Development of Phenomenological Model for RC Circular Columns
Abstract
1. Introduction
2. Database
2.1. Collection of Test Data
2.2. Design Parameters and Their Range of Values
3. The ModIMK Model and Parameters’ Calibration
3.1. The ModIMK Hysteretic Model
3.2. The Model Parameters’ Calibration
4. Empirical Equations for Model Parameters
4.1. Regression Analysis Method for Model Parameters
- (1)
- The form of the prediction equations
- (2)
- The linear regression analysis method
- (3)
- The establishment of the empirical prediction equations
4.2. Empirical Equations
- (1)
- The empirical prediction equations
- (2)
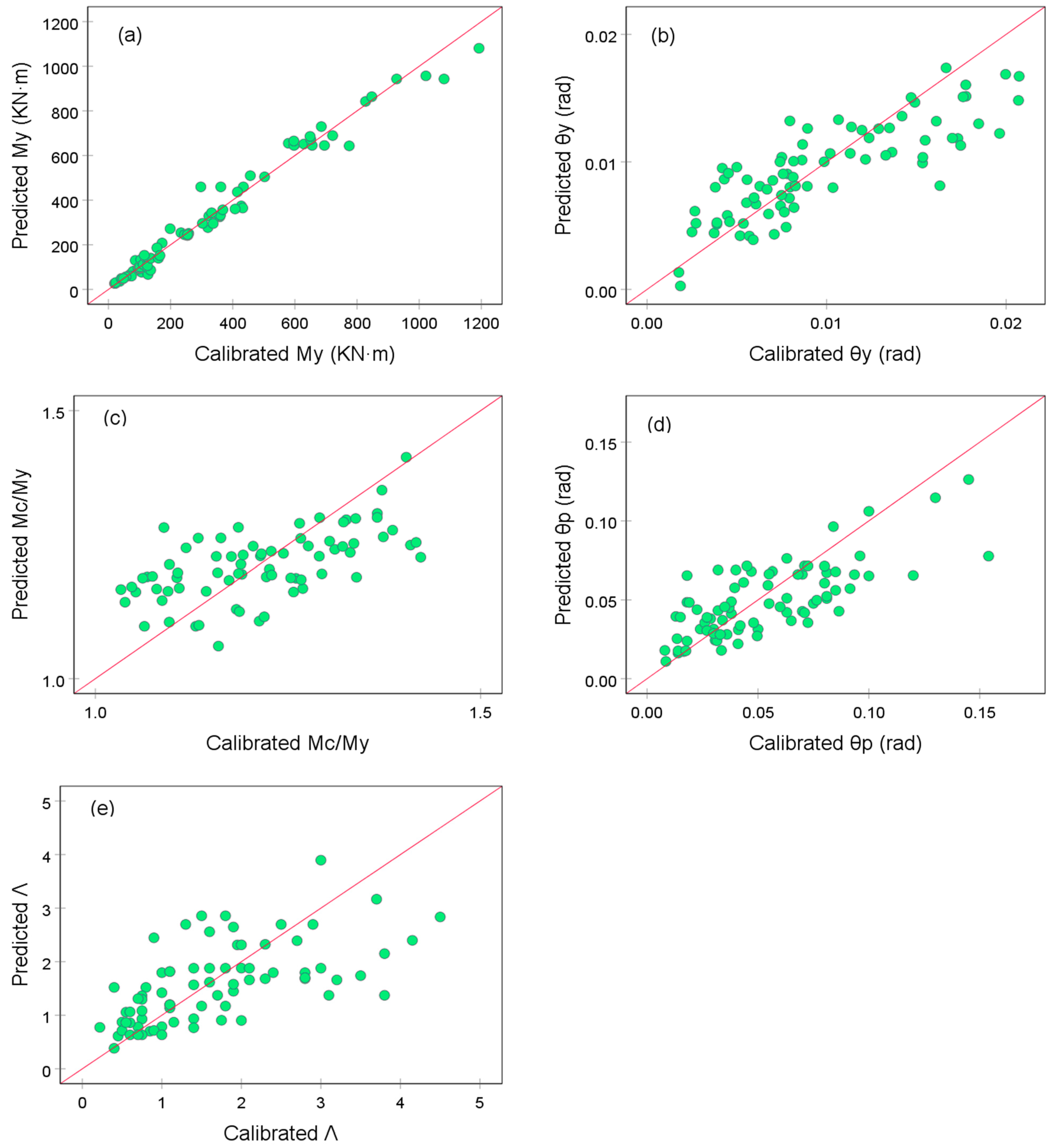
- Evaluation of the prediction equations
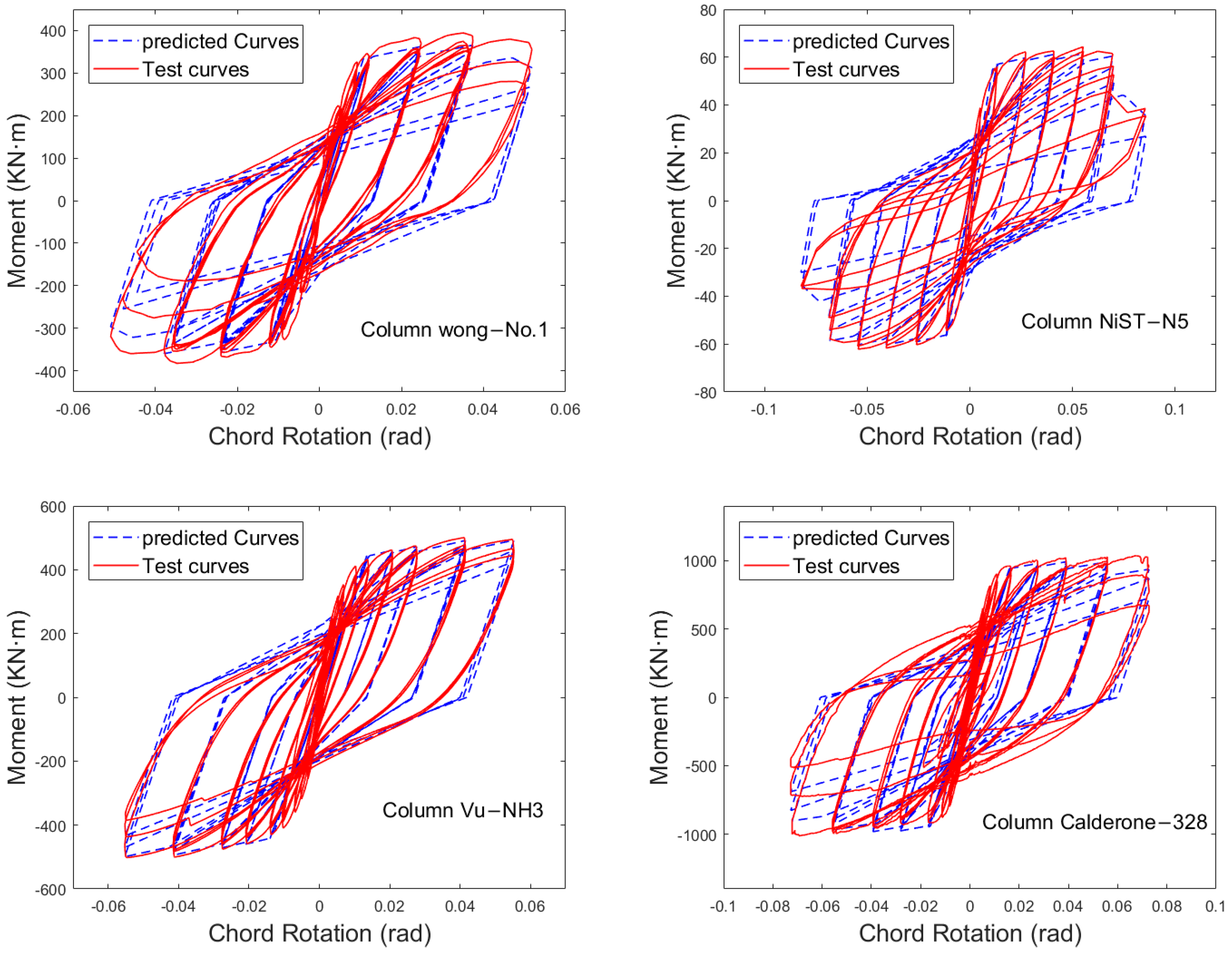
5. Validation of the Empirical Prediction Equations
5.1. Validation of the Components in the Database
5.2. Applications Outside the Database
6. Conclusions
- (1)
- A database of 80 RC circular columns undergoing flexural or flexural-shear damage had been established, containing 15 design parameters and the calibrated ModIMK model parameters. For further research on various characteristics of RC circular columns, the database is an invaluable resource.
- (2)
- The empirical prediction equations for the hysteretic model parameters based on the design parameters were established, i.e., the ModIMK model was determined. The predicted and test hysteretic curves were compared to confirm the proposed model can predict hysteretic behavior accurately. This work enabled a more comprehensive and accurate application of the ModIMK model in earthquake engineering studies involving RC circular columns.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| No. | Reference | Component No. | ||||||
|---|---|---|---|---|---|---|---|---|
| 1 | Ma [15] | c0-15 | 63.46 | 0.0085 | 1.260 | 0.0500 | — | 3.10 |
| 2 | c0-25 | 71.41 | 0.0094 | 1.254 | 0.0496 | 0.0760 | 3.80 | |
| 3 | c0-40 | 85.00 | 0.0065 | 1.173 | 0.0410 | — | 1.70 | |
| 4 | Zhu [16] | C0 | 79.20 | 0.0092 | 1.094 | 0.0130 | 0.0938 | 2.00 |
| 5 | Zhou [17] | LL | 36.13 | 0.0111 | 1.701 | 0.0545 | — | 1.95 |
| 6 | MM | 73.91 | 0.0176 | 1.532 | 0.0935 | — | 2.70 | |
| 7 | MH | 106.51 | 0.0218 | 1.463 | 0.0810 | — | 4.15 | |
| 8 | Li [18,19] | C80 | 96.21 | 0.0119 | 1.183 | 0.0307 | — | 0.75 |
| 9 | C140 | 86.40 | 0.0077 | 1.213 | 0.0085 | — | 0.45 | |
| 10 | Feng [20,21] | L0-N3-C0 | 104.44 | 0.0096 | 1.409 | 0.0225 | — | 0.90 |
| 11 | Aquino [22] | no.6 | 297.17 | 0.0090 | 1.269 | 0.0412 | 0.1134 | 1.15 |
| 12 | Lao [23] | C-0-L0-N02 | 127.18 | 0.0144 | 1.106 | 0.0240 | — | 0.40 |
| 13 | C-0-L0-N04 | 135.75 | 0.0114 | 1.067 | 0.0180 | — | 0.80 | |
| 14 | Xie [24] | BW-1 | 44.90 | 0.0101 | 1.176 | 0.0286 | — | 0.75 |
| 15 | PEER [25] | 267 | 233.50 | 0.0213 | 1.087 | 0.0260 | — | 0.70 |
| 16 | 268 | 172.50 | 0.0079 | 1.052 | 0.0380 | — | 2.00 | |
| 17 | 269 | 258.00 | 0.0236 | 1.039 | 0.0150 | — | 0.50 | |
| 18 | 271 | 254.00 | 0.0225 | 1.080 | 0.0180 | — | 0.55 | |
| 19 | 274 | 358.00 | 0.0195 | 1.226 | 0.0320 | 0.0946 | 0.75 | |
| 20 | 275 | 355.00 | 0.0175 | 1.339 | 0.0550 | — | 1.90 | |
| 21 | 276 | 360.50 | 0.0203 | 1.163 | 0.0360 | — | 0.60 | |
| 22 | 277 | 323.00 | 0.0193 | 1.033 | 0.0135 | — | 0.22 | |
| 23 | 278 | 320.00 | 0.0210 | 1.074 | 0.0370 | — | 1.10 | |
| 24 | 279 | 337.00 | 0.0169 | 1.267 | 0.0630 | — | 0.70 | |
| 25 | 280 | 245.00 | 0.0120 | 1.219 | 0.0340 | 0.1873 | 0.60 | |
| 26 | 281 | 156.00 | 0.0078 | 1.257 | 0.0480 | — | 1.40 | |
| 27 | 289 | 256.00 | 0.0118 | 1.118 | 0.0300 | — | 0.85 | |
| 28 | 290 | 254.50 | 0.0126 | 1.189 | 0.0725 | — | 1.00 | |
| 29 | 297 | 361.50 | 0.0067 | 1.109 | 0.0080 | 0.0536 | 0.50 | |
| 30 | 298 | 434.00 | 0.0095 | 1.205 | 0.0270 | 0.0505 | 1.10 | |
| 31 | 303 | 19.65 | 0.0247 | 1.047 | 0.0395 | 0.1445 | 1.90 | |
| 32 | 304 | 22.40 | 0.0189 | 1.222 | 0.0700 | — | 2.10 | |
| 33 | 305 | 23.50 | 0.0182 | 1.061 | 0.0650 | — | 3.00 | |
| 34 | 308 | 47.20 | 0.0120 | 1.331 | 0.1540 | — | 1.60 | |
| 35 | 309 | 56.00 | 0.0115 | 1.291 | 0.1200 | — | 1.40 | |
| 36 | 310 | 41.60 | 0.0083 | 1.594 | 0.0700 | — | 2.80 | |
| 37 | 311 | 47.00 | 0.0103 | 1.244 | 0.0960 | — | 1.80 | |
| 38 | 313 | 48.20 | 0.0115 | 1.187 | 0.1000 | — | 2.30 | |
| 39 | 346 | 100.00 | 0.0139 | 1.294 | 0.0470 | — | 2.40 | |
| 40 | 347 | 98.50 | 0.0114 | 1.159 | 0.0320 | — | 5.30 | |
| 41 | 349 | 109.50 | 0.0162 | 1.229 | 0.0400 | — | 2.80 | |
| 42 | 350 | 101.00 | 0.0122 | 1.190 | 0.0565 | — | 1.00 | |
| 43 | 357 | 332.00 | 0.0059 | 1.265 | 0.0315 | — | 0.90 | |
| 44 | 358 | 456.50 | 0.0058 | 1.266 | 0.0140 | 0.0320 | 0.40 | |
| 45 | 359 | 1192.50 | 0.0160 | 1.186 | 0.0800 | — | 3.80 | |
| 46 | 360 | 502.50 | 0.0122 | 1.326 | 0.0420 | — | 1.10 | |
| 47 | 361 | 198.50 | 0.0082 | 1.366 | 0.0180 | — | 1.10 | |
| 48 | 363 | 775.00 | 0.0240 | 1.372 | 0.0850 | — | 1.60 | |
| 49 | 364 | 302.50 | 0.0154 | 1.386 | 0.0750 | — | 1.75 | |
| 50 | 365 | 827.50 | 0.0201 | 1.403 | 0.0850 | — | 3.50 | |
| 51 | 366 | 579.00 | 0.0247 | 1.192 | 0.0765 | 0.3986 | 2.10 | |
| 52 | 367 | 600.00 | 0.0207 | 1.144 | 0.0380 | 0.0574 | 1.40 | |
| 53 | 368 | 629.00 | 0.0218 | 1.157 | 0.0810 | — | 3.20 | |
| 54 | 369 | 655.50 | 0.0107 | 1.133 | 0.0710 | — | 1.10 | |
| 55 | 370 | 695.00 | 0.0194 | 1.096 | 0.0840 | — | 1.60 | |
| 56 | 371 | 597.00 | 0.0215 | 1.107 | 0.1000 | — | 1.80 | |
| 57 | 372 | 415.00 | 0.0097 | 1.089 | 0.0450 | — | 2.00 | |
| 58 | 373 | 1021.50 | 0.0147 | 1.311 | 0.0725 | — | 1.00 | |
| 59 | 375 | 927.50 | 0.0187 | 1.214 | 0.1300 | — | 4.50 | |
| 60 | 376 | 1080.00 | 0.0216 | 1.215 | 0.1450 | — | 3.70 | |
| 61 | 377 | 651.00 | 0.0142 | 1.422 | 0.0710 | — | 1.80 | |
| 62 | 378 | 649.00 | 0.0153 | 1.484 | 0.0630 | — | 1.50 | |
| 63 | 379 | 721.50 | 0.0129 | 1.416 | 0.0915 | — | 1.90 | |
| 64 | 380 | 121.00 | 0.0164 | 1.276 | 0.0810 | — | 7.80 | |
| 65 | 381 | 112.00 | 0.0127 | 1.321 | 0.0630 | — | 6.00 | |
| 66 | 382 | 135.50 | 0.0085 | 1.322 | 0.0600 | — | 2.50 | |
| 67 | 383 | 126.00 | 0.0110 | 1.304 | 0.0190 | 0.0475 | 1.50 | |
| 68 | 384 | 123.50 | 0.0100 | 1.335 | 0.0270 | — | 2.30 | |
| 69 | 385 | 125.50 | 0.0173 | 1.366 | 0.0800 | — | 2.90 | |
| 70 | 386 | 136.50 | 0.0121 | 1.291 | 0.0350 | — | 1.30 | |
| 71 | 387 | 161.00 | 0.0129 | 1.338 | 0.0550 | — | 3.00 | |
| 72 | 388 | 427.50 | 0.0117 | 1.096 | 0.0165 | — | 0.60 | |
| 73 | 389 | 432.00 | 0.0079 | 1.064 | 0.0140 | — | 0.75 | |
| 74 | 390 | 407.50 | 0.0086 | 1.130 | 0.0335 | 0.0269 | 1.00 | |
| 75 | 391 | 368.00 | 0.0067 | 1.134 | 0.0175 | — | 0.70 | |
| 76 | 392 | 685.00 | 0.0116 | 1.374 | 0.0680 | 0.4156 | 1.10 | |
| 77 | 393 | 596.50 | 0.0123 | 1.456 | 0.0865 | — | 0.75 | |
| 78 | 394 | 847.50 | 0.0116 | 1.160 | 0.0300 | — | 0.55 | |
| 79 | 408 | 115.50 | 0.0108 | 1.228 | 0.0435 | — | 2.80 | |
| 80 | 412 | 165.50 | 0.0099 | 1.186 | 0.0330 | — | 1.40 |
| No. | Component No. | P-Δ Type | d | c | + 1 | s/d | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | c0-15 | shear | 260 | 30 | 25.92 | 16.0 | 0.0227 | 3.15 | 1.150 | 12.074 | 6.250 | 0.526 | 373.2 | 572.3 | 8.0 | 0.0100 | 327.0 |
| 2 | c0-25 | shear | 260 | 30 | 25.92 | 16.0 | 0.0227 | 3.15 | 1.250 | 12.074 | 6.250 | 0.519 | 373.2 | 572.3 | 8.0 | 0.0100 | 327.0 |
| 3 | c0-40 | shear | 260 | 30 | 25.92 | 16.0 | 0.0227 | 3.15 | 1.400 | 12.074 | 6.250 | 0.578 | 373.2 | 572.3 | 8.0 | 0.0100 | 327.0 |
| 4 | C0 | shear | 300 | 30 | 24.34 | 16.0 | 0.0171 | 3.67 | 1.200 | 8.840 | 4.688 | 0.389 | 355.6 | 538.3 | 8.0 | 0.0112 | 230.7 |
| 5 | LL | shear | 240 | 20 | 32.00 | 12.0 | 0.0150 | 5.83 | 1.057 | 8.333 | 4.167 | 0.184 | 400.0 | 540.0 | 6.0 | 0.0113 | 400.0 |
| 6 | MM | shear | 240 | 20 | 32.00 | 16.0 | 0.0355 | 7.50 | 1.071 | 6.250 | 3.125 | 0.288 | 400.0 | 540.0 | 6.0 | 0.0113 | 400.0 |
| 7 | MH | shear | 240 | 20 | 32.00 | 20.0 | 0.0563 | 7.50 | 1.071 | 5.000 | 2.500 | 0.412 | 400.0 | 540.0 | 6.0 | 0.0113 | 400.0 |
| 8 | C80 | shear | 300 | 30 | 26.48 | 14.0 | 0.0218 | 4.67 | 1.147 | 16.840 | 8.571 | 0.475 | 386.0 | 628.0 | 6.0 | 0.0039 | 367.0 |
| 9 | C140 | shear | 300 | 30 | 26.48 | 14.0 | 0.0218 | 4.67 | 1.410 | 29.470 | 15.000 | 0.485 | 386.0 | 628.0 | 6.0 | 0.0022 | 367.0 |
| 10 | L0-N3-C0 | shear | 300 | 30 | 35.44 | 16.0 | 0.0171 | 5.00 | 1.300 | 7.632 | 3.125 | 0.203 | 596.4 | 734.9 | 8.0 | 0.0167 | 404.9 |
| 11 | no.6 | shear | 500 | 25 | 32.00 | 25.4 | 0.0310 | 4.20 | 1.000 | 16.137 | 7.874 | 0.457 | 420.0 | 620.0 | 9.5 | 0.0031 | 420.0 |
| 12 | C-0-L0-N02 | shear | 250 | 25 | 26.16 | 16.0 | 0.0246 | 4.54 | 1.200 | 13.376 | 6.250 | 0.679 | 458.0 | 620.0 | 8.0 | 0.0100 | 454.0 |
| 13 | C-0-L0-N04 | shear | 250 | 25 | 26.16 | 16.0 | 0.0246 | 4.54 | 1.400 | 13.376 | 6.250 | 0.652 | 458.0 | 620.0 | 8.0 | 0.0100 | 454.0 |
| 14 | BW-1 | shear | 240 | 25 | 34.24 | 10.0 | 0.0174 | 5.83 | 1.100 | 22.334 | 10.000 | 0.237 | 498.8 | 693.6 | 8.0 | 0.0106 | 358.6 |
| 15 | 267 | shear | 400 | 18 | 37.50 | 16.0 | 0.0320 | 2.00 | 1.000 | 7.830 | 3.750 | 1.405 | 436.0 | 674.0 | 6.0 | 0.0051 | 328.0 |
| 16 | 268 | shear | 400 | 18 | 37.20 | 16.0 | 0.0320 | 2.00 | 1.000 | 6.452 | 3.750 | 0.950 | 296.0 | 457.0 | 6.0 | 0.0051 | 328.0 |
| 17 | 269 | shear | 400 | 18 | 36.00 | 16.0 | 0.0320 | 2.50 | 1.000 | 7.830 | 3.750 | 1.055 | 436.0 | 674.0 | 6.0 | 0.0051 | 328.0 |
| 18 | 271 | shear | 400 | 18 | 31.10 | 16.0 | 0.0320 | 2.00 | 1.000 | 5.220 | 2.500 | 1.223 | 436.0 | 674.0 | 6.0 | 0.0076 | 328.0 |
| 19 | 274 | shear | 400 | 18 | 28.70 | 16.0 | 0.0320 | 2.00 | 1.200 | 3.969 | 1.875 | 0.937 | 448.0 | 693.0 | 6.0 | 0.0102 | 372.0 |
| 20 | 275 | shear | 400 | 18 | 29.90 | 16.0 | 0.0320 | 2.50 | 1.200 | 3.969 | 1.875 | 0.924 | 448.0 | 693.0 | 6.0 | 0.0102 | 372.0 |
| 21 | 276 | shear | 400 | 21 | 31.20 | 16.0 | 0.0320 | 2.00 | 1.200 | 15.875 | 7.500 | 1.022 | 448.0 | 693.0 | 12.0 | 0.0102 | 332.0 |
| 22 | 277 | shear | 400 | 18 | 29.90 | 16.0 | 0.0320 | 2.00 | 1.200 | 7.937 | 3.750 | 1.184 | 448.0 | 693.0 | 6.0 | 0.0051 | 372.0 |
| 23 | 278 | shear | 400 | 18 | 28.60 | 16.0 | 0.0320 | 1.50 | 1.100 | 3.915 | 1.875 | 1.463 | 436.0 | 674.0 | 6.0 | 0.0102 | 328.0 |
| 24 | 279 | shear | 400 | 18 | 36.20 | 16.0 | 0.0320 | 2.00 | 1.100 | 3.915 | 1.875 | 1.045 | 436.0 | 679.0 | 6.0 | 0.0102 | 326.0 |
| 25 | 280 | shear | 400 | 18 | 33.70 | 24.0 | 0.0324 | 2.00 | 1.000 | 5.148 | 2.500 | 1.352 | 424.0 | 671.0 | 6.0 | 0.0051 | 326.0 |
| 26 | 281 | shear | 400 | 18 | 34.80 | 16.0 | 0.0192 | 2.00 | 1.000 | 7.830 | 3.750 | 0.932 | 436.0 | 679.0 | 6.0 | 0.0051 | 326.0 |
| 27 | 289 | shear | 400 | 21 | 32.30 | 16.0 | 0.0320 | 2.00 | 1.000 | 20.881 | 10.000 | 1.178 | 436.0 | 679.0 | 12.0 | 0.0076 | 332.0 |
| 28 | 290 | shear | 400 | 20 | 33.10 | 16.0 | 0.0320 | 2.00 | 1.000 | 14.355 | 6.875 | 1.265 | 436.0 | 679.0 | 10.0 | 0.0077 | 310.0 |
| 29 | 297 | shear | 400 | 18 | 37.00 | 16.0 | 0.0320 | 2.00 | 1.390 | 8.854 | 4.063 | 0.809 | 475.0 | 625.0 | 6.0 | 0.0047 | 340.0 |
| 30 | 298 | shear | 400 | 20 | 37.00 | 16.0 | 0.0320 | 2.00 | 1.390 | 8.173 | 3.750 | 0.962 | 475.0 | 625.0 | 10.0 | 0.0142 | 300.0 |
| 31 | 303 | Feff | 152 | 10.2 | 34.50 | 12.7 | 0.0558 | 7.50 | 1.241 | 3.667 | 1.732 | 0.228 | 448.0 | 679.6 | 3.7 | 0.0145 | 620.0 |
| 32 | 304 | Feff | 152 | 10.2 | 34.50 | 12.7 | 0.0558 | 3.75 | 1.241 | 3.667 | 1.732 | 0.448 | 448.0 | 679.6 | 3.7 | 0.0145 | 620.0 |
| 33 | 305 | Feff | 152 | 10.2 | 34.50 | 12.7 | 0.0558 | 3.75 | 1.351 | 3.667 | 1.732 | 0.475 | 448.0 | 679.6 | 3.7 | 0.0145 | 620.0 |
| 34 | 308 | shear | 250 | 9.9 | 24.10 | 7.0 | 0.0196 | 3.00 | 1.101 | 2.715 | 1.286 | 0.308 | 446.0 | 676.6 | 3.1 | 0.0141 | 441.0 |
| 35 | 309 | shear | 250 | 9.9 | 23.10 | 7.0 | 0.0196 | 3.00 | 1.211 | 2.715 | 1.286 | 0.364 | 446.0 | 676.6 | 3.1 | 0.0141 | 441.0 |
| 36 | 310 | shear | 250 | 9.7 | 25.40 | 7.0 | 0.0196 | 6.00 | 1.096 | 4.224 | 2.000 | 0.220 | 446.0 | 676.6 | 2.7 | 0.0068 | 476.0 |
| 37 | 311 | shear | 250 | 9.9 | 24.40 | 7.0 | 0.0196 | 3.00 | 1.100 | 2.715 | 1.286 | 0.322 | 446.0 | 676.6 | 3.1 | 0.0141 | 441.0 |
| 38 | 313 | shear | 250 | 9.7 | 23.30 | 7.0 | 0.0196 | 6.00 | 1.105 | 4.224 | 2.000 | 0.619 | 446.0 | 676.6 | 2.7 | 0.0068 | 476.0 |
| 39 | 346 | shear | 305 | 14.5 | 29.00 | 9.5 | 0.0204 | 4.50 | 1.094 | 4.233 | 2.000 | 0.323 | 448.0 | 690.0 | 4.0 | 0.0094 | 434.0 |
| 40 | 347 | shear | 305 | 14.5 | 35.50 | 9.5 | 0.0204 | 4.50 | 1.086 | 4.233 | 2.000 | 0.307 | 448.0 | 690.0 | 4.0 | 0.0094 | 434.0 |
| 41 | 349 | shear | 305 | 14.5 | 35.50 | 9.5 | 0.0204 | 4.50 | 1.086 | 4.233 | 2.000 | 0.341 | 448.0 | 690.0 | 4.0 | 0.0094 | 434.0 |
| 42 | 350 | shear | 305 | 14.5 | 35.50 | 9.5 | 0.0204 | 4.50 | 1.094 | 4.233 | 2.000 | 0.330 | 448.0 | 690.0 | 4.0 | 0.0094 | 434.0 |
| 43 | 357 | Feff | 610 | 15.9 | 30.00 | 12.7 | 0.0052 | 1.50 | 1.057 | 12.897 | 6.000 | 0.164 | 462.0 | 700.9 | 6.4 | 0.0028 | 361.0 |
| 44 | 358 | Feff | 610 | 15.9 | 30.00 | 12.7 | 0.0104 | 1.50 | 1.057 | 21.494 | 10.000 | 0.187 | 462.0 | 700.9 | 6.4 | 0.0017 | 361.0 |
| 45 | 359 | Feff | 610 | 27.8 | 41.10 | 22.2 | 0.0266 | 6.00 | 1.148 | 5.477 | 2.568 | 0.076 | 455.0 | 746.0 | 9.5 | 0.0089 | 414.0 |
| 46 | 360 | Feff | 457 | 24.8 | 38.30 | 15.9 | 0.0241 | 1.99 | 1.307 | 7.802 | 3.774 | 0.672 | 427.5 | 648.5 | 9.5 | 0.0114 | 430.2 |
| 47 | 361 | Feff | 457 | 24.8 | 39.20 | 15.9 | 0.0241 | 1.99 | 0.901 | 7.802 | 3.774 | 0.519 | 427.5 | 648.5 | 9.5 | 0.0114 | 430.2 |
| 48 | 363 | Feff | 457 | 26.4 | 35.00 | 19.0 | 0.0521 | 1.99 | 1.148 | 5.125 | 2.368 | 0.716 | 468.2 | 710.3 | 12.7 | 0.0270 | 434.4 |
| 49 | 364 | Feff | 457 | 24.8 | 35.20 | 15.9 | 0.0241 | 1.99 | 0.915 | 11.335 | 5.031 | 0.875 | 507.5 | 769.9 | 9.5 | 0.0085 | 448.2 |
| 50 | 365 | Feff | 457 | 26.4 | 35.00 | 19.0 | 0.0521 | 1.99 | 1.333 | 4.642 | 2.105 | 0.225 | 486.2 | 737.6 | 12.7 | 0.0304 | 434.4 |
| 51 | 366 | Feff | 457 | 30.2 | 36.60 | 15.9 | 0.0362 | 8.00 | 1.296 | 10.439 | 4.780 | 0.269 | 477.0 | 723.6 | 9.5 | 0.0092 | 445.0 |
| 52 | 367 | Feff | 457 | 30.2 | 40.00 | 15.9 | 0.0362 | 8.00 | 1.271 | 7.005 | 3.208 | 0.325 | 477.0 | 723.6 | 6.4 | 0.0060 | 437.0 |
| 53 | 368 | Feff | 457 | 30.2 | 38.60 | 15.9 | 0.0362 | 8.00 | 1.281 | 10.439 | 4.780 | 0.286 | 477.0 | 723.6 | 9.5 | 0.0092 | 445.0 |
| 54 | 369 | prrdv | 609.6 | 22.2 | 31.00 | 15.9 | 0.0149 | 4.00 | 1.072 | 4.299 | 2.000 | 0.368 | 462.0 | 630.0 | 6.4 | 0.0070 | 606.8 |
| 55 | 370 | prrdv | 609.6 | 22.2 | 31.00 | 15.9 | 0.0149 | 8.00 | 1.072 | 4.299 | 2.000 | 0.369 | 462.0 | 630.0 | 6.4 | 0.0070 | 606.8 |
| 56 | 371 | shear | 609.6 | 22.2 | 31.00 | 15.9 | 0.0149 | 10.00 | 1.072 | 4.299 | 2.000 | 0.370 | 462.0 | 630.0 | 6.4 | 0.0070 | 606.8 |
| 57 | 372 | prrdv | 609.6 | 22.2 | 31.00 | 15.9 | 0.0075 | 4.00 | 1.072 | 4.299 | 2.000 | 0.237 | 462.0 | 630.0 | 6.4 | 0.0070 | 606.8 |
| 58 | 373 | prrdv | 609.6 | 22.2 | 31.00 | 15.9 | 0.0298 | 4.00 | 1.072 | 4.299 | 2.000 | 0.595 | 462.0 | 630.0 | 6.4 | 0.0070 | 606.8 |
| 59 | 375 | prrdv | 609.6 | 28.6 | 34.50 | 19.0 | 0.0273 | 8.00 | 1.091 | 2.808 | 1.337 | 0.225 | 441.3 | 602.0 | 6.4 | 0.0089 | 606.8 |
| 60 | 376 | prrdv | 609.6 | 28.6 | 34.50 | 19.0 | 0.0273 | 10.00 | 1.091 | 2.808 | 1.337 | 0.143 | 441.3 | 602.0 | 6.4 | 0.0089 | 606.8 |
| 61 | 377 | Feff | 600 | 30.2 | 31.40 | 22.2 | 0.0192 | 3.00 | 1.045 | 9.248 | 4.369 | 0.556 | 448.0 | 739.0 | 9.5 | 0.0054 | 431.0 |
| 62 | 378 | Feff | 600 | 30.2 | 34.60 | 22.2 | 0.0192 | 3.00 | 1.041 | 9.248 | 4.369 | 0.545 | 448.0 | 739.0 | 9.5 | 0.0054 | 431.0 |
| 63 | 379 | Feff | 600 | 30.2 | 33.00 | 22.2 | 0.0192 | 3.00 | 1.043 | 6.190 | 2.883 | 0.448 | 461.0 | 775.0 | 9.5 | 0.0081 | 434.0 |
| 64 | 380 | prrdv | 250 | 13.8 | 65.00 | 16.0 | 0.0328 | 6.58 | 1.313 | 6.397 | 3.125 | 1.116 | 419.0 | 635.6 | 7.5 | 0.0015 | 1000.0 |
| 65 | 381 | prrdv | 250 | 15.6 | 65.00 | 16.0 | 0.0328 | 6.58 | 1.313 | 6.397 | 3.125 | 0.706 | 419.0 | 635.6 | 11.3 | 0.0035 | 420.0 |
| 66 | 382 | prrdv | 250 | 13.8 | 90.00 | 16.0 | 0.0328 | 6.58 | 1.419 | 6.397 | 3.125 | 0.410 | 419.0 | 635.6 | 7.5 | 0.0154 | 1000.0 |
| 67 | 383 | prrdv | 250 | 14 | 90.00 | 16.0 | 0.0328 | 6.58 | 1.419 | 6.397 | 3.125 | 0.284 | 419.0 | 635.6 | 8.0 | 0.0175 | 580.0 |
| 68 | 384 | prrdv | 250 | 15.6 | 90.00 | 16.0 | 0.0328 | 6.58 | 1.419 | 12.793 | 6.250 | 0.316 | 419.0 | 635.6 | 11.3 | 0.0174 | 420.0 |
| 69 | 385 | prrdv | 250 | 13.8 | 90.00 | 16.0 | 0.0328 | 6.58 | 1.209 | 6.397 | 3.125 | 0.338 | 419.0 | 635.6 | 7.5 | 0.0015 | 1000.0 |
| 70 | 386 | prrdv | 250 | 13.8 | 90.00 | 16.0 | 0.0328 | 6.58 | 1.419 | 6.397 | 3.125 | 0.342 | 419.0 | 635.6 | 7.5 | 0.0015 | 1000.0 |
| 71 | 387 | prrdv | 250 | 13.8 | 90.00 | 16.0 | 0.0328 | 6.58 | 1.419 | 6.397 | 3.125 | 0.219 | 419.0 | 635.6 | 11.3 | 0.0034 | 420.0 |
| 72 | 388 | prrdv | 508 | 21.3 | 56.20 | 16.0 | 0.0099 | 3.00 | 1.127 | 13.598 | 6.375 | 0.795 | 455.0 | 723.5 | 4.5 | 0.0013 | 455.0 |
| 73 | 389 | prrdv | 508 | 21.3 | 56.30 | 16.0 | 0.0099 | 3.00 | 1.109 | 13.598 | 6.375 | 0.975 | 455.0 | 723.5 | 4.5 | 0.0013 | 455.0 |
| 74 | 390 | prrdv | 508 | 21.3 | 57.00 | 16.0 | 0.0099 | 3.00 | 1.099 | 13.598 | 6.375 | 0.975 | 455.0 | 723.5 | 4.5 | 0.0013 | 455.0 |
| 75 | 391 | prrdv | 508 | 21.3 | 52.70 | 16.0 | 0.0099 | 3.00 | 1.107 | 13.598 | 6.375 | 0.926 | 455.0 | 723.5 | 4.5 | 0.0013 | 455.0 |
| 76 | 392 | prrdv | 609.6 | 22.2 | 37.20 | 15.9 | 0.0149 | 4.00 | 1.120 | 4.299 | 2.000 | 0.351 | 462.0 | 700.9 | 6.4 | 0.0070 | 606.8 |
| 77 | 393 | prrdv | 609.6 | 22.2 | 37.20 | 15.9 | 0.0149 | 4.00 | 1.060 | 8.584 | 3.994 | 0.448 | 462.0 | 700.9 | 6.4 | 0.0035 | 606.8 |
| 78 | 394 | prrdv | 609.6 | 20 | 32.60 | 19.0 | 0.0254 | 6.00 | 1.187 | 11.865 | 6.684 | 1.176 | 315.1 | 497.8 | 6.4 | 0.0017 | 351.6 |
| 79 | 408 | Feff | 406.4 | 15 | 36.50 | 12.7 | 0.0117 | 4.56 | 1.000 | 5.362 | 2.504 | 0.237 | 458.5 | 646.0 | 4.5 | 0.0053 | 691.5 |
| 80 | 412 | Feff | 406.4 | 10.4 | 35.40 | 12.7 | 0.0117 | 2.58 | 1.000 | 10.706 | 5.000 | 0.812 | 458.5 | 646.0 | 4.5 | 0.0026 | 691.5 |
References
- Ibarra, L.F.; Medina, R.A.; Krawinkler, H. Hysteretic models that incorporate strength and stiffness deterioration. Earthq. Eng. Struct. Dyn. 2005, 34, 1489–1511. [Google Scholar] [CrossRef]
- Lignos, D.G.; Krawinkler, H. Deterioration modeling of steel components in support of collapse prediction of steel moment frames under earthquake loading. J. Struct. Eng. 2011, 137, 1291–1302. [Google Scholar] [CrossRef]
- Terrenzi, M.; Spacone, E.; Camata, G. Comparison between phenomenological and fiber-section non-linear models. Front. Built Environ. 2020, 6, 38. [Google Scholar] [CrossRef]
- Zucca, M.; Reccia, E.; Longarini, N.; Eremeyev, V.; Crespi, P. On the structural behaviour of existing RC bridges subjected to corrosion effects: Numerical insight. Eng. Fail. Anal. 2023, 152, 107500. [Google Scholar] [CrossRef]
- Lu, X.; Zhu, Z.; Wang, K. Hysteretic behavior of composite beam-reinforced concrete column frame. Results Eng. 2024, 23, 102549. [Google Scholar] [CrossRef]
- Di Domenico, M.; Ricci, P.; Verderame, G.M. Empirical calibration of hysteretic parameters for modelling the seismic response of reinforced concrete columns with plain bars. Eng. Struct. 2021, 237, 112120. [Google Scholar] [CrossRef]
- Ma, Y.; Wu, Z.; Cheng, X.; Sun, Z.; Chen, X. Parameter identification of hysteresis model of reinforced concrete columns considering shear action. Structures 2023, 47, 93–104. [Google Scholar] [CrossRef]
- Haselton, C.B.; Liel, A.B.; Lange, S.T. Beam-Column Element Model Calibrated for Predicting Flexural Response Leading to Global Collapse of RC Frame Buildings; Pacific Earthquake Engineering Research Center: Berkeley, CA, USA, 2008. [Google Scholar]
- Lignos, D.G.; Krawinkler, H. Development and utilization of structural component databases for performance-based earthquake engineering. J. Struct. Eng. 2013, 139, 1382–1394. [Google Scholar] [CrossRef]
- Lignos, D.G. Sidesway Collapse of Deteriorating Structural Systems Under Seismic Excitations. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2013. [Google Scholar]
- Dai, K.Y.; Yu, X.H.; Lu, D.G. Phenomenological hysteretic model for corroded RC columns. Eng. Struct. 2020, 210, 110315. [Google Scholar] [CrossRef]
- Huang, X.; Kim, S.H.; Kwon, O. Lumped spring model parameters of RC frame elements for seismic performance assessment. Earthq. Eng. Struct. D 2022, 51, 2553–2574. [Google Scholar] [CrossRef]
- Liu, H.B.; Lin, J.Q.; Liu, J.L. Deterioration Model for Corroded Circular Columns. Appl. Sci. 2024, 14, 7983. [Google Scholar] [CrossRef]
- Dai, K.Y.; Yu, X.H.; Wang, S. Identification of the hysteretic model parameters for reinforced concrete circular columns based on the modified ibarra-medina-krawinkler material model. Eng. Mech. 2021, 38, 154–163. (In Chinese) [Google Scholar]
- Ma, Y.; Che, Y.; Gong, J. Behavior of corrosion damaged circular reinforced conc-rete columns under cyclic loading. Constr. Build. Mater. 2012, 29, 548–556. [Google Scholar] [CrossRef]
- Zhu, J. Degradation of Capacity of the Corroded Reinforced Concrete Columns. Ph.D. Thesis, Shanghai Jiao Tong University, Shanghai, China, 2013. (In Chinese). [Google Scholar]
- Zhou, M.; Yin, S.; Zhu, G.; Fu, J. Seismic performance evaluation of highway bridges under scour and chloride ion corrosion. Appl. Sci. 2022, 12, 6680. [Google Scholar] [CrossRef]
- Li, J.; Li, Y. Experimental and theoretical study on the seismic performance of corroded RC circular columns strengthened with hybrid fiber reinforced polymers. Polym. Polym. Compos. 2014, 22, 653–660. [Google Scholar] [CrossRef]
- Li, J. Experimental and Theoretical Research on Hybrid FRP and Seismic Performance for Strengthening Corroded RC Columns. Ph.D. Thesis, Beijing University of Technology, Beijing, China, 2010. (In Chinese). [Google Scholar]
- Feng, R.; Li, Y.; Zhu, J.H.; Xing, F. Behavior of corroded circular RC columns strengthened by C-FRCM under cyclic loading. Eng. Struct. 2021, 226, 111311. [Google Scholar] [CrossRef]
- Liu, J.R. Research on the Seismic Behavior of Corroded RC Columns Under the New Restoration System. Ph.D. Thesis, Harbin Institute of Technology, Harbin, China, 2019. (In Chinese). [Google Scholar]
- Aquino, W.; Hawkins, N.M. Seismic retrofitting of corroded reinforced concrete columns using carbon composites. ACI Struct. J. 2007, 104, 348–356. [Google Scholar]
- Lao, T.A. Experimental Study on Seismic Performance of Corroded Reinforced Concrete Columns Under Large Strain FRP Confinement. Ph.D. Thesis, Shenzhen University, Shenzhen, China, 2010. (In Chinese). [Google Scholar]
- Xie, M.F. Seismic Behavior of Reinforced Concrete Piers with Basalt Fiber Sheets. Ph.D. Thesis, Shijiazhuang Tie Dao University, Shijiazhuang, China, 2020. (In Chinese). [Google Scholar]
- Berry, M.; Parrish, M.; Eberhard, M. Peer Structural Performance Database User’s Manual (Version 1.0). 2004. Available online: https://nisee.berkeley.edu/spd/performance_database_manual_1-0.pdf (accessed on 12 April 2025).
- Haselton, C.B.; Liel, A.B.; Taylor-Lange, S.C.; Deierlein, G.G. Calibration of model to simulate response of reinforced concrete beam-columns to collapse. ACI Struct. J. 2016, 113, 1141–1152. [Google Scholar] [CrossRef]
- Ibarra, L.F.; Krawinkler, H. Global Collapse of Frame Structures Under Seismic Excitations. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2004. [Google Scholar]
- Zareian, F.; Medina, R.A. A practical method for proper modeling of structural damping in inelastic plane structural systems. Comput. Struct. 2010, 88, 45–53. [Google Scholar] [CrossRef]
- Haselton, C.B.; Liel, A.B.; Dean, B.S. Seismic collapse safety and behavior of modern reinforced concrete moment frame buildings. Struct. Eng. Res. Front. 2007, 1–14. [Google Scholar] [CrossRef]


| Parameter | Description | Parameter | Description |
|---|---|---|---|
| volume stirrup ratio | d | diameter of circular column, mm | |
| ratio of stirrup spacing to longitudinal bar diameter | yield strength of stirrup, MPa | ||
| c | thickness of concrete cover, mm | longitudinal reinforcement ratio | |
| ultimate strength of longitudinal reinforcement | yield strength of longitudinal reinforcement, MPa | ||
| L | shear span, mm | shear capacity ratio, Calculated according to the U.S. Code ACI318 | |
| axial load ratio | |||
| s | stirrup spacing, mm | stirrup diameter, mm | |
| shear span ratio | cylinder compressive strength of concrete, MPa | ||
| longitudinal reinforcement diameter, mm |
| Parameter | Value Range |
|---|---|
| d (mm) | 152–609.6 |
| (MPa) | 23.1–90 |
| v | −0.01–0.419 |
| 1.5–10 | |
| (MPa) | 296–496.4 |
| (MPa) | 230.68–1000 |
| 0.0052–0.0563 | |
| 0.0013–0.0304 |
| Parameter | Description | Parameter | Description |
|---|---|---|---|
| elastic stiffness | yield moment | ||
| chord rotation of strain-hardening stage in backbone curve | hardening stiffness | ||
| residual moment in backbone curve | post-capping stiffness | ||
| chord rotation of elastic stage | ultimate rotation capacity | ||
| capping moment | chord rotation of softening stage in backbone curve | ||
| c | the deterioration exponent | cyclic deterioration parameter |
| 1.01 | 1.02 | 1.02 | 1.09 | 1.13 | 1.20 | |
| 0.99 | 1.01 | 1.02 | 1.02 | 0.99 | 1.00 | |
| 0.18 | 0.22 | 0.11 | 0.44 | 0.57 | — |
| Test No | ||||||
|---|---|---|---|---|---|---|
| 298 | 459.50 | 0.0108 | 1.247 | 0.0305 | 1.197 | 0.1 |
| 360 | 504.36 | 0.0104 | 1.296 | 0.0337 | 1.196 | 0.1 |
| 384 | 139.57 | 0.0112 | 1.252 | 0.0389 | 2.324 | 0.1 |
| 392 | 729.74 | 0.0131 | 1.264 | 0.0661 | 1.815 | 0.1 |
| Component No. | P-Δ Type | d | c | + 1 | s/d | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wong-No.1 | shear | 400 | 20 | 38.00 | 16.0 | 0.0320 | 2.00 | 1.190 | 7.713 | 3.750 | 0.830 | 423.0 | 577.0 | 10.0 | 0.0142 | 300.0 |
| NiST-N5 | shear | 250 | 9.9 | 24.30 | 7.0 | 0.0196 | 3.00 | 1.200 | 2.715 | 1.286 | 0.357 | 446.0 | 676.6 | 3.1 | 0.0141 | 441.0 |
| Vu-NH3 | Feff | 457 | 24.8 | 39.40 | 15.9 | 0.0241 | 1.99 | 1.150 | 7.802 | 3.774 | 0.673 | 427.5 | 648.5 | 9.5 | 0.0114 | 430.2 |
| Calderone-328 | prrdv | 609.6 | 28.6 | 34.50 | 19.0 | 0.0273 | 3.00 | 1.091 | 2.808 | 1.337 | 0.502 | 441.3 | 602.0 | 6.4 | 0.0089 | 606.8 |
| Component No. | ||||||
|---|---|---|---|---|---|---|
| Wong-No.1 | 336.11 | 0.0108 | 1.253 | 0.0421 | 1.250 | 0.1 |
| NiST-N5 | 56.70 | 0.0114 | 1.229 | 0.0666 | 1.878 | 0.1 |
| Vu-NH3 | 410.11 | 0.0115 | 1.296 | 0.0423 | 1.196 | 0.1 |
| Calderone-328 | 943.01 | 0.0161 | 1.263 | 0.0751 | 1.745 | 0.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.; Lin, J.; Liu, J. Development of Phenomenological Model for RC Circular Columns. Appl. Sci. 2025, 15, 4841. https://doi.org/10.3390/app15094841
Liu H, Lin J, Liu J. Development of Phenomenological Model for RC Circular Columns. Applied Sciences. 2025; 15(9):4841. https://doi.org/10.3390/app15094841
Chicago/Turabian StyleLiu, Haibing, Junqi Lin, and Jinlong Liu. 2025. "Development of Phenomenological Model for RC Circular Columns" Applied Sciences 15, no. 9: 4841. https://doi.org/10.3390/app15094841
APA StyleLiu, H., Lin, J., & Liu, J. (2025). Development of Phenomenological Model for RC Circular Columns. Applied Sciences, 15(9), 4841. https://doi.org/10.3390/app15094841





