Research on High-Speed Modeling Method of Modular Multilevel Converter Valve Based on Data Derivation

Abstract

Due to the highly demanding operating conditions of the Modular Multilevel Converter (MMC), as well as its inherently complex structure, electric field analysis of the MMC valve is crucial for the safe and stable operation of MMC-HVDC (MMC high voltage direct current) transmission systems. In this paper, a high-speed modeling method for an MMC valve based on data derivation is proposed. Firstly, the relationship between the MMC valve electric field calculation model parameters and the MMC-HVDC transmission system parameters was studied. Based on this, the data derivation system for the electric field calculation model parameters of all components of the MMC valve was established. A modeling parameters database based on empirical knowledge is also used in the calculation process of the data derivation system. The output model parameter matrix includes the geometric parameters, position parameters, and electrical parameters of the components. Based on the output matrix, the electric field calculation model of the converter valve can be quickly generated. Furthermore, to improve the accuracy of the calculations and reduce computation time, a discrete modeling method that combines mesh optimization was proposed. In the process of discretized electric field modeling, a mesh division influence factor based on the accuracy of electric field calculation is proposed. By adjusting the mesh division influence factor during the electric field calculation, the accuracy of the electric field calculation can be optimized rapidly. Finally, the effectiveness and practicality of the high-speed modeling method for the MMC valve are verified through comprehensive case studies conducted on the ±320 kV onshore MMC-HVDC valve. It is demonstrated that the high-speed modeling method proposed in this paper can significantly reduce the modeling time of the converter valve and greatly improve the accuracy of electric field calculation.

1. Introduction

Due to the manufacturing or complex operating conditions, there might be insulation failures or defects in the insulation of the MMC valve. The analysis of the electric field in the MMC valve has become extremely important. In particular, the MMC-HVDC system has been widely used in recent years. With the continuous increase in voltage and capacity, the MMC valve, as a core component of the MMC-HVDC transmission system, is facing increasing challenges [1,2,3,4]. The MMC valve is subjected to highly complex and demanding current and voltage conditions during operation. Consequently, the electric field characteristics of the MMC valve have a significant impact on the safety and stability of the MMC-HVDC transmission systems. In particular, under fault conditions with impulse excitation, the distributed parameters of the MMC valves, especially the stray capacitance, can have a severe impact on the system [5,6,7]. The method of electric field analysis is commonly employed to calculate the stray parameters of converter valves.

Research on electric field analysis of high-voltage equipment is relatively mature, with a wide variety of calculation theories and commercial software available. However, the electric field analysis of complex equipment and environments, such as converter valves, remains a research hotspot in the industry. With the increasing demand for high power density in electrical equipment, the accurate and efficient calculation of the electric field for complex equipment continues to be a challenging issue.

To investigate the accurate electric field characteristics of the converter valve, the 3D model of ±420 kV flexible converter valve tower by Creo software and the electric field distribution for the valve tower are calculated by ANSOFT software in reference [8]. In reference [9], through simplification FEM model of the valve tower in ANSYS Q3D Extractor, the capacitances are calculated. However, the modeling process of the aforementioned methods for electric field calculation is relatively complex, and the amount of work involved in the computation is considerably large. To enhance the efficiency of electric field calculation for HVDC equipment, reference [10] adopted the method of the equivalent gas conductivity to achieve calculation of electric field distribution quickly. To address the problem of large calculation amount of transient field in the valve hall model, reference [11] proposes an instantaneous potential load method to study the electric field characteristics of UHV-VSC valve hall. To reduce the computational load in the process of electric field calculation, reference [12] proposes a simplified calculation model of the radiative near field of the electric field in the circuit breaker valve hall. Similarly, reference [13] presents a simplified approach for the MMC valve, employing the finite element method to compute the stray capacitances. In summary, the modeling process and methods for the electric field analysis of converter valves are of great significance. The electric field accurate analysis of the MMC valve is significant for the design of the MMC valve and the MMC-HVDC system. In the aforementioned studies, the modeling process of the converter valve has not been analyzed.

However, previous studies have focused on theoretical calculations and model simplification, neglecting a systematic and detailed process. In some of the analyses of electric field modeling method in these articles, both the insulators and the shape of the outer conductors are ignored, which will significantly reduce the accuracy of the electric field calculation. How to improve the accuracy and efficiency of electric field calculation during the modeling process has not been addressed. Meanwhile, in the model process of the converter valve, the conventional import method or the re-establishment of the electric field model based on the mechanical model is adopted. This will result in a very long time required for the establishment of the converter valve electric field model. Conventional modeling methods for MMC valve electric fields suffer from long modeling times and low accuracy.

With the rapid development of information technology, data derivation technology, as an important data processing technique, has been widely applied in various industries, especially in the era of big data. Its core principle is to generate a large amount of derived data from a small amount of raw data in a specific manner, thereby providing richer and more comprehensive data support for the analysis and modeling of complex systems [14,15,16,17]. This paper innovatively introduces data derivation technology into the electric field modeling of the MMC valve, aiming to derive the parameter matrix for MMC valve electric field modeling by combining a small number of MMC system parameters with the design process and principles of the MMC valve. This method also digitizes the modeling parameters and process of the converter valve, thereby providing an efficient and accurate new approach for the optimized design and performance evaluation of converter valves.

In this paper, a high-speed modeling method for the MMC valve is proposed to overcome these drawbacks. The data derivation is used to generate multiple sets of parameters from a limited number of system input parameters (three to four). These derived parameters are then utilized to establish the electric field analysis model for the MMC valve. Firstly, the relationship between the MMC valve electric field calculation model parameters and the MMC-HVDC transmission system parameters was studied. Based on this, the data derivation system for the electric field calculation model parameters of all components of the MMC valve was established. A modeling parameters database based on empirical knowledge is also used in the calculation process of the data derivation system. Empirical knowledge is used to constrain and correct the parameters. The output model parameter matrix includes the geometric parameters, position parameters, and electrical parameters of the components. Based on the output matrix, the electric field calculation model of the converter valve can be quickly generated. Furthermore, to improve the accuracy of the calculations and reduce computation time, a discrete modeling method that combines mesh optimization was proposed. In the process of discretized electric field modeling, a mesh division influence factor based on the accuracy of electric field calculation is proposed. By adjusting the mesh division influence factor during the electric field calculation, the accuracy of the electric field calculation can be optimized rapidly. Finally, the effectiveness and practicality of the high-speed modeling method for the MMC valve are verified through comprehensive case studies conducted on the ±320 kV onshore MMC-HVDC valve. By comparing the modeling times, calculation error rate, and calculation times, it was confirmed that the method described in this paper has significant advantages.

2. High-Speed Modeling Method for the MMC Valve

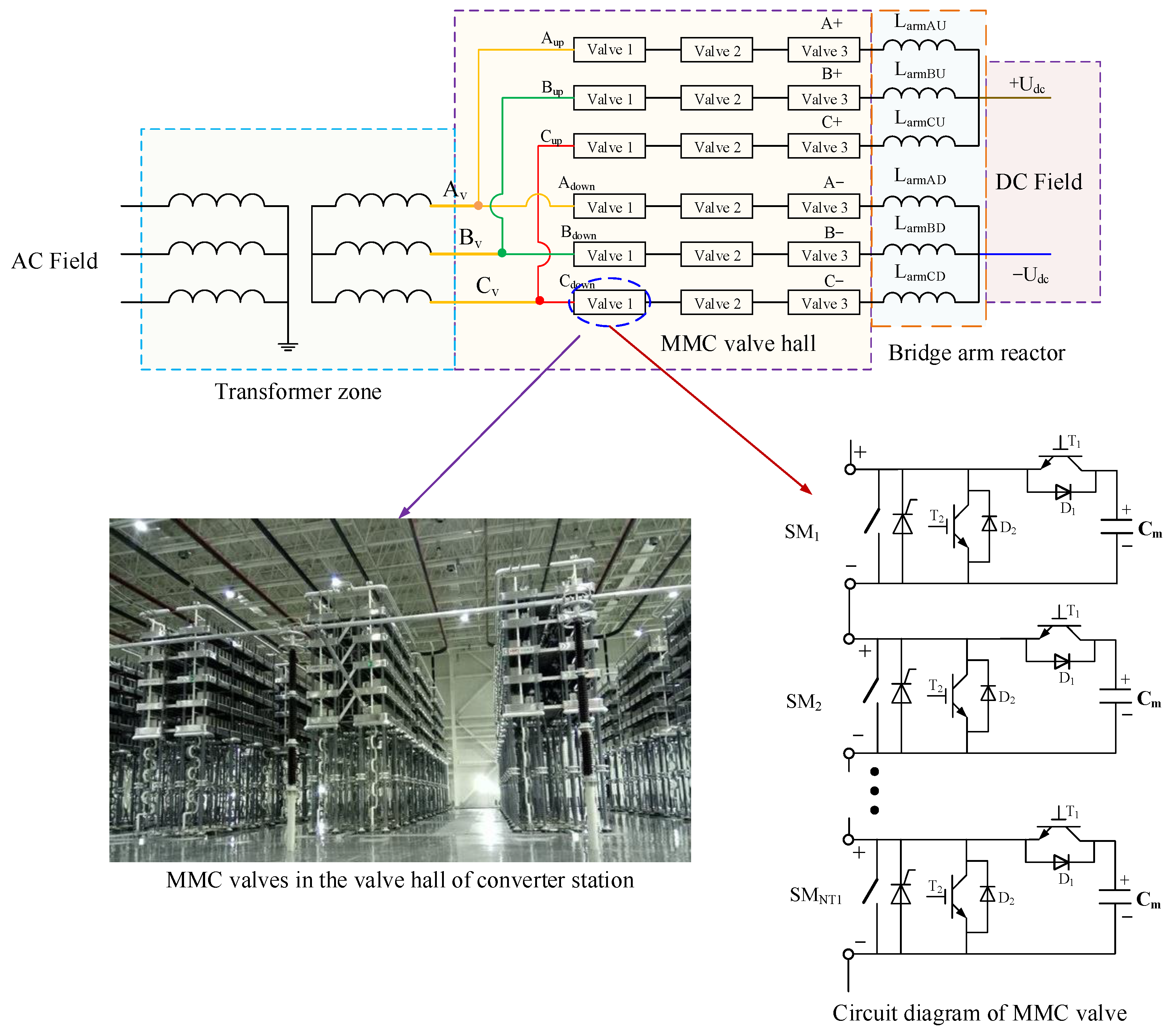
The MMC-HVDC power transmission system has been put into practice in many projects [18,19,20]. The MMC system schematic diagram and the MMC valve in hall are illustrated in Figure 1.

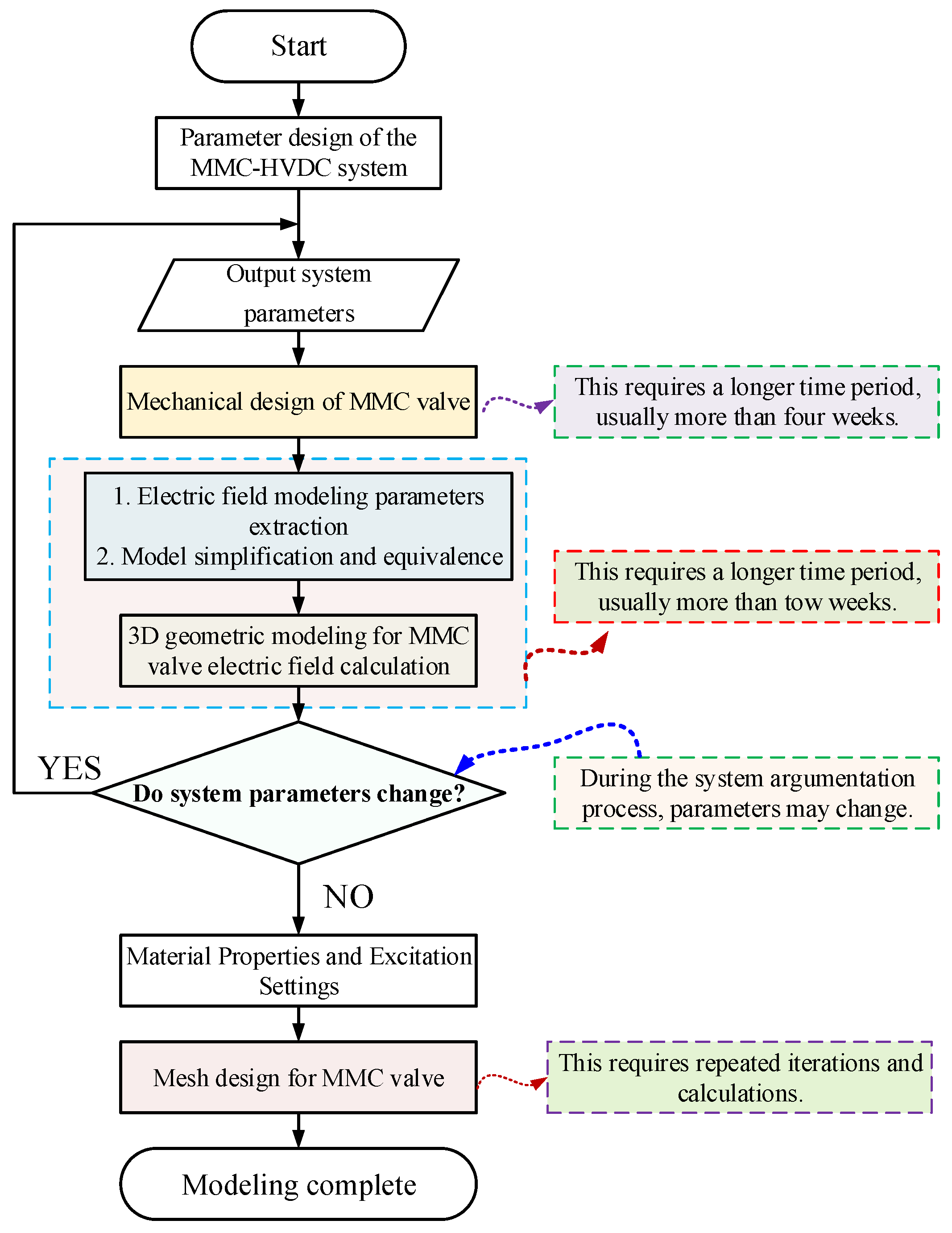
The design data and operational observation data for the MMC system are all circuit parameters such as voltage and current. In the operating environment of the valve hall, the insulation characteristics of the MMC valve need to be obtained through electric field calculation. The conventional electric field analysis process of the MMC valve, which is widely used in engineering practice, is shown in Figure 2.

As shown in Figure 2, the conventional electric field modeling method involves multiple departments and is time-consuming. When transitioning from the mechanical model to the electric field analysis model, simplifications are made to adjust the electric field analysis.

The importation of models may introduce errors, thereby reducing the computational accuracy. Moreover, if system parameters are updated or adjusted during the analysis, the mechanical model must be re-established. The conventional approach results in a lengthy analysis cycle for the converter valve’s electric field, with difficulties in controlling the computational accuracy [21,22].

To enhance the efficiency and accuracy of the electric field analysis of the MMC valve, it is necessary to establish models based on the requirements of electric field analysis and the characteristics of the MMC-HVDC power transmission system.

Based on the research of the operating characteristics of MMC-HVDC and the design process of the MMC valve, the high-speed modeling method using data derivation technology is proposed. Compared with conventional electric field analysis methods, the high-speed modeling process bypasses the steps of mechanical model construction and simplification, fully digitizes the electrostatic field modeling process, and directly generates the electrostatic field analysis model using the modeling data derived from the data derivation method. Meanwhile, mesh optimization is also included in the model establishment process, which significantly reduces the iterative computation in the electrostatic field calculation.

The high-speed modeling method for electric field analysis of the MMC valve is illustrated in Figure 3.

As shown in Figure 3, the high-speed modeling for the electric field analysis of the MMC valve used data derivation to generate multiple modeling datasets from limited input data. Within the data derivation system, the parameters necessary for the modeling process of the MMC valve are computed. The high-speed modeling method proposed in this paper can significantly reduce the time required for modeling. The remainder of this section will delve into the fundamental theories of electric field modeling that underpin the data derivation system.

According to the fundamental principles of electric fields, the electric equilibrium characteristics of a conductor reveal that the inner surface of a metallic conductor has no charge distribution, and the electric field within an air-filled cavity is zero, with a uniform potential throughout. The shape of the internal cavity does not influence the external electric field distribution of the conductor. The curvature of the conductor’s outer surface significantly affects the charge distribution and electric field. Higher curvature reduces the repulsive force between charges, leading to higher charge density and a more concentrated electric field. Conversely, lower curvature results in a more uniform electric field distribution. Therefore, while the shape of the conductor’s outer surface strongly impacts the electric field distribution, the internal cavity and its shape have no effect on the external electric field.

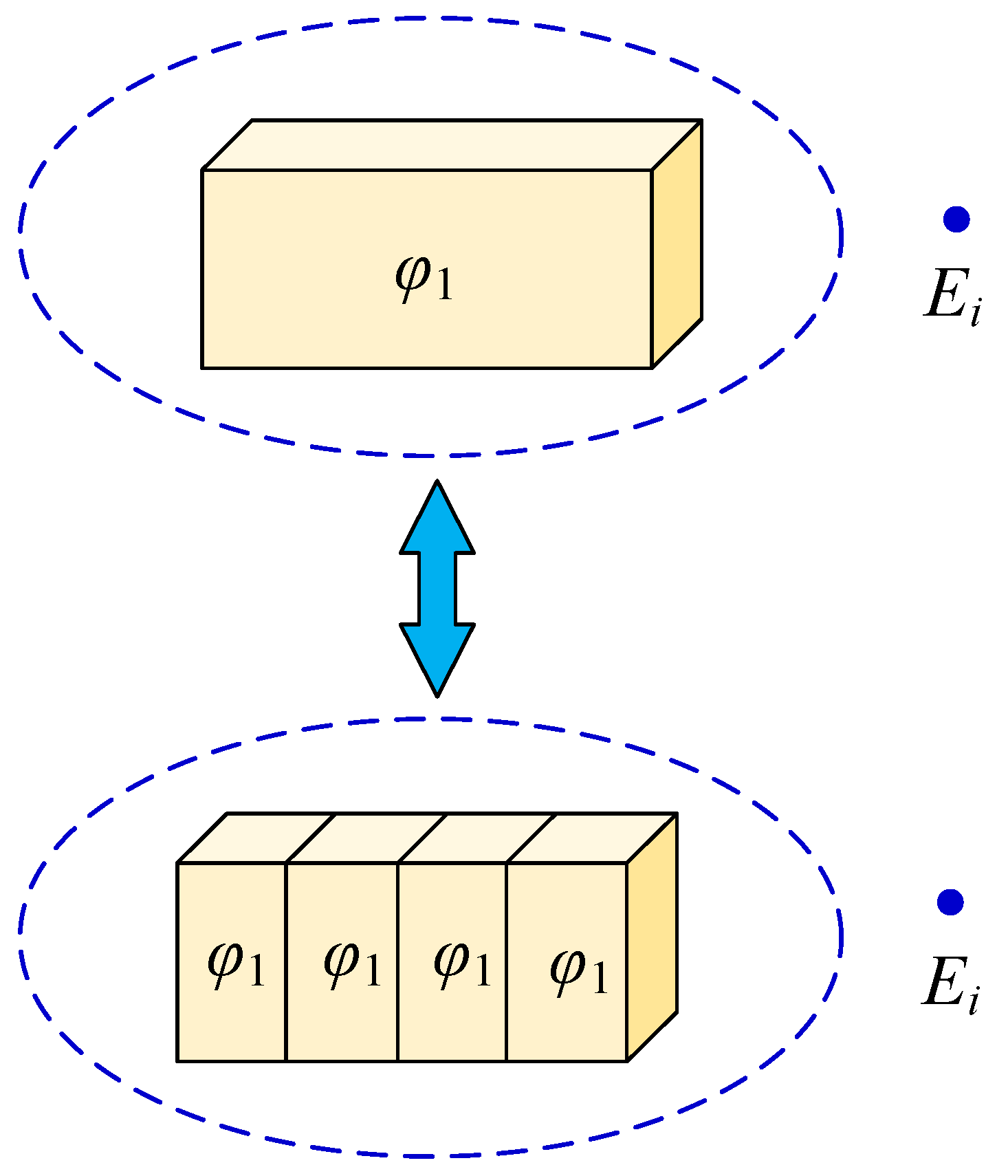
According to Gauss’s law, the electric field strength produced by multiple charged bodies in space exhibits superposition. The electric field generated by multiple conductors at a point in space can be obtained by vector summing the electric fields produced by each conductor at that point. The principle of electric field equivalence between discrete conductors and a continuous conductor is illustrated in Figure 4.

As shown in Figure 4, the electric field distribution of a continuous conductor is consistent with that of discrete equipotential conductors in space.

3. Data Derivation System of Electric Field Modeling

The MMC valve is a core component in the actual operation of the MMC-HVDC power transmission system. As a high-voltage, high-capacity power electronic device, the structure of the MMC valve is determined by the system parameters [23,24]. The design parameters of the MMC valve were derived based on the system parameters. The potential that determines the distribution of the electric field originates from the voltage parameters during system operation.

By conducting a theoretical analysis of the process of establishing the electric field model of the MMC valve and combining it with the relationship analysis of the MMC valve and the main circuit parameters.

The data derivation system for the electric field modeling parameters of the MMC valve is defined as follows:

$$\left\{\begin{array}{l}{X}_{\mathrm{in}}\to \Phi (\mathrm{x})\to Y_{\mathrm{out}}\\ X_{\mathrm{in}}=\left[\begin{array}{l}{U}_{\mathrm{dc}}\\ P_{\mathrm{dc}}\\ Q\end{array}\right]\end{array}\right.$$

(1)

In Equation (1), X_in and Y_out are the input and output matrix, Φ(x) are data derivation system, U_dc is the DC voltage of the MMC, P_dc and Q are the active power and reactive power of MMC. The data derivation system matrix Φ(x) and output Y_out are defined as follows:

$$\Phi (x)=\left[\begin{array}{l}{F}_{\mathrm{sm}}(\mathrm{x})\\ F_{\mathrm{shield}}(\mathrm{x})\\ F_{\mathrm{insulator}}(\mathrm{x})\\ F_{\mathrm{beam}}(\mathrm{x})\\ F_{\mathrm{ring}}(\mathrm{x})\end{array}\right] \mathrm{and} Y_{\mathrm{out}}=\left[\begin{array}{l}{Y}_{\mathrm{sm}}\\ Y_{\mathrm{shield}}\\ Y_{\mathrm{insulator}}\\ Y_{\mathrm{beam}}\\ Y_{\mathrm{ring}}\end{array}\right]$$

(2)

In Equation (2), F_sm(x) is the submodule parameters calculation matrix, F_sheild(x) is the shielding cover parameters calculation matrix, F_insulator(x) is the insulator parameters calculation matrix, F_beam(x) is the support frame parameters calculation matrix, F_ring(x) is the grading ring parameters calculation matrix, Y_sm is the modeling parameters of submodules, Y_sheild is the modeling parameters of shielding covers, Y_insulator is the modeling parameters of insulators, Y_beam is the modeling parameters matrix of support frames, Y_ring is the modeling parameters of grading rings.

According to the principles of electric field calculation for the MMC valve and the analysis of key factors, the electric field modeling parameters for the MMC valve are categorized into five distinct categories. The data derivation system Φ(x) and the modeling parameters data Y_out are structured accordingly. The following sections provide a detailed analysis of each of these five categories.

3.1. Multidimensional Array Modeling of Submodule

The submodule is the fundamental building block of the MMC valve and constitutes its core component of the MMC valve. Each converter valve is typically composed of multiple submodules. The parameter matrix of the submodules is defined as follows:

$$\left\{\begin{array}{l}{Y}_{\mathrm{sm}}=\left[\begin{array}{cccc}{Y}_{\mathrm{sm}11}& Y_{\mathrm{sm}12}& \cdots & Y_{\mathrm{sm}1\mathrm{NSM}}\\ Y_{\mathrm{sm}21}& Y_{\mathrm{sm}22}& \cdots & Y_{\mathrm{sm}2\mathrm{NSM}}\\ ⋮& ⋮& \ddots & ⋮\\ Y_{\mathrm{smCLT}1}& Y_{\mathrm{smCLT}2}& \cdots & Y_{\mathrm{smCLTNSM}}\end{array}\right]\\ Y_{\mathrm{sm}ij}=\left[\begin{array}{l}E{(\mathrm{sm})}_{ij}\\ G{(\mathrm{sm})}_{ij}\\ SP{(\mathrm{sm})}_{ij}\end{array}\right]\end{array}\right.$$

(3)

In Equation (3), Y_smij is the modeling parameters matrix of the submodule j in layer i of the MMC valve, MSN denotes the number of submodules in each layer of the MMC valve, and CLT denotes the number of layers in the MMC valve.

As shown in Equation (3), the electric field modeling parameters of each submodule consist of three distinct sets of parameters, the electrical parameter matrix E(sm), the spatial geometric parameter matrix G(sm), and the spatial location matrix SP(sm).

Due to the metallic conductor nature of the sub-module’s outer casing, the sub-module acquires a certain electric potential during the operation of the MMC valve, with the entire outer casing forming an equipotential body. The electrical parameter matrix E(sm) of the submodule is defined as follows:

$$\left\{\begin{array}{l}E{(\mathrm{sm})}_{ij}=\left[\begin{array}{l}G{V}_{\mathrm{sm}ij}\\ M{C}_{\mathrm{sm}}\end{array}\right]\\ G{V}_{\mathrm{sm}ij}=G{V}_{\mathrm{sm}11}+NS·{\mathrm{u}}_{\mathrm{smd}}=G{V}_{\mathrm{T}}+NS·{\mathrm{u}}_{\mathrm{smd}}\end{array}\right.$$

(4)

In Equation (4), GV_smij is electric potential of the submodule ij in the valve, NS denotes the voltage coefficient, u_smd is the rated voltage of submodule, GV_T is the electric potential of the first submodule in the valve, and MC_sm is the material property of the submodule shell.

The submodule is composed of power devices, capacitors, and other components. Once the type of power device and the parameters of the capacitor are specified, the geometric dimensions of the submodule can be determined. The geometric parameter matrix of the submodule is defined as follows:

$$G{(\mathrm{sm})}_{ij}=EC(\mathrm{sm})=f(\mathrm{Csm},U_{\mathrm{CmN}})=\left[\begin{array}{l}Form\\ X_{\mathrm{S}0}\\ Y_{\mathrm{S}0}\\ Z_{\mathrm{S}0}\end{array}\right]$$

(5)

In Equation (5), EC(sm) is a computational matrix for the geometric dimensions of the submodule, C_sm is rated capacitance of the submodule, U_cmN is the rated voltage of C_sm, Form is the shape structure of the submodule. The dimensions X₀, Y₀ and Z₀ denote the preliminary geometric sizes of the submodule.

The shape structure of the submodule capacitor is obtained from the capacitor manufacturer and typically has a limited range of options. The preliminary geometric sizes of the submodule are generally provided by the structural design engineers. The calculation of geometric parameters adopts a database format, where the geometric parameters of the submodule are determined based on its rated voltage and capacitance.

The calculation methods for the rated voltage of the submodule and the rated voltage of the capacitor are defined as follows:

$$\left\{\begin{array}{l}{I}_{\mathrm{dc}}={\displaystyle \frac{P_{\mathrm{dc}}}{2U_{\mathrm{dc}}}}\\ {\mathrm{u}}_{\mathrm{PSD}}=DB(\mathrm{powerSD}, I_{\mathrm{dc}})\\ {\mathrm{u}}_{\mathrm{smd}}<{\displaystyle \frac{1}{2}}{\mathrm{u}}_{\mathrm{PSD}}\\ U_{\mathrm{CmN}}={\mathrm{k}}_{\mathrm{CU}}{\mathrm{u}}_{\mathrm{smd}}\end{array}\right.$$

(6)

In Equation (6), DB is the parameter database for high-capacity, fully controlled power electronic devices (such as IGBTs), u_PSD is the voltage rating of the device, K_cu is the calculation coefficient for the rated voltage of capacitors.

The submodules are arranged in the MMC valve according to the wiring sequence. The positional parameters of the submodules are determined by the layout method of the valve tower. The calculation method for the positional parameters of the submodules is defined as follows:

$$SP{(\mathrm{sm})}_{ij}=\left[\begin{array}{l}{\mathrm{X}}_{\mathrm{sm}11}+x{m}_{ij}\\ Y_{\mathrm{sm}11}+y{m}_{ij}\\ Z_{\mathrm{sm}11}+z{m}_{ij}\end{array}\right]$$

(7)

In Equation (7), X_sm11, Y_sm11 and Z_sm11 are the coordinates of the first submodule in the MMC valve, xm_ij, ym_ij, zm_ij are the displacement coordinates of submodule ij relative to the first submodule in the MMC valve. These displacement coordinates can be determined based on the rated voltage of the sub-module and their layout configuration.

3.2. Multidimensional Array Modeling of Shielding Cover

The shielding cover significantly influences the electric field distribution of the MMC valve. The shielding covers of the MMC valve generally consist of two types, one with curved shapes located at the four corners, and the other with flat-plate shapes positioned at other locations. The electric field calculation parameters matrix of the MMC valve shielding covers are defined as follows:

$$\begin{array}{l}{Y}_{\mathrm{shieldC}}=\left[\begin{array}{cccc}{Y}_{\mathrm{shieldC}11}& Y_{\mathrm{shieldC}12}& \cdots & Y_{\mathrm{shieldC}1\mathrm{NSC}}\\ Y_{\mathrm{shieldC}21}& Y_{\mathrm{shieldC}22}& \cdots & Y_{\mathrm{shieldC}2\mathrm{NSC}}\\ ⋮& ⋮& \ddots & ⋮\\ Y_{\mathrm{shieldCLT}1}& Y_{\mathrm{shieldCLT}2}& \cdots & Y_{\mathrm{shieldCLTNSC}}\end{array}\right]\\ Y_{\mathrm{shieldP}}=\left[\begin{array}{cccc}{Y}_{\mathrm{shieldP}11}& Y_{\mathrm{shieldP}12}& Y_{\mathrm{shieldP}13}& Y_{\mathrm{shieldP}14}\\ Y_{\mathrm{shieldP}21}& Y_{\mathrm{shieldP}22}& Y_{\mathrm{shieldP}23}& Y_{\mathrm{shieldP}24}\\ ⋮& ⋮& ⋮& ⋮\\ Y_{\mathrm{shieldPLT}1}& Y_{\mathrm{shieldPLT}2}& Y_{\mathrm{shieldPLT}3}& Y_{\mathrm{shieldPLT}4}\end{array}\right]\end{array}$$

(8)

In Equation (8), Y_shieldC denotes the arc-shaped shielding covers at the four corners of each layer, Y_shieldP denotes the flat-plate shielding covers, LT is the number of layers in the MMC valve, and NSC is the number of shielding covers in one layer of the MMC valve.

The electric field modeling parameters of each shielding cover consist of three distinct sets of parameters. The modeling parameters matric of each shield are defined as follows:

$$\left\{\begin{array}{l}{Y}_{\mathrm{shieldC}ij}=\left[\begin{array}{l}E{(\mathrm{shieldC})}_{ij}\\ G{(\mathrm{shieldC})}_{ij}\\ SP{(\mathrm{shieldC})}_{ij}\end{array}\right]\\ Y_{\mathrm{shieldP}ij}=\left[\begin{array}{l}E{(\mathrm{shieldP})}_{ij}\\ G{(\mathrm{shieldP})}_{ij}\\ SP{(\mathrm{shieldP})}_{ij}\end{array}\right]\end{array}\right.$$

(9)

In Equation (9), Y_shieldCij denotesthe modeling parameters of the corner shielding cover j in the layer i of the MMC valve, Y_shieldPij is the modeling parameters of flat plate shielding cover j in the layer i of the MMC valve.

Shielding covers are arranged externally on the MMC valve and serves the functions of electromagnetic shielding and protection. The shielding covers are connected to the nearby submodules, forming an equipotential connection.

The electrical parameter matrix E(shield) of the shield covers is defined as follows:

$$\left\{\begin{array}{l}E{(\mathrm{shieldC})}_{ij}=\left[\begin{array}{l}G{V}_{\mathrm{shieldC}ij}\\ M{C}_{\mathrm{shield}}\end{array}\right]\\ G{V}_{\mathrm{shieldC}ij}=G{V}_{\mathrm{shieldC}11}+DS·{\mathrm{u}}_{\mathrm{smd}}=G{V}_{\mathrm{T}}+DS·{\mathrm{u}}_{\mathrm{smd}}\end{array}\right.$$

(10)

In Equation (10), GV_smij is electric potential of the shield cover ij in the valve, DS is voltage coefficient, MC_shiled is material properties of the shield covers.

During the operation of the MMC valve, the shielding cover neither carries current nor performs power transmission. It protects the MMC valve by optimizing the electric field distribution, which is the primary objective of its design.

The geometric parameter matrix of the shield is defined as follows:

$$G{(\mathrm{shieldC})}_{ij}=EC(\mathrm{shieldC})=\left[\begin{array}{l}Form\\ X_{\mathrm{S}0}\\ Y_{\mathrm{S}0}\\ Z_{\mathrm{S}0}\\ ALR\end{array}\right]$$

(11)

In Equation (11), EC(shield C) is a computational matrix for the geometric dimensions of the corner shielding cover, Form is the shape structure of the shield cover. The dimensions X₀, Y₀ and Z₀ denote the preliminary geometric sizes of the shield cover, ALR are the curvature parameters of the shielding cover.

The geometric parameters of the shielding covers are using a database format. For the MMC valve, the limited variety of shielding cover styles simplifies database establishment. The positional parameters are determined by the layout method of the valve tower. The calculation method for the positional parameters of the shield cover is defined as follows:

$$SP{(\mathrm{shieldC})}_{ij}=\left[\begin{array}{l}{\mathrm{X}}_{\mathrm{SC}11}+x{m}_{ij}\\ Y_{\mathrm{SC}11}+y{m}_{ij}\\ Z_{\mathrm{SC}11}+z{m}_{ij}\end{array}\right]$$

(12)

In Equation (12), X_sc11, Y_sc11 and Z_sc11 are the coordinates of the first shield cover in the MMC valve, xm_ij, ym_ij, and zm_ij are the displacement coordinates of shielding cover ij relative to the first shielding cover in the MMC valve.

3.3. Multidimensional Array Modeling of Grading Ring

Two voltage equalization rings are arranged at the top of the MMC valve, serving the dual functions of voltage equalization and providing connections for the incoming and outgoing lines of the MMC valve. The modeling parameters matric for the equalization rings are defined as follows:

$$\left\{\begin{array}{l}{Y}_{\mathrm{ring}i}=\left[\begin{array}{l}E{(\mathrm{ring})}_i\\ G{(\mathrm{ring})}_i\\ SP{(\mathrm{ring})}_i\end{array}\right]\\ E{(\mathrm{ring})}_i=\left[\begin{array}{l}G{V}_{ringi}\\ M{C}_{\mathrm{ring}}\end{array}\right]\\ G{V}_{ring1}=G{V}_1\\ G{V}_{ring2}=G{V}_1+N_{\mathrm{sm}}·{\mathrm{u}}_{\mathrm{smd}}\end{array}\right.$$

(13)

In Equation (13), Y_ring1 and Y_ring2 are the modeling parameters of the two-voltage equalization ring, GV_ring is electric potential of the ring, N_sm is voltage coefficient, GV₁ is the electric potential of ring 1. The electric field modeling parameters of top voltage equalization rings are also composed of three sets of parameters.

The voltage equalization rings at the top of the MMC valve are typically composed of two C-shaped tubular structures. The geometric parameters matrix and positional parameters matrix are defined as follows:

$$G{(\mathrm{ring})}_i=EC(\mathrm{ring})=\left[\begin{array}{l}Form\\ R\\ X_{r0}\\ Y_{r0}\\ Z_{r0}\\ ALR\end{array}\right] \mathrm{and} SP{(\mathrm{ring})}_i=\left[\begin{array}{l}{\mathrm{X}}_{\mathrm{sm}1}+xr{m}_i\\ Y_{\mathrm{sm}1}+yr{m}_i\\ Z_{\mathrm{sm}1}+zr{m}_i\end{array}\right]$$

(14)

In Equation (14), EC(ring) is a computational matrix for the geometric dimensions of the voltage equalization ring, Form is the shape structure, xrm_i, yrm_i, and zrm_i are the displacement coordinates of voltage equalization ring i relative to another. The geometric parameters employs a database format. This limited variety makes it easier to establish a database.

3.4. Multidimensional Array Modeling of Support Frame

The support frame is one of the most critical components in the valve tower structure, serving to support and secure the MMC valve. Typically, the MMC valve support frame is composed of insulating beams and metallic beams. The electric field calculation parameters matrix of the MMC valve shield is defined as follows:

$$\left\{\begin{array}{l}{Y}_{\sup }=\left[\begin{array}{l}{Y}_{\mathrm{beamI}}\\ Y_{\mathrm{beamM}}\end{array}\right]\\ Y_{\mathrm{beamI}}=\left[\begin{array}{cccc}{Y}_{\mathrm{beamI}11}& Y_{\mathrm{beamI}12}& \cdots & Y_{\mathrm{beamI}1\mathrm{NBC}}\\ Y_{\mathrm{beamI}21}& Y_{\mathrm{beamI}22}& \cdots & Y_{\mathrm{beamI}2\mathrm{NBC}}\\ ⋮& ⋮& \ddots & ⋮\\ Y_{\mathrm{beamICLT}1}& Y_{\mathrm{beamICLT}2}& \cdots & Y_{\mathrm{beamICLTNBC}}\end{array}\right]\\ Y_{\mathrm{beamI}ij}=\left[\begin{array}{l}E{(\mathrm{beamI})}_{ij}\\ G{(\mathrm{beamI})}_{ij}\\ SP{(\mathrm{beamI})}_{ij}\end{array}\right]\\ E{(\mathrm{beamI})}_{ij}=M{C}_{\mathrm{beamI}}={\epsilon }_{\mathrm{beamI}}\end{array}\right.$$

(15)

In Equation (15), Y_beamI is the modeling parameters of insulating beam, Y_beamM is the modeling parameters of metallic beam, CLT is the number of layers in the MMC valve, NBC is the number of insulating beams in one layer of the MMC valve.

As shown in Equation (15), the electric field modeling parameters are also composed of three sets of parameters. The electrical parameter matrix of insulating beams are solely characterized by the dielectric constant of the insulating material. The geometric parameters matrix and positional parameters matrix are defined as follows:

$$\left\{\begin{array}{lll}G{(\mathrm{beamI})}_{ij}=EC(\mathrm{beamI})=\left[\begin{array}{l}Form\\ X_{\mathrm{S}0}\\ Y_{\mathrm{S}0}\\ Z_{\mathrm{S}0}\end{array}\right]& \mathrm{and} & SP{(\mathrm{beamI})}_{ij}=\left[\begin{array}{l}{\mathrm{X}}_{\mathrm{BI}11}+xbi{m}_{ij}\\ Y_{\mathrm{BI}11}+ybi{m}_{ij}\\ Z_{\mathrm{BI}11}+zbi{m}_{ij}\end{array}\right]\end{array}\right.$$

(16)

In Equation (16), EC(beamI) is a computational matrix for the geometric dimensions of the insulating beams, Form is the shape structure, X_BI11, Y_BI11, and Z_BI11 are the displacement coordinates of beamI₁₁ in the MMC valve, xbim_ij, ybim_ij, and zbim_ij are the displacement coordinates of insulating beams i relative to the beamI₁₁.

3.5. Multidimensional Array Modeling of Insulator

The primary design of the MMC valve currently adopts the supported valve tower structure, in which the interlayer insulators and ground insulators are important components of the valve tower. The electric field modeling parameters matrix of the MMC valve insulators are defined as follows:

$$\begin{array}{l}{Y}_{\mathrm{insulatorL}}=\left[\begin{array}{cccc}{Y}_{\mathrm{insulatorL}11}& Y_{\mathrm{insulatorL}12}& \cdots & Y_{\mathrm{insulatorL}1\mathrm{NIL}}\\ Y_{\mathrm{insulatorL}21}& Y_{\mathrm{insulatorL}22}& \cdots & Y_{\mathrm{insulatorL}2\mathrm{NIL}}\\ ⋮& ⋮& \ddots & ⋮\\ Y_{\mathrm{insulatorLLT}1}& Y_{\mathrm{insulatorLLT}2}& \cdots & Y_{\mathrm{insulatorLLTNIL}}\end{array}\right]\\ Y_{\mathrm{insulatorG}}=\left[\begin{array}{cccc}{Y}_{\mathrm{insulatorG}1}& Y_{\mathrm{insulatorG}2}& \cdots & Y_{\mathrm{insulatorGNIG}}\end{array}\right]\\ Y_{\mathrm{insulator}ij}=\left[\begin{array}{l}E{(\mathrm{insulator})}_{ij}\\ G{(\mathrm{insulator})}_{ij}\\ SP{(\mathrm{insulator})}_{ij}\end{array}\right]\\ E(\mathrm{insulatorL}/\mathrm{G})=M{C}_{\mathrm{insulator}}=M{C}_{\mathrm{insulator}}={\epsilon }_{\mathrm{insulator}}\end{array}$$

(17)

In Equation (17), Y_insulatorL is the modeling parameters of interlayer insulators, Y_insulatorG is the modeling parameters of ground insulators, NIG is the number of ground insulators, NIL is the number of interlayer insulators in one layer.

The electrical parameter matrix of insulators are solely characterized by the dielectric constant of the insulating material. The geometric parameters matrix and positional parameters matrix are defined as follows:

$$\left\{\begin{array}{l}G{(\mathrm{insulatorL})}_{ij}=EC(\mathrm{insulatorL})=\left[\begin{array}{l}Form\\ R_1\\ R_2\\ NP\\ hL\end{array}\right]\\ hL=f_V(N_L·{\mathrm{u}}_{\mathrm{smd}})\\ NP=f_N(hL,F)\\ SP{(\mathrm{insulatorL})}_{ij}=\left[\begin{array}{l}{\mathrm{X}}_{\mathrm{SC}11}+x{m}_{ij}\\ Y_{\mathrm{SC}11}+y{m}_{ij}\\ Z_{\mathrm{SC}11}+z{m}_{ij}\end{array}\right]\end{array}\right.$$

(18)

In Equation (18), EC(insulatorL) is a computational matrix for the geometric dimensions of the interlayer insulators, Form is the shape structure, R₁ is the shed radius, R₂ is the core radius, hL is the height of the insulator, and NP is the number of shed layers, X_SC11, Y_SC11 and Z_SC11 are the displacement coordinates of interlayer insulator in the MMC valve, xm_ij, ym_ij and zm_ij are the displacement coordinates of insulator L ij relative to the insulator L₁₁. The hL is correlated with the voltage difference between layers, which is equal to the sum of the voltages of the sub-modules in each layer. The geometric parameters matrix and positional parameters matrix of ground insulators are defined as follows:

$$\left\{\begin{array}{l}G{(\mathrm{insulatorG})}_i=GEC(\mathrm{insulatorG})=\left[\begin{array}{l}Form\\ R_{1G}\\ R_{2G}\\ NPG\\ hG\end{array}\right]\\ NPG=f_N(hG,Form)\\ hG=f_V[SIWL(VTG),LIWL(VTG)]\\ SP{(\mathrm{insulatorG})}_i=\left[\begin{array}{l}{\mathrm{X}}_{\mathrm{SC}1}+xg{m}_i\\ Y_{\mathrm{SC}1}+yg{m}_i\end{array}\right]\end{array}\right.$$

(19)

In Equation (19), EC(insulatorG) is a computational matrix, Form is the shape structure, hG is the height of the insulator, and NPG is the number of shed layers on the insulator, X_SC1 and Y_SC1 are the displacement coordinates of ground insulator in the MMC valve, xgm_i and ygm_i are the displacement coordinates of insulatorG_i relative to the insulator G1. The height of the ground insulator corresponds to the air clearance between the MMC valve and the ground, which is determined by the withstand overvoltage of MMC-HVDC system.

The materials used in the MMC valve, including insulators, supports, sub-module enclosures, and shielding covers, are all linear materials. During the operation of the MMC valve, the permittivity, susceptibility, and other material constants of these components remain constant.

4. Geometric Discrete Modeling Method

To improve the accuracy and speed of calculations, data derivation are used to discretize and model the key metal components of the converter valve. The size discretization method is defined as follows:

$$\left\{\begin{array}{l}{L}_{\mathrm{shield}}\\ W_{\mathrm{shield}}\\ H_{\mathrm{shield}}\end{array}\right.\to M(\mathrm{x})\to \left\{\begin{array}{l}{\displaystyle \sum L_{\mathrm{shield}j}}\\ {\displaystyle \sum W_{\mathrm{shield}j}}\\ {\displaystyle \sum H_{\mathrm{shield}j}}\end{array}\right.$$

(20)

In Equation (20), M(x) denotes the discretization parameters data derivation system, L_shield, W_shield, and H_shield are the actual dimensions of the shielding cover, L_shieldj, W_shieldj, and H_shieldj are the distributed geometric parameters.

The discretization modeling dimensions of the shielding cover are derived based on the relationship between the electric field and the meshing dimensions. The shielding cover significantly impacts the electric field distribution of the MMC valve, with the maximum electric field intensity typically located on its outer surface. The following section explains the basic principles of distributed modeling for the shielding cover.

Shielding cover is divided into two parts, the cubic section and the curved section. The discrete modeling parameters for the cubic section are defined as follows:

$$\left\{\begin{array}{l}\min (X_{\mathrm{SP}0},Y_{\mathrm{SP}0},Z_{\mathrm{SP}0})=M_{L0}\\ N_{\mathrm{a}0}=⌈{\displaystyle \frac{X_{\mathrm{SP}0}}{M_{L0}}}⌉\\ N_{\mathrm{b}0}=⌈{\displaystyle \frac{Y_{\mathrm{SP}0}}{M_{L0}}}⌉\\ N_{\mathrm{c}0}=⌈{\displaystyle \frac{Z_{\mathrm{SP}0}}{M_{L0}}}⌉\end{array}\right.$$

(21)

In Equation (21), M_L0 the minimum value among the length X_SP0, width Y_sp0 and height Z_sp0, N_a0, N_b0, and N_c0 are the dimension proportionality coefficients. These ceilings have a value of the ratio between the length, width, height and their minimum value.

The dimensions of the cubic section are discretized based on the dimension proportionality coefficient. The mesh division influence factor is proposed by discretized modeling parameters and principle of mesh partitioning. The modeling of the discrete size units and the mesh division influence factor are defined as follows:

$$\left\{\begin{array}{l}{X}_{\mathrm{eL}}={\displaystyle \frac{X_{\mathrm{SP}0}}{N_{\mathrm{a}0}}}\\ Y_{\mathrm{eL}}={\displaystyle \frac{Y_{\mathrm{SP}0}}{N_{\mathrm{b}0}}}\\ Z_{\mathrm{eL}}={\displaystyle \frac{Z_{\mathrm{SP}0}}{N_{\mathrm{c}0}}}\\ \min (X_{\mathrm{eL}},Y_{\mathrm{eL}},Z_{\mathrm{eL}})=L_{m0}\\ L_{meshM}={\displaystyle \frac{L_{m0}}{N_{\mathrm{m}}}}\end{array}\right.\mathrm{and} \left\{\begin{array}{l}\min (L_{\mathrm{eLh}},r_h)=M_{0\mathrm{H}}\\ L_{mh0}={\displaystyle \frac{M_{0\mathrm{H}}}{3}}\\ L_{mMh0}={\displaystyle \frac{L_{mh0}}{N_h}}\end{array}\right.$$

(22)

In Equation (22), L_m0 is the minimum value among the discrete modeling size units, X_eL, Y_eL, and Z_eL are the discrete modeling size units of the cubic section of the shielding cover, N_m is the mesh division influence factor, L_meshM the maximum edge length of the mesh partitioning for the rectangular part of the shielding cover, M_oH is the minimum value among the curved part size units, L_eLh is the extension value or length of the curved section, r_h is the radius of the curvature, L_mh0 is the discrete modeling size units of the curved part of the shielding cover, N_h is the mesh division influence factor, L_mMh0 is the maximum edge length of the mesh partitioning for the curved section.

In distributed modeling, both computational efficiency and accuracy be considered. Based on mesh partitioning principles, a partitioning influence factor is proposed in the design of the data derivation system. By suing the factor N_m or N_h, the electric field calculation for the MMC valve achieves rapid convergence while maintaining computational accuracy.

5. Electric Field Calculation Results

Based on the aforementioned discussion, an electric field calculation model has been established using the high-speed modeling method proposed in this paper. To verify the effectiveness of the high-speed modeling method described in this paper, a ±320 kV 1000 MW MMC-HVDC system that has been in operation for more than five years was selected as the research object. In the engineering design and operation process, there have been extensive studies and verifications on the electrostatic field of the converter valve.

The main parameters of ±320 kV, 1000 MW MMC-HVDC transmission system are shown in

Table 1. Main parameters of onshore MMC-HVDC system.

Parameters of System	Value
DC voltage Udc	±320 kV
DC transmission power Pdc	1000 MW
Connection configuration	symmetrical bipolar
Sub-module topological	half-bridge

.

In accordance with data derivation system described previously, the system parameters in Table 1 are calculated and derived. The resulting parameters for the MMC valve are presented in

Table 2. Parameters calculated by the data-derived system.

Parameters of MMC Valve	Value
DC voltage Udc	1.5625 kV
Rated voltage of the IGBT	3.3 kV
Rated voltage of sub-module	1.6 kV
Rated voltage of capacitor	2.1 kV
Capacitance of sub-module	10 mF
Rated number of sub-modules per arm	200
Number of sub-modules per valve	72

.

According to the method described in Equation (6), the dimensions of the capacitor can be determined from its capacitance and rated voltage, allowing for the rapid construction of the electric field analysis model for the submodule.

The valve tower features a three-tier structure, with each valve component comprising six sub-modules. The dimensions and structure of the MMC valve support frame can be readily determined based on Equations (15) and (16). For components such as the shielding cover and grading ring, the limited available forms facilitate easy determination of their dimensions. The component models of the submodule, support frame, grading ring, and shielding cover are illustrated in Figure 5.

The modeling parameters for interlayer insulators can be derived from Equation (18) based on the number of sub-modules per layer. The insulation level of the MMC valve to ground is determined by the DC voltage of the MMC-HVDC system, and the modeling parameters of the ground insulators can be obtained from Equation (19).

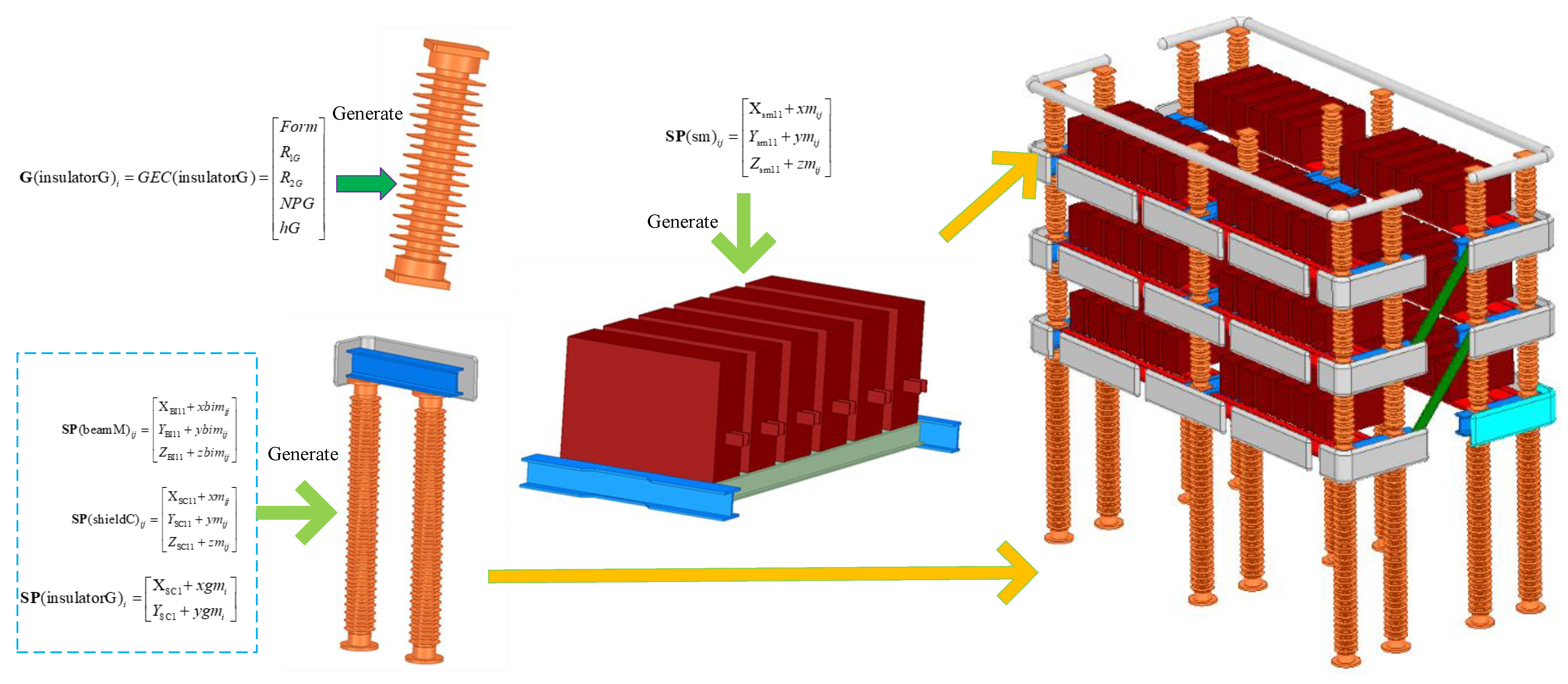
By leveraging the position parameters of all components, which are calculated using the data derivation system, the electric field model of the MMC valve can be rapidly established. The process of establishing the electric field model for the MMC valve is illustrated in Figure 6.

The maximum electric field on the outer surface of the MMC valve is located at the surface of the shielding cover. To achieve faster and more accurate calculation of the electric field strength, the shielding cover is discretized for modeling parameter calculation in accordance with Equation (22). The values of N_m and N_h m are both initially set to 1, discretization modeling and mesh are shown in Figure 7.

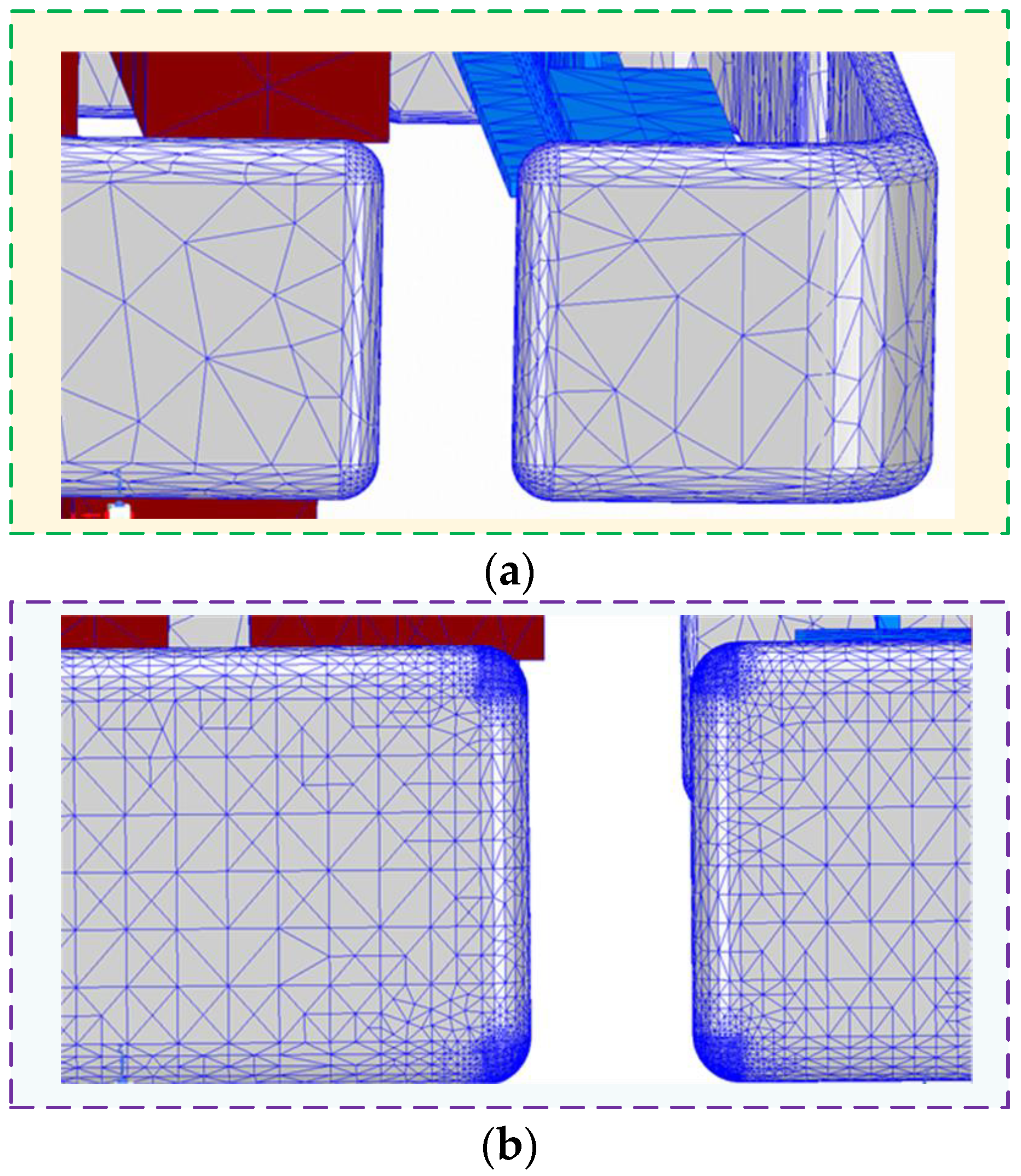
To compare the advantages of the method proposed in this paper, electric field modeling and calculation of the MMC valve are investigated using conventional analysis methods. The initial meshing diagrams for conventional modeling and distributed modeling are shown in Figure 8.

As shown in Figure 8, the electric field model established using the conventional method has poor initial meshing quality, which fails to meet the required accuracy for field computation. When using the conventional method for electric field calculation, it is necessary to perform mesh refinement and optimization specifically tailored to the layout positions.

This process typically requires multiple iterations of computation. The maximum electric field strength is used as a reference value. The relationship between calculation error rate, mesh density, and calculation time is shown in Figure 9.

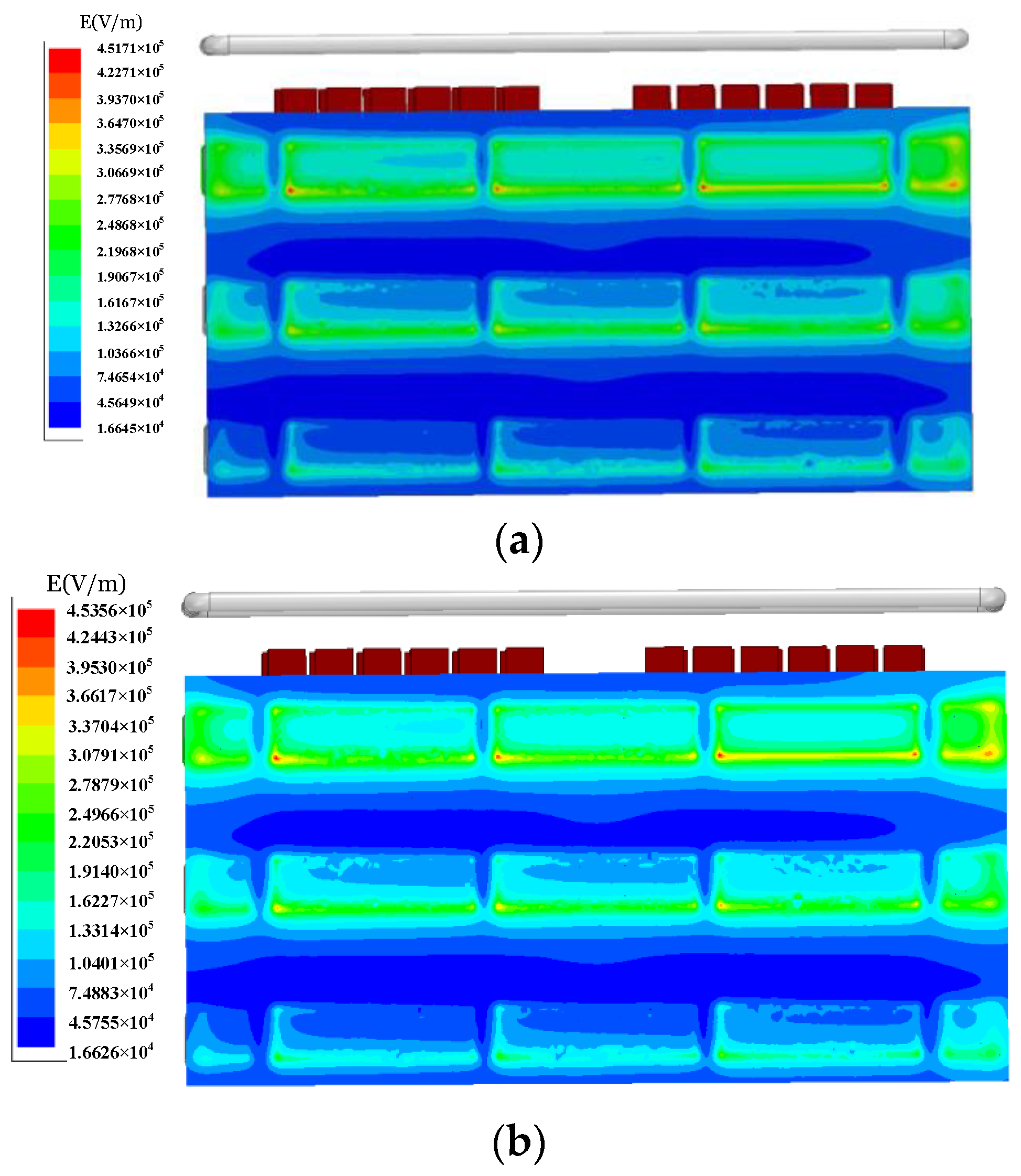
As shown in Figure 9, in the conventional calculation process, multiple mesh refinements are required to obtain a relatively accurate electric field strength, and the calculation time is relatively long. The electric field distribution obtained using the conventional method after multiple iterations and the electric field distribution obtained using the method proposed in this paper (with N_m = 1 and N_h = 1) in a single calculation are shown in Figure 10.

As shown in Figure 10, electric field strength of the MMC is calculated rapidly and accurately using the high-speed modeling method proposed in this paper.

Methods such as the boundary element method, the method of the equivalent gas conductivity, and the instantaneous potential load method are not applicable for the external electric field analysis and stray capacitance calculation of the MMC valve, and the modeling process is still too complex.

The traditional finite element electric field modeling and calculation method proposed in this paper has been applied in practical engineering and has been verified for its correctness through insulation design and fault analysis in practical engineering. The traditional method, which has been proven effective in practice, was selected in this paper to be compared with the high-speed modeling method.

The analysis in this paper focuses on the electric field distribution of the MMC valve under the normal operating conditions of MMC-HVDC system. The comparison of the electric field calculation process using the high-speed modeling method presented in this paper and the conventional method is shown in

Table 3. Comparison results of the two methods for electric field calculation.

Modeling Methods	Modeling Time	Mesh Refinement Factor	Maximum Electric Field Strength	Error Rate/%	Simulation Time
Conventional methods	>120 h	1 (initial)	0.368 kV/mm	18.4	2 h
		6	0.3939 kV/mm	12.66	10 h
		10	0.415 kV/mm	7.95	13 h
		15	0.429 kV/mm	4.88	14 h
		30	0.438 kV/mm	2.88	15 h
		40	0.452 kV/mm	0.22	20 h
		50	0.455 kV/mm	0.87	26 h
		60	0.451 kV/mm	0	30 h
		75	0.451 kV/mm	0	41 h
High-speed methods	<16 h	No operation	0.453 kV/mm	0.4	4 h

.

As shown in Table 3, the high-speed electric field modeling method proposed in this paper, based on the characteristics of electric field and its main influencing factors, directly derives modeling parameters from system parameters through data derivation. By omitting the process of simplifying mechanical models to electric field models, the modeling time is significantly reduced. Additionally, for regions of interest in the electric field, a distributed modeling approach is adopted, integrating the meshing process with model construction, thereby substantially enhancing the accuracy and speed of electrostatic field calculations.

The establishment of the modeling parameter matrix requires a high level of professional knowledge from researchers and technicians. They need to be familiar with both the MMC-HVDC system and the MMC valve equipment and possess both theoretical and practical knowledge simultaneously.

6. Conclusions

A high-speed modeling method for the MMC valve based on data derivation is proposed in this paper. The data derivation is used to generate multiple sets of parameters from a limited number of system input parameters (three to four). These derived parameters are then utilized to establish the electric field analysis model for the MMC valve. Firstly, the relationship between the MMC valve electric field calculation model parameters and the MMC-HVDC transmission system parameters was studied. Then, the data derivation system for the electric field calculation model parameters of all components of the MMC valve was established. Modeling parameters databases based on empirical knowledge is also used in the calculation process of the data derivation system. The output model parameter matrix includes the geometric parameters, position parameters, and electrical parameters of the components. The electric field calculation model of the MMC valve can be quickly generated by output model parameter matrix. Furthermore, to improve the accuracy of the calculations, a discrete modeling method that combines mesh optimization was proposed. A mesh division influence factor based on the accuracy of electric field calculation is proposed. Finally, the method proposed in this paper was applied to the ±320 kV MMC-HVDC valve for electric field modeling and calculation. The numerical results demonstrates that the high-speed modeling method proposed in this paper can significantly reduce the modeling time of the MMC valve but also enhances the accuracy of electric field calculations compared to conventional approaches.

In the next phase of our research, we will focus on further refining and enhancing the database. Additionally, we plan to digitize more equipment design processes. By utilizing operational data and test results from practical applications, we aim to improve the high-speed modeling theory. We will also incorporate intelligent algorithms to achieve more precise and intelligent electrostatic field analysis modeling of converter valves during the high-speed modeling process. In future research, it is essential to employ digitalization techniques to bridge the gap between practical device parameters and theoretical scientific research. The integration of electrostatic field analysis with system operation through unified artificial intelligence (AI) analysis and fault prediction for converter valves represents a significant direction for future development.