The Investigation of Porcelain Plates Roughness Influence on Determination of Explosives Friction Sensitivity

Abstract

The influence of the roughness of porcelain plates on the results of the friction sensitivity test of explosives was investigated. For this purpose, the roughness of selected batches of plates from several manufacturers (Julius Peters, OZM Research s.r.o., Deltima Precision s.r.o.) was determined. Subsequently, according to the standards EN 13631-3:2005 and STANAG 4487 JAIS (edition 2), friction sensitivity tests of PETN (Pentaerythritol Tetranitrate, penthrite) and RDX (Royal Demolition Explosive, hexogen) were carried out. No statistically significant correlation was established under the experimental conditions—although sanded plates had the lowest roughness (mean value 5.07 μm) and simultaneously gave the lowest sensitivity results (168 N for RDX and 80 N for PETN according to the EN 13631-3 while 216 N for RDX and 121 N for PETN according to the STANAG 4487), Julius Peters plates with a similar level of roughness (mean value 6.07 μm) did not reflect the pattern and results of the sensitivity tests that were surprisingly high (120 N for RDX and 64 N for PETN according to the EN 13631-3 while 182 N for RDX and 67 N for PETN according to the STANAG 4487). Due to these results, the human factor is indicated as a key factor in the obtained discrepancies; however, further research in this matter may be needed.

1. Introduction

Due to the development of the explosives industry, there is a need to define the requirements for their safe manufacturing, storage, transport, and use. The discrepancies between the effects of different mechanical, thermal, or electrical stimuli on the tendency of materials to undergo explosive decomposition have been noticed quite quickly. Consequently, different methods have been developed to determine the sensitivity of explosives depending on the chosen stimulus.

The most common and impossible to completely eliminate stimulus is friction, which is present during the handling, mixing, and packaging of a substance. Moreover, the ability to convert a part of the frictional energy into thermal energy must be considered when manipulating a given material. Consequently, several methods have been developed to determine the frictional sensitivity of an explosive, while minimizing the risk of other stimuli accompanying the frictional force, such as heat release and additional impact on the sample. Avrami and Hutchinson, in agreement with Brown [1,2], listed three categories of friction sensitivity testing methods:

• In which a thin layer of material is placed between two rigid elements, which then act on the material in a reciprocating or rotary-continuous motion;
• In which a block of material is rubbed violently against an abrasive surface;
• In which the explosive sample is deformed significantly during impact (a combination of frictional and impact stimuli suitable only for secondary explosives).

Most tests used worldwide belong to the first category and differ in the design of the apparatus in which the explosive is placed, the type of frictional surfaces, and the nature of the movement acting on the sample.

Among these is the Bundesanstalt für Materialforschung und -prüfung (BAM) friction test developed by Germans and standardized in Europe. It is carried out using a Julius Peters apparatus. The apparatus has a table for placing grooved porcelain plates and a notched arm on which weights of a chosen mass and at a chosen distance from the end of the arm are hung so as to obtain a target value of the friction force acting on the sample. Approximately 10 mm³ of the test substance is placed on a porcelain plate attached to the apparatus carriage. A porcelain peg attached to the arm acts on the sample from above [3]. Depending on the type of tested material (primary or secondary explosive), a smaller- or larger-scale Julius Peters apparatus is used. Loads for primary materials range from 0.1 to 10 N, while for secondary materials the range is 5 to 353 N [4,5]. Each time, the test result for a given load is assessed in terms of the occurrence of flame, sparkle, crackling, odor of decomposition products, and/or left trace on the plate. The final sensitivity of the material is determined based on the chosen standard.

There is a known issue with a certain degree of subjectivity in the evaluation of test results. The BAM friction test provides less clear results than the analogous impact test, particularly in the case of an inexperienced researcher. The method of preparing the samples for testing and the size of the crystals also have a significant impact on the obtained sensitivity parameters. For azides with different crystal sizes, Bowden and Singh noticed the divergent nature of decomposition dynamics: larger crystals displayed a tendency to decompose rapidly during heating and were also more sensitive to impact [6]. The influence of the method of sample preparation and crystal size on friction sensitivity was analyzed by Matyas et al. [7]. Radacsi et al. [8] observed that the BAM friction test yields unreliable results for sub-micrometer-sized RDX and HMX (High Molecular weight Explosive, octogen) particles, as the crystals diffuse across the surfaces of the plates and fill the grooves.

The results of the 2014 edition of the Round Robin Test on Explosives (RRTE) indicate significant differences in the results of sensitivity determinations (shown in

Table 1. Friction sensitivity of double-base propellant determined according to the European standard during the RRTE 2014 edition [9].

No. of Laboratory	Porcelain Components Manufacturer	Determined Sensitivity [N]
1	No data	192
2	OZM Research s.r.o.	168
3	Reichel and Partner GmbH	240 and 252 1
4	OZM Research s.r.o.	240
5	No data	168 and 216
6	OZM Research s.r.o.	96, 108 and 128 1
7	OZM Research s.r.o.	288
8	Julius Peters	96
9	OZM Research s.r.o.	168

¹ From various technicians.

) depending on the laboratories carrying out tests and the porcelain components used (sensitivity was determined for identical samples of double-base propellant). Limiting the number of manufacturers of plates and pegs during these studies significantly reduced the discrepancies from the range of 96–288 N to the range of 96–160 N, leaving only room for differences caused by the human factor (

Table 2. Friction sensitivity of double-base propellant determined after choosing one manufacturer of porcelain components [9].

No. of Laboratory	Porcelain Components Manufacturer	Determined Sensitivity [N]
1	OZM Research s.r.o.	128
3	OZM Research s.r.o.	108
5	OZM Research s.r.o.	160
6	OZM Research s.r.o.	96
10	OZM Research s.r.o.	112

) [9].

Furthermore, another discrepancy in the results of friction sensitivity determinations for penthrite and hexogen (according to the statistical Bruceton’s method) is mentioned in STANAG 4487 (edition 2) [10]. The sensitivity of PETN ranges between 56 and 102 N, whereas the range for RDX is 110–185 N. Such differences may be due to possible wetting of the samples (resulting in the phlegmatisation of the material [11]); nevertheless, the influence of the applied porcelain components may also be the reason. The results of comparative tests between Polish laboratories (carried out in 2008) also show differences in the obtained values, with results ranging between 107 and 240 N for crystalline RDX samples tested according to the European standard [12].

Le Roux highlighted the variation in the roughness of the available porcelain plates. He suggested using an emery paper while testing primary explosives owing to its normalized surface (in comparison with the lack of uniformity of roughness for plates) [13].

Both the plates and pegs for the BAM friction test have standardized dimensions. The grooves are made with a special sponge imprinted before firing the ceramics. The European standard [14] also specifies requirements for the surface roughness of the plates: the arithmetic mean of the plate profile deviations (Ra parameter) should be between 6 and 15 µm, with individual readings between 5 and 19 µm. STANAG 4487 (edition 2) does not specify the roughness of plates.

Currently, there are no requirements for checking the roughness of plates prior to friction sensitivity testing. However, the discrepancies in the results raise questions as to whether such a procedure should be introduced, especially when the examined explosives are on the edge of admission for civil use. According to European standards secondary explosives should not be sensitive to loads lower than 80 N (EN 13631-1:2005 [15]) whereas in the case of explosives used for detonating cords, the limit is 30 N (EN 13630-1:2003 [16]).

This paper presents an attempt to determine the effect of porcelain plate roughness on friction test results. To this end, the profiles of selected types of plates available on the European market were measured and the number of scratches on the plates was visually assessed. The results of the measurements were compared with the results of subsequent sensitivity tests for penthrite and hexogen, according to Bruceton’s statistical method and the European standard EN 13631-3:2005.

2. Materials and Methods

Porcelain plates produced by the following manufacturers were selected for testing:

• Julius Peters;
• OZM Research s.r.o. (Hrochův Týnec, Czech Republic);
• Deltima Precision s.r.o. (Praha, Czech Republic).

For each batch of plates, OZM pegs were used. Julius Peters plates were noticeably thicker (approx. 5.5 mm, while it was approx. 5 mm for the others). In addition, only one side of the plates was designed for measurements; significant scratches were carved on the other side (Figure 1).

Due to the large differences between the two batches of the owned OZM plates, it was decided to carry out the experiments for both of them. Older batch plates had an uneven shape, and their thickness, distribution, and depth of grooves varied within a single plate. The newer batch did not have such flaws. Moreover, a part of the new batch was sanded with emery paper (60 grit) to purposely reduce its roughness and compare the obtained results. Fifty plates from each plate type, including sanded ones, were tested (with the exception of the single-sided Julius Peters plates, for which one hundred units were chosen). Each time, each side of the plate was tested in triplicate.

To determine the profile of a given surface, a number of contact or non-contact methods may be applied, each of which differs in the simplicity of the application under given laboratory conditions, the range of roughness to be measured, and the ease of handling the output data [17]. In addition, more precise methods for the measurement and processing of surface profiles are still being developed [18,19]. In this study, the contact method for roughness measurement was chosen. For this purpose, a SURFTEST SJ-410 surface roughness tester (software Ver. 2.00) from Mitutoyo (Kanagawa, Japan) was used, and the following three parameters were measured:

• Ra—arithmetical mean of absolute profile deviations;
• Rt—total height of profile;
• Rz1max—maximum roughness depth [20].

A visual assessment of the roughness was also carried out. First, magnified photographs of the plates were taken to apply a filter. The number of grooves per measurement section of the plate was then assessed.

In the second part of the study, material for the indication of friction sensitivity, which included crystalline RDX (provided by NITRO-CHEM S.A., Bydgoszcz, Poland) and crystalline PETN (provided by SSE Group, Brig, Switzerland), was chosen. The results of the granulometric analysis of the substances used are shown in Figure 2 and Figure 3. The distributions of RDX and PETN fractions were obtained using a laser analyzer ANALYSETTE 22 MicroTec plus (Fritsch GmbH, Idar-Oberstein, Germany).

The assessment of friction sensitivity was carried out by a single technician (in order to avoid the variable of different operators) according to the standards EN 13631-3:2005 and STANAG 4487 JAIS (Editon 2), using Julius Peters apparatus. In the European standard friction sensitivity is defined as the lowest peg load at which a reaction occurs in at least one of the six tests [14]. However, in STANAG the sensitivity of an explosive is described with a 50% point, i.e., a load calculated using the Bruceton statistical method (also known as the up-and-down method), for which the probability of initiation is 50%. To obtain the 50% point, at least 25 tests are required [10]. Noteworthy, the up-and-down method, established in the 1940s, has many limitations (e.g., the condition of choosing an appropriate interval for loading the arm during the test, so that the value of the interval divided by the obtained standard deviation is between 0.5 and 2), which has led to the development of new methods for calculating the 50% point, like the Neyer D-optimal method or the probit analysis [21,22,23]. Nevertheless, Bruceton’s method is still commonly used to determine explosives’ sensitivity, e.g., in the United States [24].

3. Results

3.1. Roughness Evaluation

3.1.1. Plates Visual Comparison

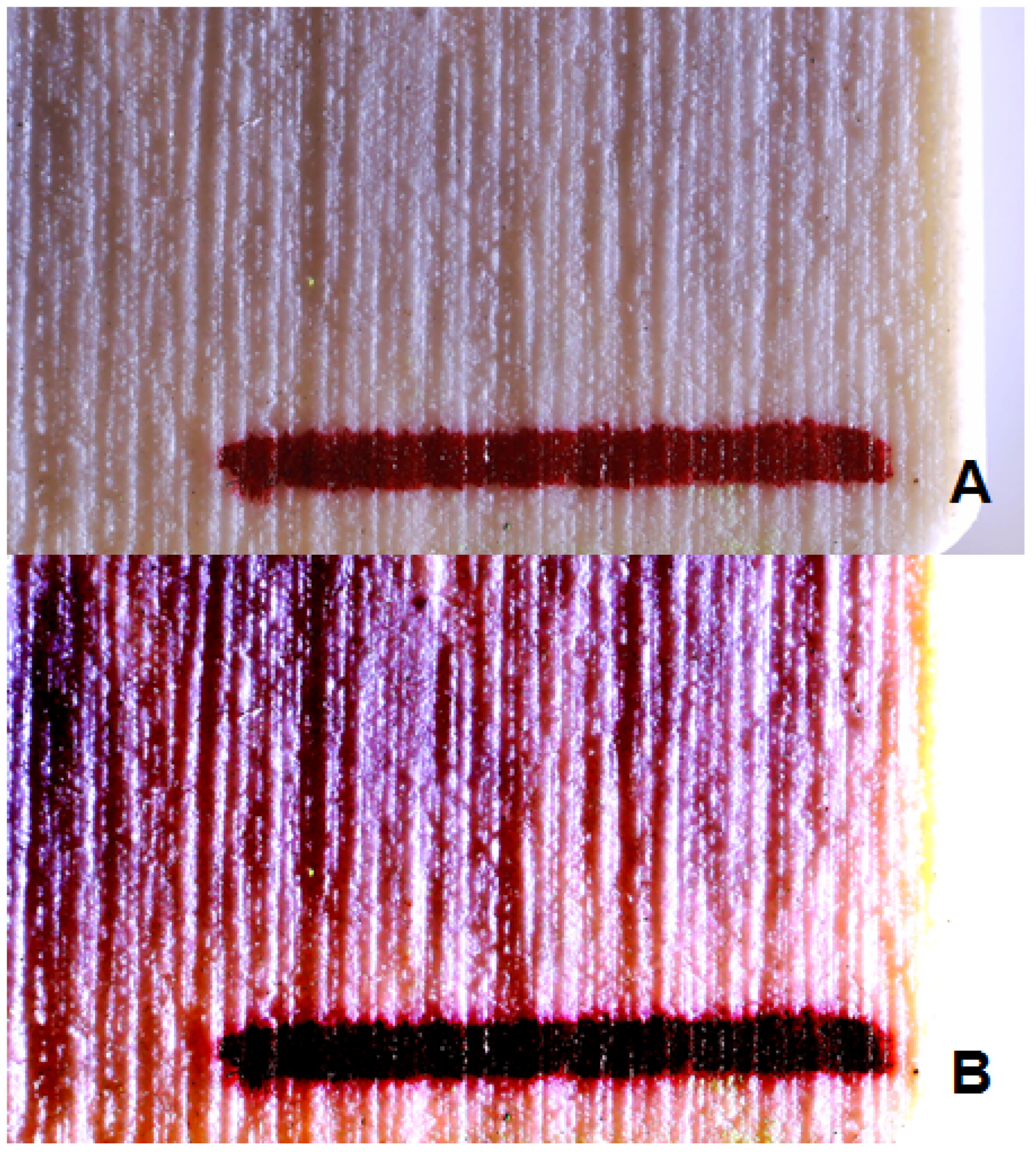
During the visual assessment of the plates surfaces, magnified photographs were taken. Photographs before and after the filter was applied are shown in Figure 4, Figure 5, Figure 6 and Figure 7. The red sections visible in the photos are the measurement sections of 1 cm, for which the number of grooves was counted (

Table 3. Results of plates visual comparison.

Plate Type	Grooves per 1 cm (Mean Value)	Easy Identification of Single Groove	Surface Homogeneity
Julius Peters	16 ± 2	No	No
Old OZM	16 ± 3	Yes	No
New OZM	18 ± 2	Yes	Yes
Deltima Precision	15 ± 2	Yes	Yes

).

3.1.2. Determining Roughness Parameters

Roughness measurements were carried out under the following parameters: cutoff wavelength $\lambda$ = 8 μm, a stylus feed rate of 0.5 mm/s, measuring distance of five single measured lengths (5 × 2.5 mm), a measuring reference Ra = 3 μm, filter type: Gauss. Sanded OZM plates were also analyzed.

Table 4. Roughness parameters for each plate type.

Plate Type	Parameter	Mean Value [μm]	Minimum Value [μm]	Maximum Value [μm]	Most Popular Range [μm]
Julius Peters	Ra	6.07 ± 0.79	4.76	7.80	6 to 7
	Rt	54.43 ± 8.36	42.57	76.61	50 to 55
	Rz1max	51.62 ± 8.41	40.40	72.89	50 to 55
Old OZM	Ra	10.89 ± 1.60	7.99	14.44	10 to 11
	Rt	84.99 ± 13.46	62.93	131.56	75 to 80
	Rz1max	80.98 ± 13.53	58.11	125.82	75 to 80
New OZM	Ra	9.87 ± 1.01	7.69	12.83	9 to 10
	Rt	83.01 ± 12.19	60.07	119.56	80 to 85
	Rz1max	78.40 ± 10.38	60.07	103.59	80 to 85
Deltima Precision	Ra	9.67 ± 1.07	6.67	12.47	9 to 10
	Rt	81.00 ± 10.26	64.35	114.03	80 to 85
	Rz1max	76.43 ± 9.55	58.75	108.79	70 to 75
Sanded OZM	Ra	5.07 ± 1.22	2.99	7.14	4 to 5
	Rt	51.69 ± 6.81	42.14	62.69	45 to 50
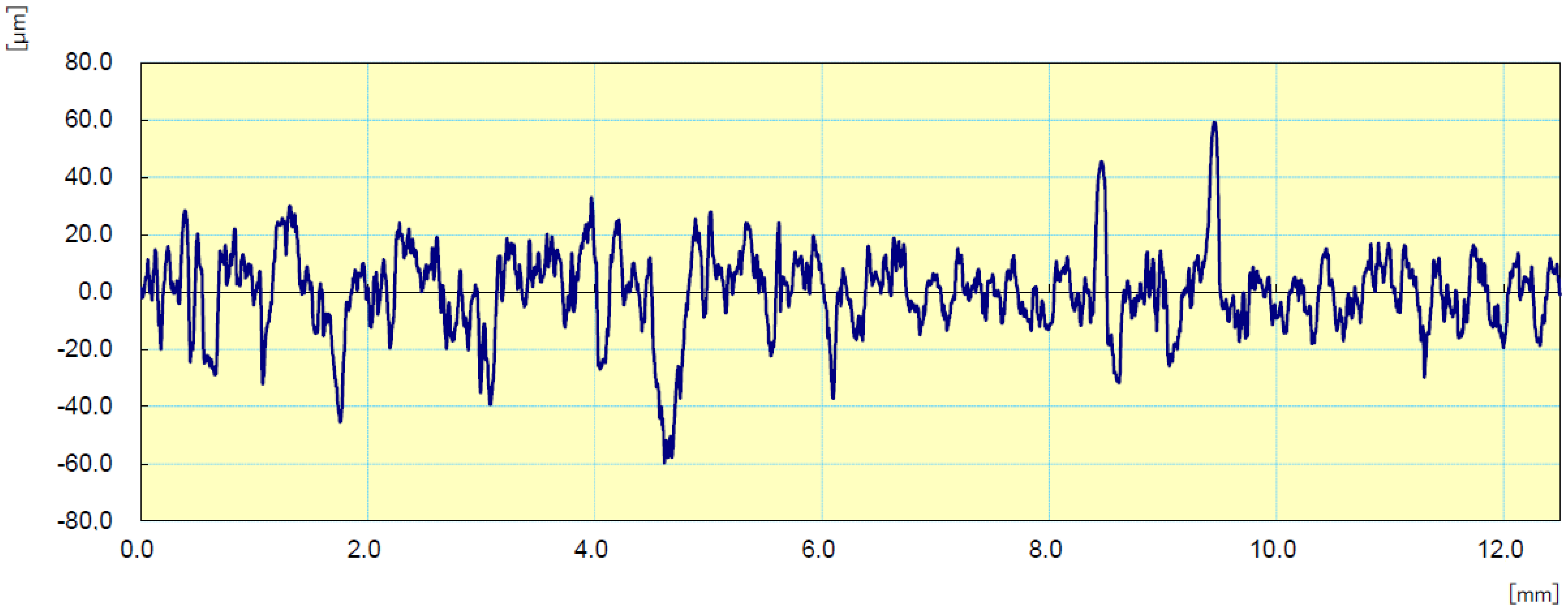
	Rz1max	51.15 ± 6.72	40.13	62.69	45 to 50

contains the aggregate results for all plate types. The plate surface profiles generated by the Mitutoyo software from the measurements (one example for each batch of plates tested) are shown in Figure 8, Figure 9, Figure 10 and Figure 11.

3.2. Friction Sensitivity

A summary of the friction test results for RDX and PETN is presented in

Table 5. Friction sensitivity of hexogen for all plate types.

Plate Type	Sensitivity (EN 13631-3) [N]	50% Point (STANAG 4487) [N]	Ra (Mean Value) [μm]	Ra Range [μm]	Grooves per 1 cm (Mean Value)
Julius Peters	120	182 ± 9	6.07 ± 0.79	4.76 to 7.80	16 ± 2
Old OZM	144	206 ± 89	10.89 ± 1.60	7.99 to 14.44	16 ± 3
New OZM	144	196 ± 49	9.87 ± 1.01	7.69 to 12.83	18 ± 2
Deltima Precision	128	215 ± 74	9.67 ± 1.07	6.67 to 12.47	15 ± 2
Sanded OZM	168	216 ± 55	5.07 ± 1.22	2.99 to 7.14	Not applicable

and

Table 6. Friction sensitivity of penthrite for all plate types.

Plate Type	Sensitivity (EN 13631-3) [N]	50% Point (STANAG 4487) [N]	Ra (Mean Value) [μm]	Ra Range [μm]	Grooves per 1 cm (Mean Value)
Julius Peters	64	67 ± 9	6.07 ± 0.79	4.76 to 7.80	16 ± 2
Old OZM	60	73 ± 21	10.89 ± 1.60	7.99 to 14.44	16 ± 3
New OZM	64	75 ± 19	9.87 ± 1.01	7.69 to 12.83	18 ± 2
Deltima Precision	56	69 ± 9	9.67 ± 1.07	6.67 to 12.47	15 ± 2
Sanded OZM	80	121 ± 29	5.07 ± 1.22	2.99 to 7.14	Not applicable

. To compare it with the plates surface differences, the parameter Ra and the number of grooves per 1 cm were chosen. Parameters Rt and Rz1max turned out to be relatable to Ra, e.g., plates with the lowest Ra value had also the lowest Rt and Rz1max values.

The obtained friction sensitivities are comparable to the hexogen and penthrite sensitivities available in the literature and STANAG 4487 (

Table 7. Friction sensitivity—RDX and PETN. All figures given in N.

Explosive	Meyer et al. [5]	50% Point-Musil et al. * [25]	50% Point-Künzel et al. * [26]	50% Point Range-STANAG 4487 [10]
Hexogen	120	127	127	110 to 185
Penthrite	60	75.5	75.1	56 to 102

* Obtained using the probit analysis.

).

4. Discussion

The visual assessment of the plates surfaces and the resulting number of grooves per measurement section are subject to a high degree of uncertainty due to the difficulty in identifying individual grooves (especially for Julius Peters plates). The obtained number of grooves for each plate type is similar due to the standard deviation value. Nonetheless, the results of this assessment highlight the unevenness of the tested surfaces. Therefore, from all tested plate types new OZM plates are mostly recommended.

During the roughness measurements, it was found that the Julius Peters plates do not meet the requirements of the standard EN 13671-3:2005. The individual deviation values from the plate profile are not within the range of 5 to 19 μm. The thickness of the plates is also inappropriate. Therefore, the Julius Peters plates may not be suitable for measurements according to this standard. Plates from other manufacturers meet the standard requirements.

No major differences in sensitivity measurements between the OZM plates from the new and the old batches were noticed, despite the discrepancies in the regularity of surfaces and ease of groove identification. Taking into consideration standard deviation, there is no huge divergence in measured Ra (10.89 ± 1.60 μm for the old batch and 9.87 ± 1.01 μm for the new one).

Analysis of the generated by the Mitutoyo software profiles indicates, that on average, the Julius Peters plate deviations have the lowest values (up to 20 μm with several values not exceeding 25 μm). The new batch of OZM plates is characterized by single deviations with large values (up to 40 ÷ 60 μm), while the average deviations reach the level from 20 to 30 μm; for the old OZM batch, the average deviation level is similar, while the single deviations reach 60 μm. For Deltima plates, the largest deviations do not exceed 50 μm with an average level of 10 to 20 μm. These observations are convergent to the measured roughness parameters.

The plate profiles are not completely uniform, with noticeably higher profile deviations or various deviation widths observed in certain areas of the plates. The presence of such irregularities can lead to the accumulation of excess samples, which may distort the results of the sensitivity test. For Deltima and old OZM plates, profile deviations are noticeably wider, while for Julius Peters plates deviations are mostly narrow and, along with their low intensity, make it mostly unlikely to gather crystals in the grooves. In the case of old OZM and Julius Peters plates, the analysis of the obtained profiles (along with a visual assessment of the plates) shows that the surface exhibits an uneven distribution of profile deviations for the same side of one plate, and depending on the selected position of the sample on the plate, a risk of different results of the sensitivity determination occurs.

The sanded OZM plates have the lowest roughness and each time they were used, the determined sensitivities of RDX and PETN were the lowest. However, the use of Julius Peters plates with a roughness at a similarly low level (5.07 ± 1.22 μm for sanded plates while 6.07 ±0.79 μm for Julius Peters) does not reflect the expected pattern. For penthrite, the friction sensitivity ranks at a similar level (European standard: 64 N for Peters and new OZM, 50% Point: 67 ± 9 N for Peters and 75 ± 19 N for new OZM), while for hexogen the sensitivity is even higher than when using plates from other manufacturers.

Deltima plates with slightly lower roughness than new OZM plates give higher friction sensitivities while determining according to the European standard, but this pattern does not coincide with the Bruceton test results for hexogen.

Neither RDX nor PETN was at the edge of admission for civil use. The tested RDX sample passed all the requirements for each type of plates, whereas crystalline PETN each time had the approvable sensibility for use as an explosive core in detonating cords.

5. Conclusions

On the basis of the obtained results, no obvious correlation between the plate roughness and the determined value of the friction sensitivity can be established. Therefore, currently, it is not justified to introduce a requirement for a roughness measurement preceding the Peters test into the standard. However, it is recommended to include information on the components used in the test reports.

Nevertheless, discrepancies between various types of plates are still being observed. This may be caused by another valid feature of used components that have not been examined in this study, e.g., the hardness of ceramics or variations in thickness. However, RRTE results have shown that the limitation of the types of plates may help lower the differences in the obtained sensitivity. Despite this, the total unanimity of the results is practically impossible to achieve, where the role of the human factor and subjectivity in the assessment of the test results seems to be currently unavoidable. Therefore, inter-laboratory comparison tests are crucial for determining the overall level of sensitivity of the tested explosives.

Applying sound and light detectors during sensitivity to friction tests may partially eliminate the above-mentioned human factor, resulting in more compliant results being obtained. Due to the development of 3D printing, another possibility for minimizing the discrepancies is to use printed plates—3D printers enable obtaining more regular grooves in technical ceramics used as filament. In each case, further tests are required.

Although the explosives tested in this study met the requirements of the standards for their approval in each case, it must be taken into account that, for some of the explosives, the results will place them on the borderline of admissibility, which may be problematic for explosives manufacturers (identical product may exclusively be approved by some certifying laboratories). Hence, further investigation of this issue is necessary to improve the comparability of the obtained results and indirectly increase the safety of the explosives used.