Historical Church Bell Tower Structural Behavior Induced by Bells Acoustic Wave Propagation

Abstract

The preservation of historical heritage requires a deep understanding of the interactions between physical and structural phenomena. This study investigates the influence of bells acoustic waves propagation on the dynamic behavior for the historical bell tower of the San Michele Arcangelo Cathedral in Caserta Vecchia, Italy. By combining environmental acoustic measurements with finite element modeling in ABAQUS, the research analyzes vibroacoustic coupling and identifies vibrational modes affected by the bell sounds. Harmonic analysis reveals the relationships between sound frequencies and structural vibrational modes, providing insights into the dynamics of historic towers subjected to repetitive acoustic loads. The findings demonstrate how sound can induce complex structural responses, offering valuable guidance for targeted conservation strategies. This interdisciplinary approach can be extended to other historic buildings, highlighting the critical role of vibroacoustic considerations in safeguarding cultural heritage.

1. Introduction

The preservation of historical structures is not only about protecting their architectural design, but also about understanding the principles that shaped construction techniques in relation to their foreseen function throughout history. These principles often include a blend of craftsmanship, materials science and engineering knowledge that evolved over centuries. One intriguing yet less-explored field of study is the interaction between audible sounds. The phenomenon of vibroacoustic coupling occurs when audible sources, between 20 Hz and 20 kHz, induce mechanical vibrations within a structure through its materials, becoming a reverse case with respect to a music instrument, where a vibrating source can produce an audible sound [1,2,3]. This interplay underscores the importance of an interdisciplinary methodology that integrates fields such as engineering, acoustics, and materials science to facilitate informed strategies for heritage conservation.

In the case of church bells, an acoustic source acts as the initiator of mechanical vibrations across a broader frequency spectrum. The vibrations produced during bell ringing can lead to complex oscillatory behaviors, manifesting as resonant frequencies that amplify selected vibrational modes of the structure [4], that are superimposed to natural and anthropogenic vibration sources [5]. This intrinsic coupling of acoustics and structural responses can produce cumulative damage over time, characterized by phenomena such as microcracking, material fatigue and eventual structural failure [6].

Research into the vibroacoustic interaction of structures has advanced significantly with the development of modeling techniques that integrate analyses of sound and structural responses. Sophisticated finite element modeling (FEM) methods have been employed to characterize the material properties of historical structures while simulating the vibrational influences of nearby sound sources [7]. In assessing how structural behavior adapts to acoustic loading, it is essential to consider not just the material composition, but also the broad spectrum of historical and environmental variables that influence its response [8].

Different materials used in the tower, including local stone and mortar, exhibit varied vibrational properties regarding acoustic wave attenuation. For instance, studies have shown that the acoustic properties of rocks are influenced by factors such as microcracks, porosity and fluid content, leading to variations in their elastic behavior and acoustic attenuation characteristics [9]. Additionally, research on fresh mortar indicates that components like sand size and content significantly affect wave attenuation behavior, with entrapped air bubbles impacting lower frequency ranges [10]. These findings underscore the importance of considering the specific vibrational properties of construction materials when assessing acoustic wave attenuation in structures.

This study focuses on the San Michele Arcangelo Cathedral historical bell tower located in Caserta Vecchia (Campania Region, Italy). The investigation examines how the propagation of its bells acoustic waves influences the tower structural behavior. This specific bell tower was chosen because of its historical relevance and since earlier studies analyzed its response to environmental vibrations at lower frequencies but did not account for the effects of acoustic forcing [11]. Additionally, prior research largely focused on seismic behavior and provided only modal analysis of the dynamic model [12,13,14]. By combining environmental acoustic measurements with finite element modeling, this study offers new perspectives on the dynamic behavior of historical bell towers subjected to vibroacoustic loads.

The San Michele Arcangelo bell tower is an example of medieval architecture. Its design, with a square base transitioning into an octagonal upper section, reflects the architectural features of its time. The tower’s structure consists of a variety of materials, including local stone and mortar, which contribute to its unique vibrational properties. These materials, while durable, also present challenges for conservation, as their mechanical properties can vary significantly over time due to weathering and other environmental factors [15,16].

In this study, an interdisciplinary approach which integrates acoustic measurements and advanced finite element simulation developed on ABAQUS software (v.2020) bridges the gap between theoretical analysis and practical conservation efforts. The finite element model on one hand incorporates detailed material properties and geometric configurations and on the other hand accurately reproduces the vibrating source, i.e., the bell, allowing for an accurate simulation of the tower’s response to acoustic forces. Furthermore, the study employs harmonic analysis to identify the relationships between the frequencies of the bell sounds and the vibrational modes of the tower.

The findings of this study are not just relevant for the tower assumed as case study. They can help us understand how sound impacts other historic structures. This knowledge is important for protecting cultural heritage. By learning more about how sound and vibrations interact, we can find better ways to preserve bell towers and similar buildings from damage caused by these forces. This research also contributes to broader fields such as architecture, engineering and the conservation of historic sites. The methodology used in this study could be extended to other types of structures, such as bridges, domes and even modern buildings that are subject to significant acoustic loads.

To achieve these objectives, the study draws on a rich body of literature that includes both historical and contemporary sources. For instance, recent advances in vibroacoustic analysis have provided new tools for examining the interaction between sound and structures [17,18,19]. These tools, combined with traditional engineering methods, enable a more comprehensive analysis of the complex dynamics involved.

2. Materials and Methods

2.1. The San Michele Arcangelo Cathedral Bell Tower

The San Michele Arcangelo Cathedral bell tower is a relevant architectural and cultural heritage asset of Caserta Vecchia, the historic fraction of Caserta municipality (Campania Region, Italy). Built during the 13th century, this monumental bell tower has withstood centuries of seismic activity, with each event leaving an indelible mark on its structural integrity. Over the years, numerous restoration and conservation efforts have been undertaken to preserve its original characteristics while ensuring its structural stability.

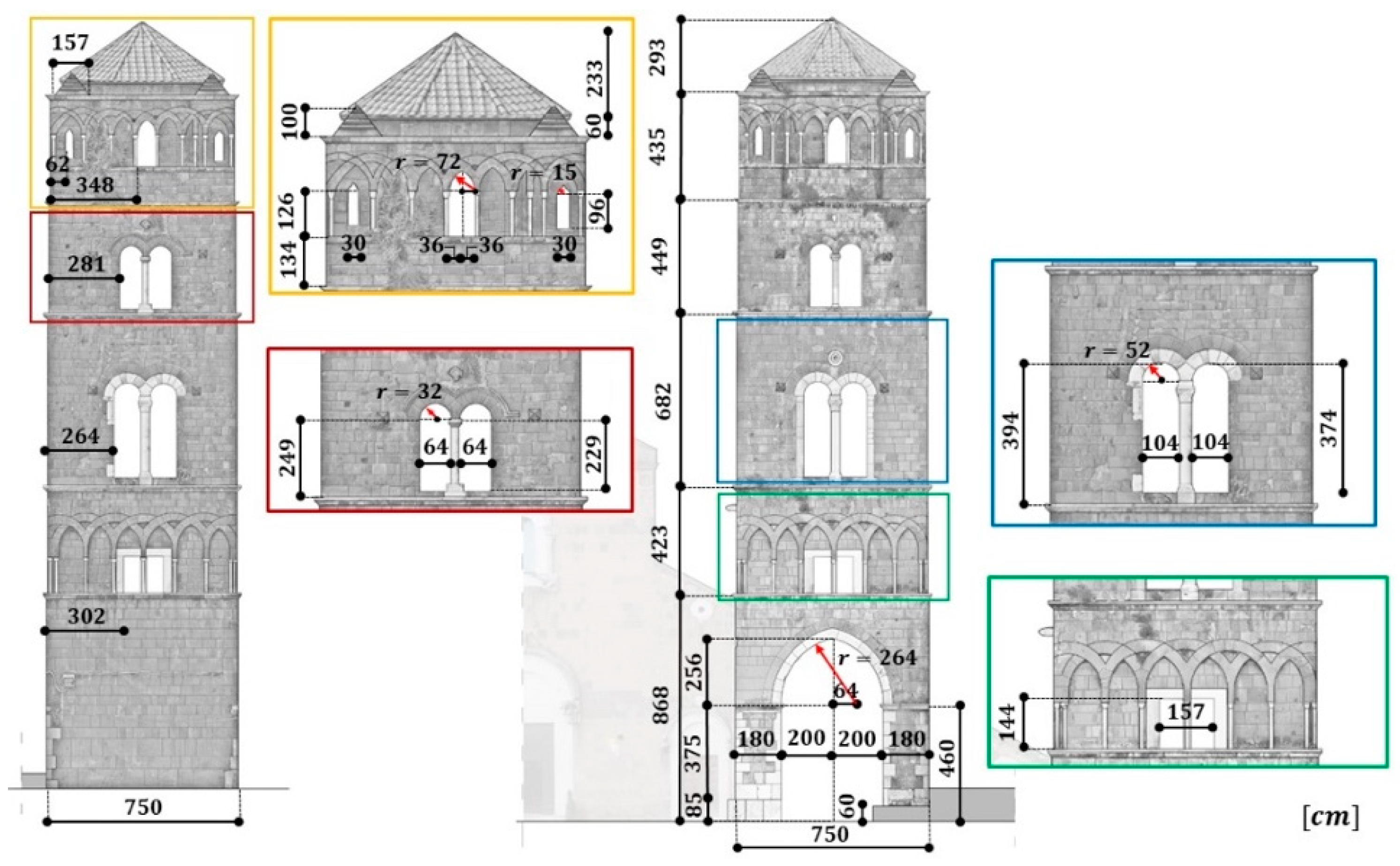
The tower features a square base measuring approximately 7.60 m per side, rising to a height of 31.5 m. Its foundational blocks, composed of large stones sourced from ancient Roman structures, remain visible due to the lowered elevation of the adjacent square. The structure is divided into five distinct levels, each showcasing unique architectural elements that highlight the craftsmanship of medieval builders. The main dimensions of the tower are reported in Figure 1.

The base level, notable for its sharply arched opening, accommodates a public road, seamlessly blending architectural functionality with urban practicality. This feature reflects similar designs observed in the bell tower of the Naples Cathedral and other medieval structures. The transition between levels is marked by protruding cornices, emphasizing the vertical progression of the structure.

The second level includes rectangular windows framed with limestone, while the third and fourth levels feature double-arched windows supported by slender marble columns. These elements are enhanced by decorative relief arches and pilasters, which add depth and texture to the façade. The fifth level transitions the tower into an octagonal shape, a hallmark of medieval architecture, and is crowned by four cylindrical turrets at its corners. These turrets are topped with conical pinnacles, completing the tower’s silhouette.

One of the most striking features of the tower is the interplay of Arab–Norman artistic influences. The sharply arched openings, intertwined arches, and alternating bands of light travertine and dark tuff stone create a visually compelling aesthetic. Historically, the bell tower underwent significant modifications. For example, the original spire, described in historical manuscripts as pyramid-like, was replaced with the current octagonal top and turrets. Restoration efforts in the late 20th century addressed structural issues, such as widespread mortar degradation and delamination of the outer masonry layers from the core. Techniques like anchoring the external layers to the inner core with reinforced rods were employed to stabilize the structure.

The bell tower houses seven bells, of which only three are rung full circle during festivities (Figure 2).

2.2. Acoustic Wave Source Recording

In this study, the mechanical pressure signal of the seven bells is considered as the primary forcing vibration source, used as input by the dynamic simulation software to reproduce the expected vibroacoustic response of the bell tower structure. In particular, the simulation environment requires the use of an uncompressed acoustic signal in *.wav format. The input audio file was recorded using a Class I USB omnidirectional microphone (UMIK-1, miniDSP™, Hong Kong, China), which provides a flat frequency response and uniform sensitivity across directions. This type of microphone ensures minimal distortion and is well suited for accurately capturing the spectral content of the bell sound, which is the key quantity of interest for the simulation.

The aim of the acoustic acquisition was not to reconstruct the full spatial distribution of the sound field, but rather to obtain a representative broadband excitation signal in the time–domain, suitable for use in vibroacoustic modeling. The procedure followed methodologies adopted in previous vibroacoustic studies [20,21], including those related to musical instruments and heritage sound sources, where the primary goal is to reproduce the energy input rather than spatial variations.

The recording was performed at 2 m from the bells, during a sunny day with stable weather conditions, to avoid the influence of wind or other environmental disturbances. No anthropic activity was detected during the recording period, ensuring a low background noise level. A windshield was also used to protect the microphone from local airflow variations.

The microphone was calibrated using the class-I calibrator ND9B, producing a tone with a peak of 1000 ± 0.01% Hz and two alternative sound pressure level settings, at 94 dB and 114 dB. Data were recorded and digitized as uncompressed *.wav files, with a sampling rate of 44,100 Hz. The recorded bell sound lasted for three minutes; a sub-set of the time–domain sound source signal, lasting 12 s and used as input for the structural vibration analysis.

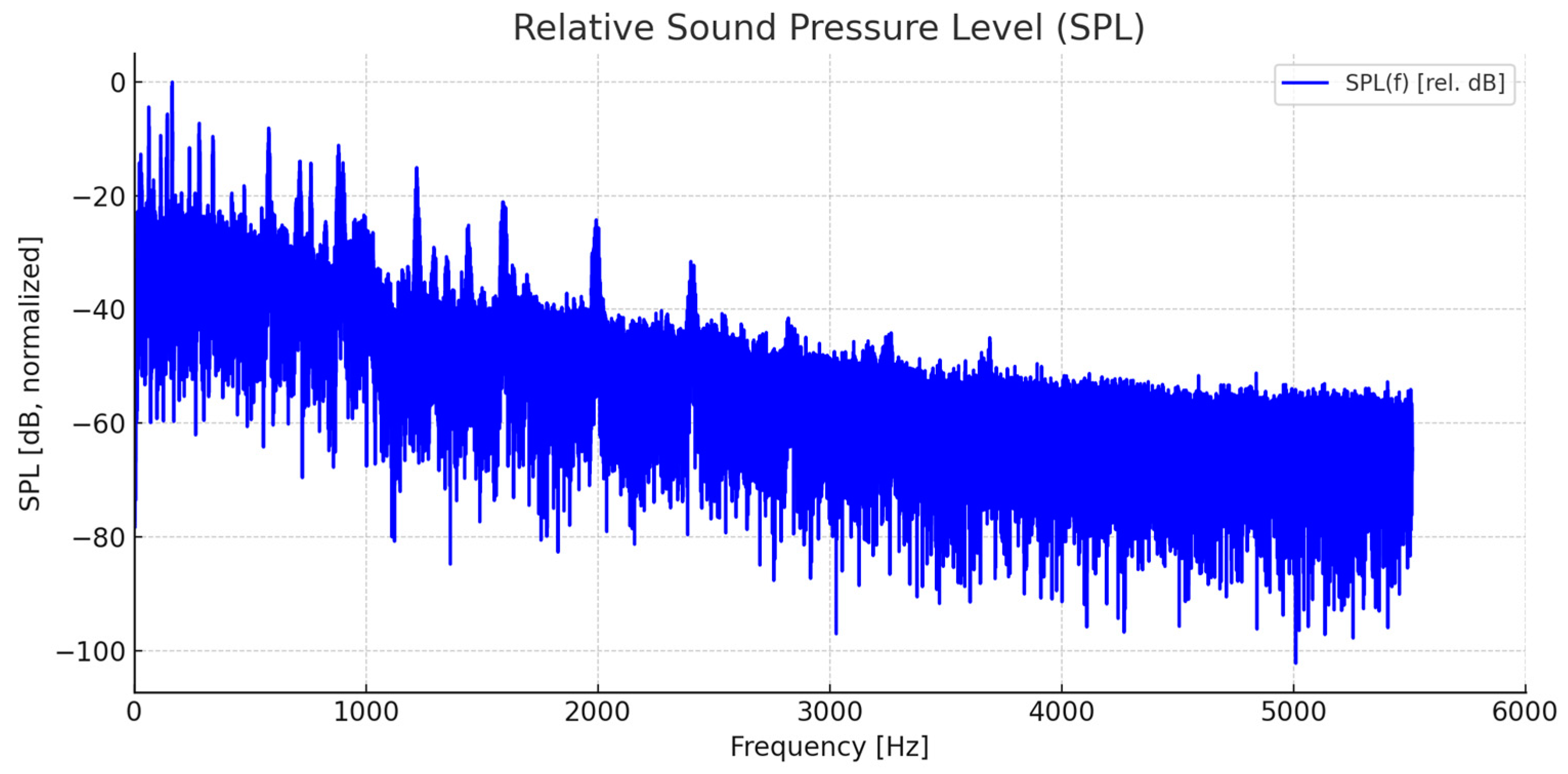
The recorded audio file was analyzed in the frequency–domain in MATLAB 2022 environment to derive the bell signal frequency peaks. In particular, the following peaks, measured in units of Hz, were detected: 127.3 Hz; 229.3 Hz; 280.5 Hz; 289.3 Hz; 330.7 Hz; 408.4 Hz; 560.3 Hz; 792.0 Hz; 856.3 Hz; 953.7 Hz; 1184.0 Hz; 1485.0 Hz; 1550.0 Hz; 1928.0 Hz; 1986.0 Hz; 2587.0 Hz; 3221.0 Hz; 3874.0 Hz; 4552.0 Hz; 5888.0 Hz; 6558.0 Hz. No relevant peak was detected above 10,000 Hz.

Following the spectral analysis of the recorded bell signal, a relative sound pressure level (SPL) spectrum was computed and is shown in Figure 3. The SPL(f) was obtained by applying a fast Fourier transform (FFT) to the 12 s acoustic waveform and calculating the amplitude spectrum, which was then expressed in decibels (dB) and normalized to the peak value. This graphical representation highlights the frequency components with the highest acoustic energy and provides a more intuitive understanding of the bell excitation signal, complementing the list of frequency peaks reported above.

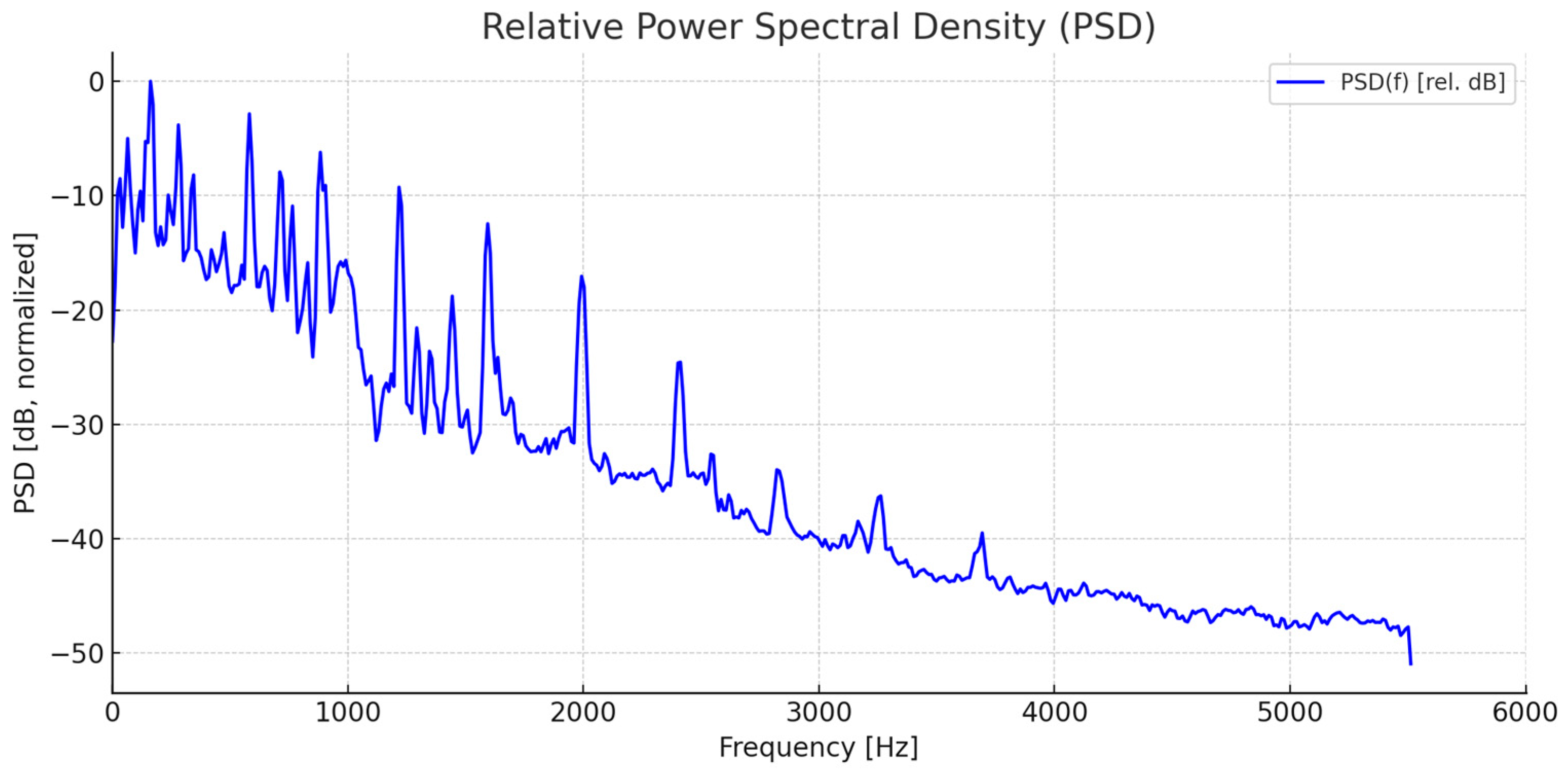
Additionally, a power spectral density (PSD) analysis was carried out to evaluate the energy distribution of the signal across frequencies. The resulting PSD spectrum, shown in Figure 4, was computed using the Welch method and confirms the presence of dominant peaks already observed in the SPL representation, while offering a smoothed view of the signal’s spectral energy content.

2.3. Finite Element Modeling

To accurately simulate the structural response of the bell tower to seismic forces and the additional dynamic loads from the bells, a detailed FE model was developed. The bell tower was first modeled geometrically, using data from a photogrammetric survey. Then, the geometrical model was partitioned into analyzable components, which allowed for targeted investigation of seismic responses and strategic application of materials and boundary conditions. Meshing was performed using structured and sweep hexahedral meshes to minimize distortion and shear locking, crucial for maintaining computational efficiency in dynamic analyses. The mesh sizes were carefully chosen to optimize both accuracy and efficiency, with finer meshes applied to areas likely to experience higher stress. The model did not consider soil effects, assuming a fixed base for the structure due to the lack of substantial soil influence under the modeled seismic conditions. Interactions with the adjacent cathedral were not considered while additional masses were included to simulate the dynamic effects of the bell ringing. The primary materials used in the bell tower include local grey tuff for most of the structure, limestone for the basement and arch framings, and recently added reinforced concrete for the roof [13,14].

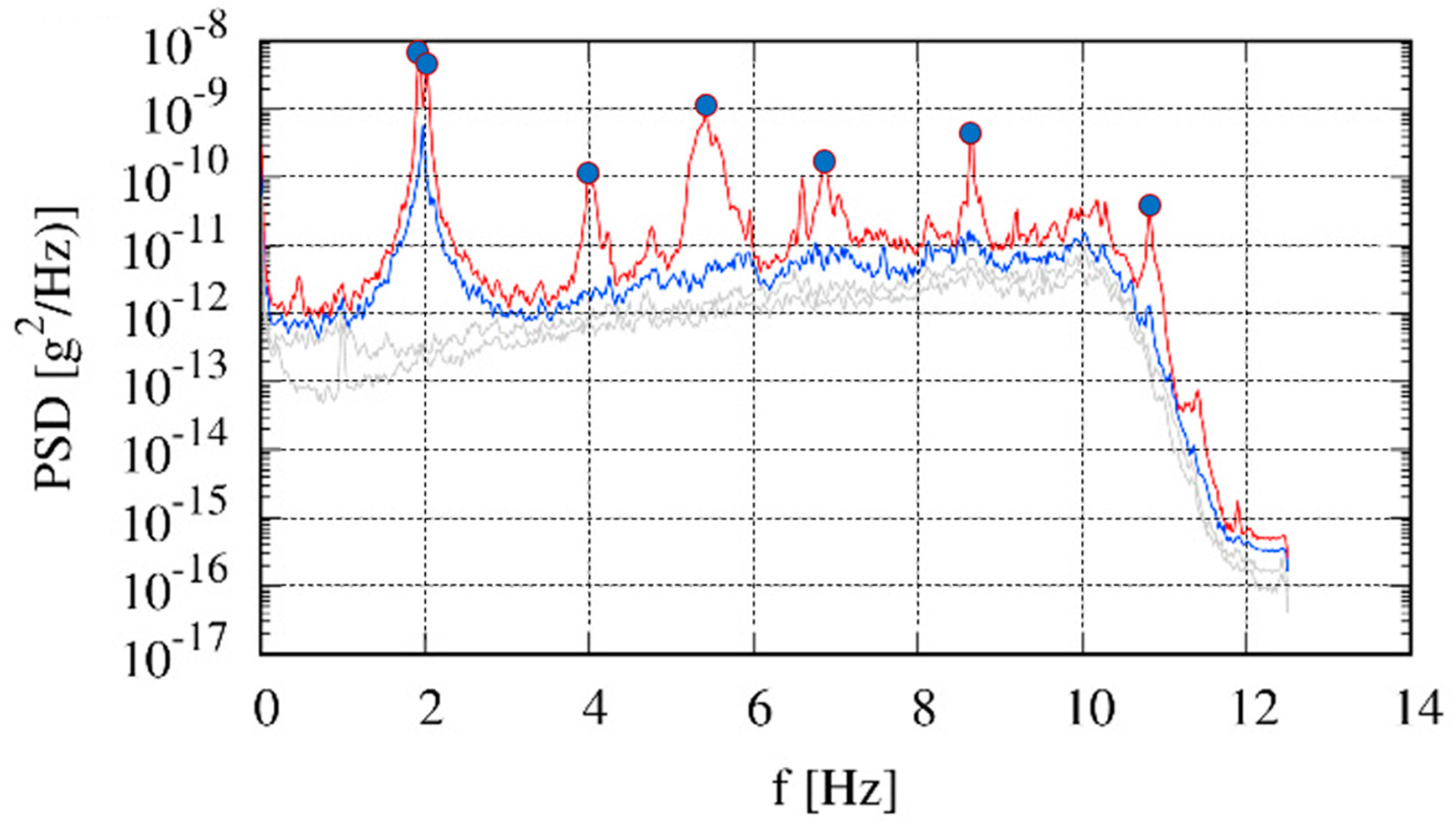
The Young static moduli of the adopted materials are 1305 MPa, 33,455 MPa and 24,147.73 MPa for tuff, limestone and concrete, respectively. The mesh typology used for the masonry and concrete part is an 8-node linear brick with reduced integration and hourglass control. This model, from a structural point of view, was calibrated by comparing the data from ambient vibration tests (AVTs), which provided essential information on the natural frequencies and principal vibrational modes of the tower [11] and the modal analysis. The Fourier spectrum resulting from the AVT is reported in Figure 5.

The bell tower was considered as fully embedded in an acoustic air domain, about three times the base and height dimension (Figure 6). The material properties of the air are parametrized through its density, equal to 1.204 × 10⁻⁶ ton/mm³, while the bulk modulus is equal to 141.8 MPa. The mesh type is an 8-node linear acoustic brick, reduced integration hourglass control (AC3D8R) with a dimension of 5 mm to ensure at least six elements per wavelength, which is considered sufficient for capturing the dominant frequencies of interest up to 10 kHz [22]. The air domain surrounding the bell tower was implemented with non-reflective (absorbing) boundary conditions. Then, the structural model of the bell tower is coupled with the acoustic model. An incident wave interaction is set up by placing the source point at the position of the bell’s main axis, approximately 21 meters above the ground; the standoff point is located on the inner surface of the bell tower, which also serves as the interaction surface. Given that the simulation focused on identifying frequency response rather than stress concentration or failure, a full sensitivity analysis was not deemed necessary for the objectives of this study.

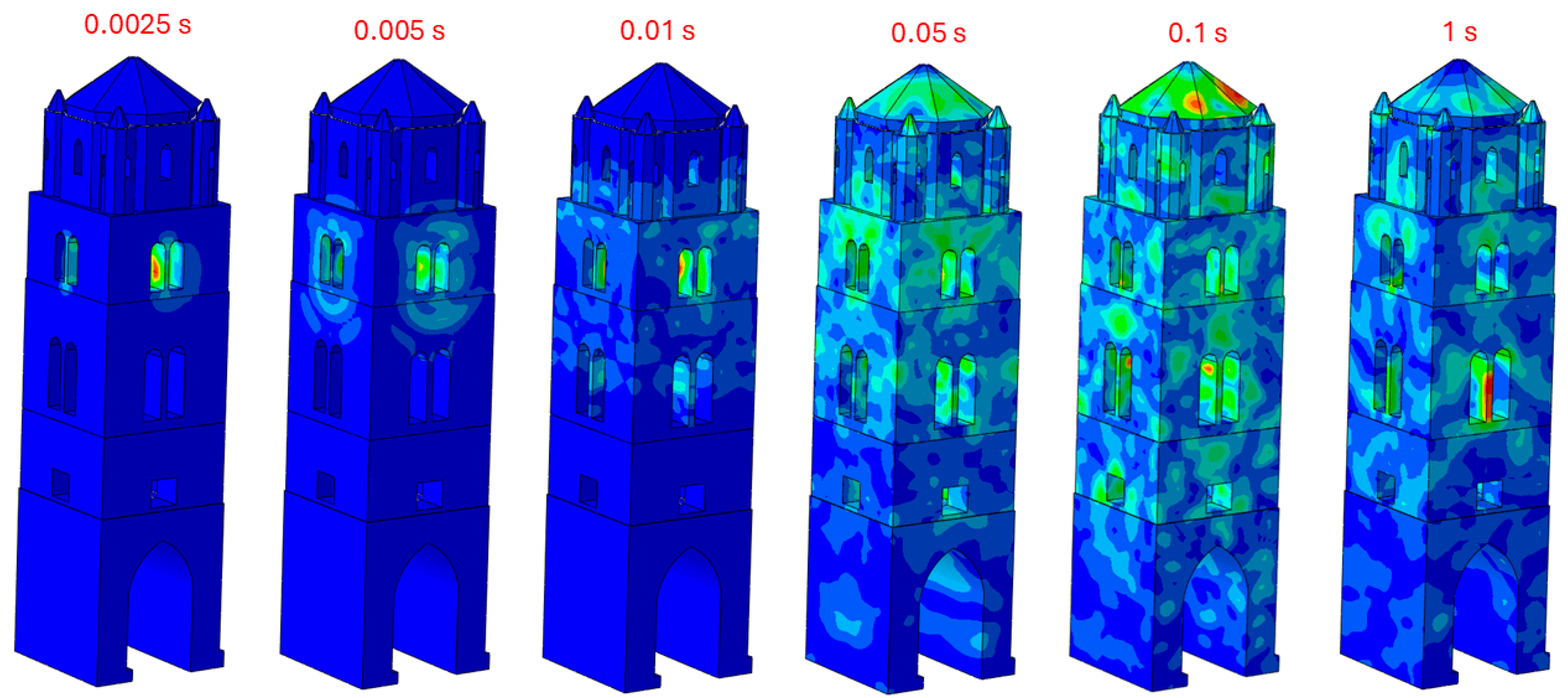
The analysis is dynamic explicit without mass scaling and with a scale time of 12 s. The pattern of Mises normal stress S is shown in Figure 7 for increasing time steps. Red areas represent regions with higher stress, while blue areas indicate under-excited regions. The frequency of output is 400 Hz. The time–domain output displacement signal was converted into the frequency–domain to assess the main frequency peaks derived from the simulation. Then, the data were partially clustered and compared with the input acoustic signal, as commonly done in musical vibroacoustics, through the identification of harmonic proportions to the observed peak frequencies, assessing also the relative percentage deviation between simulation frequency peaks and expected values derived from the computation of harmonic proportions.

2.4. Frequency Matching Technique

To assess whether the expected structural behavior depends on the excitation determined by the acoustic wave, the frequency–domain signal computed from the simulation is compared with the frequency–domain signal of the church bell acoustic wave, as experimentally determined through the measure.

With this respect, it is worth noticing that any structured sound produced by a musical instrument, including a bell, is composed of several frequency partials, identified as frequency peaks during an acoustic measure. These partials can be related to a fundamental lower frequency through specific frequency ratios, as originally discovered by Pythagoras and later mathematically modeled, in the 16th century, by the music theorist Gioseffo Zarlino [23].

The frequency peaks derived from simulated structural data are compared with the frequency partials computed based on measured frequency peaks. A frequency match was accepted for relative deviations between the two values lower than 5%. Consequently, in case of a sufficient match, it is possible to say that the identified expected structural vibrations, as determined through the simulations, are forced by the acoustic wave generated by the church bells.

3. Results and Discussion

The results of the FE model highlight the significant impact of vibroacoustic interactions on the structural response of the bell tower. Considering that the simulation output frequency is 400 Hz, a Nyquist frequency of 200 Hz is fixed. Consequently, no frequency peak above 200 Hz was included for the analysis.

Table 1. Simulation output frequency peaks and expected acoustic frequency partials (based on the calculation of harmonic proportions), their relation and their percentage relative deviation.

Structural Frequency Peak	Acoustic Frequency Partials	Relation Between Simulation Output and Computed Value	Relative Deviation
[Hz]	[Hz]		%
1.67	NA	Independent structural vibration	NA
1.91	1.93	First experimental vibration mode (1.93 Hz)	0.67%
4.08	2.04	One octave lower than the second experimental vibration mode (2.03 Hz)	−0.59%
4.67	4.67	Harmonic ratio with 32.670 Hz (sesquiseptimum)	0.00%
5.58	NA	Independent structural vibration	NA
5.83	5.85	Harmonic ratio with 29.250 Hz (sesquiquartum)	−0.29%
6.25	6.22	Harmonic ratio with 49.750 Hz (three octaves lower)	0.50%
23.00	23.04	Harmonic ratio with 46.080 Hz (one octave lower)	−0.17%
29.25	NA	Structural vibration	NA
32.67	32.76	Harmonic ratio with bell signal forcing at 229.300 Hz (sesquiseptimum)	−0.27%
43.33	NA	Independent structural vibration	NA
46.08	45.86	Harmonic ratio with bell signal forcing at 229.300 Hz (sesquiquartum)	0.48%
49.75	NA	Independent structural vibration	NA
63.83	63.85	Harmonic ratio with bell signal forcing at 127.7 Hz (lower octave)	−0.03%
80.75	80.04	Harmonic ratio with bell signal forcing at 560.300 Hz (sesquiseptimum)	0.88%
114.70	114.65	Harmonic ratio with bell signal forcing at 229.300 Hz (one octave lower)	0.04%
118.50	119.21	Harmonic ratio with bell signal forcing at 953.700 Hz (three octaves lower)	−0.60%
144.80	144.65	Harmonic ratio with bell signal forcing at 289.300 Hz (one octave lower)	0.10%

lists of the structural frequency peaks, derived from the numerical model, partially matching with the acoustic frequency partials, computed as harmonic ratios built of the measured acoustic frequency peaks. Then, the last column indicates the percentage relative deviation between values derived from the model and expected acoustic frequency partials values. It is worth noting that, when the presence of a harmonic proportion was identified, the relative deviations between the computed value and the value obtained through the frequency analysis of the simulation output were always lower than 1%.

Part of the peak values are associated to the acoustic forcing of the bell sound, while other peak values could be clustered in relation to the vibration of the structure. Moreover, a relation with the previously-measured first two modal frequencies of the bell tower was always observed [5] with a relative deviation between the measured and the computed value being lower than 1%. Finally, other structural vibrations could not be clustered, as indicated in Table 1. Based on the preliminary results of this study, it is possible to confirm that a sound source with specific a frequency pattern emission (like in the case of any musical instrument, such as a bell), also known as vibroacoustic fingerprint [1], can trigger a structural response, covering a wider range of frequencies, including those lower than the original acoustic forcing source.

The study of acoustic transmission through solid materials, especially in the context of structural systems, remains underexplored, despite its critical relevance to engineering and heritage conservation. This phenomenon, often referred to as solid-borne sound transmission, plays a significant role in understanding the vibroacoustic behavior of structures subjected to dynamic loads, including heritage buildings like bell towers. Surprisingly, only a limited number of indexed works in Scopus explicitly address this topic, highlighting a significant gap in the literature.

The foundational contribution to this area of research through the application of finite element modeling and analysis established a robust framework for analyzing fluid-structure interaction problems [24]. This work inspired subsequent research and is considered a cornerstone in the study of vibroacoustic interactions. The methodology developed in this study implemented this theoretical framework to capture the coupled behavior of fluids and solids, providing insights into eigenfrequencies and modal interactions. The validity of this approach is confirmed by a previous implementation of the mathematical background, supporting the representation of the convergence of eigenfrequencies in systems, involving fluid–solid coupling, while establishing a benchmark for computational approaches [25].

While the mathematical foundations for acoustic–structural interactions were extensively developed, real-world applications, particularly involving masonry structures until now remained scarce. In fact, studies addressing the propagation of acoustic waves through solid masonry and its implications for structural integrity are virtually absent in the literature. This gap is particularly concerning, given the increasing demand for acoustic isolation in modern and historical buildings.

The relevance of this work in proving the possibility of addressing the solid-borne sound transmission is twofold. Firstly, the validity of the results supports the possibility of adopting the same approach for an innovative approach to the understanding of acoustic comfort and noise isolation in contemporary architectural designs. Secondly, the application to the cultural heritage context proves the applicability of this method for heritage conservation, where repetitive acoustic loads (like bell ringing, as discussed above), can induce cumulative damage over time. The lack of practical investigations into these phenomena underscores the need for interdisciplinary approaches that combine advanced numerical methods with experimental studies tailored to masonry structures.

Considering the preliminary nature of the work, the experimental characterization of such an interaction phenomenon could be expanded, integrating the broadband quantification of mechanical vibrations with appropriate sensors, as already done in the literature [5,19], as well as expanding the study to other structural engineering contexts of interest.

4. Conclusions

This study investigated the influence of bell-generated acoustic waves on the structural response of a medieval bell tower through a vibroacoustic coupling analysis. The results of the finite element model suggest that certain vibrational modes of the structure can be activated or amplified by specific sound frequencies emitted during bell ringing. These observations are supported by the frequency matching between simulated structural responses and harmonic partials derived from the measured acoustic signal.

While these findings provide new insight into the interaction between sound and structure in historical masonry buildings, the authors acknowledge that the present study represents a preliminary step. The analysis was performed on a single case study, and further investigations, particularly experimental measurements of structural response under acoustic excitation, are needed to validate and generalize the approach.

Nonetheless, the proposed methodology demonstrates the potential of vibroacoustic modeling as a tool for understanding complex dynamic phenomena in cultural heritage structures. This approach could offer valuable guidance for conservation strategies, especially in cases where repetitive internal acoustic sources, such as bells, may induce long-term effects on structural integrity. Future studies may explore the application of this framework to a broader range of building typologies, including bridges, domes and modern architectural elements, where acoustic loads are relevant.