Research and Parameter Analysis of Lateral Resistance Performance of Assembled Corrugated Steel Plate Shear Wall
Abstract
1. Introduction
2. Experimental Preparation
2.1. Specimen Design
- (1)
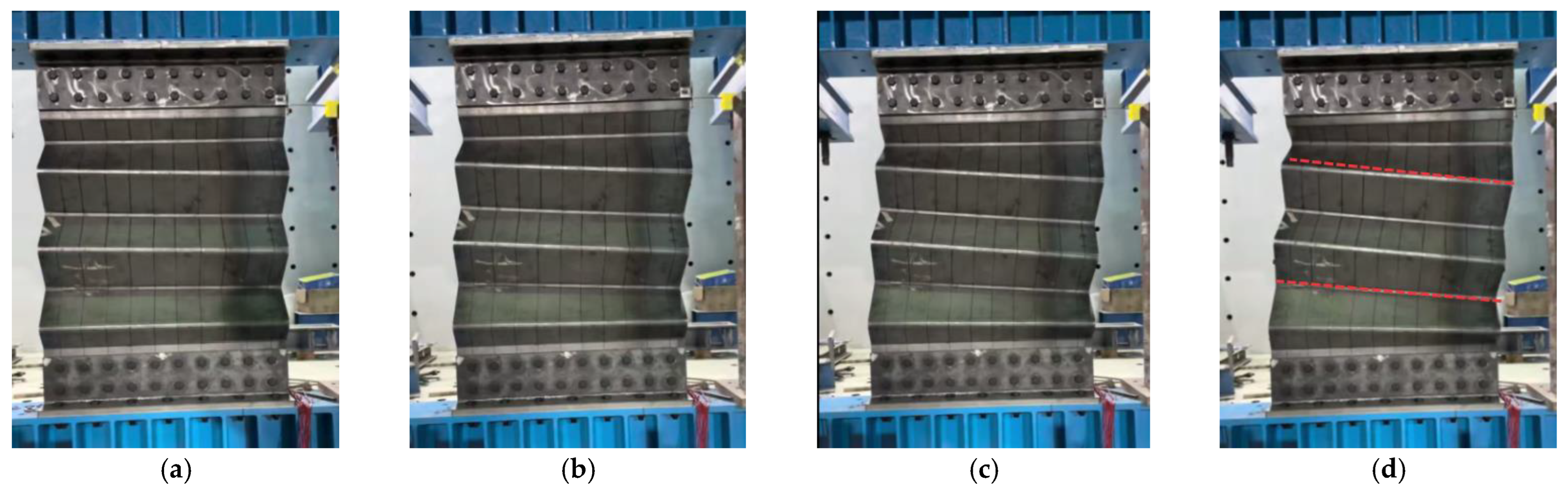
- TSPSW-M-1: Flat steel plate (Figure 1a).
- (2)
- TCSPSW-M-1: Trapezoidal corrugated plate (wave height = 75 mm, wavelength = 350 mm, Figure 1b).
- (3)
- TCSPSW-M-2: Trapezoidal corrugated plate (wave height = 75 mm, wavelength = 250 mm, Figure 1c).
- (4)
- TCSPSW-M-3: Trapezoidal corrugated plate (wave height = 150 mm, wavelength = 350 mm, Figure 1d).
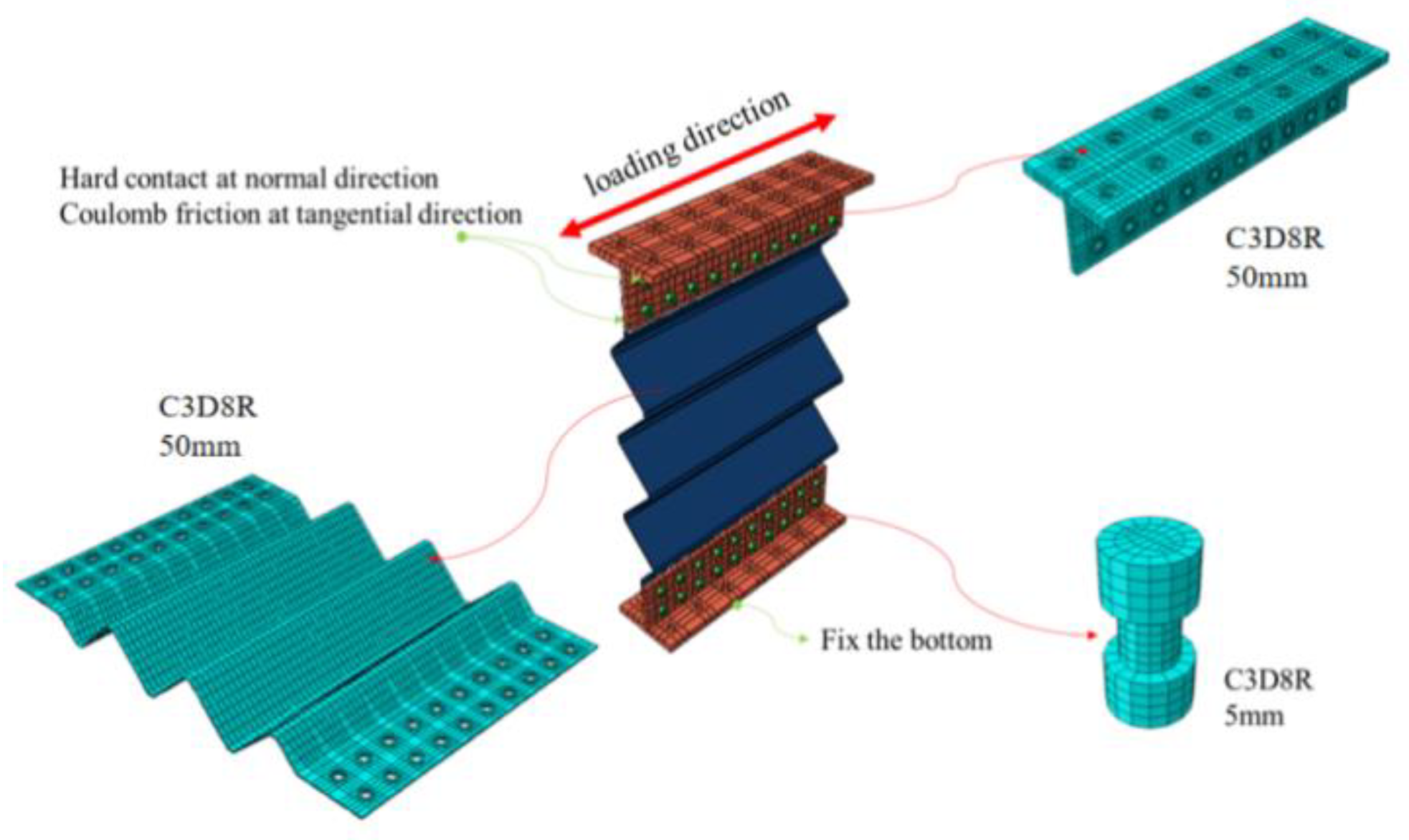
2.2. Test Setup
2.3. Measurement Scheme
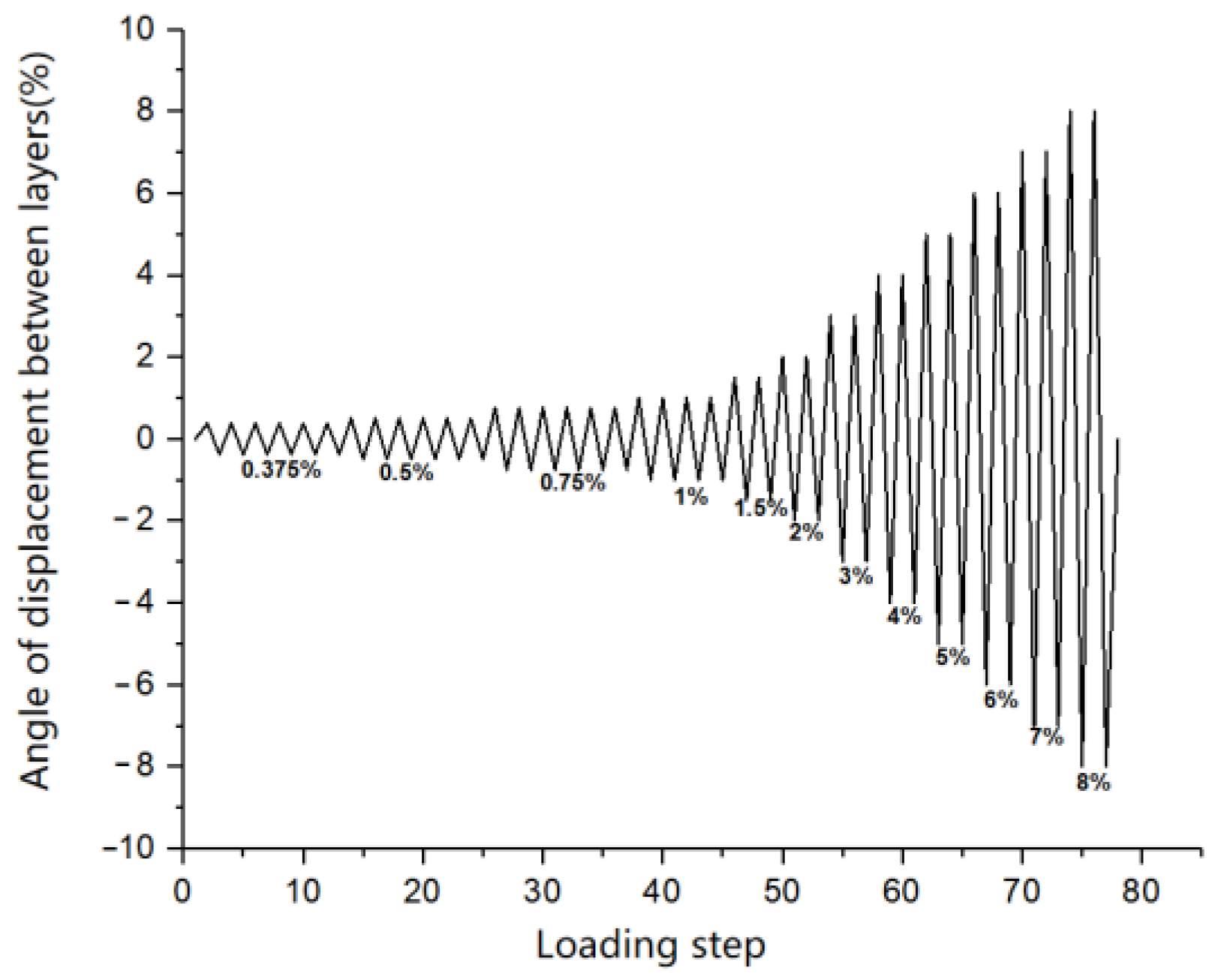
2.4. Loading Protocol
3. Experimental Phenomena and Result Analysis
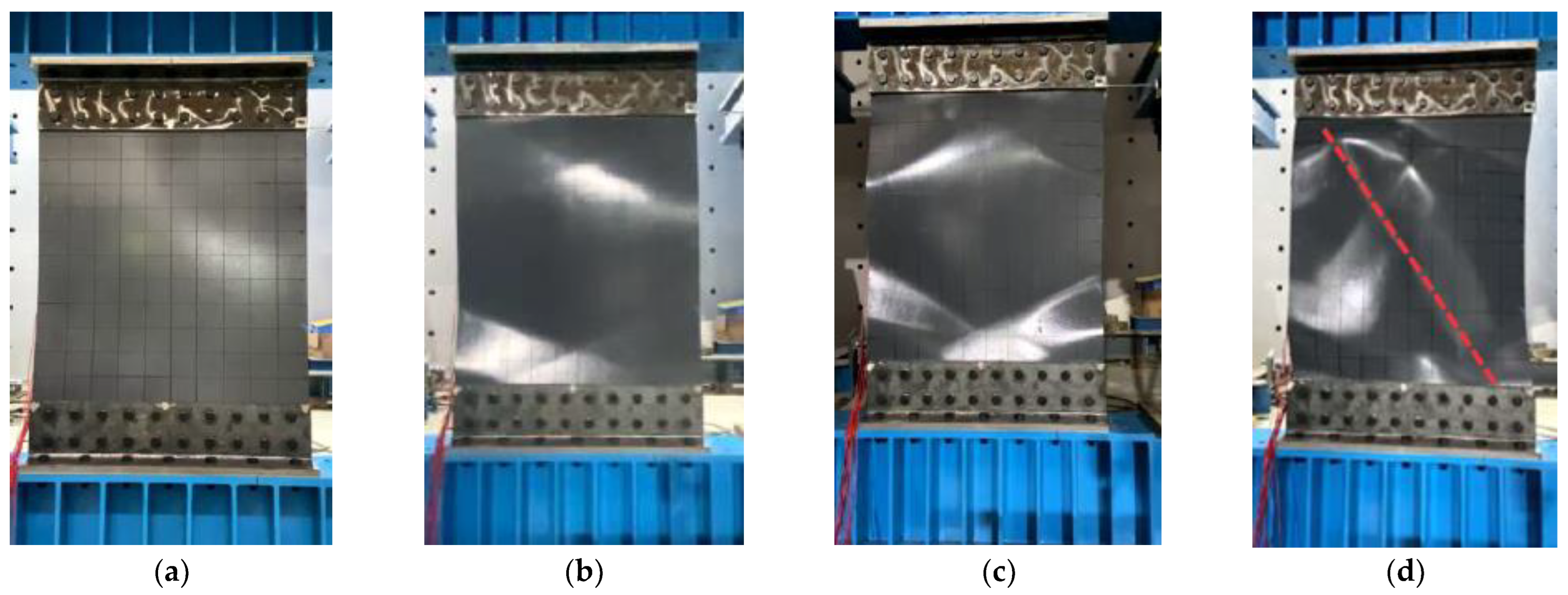
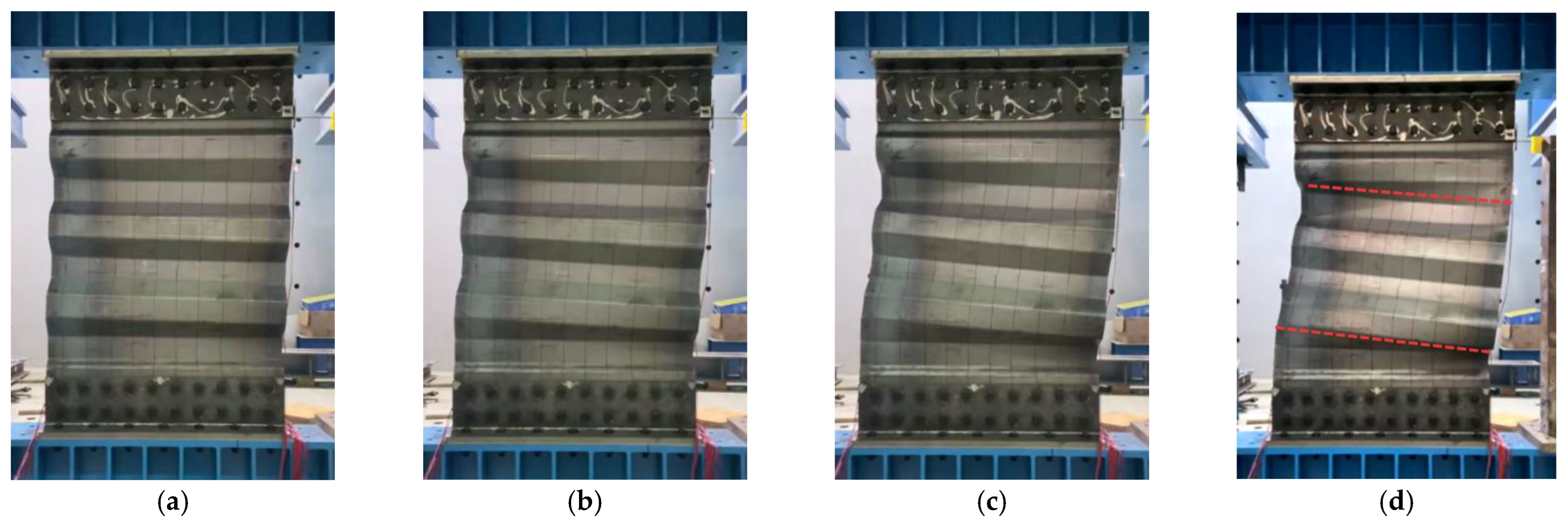
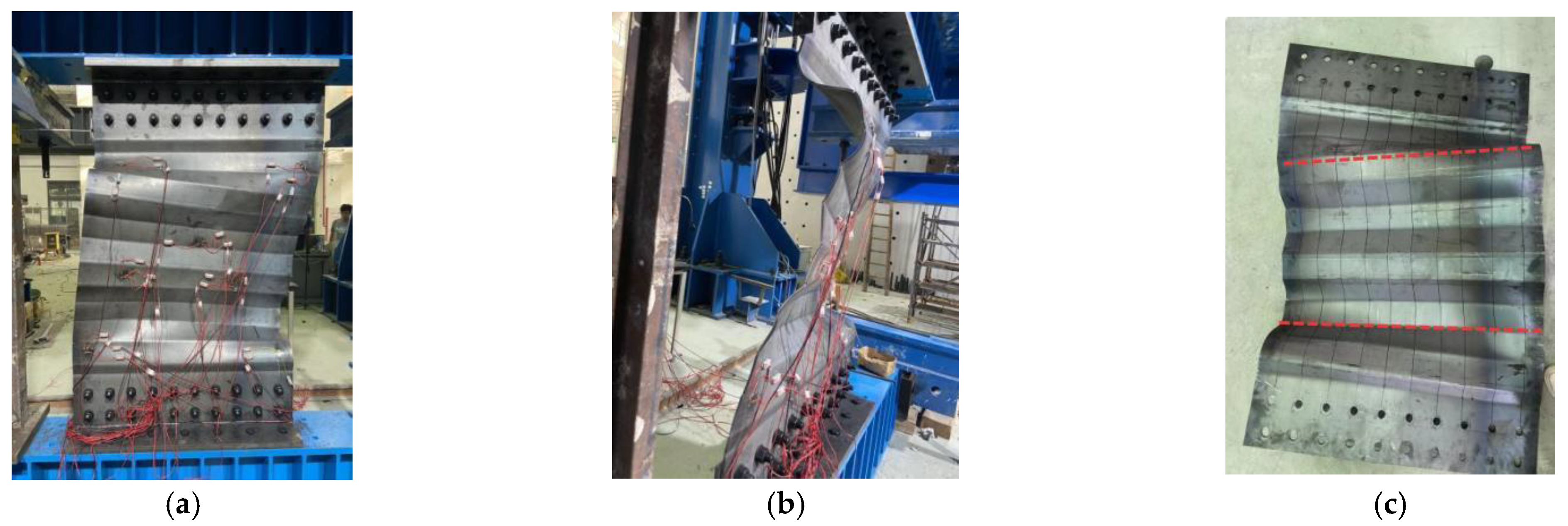
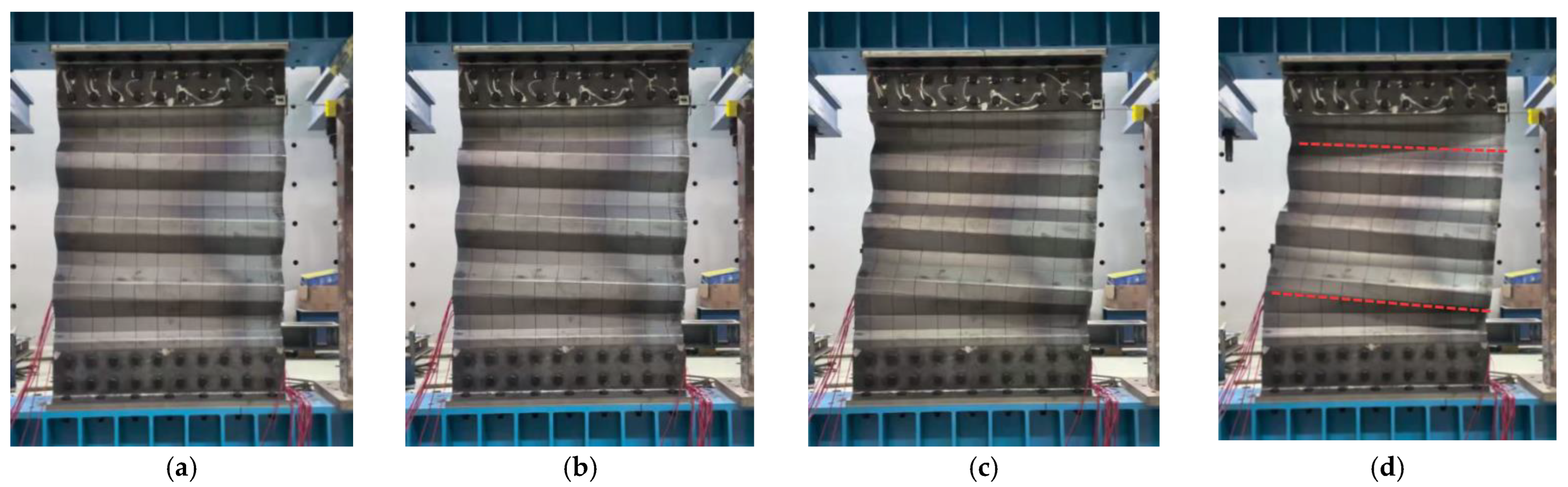
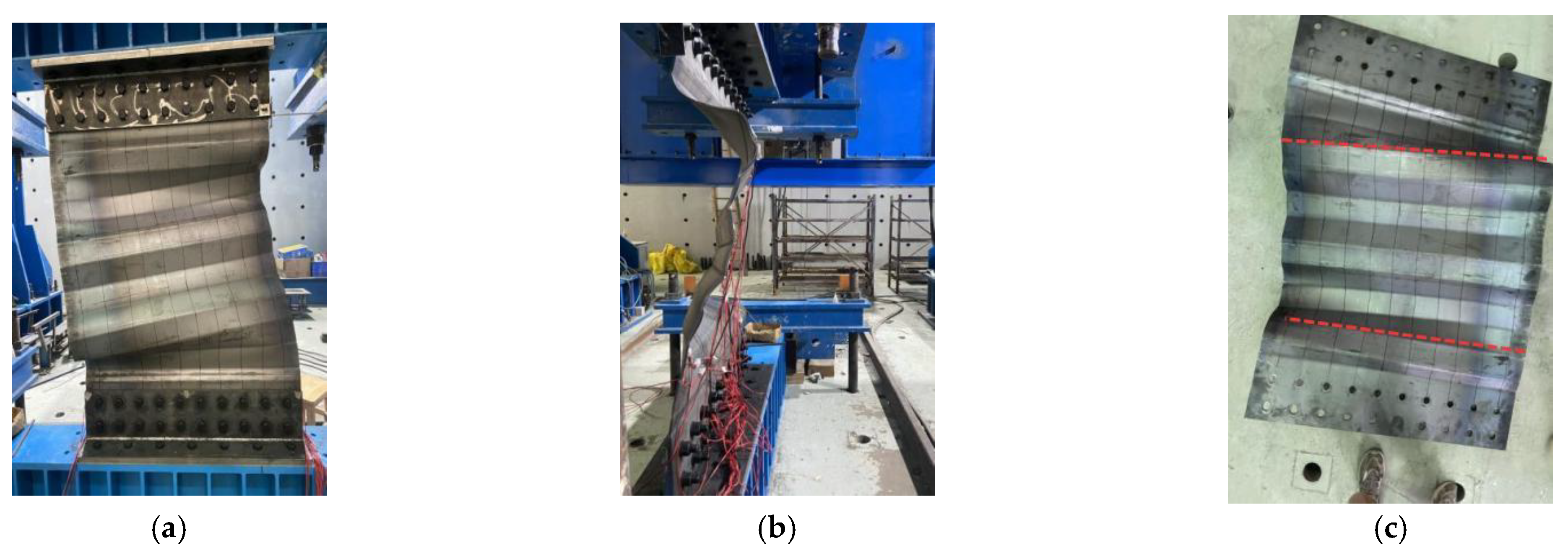
3.1. Experimental Observations
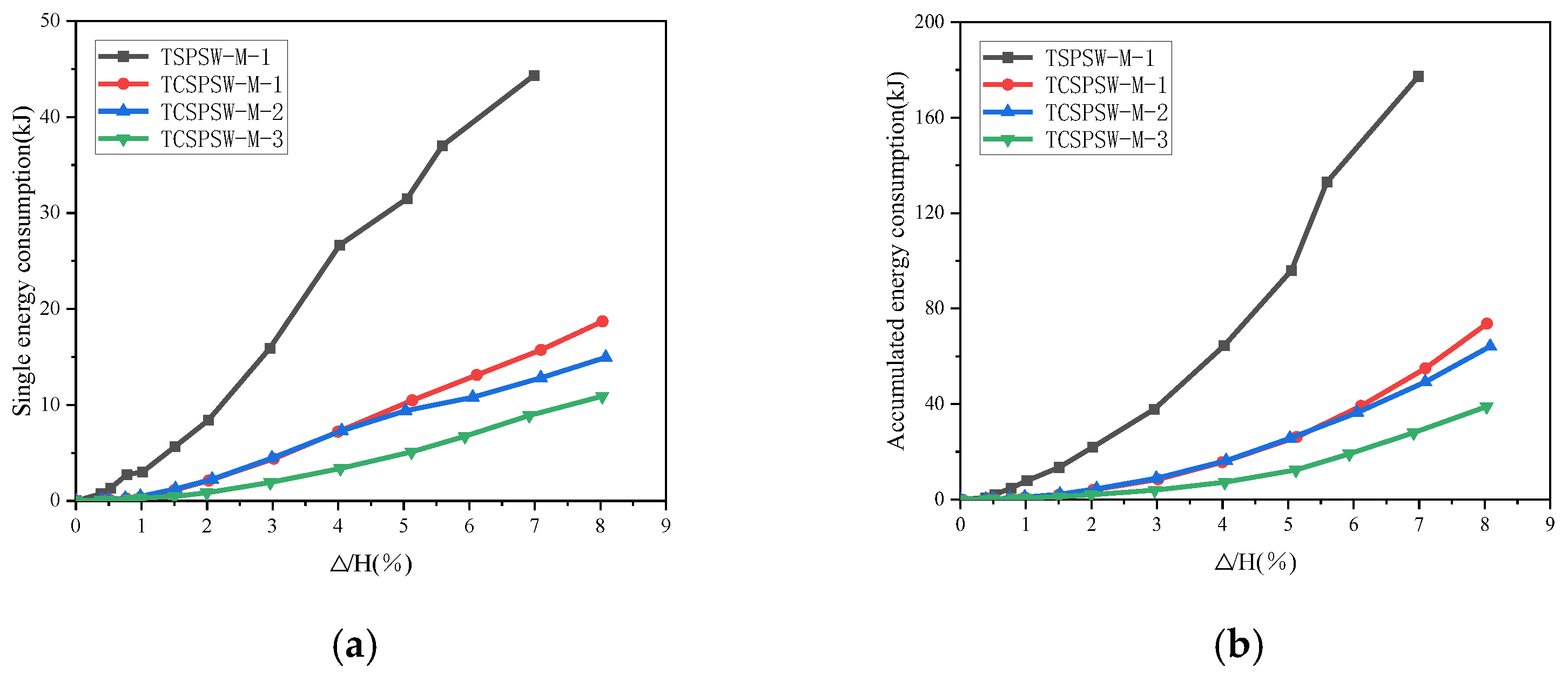
3.2. Experimental Result Analysis
4. Parametric Analysis of CSPSWs
4.1. Influence of Different Plate Thicknesses on Energy Dissipation Capacity of CSPSWs
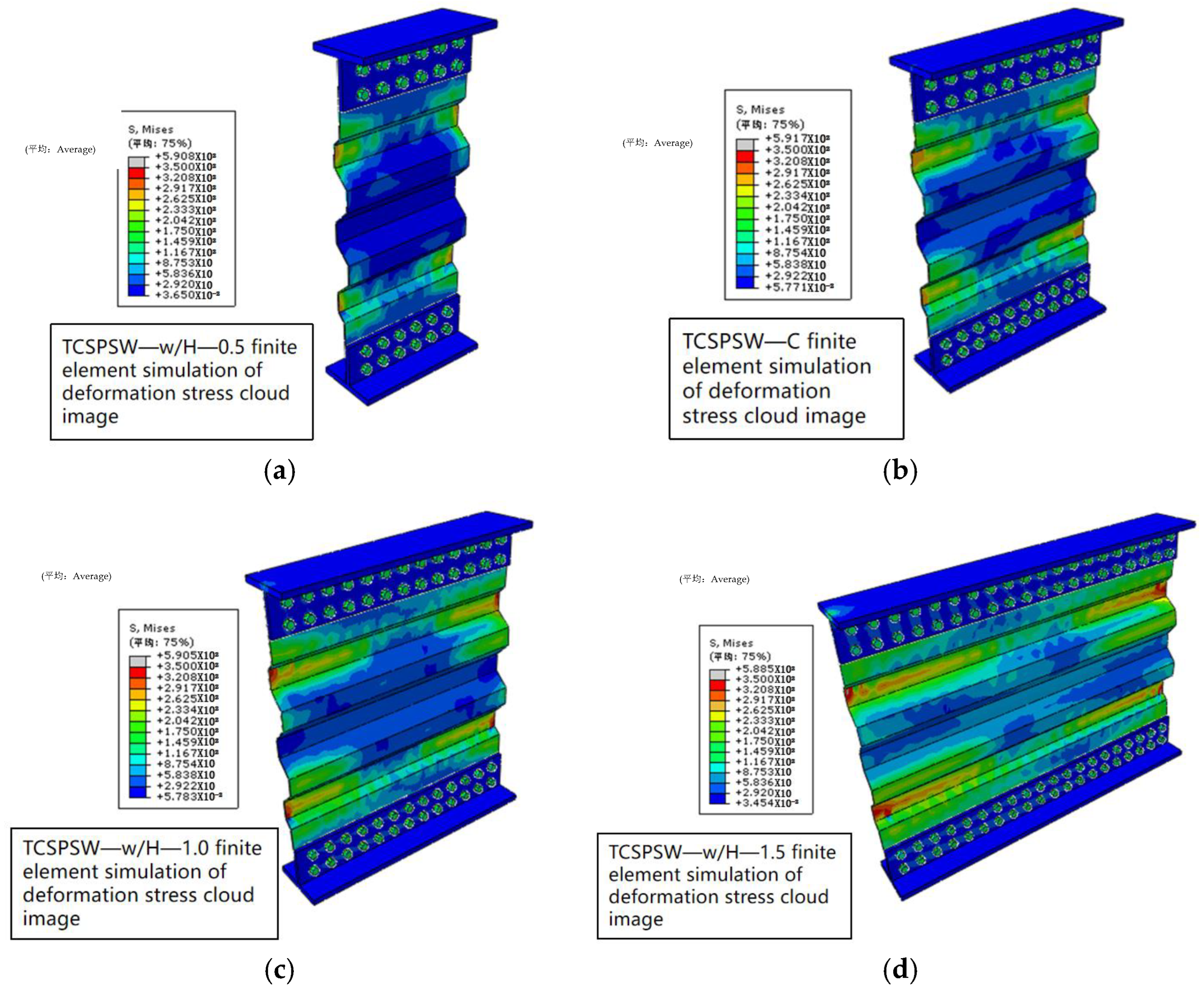
4.2. Influence of Different Steel Sheet Width/Height Ratio on Energy Dissipation Capacity of CSPSWs
4.3. Influence of Different Wave Height on Energy Dissipation Capacity of CSPSWs
5. Conclusions
- (1)
- The energy dissipation performance of corrugated steel plate shear walls is superior to that of flat steel plate shear walls. Under the same conditions, flat steel plate shear walls exhibit higher lateral stiffness and energy dissipation compared to corrugated steel plate shear walls. However, due to their unique geometric shape, namely the “accordion-like structure”, corrugated steel plate shear walls can demonstrate superior energy dissipation efficiency. Prefabricated corrugated steel plate shear walls, compared to prefabricated flat steel plate shear walls, have better resistance to out-of-plane displacement under seismic action, providing enhanced safety.
- (2)
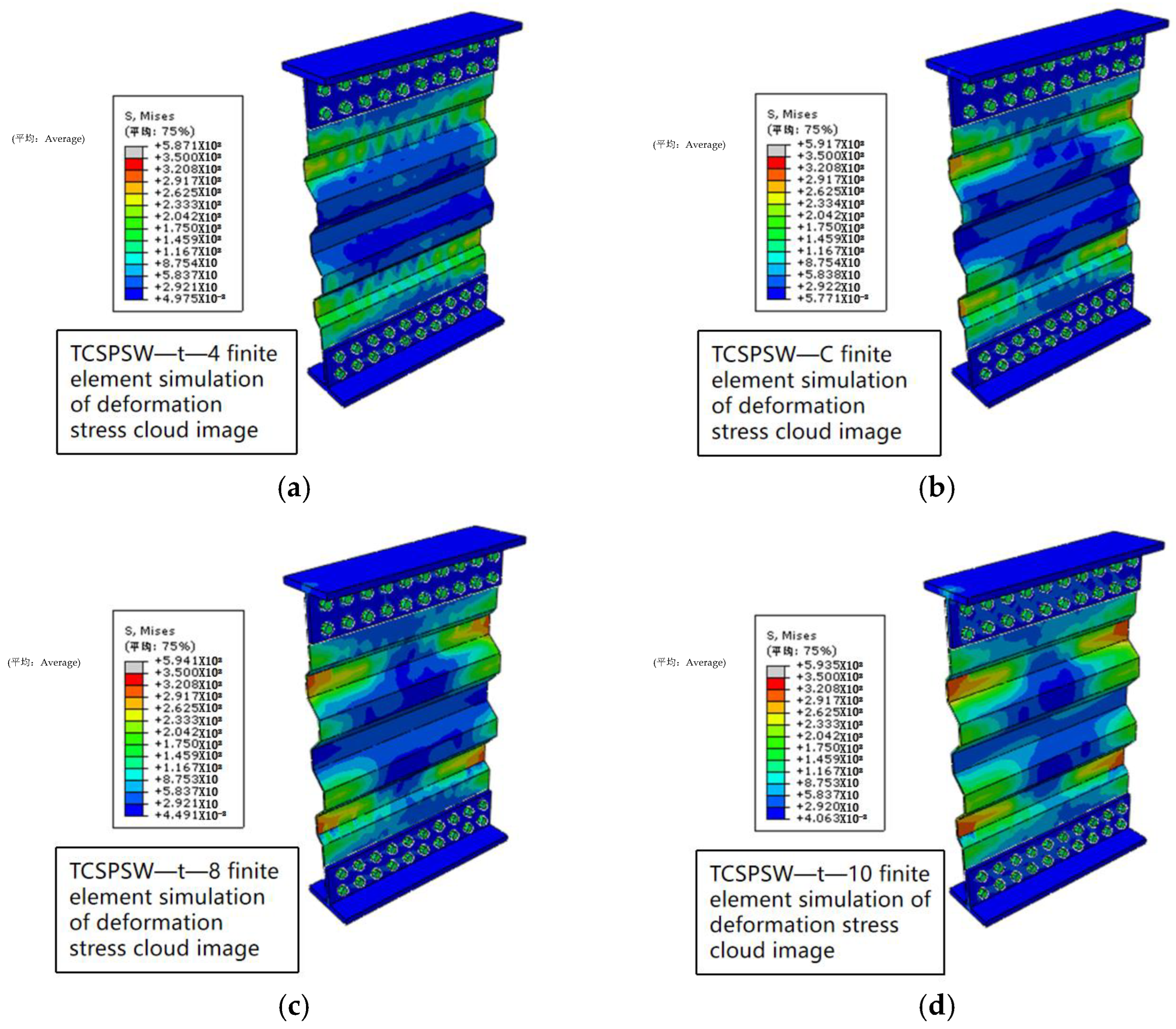
- The “accordion-like” configuration of corrugated steel plate shear walls significantly enhances out-of-plane stability. Under seismic action, the maximum out-of-plane deformation of the corrugated steel plate is reduced by 40% compared to that of the flat steel plate. Moreover, the failure mode is primarily characterized by local buckling, which avoids the risk of overall instability.
- (3)
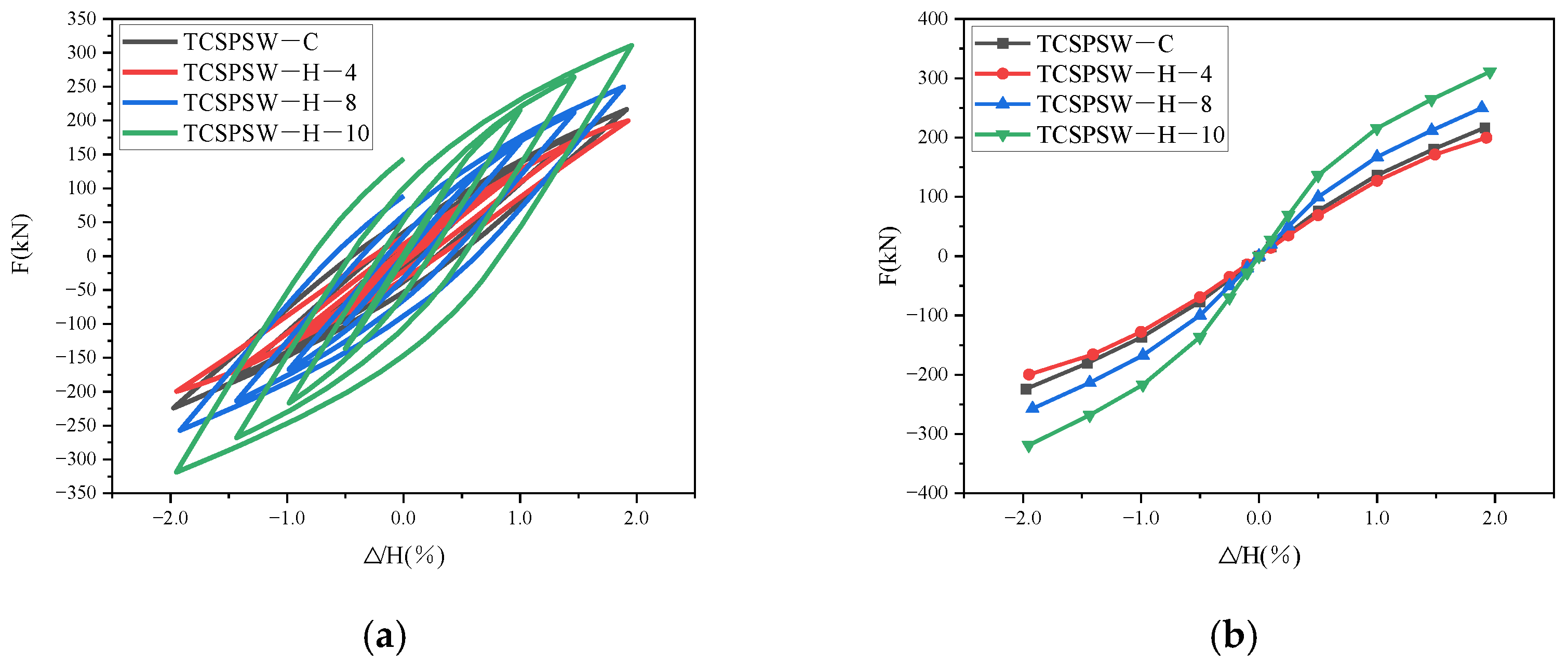
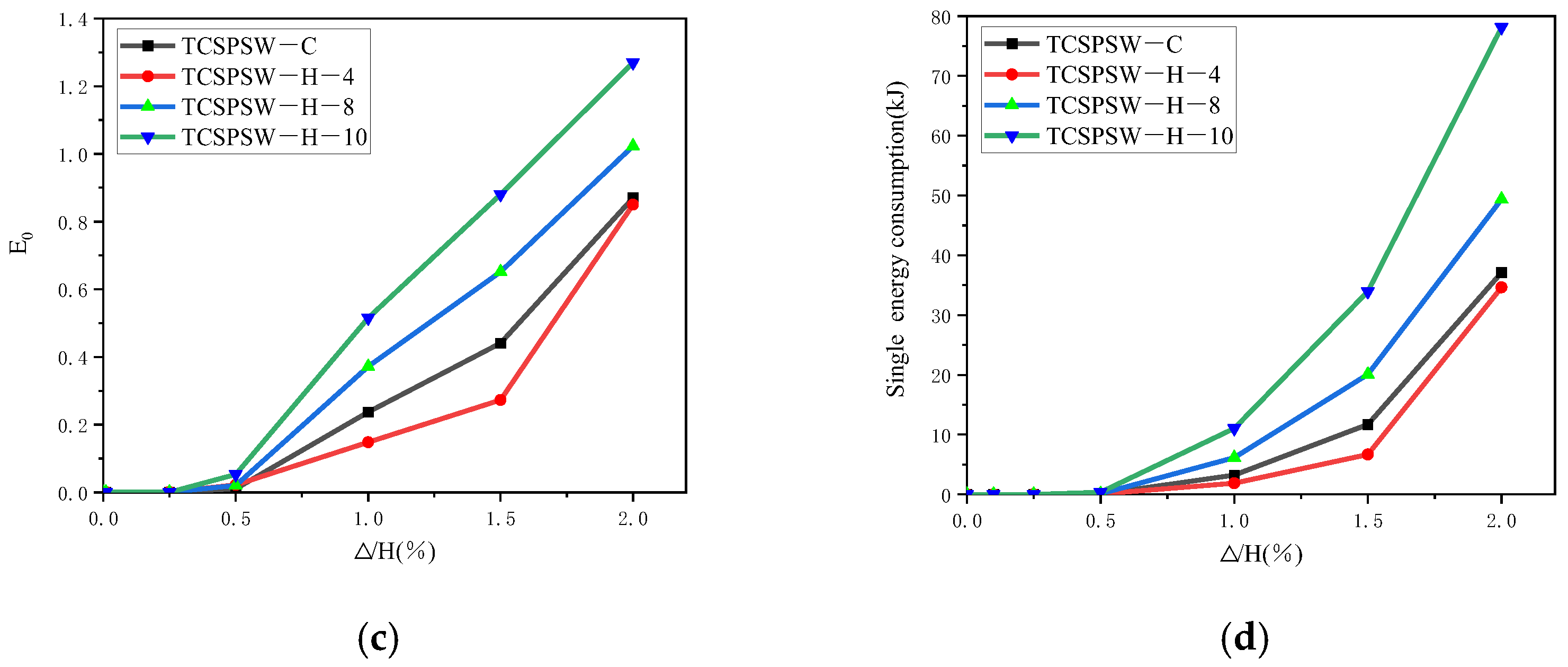
- There is a significant positive correlation between the plate thickness and the energy dissipation performance of corrugated steel plate shear walls. As the plate thickness increases, both the energy dissipation coefficient and the energy dissipation of the corrugated steel plate shear walls significantly improve. At the inter-story drift angle stage of ∆/H = 2.0%, the model with a plate thickness of 10 mm has the highest energy dissipation coefficient and energy dissipation, which are 49.38% and 125.50% higher than those of the model with a plate thickness of 4 mm, respectively. This indicates that increasing the plate thickness can effectively enhance the energy dissipation capacity of the structure, especially under larger inter-story drift angles, where the energy dissipation performance of thicker plates is more prominent.
- (4)
- The aspect ratio has a significant impact on the energy dissipation performance of corrugated steel plate shear walls. The model with an aspect ratio of 1.5 has the highest energy dissipation coefficient and energy dissipation at the stage of ∆/H = 2.0%, which is three times that of the model with an aspect ratio of 0.5. This indicates that increasing the aspect ratio of the corrugated steel plate can effectively enhance the energy dissipation performance of the structure.
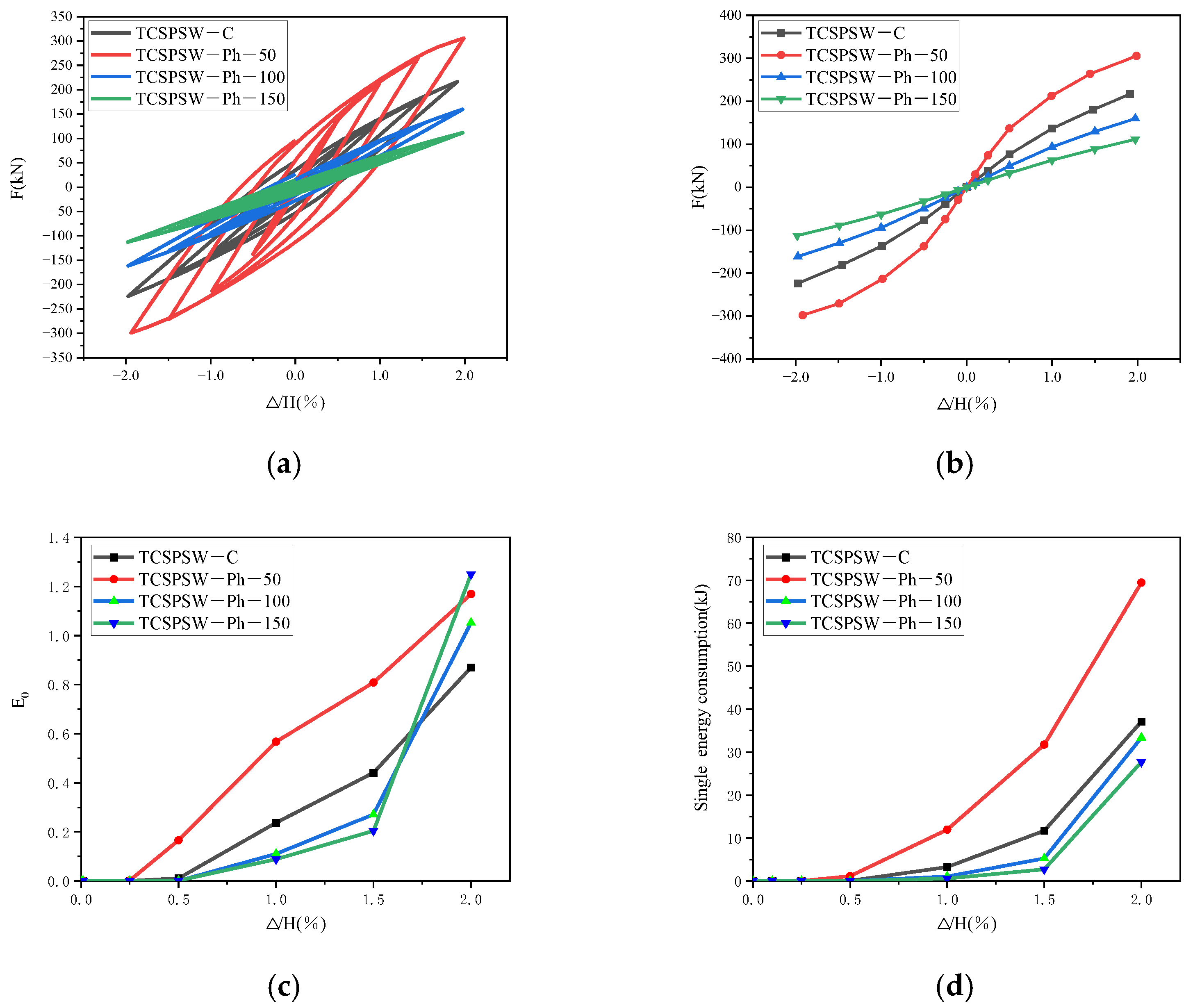
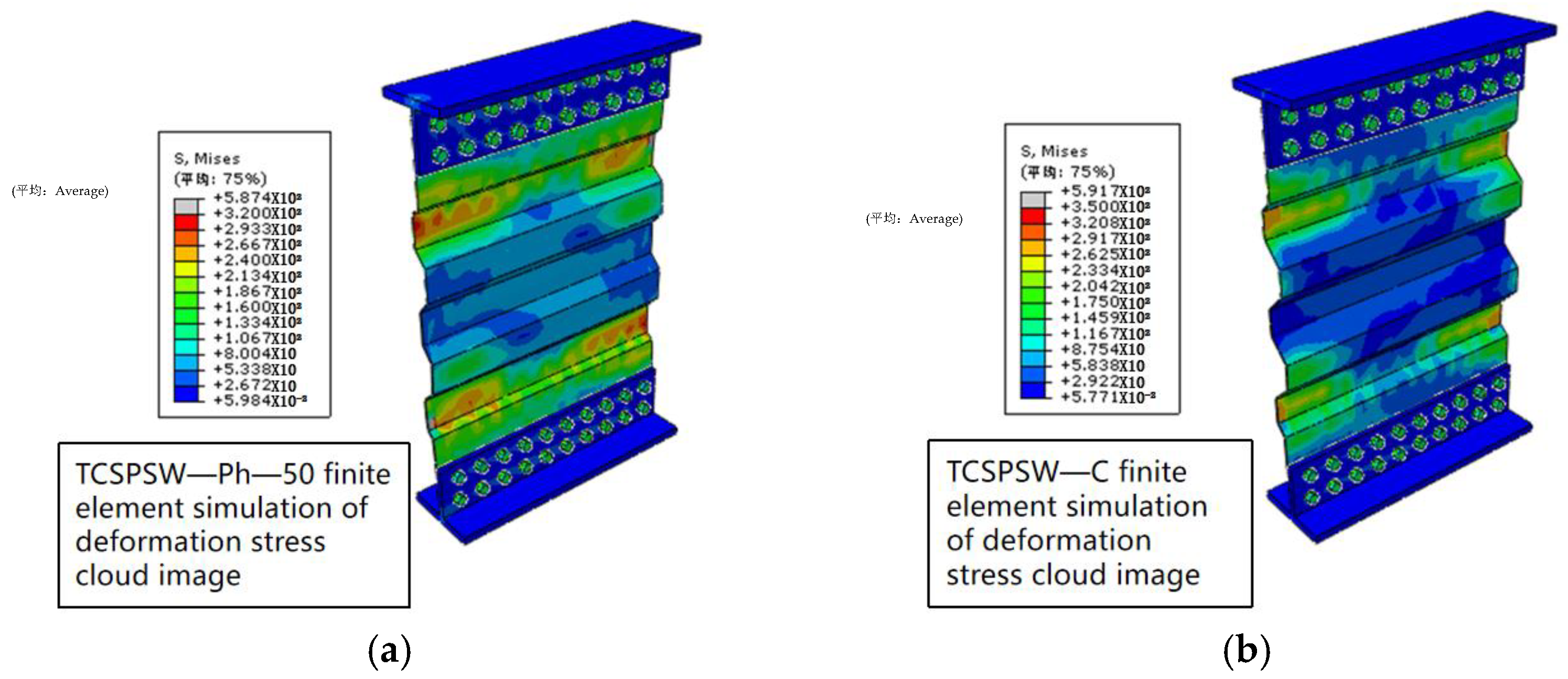
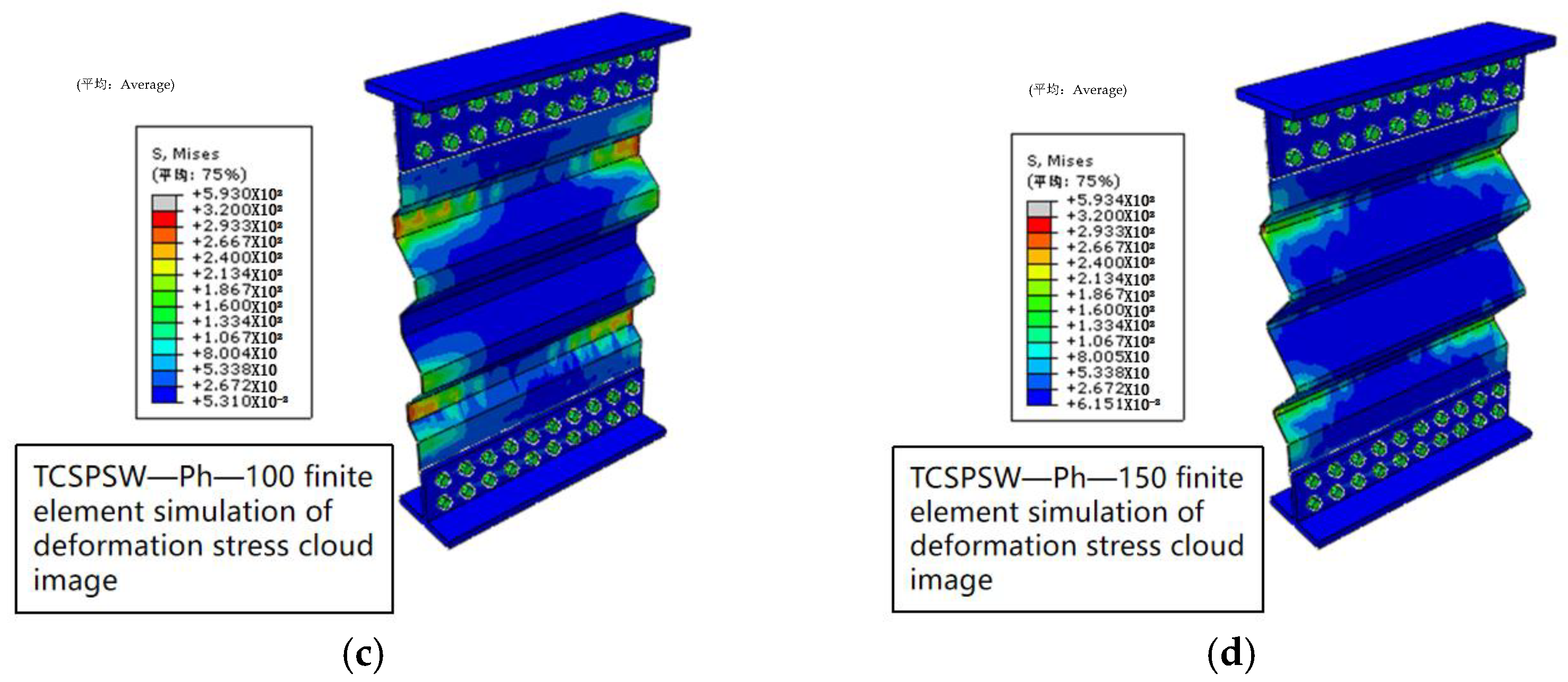
- (5)
- The increase of corrugation height has a negative impact on the energy dissipation capacity of corrugated steel plate shear walls. As the corrugation height increases from 50 mm to 150 mm, the lateral load-bearing capacity of the model decreases by about 35%, and the energy dissipation decreases by 150.6%. This indicates that increasing the corrugation height reduces the lateral load-bearing capacity and energy dissipation capacity of the corrugated steel plate. However, at a specific loading stage (∆/H = 2.0%), the energy dissipation coefficient of the high-corrugation-height model exceeded that of the low-corrugation-height model. This may be related to the structure entering a deeper nonlinear response stage. It is suggested to strengthen the trough area by welding the ribbed plate when the corrugated height is too high to avoid the early damage caused by stress concentration.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Berman, J.W.; Bruneau, M. Experimental Investigation of Light-Gauge Steel Plate Shear Walls. J. Struct. Eng. 2005, 131, 259–267. [Google Scholar] [CrossRef]
- Botros, R.B.G. Nonlinear Finite Element Analysis of Corrugated Steel Plate Shear Walls. Master’s Thesis, University of Calgary, Calgary, AB, Canada, 2006. [Google Scholar]
- Farzampour, A.; Laman, J.A.; Mofid, M. Behavior prediction of corrugated steel plate shear walls with openings. J. Constr. Steel Res. 2015, 114, 258–268. [Google Scholar] [CrossRef]
- Vaziri, E.; Gholami, M.; Gorji Azandariani, M. The Wall—Frame Interaction Effect in Corrugated Steel Plate Shear Walls Systems. Int. J. Steel Struct. 2021, 21, 1680–1697. [Google Scholar] [CrossRef]
- Cao, Q.; Huang, J. Shear Behavior and Analytical Method of Vertically Corrugated Steel Plate Shear Walls with Inelastic Buckling of Infilled Plates. Buildings 2023, 13, 2184. [Google Scholar] [CrossRef]
- Zheng, L.; Wang, W.; Ge, H.; Guo, H.; Gao, Y.; Han, Y. Seismic performance of steel corrugated plate structural walls with different corrugation inclinations. J. Constr. Steel Res. 2022, 192, 107248. [Google Scholar] [CrossRef]
- Yadollahi, Y.; Pakar, I.; Bayat, M. Evaluation and comparison of behavior of corrugated steel plate shear walls. Lat. Am. J. Solids Struct. 2015, 12, 763–786. [Google Scholar] [CrossRef]
- Deng, R.; Yang, J.-D.; Wang, Y.-H.; Li, Q.-Q.; Tan, J.-K. Cyclic shear performance of built-up double-corrugated steel plate shear walls: Experiment and simulation. Thin-Walled Struct. 2022, 181, 110077. [Google Scholar] [CrossRef]
- Zhu, B.L.; Bai, W.H.; Wen, C.B.; Zuo, J.Q.; Sun, H.J.; Wang, X.; Guo, Y.L. Experimental and numerical investigation into hysteretic performance of orthogonal double corrugated steel plate shear wall. Thin-Walled Struct. 2024, 195, 111392. [Google Scholar] [CrossRef]
- Wang, W.; Quan, C.-C.; Li, Y.; Zhen, G.-K.; Zhao, H.-T. Experimental study and numerical simulation analysis on seismic performance of corrugated steel-plate shear wall with replaceable bottom corner dampers. Soil Dyn. Earthq. Eng. 2022, 152, 107061. [Google Scholar] [CrossRef]
- Shariati, M.S.S. Numerical study on the structural performance of corrugated low yield point steel plate shear walls with circular openings. Steel Compos. Struct. Int. J. 2019, 33, 569–581. [Google Scholar]
- Bahrebar, M.; Lim, J.B.; Clifton, G.C.; Zirakian, T.; Shahmohammadi, A.; Hajsadeghi, M. Response assessment and prediction of low yield point steel plate shear walls with curved corrugated web plates and reduced beam sections. Structures 2020, 28, 1729–1745. [Google Scholar] [CrossRef]
- Yu, C.-Q.; Tong, J.-Z.; Tong, G.-S.; Xu, S.-L.; Chen, M. Axial compressive performance and design of multi-celled corrugated-plate CFST walls. Structures 2023, 57, 105303. [Google Scholar] [CrossRef]
- Yu, J.-G.; Zhu, S.-Q. Study on seismic behavior of oblique CFRP corrugated plate-steel plate light weight sandwich composite shear wall. Structures 2023, 48, 2062–2081. [Google Scholar] [CrossRef]
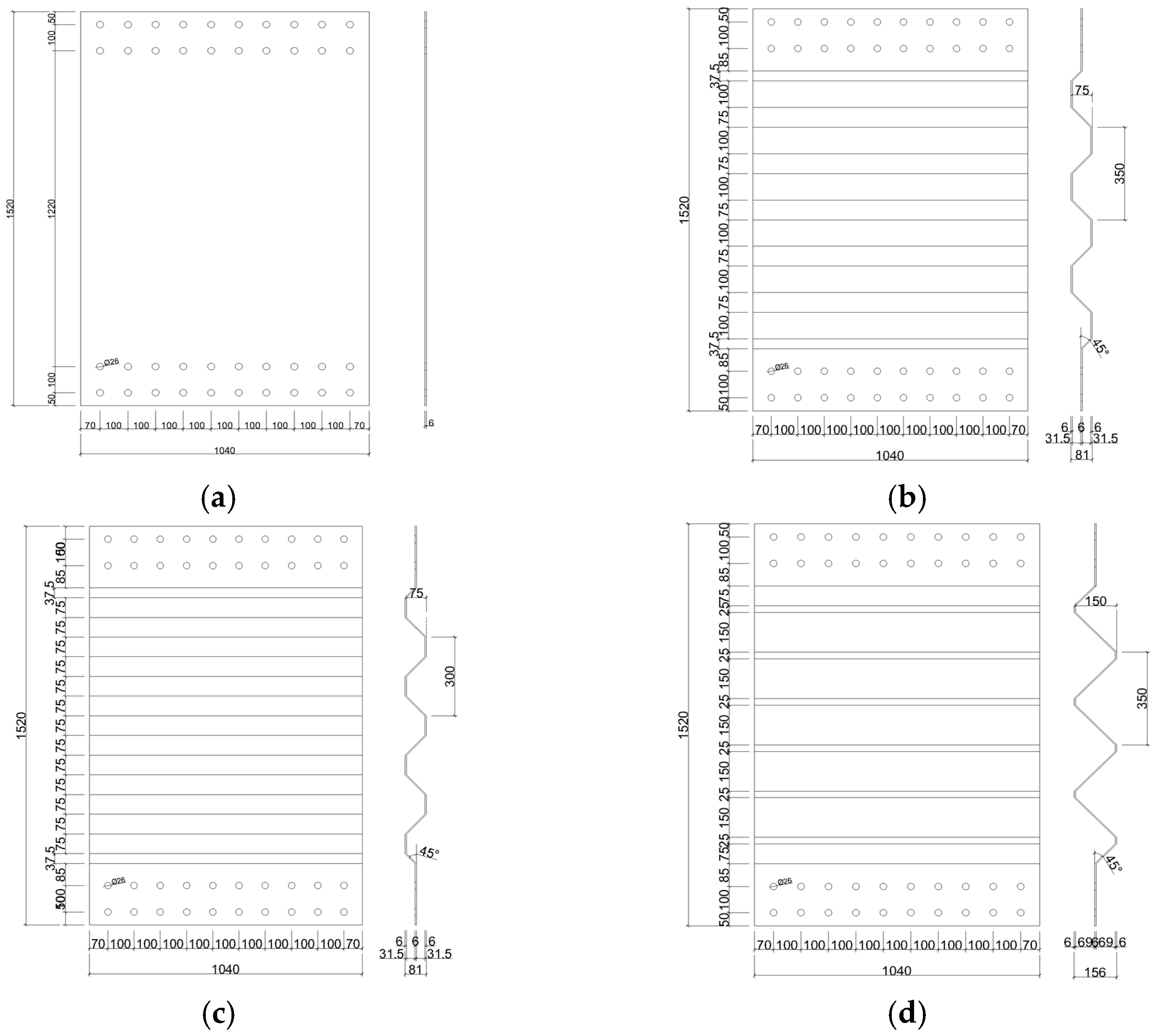






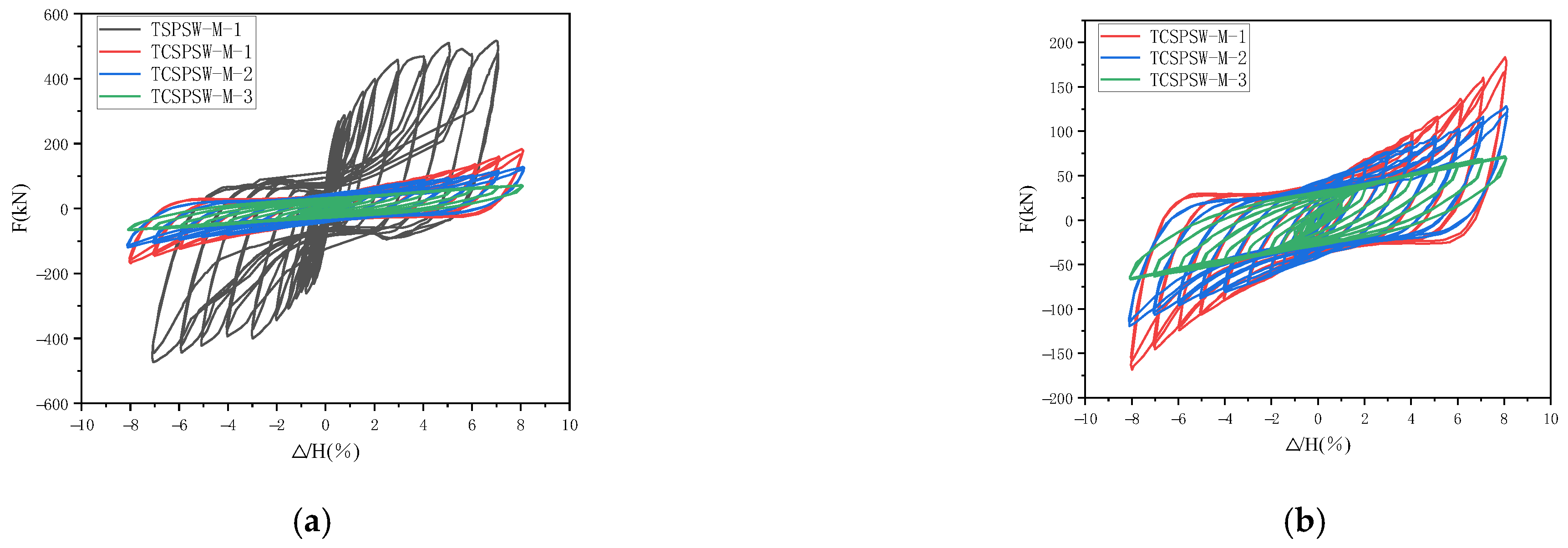
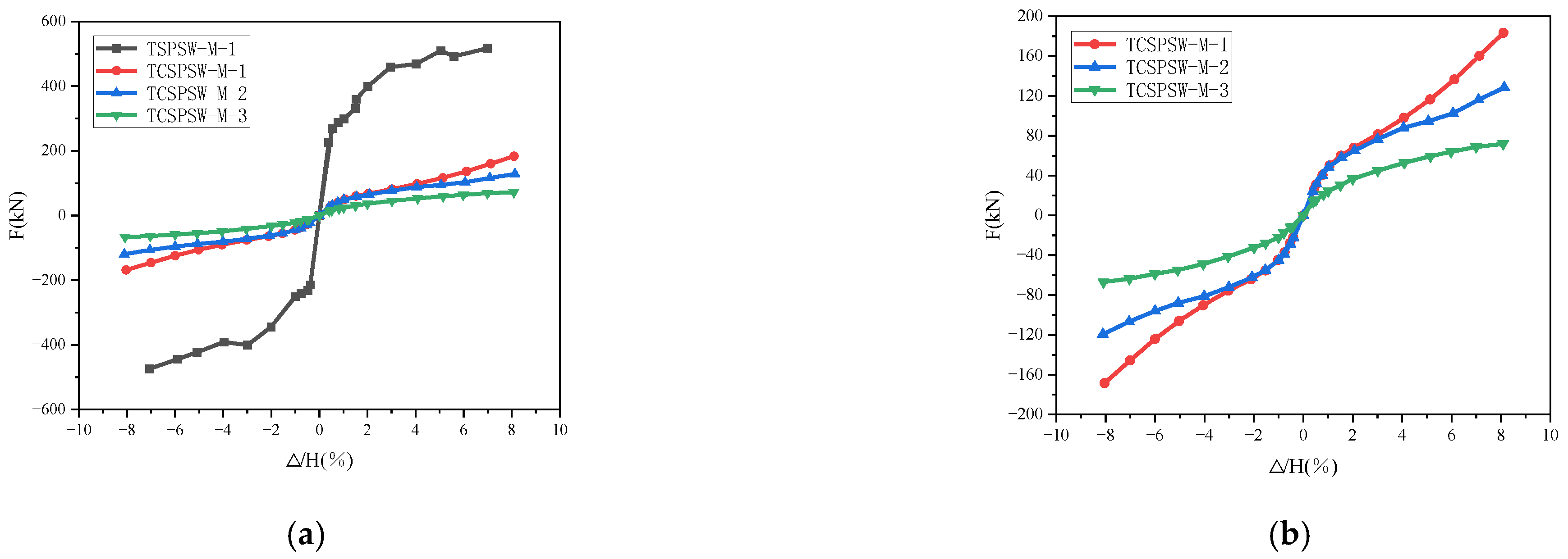


| Specimen ID | Plate Height (mm) | Plate Width (mm) | Wave Height (mm) | Wavelength (mm) | Thickness (mm) |
|---|---|---|---|---|---|
| TSPSW-M-1 | 1520 | 1040 | 6 | ||
| TCSPSW-M-1 | 1520 | 1040 | 75 | 350 | 6 |
| TCSPSW-M-2 | 1520 | 1040 | 75 | 250 | 6 |
| TCSPSW-M-3 | 1520 | 1040 | 150 | 350 | 6 |
| Material Name | Yield Strength (MPa) | Tensile Strength (MPa) | Elongation (%) | Elasticity Modulus (GPa) |
|---|---|---|---|---|
| Q235 | 228.9 | 363.6 | 26 | 196 |
| Q355 | 349.5 | 455.0 | 22 | 204 |
| M24 high-strength bolt | 934.1 | 1011.9 | 12 | 206 |
| Model Number | Variable | Plate Height (mm) | Plate Width (mm) | Plate Thickness (mm) | Wave Height (mm) |
|---|---|---|---|---|---|
| TCSPSW-C | C | 1520 | 1040 | 6 | 75 |
| TCSPSW-t-4 | t | 1520 | 1040 | 4 | 75 |
| TCSPSW-t-8 | 1520 | 1040 | 8 | 75 | |
| TCSPSW-t-10 | 1520 | 1040 | 10 | 75 | |
| TCSPSW-w/H-0.5 | w/H | 1520 | 760 | 6 | 75 |
| TCSPSW-w/H-1.0 | 1520 | 1520 | 6 | 75 | |
| TCSPSW-w/H-1.5 | 1520 | 2280 | 6 | 75 | |
| TCSPSW-Ph-50 | Ph | 1520 | 1040 | 6 | 100 |
| TCSPSW-Ph-100 | 1520 | 1040 | 6 | 125 | |
| TCSPSW-Ph-150 | 1520 | 1040 | 6 | 150 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, J.; Chen, Z.; Zhao, D.; Chen, S. Research and Parameter Analysis of Lateral Resistance Performance of Assembled Corrugated Steel Plate Shear Wall. Appl. Sci. 2025, 15, 4369. https://doi.org/10.3390/app15084369
He J, Chen Z, Zhao D, Chen S. Research and Parameter Analysis of Lateral Resistance Performance of Assembled Corrugated Steel Plate Shear Wall. Applied Sciences. 2025; 15(8):4369. https://doi.org/10.3390/app15084369
Chicago/Turabian StyleHe, Jianian, Zheng Chen, Dongzhuo Zhao, and Shizhe Chen. 2025. "Research and Parameter Analysis of Lateral Resistance Performance of Assembled Corrugated Steel Plate Shear Wall" Applied Sciences 15, no. 8: 4369. https://doi.org/10.3390/app15084369
APA StyleHe, J., Chen, Z., Zhao, D., & Chen, S. (2025). Research and Parameter Analysis of Lateral Resistance Performance of Assembled Corrugated Steel Plate Shear Wall. Applied Sciences, 15(8), 4369. https://doi.org/10.3390/app15084369






