A Model to Study the Creep Behavior of Carbon Fiber/Epoxy Resin Composites Under Temperature
Abstract
1. Introduction
- -
- Development of an interpolator model for the study of the influence of temperature on the behavior of carbon fiber-reinforced composite materials;
- -
- Experimental measurements for the analysis of the method and the study of a material frequently used in engineering applications;
- -
- Creation of an experimental basis that can also serve as the basis for verifying other models.
2. Literature Review
3. Materials and Methods
3.1. Constitutive Relations
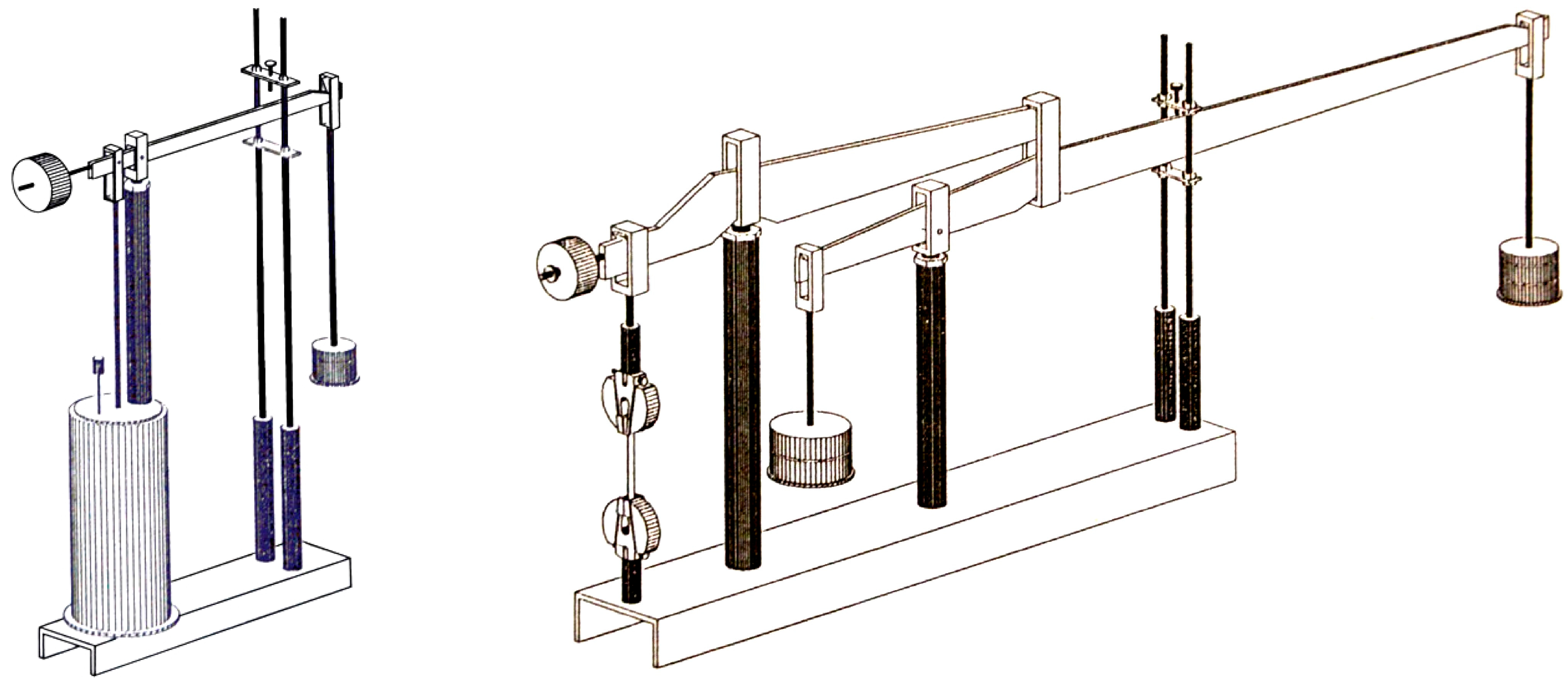
3.2. Experimental Background
3.3. Mathematical Model
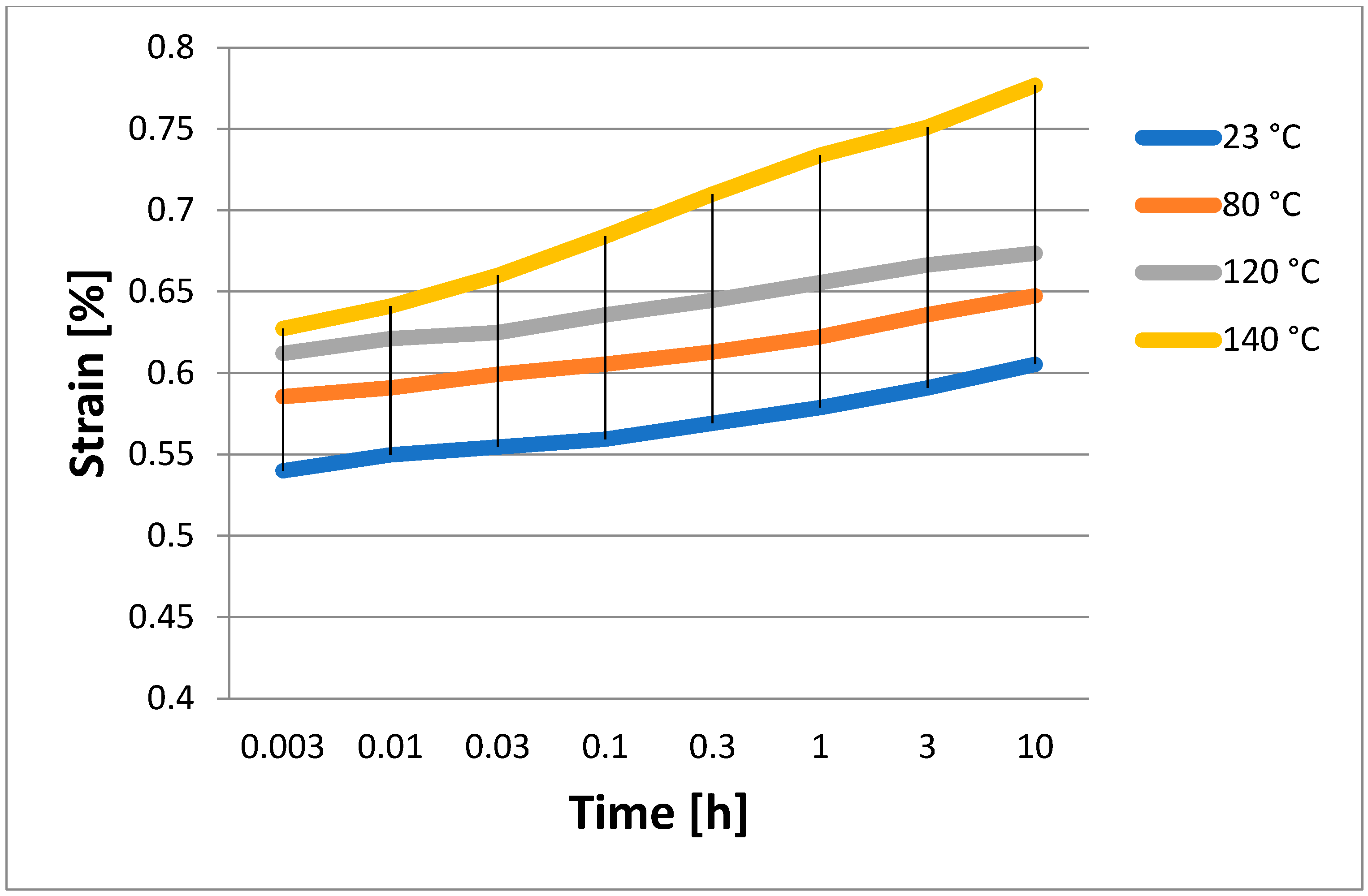
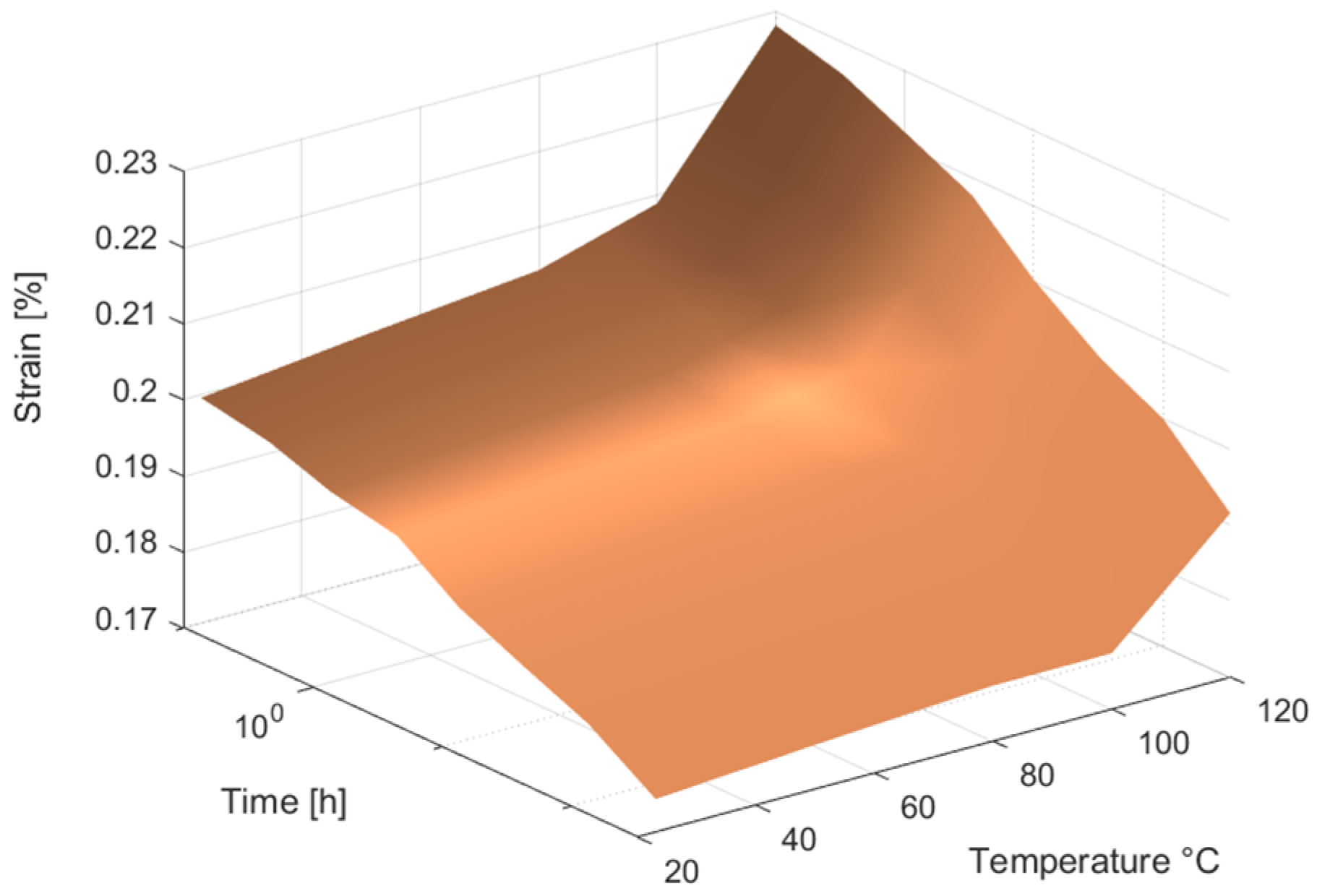
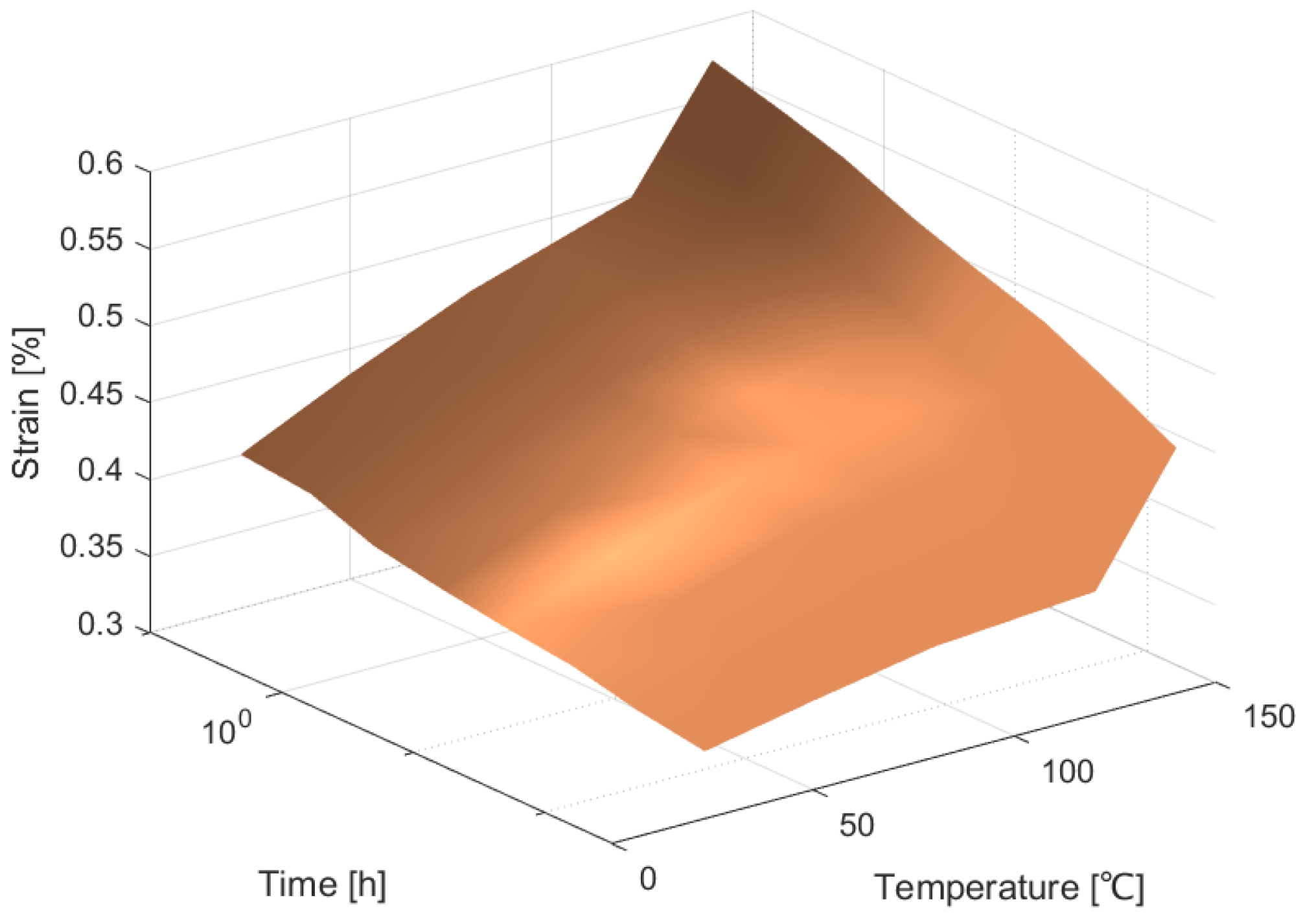
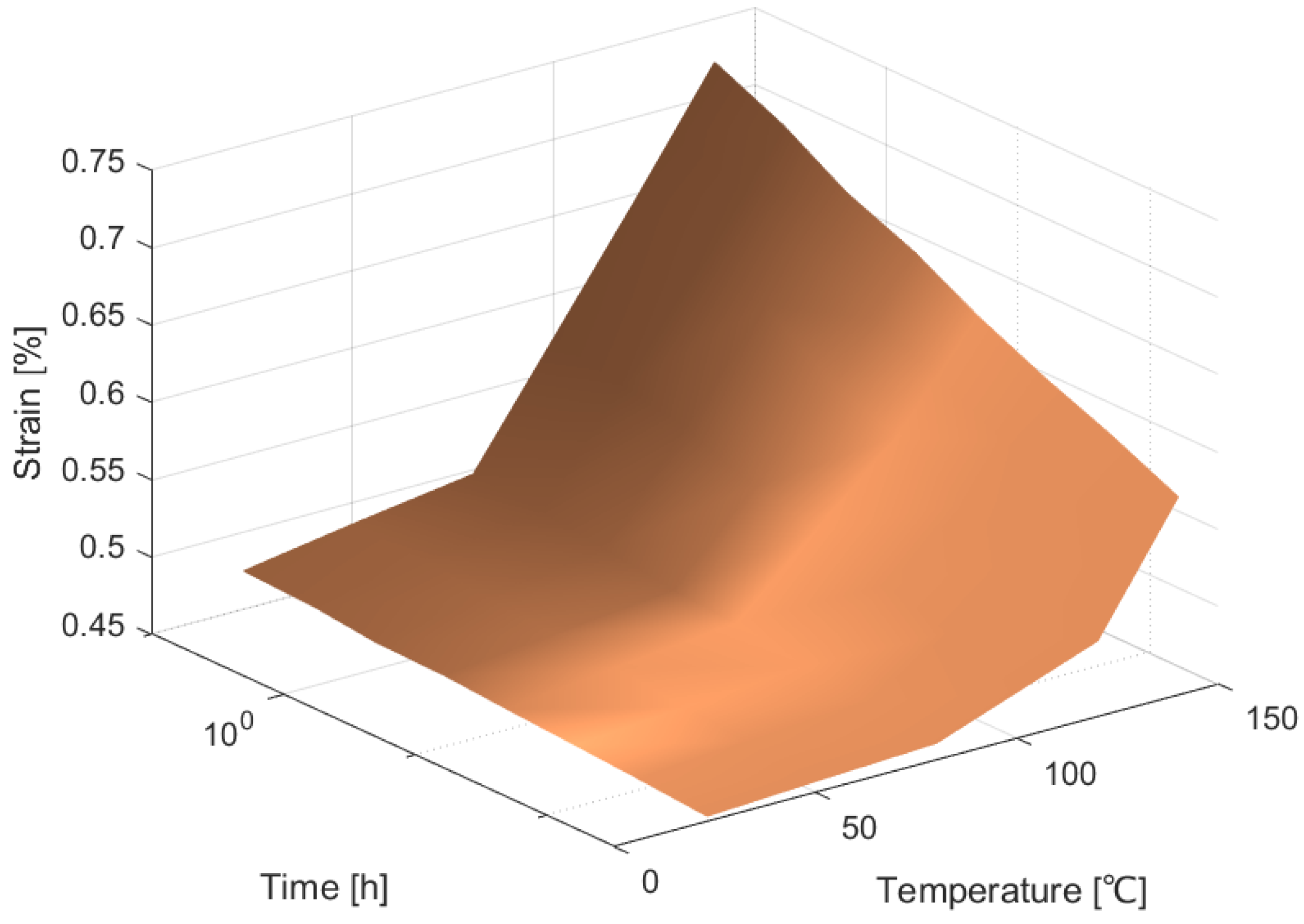
4. Results
4.1. Material and Specimens
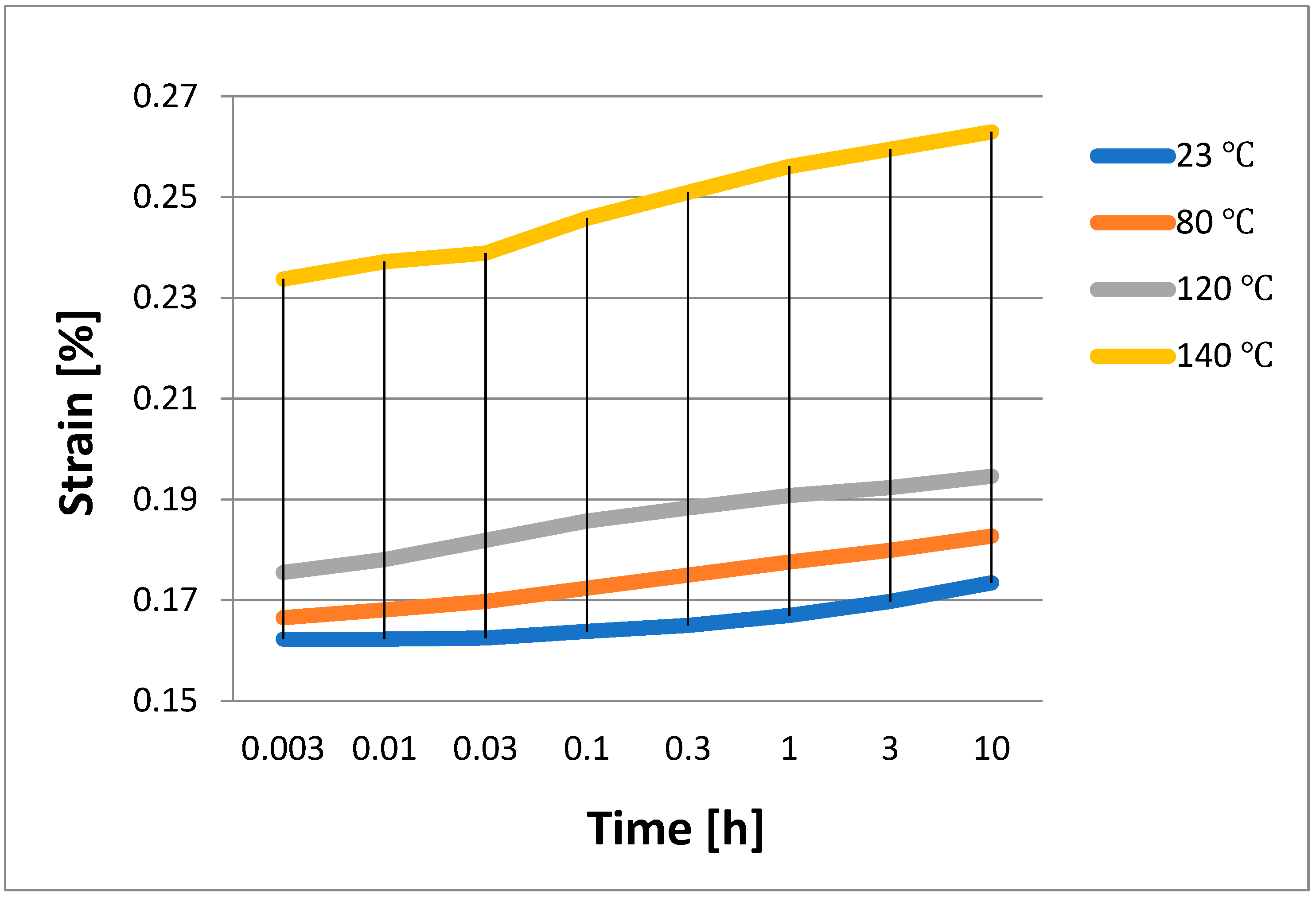
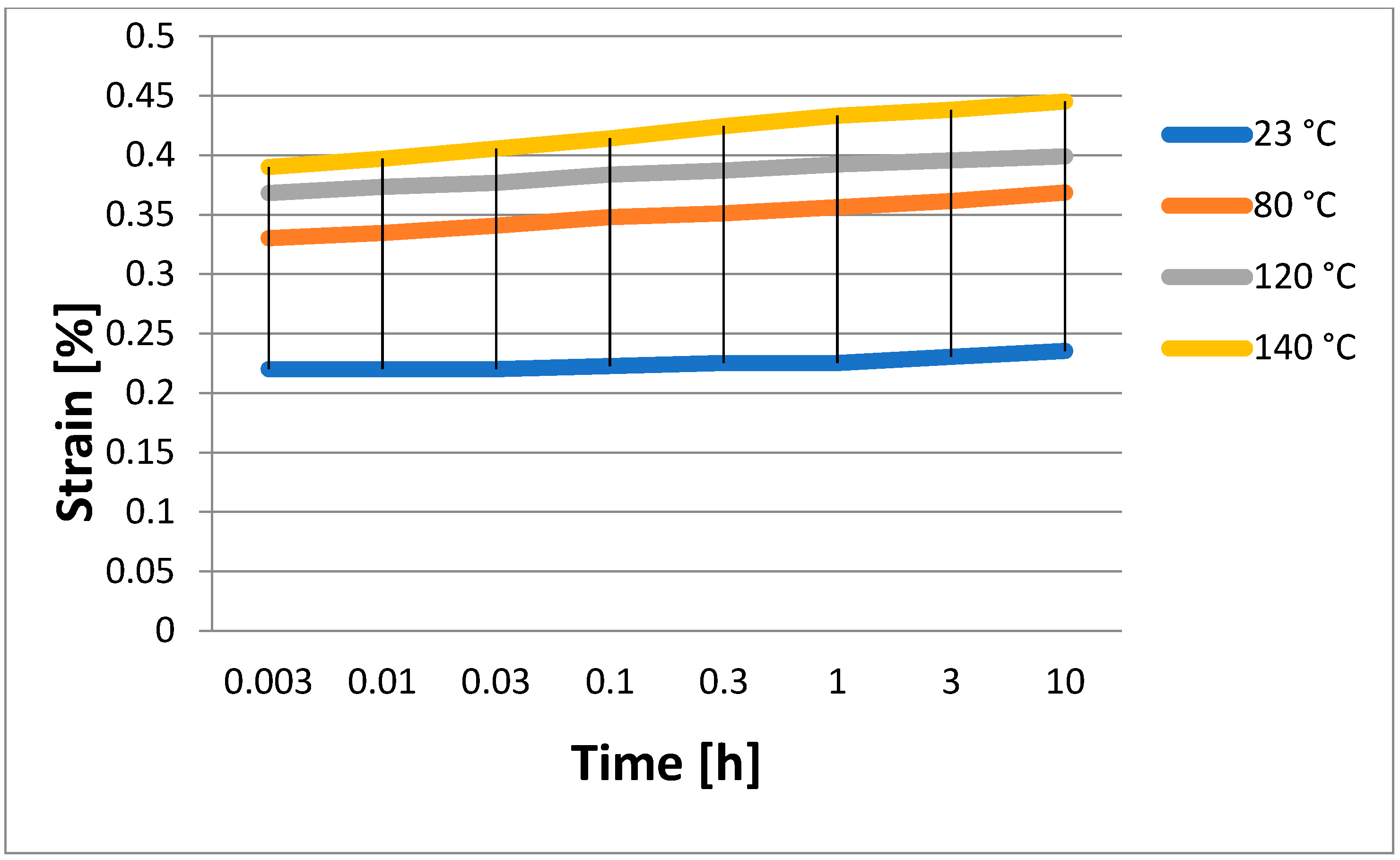
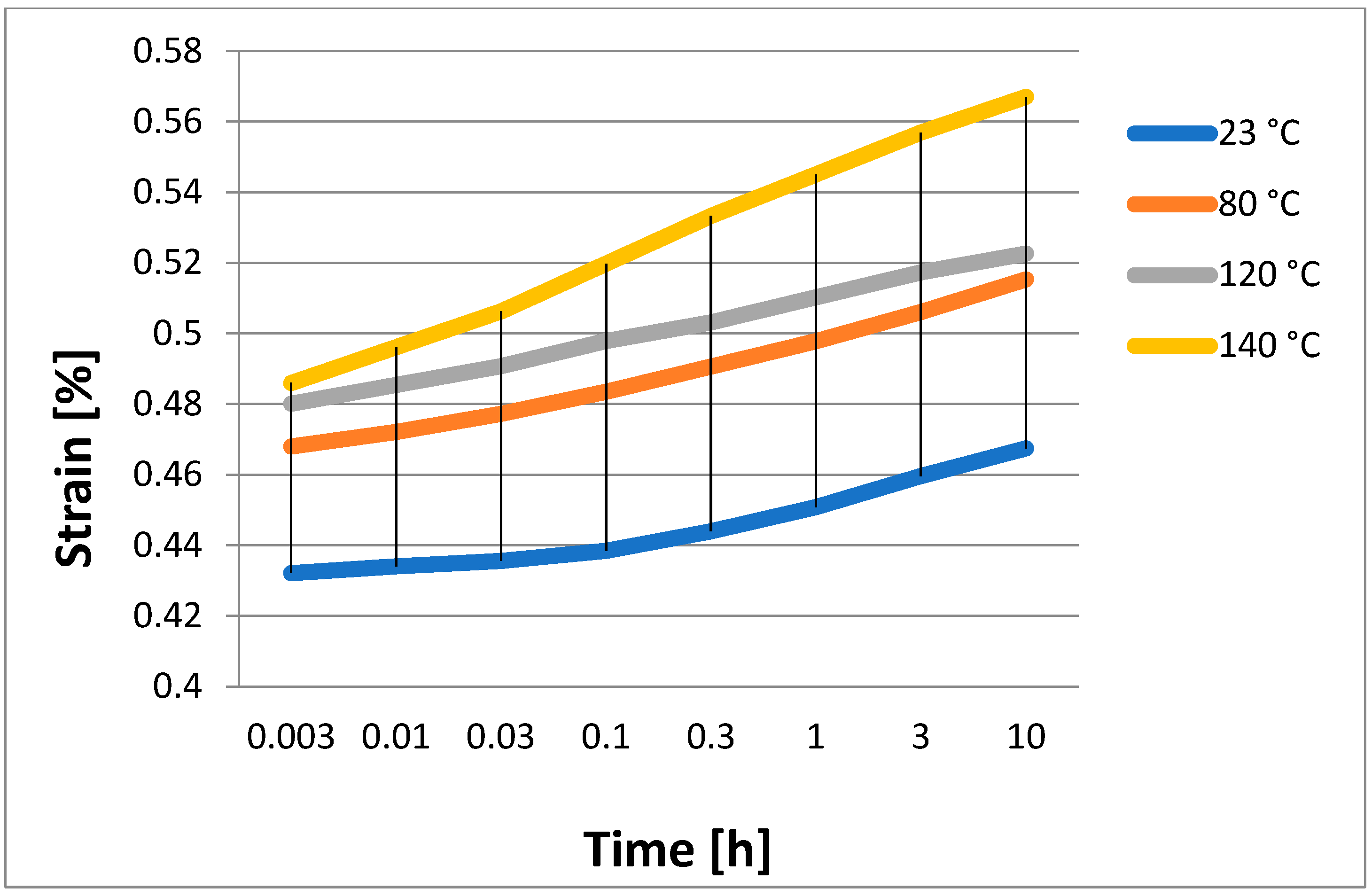
4.2. Results of Measurements
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Available online: www.hexcel.com (accessed on 5 March 2025).
- Available online: https://www.knowde.com/ (accessed on 5 March 2025).
- ISO R527; Plastics—Determination of Tensile Properties. ISO: Geneva, Switzerland, 1966.
- ISO 178; Plastics—Determination of Flexural Properties. ISO: Geneva, Switzerland, 2019.
- Li, M.Z.; Jia, X.Y.; Han, Y.C.; Gao, L.H.; Ma, Z. Investigation of damage mechanisms in carbon fiber epoxy resin composites under laser irradiation. Polym. Compos. 2025. early access. [Google Scholar]
- Yu, B.S.; Zhang, Y.W.; Liu, Y.; Jin, Y.W.; Song, Y.H.; Peng, M. Enhancing the mechanical properties of carbon fiber/epoxy resin laminates through a combination of carbon fiber coating and matrix reinforcement with an aminated para-polyamide. Compos. Part A Appl. Sci. Manuf. 2025, 191, 108740. [Google Scholar]
- Donda, G.M.; Ortega, F.D.; Xavier, G.D.; Lima, J.V.F.; Ricardo, F.V.; Passador, F.R.; de Oliveira, I.R. Effects of graphene nanoplatelets dispersion on the mechanical properties of epoxy resin and carbon fiber laminated composites. J. Compos. Mater. 2025. early access. [Google Scholar]
- Lu, J.J.; Zheng, C.X.; Wang, L.; Dai, Y.C.; Wang, Z.Y.; Song, Z.B. T700 Carbon Fiber/Epoxy Resin Composite Material Hygrothermal Aging Model. Materials 2025, 18, 369. [Google Scholar] [CrossRef]
- Duan, X.; Yuan, H.; Tang, W.; He, J.; Guan, X. A General Temperature-Dependent Stress–Strain Constitutive Model for Polymer-Bonded Composite Materials. Polymers 2021, 13, 1393. [Google Scholar] [CrossRef]
- Luo, L.; Luo, Q.; Zhang, G.; Li, Q.; Sun, G. On strain rate and temperature dependent mechanical properties and constitutive models for additively manufactured polylactic acid (PLA) materials. Thin-Walled Struct. 2022, 179, 109624. [Google Scholar] [CrossRef]
- Erartsin, O.; van Drongelen, M.; Govaert, L.E. Identification of plasticity-controlled creep and fatigue failure mechanisms in transversely loaded unidirectional thermoplastic composites. J. Compos. Mater. 2021, 55, 1947–1965. [Google Scholar] [CrossRef]
- Schapery, R.A. Stress Analysis of Viscoelastic Composite Materials. J. Compos. Mater. 1967, 1, 228. [Google Scholar]
- Jamshidi, M.; Shokrieh, M.M. On the Schapery nonlinear viscoelastic model: A review. Eur. J. Mech. A-Solids 2024, 108, 105403. [Google Scholar]
- Katouzian, M. On the Effect of Temperature on Creep Behavior of Neat and Carbon Fiber Reinforced PEEK and Epoxy—A micromechanical Approach. Ph.D. Thesis, Dresden University of Technology, Dresden, Germany, 1995. [Google Scholar]
- Kovacevic, D.; Sundararajan, B.K.; van der Meer, F.P. Micromechanical model for off-axis creep rupture in unidirectional composites undergoing finite strains. Compos. Part A Appl. Sci. Manuf. 2024, 176, 107860. [Google Scholar]
- Ha, S.K.; Wang, Q.L.; Chang, F.K. Modeling the Viscoplastic Behavior of Fiber-Reinforced Thermoplastic Matrix Composites at Elevated Temperatures. J. Compos. Mater. 1991, 25, 334–374. [Google Scholar] [CrossRef]
- Katouzian, M.; Bruller, O.S.; Horoschenkoff, A. On the Effect of Temperature on the Creep-Behavior of Neat and Carbon-Fiber-Reinforced PEEK and Epoxy-Resin. J. Compos. Mater. 1995, 29, 372–387. [Google Scholar] [CrossRef]
- Niculiţă, C.; Vlase, S.; Bencze, A.; Mihălcică, M.; Calin, M.R.; Serbina, L. Optimum stacking in a multi-ply laminate used for the skin of adaptive wings. Optoelectron. Adv. Mater.—Rapid Commun. 2011, 5, 1233–1236. [Google Scholar]
- Yadav, A.K.; Carrera, E.; Marin, M.; Othman, M.I. Reflection of hygrothermal waves in a Nonlocal Theory of coupled thermo-elasticity. Mech. Adv. Mater. Struct. 2024, 31, 1083–1096. [Google Scholar] [CrossRef]
- Marin, M.; Abbas, I.; Kumar, R. Relaxed Saint-Venant principle for thermoelastic micropolar diffusion. Struct. Eng. Mech. 2014, 51, 651–662. [Google Scholar] [CrossRef]
- Teodorescu-Draghicescu, H.; Vlase, S. Homogenization and averaging methods to predict elastic properties of pre-impregnated composite materials. Comput. Mater. Sci. 2011, 50, 1310–1314. [Google Scholar] [CrossRef]
- Vlase, S.; Teodorescu-Draghicescu, H.; Serbina, L. Simulation of the elastic properties of some fiber-reinforced composite laminates under off-axis loading system. Optoelectron. Adv. Mater.—Rapid Commun. 2011, 5, 424–429. [Google Scholar]
- Vlase, S.; Teodorescu-Draghicescu, H.; Calin, M.R. Behavior of multiphase fiber-reinforced polymers under short time cyclic loading. Optoelectron. Adv. Mater.—Rapid Commun. 2011, 5, 419–423. [Google Scholar]
- Teodorescu-Draghicescu, H.; Vlase, S.; Mihalcica, M. Advanced Pultruded Glass Fibers-Reinforced Isophthalic Polyester Resin. Plast. Mater. 2015, 52, 62–64. [Google Scholar]
- Ribeiro, B.; Botelho, E.C.; Costa, M.L.; Bandeira, C.F. Carbon nanotube bucky paper reinforced polymer composites: A review. Polymers 2017, 27, 247–255. [Google Scholar]
- Li, Y.L.; Chen, W.J.; Shen, M.Y.; Chiang, C.L.; Yip, M.C. Study on the Mechanical Properties and Creep Behaviour of Carbon Fiber Nano-Composites. International Conference on Advanced Engineering Materials and Technology (AEMT2011). Mater. Des. 2011, 284–286, 557–564. [Google Scholar]
- Daei-Sorkhabi, A.H.; Hosseinzadeh-Nodehi, S.F. Numerical study of the effect of carbon fiber/epoxy resin adhesive thickness on the creep behaviour of carbon steel plate joints. J. Adhes. Sci. Technol. 2019, 33, 1790–1805. [Google Scholar] [CrossRef]
- Nakada, M.; Miyano, Y.; Kageta, S.; Nishida, H.; Hayashi, Y.; Uzawa, K. Prediction of statistical life time for unidirectional CFRTP under cyclic loading. J. Reinf. Plast. Compos. 2021, 40, 749–758. [Google Scholar] [CrossRef]
- Gordelier, T.J.; Thies, P.R.; Turner, L.; Johanning, L. Optimizing the FDM additive manufacturing process to achieve maximum tensile strength: A state-of-the-art review. Rapid Prototyp. J. 2019, 25, 953–971. [Google Scholar] [CrossRef]
- Fairhurst, A.; Thommen, M.; Rytka, C. Comparison of short and long term creep testing in high performance polymers. Polym. Test. 2019, 78, 105979. [Google Scholar] [CrossRef]
- Vlase, S.; Teodorescu-Draghicescu, H.; Scutaru, M.L. Advanced Polylite composite laminate material behavior to tensile stress on weft direction. J. Optoelectron. Adv. Mater. 2012, 14, 658–663. [Google Scholar]
- Fujiwara, T.; Takeuchi, M.; Daoyuan, C.; Liang, Y.; Nishioka, N.; Okayasu, M. Mechanical Properties of Epoxy- and Dicyclopentadiene-Based Carbon-Fiber-Reinforced Plastics at Low and Room Temperatures. Appl. Compos. Mater. 2025. early access. [Google Scholar] [CrossRef]
- Kazanci, M. Carbon fiber reinforced microcomposites in two different epoxies. Polym. Test. 2004, 23, 747–753. [Google Scholar] [CrossRef]
- Battawi, A.A.; Abed, B.H.; Al-Filfily, A.A.H. Enhancements of Creep Compliance of Kevlar and Carbon Fibers Reinforced Sika Epoxy Composites. Rev. Des Compos. Des Mater. Av.-J. Compos. Adv. Mater. 2024, 34, 593–602. [Google Scholar] [CrossRef]
- Tan, H.; Yan, S.; Zhu, S.; Wen, P. Creep Modeling of Composite Materials Based on Improved Gene Expression Programming. Sci. Rep. 2022, 12, 22244. [Google Scholar] [CrossRef]
- Griffith, W.I. The Accelerated Characterization of Viscoelastic Composite Materials. Ph.D. Thesis, VPI & SU, Blacksburg, VA, USA, 1978. [Google Scholar]
- Yeow, Y.T. The Temperature Behavior of Graphite Epoxy Laminates. Ph.D. Thesis, VPI & SU, Blacksburg, VA, USA, 1978. [Google Scholar]
- Sturgeon, J.B. Creep of Fiber Reinforced Thermosetting Resins. In Creep of Engineering Materials; Pomeroy, C.D., Ed.; Mechanical Engineering Publications Ltd.: London, UK, 1978. [Google Scholar]
- Miyano, Y.; Nakada, M.; Morisawa, Y.; Matsuno, J.; Kageta, S. Prediction of creep failure life for unidirectional CFRP with heat-resistant epoxy resin as matrix exposed to high temperature under tension load. J. Compos. Mater. 2024, 58, 1051–1062. [Google Scholar] [CrossRef]
- Yang, J.Y.; Ma, X.F.; Wang, H.; Shang, F.L.; Hou, D.M. Long-term creep deformation of carbon fiber/epoxy composites with physical aging: Experimental investigation and constitutive modeling. Polym. Compos. 2024, 45, 2825–2840. [Google Scholar] [CrossRef]
- Santos, P.; Silva, A.P.; Reis, P. Effect of carbon nanofibers on the viscoelastic response of carbon/epoxy composites. J. Reinf. Plast. Compos. 2023, 43, 1257–1271. [Google Scholar] [CrossRef]
- Cha, J.; Yoon, S. Long-term viscoelastic properties of carbon fiber/epoxy composites using tow prepreg strand specimens. Adv. Compos. Mater. 2004, 33, 151–161. [Google Scholar] [CrossRef]
- Nakada, M.; Miyano, Y.; Nonaka, T.; Morisawa, Y.; Isaki, T.; Hirano, T.; Uzawa, K. Statistical tensile and flexural fatigue lives of unidirectional CF/PP laminates. J. Reinf. Plast. Compos. 2024, 43, 195–204. [Google Scholar] [CrossRef]
- Katouzian, M.; Vlase, S.; Scutaru, M.L. Finite Element Method-Based Simulation Creep Behavior of Viscoelastic Carbon-Fiber Composite. Polymers 2021, 13, 1017. [Google Scholar] [CrossRef]
- Monticeli, F.M.; Ornaghi, H.L.; Neves, R.M.; Cioffi, M.O.H. Creep/recovery and stress-relaxation tests applied in a standardized carbon fiber/epoxy composite: Design of experiment approach. J. Od Strain Anal. Eng. Des. 2021, 55, 109–117. [Google Scholar] [CrossRef]
- Emara, M.; Torres, L.; Baena, M.; Bards, C.; Moawad, M. Effect of sustained loading and environmental conditions on the creep behavior of an epoxy adhesive for concrete structures strengthened with CFRP laminates. Compos. Part B-Eng. 2017, 129, 88–96. [Google Scholar] [CrossRef]
- Raghavan, J.; Meshii, M. Activation Theory for Creep of Matrix Resin and Carbon-Fiber-Reinforced Polymer Composite. J. Mater. Sci. 1994, 29, 5078–5084. [Google Scholar] [CrossRef]
- Raghavan, J.; Meshii, M. Prediction of Creep-Rupture of Unidirectional Carbon-Fiber-Reinforced Polymer Composite. Mater. Sci. Eng. A Struct. Mater. Prop. Microstruct. Process. 1995, 197, 237–249. [Google Scholar] [CrossRef]
- Blassiau, S.; Thionnet, A.; Bunsell, A.R. Micromechanisms of load transfer in a unidirectional carbon fibre-reinforced epoxy composite due to fibre failures. Part 2: Influence of viscoelastic and plastic matrices on the mechanisms of load transfer. Compos. Struct. 2006, 74, 319–331. [Google Scholar] [CrossRef]
- Callister, W.D., Jr.; Rethwisch, D.G. Materials Science and Engineering: An Introduction; Wiley: Hoboken, NJ, USA, 2020. [Google Scholar]
- Fung, Y.C. Fundamentals of Solid Mechanics; Prentice-Hall: Hoboken, NJ, USA, 1965. [Google Scholar]
- Morris, D.H.; Yeow, Y.T.; Brinson, H.F. The Viscoelastic Behavior of the Principal Compliance Matrix of Unidirectional Graphite Epoxy Composite. Polym. Compos. 1980, 1, 32–36. [Google Scholar] [CrossRef]
- DIN EN ISO 8044; Corrosion of Metals and Alloys—Vocabulary. European Standards: Plzen, Czech Republic, 2024. Available online: https://www.iso.org/standard/83222.html (accessed on 11 March 2025).
- DIN 50125; Testing of Metallic Materials—Tensile Test Pieces. European Standards: Plzen, Czech Republic, 2022. Available online: https://www.en-standard.eu/din-50125-testing-of-metallic-materials-tensile-test-pieces (accessed on 11 March 2025).
- Hua, T.; Sirong, Z.; Shilin, Y.; Pin, W. Predictive Model for Creep Behavior of Composite Materials Using Gene Expression Programming. Appl. Compos. Mater. 2023, 30, 1003–1030. [Google Scholar] [CrossRef]
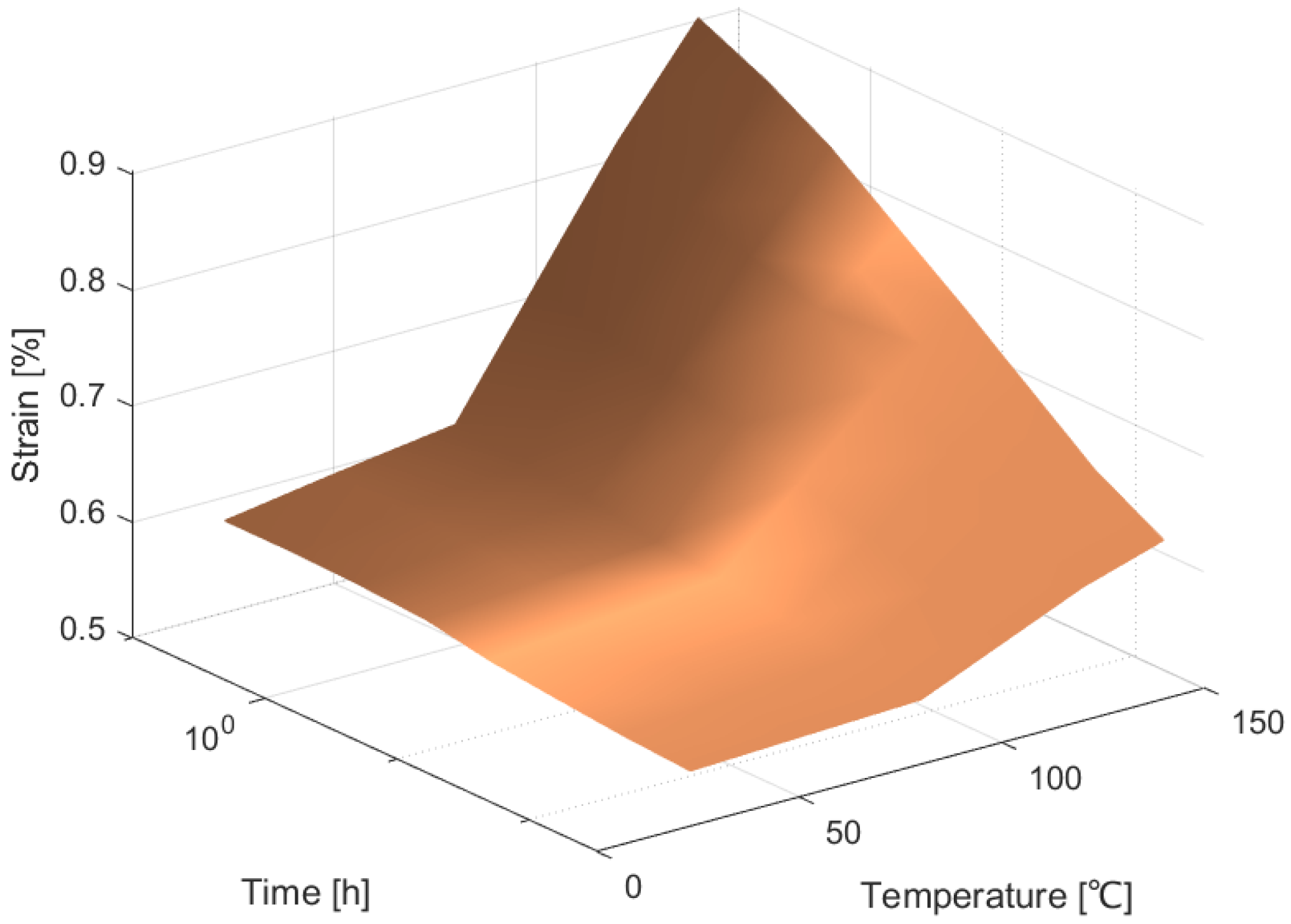
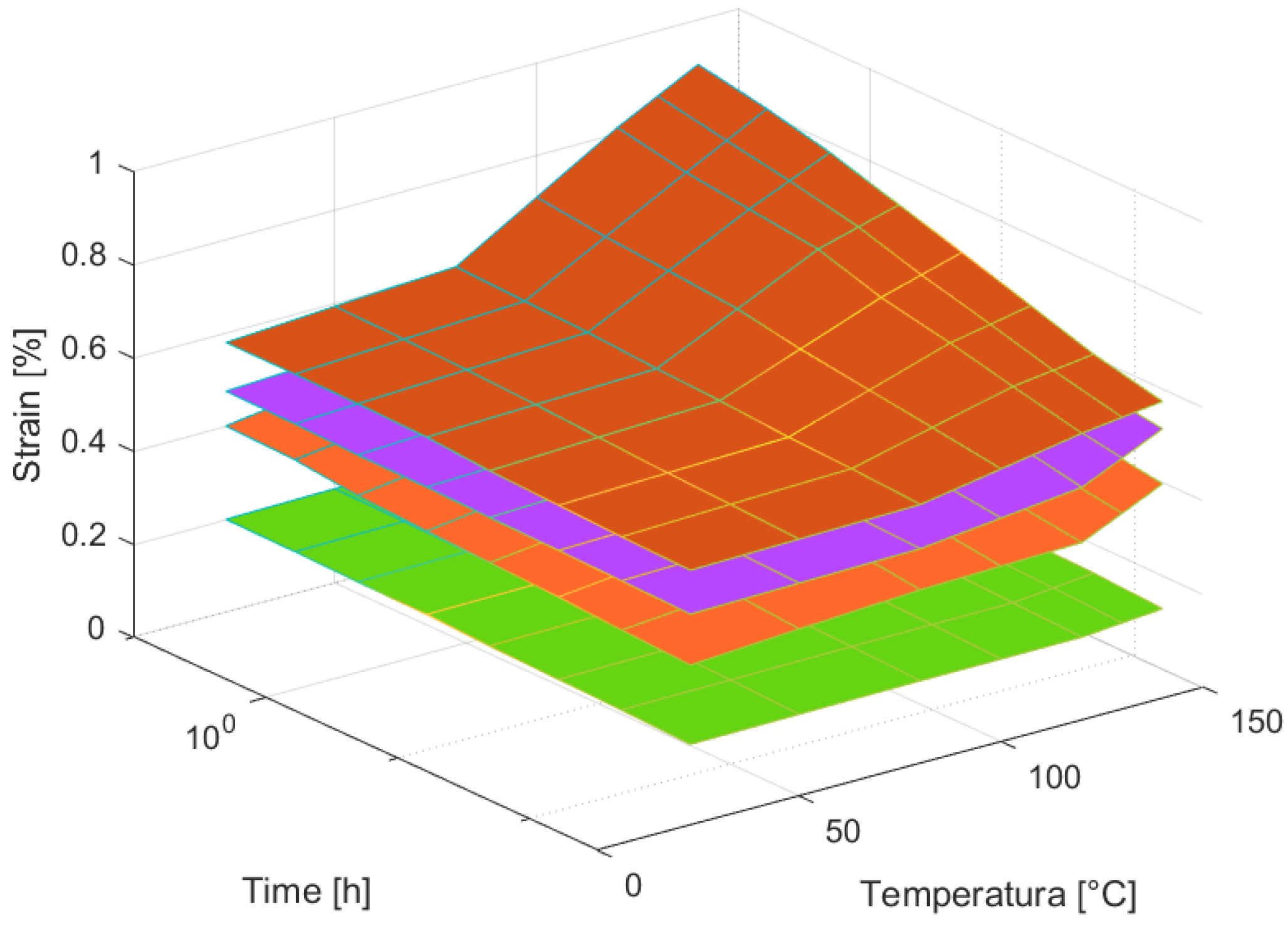

| Nr. | Property | Value |
|---|---|---|
| 1 | Tensile Strength | 105 MPa |
| 2 | Tensile Modulus | 3.60 GPa |
| 3 | Flexural Strength | 144 MPa |
| 4 | Flexural Modulus | 4.4 GPa |
| 5 | Toughness (G1C) | 432 J/m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Katouzian, M.; Vlase, S. A Model to Study the Creep Behavior of Carbon Fiber/Epoxy Resin Composites Under Temperature. Appl. Sci. 2025, 15, 4206. https://doi.org/10.3390/app15084206
Katouzian M, Vlase S. A Model to Study the Creep Behavior of Carbon Fiber/Epoxy Resin Composites Under Temperature. Applied Sciences. 2025; 15(8):4206. https://doi.org/10.3390/app15084206
Chicago/Turabian StyleKatouzian, Mostafa, and Sorin Vlase. 2025. "A Model to Study the Creep Behavior of Carbon Fiber/Epoxy Resin Composites Under Temperature" Applied Sciences 15, no. 8: 4206. https://doi.org/10.3390/app15084206
APA StyleKatouzian, M., & Vlase, S. (2025). A Model to Study the Creep Behavior of Carbon Fiber/Epoxy Resin Composites Under Temperature. Applied Sciences, 15(8), 4206. https://doi.org/10.3390/app15084206







