The Impact of Hollow Wear on the Stability of High-Speed Railway Vehicles
Abstract
1. Introduction
2. The Influence of Tread Hollow Wear on the Wheel–Rail Contact Relationship
2.1. The Profile of Worn Wheels
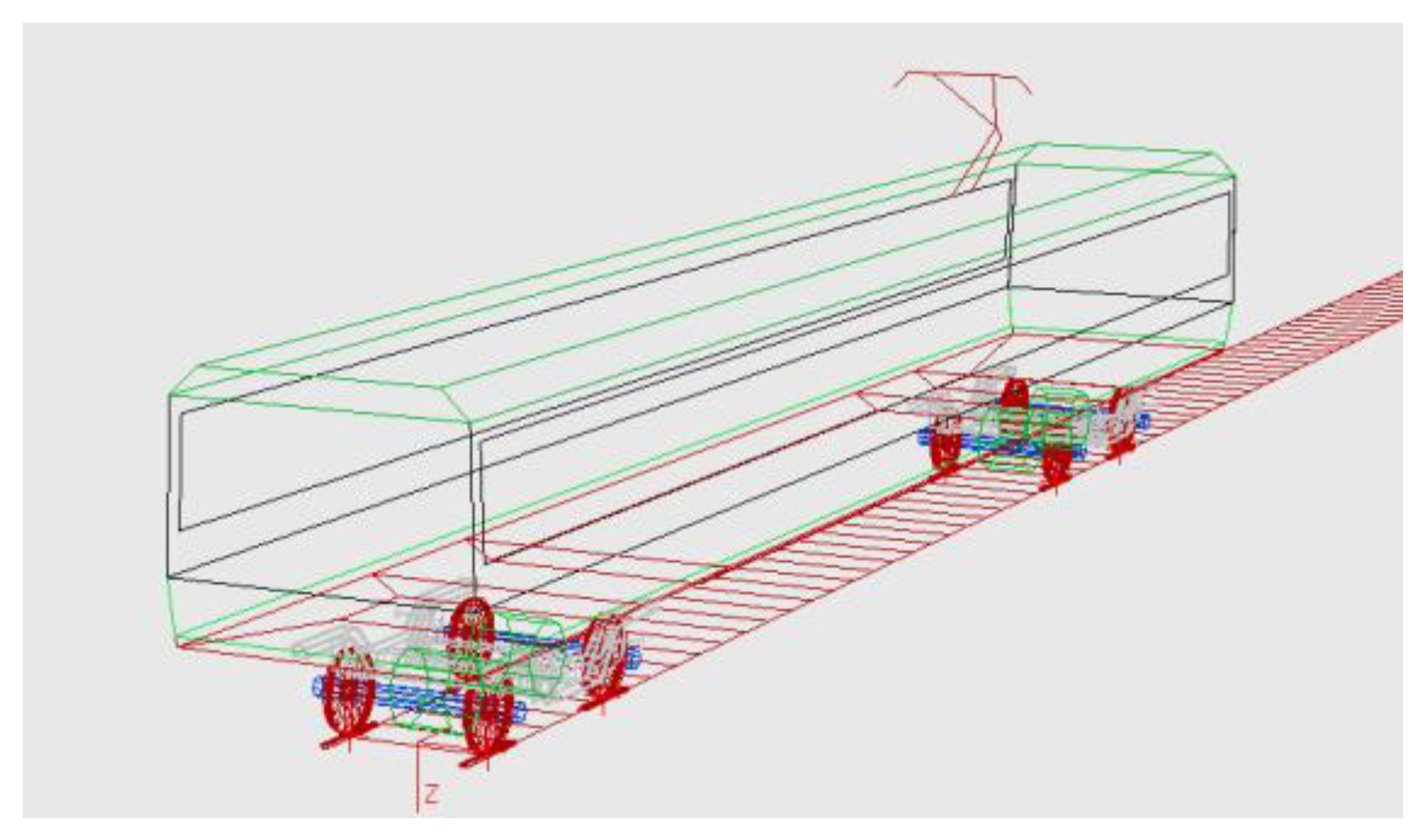
2.2. Dynamic Model
2.3. Distribution of Wheel–Rail Contact Points
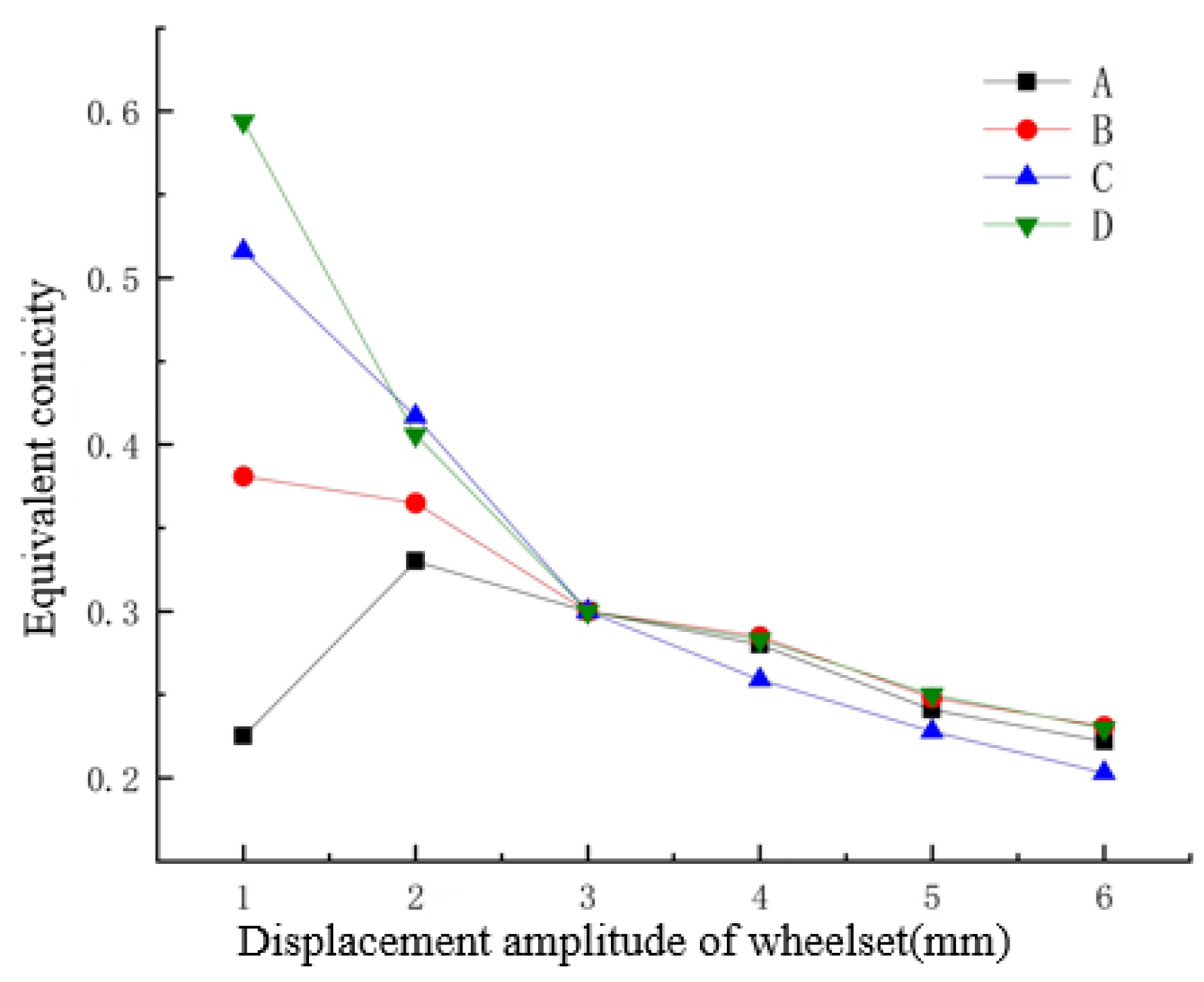
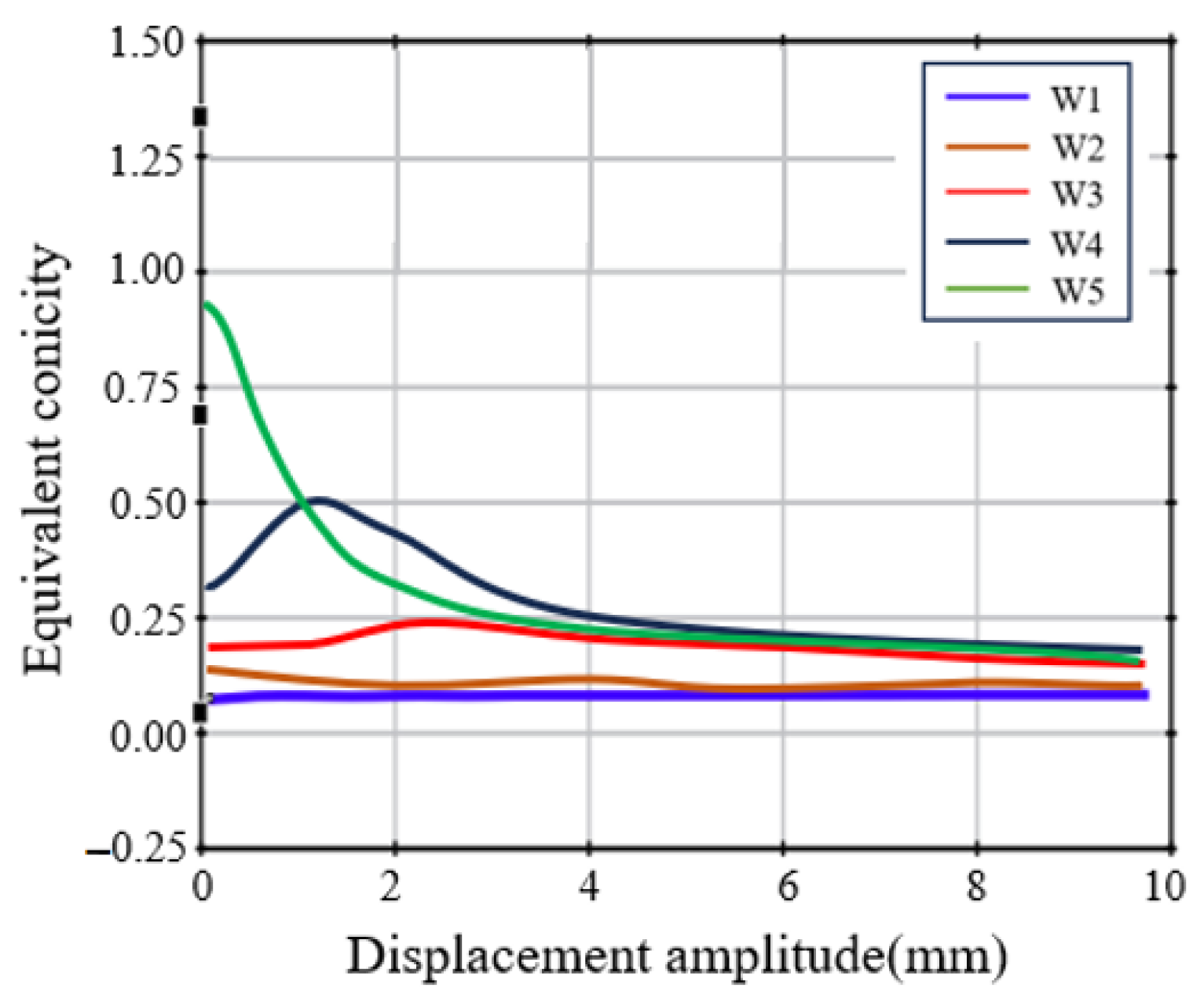
2.4. Nonlinear Characteristics and Revised Equivalent Conicity for Worn Wheels
3. Impact of Hollow Worn Wheel on Vehicle Stability
3.1. Evaluation of Vehicle Stability Using Velocity-Reducing Method
3.2. Evaluation of Vehicle Stability Using Bifurcation Configuration Method
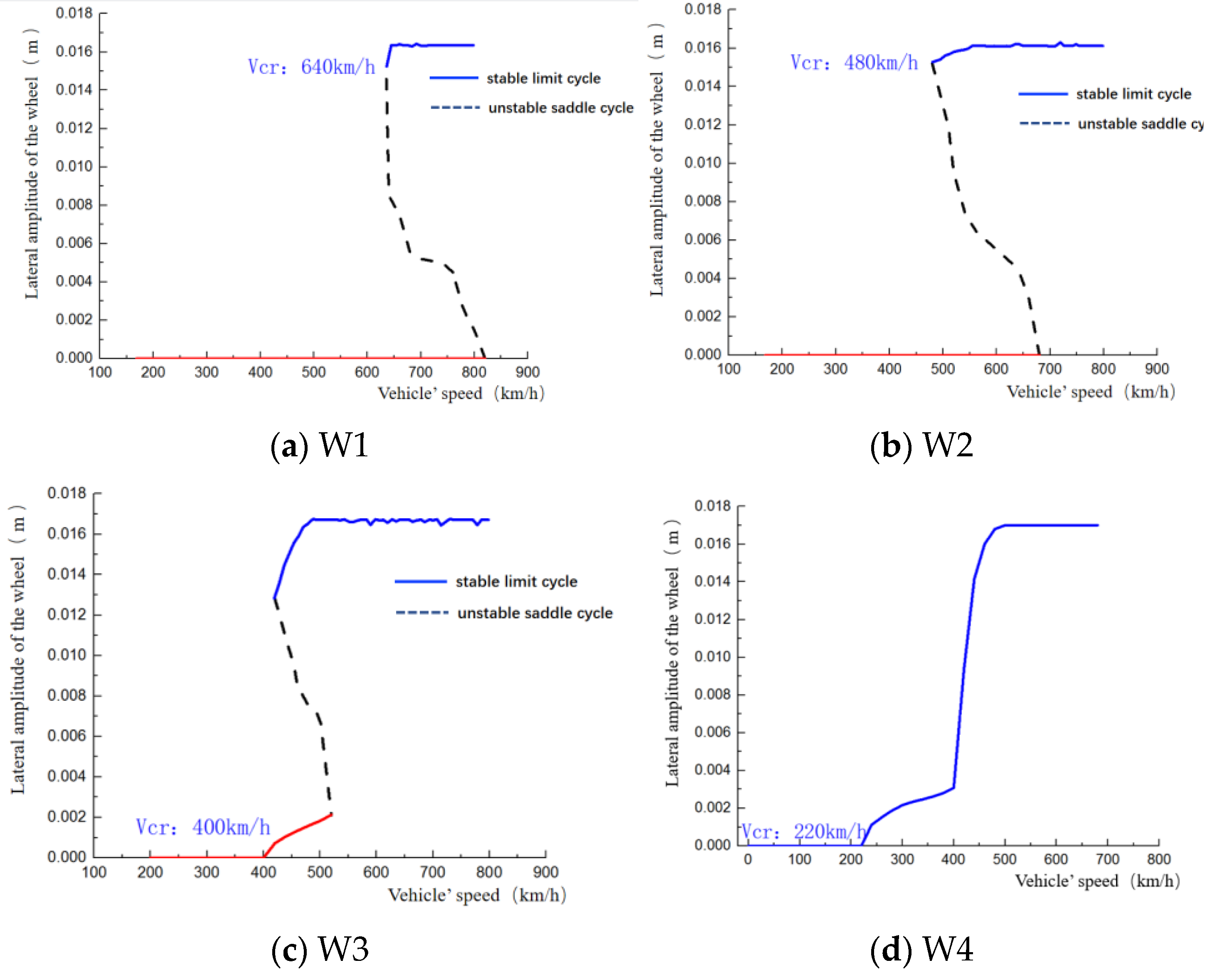
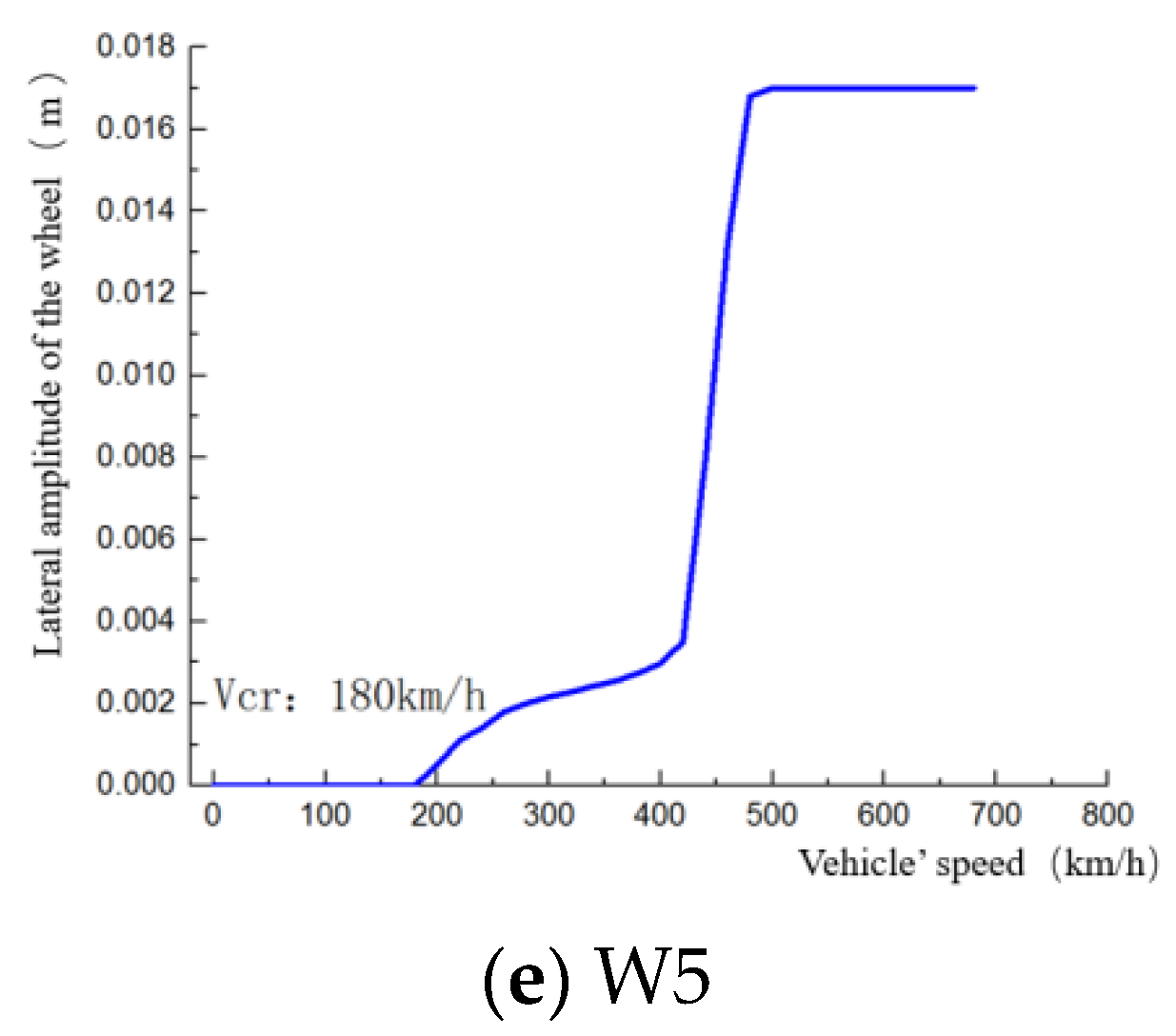
3.2.1. Critical Speed and Bifurcation Type of Vehicles
3.2.2. Analysis of Hopf Bifurcation Diagram for Wheelsets
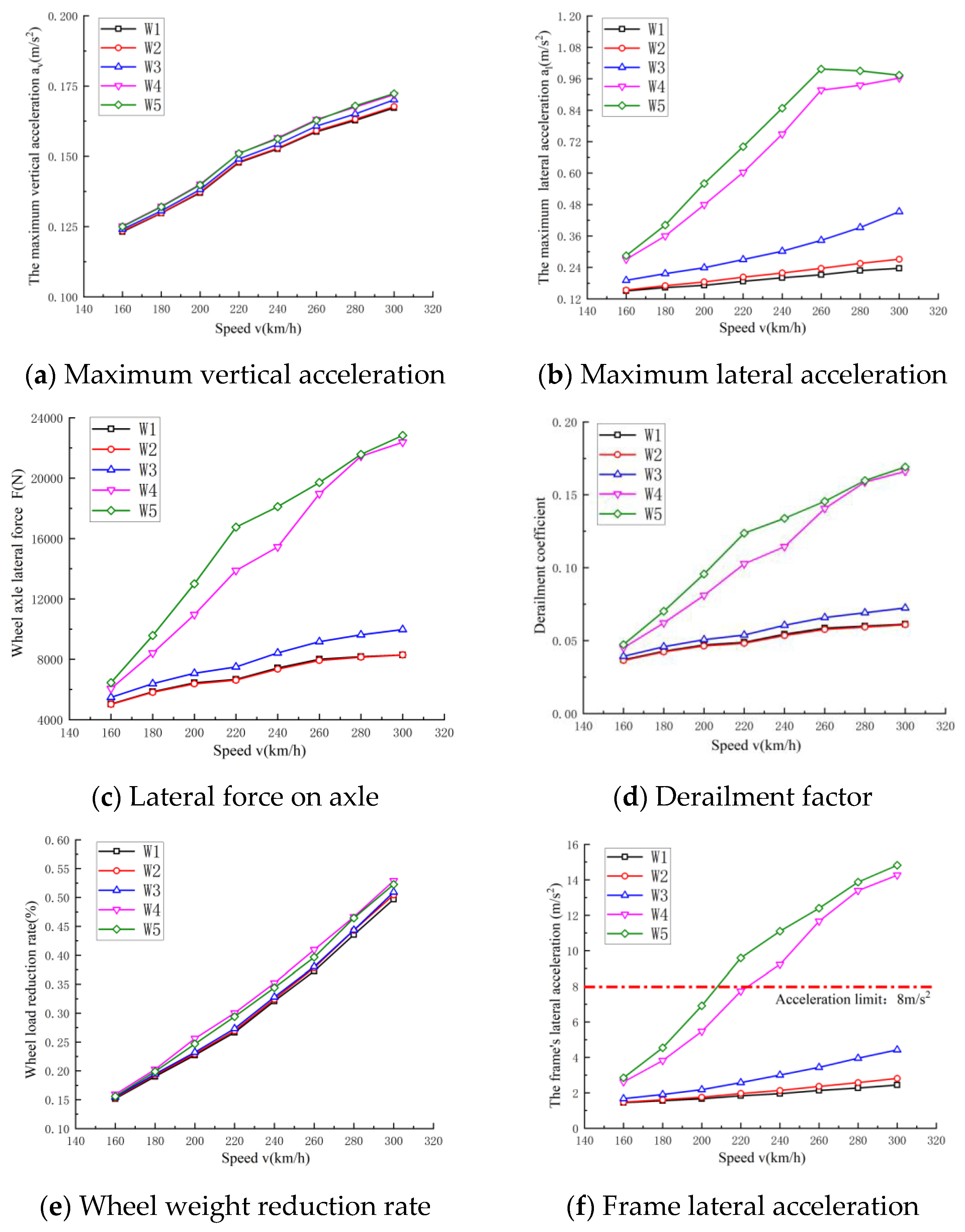
3.3. An Evaluation of Vehicle Stability Using the Lateral Vibration Acceleration Method
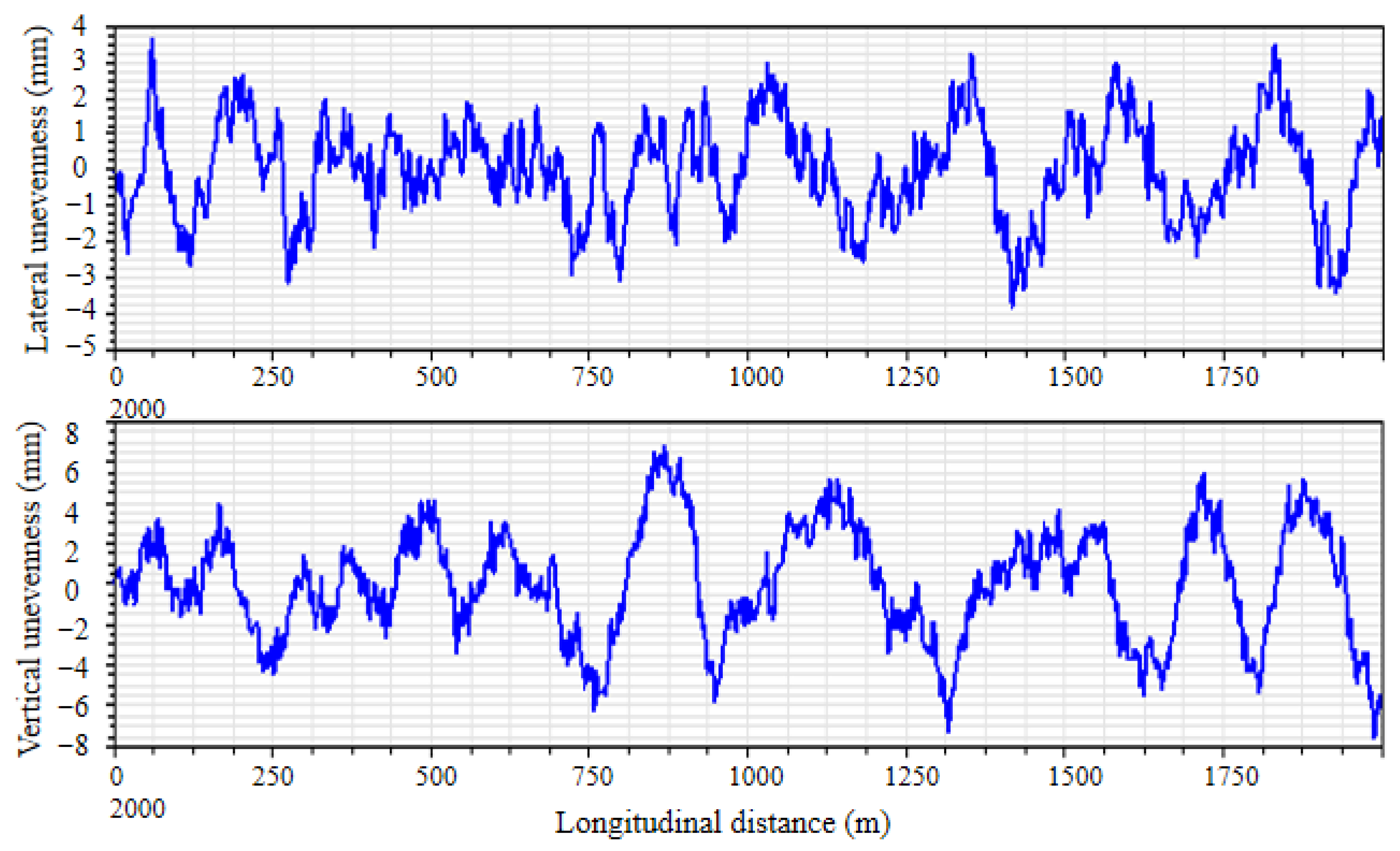
4. Experimental Analysis
5. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sawley, K.; Wu, H. The formation of hollow-worn wheels and their effect on wheel/rail interaction. Wear 2005, 258, 1179–1186. [Google Scholar]
- Sawley, K.; Urban, C.; Walker, R. The effect of hollow-worn wheels on vehicle stability in straight track. Wear 2005, 258, 1100–1108. [Google Scholar]
- Zhai, W.; Jin, X.; Wen, Z.; Zhao, X. Wear problems of high-speed wheel/rail systems: Observations, causes, and countermeasures in China. Appl. Mech. Rev. 2020, 72, 060801. [Google Scholar] [CrossRef]
- Tavakkoli, H.; Ghajar, R.; Alizadeh, K.J. The influence of tread hollowing of the railway wheels on the hunting of a coach. In Proceedings of the ASME 2010 International Mechanical Engineering Congress and Exposition, Vancouver, BC, Canada, 12–18 November 2010; Volume 11, pp. 895–900. [Google Scholar]
- Frohling, R.; Ekberg, A.; Kabo, E. The detrimental effects of hollow wear-field experiences and numerical simulations. Wear 2008, 265, 1283–1291. [Google Scholar]
- Alizadeh Kaklar, J.; Ghajar, R.; Tavakkoli, H. Modelling of nonlinear hunting instability for a high-speed railway vehicle equipped by hollow worn wheels. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2016, 230, 553–567. [Google Scholar]
- Zhai, W.; Liu, P.; Lin, J.; Wang, K. Experimental investigation on vibration behaviour of a CRH train at speed of 350 km/h. Int. J. Rail Transp. 2015, 3, 1–16. [Google Scholar]
- Li, W.; Guan, Q.; Chi, M.; Wen, Z.; Sun, J. An investigation into the influence of wheel-rail contact relationships on the carbody hunting stability of an electric locomotive. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2022, 236, 1198–1209. [Google Scholar]
- Sun, J.; Chi, M.; Cai, W.; Gao, H.; Liang, S. An investigation into evaluation methods for ride comfort of railway vehicles in the case of carbody hunting instability. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2021, 235, 586–597. [Google Scholar]
- Wei, W.; Yabuno, H. Subcritical Hopf and saddle-node bifurcations in hunting motion caused by cubic and quintic nonlinearities: Experimental identification of nonlinearities in a roller rig. Nonlinear Dyn. 2019, 98, 657–670. [Google Scholar] [CrossRef]
- Schupp, G. Bifurcation analysis of railway vehicles. Multibody Syst. Dyn. 2006, 15, 25–50. [Google Scholar]
- Polach, O. Application of nonlinear stability analysis in railway vehicle industry. Non-Smooth Probl. Veh. Syst. Dyn. 2008, 500, 15–27. [Google Scholar]
- Polach, O.; Kaiser, I. Comparison of methods analyzing bifurcation and hunting of complex rail vehicle models. J. Comput. Nonlinear Dyn. 2012, 7, 041005. [Google Scholar]
- Li, Y.; Huang, C.; Zeng, J.; Cao, H. Bifurcations in a simplified and smoothed model of the dynamics of a rolling wheelset. Nonlinear Dyn. 2023, 111, 2079–2092. [Google Scholar]
- Lei, C.; Wang, S.; Guo, B.; Ma, W.; Dong, L. Study on Low-frequency Lateral Swaying of 2B0 Locomotive. J. China Railw. Soc. 2019, 41, 42–49. [Google Scholar]
- Wang, C.; Luo, S.; Wu, P.; Xu, Z.; Ma, W.; Fang, W. Effect of Hollow Worn Tread of Electric Multiple Units on Vehicle Stability. J. Southwest Jiaotong Univ. 2021, 56, 84–91. [Google Scholar]
- Hou, M.; Chen, B.; Cheng, D. Study on the evolution of wheel wear and its impact on vehicle dynamics of high-speed trains. Coatings 2022, 12, 1333. [Google Scholar] [CrossRef]
- Cui, D.; Li, L.; Wang, H.; Wen, Z.; Xiong, J. High-speed EMU wheel re-profiling threshold for complex wear forms from dynamics viewpoint. Wear 2015, 338, 307–315. [Google Scholar]
- Wang, C.; Luo, S.; Xu, Z.; Ma, W.; Dong, X.; Wu, P. Simulation analysis and field test verification of lateral instability of high-speed EMU framework. J. China Railw. Soc. 2021, 43, 39–48. [Google Scholar]
- Polach, O. Characteristic parameters of nonlinear wheel/rail contact geometry. Veh. Syst. Dyn. 2010, 48 (Suppl. S1), 19–36. [Google Scholar]
- UIC Code 518; Testing and Approval of Railway Vehicles from the Point of View of Their Dynamic Behavior-Safety-Track Fatigue-Running Behavior. International Union of Railways (UIC): Paris, France, 2009.
- Zhang, T.; Dai, H. Bifurcation analysis of high-speed railway wheel-set. Nonlinear Dyn. 2016, 83, 1511–1528. [Google Scholar]
- True, H.; Kaas-Petersen, C. A bifurcation analysis of nonlinear oscillations in railway vehicles. Veh. Syst. Dyn. 2007, 12, 5–6. [Google Scholar]
- Zeng, J. Nonlinear oscillations and chaos in a railway vehicle system. Chin. J. Mech. Eng. 1998, 11, 231–238. [Google Scholar]
- GB/T 5599-2019; The Standardization Administration of the People’s Republic of China: GB/T 5599-2019 Specification for Locomotive and Vehicle Dynamic Performance Evaluation and Test Identification. Standards Press of China: Beijing, China, 2019.
- Ham, Y.; Lee, D.; Kwon, S.; You, W.; Oh, T. Continuous Measurement of Interaction Forces between Wheel and Rail. Int. J. Precis. Eng. Manuf. 2009, 10, 35–39. [Google Scholar]
- Zhu, T.; Wang, X.; Wu, J.; Zhang, J.; Xiao, S.; Lu, L.; Yang, B.; Yang, G. Comprehensive identification of wheel-rail forces for rail vehicles based on the time domain and machine learning methods. Mech. Syst. Signal Process. 2025, 222, 111635. [Google Scholar]
- Gong, D.; Liu, G.; Zhou, J. Research on mechanism and control methods of carbody chattering of an electric multiple-unit train. Multibody Syst. Dyn. 2021, 53, 135–172. [Google Scholar]


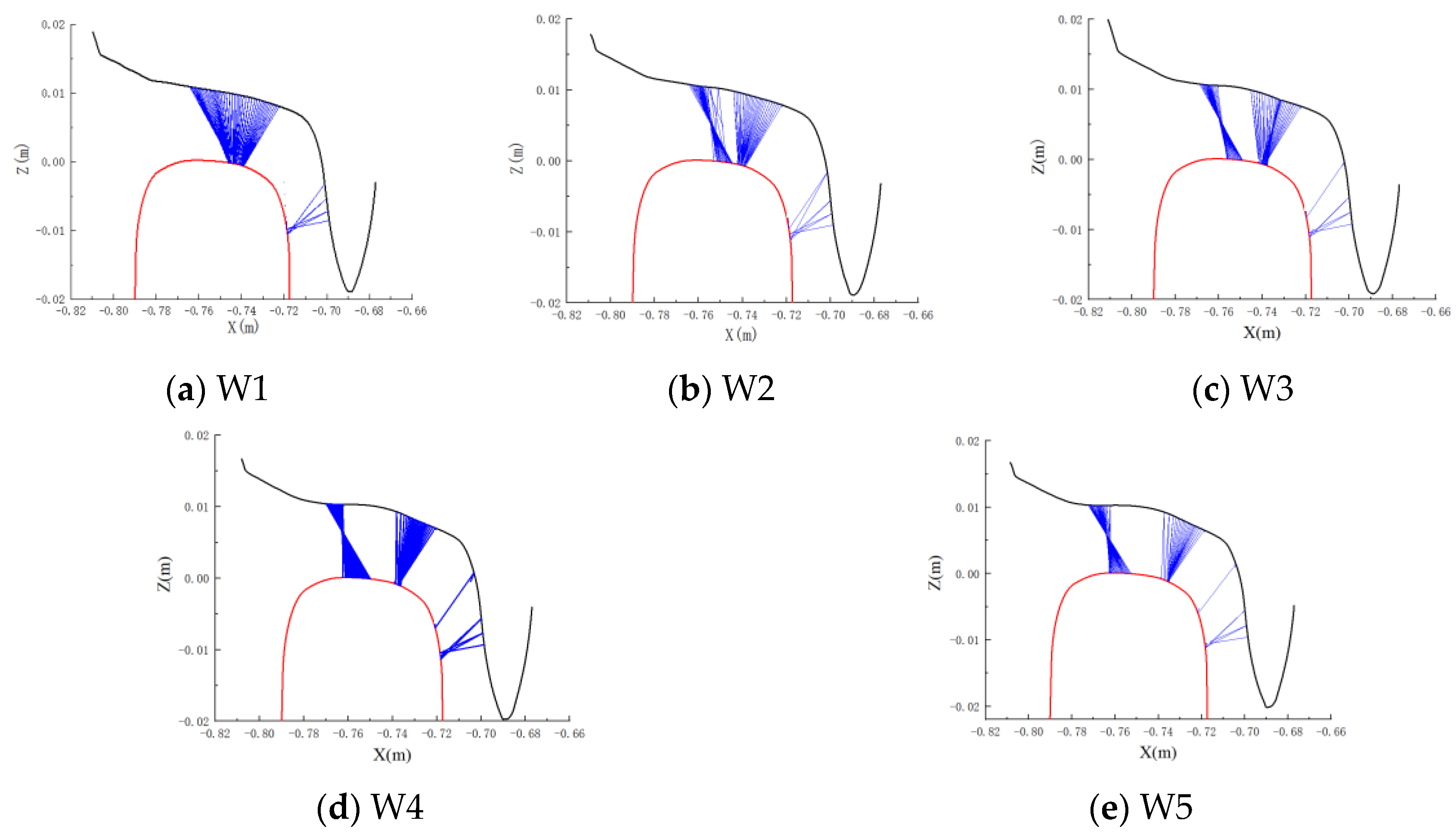


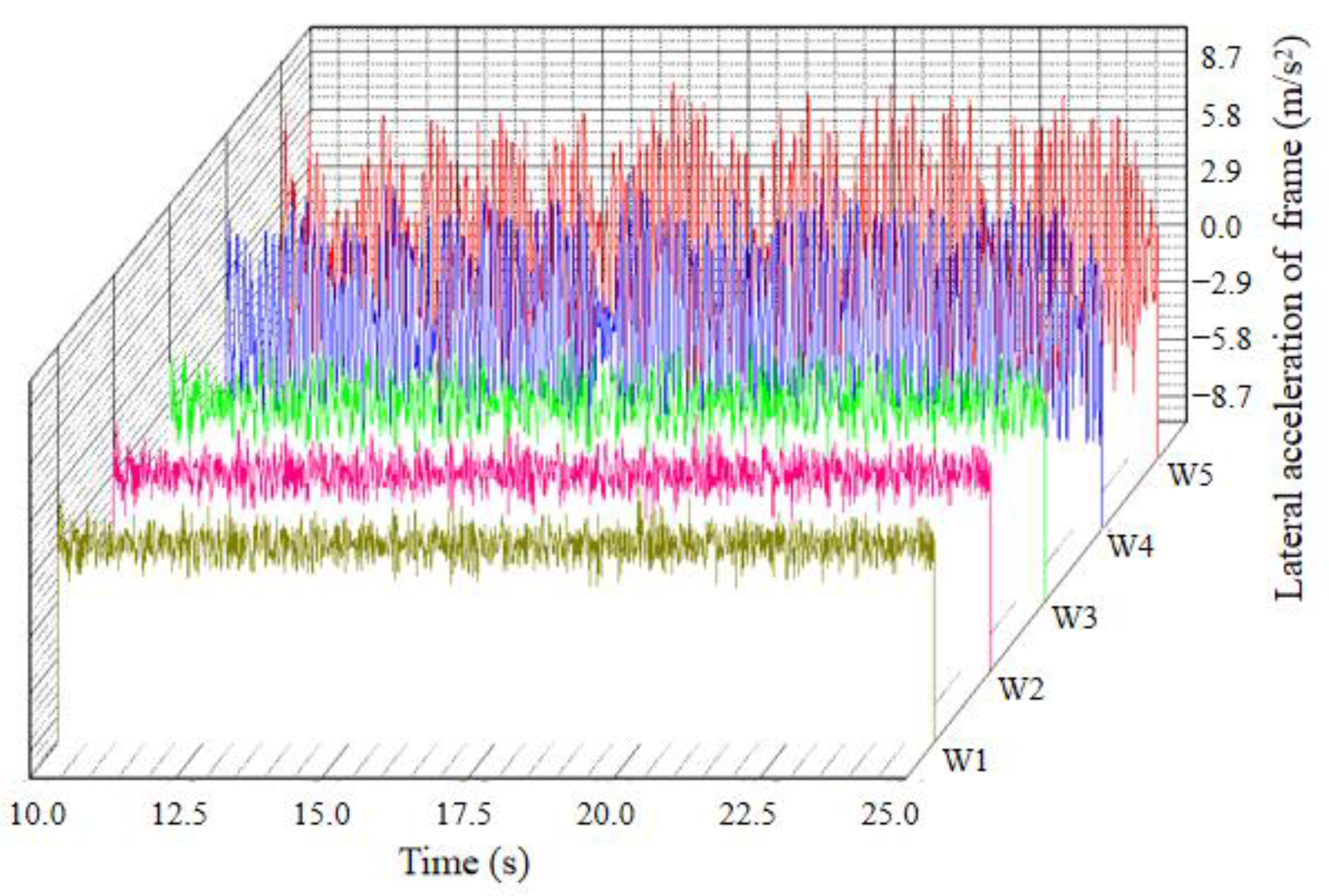

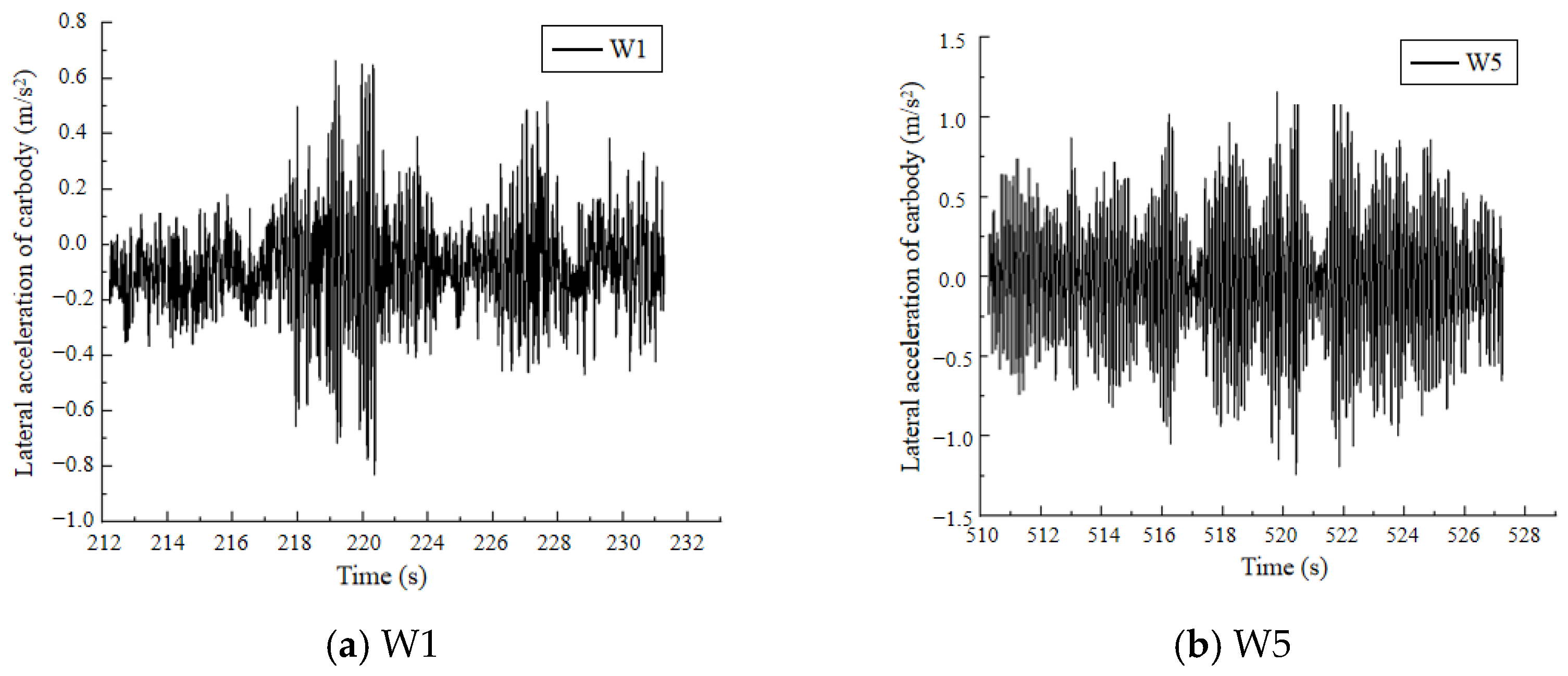

| Wheel Number | W1 | W2 | W3 | W4 | W5 |
|---|---|---|---|---|---|
| Running mileage (×104 km) | 0 | 4.5 | 9.5 | 18.5 | 26 |
| Wear width (mm) | 0 | 32 | 40 | 46 | 53 |
| Wear depth (mm) | 0 | 0.32 | 0.61 | 0.96 | 1.26 |
| Depth of hollow wear (mm) | 0 | 0.17 | 0.31 | 0.52 | 0.64 |
| Component | Degree of Freedom | |||||
|---|---|---|---|---|---|---|
| Longitudinal | Lateral | Vertical | Pitch | Yaw | Roll | |
| Carbody | ||||||
| Bogie | ||||||
| Wheelset | ||||||
| Axle box | ||||||
| Parameter Type | Parameter | Numeric Value | Unit |
|---|---|---|---|
| Vehicle parameters | Distance between front and rear bogies | 19 | m |
| Wheelbase | 2.7 | m | |
| Diameter of the wheel | 920 | mm | |
| Quality parameters | Carbody mass | 42 | t |
| Bogie mass | 2.7 | t | |
| Wheelset mass | 1.7 | t | |
| Axle box mass | 0.099 | t | |
| Suspension parameters | Vertical stiffness of primary spring | 1.2 | MN/m |
| Longitudinal stiffness of primary spring | 13.7 | MN/m | |
| Lateral stiffness of primary spring | 4.99 | MN/m | |
| Vertical stiffness of secondary spring | 0.26 | MN/m | |
| Vertical damping of secondary spring | 20 | kN·s/m | |
| Lateral damping of secondary spring | 22 | kN·s/m | |
| Damping of anti-hunting absorber | 26 | kN·s/m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Hu, J.; Wang, C.; Liu, Z. The Impact of Hollow Wear on the Stability of High-Speed Railway Vehicles. Appl. Sci. 2025, 15, 4060. https://doi.org/10.3390/app15074060
Zhang L, Hu J, Wang C, Liu Z. The Impact of Hollow Wear on the Stability of High-Speed Railway Vehicles. Applied Sciences. 2025; 15(7):4060. https://doi.org/10.3390/app15074060
Chicago/Turabian StyleZhang, Ling, Junping Hu, Chen Wang, and Zechao Liu. 2025. "The Impact of Hollow Wear on the Stability of High-Speed Railway Vehicles" Applied Sciences 15, no. 7: 4060. https://doi.org/10.3390/app15074060
APA StyleZhang, L., Hu, J., Wang, C., & Liu, Z. (2025). The Impact of Hollow Wear on the Stability of High-Speed Railway Vehicles. Applied Sciences, 15(7), 4060. https://doi.org/10.3390/app15074060






