Dynamic Characteristics on Single-Tooth Rock Cutting Considering the Change of Extrusion Zone Height
Abstract
:1. Introduction
2. Construction of New Dynamic Rock-Cutting Model and Analysis of Cutting Periodicity
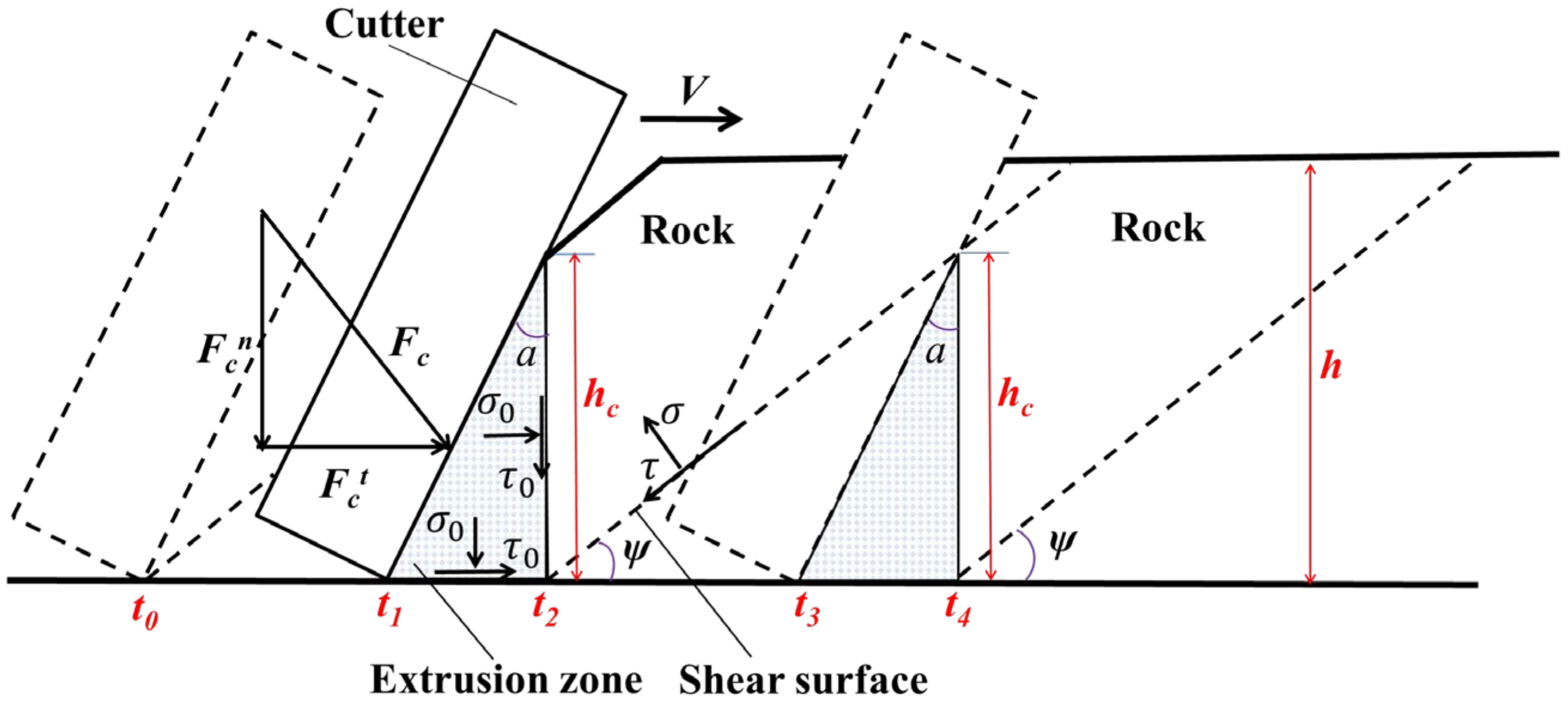
2.1. Construction of a New Dynamic Cutting Model
2.2. The Relationship Between the Variation Characteristics of Tangential Cutting Force Fct and the Dynamic Rock-Cutting Process
- To simplify the problem, the cutter is considered a rigid body, without considering its wear and thermal effects.
- Except for the rock debris forming the crushing zone, the remaining debris can be cleared promptly and will not affect the cutting due to accumulation in front of the cutter.
- To avoid the influence of uneven distribution of rock components on the cutting force, the rock is considered an isotropic, homogeneous material.
- In the analysis, the problem is simplified to a two-dimensional orthogonal cutting model.
- The effects of temperature and humidity on the cutting process are not considered.
3. Numerical Simulation of Single-Tooth Rock Cutting
3.1. Constitutive Model of Rock
3.2. Geometric Model and Material Parameters
- (1)
- Since the hardness of the tool is much greater than that of the rock, the tool is rigidly constrained;
- (2)
- The rock is considered an isotropic, homogeneous material;
- (3)
- The effects of temperature and thermo-mechanical coupling on cutting are not considered;
- (4)
- The rock mass element is deleted in real time upon failure and no longer participates in subsequent calculations;
- (5)
- The size of the cutting tooth is much larger than the cutting depth. Therefore, rock cutting is treated as a plane strain problem, and a two-dimensional model is used for numerical simulation.
3.3. Boundary Conditions and Solver Settings
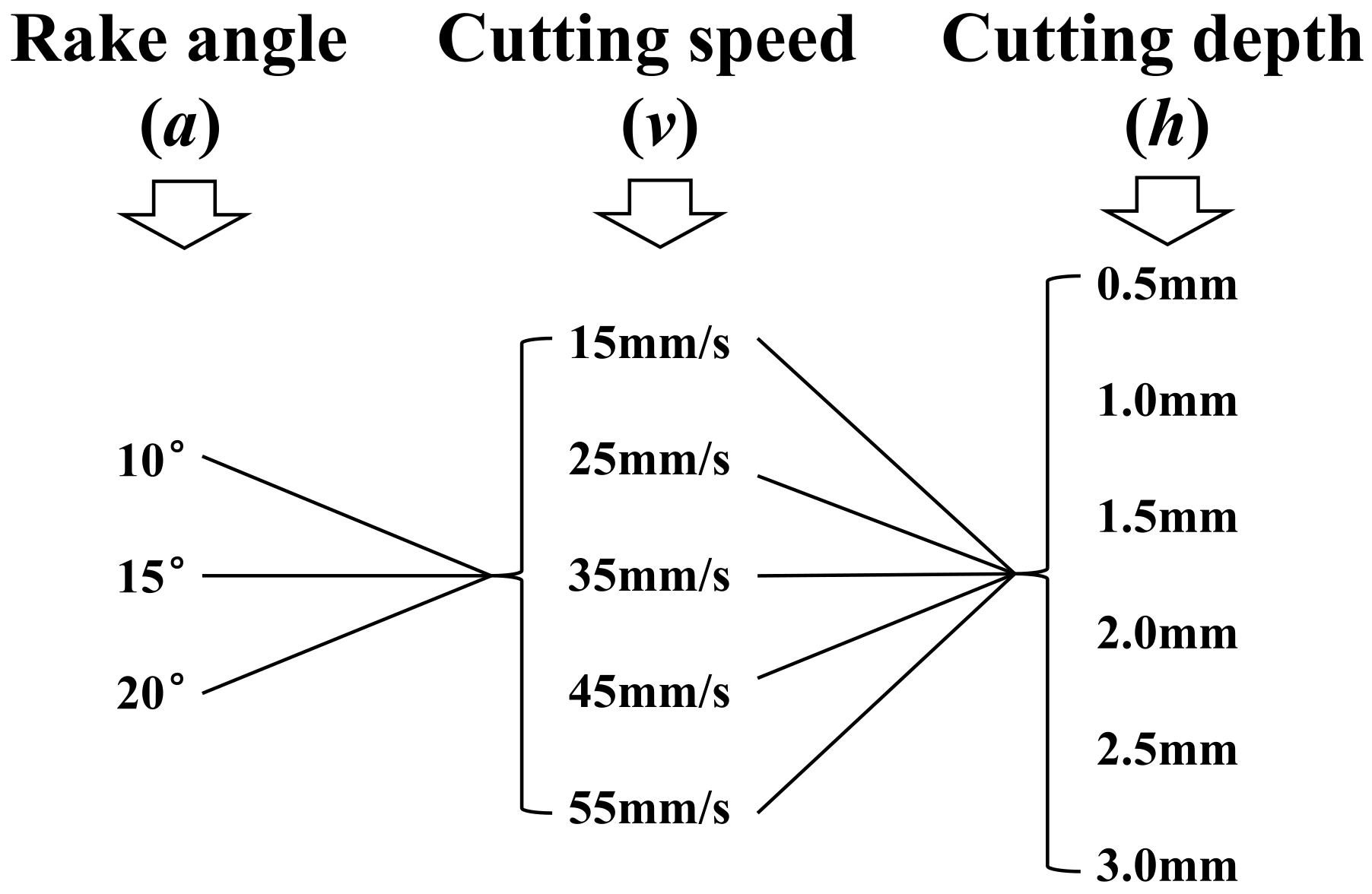
3.4. Scheme Setting
3.5. Model Validation
4. Result
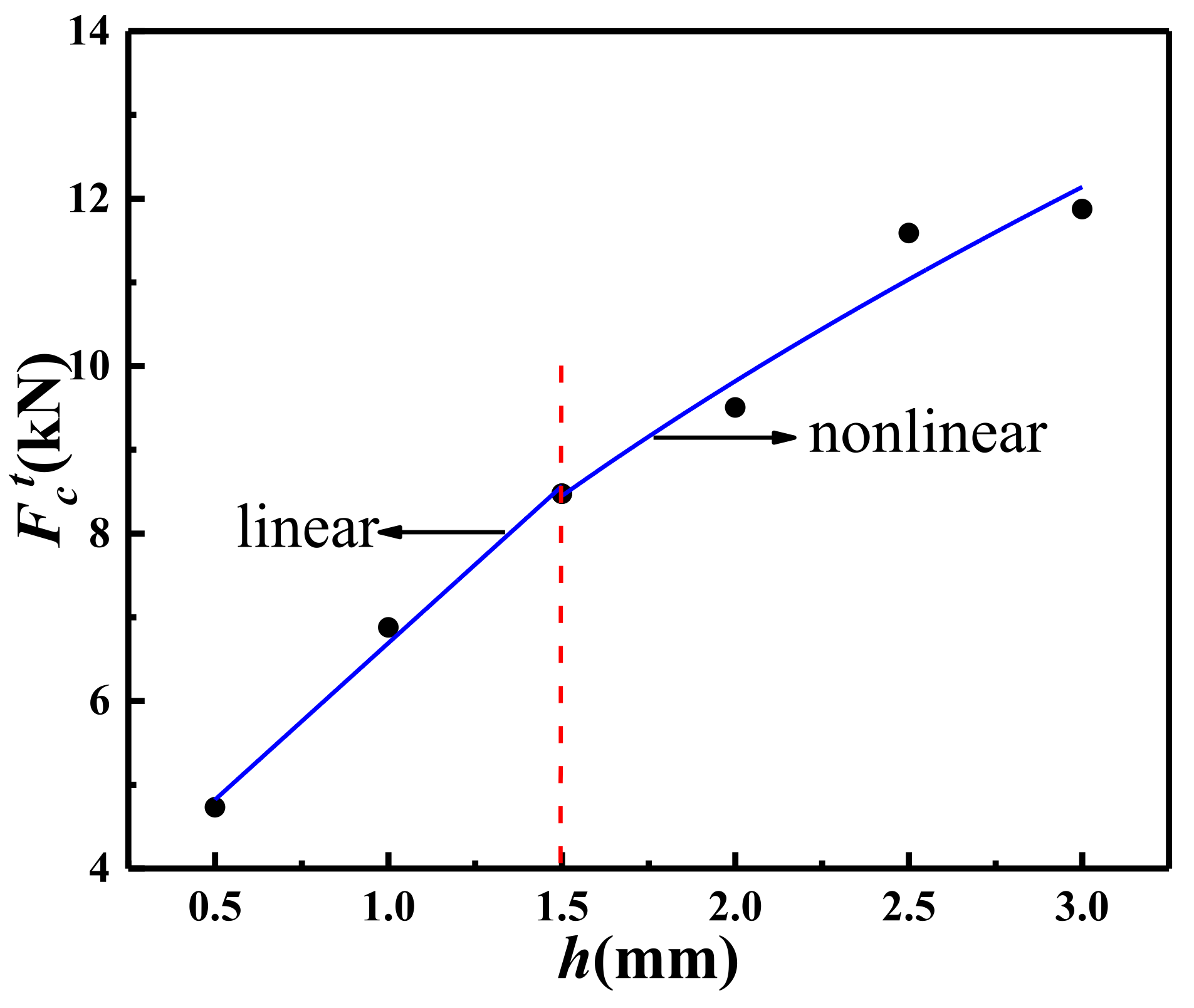
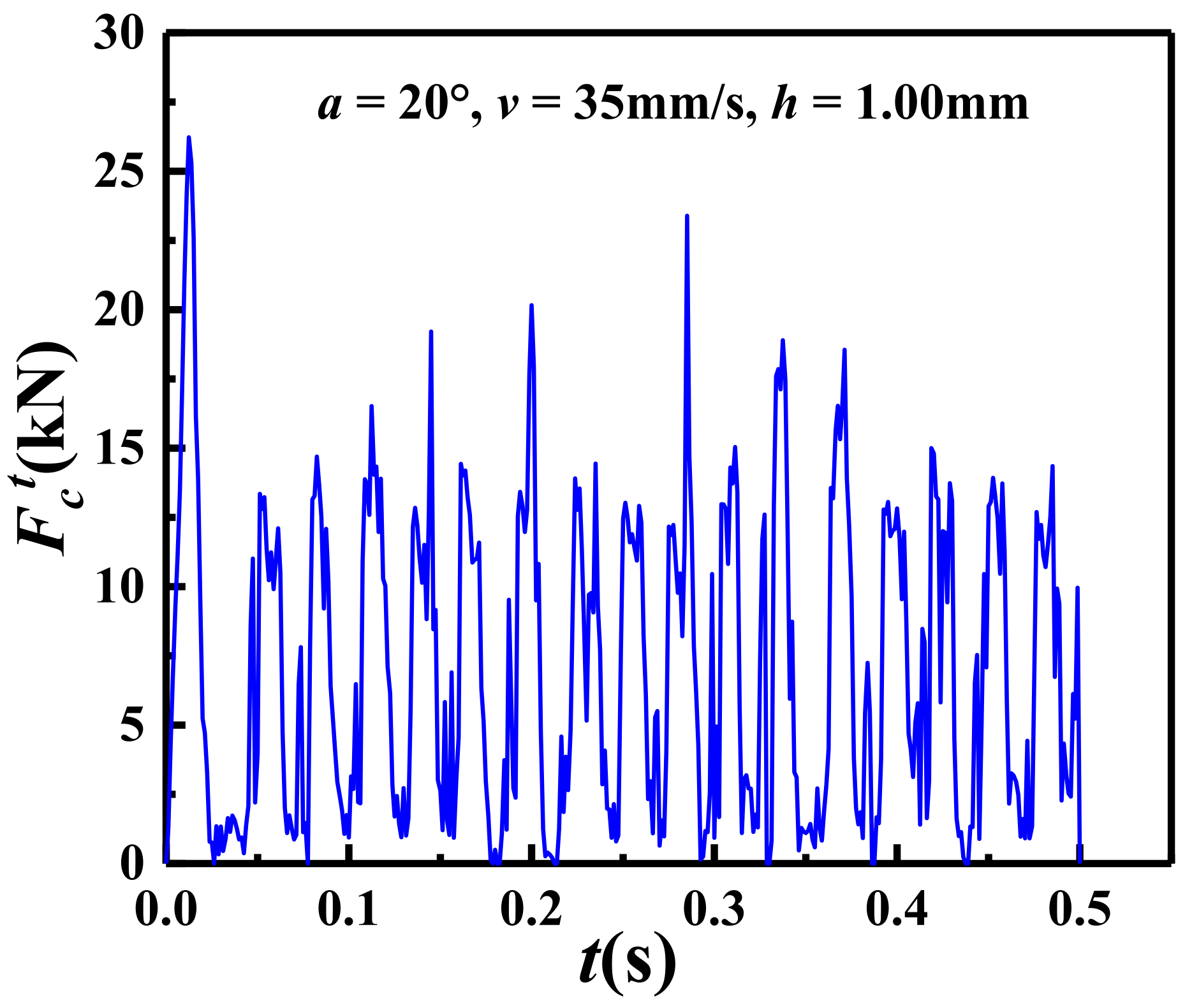
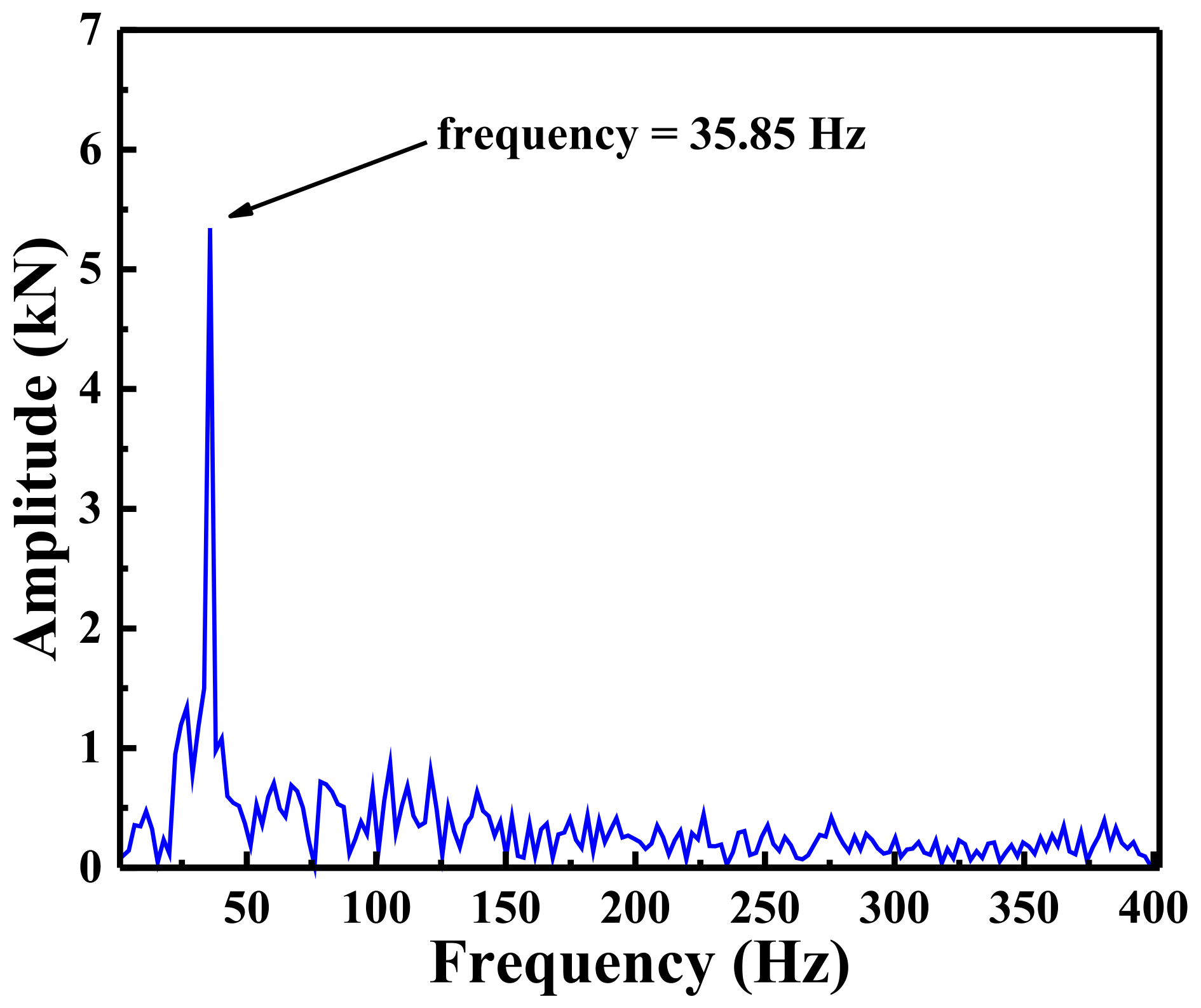
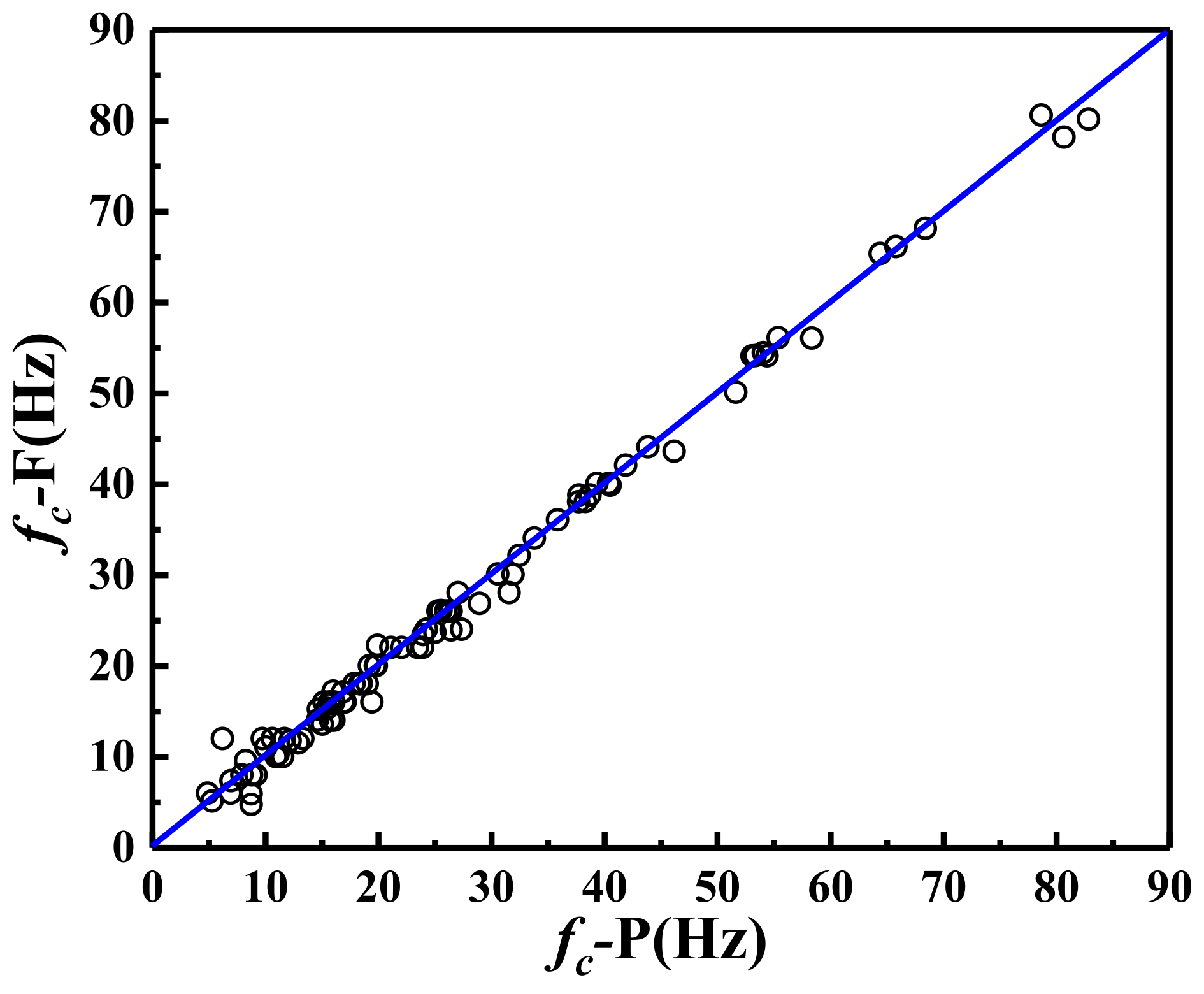
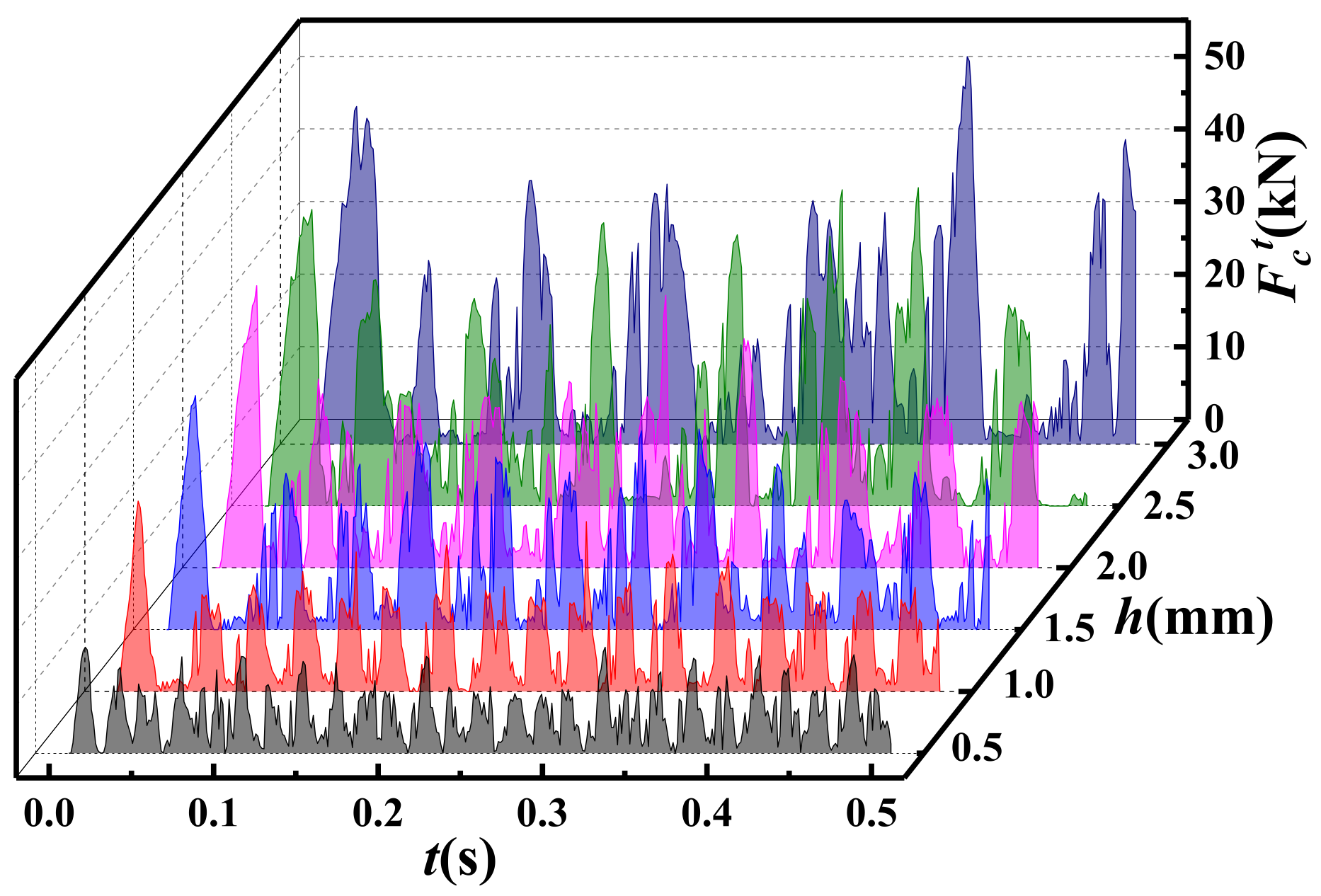
4.1. Verification of Tangential Force Periodicity and Cutting Frequency Extraction Method
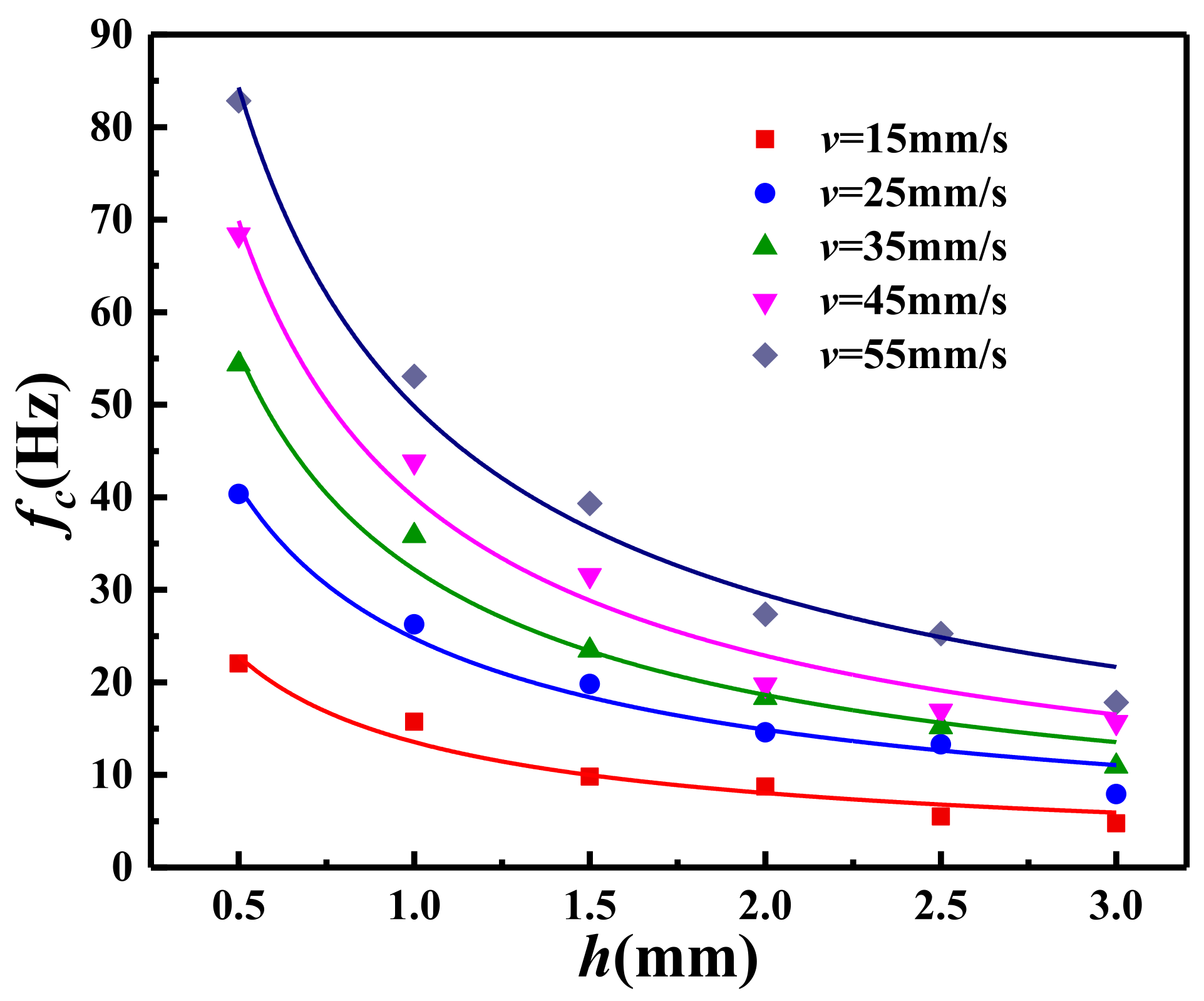
4.2. The Influence of Cutting Parameters on Cutting Frequency
4.2.1. The Influence of Rake Angle on Cutting Frequency
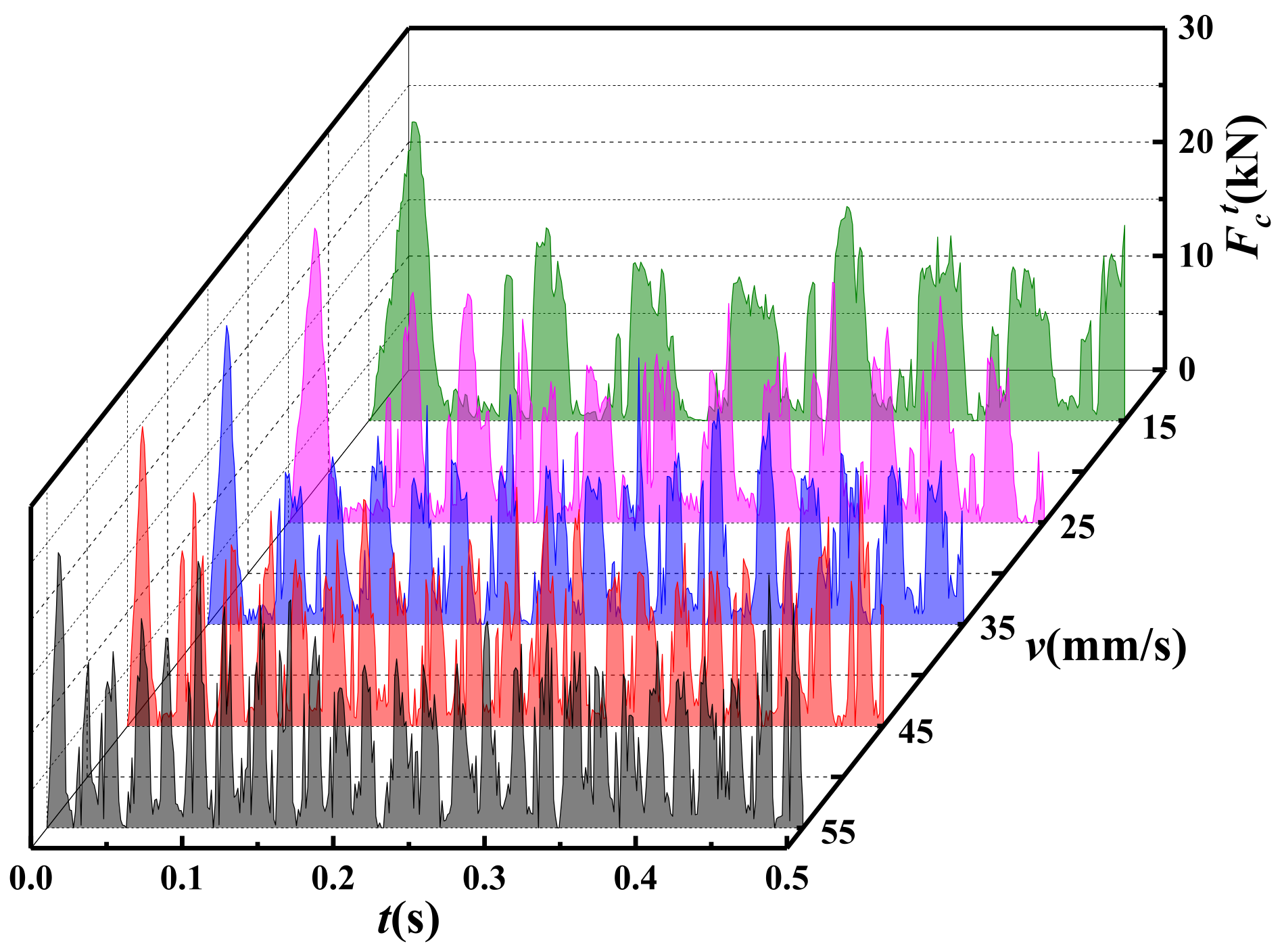
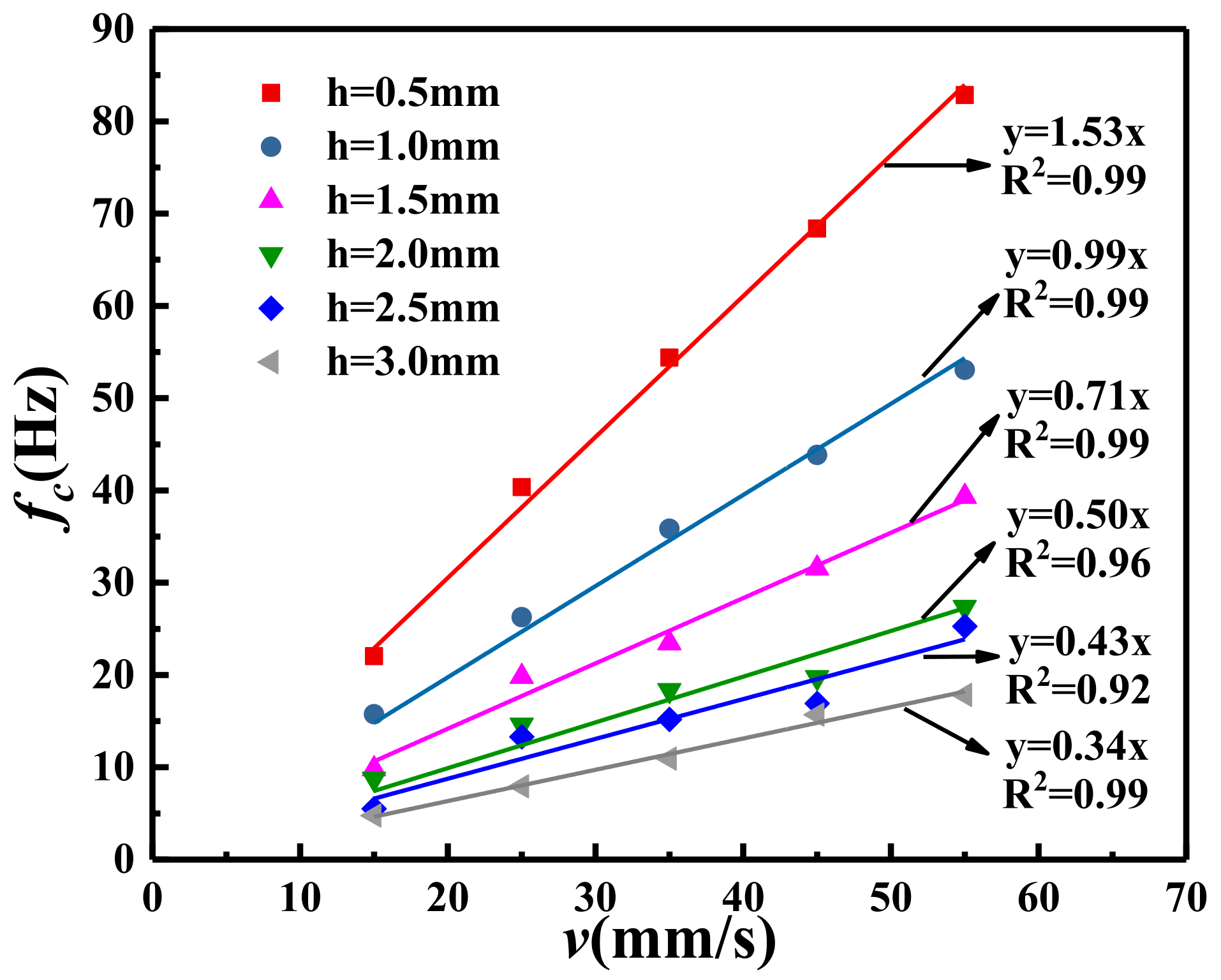
4.2.2. The Influence of Cutting Speed v on Cutting Frequency
4.2.3. The Influence of Cutting Depth H on Cutting Frequency
5. Discussion
5.1. The Influence Mechanism of Cutting Parameters on Cutting Frequency
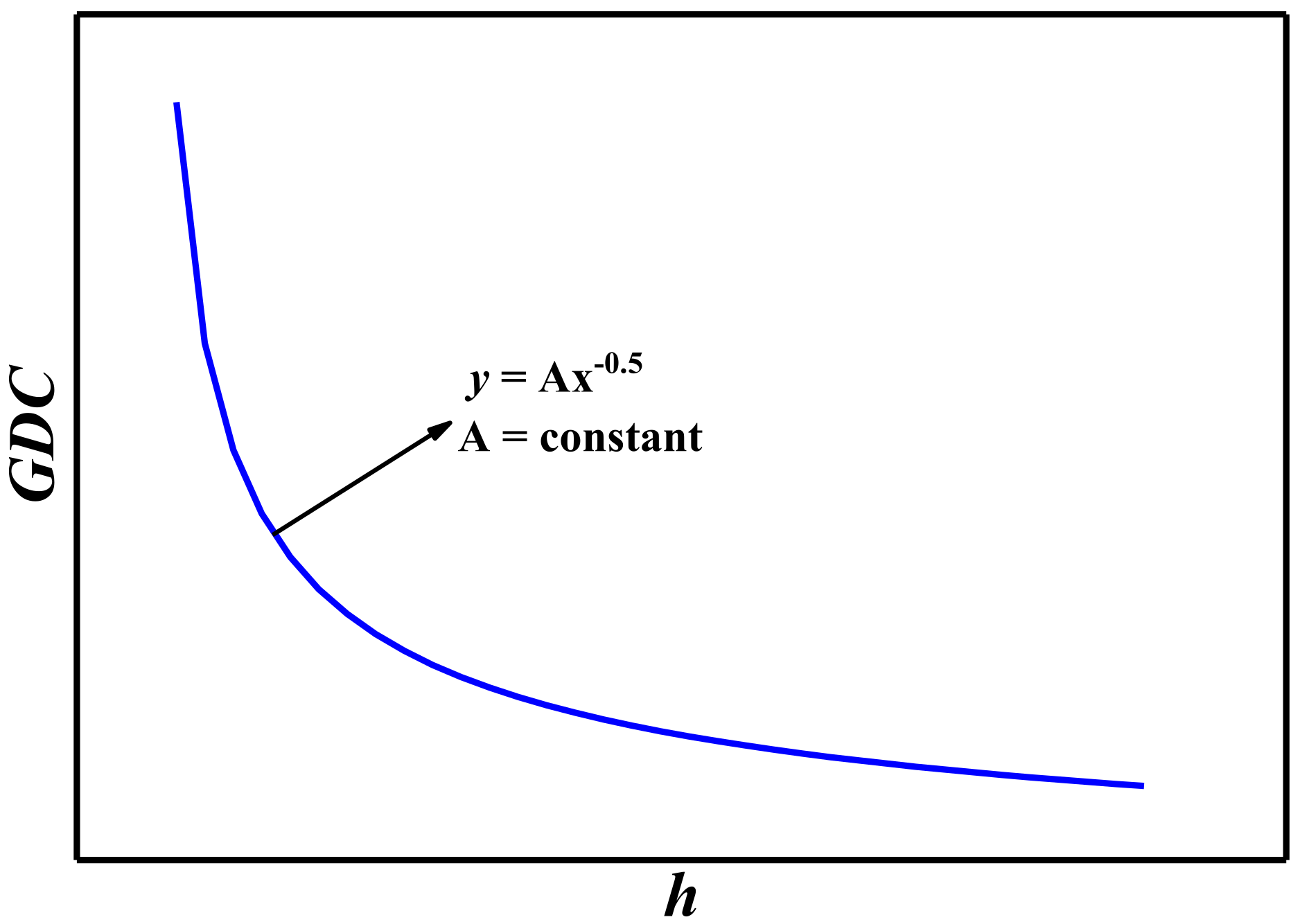
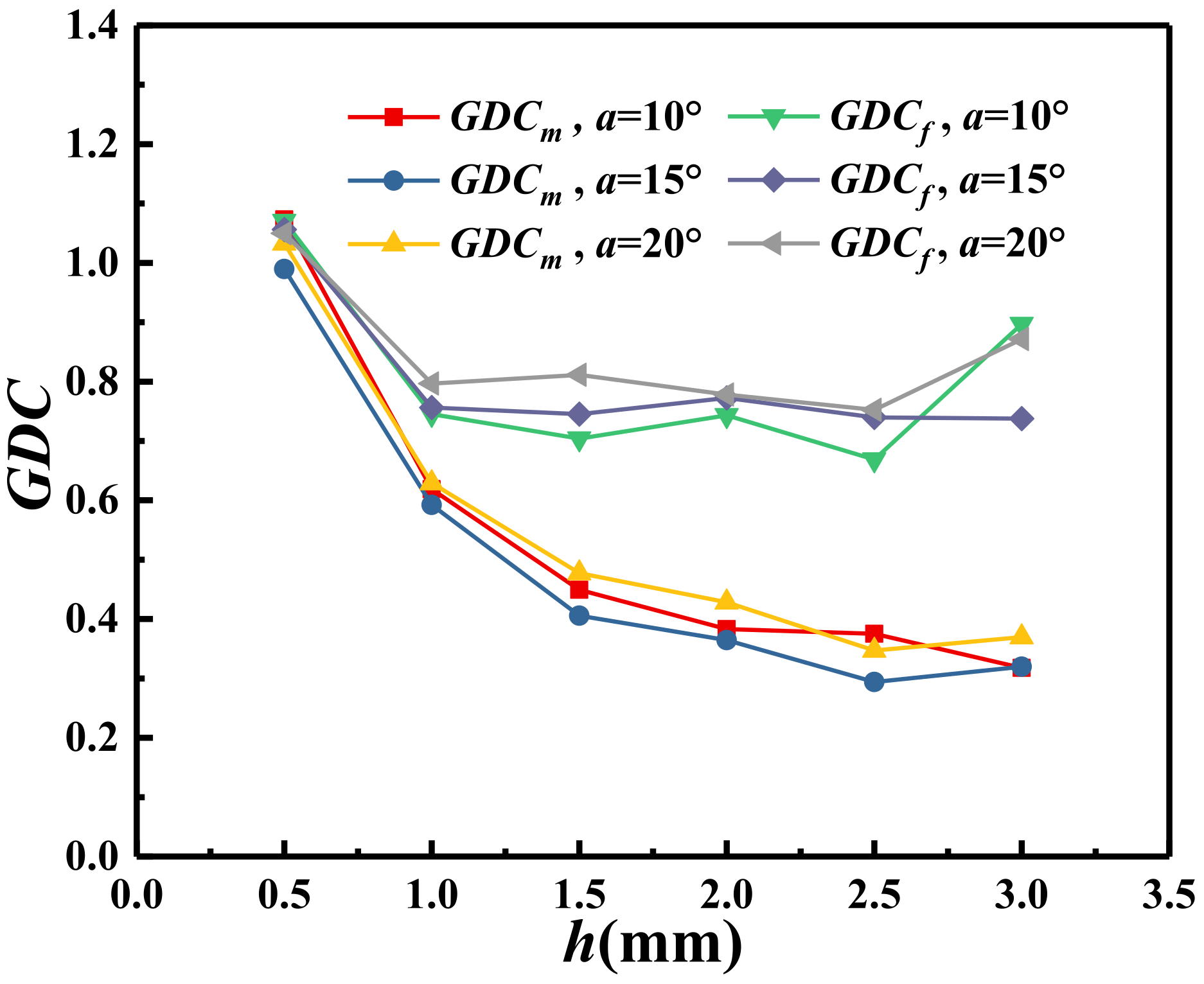
5.2. The Relationship Between the Height of Extrusion Zone and Cutting Depth
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bruton, G.; Crockett, R.; Taylor, M.; DenBoer, D.; Lund, J.; Fleming, C.; Ford, R.; Garcia, G.; White, A. PDC bit technology for the 21st century. Oilfield Rev. 2014, 26, 48–57. [Google Scholar]
- Cutting Experiments in Sandstones with Blunt PDC Cutters: Almenara, J R; Detournay, E Proc ISRM Symposium: Eurock’92, Chester, 14–17 September 1992P215–220. Publ London: Thomas Telford, 1992. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, A242. [CrossRef]
- Akbari, B.; Miska, S. The Effects of Chamfer and Back Rake Angle on PDC Cutters Friction. J. Nat. Gas Sci. Eng. 2016, 35, 347–353. [Google Scholar] [CrossRef]
- Yahiaoui, M.; Paris, J.-Y.; Delbé, K.; Denape, J.; Gerbaud, L.; Colin, C.; Ther, O.; Dourfaye, A. Quality and Wear Behavior of Graded Polycrystalline Diamond Compact Cutters. Int. J. Refract. Met. Hard Mater. 2016, 56, 87–95. [Google Scholar] [CrossRef]
- Rostamsowlat, I. Effect of Cutting Tool Properties and Depth of Cut in Rock Cutting: An Experimental Study. Rock Mech. Rock Eng. 2018, 51, 1715–1728. [Google Scholar] [CrossRef]
- Detournay, E.; Defourny, P. A Phenomenological Model for the Drilling Action of Drag Bits. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1992, 29, 13–23. [Google Scholar] [CrossRef]
- Fu, X.-K.; Xiong, C.; Shi, H.-Z.; He, W.-H.; Wang, L.-H.; Huang, Z.-W. Comparative Analysis of Rock Breakage Characteristics and Failure Mode on Conventional and Conical PDC Cutter Cutting Carbonate. Pet. Sci. 2025, 22, 821–834. [Google Scholar] [CrossRef]
- Wang, H.; He, M.; Ding, M.; Wang, J.; Hao, W.; Yu, X. Determination of Elastic Modulus and Anisotropy for Rocks Using Digital Drilling Method. Geoenergy Sci. Eng. 2024, 243, 213373. [Google Scholar] [CrossRef]
- Cheatham, J.B., Jr.; Daniels, W.H. A Study of Factors Influencing the Drillability of Shales: Single-Cutter Experiments With STRATAPAX(T) Drill Blanks. J. Energy Resour. Technol. 1979, 101, 189–195. [Google Scholar] [CrossRef]
- Wei, J.; Liu, W.; Gao, D. Modeling of PDC Bit-Rock Interaction Behaviors Based on the Analysis of Dynamic Rock-Cutting Process. Geoenergy Sci. Eng. 2024, 239, 212955. [Google Scholar] [CrossRef]
- Cheng, Z.; Sheng, M.; Li, G.; Huang, Z.; Wu, X.; Zhu, Z.; Yang, J. Imaging the Formation Process of Cuttings: Characteristics of Cuttings and Mechanical Specific Energy in Single PDC Cutter Tests. J. Pet. Sci. Eng. 2018, 171, 854–862. [Google Scholar] [CrossRef]
- Liu, W.; Deng, H.; Zhu, X.; Deng, K. The PDC Cutter-Rock Interaction Behavior in Rock Cutting: A Review. Geoenergy Sci. Eng. 2023, 229, 212168. [Google Scholar] [CrossRef]
- Che, D.; Zhang, W.; Ehmann, K. Chip Formation and Force Responses in Linear Rock Cutting: An Experimental Study. J. Manuf. Sci. Eng. 2016, 139, 011011. [Google Scholar] [CrossRef]
- Liu, W.; Luo, Y.; Zhu, X.; Yang, F. The Ductile–Brittle Failure Mode Transition of Hard Brittle Rock Cutting—New Insights from Numerical Simulation. Geomech. Geophys. Geo-Energy Geo-Resour. 2022, 8, 129. [Google Scholar] [CrossRef]
- Kadkhodaei, M.H.; Ghasemi, E.; Hamidi, J.K.; Rostami, J. Evaluation of Fine Material and Chip Formation in Rock Cutting with a Conical Tool. Bull. Eng. Geol. Environ. 2024, 83, 276. [Google Scholar] [CrossRef]
- Dai, X.; Huang, Z.; Shi, H.; Wu, X.; Xiong, C. Cutting Force as an Index to Identify the Ductile-Brittle Failure Modes in Rock Cutting. Int. J. Rock Mech. Min. Sci. 2021, 146, 104834. [Google Scholar] [CrossRef]
- PDC Cutter-Rock Interaction—Experiments and Modeling|Semantic Scholar. Available online: https://www.semanticscholar.org/paper/PDC-cutter-rock-interaction-experiments-and-Akbari/668c150bd3cfc2f2d9cb7cfd2955fb37f4e31c17 (accessed on 6 January 2025).
- Dougherty, P.S.M.; Mpagazehe, J.; Shelton, J.; Higgs III, C.F. Elucidating PDC Rock Cutting Behavior in Dry and Aqueous Conditions Using Tribometry. J. Pet. Sci. Eng. 2015, 133, 529–542. [Google Scholar] [CrossRef]
- Glowka, D.A. Use of Single-Cutter Data in the Analysis of PDC Bit Designs: Part 1—Development of a PDC Cutting Force Model. J. Pet. Technol. 1989, 41, 797–849. [Google Scholar] [CrossRef]
- Ziaja, M.B. Mathematical Model of the Polycrystalline Diamond Bit Drilling Process and Its Practical Application; OnePetro: Richardson, TX, USA, 1985. [Google Scholar]
- Richard, T.; Dagrain, F.; Poyol, E.; Detournay, E. Rock Strength Determination from Scratch Tests. Eng. Geol. 2012, 147–148, 91–100. [Google Scholar] [CrossRef]
- Kalantari, S.; Baghbanan, A.; Hashemalhosseini, H. An Analytical Model for Estimating Rock Strength Parameters from Small-Scale Drilling Data. J. Rock Mech. Geotech. Eng. 2019, 11, 135–145. [Google Scholar] [CrossRef]
- Evans, I. A Theory of the Basic Mechanics of Coal Ploughing. In Mining Research; Clark, G.B., Ed.; Pergamon: Oxford, UK, 1962; pp. 761–798. ISBN 978-1-4832-8307-4. [Google Scholar]
- Nishimatsu, Y. The Mechanics of Rock Cutting. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1972, 9, 261–270. [Google Scholar] [CrossRef]
- Li, Z.; Itakura, K. An Analytical Drilling Model of Drag Bits for Evaluation of Rock Strength. Soils Found. 2012, 52, 216–227. [Google Scholar] [CrossRef]
- Che, D.; Zhu, W.-L.; Ehmann, K.F. Chipping and Crushing Mechanisms in Orthogonal Rock Cutting. Int. J. Mech. Sci. 2016, 119, 224–236. [Google Scholar] [CrossRef]
- Kalantari, S.; Hashemolhosseini, H.; Baghbanan, A. Estimating Rock Strength Parameters Using Drilling Data. Int. J. Rock Mech. Min. Sci. 2018, 104, 45–52. [Google Scholar] [CrossRef]
- Mishnaevsky, L.L. Investigation of the Cutting of Brittle Materials. Int. J. Mach. Tools Manuf. 1994, 34, 499–505. [Google Scholar] [CrossRef]
- Yilmaz, N.G.; Yurdakul, M.; Goktan, R.M. Prediction of Radial Bit Cutting Force in High-Strength Rocks Using Multiple Linear Regression Analysis. Int. J. Rock Mech. Min. Sci. 2007, 44, 962–970. [Google Scholar] [CrossRef]
- Simon, R. Energy Balance in Rock Drilling. Soc. Pet. Eng. J. 1963, 3, 298–306. [Google Scholar] [CrossRef]
- Teale, R. The Concept of Specific Energy in Rock Drilling. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1965, 2, 57–73. [Google Scholar] [CrossRef]
- Caicedo, H.U.; Calhoun, W.M.; Ewy, R.T. Unique ROP Predictor Using Bit-Specific Coefficient of Sliding Friction and Mechanical Efficiency as a Function of Confined Compressive Strength Impacts Drilling Performance. In Proceedings of the SPE/IADC Drilling Conference, Amsterdam, The Netherlands, 23–25 February 2005; p. SPE-92576-MS. [Google Scholar]
- He, L.; Huang, J.; Luo, Y.; Liu, W.; Zhu, X.; Fan, S. The Ductile–Brittle Transition of Rock Cutting: Insight from the Discrete Element Method. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 605. [Google Scholar] [CrossRef]
- Liu, W.; Qian, X.; Li, T.; Zhou, Y.; Zhu, X. Investigation of the Tool-Rock Interaction Using Drucker-Prager Failure Criterion. J. Pet. Sci. Eng. 2019, 173, 269–278. [Google Scholar] [CrossRef]
- Aresh, B.; Khan, F.N.; Haider, J. Experimental Investigation and Numerical Simulation of Chip Formation Mechanisms in Cutting Rock-like Materials. J. Pet. Sci. Eng. 2022, 209, 109869. [Google Scholar] [CrossRef]
- Che, D.; Han, P.; Guo, P.; Ehmann, K. Issues in Polycrystalline Diamond Compact Cutter-Rock Interaction from a Metal Machining Point of View-Part I: Temperature, Stresses, and Forces. Trans. ASME-B-Journ Manuf. Sci. Eng. 2012, 134, 064001. [Google Scholar] [CrossRef]
- Che, D.; Han, P.; Guo, P.; Ehmann, K. Issues in Polycrystalline Diamond Compact Cutter–Rock Interaction from a Metal Machining Point of View—Part II: Bit Performance and Rock Cutting Mechanics. J. Manuf. Sci. Eng. 2012, 134, 064002. [Google Scholar] [CrossRef]
- Zhang, G.; Thuro, K.; Konietzky, H.; Menschik, F.M.; Käsling, H.; Bayerl, M. In-Situ Investigation of Drilling Performance and Bit Wear on an Electrical Drill Hammer. Tunn. Undergr. Space Technol. 2022, 122, 104348. [Google Scholar] [CrossRef]
- Hood, M.; Alehossein, H. A Development in Rock Cutting Technology. Int. J. Rock Mech. Min. Sci. 2000, 37, 297–305. [Google Scholar] [CrossRef]
- Gou, B.; Zhang, M. Effects of Surface Grooves on Rock Cutting Performance and Contact Behavior of a TBM Disc Cutter. Eng. Fract. Mech. 2022, 267, 108466. [Google Scholar] [CrossRef]
- Li, X.; Huang, B.; Li, C.; Jiang, S. Dynamics Analysis on Roadheader Cutting Head Based on LS-DYNA. J. Converg. Inf. Technol. 2012, 7, 333–340. [Google Scholar] [CrossRef]
- Munoz, H.; Taheri, A.; Chanda, E. Rock Cutting Characteristics on Soft-to-Hard Rocks under Different Cutter Inclinations. Int. J. Rock Mech. Min. Sci. 2016, 87, 85–89. [Google Scholar] [CrossRef]
- Rostamsowlat, I.; Richard, T.; Evans, B. An Experimental Study of the Effect of Back Rake Angle in Rock Cutting. Int. J. Rock Mech. Min. Sci. 2018, 107, 224–232. [Google Scholar] [CrossRef]
- Richard, T.; Detournay, E.; Drescher, A.; Nicodeme, P.; Fourmaintraux, D. The Scratch Test as a Means to Measure Strength of Sedimentary Rocks; OnePetro: Richardson, TX, USA, 1998. [Google Scholar]
- Gray, K.E.; Armstrong, F.; Gatlin, C. Two-Dimensional Study of Rock Breakage in Drag-Bit Drilling at Atmospheric Pressure. J. Pet. Technol. 1962, 14, 93–98. [Google Scholar] [CrossRef]
- Majidi, R.; Miska, S.; Tammineni, S. PDC Single Cutter: The Effects of Depth of Cut and RPM under Simulated Borehole Conditions. Wiert. Naft. Gaz 2011, 28, 283–295. [Google Scholar]








| Rock | Dendity (Kg/m3) | Young’s Modulus (GPa) | Passion’s Ratio | UCS (MPa) | Internal Angle (°) |
|---|---|---|---|---|---|
| Tuffeau | 1530.00 | 3.40 | 0.24 | 9.00 | 26.20 |
| Tooth | 3510.00 | 897.10 | 0.07 | — | — |
| v (mm/s) | 15 | 25 | 35 | 45 | 55 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| h (mm) | Mean (Hz) | SD | COV (%) | Mean (Hz) | SD | COV (%) | Mean (Hz) | SD | COV (%) | Mean (Hz) | SD | COV (%) | Mean (Hz) | SD | COV (%) | |
| 0.5 | 24.39 | 2.43 | 9.96 | 38.96 | 1.34 | 3.44 | 54.07 | 0.64 | 1.18 | 66.52 | 1.62 | 2.44 | 81.37 | 1.27 | 1.56 | |
| 1.0 | 15.88 | 0.16 | 1.01 | 25.40 | 1.24 | 4.88 | 37.65 | 1.59 | 4.22 | 45.87 | 3.69 | 8.04 | 55.17 | 1.84 | 3.34 | |
| 1.5 | 10.55 | 0.88 | 8.34 | 20.18 | 1.95 | 9.66 | 25.54 | 1.86 | 7.28 | 32.51 | 1.16 | 3.57 | 40.37 | 1.33 | 3.29 | |
| 2.0 | 9.17 | 0.43 | 4.69 | 14.75 | 1.23 | 8.34 | 18.55 | 0.57 | 3.07 | 23.29 | 3.37 | 14.47 | 29.8 | 2.30 | 7.72 | |
| 2.5 | 6.60 | 0.98 | 14.85 | 12.25 | 0.91 | 7.43 | 15.86 | 1.05 | 6.62 | 17.69 | 1.14 | 6.44 | 24.22 | 0.93 | 3.84 | |
| 3.0 | 4.91 | 0.20 | 4.07 | 9.25 | 1.63 | 17.62 | 11.02 | 0.47 | 4.26 | 15.66 | 0.39 | 2.49 | 18.73 | 2.08 | 11.11 | |
| H (mm) | Fitted Equation | R2 | The Slope of the Fitted Equation k |
|---|---|---|---|
| 0.5 | y = 1.53x | 0.99 | 1.53 |
| 1.0 | y = 0.99x | 0.99 | 0.99 |
| 1.5 | y = 0.71x | 0.99 | 0.71 |
| 2.0 | y = 0.50x | 0.96 | 0.50 |
| 2.5 | y = 0.43x | 0.92 | 0.43 |
| 3.0 | y = 0.34x | 0.99 | 0.34 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Y.; Li, G.; Li, N. Dynamic Characteristics on Single-Tooth Rock Cutting Considering the Change of Extrusion Zone Height. Appl. Sci. 2025, 15, 3630. https://doi.org/10.3390/app15073630
Hu Y, Li G, Li N. Dynamic Characteristics on Single-Tooth Rock Cutting Considering the Change of Extrusion Zone Height. Applied Sciences. 2025; 15(7):3630. https://doi.org/10.3390/app15073630
Chicago/Turabian StyleHu, Yanbo, Guofeng Li, and Ning Li. 2025. "Dynamic Characteristics on Single-Tooth Rock Cutting Considering the Change of Extrusion Zone Height" Applied Sciences 15, no. 7: 3630. https://doi.org/10.3390/app15073630
APA StyleHu, Y., Li, G., & Li, N. (2025). Dynamic Characteristics on Single-Tooth Rock Cutting Considering the Change of Extrusion Zone Height. Applied Sciences, 15(7), 3630. https://doi.org/10.3390/app15073630






