Collapse Potential of an Existing Reinforced Concrete Bridge Structure

Abstract

This article presents the results of a study conducted to obtain the fragility curves of an existing reinforced concrete highway bridge in Kocaeli, Turkey, to investigate the collapse potential. Bridges are key components of transportation systems, providing convenient and efficient access to different locations. However, these structures are susceptible to forces that can cause significant damage in the event of seismic activity. Thus, the fundamental target of designing earthquake-resistant bridges is to ensure that they can remain functional at an acceptable level during seismic activity. At least, they are expected to survive strong earthquakes without collapse. Turkey is a country located on active fault lines and has experienced devastating earthquakes in the past. This high earthquake risk requires the design and construction of bridges that are a critical part of the transportation infrastructure having adequate safety levels so that the collapse risk can be minimized. Therefore, damage estimation of bridges is an important part of earthquake preparedness and the response plans that will be followed immediately after earthquakes. In this study, the fragility curves of an existing reinforced concrete highway were obtained to investigate the collapse potential. The interstory drift limits related to the performance levels defined by the Turkish Bridge Seismic Design Code 2020 were determined by the incremental dynamic analysis method, and fragility curves were obtained using 10 different earthquake records based on these determined limits. The results showed that the target performance level Uninterrupted Occupancy and Collapse Prevention performance level requirements, as defined by the Turkish Bridge Seismic Design, were not met, and the Collapse Probability is %100.

1. Introduction

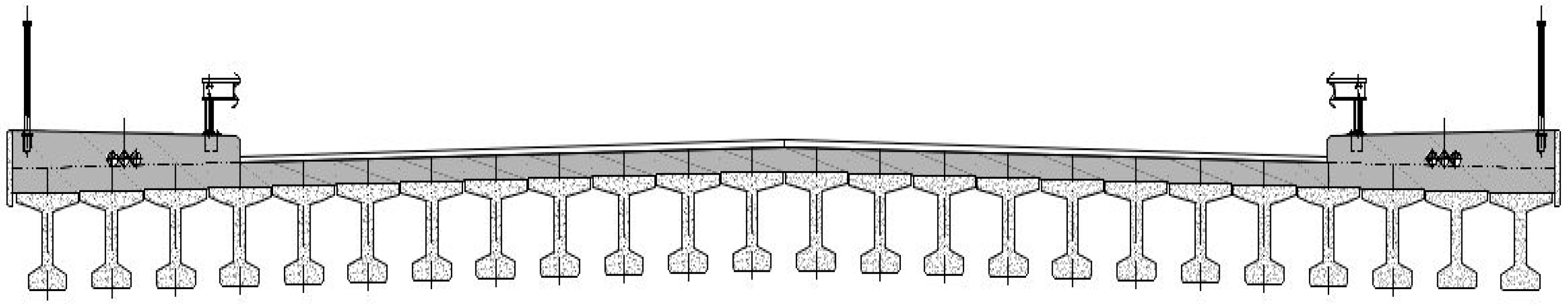
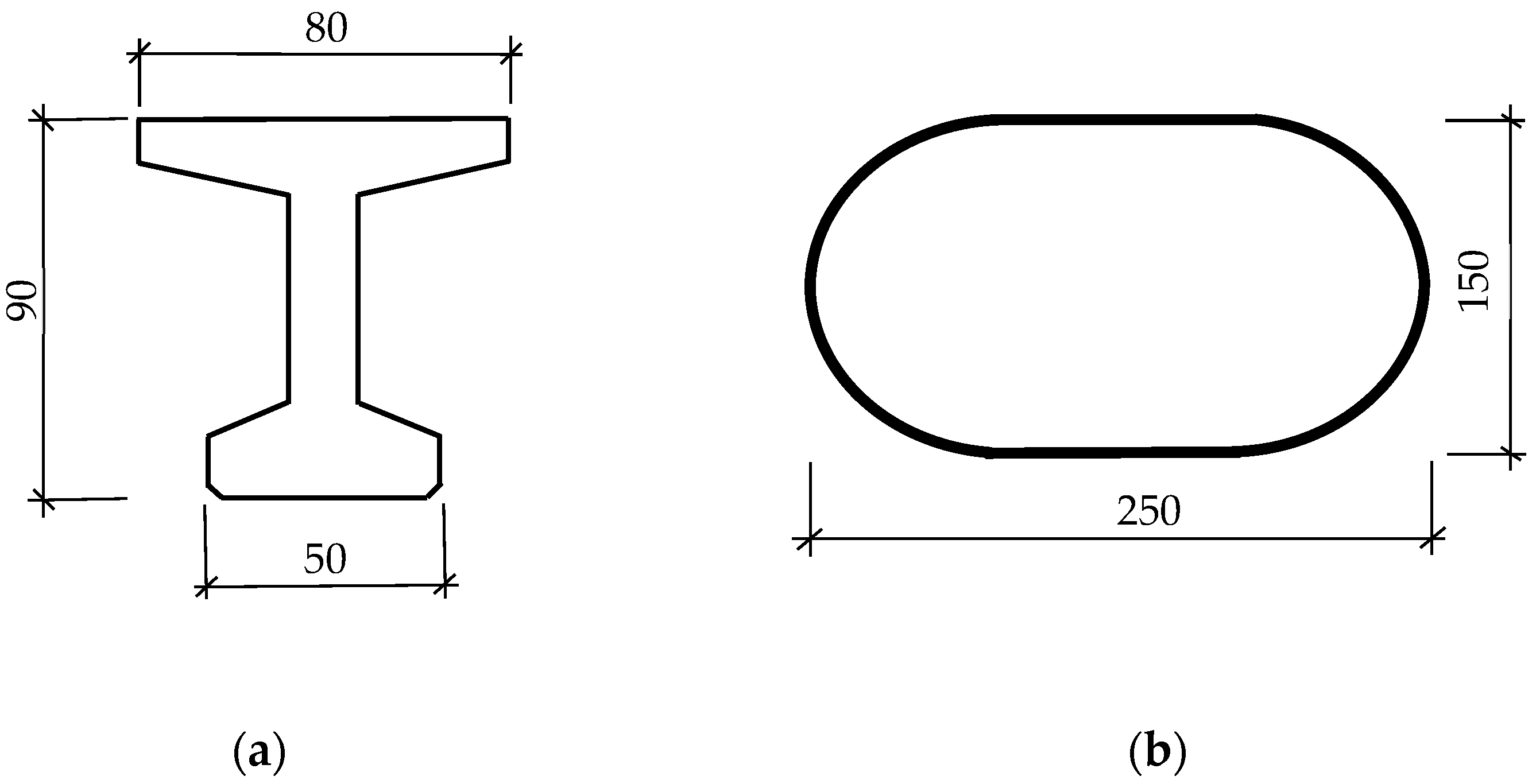
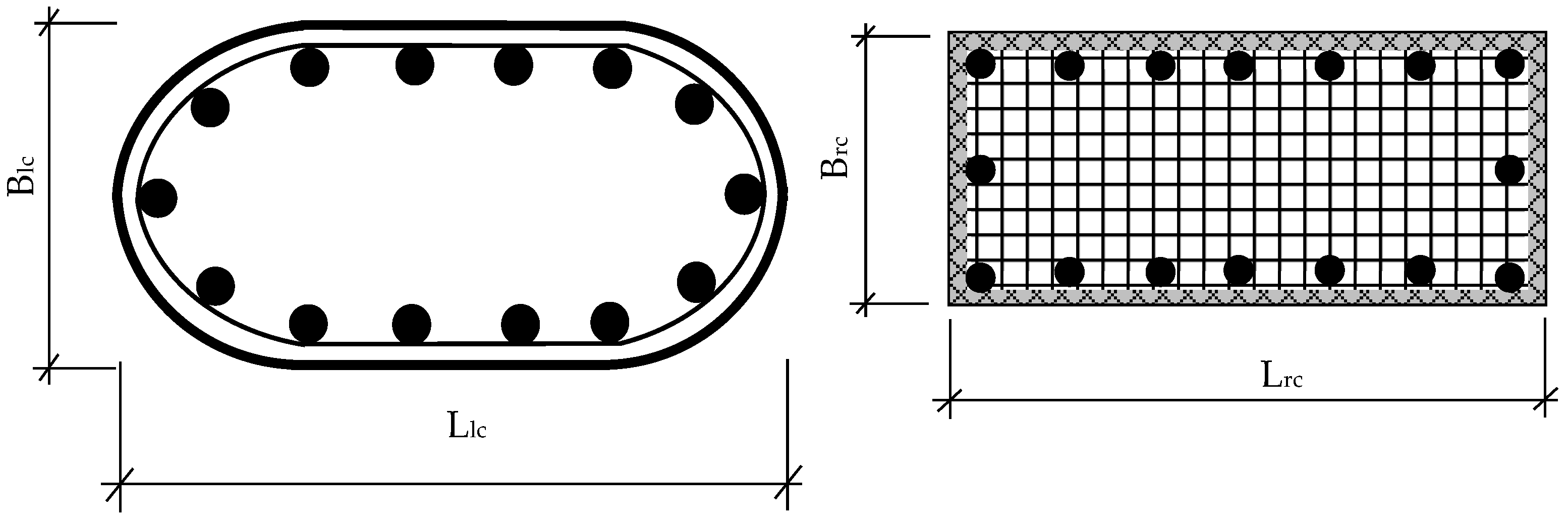
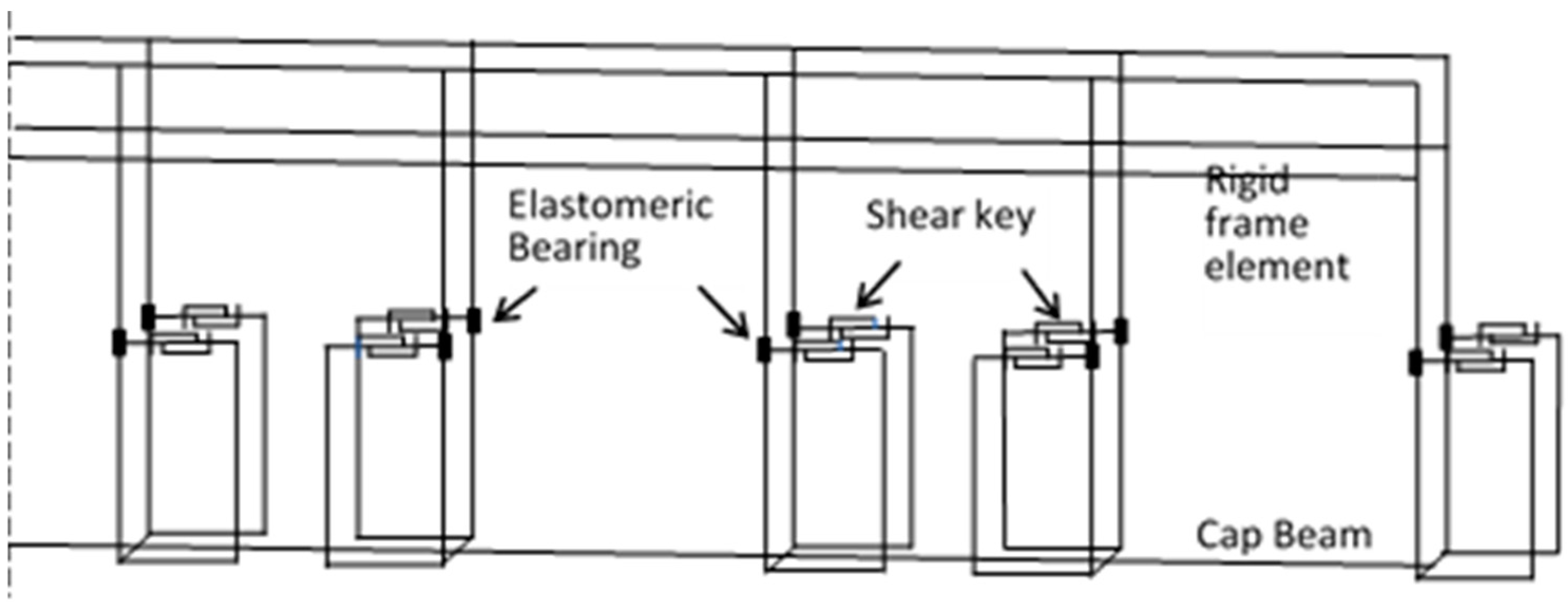
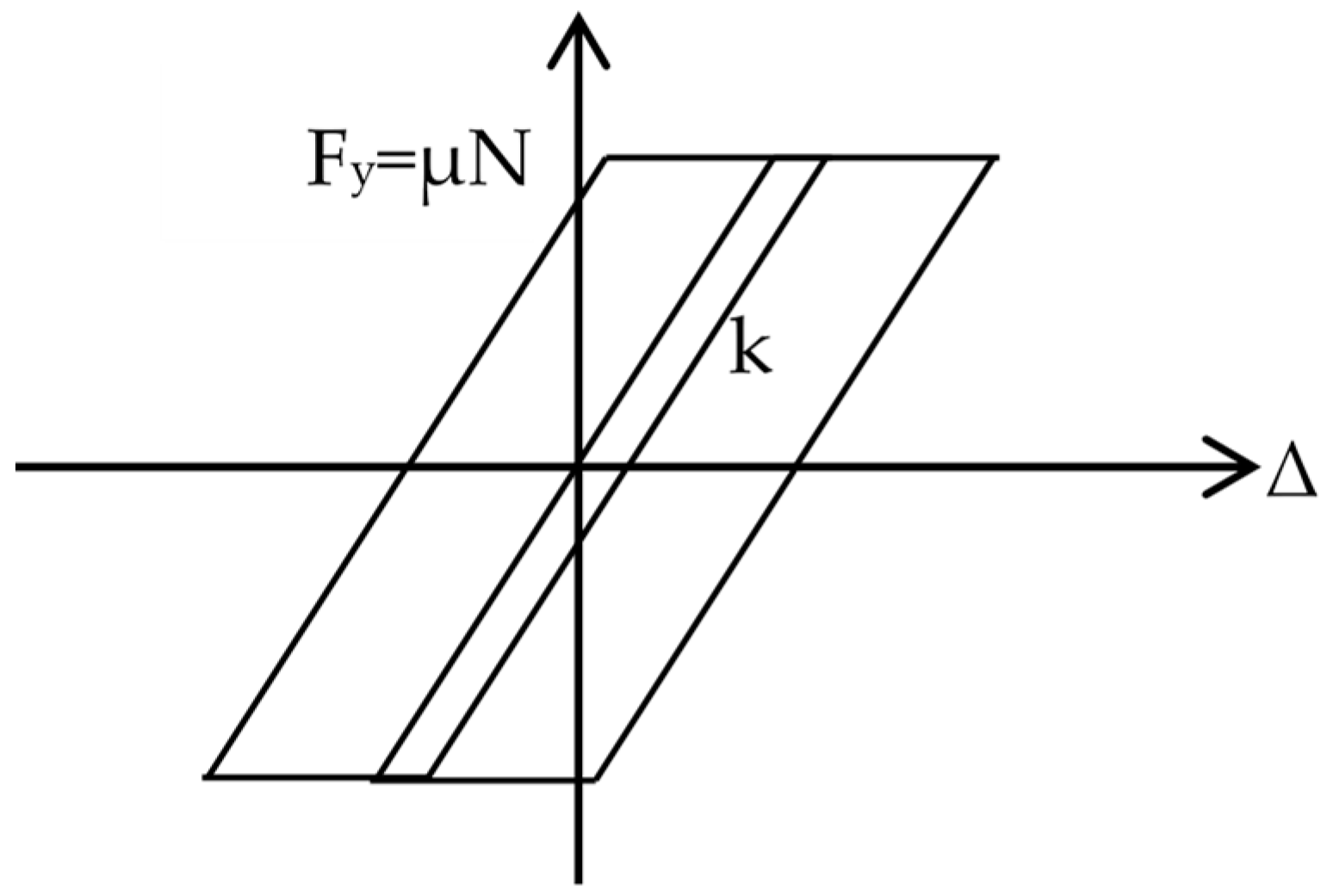
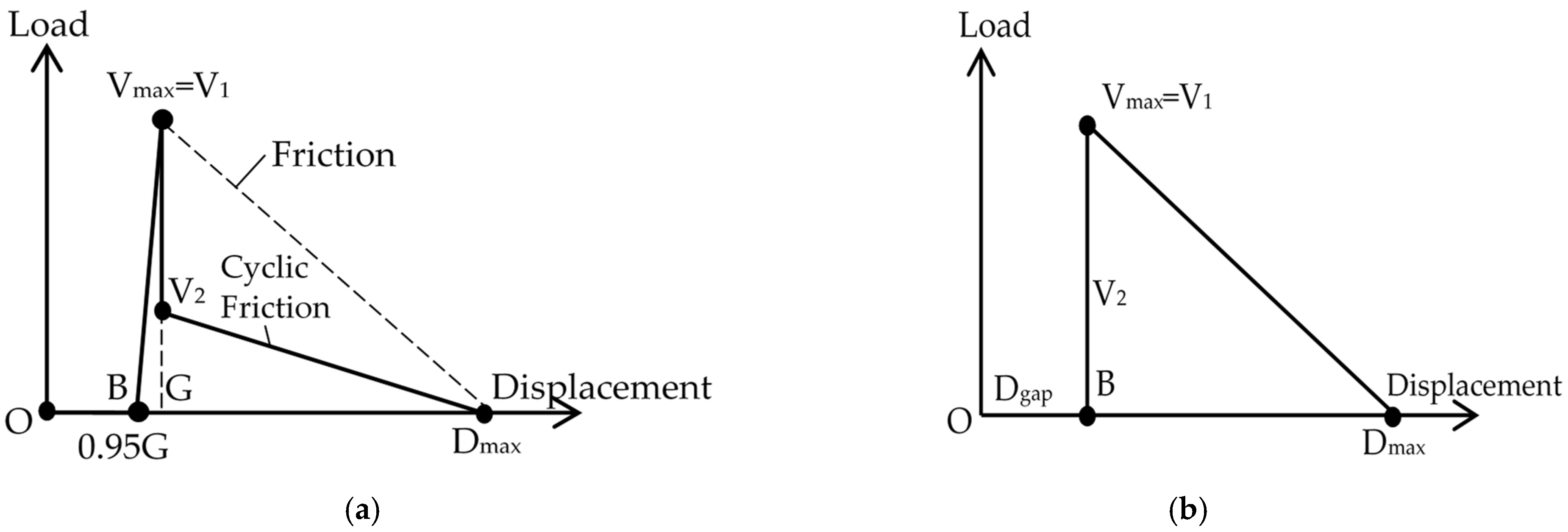
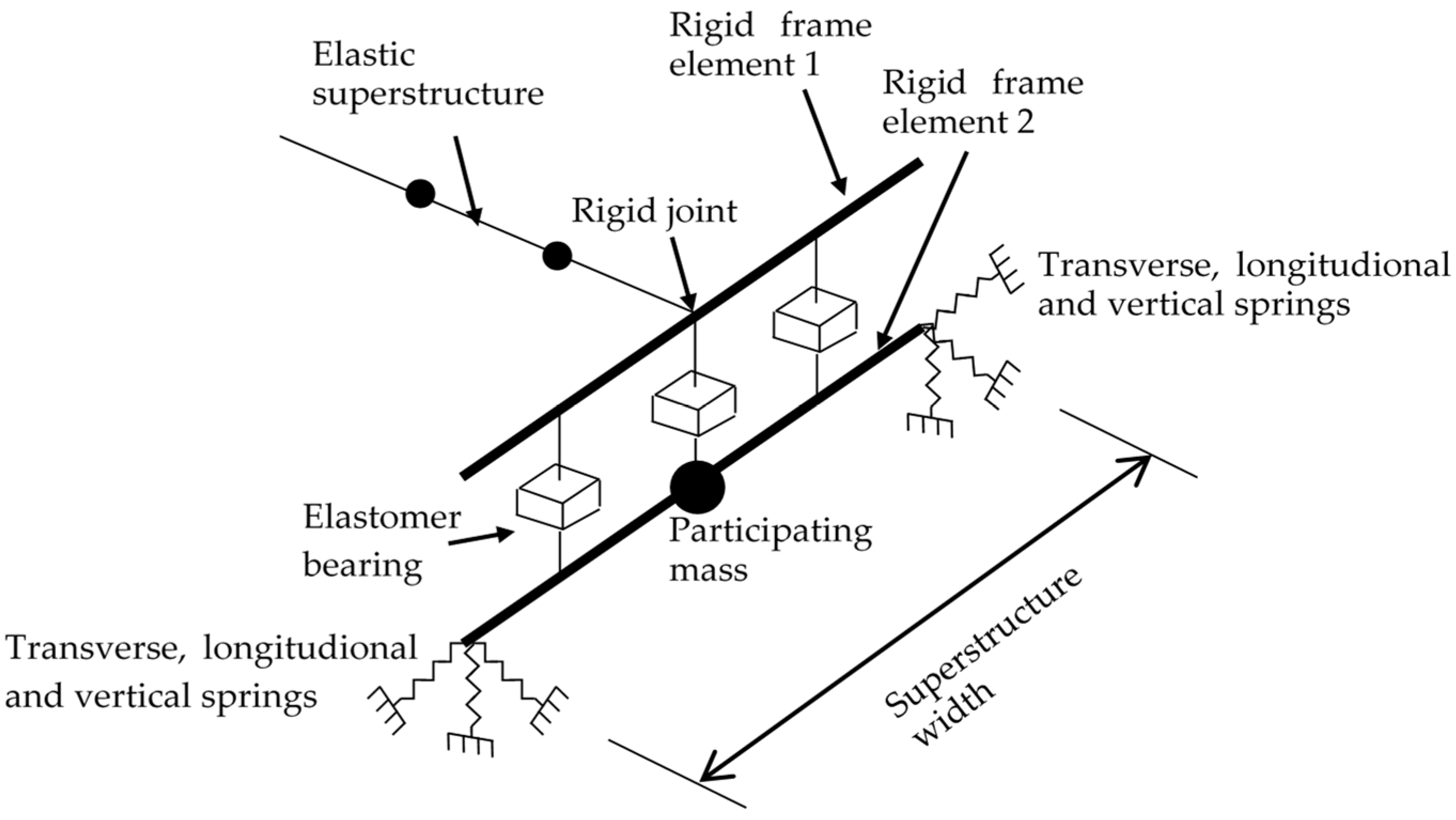
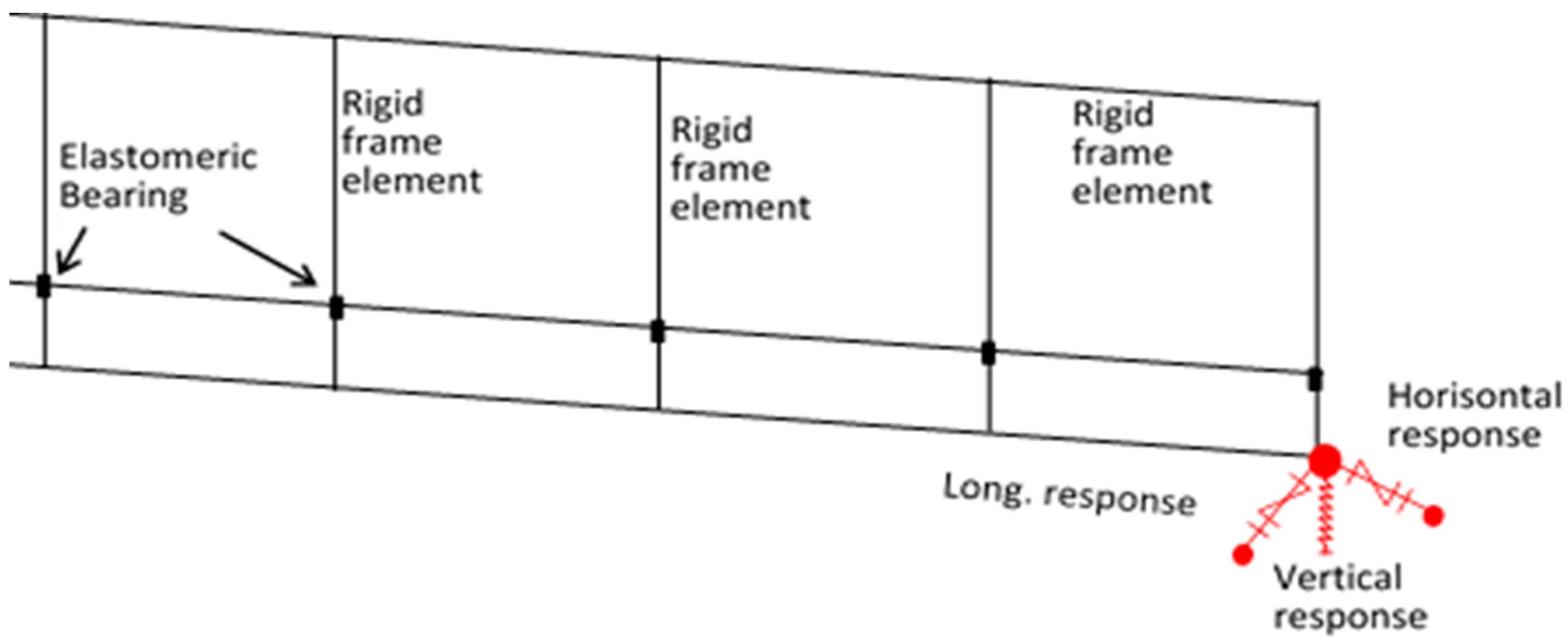
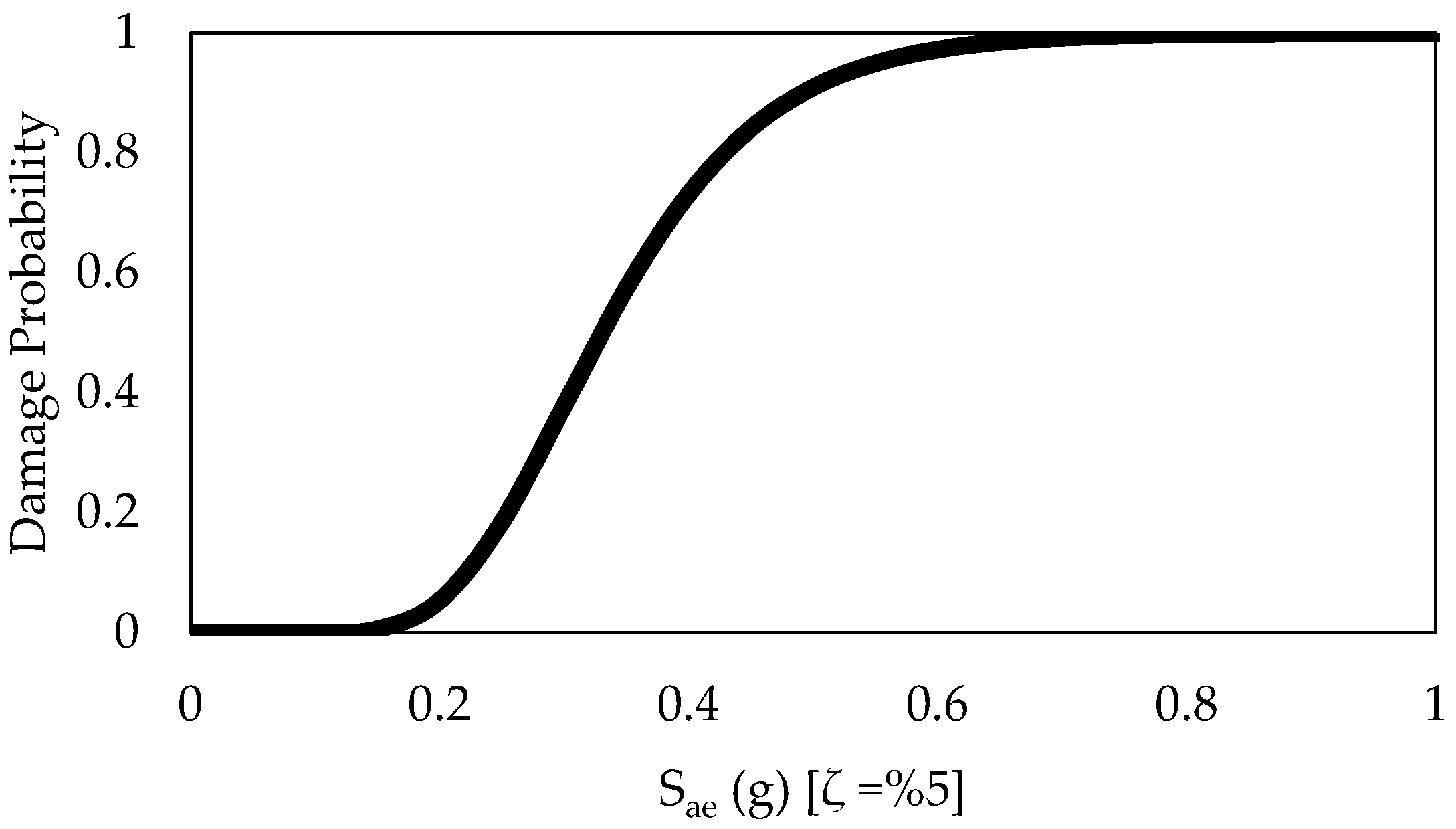
Turkey lies on active fault lines and has experienced numerous destructive earthquakes. This high earthquake risk requires ductile design and the construction of reinforced concrete bridges, which are an essential part of the transportation infrastructure, with adequate safety levels so that post-seismic serviceability can be provided or at least collapse can be prevented. However, in the case of making a design with the force-based design approach, no target performance level is defined, so it is not checked as to whether the targeted performance level’s requirements are satisfied or not. In other words, it is assumed that a bridge designed according to the code’s provisions will exhibit the intended target performance. Furthermore, there are newer versions of codes that aim for safer design and higher performance of bridges. However, bridges designed according to the older version of the seismic design codes may not fully meet the performance criteria defined by the current version of the codes as another source of uncertainty. This study mainly aims to investigate whether an existing bridge satisfies the targeted performance, as defined by the Turkish Bridge Seismic Design Code 2020 [1] through a fragility analysis. In other words, the purpose of the study is to determine whether the exceedance probabilities of the targeted performance levels are at a reasonable level. The investigated bridge has been designed in accordance with the 2012 version of the AASHTO [2] and based on the principles of force-based design, and this code aims to design bridges for ductile behavior and controlled damage. In the literature, numerous studies can be found that aim to obtain the fragility curves of different bridge types [3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24]. However, there are quite a few studies that question the validity of the above-mentioned assumption made within the scope of force-based design. Shinozuka et al. [3] compared the fragility curves of a bridge by considering 80 ground motions to examine the efficiency of two different methods used for the determination of bridge response, namely time–history analysis and the capacity spectrum method. Basöz et al. [4] compared the observed damage of bridges in Los Angeles, caused by the 1994 Northridge earthquake, with fragility curves given by ATC 13 [5] and the National Institute of Building Science. Yu et al. [6] obtained seismic fragility curves of highway bridges in Kentucky based on elastic response spectrum analysis and single-degree-of-freedom systems. Hwang et al. [7] proposed a method to estimate earthquake damage to bridges in urban areas. The proposed method was used to evaluate the expected damage to bridges in the Memphis area resulting from an M 7.0 scenario earthquake that may occur in the New Madrid Seismic Zone. Karim and Yamazaki [8] used an analytical approach to obtain the fragility curves for highway bridges based on a numerical simulation and proposed a simplified method to construct the fragility curves of non-isolated highway bridges in Japan. Elnashai et al. [9] derived analytic vulnerability functions for RC bridges based on four deformation-based limit states. They compared the obtained curves with a data set comprising observational damage data from the Northridge and Kobe earthquakes. Choi and Jeon [10] obtained a set of analytic fragility curves for the bridges of the Central and Southeastern United States. They also investigated the effectiveness of different retrofitting methods. The investigated methods are elastomeric bearings, lead–rubber bearings, and restrainer cables. Choi et al. [11] studied the fragility curves commonly located in the Central and Southeastern United States. They obtained fragility curves of the individual components of the structural system for each considered bridge type. Then, they developed fragility curves for each considered bridge structural system type by combining the fragility curves obtained for individual components based on the first-order reliability principles. They observed that the highest fragile bridge types are the multi-span simply supported and multi-span continuous steel-girder bridges, while the multi-span continuous prestressed concrete-girder bridge has the least fragility. Avşar et al. [12] carried out a study for the computation of analytical fragility curves of the ordinary highway bridges located in Turkey and designed in the 1990s. Four major bridge categories were set by considering the bridge’s skew angle, number of columns per bent, and number of spans. The obtained fragility curves show that bridges with larger skew angles or single-column bents have the highest fragility. Tong et al. [13] conducted an investigation to test the effectiveness of a retrofitting technique made with ultrahigh-performance concrete (UHPC) jackets for piers whose flexural strength is insufficient, under the effect of cyclic loading tests. The investigated pier specimens have several retrofitting techniques, namely with a mono-wide-strip jacket and a multi-narrow-strip jacket. The effectiveness of retrofitting techniques was using several indicators, such as damage evolution, hysteretic behavior, skeleton curve, ductility, and energy dissipation. A higher lateral strength was observed for the piers retrofitted with a mono-wide-strip jacket, while its sensitivity to plastic hinge relocation was also observed. In comparison, the multi-narrow-strip jacket enhanced the ductility of the piers and reduced the spread of concrete damage, provided there was a tight contact between the multi-narrow strips, sheath, and the shaft. Li et al. [14] evaluated the seismic fragility and seismic life-cycle loss of bridges with the precast segmental ultrahigh-performance concrete columns (UHPC) and by monolithic reinforced concrete piers. Pang et al. [15] investigated the seismic performance of fiber-reinforced concrete bridge piers under the effect of both far-field and near-fault ground motions. They obtained the fragility curves of the aforementioned columns with different fiber types to investigate their effectiveness and concluded that the improved ductility provided by fiber-reinforced concrete can significantly reduce the damage of bridge piers for both near-fault and far-field ground motions. Moayyedi et al. [16] developed fragility functions to investigate and compare the damage potential of the overall structural system and the components of the structural system of both regular and irregular box-girder bridges with and without pounding. Furinghetti et al. [17] carried out the seismic analysis of five case-study bridges from the Italian Road Network. The fragility curves of each considered bridge have been obtained based on the results of nonlinear a time–history analysis made with a design spectrum-compatible ground motion set. They observed that the main deficiencies of the investigated bridges are related to the bearings and connecting elements of piers. Wei et al. [18] proposed a method to evaluate the seismic damage of bridges incorporating both the maximum drift and residual drift of columns as the demand parameter. They applied the proposed method to assess the seismic resilience of a two-span reinforced concrete bridge and observed that the residual drift has a significant effect on the probability of collapse. They reported that seismic resilience decreases in the case of taking both maximum drift and residual drift into consideration. Uenaga et al. [19] investigated the effect of structural parameters on seismic resilience, such as deck radius, pier height irregularity, and seismic wave angle under short- and long-period records by using fragility curves and the resilience surfaces. They observed that the angle of incidence is the most effective parameter when long-period records are applied in one direction, and the vulnerability of bridges increases when the angle of incidence approximates 0^◦. Izhar et al. [20] carried out a study to quantify the seismic damage of RC bridges under the effect of ground motions and proposed a new definition for the damage index and damage states. They performed a seismic vulnerability evaluation, developed fragility curves, and concluded that the proposed damage model is effective. Pinto et al. [21] examined the earthquake performance of a highway bridge with RC multi-column bents, whose span number is five, under the effect of earthquakes and chloride-induced corrosion with the help of fragility surfaces. They concluded that elastomeric bearings have the highest probability of damage. Sun et al. [22] used fragility curves to examine the efficiency of a new type of hybrid pier with replaceable components to guarantee that structural damage remains repairable following earthquakes. They derived the fragility curve of the new type of pier and showed that its fragility curve is slightly higher than the fragility curve of a conventional RC pier. They also noted that, when the hybrid pier experiences damage, the decreased stiffness affects its seismic performance, leading to a reduction in the seismic forces acting on the structure and enhancing its seismic resilience. Fraioli et al. [23] developed a numerical model to simulate the response of two large-scale RC columns, which had been repaired with different techniques, and implemented it into a prototype bridge model. Incremental dynamic analyses and a fragility analysis of numerical models were carried out to investigate the effectiveness of the repair techniques and their post-repair seismic performance. Dehghanpoor et al. [24] aimed to make an investigation on the fragility of reinforced concrete bridges by incorporating the vertical component of ground motions. They obtained fragility surfaces by taking pile-cap displacement and drift ratio as damage indicators into consideration. They showed the considerable effect of vertical spectral acceleration on the increase in damage probability for both slight damage and collapse state.

2. Materials and Methods

2.1. Investigated Bridge

A girder RC bridge, with 4 spans, has been investigated within the scope of this study, with lengths of 16.25 m, 20 m, 20 m, and 14.75 m, respectively. The bridge deck is 14 m wide and is designed for a one-way traffic flow. The superstructure of the bridge consists of 24 prestressed precast I-girders. The cast-in-place reinforced concrete slab above the girder has a thickness of 22 cm. Figure 1 and Figure 2 show the transverse and longitudinal cross-sectional views of the bridge superstructure, respectively.

The girders are placed on a total of 192 elastomeric bearings, which help to reduce vibrations in girders and accommodate horizontal movements. Shear keys are installed at abutments and cap beams to restrict the transverse movement of the bridge. The continuous deck is supported by simply supported longitudinal prestressed concrete girders.

As mentioned before, the prestressed girders have an I-shaped cross-section and a height of 90 cm, which is given in Figure 3a. A total of 24 simply supported girders are placed with a center-to-center spacing of 82.5 cm, as shown in Figure 1. They are supported by cap beams that are monolithically supported on 3 column bents. The column heights are variable, as seen in Figure 2.

The bridge columns have an elliptic shape, as given in Figure 3b. Its bending and shear reinforcement are 62Ø26 and Ø16/10, respectively. The compressive strength of concrete at 28 days is 45 MPa for girders, while it is equal to 30 MPa for other structural elements. Their mean strengths are taken into consideration for the analyses. The deck and longitudinal girders were modeled as an elastic element, since they are expected to remain linear elastic during the anticipated earthquake loads. The foundation type is a continuous footing that connects the columns to each other. The dimensions of the foundations are 8 m × 19.5 m, with a height of 1.5 m. Considering that the soil type is B and from the point of engineering view, those dimensions are sufficient to prevent different settlements and minimize the soil–structure interaction effect. Thus, the columns were assumed to be fixed in the numerical model.

2.2. Analytic Model

The inelastic spine models, as shown in Figure 4, were used for the nonlinear time–history analyses by using SAP2000 v.10 [25] software. The superstructure, including the cap beams, was modeled as an elastic element, since they were expected to remain elastic. The nonlinear behavior of the other structural elements was taken into account with their proper nonlinear properties. The bridge columns are modeled as a fiber model.

The used concrete model is Mander [1,26], which defines the stress–strain relationship of concrete whether it is confined or unconfined. The reinforcement model, described by the Turkish Bridge Seismic Design Code, was used for a similar relationship of reinforcement. The used model incorporates strain hardening. Figure 5 shows the stress–strain relationship of the used material models.

The existing bridge columns have an elliptical cross-section geometry as mentioned above. To overcome the modeling difficulties, the cross-section has been idealized as a rectangular shape following the method used by Mangalathu [27], as illustrated in Figure 6. The cross-section conversion is based on the confined concrete area, as the core concrete can sustain loads even at high deformation levels. Since unconfined shell concrete reaches its compressive strength at significantly lower deformation levels under bending, it is neglected in the cross-section transformation.

The plastic hinge hypothesis assumes that nonlinear deformations were uniformly distributed along a specific length of the structural element, which is called plastic hinge length. The selected locations of the plastic hinges are at both ends of the columns. The following is the equation that gives the plastic hinge length, as proposed by the Turkish Bridge Seismic Design Code 2020 [1].

$$L_p=0.08L_k+0.022f_{ye}{d}_{bl}\ge 0.044f_{ye}{d}_{bl}$$

(1)

where L_p, L_k, f_ye, and d_bl are plastic hinge length, distance between the critical section and moment zero point, reinforcement yield strength, and diameter of the longitudinal reinforcement, respectively. Elastomeric bearings transfer the horizontal forces of the bridge, acting on the superstructure, to other structural elements by exhibiting shear behavior. They completely isolate the superstructure from the substructure, making the superstructure more susceptible to deformations. In this type of elastomeric bearing, the only mechanism preventing sliding is friction forces. Depending on the applied forces, they can undergo horizontal, axial, and rotational deformations. Thus, the analytic model should include those mechanical properties. Equations (2)–(4) present the stiffness values considered for the axial, shear, and rotational deformations. E_c, G_eff, H, I, and A show modulus of elasticity, effective shear modulus, moment of inertia, and area of bearing, respectively.

$$k_v={\displaystyle \frac{E_{c}A}{H}}$$

(2)

$$k_H={\displaystyle \frac{G_{eff}A}{H}}$$

(3)

$$k_{\theta }={\displaystyle \frac{EI}{H}}$$

(4)

Figure 7 shows a schematic representation of the analytic model of a cap beam, elastomeric bearings, and shear keys, which are located on it. This part of the bridge is indicated in Figure 4 with a red circle.

Elastomeric bearings can be damaged when their shear, rotational, or axial force capacities are exceeded. The shear force capacity of the bearing is governed by the friction force capacity between the two surfaces (the bottom rubber layer and the concrete surface). The force–deformation relationship that represents this behavior of the elastomeric bearing is assumed to be elastoplastic [27]. The friction force capacity, F_y, is the product of the dynamic friction coefficient (μ) and the axial force (N). The friction coefficient has been assumed to be 0.4 [28]. Figure 8 shows the considered load–deformation relationship for the bearings; k is the shear stiffness of the bearings.

Shear keys significantly influence a bridge’s response during an earthquake. They facilitate the transfer of seismic shear forces to the abutments and piers by restricting the transverse movement of the deck. This effect is especially critical for abutment bearings, where the superstructure may become more independent from the abutment, resulting in large displacements during seismic events. Shear keys continue to restrict the transverse movement of the deck due to friction resistance, even after reaching their maximum shear force capacity, according to the results of the study conducted by Megally et al. [29] Figure 9a illustrates the force–displacement relationship of an internal shear key. Point G represents the distance between the shear key and the adjacent structural element, while point B corresponds to 95% of this distance. From point B onward, the load increases at a constant slope until the shear force capacity of the block is reached. When the shear force capacity, denoted by V₁, is attained, shear failure occurs. This type of failure is brittle. Thus, a sudden decrease in load-bearing capacity is observed. If the block is subjected to a monolithic load, the load–displacement curve decreases linearly until it reaches the maximum displacement (D_max). In the case of cyclic loading, the shear force capacity drops abruptly from V₁ to V₂, after which the behavior resembles that observed under monotonic loading.

The following equations are the formulations used for the calculation of the shear force capacity (V_max) of the shear keys [29].

$$V_{max}=V_1=11.3\sqrt{f_c^{\prime }}bd$$

(5)

$${V_2=V}_{cyclic}=V_{max} c$$

(6)

$$c=1.5\alpha -0.25$$

(7)

$$K_1={\displaystyle \frac{V_{max}}{0.05G}}$$

(8)

where V₂, f^’_c, b, d, K₁, and α are the cyclic capacity of the shear key, concrete strength, width, length, shear stiffness, and aspect ratio, respectively. The force–displacement relationship, shown in Figure 9b, is used in this study for external shear keys that are based on the research carried out by Megally et al. [29], which involved a series of external shear key experiments on bridge abutments. Their findings revealed that shear keys can displace up to 8.9 cm before completely losing their load-bearing capacity, and then, the capacity of the seismic blocks essentially drops to zero. The shear key response is represented using zero-length elements that accurately capture this nonlinear force–deformation behavior. According to [29] the shear force capacity of an external shear key is calculated with 2 different approaches, namely shear capacity based on friction and shear capacity based on bar analogy. The lower one is taken as V_max = V₁ in Figure 9. The difference between D_max and D_gap was found to be 8.89 cm by Megally et al. [29]. The model proposed by Mackie and Stojadinović [30] was used to develop the analytical model of bridge abutment, as shown in Figure 10. It considers the transverse, vertical, and longitudinal behaviors of the abutment using spring elements. The abutment mass is calculated by taking into account both the mass of the abutment itself and a portion of the backfill soil behind the wall, according to Zank and Makris [31].

The longitudinal response of the bridge deck due to impact with the abutment occurs when the gap between the deck and the abutment closes, leading to a collision. Several factors influence this response, including the properties of the elastomeric bearings, the gap length between the deck and the abutment back wall, the geometric characteristics of the back wall, and the properties of the backfill material between the abutment and the soil. Before the impact, vertical forces from the superstructure are transmitted through the elastomeric bearings to the abutment face wall, then to the foundation and the ground. In the event of a seismic-induced impact, the longitudinal response generates an interaction between the abutment face wall and the backfill soil, mobilizing the full passive earth pressure. A similar situation occurs in the transverse direction. Horizontal forces are transmitted sequentially from the elastomeric bearings to the shear keys, then to the abutment, foundation, and finally, to the ground. Thus, the bridge’s transverse response relies on the stiffness provided by those elements and the backfill material. In this case, an interaction also occurs between the wing walls and the backfill. If there is backfill soil behind the wing walls, it decreases the horizontal displacements. Springs are used to take girder impacts on the shear keys into consideration, assuming that no displacement occurs in the backfill behind the wing walls. Several studies in the literature have adopted this approach [32]. This method is also considered to be a conservative approach to checking the shear capacity control of the shear keys. The vertical behavior of the bridge is modeled by considering the vertical stiffness of the elastomeric bearings and the soil backfill [33]. Figure 11 shows the computer model of the abutment.

In the longitudinal direction of the bridge, a structural element is defined to model nonlinear behavior, as shown in the red color in Figure 11. It consists of a rigid component with shear and moment degrees of freedom, gap elements (GAP) at both ends that restrict movement to longitudinal translation, and a zero-length element that captures displacement induced by the backfill. In the transverse direction of the bridge, a series system similar to the longitudinal model is employed to capture nonlinear behavior. This system consists of a rigid element with defined shear and moment degrees of freedom, a gap element (GAP) that acts only in compression in the transverse direction, and a zero-length element at the end representing the external shear key. When the longitudinal gap between the bridge superstructure and the abutment back wall closes, the passive earth pressure of the backfill soil is mobilized. In this case, the initial stiffness of the backfill material plays a critical role. According to the Caltrans Seismic Design Criteria [34] Section 7.8.1-1, the initial stiffness for backfill material meeting standard specifications is defined by Equation (9).

$$K_i={\displaystyle \frac{28.7 \mathrm{k}\mathrm{N}/\mathrm{m}\mathrm{m}}{m}}$$

(9)

According to the Caltrans Seismic Design Criteria [34], the passive longitudinal response of the backfill soil is modeled using an elastic perfectly plastic force–displacement relationship. This relationship is illustrated in Figure 12 for the abutment-supported model, while the abutment stiffness and the passive pressure force resisting the abutment movement are represented in Equations (10) and (11), where A_e is the area of the back wall. The gap between the bridge superstructure and the abutment back wall is denoted as Δ_gap in Figure 12, whereas Δ_eff represents the effective longitudinal abutment displacement at the idealized yield point.

$$K_{abut}=K_i{w}_b\left({\displaystyle \frac{h}{1.7}}\right)$$

(10)

$$P_{bw}=239A_e\left({\displaystyle \frac{h_{bw}}{1.7}}\right)$$

(11)

To accurately determine the vibration characteristics of the bridge, it is crucial to account for the mass of the bridge abutment and the backfill behind it. In this study, the method proposed by Zhang and Makris [31] is employed for this purpose, as mentioned before. This approach considers a specific portion of the backfill behind the wall, known as the critical length, which is calculated using Equation (12) below.

$$L_c=0.7\times \sqrt{S\times B_c\times H}$$

(12)

where S, B_c, and H are the backfill slope, abutment wall width, and height, respectively. The total mass of the abutment consists of the sum of the masses of the wall components, such as the foundation, end wall, and wing wall, along with the mass of the soil behind the wall, whose length is L_c. The density of the backfill soil is taken as 19 kN/m³ [35].

2.3. Ground Motions

The selection and spectral matching of the ground motions to be used for nonlinear time–history analysis play an important role in evaluating the seismic performance of a structure. Ten earthquake ground motion records, obtained from the “Pacific Earthquake Engineering Research Center (PEER)” ground motion database [36], with varying magnitudes, peak ground accelerations (PGA), and source distances, were selected in this study to account for the inherent randomness of seismic events. Since the investigated bridge is located on Site Class B, all selected ground motion records were taken among earthquakes recorded at the stations on Site Class B. Soil layers and their amplification effect were not considered since the used records are not on the bedrock but on the stations located on different soil profiles. Therefore, the randomness originating from the soil profiles has also been taken into account in an indirect manner. All of the considered ground motions are summarized in

Table 1. Considered ground motions.

Event	Year	Station	PGA	Magnitude	Rjb (km)	Rrup (km)	Vs30 (m/s)	Site Class
San Fernando	1971	Cedar Springs, Allen Ranch	0.015	6.61	89.37	89.72	813.48	B
Tabas, Iran	1978	Tabas	0.86	7.35	1.79	2.05	766.77	B
Morgan Hill	1984	Gilroy Array #1	0.098	6.19	14.9	14.91	1428.14	B
Landers	1992	Lucerne	0.63	7.28	2.19	2.19	1369	B
Northridge-01	1994	Vasquez Rocks Park	0.13	6.69	23.1	23.64	996.43	B
Kocaeli, Turkey	1999	Gebze	0.14	7.51	7.57	10.92	792	B
Loma Prieta	1989	Los Gatos	0.3	6.93	3.22	5.02	1070.34	B
Duzce, Turkey	1999	IRIGM 496	0.74	7.14	4.21	4.21	760	B
Parkfield-02, CA	2004	Diablo Canyon Power Plant	0.0055	6	78.14	78.24	1100	B
Kahramanmaraş	2023	Andırın	0.44	7.7	20.54	20.54	998	B

.

The selected ground motion records were matched to the target spectrum of the structure using “Seismomatch 2016” software [37]. The Turkish Bridge Seismic Design Code [1] requires that this type of bridge must meet the collapse prevention performance level requirements under the effect of an earthquake with a 2% probability of exceedance, in other words, the maximum earthquake. Thus, the target spectrum used for matching is the spectrum of the maximum earthquake. A damping ratio of 5% was applied to each input record. Figure 13 shows the acceleration spectrum of the selected ground motions.

The amplitudes of the average spectrum of all of the selected records between the periods of 0.2T₁ and 1.5T₁ must be higher than the amplitude of the design spectrum according to the Turkish Bridge Seismic Design Code [1] provisions. As can be seen from Figure 14, this provision is satisfied. T₁ is the period of the dominant mode of the bridge, which is equal to 0.238 s. Figure 14 shows the mean spectrum of the matched ground motion records and the design spectrum.

2.4. Damage Levels

The Turkish Bridge Seismic Design Code [1] defines 4 different performance levels: Uninterrupted Occupancy Performance Level, Limited Damage Performance Level, Controlled Damage Performance Level, and Collapse Prevention Performance Level. The Uninterrupted Performance Level defines no or negligible damage in the main structural system elements. The Limited Damage Performance Level represents limited and easily repairable damage in the main structural system elements. The Controlled Damage Performance Level corresponds to the damage level in the main structural system elements that is not severe and is mostly repairable. Each performance level has a damage limit in terms of plastic rotation. This study aims to evaluate the probability of exceeding the collapse prevention performance level of an existing bridge in accordance with the current Turkish Bridge Seismic Design Code [1]. Therefore, the collapse limit has been established based on the damage criterion specified in the aforementioned code. The Code requires that this type of bridge must meet the collapse prevention performance level for an earthquake with a 2% probability of exceedance. The Turkish Bridge Seismic Design Code [1] defines the Collapse Prevention Performance Level as the onset of collapse where the bridge’s structural elements experience extensive severe damage. The acceptable damage levels, in terms of the plastic rotations of an RC section for each Performance Level, are given in Equations (13)–(15).

$${\theta }_p^{\left(LD\right)}=\left({\varphi }^{LD}-{\varphi }_y\right)L_p$$

(13)

$${\theta }_p^{\left(CD\right)}=\left(0.5{\varphi }_u-{\varphi }_y\right)L_p$$

(14)

$${\theta }_p^{\left(CP\right)}=\left(0.67{\varphi }_u-{\varphi }_y\right)L_p$$

(15)

where ${\varphi }_y$ and ${\varphi }_u$ are yielding curvature and ultimate curvature of the section, respectively. ${\varphi }^{LD}$ is the curvature calculated based on the existing longitudinal and transverse reinforcement and the unit strain capacities given by the Turkish Bridge Seismic Design Code [1], with 0.004 for concrete and 0.015 for reinforcement. The interstory drift ratio, obtained from IDA, which initiates the exceedance of the plastic rotation limits previously mentioned above, is accepted as the limit of the bridge’s structural performance level, and the corresponding spectral acceleration level is taken as the limit value of spectral acceleration for the reached or exceeded bridge performance level.

Table 2. Bridge performance levels and section damages.

Bridge Performance Level	Section Damage Level of a Structural System Element
	Interstory Drift Ratio of Limited Damage	Interstory Drift Ratio of Controlled Damage	Interstory Drift Ratio of Collapse Prevention
Limited Damage	X
Controlled Damage		X
Collapse Prevention			X

shows the bridge performance level and section damage levels based on the approach explained above.

The damage limits for the shear keys and elastomeric bearings, taken from Cordone [38], are given in

Table 3. Damage limits for bearing and shear key.

Section Damage	Limited Damage	Controlled Damage	Collapse Prevention
Elastomeric Bearing	dfr	dpad	duns
Shear Key	dgap	du

below.

d_fr is the displacement limit of the bearing calculated based on friction resistance, as given in Equation (16).

$$d_{fr}=\mu P/K_r$$

(16)

where μ, P, and K_r are the friction coefficient, the axial force of the bearing, and K_r stiffness, respectively. d_pad is the dimension of the elastomeric bearing in the direction of motion. d_uns refers to the displacement associated with the bridge deck sliding off the cap beam, and d_gap refers to the distance between the shear key and the adjacent structural element. Finally, d_u is the displacement corresponding to the shear strength of the shear key, which is calculated by dividing shear strength by its stiffness.

2.5. Incremental Dynamic Analysis

Incremental dynamic analysis is essentially a method that involves multiple nonlinear time–history analyses and can reveal both the elastic and post-yield behavior of the structure under investigation. Thus, the analytical damage limits are established by identifying at least the yield and collapse points under the effect of several ground motions. The graphical representation illustrates the relationship between the increasing load in terms of a selected ground motion intensity measure and increasing displacement, along with the resulting damage, which is referred to as the incremental dynamic analysis (IDA) curve. A comprehensive discussion of IDA can be found in the study by Vamvatsikos and Cornell [39]. IDA also allows for the identification of the different damage phases observed between the yield and collapse limits. The investigated structure is analyzed under the effect of scaled ground motions. The scaling procedure is carried out based on the 5%-damped elastic spectral acceleration corresponding to the structural period in the considered direction. In addition to elastic spectral acceleration, which is considered an intensity parameter, the corresponding interstory drift ratio of the columns serves as the damage indicator in this study. The spectral acceleration–maximum interstory drift ratio relationship is linear until the yield point is reached. The IDA curve loses its linearity due to the reduction in stiffness when the bridge reaches the yielding level of spectral acceleration. In cases of dynamic instability caused by non-converging analyses, the spectral acceleration level at which the analysis fails to converge is considered the collapse capacity. As mentioned above, SAP2000 [25] software was used for the nonlinear time–history analyses. Figure 15 shows the IDA curve obtained under the effect of the Duzce ground motion record.

As seen from the figure, the IDA curve is linear up to a spectral acceleration level of 0.31 g, and yielding is observed at the latter spectral acceleration value, which is equal to 0.32 g. At this moment the column relative drift is 0.0019. Yielding occurs at 10.168 s of the earthquake. Limited damage, controlled damage, and collapse prevention section damage levels are also reached at 10.192, 10.252, and 10.26 s of the same analysis. The relative story drifts related to those performance levels are 0.0095, 0.023, and 0.0237, respectively. Section damages were developed in different columns. All bridge performance level limits are reached at the same spectral acceleration level. The same structural behavior was observed for all of the considered ground motions. Figure 16 shows all of the IDA curves.

Figure 17 shows the plastic hinge locations at the collapse state.

2.6. Fragility Curves

Fragility curves give the probability of exceedance of a specific damage state or performance level in terms of the considered ground motion indices, which is taken as the elastic spectral acceleration in this study. Fragility curves are drawn based on the lognormal distribution assumption made in the literature. The probability of reaching or exceeding a performance level at a specific ground motion index can be given as follows:

$$P\left(PL\right)=P\left(d_{PL}\le d_{max}\right)=1-\Phi \left(r\right)$$

(17)

where Φ is the standard normal distribution parameter. d_PL and d_max show the performance level capacity and the maximum value in terms of the considered damage indicator corresponding to the considered intensity parameter, respectively. The standard normal variant r can be expressed as follows

$$r={\displaystyle \frac{\ln d_{LS}-{\lambda }_D}{\sqrt{{\zeta }_{LS}^2+{\zeta }_D^2}}}$$

(18)

λ_D and ζ_D are the required parameters to define the lognormal distribution function. λ_D shows the mean value of the considered variable, where it is assumed that the distribution is lognormal. It shows the mean value of the maximum response in terms of demand. ζ_D is the standard deviation, which reflects the dispersion of maximum demand. They can be calculated with Equations (19) and (20).

$${\lambda }_D=ln{\overline{d}}_{max}-{\displaystyle \frac{{\zeta }_D^2}{2}}$$

(19)

$$ln{\overline{d}}_{max}={\displaystyle \frac{1}{n}}{\sum }_{i=1}^n\ln d_{max,i}$$

(20)

ζ_LS is the lognormal standard deviation of a damage limit and is taken as 0.3 in this study, following the study carried out by Jeong and Elnashai [40]. ζ_D, is calculated as the combination of several uncertainties associated with demand estimation as given in Equation (21):

$${\zeta }_D=\sqrt{ln\left(1+{\left({\displaystyle \frac{{\sigma }_r}{{\overline{d}}_{max}}}\right)}^2\right)+ln\left(1+{\left({\displaystyle \frac{{\sigma }_c}{{\overline{d}}_{max}}}\right)}^2\right)+ln\left(1+{\left({\displaystyle \frac{{\sigma }_D}{{\overline{d}}_{max}}}\right)}^2\right)}$$

(21)

σ_r shows the standard deviation, which reflects the effect of randomness in the considered earthquake records and is taken into consideration by using 10 ground motion records. Standard deviation σ_c, caused by randomness in the material properties, is ignored within the scope of this study, following the results of the study carried out by Jeong and Elnashai [40]. They concluded that the mean response of 30 sample buildings, created to account for material uncertainty, closely matches the response of a building with the average material strength. Thus, mean material strength values are considered. σ_D can be expressed as follows:

$${\sigma }_D={\left[{\displaystyle \frac{1}{n-1}}{\sum }_{i=1}^n\left(\ln d_{max,i}-ln{\overline{d}}_{max}\right)\right]}^{0.5}$$

(22)

The fragility curve of the collapse state obtained for the shorter direction of the considered bridge is given in Figure 18.

3. Results

Table 4. Modal information on the investigated bridge.

Mode	Period	Modal Mass Ratio
		Long.	Trans.
1	0.811	0.904	1 × 10−10
2	0.289	2.76 × 10−8	0.012
3	0.238	3.5 × 10−12	0.983
4	0.178	7.92 × 10−5	1.62 × 10−11
5	0.177	1.1 × 10−6	5.5 × 10−8
6	0.176	0.096	1.88 × 10−9

shows vibration periods and modal mass ratios of each mode of the investigated bridge.

The first three modes are shown in Figure 19, Figure 20 and Figure 21.

Table 5. Interstory drift ratio at each performance level for all the considered ground motions.

Ground Motion	Yielding	Limited Damage	Controlled Damage	Collapse Prevention
San Fernando	0.0023	0.01	0.018	0.046
Tabas, Iran	0.0028	0.01	0.02	0.046
Morhan Hill	0.002	0.001	0.019	0.025
Landers	0.0018	0.012	0.014	0.027
Northridge-01	0.003	0.009	0.02	0.044
Kocaeli, Turkey	0.0017	0.007	0.02	0.024
Loma Prieta	0.0019	0.01	0.02476	0.02482
Duzce, Turkey	0.002	0.01	0.0237	0.023713
Parkfield-02, CA	0.0017	0.01	0.017	0.033
Kahramanmaraş	0.0015	0.01	0.025476	0.025636

shows the Interstory drift ratio values, which were obtained for each performance level and yielding by IDA, for each considered ground motion record. Similarly, Table 5 gives the same information but in terms of spectral acceleration.

As seen in Figure 16 and

Table 6. Spectral Acceleration at each performance level for all the considered ground motions.

Ground Motion	Yielding	Limited Damage	Controlled Damage	Collapse Prevention
San Fernando	0.34	0.34	0.34	0.34
Tabas, Iran	0.31	0.31	0.31	0.31
Morhan Hill	0.38	0.38	0.38	0.38
Landers	0.32	0.32	0.32	0.32
Northridge-01	0.3	0.3	0.3	0.3
Kocaeli, Turkey	0.34	0.34	0.34	0.34
Loma Prieta	0.33	0.33	0.33	0.33
Duzce, Turkey	0.32	0.32	0.32	0.32
Parkfield-02, CA	0.35	0.35	0.35	0.35
Kahramanmaraş	0.32	0.32	0.32	0.32

, all performance levels are reached at the same spectral acceleration level for the IDA curve of each ground motion record. The fragility curve, which is given in Figure 18, was obtained only for the Collapse Prevention Performance Level since all of the ground motions were scaled to spectrum with a 2% probability of exceedance in 50 years.

4. Discussion

The target performance levels defined by the Turkish Bridge Seismic Design Code [1] are uninterrupted occupancy under the effect of an earthquake with a 50% probability of being exceeded in 50 years and collapse prevention under the effect of an earthquake with a 2% probability of being exceeded in 50 years. The spectral acceleration levels of those earthquake levels are 0.99 g and 1.98 g, respectively. The probability of reaching or exceeding the Collapse Prevention Performance Level is 100%. To determine the probability of reaching or exceeding the Uninterrupted Occupancy Performance Level, all ground motions must be scaled to the spectrum with a 50% probability of exceedance in 50 years, followed by performing the IDA analyses. The fragility curve for this performance level can only be accurately derived through this approach. Although the ground motions in this study were not scaled to this level of ground motion, and fragility curves were not derived for this specific ground motion level, it is evident that the median value of the resulting fragility curves would be lower. Consequently, the probability corresponding to a spectral acceleration level of 0.99 g would still be 100%. Both the probability of reaching the Uninterrupted Occupancy Performance Level and reaching the Collapse Prevention Performance Level under the effect of the corresponding ground motion levels far exceed the acceptable or reasonable limits.

5. Conclusions

The following conclusions can be drawn from the results of this study.

• No damage has been observed in the longitudinal (traffic) direction of the bridge. Damage was only detected in the transverse direction;
• In the analyses conducted for each earthquake record, it was observed that the performance limits were reached at different spectral acceleration values for each record. However, for the same ground motion, all performance level limits were achieved at the same spectral acceleration level;
• It was observed that the columns reached the specified section damage limits before the elastomeric bearings and shear keys. Consequently, in case of collapse, the main reason is not the probable damage of the shear keys or elastomeric bearings;
• The median and standard deviation values characterizing the fragility curve were found to be 0.33 and 0.31, respectively.
• The Turkish Bridge Seismic Design Code requires that this type of bridge must satisfy the Collapse Prevention Performance Level under the effect of an earthquake with a 2% probability of exceedance in 50 years. In such a case, the probability of collapse is expected to be minimal from the point of view of code-compatible design. According to the spectrum provided by the Turkish Bridge Seismic Code for an earthquake with a 2% probability of exceedance in 50 years, the spectral acceleration corresponding to the structural period in a shorter direction (0.24 s) is 1.98 g. The probability of exceeding the Collapse Prevention Performance Level is 100%. In other words, the investigated bridge will collapse at this ground motion level;
• The spectral acceleration value used for the design of the bridge is 1.51 g. This corresponds to the design earthquake with a 10% probability of exceedance in 50 years. Even at this spectral acceleration level, the probability of collapse is 100%, which is already high. Given that the spectral acceleration for an earthquake with a 2% probability of exceedance in 50 years is even higher, the collapse probability will still be 100% in the case of determination of the probability based on the design spectrum;
• The Turkish Bridge Seismic Design Code requires that this type of bridge must meet the Uninterrupted Occupancy Performance Level under the effect of an earthquake with a 50% probability of exceedance in 50 years. According to the Turkish Bridge Seismic Design Code [1], the spectral acceleration value for this level of earthquake is 0.99 g. The probability of exceeding the Uninterrupted Occupancy Performance Level at this spectral acceleration level would still be 100%, even in case of obtaining a fragility curve of this performance level under the effect of ground motion records scaled to this ground motion level;
• No shear failure has been observed in any of the bridge’s structural elements.

As observed, the bridge does not meet the performance requirements specified by the code. The absence of shear failure in any of the structural elements suggests that the bridge does not exhibit significant deficiencies in ductility or shear strength. The observed damage is primarily attributed to the insufficient lateral stiffness of the piers, which fails to sufficiently prevent excessive lateral displacement. This highlights the importance of ensuring not only adequate strength and ductility but also adequate stiffness in bridge design. Furthermore, the fact that all damage thresholds are reached at the same spectral acceleration level for a given earthquake indicates a lack of redundancy or insufficient reserve capacity in the structural system of the bridge.