Theoretical and Experimental Studies of Thermal Parameters of Annealed Polybutyl Methacrylate–Siloxane Protective Coatings by Means of Nondestructive Photothermal Radiometry and Photothermal Beam Deflection Methods
Abstract
1. Introduction
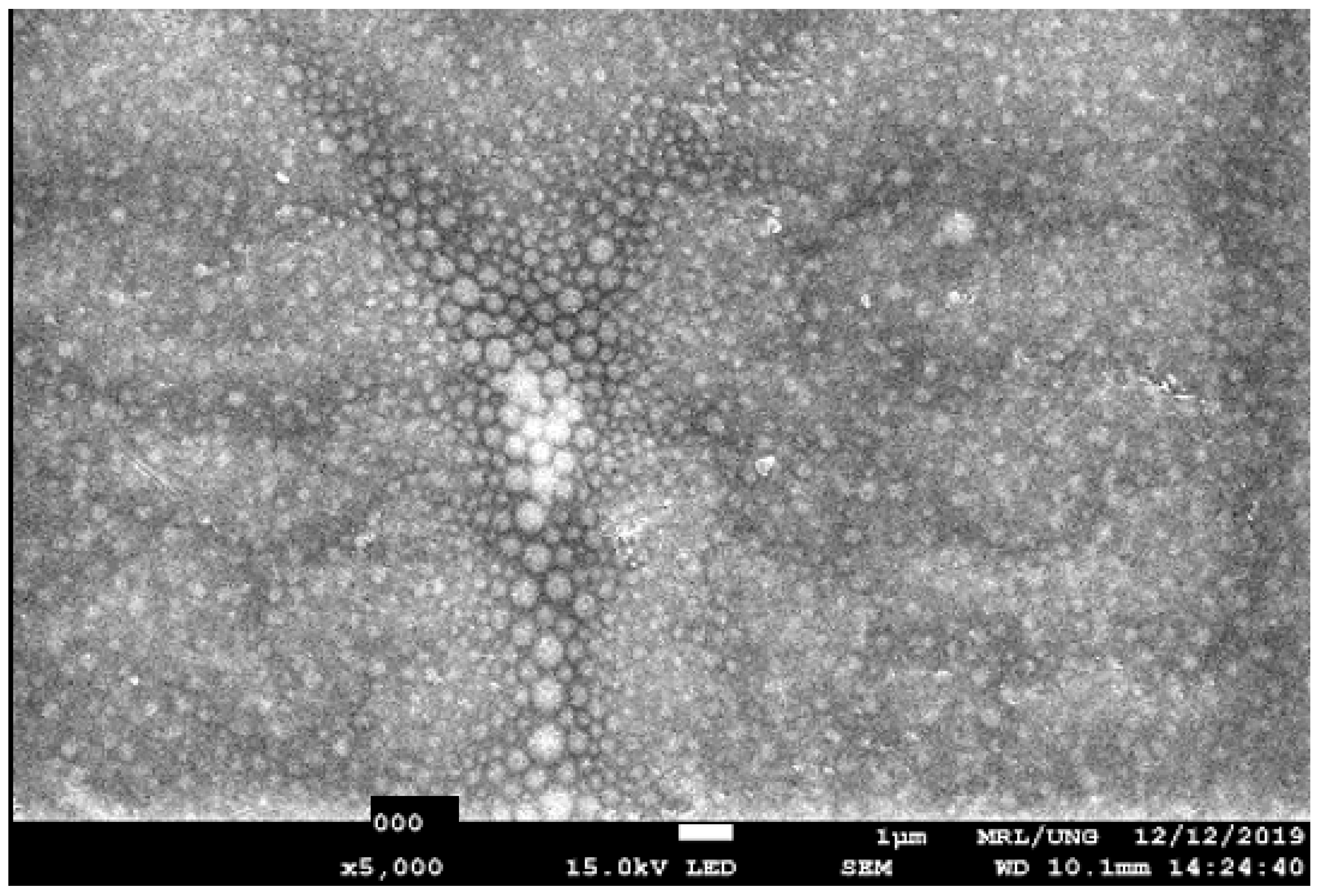
2. Sample Preparation
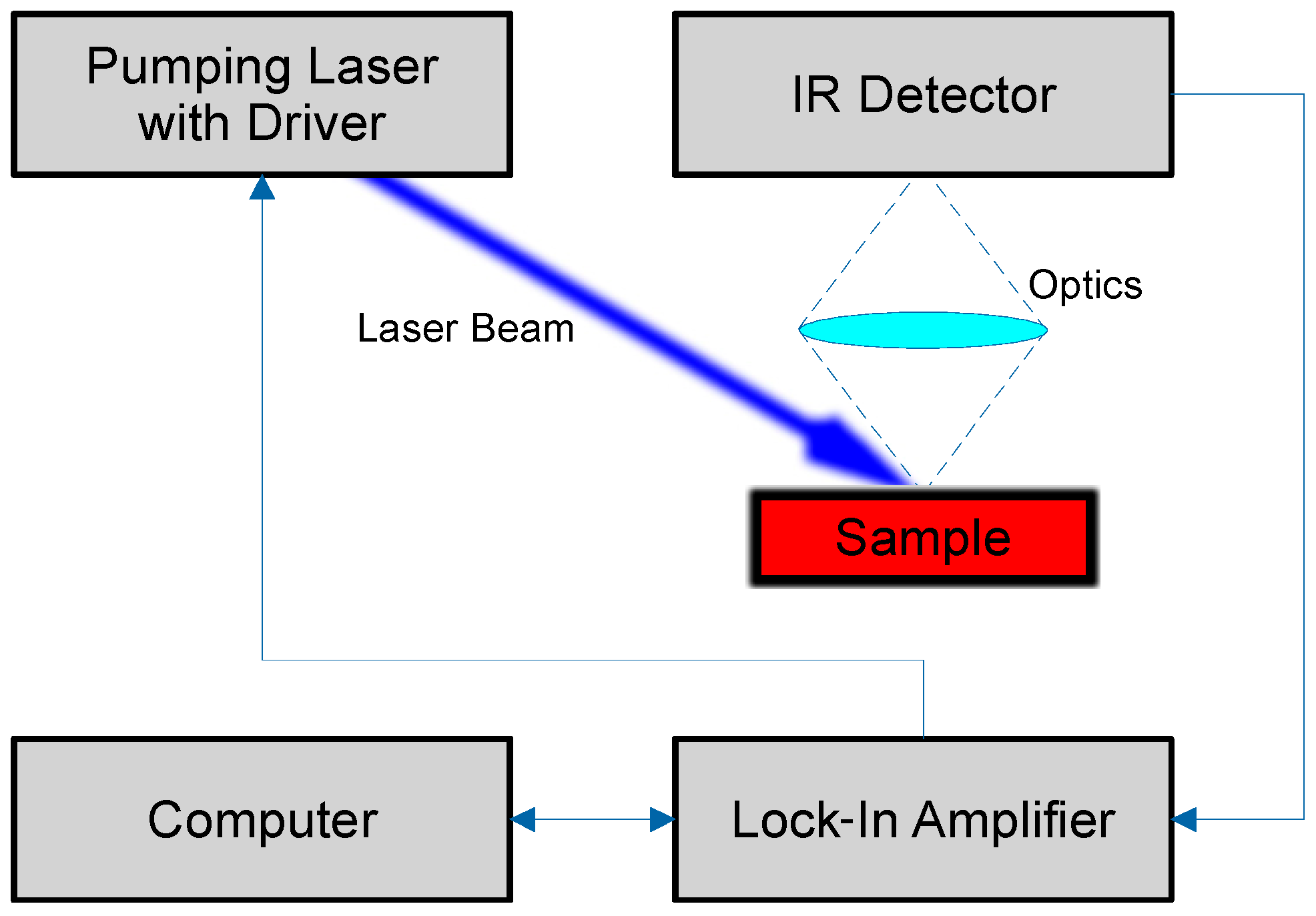
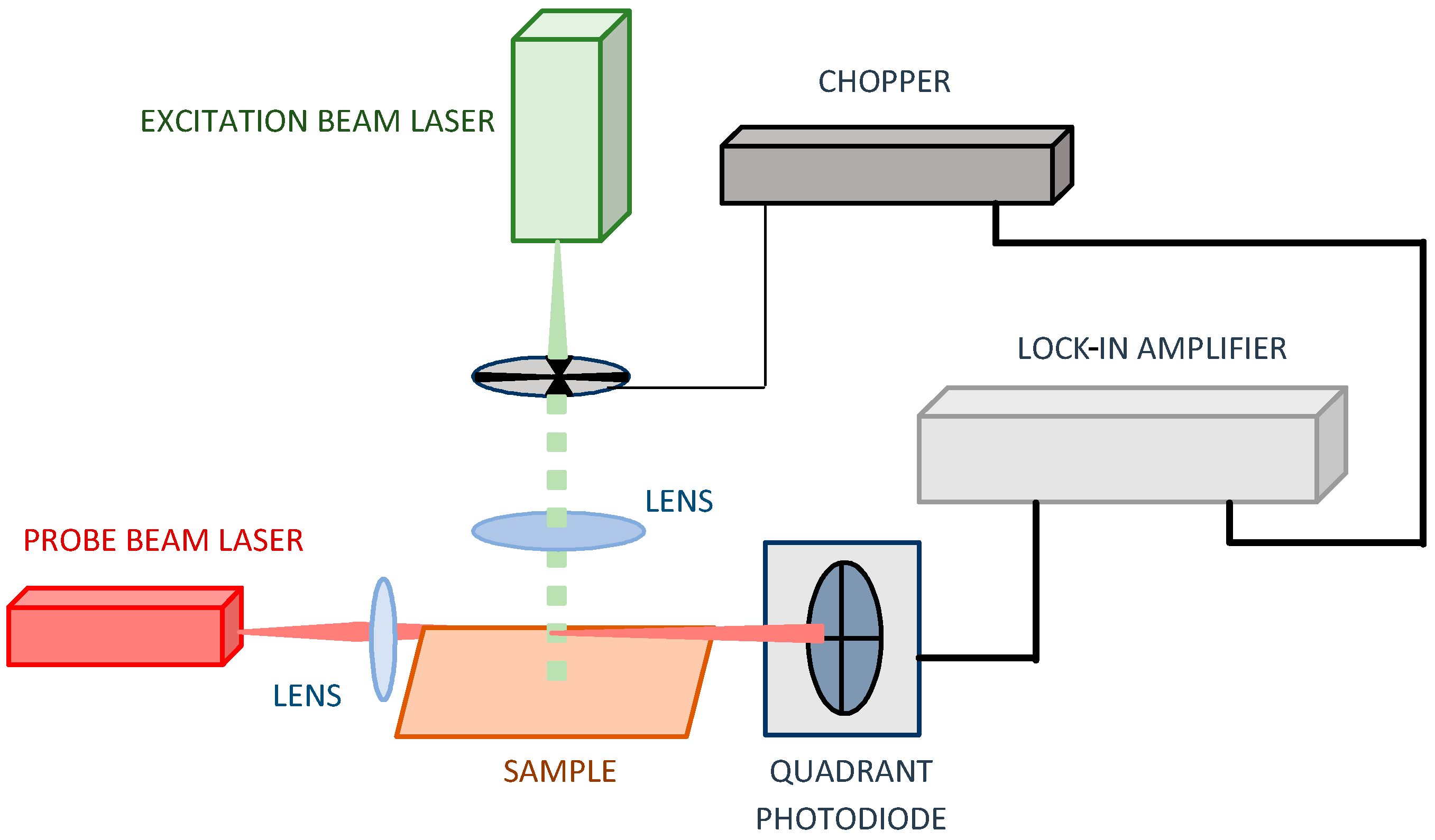
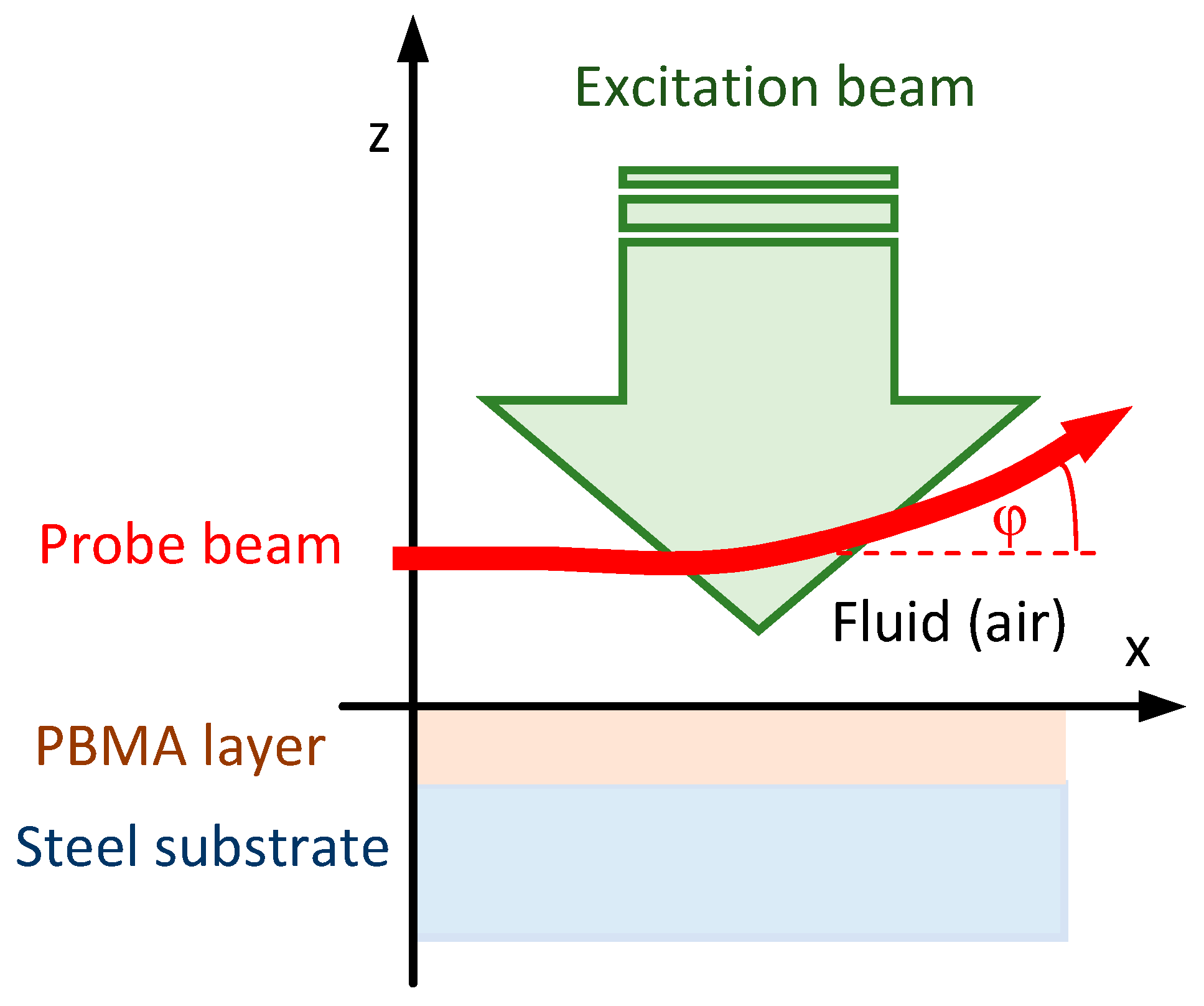
3. Experimental Methods
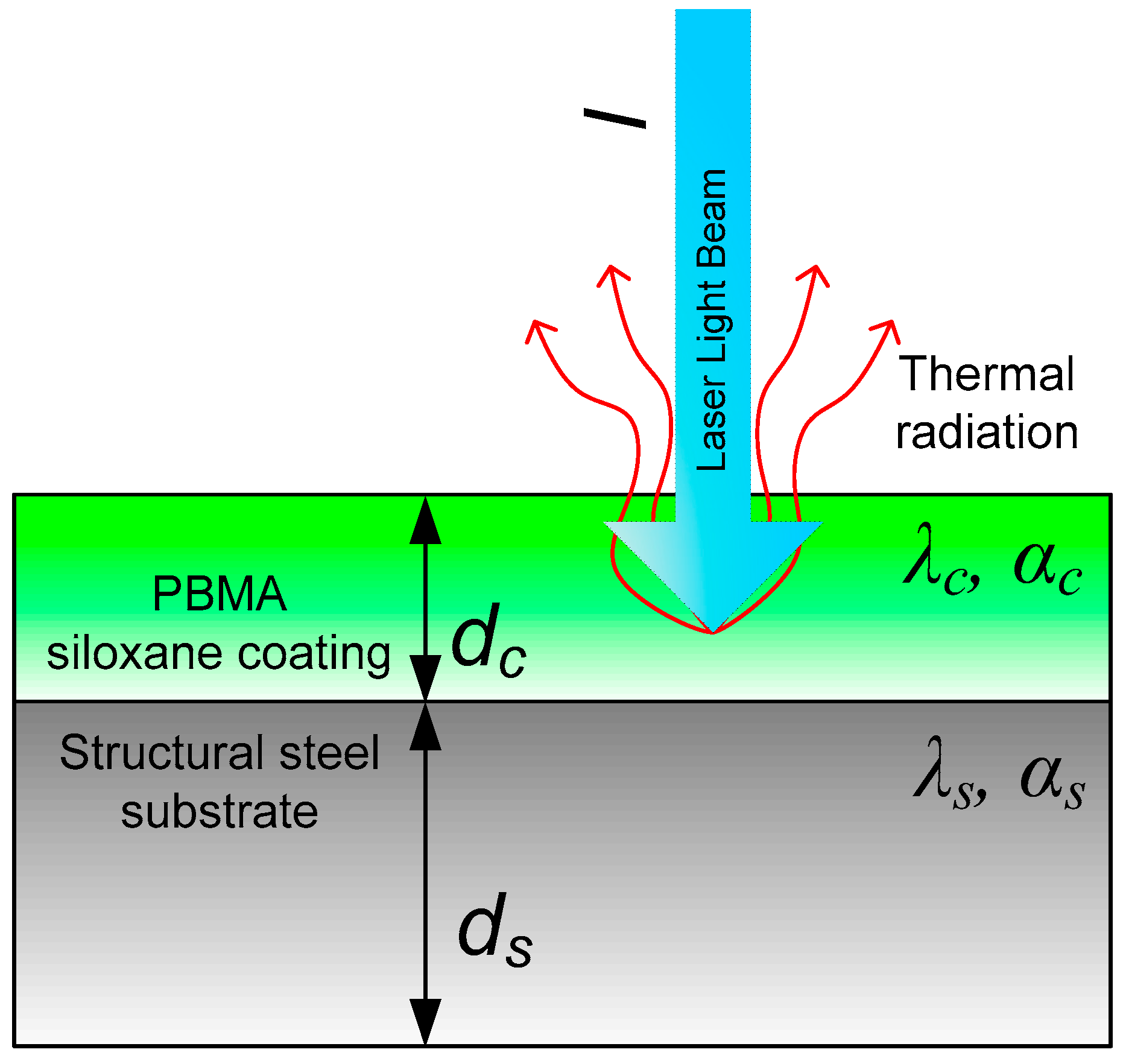
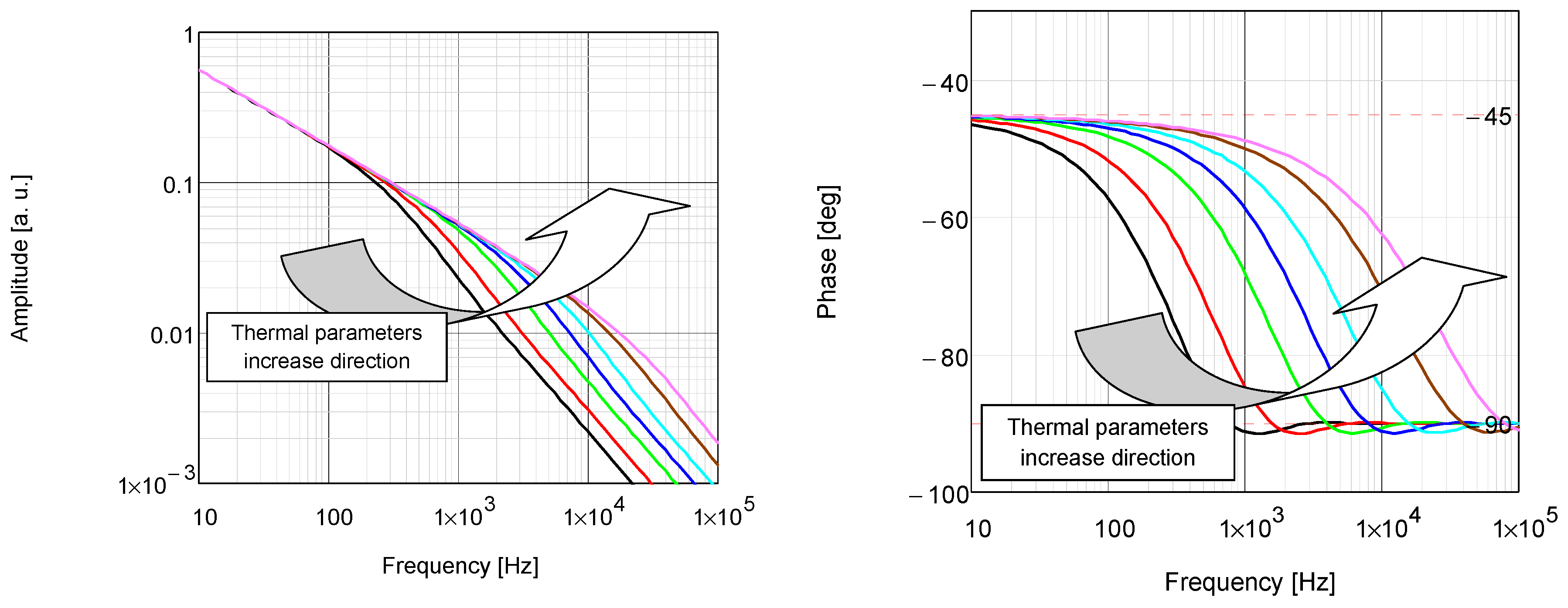
4. Theoretical Considerations
4.1. PTR Method
4.2. BDS Method
5. Experimental Results
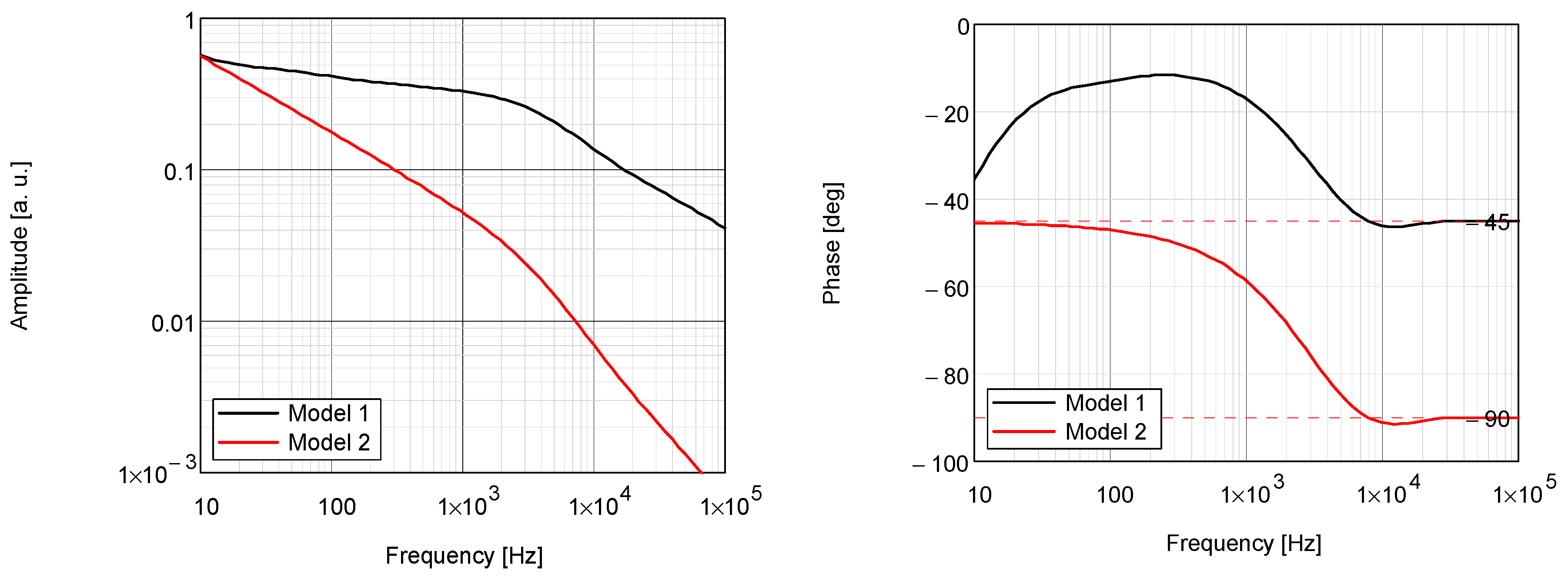
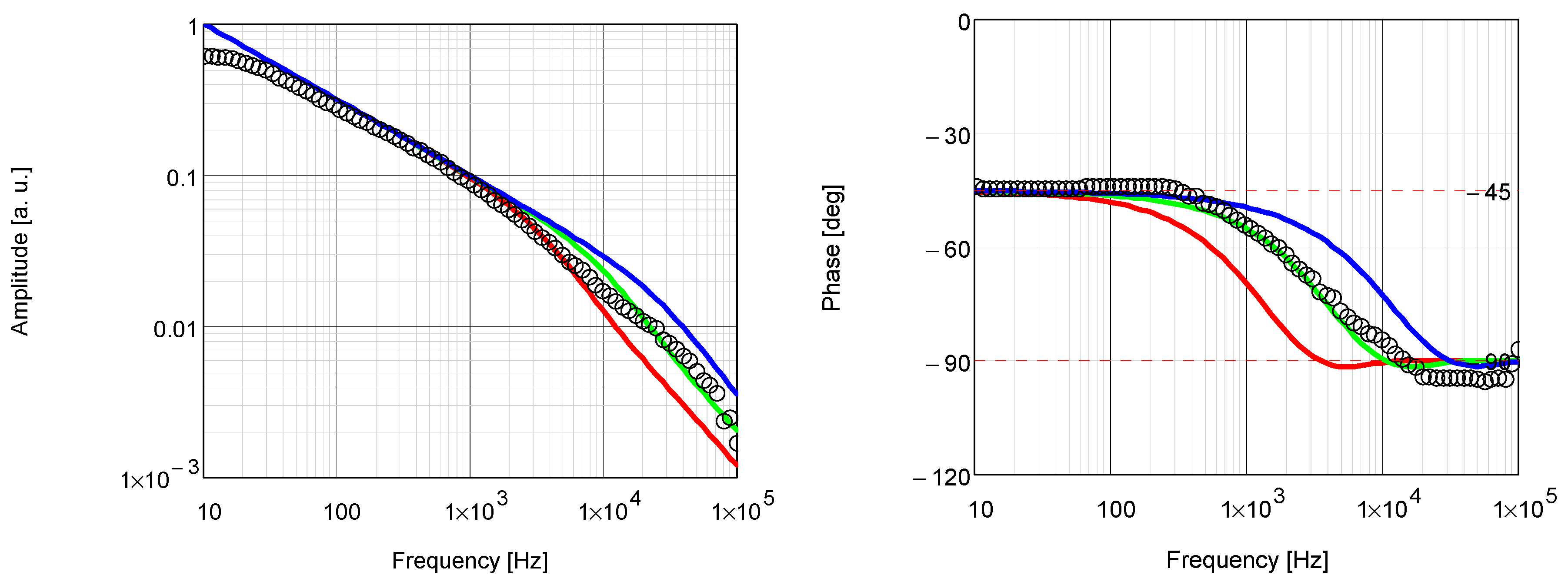
5.1. PTR Results
5.2. BDS Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Stankiewicz, A.; Szczygieł, I.; Szczygieł, B. Self-Healing Coatings in Anti-Corrosion Applications. J. Mater. Sci. 2013, 48, 8041. [Google Scholar]
- Rodič, P.; Katić, J.; Korte, D.; Desimone, P.M.; Franko, M.; Ceré, S.M.; Milošev, I. The Effect of Cerium Ions on the Structure, Porosity and Electrochemical Properties of Si/Zr-Based Hybrid Sol-Gel Coatings Deposited on Aluminum. Metals 2018, 8, 248. [Google Scholar] [CrossRef]
- Rodič, P.; Kapun, B.; Milošev, I. Durable Polyacrylic/Siloxane-Silica Coating for the Protection of Cast AlSi7Mg0.3 Alloy against Corrosion in Chloride Solution. Polymers 2023, 15, 3992. [Google Scholar] [CrossRef]
- Conde, J.J.; Ferreira-Aparicio, P.; Chaparro, A.M. Anti-Corrosion Coating for Metal Surfaces Based on Superhydrophobic Electrosprayed Carbon Layers. Appl. Mater. Today 2018, 13, 100. [Google Scholar]
- Wei, X.X.; Zhang, B.; Wu, B. Enhanced Corrosion Resistance by Engineering Crystallography on Metals. Nat. Commun. 2022, 13, 726. [Google Scholar]
- Hamulić, D.; Rodič, P.; Poberžnik, M.; Jereb, M.; Kovač, J.; Milošev, I. The Effect of the Methyl and Ethyl Group of the Acrylate Precursor in Hybrid Silane Coatings Used for Corrosion Protection of Aluminium Alloy 7075-T6. Coatings 2020, 10, 172. [Google Scholar] [CrossRef]
- Ma, H. First-Principles Modeling of Anisotropic Anodic Dissolution of Metals and Alloys in Corrosive Environments. Acta Mater. 2017, 130, 137–146. [Google Scholar]
- Nordal, P.E.; Kanstad, S.O. Photothermal Radiometry. Phys. Scr. 1979, 20, 659. [Google Scholar]
- Corona, J.; Nirmala, K. Recent Progress in Modulated Photothermal Radiometry. Sensors 2023, 23, 4935. [Google Scholar] [CrossRef]
- Jackson, W.B.; Amer, N.M.; Boccara, A.C.; Fournier, D. Photothermal Deflection Spectroscopy and Detection. Appl. Opt. 1981, 20, 1333–1344. [Google Scholar]
- Boccara, A.C.; Fournier, D.; Badoz, J. Thermo optical Spectroscopy: Detection by the “mirage Effect”. Appl. Phys. Lett. 1980, 36, 130–132. [Google Scholar]
- Boccara, A.C.; Fournier, D.; Jackson, W.; Amer, N.M. Sensitive Photothermal Deflection Technique for Measuring Absorption in Optically Thin Media. Opt. Lett. 1980, 5, 377–379. [Google Scholar]
- Wemhoner, J.; Wermer, L.; Silva, C.L.; Barnett, P.; Radosevich, C.; Patel, S.; Edens, H. Lightning Radiometry in Visible and Infrared Bands. Atmos. Res. 2023, 292, 106855. [Google Scholar]
- Fomina, P.S.; Proskurnin, M.A. Photothermal Radiometry Methods in Materials Science and Applied Chemical Research. J. Appl. Phys. 2022, 132, 040701. [Google Scholar]
- Mateos-Canseco, A.; Kusiak, A.; Battaglia, J.L. Thermal Imaging by Scanning Photothermal Radiometry. Rev. Sci. Instrum. 2023, 94, 104902. [Google Scholar]
- Sade, S.; Eyal, O.; Scharf, V.; Katzir, A. Fiber-Optic Infrared Radiometer for Accurate Temperature Measurements. Appl. Opt. 2002, 41, 1908–1914. [Google Scholar]
- Hristov, H.I.; Dimitrov, K.L.; Kolev, S.V. Use of Infrared Radiometry in Temperature Measurement of Plant Leaf. Int. J. Reason.-Based Intell. Syst. 2021, 13, 219–226. [Google Scholar]
- Salnick, A.; Mandelis, A.; Jean, C. Noncontact Measurement of Transport Properties of Long-bulk-carrier-lifetime Si Wafers Using Photothermal Radiometry. Appl. Phys. Lett. 1996, 69, 2522–2524. [Google Scholar]
- Salnick, A.; Jean, C.; Mandelis, A. Noncontacting Photothermal Radiometry of SiO2/Si MOS Capacitor Structures. Solid-State Electron. 1997, 41, 591–597. [Google Scholar]
- Macedo, F.; Vaz, F.; Rebouta, L.; Carvalho, P.; Haj-Daoud, A.; Junge, K.H.; Pelzl, J.; Bein, B.K. Modulated IR Radiometry of (TiSi)N Thin Films. Vacuum 2008, 82, 1457–1460. [Google Scholar]
- Dorywalski, K.; Chrobak, Ł.; Maliński, M. Comparative Studies of the Optical Absorption Coefficient Spectra in the Implanted Layers in Silicon with the Use of Nondestructive Spectroscopic Techniques. Metrol. Meas. Syst. 2020, 27, 323–337. [Google Scholar]
- Martinez-Hernandez, H.D.; Martinez-Munoz, P.E.; Ramirez-Gutierrez, C.F.; Martinez-Ascencio, E.U.; Millan-Malo, B.M.; Rodriguez-Garcia, M.E. Effect of Intrinsic and Extrinsic Defects on the Structural, Thermal, and Electrical Properties in p-Type CZ-Si Wafers with Different Carrier Concentrations. Int. J. Thermophys. 2022, 43, 181. [Google Scholar]
- Chrobak, Ł.; Maliński, M. Investigations of the Possibility of Determination of Thermal Parameters of Si and SiGe Samples Based on the Photo Thermal Radiometry Technique. Infrared Phys. Technol. 2018, 89, 46–51. [Google Scholar]
- Chrobak, Ł.; Maliński, M. On Investigations of the Optical Absorption Coefficient of Gold and Germanium Implanted Silicon with the Use of the Non-Destructive Contactless Photo Thermal Infrared Radiometry. J. Electron. Mater. 2019, 48, 5273–5278. [Google Scholar]
- Fontenot, R.S.; Mathur, V.K.; Barkyoumb, J.H. New Photothermal Deflection Technique to Discriminate between Heating and Cooling. J. Quant. Spectrosc. Radiat. Transf. 2018, 204, 1–6. [Google Scholar]
- Afjeh-Dana, E.; Asadian, E.; Razzaghi, M.R.; Rafii-Tabar, H.; Sasanpour, P. Deflection-Based Laser Sensing Platform for Selective and Sensitive Detection of H2S Using Plasmonic Nanostructures. Sci. Rep. 2022, 12, 15789. [Google Scholar]
- Olmstead, M.A.; Amer, N.M.; Kohn, S.; Fournier, D.; Boccara, A.C. Photothermal Displacement Spectroscopy: An Optical Probe for Solids and Surfaces. Appl. Phys. A 1983, 32, 141–154. [Google Scholar]
- Bialkowski, S.E.; Mandelis, A. Photothermal Spectroscopy Methods for Chemical Analysis. Phys. Today 1996, 49, 76. [Google Scholar]
- Barbero, C.; Kőtz, R.; Haas, O. Differential Photothermal Deflection Spectroscopy (Dpds). a Technique to Study Electrochromism of Synthetic Metals. Synth. Met. 1999, 101, 170. [Google Scholar]
- Sánchez-Lavega, A.; Salazar, A.; Ocariz, A.; Pottier, L.; Gomez, E.; Villar, L.M.; Macho, E. Thermal Diffusivity Measurements in Porous Ceramics by Photothermal Methods. Appl. Phys. A 1997, 65, 15–22. [Google Scholar]
- Leonid, S. Light-Induced Absorption in Materials Studied by Photothermal Methods. Recent Pat. Eng. 2009, 3, 129–145. [Google Scholar] [CrossRef]
- Xu, X.; Cuautle, J.J.A.F.; Kouyate, M.; Roozen, N.B.; Goossens, J.; Menon, P.; Malayil, M.K.; Salenbien, R.; Rajesh, R.N.; Glorieux, C.; et al. Evolution of Elastic and Thermal Properties during TMOS-Gel Formation Determined by Ringing Bottle Acoustic Resonance Spectroscopy, Impulsive Stimulated Scattering, Photopyroelectric Spectroscopy and the Hot Ball Method. J. Phys. D Appl. Phys. 2016, 49, 085502. [Google Scholar] [CrossRef]
- Milošev, I.; Hamulić, D.; Rodič, P.; Carrière, C.; Zanna, S.; Budasheva, H.; Korte, D.; Franko, M.; Mercier, D.; Seyeux, A.; et al. Siloxane Polyacrylic Sol-Gel Coatings with Alkly and Perfluoroalkyl Chains: Synthesis, Composition, Thermal Properties and Log-Term Corrosion Protection. Appl. Surf. Sci. 2022, 574, 151578. [Google Scholar] [CrossRef]
- Hanh, B.D.; Faubel, W.; Heissler, S.; Wartewig, S.; Neubert, R.H. Pharmaceutical Applications of Photothermal Beam Deflection. Laser Phys. 2006, 16, 794–798. [Google Scholar] [CrossRef]
- Rudnicki, J.D.; Russo, R.E.; Shoesmith, D.W. Photothermal Deflection Spectroscopy Investigations of Uranium Dioxide Oxidation. J. Electroanal. Chem. 1994, 372, 63–74. [Google Scholar] [CrossRef]
- Mandelis, A. Laser Infrared Photothermal Radiometry of Semiconductors: Principles and Applications to Solid State Electronics. Solid-State Electron. 1998, 42, 1–15. [Google Scholar] [CrossRef]
- Mandelis, A.; Batista, J.; Shaughnessy, D. Infrared Photocarrier Radiometry of Semiconductors: Physical Principles, Quantitative Depth Profilometry, and Scanning Imaging of Deep Subsurface Electronic Defects. Phys. Rev. B 2003, 67, 205208. [Google Scholar] [CrossRef]
- Chrobak, Ł.; Korte, D.; Budasheva, H.; Maliński, M.; Rodič, P.; Milošev, I.; Janta-Lipińska, S. Investigations of the Thermal Parameters of Hybrid Sol–Gel Coatings Using Nondestructive Photothermal Techniques. Energies 2022, 15, 4122. [Google Scholar] [CrossRef]
- Chrobak, Ł.; Maliński, M.; Korte, D. Two-Layer Hybrid Sol-Gel System’s Thermal Parameters Investigated with the Optical Nondestructive Photoacoustic Method in the Frequency Domain. Mater. Sci. Eng. B 2023, 295, 116585. [Google Scholar]
- Li, B.; Deng, Y.; Cheng, J. Pulsed Photothermal Deflection Spectroscopy with Optically Dense Samples. Appl. Spectrosc. 1995, 49, 279–285. [Google Scholar] [CrossRef]
- Korte, D.; Franko, M. Application of Complex Geometrical Optics to Determination of Thermal, Transport, and Optical Parameters of Thin Films by the Photothermal Beam Deflection Technique. JOSA A 2015, 32, 61–74. [Google Scholar] [PubMed]
- Kirkup, L. An Introduction to Uncertainty in Measurement: Using The Gum, 1st ed.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2006; ISBN 978-0-521-60579-3. [Google Scholar]
- Williams, J.H. Quantifying Measurement: The Tyranny of Numbers; Morgan & Claypool Publishers: San Rafael, CA, USA, 2016; ISBN 978-1-68174-433-9. [Google Scholar]
- Hamulić, D.; Medoš, G.; Korte, D.; Rodič, P.; Milošev, I. The Effect of Curing Temperature and Thickness of Polybutyl Methacrylate Siloxane Coatings on the Corrosion Protection of Structural Steel S355. Coatings 2023, 13, 675. [Google Scholar] [CrossRef]
- Greenland, S.; Senn, S.J.; Rothman, K.J.; Carlin, J.B.; Poole, C.; Goodman, S.N.; Altman, D.G. Statistical Tests, P Values, Confidence Intervals, and Power: A Guide to Misinterpretations. Eur. J. Epidemiol. 2016, 31, 337–350. [Google Scholar] [PubMed]


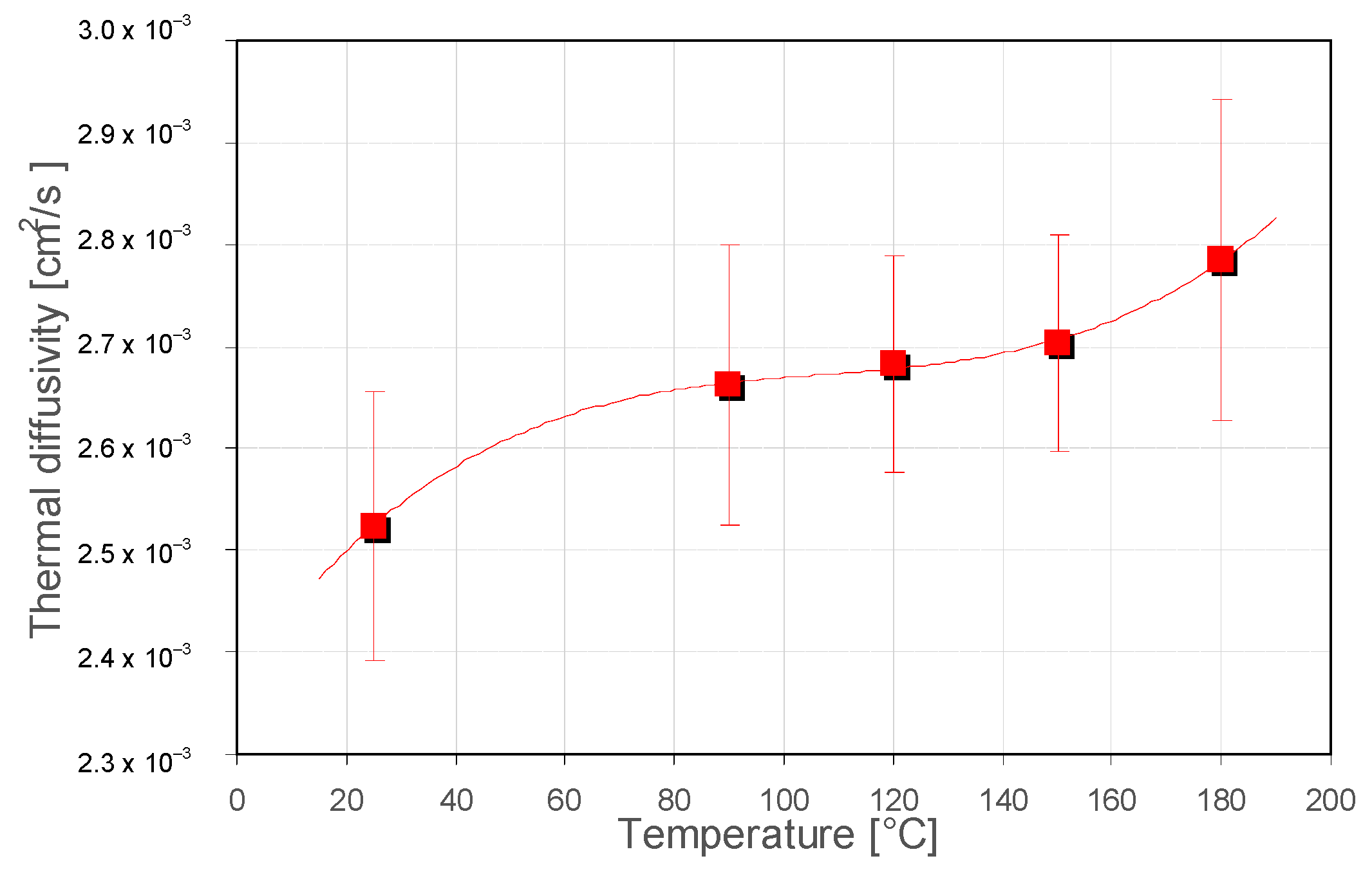

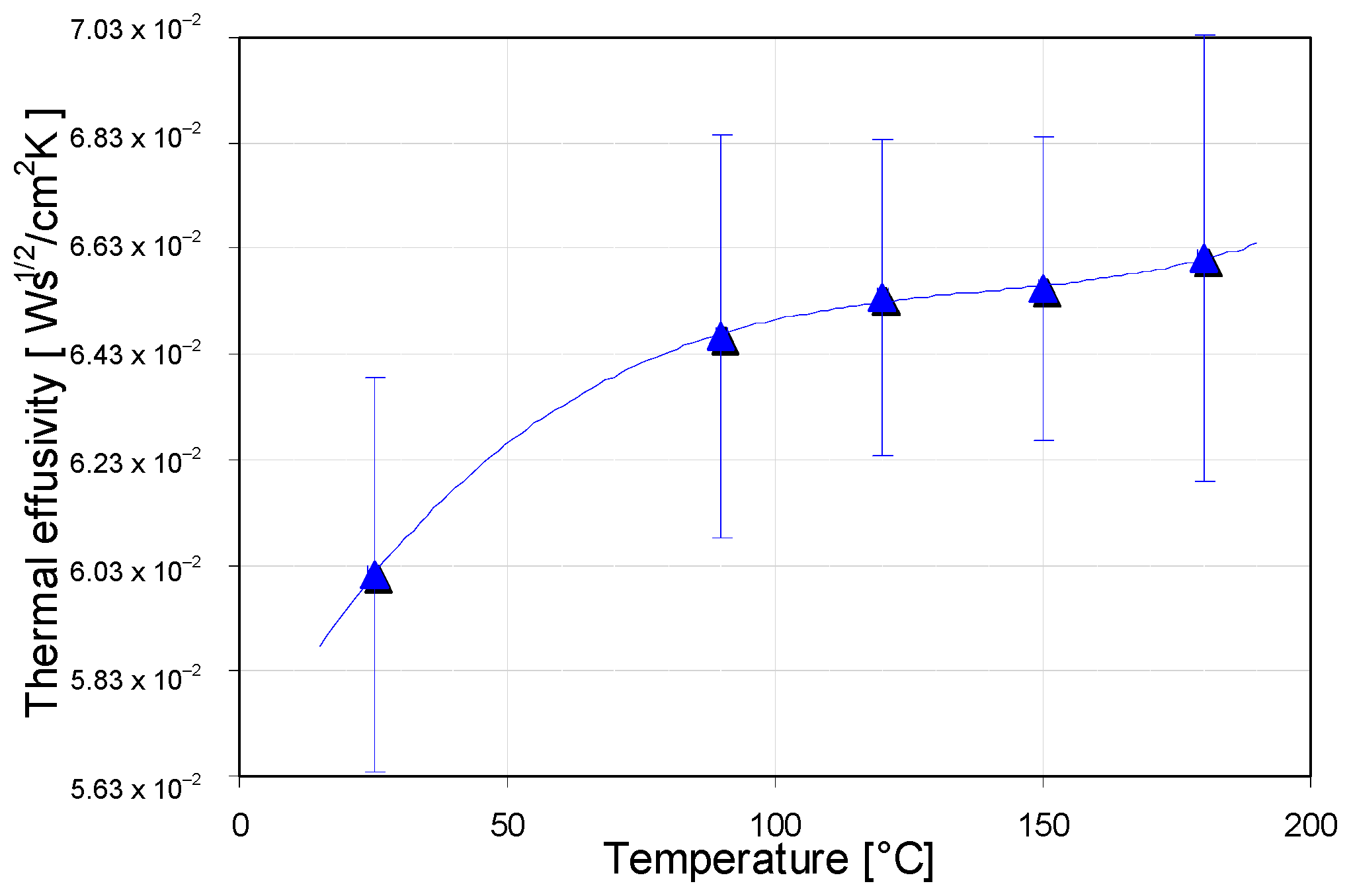
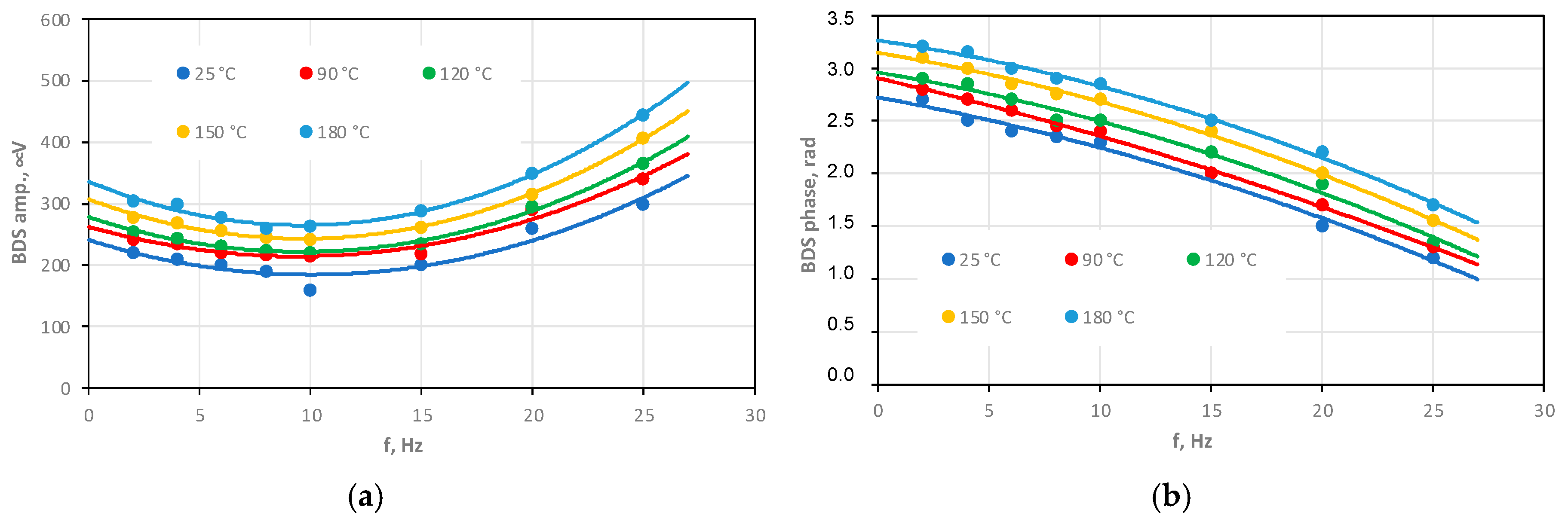
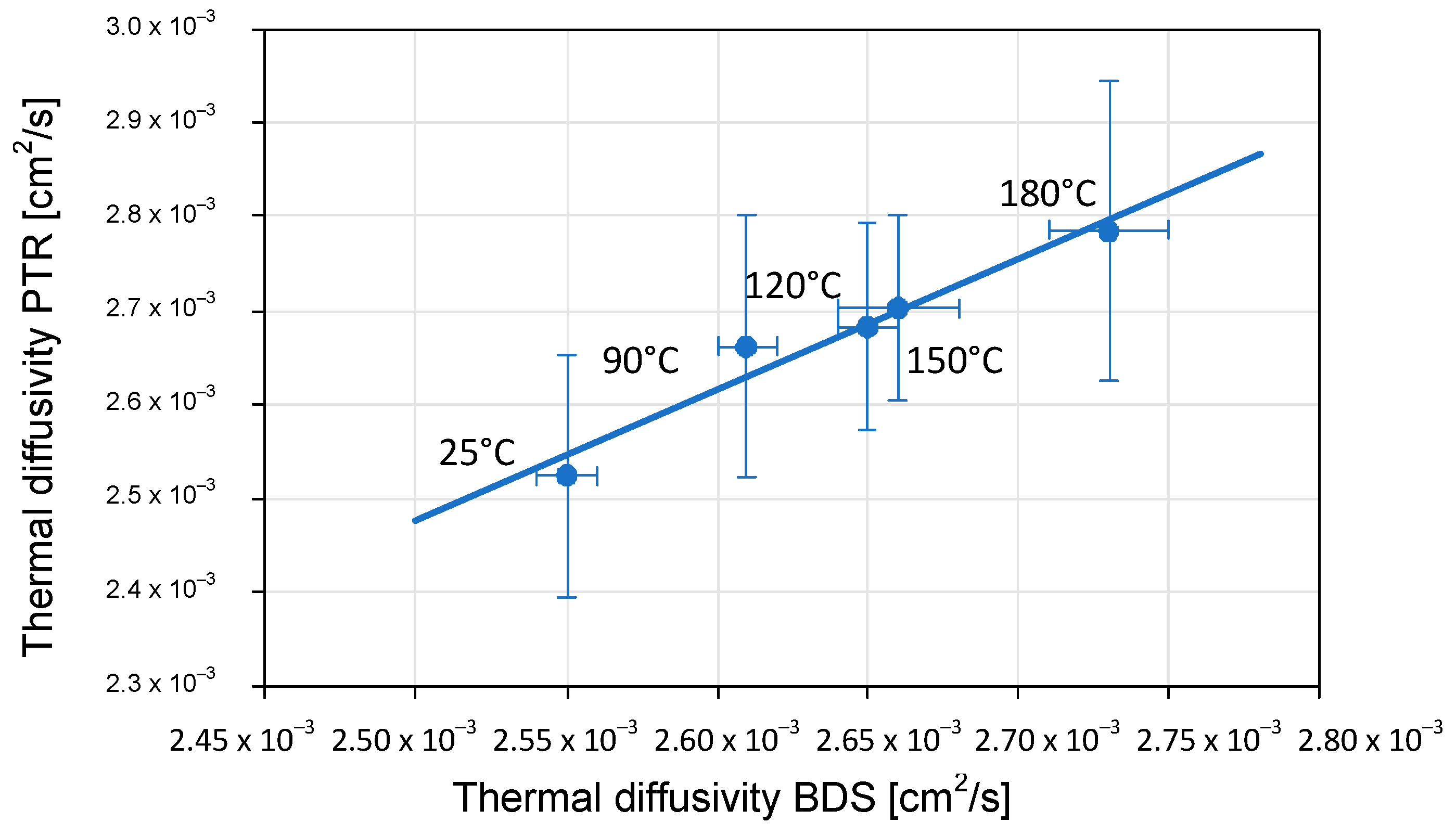
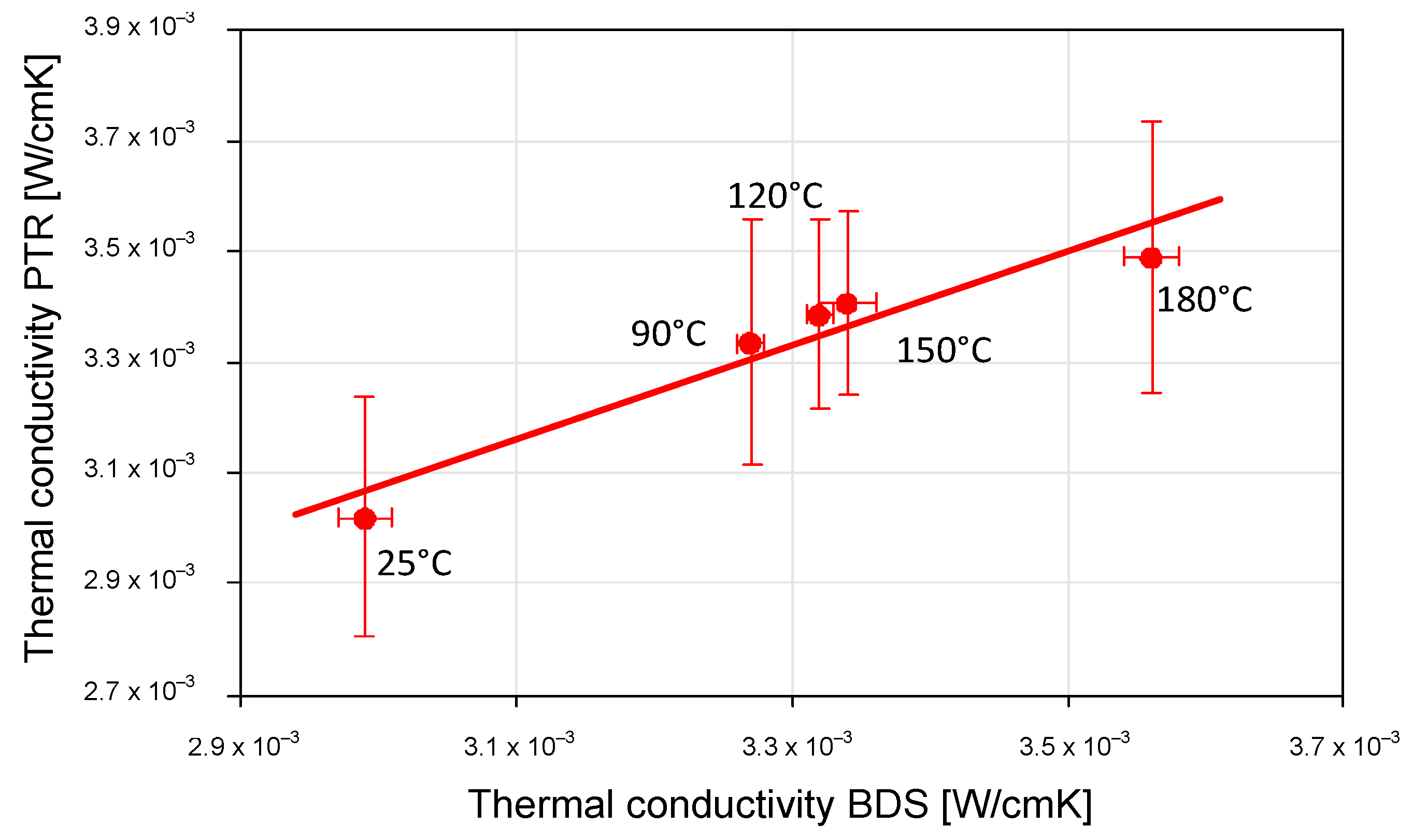
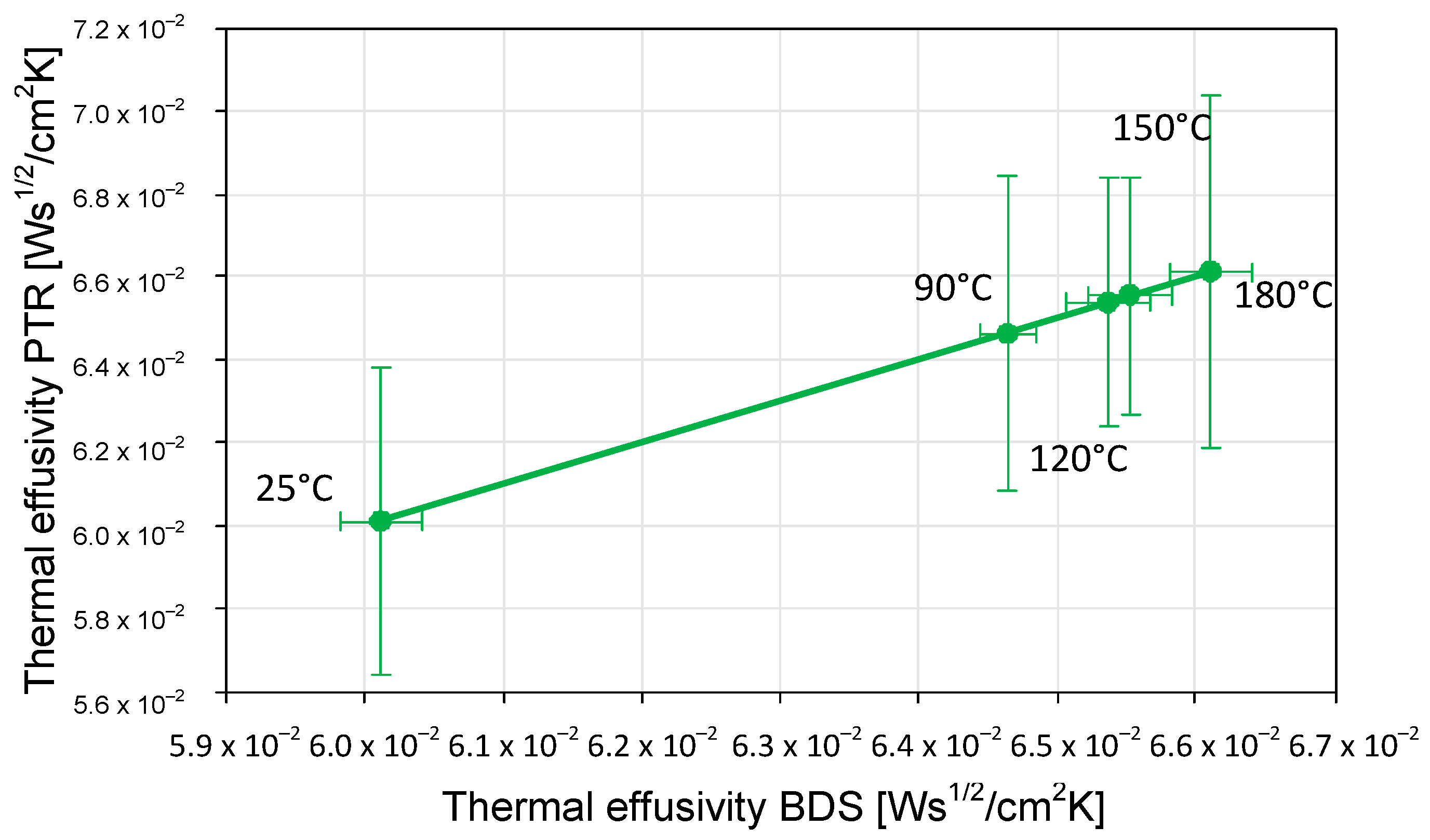
| Annealing Temperature [°C] | Thermal Diffusivity αc [cm2 s−1] | Thermal Conductivity λc [Wcm−1K−1] | Thermal Effusivity ec × 10−3 [Ws1/2cm−2K−1] |
|---|---|---|---|
| 25 | 2.52·10−3 ± 1.31·10−4 | 3.02·10−3 ± 2.15·10−4 | 6.01·10−2 ± 3.72·10−3 |
| 90 | 2.66·10−3 ± 1.37·10−4 | 3.33·10−3 ± 2.20·10−4 | 6.46·10−2 ± 3.81·10−3 |
| 120 | 2.68·10−3 ± 1.06·10−4 | 3.38·10−3 ± 1.71·10−4 | 6.53·10−2 ± 3.00·10−3 |
| 150 | 2.70·10−3 ± 1.06·10−4 | 3.40·10−3 ± 1.68·10−4 | 6.55·10−2 ± 2.88·10−3 |
| 180 | 2.78·10−3 ± 1.57·10−4 | 3.48·10−3 ± 2.46·10−4 | 6.61·10−2 ± 4.23·10−3 |
| Annealing Temperature [°C] | Thermal Diffusivity αc [cm2·s−1] | Thermal Conductivity λc [W·cm−1·K−1] | Thermal Effusivity ec × 10−3 [W·s1/2cm−2K−1] |
|---|---|---|---|
| 25 | 2.55·10−3 ± 0.1·10−4 | 2.99·10−3 ± 2·10−4 | 5.92·10−2 ± 0.03·10−3 |
| 90 | 2.61·10−3 ± 0.1·10−4 | 3.27·10−3 ± 1·10−4 | 6.40·10−2 ± 0.02·10−3 |
| 120 | 2.65·10−3 ± 0.1·10−4 | 3.32·10−3 ± 2·10−4 | 6.45·10−2 ± 0.03·10−3 |
| 150 | 2.66·10−3 ± 0.2·10−4 | 3.34·10−3 ± 1·10−4 | 6.48·10−2 ± 0.03·10−3 |
| 180 | 2.73·10−3 ± 0.2·10−4 | 3.56·10−3 ± 1·10−4 | 6.81·10−2 ± 0.03·10−3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chrobak, Ł.; Maliński, M.; Korte, D.; Janta-Lipińska, S. Theoretical and Experimental Studies of Thermal Parameters of Annealed Polybutyl Methacrylate–Siloxane Protective Coatings by Means of Nondestructive Photothermal Radiometry and Photothermal Beam Deflection Methods. Appl. Sci. 2025, 15, 3416. https://doi.org/10.3390/app15073416
Chrobak Ł, Maliński M, Korte D, Janta-Lipińska S. Theoretical and Experimental Studies of Thermal Parameters of Annealed Polybutyl Methacrylate–Siloxane Protective Coatings by Means of Nondestructive Photothermal Radiometry and Photothermal Beam Deflection Methods. Applied Sciences. 2025; 15(7):3416. https://doi.org/10.3390/app15073416
Chicago/Turabian StyleChrobak, Łukasz, Mirosław Maliński, Dorota Korte, and Sylwia Janta-Lipińska. 2025. "Theoretical and Experimental Studies of Thermal Parameters of Annealed Polybutyl Methacrylate–Siloxane Protective Coatings by Means of Nondestructive Photothermal Radiometry and Photothermal Beam Deflection Methods" Applied Sciences 15, no. 7: 3416. https://doi.org/10.3390/app15073416
APA StyleChrobak, Ł., Maliński, M., Korte, D., & Janta-Lipińska, S. (2025). Theoretical and Experimental Studies of Thermal Parameters of Annealed Polybutyl Methacrylate–Siloxane Protective Coatings by Means of Nondestructive Photothermal Radiometry and Photothermal Beam Deflection Methods. Applied Sciences, 15(7), 3416. https://doi.org/10.3390/app15073416







