Research on the Mechanism and Dynamic Characteristics of Intermittent Failure of Electrical Connectors
Abstract
1. Introduction
2. Analysis of the Electrical Connector Intermittent Failure Mechanism
2.1. Contact Resistance Model
2.2. Analysis of the Degradation of the Contact Resistance
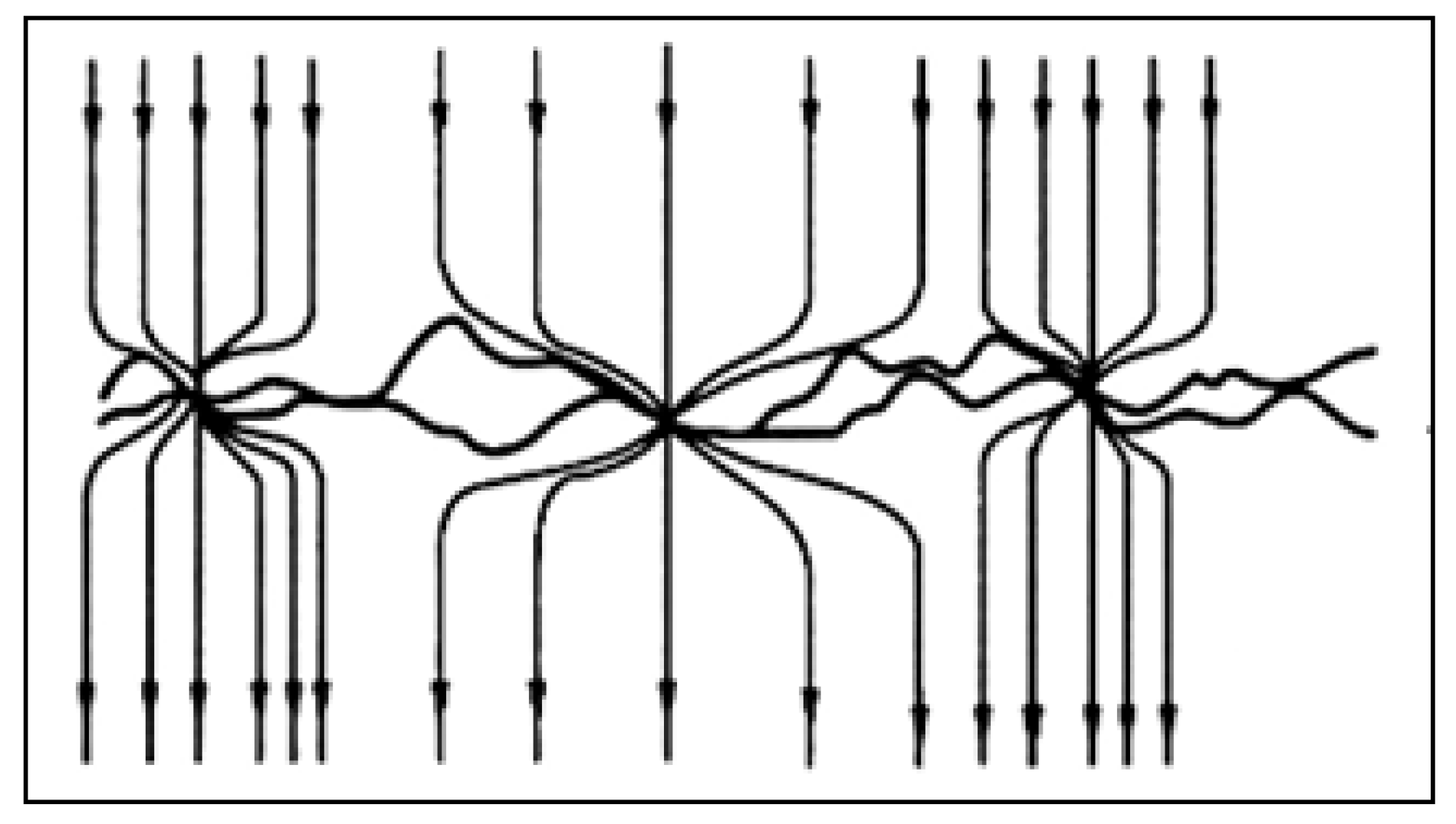
- Creep and stress relaxation. The creep and stress relaxation both lead to a decrease in the elastic deformation of connectors. According to the Hertz contact theory [32], the conductive area of the contact spot will decrease with the decrease in elastic deformation, resulting in a significant increase in contact resistance.
- Metal migration. The metal migration mainly causes variation in contact area, then contact resistance fluctuates.
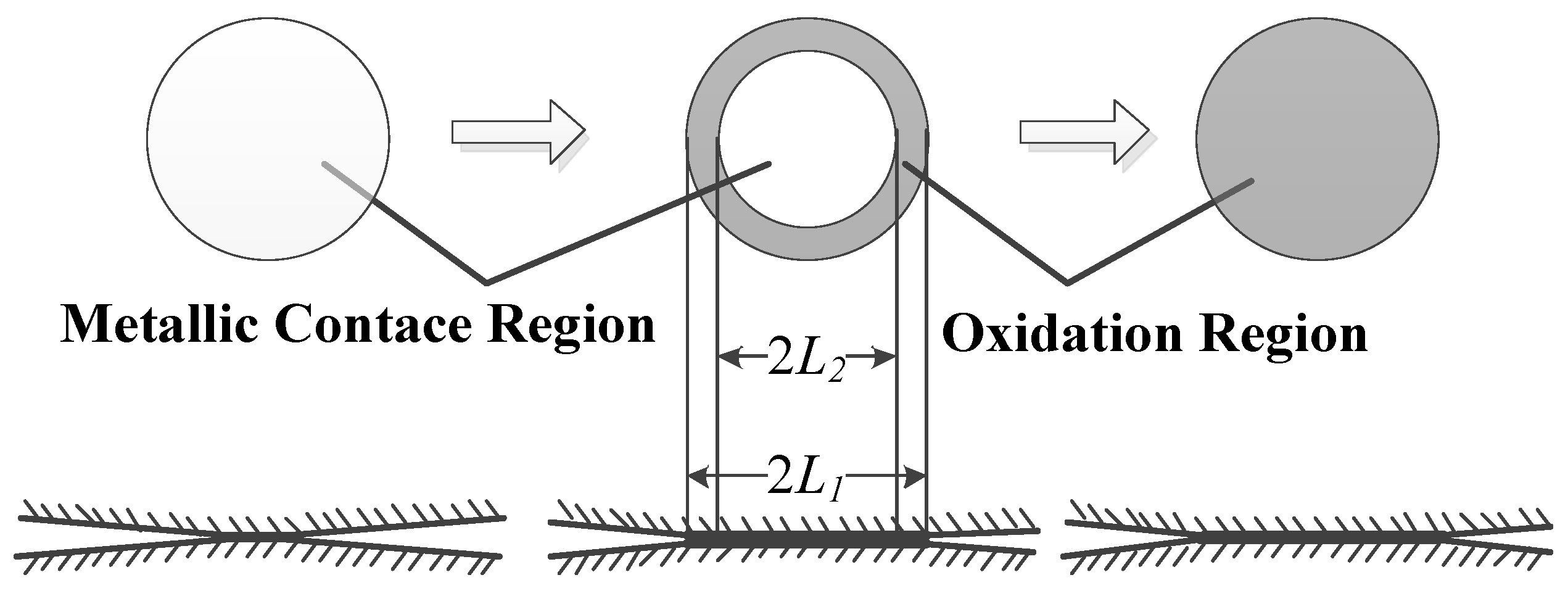
- Oxidation and corrosion. The contact area is easily corroded by oxygen. Thus, the oxidized film, which is composed of an insulating material, will cover the conductive spot gradually. Then, the size of the conductive spot will decrease, and the resistance will increase.
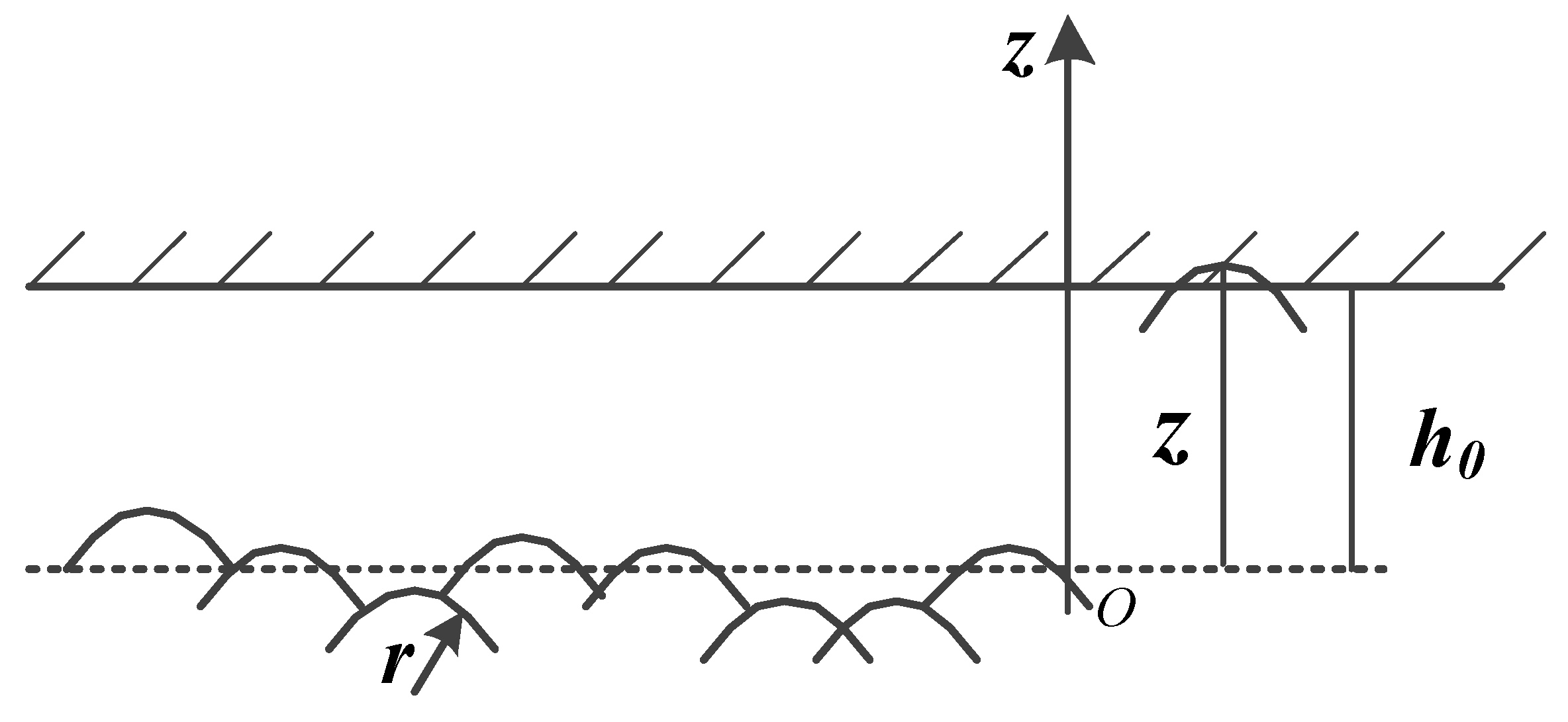
2.3. Electrical Contact Model Considering the Plowing Effect
2.4. The Intermittent Failure Mechanism of the Electrical Connector
2.5. Analysis of the Mechanism of Electrical Connector Intermittent Failure Induced by Vibration
3. Analysis of Intermittent Failure Dynamic Characteristics
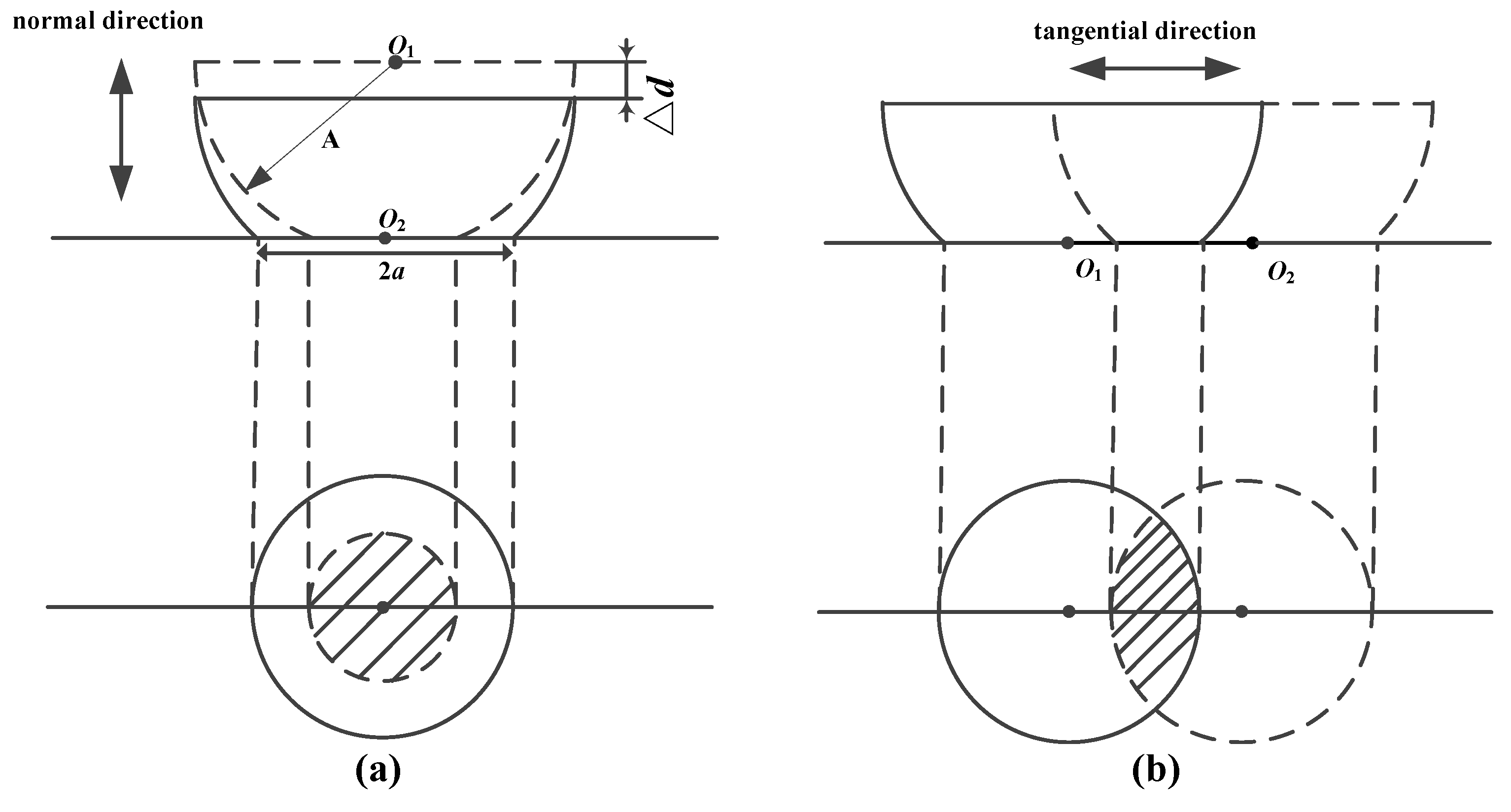
3.1. G-W Model
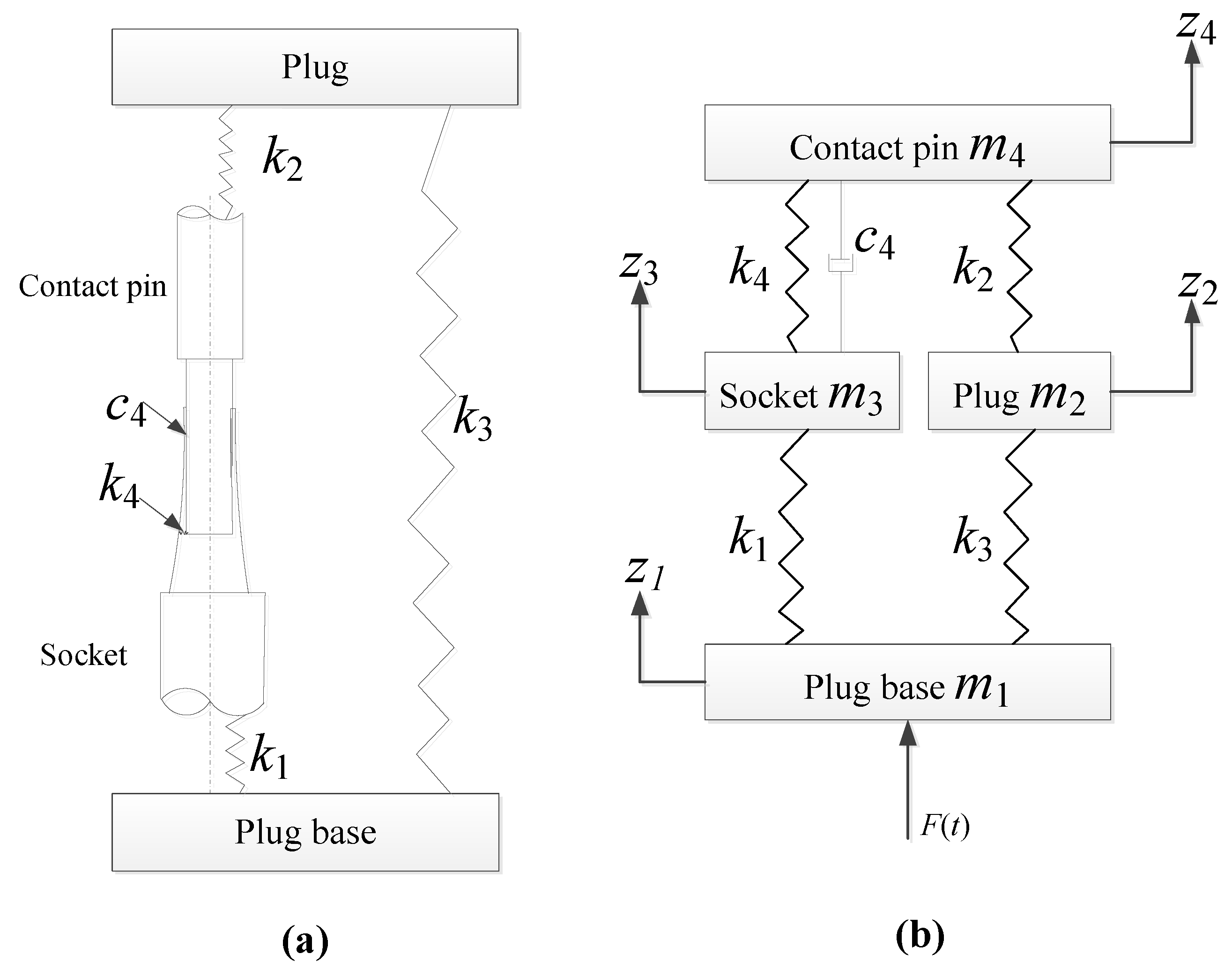
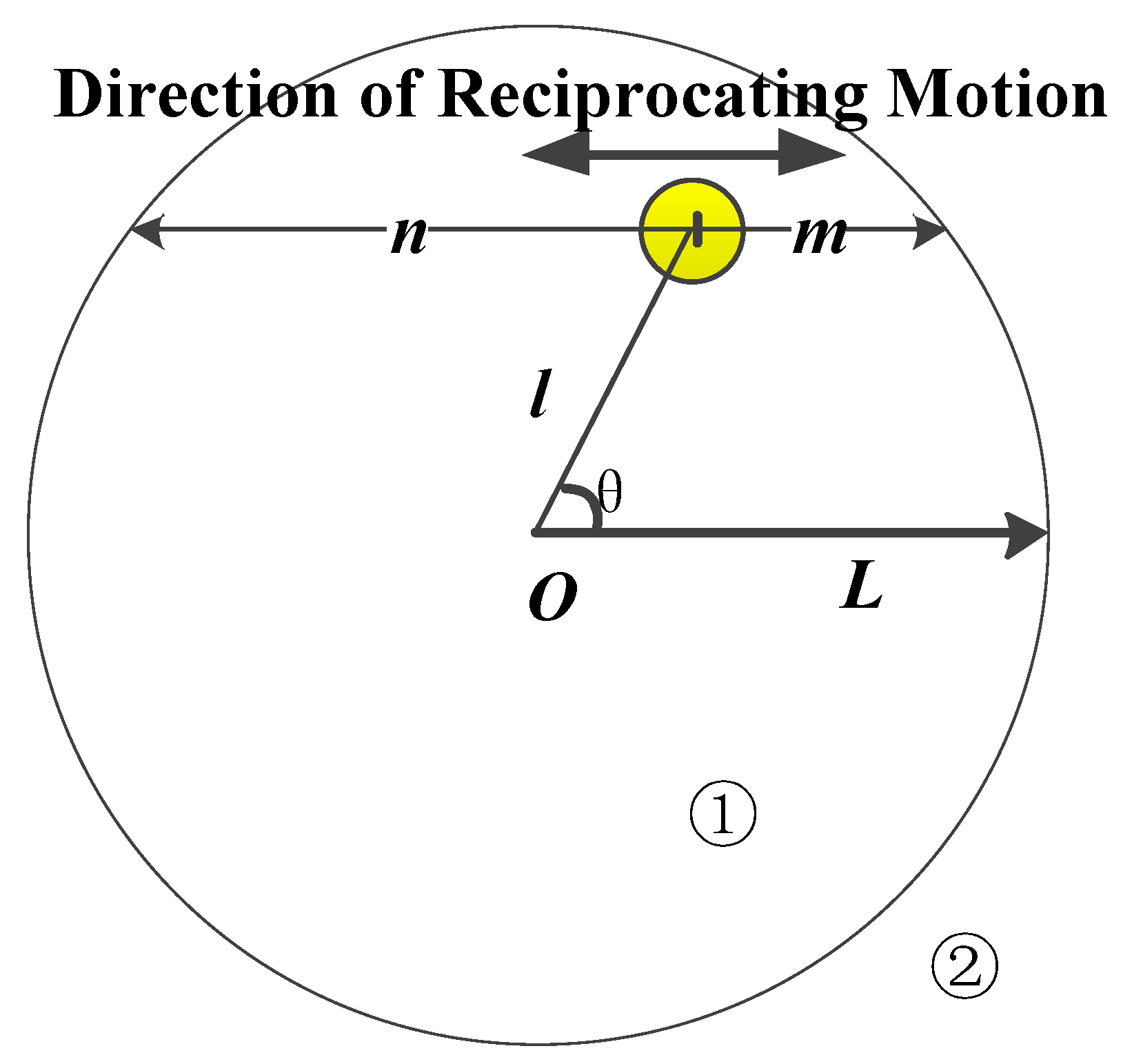
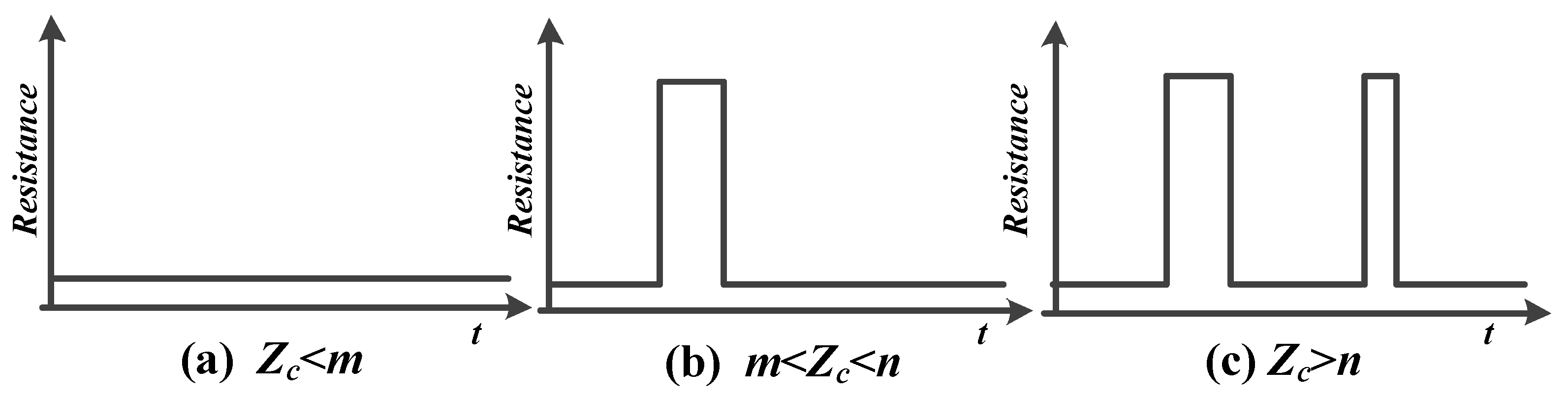
3.2. Parameter Framework of the Dynamic Model of Intermittent Failure
3.3. Dynamic Model of Intermittent Failure
4. Simulation Case
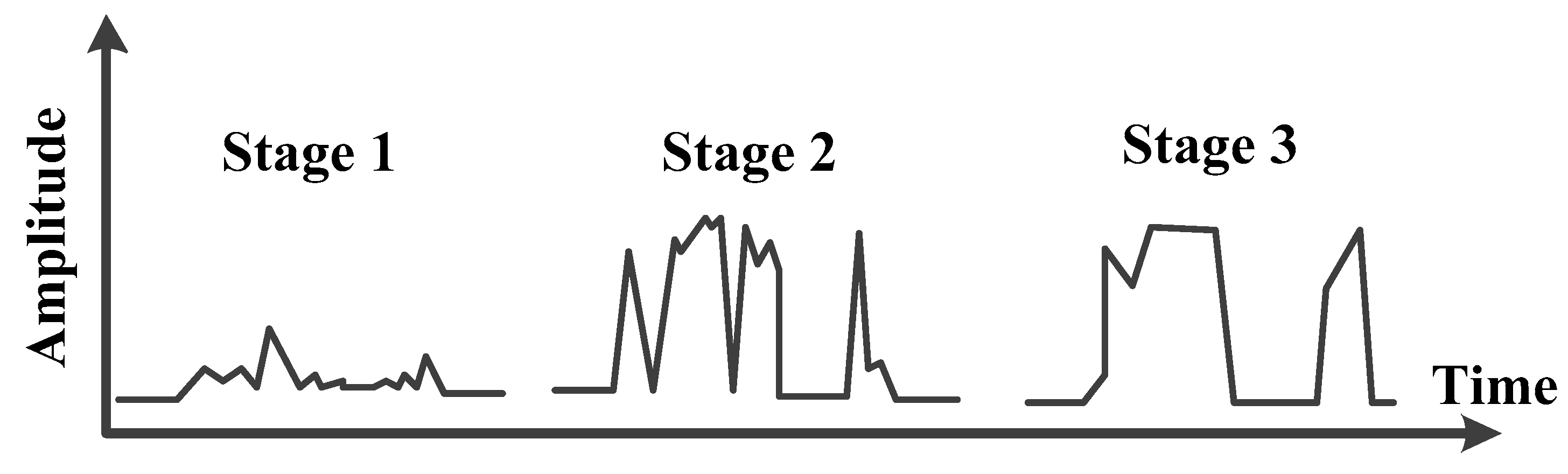
4.1. Dynamics of Intermittent Failure Under Different Degradation Stages
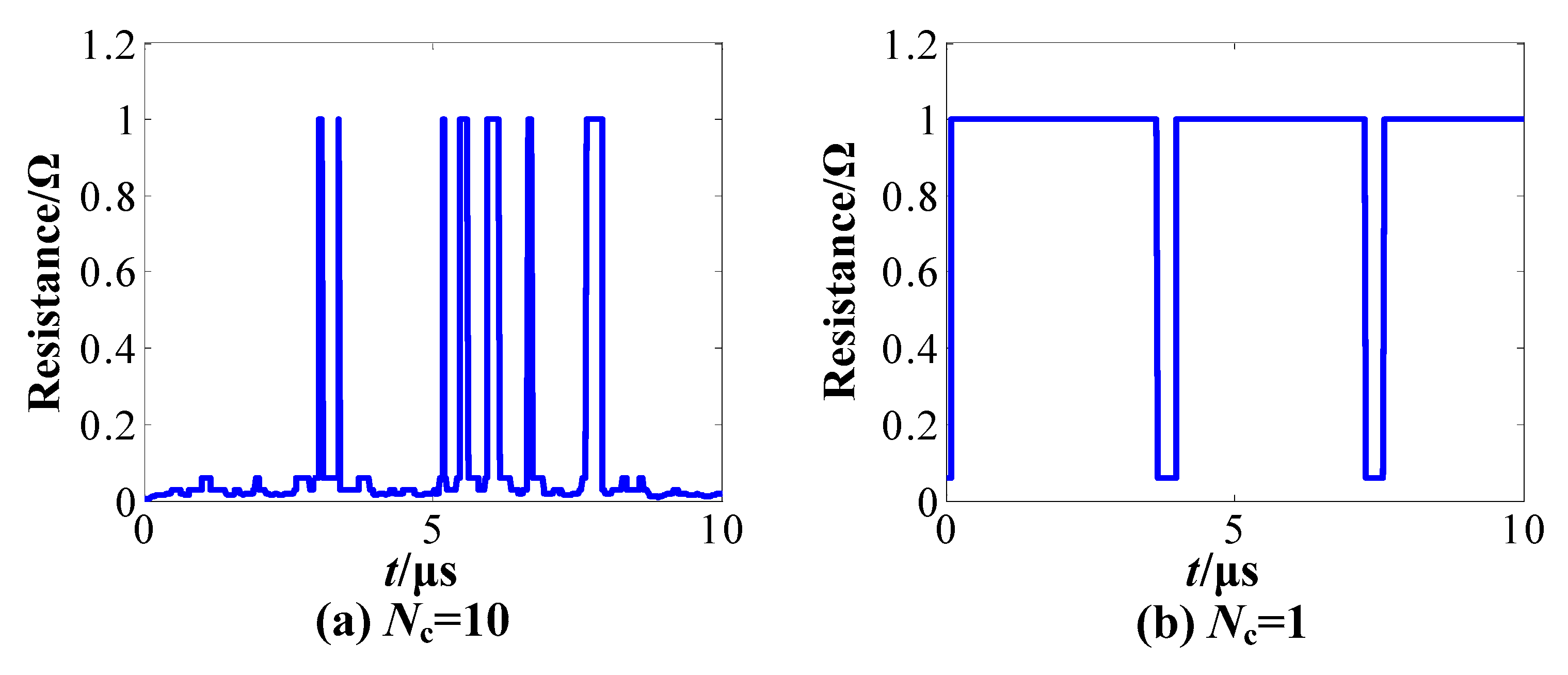
4.2. Dynamics of Intermittent Failure Under Different Contact Pressures
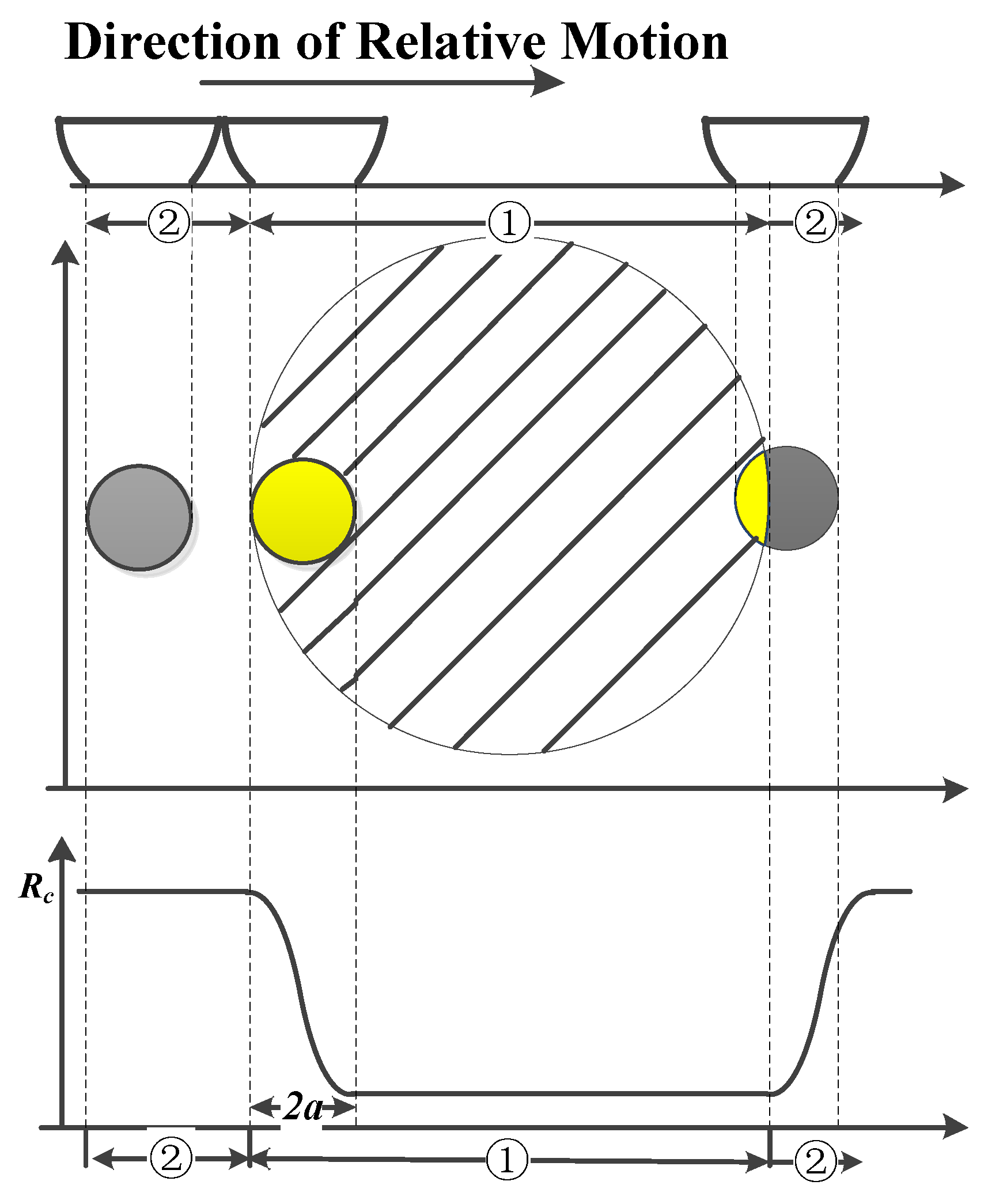
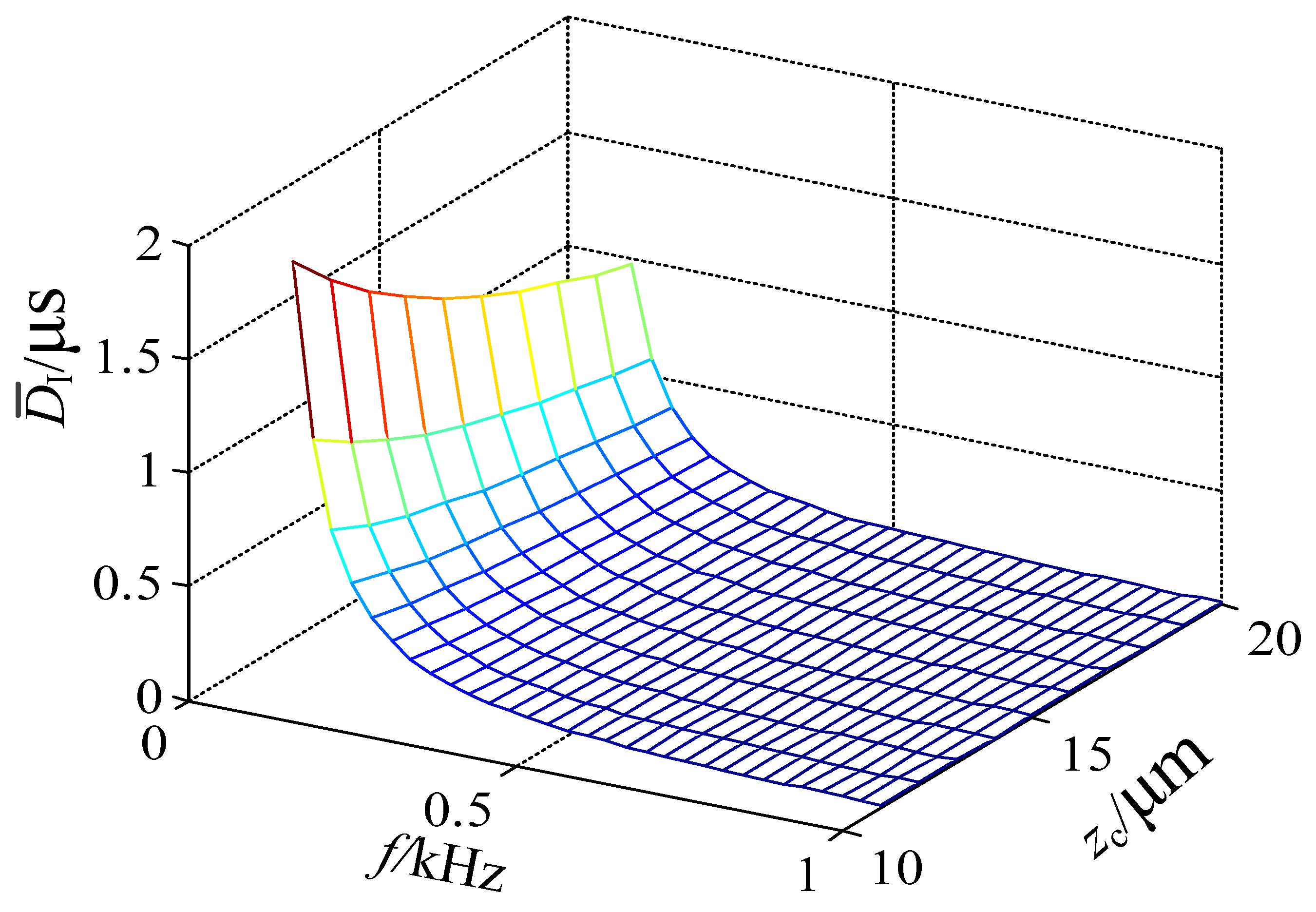
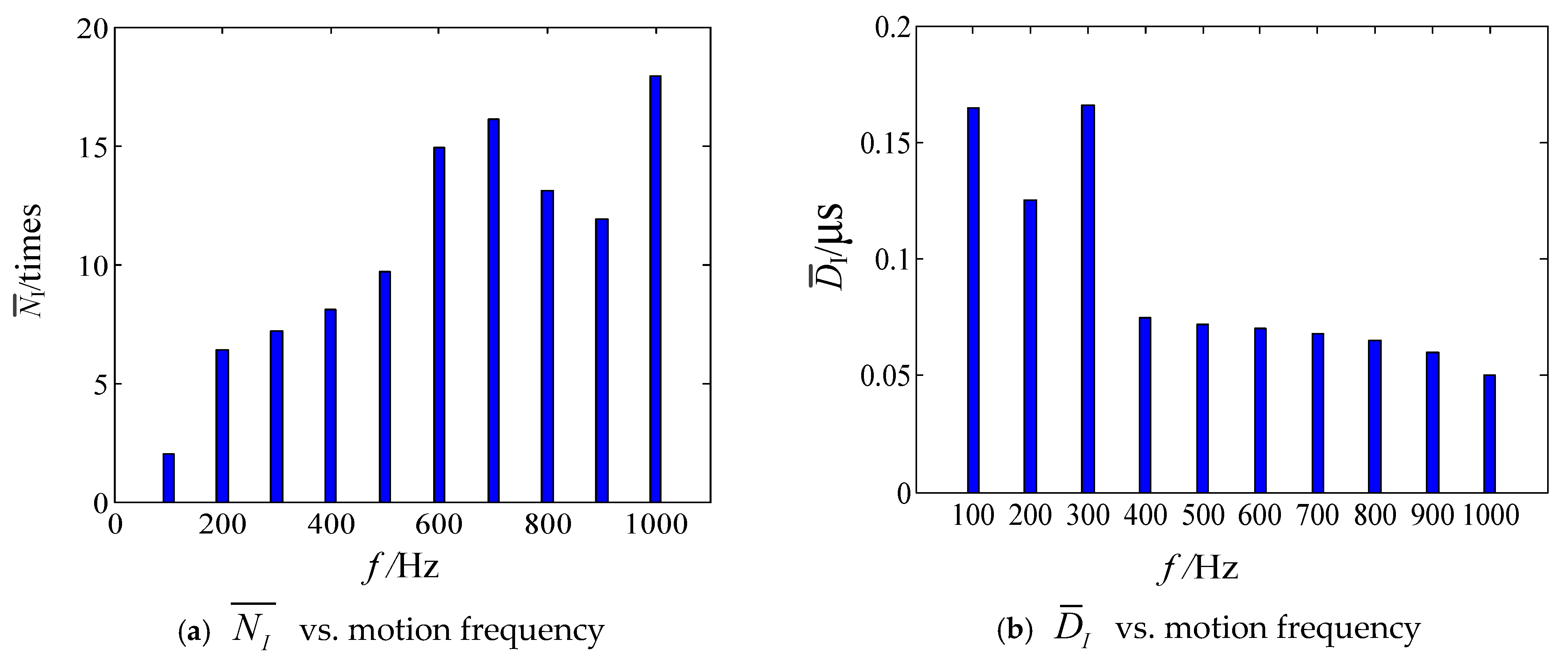
4.3. Dynamics of Intermittent Failure Under Different Relative Motion Parameters
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Arulvel, S.; Jain, A.; Kandasamy, J.; Singhal, M. Laser processing techniques for surface property enhancement: Focus on material advancement. Surf. Interfaces 2023, 42, 103293. [Google Scholar]
- Gu, D.; Shi, X.; Poprawe, R.; Bourell, D.L.; Setchi, R.; Zhu, J. Material-structure-performance integrated laser-metal additive manufacturing. Science 2021, 372, eabg1487. [Google Scholar] [CrossRef] [PubMed]
- Wei, C.; Zhang, Z.; Cheng, D.; Sun, Z.; Zhu, M.; Li, L. An overview of laser-based multiple metallic material additive manufacturing: From macro-to micro-scales. Int. J. Extrem. Manuf. 2020, 3, 012003. [Google Scholar] [CrossRef]
- Romic, I.; Augustin, G.; Bogdanic, B.; Bruketa, T.; Moric, T. Laser treatment of pilonidal disease: A systematic review. Lasers Med. Sci. 2022, 37, 723–732. [Google Scholar] [CrossRef] [PubMed]
- Farooq, A.; Alquaity, A.B.; Raza, M.; Nasir, E.F.; Yao, S.; Ren, W. Laser sensors for energy systems and process industries: Perspectives and directions. Prog. Energy Combust. Sci. 2022, 91, 100997. [Google Scholar] [CrossRef]
- Klotzkin, D.J. Introduction to Semiconductor Lasers for Optical Communications; Springer International Publishing: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- He, S.; Chen, S.; Zhao, Y.; Qi, N.; Zhan, X. Study on the intelligent model database modeling the laser welding for aerospace aluminum alloy. J. Manuf. Process. 2021, 63, 121–129. [Google Scholar] [CrossRef]
- Ren, Z.; Li, Q.; Qiu, B.; Zhang, J.; Li, X.; Xu, B.; Song, K.; Li, B. Failure mode characterizations of semiconductor lasers. AIP Adv. 2023, 13, 095310. [Google Scholar] [CrossRef]
- Guanjun, L.; Hui, Z.; Jing, Q.; Kehong, L.; Qinmu, S. Mechanism of intermittent failures in extreme vibration environment and online diagnosis technology. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2015, 229, 363–369. [Google Scholar] [CrossRef]
- Sharma, R.; Saluja, K.K. An implementation and analysis of a concurrent built-in self-test technique. In Proceedings of the 1988 The Eighteenth International Symposium on Fault-Tolerant Computing. Digest of Papers, Tokyo, Japan, 27–30 June 1988; IEEE: New York, NY, USA, 1988; pp. 164–169. [Google Scholar]
- Li, Q.; Lv, K.; Qiu, J.; Liu, G. Evaluation method of electrical connector performance based on intermittent failure. In Proceedings of the Prognostics and System Health Management Conference, Harbin, China, 9–12 July 2017; IEEE: New York, NY, USA, 2017; pp. 1–6. [Google Scholar]
- Wang, S.; Liu, Z.; Jia, Z.; Zhao, W.; Li, Z. Intermittent fault diagnosis for electronics-rich analog circuit systems based on multi-scale enhanced convolution transformer network with novel token fusion strategy. Expert Syst. Appl. 2024, 238, 121964. [Google Scholar] [CrossRef]
- Asaadi, M.; Izadi, I.; Hassanzadeh, A.; Yang, F. Assessment of alarm systems for mixture processes and intermittent faults. J. Process. Control. 2022, 114, 120–130. [Google Scholar] [CrossRef]
- Hockley, C.J. The Impact of No Fault Found (NFF) on Through Life Engineering Services. J. Qual. Maint. Eng. 2012, 18, 141–153. [Google Scholar] [CrossRef]
- Zhang, S.; Sheng, L.; Gao, M. Intermittent fault detection for delayed stochastic systems over sensor networks. J. Frankl. Inst. 2021, 358, 6878–6896. [Google Scholar] [CrossRef]
- Kothawade, S.; Chakraborty, K.; Roy, S.; Han, Y. Analysis of intermittent timing fault vulnerability. Microelectron. Reliab. 2012, 52, 1515–1522. [Google Scholar] [CrossRef]
- Fang, X.; Qu, J.; Chai, Y. Self-supervised intermittent fault detection for analog circuits guided by prior knowledge. Reliab. Eng. Syst. Saf. 2023, 233, 109108. [Google Scholar] [CrossRef]
- Aylstock, F.; Elerin, L.; Hintz, J.; Learoyd, C.; Press, R. Neural network false alarm filter, volume 2. Neural Netw. False Alarm. Filter 1994, 95, 30886. [Google Scholar]
- Cui, Y.; Shi, J.; Wang, Z. Intermittent failure process and false alarm interaction modelling of threshold-based monitoring built-in tests (BITs). Int. J. Prod. Res. 2016, 54, 1610–1626. [Google Scholar] [CrossRef]
- Carl, J.D.; Tantawy, A.; Biswas, G.; Koutsoukos, X.D. Detection and Estimation of Multiple Fault Profiles Using Generalized Likelihood Ratio Tests: A Case Study. In Proceedings of the 16th IFAC Symposium on System Identification, Brussels, Belgium, 11–13 July 2012; pp. 386–391. [Google Scholar]
- Kehong, L. Research on BIT False Alarm Reducing and Fault Prediction Technologies Based on Time Stress Analysis. Ph.D. Thesis, National University of Defense Technology, Changsha, China, 2018. [Google Scholar]
- Huakang, L.; Kehong, L.; Jing, Q.; Guanjun, L.; Bailiang, C. Selection of test paths for solder joint intermittent connection faults under DC stimulus. Int. J. Electron. 2018, 105, 1011–1024. [Google Scholar] [CrossRef]
- Deng, G.Q. Research on Key Technology of Intermittent Faults Diagnosis in Extreme Temperature Environment. Ph.D. Thesis, National University of Defense Technology, Changsha, China, 2013. [Google Scholar]
- Wang, Z.; Zhai, G.; Ren, W.; Huang, X.; Yu, Q. Research on Accelerated Storage Degradation Testing for Aerospace Electromagnetic Relay. In Proceedings of the IEEE, Holm Conference on Electrical Contacts, Portland, OR, USA, 23–26 September 2012; IEEE: New York, NY, USA, 2012; pp. 1–8. [Google Scholar]
- Antler, M. Survey of Contact Fretting in Electrical Connectors. IEEE Trans. Compon. Hybrids Manuf. Technol. 1985, 8, 87–104. [Google Scholar] [CrossRef]
- Queffelec, J.L.; Jamaa, N.B.; Travers, D.; Pethieu, G. Materials and contact shape studies for automobile connectors development. IEEE Trans. Compon. Hybrids Manuf. Technol. 1991, 14, 90–94. [Google Scholar] [CrossRef]
- Li, Q.; Gao, J.; Flowers, G.T.; Yi, W.; Jackson, R.L.; Hamilton, M. Investigation on vibration induced fretting in degraded contact interface. Microelectron. Reliab. 2022, 139, 114794. [Google Scholar] [CrossRef]
- Liu, X.-L.; Hu, S.-X.; Xiao, Q.; Deng, G.-H.; Zheng, Y.-T.; Gao, M.-S.; Zhang, D.; Cao, H.-Y.; Wang, Z.; Chen, D.-Y.; et al. An investigation of the electrical contact failure of JPT electric connectors used in automobiles under multiple stresses. Wear 2024, 552–553, 205458. [Google Scholar] [CrossRef]
- Liu, X.-L.; Cai, Z.-B.; He, J.-F.; Peng, J.-F.; Zhu, M.-H. Effect of elevated temperature on fretting wear under electric contact. Wear 2017, 376-377, 643–655. [Google Scholar] [CrossRef]
- Jemaa, N.B.; Carvou, E. Electrical contact behaviour of power connector during fretting vibration. In Proceedings of the 52nd IEEE Holm Conference on Electrical Contacts, Montreal, QC, Canada, 25–27 September 2006; IEEE: New York, NY, USA, 2007; pp. 263–266. [Google Scholar]
- El Mossouess, S.; Carvou, E.; El Abdi, R.; Benjemâa, N.; Obame, H.; Doublet, L.; Rodari, T. Analysis of temporal and spatial contact voltage fluctuation during fretting in automotive connectors. In Proceedings of the International Conference on Entertainment Computing, ICEC 2014, Dresden, Germany, 22–26 June 2014; VDE: Berlin, Germany, 2014; pp. 1–5. [Google Scholar]
- Braunovic, M.; Konchits, V.V.; Myshkin, N.K. Electrical Contacts: Fundamentals, Applications and Technology; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Li, Q.; Lyu, K.; Qiu, J.; Liu, G. Research on intermittent failure re-presentation of electrical connector based on accelerated test. Proc. Inst. Mech. Eng. Part O J. Risk Reliab. 2018, 233, 317–327. [Google Scholar] [CrossRef]
- Shen, Q.; Lv, K.; Liu, G.; Qiu, J. Dynamic Performance of Electrical Connector Contact Resistance and Intermittent Fault Under Vibration. IEEE Trans. Compon. Packag. Manuf. Technol. 2017, 8, 216–225. [Google Scholar] [CrossRef]
- Li, Q. Research on Key Technologies of Intermittent Failures Duplication and Evaluation for Electrical Connectors. Ph.D. Thesis, National University of Defense Technology, Changsha, China, 2018. [Google Scholar]




| Parameter | Value |
|---|---|
| 1.45 μm | |
| Nc | 7 |
| L | 10 μm |
| l | U(0,L) |
| θ | U(0,2π) |
| Zc | 16 μm |
| f | 100 Hz |
| tc | 10 μs |
| Δt | 10−3 μs |
| Rth | 0.8 Ω |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, L.; Zhang, X.; Lan, X. Research on the Mechanism and Dynamic Characteristics of Intermittent Failure of Electrical Connectors. Appl. Sci. 2025, 15, 3328. https://doi.org/10.3390/app15063328
Ren L, Zhang X, Lan X. Research on the Mechanism and Dynamic Characteristics of Intermittent Failure of Electrical Connectors. Applied Sciences. 2025; 15(6):3328. https://doi.org/10.3390/app15063328
Chicago/Turabian StyleRen, Libing, Xiaofei Zhang, and Xuke Lan. 2025. "Research on the Mechanism and Dynamic Characteristics of Intermittent Failure of Electrical Connectors" Applied Sciences 15, no. 6: 3328. https://doi.org/10.3390/app15063328
APA StyleRen, L., Zhang, X., & Lan, X. (2025). Research on the Mechanism and Dynamic Characteristics of Intermittent Failure of Electrical Connectors. Applied Sciences, 15(6), 3328. https://doi.org/10.3390/app15063328






