Full-Scale Simulation and Experimental Study of Heat Transfer in Landing Gear Brake Discs for Medium-Sized Passenger Aircraft
Abstract
Featured Application
Abstract
1. Introduction
2. Model Description
2.1. Full-Size Brake Disc Geometry Mode
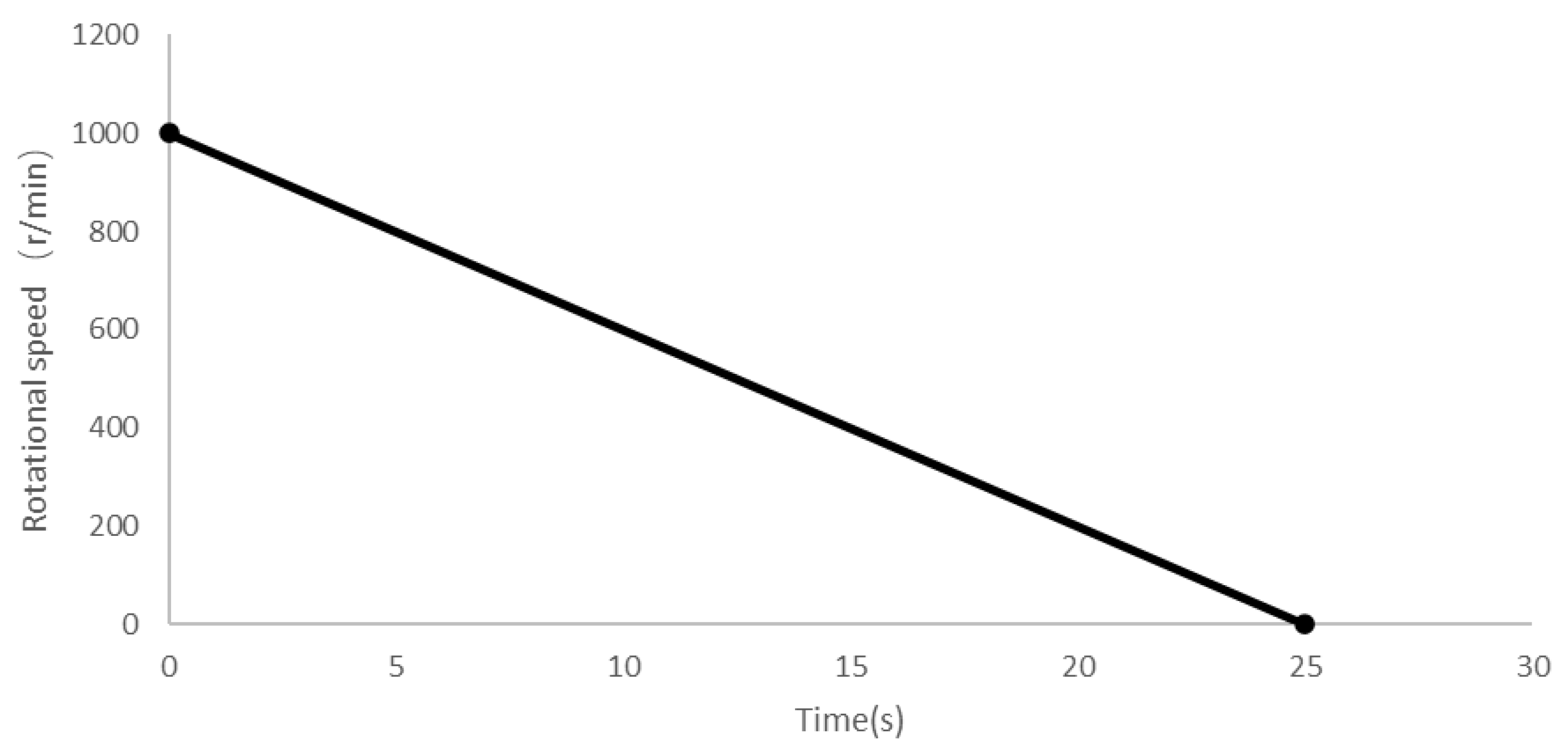
2.2. Braking Condition Analysis
3. Simulation Model and Simulation Methods
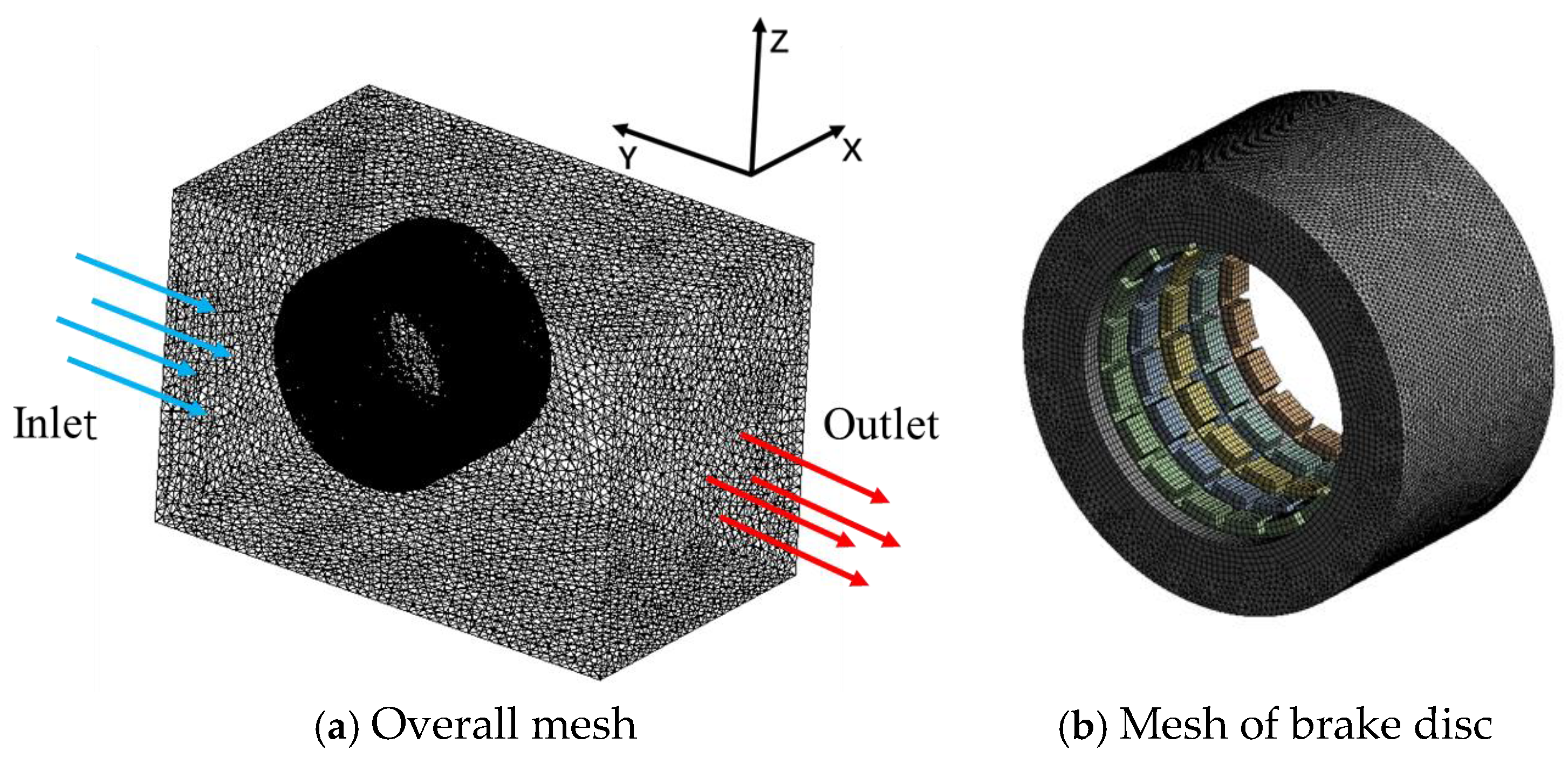
3.1. Finite Element Model
3.2. Mesh
3.3. Governing Equations and Boundary Condition
- (1)
- The rotational angular velocity of the brake disc solid domain and the cylindrical fluid calculation area is equal to the rotational angular velocity of the aircraft axis, and the rotation axis is the X axis.
- (2)
- Inlet boundary: the air inlet surface is set as the velocity inlet, and the air flow direction is perpendicular to the surface, along the Y axis, and the wind speed is equal to the aircraft braking speed, simulating the wind speed that the aircraft is subjected to during actual braking.
- (3)
- Outlet boundary: the air outlet surface is set as the pressure outlet, the pressure is equal to the atmospheric pressure, and the wall temperature is set to 300 K.
- (4)
- Wall conditions: the two friction walls of the brake disc solid domain are applied with time-varying heat flux density, and the initial temperature of the other fluid–solid coupling walls is set to 300 K. The other four surfaces of the rectangular fluid domain are stationary walls, and the thermodynamic boundaries are set as adiabatic.
3.4. Numerical Method
4. Results and Discussion
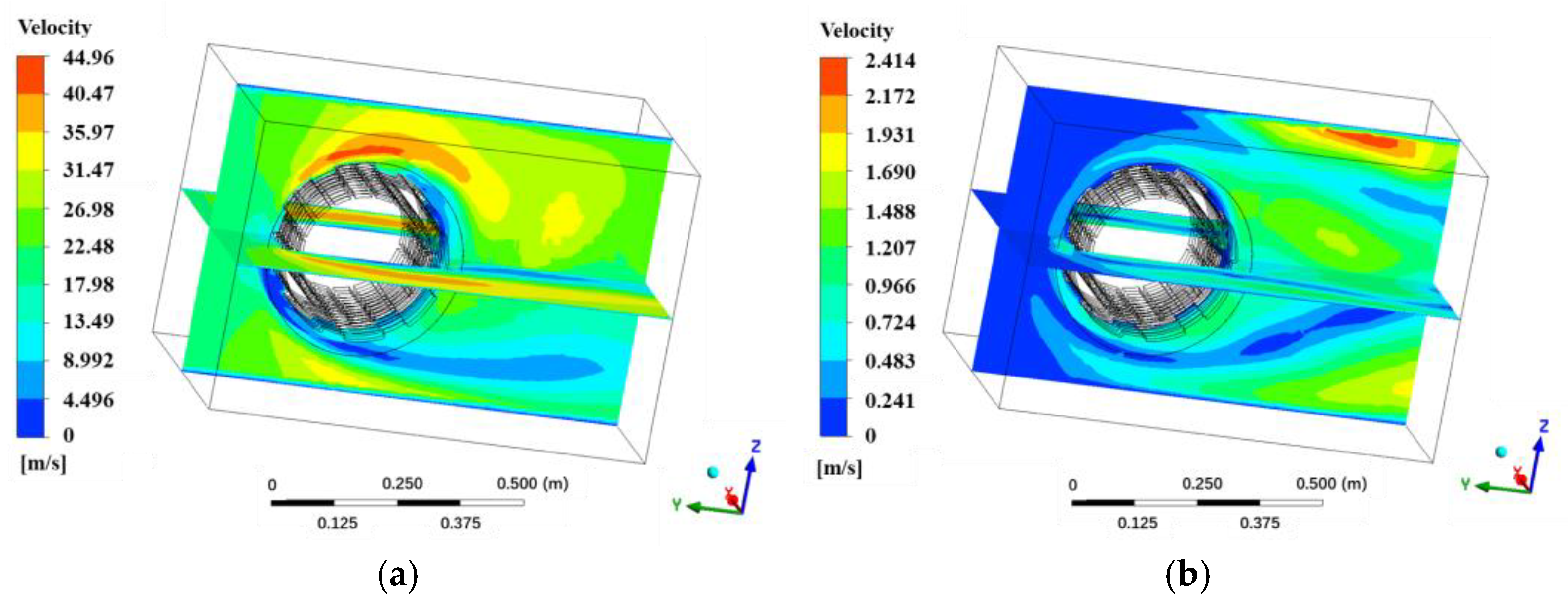
4.1. Analysis of the Airflow Field Around the Brake Disc
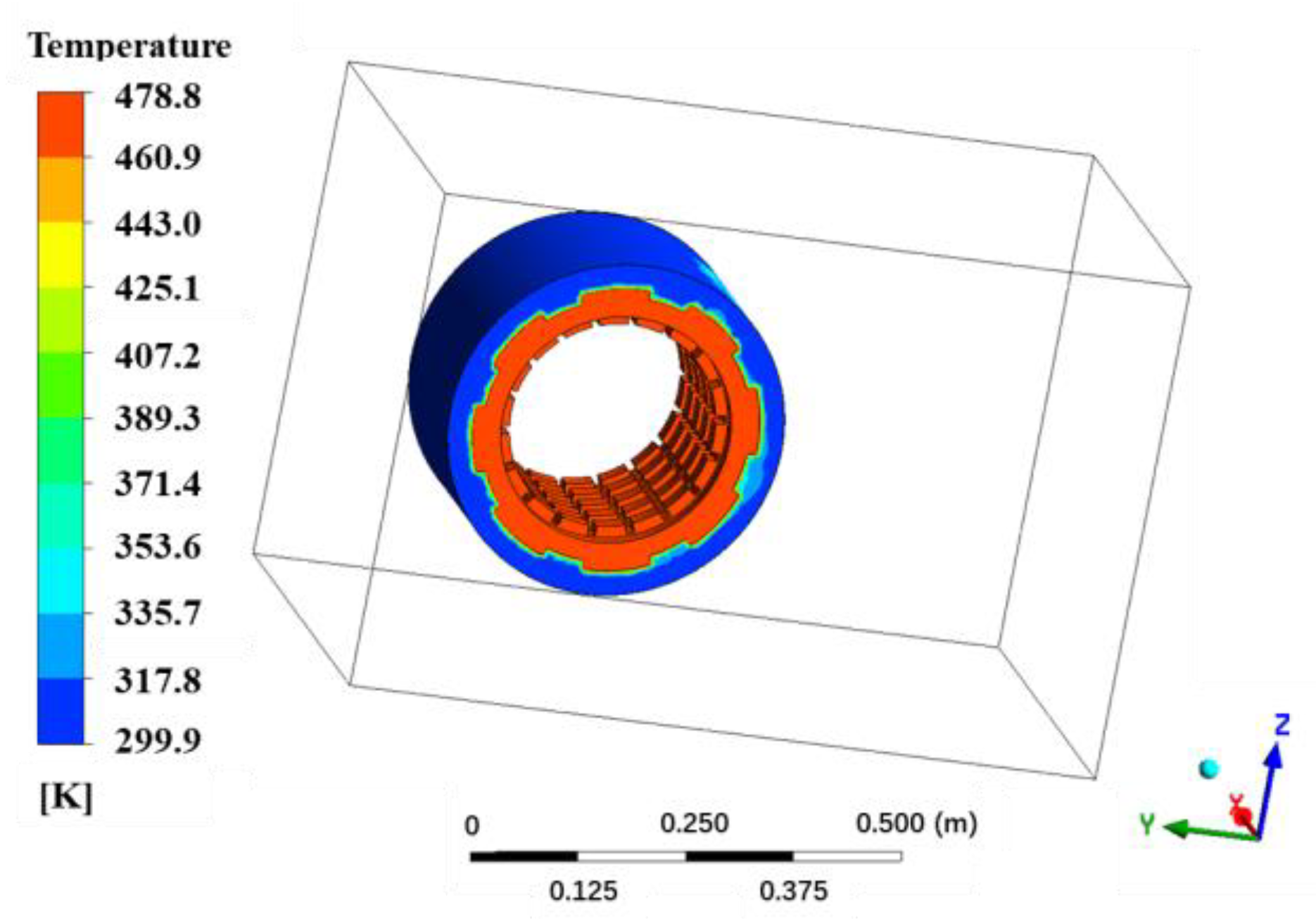
4.2. Analysis of Brake Disc Temperature Field
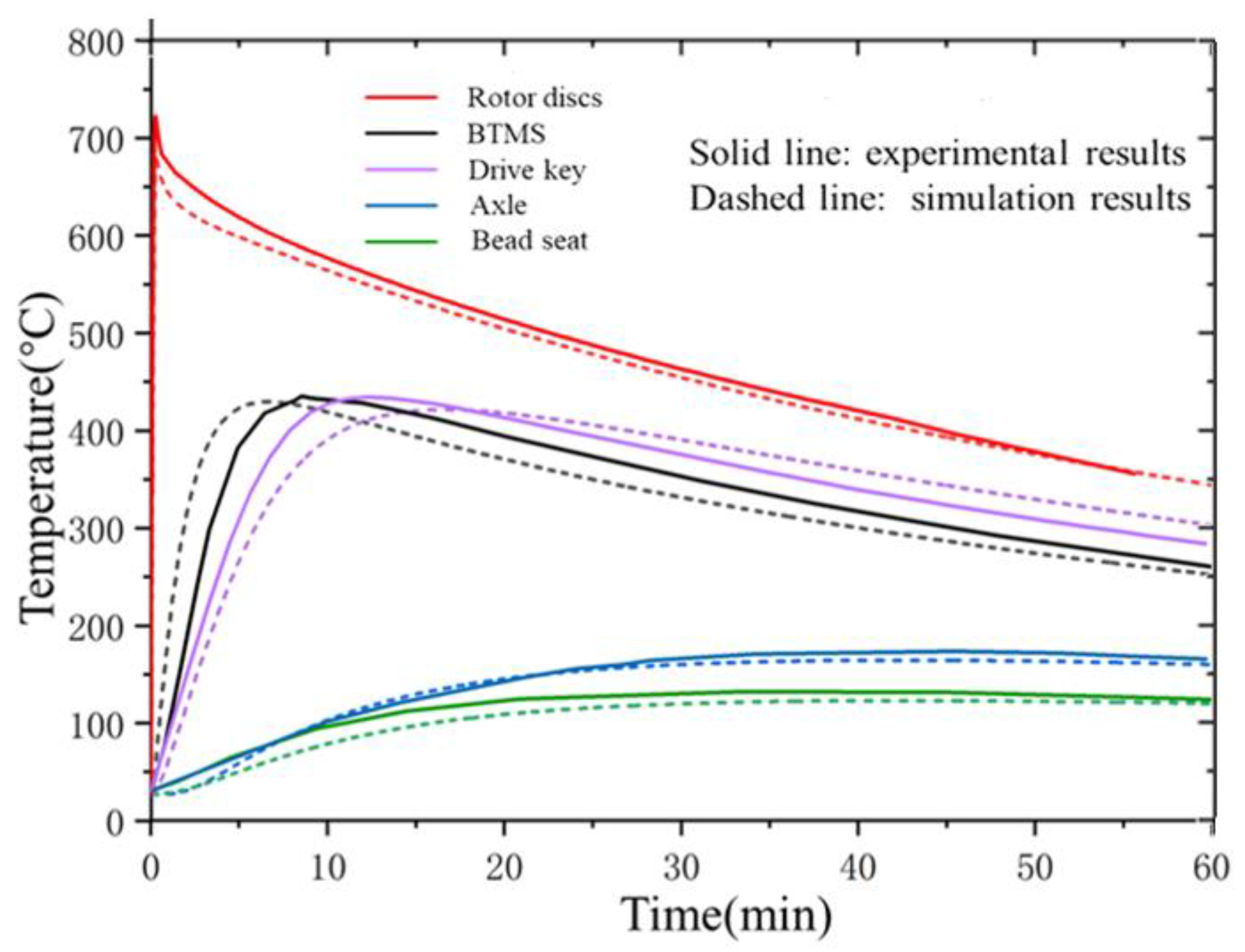
4.3. Experiment Analysis
5. Conclusions
- (1)
- The surface temperature of the brake disc rapidly increases during the braking process, creating a significant temperature gradient, especially in the early stages of braking. This temperature gradient may cause thermal stress and material fatigue. Therefore, during design, special attention should be paid to material selection and structural design in areas with high temperature gradients to prevent cracks or deformations caused by thermal stress.
- (2)
- The air flow has a significant impact on the heat dissipation efficiency of brake discs, and the higher the flow speed, the better the heat dissipation effect. Even in the later stages of braking, air disturbance remains significant, indicating that forced convection (such as with a fan) at low speeds or when stopped can significantly improve heat dissipation efficiency. Therefore, the introduction of forced convection devices in practical applications can effectively accelerate the cooling of brake discs.
- (3)
- Experimental verification shows that the maximum relative error between numerical simulation results and experimental data is about 4.5%, indicating the high reliability of the model. The errors mainly come from the simplification of material properties, boundary condition settings, and experimental measurement uncertainties. To improve simulation accuracy, future research can adopt material thermophysical parameters that vary with temperature and optimize boundary conditions, especially the dynamic changes in convective heat transfer coefficients.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pevec, M.; Potrc, I.; Bombek, G.; Vranesevic, D. Prediction of the cooling factors of a vehicle brake disc and its influence on the results of a thermal numerical simulation. Int. J. Automot. Technol. 2012, 13, 725–733. [Google Scholar] [CrossRef]
- Deren, E.A.; Volkhonsky, A.E.; Konoval, D.V. Computer simulation of heat transfer processes in aircraft landing gear braking mechanisms. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1111, 012018. [Google Scholar] [CrossRef]
- Schmidt, D.L.; Davidson, K.E.; Theibert, L.S. Unique applications of carbon-carbon composite materials (part three). Sampe J. 1999, 35, 47–55. [Google Scholar]
- Afzal, A.; Abdul Mujeebu, M. Thermo-mechanical and structural performances of automobile disc brakes: A review of numerical and experimental studies. Arch. Comput. Methods Eng. 2019, 26, 1489–1513. [Google Scholar] [CrossRef]
- Bauzin, J.-G.; Laraqi, N. Three-dimensional analytical calculation of the temperature in a brake disc of a high-speed train. Appl. Therm. Eng. 2019, 154, 668–675. [Google Scholar] [CrossRef]
- Singh, S.K.; Abbassi, H.; Tamamidis, P. 3D investigation into the thermal behavior of the wet multi-disk axle brake of an off-highway machinery. Appl. Therm. Eng. 2018, 136, 576–588. [Google Scholar] [CrossRef]
- Belhocine, A.; Omar, W.Z.W. CFD analysis of the brake disc and the wheel house through air flow: Predictions of Surface heat transfer coefficients (STHC) during braking operation. J. Mech. Sci. Technol. 2018, 32, 481–490. [Google Scholar] [CrossRef]
- Kennedy, F.E., Jr.; Ling, F. A thermal, thermoelastic, and wear simulation of a high-energy sliding contact problem. J. Lubr. Technol. 1974, 96, 497–505. [Google Scholar] [CrossRef]
- Qi, H.S.; Day, A.J. Investigation of disc/pad interface temperatures in friction braking. Wear 2007, 262, 505–513. [Google Scholar] [CrossRef]
- Grzes, P. Maximum temperature of the disc during repeated braking applications. Adv. Mech. Eng. 2019, 11, 1687814019837826. [Google Scholar] [CrossRef]
- Nosko, A.L.; Mozalevb, V.V.; Nosko, A.P.; Suvorov, A.V.; Lebedeva, V.N. Calculation of temperature of carbon disks of aircraft brakes with account of heat exchange with the environment. J. Frict. Wear 2012, 33, 233–238. [Google Scholar] [CrossRef]
- Lei, L.; Yi, M.; Xu, H. 3-D temperature field for C/C composite braking discs. Acta Mater. Compos. Sin. 2009, 26, 113–117. [Google Scholar]
- Peszynska-Bialczyk, K.; Anderson, K.; Szymanski, T.; Krkoska, M.; Filipet, P. Thermal analysis of bulk carbon–carbon composite and friction products derived from it during simulated aircraft braking. Carbon 2007, 45, 524–530. [Google Scholar] [CrossRef]
- Bauzin, J.; Keruzore, N.; Laraqi, N.; Gapin, A.; Diebold, J. Identification of the heat flux generated by friction in an aircraft braking system. Int. J. Therm. Sci. 2018, 130, 449–456. [Google Scholar] [CrossRef]
- Cant, R.S.; Ahmed, U.; Fang, J.; Chakarborty, N.; Nivarti, G.; Moulinec, C.; Emerson, D.R. An unstructured adaptive mesh refinement approach for computational fluid dynamics of reacting flows. J. Comput. Phys. 2022, 468, 111480. [Google Scholar] [CrossRef]
- Shen, H.; Liu, C.; Yang, L.; Wang, Y. Pore-scale numerical investigation on comprehensive heat transfer performance of homogeneous and graded metal foam heat sinks. Therm. Sci. 2024, 28, 1529–1544. [Google Scholar] [CrossRef]


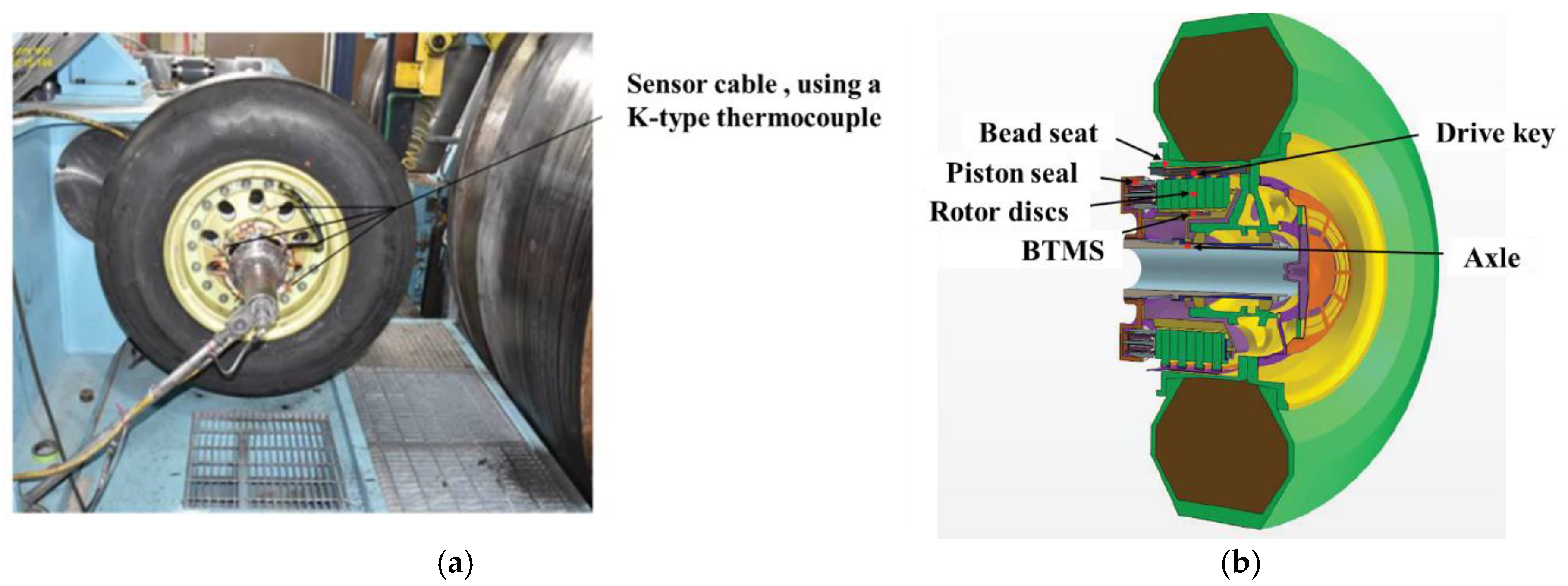

| Structure Parameters | Value |
|---|---|
| Outer diameter of the rotor disc (mm) | 341.25 |
| Inner diameter of the rotor disc (mm) | 273.34 |
| Thickness of the rotor disc (mm) | 20 |
| Outer diameter of the stator disc (mm) | 316.78 |
| Inner diameter of the stator disc (mm) | 245.01 |
| Thickness of the stator disc (mm) | 23.5 |
| Thermophysical Parameters | Value |
|---|---|
| Density ρ (kg/m3) | 2000 |
| Elastic modulus E (GPa) | 82 |
| Poisson’s ratio | 0.3 |
| Specific heat capacity c (J/(kg °C)) | 1000 |
| Thermal conductivity k (W/(m·°C)) | 40 |
| (10−6/°C) | 5 |
| (MPa) | 600 |
| Parameters | Value |
|---|---|
| Initial speed v0 (m/s) | 36.7 |
| Braking distance s (m) | 458.75 |
| (m/s2) | −1.468 |
| Gravitational acceleration g (m/s2) | 9.8 |
| Braking time t (s) | 25 |
| Number of brake discs n | 5 |
| Aircraft wheel radius Rw (mm) | 350 |
| Friction coefficient f | 0.3 |
| (m2) | 0.2013 |
| t (s) | v (m/s) | (rad/s) | q (W/m2) |
|---|---|---|---|
| 0 | 36.7 | 104.86 | 80,090,208.97 |
| 2.5 | 33.03 | 94.37 | 72,081,188.07 |
| 5 | 29.36 | 83.89 | 64,072,167.18 |
| 7.5 | 25.69 | 73.40 | 56,063,146.28 |
| 10 | 22.02 | 62.91 | 48,054,125.38 |
| 12.5 | 18.35 | 52.43 | 40,045,104.48 |
| 15 | 14.68 | 41.94 | 32,036,083.59 |
| 17.5 | 11.01 | 31.46 | 24,027,062.69 |
| 20 | 7.34 | 20.97 | 16,018,041.79 |
| 22.5 | 3.67 | 10.49 | 8,009,020.90 |
| 25 | 0 | 0 | 0 |
| Material | Density (kg/m3) | Heat Capacity (J/(kg·K)) | Thermal Conductivity (W/(m·K)) | Viscosity (kg/(m·s)) |
|---|---|---|---|---|
| Fluid material, air | 1.225 | 1006.43 | 0.0242 | 1.7894 × 10−5 |
| Solid material, C/C | 2000 | 1000 | 40 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, Q.; Shen, H.; Li, B.; Jiao, Z. Full-Scale Simulation and Experimental Study of Heat Transfer in Landing Gear Brake Discs for Medium-Sized Passenger Aircraft. Appl. Sci. 2025, 15, 3023. https://doi.org/10.3390/app15063023
Meng Q, Shen H, Li B, Jiao Z. Full-Scale Simulation and Experimental Study of Heat Transfer in Landing Gear Brake Discs for Medium-Sized Passenger Aircraft. Applied Sciences. 2025; 15(6):3023. https://doi.org/10.3390/app15063023
Chicago/Turabian StyleMeng, Qingtang, Hangming Shen, Bingchu Li, and Zongxia Jiao. 2025. "Full-Scale Simulation and Experimental Study of Heat Transfer in Landing Gear Brake Discs for Medium-Sized Passenger Aircraft" Applied Sciences 15, no. 6: 3023. https://doi.org/10.3390/app15063023
APA StyleMeng, Q., Shen, H., Li, B., & Jiao, Z. (2025). Full-Scale Simulation and Experimental Study of Heat Transfer in Landing Gear Brake Discs for Medium-Sized Passenger Aircraft. Applied Sciences, 15(6), 3023. https://doi.org/10.3390/app15063023






