Experimental and Analytical Study on Multiscale Cushioning and Energy Absorption of Aluminum Foam at Different Strain Rates

Abstract

In this research, the compressive behavior of closed-cell aluminum foam was examined across a broad strain rate spectrum, spanning from 0.005 s⁻¹ to 2000 s⁻¹. Utilizing an enhanced Hopkinson bar apparatus, the deformation and fracture mechanisms of the foam were captured through high-speed videography. Additionally, a microscale finite element model was developed to elucidate the dynamic deformation characteristics of aluminum foam at multiple scales. The findings indicate that the material’s response is highly strain rate-dependent, with both stress levels and energy absorption efficiency escalating as the strain rate rises. Moreover, the damage progression in aluminum foam manifests as a progressive collapse pattern.

1. Introduction

Aluminum foam is a porous material produced by the foaming process of aluminum or aluminum alloys [1,2,3]. Its unique microstructure endows it with extensive application potential in numerous fields [4,5,6]. Composed of a continuous metal matrix and dispersed air phase, aluminum foam features closed-cell or open-cell structures, and combines excellent properties such as low density, sound absorption, energy absorption, vibration damping, thermal insulation, and electromagnetic shielding [7,8,9]. These characteristics have led to its widespread use in industries like automotive manufacturing [10,11], aerospace [12,13,14,15,16,17], defense, electronics [11], architectural acoustics [18], and petrochemicals. Compared with traditional metal materials, although aluminum foam has a lower yield strength, its longer plateau stage and stronger energy absorption capacity provide unique advantages for its application in protective structures.

In recent years, many scholars have conducted in-depth research on the mechanical properties of aluminum foam [19,20,21,22,23]. PAKA et al. [24] analyzed in detail the load-displacement response characteristics of aluminum foam under compressive loads through uniaxial and biaxial compression tests. Gibson et al. [25] quasi-static compression tests showed that aluminum foam has a wide yield plateau during compression, and can absorb a large amount of energy at an approximately constant stress on this plateau. PAUL et al. [26] further revealed the strain rate sensitivity of closed-cell aluminum foam, finding that its energy absorption capacity increases with the increase of strain rate. Miran Ulbin et al. [27] observed significant changes in the internal pore structure of cylindrical foam samples during deformation using micro-computed tomography and CT image analysis. Samples with smaller spatial variations in porosity can withstand greater strain until failure under compressive loads. Lin Jing et al. [28] research indicated that the crushing stress and plateau stress of foam metals both increase with the increase of initial density, and the relationship between them is basically a power function. Movahedi et al. [29] explored the temperature effects on the compressive mechanical behavior of closed-cell aluminum foam, providing theoretical basis for its application in high-temperature environments. Liu et al. [30] investigated the strain rate sensitivity of aluminum foams by numerical simulation and found that their mechanical properties changed significantly with increasing strain rate.

Despite the wide application of aluminum foam in many fields, its application in the packaging engineering field, especially in the packaging protection of valuable goods under special conditions (such as energy absorption protection under free fall and collision conditions), is still relatively limited. In addition, the mechanical properties and energy absorption efficiency of aluminum foam-polyurethane composite materials under quasi-static compression are affected by relative density and pore size, which provides new ideas for the application of aluminum foam in packaging protection. Given this, the present study aims to systematically investigate the lightweight and high-toughness properties of closed-cell aluminum foam under static and dynamic loads. By conducting quasi-static and dynamic compression experiments at room temperature, and combining the establishment of constitutive models and damage research, the strain rate dependence of its mechanical properties will be revealed. Moreover, this study will also observe the deformation and fracture behavior under high-speed loading, in order to provide scientific guidance for the structural design of packaging protection engineering, and promote the application development of aluminum foam in the field of packaging protection.

2. Materials and Methods

2.1. Materials and Sample Design

The specimens used in the experiments were provided by Rongda New Materials Technology Co., Ltd. in Liaoning Province, Shantou, China. These specimens were produced by processing industrial pure aluminum through a direct melt foaming technique. In previous studies, relative density has been used as a key indicator to evaluate closed-cell aluminum foam, defined as the ratio of the specimen density (ρ0) to the density of the base material (ρs), where the base material is pure aluminum. The relative density of the closed-cell aluminum foam used in this experiment is 0.5 g/cm³. However, it should be noted that due to production errors, the relative density of the specimens exhibits some variability. The experimental specimens are divided into two categories: one for static compression tests, with sizes of 20 mm × 20 mm × 20 mm, and the other for dynamic loading tests, with dimensions of ∅20 mm × 10 mm. Figure 1 displays the appearance of these two types of closed-cell aluminum foam specimens.

To ensure the consistency of the samples used in the study, multiple specimens were tested under identical conditions. The stress-strain curves of five different samples are presented in Figure 2, showing the results obtained at a strain rate of 0.01 s⁻¹. As shown in the figure, the stress-strain curves of the five samples exhibit very similar trends, indicating that the samples have consistent mechanical properties. The curves show a clear three-stage characteristic: elastic stage, stress plateau stage, and densification stage. The yield stress, plateau stress, and densification strain are consistent across all samples, with only minor variations observed, which can be attributed to normal experimental fluctuations. The high degree of similarity in the stress-strain curves of the different samples demonstrates that the foam cell structure was controlled effectively and that the samples were consistent in their mechanical properties.

2.2. Quasi-Static Compression Device for Closed-Cell Aluminum Foam

Experiments were conducted using a testing machine equipped with high-precision force sensors, the schematic diagram of the device is shown in Figure 3. The loading rate was controlled by adjusting the displacement of the indenter to ensure the accuracy and repeatability of the experimental process. All tests were strictly performed in accordance with a loading strain rate set at 0.1 s⁻¹, which effectively simulates the quasi-static mechanical behavior of the material in practical applications. To ensure the reliability and consistency of the experimental data, each test condition was repeated three times, and the data were statistically analyzed. For data points with significant deviations, additional tests were conducted to eliminate the influence of random factors on the experimental results. In each test, the closed-cell aluminum foam specimens were compressed to a nominal strain exceeding 80%, a range that fully captures the complete compression response process, from elastic deformation to plastic deformation and finally to densification. By recording and analyzing the stress-strain curves, key mechanical properties of the closed-cell aluminum foam, such as compressive strength, energy absorption capacity, and deformation mechanisms, were further investigated.

2.3. Split-Hopkinson Pressure Bar (SHPB) System

This study employs an improved Split Hopkinson Pressure Bar (SHPB) device to conduct dynamic impact loading experiments on closed-cell aluminum foam samples (Φ40 mm × 20 mm). The SHPB device is an experimental setup widely used for investigating the dynamic mechanical properties of porous materials under high strain rates and can effectively simulate the mechanical response of materials under extreme conditions such as impact or explosion. As shown in Figure 4, the experimental setup mainly consists of the following components: the striker bar, the incident bar, the transmitted bar, and the absorber bar. During the experiment, the striker bar is accelerated by a pneumatic device to impact the incident bar, generating a stress pulse that is transmitted to the specimen. The incident and transmitted bars record the incident wave, reflected wave, and transmitted wave signals, respectively. By analyzing these signals, the stress-strain relationship, energy absorption characteristics, and deformation mechanisms of the specimen under dynamic loading can be calculated. To ensure the accuracy and reliability of the experimental data, the device was strictly calibrated before the experiment, and multiple repeated experiments were conducted on each specimen to verify the consistency of the results. All bars have a diameter of 60 mm, with the striker bar being an aluminum rod of 1000 mm in length, while the incident and transmitted bars are aluminum rods of 3000 mm in length. These aluminum rods have a material density of 2.7 × 10³ kg/m³ and a Young’s modulus of 71 GPa, possessing excellent elasticity and wave-guiding characteristics, which ensure the efficient propagation of stress waves within the bars and precise measurement.

By utilizing lead sheets as a pulse shaper, we can effectively extend the duration of the incident pulse and ensure that the stress waves undergo multiple reflections within the closed-cell aluminum foam samples, thereby achieving a state of stress equilibrium. The strain gauges mounted on the incident and transmitted bars are used to capture the signals of the incident wave, reflected wave, and transmitted wave, respectively. A typical signal diagram is shown in Figure 5. By accurately measuring these strain signals and combining them with the theory of one-dimensional stress waves, we can calculate the stress, strain, and strain rate of the samples under dynamic conditions using the wave velocity, the material’s elastic modulus, and relevant geometric parameters. The specific derivation formulas are shown below:

$$\dot{\epsilon }=-{\displaystyle \frac{2c_0}{l_s}}{\epsilon }_r$$

(1)

$$\epsilon ={\displaystyle {\int }_0^t\dot{\epsilon }dt={\displaystyle {\int }_0^t\frac{2c_0}{l_s}}}{\epsilon }_{r}dt$$

(2)

$$\sigma ={\displaystyle \frac{A_0}{A_s}}E{\epsilon }_t$$

(3)

where $l_s$ and $A_s$ denote the uncompressed length of the closed-cell aluminum foam and its cross-sectional area, respectively; E, c₀ and A₀ denote the Young’s modulus of the aluminum bar, the wave speed of the bar and the cross-sectional area of the bar, respectively.

2.4. Numerical Simulation Method

Voronoi diagrams can effectively simulate the microstructure of aluminum foam, generating foam structures with random or regular distributions. By randomly placing certain points in space and defining their nearest-neighbor regions, foam cells can be created, thereby constructing the complex cellular structure of aluminum foam. This method can well reflect the randomness and irregularity inside the aluminum foam, making it closer to the actual microstructure of the material. The generation process of Voronoi diagrams is relatively simple and efficient, capable of quickly generating complex foam structure models. This is a significant advantage for research that requires extensive computation and simulation. Additionally, the computational cost of Voronoi diagrams is low, making them suitable for large-scale simulation and optimization. By adjusting parameters in the Voronoi diagram, such as the distribution of nuclei, the size and shape of the cells, the performance of aluminum foam can be optimized. For example, increasing the thickness of the cell walls can enhance the load-bearing capacity of aluminum foam under the same load. Furthermore, by controlling the distribution and shape of the cells, the deformation mode and mechanical properties of aluminum foam can be altered, as shown in Figure 6 for the specific modeling process.

2.5. Finite Element Model

The Johnson-Cook model is a widely used constitutive model that describes the relationship between stress, strain, strain rate, and temperature in materials subjected to large deformations. It is particularly useful for simulating the behavior of materials under high strain rates and elevated temperatures, such as in metal forming, high-speed impact, and explosive loading scenarios. The model is expressed as:

$$\sigma =\left[A+B{\left({\epsilon }^{pl}\right)}^n\right]\left[1+C\ln \left({\displaystyle \frac{{\dot{\epsilon }}^{pl}}{{\dot{\epsilon }}_0}}\right)\right]\left(1-T^{\ast m}\right)$$

(4)

where T is temperature; ${\epsilon }^{pl}$ and ${\dot{\epsilon }}^{pl}$ are the plastic strain and strain rate, and all other parameters are constants;

Table 1. Parameters for closed-cell foam aluminum pure aluminum matrix.

A/MPa	B/MPa	C	n	m	$\dot{\mathit{\epsilon }}$/s−1	${\mathbf{T}}_{\mathit{r}}$/K	${\mathbf{T}}_{\mathit{m}}$/K
320	452	0.0021	0.59	1.31	5 × 10−4	298	883
D1	D2	D3	D4	D5	ρ/kg·m−3	E/GPa	v
0.096	0.049	3.342	0.0132	1.067	2700	68	0.32

gives a detailed list of parameters.

To enhance computational efficiency, shell elements were employed in the simulation. To avoid the formation of excessively small and distorted elements, edges shorter than a critical threshold and surfaces smaller than a critical area were removed. Moreover, the aspect ratio of the cell walls was maintained between 1/20 and 1/35 for all samples. During this optimization process, only 1.06% of the faces were eliminated, accounting for just 0.003% of the total surface area. As a result, the removal of these minor edges and faces had a minimal effect on the model’s geometry and stiffness. This approach ensured that the simulation remained both accurate and computationally efficient, allowing for the effective analysis of complex foam structures.

3. Results and Discussions

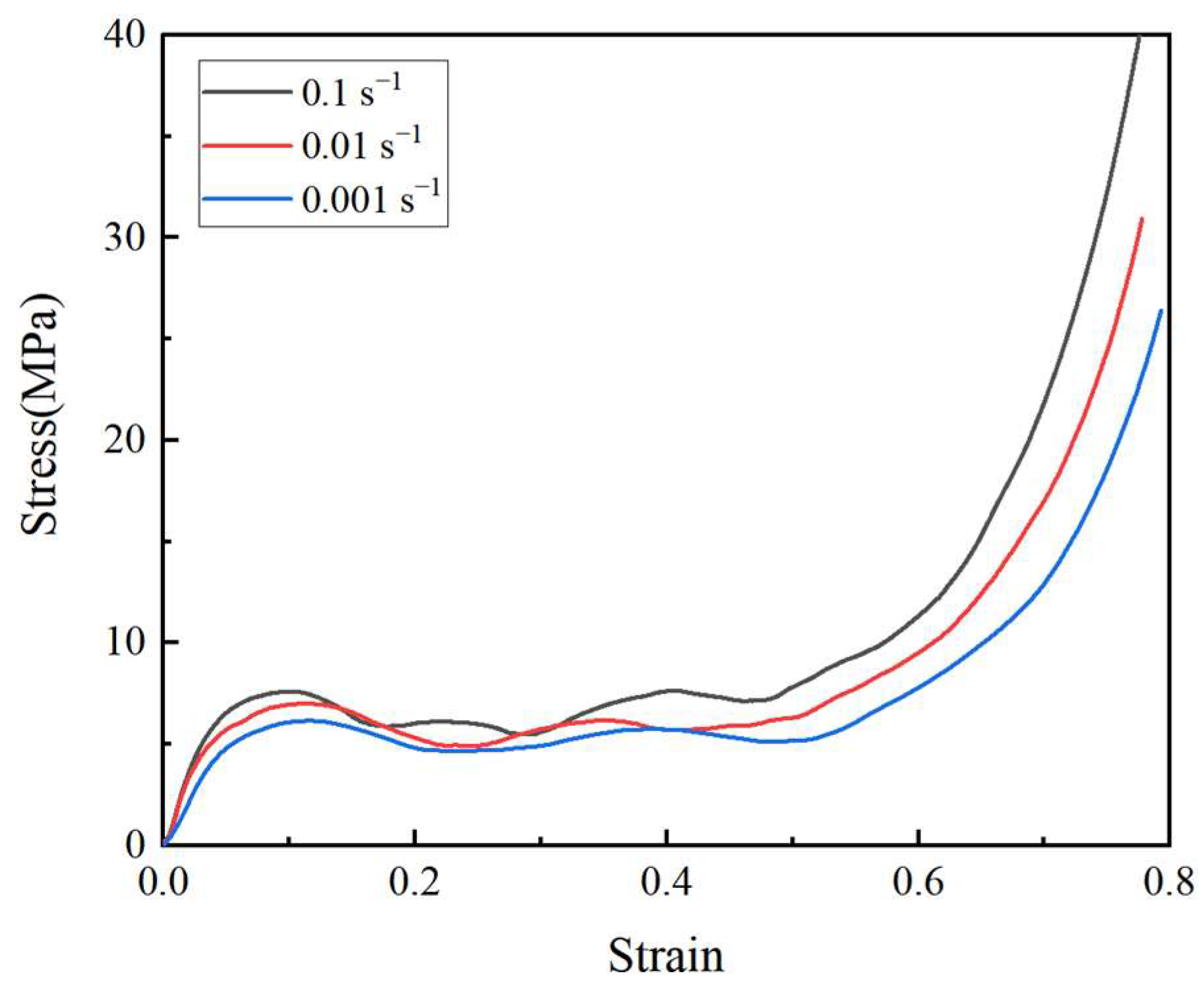
3.1. Quasi-Static Compression Result

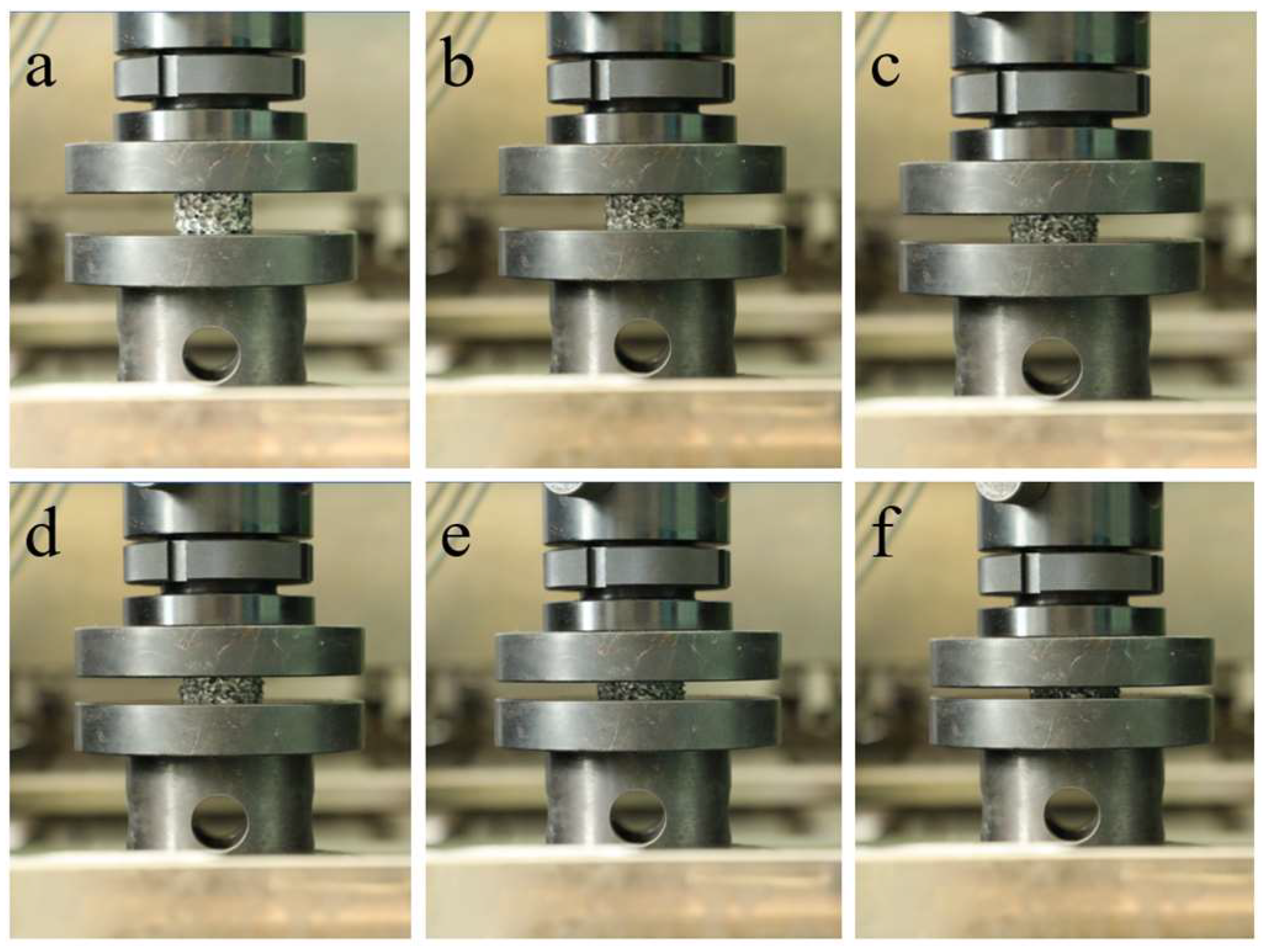
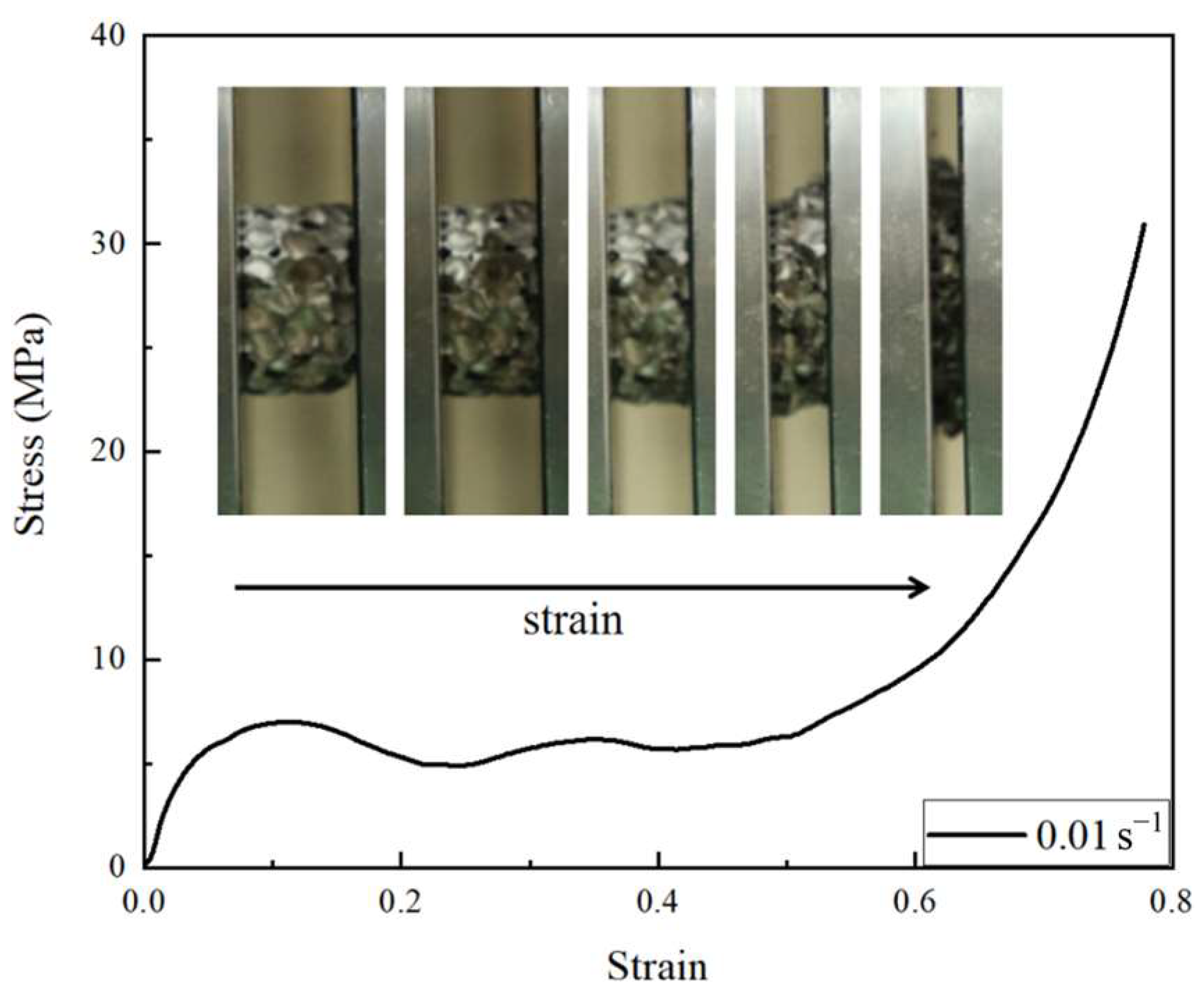
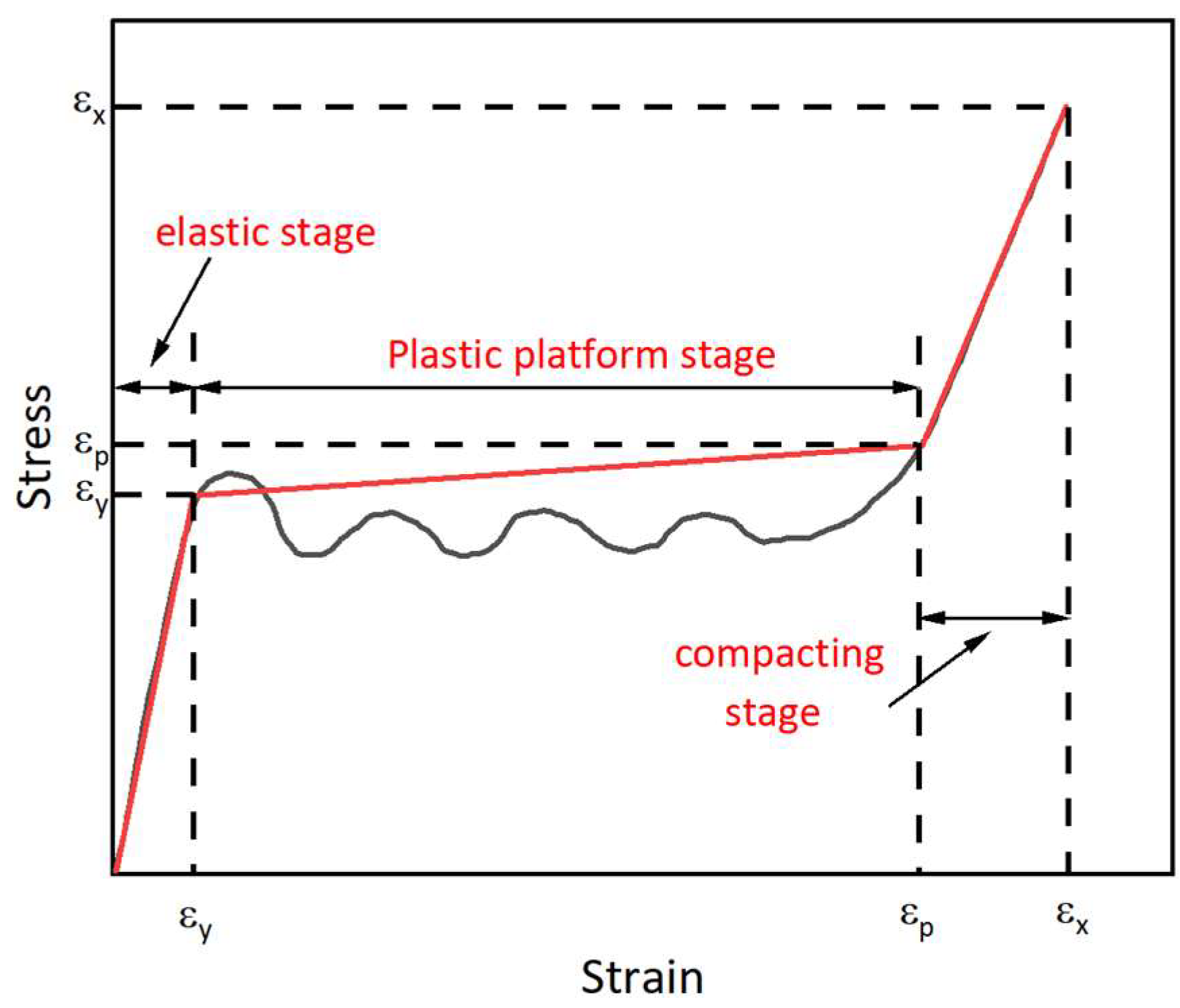
By conducting quasi-static compression experiments, the stress-strain curves of aluminum foam under quasi-static compression were obtained, as shown in Figure 7. The “failure point” is defined as the point at which the material begins to exhibit significant deformation or fracture. This point corresponds to the location on the stress-strain curve where the curve starts to deviate from its initial linear behavior, indicating the onset of plastic deformation or the beginning of the plateau region. In this plateau region, the cells of the material start to buckle and collapse. For aluminum foam, this point is particularly important because it signifies the transition from elastic deformation to plastic deformation.

As shown in Figure 7 and Figure 8, in quasi-static compression, the aluminum foam has obvious 3-stage characteristics: elastic section, stress plateau section and compaction section. When the compressive stress reaches the vicinity of the yield stress, due to the sudden collapse and buckling of the pore wall, the stress-strain relationship curve has some fluctuations in the yielding process, and with the further compaction of the aluminum foam, this fluctuation due to the sudden collapse is obviously reduced, and the curve is smoother. From the change of yield point stress of aluminum foam under different strain rates, it can be seen that there is not much influence on the yield point stress during compression of aluminum foam between each strain rate, and the phenomenon of yield point stress elevation is not obvious, and the platform section is basically the same, which indicates that there is almost no influence on the platform stress of aluminum foam under low strain rate.

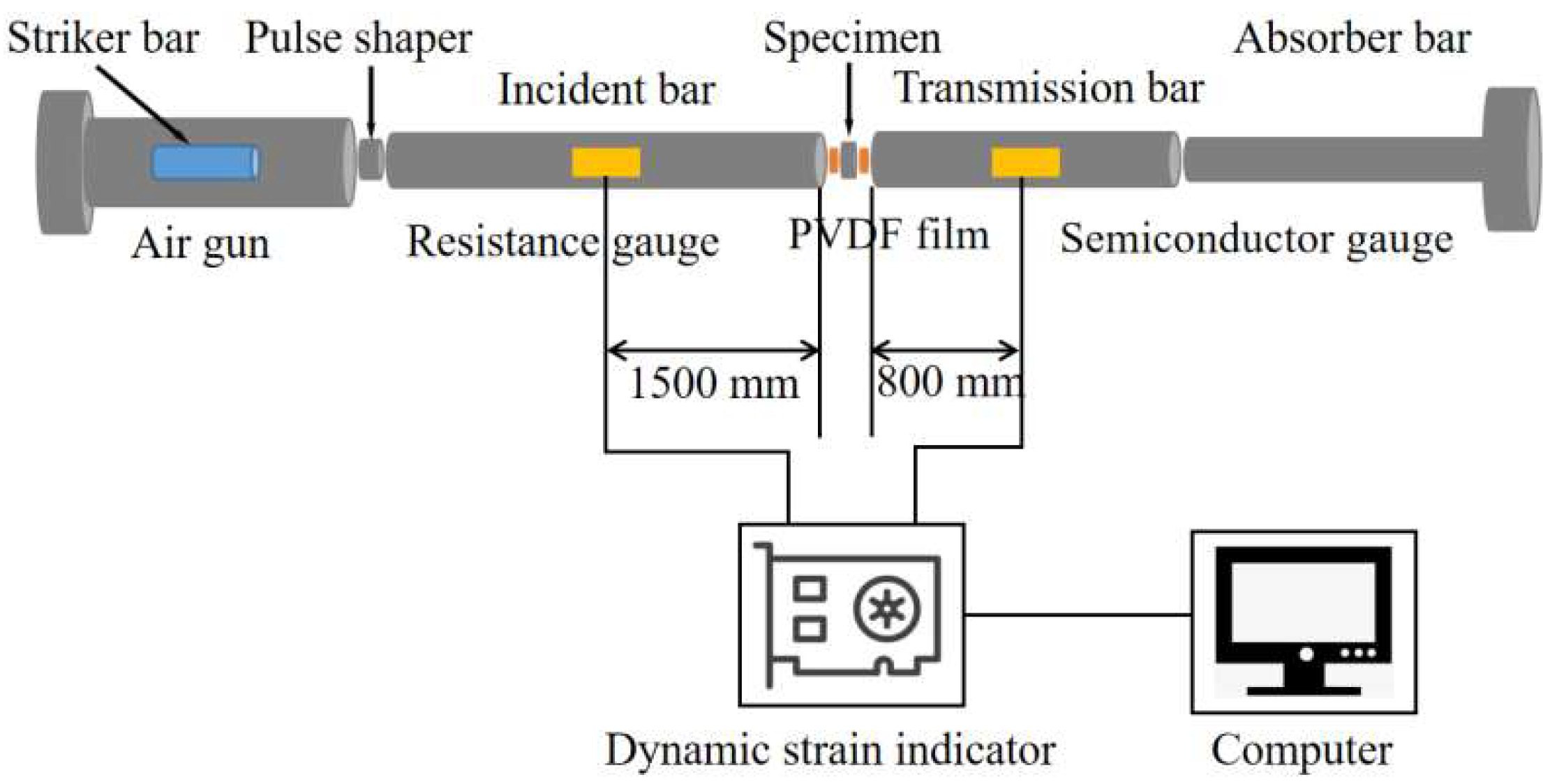
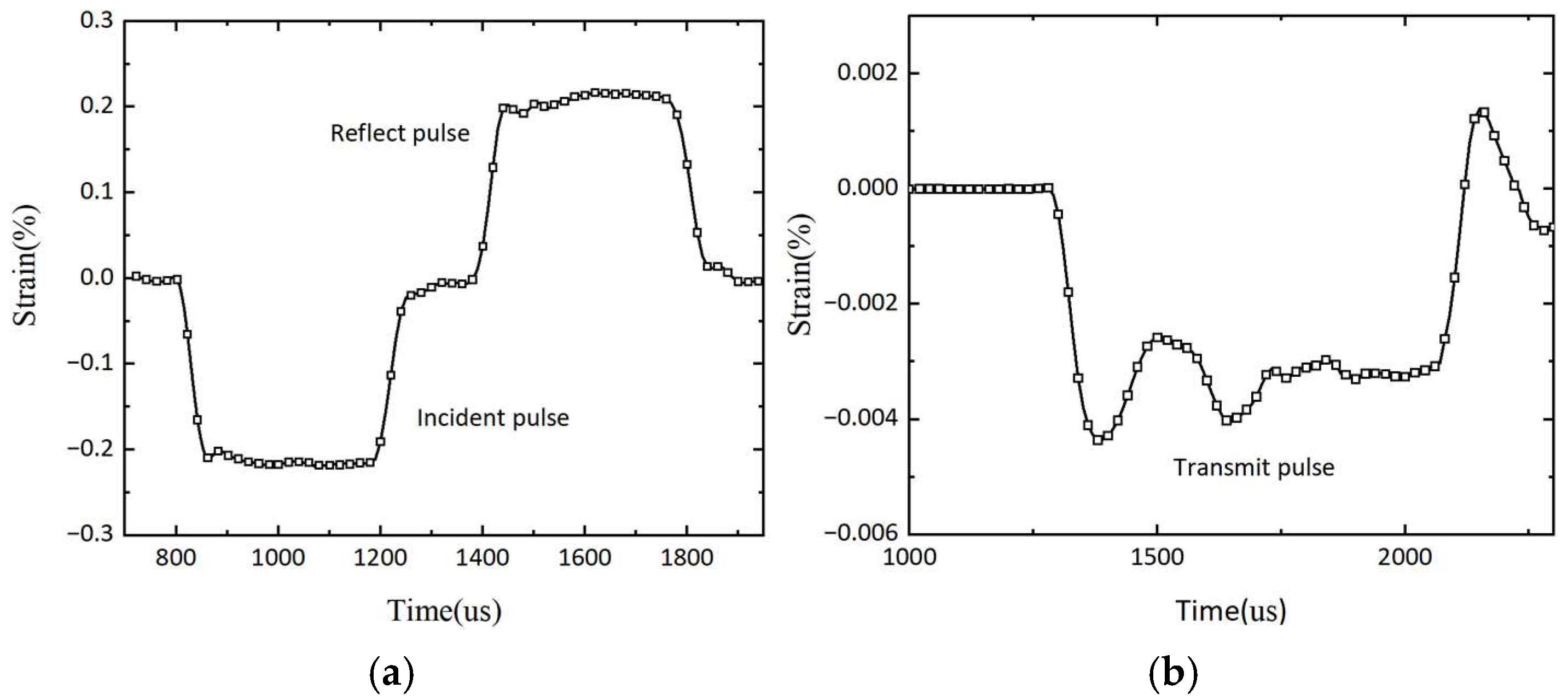
3.2. Dynamic Compression

As shown in Figure 9 and Figure 10, it can be seen that in dynamic compression, the aluminum foam has obvious 3-stage characteristics: elastic section, stress platform section, and no densification stage is found, which is due to the fact that the forward bar of SHPB equipment gives impact force to the incoming bar, and the incoming bar strikes the aluminum foam specimen at a certain speed, and the specimen absorbs the impact energy brought by the incoming bar in the compression and denaturation process, and the impact energy of incoming bar gradually decreases with the compression of aluminum foam, which leads to the smaller impact energy of incoming bar, the lower strain rate, and the lower strain rate, and no densification stage occurs. The impact energy of the incident rod decreases gradually with the compression of the aluminum foam, which leads to the smaller impact energy of the incident rod, the lower strain rate, the smaller strain of the aluminum foam, and the stage of densification does not occur. Based on the compression process of the aluminum foam, it is reasonable to infer that if there is a continuous impact energy, the aluminum foam will eventually develop a densification stage.

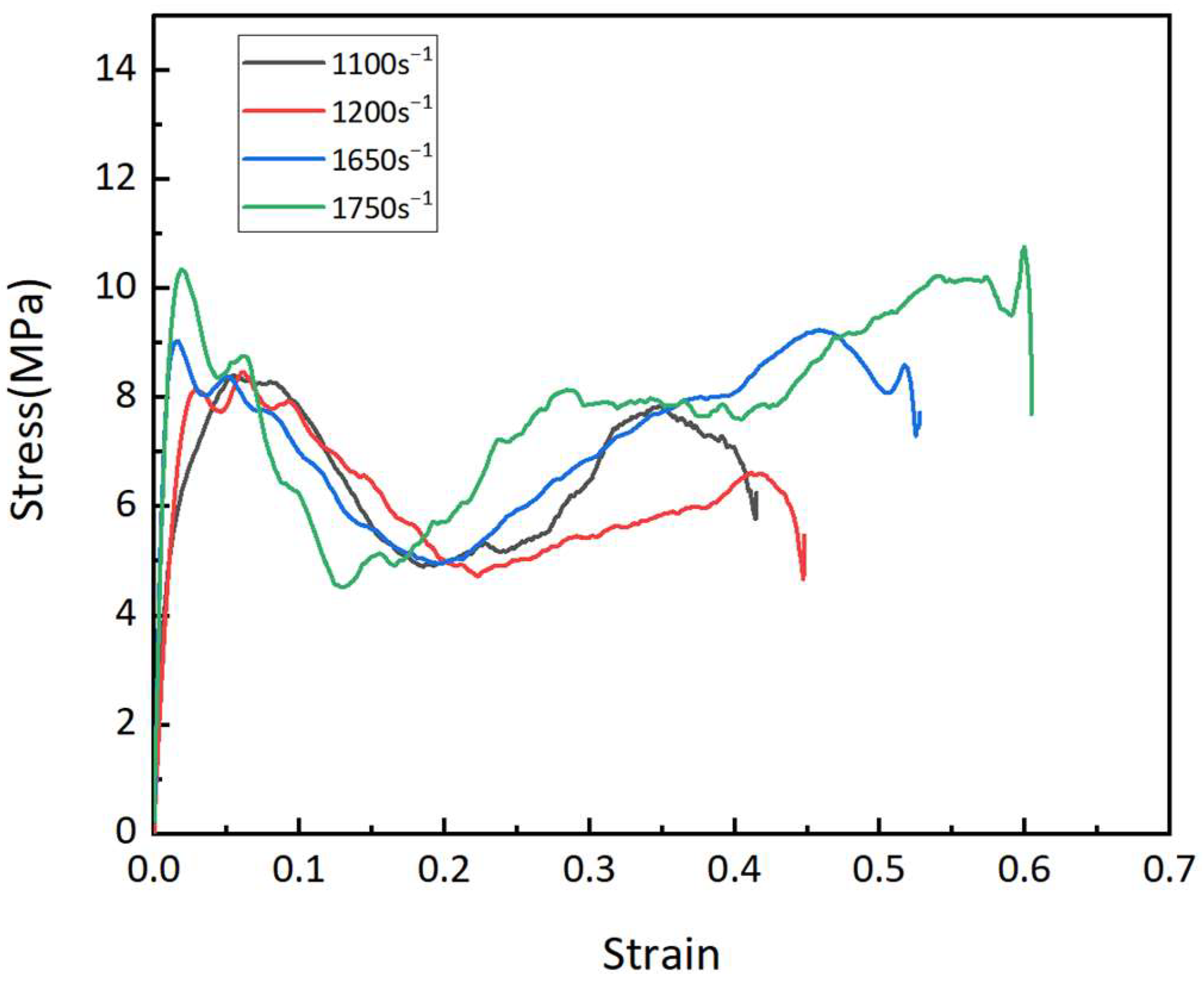
From the change of yield point stress of aluminum foam under different strain rates, it can be seen that different strain rates have a certain effect on the yield point stress of aluminum foam in compression, and with the increase of strain rate, the yield stress gradually increases and the elastic strain gradually decreases. The stress in the platform section has a small enhancement with the increase of strain rate, and the trend of stress change is basically the same, indicating that there is a slight strain rate effect on the yield stress and platform stress in aluminum foam.

3.3. The Effect of Different Strain Rate

The compressive stress-strain curve is shown in Figure 11. The plateau pressure σ_pl is expressed as, where ε₀ is the yield strain of the foam, and ε_d belongs to the densification strain.

$${\sigma }_{pl}={\displaystyle \frac{1}{{\epsilon }_d-{\epsilon }_0}}{\int }_{{\epsilon }_0}^{{\epsilon }_d}\sigma d\epsilon$$

(5)

The individual eigenvalues of aluminum foam at different strain rates were extracted and the results are shown in the

Table 2. Effect of strain rate on mechanical properties of aluminum foam.

Strain Rate/s−1	Yield Stress (MPa)	Elastic Modulus (MPa)	Densification Strain	Platform Modulus (MPa)	Platform Stress (σ)
0.001	4.142	11.477	0.693	10.12	5.739
0.01	3.87	17.750	0.675	13.00	6.1
0.1	5.25	15.130	0.64	11.62	7.142
1000	9.89	37.784	0.63	5.85	6.75
1100	10.9	37.688	0.623	6.31	7.10
1200	15.02	38.216	0.613	6.80	7.05
1650	9.28	56.901	0.634	6.17	7.45
1750	7.49	80.823	0.635	6.89	7.63

below:

As shown in Figure 12, the mechanical properties of foam aluminum change significantly with the increase of strain rate: the yield stress, elastic gradient, and plateau stress all increase, while the yield strain and densification strain decrease. The reason for these changes is that under high strain rates, the pore structure of foam aluminum is affected by rapid loading, the stress concentration in pore walls and edges is more pronounced, internal defects (such as pores, cracks, etc.) do not have enough time to expand fully, dislocation movement is inhibited, dislocation density increases, thereby hindering further plastic deformation. At the same time, the deformation of the pore structure is restricted, the rigidity of the pore walls is enhanced, and the elastic modulus increases. In terms of micro-mechanisms, under high strain rates, the deformation rate of the pore structure accelerates, the stress transfer within the material is faster, the contact between pore walls and edges is tighter, and the material can reach a dense state at a lower strain.

3.4. Deformation Mechanism and Failure Mechanism

As shown in Figure 13, the mechanical properties of foam aluminum are significantly affected by different strain rates under static and dynamic conditions. With the increase of strain rate, the yield stress and plateau stress gradually increase, while the elastic strain gradually decreases. The yield point stress increases by nearly 40%. The stress-strain curve trend under low strain rate is basically consistent with that under high strain rate.

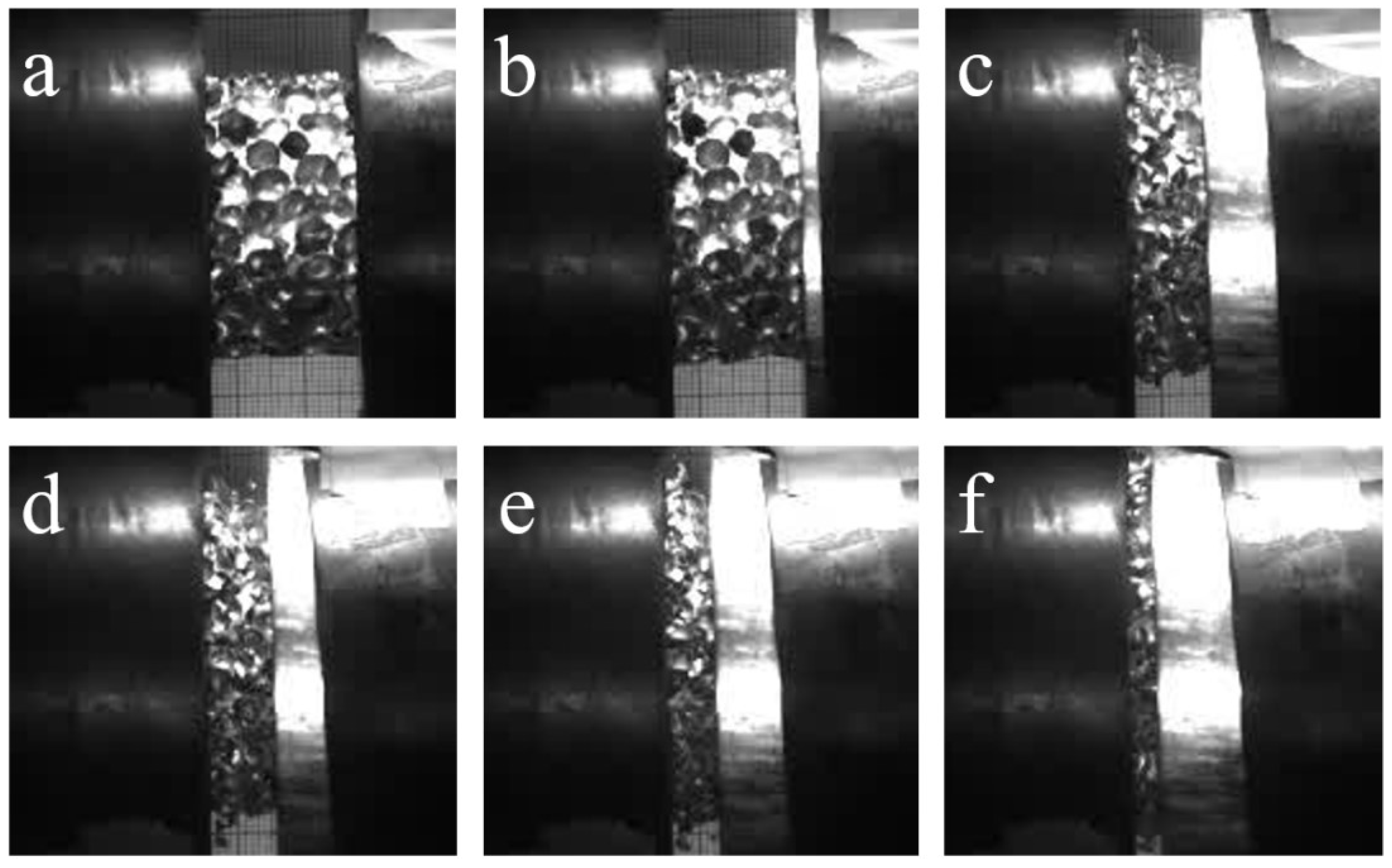
As shown in Figure 14, during the elastic stage, the deformation of foam aluminum occurs within a very small region. The stress-strain curve in this stage can be approximated as a straight line, indicating that the pore shapes of foam aluminum have not yet changed significantly and the pore walls have not yet buckled. The end of the elastic segment is its yield point. When the strain of foam aluminum enters the plateau segment, the strain gradually increases while the stress does not rise significantly, because during this period, the pore walls of foam aluminum begin to buckle and collapse gradually. As the pores in foam aluminum are completely compacted and enter the compaction segment, the material itself begins to undergo compressive deformation, and the stress rises sharply.

The static compression and dynamic deformation processes of closed-cell aluminum foam stress-strain curves are shown in Figure 15. During the compression process, the cell structure first deforms, causing stress concentration. As the load increases, the cell walls bend and fracture, and the pores collapse. Subsequently, the collapse expands layer by layer, resulting in a step-by-step collapse deformation, and eventually the entire specimen is compacted. The deformation and failure process is divided into three stages: (1) elastic deformation stage; (2) plateau collapse deformation stage; (3) densification stage. The linear elastic deformation stage of closed-cell foam aluminum is mainly characterized by the elastic bending and extension of the cell walls, and the work done by the external force is converted into the elastic deformation energy of the foam aluminum. This stage mainly reflects the strength characteristics of the pore structure. When the compressive stress reaches the yield strength that foam aluminum can withstand, the recoverable elastic deformation of the cell walls evolves into irreversible plastic yielding. Due to the inhomogeneity of the foam aluminum material structure, plastic deformation cannot occur simultaneously when subjected to external forces. The failure first occurs at the weak points of the cell walls, where the local cell walls first transition from elastic bending to plastic bending, leading to stress concentration in the plane perpendicular to the external force and containing this pore, causing the cell walls to be crushed. The crushed cell walls connect to form the first deformation band, with most of the cell walls outside the deformation band still in the elastic deformation stage. As the load increases, new weak deformation bands gradually form and develop, and the continuous formation of weak deformation bands indicates that foam aluminum exhibits significant localization during the compression process. When collapse occurs again, the failure occurs within a new layer and repeats the above process, compacting the material layer by layer, with little change in stress throughout the process. This stage mainly reflects the yielding and crushing process of the pore structure. The deformation mechanism in the densification stage is mainly due to plastic collapse causing the cell walls to wrinkle along the compression direction, leading to the cell walls pressing together and the pores being compacted. At this point, the compressive properties of foam aluminum are equivalent to those of solid metal, with stress increasing rapidly within a small strain range.

From the above analysis, it can be known that the deformation mechanism of foam aluminum is that stress concentration first occurs at the weak pore walls, forming deformation bands. These deformation bands continuously develop and collapse layer by layer. The micro-deformation is irregular and is the result of the combined action of pore wall bending and folding. It is precisely because of the unique compressive behavior of foam aluminum materials, that is, when subjected to external impact, they are prone to deformation and can maintain stress at a low level while deforming. During the compressive deformation process, a large amount of work is consumed and transformed into various forms of energy dissipation such as plastic deformation, collapse, and rupture of pores in the structure, thereby effectively absorbing external impact energy. The deformation process of closed-cell aluminum foam is shown in Figure 16.

As analyzed above, the deformation mechanism of foam aluminum begins with stress concentration at the weak pore walls, leading to the formation of deformation bands. These bands continuously evolve and collapse layer by layer. The micro-deformation is irregular, resulting from the combined effects of pore wall bending and folding. It is the unique compressive behavior of foam aluminum materials that makes them prone to deformation when subjected to external impacts, while maintaining stress at a low level during deformation. This behavior allows for the consumption of a large amount of work during the compressive deformation process, which is then transformed into various forms of energy dissipation, such as plastic deformation, collapse, and rupture of pores within the structure, thereby effectively absorbing external impact energy.

3.5. Finite Element Model Accuracy Verification

The Figure 17 compares experimental data (black line with square markers) and numerical simulation results (red line with circular markers). The overall trends show good agreement, indicating the numerical model accurately captures the system’s behavior. However, discrepancies are noted in regions where experimental data fluctuates more significantly. These differences may stem from experimental errors, model simplifications, or unaccounted material property variations. Overall, the numerical simulation reliably predicts experimental outcomes with minor deviations in certain areas.

3.6. Damage Mechanisms

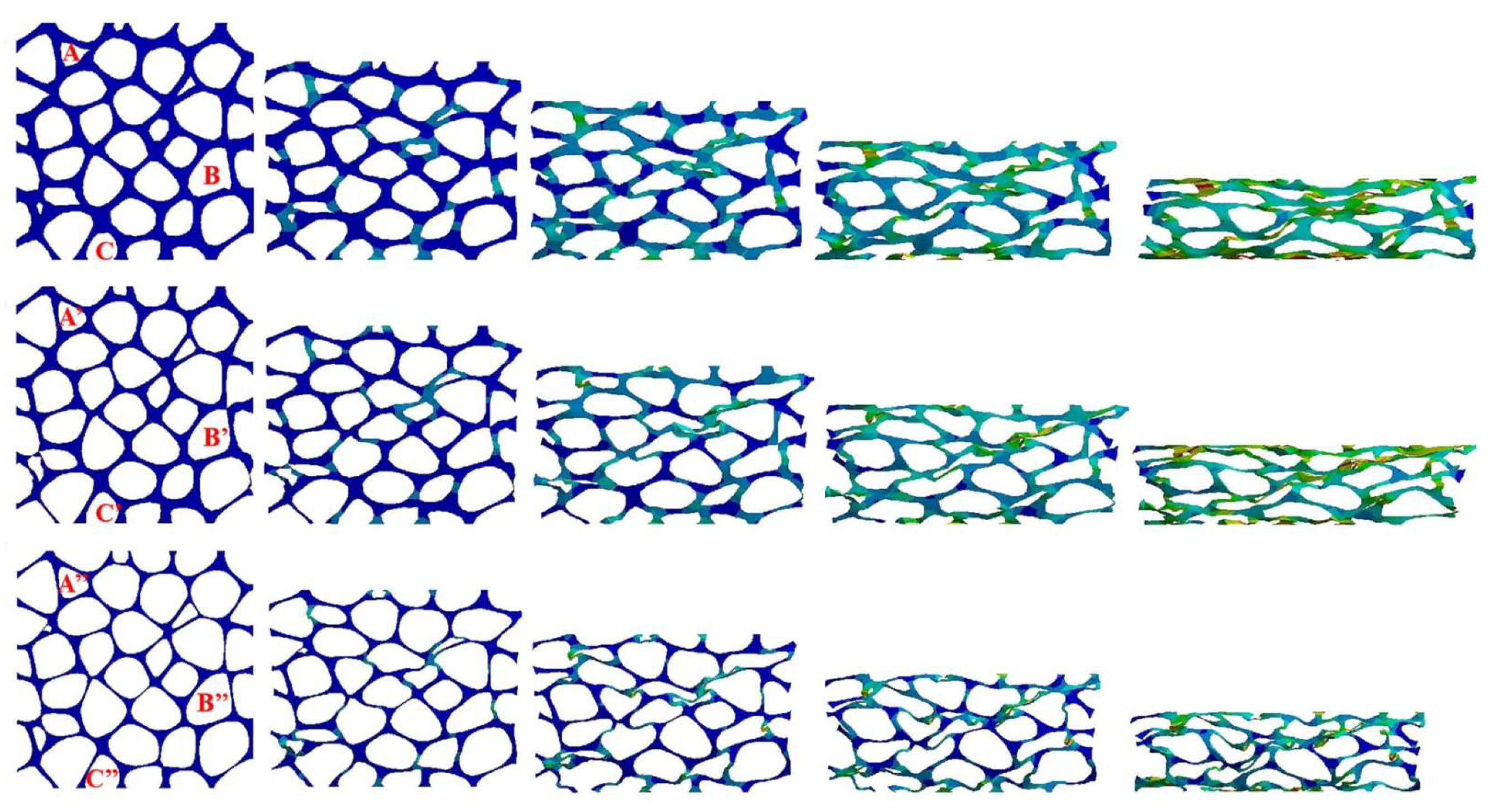
Simulation analysis was conducted using the validated mesoscale closed-cell foam aluminum finite element model to study its deformation patterns under impact loads. As shown in Figure 18, the results indicate that as the compressive strain gradually increases, deformation bands begin to appear on the cross-section of the foam aluminum. These deformation bands are initially sparsely distributed, but as the compressive strain further increases, the range of the deformation bands gradually narrows and eventually converges to form a concentrated deformation band. This process reflects the characteristic of foam aluminum transitioning from initial uniform deformation to localized concentrated deformation under impact loads.

The finite element model is sliced as shown in Figure 19 to analyses the different stages of deformation of closed cell aluminum foam. Initial stage: At lower compressive strains, the pore structure of the foam aluminum is in a state of uniform deformation as a whole, and no obvious local deformation characteristics have yet appeared. Deformation band formation stage: As the compressive strain increases, stress begins to concentrate in some weak areas of the foam aluminum (such as the connections of pore walls or the edges of pores), and these areas gradually form deformation bands. At this time, the distribution of deformation bands is relatively scattered, but a trend of local deformation has already appeared. Deformation band contraction stage: Further increasing the compressive strain, the stress concentration effect becomes more significant, and the range of the deformation bands gradually narrows. This is because the stress is further concentrated in a smaller area, resulting in relatively weaker deformation in other areas. Concentrated deformation band formation stage: Finally, at higher compressive strains, the deformation bands converge to form a concentrated deformation band. At this time, the deformation of the foam aluminum is mainly concentrated in this area, showing obvious localized deformation characteristics.

This deformation pattern reflects the complex mechanical behavior of foam aluminum under impact loads, revealing its transition process from overall deformation to localized concentrated deformation, and provides an important basis for understanding the energy absorption characteristics and failure mechanisms of foam aluminum.

4. Conclusions

This study thoroughly investigates the mechanical behavior of closed-cell foam aluminum under varying strain rates through both experimental and modeling approaches. The research reveals that the material’s mechanical properties, including yield stress, elastic modulus, and platform stress, significantly increase with strain rate, while yield strain and densification strain decrease. For example, the yield stress increased from 4.142 MPa at a strain rate of 0.001 s⁻¹ to 15.02 MPa at a strain rate of 1200 s⁻¹. The elastic modulus also increased from 11.477 MPa to 38.216 MPa over the same range of strain rates.

The deformation mechanism involves stress concentration at weak pore walls, leading to the formation and evolution of deformation bands, which eventually result in localized concentrated deformation. In order to further investigate the deformation mechanism, a fine-scale finite element model of closed-cell aluminum foam was established and validated. The results showed that the established multiscale finite element model could accurately describe the stress-strain response of closed-cell aluminum foam under impact loading. Based on the fine-view finite element model, the multiscale deformation mechanism of aluminum foam under impact loading was investigated, and three stages of the deformation band evolution of closed-cell aluminum foam under impact loading were proposed. The deformation evolution process was described in detail, providing valuable insights into the energy absorption characteristics and failure mechanisms of the material.