Numerical Study on the Coupled Impact of Periodic Wake and Pulsating Jet on Film Cooling Efficiency on a Flat Wall
Abstract
1. Introduction
2. Numerical Methods
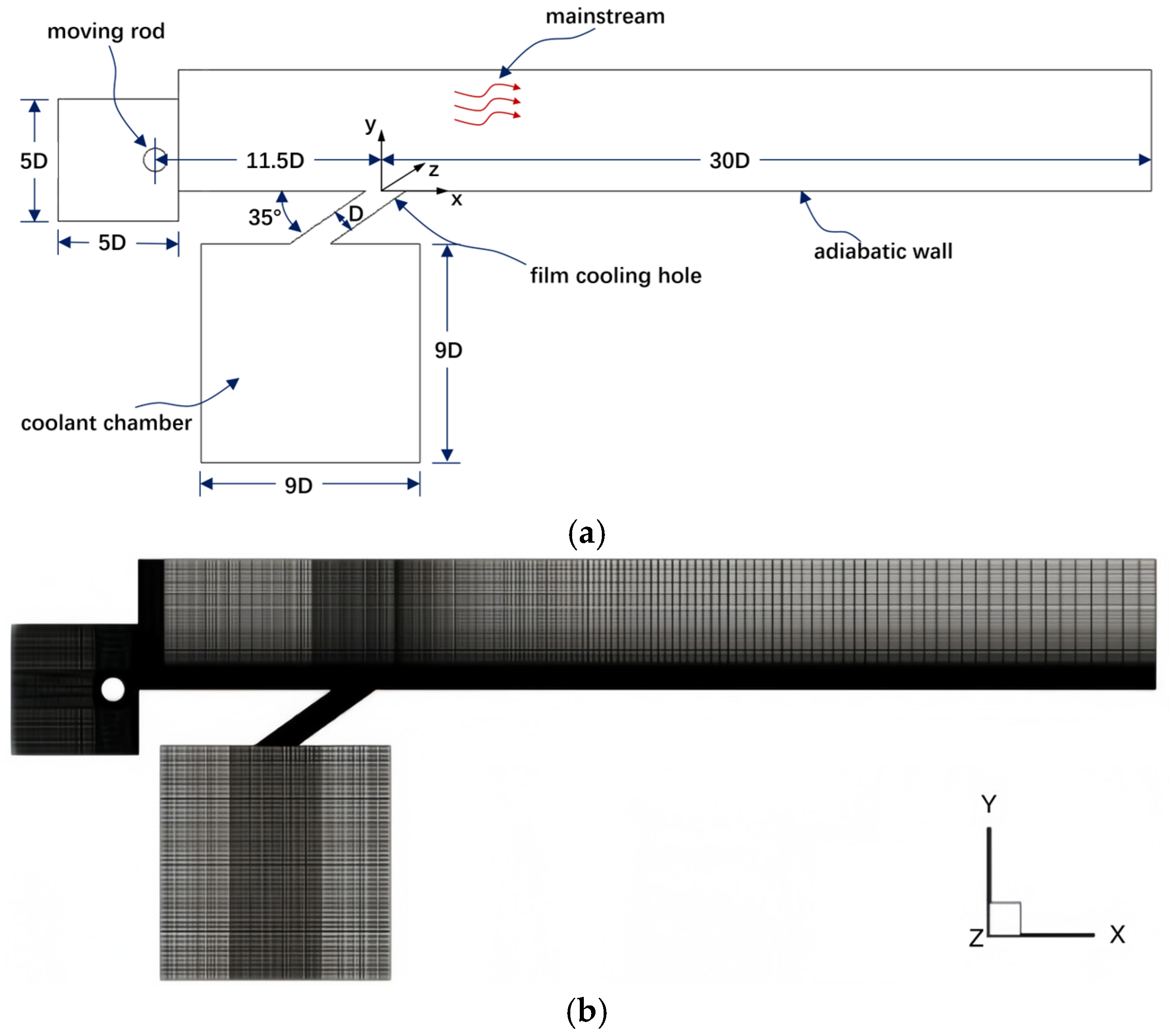
2.1. Numerical Setup
2.2. Parameter Definition
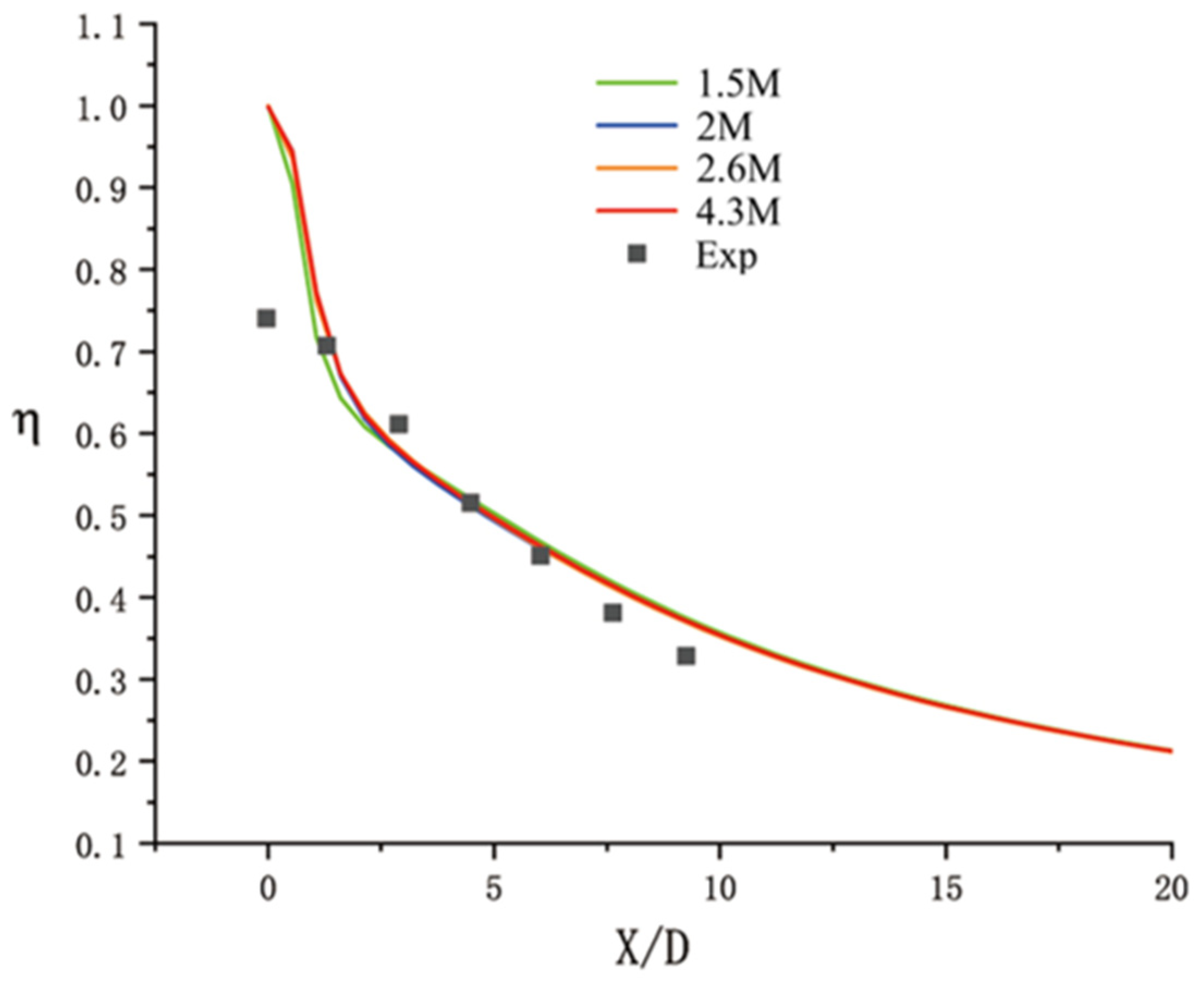
2.3. Grid Independence Study
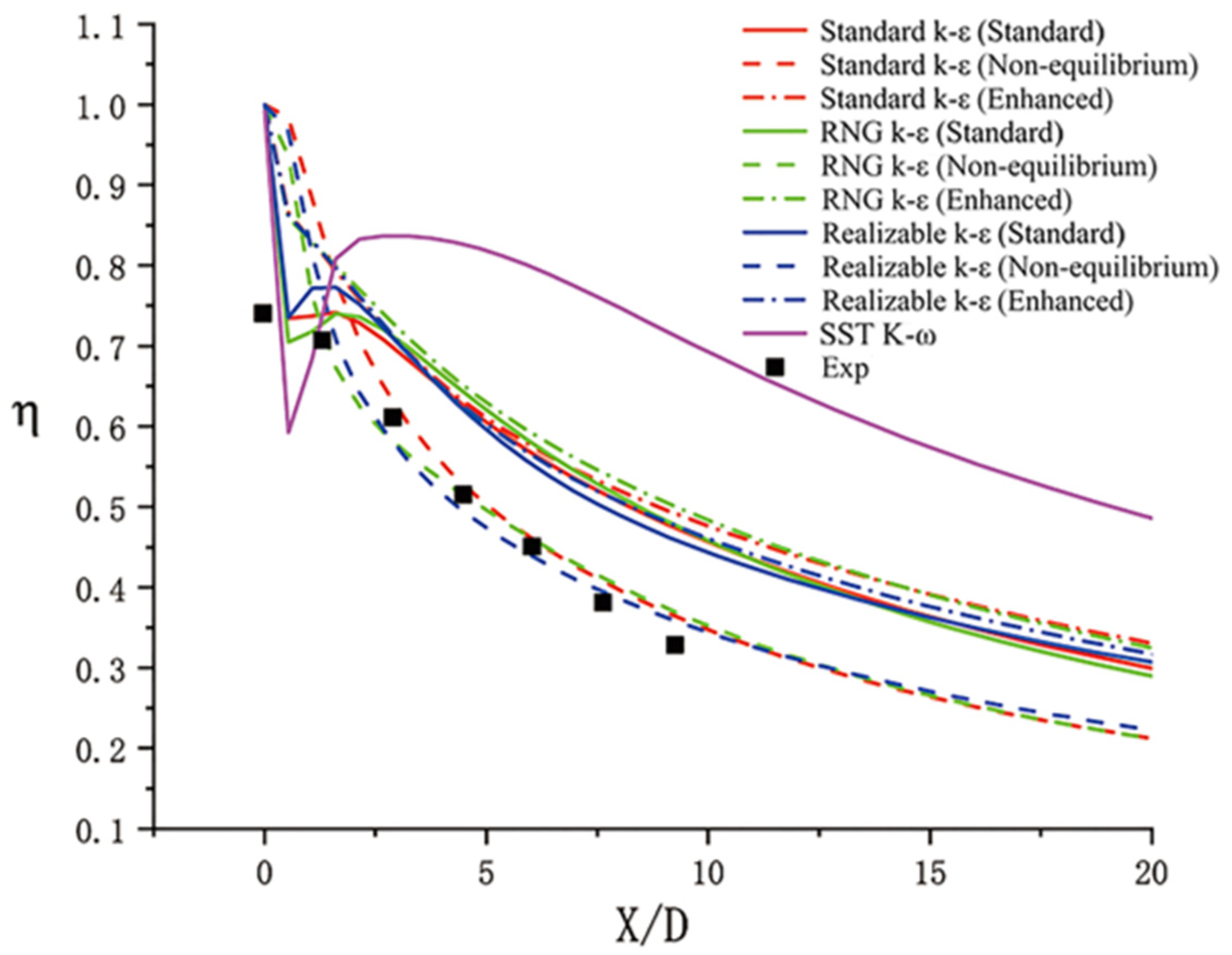
2.4. Turbulence Model Independence Study
3. Results and Discussion
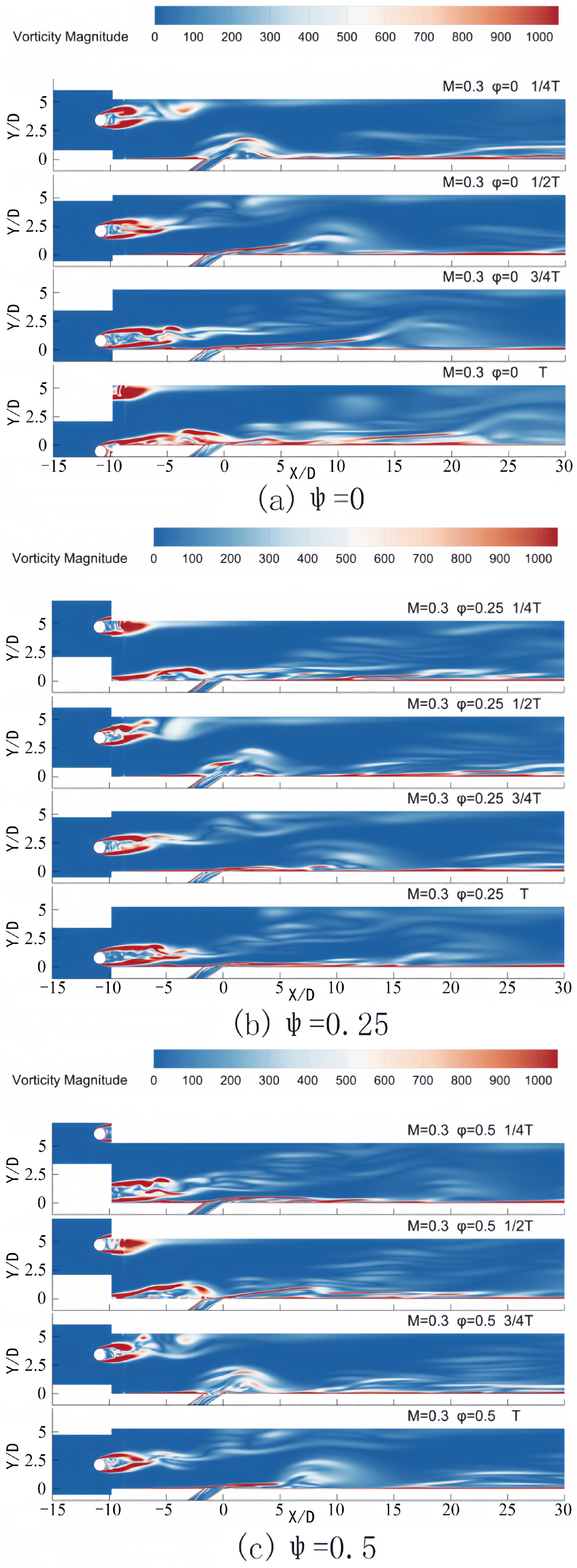
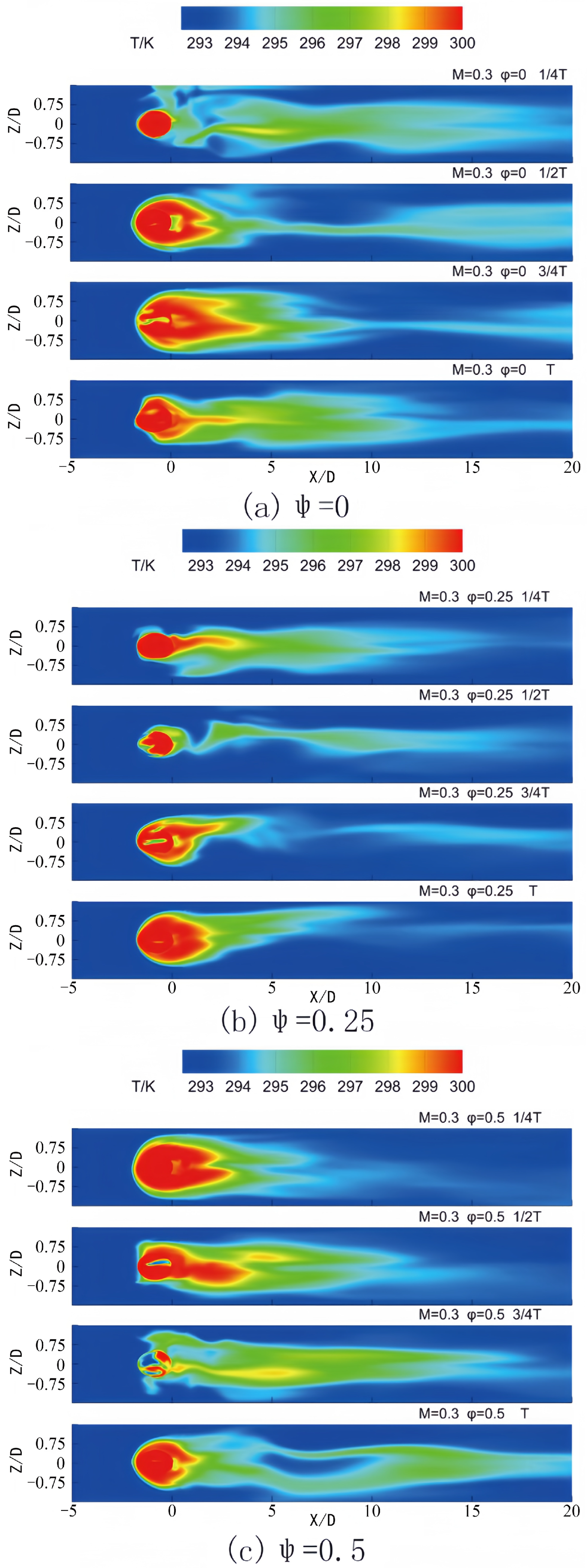
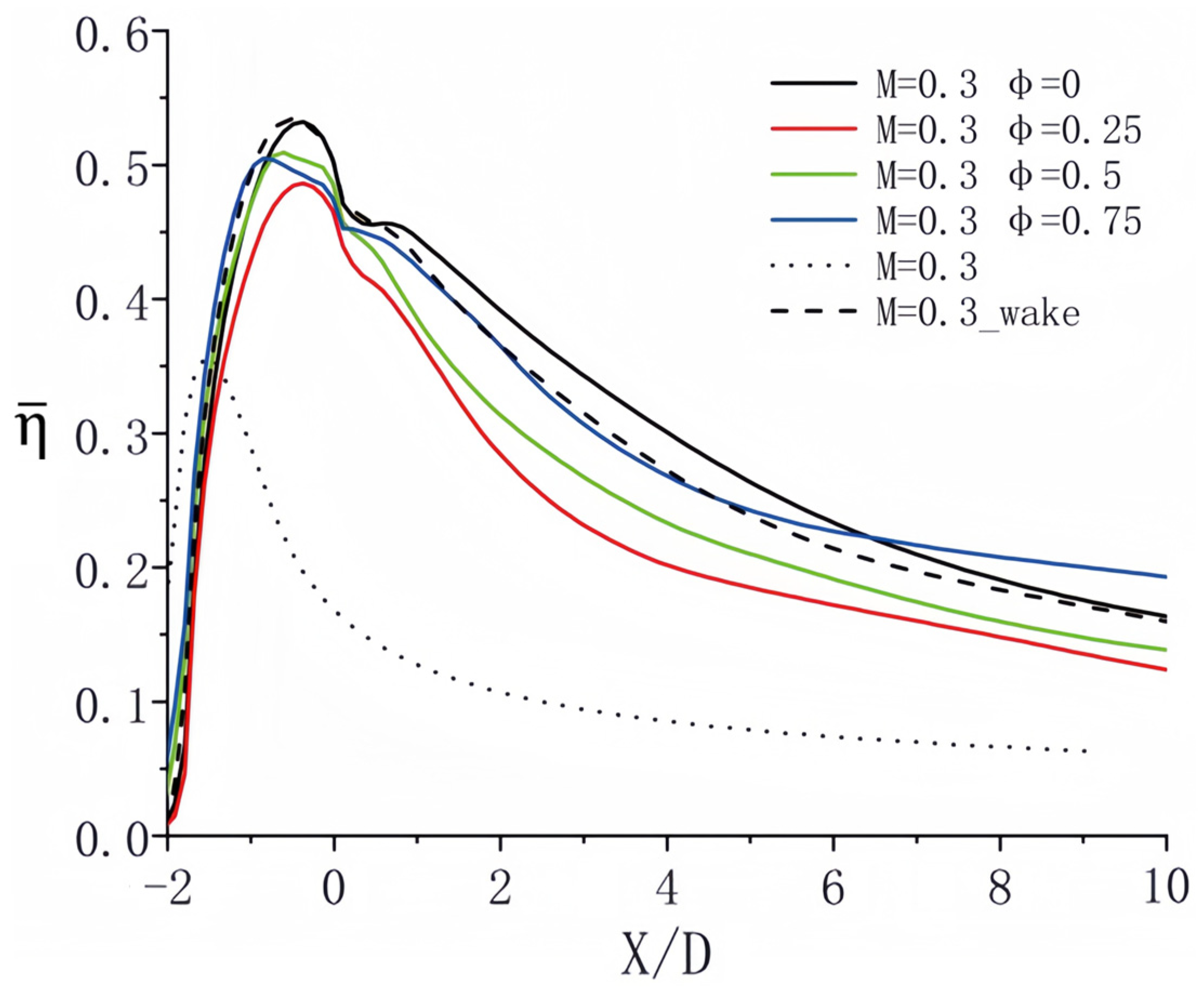
3.1. Blowing Ratio M = 0.3
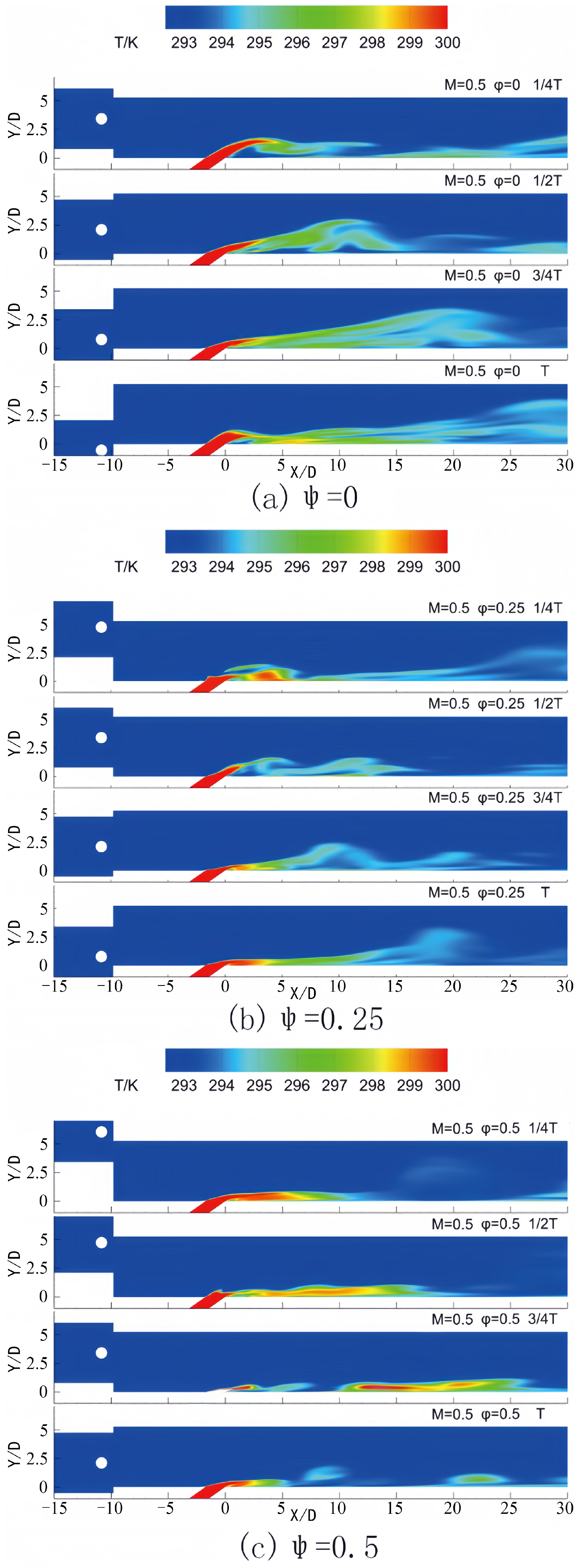
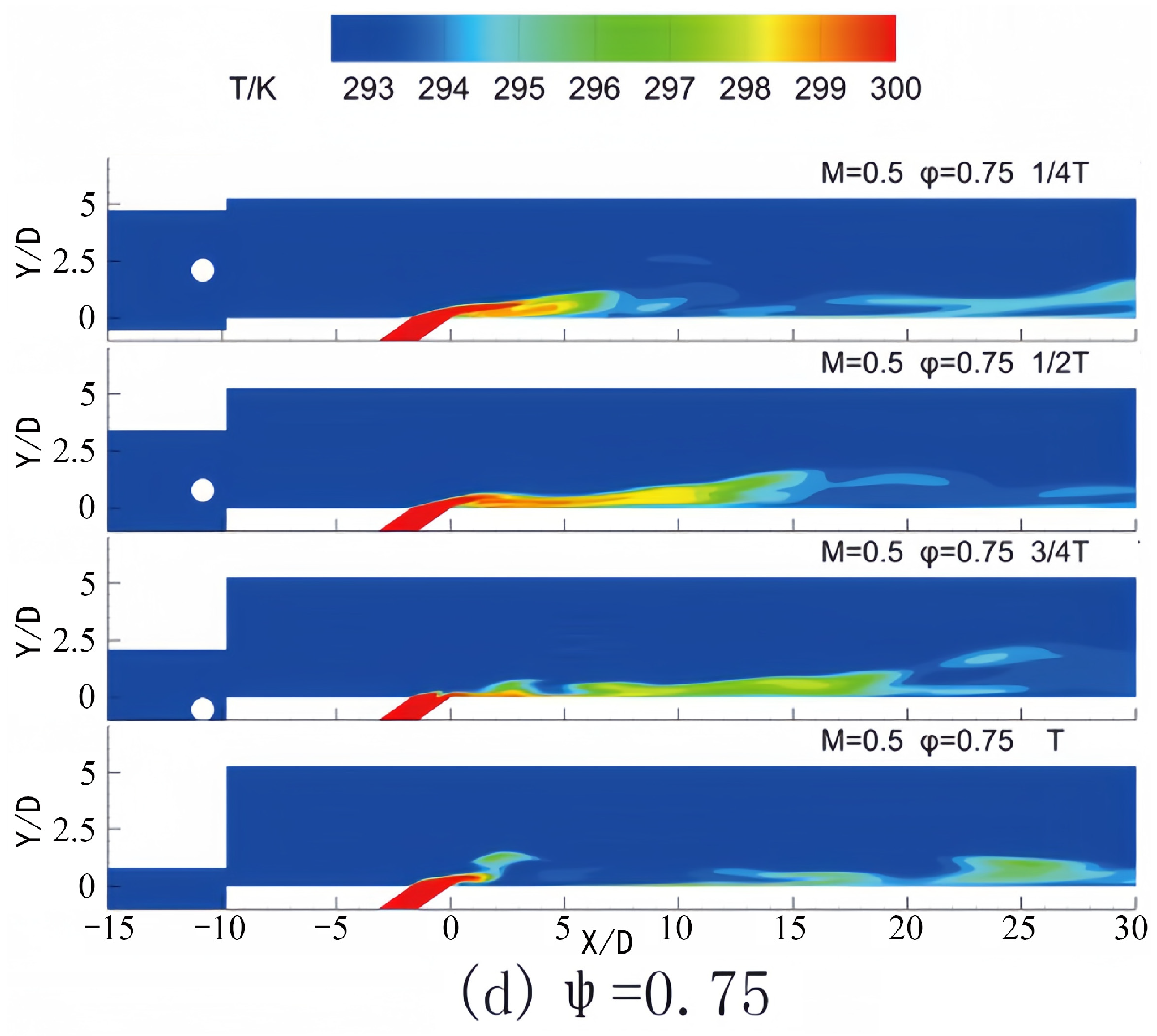
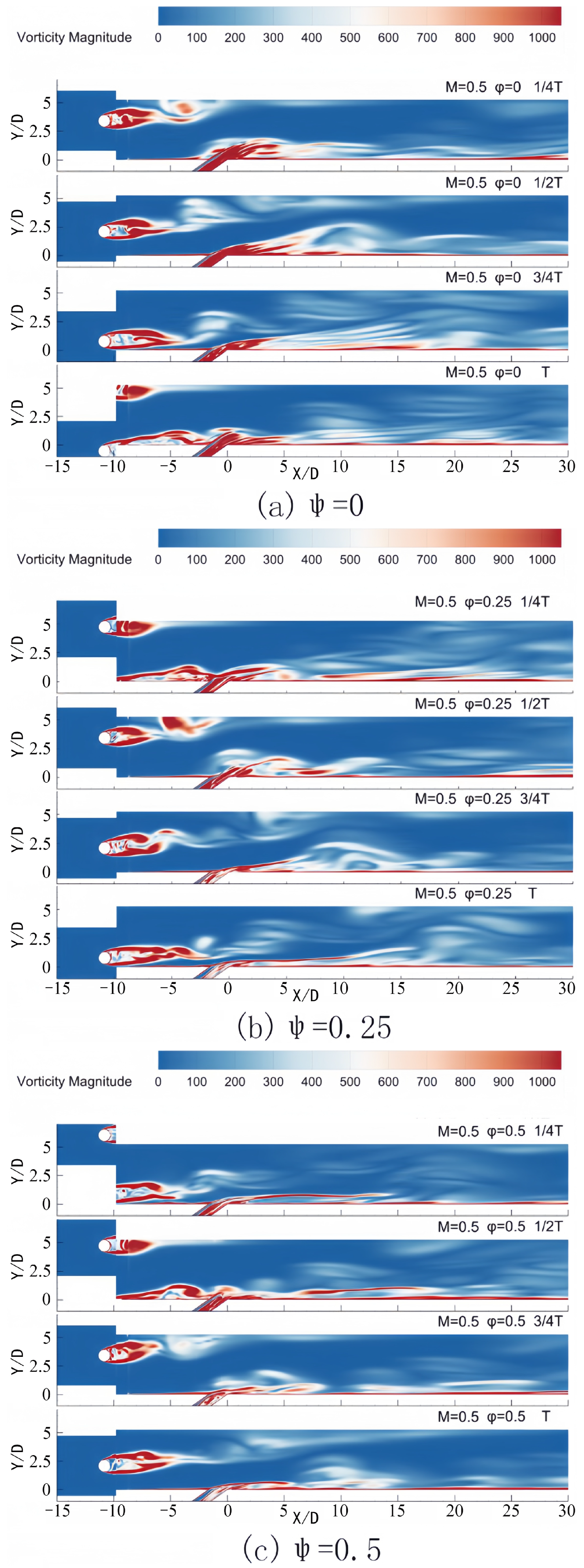
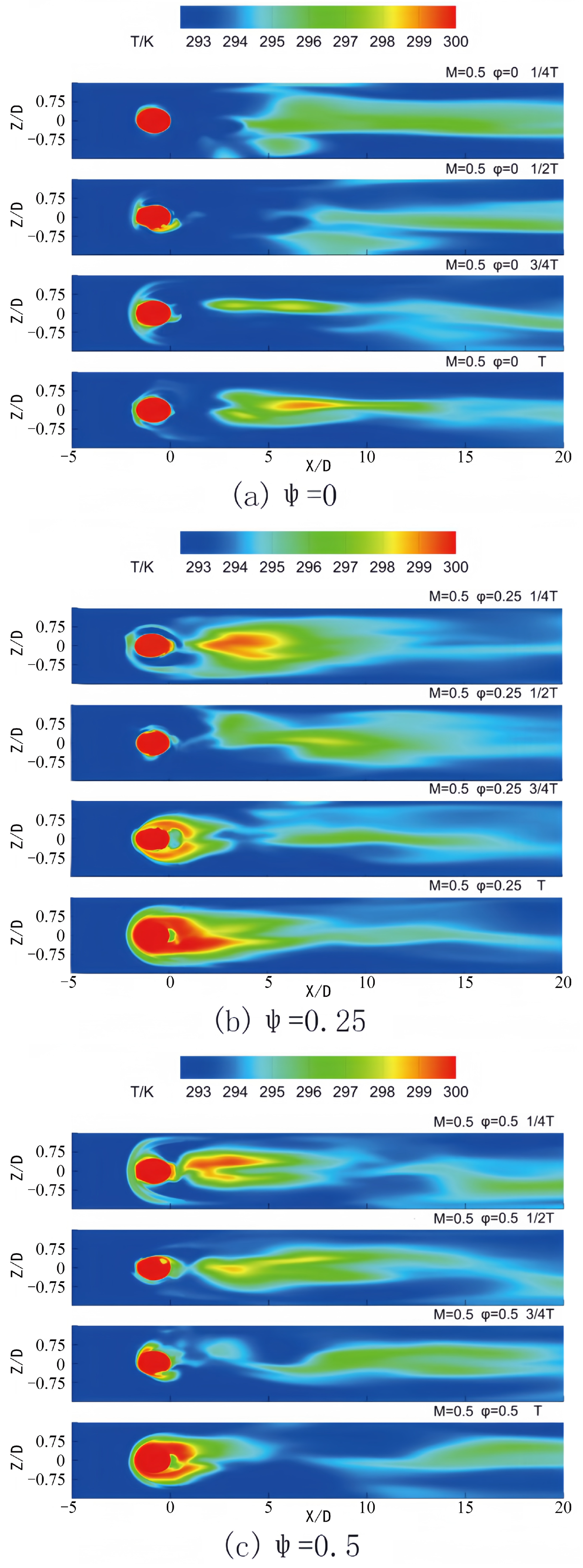
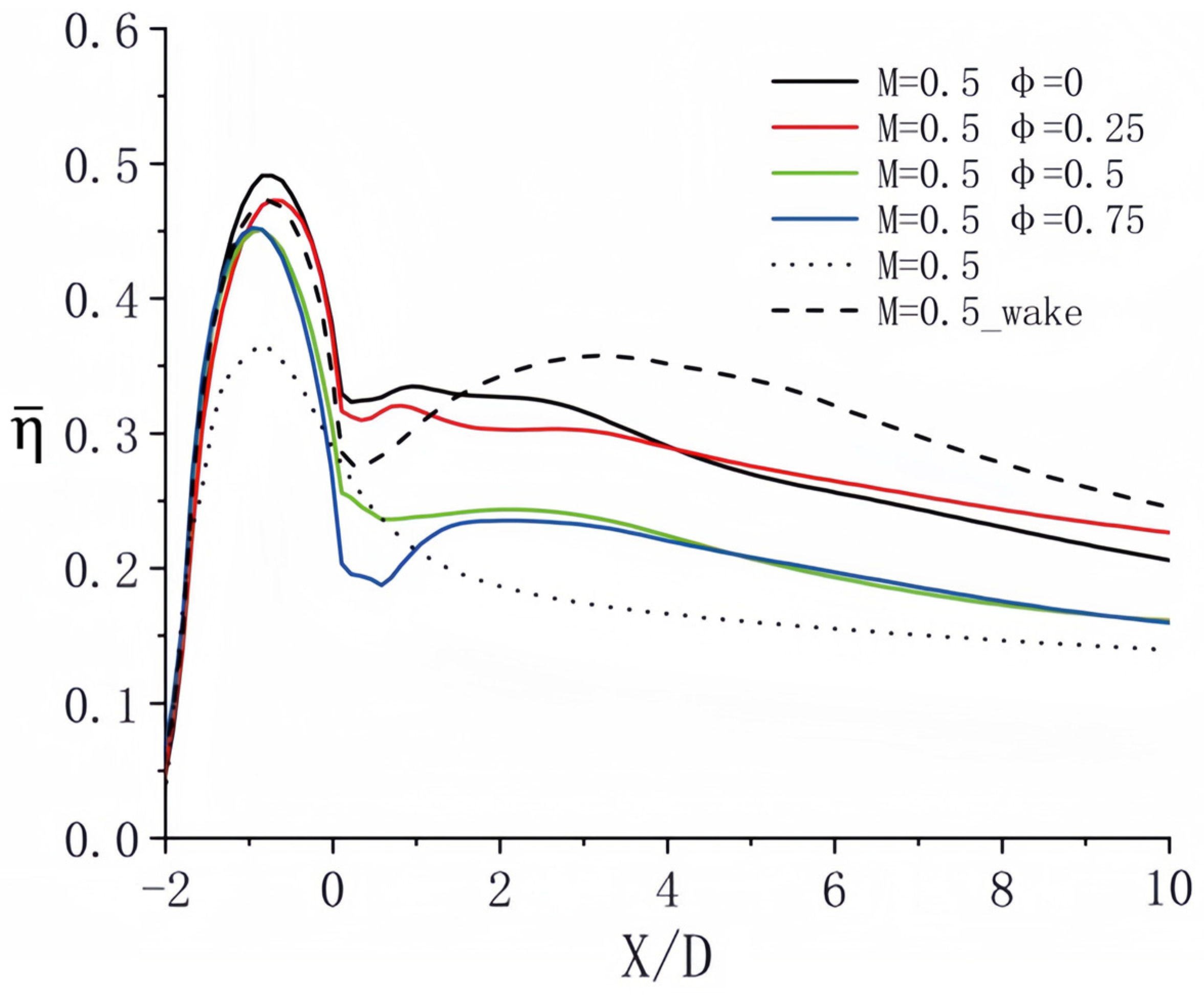
3.2. Blowing Ratio M = 0.5
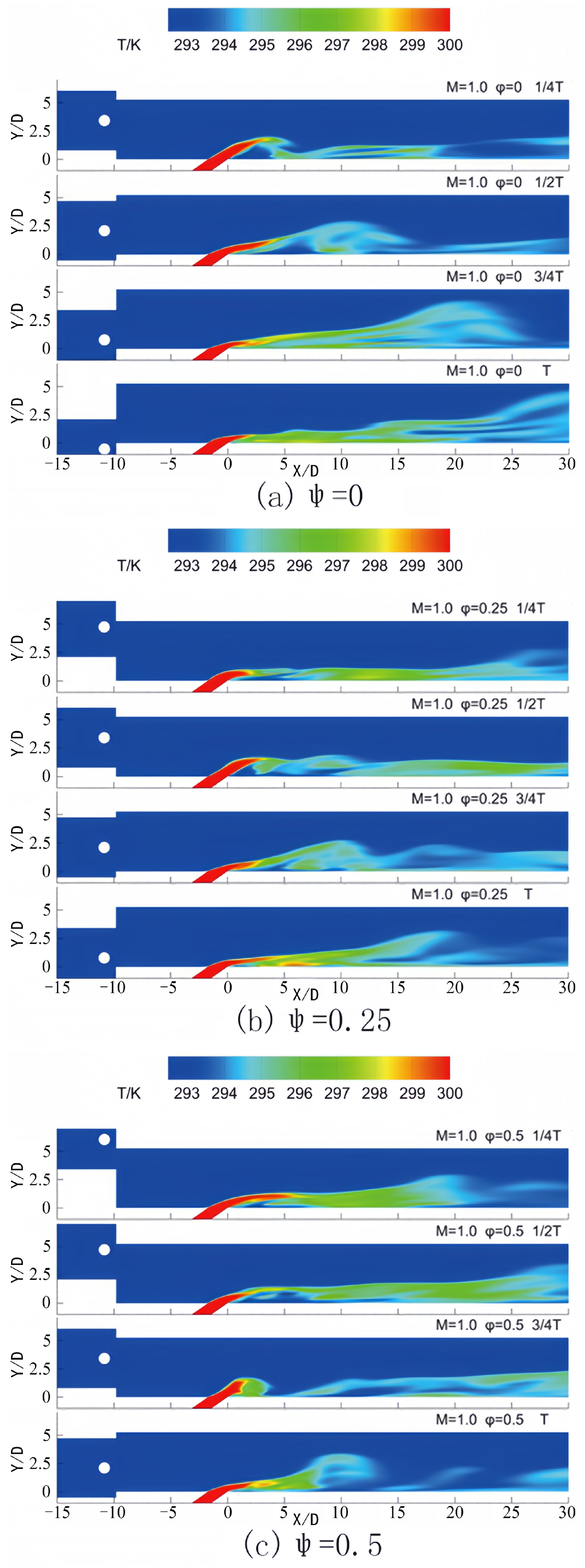
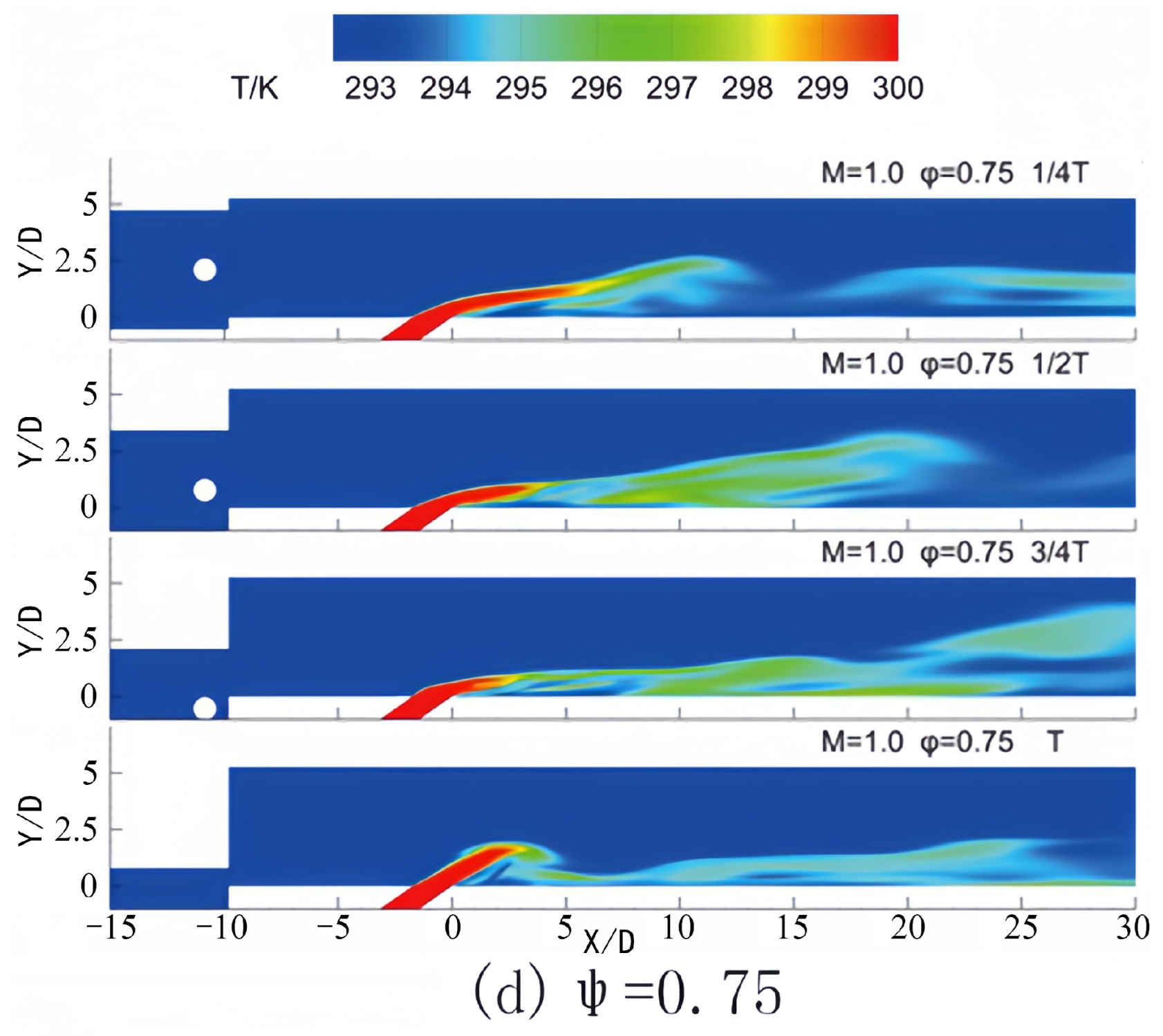
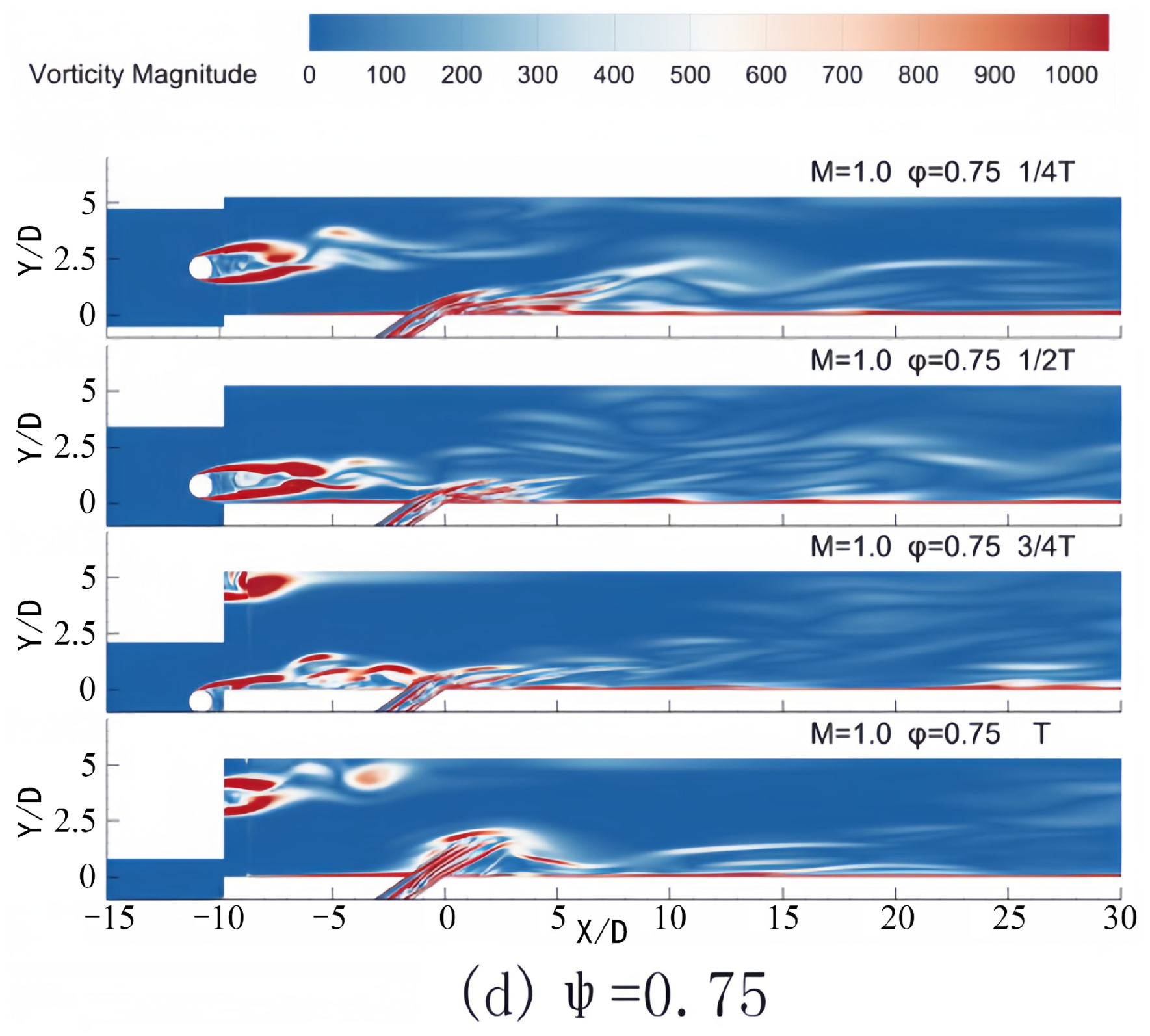
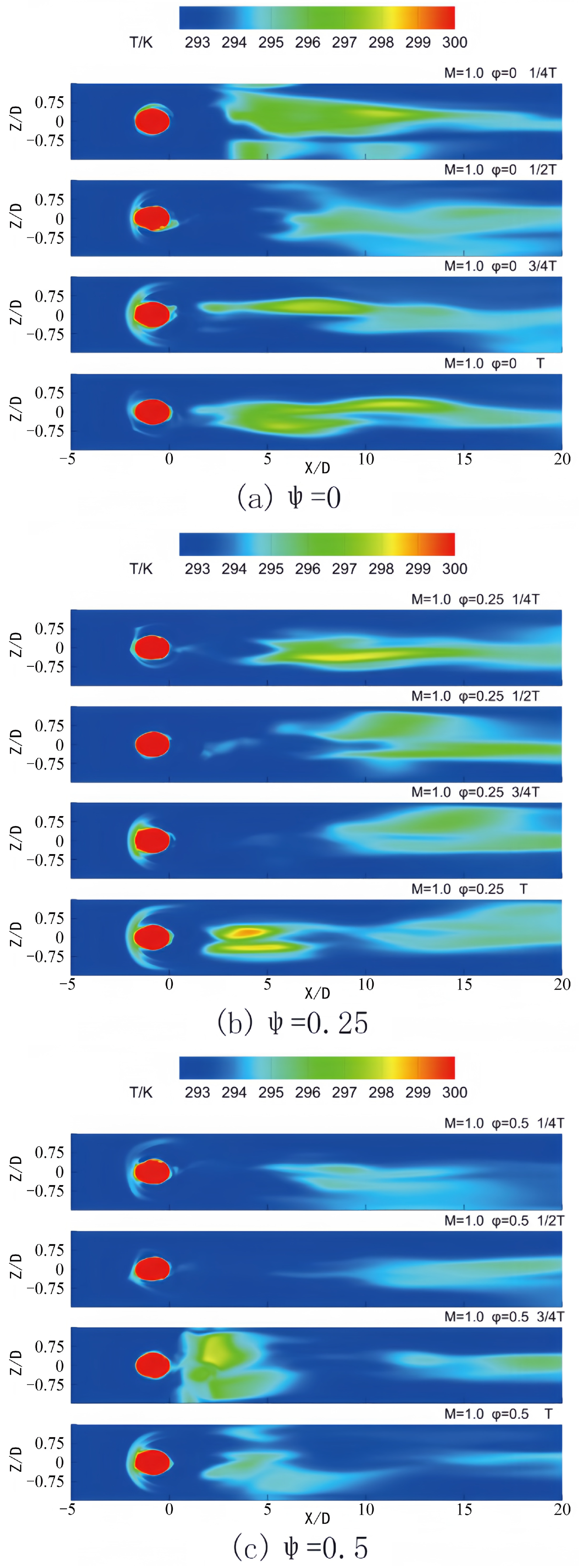
3.3. Blowing Ratio M = 1.0
4. Conclusions
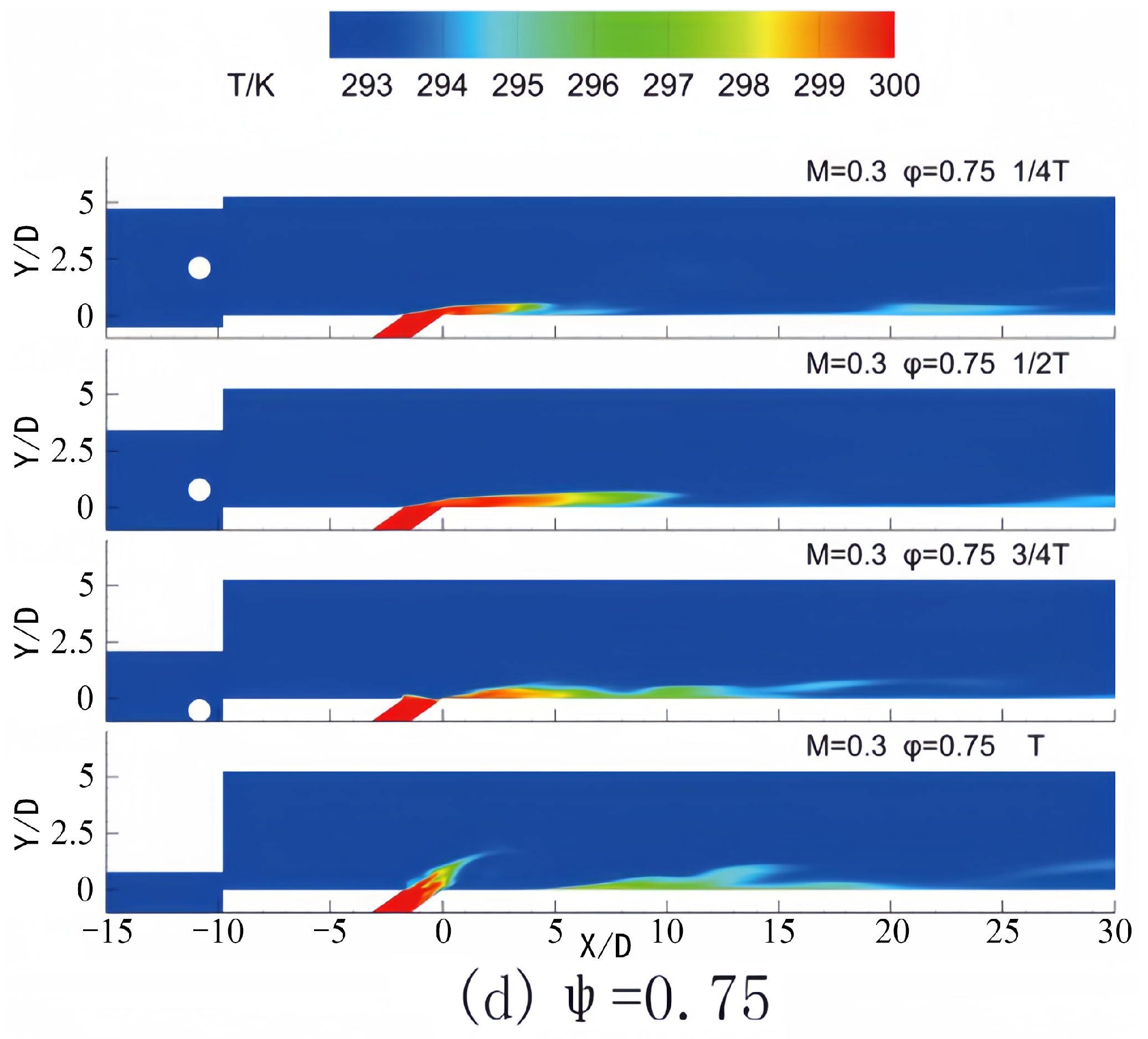
- For a blowing ratio of M = 0.3, the variations in the blowing ratio are minimal, resulting in the predominance of the wake sweeping process. Therefore, it was essential to couple the surface affected by the wake with the low blowing ratio stage of the pulsating jet. Moreover, due to the low overall blowing ratio, the film structure was susceptible to being lifted and damaged by the wake, adversely affecting the cooling efficiency.
- At a blowing ratio of M = 0.5, an increase in the average blowing ratio led to the predominance of the lift generated by the wake at the film hole. The jet lift induced by the wake was aligned with the high blowing ratio phase of the cycle. Consequently, when this lift coincided, the influence of the wake on jet pulsation was relatively diminished.
- At a blowing ratio of M = 1.0, the elevated average blowing ratio caused significant jet lift. The wake’s influence on the film’s cooling efficiency was primarily concentrated on the leading and trailing edges of the lifted area. The interaction at these regions encouraged the higher jet to adhere closely to the wall, thereby enhancing the film-cooling effect.
- In scenarios where the blowing ratio was low, coupling the wake-affected surface with the low-blowing ratio stage of the pulsating jet can achieve improved film cooling efficiency. Conversely, at higher blowing ratios, integrating the pulsating low-pressure phase at the film hole with the jet pulsating high-blowing ratio can optimize the film cooling effect. When the phase difference was equal to zero, the cooling efficiency of the pulsating film attains its peak across all blowing ratios, indicating a highly effective coupling strategy.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| D | diameter of the hole, mm |
| M | blowing ratio |
| T | cycle of the jet, s |
| adiabatic wall surface temperature, K | |
| jet temperature, K | |
| main flow temperature, K | |
| coolant velocity, m/s | |
| mainstream velocity, m/s | |
| x | Streamwise coordinate |
| y | vertical coordinate |
| z | spanwise coordinate |
| Greek symbols | |
| coolant density, kg/m3 | |
| mainstream density, kg/m3 | |
| adiabatic film cooling efficiency | |
| averaged film cooling efficiency | |
| phase lag, T |
References
- Zhang, J.; Zhang, S.; Wang, C.; Tan, X. Recent advances in film cooling enhancement: A review. Chin. J. Aeronaut. 2020, 33, 1119–1136. [Google Scholar] [CrossRef]
- Jia, Q.; Du, W.; Li, X.; Luo, L.; Jiao, Y.; Yan, H. Effects of blowing ratios and lip thicknesses on film cooling for trailing edge cutback with latticework ducts. Appl. Therm. Eng. 2024, 252, 123640. [Google Scholar] [CrossRef]
- Cao, N.; Li, X.; Wu, Z.; Luo, X. Effect of film hole geometry and blowing ratio on film cooling performance. Appl. Therm. Eng. 2020, 165, 114578. [Google Scholar] [CrossRef]
- Wang, C.; Wang, Y.; Wang, H.; Zhang, J.; Alting, S.A. Effect of coolant pulsation on film cooling performance on flat plate. Appl. Therm. Eng. 2023, 219, 119493. [Google Scholar] [CrossRef]
- Wang, Y.; Luo, Z.; Zhou, Y.; Peng, W. Large eddy simulation of pulsating film cooling on turbine vane. Aerosp. Sci. Technol. 2024, 148, 109103. [Google Scholar] [CrossRef]
- Song, H.; Ye, L.; Wang, X.; Liu, C.; Liang, X.; Ji, X. Assessing the effect of swirl flow on the film cooling effectiveness of a vane pressure surface. Int. Commun. Heat Mass Transf. 2025, 160, 108327. [Google Scholar] [CrossRef]
- Goldstein, R.J. Film Cooling. Adv. Heat Transf. 1971, 7, 321–379. [Google Scholar]
- Cheng, H.; Wen, Z.X.; Zhao, Y.C.; Wu, Z.Y.; Ren, X.; Yue, Z.F. Effect and optimization of geometric parameters and arrangement on film cooling performance of fan-shaped holes based on generalized regression neural network. Int. Commun. Heat Mass Transf. 2024, 158, 107868. [Google Scholar] [CrossRef]
- Ni, H.; Wang, M.J.; Jiang, P.X.; Peng, W.; Zhu, Y.H. A numerical study of segmented cooling-stream injection in supersonic film cooling. Chin. J. Aeronaut. 2022, 35, 156–171. [Google Scholar] [CrossRef]
- Saeed, R.; Khodayar, J.; Ali, A.; Vahid, V. Proposal of innovative arc-shaped wall-jet film cooling: A numerical investigation. Int. J. Thermofluids 2024, 24, 100842. [Google Scholar]
- Li, B.R.; Liu, C.L.; Ye, L.; Ren, M.; Wang, S.Y.; Liu, S. Film cooling performance evaluation of bi-directional diffusion hole with compound-angle on turbine blade: Study on spanwise width. Appl. Therm. Eng. 2024, 256, 124122. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, J.Z.; Wang, C.H.; Zhu, X.D. Multi-objective optimization of laidback fan-shaped film cooling hole on turbine vane suction surface. Heat Mass Transf. 2019, 55, 1181–1194. [Google Scholar] [CrossRef]
- Kalghatgi, P.; Acharya, S. Improved film cooling effectiveness with a round film cooling hole embedded in a contoured crater. J. Turbomach. 2015, 137, 101006. [Google Scholar] [CrossRef]
- Zhang, S.C.; Zhang, J.Z.; Tan, X.M. Improvement on shaped-hole film cooling effectiveness by integrating upstream sand-dune-shaped ramps. Chin. J. Aeronaut. 2021, 34, 42–55. [Google Scholar] [CrossRef]
- Kim, J.H.; Kang, C.W. A novel design for film-cooling: Cooling holes with inlet groove. Int. J. Therm. Sci. 2024, 195, 108649. [Google Scholar] [CrossRef]
- Zhang, K.; Li, Z.; Hao, M.; Li, Z.; Li, J. Experimental and numerical study on integrated film cooling of turbine endwall by upstream slot leakage and blade showerhead jets. Appl. Therm. Eng. 2024, 257, 124423. [Google Scholar] [CrossRef]
- Yang, X.; Zhao, Q.; Feng, Z. Experimental evaluation of cooling effectiveness from novel film holes over turbine endwalls with inlet swirl. Int. J. Therm. Sci. 2022, 174, 107434. [Google Scholar] [CrossRef]
- Yang, X.; Zhao, Q.; Wu, H.; Feng, Z. Film cooling effectiveness from upstream purge slots of a turbine vane endwall: Experiment, modeling, and correlation. Int. J. Heat Mass Transf. 2024, 219, 124899. [Google Scholar] [CrossRef]
- Yang, X.; Zhang, K.; Wu, J.; Lei, J.; Su, P.; Fang, Y. Numerical analysis of vane endwall film cooling and heat transfer with different mainstream turbulence intensities and blowing ratios. Int. J. Therm. Sci. 2022, 175, 107482. [Google Scholar] [CrossRef]
- Xu, Q.; Du, Q.; Wang, P.; Xiao, X. Numerical investigation of blowing ratio, density ratio and axial position of film holes on the vane endwall film cooling effectiveness with upstream step. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2022, 236, 541–553. [Google Scholar] [CrossRef]
- Vargas, S.G.; Alvarado, G.D.; Daza, D.C. The Effects of Mainstream Reynolds Number and Blowing Ratio on Film Cooling of Gas Turbine Vanes. Fluids 2023, 8, 263. [Google Scholar] [CrossRef]
- Liu, Y.; Jia, Y.; He, X.; Meng, Z.; Xia, G.; Li, K. Investigation of the endwall film holes layout strategy based on the conjugate temperature gradient distribution in gas turbine blade cooling. Appl. Therm. Eng. 2025, 263, 125447. [Google Scholar] [CrossRef]
- Bogard, D.G.; Thole, K. A Gas Turbine Film Cooling. J. Propuls. Power 2012, 22, 249–270. [Google Scholar] [CrossRef]
- Zhao, Z.; Wen, F.; Tang, X.; Song, J.; Wang, Z. Large eddy simulation of pulsed film cooling with vortex generators. Int. J. Heat Mass Transf. 2021, 180, 121806. [Google Scholar] [CrossRef]
- Ke, Z.; Wang, J. Numerical investigations of pulsed film cooling on an entire turbine vane. Appl. Therm. Eng. 2015, 87, 117–126. [Google Scholar] [CrossRef]
- Du, K.; Pei, X.; Liang, T.; Wang, H.; Liu, C.; Sunden, B. Experimental and computational investigation of the regional endwall cooling performance considering influences of film holes layouts and purge slot shape. Therm. Sci. Eng. Prog. 2025, 59, 103333. [Google Scholar] [CrossRef]
- Hossain, A.M.; Ameri, A.; Gregory, W.J.; Bons, J.P. Sweeping Jet Film Cooling at High Blowing Ratio on a Turbine Vane. J. Turbomach. 2020, 142, 121010. [Google Scholar] [CrossRef]
- Ye, Q.; Zhang, Y.; Wei, J. A comprehensive review of pulsating flow on heat transfer enhancement. Appl. Therm. Eng. 2021, 196, 117275. [Google Scholar] [CrossRef]
- Du, W.; Luo, L.; Wang, S.; Sunden, B. Film cooling in the trailing edge cutback with different land shapes and blowing ratios. Int. Commun. Heat Mass Transf. 2021, 125, 105311. [Google Scholar] [CrossRef]
- Wang, Q.; Moosania, M.; Zhou, C. Effects of an incoming vortex on the film cooling jet. Int. J. Heat Mass Transf. 2021, 185, 122323. [Google Scholar] [CrossRef]
- Sultan, Q.; Lalizel, G.; Fenot, M.; Dorignac, E. Influence of coolant jet pulsation on the convective film cooling of an adiabatic wall. J. Heat Transf. 2017, 139, 022201. [Google Scholar] [CrossRef]
- Burdet, A.; Abhari, R.S. Influence of Near Hole Pressure Fluctuation on the Thermal Protection of a Film-Cooled Flat Plate. J. Heat Transfer. 2009, 131, 022202. [Google Scholar] [CrossRef]
- Huo, D.C.; Fang, H.L.; Gao, J.; Liao, Y.N.; Jiang, Z.Y. Unsteady vane-rotor secondary flow interaction of the endwall region in a 1.5-stage variable-geometry turbine. Energy 2024, 309, 133027. [Google Scholar] [CrossRef]
- Wang, T.Y.; Xuan, Y.M.; Han, X.S. The effects of stator-rotor interaction on unsteady characteristics of turbine tip leakage flow. Aerosp. Sci. Technol. 2023, 141, 108544. [Google Scholar] [CrossRef]
- Chen, D.W.; Zhu, H.R.; Liu, C.L.; Li, H.T.; Li, B.R.; Zhou, D.E. Combined effects of unsteady wake and free-stream turbulence on turbine blade film cooling with laid-back fan-shaped holes using PSP technique. Int. J. Heat Mass Transf. 2019, 133, 382–392. [Google Scholar] [CrossRef]
- Abadi, S.M.H.B.; Zirak, S.; Zargarabadi, M.R. Effect of pulsating injection and mainstream attack angle on film cooling performance of a gas turbine blade. Phys. Fluids 2020, 32, 117102. [Google Scholar] [CrossRef]
- Zhao, Z.; Wen, F.; Li, Z.; Wan, C.; Zhang, X.; Wang, S. Effects of vortex generators and pulsation on compound angle film cooling. Appl. Therm. Eng. 2024, 244, 122737. [Google Scholar] [CrossRef]
- Li, F.; Liu, Z.; Tao, Y.; Zhang, W.; Feng, Z. A conjugate study on the application of combined impingement and film cooling for a turbine blade tip. Appl. Therm. Eng. 2024, 252, 123594. [Google Scholar] [CrossRef]
- Wang, L.; Li, H.; Xie, G.; Zhou, Z. Effect of blowing ratio, rotation, and film hole row location on film cooling on the suction surface of a rotating turbine blade. Int. J. Heat Mass Transf. 2023, 208, 124048. [Google Scholar] [CrossRef]
- Coulthard, S.M.; Volino, R.J.; Flack, K.A. Effect of Jet Pulsing on Film Cooling: Part 1—Effectiveness and Flowfield Temperature Results. J. Turbomach. 2007, 129, 232–246. [Google Scholar] [CrossRef]









Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, Q.; Shi, L. Numerical Study on the Coupled Impact of Periodic Wake and Pulsating Jet on Film Cooling Efficiency on a Flat Wall. Appl. Sci. 2025, 15, 2558. https://doi.org/10.3390/app15052558
Peng Q, Shi L. Numerical Study on the Coupled Impact of Periodic Wake and Pulsating Jet on Film Cooling Efficiency on a Flat Wall. Applied Sciences. 2025; 15(5):2558. https://doi.org/10.3390/app15052558
Chicago/Turabian StylePeng, Qiushou, and Liuliu Shi. 2025. "Numerical Study on the Coupled Impact of Periodic Wake and Pulsating Jet on Film Cooling Efficiency on a Flat Wall" Applied Sciences 15, no. 5: 2558. https://doi.org/10.3390/app15052558
APA StylePeng, Q., & Shi, L. (2025). Numerical Study on the Coupled Impact of Periodic Wake and Pulsating Jet on Film Cooling Efficiency on a Flat Wall. Applied Sciences, 15(5), 2558. https://doi.org/10.3390/app15052558





