Single-Glazed Vacuum Tube Collector with SnAl2O3 Selective Flat Absorber Plate and Gravity Single-Stage Direct Water Flow: A Comprehensive Geometric Optimization
Abstract
1. Introduction
2. Materials and Methods
2.1. Research Subject
2.2. Mathematical Model
2.2.1. Thermal Efficiency and Solar Heat
2.2.2. Useful Heat Power
2.2.3. Heat Losses
2.2.4. Incident Solar Irradiance
2.2.5. Absorbed Solar Irradiance
2.2.6. Optical Efficiency
2.2.7. Solar Incident Angle
2.2.8. Incoming Solar Irradiance
2.2.9. Iterative Calculation Algorithm
- (1)
- (2)
- the glass tube temperature Tgl [K] is assumed;
- (3)
- the results of the initial simulations are printed: Qnet, Qloss, Qheat and η;
- (4)
- the results of the control Equations (32) and (33) are printed;
- (5)
- the fulfillment of the condition |qabs-gt-qgt-o| ≤ 0.01 W/m2 (from Equations (32) and (33)) is checked.
- (6)
- If the condition from point 5 is met—the final simulation results are printed: Qnet, Qloss, Qheat and η;
- (7)
- if the condition from point 5 is not met—the value of Tgl [K] is changed and the entire process is repeated until the condition from point 5 is met.
2.3. Multi-Criteria Analysis
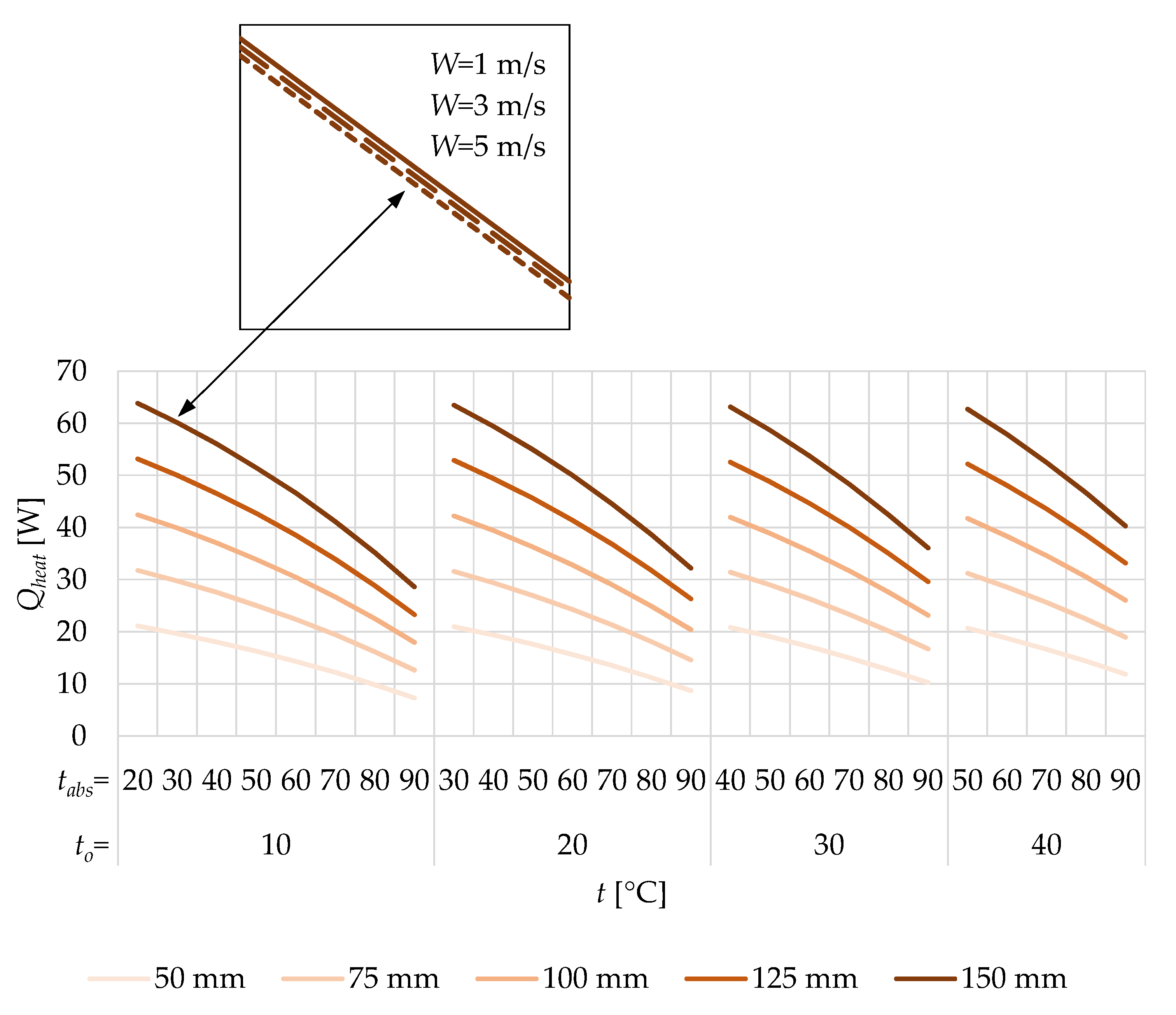
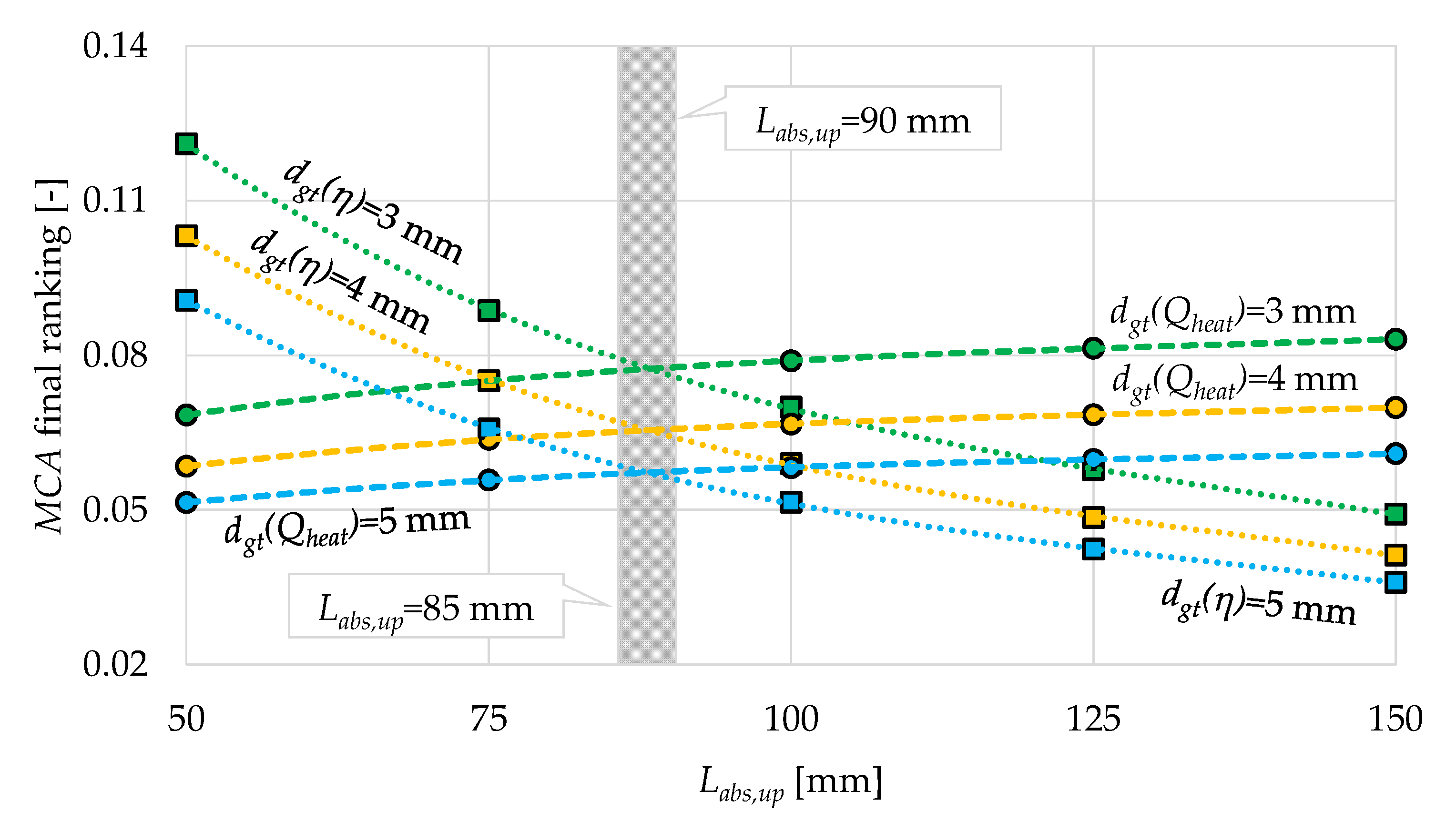
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- National Renewable Energy Laboratory. Available online: https://www.nrel.gov/ (accessed on 3 January 2025).
- Alam, T.; Balam, N.B.; Kulkarni, K.S.; Siddiqui, M.I.H.; Kapoor, N.R.; Meena, C.S.; Kumar, A.; Cozzolino, R. Performance augmentation of the flat plate solar thermal collector: A review. Energies 2021, 14, 6203. [Google Scholar] [CrossRef]
- Olia, H.; Torabi, M.; Bahiraei, M.; Ahmadi, M.H.; Goodarzi, M.; Safaei, M.R. Application of nanofluids in thermal performance enhancement of parabolic trough solar collector: State-of-the-art. Appl. Sci. 2019, 9, 463. [Google Scholar] [CrossRef]
- Tyagi, V.V.; Nagilla, D.K.; Selvaraj, J.; Chopra, K.; Kothari, R.; Pandey, A.K. Thermal energy storage in phase change material integrated solar collectors for air heating application. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1127, 012006. [Google Scholar] [CrossRef]
- Omeiza, L.A.; Abid, M.; Dhanasekaran, A.; Subramanian, Y.; Raj, V.; Kozak, K.; Mamudu, U.; Azad, A.K. Application of solar thermal collectors for energy consumption in public buildings—An updated technical review. J. Eng. Res. 2023, 12, 994–1010. [Google Scholar] [CrossRef]
- Evangelisti, L.; Vollaro, R.D.L.; Asdrubali, F. Latest advances on solar thermal collectors: A comprehensive review. Renew. Sustain. Energy Rev. 2019, 114, 109318. [Google Scholar] [CrossRef]
- Barrasso, M.; Langella, G.; Amoresano, A.; Iodice, P. Latest advances in thermal energy storage for solar plants. Processes 2023, 11, 1832. [Google Scholar] [CrossRef]
- Solar Water Heater. Available online: https://www.jinyi-solar.com/ (accessed on 12 January 2025).
- Lukić, N.; Babić, M. Solar Energy—Monography, 1st ed.; Faculty of Engineering University of Kragujevac: Kragujevac, Serbia, 2008. [Google Scholar]
- Sakhrieh, A.; Al-Ghandoor, A. Experimental investigation of the performance of five types of solar collectors. Energy Convers. Manag. 2013, 65, 715–720. [Google Scholar] [CrossRef]
- Deng, J.; O’Donovan, T.S.; Tian, Z.; King, J.; Speake, S. Thermal performance predictions and tests of a novel type of flat plate solar thermal collectors by integrating with a freeze tolerance solution. Energy Convers. Manag. 2019, 198, 111784. [Google Scholar] [CrossRef]
- Nikolić, N.; Lukić, N. Theoretical and experimental investigation of the thermal performance of a double exposure flat-plate solar collector. Sol. Energy 2015, 119, 100–113. [Google Scholar] [CrossRef]
- Abd, H.M.; Alomar, O.R.; Ali, F.A.; Salih, M.M.M. Experimental study of compound parabolic concentrator with flat plate receiver. Appl. Therm. Eng. 2020, 166, 114678. [Google Scholar]
- Nikolić, N.N.; Lukić, N.S.; Nešović, A.M.; Nikolić, D.M. Optimal size and position of the planar back reflector moveable only in the direction normal to the bifacial solar collector plane. Therm. Sci. 2024, 28, 4483–4497. [Google Scholar] [CrossRef]
- Neville, R.C. Solar energy collector orientation and tracking mode. Sol. Energy 1978, 20, 7–11. [Google Scholar] [CrossRef]
- Drago, P.A. A simulated comparison of the useful energy gain in a fixed and a fully tracking flat plate collector. In Proceedings of the Solar Energy International Progress, Cairo, Egypt, 16–22 June 1978. [Google Scholar]
- Maia, C.B.; Ferreira, A.G.; Hanriot, S.M. Evaluation of a tracking flat-plate solar collector in Brazil. Appl. Therm. Eng. 2014, 73, 953–962. [Google Scholar] [CrossRef]
- Nešović, A.M.; Lukić, N.S.; Josijević, M.M.; Jurišević, N.M.; Nikolić, N.N. Novel flat-plate solar collector with an inclined NS axis and relative EW tracking absorbers and the numerical analysis of its potentials. Therm. Sci. 2024, 28, 2905–2916. [Google Scholar] [CrossRef]
- Akhter, J.; Gilani, S.I.; Al-Kayiem, H.H.; Ali, M. Optical performance analysis of single flow through and concentric tube receiver coupled with a modified CPC collector under different configurations. Energies 2019, 12, 4147. [Google Scholar] [CrossRef]
- Martínez-García, A.; Vincent, M.; Rubiolo, V.; Domingos, M.; Canela, M.C.; Oller, I.; Fernández-Ibáñez, P.; Polo-López, M.I. Assessment of a pilot solar V-trough reactor for solar water disinfection. Chem. Eng. J. 2020, 399, 125719. [Google Scholar] [CrossRef]
- Kurhe, N.; Pathak, A.; Deshpande, K.; Jadkar, S. Compound parabolic solar collector–performance evaluation as per standard test method and actual field conditions for industrial process heat application in Indian context. Energy Sustain. Dev. 2020, 57, 98–108. [Google Scholar] [CrossRef]
- Chai, S.; Yao, J.; Liang, J.D.; Chiang, Y.C.; Zhao, Y.; Chen, S.L.; Dai, Y. Heat transfer analysis and thermal performance investigation on an evacuated tube solar collector with inner concentrating by reflective coating. Sol. Energy 2021, 220, 175–186. [Google Scholar] [CrossRef]
- Schumann, J.; Schiebler, B.; Giovannetti, F. Performance evaluation of an evacuated tube collector with a low-cost diffuse reflector. Energies 2021, 14, 8209. [Google Scholar] [CrossRef]
- Dinesh, S.N.; Ravi, S.; Kumar, P.M.; Subbiah, R.; Karthick, A.; Saravanakumar, P.T.; Pranav, R.A. Study on an ETC solar water heater using flat and wavy diffuse reflectors. Mater. Today Proc. 2021, 47, 5228–5232. [Google Scholar] [CrossRef]
- Li, Q.; Zheng, C.; Shirazi, A.; Mousa, O.B.; Moscia, F.; Scott, J.A.; Taylor, R.A. Design and analysis of a medium-temperature, concentrated solar thermal collector for air-conditioning applications. Appl. Energy 2017, 190, 1159–1173. [Google Scholar] [CrossRef]
- Li, Q.; Zheng, C.; Mesgari, S.; Hewkuruppu, Y.L.; Hjerrild, N.; Crisostomo, F.; Rosengarten, G.; Scott, J.A.; Taylor, R.A. Experimental and numerical investigation of volumetric versus surface solar absorbers for a concentrated solar thermal collector. Sol. Energy 2016, 136, 349–364. [Google Scholar] [CrossRef]
- Li, Q.; Tehrani, S.S.M.; Taylor, R.A. Techno-economic analysis of a concentrating solar collector with built-in shell and tube latent heat thermal energy storage. Energy 2017, 121, 220–237. [Google Scholar] [CrossRef]
- Nešović, A.; Lukić, N.; Taranović, D.; Nikolić, N. Theoretical and experimental investigation of the glass tube solar collector with inclined NS axis and relative EW single-axis tracking flat absorber. Appl. Therm. Eng. 2024, 236, 121842. [Google Scholar] [CrossRef]
- Bellos, E.; Tzivanidis, C. A detailed investigation of an evacuated flat plate solar collector. Appl. Therm. Eng. 2023, 234, 121334. [Google Scholar] [CrossRef]
- Nešović, A.; Kowalik, R.; Cvetković, D.; Janaszek, A. Multi-criteria decision-making method for simple and fast dimensioning and selection of glass tube collector type based on the iterative thermal resistance calculation algorithm with experimental validation. Appl. Sci. 2024, 14, 6603. [Google Scholar] [CrossRef]
- Ditta, A.; Tabish, A.N.; Mujtaba, M.A.; Amjad, M.; Yusuf, A.A.; Chaudhary, G.Q.; Razzaq, L.; Abdelrahman, A.; Kalam, M.A. Experimental investigation of a hybrid configuration of solar thermal collectors and desiccant indirect evaporative cooling system. Front. Energy Res. 2022, 10, 979942. [Google Scholar] [CrossRef]
- Supankanok, R.; Sriwong, S.; Ponpo, P.; Wu, W.; Chandra-Ambhorn, W.; Anantpinijwatna, A. Modification of a solar thermal collector to promote heat transfer inside an evacuated tube solar thermal absorber. Appl. Sci. 2021, 11, 4100. [Google Scholar] [CrossRef]
- Ma, L.; Lu, Z.; Zhang, J.; Liang, R. Thermal performance analysis of the glass evacuated tube solar collector with U-Tube. Build. Environ. 2010, 45, 1959–1967. [Google Scholar] [CrossRef]
- Kaya, H.; Arslan, K. Numerical investigation of efficiency and economic analysis of an evacuated U-tube solar collector with different nanofluids. Heat Mass Transf. 2019, 55, 581–593. [Google Scholar] [CrossRef]
- Morrison, G.L.; Budihardjo, I.; Behnia, M. Measurement and simulation of flow rate in a water-in-glass evacuated tube solar water heater. Sol. Energy 2005, 78, 257–267. [Google Scholar] [CrossRef]
- Budihardjo, I.; Morrison, G.L.; Behnia, M. Natural circulation flow through water-in-glass evacuated tube solar collectors. Sol. Energy 2007, 81, 1460–1472. [Google Scholar] [CrossRef]
- Glembin, J.; Rockendorf, G.; Scheuren, J. Internal thermal coupling in direct-flow coaxial vacuum tube collectors. Sol. Energy 2010, 84, 1137–1146. [Google Scholar] [CrossRef]
- Abokersh, M.H.; El-Morsi, M.; Sharaf, O.; Abdelrahman, W. An experimental evaluation of direct flow evacuated tube solar collector integrated with phase change material. Energy 2017, 139, 1111–1125. [Google Scholar] [CrossRef]
- Rezaeian, M.; Dehaj, M.S.; Mohiabadi, M.Z.; Salarmofrad, M.; Shamsi, S. Experimental investigation into a parabolic solar collector with direct flow evacuated tube. Appl. Therm. Eng. 2021, 189, 116608. [Google Scholar] [CrossRef]
- Rabl, A. Active Solar Collectors and Their Applications, 1st ed.; Oxford University Press: New York, NY, USA, 1985. [Google Scholar]
- Yang, X.; Heng, K.; Dai, X.; Wu, X.; Liu, G. A Temperature-Dependent Cauer Model Simulation of IGBT Module With Analytical Thermal Impedance Characterization. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 3055–3065. [Google Scholar] [CrossRef]
- Zhang, J.; Shen, H.; Du, X.; Chen, R. Condition Monitoring the Inhomogeneous Thermal Fatigue of Multichip IGBT Module Based on the Thermal Attenuation Coefficient. IEEE Trans. Power Electron. 2025, 40, 2114–2125. [Google Scholar] [CrossRef]
- Orman, Ł.J. Enhancement of pool boiling heat transfer with pin-fin microstructures. J. Enhanc. Heat Transf. 2016, 23, 137–153. [Google Scholar] [CrossRef]
- Kowalik, R.; Nešović, A.; Cvetković, D.; Janaszek, A.; Kozłowski, T. Numerical Simulation of Climate Change Impact on Energy, Environmental and Economic Performances of Small Single-Family Houses Equipped with Trombe Walls and Fixed Horizontal Overhangs. Energies 2024, 17, 6275. [Google Scholar] [CrossRef]
- Rajput, R.K. Engineering Thermodynamics, 3rd ed.; Jones & Bartlett Publishers: Boston, MA, USA, 2007. [Google Scholar]
- Kalogirou, S.A.; Karellas, S.; Braimakis, K.; Stanciu, C.; Badescu, V. Exergy analysis of solar thermal collectors and processes. Prog. Energy Combust. Sci. 2016, 56, 106–137. [Google Scholar] [CrossRef]
- Dąbek, L.; Kapjor, A.; Orman, Ł.J. Ethyl alcohol boiling heat transfer on multilayer meshed surfaces. AIP Conf. Proc. 2016, 1745, 020005. [Google Scholar]
- Majewski, G.; Telejko, M.; Orman, Ł.J. Preliminary results of thermal comfort analysis in selected buildings. E3S Web Conf. 2017, 17, 00056. [Google Scholar] [CrossRef]
- Tang, R.; Gao, W.; Yu, Z.; Chen, H. Optimal tilt-angles of all-glass evacuated tube solar collectors. Energy 2009, 34, 1387–1395. [Google Scholar] [CrossRef]
- Mehregan, M.; Abbasi, M.; Khalilian, P.; Majid Hashemian, S.; Madadi, A. Energy, economic, environmental investigations and optimization of a combined cooling, heating and power system with hybrid prime mover of gas engine and flat plate solar collector. Energy Convers. Manag. 2022, 251, 115018. [Google Scholar] [CrossRef]
- Shah, L.J.; Furbo, S. Vertical evacuated tubular-collectors utilizing solar radiation from all directions. Appl. Energy 2004, 78, 371–395. [Google Scholar]
- Hellstrom, B.; Adsten, M.; Nostell, P.; Karlsson, B.; Wackelgard, E. The impact of optical and thermal properties on the performance of flat plate solar collectors. Renew. Energy 2003, 28, 331–344. [Google Scholar] [CrossRef]
- Porowski, R.; Kowalik, R.; Grzmiączka, M.; Jurišević, N.; Gawdzik, J. Influence of initial temperature on laminar burning velocity in hydrogen-air mixtures as potential for green energy carrier. Int. Commun. Heat Mass Transf. 2023, 146, 106861. [Google Scholar] [CrossRef]
- Nešović, A. Theoretical model of solar incident angle for an optionally oriented fixed flat surface. Technique 2022, 72, 328–333. [Google Scholar] [CrossRef]
- Erbs, D.G.; Klein, S.A.; Duffie, J.A. Estimation of the diffuse radiation fraction for hourly, daily and monthly-average global radiation. Sol. Energy 1982, 28, 293–302. [Google Scholar] [CrossRef]
- Recycling Today. Available online: https://www.recyclingtoday.com (accessed on 10 January 2025).
- Ramesh, T.; Prakash, R.; Shukla, K.K. Life cycle approach in evaluating energy performance of residential buildings in Indian context. Energy Build. 2012, 54, 259–265. [Google Scholar] [CrossRef]
- Ministry of Construction Transport and Infrastructure. Rulebook of Energy Efficiency. Available online: https://www.mgsi.gov.rs (accessed on 9 January 2025).
- Mitra, S.; Goswami, S.S. Application of simple average weighting optimization method in the selection of best desktop computer model. Adv. J. Grad. Res. 2019, 6, 60–68. [Google Scholar] [CrossRef]
- Kumar, A.; Sah, B.; Singh, A.R.; Deng, Y.; He, X.; Kumar, P.; Bansal, R.C. A review of multi-criteria decision making (MCDM) towards sustainable renewable energy development. Renew. Sustain. Energy Rev. 2017, 69, 596–609. [Google Scholar] [CrossRef]
- Puška, A.; Beganović, A.I.; Šadić, S. Model for investment decision making by applying the multi-criteria analysis method. Serbian J. Manag. 2018, 13, 7–28. [Google Scholar] [CrossRef]
- Nešović, A.; Kowalik, R.; Bojović, M.; Janaszek, A.; Adamczak, S. Elevational Earth-Sheltered Buildings with Horizontal Overhang Photovoltaic-Integrated Panels—New Energy-Plus Building Concept in the Territory of Serbia. Energies 2024, 17, 2100. [Google Scholar] [CrossRef]


| SC Type | Modification | Method | Description | Main Results | Year | Source |
|---|---|---|---|---|---|---|
| FPC | Reflector | Math/Exp | Manually operated mirror in all three orthogonal directions | η higher by 29.55% | 2015 | [12] |
| Exp | CPC/External | η higher by 26.5% | 2020 | [13] | ||
| Math | Moveable in the direction normal to the bifacial SC plane | η higher by 54–74% | 2024 | [14] | ||
| Tracking mechanism | Math | SAT/Inclined axis/E-W direction DAT | Gtot = 1370 kW/hr/m2 Gtot = 1460 kW/hr/m2 | 1978 | [15] | |
| DAT | η higher by 20% | [16] | ||||
| SAT/Horizontal axis/N-S direction SAT/Horizontal axis/E-W direction SAT/Vertical axis/E-W direction SAT/Inclined axis/E-W direction DAT | η = 57.12% η = 62.17% η = 59.51% η = 64.36% η = 67.25% | 2014 | [17] | |||
| Num | rSAT/Inclined axis/E-W direction | η higher by 20% | 2024 | [18] | ||
| ETC | Reflector | Num | CPC/External/N-S installation CPC/External/E-W installation | η = 69% η = 66.5% | 2019 | [19] |
| Exp | V-trough | Water treatment higher by 66% | 2020 | [20] | ||
| CPC/External | η = 45–64% | 2020 | [21] | |||
| Math/Exp | CPC/Internal | η = 65–72% | 2021 | [22] | ||
| Math/Num/Exp | Low-cost/Trapezoidal | Qheat higher by 30% | 2021 | [23] | ||
| Exp | Flat Wavy | ttw higher by 4 °C ttw higher by 6 °C | [24] | |||
| Tracking mechanism | Math/Num/Exp | CPC/Internal/rSAT/ Inclined axis/E-W direction | η = 54% at 80 °C η = 26% at 200 °C | 2016/2017 | [25,26,27] | |
| FPC/ETC | Tracking mechanism | Math/Exp | ABS/GT/rSAT/Inclined axis/ E-W direction | Qheat higher by 14–23% | 2024 | [28] |
| Specific solutions | Math/Num | Different temperature operation | η = 47.24% | 2023 | [29] | |
| Math/Exp | ABS/SGT/AL ABS/SGT/VL ABS/DGT/AL ABS/DGT/VL | Qloss = 5.29–64.42 W Qloss = 2.74–27.11 W Qloss = 12.65–47.14 W Qloss = 6.48–23.35 W | 2024 | [30] |
| Component | Material | ρ [kg/m3] | k [W/(mK)] | τ [-] | α [-] | ε [-] |
|---|---|---|---|---|---|---|
| FAP | Aluminum | 2700 | 203 | - | 0.88 | 0.25 |
| GT | Glass | 2200 | 0.8 | 0.9 | - | 0.9 |
| Scenario | m [kg] | SO [m2] | TSO [m2] | VO [m3] |
|---|---|---|---|---|
| Labs,up = 50 mm | 0.468 | 0.4 | 0.104 | 0.000315 |
| Labs,up = 75 mm | 0.575 | 0.6 | 0.144 | 0.000355 |
| Labs,up = 100 mm | 0.683 | 0.8 | 0.184 | 0.000395 |
| Labs,up = 125 mm | 0.791 | 0.1 | 0.224 | 0.000435 |
| Labs,up = 150 mm | 0.898 | 0.12 | 0.264 | 0.000475 |
| Scenario | m [kg] | SO [m2] | TSO [m2] | VO [m3] | |
|---|---|---|---|---|---|
| Labs,up = 50 mm | dgt = 3 mm | 0.946 | 0.048 | 0.151 | 0.00226 |
| dgt = 4 mm | 1.283 | 0.0496 | 0.156 | 0.00241 | |
| dgt = 5 mm | 1.632 | 0.0512 | 0.161 | 0.00257 | |
| Labs,up = 75 mm | dgt = 3 mm | 1.361 | 0.068 | 0.214 | 0.00454 |
| dgt = 4 mm | 1.836 | 0.0696 | 0.219 | 0.00475 | |
| dgt = 5 mm | 2.323 | 0.0712 | 0.224 | 0.00497 | |
| Labs,up = 100 mm | dgt = 3 mm | 1.775 | 0.088 | 0.276 | 0.0076 |
| dgt = 4 mm | 2.389 | 0.0896 | 0.281 | 0.00788 | |
| dgt = 5 mm | 3.014 | 0.0912 | 0.286 | 0.00816 | |
| Labs,up = 125 mm | dgt = 3 mm | 2.19 | 0.108 | 0.339 | 0.01145 |
| dgt = 4 mm | 2.942 | 0.1096 | 0.344 | 0.01179 | |
| dgt = 5 mm | 3.706 | 0.1112 | 0.349 | 0.01213 | |
| Labs,up = 150 mm | dgt = 3 mm | 2.605 | 0.128 | 0.402 | 0.01608 |
| dgt = 4 mm | 3.495 | 0.1296 | 0.407 | 0.01648 | |
| dgt = 5 mm | 4.397 | 0.1312 | 0.412 | 0.01689 | |
| Variable | Used Values | |
|---|---|---|
| V3 | tabs [°C] | 20; 30; 40; 50; 60; 70; 80; 90 |
| V4 | to [°C] | 10; 20; 30; 40 |
| V5 | W [m/s] | 1; 3; 5 |
| Scenario | CM [€] | CE [€] | Eemb [kWh] | eCO2 [kg] | |
|---|---|---|---|---|---|
| Labs,up = 50 mm | dgt = 3 mm | 57.605 | 28.803 | 2.46 | 1048.943 |
| dgt = 4 mm | 72.047 | 36.024 | 2.895 | 1043.093 | |
| dgt = 5 mm | 86.963 | 43.482 | 3.344 | 1037.29 | |
| Labs,up = 75 mm | dgt = 3 mm | 79.303 | 39.651 | 3.28 | 1625.579 |
| dgt = 4 mm | 99.664 | 49.832 | 3.894 | 1617.312 | |
| dgt = 5 mm | 120.499 | 60.249 | 4.522 | 1609.072 | |
| Labs,up = 100 mm | dgt = 3 mm | 101 | 50.5 | 4.1 | 2202.293 |
| dgt = 4 mm | 127.28 | 63.64 | 4.892 | 2191.592 | |
| dgt = 5 mm | 154.034 | 77.017 | 5.699 | 2180.907 | |
| Labs,up = 125 mm | dgt = 3 mm | 122.697 | 61.349 | 4.921 | 2779.042 |
| dgt = 4 mm | 154.897 | 77.448 | 5.891 | 2765.9 | |
| dgt = 5 mm | 187.57 | 93.785 | 6.876 | 2752.768 | |
| Labs,up = 150 mm | dgt = 3 mm | 144.395 | 72.197 | 5.741 | 3355.801 |
| dgt = 4 mm | 182.513 | 91.257 | 6.89 | 3340.215 | |
| dgt = 5 mm | 221.105 | 110.553 | 8.053 | 3324.632 | |
| Criterion | Geometric | Economic | Ecological | Sum | |||||
|---|---|---|---|---|---|---|---|---|---|
| wj [%] | 14 | 50 | 36 | 100 | |||||
| Sub-criterion | m | SO | TSO | VO | CM | CE | Eemb | eCO2 | SGVTC |
| wj,sc [%] | 5 | 5 | 2 | 2 | 35 | 15 | 18 | 18 | 100 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nešović, A.; Kowalik, R. Single-Glazed Vacuum Tube Collector with SnAl2O3 Selective Flat Absorber Plate and Gravity Single-Stage Direct Water Flow: A Comprehensive Geometric Optimization. Appl. Sci. 2025, 15, 1838. https://doi.org/10.3390/app15041838
Nešović A, Kowalik R. Single-Glazed Vacuum Tube Collector with SnAl2O3 Selective Flat Absorber Plate and Gravity Single-Stage Direct Water Flow: A Comprehensive Geometric Optimization. Applied Sciences. 2025; 15(4):1838. https://doi.org/10.3390/app15041838
Chicago/Turabian StyleNešović, Aleksandar, and Robert Kowalik. 2025. "Single-Glazed Vacuum Tube Collector with SnAl2O3 Selective Flat Absorber Plate and Gravity Single-Stage Direct Water Flow: A Comprehensive Geometric Optimization" Applied Sciences 15, no. 4: 1838. https://doi.org/10.3390/app15041838
APA StyleNešović, A., & Kowalik, R. (2025). Single-Glazed Vacuum Tube Collector with SnAl2O3 Selective Flat Absorber Plate and Gravity Single-Stage Direct Water Flow: A Comprehensive Geometric Optimization. Applied Sciences, 15(4), 1838. https://doi.org/10.3390/app15041838







