1. Introduction
Over time, the fundamental mission of engineers has been to create safe, strong, and resource-efficient structures. However, this goal has been complicated by the need to integrate new materials, methods, and technologies that can ensure the sustainability (i.e., economic, environmental and social) of building projects in a society with limited natural resources [
1,
2].
In this context, sustainability refers to meeting today’s societal needs without endangering future generations, guaranteeing a balance between economic, social, cultural, and environmental aspects [
3]. The amount of waste generated in construction by the demolition of existing buildings is considerable [
4,
5]. This increasing waste production represents a major challenge in many developing cities, which are experiencing remarkable growth due to increasing urban population and widespread urban development projects [
6,
7]. Globally, 35% of all solid waste is made up of construction and demolition waste (CDW) [
8], with more than 80% of this waste corresponding to concrete rubble, bricks, tiles, decorative materials, dust, and other materials used in construction [
9].
With over 25 billion tons produced each year, concrete is the most frequently used building material in the world [
10]. The volume of concrete comprises 70% to 80% aggregates, and in 2023 the estimated extraction of natural aggregates (NA) for use in concrete worldwide was close to 47.5 billion tons [
11,
12]. This is one of the reasons that has led to a shortage of natural aggregates around the world [
13,
14], posing a major challenge for future generations if natural resource consumption continues at the current rate. In addition, there has been growing concern regarding the environmental repercussions of concrete production, primarily related to cement, as it is thought to be the source of about 90% of concrete’s CO
2 emissions [
15,
16,
17].
Consequently, a significant amount of empirical research has been undertaken recently with the aim of alleviating the negative environmental effects related to the production and utilization of concrete. One strategy involves the valorization of various CDW types, with recycled concrete aggregates (RCA) being extensively studied due to their properties and characteristics [
7,
18]. Therefore, the primary goal is to diminish the utilization of natural aggregates alongside the amount of waste sent to landfill sites [
19].
There are clear differences between recycled and natural aggregates; for example, the former has a rough and porous surface texture resulting from the residual mortar attached to the particles of the aggregate. The properties of recycled aggregates (RA) are influenced by the characteristics of the source materials as well as by the characteristics of the concrete from which they are derived. Recycled aggregates exhibit a significant increase in water absorption capacity, which is their main difference with to natural aggregates. These differences can cause modifications in both the fresh and hardened state when using RA to manufacture concrete [
20]. In general, the partial or complete substitution of recycled aggregates in the concrete composition results in lower mechanical performance than for concrete made entirely from natural aggregates [
21,
22]. Replacing NA with RA can reduce concrete strength by up to 40%, and can also cause a decrease in other key properties [
17]. For this reason, several studies are currently analyzing ways to improve recycled aggregates to maximize their use and reduce their impact on new concrete [
23,
24,
25].
Despite this effect, the structural performance of reinforced concrete with RA is not clearly evident, as the flexural behavior is primarily influenced by the incorporation of steel reinforcement [
26]. Research into the use of recycled concrete aggregates has progressed in both unreinforced and reinforced concrete applications [
27,
28]. Studies of the structural performance and economic aspects of using recycled aggregate concrete elements in nonstructural elements shows that RA can be considered a reliable material for use in structural elements provided that it is properly applied [
29].
This trend has led to the development of specific regulations in several countries that control and promote the use of recycled aggregates as either a partial or complete substitute for natural aggregates. However, current regulations governing the use of RAs in concrete mostly limit their use to low replacement percentages. Therefore, it is necessary to look for processes that can improve the properties of RAs to increase the percentages of use without significantly affecting the properties of new concretes. The objective of this study was to analyze the behavior of coarse recycled aggregate (CRA) with an improvement process (i.e., coating with recycled binder paste, RBP) and verify the feasibility of using RAs in high percentages in reinforced concrete beams. The tested beams were designed using different percentages of treated RA. In addition, the load-deflection response, critical and end moments, load capacity, and critical and end strains of the beams were studied by evaluating the extent to which the provisions of existing codes such as ACI 318-19 and the Eurocode 2 predict the aforementioned characteristics. By providing a comparison with experimental results, this research can promote the use of recycled aggregates in structural projects.
3. Results
3.1. Mechanical Properties of Concrete
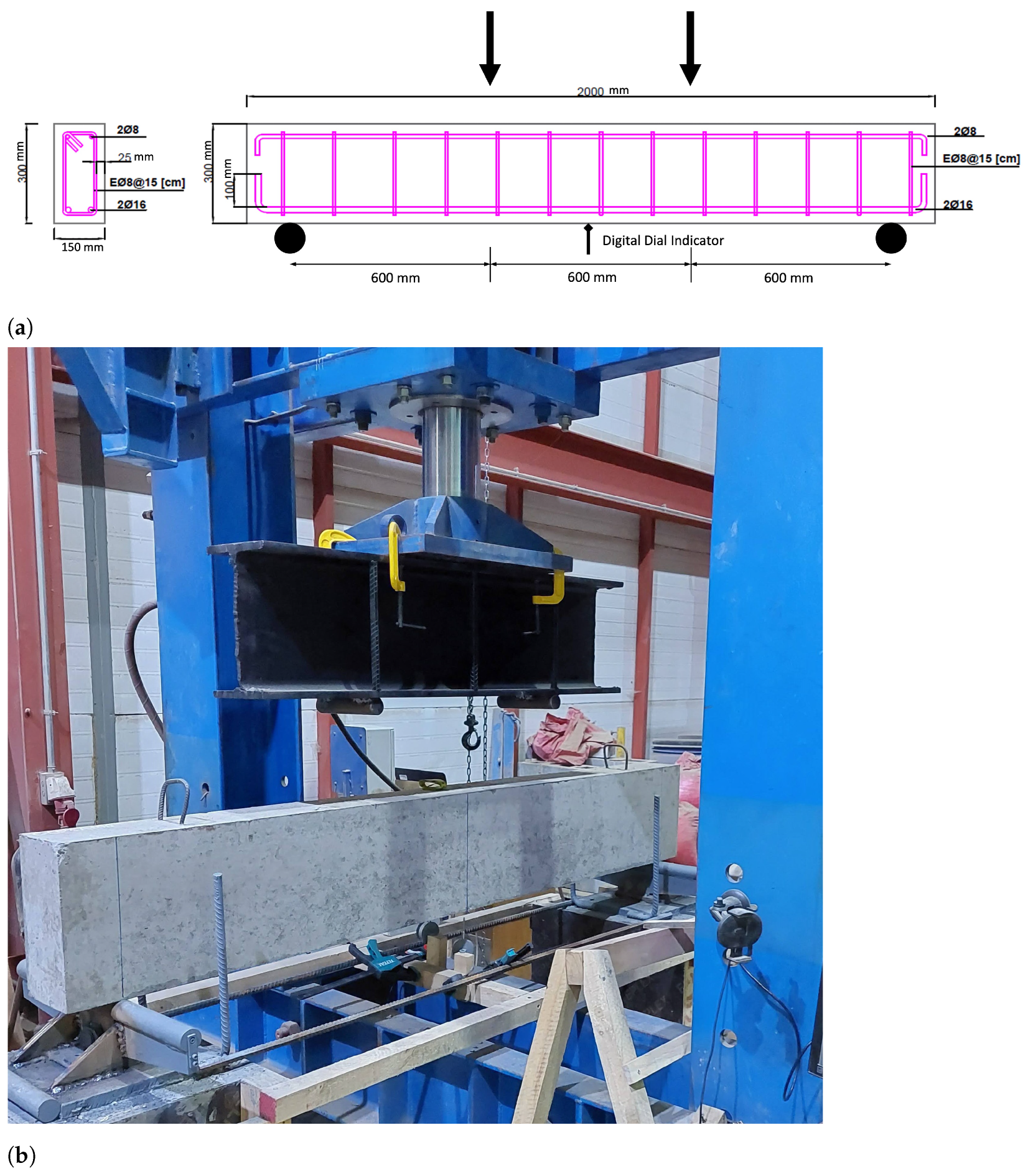
The compressive strength and modulus of elasticity of the series were obtained by testing three cylindrical specimens per series of
150 mm × 300 mm after 28 days of curing according to ASTM C39/C39M-21 [
36] and ASTM C469/C469M-14 [
37], respectively. Assessment of the flexural strength was conducted on prismatic specimens with dimensions of 150 mm × 150 mm × 550 mm with a curing period of 28 days, employing the third-point loading methodology with a four-point flexural test loading frame in accordance with ASTM C78/C78M-18 [
38]. The loads were applied to the edges of the central third of the prismatic specimens.
It is worth noting that the strength of recycled aggregate concrete generally exhibits a reduction in comparison to natural aggregate concrete [
23]. This reduction in strength is primarily attributed to the presence of old mortar attached to to the surface of the RA, resulting in a porous layer that increases water absorption and results in a lower density, leading to a decrease in mechanical properties [
13].
Therefore, the modulus of elasticity decreases when increasing the percentage of substitution of recycled aggregate [
39]. This is evident from the results obtained in
Table 3, where the series with only natural aggregates exhibits the highest modulus of elasticity among the specimens compared to the series using recycled aggregates.
After 28 days, the control series achieved the specified design strength of 30 MPa. In contrast, for the series containing recycled aggregates, an increase in the substitution percentage resulted in a reduction in the compressive strength of the concrete, indicating a negative relationship between the percentage of RA replacement and the compressive strength Kwan et al. [
40].
3.2. Beam Behavior—Experimental Results
3.2.1. Cracking Pattern and Mode of Failure
Figure 4 shows the cracking pattern of the beams tested at 28 days for the N100, CR50, and CR100 series. These cracks were marked and numbered at the end of the test and their length and thickness dimensions were recorded. All tested beams showed a similar failure pattern and number of cracks; vertical cracks were first generated within the central third, which is associated with flexural bending developing from the lower part of the beam towards the compression zone [
41]. During the last part of the test, diagonal cracks associated with shear failure formed, and tended to be more diagonal in shape as they grew further away from the central third [
19].
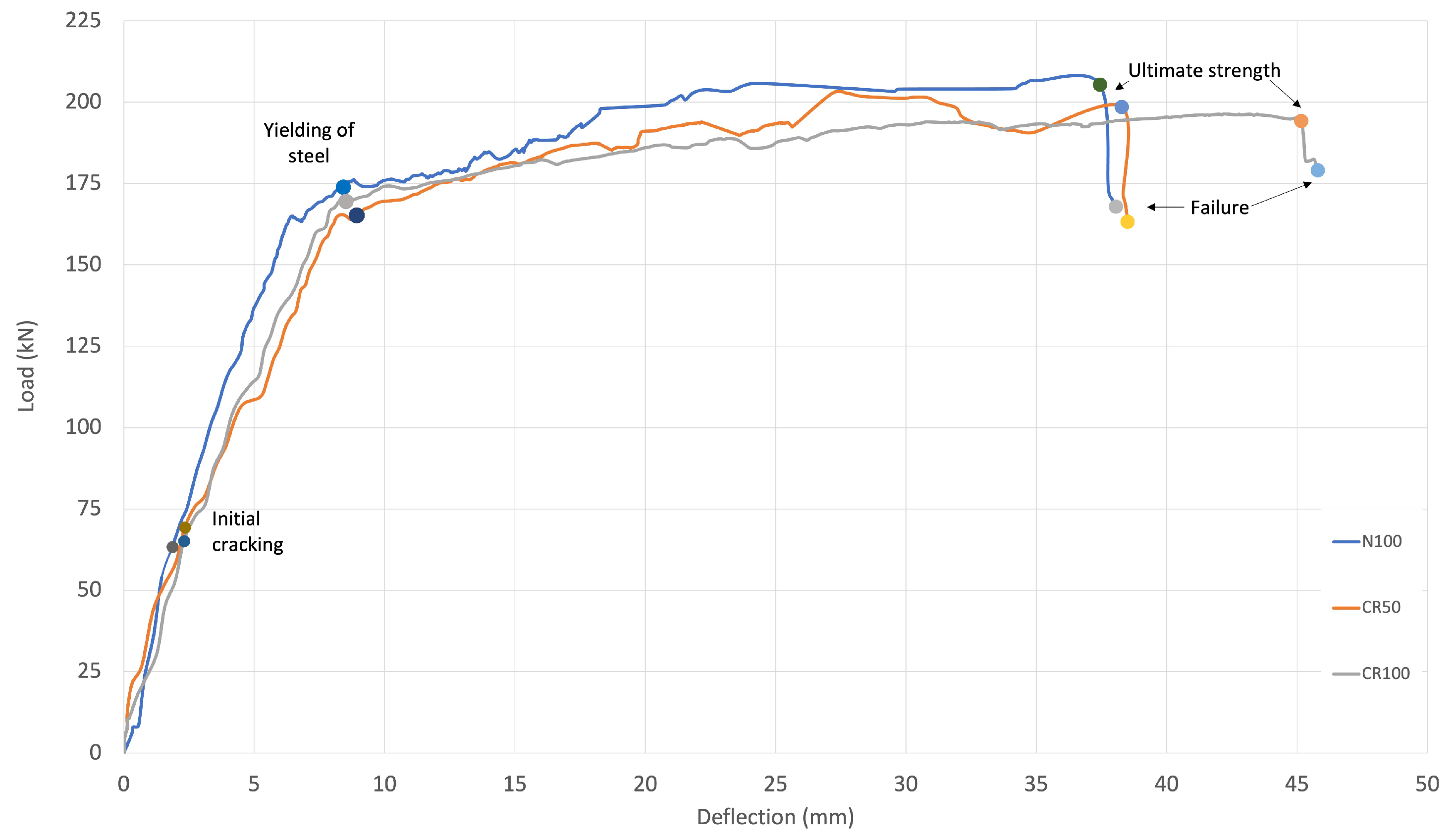
3.2.2. Load–Strain
Figure 5 provides information on how the strains and load in the concrete vary in relation to the concrete mix for the different series tested at 28 days. The curves start with a steep slope until the appearance of flexural cracks in the central third of the beams, after which the slopes are reduced. Beyond the yield point of the reinforcement, the load–strain ratio becomes curved with a parabolic tendency. Notably, the beams with 50% replacement (CR50) and 100% replacement (CR100) do not generally present major differences in their loading pattern; however, the control beam (N100) has a steeper slope in the linear elastic range, which is associated with the higher modulus of elasticity of this series Arezoumandi et al. [
42]. In addition, the behavior of the control series remains slightly above the recycled aggregate beams at all times, implying the ability to withstand a higher load for the same strain [
43].
Table 4 shows the data for the points shown in
Figure 5.
3.3. Strength Prediction/Theoretical Results
Chile has no design standards for calculating the flexural and deflection strength of beams with RA. Therefore, it was necessary to investigate whether beams with RA could be designed while applying the current design codes. For this purpose, a comparative study was conducted between the experimental results of this study and the theoretical results obtained from Eurocode 2 [
44] and ACI 318-19 [
45].
Table 5 and
Table 6 show the equations used to determine the theoretical cracking moments, and ultimate moments, capacity and deflection of the beams, respectively, while
Table 7 shows the comparison with the experimental results. The end load (
) was recorded from the load–strain curve at the end failure stage for all beams (237.66 kN, 202.80 kN, and 193.95 kN for beams containing 0%, 50%, and 100% treated recycled aggregates, respectively), then the end moments were calculated using these loads. The critical load (
) was determined from the experimental critical moment calculated from characteristics obtained from previous tests. Details of the calculations are provided in
Appendix A.
3.4. Comparison of Results
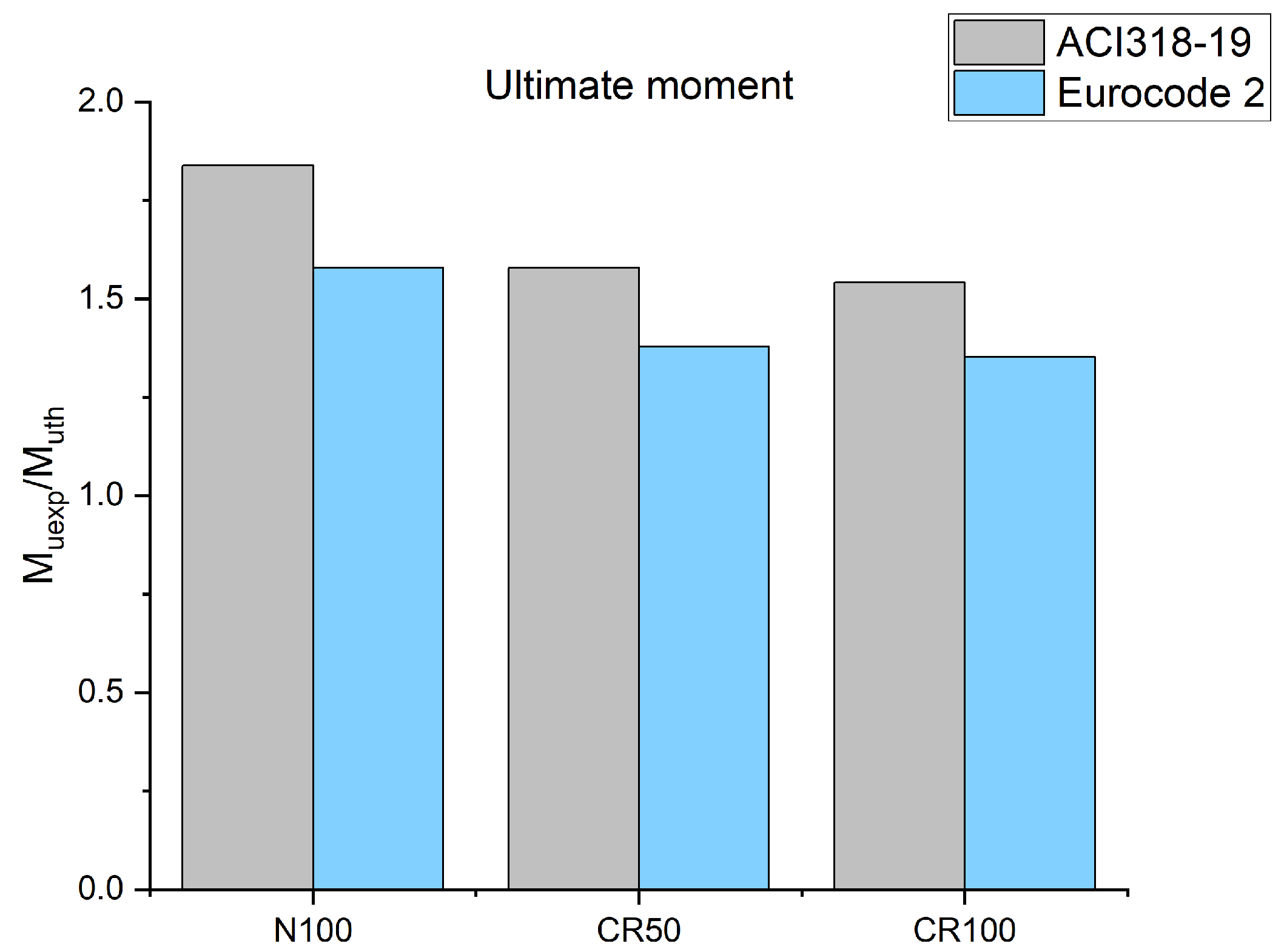
Figure 6 and
Figure 7 illustrate the relationship between the experimental and theoretical critical moments for all tested beams. A ratio above 1 represents a conservative estimation, while a ratio below 1 indicates a value greater than the experimentally predicted.
The ratios obtained for N100, CR50, and CR100 were 1.11, 1.04, and 0.99, respectively, according to the ACI 318 code, while the Eurocode 2 ratios were 1.29, 1.21, and 1.18, respectively. The ratios obtained for N100, CR50, and CR100 were 1.84, 1.58, and 1.54, respectively, according to the ACI 318 code, while the Eurocode 2 ratios were 1.58, 1.38, and 1.35, respectively.
For the critical moment, the prediction of the ACI 318 code was closer to the experimental results. In the case of high replacement percentages (100%), the experimental values were slightly below the theoretical values (0.99). Eurocode 2 yielded a more conservative prediction (1.18). The ACI 318 was more conservative than Eurocode 2 for the end moment prediction.
Figure 8 and
Figure 9 shows the relation between the experimental and theoretical critical and end strains of the studied beams. It can be observed that Eurocode 2 was more conservative in calculating the critical strain; at the same time, both codes overestimated the calculation of the end strains of all series [
46].
The ratio obtained for the N100, CR50, and CR100 beams was 1.18, 1.24, and 1.29, respectively, according to the ACI 318 code. However, according to Eurocode 2, they were 1.36, 1.44, and 1.52, which shows that the critical strain increases with increasing use of recycled aggregates, mainly due to the lower modulus of elasticity of these series.
The ratio obtained for N100, CR50, and CR100 was 0.81, 0.82, and 0.83, respectively, when using the ACI 318 code, while they were 0.69, 0.70, and 0.72 when using the Eurocode 2.
4. Conclusions
In this study, we conducted experimental and theoretical research on the flexural behavior of concrete beams with RA. Three concrete beams reinforced with different percentages of coated recycled aggregate (0%, 50%, and 100%) were tested. The structural characteristics predicted by the ACI 318-19 and Eurocode 2 codes were assessed. Based on the results, the following conclusions can be drawn:
When using the RA, the compressive strength decreased by 3.00% with 50% replacement and 12.73% with 100% replacement, whilehe flexural strength decreased by 8.44% with 50% replacement and 16.62% with 100% replacement. Although the treatment showed slight improvements in the physical properties, inferior results were obtained in terms of the mechanical properties, which could be linked to the different w/c ratios used to ensure the workability of the mixtures and comply with the requirements of industry.
The use of enhanced RA revealed a similar cracking pattern in terms of the number and spacing of cracks, indicating that the beams failed in a similar manner.
Increasing the percentage of RA reduced the load capacity; in addition, it reduced the end moment by 14.67% and the failure moment by 7.98% for the 50% replacement. For the 100% replacement, the end moment was reduced by 18.39% and the failure moment by 15.48%. For the 50% replacement, the end deflection in the central third was increased by 2.56% and the critical deflection was decreased by 25.71%. Conversely, when 100% replacement was used, the end and critical deflection both increased, by 1.92% and 4.76%, respectively.
The theoretical results provided by the equations in the Eurocode 2 and ACI 318-19 codes were compared with the experimental results. The most conservative critical moment standard was Eurocode 2; however, all the experimental critical moments were higher than the theoretical ones, with the exception of the critical moment calculated using ACI 318-19 for the 100% replacement, which resulted in a ratio of approximately 1.
The beams with RA had higher critical and end load capacities than the results predicted by the Eurocode 2 and ACI 318-19 codes, indicating that the moment capacity can be estimated using these design.
It should be noted that our study was limited to the situation described above, i.e., the use of low-quality recycled aggregates. It is recommended that further studies be carried out in order to validate the results obtained in the present study.