Design of Four-Plate Parallel Dynamic Capacitive Wireless Power Transfer Coupler for Mobile Robot Wireless-Charging Applications
Abstract
1. Introduction
- This study presents high-accuracy validation results through a theoretical design based on the practical equivalent circuit (PEC) model of a four-plate parallel DCPT coupler, compared with electromagnetic simulation results.
- It provides an analysis of the correlation between S21 and mutual capacitance in the four-plate parallel DCPT coupler.
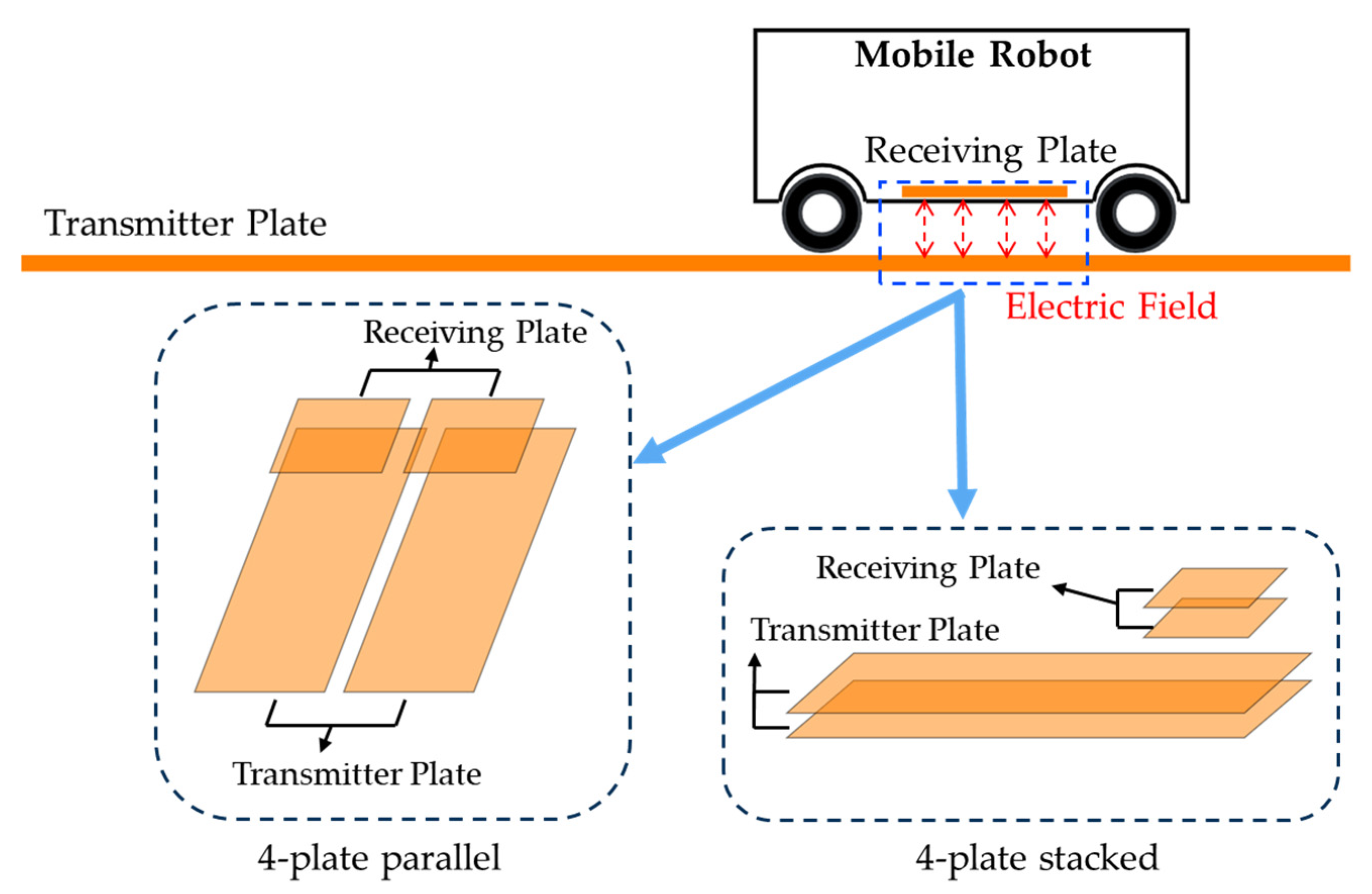
2. Model of Four-Plate Parallel DCPT Coupler
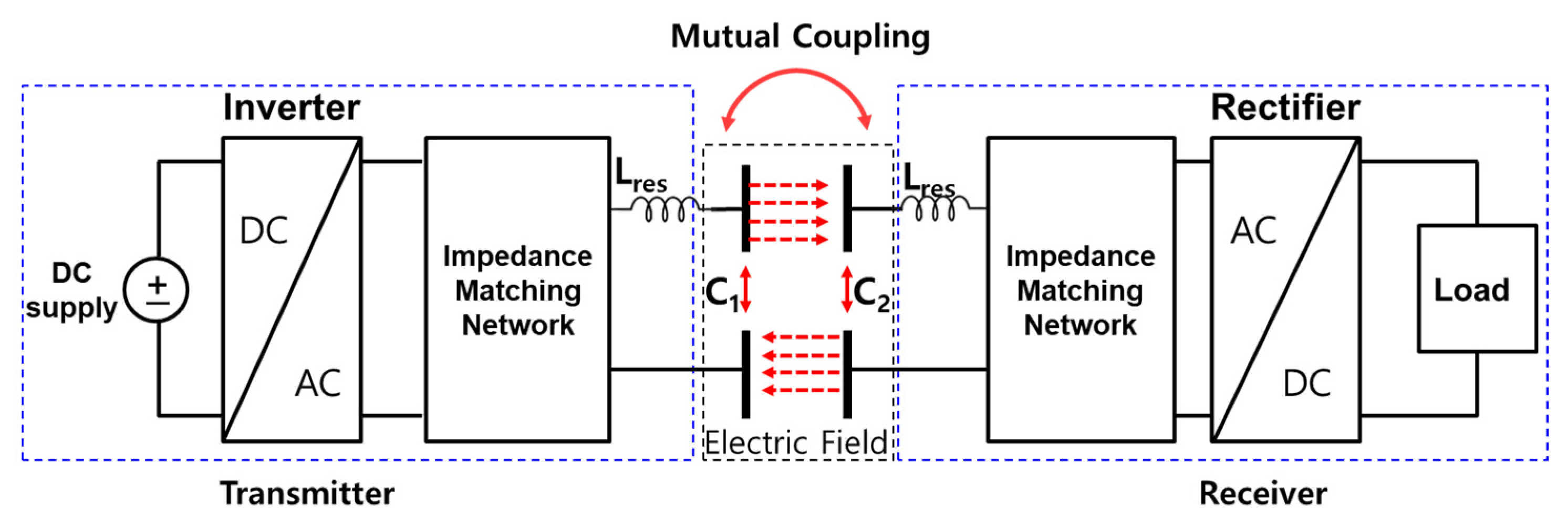
2.1. Theoretical Design Method of Equivalent Circuit
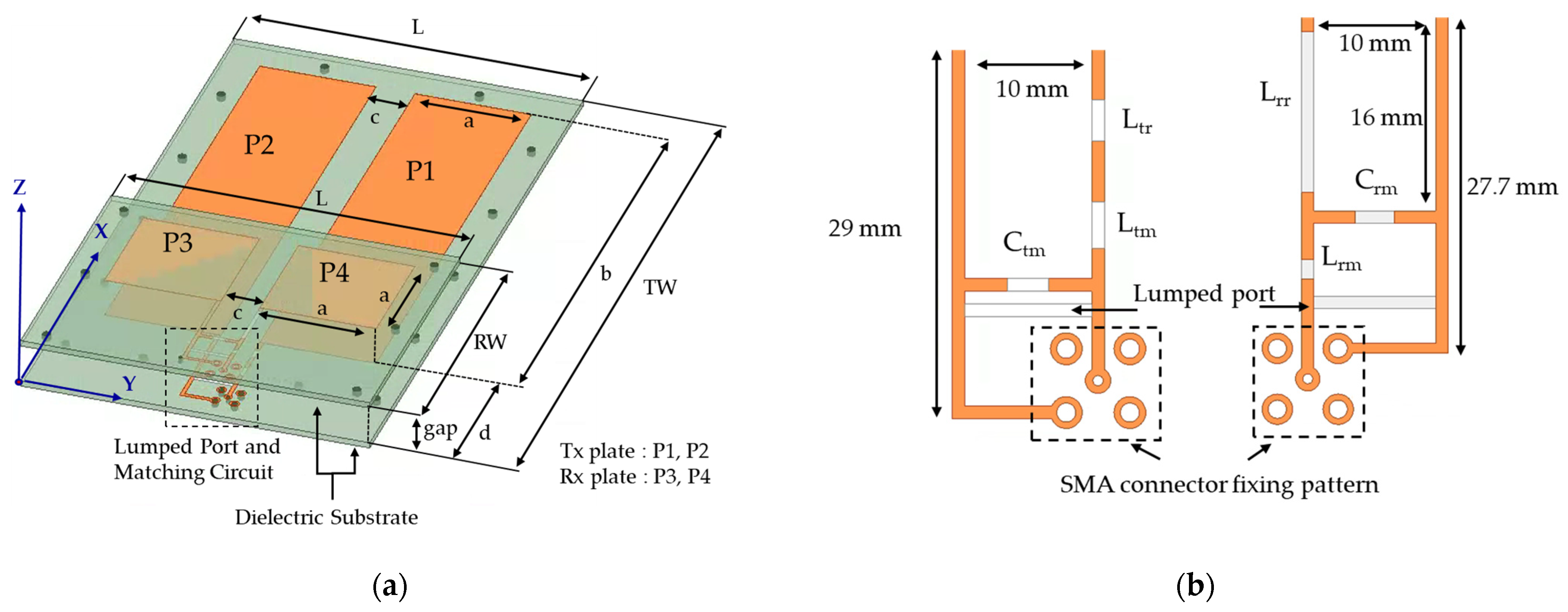
2.2. Simulation Model of Four-Plate Parallel DCPT
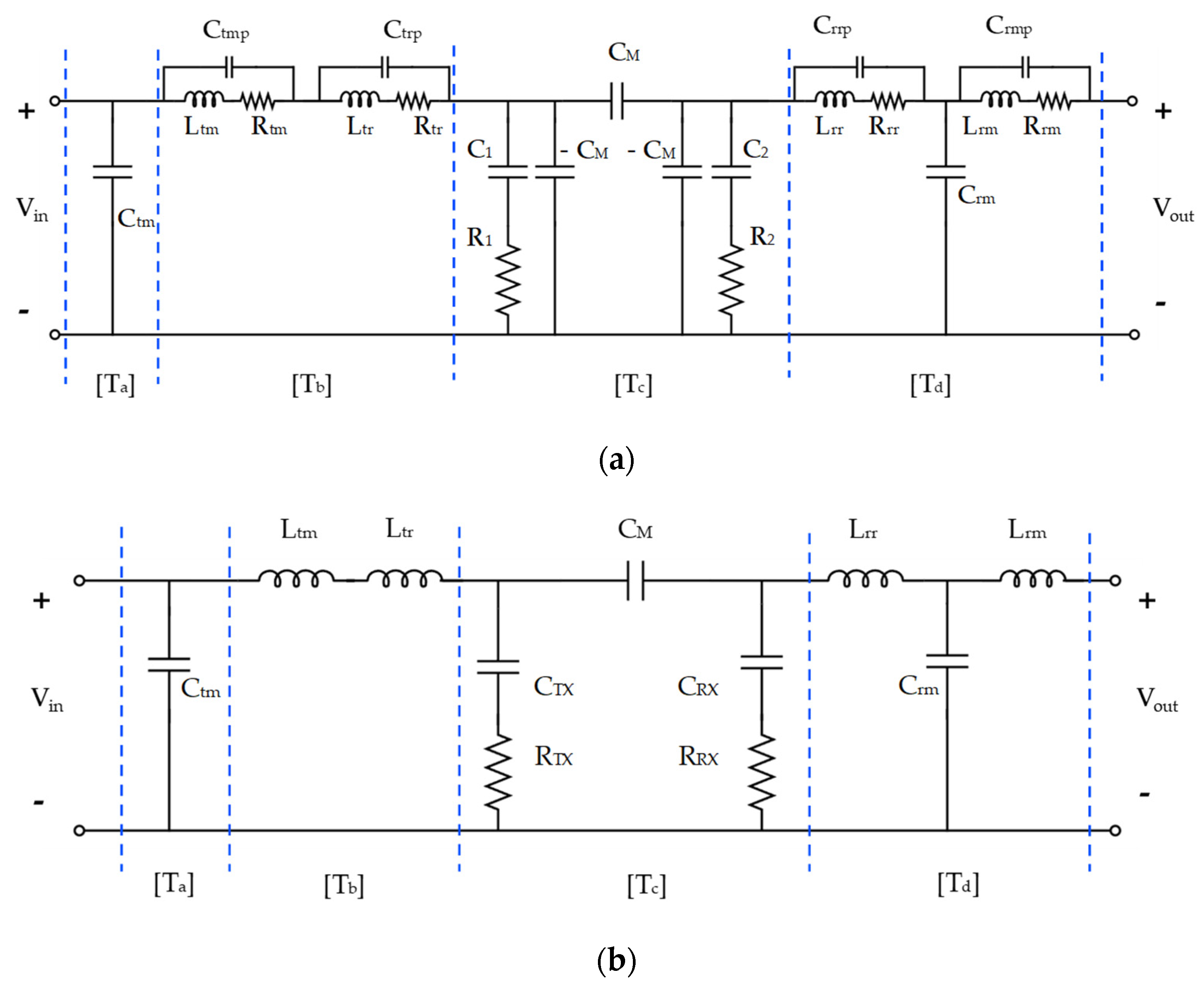
2.3. Equivalent Circuit Model of Four-Plate Parallel DCPT
| Matrix | Parameter |
|---|---|
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, W.; Chau, K.T.; Tian, X.; Wang, H.; Hua, Z. Smart Wireless Power Transfer—Opportunities and Challenges. Renew. Sustain. Energy Rev. 2023, 180, 113298. [Google Scholar] [CrossRef]
- Azad, A.; Tavakoli, R.; Pratik, U.; Varghese, B.; Coopmans, C.; Pantic, Z. A Smart Autonomous WPT System for Electric Wheelchair Applications With Free-Positioning Charging Feature. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 3516–3532. [Google Scholar] [CrossRef]
- Meile, L.; Ulrich, A.; Magno, M. Wireless Power Transmission Powering Miniaturized Low Power IoT Devices: A Revie. In Proceedings of the 2019 IEEE 8th International Workshop on Advances in Sensors and Interfaces (IWASI), Otranto, Italy, 13–14 June 2019; pp. 312–317. [Google Scholar] [CrossRef]
- Colmiais, I.; Dinis, H.; Mendes, P.M. Long-Range Wireless Power Transfer for Moving Wireless IoT Devices. Electronics 2024, 13, 2550. [Google Scholar] [CrossRef]
- Choi, K.W.; Aziz, A.A.; Setiawan, D.; Tran, N.M.; Ginting, L.; Kim, D.I. Distributed Wireless Power Transfer System for Internet of Things Devices. IEEE Internet Things J. 2018, 5, 2657–2671. [Google Scholar] [CrossRef]
- Deng, Z.; Hu, H.; Su, Y.; Chen, F.; Xiao, J.; Tang, C.; Lin, T. Design of a 60-kW EV Dynamic Wireless Power Transfer System With Dual Transmitters and Dual Receivers. IEEE J. Emerg. Sel. Top. Power Electron. 2024, 12, 316–327. [Google Scholar] [CrossRef]
- Huh, J.; Lee, S.W.; Lee, W.Y.; Cho, G.H.; Rim, C.T. Narrow-Width Inductive Power Transfer System for Online Electrical Vehicles. IEEE Trans. Power Electron. 2011, 26, 3666–3679. [Google Scholar] [CrossRef]
- Fujita, T.; Yasuda, T.; Akagi, H. A Dynamic Wireless Power Transfer System Applicable to a Stationary System. IEEE Trans. Ind. Applicat. 2017, 53, 3748–3757. [Google Scholar] [CrossRef]
- Villa, J.; Sanz, J.; Acerete, R.; Perie, M. Design Considerations for WPT Dynamic Charging Applications. In Proceedings of the 2019 AEIT International Conference of Electrical and Electronic Technologies for Automotive (AEIT AUTOMOTIVE), Turin, Italy, 2–4 July 2019; IEEE: Torino, Italy, 2019; pp. 1–6. [Google Scholar] [CrossRef]
- Zhao, J.; Cai, T.; Duan, S.; Feng, H.; Chen, C.; Zhang, X. A General Design Method of Primary Compensation Network for Dynamic WPT System Maintaining Stable Transmission Power. IEEE Trans. Power Electron. 2016, 31, 8343–8358. [Google Scholar] [CrossRef]
- Lin, C.-H.; Amir, M.; Tariq, M.; Shahvez, M.; Alamri, B.; Alahmadi, A.; Siddiqui, M.; Beig, A. Comprehensive Analysis of IPT v/s CPT for Wireless EV Charging and Effect of Capacitor Plate Shape and Foreign Particle on CPT. Processes 2021, 9, 1619. [Google Scholar] [CrossRef]
- Wang, J.; Hu, M.; Cai, C.; Lin, Z.; Li, L.; Fang, Z. Optimization Design of Wireless Charging System for Autonomous Robots Based on Magnetic Resonance Coupling. AIP Adv. 2018, 8, 055004. [Google Scholar] [CrossRef]
- Liu, H.; Huang, X.; Tan, L.; Guo, J.; Wang, W.; Yan, C.; Xu, C. Dynamic Wireless Charging for Inspection Robots Based on Decentralized Energy Pickup Structure. IEEE Trans. Ind. Inf. 2018, 14, 1786–1797. [Google Scholar] [CrossRef]
- Jo, H.; Seo, S.; Kim, J.; Bien, F. A Coreless Track-Type Seamless Wireless Charging System Using Co-Planar Wires Enabling Quasi-Free Planar Movements for Mobile Logistics Robots. Appl. Energy 2024, 375, 123943. [Google Scholar] [CrossRef]
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.D.; Fisher, P.; Soljačić, M. Wireless Power Transfer via Strongly Coupled Magnetic Resonances. Science 2007, 317, 83–86. [Google Scholar] [CrossRef] [PubMed]
- Shah, I.A.; Cho, Y.; Yoo, H. Safety Evaluation of Medical Implants in the Human Body for a Wireless Power Transfer System in an Electric Vehicle. IEEE Trans. Electromagn. Compat. 2021, 63, 681–691. [Google Scholar] [CrossRef]
- Christ, A.; Douglas, M.; Nadakuduti, J.; Kuster, N. Assessing Human Exposure to Electromagnetic Fields From Wireless Power Transmission Systems. Proc. IEEE 2013, 101, 1482–1493. [Google Scholar] [CrossRef]
- Tarusawa, Y.; Ohshita, K.; Suzuki, Y.; Nojima, T.; Toyoshima, T. Experimental Estimation of EMI From Cellular Base-Station Antennas on Implantable Cardiac Pacemakers. IEEE Trans. Electromagn. Compat. 2005, 47, 938–950. [Google Scholar] [CrossRef]
- Hikage, T.; Kawamura, Y.; Nojima, T.; Cabot, E. Numerical Assessment Methodology for Active Implantable Medical Device EMI Due to Magnetic Resonance Wireless Power Transmission Antenna. In Proceedings of the International Symposium on Electromagnetic Compatibility-EMC EUROPE, Rome, Italy, 17–21 September 2012; IEEE: Rome, Italy, 2012; pp. 1–6. [Google Scholar] [CrossRef]
- Hikage, T.; Kawamura, Y.; Nojima, T.; Koike, B.; Fujimoto, H.; Toyoshima, T. Active Implantable Medical Device EMI Assessments for Electromagnetic Emitters Operating in Various RF Bands. In Proceedings of the 2011 IEEE MTT-S International Microwave Workshop Series on Innovative Wireless Power Transmission: Technologies, Systems, and Applications, Kyoto, Japan, 12–13 May 2011; IEEE: Uji, Japan, 2011; pp. 109–112. [Google Scholar] [CrossRef]
- Cruciani, S.; Campi, T.; Maradei, F.; Feliziani, M. Wireless Charging in Electric Vehicles: EMI/EMC Risk Mitigation in Pacemakers by Active Coils. In Proceedings of the 2019 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), London, UK, 18–21 June 2019; IEEE: London, UK, 2019; pp. 173–176. [Google Scholar] [CrossRef]
- International Commission on Non-Ionizing Radiation Protection. Guidelines for Limiting Exposure to Electromagnetic Fields (100 kHz to 300 GHz). Health Phys. 2020, 118, 483–524. [Google Scholar] [CrossRef]
- Bang, K.-W.; Park, S.-W.; Bae, H.-G.; Lee, B.-Y.; Oh, H.-M.; Park, C.-U.; Baek, S.-H. Evaluation of Electromagnetic Exposure in Wireless Power Transfer Systems for Electric Vehicles. J. Electromagn. Eng. Sci. 2024, 24, 34–41. [Google Scholar] [CrossRef]
- Liu, C.; Hu, A.P. Steady State Analysis of a Capacitively Coupled Contactless Power Transfer System. In Proceedings of the 2009 IEEE Energy Conversion Congress and Exposition, San Jose, CA, USA, 20–24 September 2009; IEEE: San Jose, CA, USA, 2009; pp. 3233–3238. [Google Scholar] [CrossRef]
- Wang, C.; Zhu, C.; Wei, G.; Feng, J.; Jiang, J.; Lu, R. Design of Compact Three-Phase Receiver for Meander-Type Dynamic Wireless Power Transfer System. IEEE Trans. Power Electron. 2020, 35, 6854–6866. [Google Scholar] [CrossRef]
- Song, B.; Cui, S.; Li, Y.; Zhu, C. A Narrow-Rail Three-Phase Magnetic Coupler With Uniform Output Power for EV Dynamic Wireless Charging. IEEE Trans. Ind. Electron. 2021, 68, 6456–6469. [Google Scholar] [CrossRef]
- Zhou, W.; Li, M.; Zhang, Q.; Li, Z.; Xie, S.; Fan, Y. Potential and Challenges of Capacitive Power Transfer Systems for Wireless EV Charging: A Review of Key Technologies. Green Energy Intell. Transp. 2024, 3, 100174. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, S.; Li, X.; Yan, Z.; Tang, Y. A Compact Dynamic Wireless Power Transfer System via Capacitive Coupling Achieving Stable Output. In Proceedings of the 2020 IEEE 9th International Power Electronics and Motion Control Conference (IPEMC2020-ECCE Asia), Nanjing, China, 29 November–2 December 2020; IEEE: Nanjing, China, 2020; pp. 3269–3275. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, H.; Lu, F. Review, Analysis, and Design of Four Basic CPT Topologies and the Application of High-Order Compensation Networks. IEEE Trans. Power Electron. 2022, 37, 6181–6193. [Google Scholar] [CrossRef]
- Bang, K.; Bae, H.; Park, S. Resonant-Based Wireless Power Transfer System Using Electric Coupling for Transparent Wearable Devices and Null Power Points. Sensors 2023, 23, 1535. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Lu, F.; Hofmann, H.; Liu, W.; Mi, C. A 4-Plate Compact Capacitive Coupler Design and LCL-Compensated Topology for Capacitive Power Transfer in Electric Vehicle Charging Applications. IEEE Trans. Power Electron. 2016, 31, 8541–8551. [Google Scholar] [CrossRef]
- Mahdi, H.; Hattori, R.; Hoff, B.; Uezu, A.; Akiyoshi, K. Design Considerations of Capacitive Power Transfer Systems. IEEE Access 2023, 11, 57806–57818. [Google Scholar] [CrossRef]
- Erel, M.Z.; Bayindir, K.C.; Aydemir, M.T. A New Capacitive Coupler Design for Wireless Capacitive Power Transfer Applications. Eng. Sci. Technol. Int. J. 2023, 40, 101364. [Google Scholar] [CrossRef]
- Li, S.; Liu, Z.; Zhao, H.; Zhu, L.; Shuai, C.; Chen, Z. Wireless Power Transfer by Electric Field Resonance and Its Application in Dynamic Charging. IEEE Trans. Ind. Electron. 2016, 63, 6602–6612. [Google Scholar] [CrossRef]
- Lu, F.; Zhang, H.; Hofmann, H.; Mei, Y.; Mi, C. A Dynamic Capacitive Power Transfer System with Reduced Power Pulsation. In Proceedings of the 2016 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), Knoxville, TN, USA, 4–6 October 2016; IEEE: Knoxville, TN, USA, 2016; pp. 60–64. [Google Scholar] [CrossRef]
- Zhang, J.; Yao, S.; Pan, L.; Liu, Y.; Zhu, C. A Review of Capacitive Power Transfer Technology for Electric Vehicle Applications. Electronics 2023, 12, 3534. [Google Scholar] [CrossRef]
- Jeong, S.Y.; Kwak, H.G.; Jang, G.C.; Rim, C.T. Living Object Detection System Based on Comb Pattern Capacitive Sensor for Wireless EV Chargers. In Proceedings of the 2016 IEEE 2nd Annual Southern Power Electronics Conference (SPEC), Auckland, New Zealand, 5–8 December 2016; IEEE: Auckland, New Zealand, 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Lu, J.; Zhu, G.; Mi, C.C. Foreign Object Detection in Wireless Power Transfer Systems. IEEE Trans. Ind. Appl. 2022, 58, 1340–1354. [Google Scholar] [CrossRef]
- Lee, T.-S.; Huang, S.-J.; Tai, C.-C.; Chen, R.-Y.; Jiang, B.-R. Design of Wireless Power Transfer for Dynamic Power Transmission with Position-Detection Mechanism. In Proceedings of the 2015 IEEE International Conference on Industrial Technology (ICIT), Seville, Spain, 17–19 March 2015; IEEE: Seville, Spain, 2015; pp. 976–981. [Google Scholar] [CrossRef]
- Herpers, C.; Rouse, C.D. Matrix Persymmetry Analysis for Misalignment and Foreign Object Detection in Resonant Capacitive Power Transfer. IEEE Access 2024, 12, 65078–65087. [Google Scholar] [CrossRef]
- Pahlavan, S.; Shooshtari, M.; Jafarabadi Ashtiani, S. Star-Shaped Coils in the Transmitter Array for Receiver Rotation Tolerance in Free-Moving Wireless Power Transfer Applications. Energies 2022, 15, 8643. [Google Scholar] [CrossRef]
- Pahlavan, S.; Jafarabadi-Ashtiani, S.; Mirbozorgi, S.A. Maze-Based Scalable Wireless Power Transmission Experimental Arena for Freely Moving Small Animals Applications. IEEE Trans. Biomed. Circuits Syst. 2024, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Hyun, S.; Bae, H.; Park, S. Characteristics Analysis of Resonance-Based Wireless Power Transfer Using Magnetic Coupling and Electric Coupling. Eng. Sci. Technol. Int. J. 2023, 42, 101419. [Google Scholar] [CrossRef]
- Luo, B.; Mai, R.; Guo, L.; Wu, D.; He, Z. LC–CLC Compensation Topology for Capacitive Power Transfer System to Improve Misalignment Performance. IET Power Electron. 2019, 12, 2626–2633. [Google Scholar] [CrossRef]


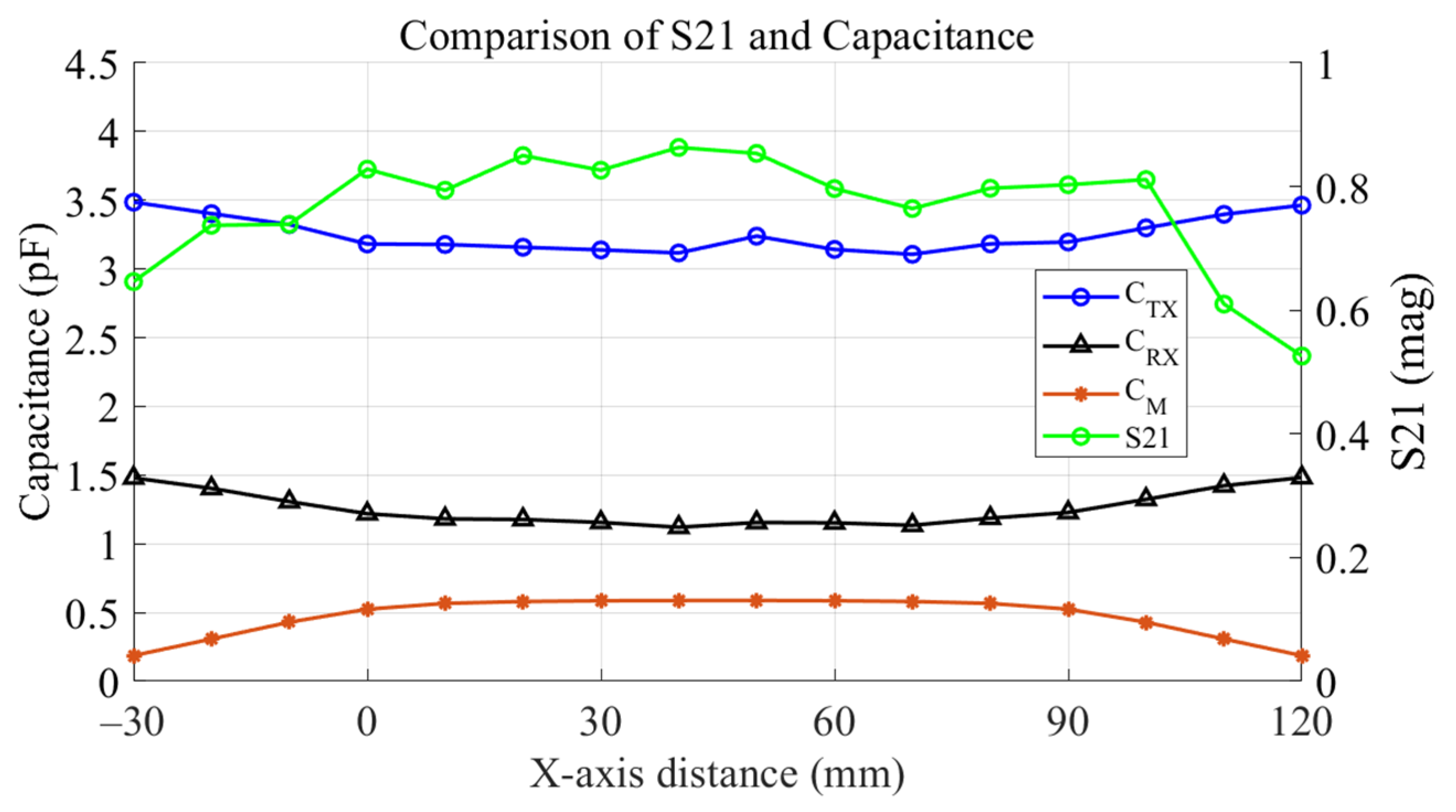
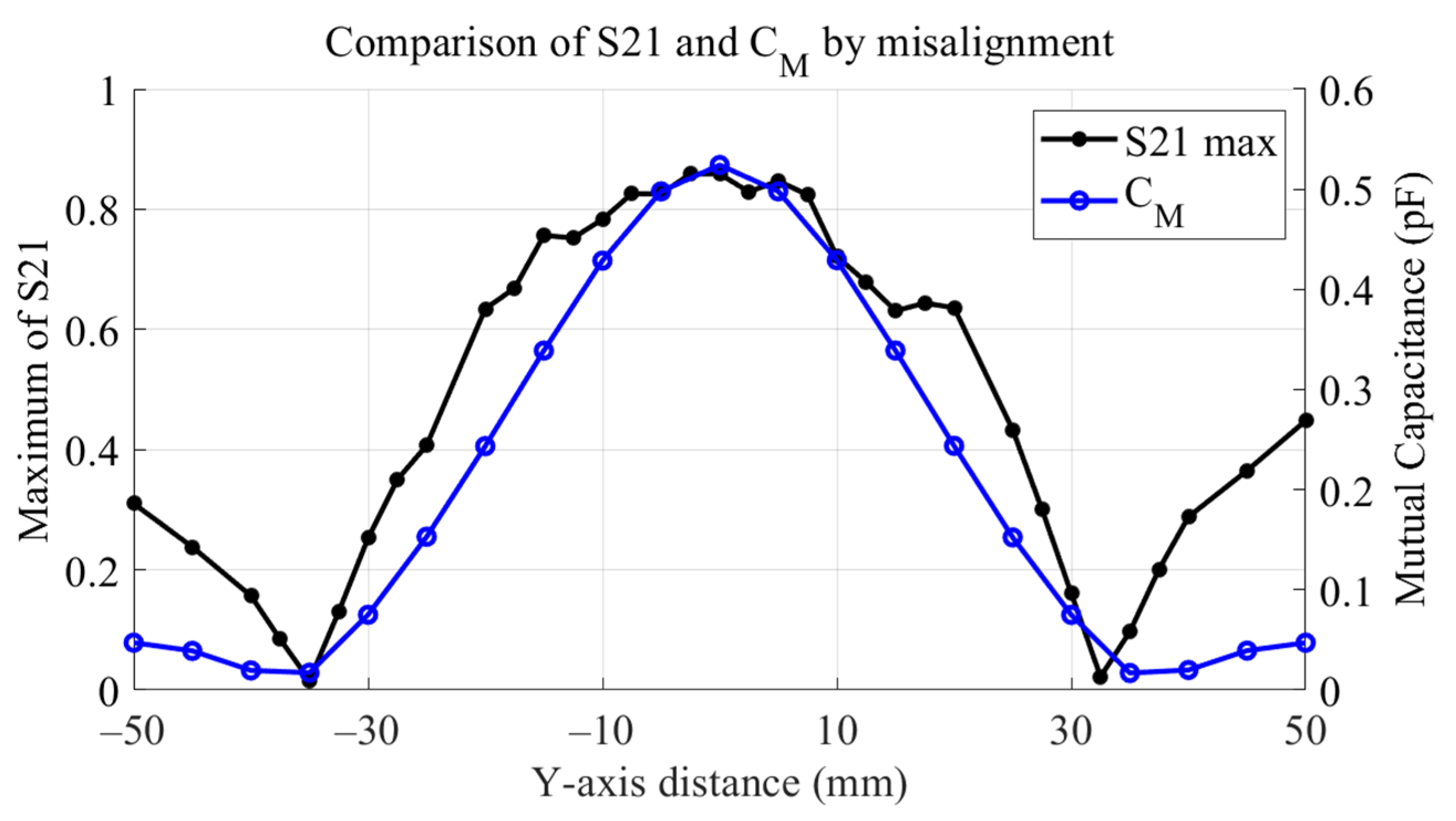
| Components | Notation | Value |
|---|---|---|
| Width of Tx and Rx substrate | L | 90 mm |
| Length of Tx substrate | TW | 165 mm |
| Length of Rx substrate | RW | 75 mm |
| Width of P1~P4 | a | 30 mm |
| Length of P1, P2 | b | 120 mm |
| Distance between metal plates of P1 and P2 | c | 10 mm |
| X-axis length from the metal plate to the origin | d | 35 mm |
| Distance between Tx and Rx | gap | 10 mm |
| Component | Notation | Value |
|---|---|---|
| Coupler self-capacitance of Tx | C1 | 3.504 pF |
| Coupler self-capacitance of Rx | C2 | 1.292 pF |
| Coupler intrinsic resistance | RTX, RRX | 1 Ω |
| Tx’s lumped L | Ltr | 56 μH |
| Rx’s lumped L | Lrr | 350 μH |
| Tx’s impedance-matching L | Ltm | 4.3 μH |
| Rx’s impedance-matching L | Lrm | 650 nH |
| Tx’s impedance-matching C | Ctm | 100 pF |
| Rx’s impedance-matching C | Crm | 750 pF |
| Mutual capacitance | CM | 0.43 pF |
| Point1 (−50 mm) | Point2 (−35 mm) | Point3 (0 mm) | Point4 (32.5 mm) | Point5 (50 mm) | |
|---|---|---|---|---|---|
| S21_max | 0.31 | 0.01 | 0.86 | 0.02 | 0.45 |
| CM (pF) | 0.04 | 0.08 | 0.52 | 0.07 | 0.04 |
| CTX (pF) | 3.79 | 3.89 | 3.5 | 3.9 | 3.86 |
| CRX (pF) | 1.7 | 1.74 | 1.38 | 1.75 | 1.72 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bae, H.; Park, S. Design of Four-Plate Parallel Dynamic Capacitive Wireless Power Transfer Coupler for Mobile Robot Wireless-Charging Applications. Appl. Sci. 2025, 15, 823. https://doi.org/10.3390/app15020823
Bae H, Park S. Design of Four-Plate Parallel Dynamic Capacitive Wireless Power Transfer Coupler for Mobile Robot Wireless-Charging Applications. Applied Sciences. 2025; 15(2):823. https://doi.org/10.3390/app15020823
Chicago/Turabian StyleBae, Hongguk, and Sangwook Park. 2025. "Design of Four-Plate Parallel Dynamic Capacitive Wireless Power Transfer Coupler for Mobile Robot Wireless-Charging Applications" Applied Sciences 15, no. 2: 823. https://doi.org/10.3390/app15020823
APA StyleBae, H., & Park, S. (2025). Design of Four-Plate Parallel Dynamic Capacitive Wireless Power Transfer Coupler for Mobile Robot Wireless-Charging Applications. Applied Sciences, 15(2), 823. https://doi.org/10.3390/app15020823










