Experimental Study of Influence of Plant Roots on Dynamic Characteristics of Clay
Abstract
1. Introduction
2. Experimental Materials with Tested Material
2.1. Experimental Materials
2.2. Tested Equipment
2.3. Experimental Procedure
3. Experimental Results Analysis
3.1. Influence of Plant Root Systems on Soil Shear Modulus
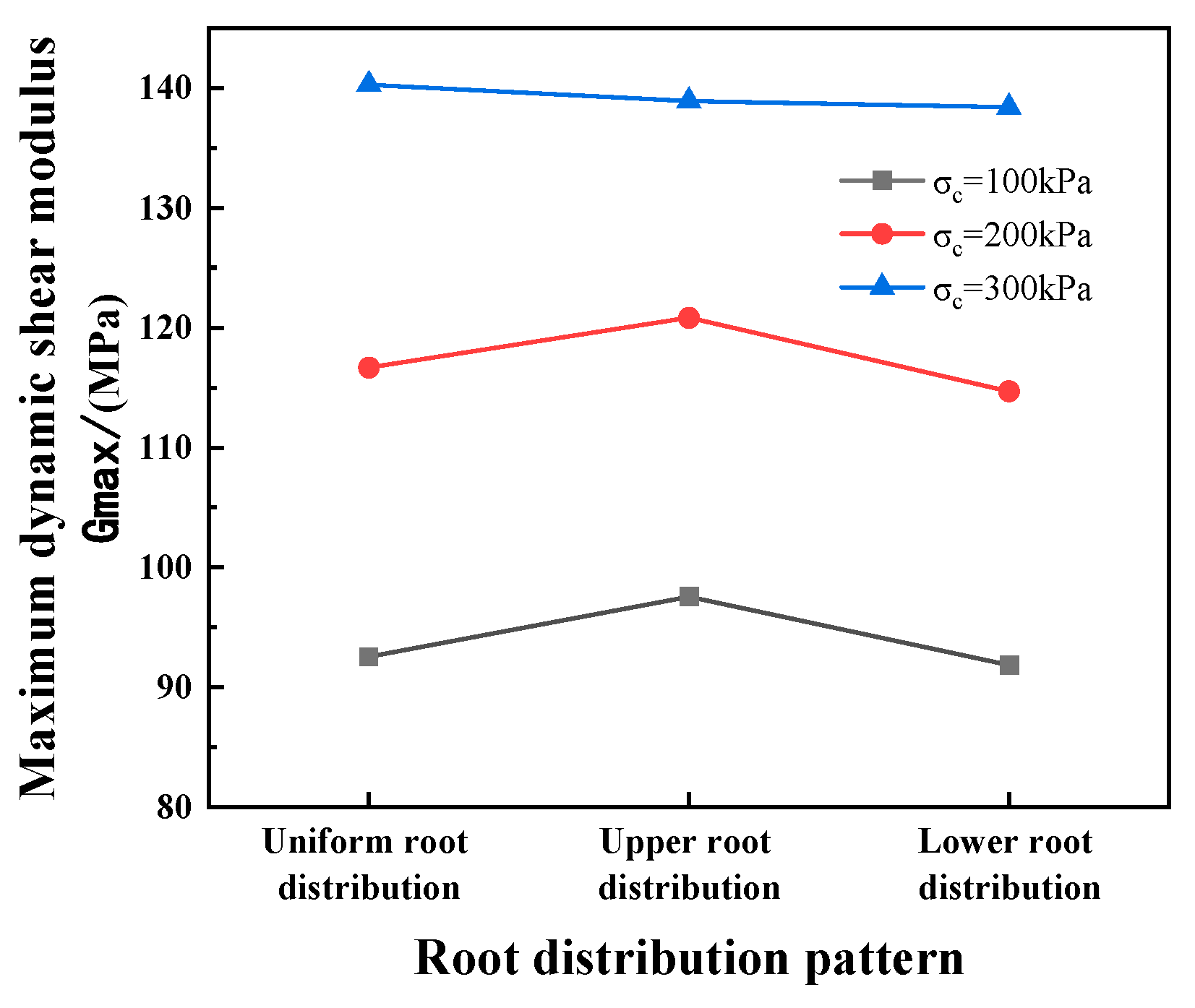
3.1.1. Different Root Distribution Patterns
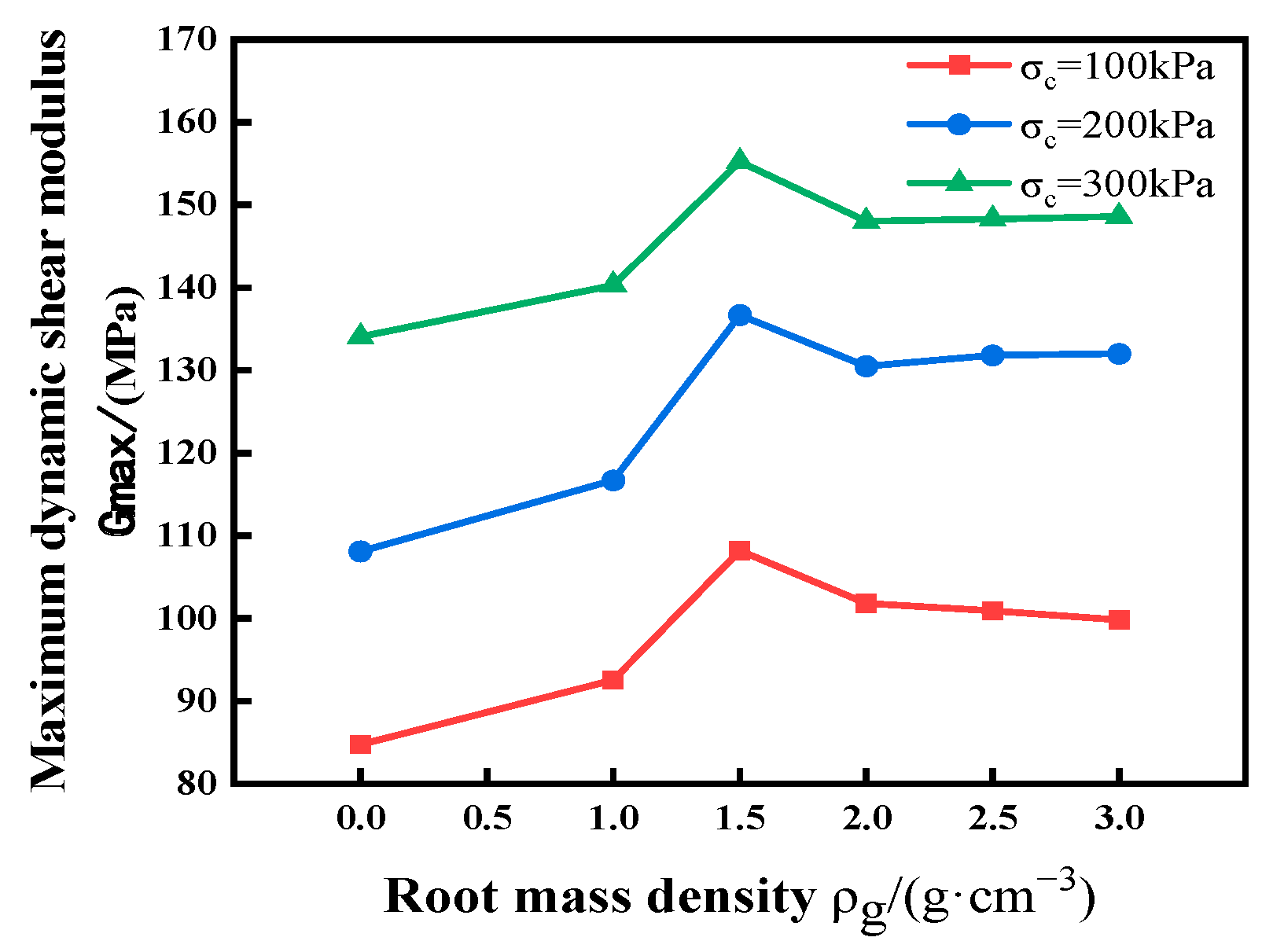
3.1.2. Different Root Mass Density
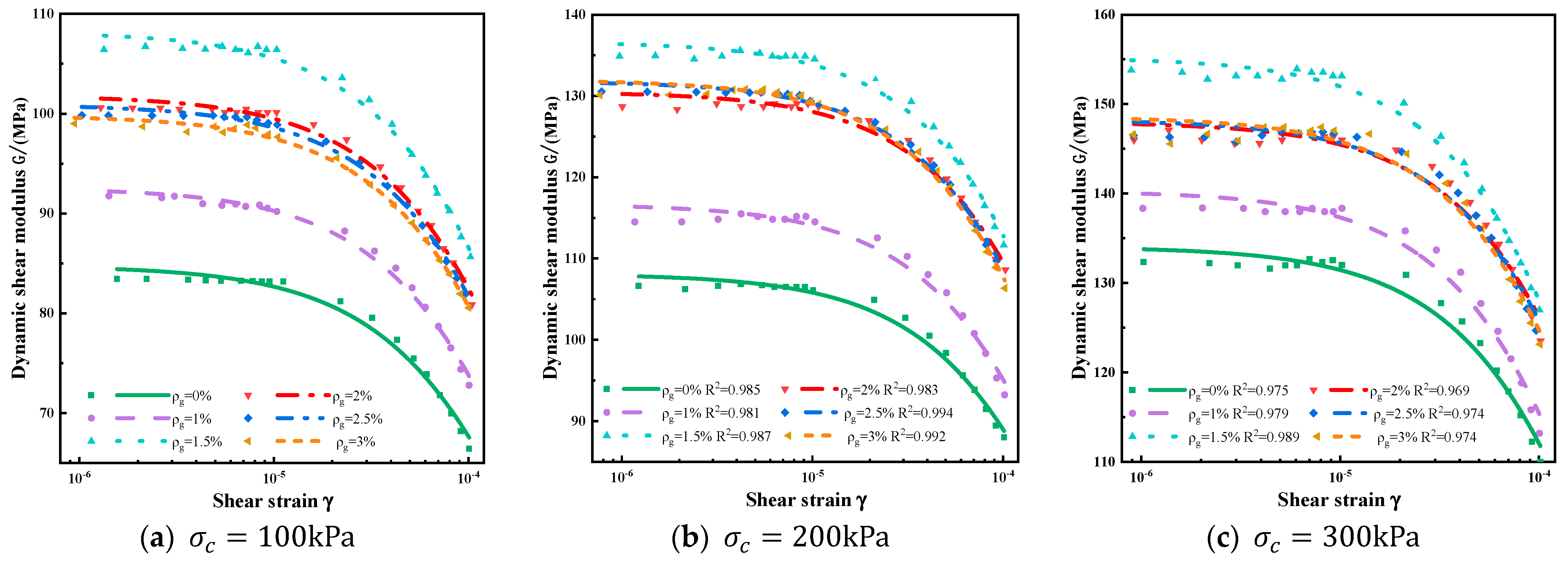
3.1.3. Shear Modulus Fitting Results of the Hardin–Drnevich Hyperbolic Model
3.2. Influence of Plant Root Systems on Soil Damping Ratio
3.2.1. Different Root Distribution Patterns
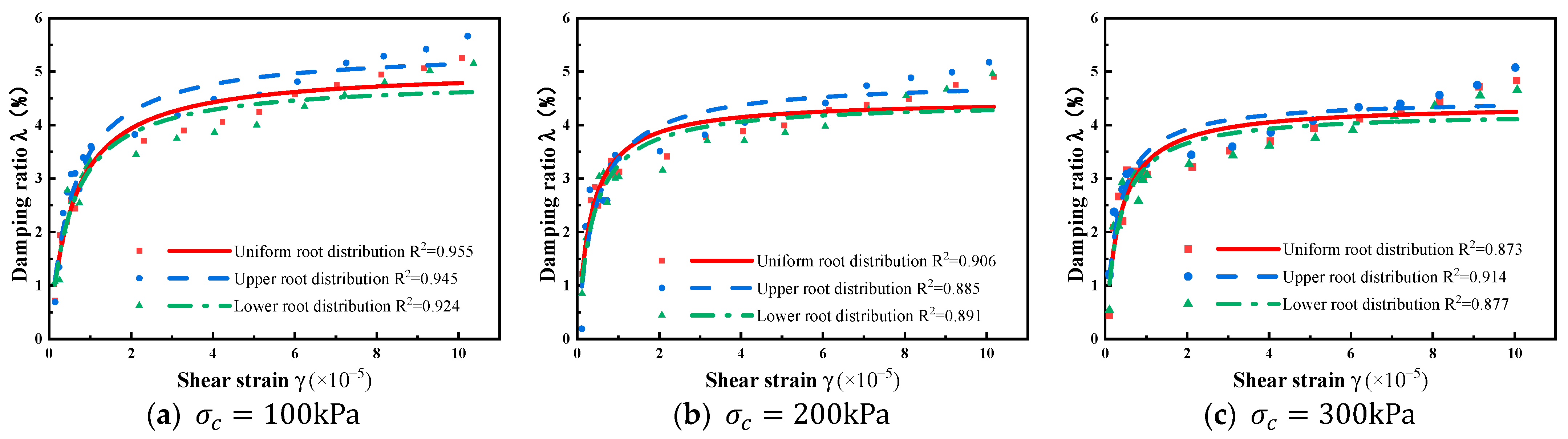
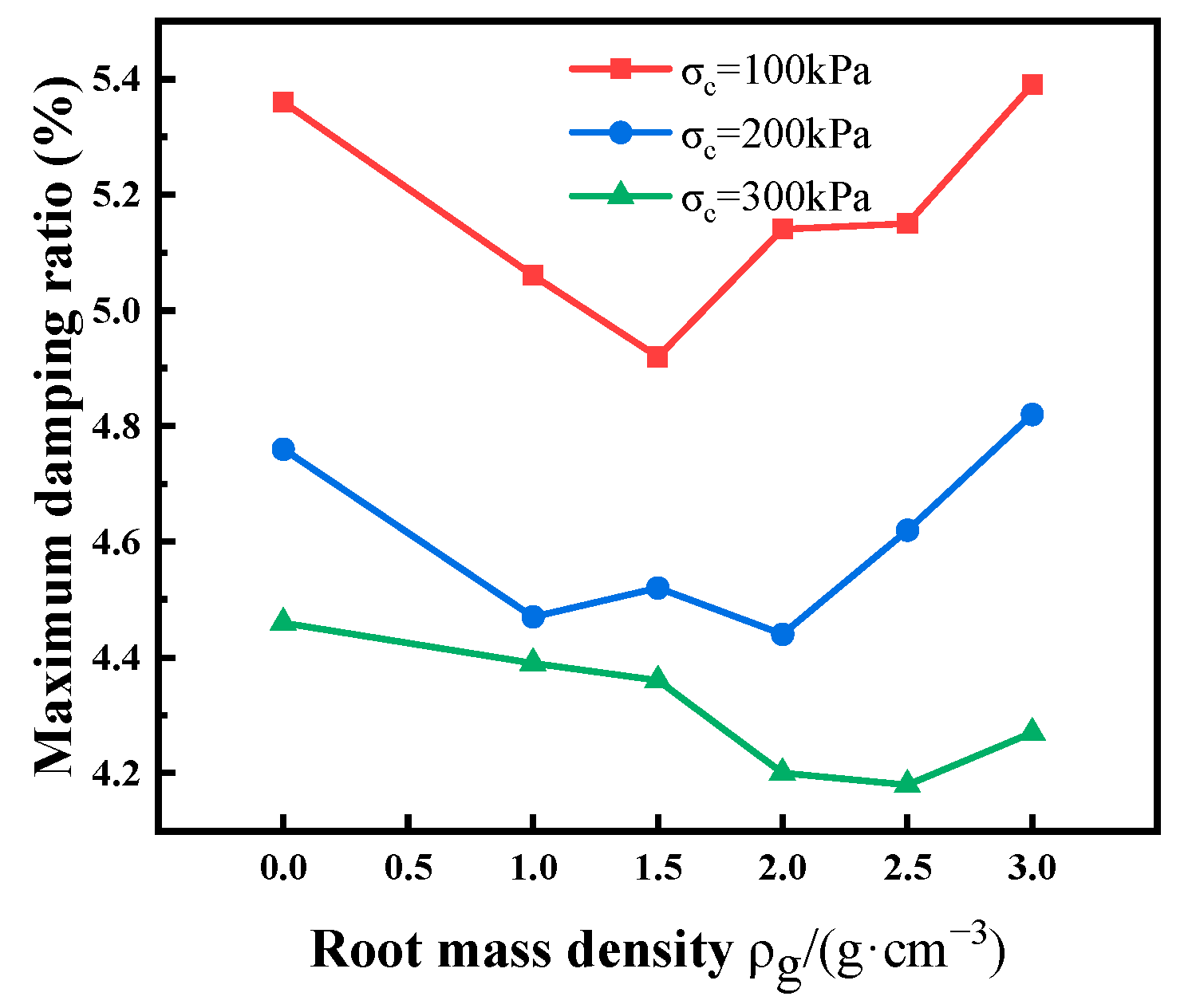
3.2.2. Different Root Mass Density
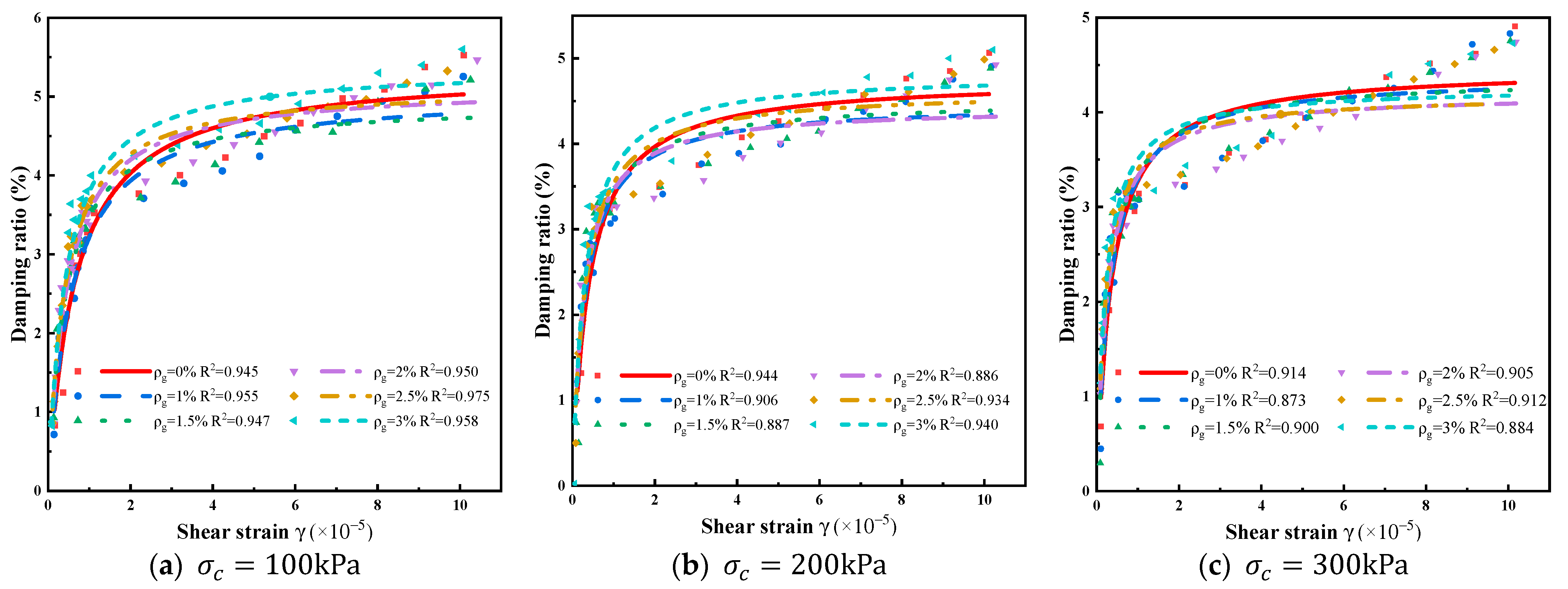
3.2.3. Damping Ratio Fitting Results of the Hardin–Drnevich Hyperbolic Model
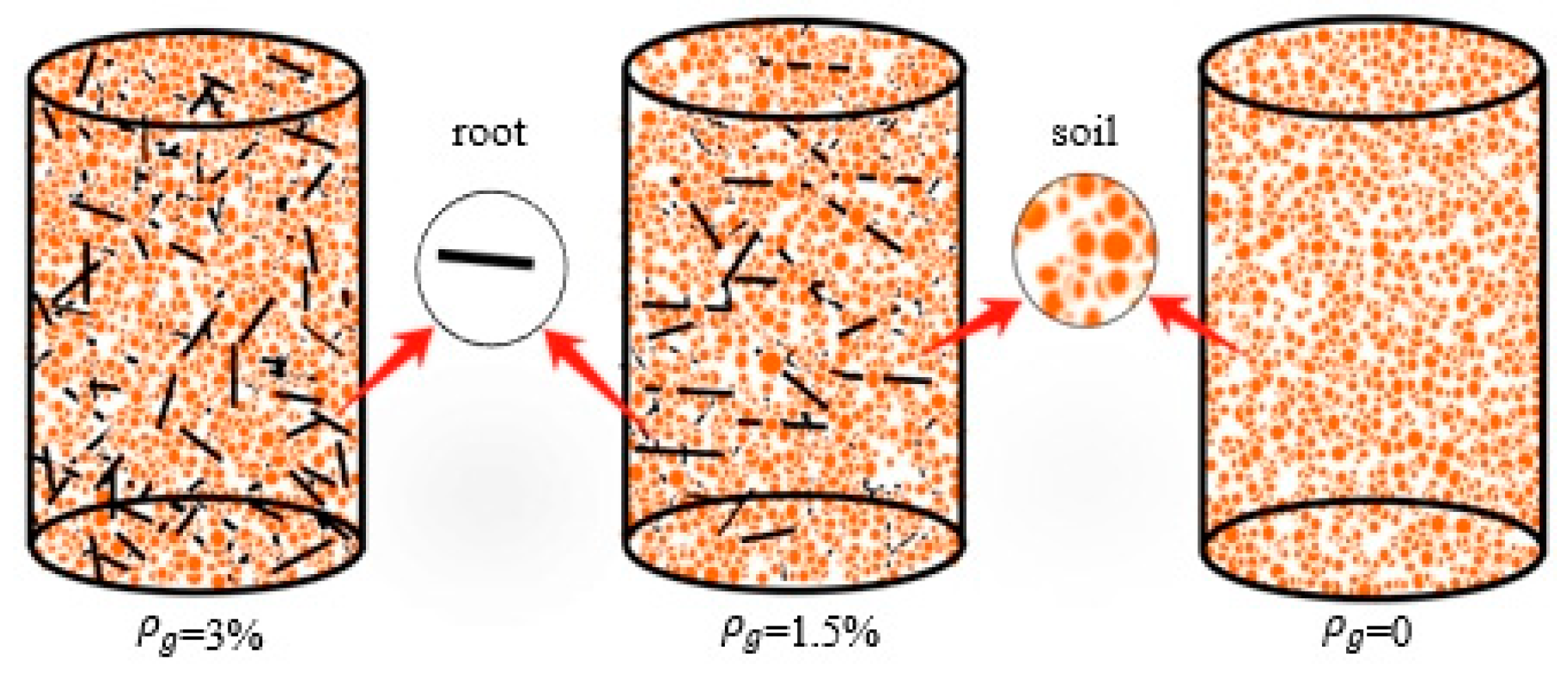
3.3. Reinforcement Mechanism of Roots
4. Conclusions
- The upper root distribution significantly increased the dynamic shear modulus of the soil under confining pressures of 100–200 kPa, demonstrating greater stiffness and strength, which enhanced dynamic soil properties and reduced dynamic strain amplitude.
- Plant roots improved the dynamic shear modulus of soil, with the effect varying according to root mass density. The dynamic shear modulus peaked at a root mass density of 1.5% , then stabilized. Increased confining pressure reduced the influence of high root mass density, with the relationship curve gradually converging. This indicates that optimizing root distribution and density can effectively enhance soil dynamic properties.
- The upper root distribution significantly increased the soil damping ratio, enhancing energy dissipation capacity due to increased upper stiffness and accelerated vibration response. In contrast, the lower root distribution exhibited a lower damping ratio due to confinement effects. This suggests that placing roots in the upper soil layer can improve dynamic soil stability, consistent with the root distribution characteristics on embankment surfaces.
- The damping ratio decreased initially and then increased with rising root mass density, with critical values observed at each confining pressure (1.5% for 100 kPa, 2% for 200 kPa, and 2.5% for 300 kPa). At a low density, roots enhanced soil compaction and reduced the damping ratio, while at a high density, they improved ductility and increased energy dissipation. Optimizing root mass density can help improve the dynamic properties of slope soils.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gong, C.; Ni, D.; Liu, Y.; Li, Y.; Huang, Q.; Tian, Y.; Zhang, H. Herbaceous Vegetation in Slope Stabilization: A Comparative Review of Mechanisms, Advantages, and Practical Applications. Sustainability 2024, 16, 7620. [Google Scholar] [CrossRef]
- Löbmann, M.T.; Geitner, C.; Wellstein, C.; Zerbe, S. The influence of herbaceous vegetation on slope stability—A review. Earth-Sci. Rev. 2020, 209, 103328. [Google Scholar] [CrossRef]
- Bordoloi, S.; Ng, C.W. The effects of vegetation traits and their stability functions in bio-engineered slopes: A perspective review. Eng. Geol. 2020, 275, 105742. [Google Scholar] [CrossRef]
- Zhu, Y.G.; Wang, C.; Liu, Z.Q. Effects of rainfall and vegetation cover on soil erosion railway bed slope. Bull. Soil Water Conserv. 2021, 41, 8–14. [Google Scholar]
- Yang, Z.G.; Xi, W.F.; Shi, Z.T.; Yang, Z.Q.; Xiao, B. Study on the relationship between landslide creep and vegetation cover in mountainous areas with complex vegetation. Sci. Surv. Mapp. 2023, 48, 157–165+175. [Google Scholar]
- Zhou, Y.; Ma, W. Plant roots in the active zone improving the performance of ecological reinforced soil retaining walls. Bull. Eng. Geol. Environ. 2023, 82, 414. [Google Scholar] [CrossRef]
- Seed, H.B. Soil moduli and damping factors for dynamic response analyses. J. Terramech. 1972, 8, 109. [Google Scholar] [CrossRef]
- Hardin, B.O.; Drnevich, V.P. Shear modulus and damping in soils: Design equations and curves. J. Soil Mech. Found. Div. 1972, 98, 667–692. [Google Scholar] [CrossRef]
- Zhong, X.G.; Zeng, X.; Rose, J.G. Shear modulus and damping ratio of rubber-modified asphalt mixes and unsaturated subgrade soils. J. Mater. Civ. Eng. 2002, 14, 496–502. [Google Scholar] [CrossRef]
- Chen, G.; Xie, J.; Zhang, K. The empirical evaluation of soil moduli and damping ratio for dynamic analysis. Earthq. Eng. Eng. Dyn. 1995, 15, 73–84. [Google Scholar]
- Wang, H.; He, M.; Zhu, J.; Guo, S.; Chen, Y.; Li, N. Experimental investigation of linear damping characteristics on granite and red sandstone under dynamic cyclic loading. Eur. J. Environ. Civ. Eng. 2022, 26, 5259–5278. [Google Scholar] [CrossRef]
- Yang, B.; He, M.; Zhang, Z.; Zhu, J.; Chen, Y. Experimental investigation and empirical model on damping properties of rock under multistage cyclic loading. Soil Dyn. Earthq. Eng. 2022, 163, 107557. [Google Scholar] [CrossRef]
- Wang, W.; Cao, G.; Li, Y.; Zhou, Y.; Lu, T.; Wang, Y.; Zheng, B. Experimental study of dynamic characteristics of tailings with different reconsolidation degrees after liquefaction. Front. Earth Sci. 2022, 10, 876401. [Google Scholar] [CrossRef]
- Zhang, Q.; Yin, G.; Wei, Z.; Fan, X.; Wang, W.; Nie, W. An experimental study of the mechanical features of layered structures in dam tailings from macroscopic and microscopic points of view. Eng. Geol. 2015, 195, 142–154. [Google Scholar] [CrossRef]
- Park, D.; Kishida, T. Shear modulus reduction and daming ratio curves for earth core materials of dams. Can. Geotech. J. 2019, 56, 14–22. [Google Scholar] [CrossRef]
- Zhao, Y.; Yang, Y.; Ling, X.; Gong, W.; Li, G.; Su, L. Dynamic behavior of natural sand soils and fiber reinforced soils in heavy-haul railway embankment under multistage cyclic loading. Transp. Geotech. 2021, 28, 100507. [Google Scholar] [CrossRef]
- Zhao, J.; Bai, X.; Xie, M.; Zheng, J. Experimental study on dynamic response characteristics of small-spaced reinforced soil under cvclic loading. J. Civ. Environ. Eng. 2023, 45, 125–133. [Google Scholar]
- Rollins, K.M.; Evans, M.D.; Diehl, N.B.; III, W.D. Shear modulus and daming relationships for gravels. J. Geotech. Geoenviron. Eng. 1998, 124, 396–405. [Google Scholar] [CrossRef]
- Tang, F.C.; Zhang, W.Y.; Tang, X.; Liu, C.K. Study on the dynamic characteristics of geogrid reinforced loess under cyclic loading. Water Resour. Hydropow. Eng. 2024, 55, 148–161. [Google Scholar]
- Vahedifard, F.; Abdollahi, M.; Leshchinsky, B.A.; Stark, T.D.; Sadegh, M.; AghaKouchak, A. Interdependencies between wildfire—Induced alterations in soil properties, near—Surface processes, and geohazards. Earth Space Sci. 2024, 11, e2023EA003498. [Google Scholar] [CrossRef]
- Sonnenberg, R.; Bransby, M.F.; Hallett, P.D.; Bengough, A.G.; Mickovski, S.B.; Davies, M.C.R. Centrifuge modelling of soil slopes reinforced with vegetation. Can. Geotech. J. 2010, 47, 1415–1430. [Google Scholar] [CrossRef]
- Chenari, R.J.; Pishkhani, S.S.; Fard, M.K.; Sosahab, J.S. Experimental and numerical investigation of dynamic properties of soil stabilized by Young Vetiver. Riv. Ital. Di Geotec. 2018, 52, 49–58. [Google Scholar]
- Yan, Z. Dynamic experimental study on remolded soil and plant-root reinforced soil under cyclic load. Port Waterw. Eng. 2021, 2021, 186–191. [Google Scholar]
- Liu, S.; Li, J.; Ji, X.; Fang, Y. Influence of root distribution patterns on soil dynamic characteristics. Sci Rep. 2022, 12, 13448. [Google Scholar] [CrossRef] [PubMed]
- GB/T 50123; Ministry of Water Resources of the People’s Republic of China. Standard for Geotechnical Testing Method. China Planning Press: Beijing, China, 2019; pp. 41–125.
- Peng, Q.F. Study on the Influence of Particle Shape and Average Particle Size Ratio on the Dynamic Characteristics of Rubber-Sand Mixtures. Master’s Thesis, Hunan University of Technology, Zhuzhou, China, 2021. [Google Scholar]
- Eshel, A.; Beeckman, T. (Eds.) Plant Roots: The Hidden Half; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Agnihotri, L.S.V. Estimating the genetic relationship in various warm season turfgrass genotypes using RAPD and ISSR markers. Natl. Acad. Sci. Lett. 2018, 41, 197–201. [Google Scholar] [CrossRef]
- Ahn, S.; Ryou, J.-E.; Ahn, K.; Lee, C.; Lee, J.-D.; Jung, J. Evaluation of dynamic properties of sodium-alginate-reinforced soil using a resonant-column test. Materials 2021, 14, 2743. [Google Scholar] [CrossRef]
- Lu, Z.C.; Yang, Z.G.; Liu, J.M.; Lv, J.H.; Zhang, Q.; Ling, X.Z. Low-Temperature dynamic triaxial testing of frozen expansive soil-rubber(ESR) mixtures. J. Eng. Geol. 2021, 29, 1312–1319. [Google Scholar]
- White, R.G.; Kirkegaard, J.A. The distribution and abundance of wheat roots in a dense, structured subsoil–implications for water uptake. Plant Cell Environ. 2010, 33, 133–148. [Google Scholar] [CrossRef] [PubMed]
- Foresta, V.; Capobianco, V.; Cascini, L. Influence of grass roots on shear strength of pyroclastic soils. Can. Geotech. J. 2020, 57, 1320–1334. [Google Scholar] [CrossRef]
- Fan, C.C.; Su, C.F. Role of roots in the shear strength of root-reinforced soils with high moisture content. Ecol. Eng. 2008, 33, 157–166. [Google Scholar] [CrossRef]
- Baets, D.S.; Poesen, J.; Reubens, B.; Wemans, K.; De Baerdemaeker, J.; Muys, B. Root tensile strength and root distribution of typical Mediterranean plant species and their contribution to soil shear strength. Plant Soil 2008, 305, 207–226. [Google Scholar] [CrossRef]
- Meijer, G.J.; Muir Wood, D.; Knappett, J.A.; Bengough, G.A.; Liang, T. Analysis of coupled axial and lateral deformation of roots in soil. Int. J. Numer. Anal. Methods Geomech. 2019, 43, 684–707. [Google Scholar] [CrossRef]
- Fan, C.C.; Su, C.F. Effect of soil moisture content on the deformation behaviour of root-reinforced soils subjected to shear. Plant Soil 2009, 324, 57–69. [Google Scholar] [CrossRef]
- Gao, W.; Hodgkinson, L.K.; Watts, C.W.; Ashton, R.W.; Shen, J.; Ren, T.; Dodd, I.C.; Binley, A.; Phillips, A.L.; Hedden, P. Deep roots and soil structure. Plant Cell Environ. 2016, 39, 1662–1668. [Google Scholar] [CrossRef]
- Ghestem, M.; Veylon, G.; Bernard, A.; Vanel, Q.; Stokes, A. Influence of plant root system morphology and architectural traits on soil shear resistance. Plant Soil 2014, 377, 43–61. [Google Scholar] [CrossRef]
- Angers, D.A.; Caron, J. Plant-induced changes in soil structure: Processes and feedbacks. Bioge-Ochemistry 1998, 42, 55–72. [Google Scholar]
- Wu, T.H.; McKinnell, W.P., III; Swanston, D.N. Strength of tree roots and landslides on Prince of Wales Island, Alaska. Can. Geotech. J. 1979, 16, 19–33. [Google Scholar] [CrossRef]
- Wu, T.H.; McOmber, R.M.; Erb, R.T.; Beal, P.E. Study of soil-root interaction. J. Geotech. Eng. 1988, 114, 1351–1375. [Google Scholar] [CrossRef]
- Pollen, N.; Simon, A. Estimating the mechanical effects of riparian vegetation on stream bank stability using a fiber bundle model. Water Resour Res. 2005, 41, W07025. [Google Scholar] [CrossRef]
- Schwarz, M.; Cohen, D.; Or, D. Root-soil mechanical interactions during pullout and failure of root bundles. J. Geophys. Res. Earth Surf. 2010, 35, 354–361. [Google Scholar]
- Schwarz, M.; Lehmann, P.; Or, D. Quantifying lateral root reinforcement in steep slopes—From a bundle of roots to tree stands. Earth Surf Process Landf. 2010, 35, 354–367. [Google Scholar] [CrossRef]

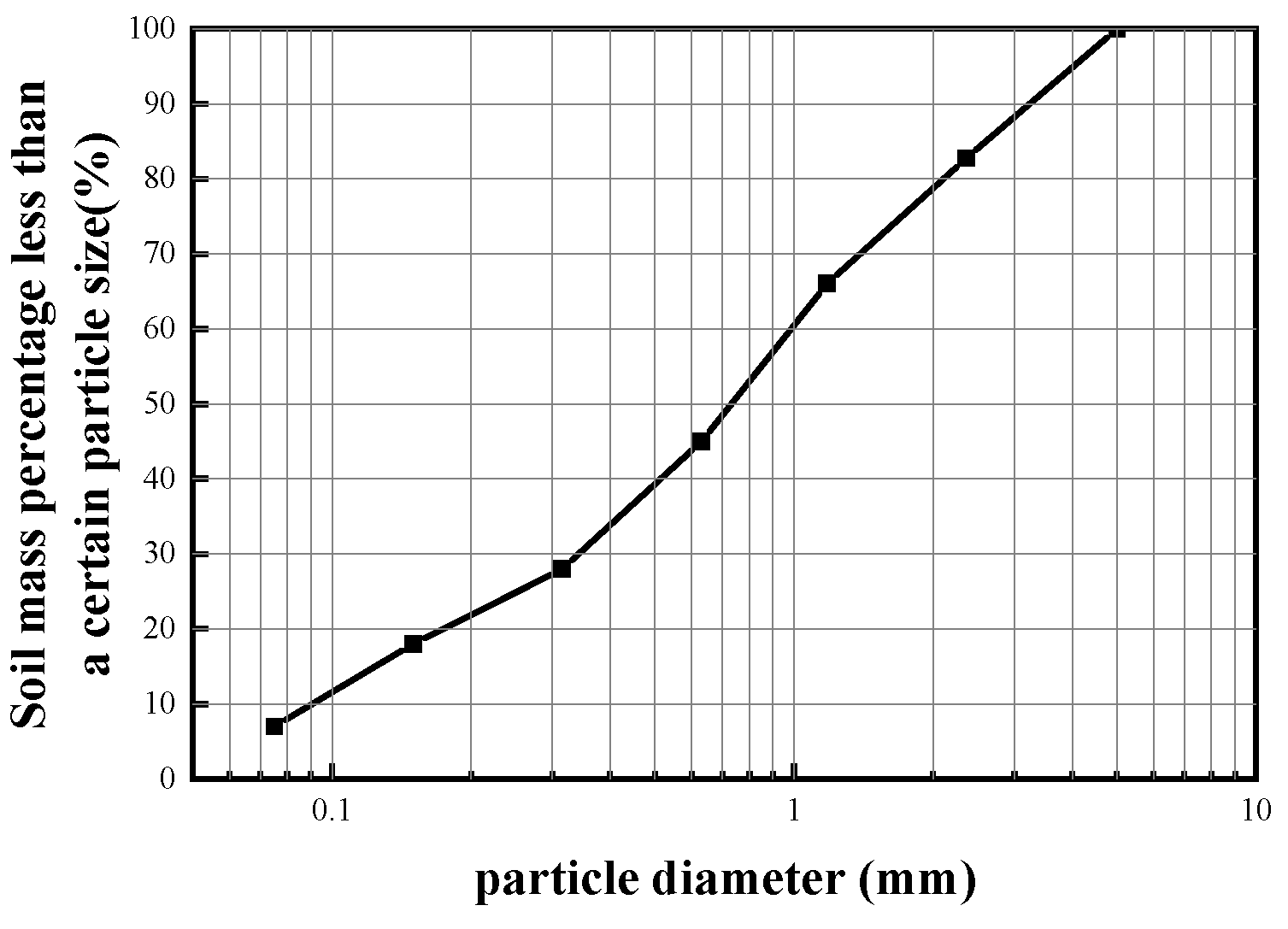
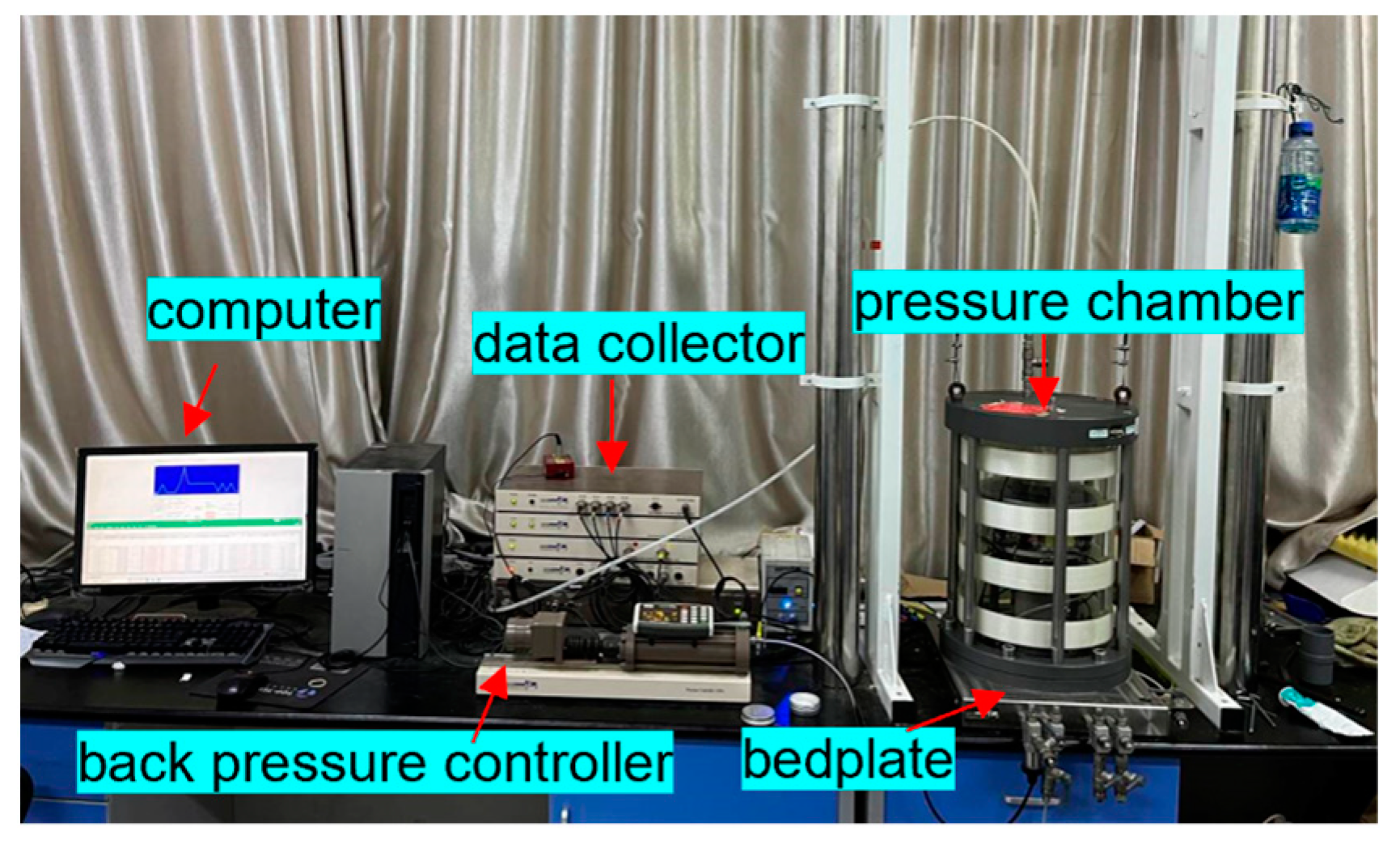

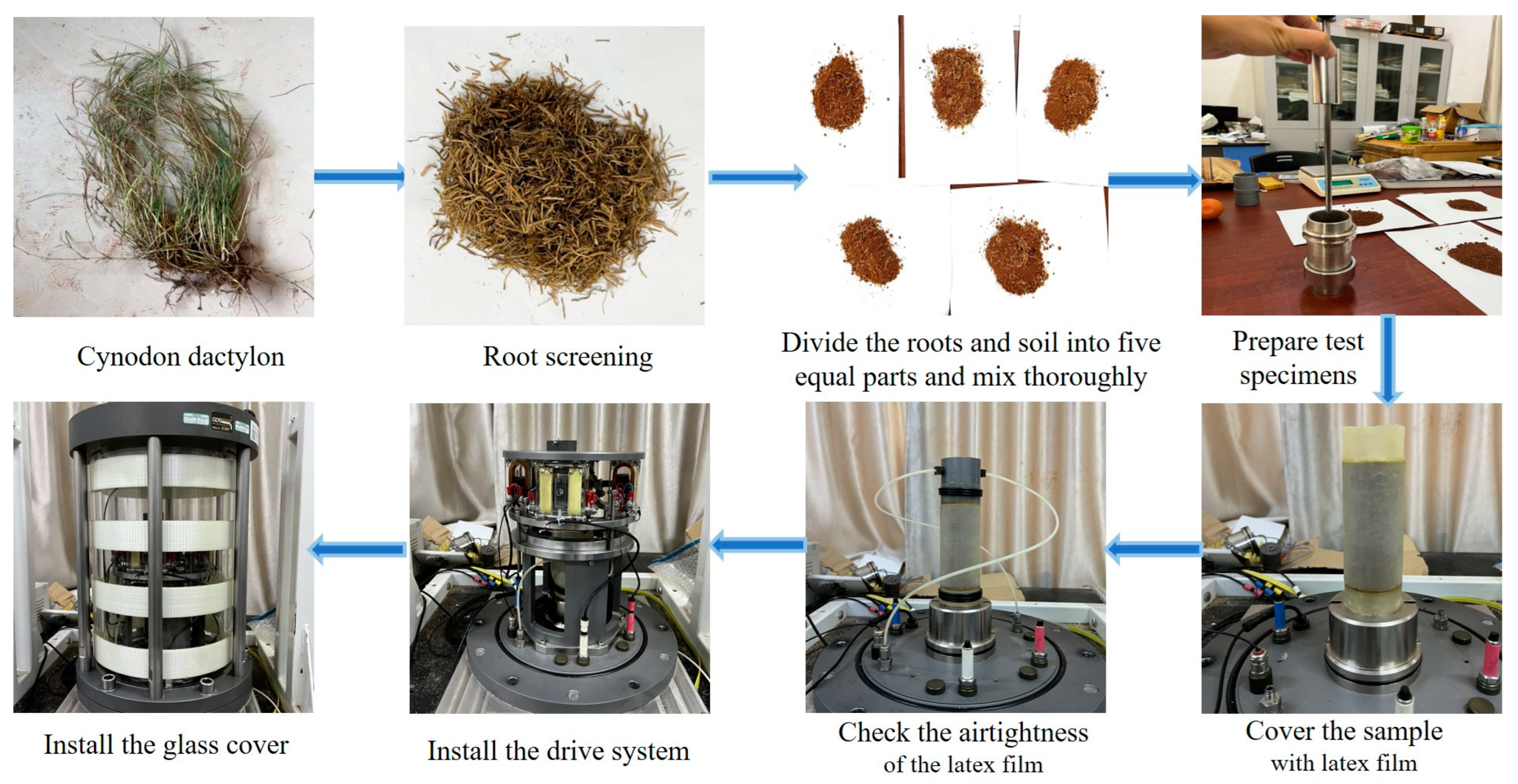


| Coefficient of Uniformity | Curvature Coefficient | Plasticity Limit | Liquid Limit | Plasticity Index |
|---|---|---|---|---|
| 14.29 | 1.11 | 22.87% | 41.63% | 18.76 |
| 100 | 200 | 300 | ||||
|---|---|---|---|---|---|---|
| 0 | 84.75 | 3.97 | 108.08 | 4.66 | 134.03 | 5.1 |
| 1% Uniform Distribution | 92.55 | 3.95 | 116.67 | 4.41 | 140.28 | 4.64 |
| 1% Upper Distribution | 97.57 | 3.67 | 120.83 | 4.19 | 138.91 | 4.34 |
| 1% Lower Distribution | 91.86 | 4.4 | 114.71 | 4.81 | 138.4 | 5.22 |
| 1.5% Uniform Distribution | 108.16 | 4.02 | 136.64 | 4.75 | 155.19 | 4.72 |
| 2% Uniform Distribution | 101.81 | 4.27 | 130.49 | 5.23 | 147.99 | 5.79 |
| 2.5% Uniform Distribution | 100.9 | 4.26 | 131.8 | 4.73 | 148.23 | 5.59 |
| 3% Uniform Distribution | 99.8 | 4.23 | 131.97 | 4.44 | 148.58 | 5.23 |
| 100 | 200 | 300 | ||||
|---|---|---|---|---|---|---|
| 0 | 5.36 | 6.58 | 4.76 | 4.03 | 4.46 | 3.63 |
| 1% Uniform Distribution | 5.06 | 5.71 | 4.47 | 3.15 | 4.39 | 3.32 |
| 1% Upper Distribution | 5.42 | 5.55 | 4.85 | 4.39 | 4.49 | 2.91 |
| 1% Lower Distribution | 4.85 | 5.31 | 4.43 | 3.68 | 4.24 | 3.20 |
| 1.5% Uniform Distribution | 4.92 | 4.18 | 4.52 | 3.08 | 4.36 | 3.06 |
| 2% Uniform Distribution | 5.14 | 4.49 | 4.44 | 2.81 | 4.20 | 2.62 |
| 2.5% Uniform Distribution | 5.15 | 4.11 | 4.62 | 3.08 | 4.18 | 2.31 |
| 3% Uniform Distribution | 5.39 | 4.20 | 4.82 | 3.04 | 4.27 | 2.19 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, Q.; Tang, C.; Zhang, C.; Ma, Y. Experimental Study of Influence of Plant Roots on Dynamic Characteristics of Clay. Appl. Sci. 2025, 15, 495. https://doi.org/10.3390/app15020495
Shen Q, Tang C, Zhang C, Ma Y. Experimental Study of Influence of Plant Roots on Dynamic Characteristics of Clay. Applied Sciences. 2025; 15(2):495. https://doi.org/10.3390/app15020495
Chicago/Turabian StyleShen, Quan, Cong Tang, Chengli Zhang, and Yongchun Ma. 2025. "Experimental Study of Influence of Plant Roots on Dynamic Characteristics of Clay" Applied Sciences 15, no. 2: 495. https://doi.org/10.3390/app15020495
APA StyleShen, Q., Tang, C., Zhang, C., & Ma, Y. (2025). Experimental Study of Influence of Plant Roots on Dynamic Characteristics of Clay. Applied Sciences, 15(2), 495. https://doi.org/10.3390/app15020495






