Study of Basalt Fibers and Graphene Enriched Polymers on Bond Behavior of FRP Bars in Concrete
Abstract
1. Introduction
2. Experimental Study
2.1. Materials
2.2. Pull-Out Tests
3. Numerical Model
3.1. Determination of the Parameters
3.2. Thermal Degradation Model
3.3. FE Model
4. Results and Discussions
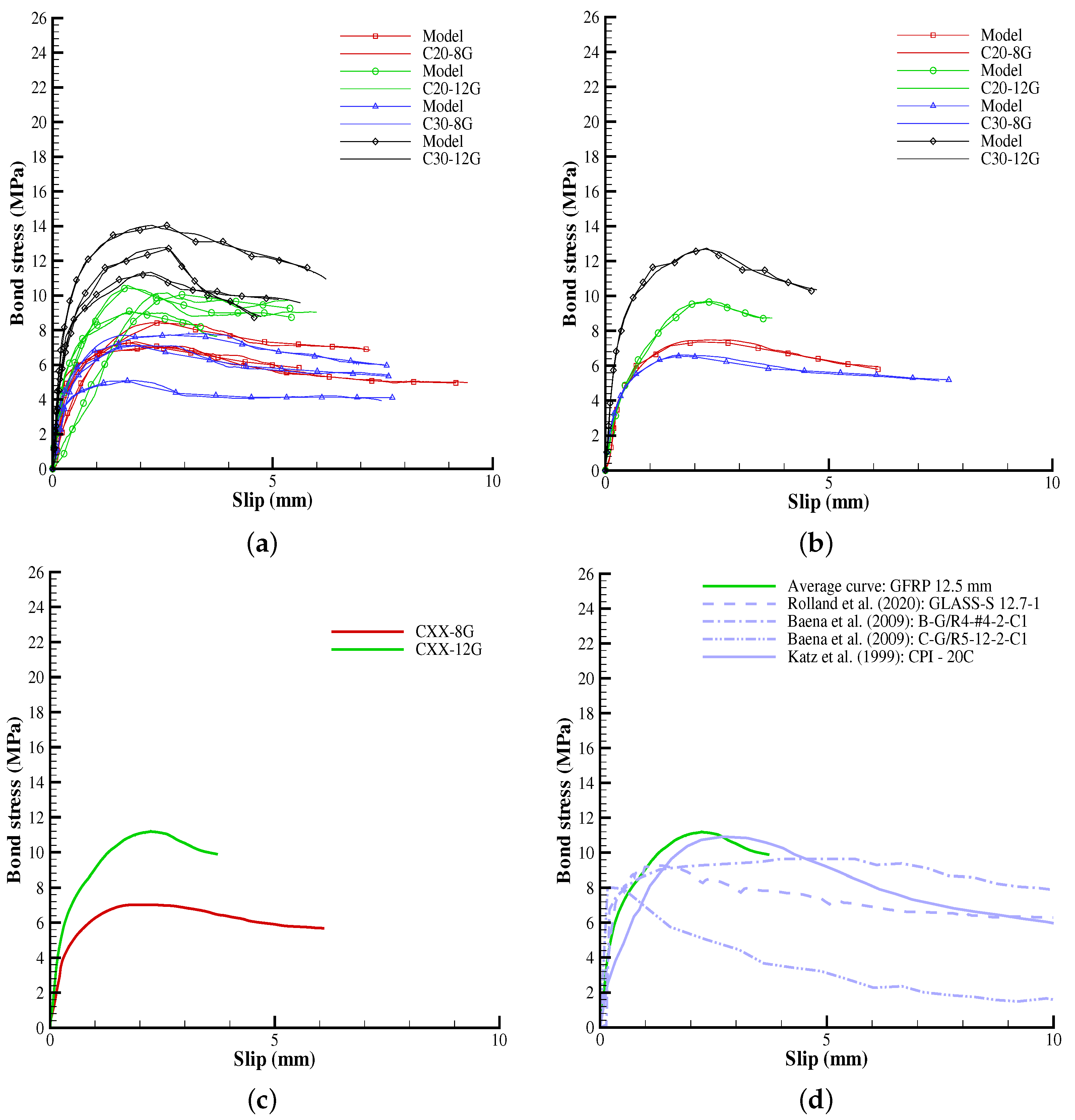
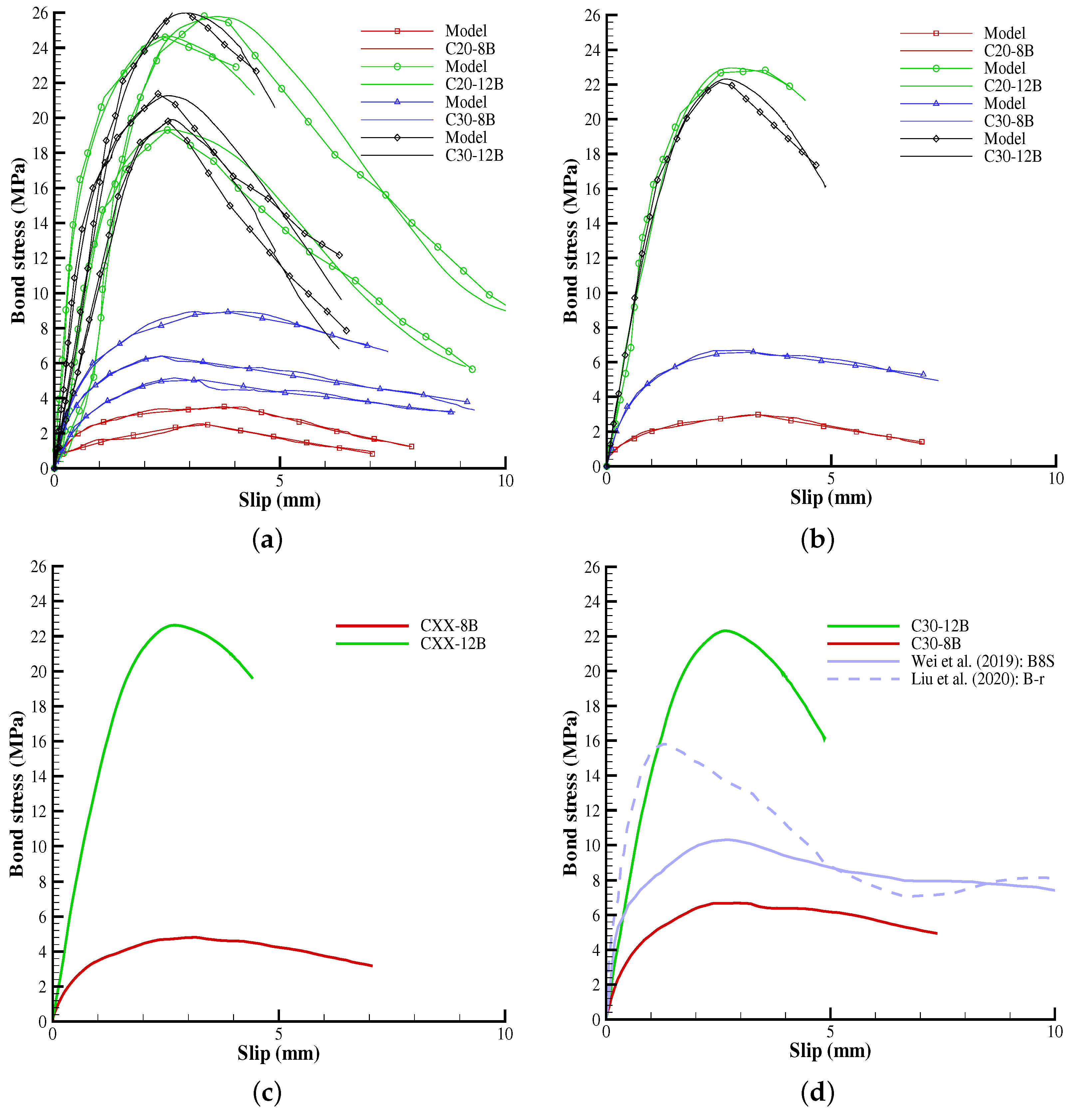
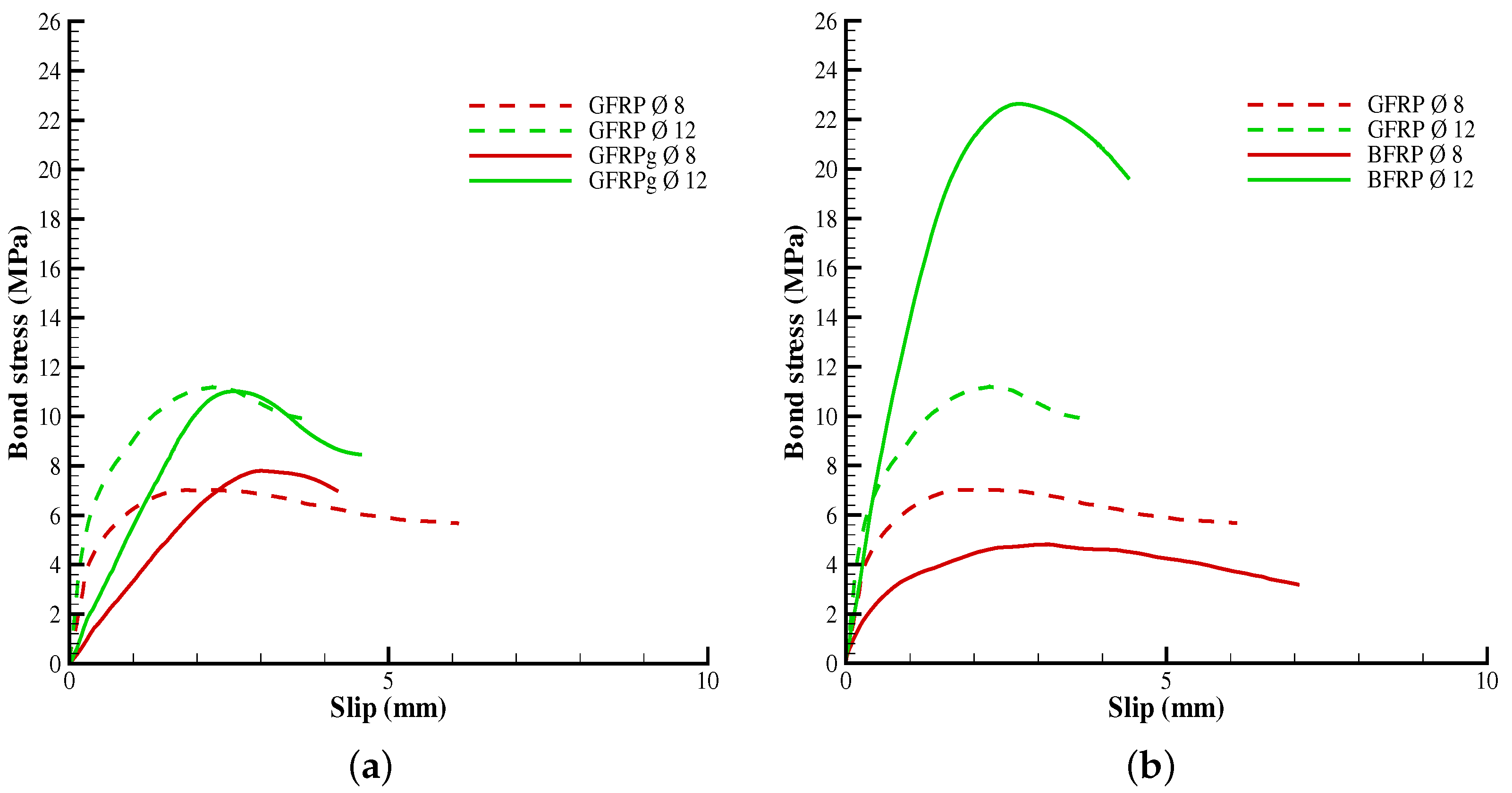
4.1. Room Temperature Results
Discussion
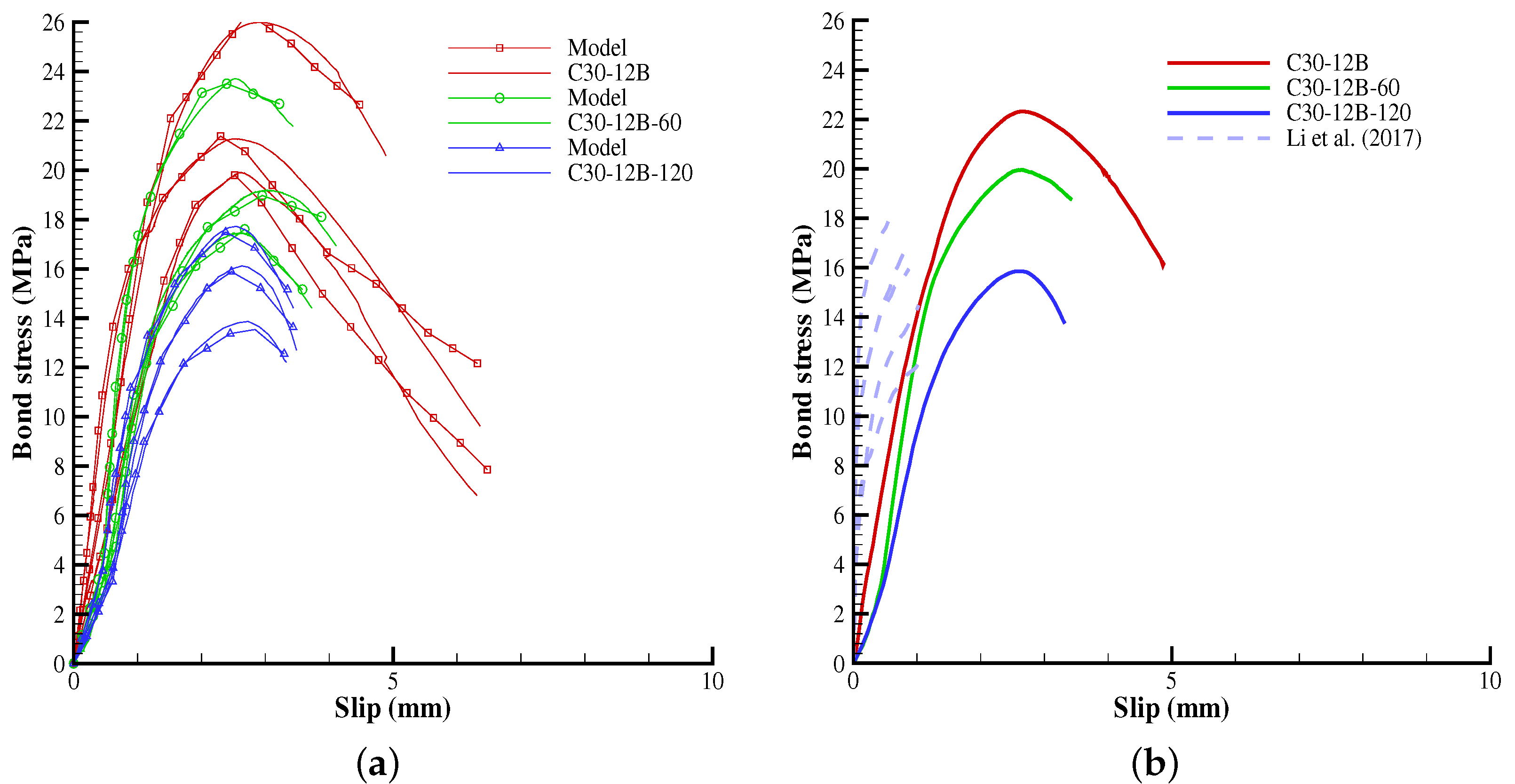
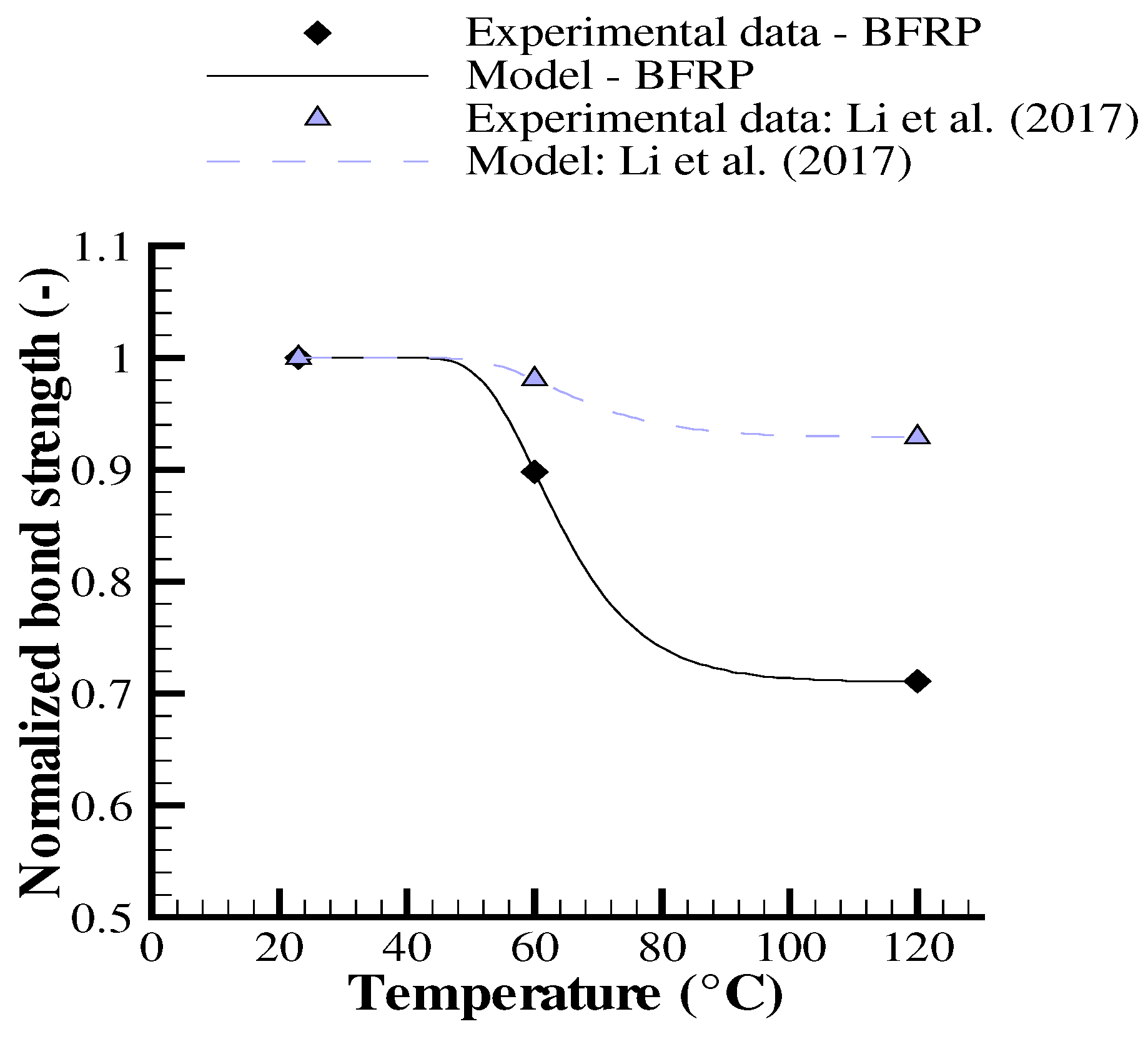
4.2. Thermal Effects
Discussion
5. Conclusions
- Combination BRFP/helically-wounded presented a superior behavior in terms of strength than other cases studied in this work and other BRFP cases considered in the literature at room and higher temperatures. The former was the only case to have a bond strength above minimum levels defined in project standards such as GOST 31938-2012 [57] and CAN/CSA S807-19 [58], in all situations tested in this work.
- Cases were GNP was introduced in a polyester matrix (GFRPg) presented a similar behavior of bars were vinyl-ester matrix was used (GFRP). However, behavior of the former was far superior than similar cases taken from the literature (polyester without GNP), at room and higher temperatures. Therefore results are encouraging, but more studies are necessary regarding GNP use.
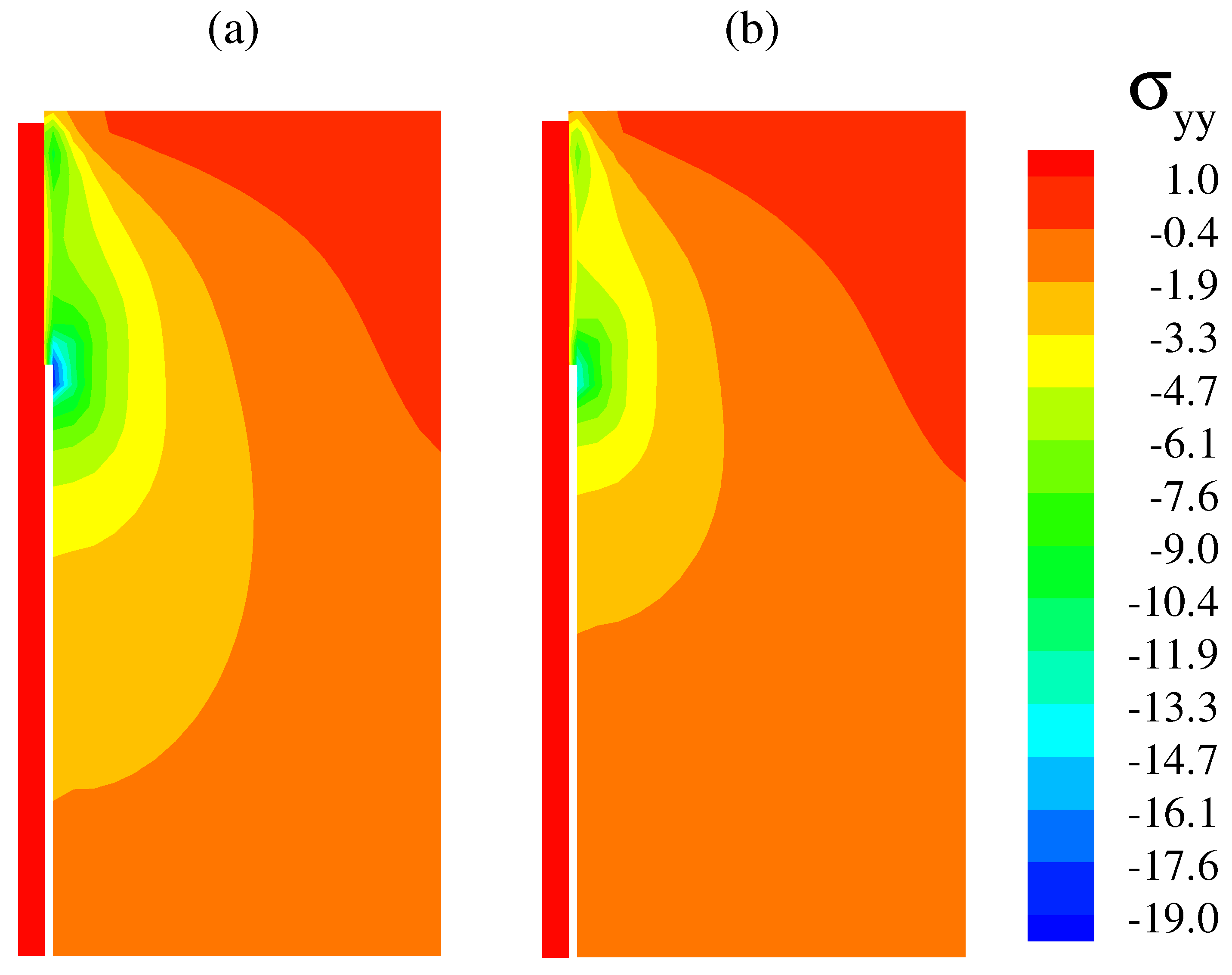
- Numerical simulations combining Rolland et al. model and an optimization method to search for parameters showed to be an excellent tool to fit experimental results. Stresses in the concrete remained always below concrete strength. At the adherent zone, these stresses stayed mostly uniform, except near the end of the zone where stress concentrations were observed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Combinations | Interpolated Data | Optimized Parameters | |||||
|---|---|---|---|---|---|---|---|
| (MPa) | (mm) | (mm) | (MPa) | (MPa) | |||
| C20-8G | 7.4858 | 2.36 | 0.11751 | 0.9314 | −9.0998 | 7.7094 | 0.066628 |
| C20-12G | 9.7153 | 2.24 | 0.027745 | 0.7701 | −9.9223 | 2.4664 | 0.09007 |
| C30-8G | 6.6223 | 1.77 | 0.034744 | 1.01 | 3.9016 | 5.392 | 0.331 |
| C30-12G | 12.6777 | 2.23 | 0.10928 | 3.7823 | −0.83611 | 5.7853 | 0.19301 |
| Combinations | Interpolated Data | Optimized Parameters | |||||
|---|---|---|---|---|---|---|---|
| (MPa) | (mm) | (mm) | (MPa) | (MPa) | |||
| C20-8Gg | 5.9414 | 3.01 | 2.12061 | 4.92525 | −31.3836 | 1.0618 | 0.0133 |
| C20-12Gg | 12.8028 | 2.46 | 1.45425 | 8.66494 | −40.6756 | 1.91502 | 0.0757943 |
| C30-8Gg | 9.6720 | 2.95 | 0.1852538 | 0.421723 | −171.9659 | 0.984 | 0.0163 |
| C30-12Gg | 9.3242 | 2.73 | 0.45663 | 2.9981 | 4.7371 | 0.83246 | 1.1013 |
| Combinations | Interpolated Data | Optimized Parameters | |||||
|---|---|---|---|---|---|---|---|
| (MPa) | (mm) | (mm) | (MPa) | (MPa) | |||
| C20-8B | 2.9619 | 3.28 | 0.0540546 | 0.6687305 | −170.1165 | 2.425713 | 0.00798 |
| C30-8B | 6.6866 | 2.93 | 0.225253 | 2.0323 | −156.5318 | 3.140931 | 0.0061006 |
| C20-12B | 22.9582 | 2.78 | 0.5645342 | 6.899 | −126.3175 | 3.421 | 0.0153539 |
| C30-12B | 22.3209 | 2.67 | 0.836405 | 12.3363 | −32.8685 | 1.851 | 0.122 |
| Combinations | Interpolated Data | Optimized Parameters | |||||
|---|---|---|---|---|---|---|---|
| (MPa) | (mm) | (mm) | (MPa) | (MPa) | |||
| C30-12G-60C | 10.2925 | 2.05 | 0.26389 | 3.498 | 5.1516 | 4.3894 | 0.3236 |
| C30-12G-120C | 7.8488 | 2.10 | 0.21999 | 2.5257 | −5.5794 | 4.1643 | 0.17586 |
| C30-12Gg-60C | 8.0323 | 2.89 | 0.266385 | 1.88402 | -75.5048 | 3.24813 | 0.0306622 |
| C30-12Gg-120C | 6.2872 | 2.74 | 0.26998 | 1.53913 | −49.7424 | 3.31262 | 0.0413618 |
| C30-12B-60C | 19.9539 | 2.64 | 0.54549 | 4.0614 | 3.2486 | 3.588 | 0.20887 |
| C30-12B-120C | 15.8601 | 2.63 | 0.614717 | 4.1399 | −31.1235 | 2.68845 | 0.13722 |
References
- Achillides, Z.; Pilakoutas, K. Bond behavior of fiber reinforced polymer bars under direct pullout conditions. J. Compos. Constr. 2004, 8, 173–181. [Google Scholar] [CrossRef]
- Baena, M.; Torres, L.; Turon, A.; Barris, C. Experimental study of bond behaviour between concrete and FRP bars using a pull-out test. Compos. B Eng. 2009, 40, 784–797. [Google Scholar] [CrossRef]
- El Refai, A.; Ammar, M.A.; Masmoudi, R. Bond performance of basalt fiber-reinforced polymer bars to concrete. J. Compos. Constr. 2015, 19, 395–406. [Google Scholar] [CrossRef]
- Roll, A.; Quiertant, M.; Khadour, A.; Chataigner, S.; Benzarti, K.; Argoul, P. Experimental investigations on the bond behavior between concrete and FRP reinforcing bars. Constr. Build. Mater. 2018, 173, 136–148. [Google Scholar]
- Aydin, F. Experimental investigation of thermal expansion and concrete strength effects on FRP bars behavior embedded in concrete. Constr. Build. Mater. 2018, 163, 1–8. [Google Scholar] [CrossRef]
- Aravas, N. Bond behaviour of sand coated GFRP bars to concrete at elevated temperature—Definition of bond vs. slip relations. Compos. B Eng. 2019, 160, 329–340. [Google Scholar]
- D’Antino, T.; Pisani, M.A.; Poggi, C. Effect of the environment on the performance of GFRP reinforcing bars. Compos. B Eng. 2018, 141, 123–136. [Google Scholar] [CrossRef]
- Basaran, B.; Kalkan, I. Investigation on variables affecting bond strength between FRP reinforcing bar and concrete by modified hinged beam tests. Compos. Struct. 2020, 242, 112185. [Google Scholar] [CrossRef]
- Tighiouart, B.; Benmokrane, B.; Gao, D. Investigation of bond in concrete member with fibre reinforced Polymer. Constr. Build. Mater. 1998, 12, 453–462. [Google Scholar] [CrossRef]
- Godat, A.; L’Hady, A.; Chaallal, O.; Neale, K.W. Bond behavior of the ETS FRP bar shear-strengthening method. J. Compos. Constr. 2012, 16, 529–534. [Google Scholar] [CrossRef]
- Miranda, M.P.; Morsch, I.B.; Brisotto, D.D.S.; Bittencourt, E.; Carvalho, E.P. Steel-concrete bond behavior: An experimental and numerical study. Constr. Build. Mater. 2021, 271, 121918. [Google Scholar] [CrossRef]
- Cosenza, E.; Manfredi, G.; Realfonzo, R. Behavior and modeling of bond of FRP rebars to concrete. J. Compos. Constr. 1997, 1, 40–51. [Google Scholar] [CrossRef]
- Shen, D.; Ojha, B.; Shi, X.; Zhang, H.; Shen, J. Bond stress–slip relationship between basalt fiber-reinforced polymer bars and concrete using a pull-out test. J. Reinf. Plast. Compos. 2016, 35, 747–763. [Google Scholar] [CrossRef]
- Benmokrane, B.; Ali, A.H.; Mohamed, H.M.; ElSafty, A.; Manalo, A. Laboratory assessment and durability performance of vinyl-ester, polyester, and epoxy glass-FRP bars for concrete structures. Compos. B Eng. 2017, 114, 163–174. [Google Scholar] [CrossRef]
- FIB. FIB Bulletin 40: FRP Reinforcement in RC Structures; International Federation for Structural Concrete: Lausanne, Switzerland, 2007. [Google Scholar]
- Bank, L.C. Composites for Construction: Structural Design with FRP Materials; John Wiley and Sons: Hoboken, NJ, USA, 2006. [Google Scholar]
- Prolongo, S.G.; Moriche, R.; Jiménez-Suárez, A.; Sánchez, M.; Ureña, A. Advantages and disadvantages of the addition of graphene nanoplatelets to epoxy resins. Eur. Polym. J. 2016, 61, 231–259. [Google Scholar] [CrossRef]
- Saleem, H.; Edathil, A.; Ncube, T.; Pokhrel, J.; Khoori, S.; Abraham, A.; Mittal, V. Mechanical and thermal properties of thermoset–graphene nanocomposites. Macromol. Mater. Eng. 2016, 301, 231–259. [Google Scholar] [CrossRef]
- Cataldi, P.; Athanassiou, A.; Bayer, I.S. Graphene nanoplatelets-based advanced materials and recent progress in sustainable applications. J. Appl. Sci. 2018, 8, 1438. [Google Scholar] [CrossRef]
- Dittrich, B.; Wartig, K.A.; Hofmann, D.; Mülhaupt, R.; Schartel, B. Flame retardancy through carbon nanomaterials: Carbon black, multiwall nanotubes, expanded graphite, multi-layer graphene and graphene in polypropylene. Polym. Degrad. Stab. 2013, 98, 1495–1505. [Google Scholar] [CrossRef]
- Inuwa, I.M.; Hassan, A.; Wang, D.Y.; Samsudin, S.A.; Haafiz, M.M.; Wong, S.L.; Jawaid, M. Influence of exfoliated graphite nanoplatelets on the flammability and termal properties of polyethylene terephthalate/polypropylene nanocomposites. Polym. Degrad. Stab. 2014, 110, 137–148. [Google Scholar] [CrossRef]
- Carter, J.; Genikomsou, A.S. Investigation on modeling parameters of concrete beams reinforced with basalt FRP bars. Front. Struct. Civ. Eng. 2019, 12, 1520–1530. [Google Scholar] [CrossRef]
- Jumaa, G.B.; Yousif, A.R. Size effect on the shear failure of high-strength concrete beams reinforced with basalt FRP bars and stirrups. Constr. Build. Mater. 2020, 209, 77–94. [Google Scholar] [CrossRef]
- Attia, K.; El Refai, A.; Alnahhal, W. Flexural Behavior of Basalt Fiber–Reinforced Concrete Slab Strips with BFRP Bars: Experimental Testing and Numerical Simulation. J. Compos. Constr. 2020, 24, 04020007. [Google Scholar] [CrossRef]
- Atutis, M.; Kawashima, S. Analysis of Flexural Concrete Beams Prestressed with Basalt Composite Bars. Compos. Struct. 2020, 243, 112172. [Google Scholar] [CrossRef]
- Lu, Z.; Su, L.; Xian, G.; Lu, B.; Xie, J. Durability study of concrete-covered basalt fiber-reinforced polymer (BFRP) bars in marine environment. Compos. Struct. 2020, 234, 111650. [Google Scholar] [CrossRef]
- Cousin, P.; Hassan, M.; Vijay, P.V.; Robert, M.; Benmokrane, B. Chemical resistance of carbon, basalt, and glass fibers used in FRP reinforcing bars. J. Compos. Mater. 2019, 53, 3651–3670. [Google Scholar] [CrossRef]
- Zhou, A.; Qiu, Q.; Chow, C.L.; Lau, D. Interfacial performance of aramid, basalt and carbon fiber reinforced polymer bonded concrete exposed to high temperature. Compos. Part A Appl. Sci. Manuf. 2020, 131, 105802. [Google Scholar] [CrossRef]
- Wang, H.; Sun, X.; Peng, G.; Luo, Y.; Ying, Q. Experimental study on bond behaviour between BFRP bar and engineered cementitious composite. Constr. Build. Mater. 2015, 95, 448–456. [Google Scholar] [CrossRef]
- Liu, X.; Wang, X.; Xie, K.; Wu, Z.; Li, F. Bond Behavior of Basalt Fiber-Reinforced Polymer Bars Embedded in Concrete Under Mono-tensile and Cyclic Loads. Int. J. Concr. Struct. Mater. 2020, 14, 19. [Google Scholar] [CrossRef]
- Henin, E.; Tawadrous, R.; Morcous, G. Effect of surface condition on the bond of Basalt Fiber-Reinforced Polymer bars in concrete. Constr. Build. Mater. 2019, 226, 449–458. [Google Scholar] [CrossRef]
- Wei, W.; Liu, F.; Xiong, Z.; Lu, Z.; Li, L. Bond performance between fibre-reinforced polymer bars and concrete under pull-out tests. Constr. Build. Mater. 2019, 227, 116803. [Google Scholar] [CrossRef]
- Katz, A.; Berman, N.; Bank, L.C. Effect of high temperature on bond strength of FRP rebars. J. Compos. Constr. 1999, 3, 73–81. [Google Scholar] [CrossRef]
- Solyom, S.; Di Benedetti, M.; Guadagnini, M.; Balázs, G.L. Effect of temperature on the bond behaviour of GFRP bars in concrete. Compos. B Eng. 2020, 183, 107602. [Google Scholar] [CrossRef]
- Li, C.; Gao, D.; Wang, Y.; Tang, J. Effect of high temperature on the bond performance between basalt fibre reinforced polymer (BFRP) bars and concrete. Constr. Build. Mater. 2017, 141, 44–51. [Google Scholar] [CrossRef]
- Hamad, R.J.; Johari, M.M.; Haddad, R.H. Mechanical properties and bond characteristics of different fiber reinforced polymer rebars at elevated temperatures. Constr. Build. Mater. 2017, 142, 521–535. [Google Scholar] [CrossRef]
- Salameh, A.; Hawileh, R.; Safieh, H.; Assad, M.; Abdalla, J. Elevated Temperature Effects on FRP–Concrete Bond Behavior: A Comprehensive Review and Machine Learning-Based Bond Strength Prediction. Infrastructures 2024, 10, 183. [Google Scholar] [CrossRef]
- Kazemi, F.; Asgarkhani, N.; Shafighfard, T.; Jankowski, R.; Yoo, D.Y. Machine-learning methods for estimating performance of structural concrete members reinforced with fiber-reinforced polymers. Arch. Comput. Methods Eng. 2025, 32, 571–603. [Google Scholar] [CrossRef]
- Fahmy, M.F.; Ahmed, S.A.; Wu, Z. Bar surface treatment effect on the bond-slip behavior and mechanism of basalt FRP bars embedded in concrete. Constr. Build. Mater. 2021, 289, 122844. [Google Scholar] [CrossRef]
- NBR 6118; Design of Concrete Structures—Procedure. ABNT: Rio de Janeiro, Brazil, 2014. (In Portuguese)
- ISO 21920-1; Geometrical Product Specifications (GPS)—Surface Texture: Profile—Part 1: Indication of Surface Texture. ISO: Geneva, Switzerland, 2021.
- ASTM D7957; Standard Specification for Solid Round Glass Fiber Reinforced Polymer Bars for Concrete Reinforcement. ASTM: West Conshohocken, PA, USA, 2022.
- D7205; Standard Test Method for Tensile Properties of Fiber Reinforced Polymer Matrix Composite Bars. ASTM: West Conshohocken, PA, USA, 2016.
- NBR 17054; Aggregates—Determination of Granulometric Composition—Test Method. ABNT: Rio de Janeiro, Brazil, 2022. (In Portuguese)
- NBR 16916; Fine Aggregate—Determination of Density and Water Absorption. ABNT: Rio de Janeiro, Brazil, 2021. (In Portuguese)
- NBR 16917; Coarse Aggregate—Determination of Density and Water Absorption. ABNT: Rio de Janeiro, Brazil, 2021. (In Portuguese)
- NBR 5739; Concrete—Compression Test of Cylindrical Specimens. ABNT: Rio de Janeiro, Brazil, 2018. (In Portuguese)
- FIB. FIB Model Code for Concrete Structures 2010; International Federation for Structural Concrete: Lausanne, Switzerland, 2013. [Google Scholar]
- D7913; Standard Test Method for Bond Strength of Fiber-Reinforced Polymer Matrix Composite Bars to Concrete by Pullout Testing. ASTM: West Conshohocken, PA, USA, 2020.
- Cox, J.V.; Herrmann, L.R. Development of a plasticity bond model for steel reinforcement. Mech. Cohesive Frict. Mater. 1998, 3, 155–180. [Google Scholar] [CrossRef]
- Li, J. An Investigation of Behavior and Modeling of Bond for Reinforced Concrete. Ph.D Thesis, University of Washington, Seattle, WA, USA, 2010. [Google Scholar]
- Seok, S.; Haikal, G.; Ramirez, J.A.; Lowes, L.N. High-resolution finite element modeling for bond in high-strength concrete beam. Eng. Struct. 2018, 173, 918–932. [Google Scholar] [CrossRef]
- Rolland, A.; Argoul, P.; Benzarti, K.; Quiertant, M.; Chataigner, S.; Khadour, A. Analytical and numerical modeling of the bond behavior between FRP reinforcing bars and concrete. Constr. Build. Mater. 2020, 231, 117160. [Google Scholar] [CrossRef]
- Mirjalili, S.; Lewis, A. The Whale Optimization Algorithm. Adv. Eng. Softw. 2016, 95, 51–67. [Google Scholar] [CrossRef]
- Correia, J.R.; Gomes, M.M.; Pires, J.M.; Branco, F.A. Mechanical behaviour of pultruded glass fibre reinforced polymer composites at elevated temperature: Experiments and model assessment. Compos. Struct. 2013, 98, 303–313. [Google Scholar] [CrossRef]
- Solyom, S.; Balázs, G.L. Bond of FRP bars with different surface characteristics. Constr. Build. Mater. 2020, 264, 119839. [Google Scholar] [CrossRef]
- GOST 31938-2012; Fiber-Reinforced Polymer Bar for Concrete Reinforcement. General Specifications. GOST: Moscow, Russia, 2012.
- CAN/CSA-S6-06 (R2012); Canadian Highway Bridge Design Code. CSA: Mississauga, ON, Canada, 2012.
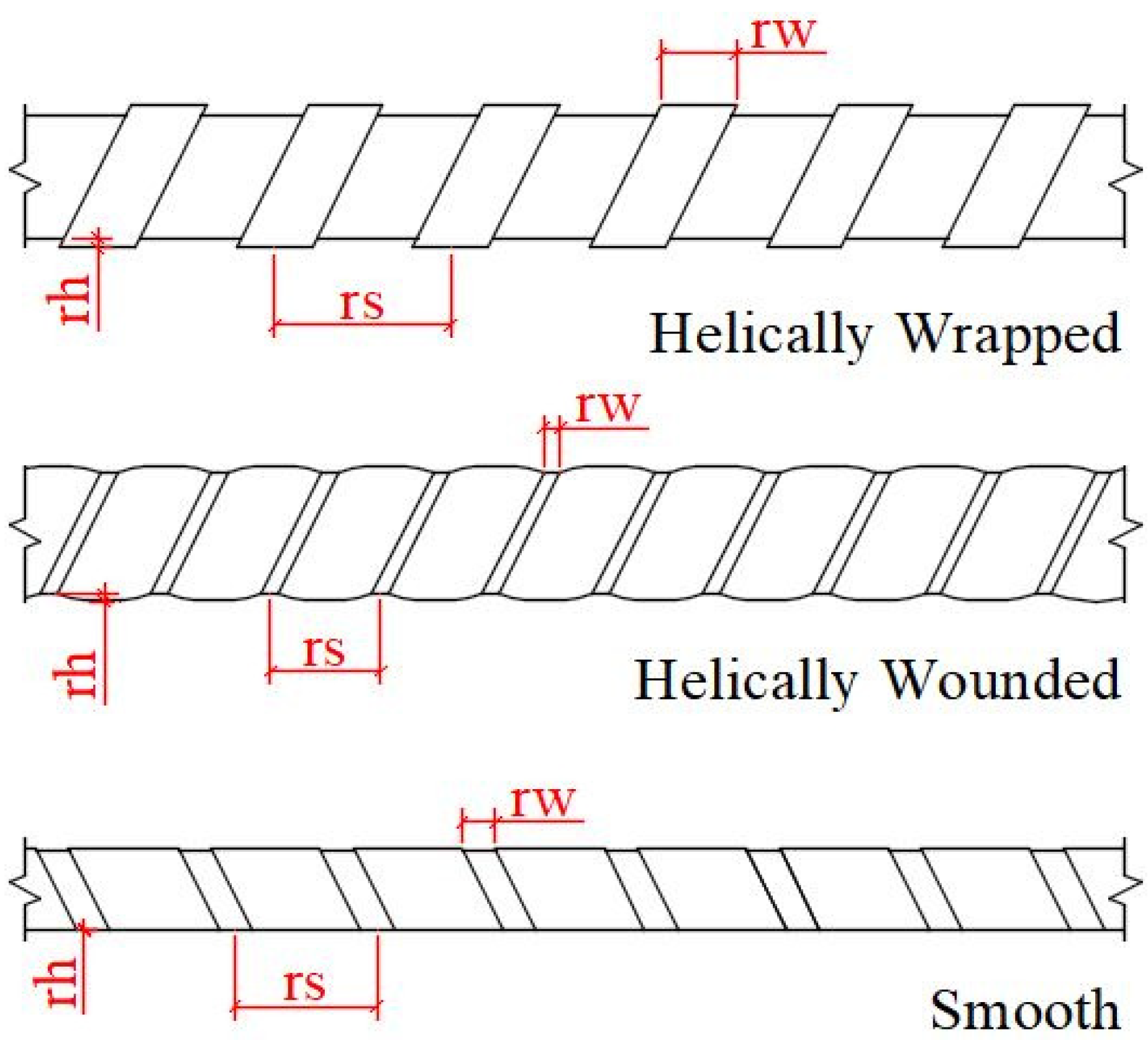
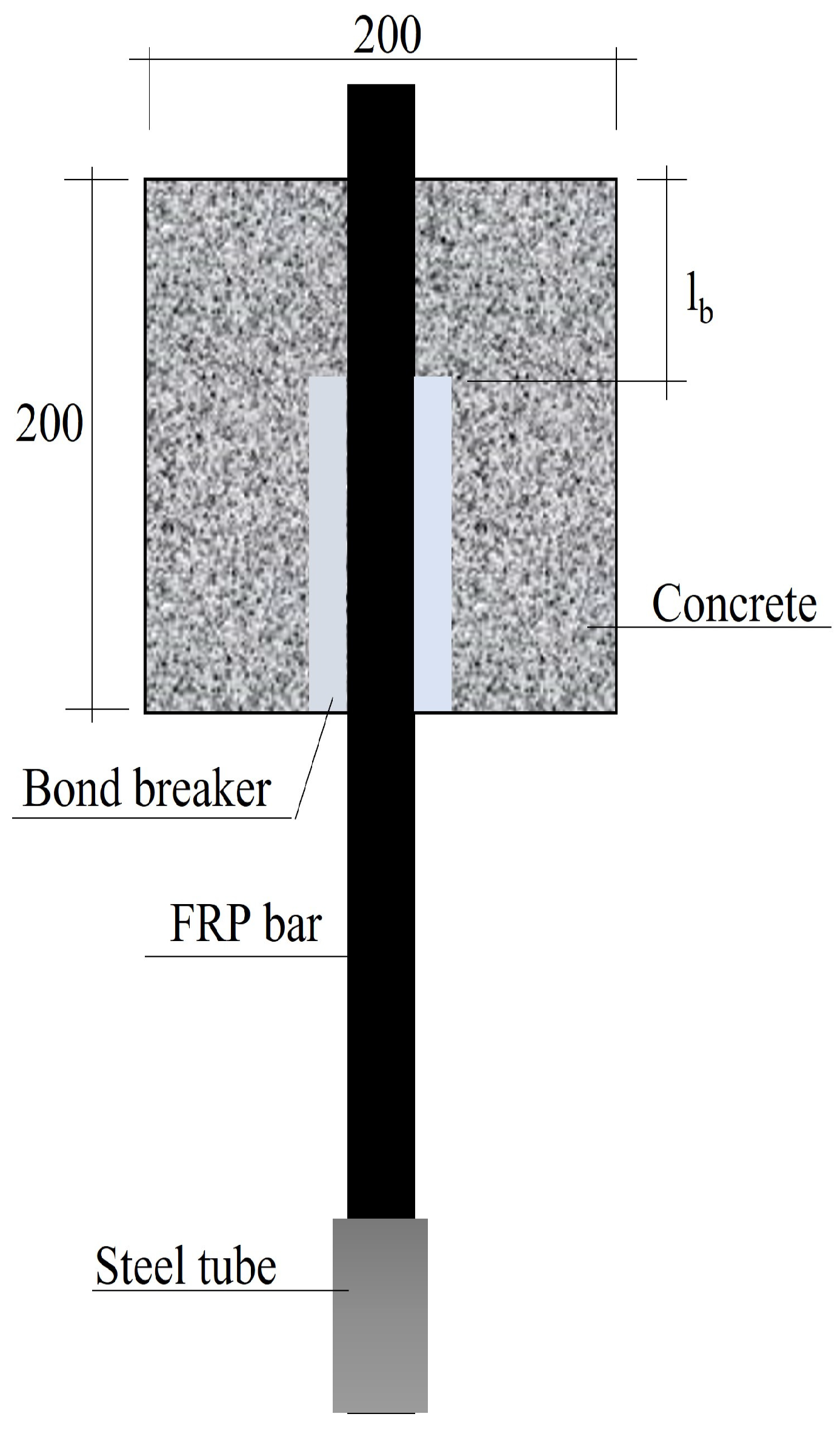

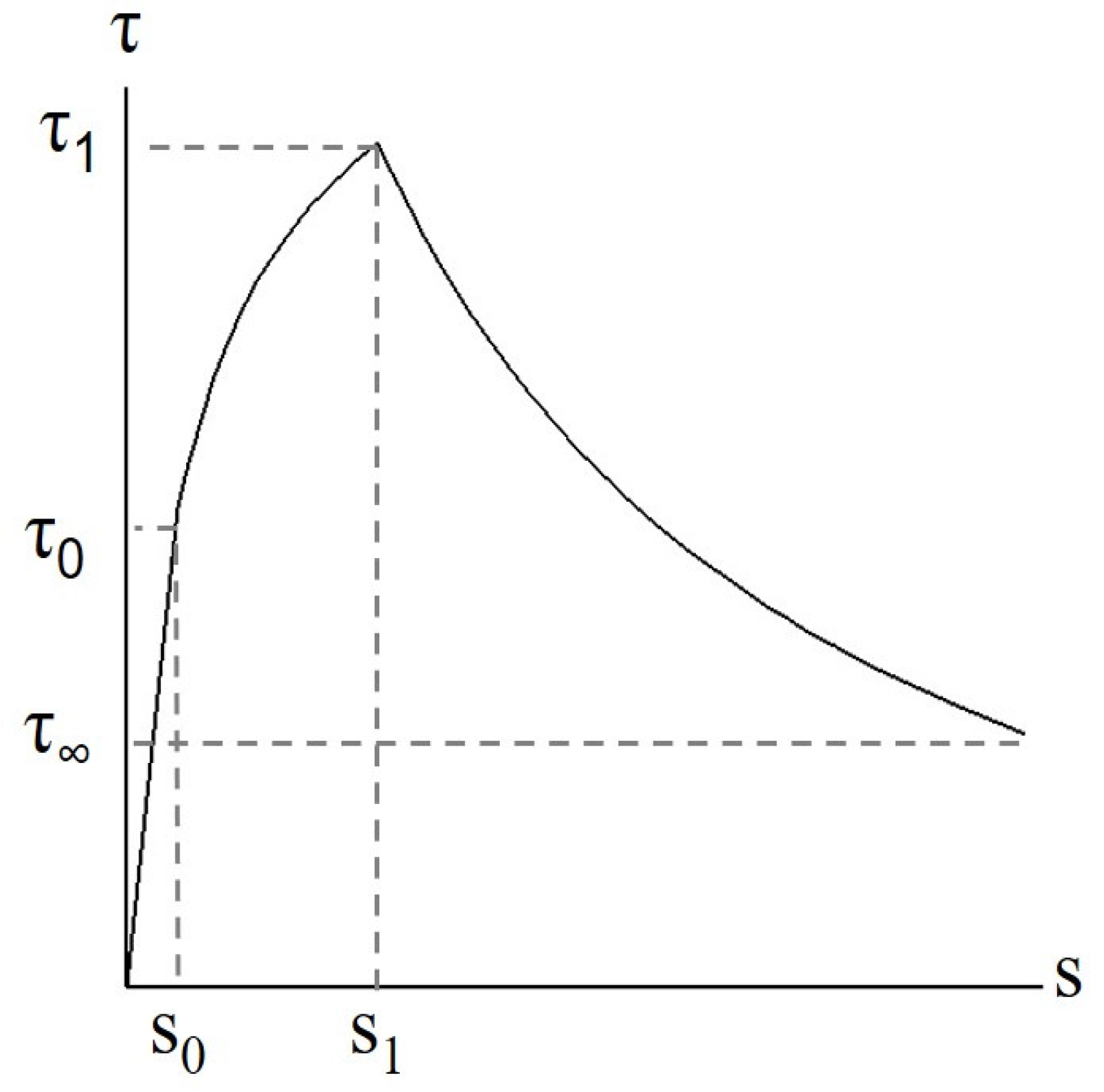
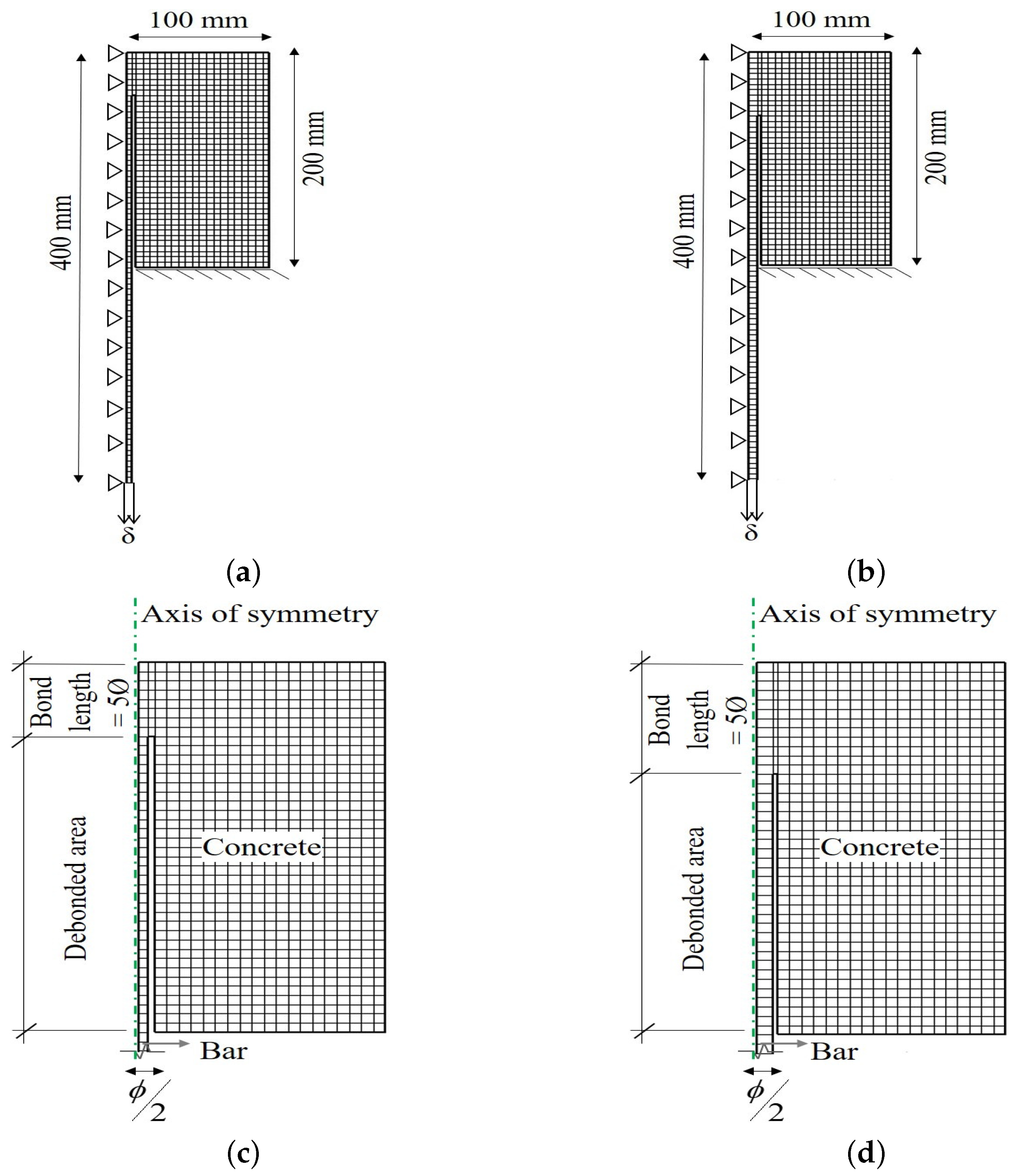







| Bar | rh (mm) | rs (mm) | rw (mm) | Ra (μm) | Fiber Fraction (%) | (MPa) | E (GPa) | Tg (°C) |
|---|---|---|---|---|---|---|---|---|
| Cxx-8G-yy | 0.30 | 22.52 | 7.59 | 4.79 | 86.07 | 955.8 | 53.1 | 66.99 |
| Cxx-12G-yy | 0.71 | 16.73 | 6.42 | 6.94 | 79.06 | 917.1 | 50.4 | 66.99 |
| Cxx-8Gg-yy | 0.35 | 8.24 | 3.16 | 4.79 | 85.60 | 817.6 | 54.4 | 62.53 |
| Cxx-12Gg-yy | 0.67 | 10.42 | 2.82 | 6.94 | 85.29 | 707.9.1 | 51.2 | 62.53 |
| Cxx-8B-yy | 0.04 | 13.45 | 3.15 | 4.53 | 83.87 | 702.9 | 55.0 | 99.02 |
| Cxx-12B-yy | 0.61 | 10.46 | 2.12 | 5.65 | 79.20 | 1013.2 | 52.0 | 99.02 |
| Concrete | Cement Consumption (kg/m3) | Unit Mix | |||
|---|---|---|---|---|---|
| Cement | Fine Aggregate | Coarse Aggregate |
Water/Cement
Ratio | ||
| C20 | 292.78 | 1.0 | 2.9 | 3.6 | 0.66 |
| C30 | 452.52 | 1.0 | 1.6 | 2.4 | 0.47 |
| Combinations | Slump (mm) | Average Compressive Strength (MPa) | Standard Deviation | Elasticity Modulus (GPa) | Poisson’s Ratio |
|---|---|---|---|---|---|
| C20-8G | 140 | 23.2 | 0.91 | 34.2 | 0.2 |
| C30-8G | 145 | 33.3 | 0.77 | 38.5 | 0.2 |
| C20-12G | 140 | 23.2 | 0.91 | 34.2 | 0.2 |
| C30-12G | 145 | 33.3 | 0.77 | 38.5 | 0.2 |
| C20-8Gg | 140 | 20.8 | 0.95 | 32.9 | 0.2 |
| C30-8Gg | 140 | 30.3 | 0.42 | 37.3 | 0.2 |
| C20-12Gg | 130 | 23.8 | 0.17 | 34.4 | 0.2 |
| C30-12Gg | 130 | 32.4 | 0.76 | 38.2 | 0.2 |
| C20-8B | 145 | 22.6 | 0.48 | 33.9 | 0.2 |
| C30-8B | 125 | 30.8 | 0.32 | 37.5 | 0.2 |
| C20-12B | 145 | 22.6 | 0.48 | 33.9 | 0.2 |
| C30-12B | 125 | 30.8 | 0.32 | 37.5 | 0.2 |
| C30-12G-60 | 120 | 34.3 | 1.95 | 38.9 | 0.2 |
| C30-12Gg-60 | 120 | 34.3 | 1.95 | 38.9 | 0.2 |
| C30-12B-60 | 120 | 34.3 | 1.95 | 38.9 | 0.2 |
| C30-12G-120 | 120 | 33.9 | 1.61 | 38.8 | 0.2 |
| C30-12Gg-120 | 120 | 33.9 | 1.61 | 38.8 | 0.2 |
| C30-12B-120 | 120 | 33.9 | 1.61 | 38.8 | 0.2 |
| Combinations | Exposure Temperature (°C) | Average Compressive Strength (MPa) | Standard Deviation | Elasticity Modulus (GPa) | Poisson’s Ratio |
|---|---|---|---|---|---|
| C30-12G-60 | 60 | 31.7 | 2.09 | 37.9 | 0.2 |
| C30-12Gg-60 | 60 | 31.7 | 2.09 | 37.9 | 0.2 |
| C30-12B-60 | 60 | 31.7 | 2.09 | 37.9 | 0.2 |
| C30-12G-120 | 120 | 29.6 | 2.91 | 37.0 | 0.2 |
| C30-12Gg-120 | 120 | 29.6 | 2.91 | 37.0 | 0.2 |
| C30-12B-120 | 120 | 29.6 | 2.91 | 37.0 | 0.2 |
| Combinations | Specimens | (MPa) | (mm) | Rupture | (MPa) | Std Dev. (MPa) |
|---|---|---|---|---|---|---|
| GFRP bars | ||||||
| SP1 | 8.42 | 2.40 | pull-out | |||
| C20-8G | SP2 | 7.26 | 1.75 | pull-out | 7.58 | 0.73 |
| SP3 | 7.10 | 2.94 | pull-out | |||
| SP1 | 10.16 | 2.59 | pull-out | |||
| C20-12G | SP2 | 10.45 | 1.66 | pull-out | 9.88 | 0.74 |
| SP3 | 9.04 | 1.88 | pull-out | |||
| SP1 | 7.83 | 3.09 | pull-out | |||
| C30-8G | SP2 | 7.12 | 1.87 | pull-out | 6.69 | 1.41 |
| SP3 | 5.111 | 1.83 | pull-out | |||
| SP1 | 12.76 | 2.59 | pull-out | |||
| C30-12G | SP2 | 11.34 | 2.23 | pull-out | 12.71 | 1.35 |
| SP3 | 14.04 | 2.25 | pull-out | |||
| GFRPg bars | ||||||
| SP1 | 6.02 | 2.80 | pull-out | |||
| C20-8Gg | SP2 | 7.75 | 3.77 | pull-out | 6.16 | 1.53 |
| SP3 | 4.70 | 3.07 | pull-out | |||
| SP1 | 12.81 | 2.88 | pull-out | |||
| C20-12Gg | SP2 | 12.97 | 2.54 | pull-out | 12.91 | 0.09 |
| SP3 | 12.96 | 2.33 | pull-out | |||
| SP1 | 7.85 | 3.31 | pull-out | |||
| C30-8Gg | SP2 | 8.71 | 2.83 | pull-out | 9.78 | 2.64 |
| SP3 | 12.78 | 3.27 | pull-out | |||
| SP1 | 12.89 | 2.65 | pull-out | |||
| C30-12Gg | SP2 | 8.08 | 3.47 | pull-out | 9.82 | 2.67 |
| SP3 | 8.48 | 2.34 | pull-out | |||
| BFRP bars | ||||||
| SP1 | 3.50 | 4.14 | pull-out | |||
| C20-8B | SP2 | 2.54 | 3.28 | pull-out | 3.02 | 0.68 |
| SP3 * | - | - | - | |||
| SP1 | 25.79 | 3.64 | pull-out | |||
| C20-12B | SP2 | 19.33 | 2.60 | pull-out | 23.26 | 3.45 |
| SP3 | 24.66 | 2.53 | pull-out | |||
| SP1 | 5.16 | 2.69 | pull-out | |||
| C30-8B | SP2 | 6.41 | 2.39 | pull-out | 6.84 | 1.94 |
| SP3 | 8.95 | 3.96 | pull-out | |||
| SP1 | 25.99 | 2.96 | pull-out | |||
| C30-12B | SP2 | 19.91 | 2.60 | pull-out | 22.39 | 3.19 |
| SP3 | 21.27 | 2.52 | pull-out | |||
| Average Combinations | Parameters | ||||||
|---|---|---|---|---|---|---|---|
| (MPa) | (mm) | (mm) | (MPa) | (MPa) | |||
| Cxx-8G | 7.0224 | 2.26 | 0.0857 | 1.0096 | −7.1129 | 7.7062 | 0.066633 |
| Cxx-12G | 11.1963 | 2.24 | 0.10931 | 3.0007 | −4.8165 | 3.431 | 0.13681 |
| Cxx-8Gg | 7.8026 | 3.01 | 1.49521 | 4.91998 | −89.6102 | 0.978 | 0.015289 |
| Cxx-12Gg | 11.0204 | 2.6 | 1.4489 | 7.94637 | −10.8328 | 1.50158 | 0.181161 |
| Cxx-8B | 4.8111 | 3.16 | 0.188973 | 1.411561 | −170.099 | 3.1396 | 0.006711 |
| Cxx-12B | 22.6302 | 2.69 | 0.83574 | 12.20998 | −100.1095 | 1.87643 | 0.030432 |
| Combinations | Specimens | (MPa) | (mm) | Rupture | (MPa) | Standard Deviation (MPa) |
|---|---|---|---|---|---|---|
| GFRP bars | ||||||
| SP1 | 10.05 | 2.97 | pull-out | |||
| C30-12G-60 | SP2 | 11.35 | 1.93 | pull-out | 10.65 | 0.65 |
| SP3 | 10.55 | 2.05 | pull-out | |||
| SP1 | 8.59 | 2.24 | pull-out | |||
| C30-12G-120 | SP2 | 7.98 | 2.10 | pull-out | 7.87 | 0.79 |
| SP3 | 7.03 | 2.28 | pull-out | |||
| GFRPg bars | ||||||
| SP1 | 8.46 | 2.41 | pull-out | |||
| C30-12Gg-60 | SP2 | 8.32 | 3.54 | pull-out | 8.30 | 0.18 |
| SP3 | 8.11 | 2.67 | pull-out | |||
| SP1 | 6.48 | 2.95 | pull-out | |||
| C30-12Gg-120 | SP2 | 5.64 | 3.26 | pull-out | 6.32 | 0.62 |
| SP3 | 6.85 | 2.74 | pull-out | |||
| BFRP bars | ||||||
| SP1 | 23.70 | 2.53 | pull-out | |||
| C30-12B-60 | SP2 | 17.45 | 2.65 | pull-out | 20.11 | 3.23 |
| SP3 | 19.19 | 3.04 | pull-out | |||
| SP1 | 17.72 | 2.55 | pull-out | |||
| C30-12B-120 | SP2 | 13.87 | 2.73 | pull-out | 15.90 | 1.94 |
| SP3 | 16.12 | 2.63 | pull-out | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bueno, G.M.; Bittencourt, E. Study of Basalt Fibers and Graphene Enriched Polymers on Bond Behavior of FRP Bars in Concrete. Appl. Sci. 2025, 15, 5838. https://doi.org/10.3390/app15115838
Bueno GM, Bittencourt E. Study of Basalt Fibers and Graphene Enriched Polymers on Bond Behavior of FRP Bars in Concrete. Applied Sciences. 2025; 15(11):5838. https://doi.org/10.3390/app15115838
Chicago/Turabian StyleBueno, Guilherme M., and Eduardo Bittencourt. 2025. "Study of Basalt Fibers and Graphene Enriched Polymers on Bond Behavior of FRP Bars in Concrete" Applied Sciences 15, no. 11: 5838. https://doi.org/10.3390/app15115838
APA StyleBueno, G. M., & Bittencourt, E. (2025). Study of Basalt Fibers and Graphene Enriched Polymers on Bond Behavior of FRP Bars in Concrete. Applied Sciences, 15(11), 5838. https://doi.org/10.3390/app15115838





