The Effect of Nodal Deviation on the Reliability Performance of the Optimized Free-Form Single-Layer Reticulated Shell
Abstract
Featured Application
Abstract
1. Introduction
2. Formulation of the Shape Optimization Problem
2.1. Shape Optimization Under Static Loads
2.2. Shape Expression
2.3. Probability Density Evolution Method
- The value range of the basic random parameter is divided, and the qth region is set as . The probability of assigning each part is calculated as . In each divided unit, it is defined as follows:Then, Equation (7) will become a series of new equations as follows:
- Select representative points within each . Solve the physical equations deterministically at these points to obtain , where and is the time step for solving physical equations.
- Substitute the physical solutions into Equation (8) with initial conditions and solve numerically. The joint PDF is computed at discrete points ( is the discrete step of y) and ( is the time step for the probability density evolution equation), and ∆τ is usually smaller than .
- Integrate the probability density solution obtained in the previous step with respect to to obtain the probability density solution of the system response y.
3. Numerical Examples
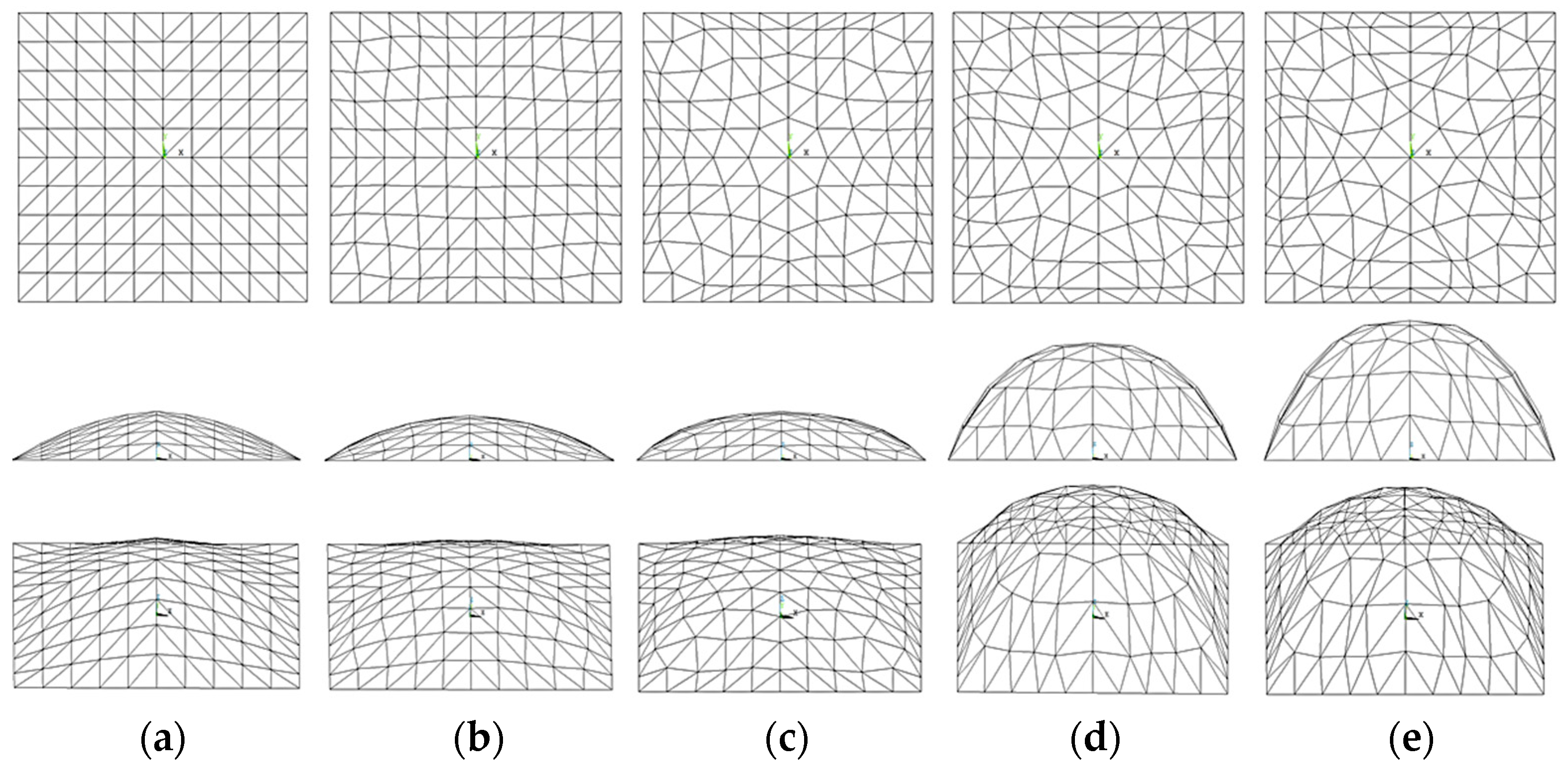
3.1. Shape Optimization
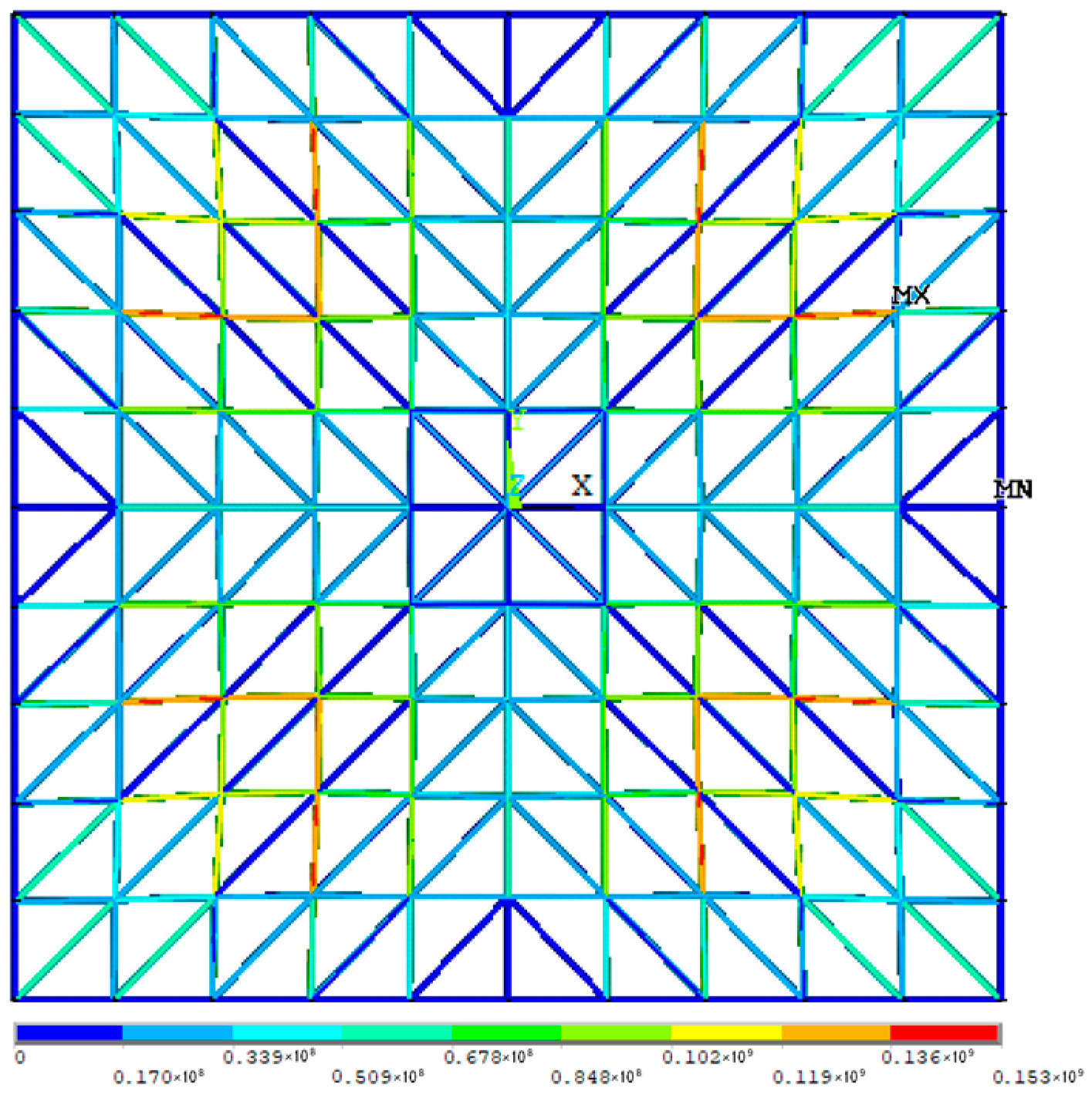
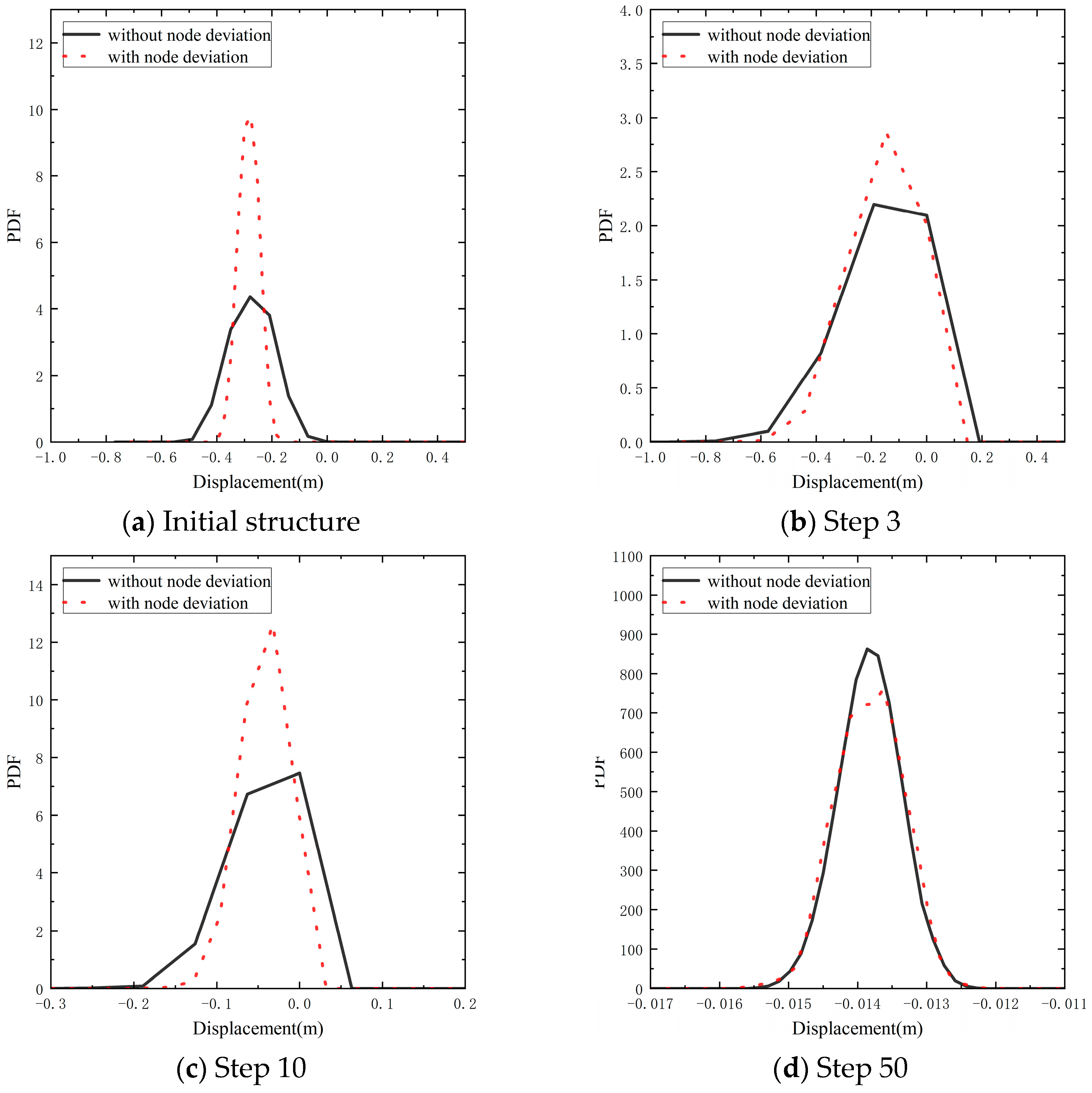
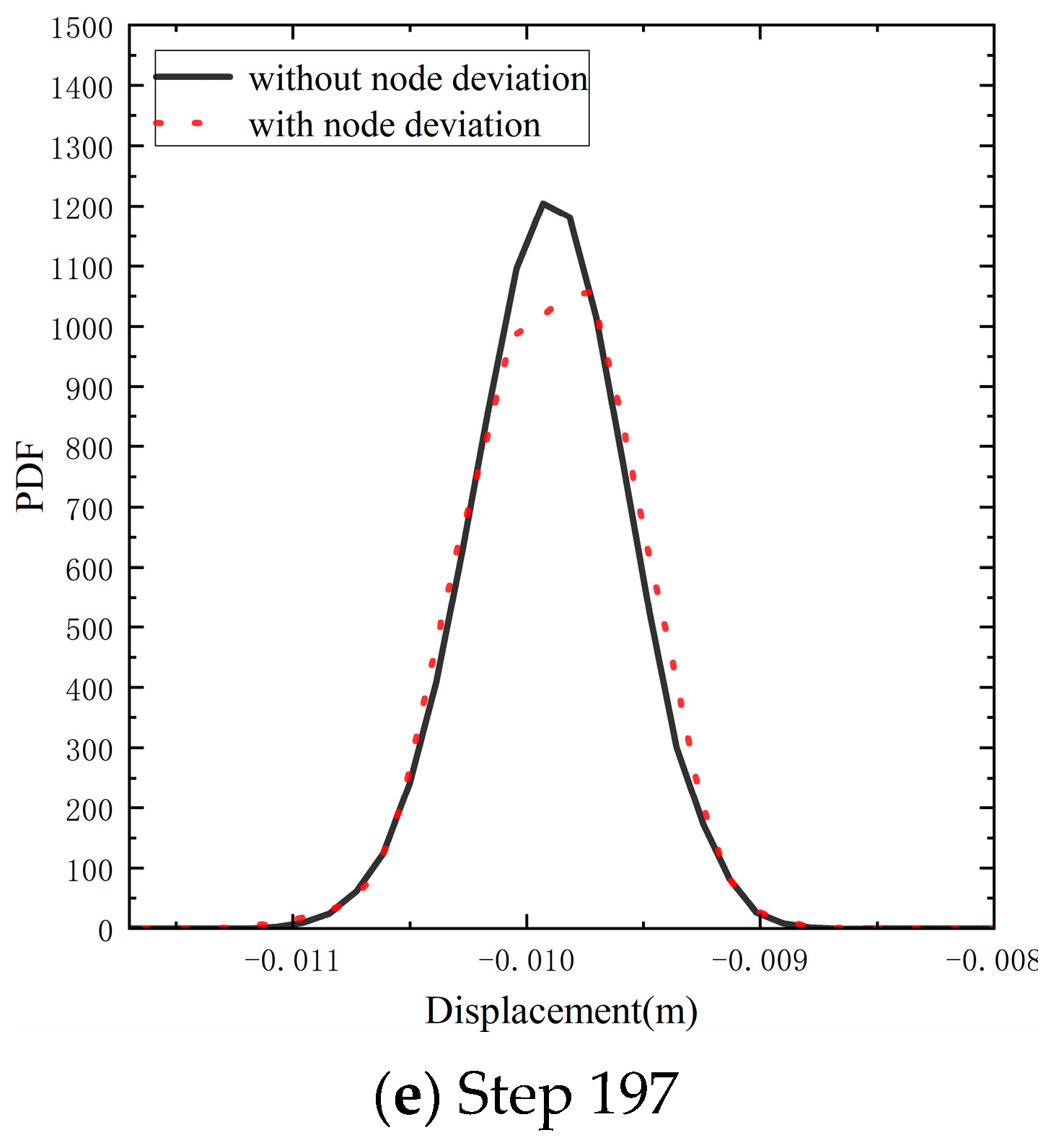
3.2. Influence of Node Deviations on the Reliability Performance of Optimized Structures
- Initial structure (without nodal deviations):Expected displacement: −2.73 × 10−2 m (upward direction defined as positive);Variance: 6.68 × 10−3 m2.Initial structure (with nodal deviations):Expected displacement: −2.84 × 10−2 m;Variance: 1.42 × 10−3 m2;Relative differences: 4.05% in expectation and 78.69% in variance.
- Optimized structure (without nodal deviations):Expected displacement: −9.90 × 10−3 m;Variance: 1.09 × 10−7 m2.Initial structure (with nodal deviations):Expected displacement: −9.89 × 10−3 m;Variance: 1.22 × 10−7 m2;Relative differences: 0.11% in expectation and 11.76% in variance.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Probability Density Function |
References
- Cui, C.; Wang, Y.; Jiang, B.; Cui, G. Research on the Morphological Creation Method of Free-Form Single-Layer Lattice Shell Structure. China Civ. Eng. J. 2013, 46, 57–63. [Google Scholar]
- Li, X.; Wu, Y.; Cui, C. NURBS-GM Method for Free-Form Surface Structure Creation. China Civ. Eng. J. 2011, 44, 60–66. [Google Scholar]
- Wu, Y.; Li, Q.; Shen, S. Numerical Creation Method of Spatial Structure Morphology Based on Inverted Hanging Experiment Principle. J. Build. Struct. 2014, 35, 41–48. [Google Scholar]
- Ding, C.; Seifi, H.; Dong, S.; Xie, Y.M. A New Node-Shifting Method for Shape Optimization of Reticulated Spatial Structures. Eng. Struct. 2017, 152, 727–735. [Google Scholar] [CrossRef]
- Falco, S.; Afonso, S.; Vaz, L. Analysis and optimal design of plates and shells under dynamic loads—II: Optimization. Struct. Multidisc. Optim. 2004, 27, 197–209. [Google Scholar] [CrossRef]
- Shimoda, M.; Okada, T.; Nagano, T.; Shi, J.X. Free-form optimization method for buckling of shell structures under out-of-plane and in-plane shape variations. Struct. Multidisc. Optim. 2016, 54, 275–288. [Google Scholar] [CrossRef]
- Shi, K.; Lin, J.; Jiang, Z.; Qu, T. Shape Optimization Method of Free-Form Surface Spatial Grid Structure Based on Plant Growth Simulation Algorithm. J. Build. Struct. 2022, 43, 276–289. [Google Scholar]
- Yang, J.-S.; Chen, J.-B.; Beer, M. Seismic Topology Optimization Considering First-Passage Probability by Incorporating Probability Density Evolution Method and Bi-Directional Evolutionary Structural Optimization. Eng. Struct. 2024, 314, 118382. [Google Scholar] [CrossRef]
- Hu, Q.; Li, Q.; Wu, Y. Topology Optimization Method of Single-Layer Lattice Shell Structure Based on Unit Coding. Spat. Struct. 2020, 26, 19–26. [Google Scholar]
- Jiang, B.; Cui, C. Structural Topology Optimization Method Considering Both Static and Dynamic Loads. Chin. J. Comput. Mech. 2023, 40, 7–13. [Google Scholar]
- Jiang, B.; Zhang, J.; Ohsaki, M. Shape Optimization of Free-Form Shell Structures Combining Static and Dynamic Behaviors. Structures 2021, 29, 1791–1807. [Google Scholar] [CrossRef]
- Liu, F.; Feng, R. Study on Morphological Optimization of Single-Layer Space Grid Structure Considering the Influence of Structural Defect Sensitivity. J. Build. Struct. 2018, 39, 25–32. [Google Scholar]
- Piegl, L.; Tiller, W. The NURBS Book; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; ISBN 978-3-642-59223-2. [Google Scholar]
- Li, J.; Chen, J. Stochastic Dynamics of Structures; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Li, J.; Chen, J. The Principle of Preservation of Probability and the Generalized Density Evolution Equation. Struct. Saf. 2008, 30, 65–77. [Google Scholar] [CrossRef]
- Li, J. Fundamental of Structural Reliability Analysis; China Science Publishing & Media Ltd.: Beijing, China, 2021; ISBN 978-7-03-068868-2. [Google Scholar]
- Jia-Shu, Y. Tree-Yang/Process_Oriented_PDEM. Available online: https://github.com/Tree-Yang/Process_Oriented_PDEM (accessed on 9 October 2024).
- Li, J.; Chen, J. Probability Density Evolution Method for Dynamic Response Analysis of Structures with Uncertain Parameters. Comput. Mech. 2004, 34, 400–409. [Google Scholar] [CrossRef]
- Chen, J.; Yang, J.; Jensen, H. Structural Optimization Considering Dynamic Reliability Constraints via Probability Density Evolution Method and Change of Probability Measure. Struct. Multidiscip. Optim. 2020, 62, 2499–2516. [Google Scholar] [CrossRef]
- Yang, J.; Chen, J.; Jensen, H. Structural Design Optimization under Dynamic Reliability Constraints Based on the Probability Density Evolution Method and Highly-Efficient Sensitivity Analysis. Probabilistic Eng. Mech. 2022, 68, 103205. [Google Scholar] [CrossRef]
- Yang, J.; Jensen, H.; Chen, J. Structural Optimization under Dynamic Reliability Constraints Utilizing Probability Density Evolution Method and Metamodels in Augmented Input Space. Struct. Multidiscip. Optim. 2022, 65, 107. [Google Scholar] [CrossRef]
- GB 50011-2010 (2016); Code for Seismic Design of Buildings. China Architecture Building Press: Beijing, China, 2016. (In Chinese)
- Dai, G.; Li, L.; Xia, Z.; Huang, Y. Statistics and Analysis of New Material Parameters of Building Structural Steel. Build. Struct. 2000, 30, 31–32. [Google Scholar]
- Vrouwenvelder, T. The JCSS Probabilistic Model Code. Struct. Saf. 1997, 19, 245–251. [Google Scholar] [CrossRef]


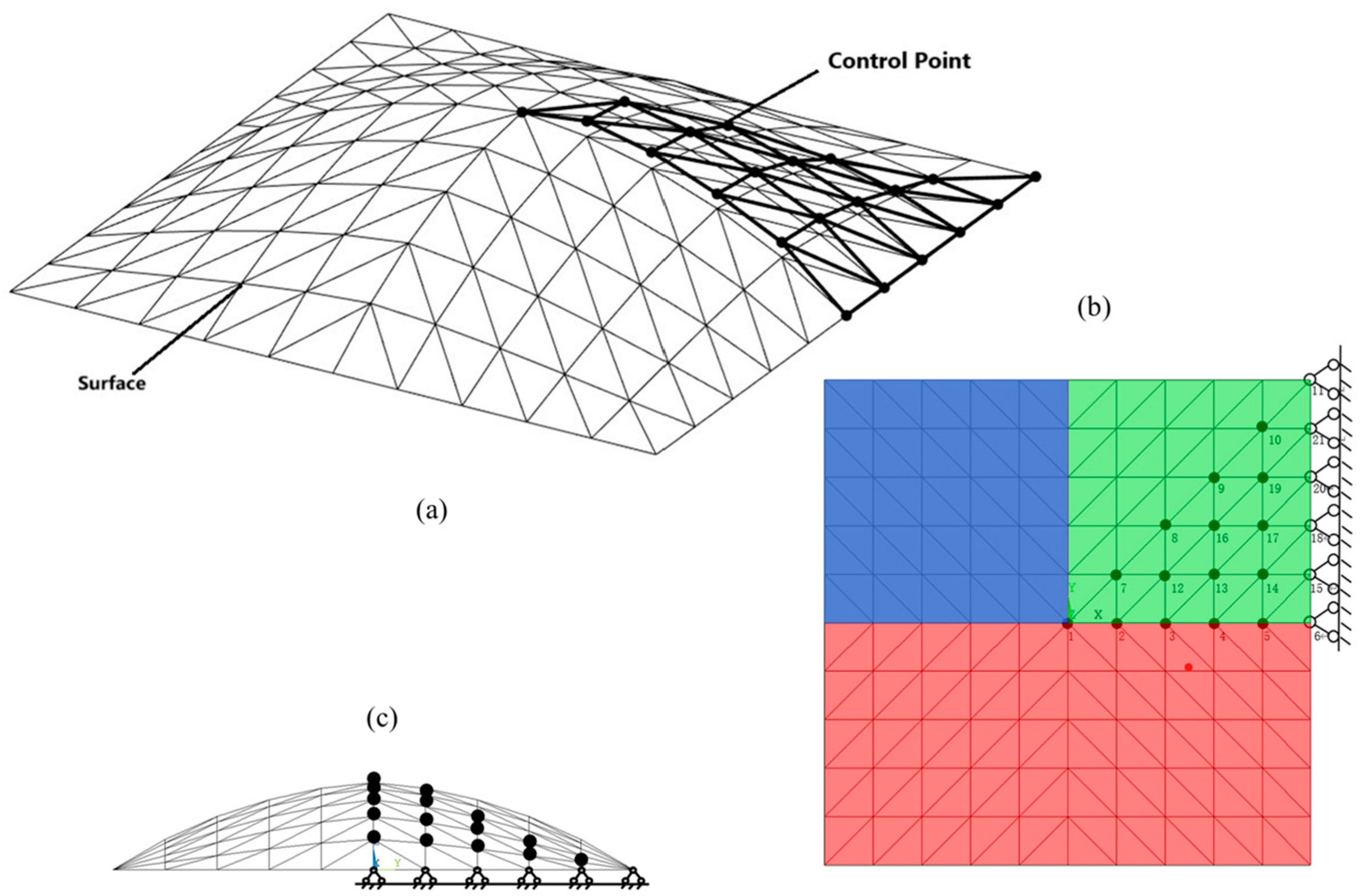

| Point Number | x | y | z | Point Number | x | y | z |
|---|---|---|---|---|---|---|---|
| 1 | 0.00 | 0.00 | 5.00 | 12 | 6.00 | 3.00 | 3.99 |
| 2 | 3.00 | 0.00 | 4.82 | 13 | 9.00 | 3.00 | 3.10 |
| 3 | 6.00 | 0.00 | 4.27 | 14 | 12.00 | 3.00 | 1.80 |
| 4 | 9.00 | 0.00 | 3.32 | 15 | 15.00 | 3.00 | 0.00 |
| 5 | 12.00 | 0.00 | 1.93 | 16 | 9.00 | 6.00 | 2.47 |
| 6 | 15.00 | 0.00 | 0.00 | 17 | 12.00 | 6.00 | 1.42 |
| 7 | 3.00 | 3.00 | 4.51 | 18 | 15.00 | 6.00 | 0.00 |
| 8 | 6.00 | 6.00 | 3.19 | 19 | 12.00 | 9.00 | 0.87 |
| 9 | 9.00 | 9.00 | 1.52 | 20 | 15.00 | 9.00 | 0.00 |
| 10 | 12.00 | 12.00 | 0.30 | 21 | 15.00 | 12.00 | 0.00 |
| 11 | 15.00 | 15.00 | 0.00 |
| Iterations | 0 | 3 | 10 | 50 | 197 |
|---|---|---|---|---|---|
| Relative Strain Energy 1 | 100.00% | 37.09% | 23.19% | 7.66% | 6.94% |
| Relative Volume 2 | 100.00% | 99.56% | 99.24% | 119.07% | 126.24% |
| Structural Height (m) | 5 | 4.336 | 4.994 | 12.055 | 14.401 |
| Random Variable | Probability Distribution Model | Source | Mean | Coefficient of Variation |
|---|---|---|---|---|
| Yield Strength of Material (MPa) | Lognormal | Article [23] | 210 | 0.081 |
| Elastic Modulus (×105 Mpa) | Normal | JCSS [24] | 2.1 | 0.03 |
| Random Variable | Probability Distribution Model | Source | Mean | Coefficient of Variation |
|---|---|---|---|---|
| Yield Strength of Material (MPa) | Lognormal | Article [23] | 210 | 0.081 |
| Elastic Modulus (×105 Mpa) | Normal | JCSS [24] | 2.1 | 0.03 |
| Node deviations (m) | Normal | JCSS [24] | 0 | 1/1000 L 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; Jiang, B. The Effect of Nodal Deviation on the Reliability Performance of the Optimized Free-Form Single-Layer Reticulated Shell. Appl. Sci. 2025, 15, 5379. https://doi.org/10.3390/app15105379
Li D, Jiang B. The Effect of Nodal Deviation on the Reliability Performance of the Optimized Free-Form Single-Layer Reticulated Shell. Applied Sciences. 2025; 15(10):5379. https://doi.org/10.3390/app15105379
Chicago/Turabian StyleLi, Dong, and Baoshi Jiang. 2025. "The Effect of Nodal Deviation on the Reliability Performance of the Optimized Free-Form Single-Layer Reticulated Shell" Applied Sciences 15, no. 10: 5379. https://doi.org/10.3390/app15105379
APA StyleLi, D., & Jiang, B. (2025). The Effect of Nodal Deviation on the Reliability Performance of the Optimized Free-Form Single-Layer Reticulated Shell. Applied Sciences, 15(10), 5379. https://doi.org/10.3390/app15105379






