Experimental Study on the Uplift Bearing Capacity of Pre-Drilled Planted Piles in Isolated Stone Strata Based on Transparent Soil Technology
Abstract
1. Introduction
2. Design of the Planted Pile Foundation Model Test Based on Transparent Soil
2.1. Planted Pile Foundation Model Test Apparatus
2.2. Preparation of Transparent Soil and Planted Pile Foundation Model
2.3. Experimental Conditions for the Planted Pile Model
3. The Numerical Model for Planted Pile
3.1. Discrete-Continuous Coupled Three-Dimensional Numerical Calculation Method
3.2. Constitutive Model and Material Parameters
4. Results
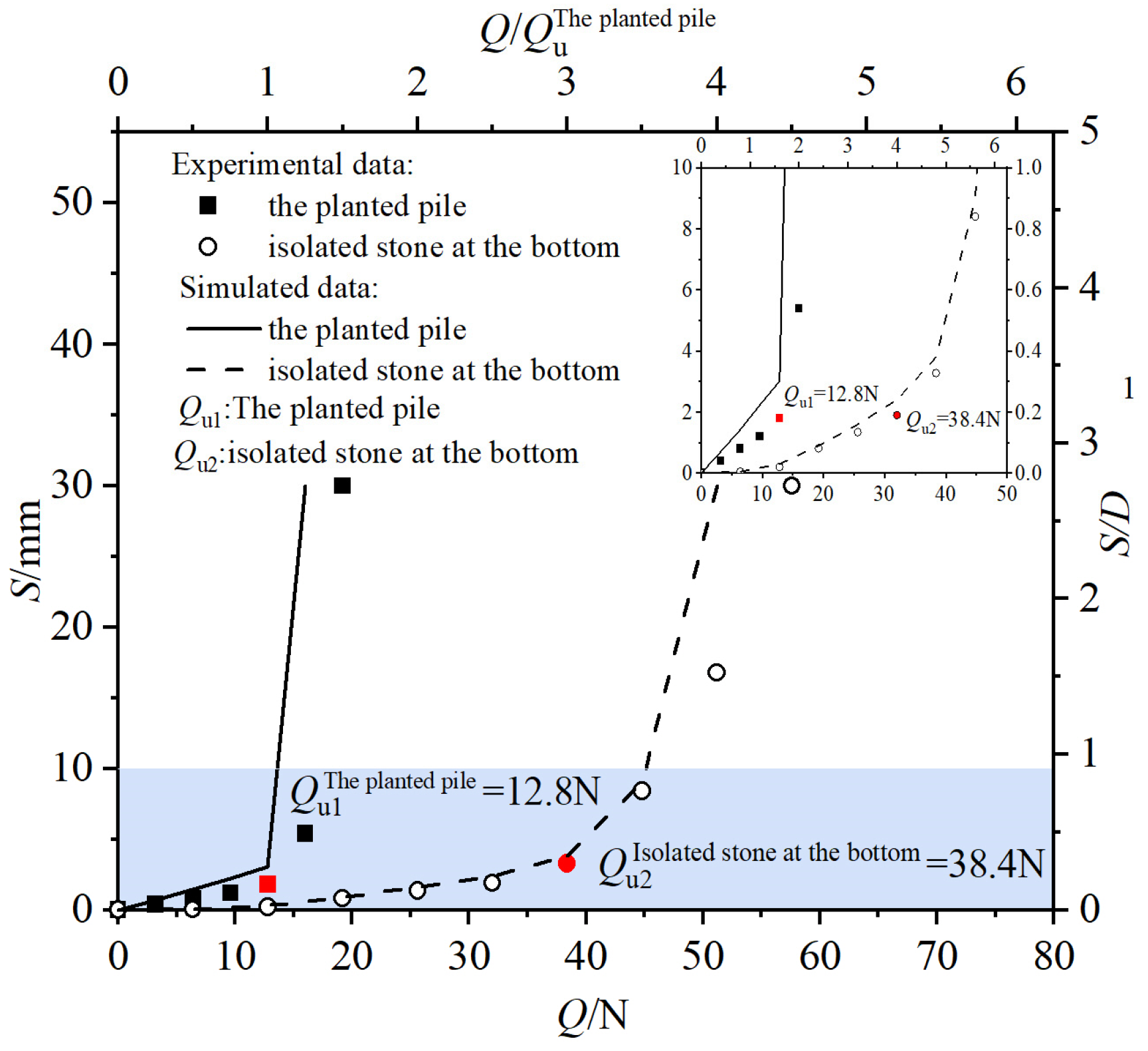
4.1. Q-S Curve Analysis
4.2. Analysis of Axial Force Transfer in the Pile Body
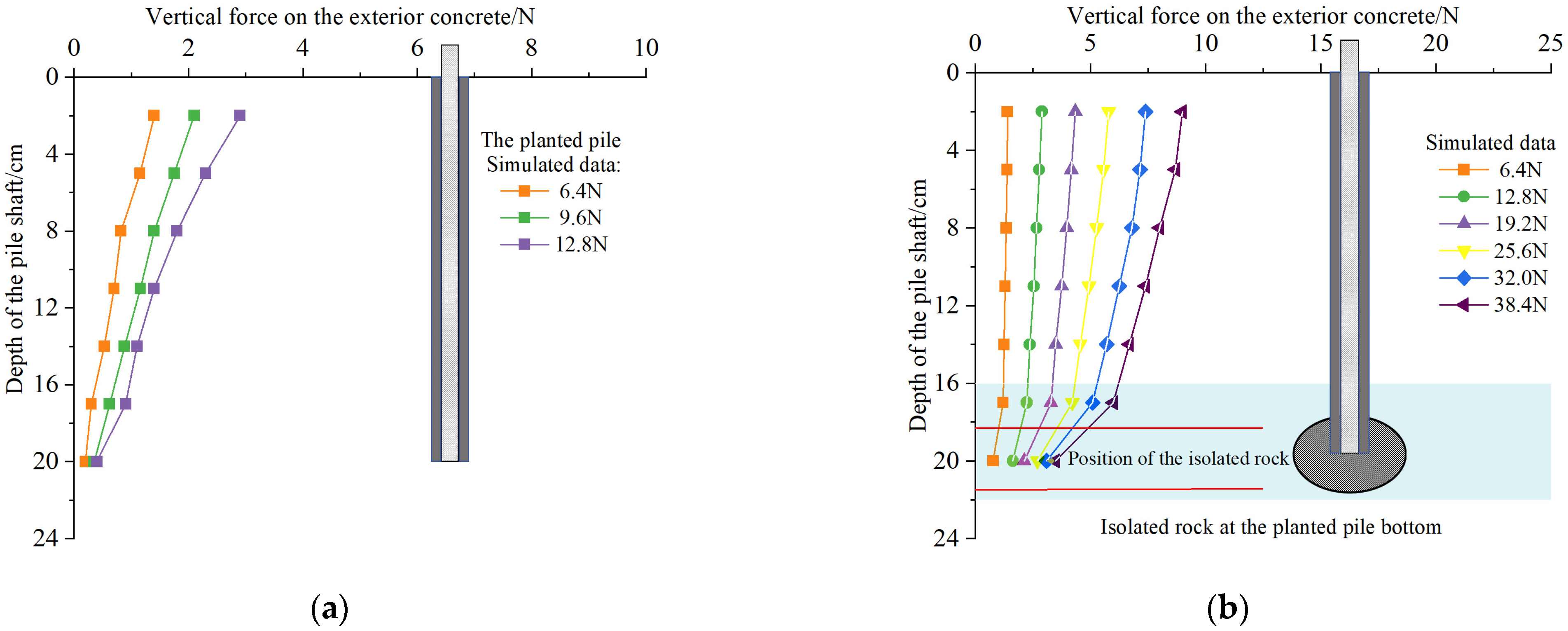
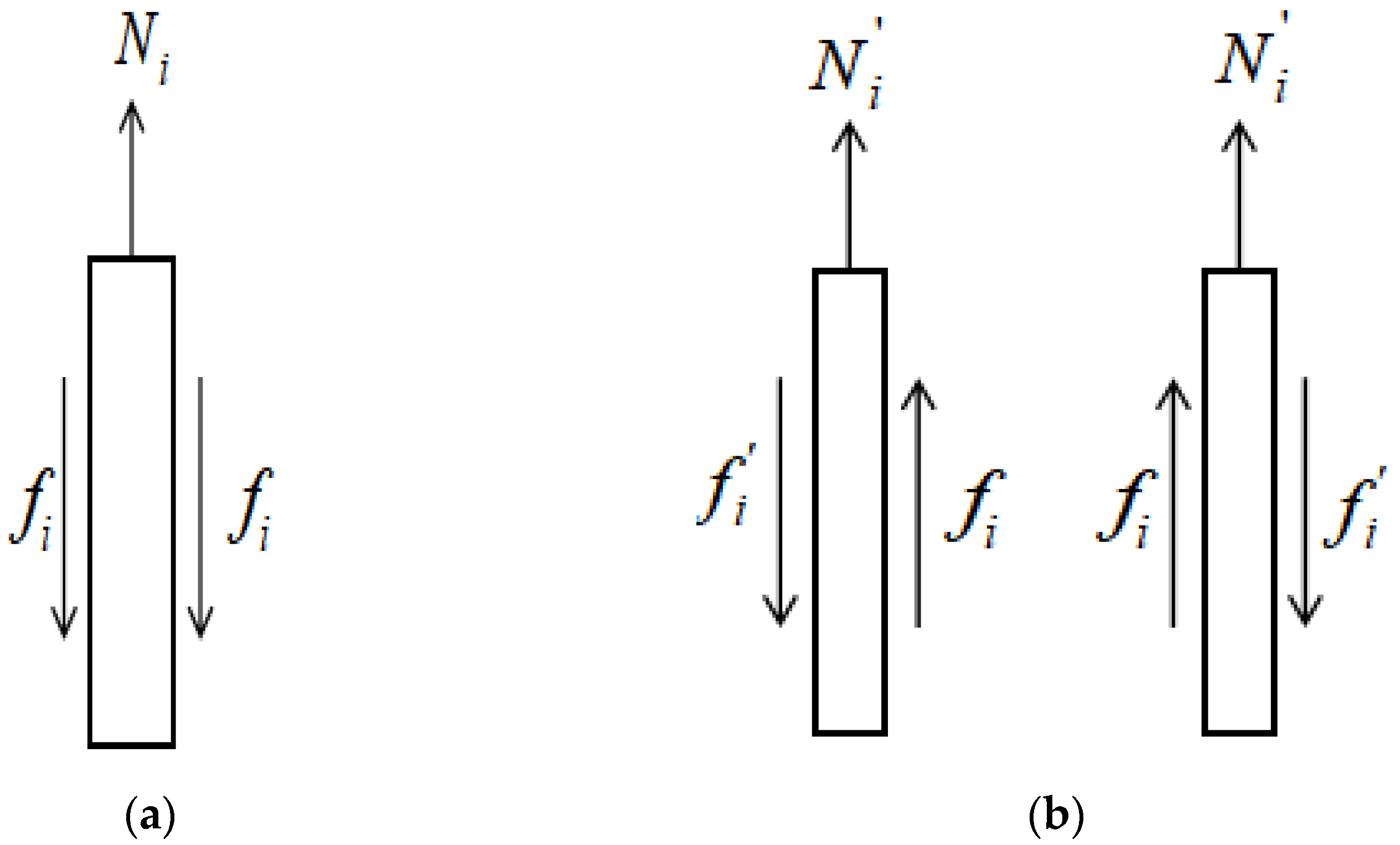
4.3. Analysis of Vertical Forces in the Outer Concrete
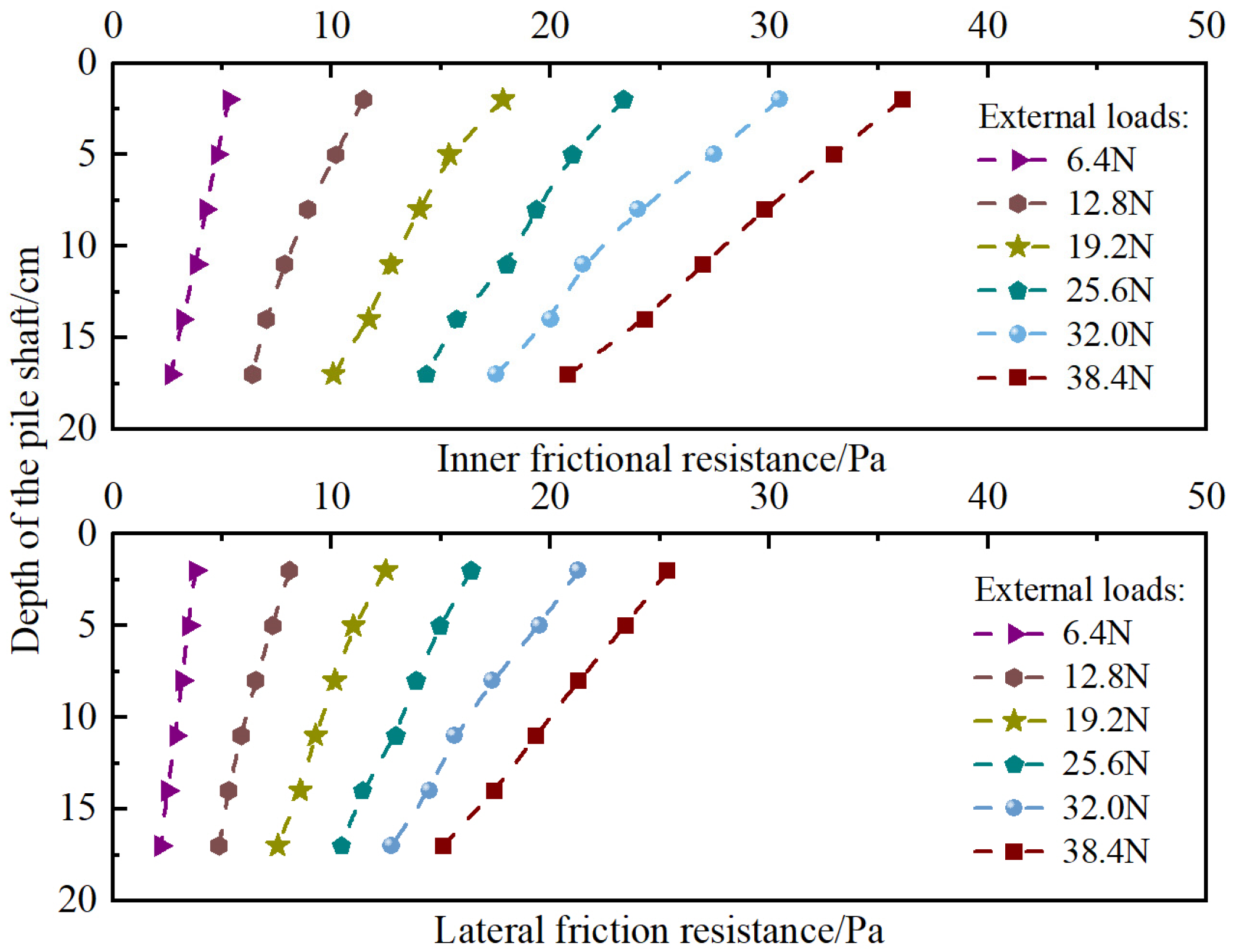
4.4. Analysis of Lateral Friction Resistance of the Pile Body
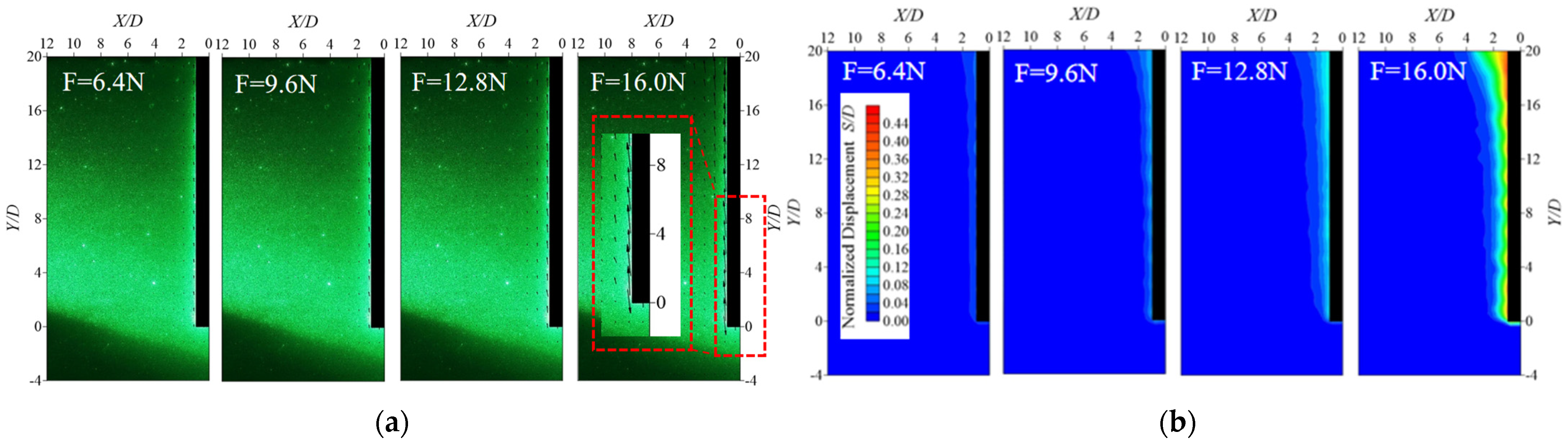
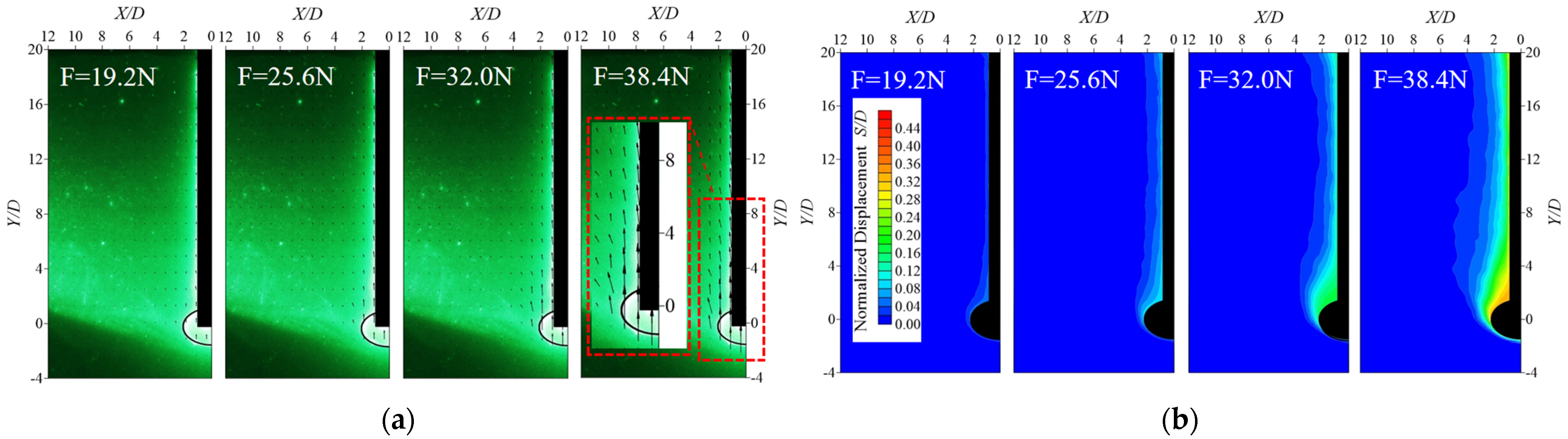
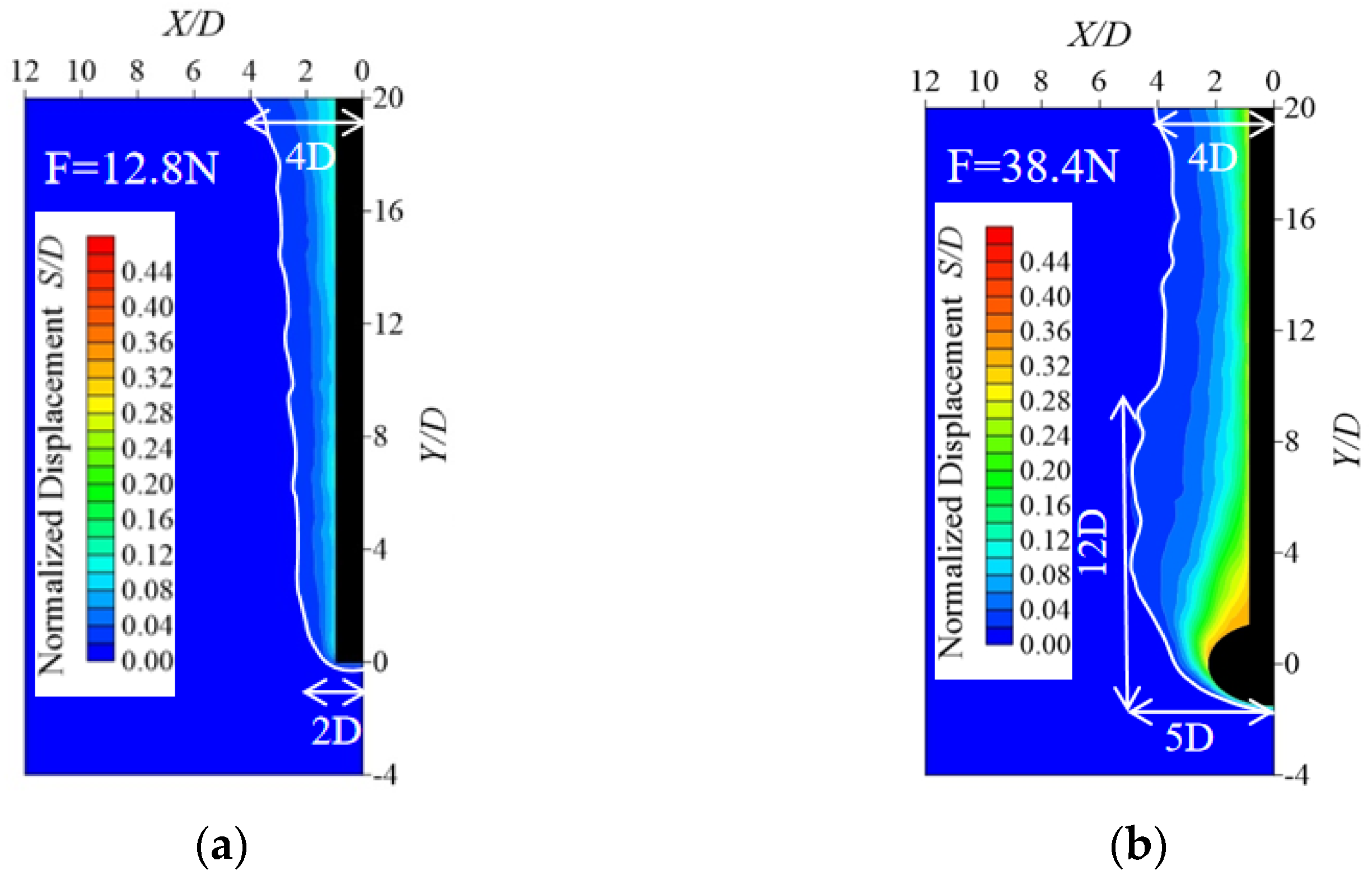
4.5. Analysis of Soil Displacement Field
5. Conclusions
- (1)
- The upper load on the planted piles is mainly borne by the high-strength prestressed pipe core piles, which not only transmit the load downwards, but also disperse it through the concrete surrounding the pipes into the surrounding soil. In areas where isolated stone is present, the load diffuses from the outer concrete layer to the isolated stone and then into the surrounding soil, creating a three-layered diffusion pattern. This effectively transmits the upper load to a larger affected area than that of planted piles without isolated stone, resulting in superior bearing capacity and doubling the ultimate pullout bearing capacity of the test piles.
- (2)
- The deformation of the planted piles is primarily controlled by the core piles, with the core and outer concrete layers deforming in a coordinated manner. The axial force in the core pile decreases with depth, and the vertical force in the outer concrete layer also decreases with depth. In planted piles with an isolated stone at the end, the axial force of the core pile and the vertical force in the outer concrete layer show a sudden decrease in the isolated stone region due to the larger contact area formed with the surrounding soil, which enhances the lateral frictional resistance in that area.
- (3)
- The distribution patterns of internal and external friction forces along the pile stem decrease gradually. The internal friction of the planted pile is approximately 1.47 times that of the external friction and about 0.8 times the ratio of the diameters of the planted pile and the core pile. The internal friction of planted piles with an isolated stone at the end is approximately 1.37 times that of the external friction.
- (4)
- The presence of the isolated stone enhances the soil-bearing capacity and also expands the area of stress distribution around the pile. Under ultimate loading conditions, the displacement field of the surrounding soil for planted piles without isolated stones is distributed in an “inverted triangle,” with a maximum width of 4.0 D at the pile top and about 2.0 D at the pile end. For planted piles with an isolated stone at the end, the displacement field of the surrounding soil exhibits a trapezoidal distribution that is wider at the bottom and narrower at the top, with the influenced area reaching 12.0 D vertically and 5.0 D horizontally at the pile end, and the maximum impact width of the soil at the pile top measuring 4.0 D.
- (5)
- Overall, the presence of isolated stones at the base of the pile can provide additional support, enhancing the load transfer pathways and the influence range of the surrounding soil. This indicates that the incorporation of isolated stones into the load-bearing system of the pile can not only mitigate the effects of the stones on the pile foundation, but also effectively enhance the overall load-bearing capacity of the pile.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Doi, T.; Murono, Y.; Zhang, F.; Hirayama, Y. In-situ horizontal cyclic loading tests on composite foundation composed of soilbags and piles. Soils Found. 2023, 63, 101327. [Google Scholar] [CrossRef]
- Li, C.; Lu, X.; Wang, P. An analytical solution for the consolidation of a composite foundation reinforced by vertical drains and high replacement ratio gravel piles by considering the radial flow within gravel piles. Soils Found. 2023, 63, 101393. [Google Scholar] [CrossRef]
- He, Z.; Ye, A.; Wang, X. Local scour effects on seismic behavior of pile-group foundations in cohesionless soils: Insights from quasi-static tests. Ocean. Eng. 2024, 311, 118886. [Google Scholar] [CrossRef]
- Honda, T.; Hirai, Y.; Sato, E. Uplift capacity of belled and multi-belled piles in dense sand. Soils Found. 2011, 51, 483–496. [Google Scholar] [CrossRef]
- Karkee, M.B.; Kanai, S.; Horiguchi, T. Quality Assurance in bored PHC nodular piles through control of design capacity based on loading test data. In Proceedings of the 7th International Conference and Exhibition, Piling and Deep Foundations, Vienna, Austria, 15–17 June 1998. [Google Scholar]
- Ping, Y.; Li, Q. Study on the construction technology and load-bearing behavior of composite piles with rigid core embedded in hard bearing layer through strong mixing soil. Chin. J. Geotech. Eng. 2013, 35, 1033–1036. [Google Scholar]
- Lou, Y.; Fang, P.; Xie, X.; Zhang, R.; Chong, C.S.; Wang, Z.; Zhu, D. Experimental study on thermo-mechanical responses of pre-bored grouted planted piles with different restraint conditions. Energy Build. 2022, 268, 112232. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, Z.; Mao, Y.; Zhao, W. Studies on applications of precast pile planting method. In Smart Geotechnics for Smart Societies; CRC Press: Boca Raton, FL, USA, 2023. [Google Scholar]
- Aizebeokhai, A.P.; Olayinka, A.I.; Singh, V.S. Application of 2D and 3D geoelectrical resistivity imaging for engineering site investigation in a crystalline basement terrain, southwestern Nigeria. Environ. Earth Sci. 2010, 61, 1481–1492. [Google Scholar] [CrossRef]
- Hasan, M.; Shang, Y.; Meng, H.; Yi, X.; Shao, P. A novel approach to determine rock mechanical parameters using non-invasive geophysical methods. Int. J. Rock Mech. Min. Sci. 2022, 160, 105260. [Google Scholar] [CrossRef]
- Li, B.; Wu, L.; Xu, C.; Zuo, Q.; Wu, S.; Zhu, B. The detection of the ns in metro tunneling in granite strata using a shield tunneling method and a new method of coping with boulders. Geotech. Geol. Eng. 2016, 34, 1155–1169. [Google Scholar] [CrossRef]
- Lv, Y.; Liu, H.; Ding, X.; Kong, G. Field tests on bearing characteristics of X-Section pile composite foundation. J. Perform. Constr. Facil. 2012, 26, 180–189. [Google Scholar] [CrossRef]
- Ma, T.; Zhu, Y.; Yang, X. Calculation of bearing capacity and deformation of composite pile foundation with long and short piles in loess areas. Adv. Civ. Eng. 2020, 2020, 8829779. [Google Scholar] [CrossRef]
- Chen, Q.; Zhao, M.; Zhou, G.; Zhang, Z. Bearing capacity and mechanical behavior of CFG pile composite foundation. J. Cent. South Univ. Technol. 2008, 15 (Suppl. S2), 45–49. [Google Scholar] [CrossRef]
- Guades, E.; Aravinthan, T.; Islam, M.; Manalo, A. A review on the driving performance of FRP composite piles. Compos. Struct. 2012, 94, 1932–1942. [Google Scholar] [CrossRef]
- Li, H.L.; Ren, L.W.; Zheng, H.; Xiao, Y.Z. Study on the load transfer mechanism of high jet grouting and insert core composite piles through full-scale model tests. Rock Soil Mech. 2010, 31, 1395–1401. [Google Scholar]
- Li, J.C.; Zhang, Y.G.; Deng, Y.G.; Hua, X.L. Study on the load transfer mechanism of composite piles composed of pipe piles and cement-soil. J. Rock Mech. Eng. 2014, 33, 3068–3076. [Google Scholar]
- Gao, S.; Chen, C.; Jiang, X.; Zhu, S.; Cai, H.; Li, W. Transformer-based settlement prediction model of pile composite foundation under embankment loading. Comput. Geotech. 2024, 176, 106783. [Google Scholar] [CrossRef]
- Shao, W.; Sun, Q.; Xu, X.; Yue, W.; Shi, D. Durability life prediction and horizontal bearing characteristics of CFRP composite piles in marine environments. Constr. Build. Mater. 2023, 367, 130116. [Google Scholar] [CrossRef]
- Kobayashi, K.; Ogura, H. Vertical bearing capacity of bored pre-cast pile with enlarged base considering diameter of the enlarged excavation around pile toe. In Advances in Deep Foundations; CRC Press: Boca Raton, FL, USA, 2007; pp. 277–283. [Google Scholar]
- Yu, X.; Wang, L.; Xu, Y.; Huang, J.; Zhang, H. Consolidation of unsaturated composite foundation reinforced by T-shaped deep cement mixing piles. Comput. Geotech. 2024, 175, 106656. [Google Scholar] [CrossRef]
- Voottipruex, P.; Suksawat, T.; Bergado, D.T.; Jamsawang, P. Numerical simulations and parametric study of SDCM and DCM piles under full scale axial and lateral loads. Comput. Geotech. 2011, 38, 318–329. [Google Scholar] [CrossRef]
- Jamsawang, P.; Bergado, D.T.; Voottipruex, P. Field behaviour of stiffened deep cement mixing piles. Ground Improv. 2011, 164, 33–49. [Google Scholar] [CrossRef]
- Jiajin, Z.; Wang, K.; Gong, X.; Zhang, R. Study on the bearing capacity and load transfer mechanism of static drill rooted nodular piles. Rock Soil Mech. 2014, 14, 1367–1376. [Google Scholar]
- Schafer, M.; Madabhushi, S.P.G. Uplift Resistance of Enlarged Base Pile Foundations. Indian Geotech. Soc. 2019, 50, 426–441. [Google Scholar] [CrossRef]
- Yang, Y.S.; Qiu, L.C. MPM simulation of uplift resistance of enlarged base piles in sand. Soils Found. 2020, 60, 1322–1330. [Google Scholar] [CrossRef]
- Zhaohu, C.; Wang, L.; Zhang, J.; Jia, H.; Pan, J. Model test study on soil displacement field during pile driving process based on PIV technology. Eng. Mech. 2014, 31, 168–174. [Google Scholar]
- Junjie, Y. Similarity Theory and Structural Model Testing; Wuhan University of Technology Press: Wuhan, China, 2005. [Google Scholar]
- Jiang, M.J.; Konrad, J.M.; Leroueil, S. An efficient technique for generating homogeneous specimens for DEM studies. Comput. Geotech. 2003, 30, 579–597. [Google Scholar] [CrossRef]
- Liu, R.; Liang, C. Study of the Bearing Capacity at the Variable Cross-Section of a Riser-Surface Casing Composite Pile. China Ocean. Eng. 2021, 35, 262–271. [Google Scholar] [CrossRef]
- Jiajin, Z.; Gong, X.; Zhang, R.; El Naggar, M.H.; Wang, K. Field behavior of pre-bored grouted planted nodular pile embedded in deep clayey soil. Acta Geotech. 2020, 15, 1847–1857. [Google Scholar]
- Jiajin, Z.; Yu, J.; Gong, X.; El Naggar, M.H.; Zhang, R. The effect of cemented soil strength on the frictional capacity of precast concrete pile-cemented soil interface. Acta Geotech. 2020, 15, 3271–3282. [Google Scholar]
- Wang, W.; Wu, J.; Wang, X. Analysis of the uplift behavior and deformation characteristics of enlarged base piles based on ultimate load Tests. Chin. J. Geotech. Eng. 2016, 38, 1330–1338. [Google Scholar]
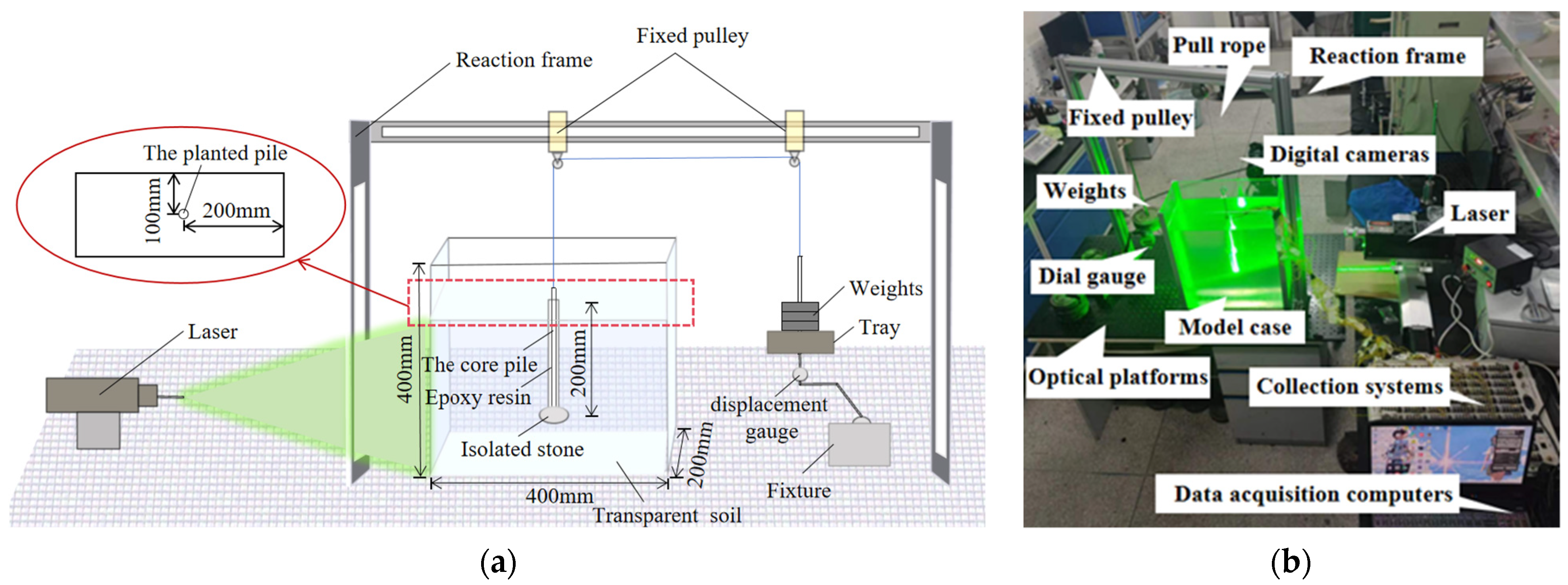


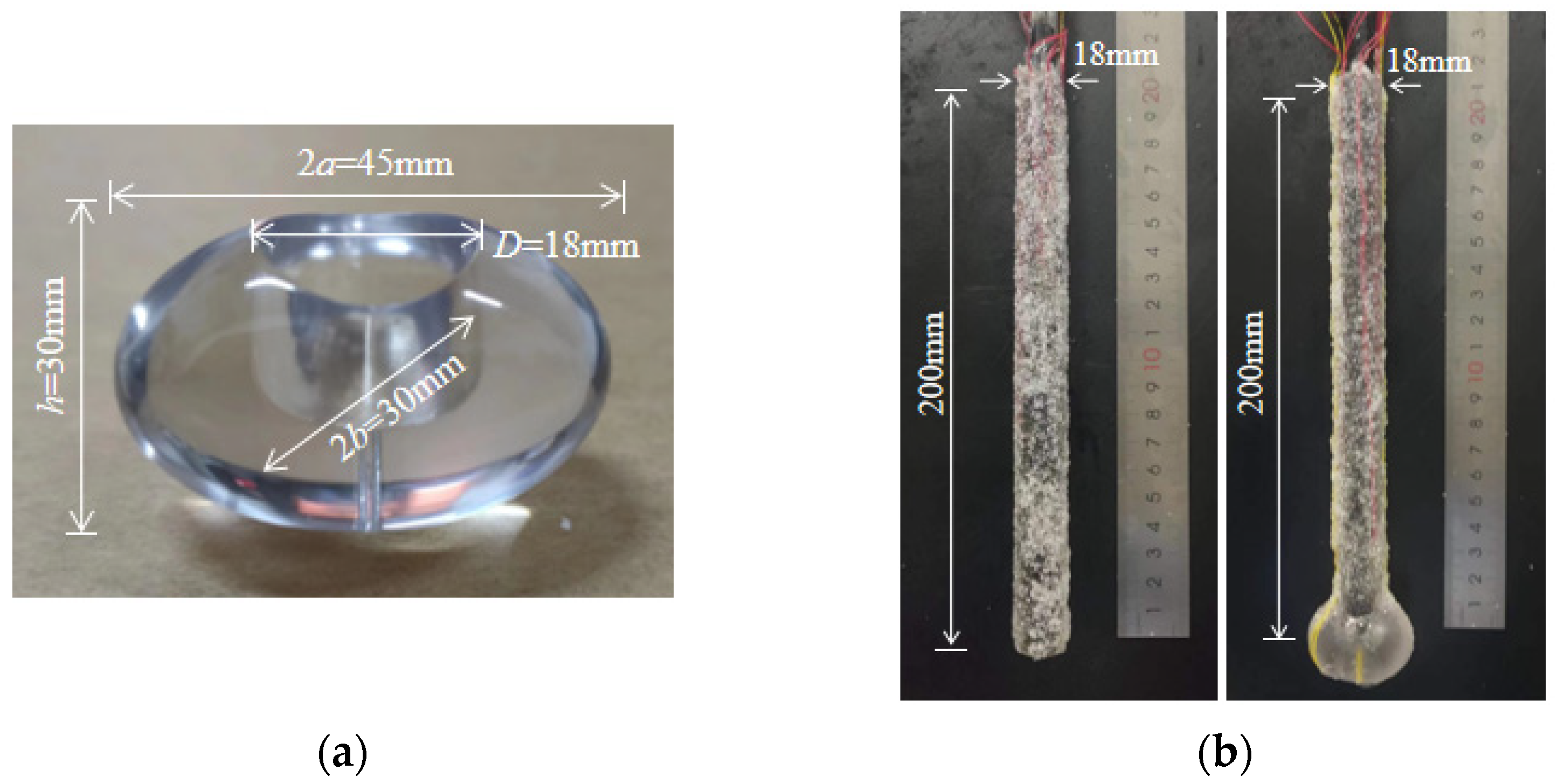
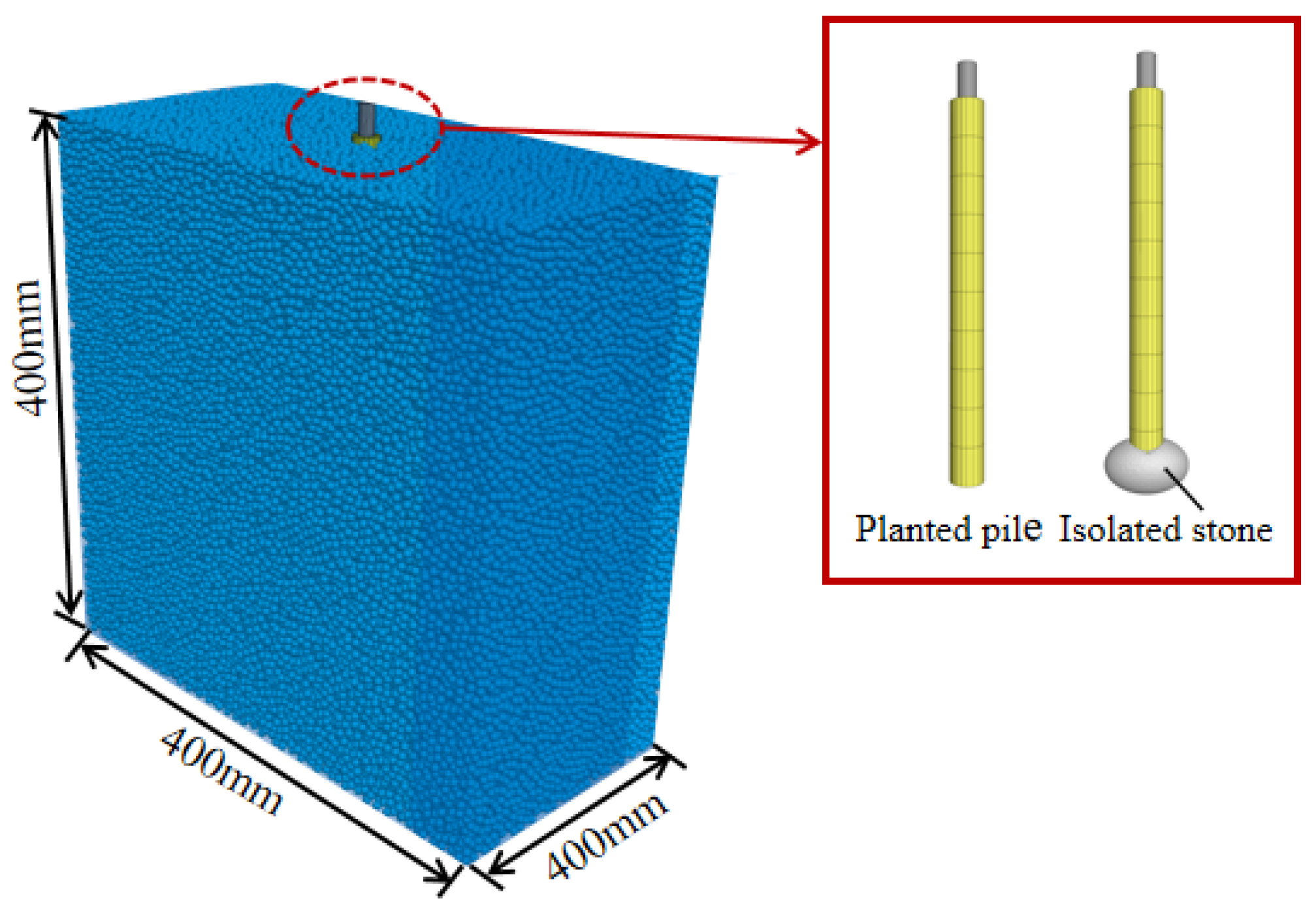


| Experiment Number | Pile Type | Isolated Stone | Isolated Stone Location |
|---|---|---|---|
| 1 | Planted pile | No | - |
| 2 | Planted pile | Yes | Pile bottom |
| Name | Unit | Effective Modulus E/MPa | Stiffness Ratio | Friction Coefficient f |
|---|---|---|---|---|
| Soil-soil | Ball-Ball | 100 | 1.5 | 0.4 |
| Soil-isolated stone | Ball-Ball | 50 | 1.5 | 0.3 |
| Soil-pile | Ball-Ball | 50 | 1.5 | 0.5 |
| Pile-isolated stone | Ball-Ball | 500 | 1.5 | 0.7 |
| Name | Constitutive Model | Weight Density γ kN/m3 | Elastic Modulus E GPa | Poisson’s Ratio - - | Cohesion c kPa | Friction Angle φ ° |
|---|---|---|---|---|---|---|
| The core pile | Elasticity | 27 | 70 | 0.33 | - | - |
| The outer concrete | Elasticity | 12 | 2 | 0.38 | - | - |
| Experiment Number | Pile Type | Ultimate Bearing Capacity (N) | Pile Top Displacement (D) |
|---|---|---|---|
| 1 | Planted pile | 12.8 | 0.2 D (2 mm) |
| 2 | Planted pile | 38.4 | 0.35 D (3.5 mm) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liao, W.; Cai, Q.; Guo, X.; Lin, H.; Zhou, J.; Su, S. Experimental Study on the Uplift Bearing Capacity of Pre-Drilled Planted Piles in Isolated Stone Strata Based on Transparent Soil Technology. Appl. Sci. 2025, 15, 304. https://doi.org/10.3390/app15010304
Liao W, Cai Q, Guo X, Lin H, Zhou J, Su S. Experimental Study on the Uplift Bearing Capacity of Pre-Drilled Planted Piles in Isolated Stone Strata Based on Transparent Soil Technology. Applied Sciences. 2025; 15(1):304. https://doi.org/10.3390/app15010304
Chicago/Turabian StyleLiao, Wenli, Qipeng Cai, Xiangyu Guo, Hao Lin, Jiajin Zhou, and Shizhuo Su. 2025. "Experimental Study on the Uplift Bearing Capacity of Pre-Drilled Planted Piles in Isolated Stone Strata Based on Transparent Soil Technology" Applied Sciences 15, no. 1: 304. https://doi.org/10.3390/app15010304
APA StyleLiao, W., Cai, Q., Guo, X., Lin, H., Zhou, J., & Su, S. (2025). Experimental Study on the Uplift Bearing Capacity of Pre-Drilled Planted Piles in Isolated Stone Strata Based on Transparent Soil Technology. Applied Sciences, 15(1), 304. https://doi.org/10.3390/app15010304






