Numerical Investigation of Stratified Slope Failure Containing Rough Non-Persistent Joints Based on Distinct Element Method
Abstract
1. Introduction
2. Principles of the RDFN Model
3. The Jointed Rock Slope Models
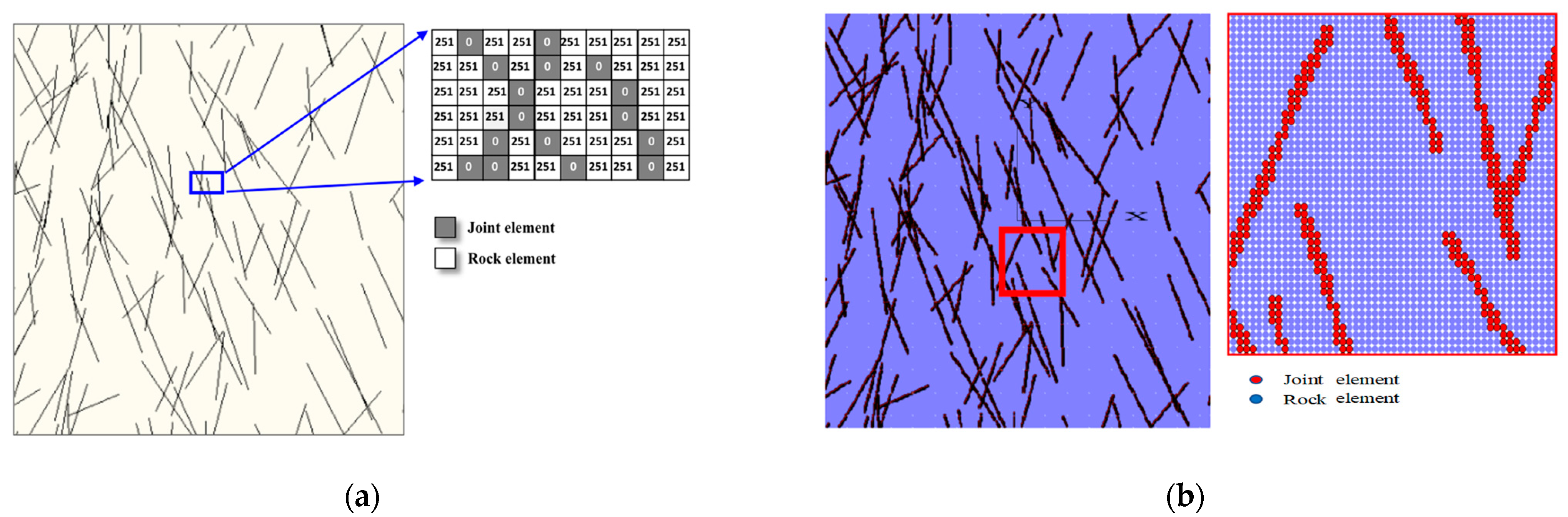
3.1. Numerical Model Based on Digital Image Processing
- The image was uploaded into the MATLAB 2023b program, and the RDFN gray digital image was established using the principle of gray image recognition, as illustrated in Figure 3a;
- The various types of pixel points will be classified based on gray image pixel recognition. In this case, 251 corresponds to white pixels and 0 corresponds to the black pixels. After classification, the spatial distribution coordinates of the bedrock model and joint model will be obtained.
- The joint and rock models will be grouped and assigned based on the PFC code. The mechanical modeling of the RDFN model will then ultimately be carried out, as shown in Figure 3b.
3.2. Mechanical Parameters
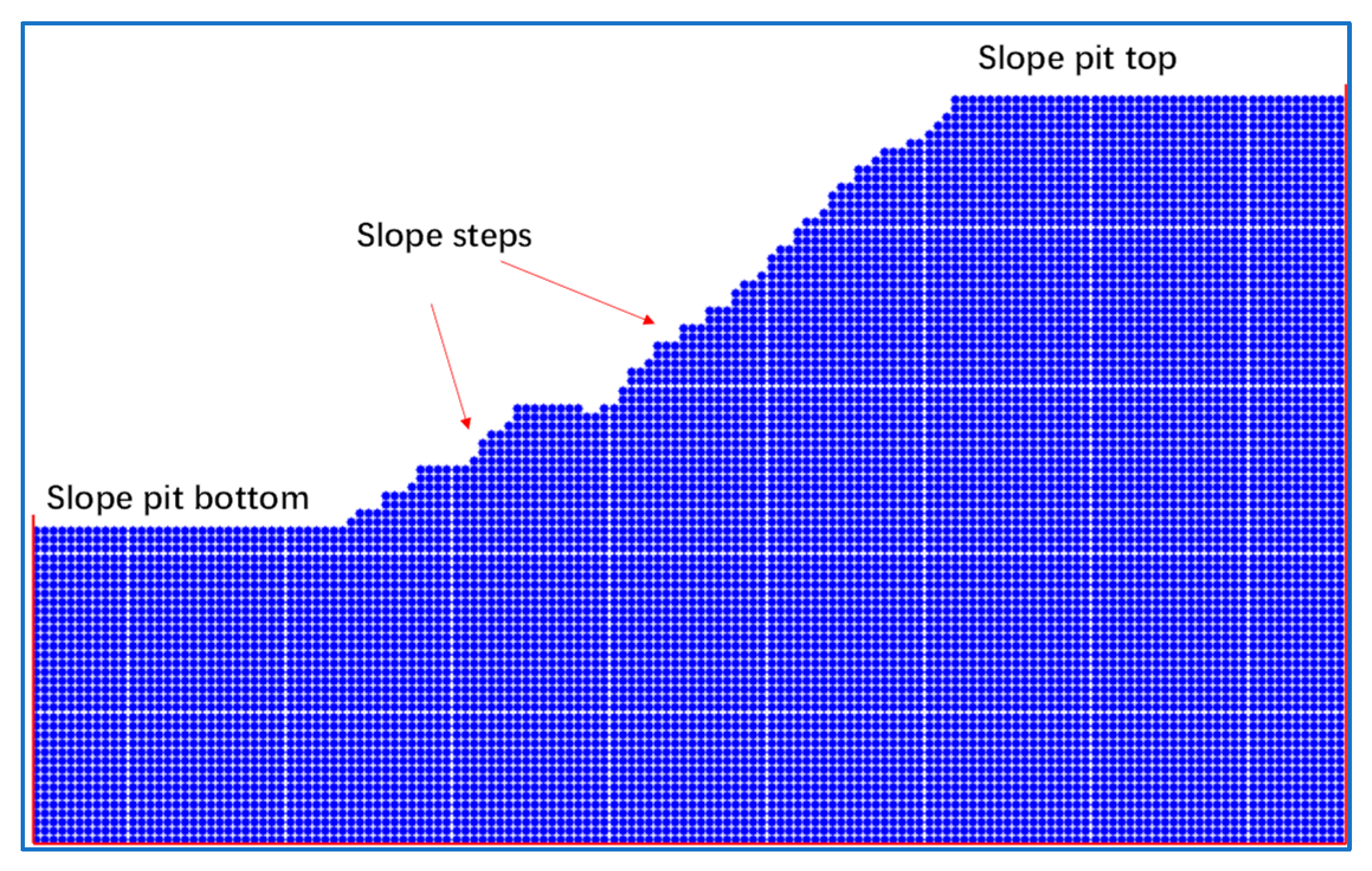
3.3. Establishment of Open-Pit Slope
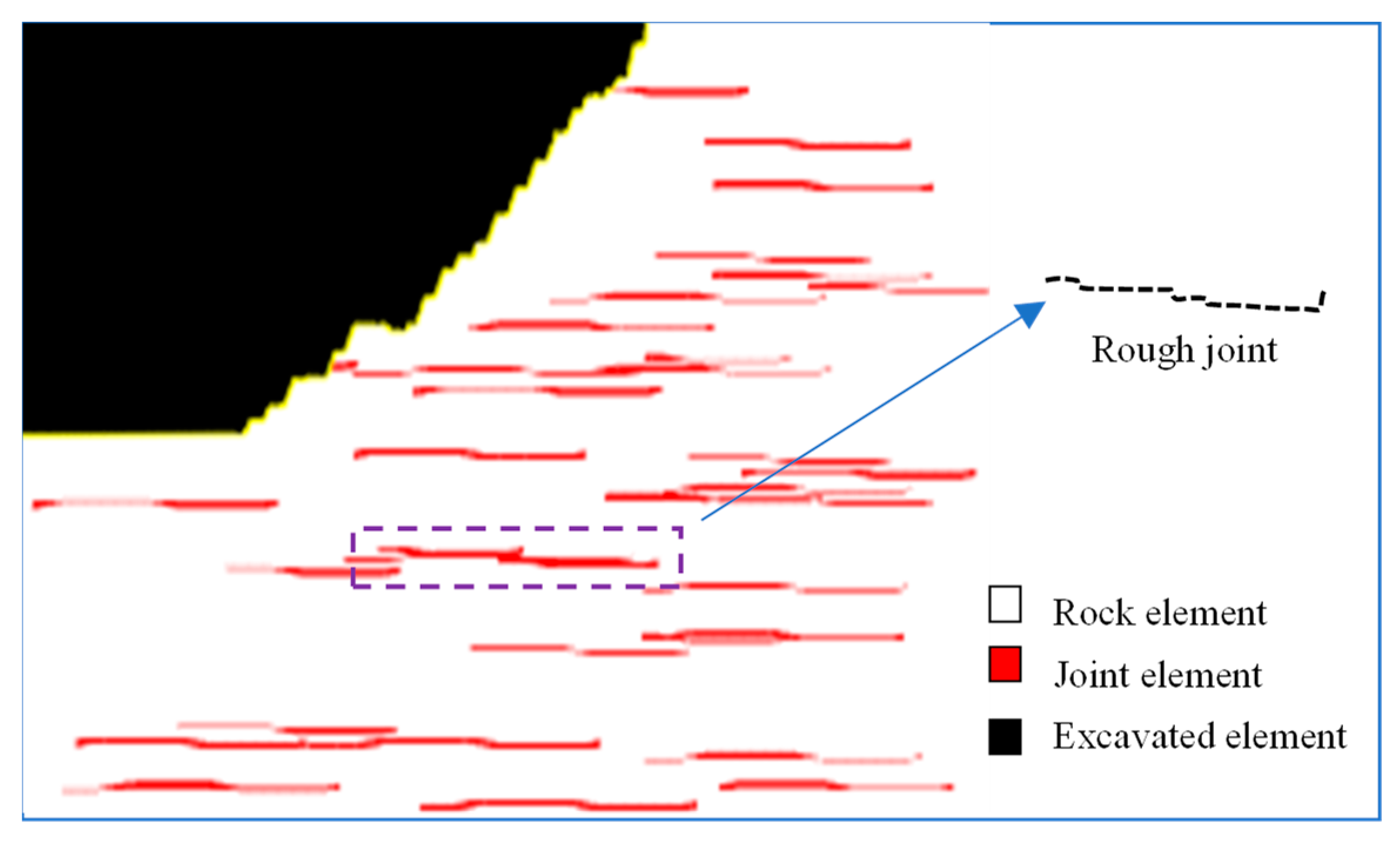
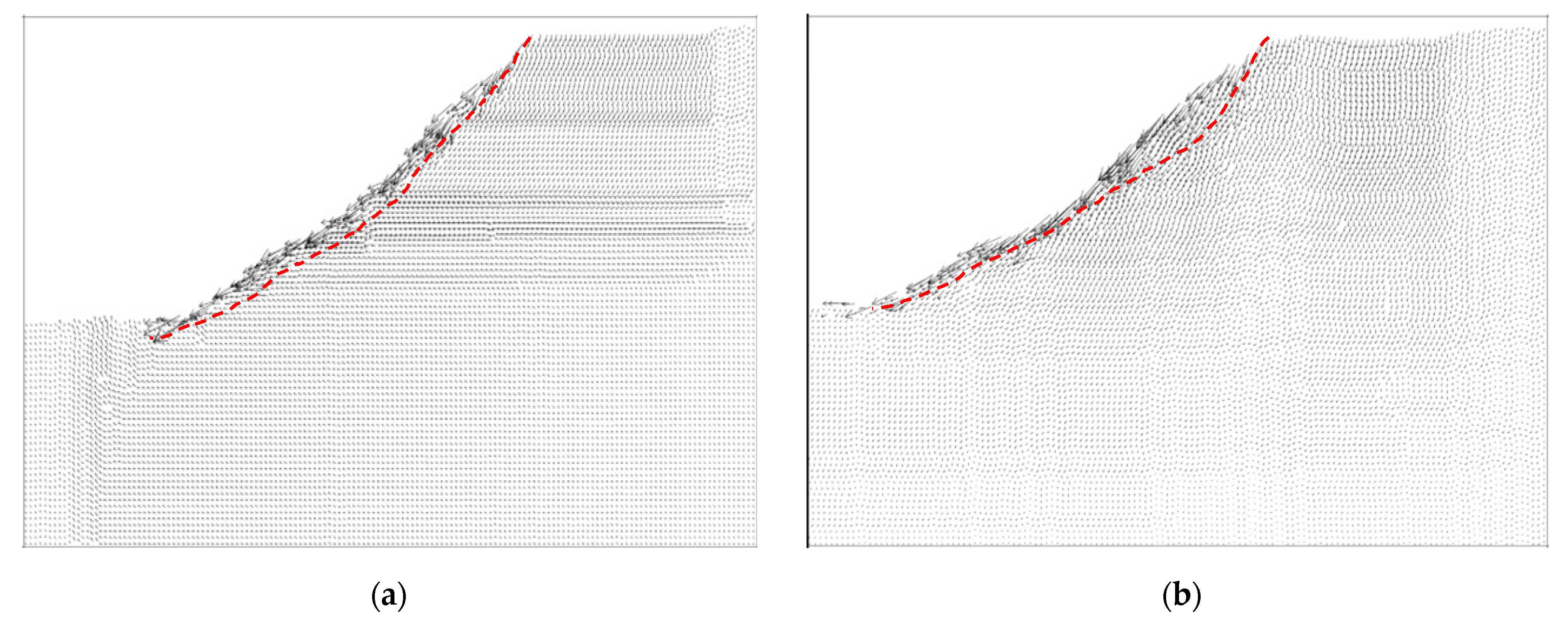
4. Comparison of Failure Modes of RDFN with DFN Models
5. Influence of the Inclination Angle
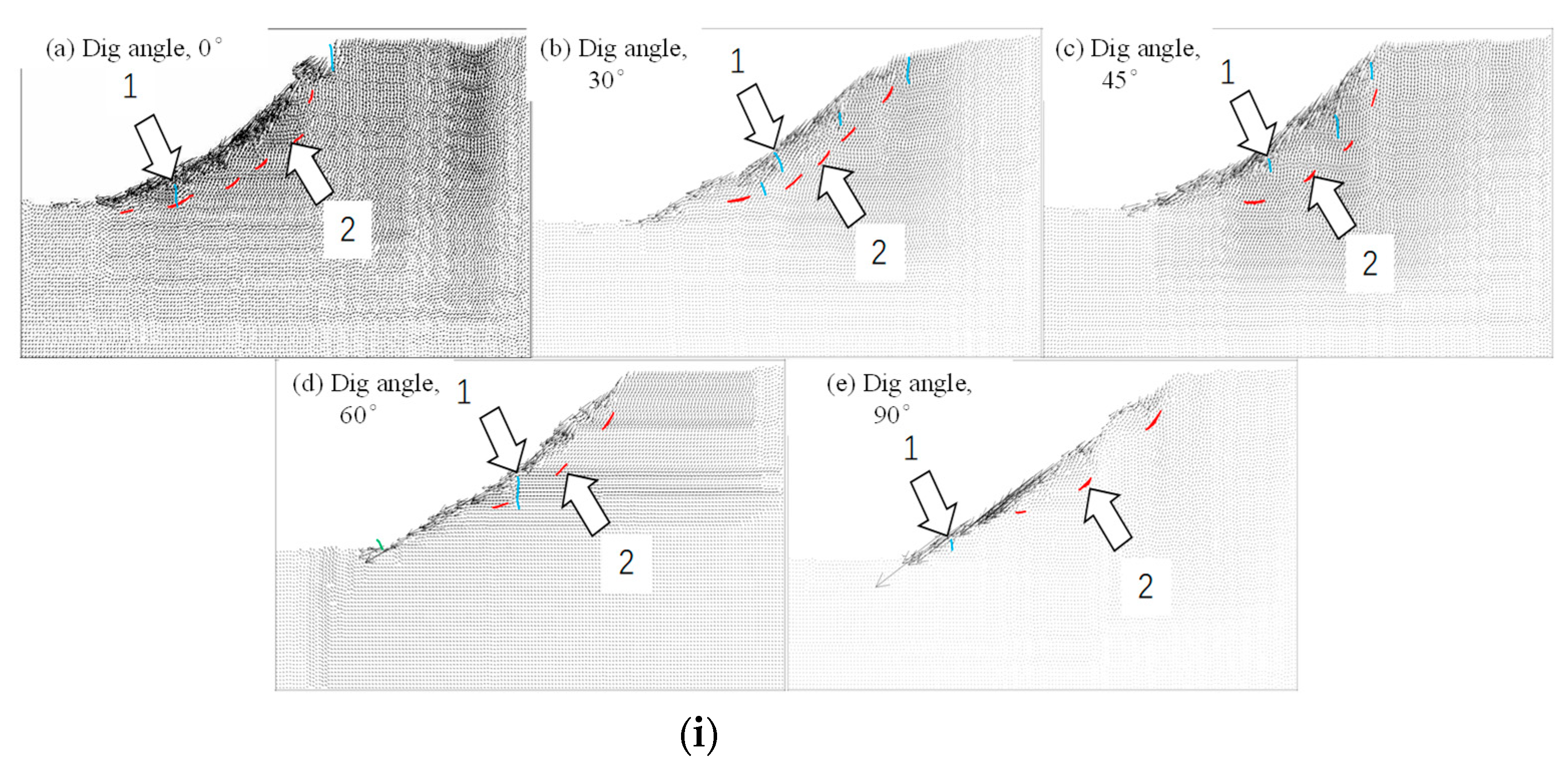
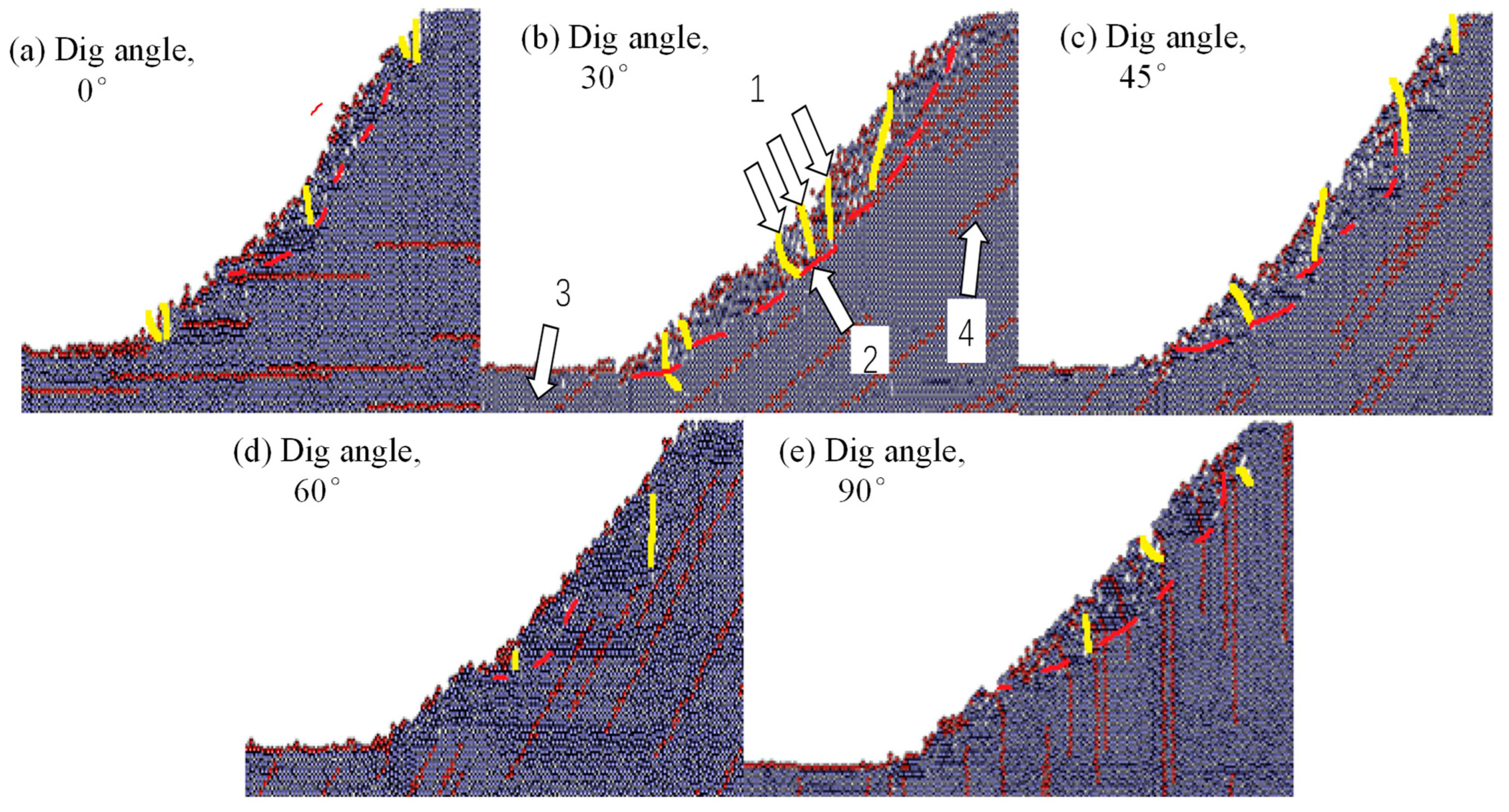
5.1. Failure Modes
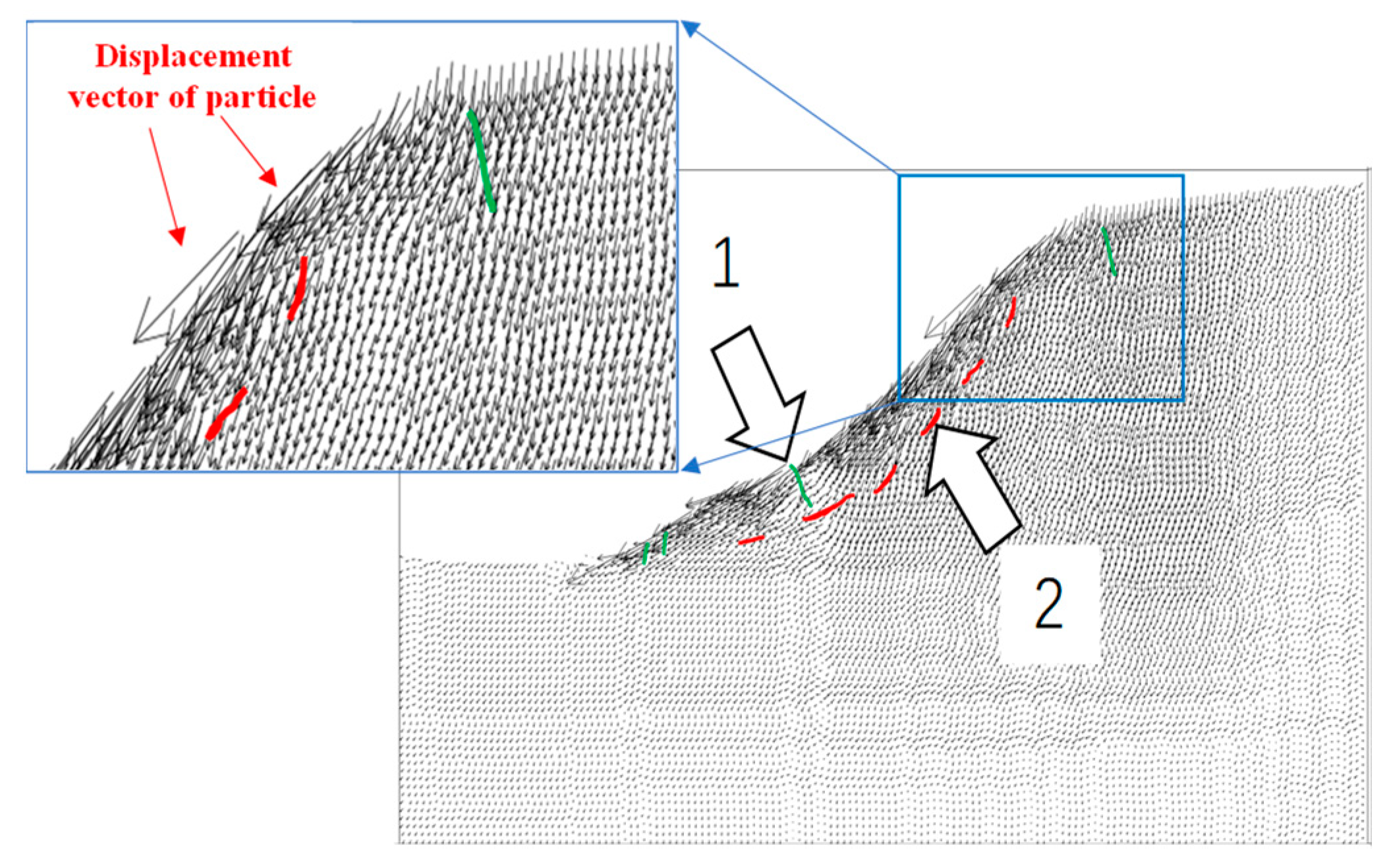
5.2. Displacement
6. Conclusions
- According to this study, a rough slope is more stable than a linear slope for a single fracture inclination angle. Although both slope types show a similar overall displacement trend, they differ significantly in their slip surface and through-tension crack distribution.
- The vectors of displacement for rough-jointed slopes having inclination angles of 0° and 45° are concentrated at the center of the side slope surface and are tangential to the slope surface. This leads to the sliding of the entire slope, resulting in local caving on the surface. Inclination angles of 30° in the joints produce weaknesses in the structural plane, reducing shear strength and causing a breakdown in the bedding slope. For fracture inclination angles of 60° and 90°, significant displacement of the slope is concentrated in the middle, along with through cracks at the top, which result in local slippage.
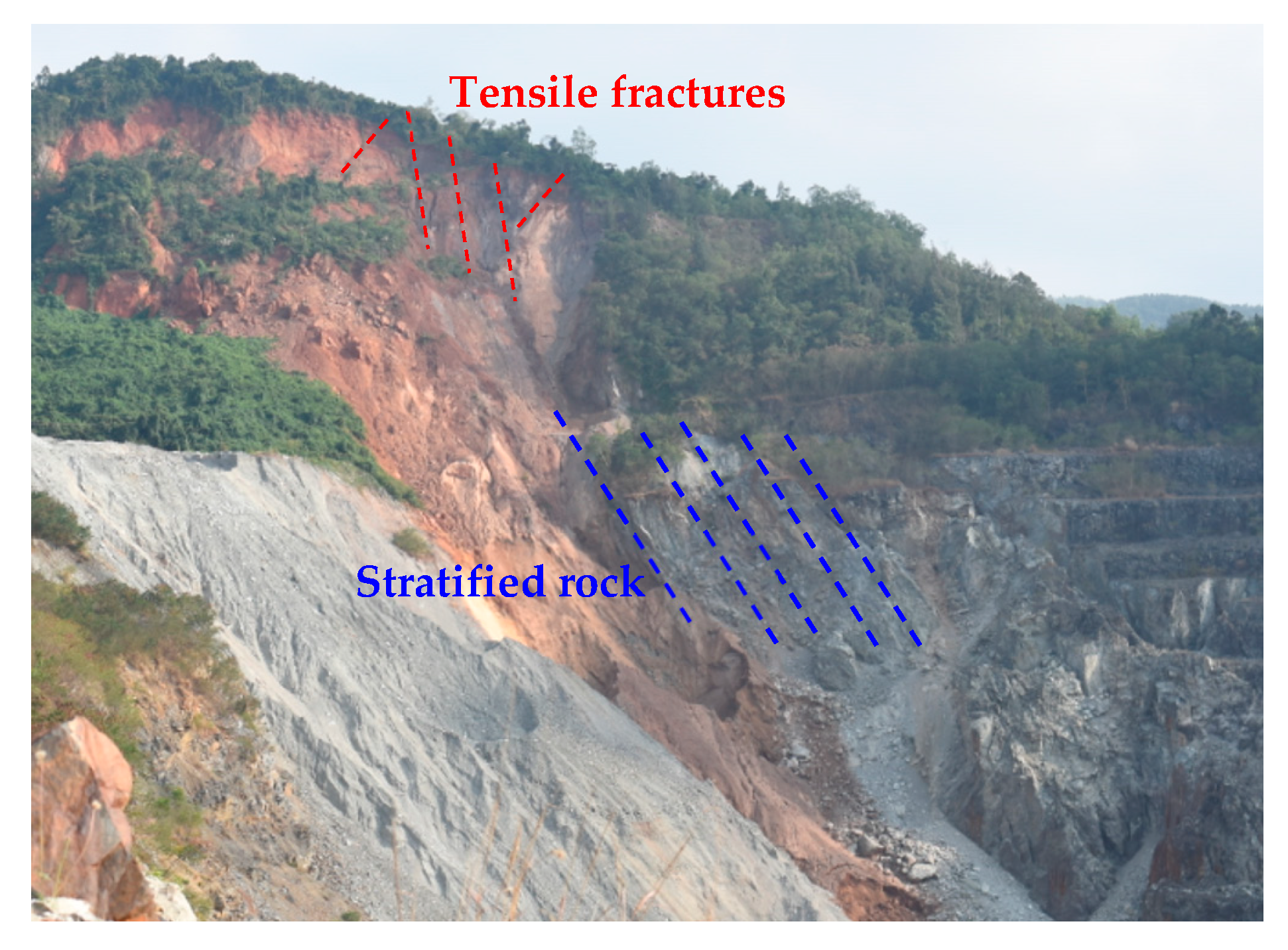
- After comparing simulation results with actual engineering slope failure characteristics and related research, we found that the stability analysis model of rough-jointed rock slopes, based on PFC2D, is closer to the real-world results than the linear-jointed model. This indicates an effective and reliable new method for modeling jointed-rock slopes with complex structures. However, the current modeling method only considers a single fracture distribution mode and a single dip angle, and it needs further optimization to consider multiple dip angles and fracture distributions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sellers, E.J.; Klerck, P. Modeling of the effect of discontinuities on the extent of the fracture zone surrounding deep tunnels. Tunneling Undergr. Space Technol. 2000, 15, 463–469. [Google Scholar] [CrossRef]
- Malan, D.F.; Spottiswoode, S.M. Time-dependent fracture zone behavior and seismicity surrounding deep level stopping operations. In Rockburst and Seismicity in Mines; A. A. Balkema: Rotterdam, The Netherlands, 1997; pp. 173–177. [Google Scholar]
- Li, M.; Yue, Z.; Ji, H.; Xiu, Z.; Han, J.; Meng, F. Numerical Analysis of Interbedded Anti-Dip Rock Slopes Based on Discrete Element Modeling: A Case Study. Appl. Sci. 2023, 13, 12583. [Google Scholar] [CrossRef]
- Liu, G.; Zhao, J.; Song, H. Numerical simulation on influence of joint distributions on failures of rock mass. J. China Univ. Min. Technol. 2007, 36, 17–22. [Google Scholar]
- Pariseau, W.G.; Puri, S.; Schmelter, S.C. A new model for effects of impersistent joint sets on rock slope stability. Int. J. Rock Mech. Min. Sci. 2008, 45, 122–131. [Google Scholar] [CrossRef]
- Li, H.; He, Y.; Xu, Q.; Deng, J.; Li, W.; Wei, Y. Detection and segmentation of loess landslides via satellite images: A two-phase framework. Landslides 2022, 19, 673–686. [Google Scholar] [CrossRef]
- Li, H.; He, Y.; Xu, Q.; Deng, J.; Li, W.; Wei, Y.; Zhou, J. Sematic segmentation of loess landslides with STAPLE mask and fully connected conditional random field. Landslides 2023, 20, 367–380. [Google Scholar] [CrossRef]
- Feng, J.; Wang, J.; Cai, M. Udy on the optimization of the slope angle and ability of high eep open pit slope. China Min. Mag. 2005, 14, 45–48. [Google Scholar]
- Gao, Y.; Xiao, S.; Wu, S.; Tian, Q.; Liu, B. Numerical simulation of the deformation and failure characteristics of consequent rock slopes and their stability. Chin. J. Eng. 2015, 37, 1403–1409. [Google Scholar]
- Zhao, W.; Wu, S.; Gao, Y.; Zhou, Y.; Xiao, S. Numerical modeling and mechanical parameters determination of jointed rock mass. Chin. J. Eng. 2015, 37, 1542–1549. [Google Scholar]
- Zhang, Y.; Ren, G.; Chang, W.; Dong, B.; Tang, Y. Probabilistic analysis of stability of jointed rock slope. J. Chengdu Univ. Technol. 2021, 48, 235–241. [Google Scholar]
- Li, D.; Jia, W.; Cheng, X.; Zhao, L.; Zhang, Y.; Yu, P. Stability of stepped sliding of bedding rock slopes with discontinuous joints. Chin. J. Geotech. Eng. 2022, 44, 2125–2134. [Google Scholar]
- Discenza, M.E.; Di Luzio, E.; Martino, S.; Minnillo, M.; Esposito, C. Role of Inherited Tectonic Structures on Gravity-Induced Slope Deformations: Inference from Numerical Modeling on the Luco dei Marsi DSGSD (Central Apennines). Appl. Sci. 2023, 13, 4417. [Google Scholar] [CrossRef]
- Ren, Z.; Chen, C.; Sun, C.; Wang, Y. Dynamic Analysis of the Seismo-Dynamic Response of Anti-Dip Bedding Rock Slopes Using a Three-Dimensional Discrete-Element Method. Appl. Sci. 2022, 12, 4640. [Google Scholar] [CrossRef]
- Nasseri, M.H.B.; Grasselli, G.; Mohanty, B. Fracture toughness and fracture roughness in anisotropic granitic rocks. Rock Mech. Rock Eng. 2010, 43, 403–415. [Google Scholar] [CrossRef]
- Mohd-Nordin, M.M.; Song, K.-I.; Kim, D.; Chang, I. Evolution of joint roughness degradation from cyclic loading and its effect on the elastic wave velocity. Rock Mech. Rock Eng. 2016, 49, 3363–3370. [Google Scholar] [CrossRef]
- Gao, Z.; Wang, S.; Yin, H.; Zhao, Q.; Vladimr, P.; Li, Y. Estimation of Shear Strength Parameters Considering Joint Roughness: A Stability Case Analysis of Bedding Rock Slopes in an Open-Pit Mine. Appl. Sci. 2023, 13, 5730. [Google Scholar] [CrossRef]
- Wang, P.; Liu, C.; Qi, Z.; Liu, Z.; Cai, M. A rough discrete fracture network model for geometrical modeling of jointed rock masses and the anisotropic behaviour. Appl. Sci. 2022, 12, 1720. [Google Scholar] [CrossRef]
- Wu, C.; Bian, K.; Liu, J.; Hu, X.; Gao, Y. Particle discrete element simulation of water absorption and softening effect of heterogeneous rocks. Water Resour. Power 2021, 39, 151–154+150. [Google Scholar]
- Wang, P.; Meifeng, C.; Fenhua, R.; Li, C.; Yang, T. A digital image-based discrete fracture network model and its numerical investigation of direct shear tests. Rock Mech. Rock Eng. 2017, 50, 1801–1816. [Google Scholar] [CrossRef]
- Yoon, J. Application of experimental design and optimization to PFC model calibration in uniaxial compression simulation. Int. J. Rock Mech. Min. Sci. 2007, 44, 871–889. [Google Scholar] [CrossRef]
- Chang, J.; Hou, C.; Zhao, X. Failure mechanism analysis and shape optimization of multi weak layer slope. Mod. Min. 2022, 38, 81–84. [Google Scholar]
- Yang, P.; Li, S.; Chao, W.; Tu, X.; Wang, Y.; Jin, Y.; Zhou, K. Analysis on deformation and failure mechanism of multi-weak layer composite slope in karst region. Sci. Technol. Eng. 2022, 22, 6264–6269. [Google Scholar]
- Zhang, S.; Zhang, Y.; Liang, D. Failure mechanism of inclination joint rock slope based by discrete elements method. J. Water Resour. Archit. Eng. 2022, 20, 19–25+42. [Google Scholar]
- Yang, X.; Lu, Z.; Chen, C.; Sun, C.; Liu, X. Analysis of mechanical model of sliding-bending failure in bedding rock slopes with slab-rent structure. Rock Soil Mech. 2022, 43, 258–566. [Google Scholar]
- Liu, X.; Wang, Y.; Xu, B.; Zhou, X.; Yi, L.; Huang, J.; Wang, Z. Investigation on dynamic response mechanism of slopes with serrated structural planes under degradation of rock mass in hydro-fluctuation belt. Chin. J. Rock Mech. Eng. 2022, 41, 2377–2388. [Google Scholar]
- Zhong, Z.; Huang, D.; Song, Y.; Cen, D. Three-dimensional cracking and coalescence of two spatial-deflection joints in rock-like specimens under uniaxial compression. Int. J. Rock Mech. Min. Sci. 2022, 159, 105196. [Google Scholar] [CrossRef]
- Yin, Y.; Sun, P.; Zhang, M.; Li, B. Mechanism on apparent dip sliding of oblique inclined bedding rockslide at Jiweishan, Chongqing, China. Landslides 2011, 8, 49–65. [Google Scholar] [CrossRef]

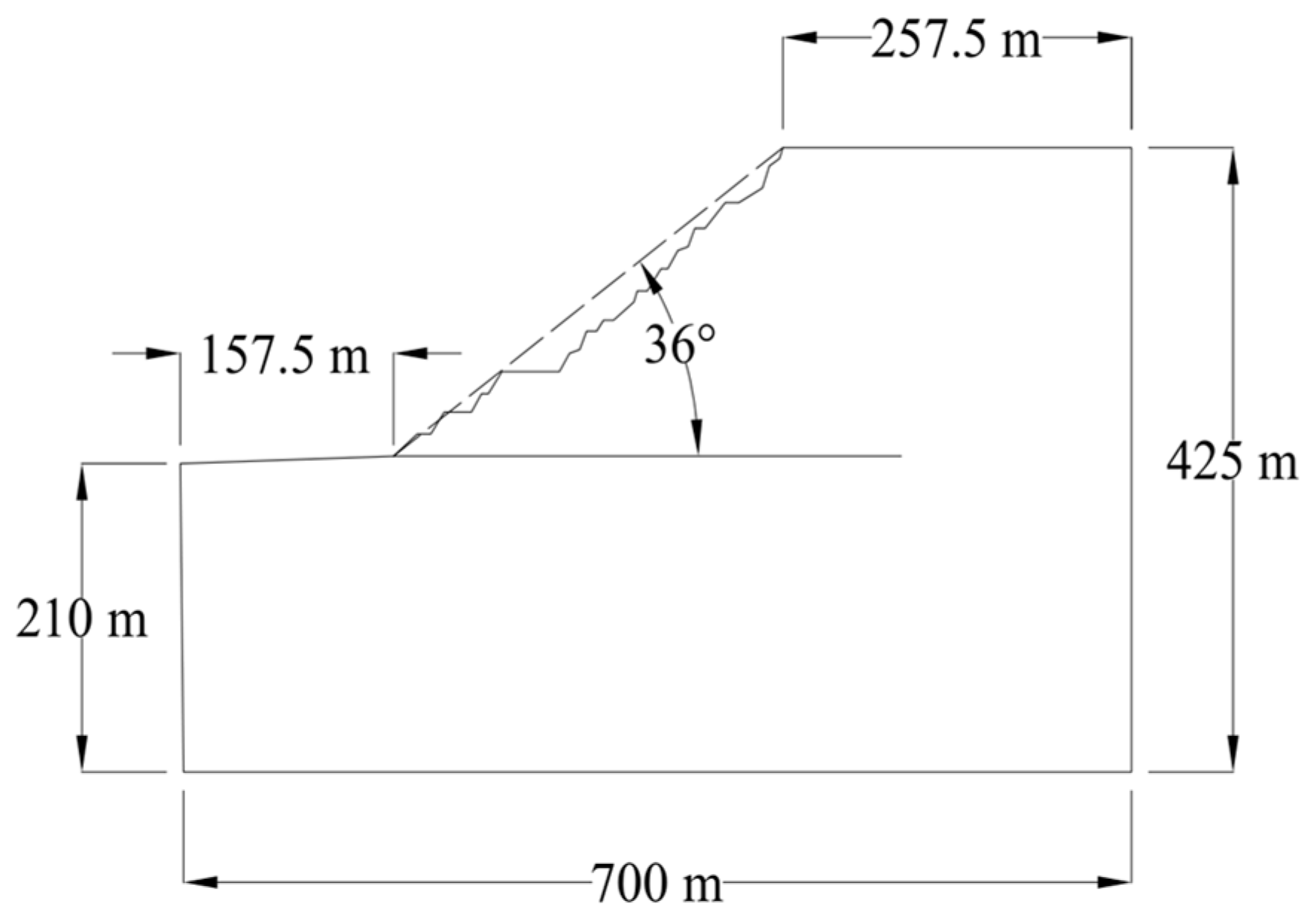



| No. | Amplitude (Amax)/m | Frequency (ω) | Climbing Width (d)/m | Fourier Series (n) |
|---|---|---|---|---|
| 1 | 3 | 3 | 0.1 | 50 |
| 2 | 4 | 2 | 0.1 | 50 |
| 3 | 9 | 2 | 0.5 | 30 |
| 4 | 2 | 3 | 0.5 | 50 |
| 5 | 6 | 2 | 0.001 | 50 |
| Parameters | Density/g·cm−3 | Modulus of Elasticity/GPa | Uniaxial Compressive Strength/(MPa) |
|---|---|---|---|
| Value | 3.527 | 12.0 | 131.73 |
| Type | Micro Parameters | Value |
|---|---|---|
| Intact rock | Particle radius ratio | 1.66 |
| Density/g·cm−3 | 3.527 | |
| Particle contact modulus E/GPa | 14.0 | |
| kn/ks | 1.5 | |
| Normal particle contact strength/N | 100 × 106 | |
| Shear contact strength/N | 135 × 106 | |
| Particle friction coefficient | 0.86 | |
| Joint element | Joint friction coefficient, fric | 0.68 |
| Normal contact bonding strength/N | 3.00 × 106 | |
| Shear contact bonding strength/N | 4.035 × 106 |
| Numerical Models | Dip Angle (°) | Total Number of Elements | Number of Rock Elements | Number of Joint Elements | Joint Element Proportion |
|---|---|---|---|---|---|
| Rough joint | 30° | 9147 | 7978 | 1169 | 12.7% |
| Linear joint | 30° | 9109 | 7924 | 1185 | 13.0% |
| Rough joint | 60° | 9703 | 8735 | 968 | 9.9% |
| Linear joint | 60° | 9554 | 8578 | 976 | 10.0% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Fu, Y.; Qin, R.; Wang, P. Numerical Investigation of Stratified Slope Failure Containing Rough Non-Persistent Joints Based on Distinct Element Method. Appl. Sci. 2024, 14, 3665. https://doi.org/10.3390/app14093665
Zhang Y, Fu Y, Qin R, Wang P. Numerical Investigation of Stratified Slope Failure Containing Rough Non-Persistent Joints Based on Distinct Element Method. Applied Sciences. 2024; 14(9):3665. https://doi.org/10.3390/app14093665
Chicago/Turabian StyleZhang, Yishan, Yilin Fu, Ran Qin, and Peitao Wang. 2024. "Numerical Investigation of Stratified Slope Failure Containing Rough Non-Persistent Joints Based on Distinct Element Method" Applied Sciences 14, no. 9: 3665. https://doi.org/10.3390/app14093665
APA StyleZhang, Y., Fu, Y., Qin, R., & Wang, P. (2024). Numerical Investigation of Stratified Slope Failure Containing Rough Non-Persistent Joints Based on Distinct Element Method. Applied Sciences, 14(9), 3665. https://doi.org/10.3390/app14093665







