Experimental Investigation of the Influence of Longitudinal Tilt Angles on the Thermal Performance of a Small-Scale Linear Fresnel Reflector
Abstract
1. Introduction
- (i)
- (ii)
- The solar tracking system. A solar tracking system is mandatory in a system. However, solar tracking is optional in an system even though the use thereof increases electricity production. The use of solar tracking systems in and systems complicates their implementation and increases their cost.
- (iii)
- Incident solar irradiance on cells. The beam, diffuse, and ground-reflected components of solar irradiance are incident on the cells in systems. In contrast, only the beam component is incident on the cells in systems. The available diffuse solar irradiance often represents a significant fraction of the solar irradiance on a tilted surface. There are even some locations where this component is decisive.
- (iv)
- The operating temperature of the cells. The energy production of a system depends on the operating temperature of the cells [5]. For example, manufacturers of crystalline photovoltaic cells estimate a decrease of between and in electrical efficiency per °C increase over the reference operating temperature [6]. In systems, the concentration of solar irradiance causes an increase in the temperature of the cells, but it is easier to implement a cooling system due to the size of the cell system. In contrast, it is not possible to implement a cooling system in system due to the large size of the cell system. In addition to reducing the electrical efficiency of cells, the high temperatures to which a cell system is subjected damage its backsheet, encapsulants, edge seals, and optical coatings [7].
- (v)
- The system cost. Due to the low efficiency of cells, systems require large cell surfaces. In contrast, systems are equipped with cheaper optical materials (e.g., mirrors or lenses), which reduces the cost of these devices. Even so, it has been estimated that the cost of an system can be more than double ( times) the cost of an system [8]. However, Moreno et al. [9] presented a study showing that under certain conditions ((i) high beam solar irradiance (>2.5 (MWh/m2 year)) and (ii) at utility scale) technologies can be competitive with systems.
- (vi)
- Waste cells. The lifetime of modules is estimated at 25 years. modules must be recycled after this period. In its report [10], the International Energy Agency Photovoltaic Power Systems () estimates that million tonnes of modules will need to be recycled by 2030 and 60 million tonnes by 2050. systems use a fraction of the cell surface area that systems use, so the resulting waste in systems will also be a fraction. This is one of the main advantages of systems.
- (vii)
- The cogeneration system. Cogeneration systems are a very important element nowadays in reducing global warming [11]. Both thermal energy and electrical energy are necessary in the building sector. systems can produce heat and electricity simultaneously, for increased efficiency, whereas systems can only produce electrical energy.
- (viii)
- The surface area required for system implementation. The deployment of technologies in buildings is limited by the available surface area on building roofs. Silva and Fernandes [12] presented a study showing that systems require less surface area to produce the same thermal and electrical performance compared to the combined use of flat thermal and systems.
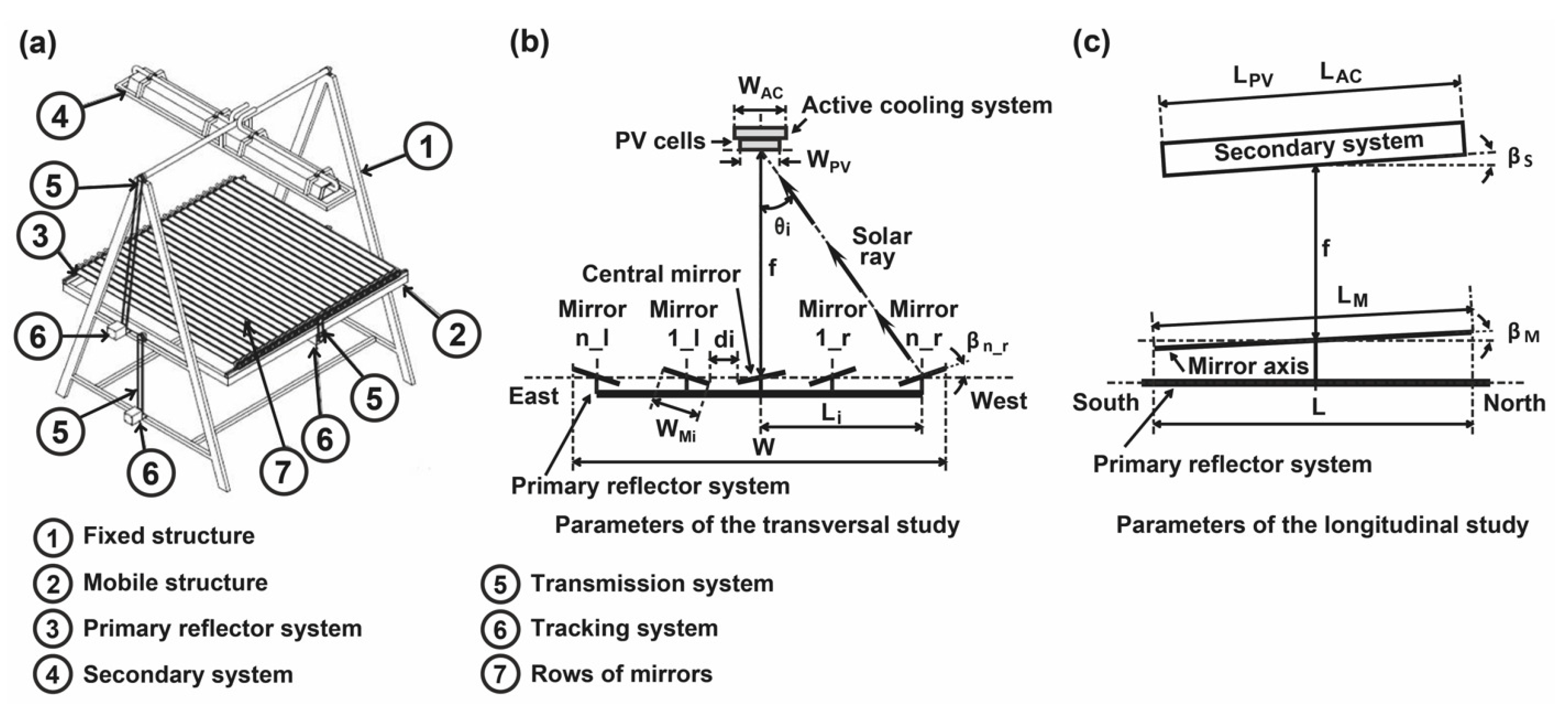
2. An Overview of the LCPV System Based on an SSLFR
2.1. Mirrors’ Movement
2.2. The Longitudinal Tilt Angle of the Secondary System
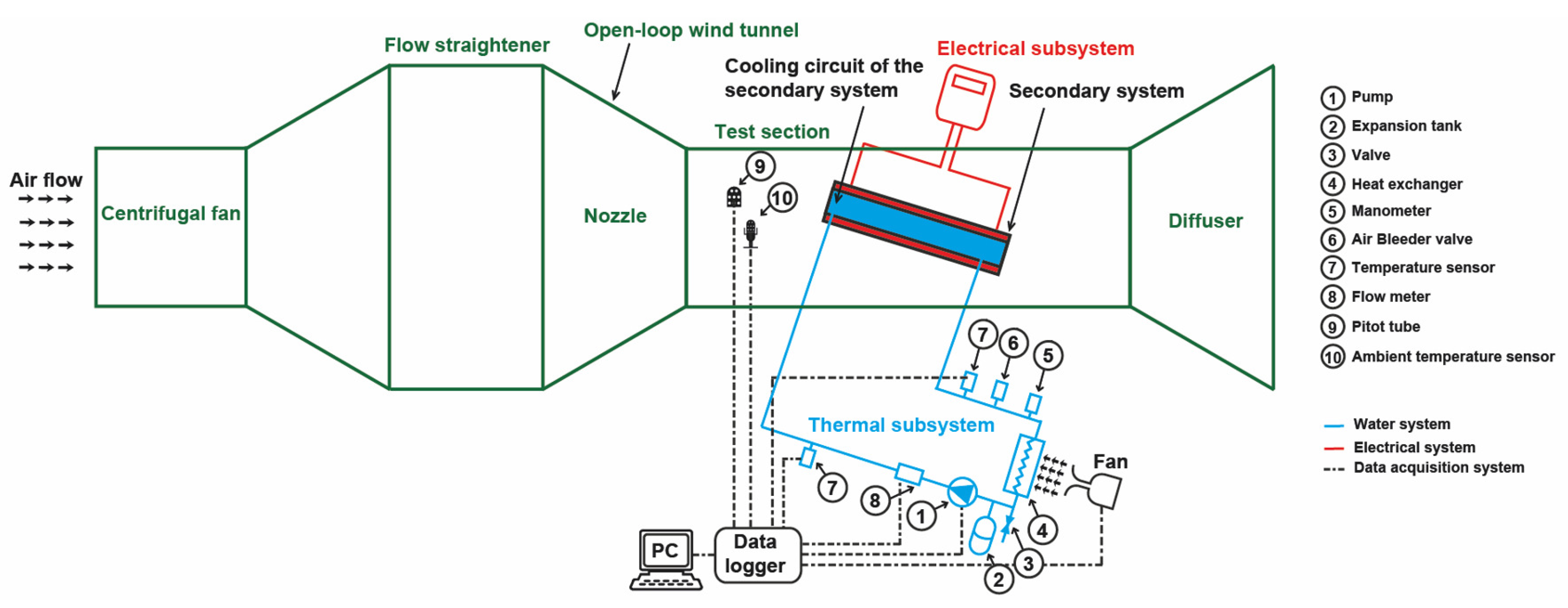
3. Experimental Setup
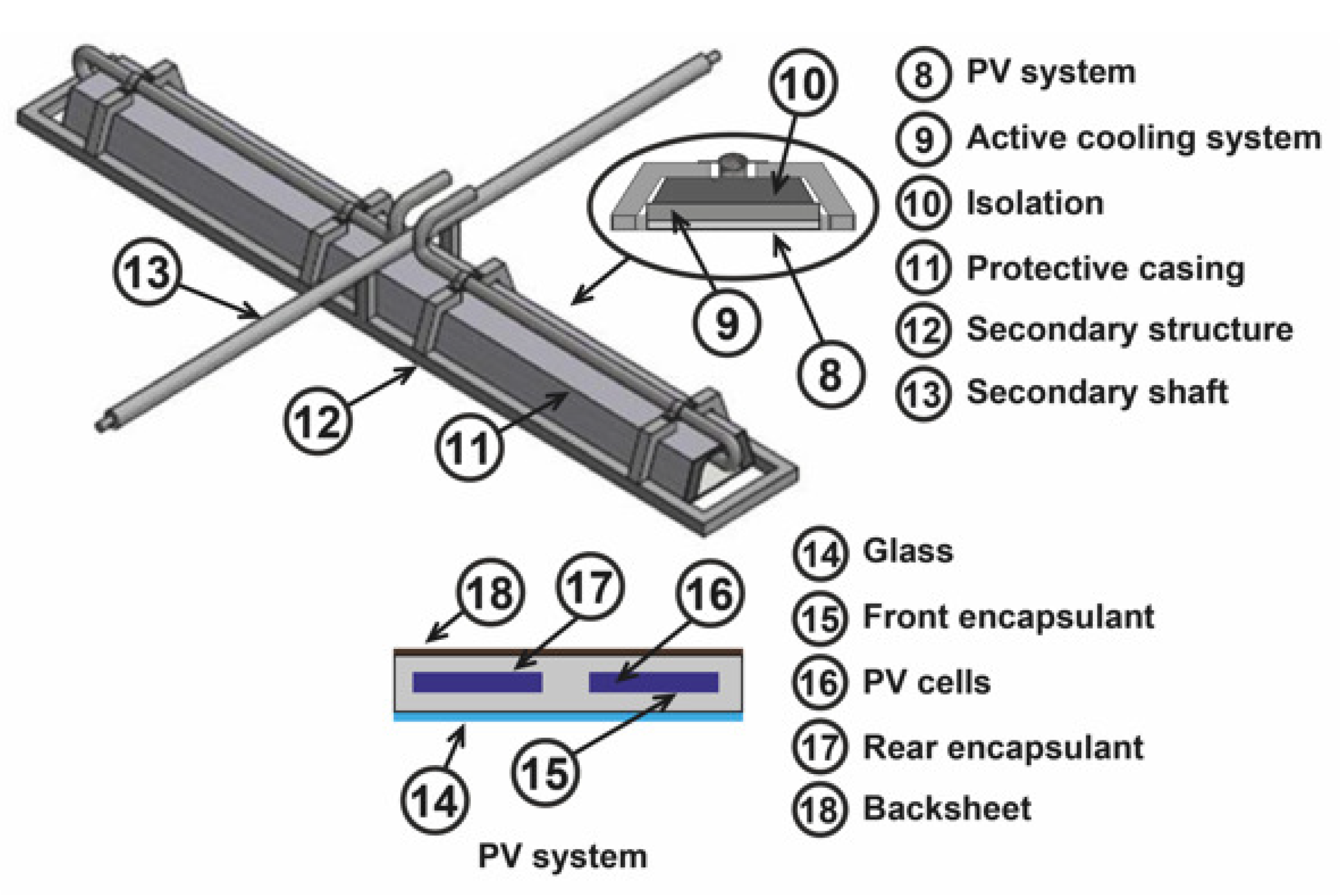
3.1. Experimental Platform
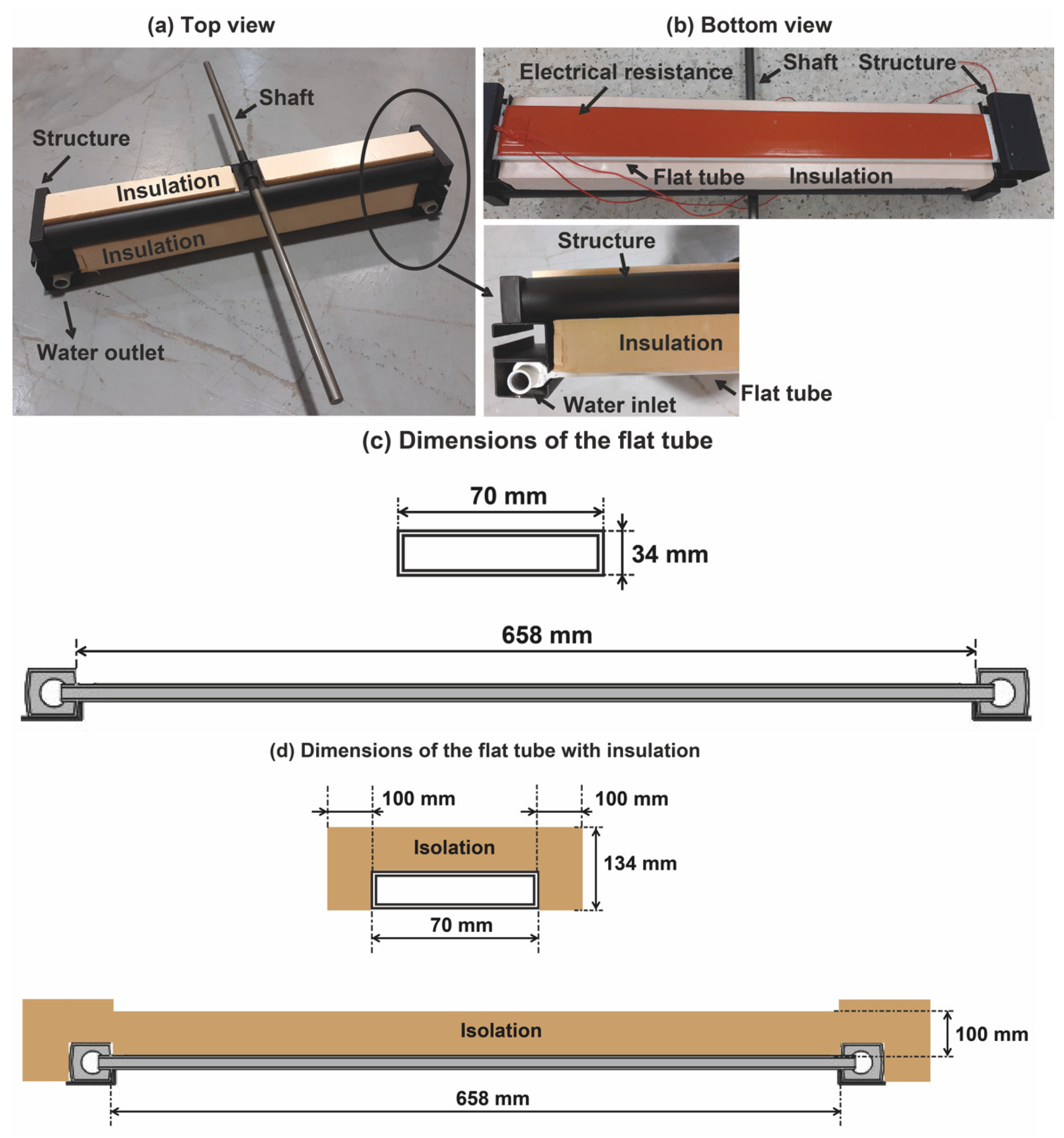
3.2. The Prototype
- (i)
- The width and length of the prototype are limited by the dimensions of the available wind tunnel. Therefore, a commercial cell with a width of 60 (mm) and a length of 30 (mm) was chosen. A total of 21 cells were arranged in the system. Therefore, the length of the system was 658 (mm) ( (mm)).
- (ii)
- (iii)
- (iv)
- The choice of the number of mirrors is directly related to the cost of the [35]. Nine mirrors were chosen so the cost would not be too high. Therefore, .
- (v)
- Based on the length of the system, a mirror length of 658 (mm) was chosen.
- (vi)
- (vii)
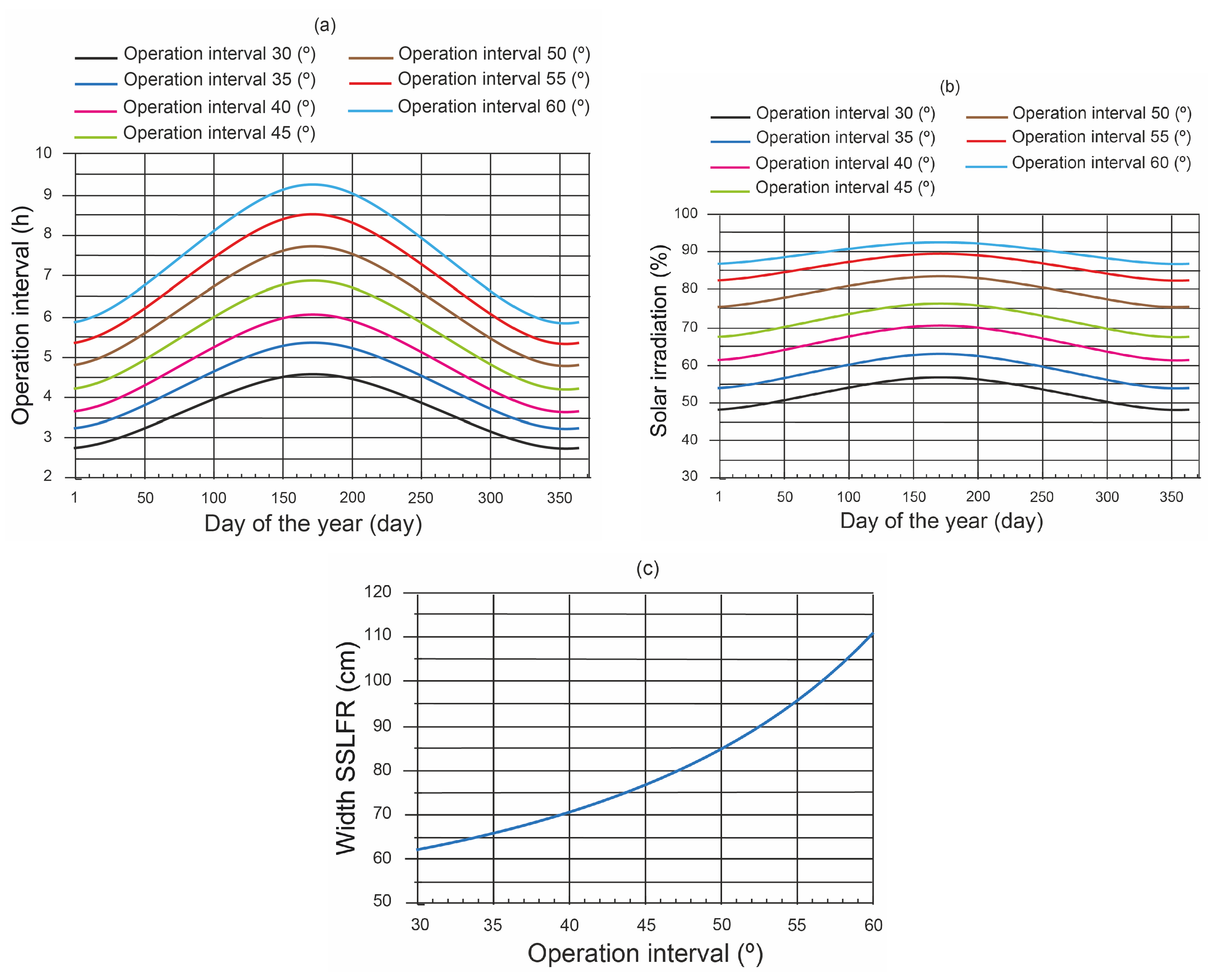
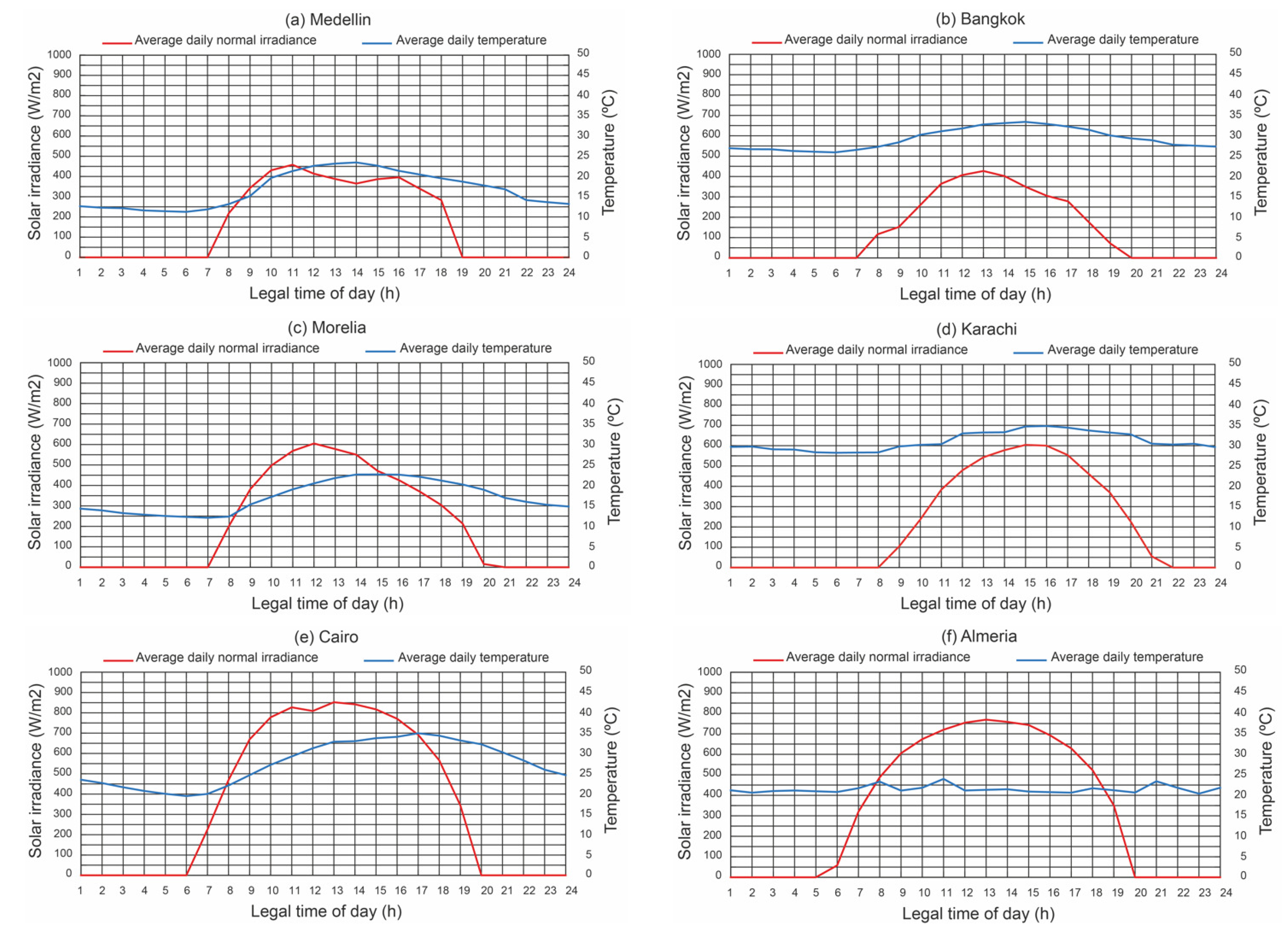
- The optimal design conditions are impossible to meet from sunrise to sunset. Therefore, Ref. [14] defines the so-called “optimum operation interval” (). This guarantees the homogeneous distribution of solar irradiance without shadows or blocking. Several aspects have to be taken into account when choosing , such as the length of the , the optimal hours of operation, and the total annual solar irradiation effectively reaching the cells. As an example, Figure 5 shows three curves related to the choice of for the city of Almeria, Spain (latitude N, longitude W, and elevation 22 (m)), as well as the parameters chosen in this section.
3.3. The Electrical Subsystem
3.4. The Thermal Subsystem
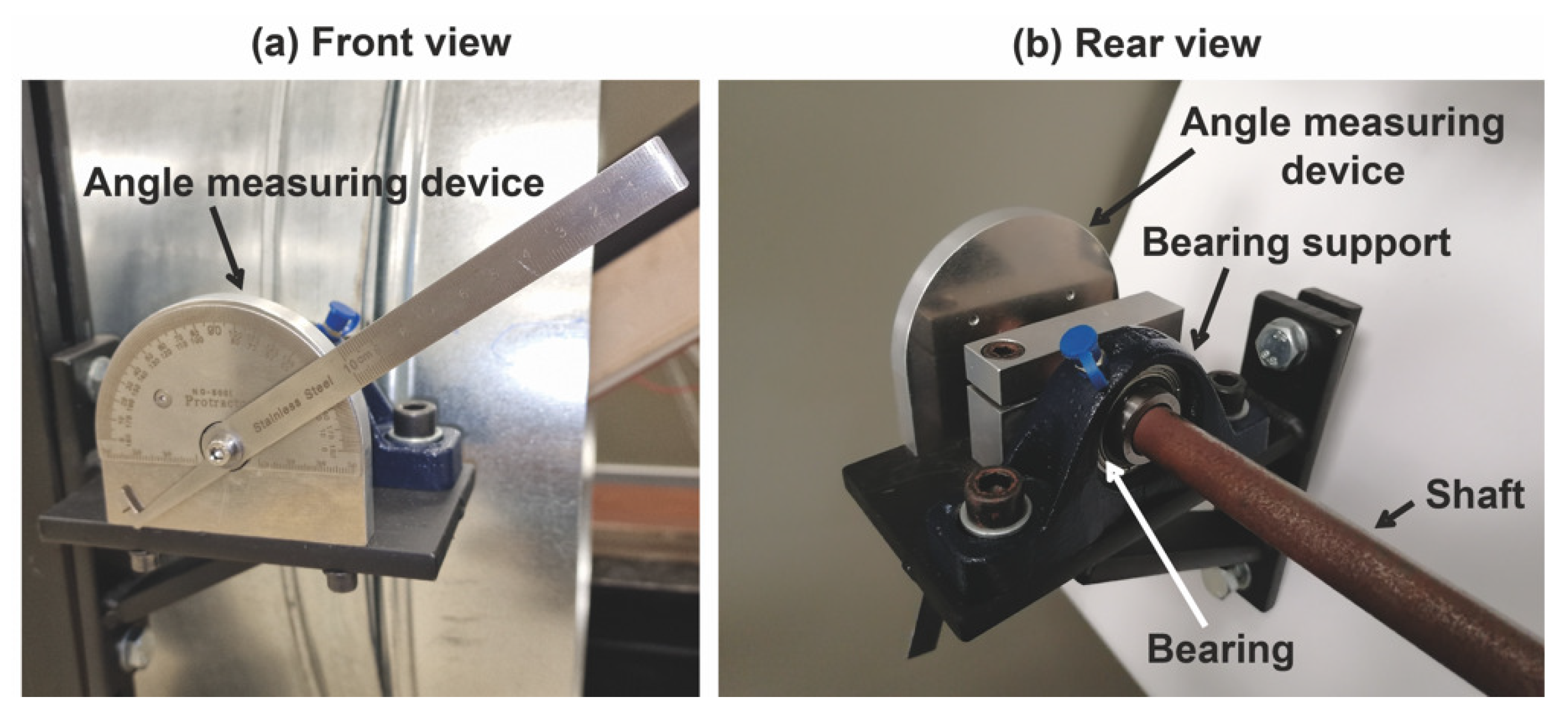
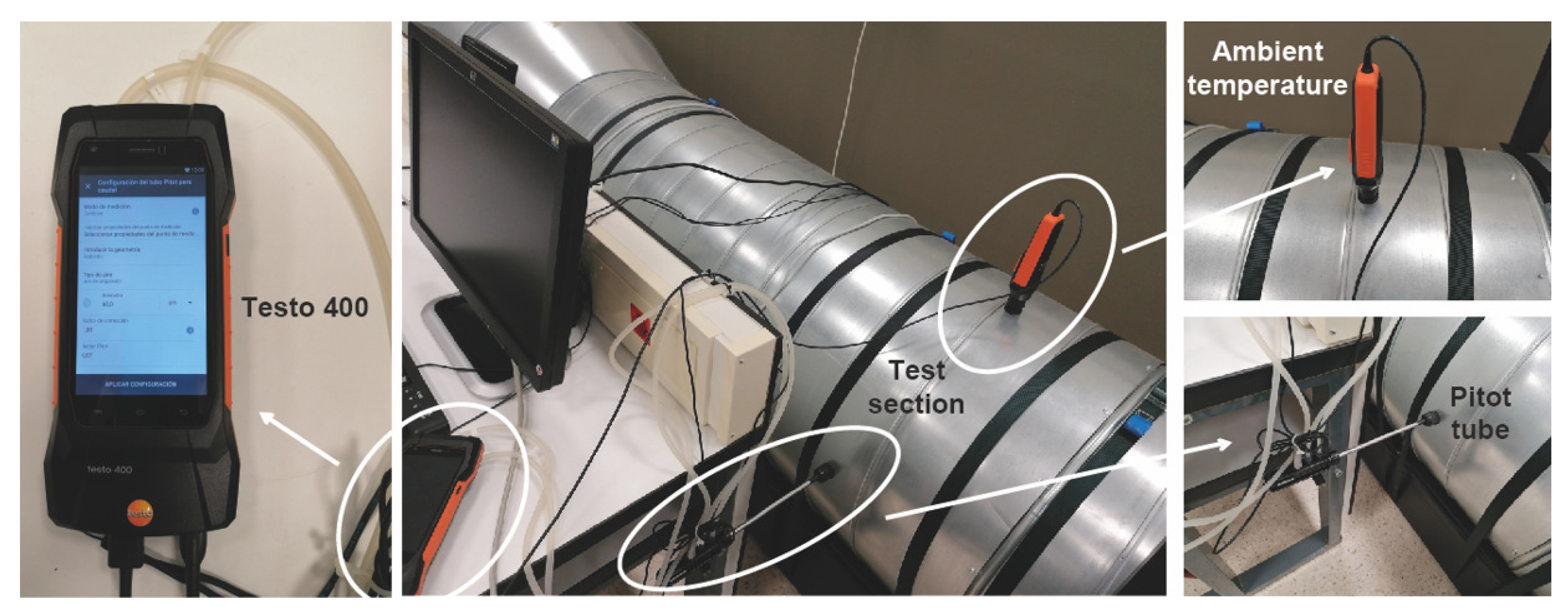
3.5. Measuring Instruments
3.6. Test Conditions
3.6.1. Longitudinal Tilt Angle
- (i)
- The cities are located in the Northern Hemisphere. There are several reasons for focusing this study on this hemisphere: (a) Ninety percent of the world’s population is concentrated in the Northern Hemisphere [51]. (b) The Northern Hemisphere has the largest building roof area, which is the ideal location for energy production in cities. In the European Union, there is a total roof area of residential buildings of about 19 billion (m2) [52].
- (ii)
- There is high beam incident solar irradiance in the chosen city. This is one of the conditions that had to be met for this type of technology to be cost-effective. The SolarGis software [53] uses data from 19 high-precision satellites for its simulations and provides maps with a rigorous, systematic approach of different parts of the world. Figure 10 shows a map of the direct normal solar irradiation all over world.
- (iii)
- There are different climate zones with quite different latitudes. In [24], they propose the choice of study locations with a difference of about 6 (°) latitude starting at latitudes of 36 (°) up to 60 (°). In our study, locations higher than 40 (°) latitude are not considered due to the value of the incident direct solar irradiance.
- (iv)
- The optimal longitudinal tilt angle of the secondary system demonstrated in [24] is used to define the longitudinal tilt angle at each chosen location. This angle coincides with the latitude of the location.
3.6.2. Wind Speed
3.6.3. Water Flow Rate
3.6.4. Ambient Temperature
3.6.5. Duration of Each Test
3.6.6. Thermal Power
3.6.7. Electrical Efficiency
3.6.8. Thermal Energy Chosen for the Test
- (i)
- The concentration factor of the system is . To determine this value, the parameters of the primary reflector system shown in Table 1 and the dimensions of the prototype were used. With the incident solar irradiance at the Cairo and Almeria locations, a thermal power not converted into electricity by the PV system of approximately 300 (W) is obtained.
- (ii)
- The choice of 300 (W) allows the Medellin, Bangkok, Morelia, and Karachi locations to increase the concentration factor in future studies.
- (iii)
- The choice of 400 (W) allows all locations under study to be able to increase the concentration factor in future studies.
3.7. Experimental Procedure
- (i)
- The water was circulated through the cooling system by the pump to simulate thermosyphon operation. The flow meter registered the water flow rate, and a valve was used to keep it constant.
- (ii)
- The electrical resistance simulated the energy that the photovoltaic cells do not convert into electricity. An autotransformer was used to ensure that the thermal power remained constant throughout the test.
- (iii)
- The test started with a wind speed of 0 (m/s), and every hour the wind speed was increased up to (m/s).
- (iv)
- The following parameters were measured during the test: the power absorbed by the electrical resistance, the ambient temperature, the water flow rate, the temperature at the water inlet/outlet, and the wind speed.
3.8. Assessment Parameters
3.8.1. Useful Heat Gain
3.8.2. Thermal Efficiency
3.8.3. Solar Irradiation Gain Incident on the Cells
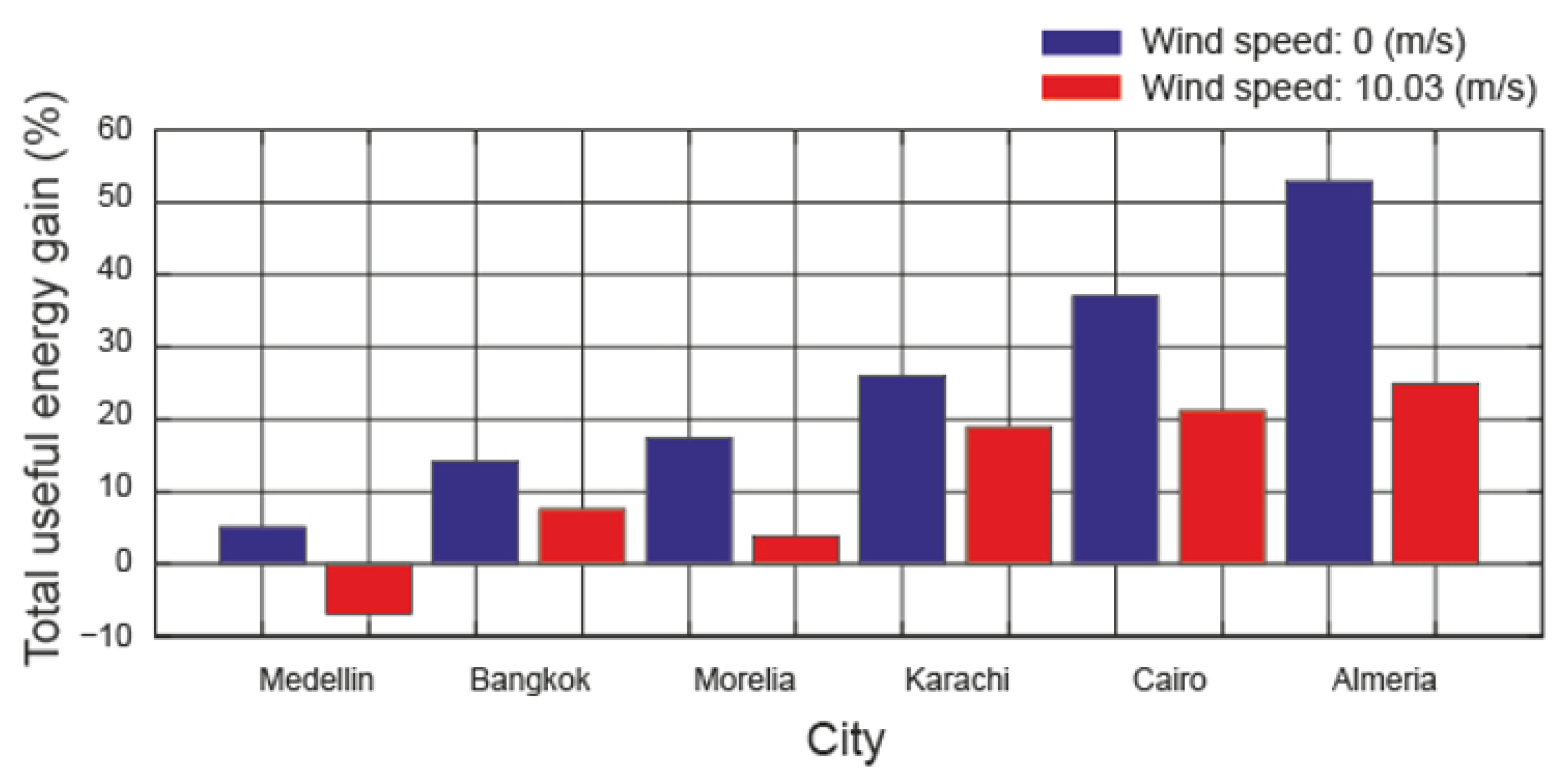
3.8.4. Total Useful Energy Gain
4. Experimental Results and Discussion
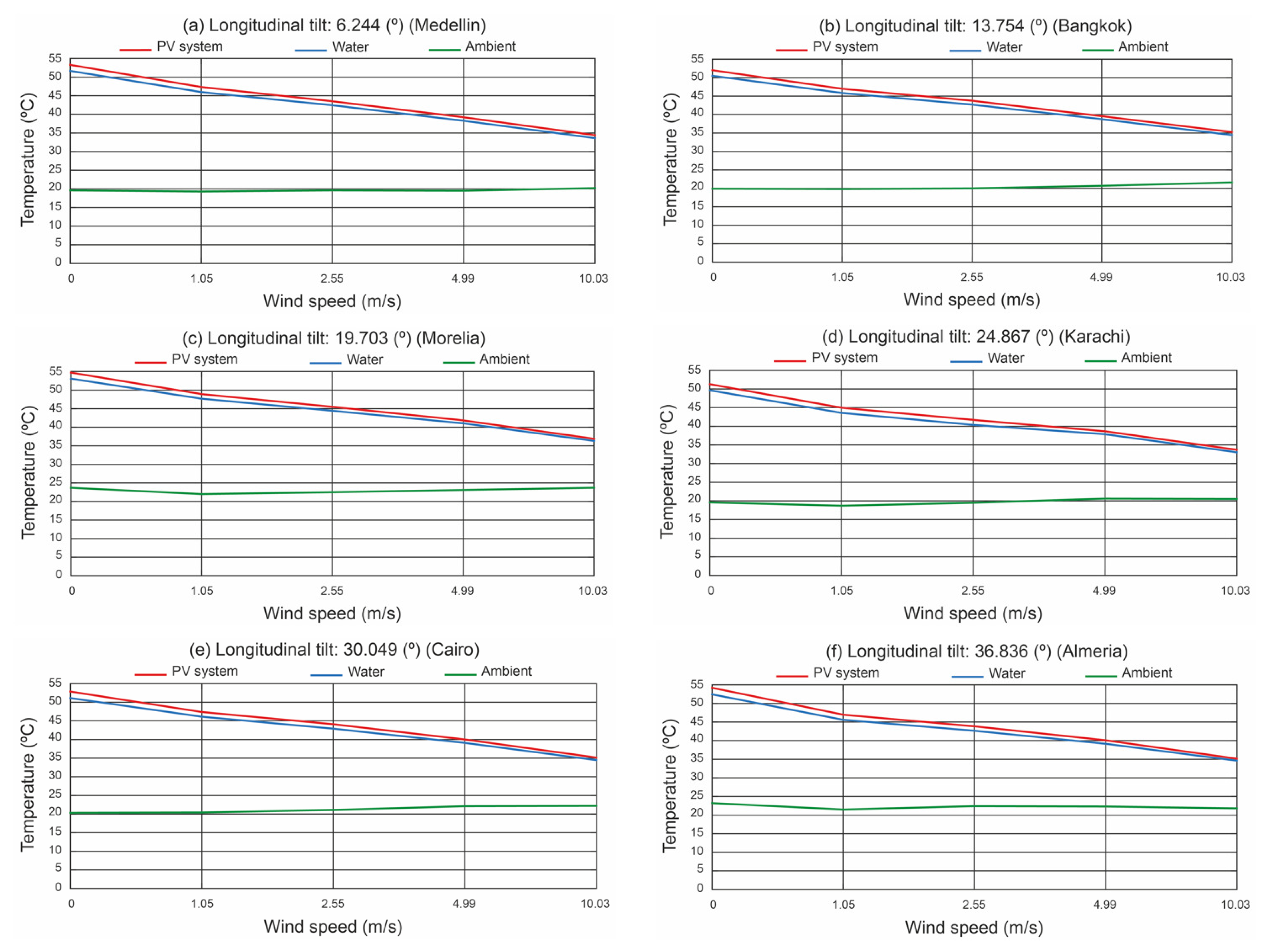
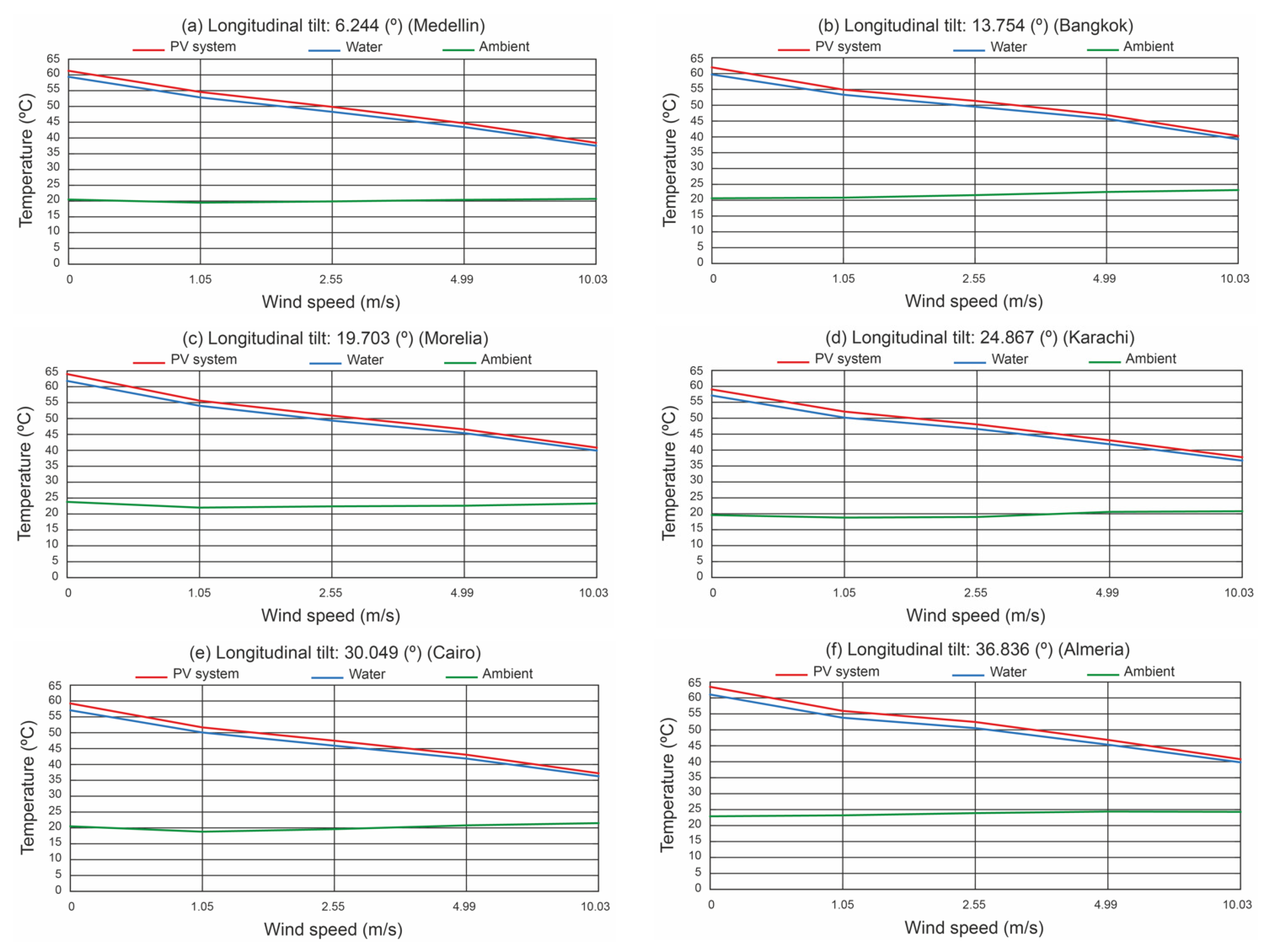
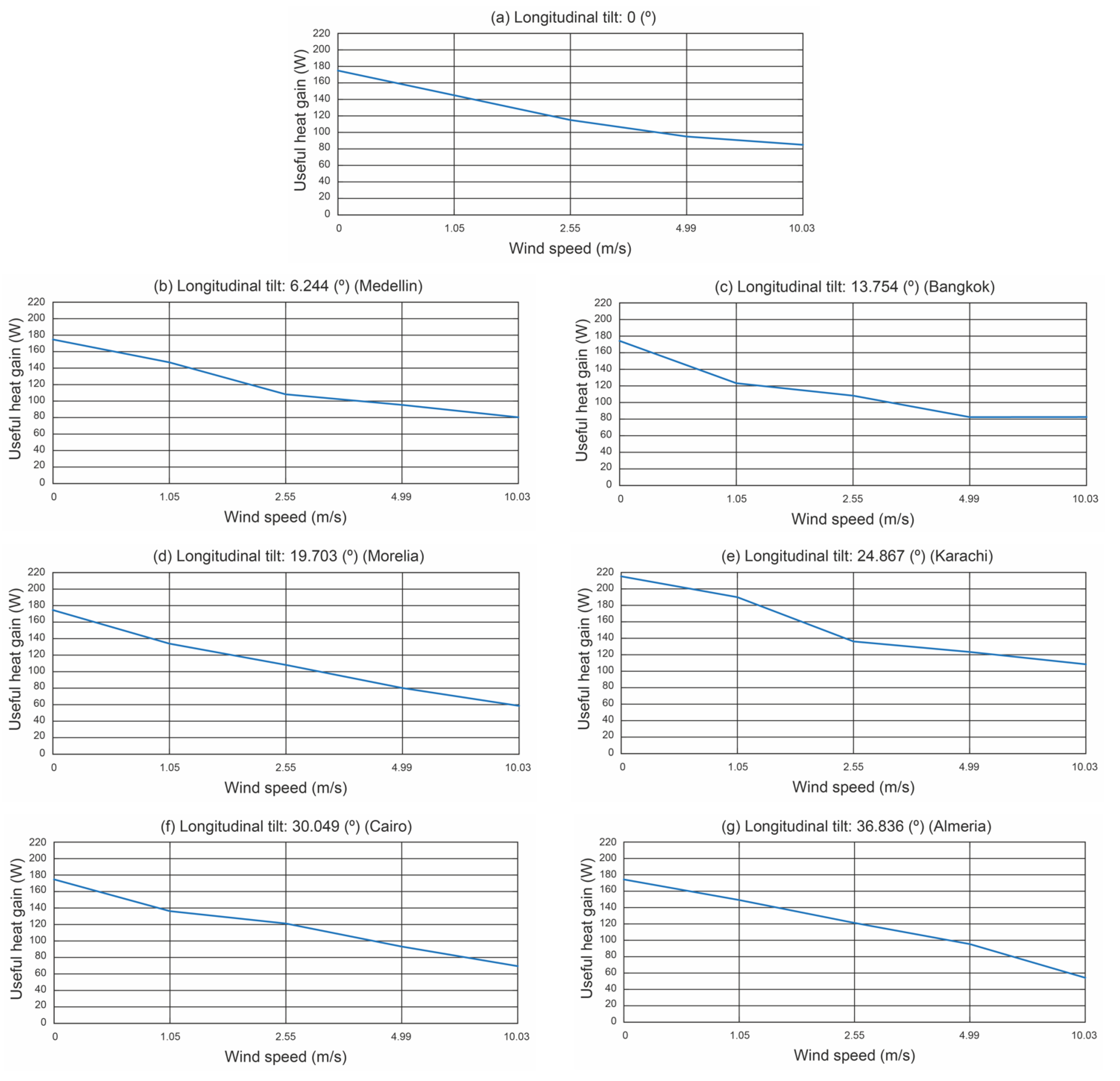
4.1. Effect of the Longitudinal Tilt Angle of the Secondary System on Useful Heat
- (i)
- Useful heat obviously decreases as the wind speed increases. This effect is more noticeable as the longitudinal tilt angle increases.
- (ii)
- When the wind speed is (m/s), the useful heat gain () of the longitudinal tilt configuration is between (Medellin) and (Almeria) with respect to the base configuration. For this wind speed, there is a noticeable difference in UHG between the lower latitude and higher latitude locations.
- (iii)
- If the wind speed is (m/s), the is between (Medellin) and (Almeria). The difference in useful heat gain between the most extreme locations decreases considerably with this wind speed.
- (iv)
- When the wind speed is (m/s), the useful heat gain in the longitudinal tilt configuration is still lower than in the base configuration. is approximately . In this case, the difference in useful heat gain between the most extreme locations is negligible. The same is true for a wind speed of (m/s).
- (v)
- When the wind speed is 0 (m/s), the useful heat gain in the longitudinal tilt configuration is similar to that of the base configuration.
- (vi)
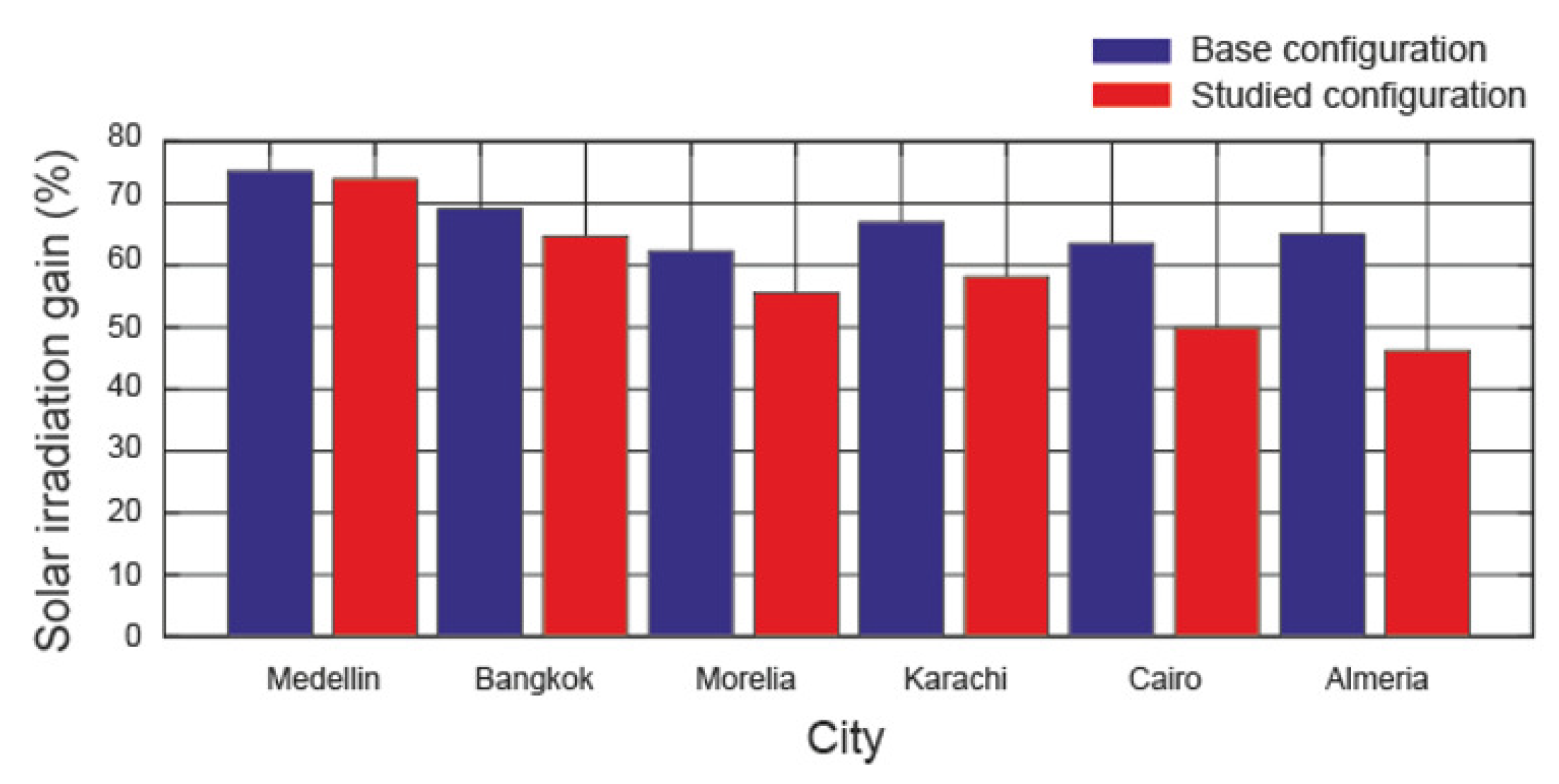
- It can be concluded that the presence of wind is detrimental to the longitudinal tilt configuration at all locations as concerns the useful heat. However, it is important to note that this type of technology also generates electricity. Furthermore, according to Table 5, the longitudinal tilt configuration performs better as concerns incident solar irradiation on the system. This will be assessed in the study below.
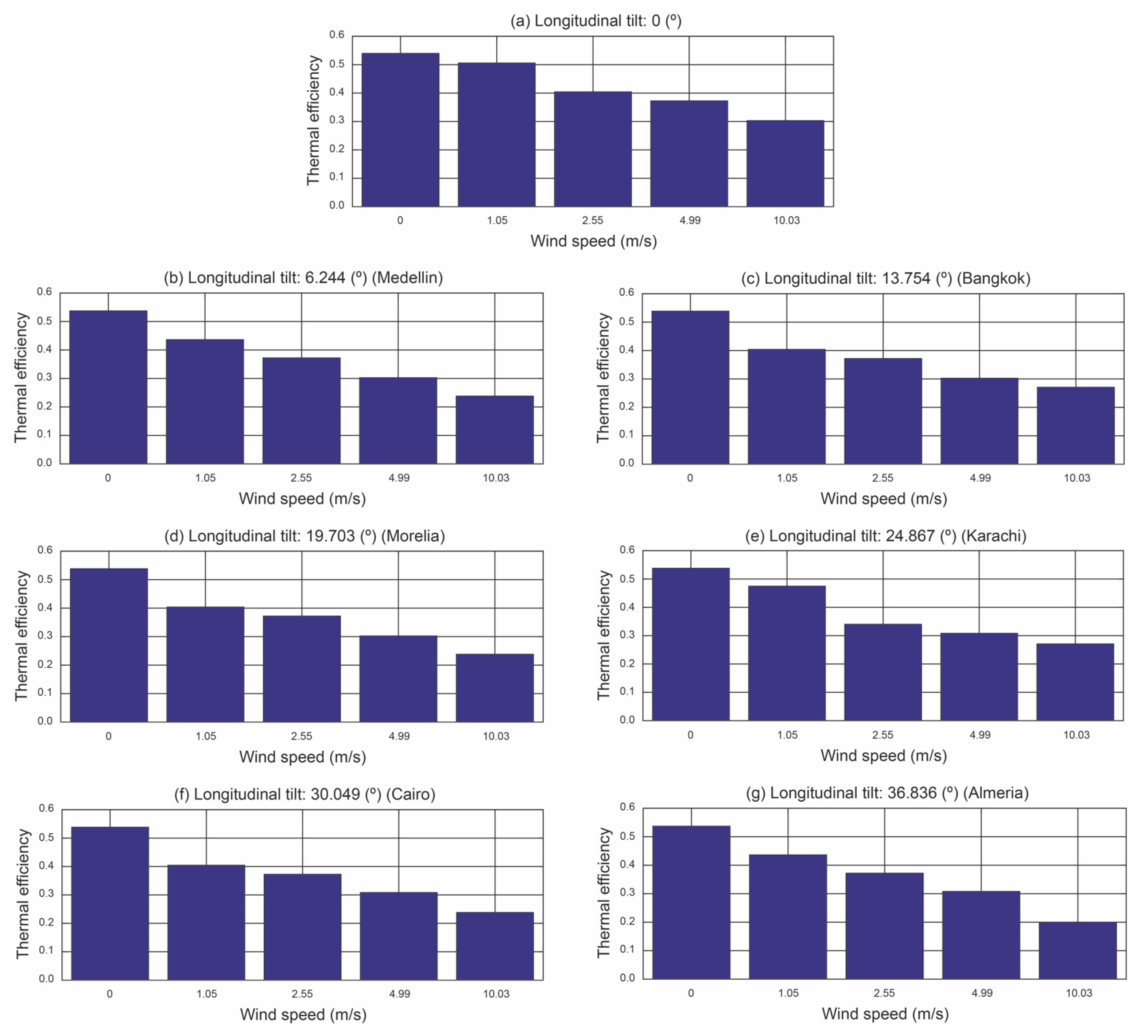
4.2. Effect of the Longitudinal Tilt Angle of the Secondary System on Thermal Efficiency
- (i)
- The trend obtained with useful heat (see Figure 15) is also obviously true for thermal efficiency.
- (ii)
- The thermal efficiency is always below . As wind speed increases, this value decreases. This effect is more pronounced as the longitudinal tilt angle increases.
- (iii)
- When the wind speed is (m/s), the thermal efficiency ranges between and . For wind speeds of (m/s), it ranges between and . For wind speeds of (m/s) and (m/s), the thermal efficiency is between and . This is valid for all locations. The lowest thermal efficiency is obtained in Almeria (location with the highest latitude).
4.3. Effect of the Longitudinal Tilt Angle of the Secondary System on Total Useful Energy Gain
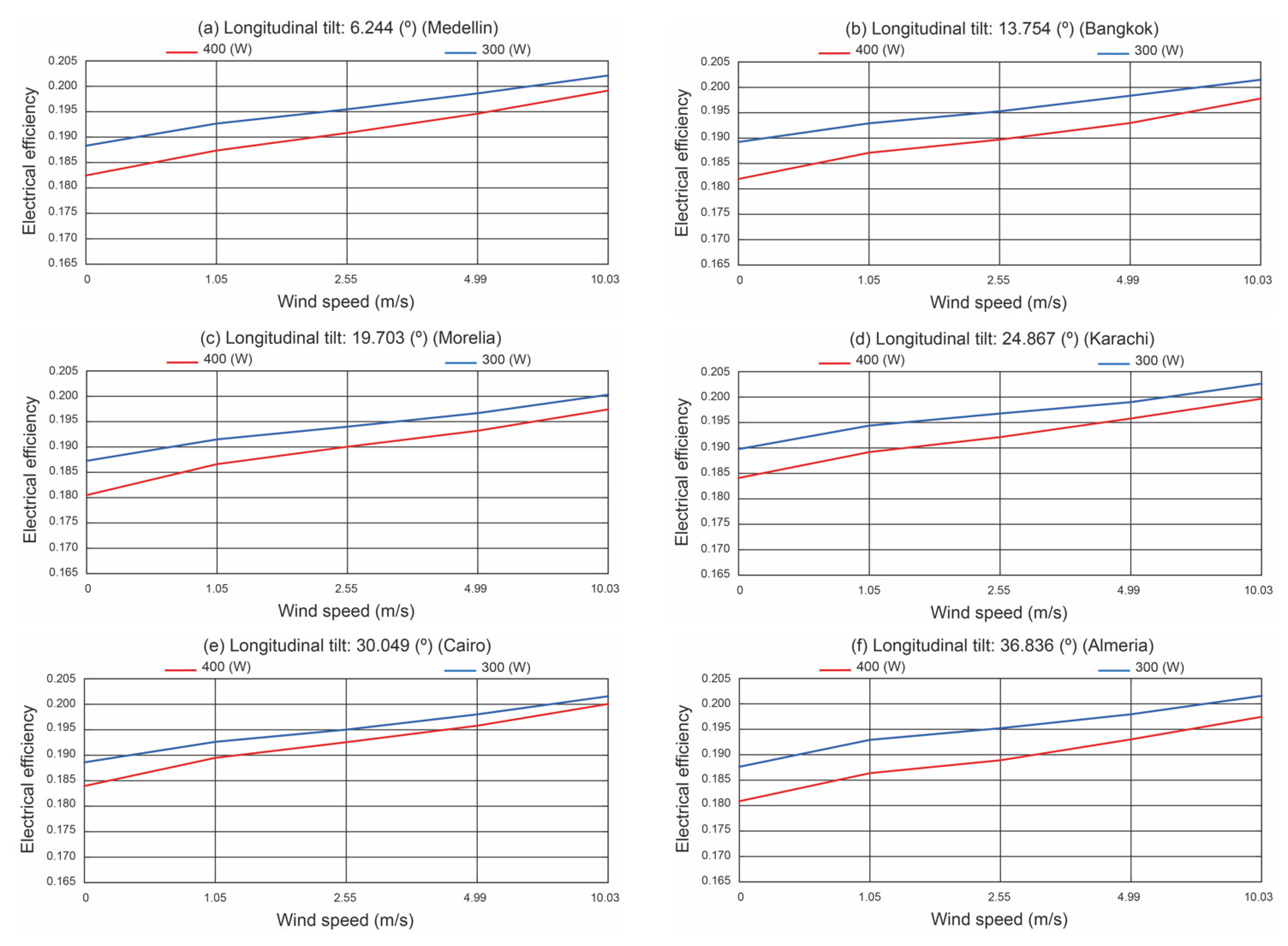
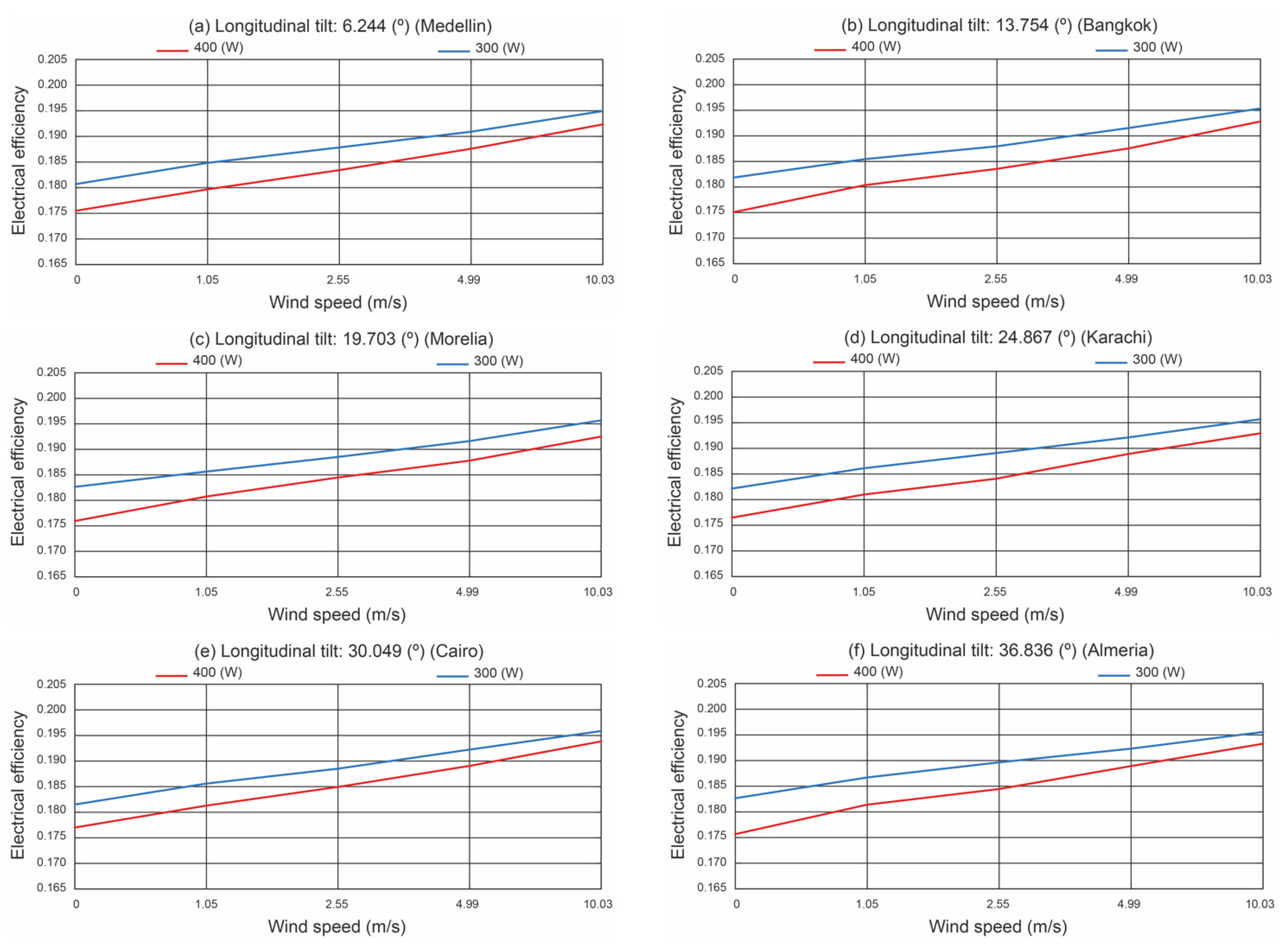
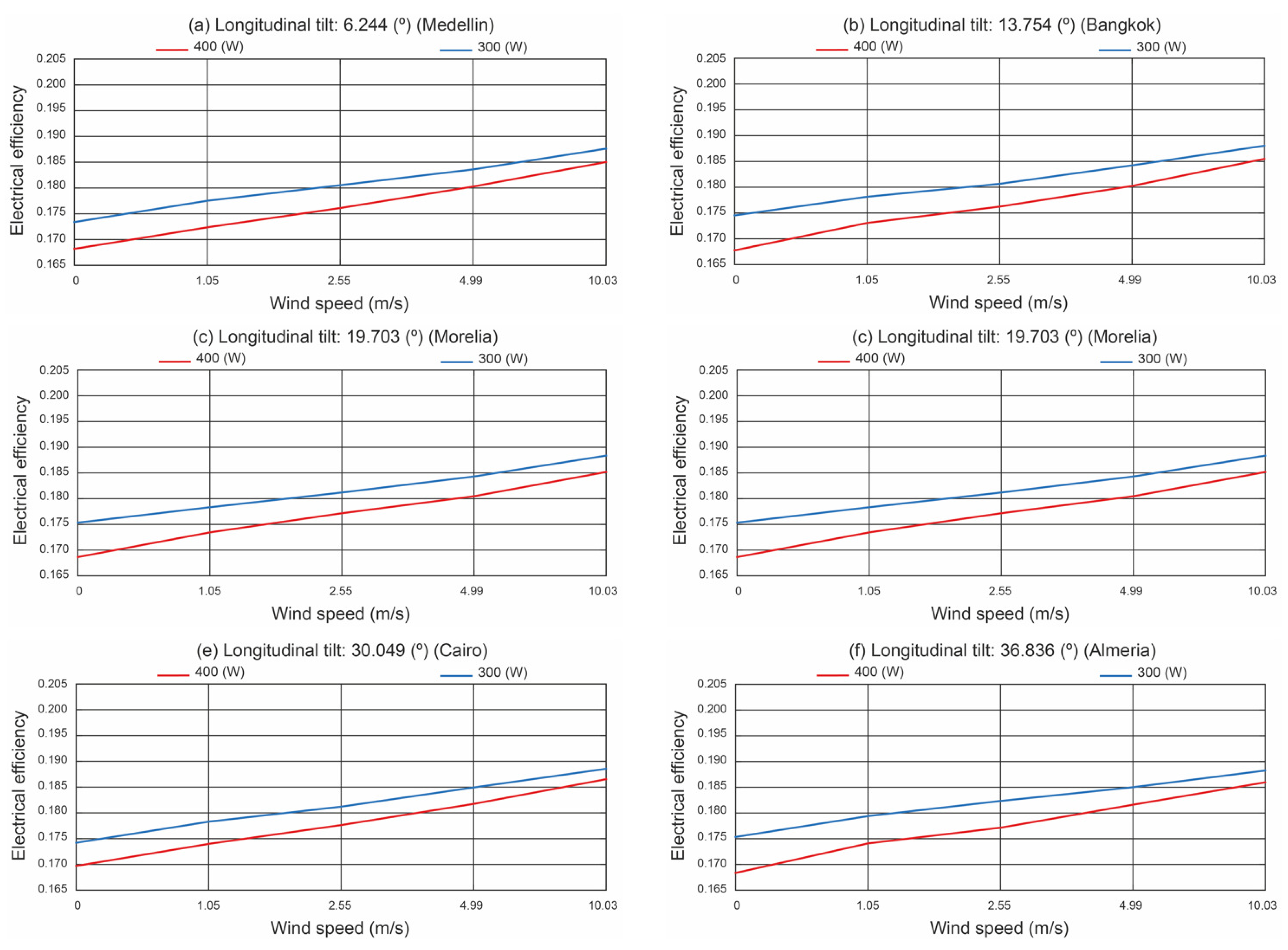
4.4. Effect of Thermal Power on the Electrical Efficiency of the PV System
- (i)
- Electrical efficiency increases as the wind speed increases and as the operating temperature of the cell decreases.
- (ii)
- Electrical efficiency decreases as the thermal power of the system increases and as the incident solar irradiance on the system increases.
- (iii)
- The difference in electrical efficiency for 300 and 400 (W) decreases with increasing wind speed as the operating temperature of the cells decreases.
4.5. Effect of Ambient Temperature on the Electrical Efficiency of the PV System
- (i)
- The electrical efficiency obviously decreases as the ambient temperature increases, since the operating temperature of the cell increases.
- (ii)
- The increase in wind speed dampens the decrease in electrical efficiency, but not enough to offset the increase in ambient temperature.
- (iii)
- Therefore, increasing the ambient temperature decreases the electrical efficiency but increases the thermal efficiency of the system by decreasing the thermal jump between the ambient temperature and the heat source.
4.6. Efficiency Comparison between Concentrating and Nonconcentrating PV Systems
- (i)
- The available roof area of the building was considered to be the same for both technologies. Specifically, the flat roof has a width of (mm) and a length of 658 (mm).
- (ii)
- According to Figure 20, Figure 21 and Figure 22, the electrical efficiency varies with incident solar irradiance, ambient temperature, and wind speed. Figure 11 shows how the ambient temperature varies as a function of the time of day at each location under study. Therefore, the electrical efficiency can take a large number of values. The electrical efficiency of cells was considered to be the same for both technologies, i.e., . The operating temperature of the cells was also assumed to be the same. It can be considered that the losses and remain constant if the solar irradiance remains constant.
- (iii)
- The system has a southern orientation and an optimal tilt angle, as determined by the procedure used in [62].
- (iv)
- The method proposed by [60] was used to determine solar irradiance for both technologies.
5. Conclusions
- (i)
- As concerns useful heat production, the longitudinal tilt configuration performs worse as the longitudinal tilt angle and wind speed increase. For example, the following is true for the power not converted into electricity by the photovoltaic cells of 400 (W): (a) When the wind speed is (m/s), the useful heat gain shows the worst results, ranging from (Medellin) to (Almeria). (b) If the wind speed drops below this value, e.g., (m/s), the useful heat gain also decreases, resulting in useful heat gains between (Medellín) and (Almería). (c) For (m/s), the useful heat gain is approximately . (d) When the wind speed is 0 (m/s), the useful heat in the longitudinal tilt configuration is similar to that of the base configuration. As wind speed increases, the difference in useful heat gain between lower and higher latitude locations increases.
- (ii)
- The thermal efficiency decreased with increasing wind speed and a longitudinal tilt angle. The thermal efficiency was always below . As wind speed increases, this value decreases. This effect is more pronounced as the longitudinal tilt angle increases. For example, the following is true for the power not converted into electricity by the photovoltaic cells of 400 (W): (a) When the wind speed is (m/s), the thermal efficiency ranges between and . (b) For wind speeds of (m/s), it ranges between and . (c) For wind speeds of (m/s) and (m/s), the thermal efficiency is between and . The lowest thermal efficiency is obtained in Almeria (location with the highest latitude).
- (iii)
- The total useful energy in a low-concentration photovoltaic system is the sum of the electrical energy generated and the useful thermal energy produced. The longitudinal tilt configuration shows the best increase in total useful energy in the absence of wind. The lowest latitude site (Medellín) has the lowest increase. On the other hand, for higher latitudes, this total useful energy gain takes values up to (Almeria). The longitudinal tilt configuration remains the best-performing when the wind speed is (m/s), except for the lowest latitude location (Medellin). At this wind speed, the total useful energy gain decreases to for the highest latitude location (Almeria). Therefore, it can be concluded that secondary system designs with longitudinal tilt angles perform well at locations with latitudes above 10 (°). These results are very good if the latitude is higher than 24 (°) and lower than 37 (°). It can be concluded that the effect of the longitudinal tilt of the secondary system has a positive effect.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Area of the flat tube (m2) | |
| Total area of the cells (m2) | |
| Heat capacity of the water (J/kg °C) | |
| Cleanliness factor of the glass () | |
| Cleanliness factor of the mirror () | |
| Hydraulic diameter of the flat tube (m) | |
| Separation between and mirrors (m) | |
| Total useful energy gain () | |
| Total useful energy in a configuration (kWh) | |
| Total useful energy in system (kWh) | |
| Total useful energy in system (kWh) | |
| Overall uncertainty associated with the measurement (%) | |
| f | Height of the receiver (m) |
| Solar irradiation gain () | |
| Beam solar irradiation incident (kWh) | |
| Solar irradiation incident on the cells (kWh) | |
| Solar irradiation incident on the cells (kWh) | |
| Total absorbed solar irradiance for system (W/m2) | |
| k | Thermal conductivity (W/m °C) |
| Position of mirror (m) | |
| Length of the active cooling system (m) | |
| Length of the mirrors (m) | |
| Length of the system (m) | |
| m | Fluid mass flow rate (kg/s) |
| N | Number of mirrors on each side of the SSLFR |
| Prandtl number of the working fluid () | |
| Conduction heat transfer (W) | |
| Convection heat transfer (W) | |
| Radiation heat transfer (W) | |
| Internal heat generation in cells (W) | |
| Useful heat (W) | |
| Reynolds number of the working fluid () | |
| Ambient temperature (°C) | |
| Temperature of the flat tube (°C) | |
| Temperature of the cells (°C) | |
| Temperature of the inlet fluid to the secondary system (°C) | |
| Temperature of the outlet fluid to the secondary system (°C) | |
| Reference temperature (°C) | |
| Working fluid temperature (°C) | |
| Useful heat gain () | |
| Width of the active cooling system (m) | |
| Width of the mirror (m) | |
| Width of the cells (m) | |
| Absorptivity of the cell () | |
| Angle which mirror i forms with the horizontal (°C) | |
| Angle between the mirror axis and the horizontal plane (°C) | |
| Temperature coefficient (1/°C) | |
| Angle between the system and the horizontal plane (°C) | |
| Wall thickness of the flat tube (m) | |
| Electrical efficiency of the system () | |
| Reference electrical efficiency () | |
| Thermal efficiency () | |
| Angle between the vertical and the line centre point of | |
| each mirror with the focal point (°) | |
| Longitudinal incidence angle (°C) | |
| Transversal incidence angle (°C) | |
| Operation interval (°C) | |
| Latitude (°C) | |
| Reflectivity of the primary mirrors () | |
| Transmissivity of encapsulant () | |
| Transmissivity of glass () |
References
- Paris Agreement. In Proceedings of the Report of the Conference of the Parties to the United Nations Framework Convention on Climate Change (21st Session), Paris, Fance, 30 November–13 December 2015.
- Statistical Review of World Energy, 72nd ed.; 2023. Available online: https://www.energyinst.org/statistical-review/resources-and-data-downloads (accessed on 20 June 2023).
- Alzahrani, M.; Shanks, K.; Mallick, T.K. Advances and limitations of increasing solar irradiance for concentrating photovoltaics thermal system. Renew. Sustain. Energy Rev. 2021, 138, 110517. [Google Scholar] [CrossRef]
- IRENA. Future of Solar Photovoltaic: Deployment, Investment, Technology, Grid Integration and Socio-Economic Aspects. International Renewable Energy Agency. 2019. Available online: https://www.irena.org/publications/2019/Nov/Future-of-Solar-Photovoltaic (accessed on 11 June 2023).
- Huang, M.; Wang, Y.; Li, M.; Keovisar, V.; Li, X.; Kong, D.; Yu, Q. Comparative study on energy and exergy properties of solar photovoltaic/thermal air collector based on amorphous silicon cells. Appl. Thermal Eng. 2021, 185, 116376. [Google Scholar] [CrossRef]
- Darkwa, J.; Calautit, J.; Du, D.; Kokogianakis, G. A numerical and experimental analysis of an integrated TEG-PCM power enhancement system for photovoltaic cells. Appl. Energy 2019, 248, 688–701. [Google Scholar] [CrossRef]
- Poulek, V.; Šafránková, J.; Cerná, L.; Libra, M.; Beránek, V.; Finsterle, T.; Hrzina, P. PV panel and PV inverter damages caused by combination of edge delamination, water penetration, and high string voltage in moderate climate. IEEE J. Photovoltaics 2021, 11, 561–565. [Google Scholar] [CrossRef]
- Kamath, H.G.; Ekins-Daukes, N.J.; Araki, K.; Ramasesha, S.K. The potential for concentrator photovoltaics: A feasibility study in India. Prog. Photovoltaics Res. Appl. 2018, 27, 316–327. [Google Scholar] [CrossRef]
- Moreno, A.; Chemisana, D.; Fernández, E.F. Hybrid high-concentration photovoltaic-thermal solar systems for building applications. Appl. Energy 2021, 304, 117647. [Google Scholar] [CrossRef]
- IRENA; IEA. End-of-Life Management: Solar Photovoltaic Panels. International Renewable Energy Agency and International Energy Agency Photovoltaic Power Systems. 2016. Available online: https://www.irena.org/publications/2016/Jun/End-of-life-management-Solar-Photovoltaic-Panels (accessed on 11 June 2023).
- Vellini, M.; Gambini, M.; Stilo, T. High-efficiency cogeneration systems for the food industry. J. Clean. Prod. 2020, 260, 121133. [Google Scholar] [CrossRef]
- Silva, R.M.; Fernandes, J.L.M. Hybrid photovoltaic/thermal (PV/T) solar systems simulation with Simulink/Matlab. Sol. Energy 2010, 84, 1985–1996. [Google Scholar] [CrossRef]
- Tian, M.; Su, Y.; Zheng, H.; Pei, G.; Li, G.; Riffat, S. A review on the recent research progress in the compound parabolic concentrator (CPC) for solar energy applications. Renew. Sustain. Energy Rev. 2018, 82, 1272–1296. [Google Scholar] [CrossRef]
- Barbón, A.; Fortuny Ayuso, P.; Bayón, L.; Fernández-Rubiera, J.A. Non-uniform illumination in low concentration photovoltaic systems based on smallscale linear Fresnel reflectors. Energy 2022, 239, 122217. [Google Scholar] [CrossRef]
- Ghodbane, M.; Said, Z.; Amine Hachicha, A.; Boumeddane, B. Performance assessment of linear Fresnel solar reflector using MWCNTs/DW nanofluids. Renew. Energy 2020, 151, 43–56. [Google Scholar] [CrossRef]
- Wang, G.; Chen, Z.; Hu, P.; Cheng, X. Design and optical analysis of the band-focus Fresnel lens solar concentrator. Appl. Therm. 2016, 102, 695–700. [Google Scholar] [CrossRef]
- Wang, G.; Yao, Y.; Chen, Z.; Hu, P. Thermodynamic and optical analyses of a hybrid solar CPV/T system with high solar concentrating uniformity based on spectral beam splitting technology. Energy 2019, 166, 256–266. [Google Scholar] [CrossRef]
- Wang, K.-J.; Yang, J.-W. Sunlight concentrator design using a revised genetic algorithm. Renew. Energy 2014, 72, 322–335. [Google Scholar] [CrossRef]
- Wang, G.; Wang, F.; Shen, F.; Jiang, T.; Chen, Z.; Hu, P. Experimental and optical performances of a solar CPV device using a linear Fresnel reflector concentrator. Renew. Energy 2020, 146, 2351–2361. [Google Scholar] [CrossRef]
- Wang, G.; Chen, Z.; Hu, P. Design and experimental investigation of a multi-segment plate concentrated photovoltaic solar energy system. Appl. Therm. Eng. 2017, 116, 147–152. [Google Scholar] [CrossRef]
- Jiang, S.; Hu, P.; Mo, S.; Chen, Z. Optical modeling for a two-stage parabolic trough concentrating photovoltaic/thermal system using spectral beam splitting technology. Sol. Energy Mater. Sol. Cells 2010, 94, 1686–1696. [Google Scholar] [CrossRef]
- Barbón, A.; Barbón, N.; Bayón, L.; Sánchez-Rodríguez, J.A. Parametric study of the small-scale linear Fresnel reflector. Renew. Energy 2018, 116, 64–74. [Google Scholar] [CrossRef]
- Barbón, A.; Bayón-Cueli, C.; Bayón, L.; Rodríguez, L. Investigating the influence of longitudinal tilt angles on the performance of small scale linear Fresnel reflectors for urban applications. Renew. Energy 2019, 143, 1581–1593. [Google Scholar] [CrossRef]
- Barbón, A.; Bayón-Cueli, C.; Fernández-Rubiera, J.A.; Bayón, L. Theoretical deduction of the optimum tilt angles for small-scale linear Fresnel reflectors. Energies 2021, 14, 2883. [Google Scholar] [CrossRef]
- Barbón, A.; López-Smeetz, C.; Bayón, L.; Pardellas, A. Wind effects on heat loss from a receiver with longitudinal tilt angle of small-scale linear Fresnel reflectors for urban applications. Renew. Energy 2020, 162, 2166–2181. [Google Scholar] [CrossRef]
- Barbón, A.; Fernández-Rubiera, J.A.; Martínez-Valledor, L.; Pérez-Fernández, A.; Bayón, L. Design and construction of a solar tracking system for small-scale linear Fresnel reflector with three movements. Appl. Energy 2021, 285, 116477. [Google Scholar] [CrossRef]
- Morin, G.; Dersch, J.; Platzer, W.; Eck, M.; Häberle, A. Comparison of linear Fresnel and parabolic trough collector power plants. Sol. Energy 2012, 86, 1–12. [Google Scholar] [CrossRef]
- Barbón, A.; Bayón, L.; Bayón-Cueli, C.; Barbón, N. A study of the effect of the longitudinal movement on the performance of small-scale linear Fresnel reflectors. Renew. Energy 2019, 138, 128–138. [Google Scholar] [CrossRef]
- Li, G.; Xuan, Q.; Pei, G.; Su, Y.; Ji, J. Effect of non-uniform illumination and temperature distribution on concentrating solar cell—A review. Energy 2018, 144, 1119–1136. [Google Scholar] [CrossRef]
- Guerriero, P.; Tricoli, P.; Daliento, S. A bypass circuit for avoiding the hot spot in PV modules. Sol. Energy 2019, 181, 430–438. [Google Scholar] [CrossRef]
- Shi, S.; Zhang, D.; Bi, L.; Ding, R.; Ren, W.; Tang, X.; He, Y. Enhancement of thermal conductivity and insulation of silicone thermal interface material through surface modification and synergistic effects of nanofillers for thermal management of electronic devices. Diam. Relat. Mater. 2024, 145, 111064. [Google Scholar] [CrossRef]
- RD. Available online: https://es.rs-online.com/web/p/grasa-termica/0554311 (accessed on 15 April 2024).
- Zhu, Y.; Shi, J.; Li, Y.; Wang, L.; Huang, Q.; Xu, G. Design and thermal performances of a scalable linear Fresnel reflector solar system. Energy Convers. Manag. 2017, 146, 174–181. [Google Scholar] [CrossRef]
- Zhu, Y.; Shi, J.; Li, Y.; Wang, L.; Huang, Q.; Xu, G. Design and experimental investigation of a stretched parabolic linear Fresnel reflector collecting system. Energy Convers. Manag. 2016, 126, 89–98. [Google Scholar] [CrossRef]
- Barbón, A.; Sánchez-Rodríguez, J.A.; Bayón, L.; Bayón-Cueli, C. Cost estimation relationships of a small scale linear Fresnel reflector. Renew. Energy 2019, 134, 1273–1284. [Google Scholar] [CrossRef]
- Fernández-Rubiera, J.A.; Barbón, A.; Bayón, L.; Ghodbane, M. Sawtooth V-trough cavity for low concentration photovoltaic systems based on small-scale linear Fresnel reflectors: Optimal design, verification and construction. Electronics 2023, 12, 2770. [Google Scholar] [CrossRef]
- Duffie, J.A.; Beckman, W.A. Solar Engineering of Thermal Processes, 4th ed.; John Wiley & Sons: New York, NY, USA, 2013. [Google Scholar]
- Sharma, V.M.; Nayak, J.K.; Kedare, S.B. Effects of shading and blocking in linear Fresnel reflector field. Sol. Energy 2015, 113, 114–138. [Google Scholar] [CrossRef]
- Theunissen, P.H.; Beckman, W.A. Solar transmittance characteristics of evacuated tubular collectors with diffuse back reflectors. Sol. Energy 1985, 35, 311–320. [Google Scholar] [CrossRef]
- Kohan, H.R.F.; Lotfipour, F.; Eslami, M. Numerical simulation of a photovoltaic thermoelectric hybrid power generation system. Sol. Energy 2018, 174, 537–548. [Google Scholar] [CrossRef]
- Giffith, B.; Torcellini, P.; Long, N. Assessment of the Technical Potential for Achieving Zero-Energy Commercial Buildings; ACEEE Summer Study Pacific Grove; National Renewable Energy Lab (NREL): Golden, CO, USA, 2006. [Google Scholar]
- ISO 9806:2017; Solar Energy—Solar Thermal Collectors—Test Methods. ISO: Geneva, Switzerland, 2017.
- Herrando, M.; Ramos, A.; Zabalza, I.; Markides, C.N. A comprehensive assessment of alternative absorber-exchanger designs for hybrid PVT-water collectors. Appl. Energy 2019, 235, 1583–1602. [Google Scholar] [CrossRef]
- Kalogirou, S.A.; Agathokleous, R.; Barone, G.; Buonomano, A.; Forzano, C.; Palombo, A. Development and validation of a new TRNSYS Type for thermosiphon flat-plate solar thermal collectors: Energy and economic optimization for hot water production in different climates. Renew. Energy 2019, 136, 632–644. [Google Scholar] [CrossRef]
- MATRIX. Available online: https://www.ujaen.es/departamentos/ingele/sites/departamento_ingele/files/uploads/manualwatimetrodigitalPX110.PDF (accessed on 18 August 2023).
- TESTO. Available online: https://www.testo.com/en/ (accessed on 18 August 2023).
- AICHITOKEI. Available online: https://www.aichitokei.net/products/microflow-sensor-of-z/ (accessed on 18 August 2023).
- Li, M.; Zhang, Q.; Li, G.; Shao, S. Experimental investigation on performance and heat release analysis of a pilot ignited direct injection natural gas engine. Energy 2015, 90, 1251–1260. [Google Scholar] [CrossRef]
- Rahman, M.M.; Hamada, K.I.; Aziz, A.R.A. Characterization of the timeaveraged overall heat transfer in a direct-injection hydrogen-fueled engine. Int. J. Hydrog. Energy 2013, 38, 4816–4830. [Google Scholar] [CrossRef]
- Al-Waeli, A.H.A.; Chaichan, M.T.; Sopian, K.; Kazem, H.A.; Mahood, H.B.; Khadom, A.A. Modeling and experimental validation of a PVT system using nanofluid coolant and nano-PCM. Sol. Energy 2019, 177, 178–191. [Google Scholar] [CrossRef]
- UN. World Population Prospects 2019. United Nations 2019. Available online: https://population.un.org/wpp/ (accessed on 6 March 2023).
- Odyssee-Mure, Energy Efficiency Trends in Buildings in the EU Lessons from the ODYSSEE MURE Project. 2015. Available online: http://www.odyssee-mure.eu/publications/br/energy-efficiency-trends-policies-buildings.pdf (accessed on 6 March 2021).
- SOLARGIS. Available online: https://solargis.com/maps-and-gis-data/download/world (accessed on 20 June 2023).
- PVGIS. Joint Research Centre (JRC). Available online: http://re.jrc.ec.europa.eu/pvg_tools/en/tools.html#PVP (accessed on 11 June 2023).
- Aly, S.P.; Ahzi, S.; Barth, N.; Abdallah, A. Using energy balance method to study the thermal behavior of PV panels under time-varying field conditions. Energy Convers. Manag. 2018, 175, 246–262. [Google Scholar] [CrossRef]
- Cengel, Y.A. Heat Transfer and Mass Transfer: A Practical Approach, 3rd ed.; McGraw Hill Book Company: New York, NY, USA, 2006. [Google Scholar]
- Guarracino, I.; Mellor, A.; Ekins-Daukes, N.J.; Markides, C.N. Dynamic coupled thermal-and-electrical modelling of sheet-and-tube hybrid photovoltaic/thermal (PVT) collectors. Appl. Therm. Eng. 2016, 101, 778–795. [Google Scholar] [CrossRef]
- Evans, D.L. Simplified method for predicting photovoltaic array output. Sol. Energy 1981, 27, 555–560. [Google Scholar] [CrossRef]
- Mills, A.F. Heat Transfer, 2nd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2005. [Google Scholar]
- Barbón, A.; Fortuny Ayuso, P.; Bayón, L.; Fernández-Rubiera, J.A. Predicting beam and diffuse horizontal irradiance using Fourier expansions. Renew. Energy 2020, 154, 46–57. [Google Scholar] [CrossRef]
- Mattei, M.; Notton, G.; Cristofari, C.; Muselli, M.; Poggi, P. Calculation of the polycrystalline PV module temperature using a simple method of energy balance. Renew. Energy 2006, 31, 553–567. [Google Scholar] [CrossRef]
- Jacobson, M.Z.; Jadhav, V. World estimates of PV optimal tilt angles and ratios of sunlight incident upon tilted and tracked PV panels relative to horizontal panels. Sol. Energy 2018, 169, 55–66. [Google Scholar] [CrossRef]







| Mirror | (mm) | (mm) |
|---|---|---|
| 1 | ||
| 2 | ||
| 3 | ||
| 4 | ||
| 5 | 0 | |
| 6 | ||
| 7 | ||
| 8 | ||
| 9 |
| Parameter | Apparatus | Specifications |
|---|---|---|
| Wind speed | Testo 400 | Measurement range: 0/40 (m/s) Precision/resolution: 0.5 (%) |
| Pitot tube | Measurement range: 0/40 (m/s) Uncertainty: 0.5 (%) | |
| Ambient temperature | Testo 400 | Measurement range: −40/150 (°C) Precision/resolution: 0.1 (°C) |
| Thermocouple | Measurement range: −40/150 (°C) Uncertainty: 0.1 (°C) | |
| Flow rate | AICHITOKEI | Measurement range: 0.35/5 (L/min) Precision/resolution: 2 (%) |
| Water temperature | Thermocouple | Measurement range: 0/85 (°C) Uncertainty: 0.0625 (°C) |
| Electrical power | PX120 | Measurement range: 0/1000 (W) Precision/resolution: 1 (%) |
| Parameter | Parameter Uncertainty (%) |
|---|---|
| Wind speed | 0.7% |
| Ambient temperature | 0.5% |
| Flow rate | 2% |
| Water temperature | 0.5% |
| Electrical power | 1% |
| Cities | Latitude | Longitude | Altitude | |
|---|---|---|---|---|
| 1 | Medellin (Colombia) | 06°14′38″ N | 75°34′04″ W | 1469 (m) |
| 2 | Bangkok (Thailand) | 13°45′14″ N | 100°29′34″ E | 9 (m) |
| 3 | Morelia (Mexico) | 19°42′10″ N | 101°11′24″ W | 1921 (m) |
| 4 | Karachi (Pakistan) | 24°52′01″ N | 67°01′51″ E | 14 (m) |
| 5 | Cairo (Egypt) | 30°29′24″ N | 31°14′38″ W | 41 (m) |
| 6 | Almeria (Spain) | 36°50′07″ N | 02°24′08″ W | 22 (m) |
| Cities | (kWh) | (kWh) | (%) | |
|---|---|---|---|---|
| 1 | Medellin (Colombia) | |||
| 2 | Bangkok (Thailand) | |||
| 3 | Morelia (Mexico) | |||
| 4 | Karachi (Pakistan) | |||
| 5 | Cairo (Egypt) | |||
| 6 | Almeria (Spain) |
| Cities | Optimum Tilt Angle (°) | (kWh) | |
|---|---|---|---|
| 1 | Medellin (Colombia) | ||
| 2 | Bangkok (Thailand) | ||
| 3 | Morelia (Mexico) | ||
| 4 | Karachi (Pakistan) | ||
| 5 | Cairo (Egypt) | ||
| 6 | Almeria (Spain) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
López-Smeetz, C.; Barbón, A.; Bayón, L.; Bayón-Cueli, C. Experimental Investigation of the Influence of Longitudinal Tilt Angles on the Thermal Performance of a Small-Scale Linear Fresnel Reflector. Appl. Sci. 2024, 14, 3666. https://doi.org/10.3390/app14093666
López-Smeetz C, Barbón A, Bayón L, Bayón-Cueli C. Experimental Investigation of the Influence of Longitudinal Tilt Angles on the Thermal Performance of a Small-Scale Linear Fresnel Reflector. Applied Sciences. 2024; 14(9):3666. https://doi.org/10.3390/app14093666
Chicago/Turabian StyleLópez-Smeetz, Carmen, Arsenio Barbón, Luis Bayón, and Covadonga Bayón-Cueli. 2024. "Experimental Investigation of the Influence of Longitudinal Tilt Angles on the Thermal Performance of a Small-Scale Linear Fresnel Reflector" Applied Sciences 14, no. 9: 3666. https://doi.org/10.3390/app14093666
APA StyleLópez-Smeetz, C., Barbón, A., Bayón, L., & Bayón-Cueli, C. (2024). Experimental Investigation of the Influence of Longitudinal Tilt Angles on the Thermal Performance of a Small-Scale Linear Fresnel Reflector. Applied Sciences, 14(9), 3666. https://doi.org/10.3390/app14093666






