Application of Unsteady Fluid Flow Simulation in the Process of Regulating an Industrial Hydraulic Network
Abstract
1. Introduction
2. Mathematical Model
- The inside diameter of the pipeline is free of any internal incrustation;
- With regard to the pressure differences, the pipe expansion is neglected, and the pipes are regarded as perfectly rigid;
- The values of local losses caused by hydraulic components (valves, check valves, etc.) are known.
2.1. A Model for Calculating the Pipeline Parameters through an Experiment
2.2. Application of the Analytical Approach to the Regulation of the Hydraulic System
3. Results and Discussion
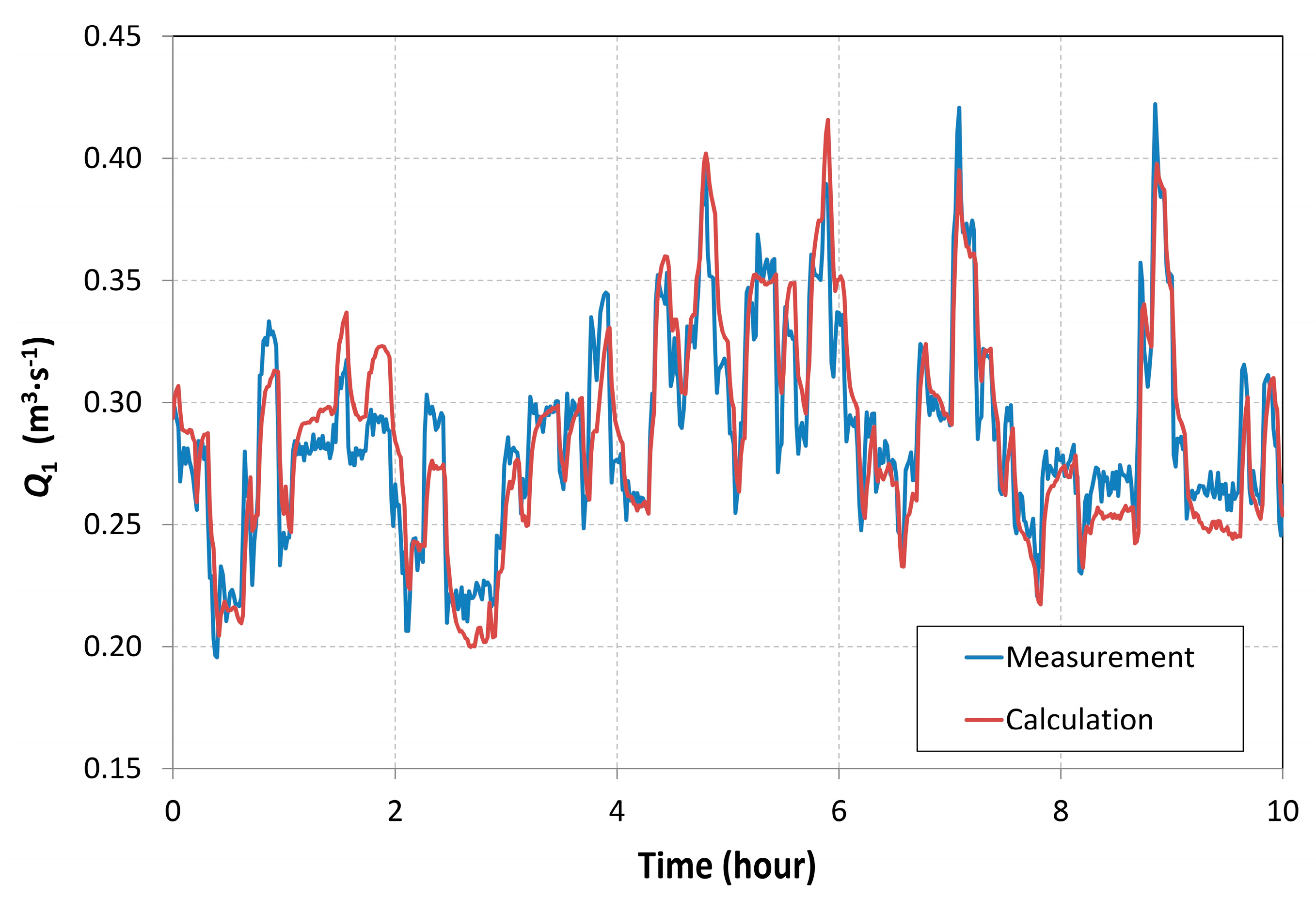
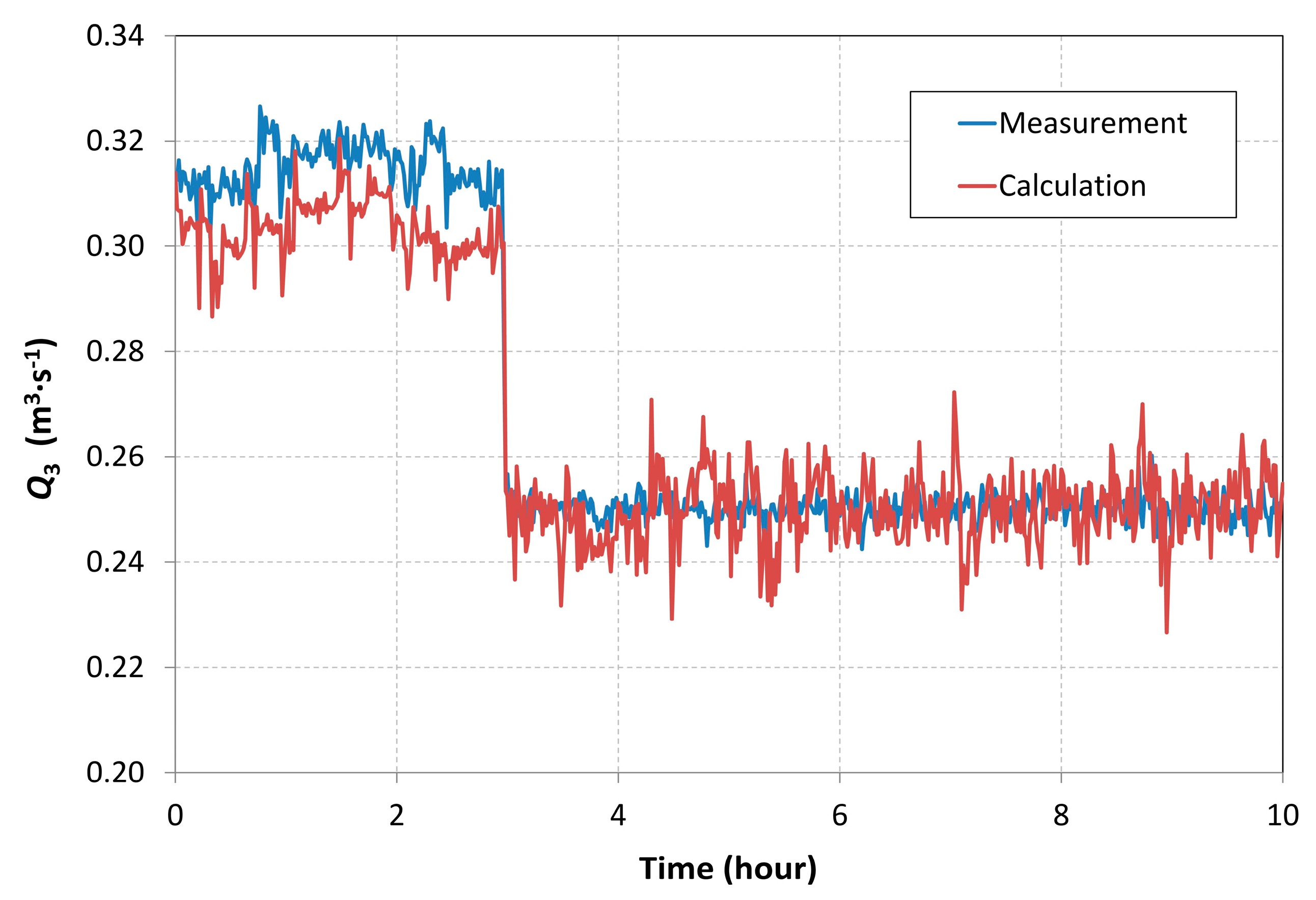
3.1. A Comparison of the Experimental Data and the Model
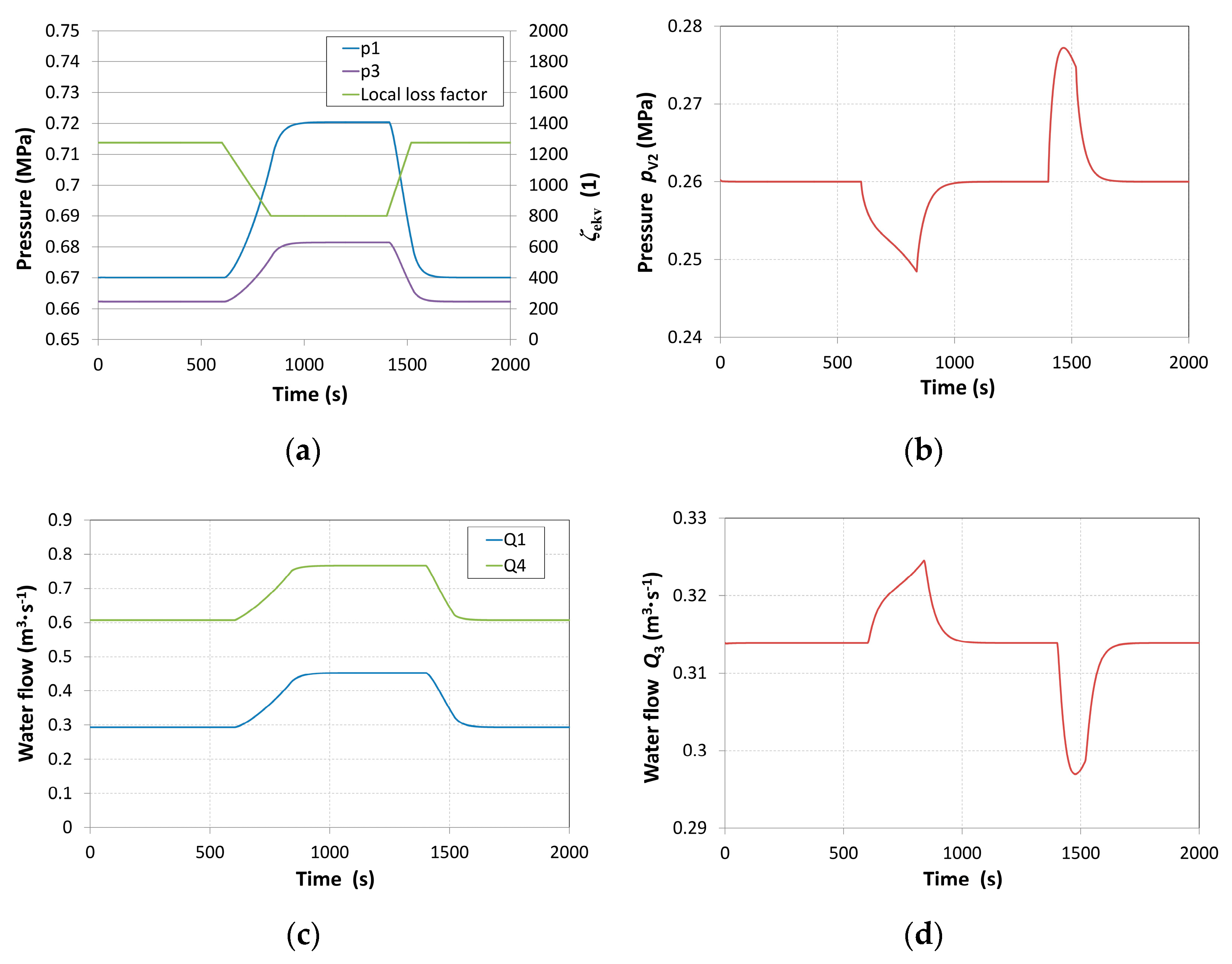
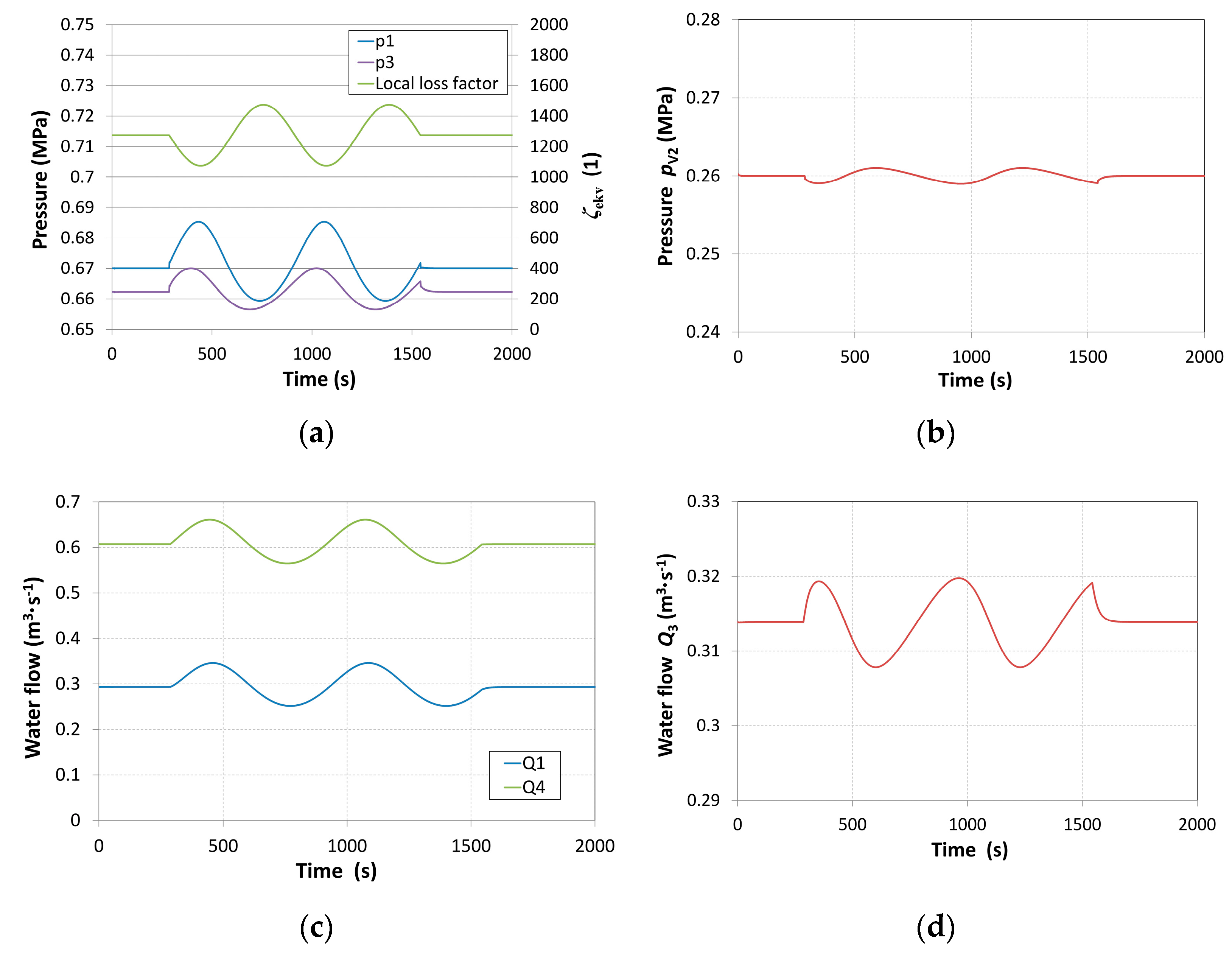
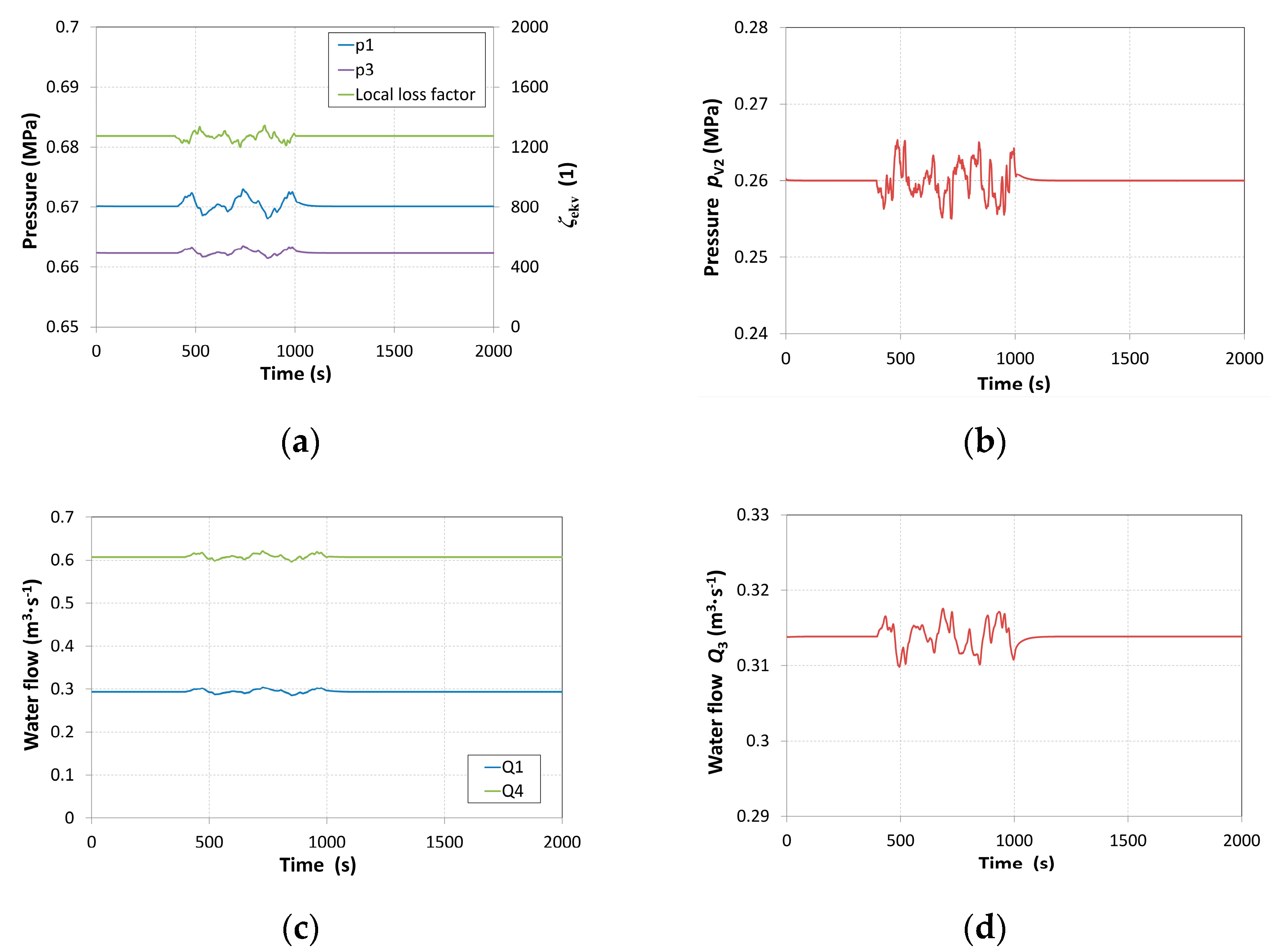
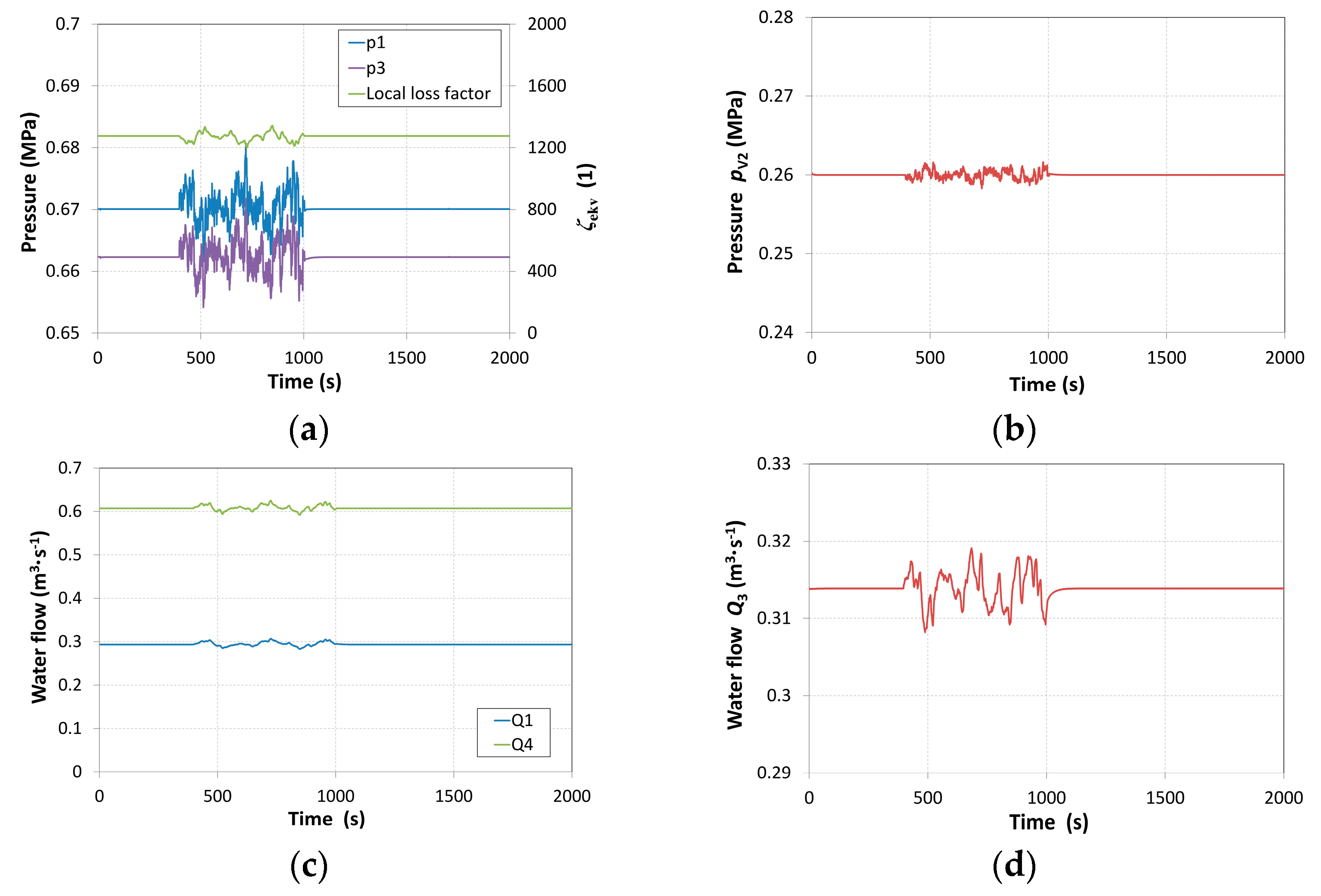
3.2. A Regulation of the Hydraulic System through the Defined Mathematical Model
- ➢
- Model Example 1: constant increasing of the flow rate, subsequent stabilising and then reducing the flow rate to the initial value;
- ➢
- Model Example 2: a sine curve of the output flow rate;
- ➢
- Model Example 3: a stochastic curve of the output flow rate.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sivakumar, P.; Prasad, R.K. Simulation of Water Distribution Network under Pressure-Deficient Condition. Water. Resour. Manag. 2014, 28, 3271–3290. [Google Scholar] [CrossRef]
- Jinesh Babu, K.S.; Mohan, S. Extended Period Simulation for Pressure-Deficient Water Distribution Network. J. Comput. Civ. Eng. 2012, 26, 498–505. [Google Scholar] [CrossRef]
- Jablonská, J. The Dynamic Behavior of the Flow in the Pipeline with Oil. Acta Mech. Slovaca 2017, 21, 40–45. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, M.; Samanta, S.; Ukamanal, M.; Pradhan, A.R.; Prasad, S.B. Simulations of water flow in a horizontal and 900 pipe bend. Mat. Today Proc. 2022, 56, 889–895. [Google Scholar] [CrossRef]
- Wagner, J.M.; Shamir, U.; Marks, D.H. Water Distribution Reliability: Simulation Methods. J. Water Resour. Plann. Manag. 1988, 114, 276–294. [Google Scholar] [CrossRef]
- Lukáč, P.; Horbaj, P.; Sedláková, J. Options to Evaluate the Effects of a Change in the Shape of the Flow Cross-Section of a Ball Valve on Its Flow Control Properties. Acta Mech. Slovaca 2014, 18, 38–43. [Google Scholar] [CrossRef]
- Vachová, L. Sestavení Hydraulického Modelu a Analýza Tlakových Poměrů Vodovodní Sítě Městské Části Praha Přední Kopanina. Master’s Thesis, Faculty of Civil Engineering CTU, Prague, Czech Republic, 2020. [Google Scholar]
- Sohaili, J.; Shi, H.S.; Zardari, N.H.; Ahmad, N.; Muniyandi, S.K. Removal of scale deposition on pipe walls by using magnetic field treatment and the effects of magnetic strength. J. Clean. 2016, 139, 1393–1399. [Google Scholar] [CrossRef]
- Blejchař, T.; Drábková, S.; Janus, V. Numerical Modelling of Flow in Fluidic Oscillator. Acta Mech. Slovaca 2023, 21, 40–47. [Google Scholar] [CrossRef]
- Mičko, P.; Kapjor, A.; Holubčík, M.; Hečko, D. Experimental Verification of CFD Simulation When Evaluating the Operative Temperature and Mean Radiation Temperature for Radiator Heating and Floor Heating. Processes 2021, 9, 1041. [Google Scholar] [CrossRef]
- Brestovič, B.; Jasminská, N.; Lázár, M.; Bednárová, Ľ. Numerical Simulation of Cooling the Adsorbent during Hydrogen Storage Process at 77 K. Acta Mech. Slovaca 2017, 21, 18–24. [Google Scholar] [CrossRef]
- Ghostine, R.; Vazquez, J.; Terfous, A.; Mose, R.; Ghenaim, A. Comparative study of 1D and 2D flow simulations at open-channel junctions. J. Hydr. Res. 2012, 50, 164–170. [Google Scholar] [CrossRef]
- Ferrari, M.; Bonzanini, A.; Poesio, P. A five-equation, transient, hyperbolic, one-dimensional model for slug capturing in pipes. Int. J. Numer. Meth. Fluids 2016, 85, 2–60. [Google Scholar]
- Bonzanini, A.; Picchi, D.; Ferrari, M.; Poesio, P. Velocity profiles description and shape factors inclusion in a hyperbolic, one-dimensional, transient two-fluid model for stratified and slug flow simulations in pipes. Petroleum 2019, 5, 191–198. [Google Scholar] [CrossRef]
- Ezzeldin, R.; Zelenakova, M.; Abd-Elhamid, H.F.; Pietrucha-Urbanik, K.; Elabd, S. Hybrid Optimization Algorithms of Firefly with GA and PSO for the Optimal Design of Water Distribution Networks. Water 2023, 15, 1906. [Google Scholar] [CrossRef]
- Studziński, A.; Pietrucha-Urbanik, K. Failure risk analysis of water distributions systems using hydraulic models on real field data. Ekon. I Sr. 2019, 1, 152–165. [Google Scholar]
- Cooper, J.P. M32 Computer Modeling of Water Distribution Systems; American Water Works Association: Denver, CO, USA, 2017. [Google Scholar]
- Branco, R.L.F.C.; Carneiro, J.N.E.; Nieckele, A.O. A model for the momentum flux parameters of the 1D Two-Fluid Model in vertical annular flows: Linear stability and numerical analysis. Int. J. Multiphase Flow 2023, 168, 104563. [Google Scholar] [CrossRef]
- Črnjarić-Žic, N.; Mujaković, N. Numerical analysis of the solutions for 1d compressible viscous micropolar fluid flow with different boundary conditions. Math. Comp. Sim. 2016, 126, 45–62. [Google Scholar] [CrossRef]
- Creaco, E.; Campisano, A.; Franchini, M.; Modica, C. Unsteady Flow Modeling of Pressure Real-Time Control in Water Distribution Networks. J. Water Resour. Plann. Manag. 2017, 143, 04017056. [Google Scholar] [CrossRef]
- Pop, I.; Grosan, T.; Revnic, C.; Rosca, A.V. Unsteady flow and heat transfer of nanofluids, hybrid nanofluids, micropolar fluids and porous media: A review. Therm. Sci. Eng. Prog. 2023, 46, 102248. [Google Scholar] [CrossRef]
- Moyers-González, M.; Castelain, C.; Burghelea, T. Numerical study of unsteady pipe flow of an elastoviscoplastic fluid. J. Non-Newt. Fluid Mech. 2022, 309, 104898. [Google Scholar] [CrossRef]
- Prandtl, L.; Tietjens, O.G. Fundamentals of Hydro- and Aeromechanics; Dover Publications: Mineola, NY, USA, 1934. [Google Scholar]
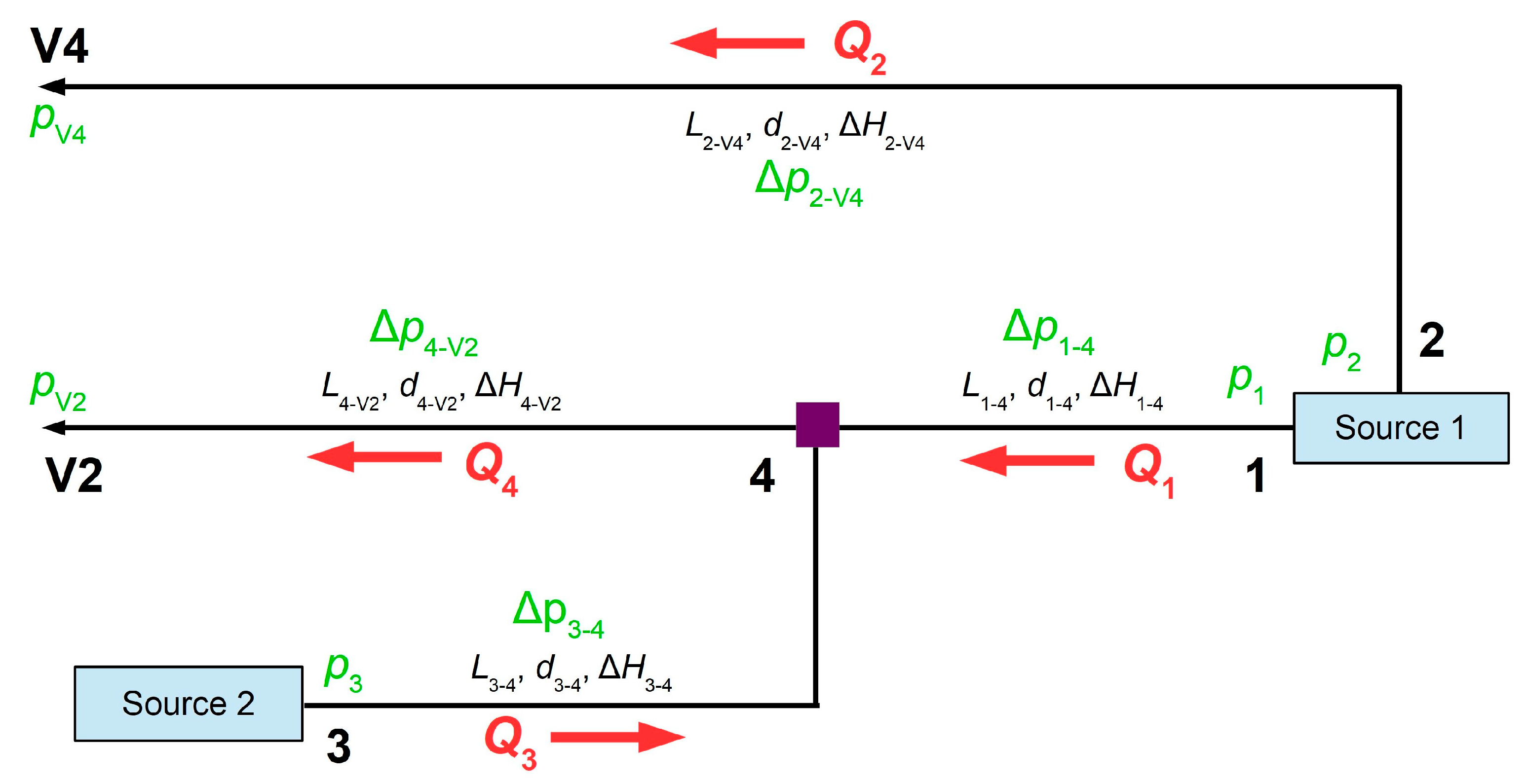




| Branch elevation | ||||
| Pipe diameter | ||||
| Pipe length |
| k1 | k2 | k3 | k4 | k5 | k6 | k7 | k8 |
|---|---|---|---|---|---|---|---|
| 262,251 | 232,853 | 87,275 | 122,502 | 1,190,691 | 130,343 | 292,535 | 334,896 |
| Q1 (m3·s−1) | Q2 (m3·s−1) | Q3 (m3·s−1) |
|---|---|---|
| 0.294 | 0.53 | 0.314 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fil’o, M.; Brestovič, T.; Lázár, M.; Jasminská, N.; Dobáková, R.; Kender, Š. Application of Unsteady Fluid Flow Simulation in the Process of Regulating an Industrial Hydraulic Network. Appl. Sci. 2024, 14, 2393. https://doi.org/10.3390/app14062393
Fil’o M, Brestovič T, Lázár M, Jasminská N, Dobáková R, Kender Š. Application of Unsteady Fluid Flow Simulation in the Process of Regulating an Industrial Hydraulic Network. Applied Sciences. 2024; 14(6):2393. https://doi.org/10.3390/app14062393
Chicago/Turabian StyleFil’o, Milan, Tomáš Brestovič, Marián Lázár, Natália Jasminská, Romana Dobáková, and Štefan Kender. 2024. "Application of Unsteady Fluid Flow Simulation in the Process of Regulating an Industrial Hydraulic Network" Applied Sciences 14, no. 6: 2393. https://doi.org/10.3390/app14062393
APA StyleFil’o, M., Brestovič, T., Lázár, M., Jasminská, N., Dobáková, R., & Kender, Š. (2024). Application of Unsteady Fluid Flow Simulation in the Process of Regulating an Industrial Hydraulic Network. Applied Sciences, 14(6), 2393. https://doi.org/10.3390/app14062393






