Investigation of Heat and Moisture Transfer during the Drying of Packed-Bed Porous Media in Soybeans
Abstract
1. Introduction
2. Methodology
2.1. Model Building
2.2. DEM Simulation Parameters
3. Simulation Setup
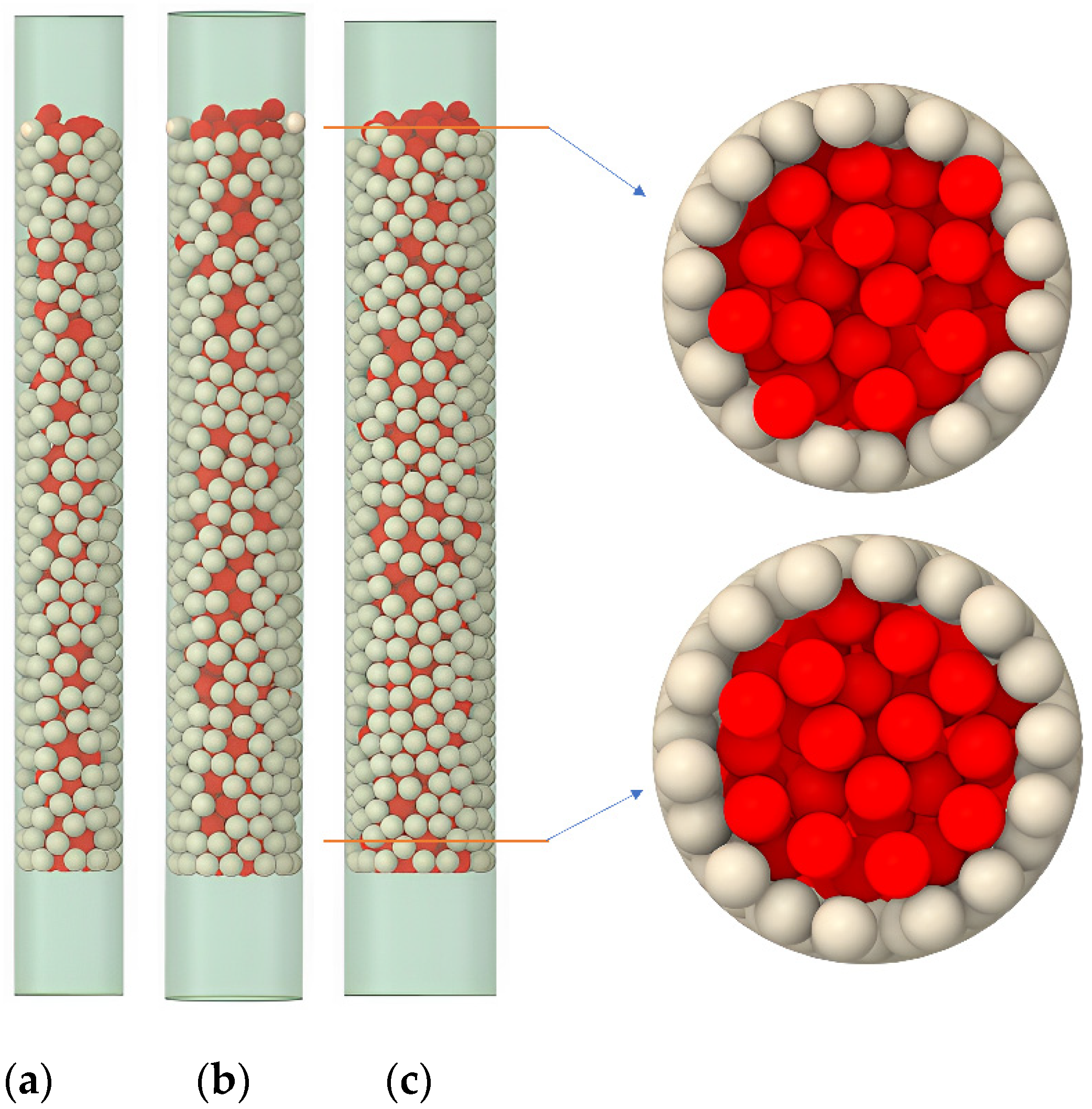
3.1. Geometry and Physical Model
3.2. Accumulation Bed and Particle-to-Particle Contact Point Treatment
4. Results and Discussion
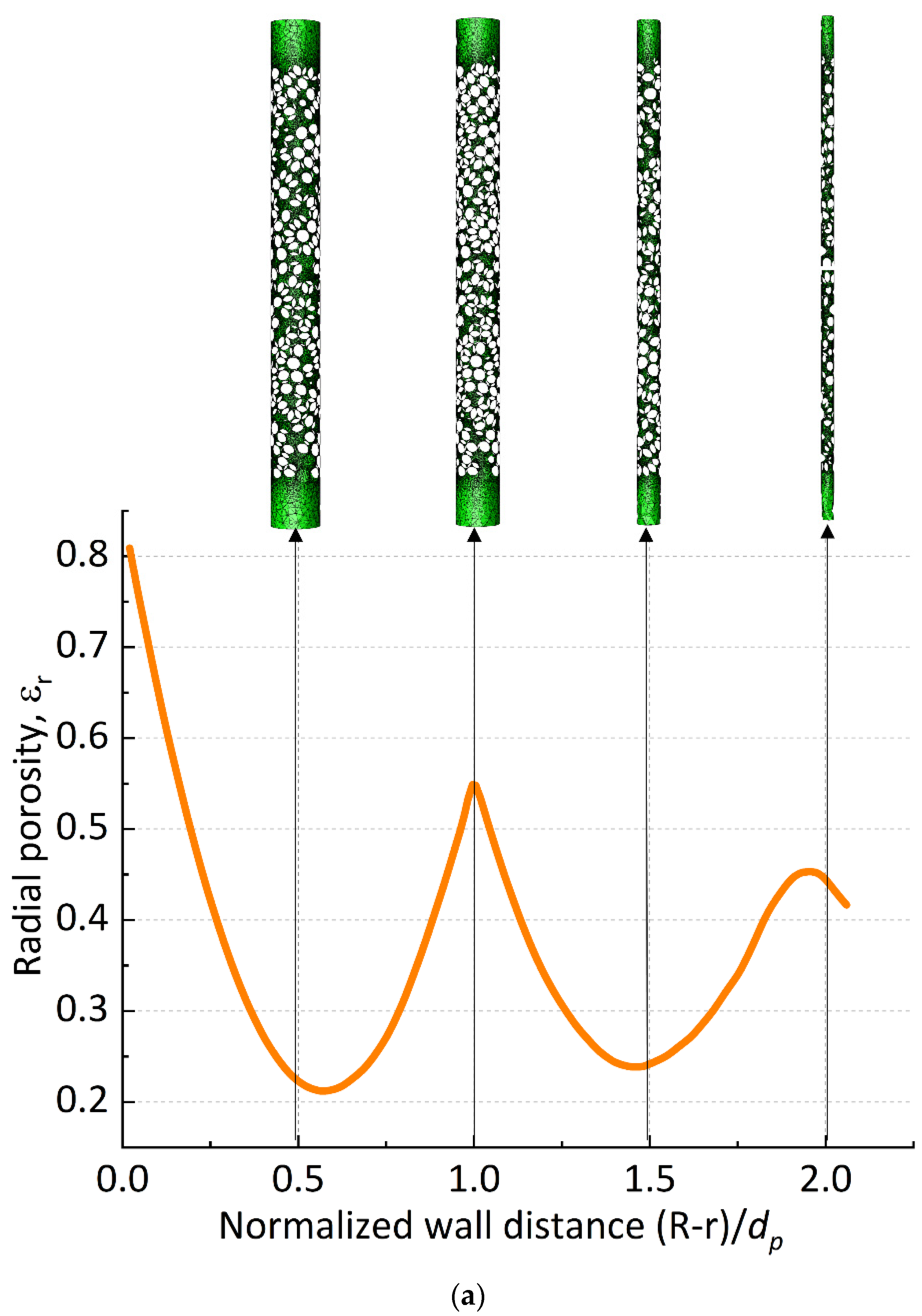
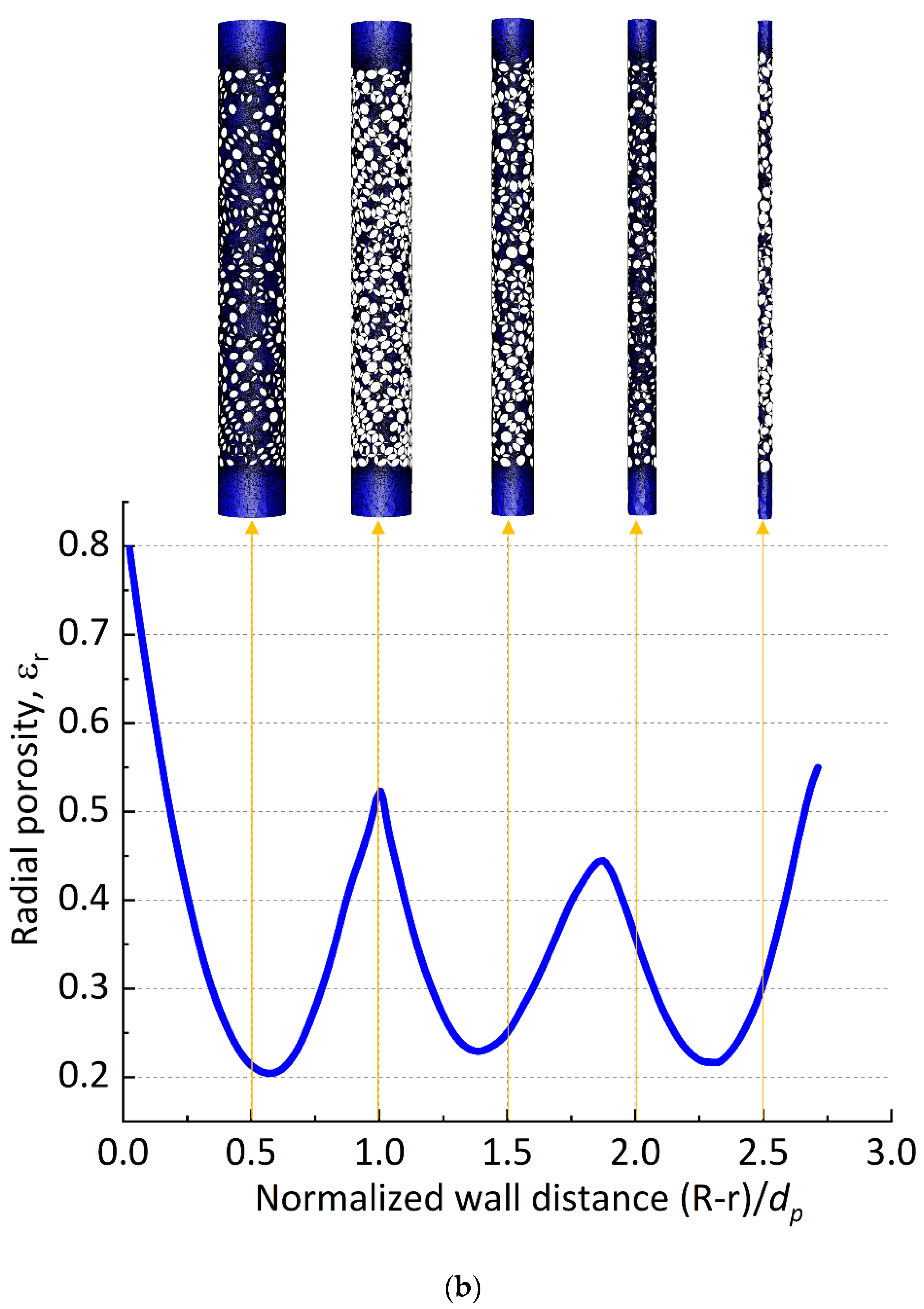
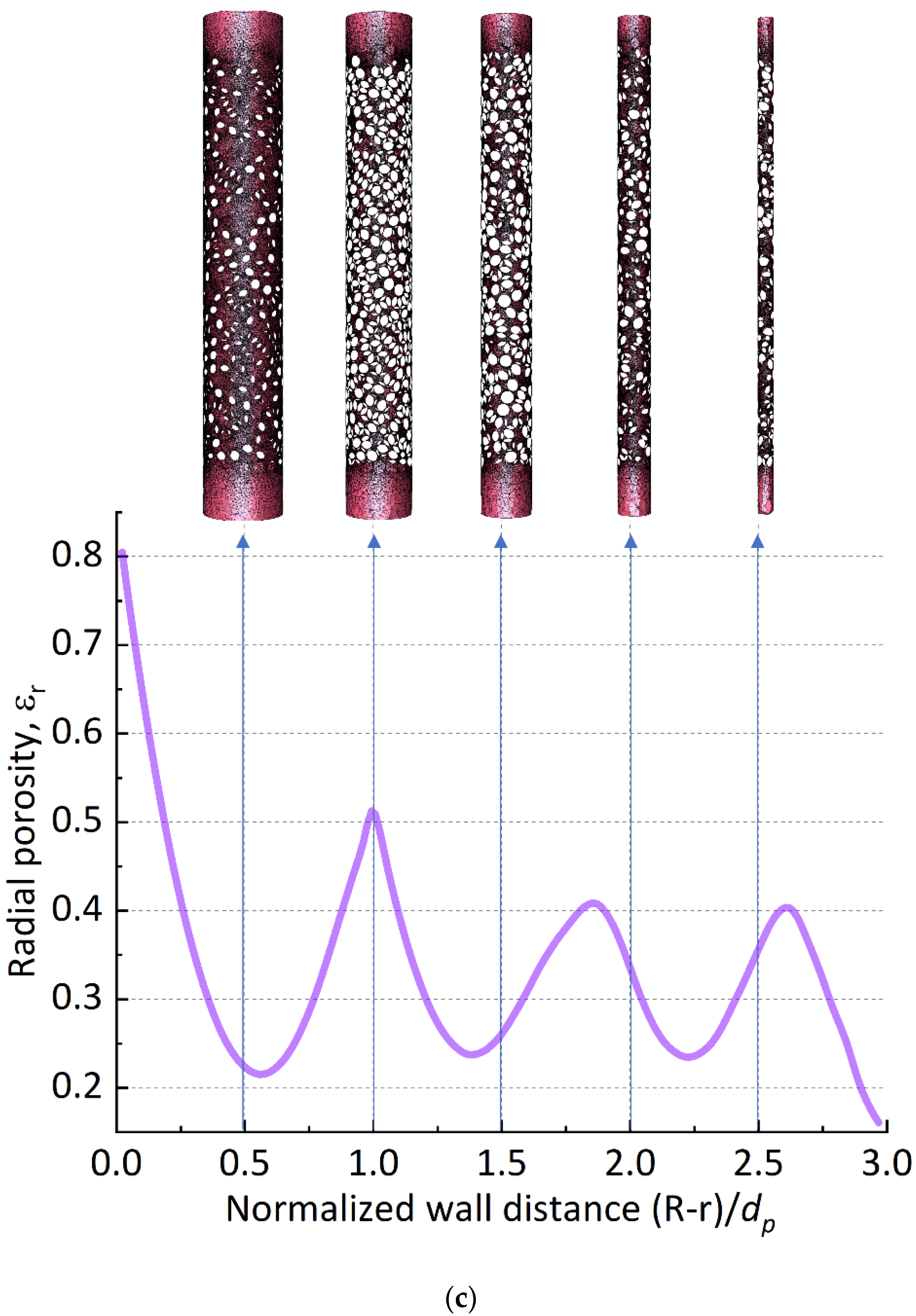
4.1. Radial Porosity Distribution
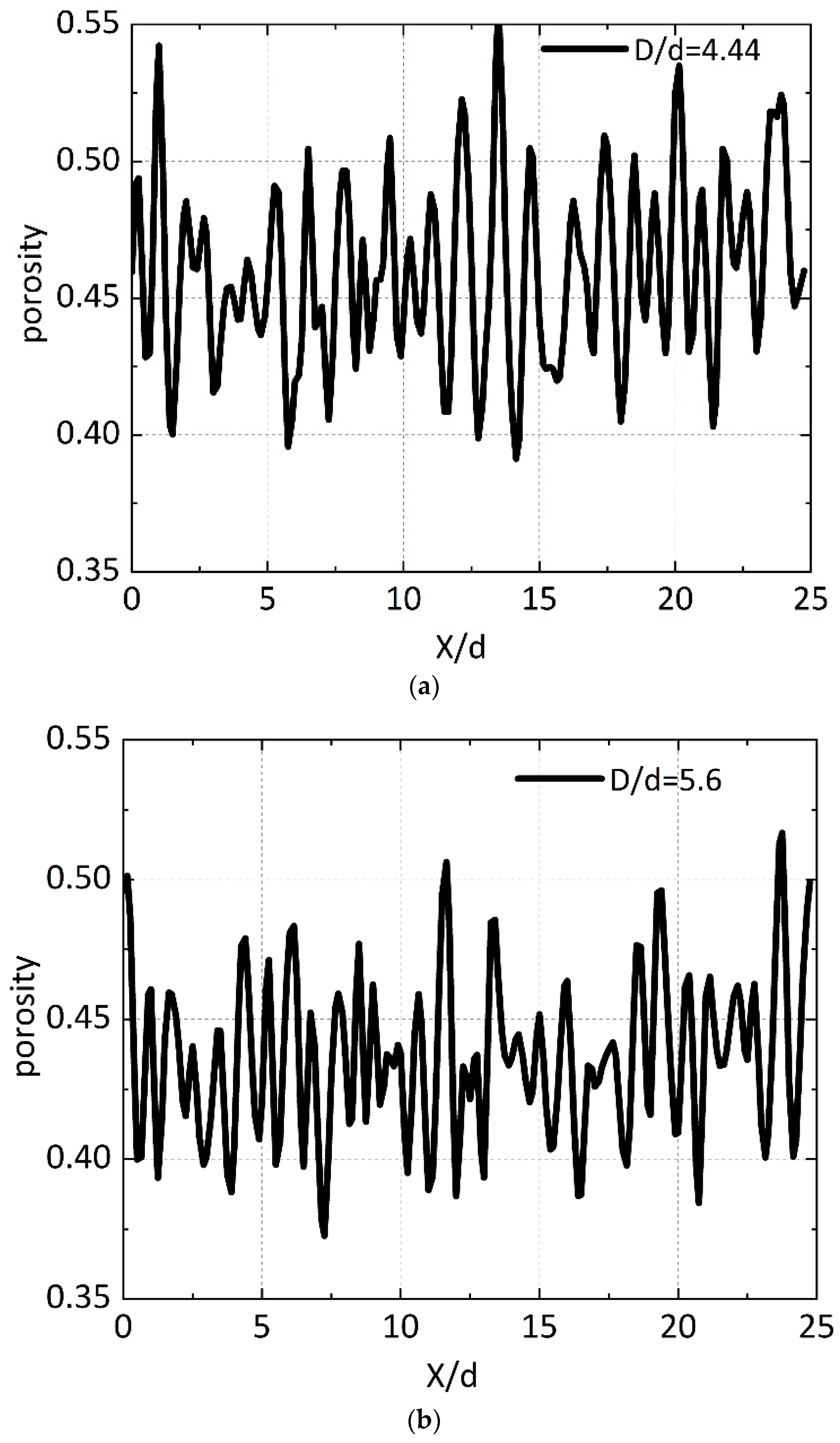
4.2. Axial Porosity Distribution
4.3. Hydrodynamics
4.3.1. Control Equations and Boundary Conditions
4.3.2. Model Validation
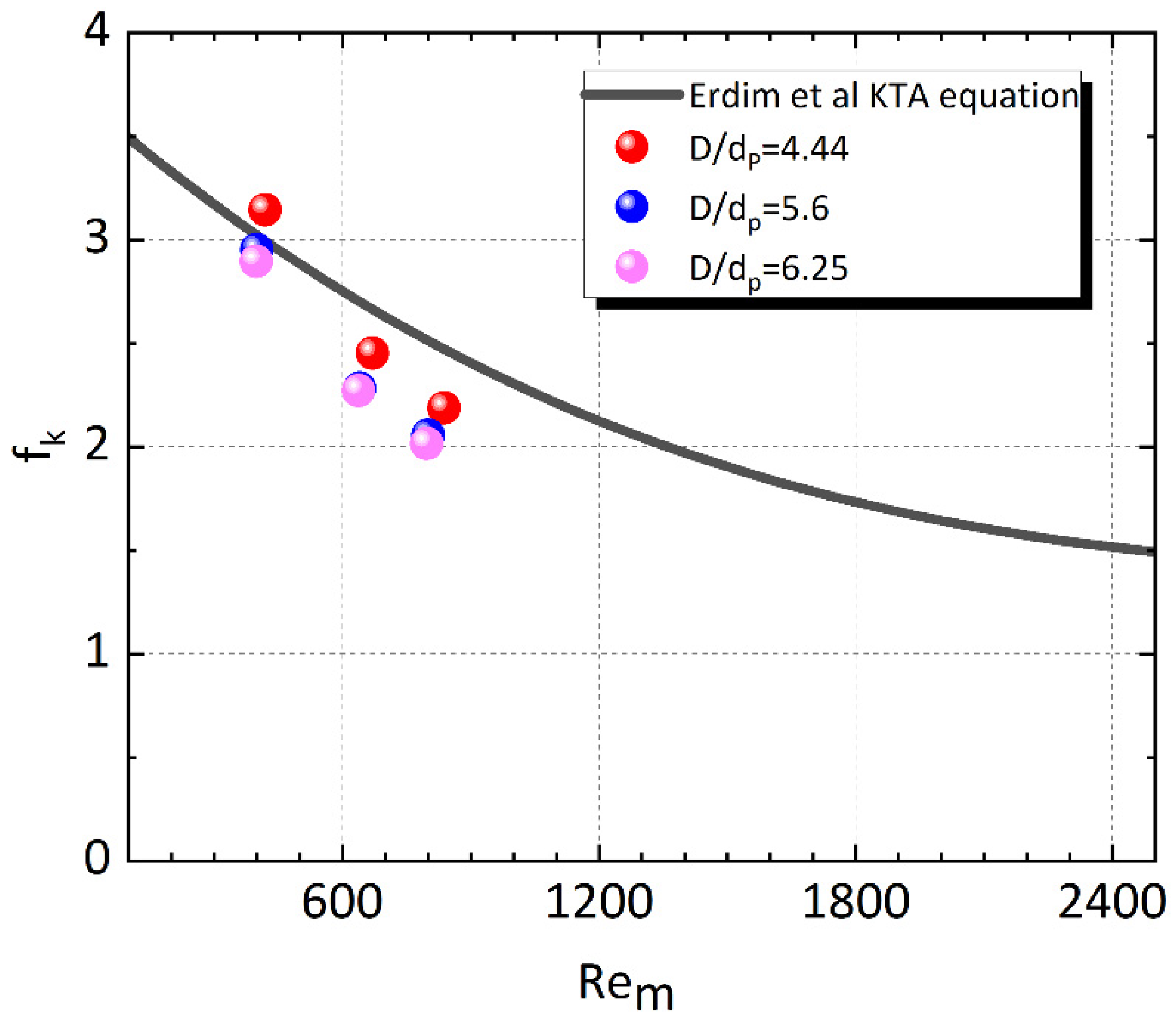
4.3.3. Packed-Bed Pressure Drop
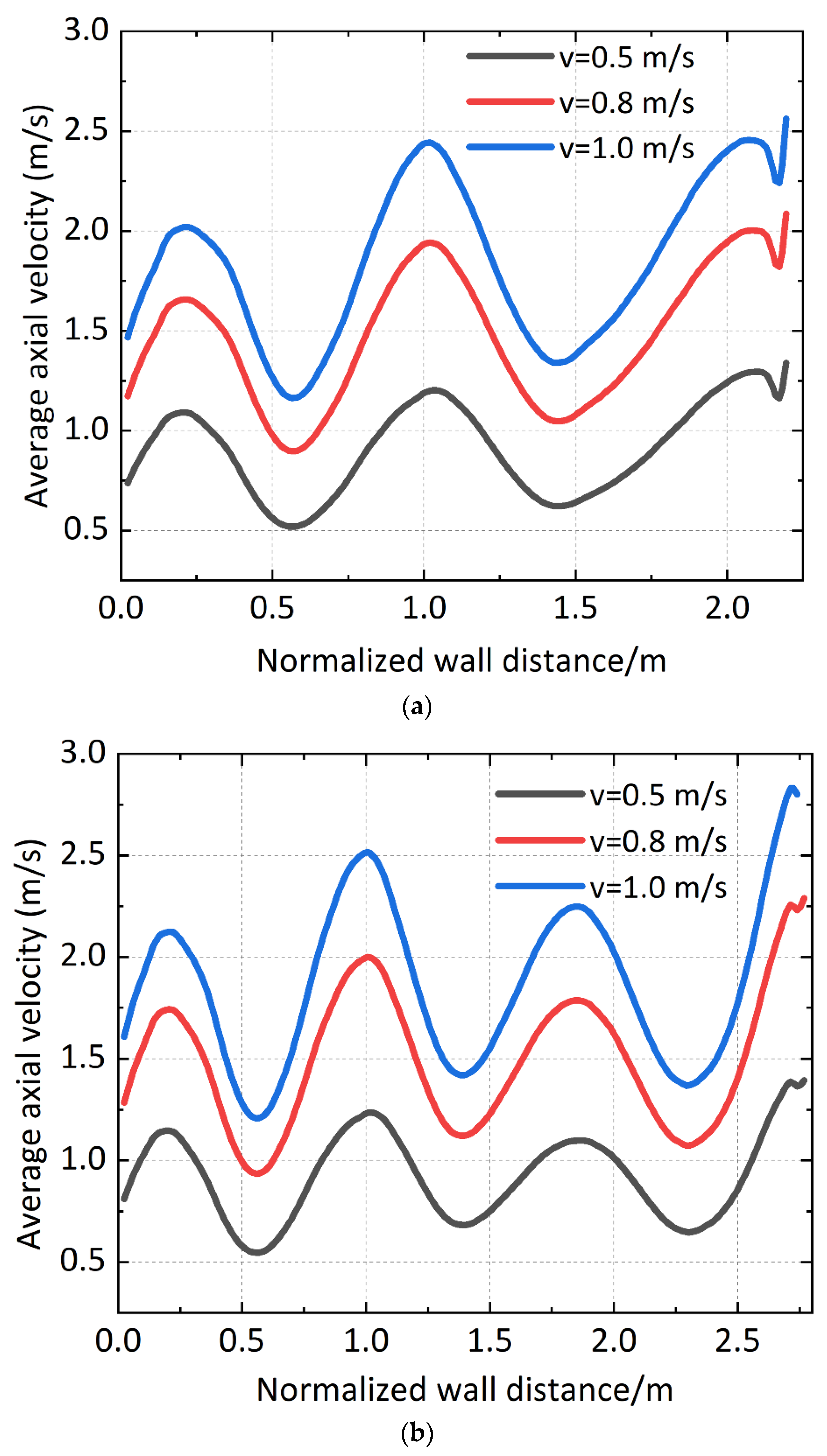
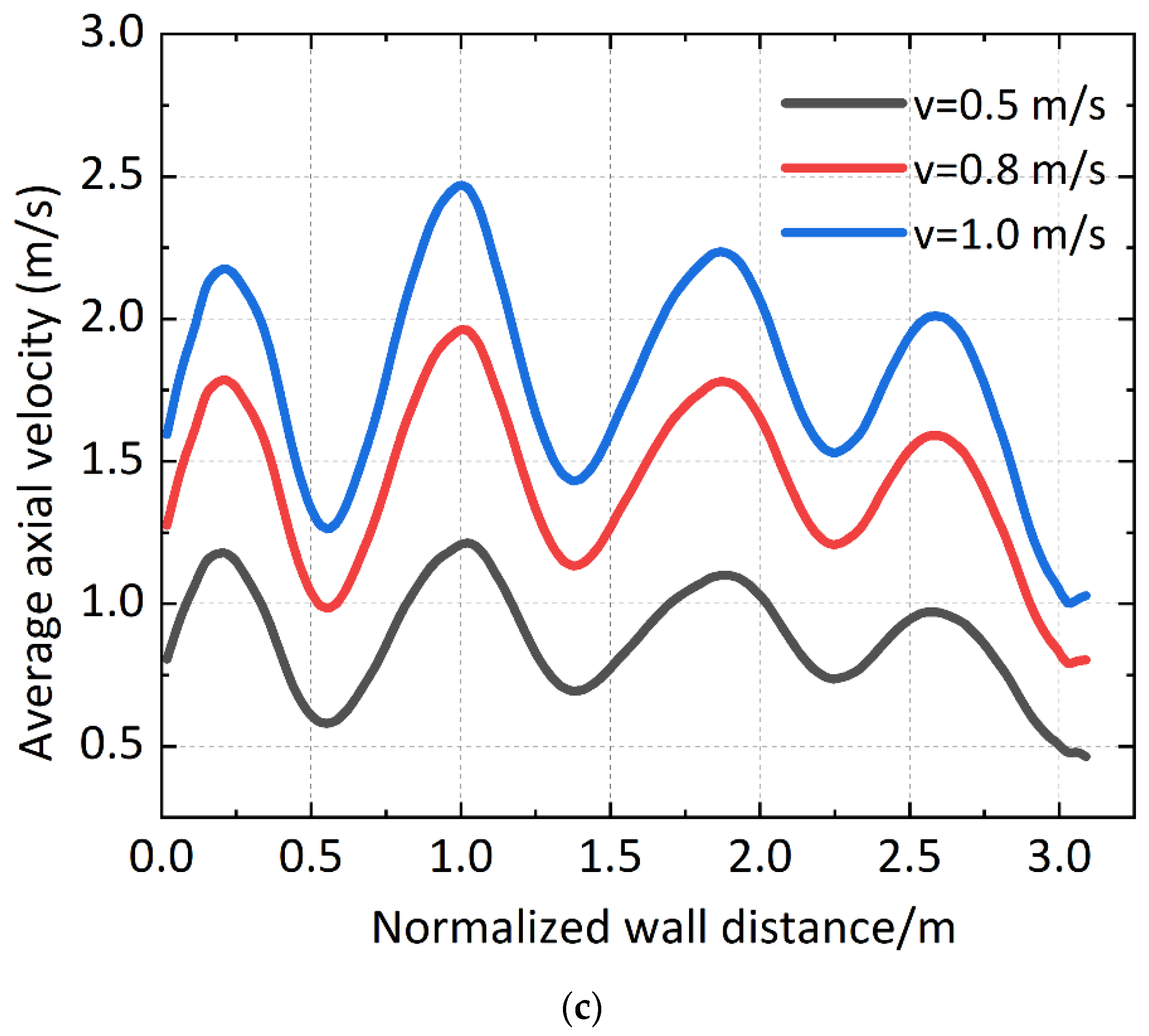
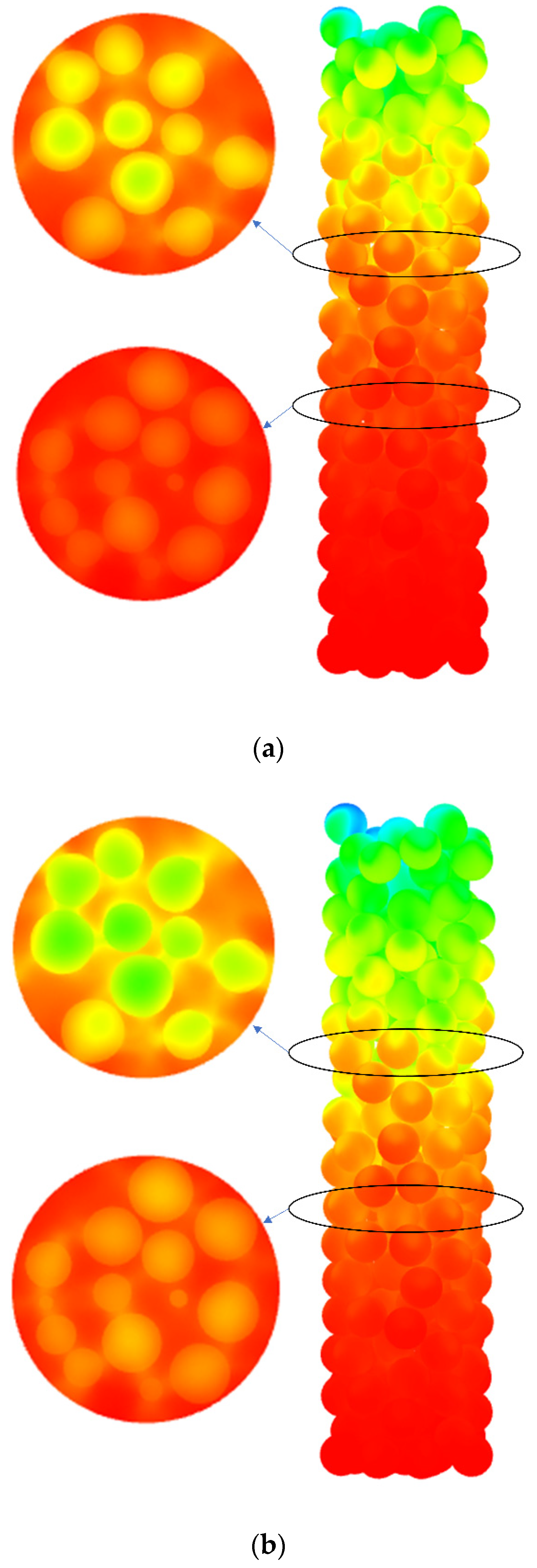
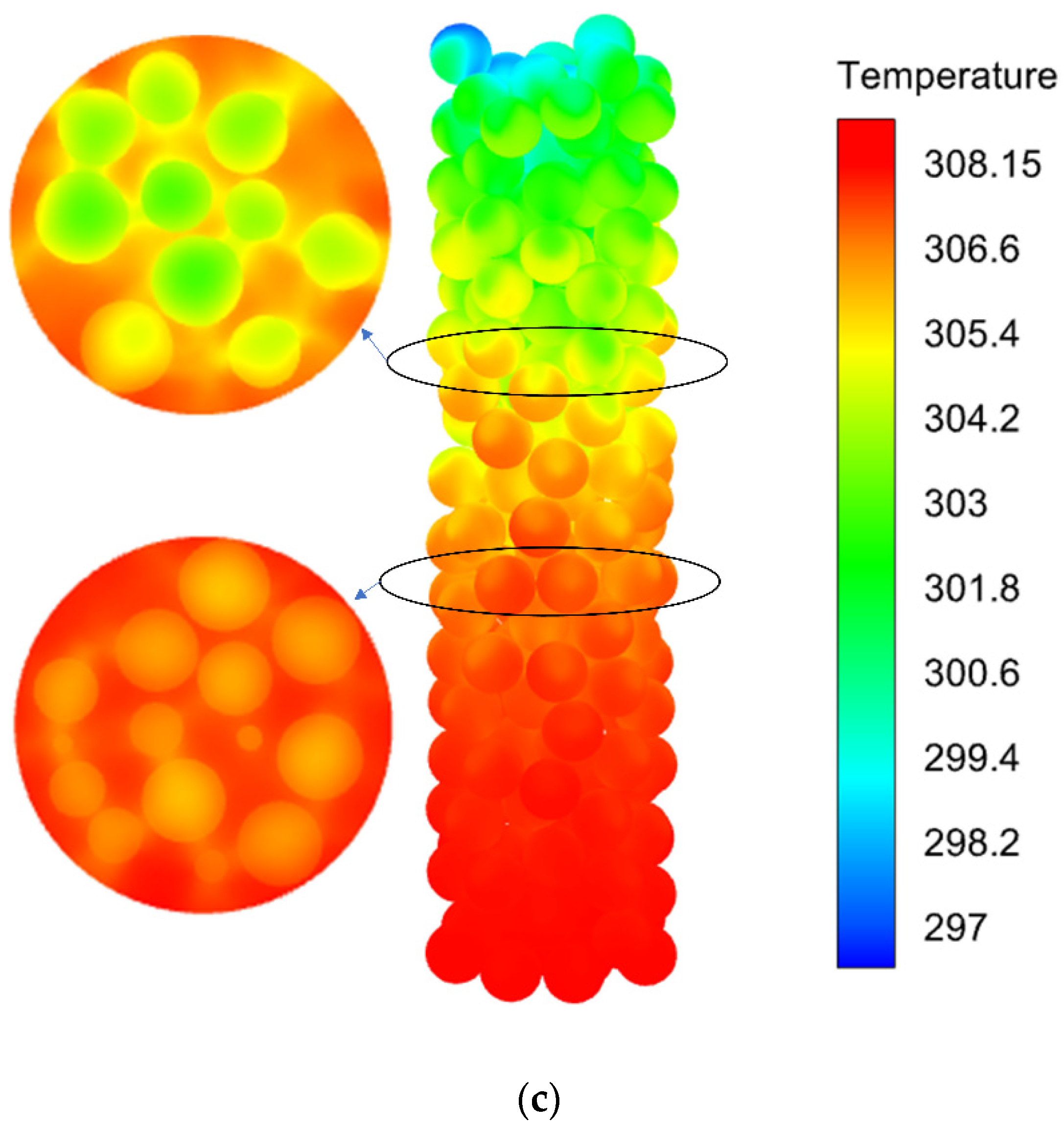
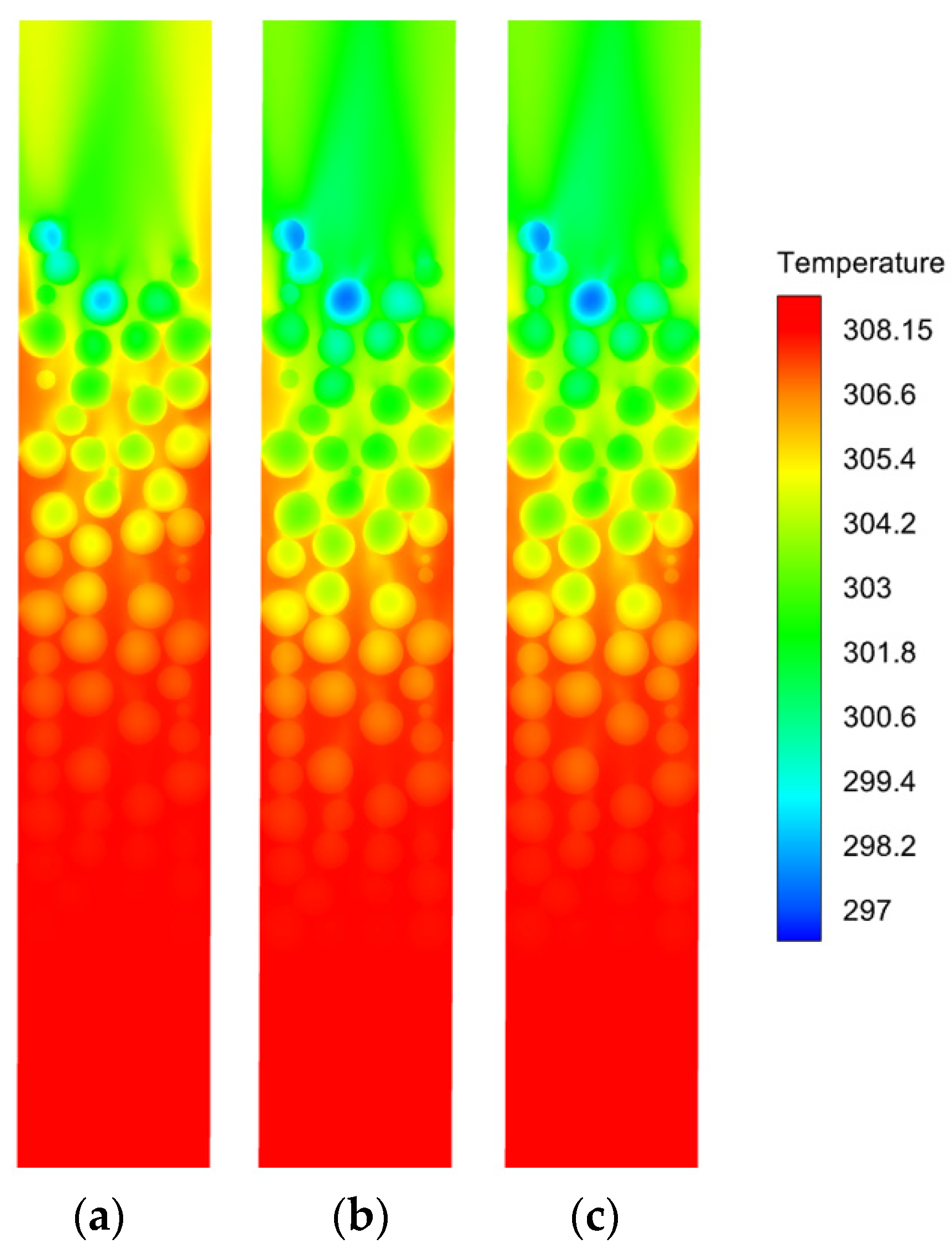
4.4. Localized Fluid Flow and Steady Temperature
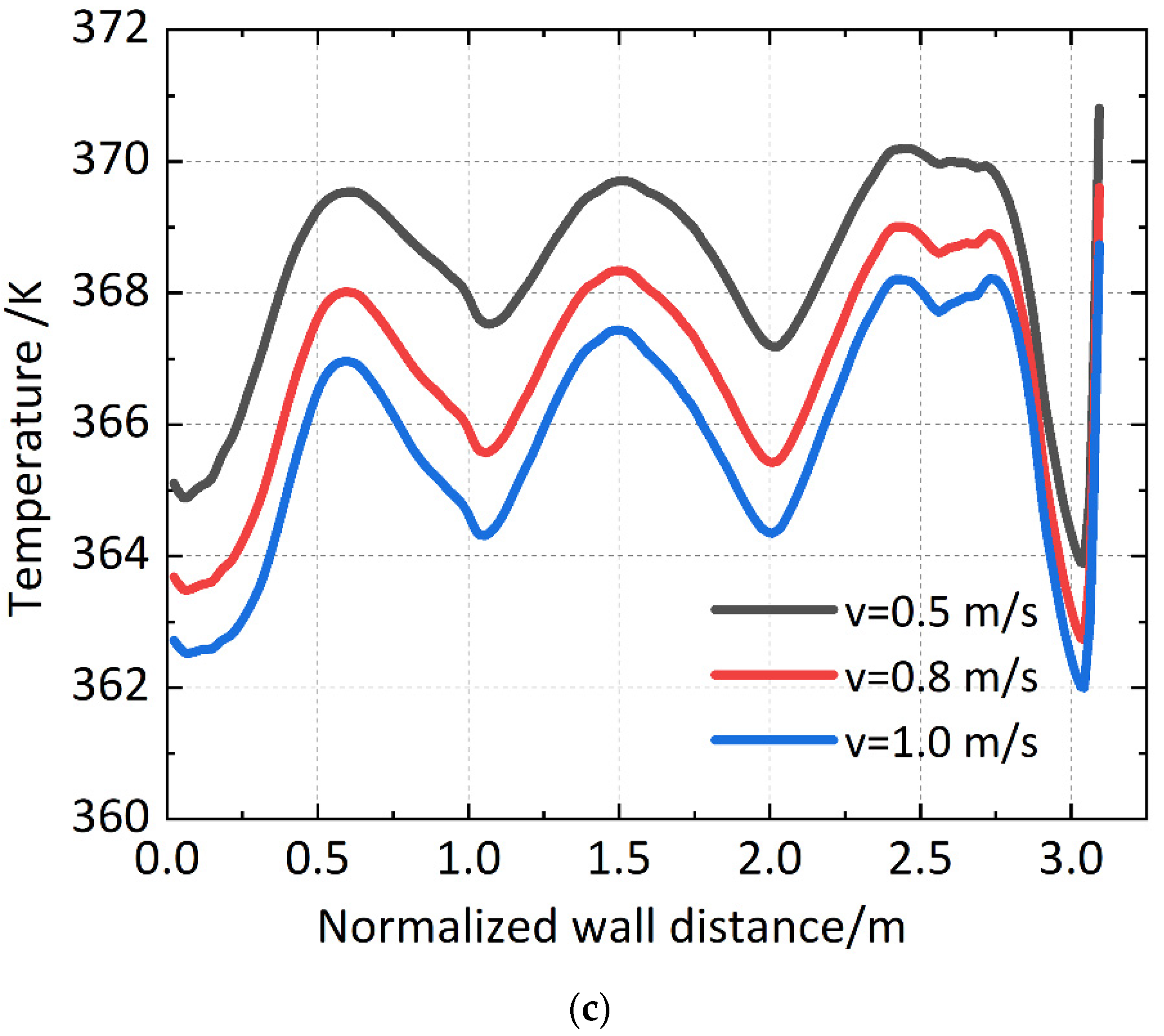
4.5. Factors Influencing Flow and Steady Temperature Characteristics
4.6. Transient Temperature Distribution
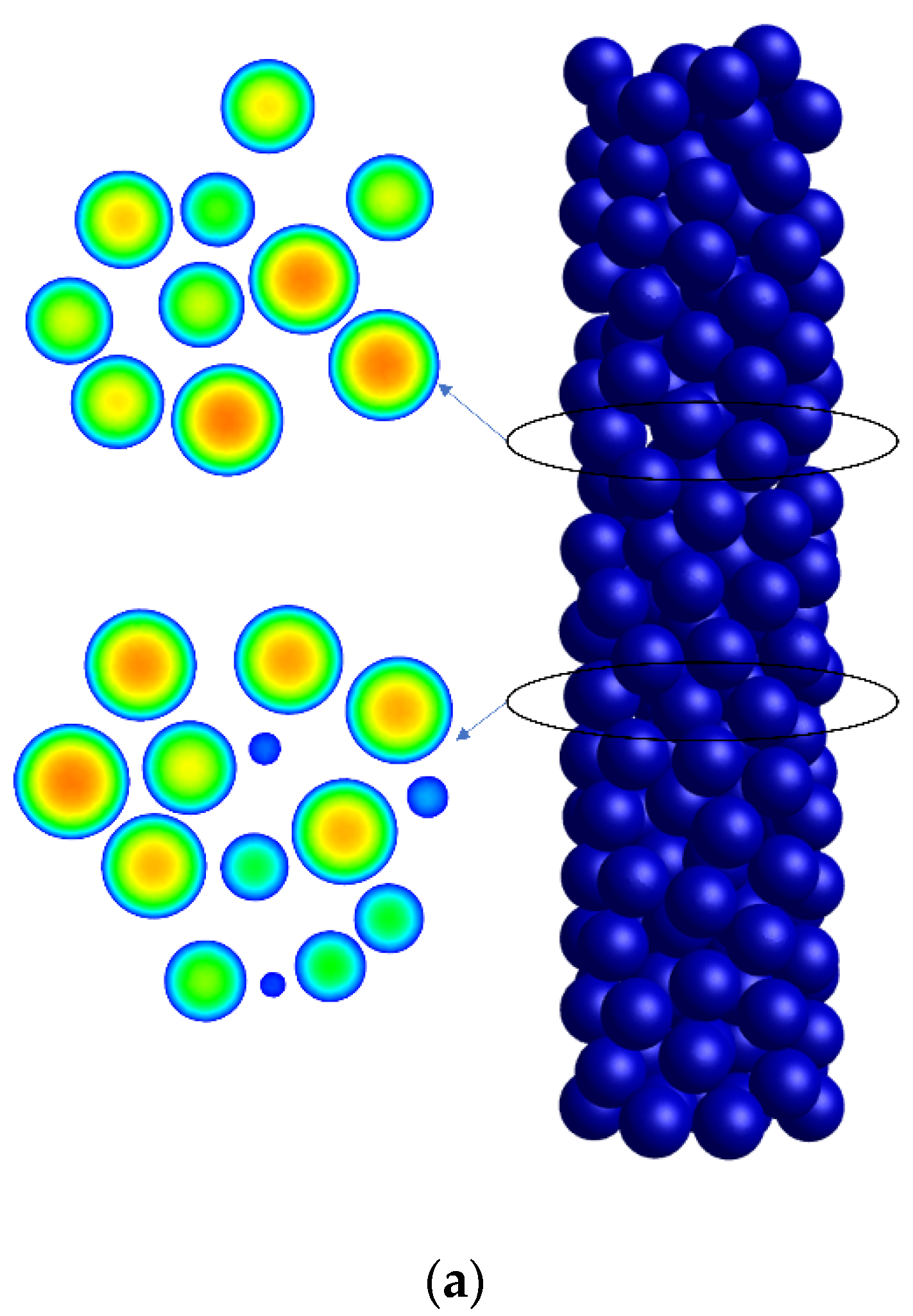
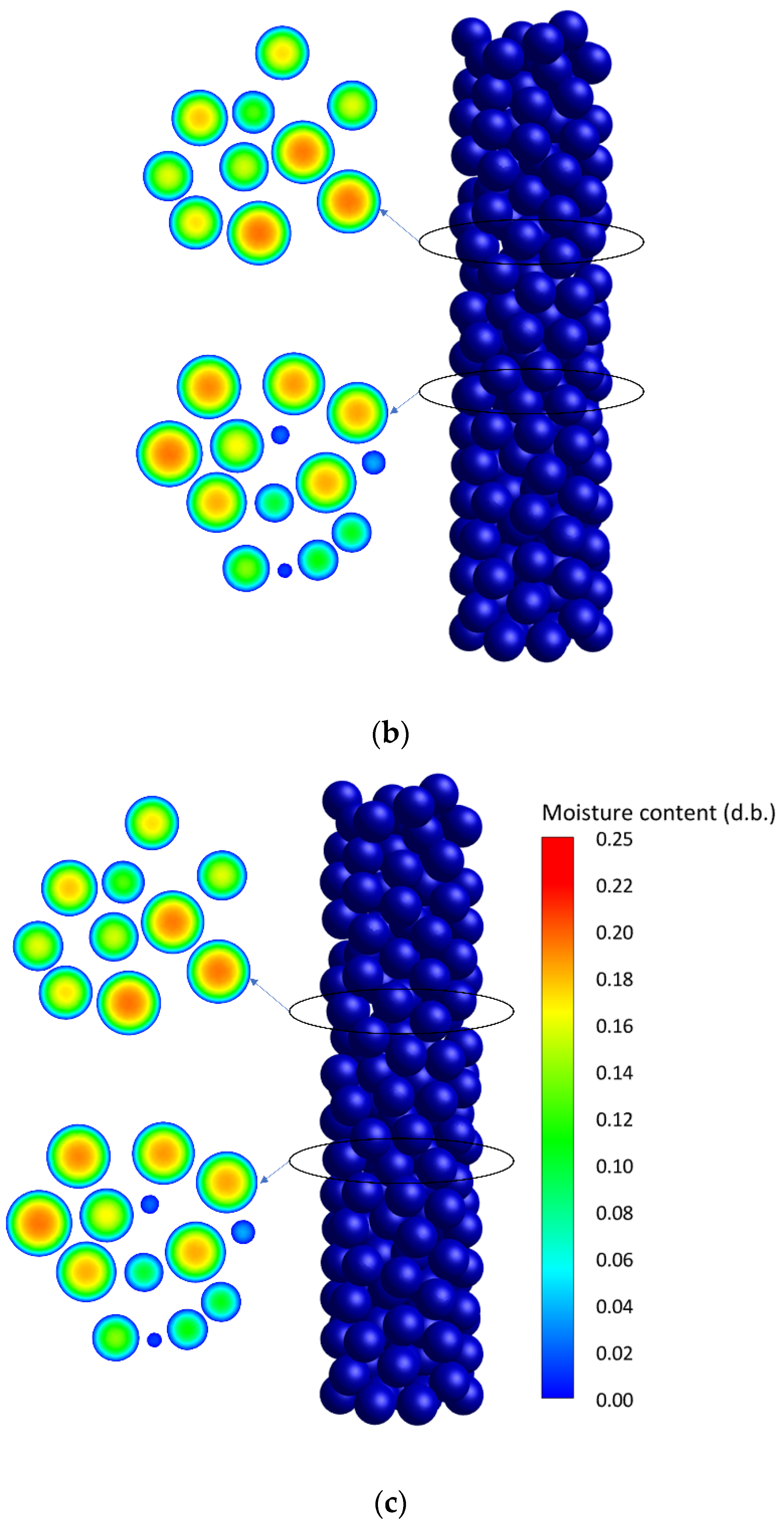
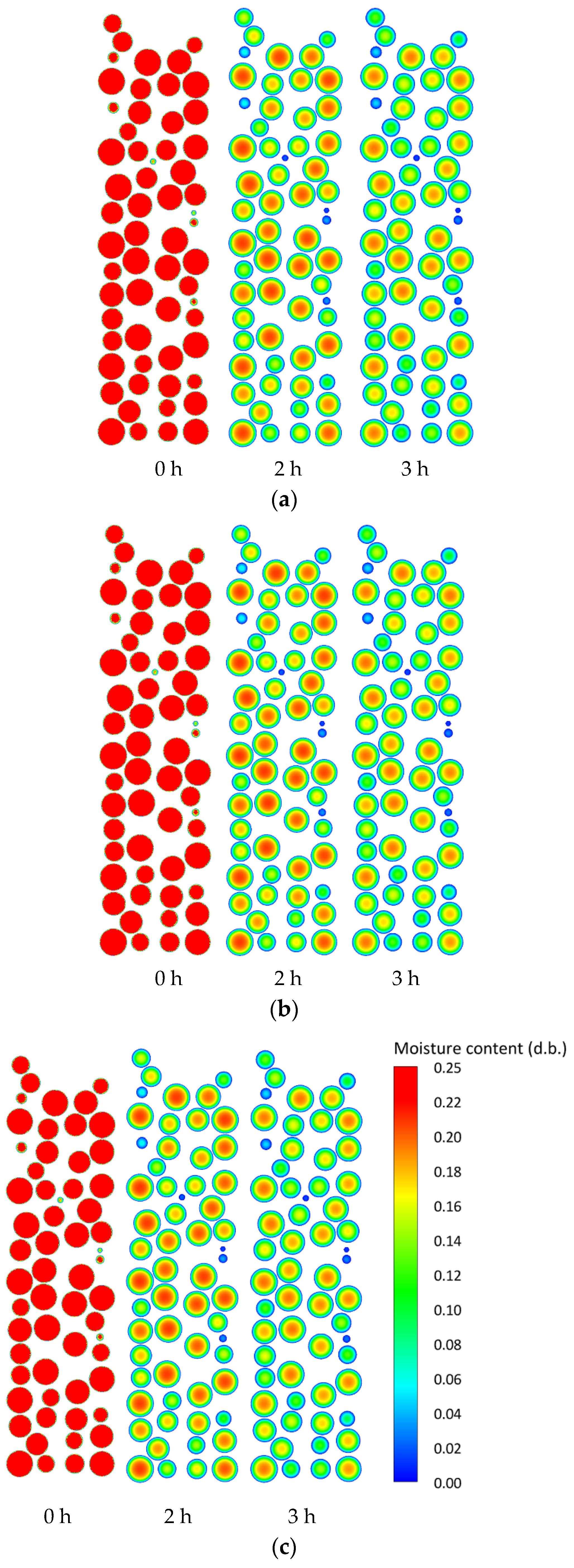
4.7. Moisture Distribution
5. Conclusions
- (1)
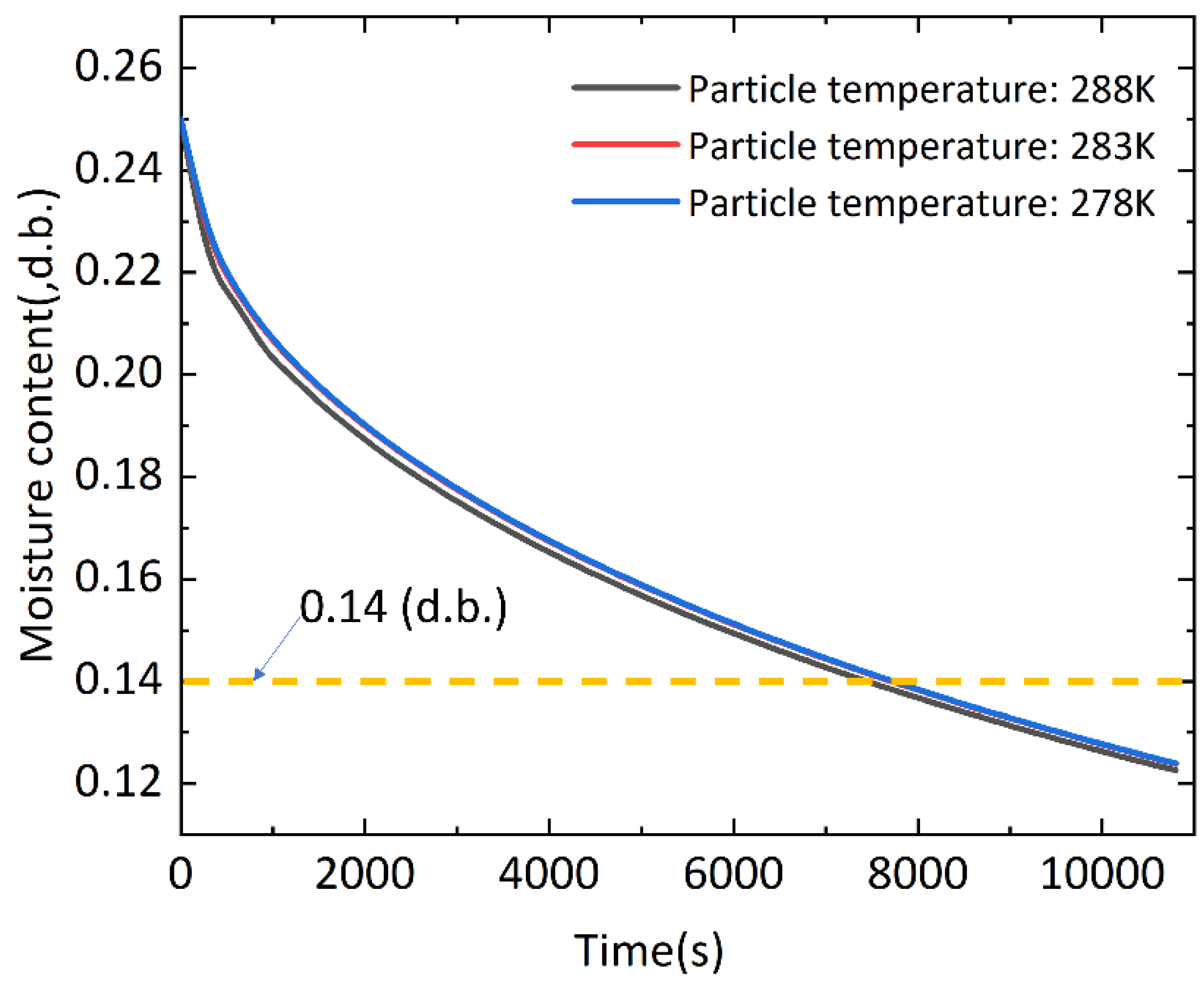
- The results indicate that the impact of the tube wall is minimal when the particle size ratio is low. The structural characteristics of the bed have a significant influence on the flow characteristics. Stagnation or backflow can occur in the wakes of the particles, while high velocities are present in areas with high porosity. Furthermore, altering the temperature of soybean particles changes the parameters of transient heat transfer, thereby affecting the convective heat transfer process. As the temperature of soybean granules increases, the water transfer rate in the packed bed also increases, along with the mass transfer rate.
- (2)
- The temperature distribution of the packed bed is uniformly distributed in steps. The temperature of the hot air gradually increases and the drying rate is accelerated, so the elapsed time to reach safe moisture becomes increasingly less. The molecular diffusion is incomplete, and the temperature uniformity becomes worse.
- (3)
- Analysis of ventilated drying of soybean pellets reveals that the temperature and moisture of the pellets in the front of the vented inlet change the fastest, while the pellet’s interior remains high in moisture. The moisture differential between the interior and surface of the particles decreases as drying time increases. The local non-equilibrium heat and moisture transfer model proposed in this study correctly predicts soybean particle temperature and moisture fluctuations at various scales.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| fk | friction factor |
| Rem | modified Reynolds number |
| εr | mean porosity of packed bed |
| R | packed bed radius |
| r | particle radius |
| dp | particle diameter |
| x | packed bed length |
| ΔP | pressure drop of packed bed |
| Z | axial height from the center of the packed bed as the origin |
| N | particle diameter ratio |
References
- Fleurat-Lessard, F. Integrated management of the risks of stored grain spoilage by seedborne fungi and contamination by storage mould mycotoxins—An update. J. Stored Prod. Res. 2017, 71, 22–40. [Google Scholar] [CrossRef]
- Jian, F.; Jayas, D.S. The Ecosystem Approach to Grain Storage. Agric. Res. 2012, 1, 148–156. [Google Scholar] [CrossRef]
- Flinn, P.W.; Subramanyam, B.; Arthur, F.H. Comparison of Aeration and Spinosad for Suppressing Insects in Stored Wheat. J. Econ. Entomol. 2004, 4, 1465–1473. [Google Scholar] [CrossRef]
- Manandhar, A.; Milindi, P.; Shah, A. An Overview of the Post-Harvest Grain Storage Practices of Smallholder Farmers in Developing Countries. Agriculture 2018, 8, 57. [Google Scholar] [CrossRef]
- Lawrence, J.; Maier, D.E.; Stroshine, R.L. Three-Dimensional Transient Heat, Mass, Momentum, and Species Transfer in the Stored Grain Ecosystem: Part I. Model Development and Evaluation. Trans. ASABE 2013, 56, 179–188. [Google Scholar] [CrossRef]
- Subrot Panigrahi, S.; Singh, C.B.; Fielke, J.; Zare, D. Modeling of heat and mass transfer within the grain storage ecosystem using numerical methods: A review. Dry. Technol. 2019, 38, 1677–1697. [Google Scholar] [CrossRef]
- Godfray, H.C.J.; Beddington, J.R.; Crute, I.R.; Haddad, L.; Lawrence, D.; Muir, J.F.; Pretty, J.; Robinson, S.; Thomas, S.M.; Toulmin, C. Food Security: The Challenge of Feeding 9 Billion People. Science 2010, 327, 812–818. [Google Scholar] [CrossRef] [PubMed]
- Mohseni, M.; Peters, B.; Baniasadi, M. Conversion analysis of a cylindrical biomass particle with a DEM-CFD coupling approach. Case Stud. Therm. Eng. 2017, 10, 343–356. [Google Scholar] [CrossRef]
- Niu, Q.; Wang, N.-X. Study of heat transfer by using DEM–CFD method in a randomly packed pebble-bed reactor. Nucl. Sci. Tech. 2019, 30, 28. [Google Scholar] [CrossRef]
- Nourmohamadi-Moghadami, A.; Zare, D.; Singh, C.B.; Stroshine, R.L. Filling of a grain silo. Part 1: Investigation of fine material distribution in a small scale centre-filled silo. Biosyst. Eng. 2020, 191, 145–156. [Google Scholar] [CrossRef]
- Mandas, N.; Habte, M. PH—Postharvest Technology: Numerical Simulation Static-Bed Drying of Barley. Biosyst. Eng. 2002, 82, 313–319. [Google Scholar] [CrossRef]
- Bai, H.; Theuerkauf, J.; Gillis, P.A.; Witt, P.M. A Coupled DEM and CFD Simulation of Flow Field and Pressure Drop in Fixed Bed Reactor with Randomly Packed Catalyst Particles. Ind. Eng. Chem. Res. 2009, 48, 4060–4074. [Google Scholar] [CrossRef]
- Wehinger, G.D.; Fütterer, C.; Kraume, M. Contact Modifications for CFD Simulations of Fixed-Bed Reactors: Cylindrical Particles. Ind. Eng. Chem. Res. 2017, 56, 87–99. [Google Scholar] [CrossRef]
- Dixon, A.G.; Nijemeisland, M.; Stitt, E.H. Systematic mesh development for 3D CFD simulation of fixed beds: Contact points study. Comput. Chem. Eng. 2013, 48, 135–153. [Google Scholar] [CrossRef]
- Dixon, A.G.; Nijemeisland, M.; Stitt, H. CFD Simulation of Reaction and Heat Transfer Near the Wall of a Fixed Bed. Int. J. Chem. React. Eng. 2003, 1, 20121029. [Google Scholar] [CrossRef]
- Guardo, A.; Coussirat, M.; Larrayoz, M.A.; Recasens, F.; Egusquiza, E. CFD Flow and Heat Transfer in Nonregular Packings for Fixed Bed Equipment Design. Ind. Eng. Chem. Res. 2004, 43, 7049–7056. [Google Scholar] [CrossRef]
- Kuroki, M.; Ookawara, S.; Ogawa, K. A High-Fidelity CFD Model of Methane Steam Reforming in a Packed Bed Reactor. J. Chem. Eng. Jpn. 2009, 42, s73–s78. [Google Scholar] [CrossRef]
- Guo, Z.; Sun, Z.; Zhang, N.; Ding, M.; Cao, X. Radial porosity peak at the centerline of packed beds with small tube to particle diameter ratios. Powder Technol. 2017, 319, 445–451. [Google Scholar] [CrossRef]
- Eppinger, T.; Seidler, K.; Kraume, M. DEM-CFD simulations of fixed bed reactors with small tube to particle diameter ratios. Chem. Eng. J. 2011, 166, 324–331. [Google Scholar] [CrossRef]
- Mueller, G.E. Radial porosity in packed beds of spheres. Powder Technol. 2010, 203, 626–633. [Google Scholar] [CrossRef]
- Liu, H.B.; Zhao, C.Y. Effect of Radial porosity oscillation on the thermal performance of packed bed latent heat storage. Engineering 2020, 7, 515–525. [Google Scholar] [CrossRef]
- Niven, R.K. Reply to the comments by D. Stevenson on “Physical insight into the Ergun and Wen and Yu equations for fluid flow in packed and fluidised beds” by R.K. Niven [Chemical Engineering Science, 57 (2002), 527–534]. Chem. Eng. Sci. 2005, 60, 299–300. [Google Scholar] [CrossRef]
- Erdim, E.; Akgiray, Ö.; Demir, İ. A revisit of pressure drop-flow rate correlations for packed beds of spheres. Powder Technol. 2015, 283, 488–504. [Google Scholar] [CrossRef]
- Ergun, S.; Orning, A.A. Fluid Flow through Randomly Packed Columns and Fluidized Beds. Ind. Eng. Chem. 1949, 41, 1179–1184. [Google Scholar] [CrossRef]
- Anthony, G.D. General correlation for pressure drop through randomly-packed beds of spheres with negligible wall effects. AIChE J. 2023, 69, e18035. [Google Scholar] [CrossRef]
- Dixon, A.G.; Walls, G.; Stanness, H.; Nijemeisland, M.; Stitt, E.H. Experimental validation of high Reynolds number CFD simulations of heat transfer in a pilot-scale fixed bed tube. Chem. Eng. J. 2012, 200–202, 344–356. [Google Scholar] [CrossRef]
- Wehinger, G.D.; Eppinger, T.; Kraume, M. Evaluating Catalytic Fixed-Bed Reactors for Dry Reforming of Methane with Detailed CFD. Chem. Ing. Tech. 2015, 87, 734–745. [Google Scholar] [CrossRef]
- Winterberg, M.; Tsotsas, E.; Krischke, A.; Vortmeyer, D. A simple and coherent set of coefficients for modelling of heat and mass transport with and without chemical reaction in tubes filled with spheres. Chem. Eng. Sci. 2000, 55, 967–979. [Google Scholar] [CrossRef]
- Wang, L.-B.; Tao, W.-Q.; Wang, Q.-W.; Wong, T.T. Experimental study of developing turbulent flow and heat transfer in ribbed convergent/divergent square ducts. Int. J. Heat Fluid Flow 2001, 22, 603–613. [Google Scholar] [CrossRef]
- Hasan, M.D.M.; Hu, X.; Gunawan, R.; Li, C.-Z. Pyrolysis of large mallee wood particles: Temperature gradients within a pyrolysing particle and effects of moisture content. Fuel Process. Technol. 2017, 158, 163–171. [Google Scholar] [CrossRef]
- Obeng, G.Y.; Adjaloo, M.K.; Donkor, P. Effect of Temperature, Moisture Content, Particle Size and Roasting on Shea Butter Extraction Efficiency. Int. J. Food Eng. 2010, 6. [Google Scholar] [CrossRef]
- Ishii, K.; Furuichi, T. Influence of moisture content, particle size and forming temperature on productivity and quality of rice straw pellets. Waste Manag. 2014, 34, 2621–2626. [Google Scholar] [CrossRef] [PubMed]








| Parameter | Symbol | Unit | Value |
|---|---|---|---|
| Cylinder radius | D | mm | 26.64/33.6/37.5 |
| Particle radius | dp | mm | 6 |
| Cylinder-to-particle size ratio | D/dp | 4.44/5.6/6.25 | |
| Particle density | ρp | kg/m3 | 1257 |
| Shear modulus | G | Pa | 2.71 × 108 |
| Young’s modulus | E | Pa | 7.588 × 108 |
| Poisson’s ratio | ν | 0.4 | |
| Restitution coefficient | epp | 0.627 | |
| Static friction coefficient | μst | 0.2 | |
| Rolling friction coefficient | μr | 0.02 |
| Material | Symbol | Value |
|---|---|---|
| Soybean | ρs, kg/m3 | 1257 |
| ks, W/(m·K) | 0.136 | |
| cs, J/(kg·K) | cs = 2000 + 35.5(Ys,db/1 + Ys,db) | |
| Air | ρf, kg/m3 | ρf = 8.666 × 10−6Tf2 − 4.318 × 10−3Tf + 1.228 |
| µf, kg/(m·s) | µf = 1.691 × 10−5 + 4.984 × 10−8Tf | |
| cf, J/(kg·K) | cf = 1002.9 + 0.0054Tf | |
| kf, W/(m·K) | kf = −2.401 × 10−8Tf 2 + 7.554 × 10−5Tf + 2.364 × 10−2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niu, Z.; Lu, X.; Li, Z. Investigation of Heat and Moisture Transfer during the Drying of Packed-Bed Porous Media in Soybeans. Appl. Sci. 2024, 14, 1935. https://doi.org/10.3390/app14051935
Niu Z, Lu X, Li Z. Investigation of Heat and Moisture Transfer during the Drying of Packed-Bed Porous Media in Soybeans. Applied Sciences. 2024; 14(5):1935. https://doi.org/10.3390/app14051935
Chicago/Turabian StyleNiu, Zhuang, Xiangyou Lu, and Zhiqiang Li. 2024. "Investigation of Heat and Moisture Transfer during the Drying of Packed-Bed Porous Media in Soybeans" Applied Sciences 14, no. 5: 1935. https://doi.org/10.3390/app14051935
APA StyleNiu, Z., Lu, X., & Li, Z. (2024). Investigation of Heat and Moisture Transfer during the Drying of Packed-Bed Porous Media in Soybeans. Applied Sciences, 14(5), 1935. https://doi.org/10.3390/app14051935





