A Coordinated Supply Contract for a Two-Echelon Supply Chain Considering Learning Effects
Abstract
1. Introduction
2. Literature Review
2.1. The Development of the Learning Effect
2.2. The Application of Learning Effect to Supply-Chain Management
2.2.1. Inventory Management and Lot Sizing
2.2.2. Supplier Selection and Outsourcing
2.2.3. Scheduling and Line-Balancing Decisions
2.2.4. Pricing
- (1)
- We investigate the coordination of supply contracts by integrating the learning effect in revenue-sharing contracts. By integrating the learning effect into the design of supply contracts, our aim is to pinpoint strategies that enhance the profitability of individual stakeholders while also bolstering the overall profitability of the entire supply chain;
- (2)
- We consider the bargaining power between the manufacturer and the retailer in contract-building processes. This consideration is essential in understanding how the distribution of profits is affected by the negotiation strength of each party, further enhancing the real-world applicability of our findings;
- (3)
- We use a numerical method, Newton–Raphson method, to find the optimal order quantity in the system with learning-curve effects. We will demonstrate that this approach is a simple and efficient solution method for the problem in this study.
3. Basic Supply-Chain Models without Learning-Curve Effects
4. Coordinated Supply Contract under the Learning Curve (LC)
- The manufacturer predicts production costs, , depending on the production amount and determines so that the SC profit can be maximized. The production costs are assumed to follow the log-linear function given in expression (2);
- The manufacturer designs the revenue-sharing contract with the wholesale price w and the profit-share ratio ϕ, and presents the contract to the retailer;
- The retailer determines the order quantity to maximize the profit based on the uncertain demand, and places an order to the manufacturer;
- The manufacturer produces and delivers a quantity Q and receives payment from the retailer.
- The retailer sells the product at a given selling price during the sales season and gives a portion of the revenue to the manufacturer (revenue sharing);
- At the end of the season, the retailer disposes of unsold products for a salvage price.
4.1. Model Construction with LC Effects for Maximizing SC Profit
4.2. The Determination of Optimal Order Quantity by Using the Newton–Raphson Method
4.3. The Development of Coordinated Contract Parameters
4.4. The Identification of the Feasible Range of ϕ with Bargaining Power α
5. Numerical Illustrations
Additional Discussions
6. Conclusions
- −
- The proposed contract is based on the log-linear learning-curve model. Further research is needed to explore the implications of other learning-curve models, as various alternative models also exist;
- −
- Our study only considers risk-neutral supply-chain participants. Investigating risk-averse and risk-seeking participants would be an interesting research avenue;
- −
- While we examine revenue-sharing contracts to coordinate the supply chain under learning-curve effects, exploring other contract types like option contracts and buyback contracts with learning effects is warranted;
- −
- We solely consider learning effects, neglecting forgetting effects. Future research incorporating both learning and forgetting effects would provide a more comprehensive understanding of production efficiencies over time;
- −
- This study focuses on the two-echelon supply-chain structure. Investigating the impact of the learning effects on various supply-chain structures, especially those involving multiple echelons or complex network relationships, could provide deeper insights into the applicability of coordination strategies across different supply-chain models;
- −
- Further analyses could also be conducted, incorporating psychological factors such as retailers’ overconfidence in supply-chain decisions for enhanced supply-chain coordination.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Description | |
| w | Per-unit wholesale price for retailer |
| c | Per-unit production cost at the manufacturer |
| Cumulative average per-unit production cost up to Qth products made with the learning-curve effect | |
| p | Selling price at the market (revenue) |
| s | Salvage price for the unsold product |
| Q | Order quantity from the retailer (i.e., the production quantity) |
| X | Uncertain market demand with the cumulative distribution function (cdf), F(x) and the probability density function (pdf), f(x) |
| S(Q) | Expected amount sold during the sale season |
| I(Q) | Expected amount unsold until the end of the sale season |
| Retailer’s revenue-sharing ratio (0 ≤ ∅ ≤ 1) | |
| r | Learning rate (0 < r < 1) |
| Expected profit of supply chain, manufacturer, and retailer without learning-curve effects, respectively | |
| Expected profit of supply chain, manufacturer, and retailer with learning-curve effects, respectively |
Appendix A
References
- Lee, H.L.; Padmanabhan, V.; Taylor, T.A.; Whang, S. Price protection in the personal computer industry. Manag. Sci. 2000, 46, 467–482. [Google Scholar] [CrossRef]
- Suo, H.; Wang, J.; Jin, Y. Supply chain coordination with sales effort effects and impact of loss aversion on effort decision. Tsinghua Sci. Technol. 2005, 10, 102–107. [Google Scholar] [CrossRef]
- Li, T.; Sethi, S.P.; He, X. Dynamic pricing, production, and channel coordination with stochastic learning. Pro. Oper. Manag. 2015, 24, 857–882. [Google Scholar] [CrossRef]
- Becker-Peth, U.W.; Thonemann, U.W. Reference points in revenue sharing contracts—How to design optimal supply chain contracts. Eur. J. Oper. Res. 2016, 249, 1033–1049. [Google Scholar] [CrossRef]
- Tsao, Y.; Sheen, G. Effects of promotion cost sharing policy with the sales learning curve on supply chain coordination. Comput. Oper. Res. 2012, 39, 1872–1878. [Google Scholar] [CrossRef]
- Tsay, A.A.; Nahmias, S.; Agrawal, N. Modeling supply chain contracts: A review. Quant. Models Supply Chain. Manag. 1999, 17, 299–336. [Google Scholar] [CrossRef]
- Luo, J.; Zhang, X.; Wang, C. Using put option contracts in supply chains to manage demand and supply uncertainty. Ind. Manag. Data Syst. 2018, 118, 477–1497. [Google Scholar] [CrossRef]
- Lee, C.; Li, X.; Xie, Y. Procurement risk management using capacitated option contracts with fixed ordering costs. IIE Trans. 2013, 45, 845–864. [Google Scholar] [CrossRef]
- Wright, T.P. Factors affecting the cost of airplanes. J. Aeronaut. Sci. 1936, 3, 122–128. [Google Scholar] [CrossRef]
- Teplitz, C.J. The Learning Curve Deskbook: A Reference Guide to Theory, Calculations and Applications; Quorum Books: New York, NY, USA, 1991; Available online: https://cir.nii.ac.jp/crid/1130282272771944064 (accessed on 6 February 2024).
- Adler, P.S.; Clark, K.B. Behind the learning curve: A sketch of the learning process. Manag. Sci. 1991, 37, 267–281. [Google Scholar] [CrossRef]
- Anzanello, M.J. Learning curve models and applications: Literature review and research directions. Int. J. Ind. Ergon. 2011, 41, 573–583. [Google Scholar] [CrossRef]
- Simatupang, T.M.; Wright, A.C.; Sridharan, R. The knowledge of coordination for supply chain integration. Bus. Process. Manag. J. 2002, 8, 289–308. [Google Scholar] [CrossRef]
- Dar-El, E.M. Human Learning: From Learning Curves to Learning Organizations; Kluver Academic: Norwell, MA, USA, 2000; Volume 29. [Google Scholar] [CrossRef]
- Xu, K.; Chiang, W.; Liang, L. Dynamic pricing and channel efficiency in the presence of the cost learning effect. Int. Trans. Oper. Res. 2011, 18, 579–604. [Google Scholar] [CrossRef]
- Shum, S.; Tong, S.; Xiao, T. On the impact of uncertain cost reduction when selling to strategic customers. Manag. Sci. 2016, 63, 843–860. [Google Scholar] [CrossRef]
- Wong, L.F. A Generalized learning curve adapted for purchasing and cost reduction negotiations. Adv. Oper. Res. 2013, 2013, 584762. [Google Scholar] [CrossRef]
- Timothy, L.S.; Thomas, E. Morton. The effects of learning on optimal lot sizes: Further developments on the single product case. IIE Trans. 1985, 17, 33–37. [Google Scholar] [CrossRef]
- Chand, S. Lot sizes and setup frequency with learning in setups and process quality. Eur. J. Oper. Res. 1989, 42, 190–202. [Google Scholar] [CrossRef]
- Jaber, M.; Bonney, M. Lot sizing with learning and forgetting in set-ups and in product quality. Int. J. Prod. Econ. 2003, 83, 95–111. [Google Scholar] [CrossRef]
- Jaber, M.; Guiffrida, A. Learning curves for processes generating defects requiring reworks. Eur. J. Oper. Res. 2004, 159, 663–672. [Google Scholar] [CrossRef]
- Jaber, M.; Bonney, M.; Guiffrida, A. Coordinating a three-level supply chain with learning-based continuous improvement. Int. J. Prod. Econ. 2010, 127, 27–38. [Google Scholar] [CrossRef]
- Gray, J.; Tomlin, B.; Roth, A. Outsourcing to a powerful contract manufacturer: The effect of learning-by-doing. Prod. Oper. Manag. 2009, 18, 487–505. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, J. Contract preference with stochastic cost learning in a two-period supply chain under asymmetric information. Int. J. Prod. Econ. 2018, 196, 226–247. [Google Scholar] [CrossRef]
- Deng, S.; Guan, X.; Xu, J. The coopetition effect of learning-by-doing in outsourcing. Int. J. Prod. Res. 2021, 59, 516–541. [Google Scholar] [CrossRef]
- Huang, Y.; Wang, Z. Information sharing in a closed-loop supply chain with learning effect and technology licensing. J. Clean. Prod. 2020, 271, 122544. [Google Scholar] [CrossRef]
- Azzouz, A.; Ennigrou, M.; Said, L.B. Scheduling problems under learning effects: Classification and cartography. Int. J. Prod. Res. 2018, 56, 1642–1661. [Google Scholar] [CrossRef]
- Koltai, T.; Kalló, N. Analysis of the effect of learning on the throughput-time in simple assembly lines. Comput. Ind. Eng. 2017, 111, 507–515. [Google Scholar] [CrossRef]
- Li, Y.; Liu, D.; Kucukkoc, I. Mixed-model assembly line balancing problem considering learning effect and uncertain demand. J. Comput. Appl. Math. 2023, 422, 114823. [Google Scholar] [CrossRef]
- Otto, C.; Otto, A. Extending assembly line balancing problem by incorporating learning effects. Int. J. Prod. Res. 2014, 52, 7193–7208. [Google Scholar] [CrossRef]
- Basu, A.; Jain, T.; Hazra, J. Supplier selection under production learning and process improvements. Int. J. Prod. Econ. 2018, 204, 411–420. [Google Scholar] [CrossRef]
- Silbermayr, L.; Minner, S. Dual sourcing under disruption risk and cost improvement through learning. Eur. J. Oper. Res. 2016, 250, 226–238. [Google Scholar] [CrossRef]
- Keachie, E.C.; Fontana, R.J. Effects of learning on optimal lot size. Manage. Sci. 1966, 13, 102–108. [Google Scholar] [CrossRef]
- Yelle, L.E. The learning curve: Historical review and comprehensive survey. Decis. Sci. 1979, 10, 302–328. [Google Scholar] [CrossRef]
- Li, G.; Rajagopalan, S. A learning curve model with knowledge depreciation. Eur. J. Oper. Res. 1998, 105, 143–154. [Google Scholar] [CrossRef]
- Knecht, G. Costing technological growth and generalized learning curves. Oper. Res. Q. 1974, 25, 487–491. [Google Scholar] [CrossRef]
- Nembhard, D.A.; Uzumeri, M.V. An individual-based description of learning within an organization. IEEE Tran. Eng. Manag. 2000, 47, 370–378. [Google Scholar] [CrossRef]
- Glock, C.H.; Grosse, E.H.; Jaber, M.Y.; Smunt, T.L. Applications of learning curves in production and operations management: A systematic literature review. Comput. Ind. Eng. 2019, 131, 422–441. [Google Scholar] [CrossRef]
- Jaber, M.Y.; Peltokorpi, J.; Smunt, T.L. The lot size problem and the learning curve: A review of mathematical modeling (1950’s–2020). Appl. Math. Model. 2022, 105, 832–859. [Google Scholar] [CrossRef]
- Tortorella, G.L.; Flavio, S.F.; Michel, J.A.; Roberto, V.; Jiju, A.; Kevin, O.; Mike, K. Learning curve applications in Industry 4.0: A scoping review. Prod. Plan. Control 2022, 1–13. [Google Scholar] [CrossRef]
- Grosse, E.H.; Glock, C.H.; Müller, S. Production economics and the learning curve: A meta-analysis. Int. J. Prod. Econ. 2015, 170, 401–412. [Google Scholar] [CrossRef]
- Abernathy, W.J.; Wayne, K. Limits of the learning curve. Harv. Bus. Rev. 1974, 52, 109–119. [Google Scholar]
- Jaber, M.; Bonney, M. The economic manufacture/order quantity (EMQ/EOQ) and the learning curve: Past, present, and future. Int. J. Prod. Econ. 1999, 59, 93–102. [Google Scholar] [CrossRef]
- Jaber, M.Y.; Bonney, M. Economic manufacture quantity (EMQ) model with lot-size dependent learning and forgetting rates. Int. J. Prod. Econ. 2007, 108, 359–367. [Google Scholar] [CrossRef]
- Jaber, M.Y.; Bonney, M. Economic lot sizing with learning and continuous time discounting: Is it significant? Int. J. Prod. Econ. 2001, 71, 135–143. [Google Scholar] [CrossRef]
- Heese, H. Single versus multiple sourcing and the evolution of bargaining positions. Omega 2015, 54, 125–133. [Google Scholar] [CrossRef]
- Agrawal, A.; Kim, Y.; Kwon, H.D.; Muthulingam, S. Investment in shared suppliers: Effect of learning, spillover, and competition. Prod. Oper. Manag. 2016, 25, 736–750. [Google Scholar] [CrossRef]
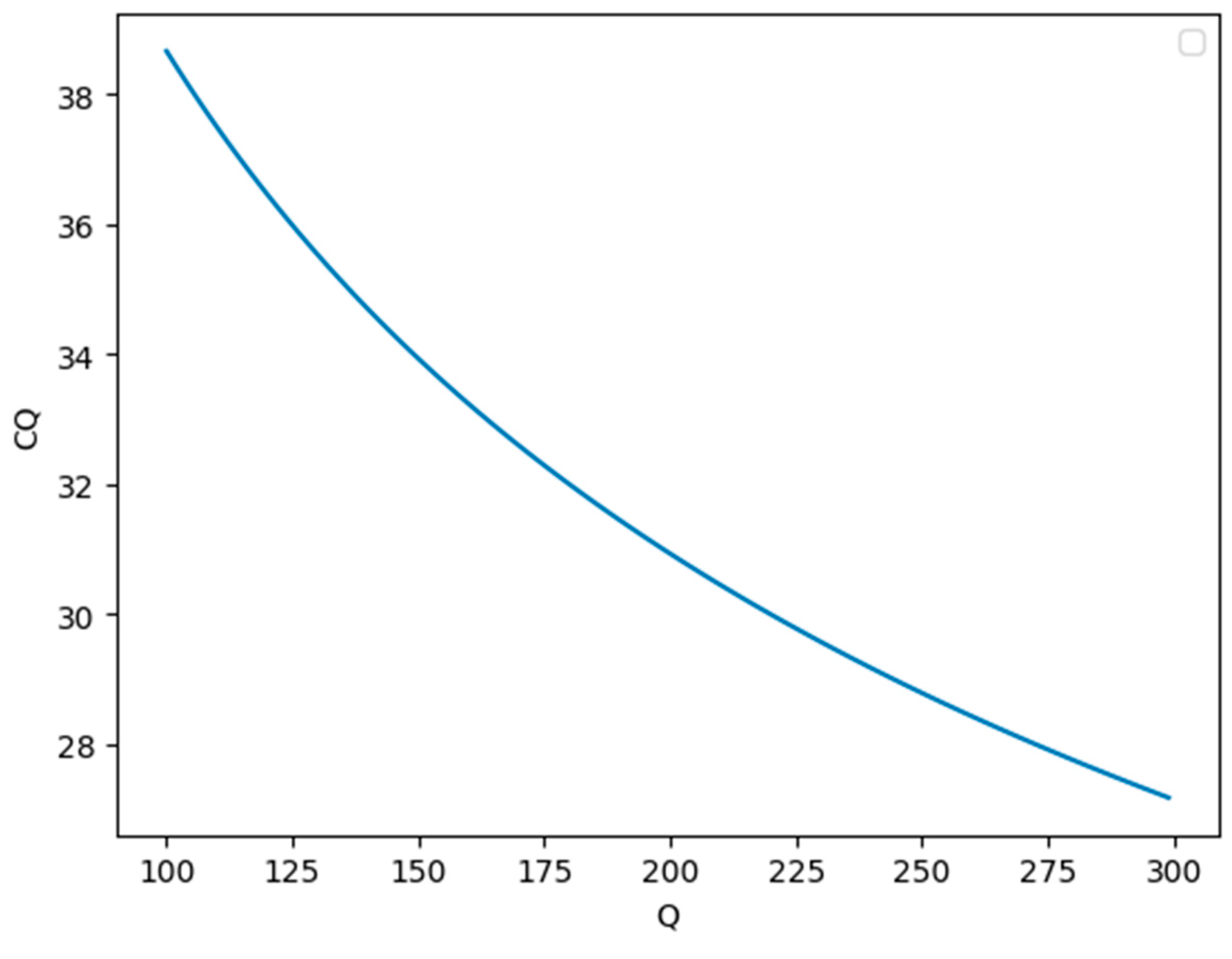
| w* | α | ||||
|---|---|---|---|---|---|
| 0.167 | 8.161 | 0 | 5011 | 5011 | 0.000 |
| 0.200 | 10.368 | 296 | 4714 | 5011 | 0.059 |
| 0.300 | 11.472 | 1185 | 3825 | 5011 | 0.237 |
| 0.400 | 12.576 | 2074 | 2936 | 5011 | 0.414 |
| 0.500 | 13.681 | 2964 | 2047 | 5011 | 0.591 |
| 0.600 | 14.785 | 3853 | 1158 | 5011 | 0.769 |
| 0.700 | 15.889 | 4742 | 269 | 5011 | 0.946 |
| 0.730 | 16.224 | 5011 | 0 | 5011 | 1.000 |
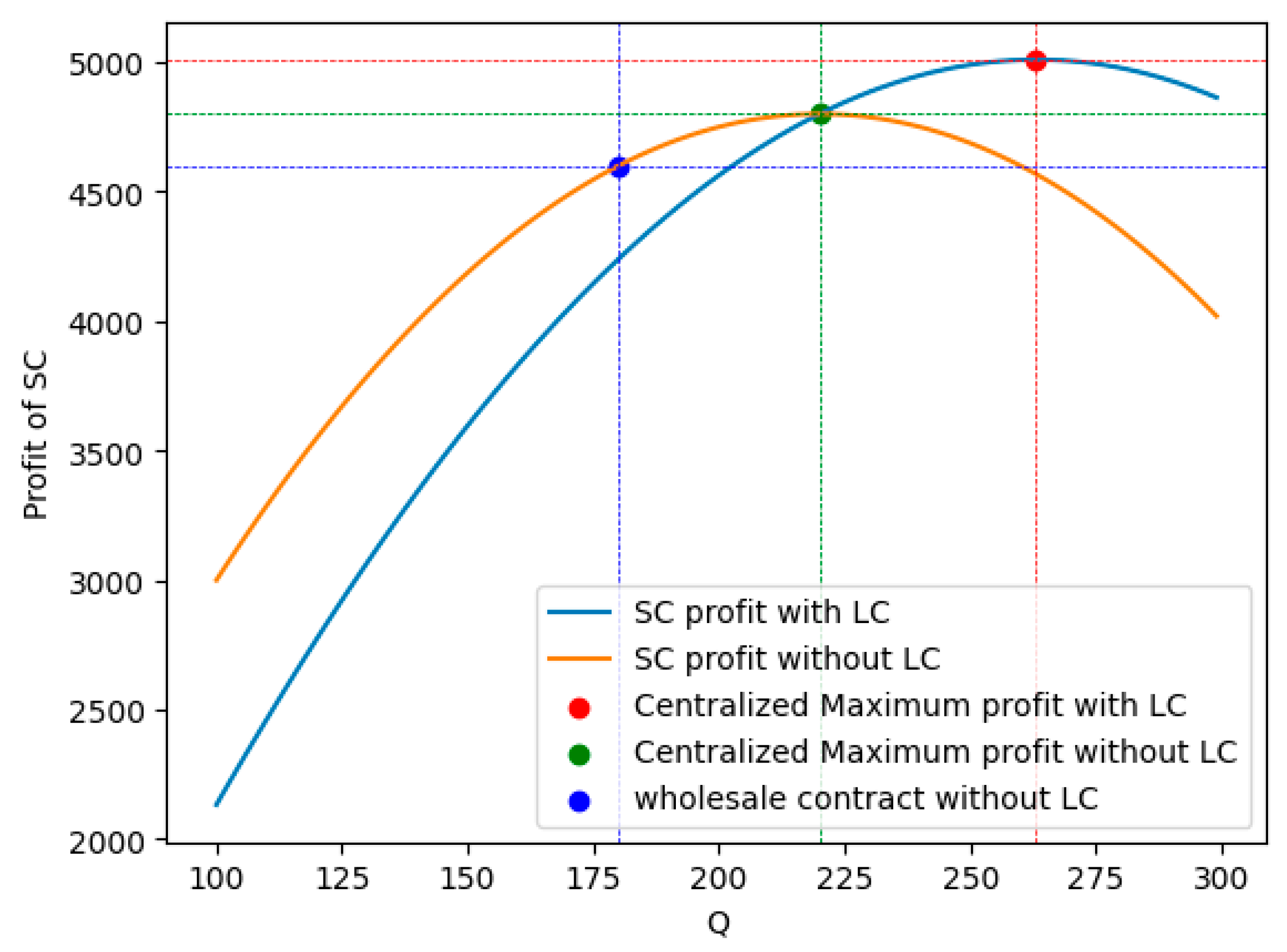
| Wholesale | Revenue-Sharing without LC | Revenue-Sharing with LC | |
|---|---|---|---|
| 180 | 220 | 263 | |
| w | 40 | 22.17 | 13.79 |
| ϕ | - | 0.6739 | 0.5101 |
| 2800 | 2922 | 3053 | |
| 1800 | 1878 | 1958 | |
| 4600 | 4800 | 5011 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tao, Z.-J.; Koo, P.-H. A Coordinated Supply Contract for a Two-Echelon Supply Chain Considering Learning Effects. Appl. Sci. 2024, 14, 1513. https://doi.org/10.3390/app14041513
Tao Z-J, Koo P-H. A Coordinated Supply Contract for a Two-Echelon Supply Chain Considering Learning Effects. Applied Sciences. 2024; 14(4):1513. https://doi.org/10.3390/app14041513
Chicago/Turabian StyleTao, Ze-Jin, and Pyung-Hoi Koo. 2024. "A Coordinated Supply Contract for a Two-Echelon Supply Chain Considering Learning Effects" Applied Sciences 14, no. 4: 1513. https://doi.org/10.3390/app14041513
APA StyleTao, Z.-J., & Koo, P.-H. (2024). A Coordinated Supply Contract for a Two-Echelon Supply Chain Considering Learning Effects. Applied Sciences, 14(4), 1513. https://doi.org/10.3390/app14041513






