Improving Passband Characteristics in Chebyshev Sharpened Comb Decimation Filters
Abstract
Featured Application
Abstract
1. Introduction
- A generalized approach is provided since all designs are based on sinusoidal magnitude responses and optimizing the amplitudes of sinusoidal responses using PSO.
- The flexibility of the design is shown in choosing narrowband or wideband designs and a trade-off between the quality of compensation and the number of required adders.
- The design is simple since only one parameter in the narrowband case and two parameters in the wideband case should be optimized, unlike the approaches in the literature based on optimizing the compensator filter coefficients.
2. Compensators: Applications and Design
2.1. What Is a Compensated Modified Comb Decimator, and Why Is It Important?
- Efficient Sample Rate Reduction. The compensated modified comb decimator impressively reduces the sampling rate without introducing aliasing and distortion, thereby saving memory and processing resources. This efficiency is particularly valuable in high-speed analog-to-digital converter (ADC) systems.
- Low Complexity. The compensated modified comb decimators, with their simple structure involving addition and subtraction operations, offer reassurance in terms of computational efficiency. This simplicity makes them highly suitable for hardware implementation.
- Improved Signal Integrity. The compensated modified comb decimator plays a crucial role in ensuring improved signal integrity. By attenuating undesired spectral components and artifacts, they instill confidence in the fidelity of the decimated signal.
- Scalable Decimation. Compensated modified comb decimators can be cascaded for multi-stage decimation, reducing the complexity of handling large decimation factors.
2.2. Design
2.2.1. Narrowband Design
2.2.2. Wideband Design
Extension of Narrowband Design
Simplified Version of Compensator in [14]
2.3. How to Obtain the Compensator Design Parameters?
3. Passband Compensation of Chebyshev Sharpened Combs from [8,10,11]
3.1. Steps of Design
3.2. Coleman Chebyshev Sharpened Comb [8]
3.2.1. Narrowband Compensation
3.2.2. Wideband Compensation
- Compensator C2
- Compensator C3
3.3. Chebyshev Sharpened Comb Filters [10,11]
3.3.1. Narrowband Compensation
3.3.2. Wideband Compensation
- Compensator C2
- Compensator C3
4. Results
4.1. Comparisons
4.1.1. Comparison with Method in [10]
- Chebyshev sharpening polynomial p(x) = 1 − 29x + 215x2, x = H(z, 1, 32)
- Chebyshev Sharpening Polynomial p(x) = 1 − 210x + 217x2, x = H(z, 1, 32)
- Chebyshev sharpening polynomial p(x) = −1 + 27(26x − 214x2 + 220x3), x = H(z, 1, 32)
- Chebyshev sharpening polynomial p(x) = −1 + 27(24x − 210x2 + 214x3), x = H(z, 1, 32)
4.1.2. Comparison with Method in [11]
- Chebyshev sharpening polynomial p(x) = 1 − 29x2 + 215x4, x = H(z, 1, 32)
- Chebyshev sharpening polynomial p(x) = −1 + 27(24x − 210x2 + 214x3), x = H(z, 1, 32)
4.1.3. Comparison with Method in [12]
4.1.4. Comparison with Method in [7]
4.2. Principal Features of the Proposed Method
- The method includes the compensation of Coleman Chebyshev comb filters, which have a favorite characteristic of providing high and equal attenuations in all folding bands.
- The choice of narrowband and wideband compensators depends on the passband of interest. For the wideband case, there is a choice between two compensators depending on the number of adders and the compensation quality.
- All designs have the option of optimal multiplier and multiplierless designs.
- Design flexibility presented as a trade-off between the number of adders and the quality of compensations.
- Simplicity of design since there is only one parameter in the narrowband case and two parameters in the wideband case to optimize.
- The comparisons with the methods from the literature demonstrate the advantages of the proposed method.
4.3. Possible Practical Applications
- Communication Systems. Providing efficient decimation since the signals are often decimated to match a target sampling rate. In radio frequency (RF) signal processing, these filters can significantly improve the performance of intermediate frequency (IF) decimation stages by reducing signal distortion and improving signal-to-noise ratio.
- Biomedical Signal Processing. These filters can be used to decimate high-frequency sampled biomedical signals to preserve signal information by accurately retaining the key features of the original signal while reducing the data rate.
- Audio Signal Processing. To maintain high sound quality during resampling or multi-rate processing, minimize aliasing and maintain audio integrity.
- Scientific Instrumentation. Preservation signal quality in high-resolution imaging or spectroscopy applications. Accurate decimation of geophysical signals aids in the real-time monitoring and analysis of seismic data.
4.4. Potential Future Research Work
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kaiser, J.; Hamming, R. Sharpening the response of a symmetric non-recursive filter by multiple use of the same filter. IEEE Trans. Acoust. Speech Signal Process 1977, 25, 415–422. [Google Scholar] [CrossRef]
- Kwentus, A.Y.; Zhongnong, J.; Willson, A.N. Application of filter sharpening to cascaded integrator-comb decimation filters. IEEE Trans. Signal Process 1997, 45, 457–467. [Google Scholar] [CrossRef]
- Neeraja, P.K.; Bindiya, T.S.; Ragh, C.W. Low Power Implementation of Compensated and Sharpened CIC Decimation Filter. In Proceedings of the IEEE 9th Uttar Pradesh International Conference on Electrical, Electronics and Computer Engineering (UPCON 2022), Allahabad, India, 2–4 December 2022. [Google Scholar] [CrossRef]
- Dudarin, A.; Goran Molnar, G.; Vucic, M. Optimum multiplierless sharpened cascaded-integrator-comb filters. Dig Sig. Proc 2022, 127, 103564. [Google Scholar] [CrossRef]
- Aggarwal, S. Efficient design of decimation filter using linear programming and its FPGA implementation. Integration 2021, 79, 94–106. [Google Scholar] [CrossRef]
- Aggarwal, S.; Meher, P.K. Enhanced sharpening of CIC decimation filters implementation and applications. Circ. Syst. Signal Proc. 2022, 41, 4581–4603. [Google Scholar] [CrossRef]
- Gautam, D.; Khare, K.; Shrivastava, B.P. A novel approach for optimal design of sample rate conversion filter using linear optimization technique. IEEE Access 2021, 9, 44436–44444. [Google Scholar] [CrossRef]
- Coleman, J.O. Chebyshev stopbands for CIC decimation filters and CIC-implemented array tapers in 1D and 2D. IEEE Trans. Circ. Syst. I Reg. Pap. 2012, 59, 2956–2968. [Google Scholar] [CrossRef]
- Molnar, G.; Dudarin, A.; Vucic, M. Minimax design of multiplierless sharpened CIC filters based on interval analysis. In Proceedings of the 39th International Convention on Information and Communication Technology, Electronics and Microelectronics, (MIPRO 2016), Opatija, Croatia, 30 May–3 June 2016; pp. 94–98. [Google Scholar] [CrossRef]
- Molnar, G.; Dudarin, A.; Vucic, M. Design and multiplierless realization of maximally flat sharpened-CIC compensators. IEEE Trans. Circ. Syst. II Express Briefs 2018, 65, 51–55. [Google Scholar] [CrossRef]
- Dudarin, A.; Molnar, G.; Vucic, M. Optimum multiplierless compensators for sharpened cascaded-integrator-comb decimation filters. Electron. Lett. 2018, 54, 971–972. [Google Scholar] [CrossRef]
- Jovanovic Dolecek, G.; Martinez Novelo, G. Design of compensator for Chebyshev sharpened comb filter. In Proceedings of the 2023 IEEE 33rd International Conference on Microelectronics (MIEL), Nis, Serbia, 16–18 October 2023. [Google Scholar] [CrossRef]
- Jovanovic Dolecek, G.; Fernandez-Vazquez, A. Trigonometrical approach to design a simple wideband comb compensator. AEU-Int. J. Electron. Commun. 2014, 68, 437–441. [Google Scholar] [CrossRef]
- Jovanovic Dolecek, G.; Garcia Baez, R.; Molina Salgado, G.; de la Rosa, J.M. Novel multiplierless wideband comb compensator with high compensation capability. Circ. Syst. Signal Proc. 2017, 36, 2031–2049. [Google Scholar] [CrossRef]
- Jovanovic Dolecek, G.; de la Rosa, J.M. Design of wideband comb compensator based on magnitude response using two sinusoidals and particle swarm optimization. AEU-Int. J. Electron. Commun. 2021, 130, 153570. [Google Scholar] [CrossRef]
- Xu, L.; Yang, L.W.; Tian, H. Design of wideband CIC compensator based on particle swarm optimization. Circ. Syst. Signal Proc. 2019, 38, 1833–1846. [Google Scholar] [CrossRef]
- Zhibin, L.; Ruotong, Y.; Min, G. Efficient sharpening CIC filter embedding fifth-order filter with coefficient optimisation algorithm. Electron. Lett. 2020, 56, 1241–1243. [Google Scholar] [CrossRef]
- Harris, F. Multirate Signal Processing for Communications Systems; River Publisher: Gistrup, Denmark, 2021. [Google Scholar]
- Milic, L.J. Multirate Filtering for Digital Signal Processing; IGI Global: Hershey, PA, USA, 2009. [Google Scholar]
- de la Rosa, J.M. Sigma-Delta Converters, Practical Design Guide, 2nd ed.; Wiley-IEEE Press: New Jersey, NY, USA, 2018; pp. 20–27. [Google Scholar]
- Tobin, P. PSpice for Digital Signal Processing. Synthesis Lectures on Digital Circuits & Systems; Springer: Cham, Switzerland, 2007; pp. 109–131. [Google Scholar] [CrossRef]
- White, B.A.; Elmasri, M.I. Low-power design of decimation filters for a digital IF receiver. IEEE Trans. Very Large Scale Integr. (Vlsi) Syst. 2000, 8, 339–345. [Google Scholar] [CrossRef]
- Abinaya, A.; Maheswari, M. A survey of digital down converter architecture for next generation wireless applications. Proc. Iop Conf. Ser. Mater. Sci. Eng. 2000, 872, 012037. [Google Scholar] [CrossRef]
- Chukwuchekwa, N. Illustration of Decimation in Digital Signal Processing (DSP) Systems Using MATLAB. 2009. Available online: https://www.researchgate.net/publication/334432641 (accessed on 10 November 2024).
- Wang, W.; Jin, X.; Quan, D.; Zhu, M.; Wang, X.; Zheng, M.; Li, J.; Chen, J. Rate adaptive compressed sampling based on region division for wireless sensor networks. Sci. Rep. 2024, 14, 1–13. [Google Scholar] [CrossRef]
- Gan, C.; Li, X. Improved CIC Decimation Filter on Software Defined Radio. In Proceedings of the 2021 9th International Conference on Communications and Broadband Networking (ICCBN 21), Shanghai China, 25–27 February 2021; pp. 232–238. [Google Scholar] [CrossRef]
- Ainsworth, M.; Klasky, S.; Whitney, B. Compression using lossless decimation analysis and application. SIAM J. Sci. Comput. 2017, 39, B732–B757. [Google Scholar] [CrossRef]
- Yang, M.; Guerra, R.; Han, C.; López, J.F. A near lossless data compression method for the geostationary interferometric infrared sounder (GIIRS) based on the combination of decimation and quantization. In Proceedings of the IEEE 8th Joint Int Inf Tech and Artific Intell Conf (ITAIC2019), Chongqing, China, 24–26 May 2019. [Google Scholar] [CrossRef]
- Rahate, T.W.; Ladhake, S.A.; Ghate, U.S. Decimator filter for hearing aid application based on FPGA. Int. Res. J. Eng. Tech. (IRJET) 2018, 5, 1175–1183. [Google Scholar]
- Lin, L.; Gao, B.; Gong, M. An area-efficient decimation filter on high resolution Sigma Delta A/D converter for biomedical signal processing. In Proceedings of the 2020 IEEE International Conference on Advances in Electrical Engineering and Computer Applications (AEECA), Dalian, China, 25–27 August 2020. [Google Scholar] [CrossRef]
- Yang, R.; Liu, H.; Luo, Z. Optimization design of decimation filter for the phasemeter in the space gravitational wave detection. IEEE Trans. Inst. Meas. 2024, 73, 1–8. [Google Scholar] [CrossRef]
- Smalt, C.J.; Brungart, D.S. Digital sampling of acoustic impulse noise: Implications for exposure measurement and damage risk criteria. J. Acoust. Soc. Am. 2022, 152, 1283–1291. [Google Scholar] [CrossRef] [PubMed]
- Available online: https://www.mathworks.com/help/gads/particleswarm.html (accessed on 15 September 2024).
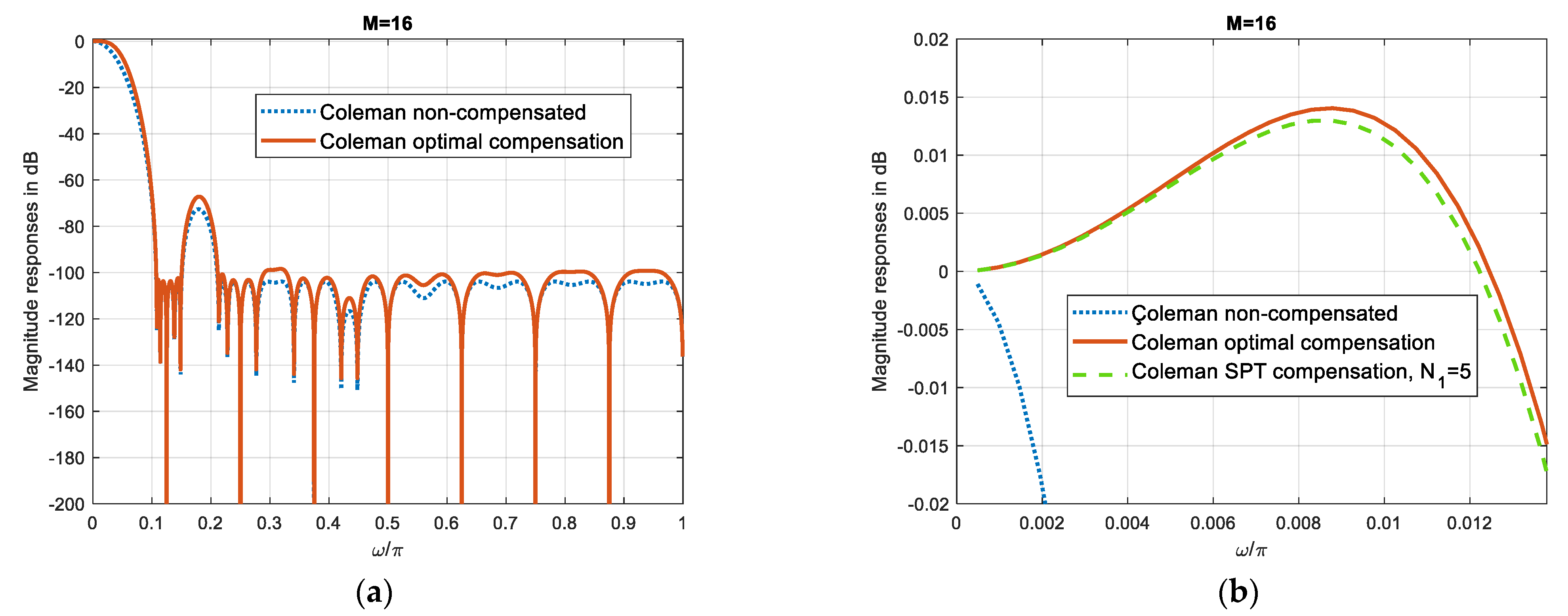

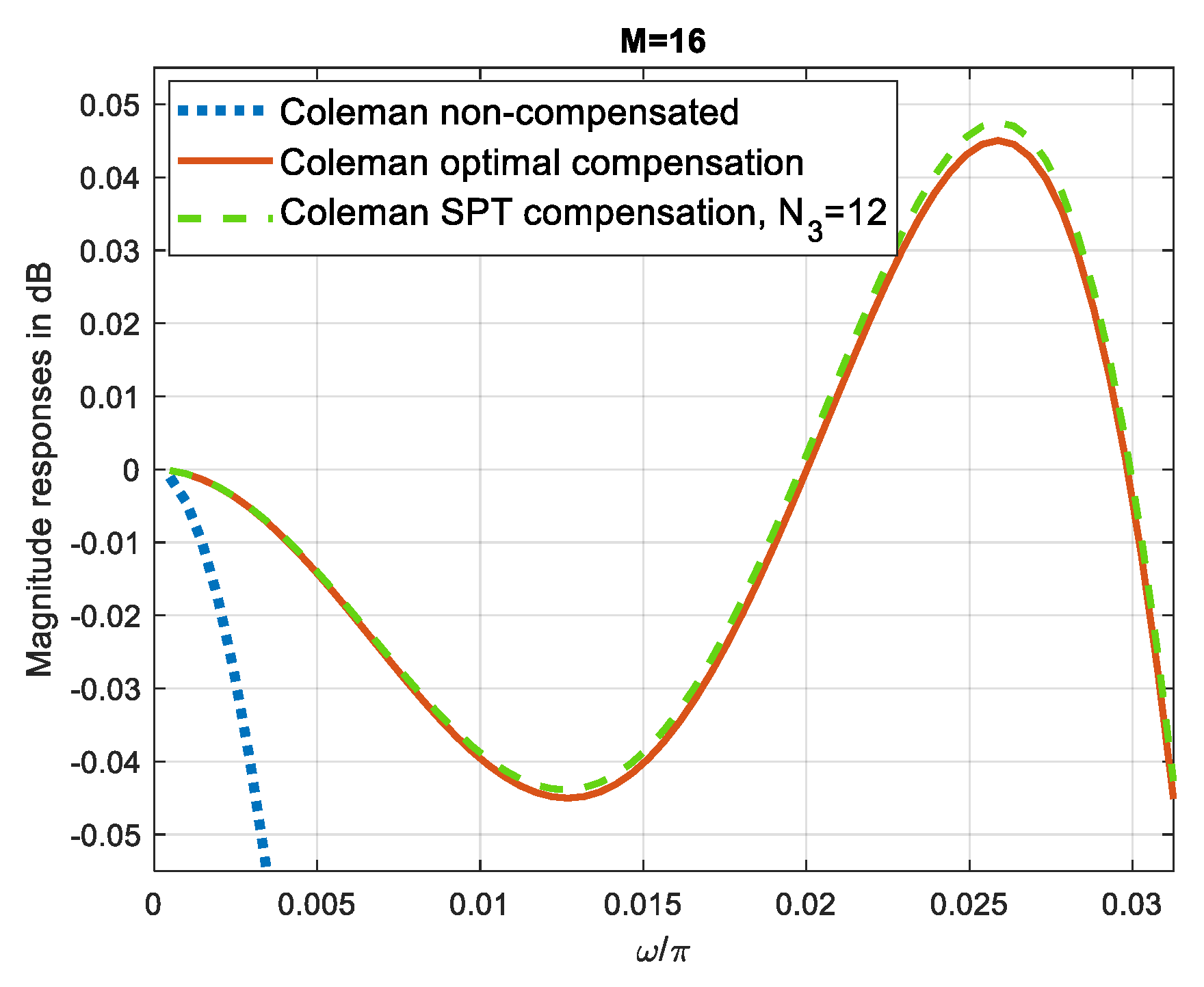
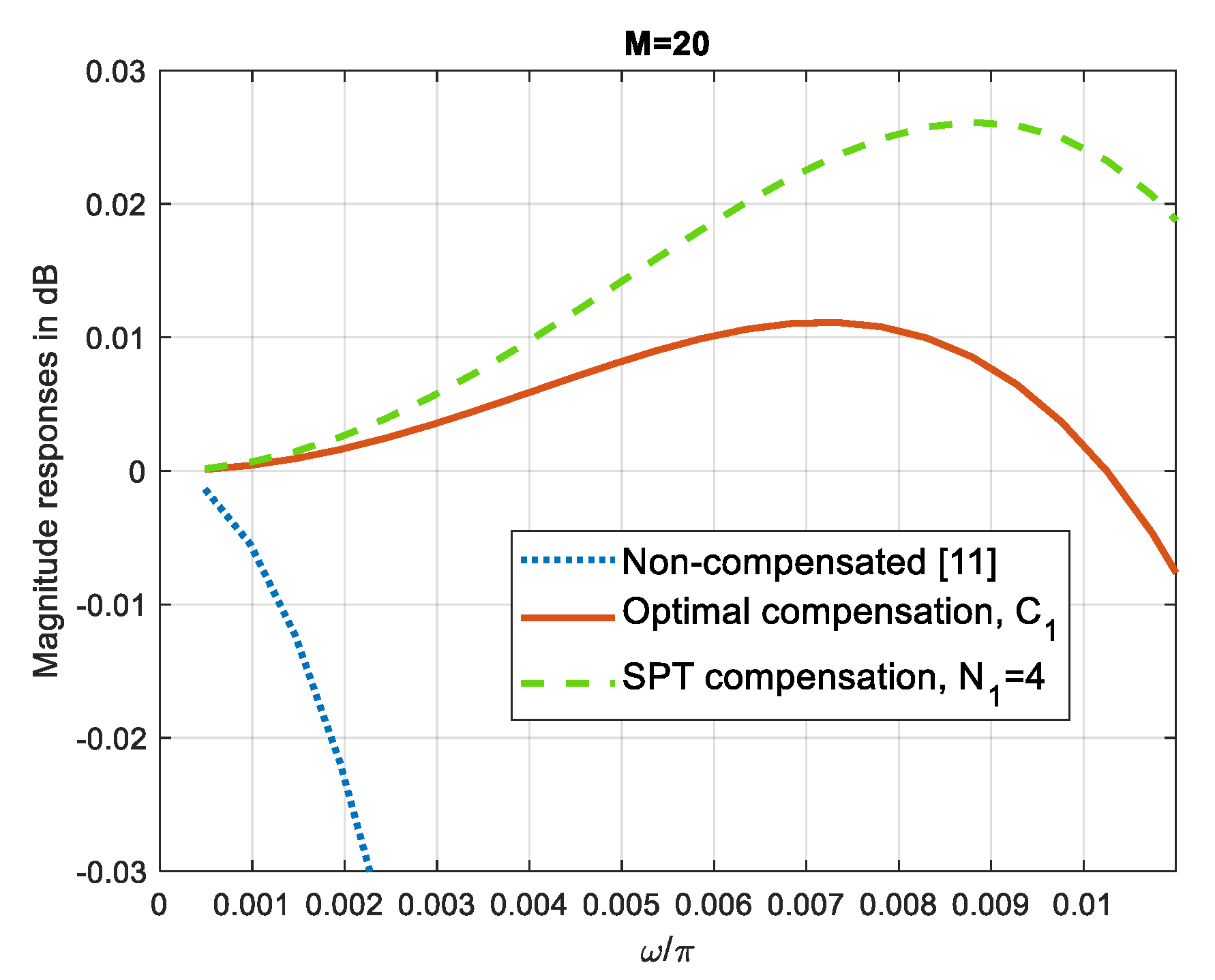
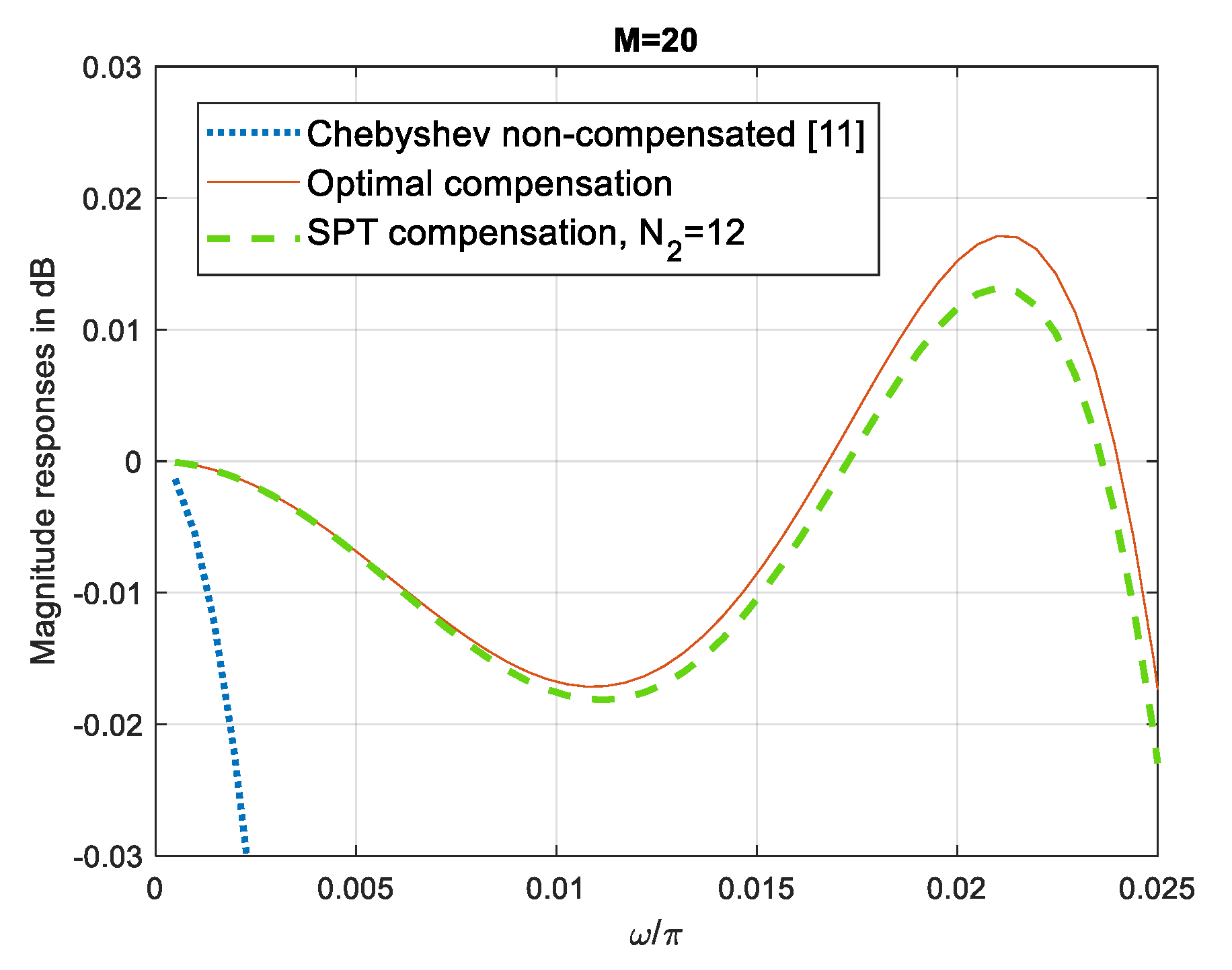

| C11 | δ1 [dB] | N1 |
|---|---|---|
| 20-2−4 | 0.0274 | 4 |
| 20-2−3 + 2−5 | 0.0165 | 5 |
| 20-2−3 + 2−5 + 2−8 | 0.0145 | 6 |
| 20-2−3 + 2−5 + 2−8-2−10 | 0.0142 | 7 |
| C21 | C22 | δ2 [dB] | N2 |
|---|---|---|---|
| 20-2−2 | 20-2−3 | 0.1232 | 11 |
| 20-2−2 + 2−5 | 20-2−3 | 0.0307 | 12 |
| 20-2−2 + 2−5 | 20-2−3 + 2−7 | 0.0297 | 13 |
| 20-2−2 + 2−5 + 2−8 | 20-2−3-2−10 | 0.0271 | 14 |
| 20-2−2 + 2−5 + 2−7 | 20-2−3-2−6 + 2−8 | 0.0247 | 15 |
| C31 | C32 | δ3 [dB] | N3 |
|---|---|---|---|
| 20-2−2 | 20-2−2 + 2−6 | 0.0616 | 9 |
| 20-2−2-2−8 | 20-2−2 + 2−6 | 0.0532 | 11 |
| 20-2−2-2−5 + 2−7 | 20 + 2−2 + 2−4 | 0.0474 | 12 |
| 20-2−2-2−5 + 2−7 | 20 + 2−2 + 2−4-2−9 | 0.04591 | 13 |
| 20-2−2-2−5 + 2−7-2−10 | 20-2−2 + 2−4-2−14 | 0.0452 | 16 |
| C11 | δ1 [dB] | N1 |
|---|---|---|
| 20-2−2 | 0.0299 | 4 |
| 20-2−2-2−5 | 0.0125 | 6 |
| 20-2−2-2−5-2−8 | 0.0115 | 7 |
| 20-2−2-2−5-2−8 + 2−11 | 0.0110 | 8 |
| C21 | C22 | δ2 [dB] | N2 |
|---|---|---|---|
| 2−1 + 2−3 | 2−1 + 2−3 | 0.0485 | 11 |
| 2−1 + 2−3 + 2−7 | 2−1 + 2−3 | 0.0227 | 12 |
| 2−1 + 2−3 + 2−7 | 2−1 + 2−3 + 2−8 | 0.00177 | 13 |
| 2−1 + 2−3 + 2−7 + 2−11 | 2−1 + 2−3 + 2−9 | 0.0174 | 14 |
| 2−1 + 2−3 + 2−7 + 2−10 | 2−1 + 2−3 + 2−10 + 2−12 | 0.0172 | 15 |
| C31 | C32 | δ3 [dB] | N |
|---|---|---|---|
| 2−1-2−4 | 20-2−4 | 0.0597 | 9 |
| 2−1 + 2−4-2−6 | 20-2−4 | 0.0404 | 10 |
| 2−1 + 2−3 + 2−6 | 20-2−3-2−6 | 0.0373 | 9 |
| 2−1 + 2−3-2−5 + 2−9 | 20-2−3 + 2−6 | 0.0317 | 13 |
| 2−1 + 2−3-2−5 + 2−11 | 20-2−3 + 2−6 + 2−8 | 0.0304 | 14 |
| C11 | δ1 [dB] | N1 |
|---|---|---|
| 2−1-2−3 | 0.0169 | 4 |
| 2−1-2−3-2−6 | 0.0064 | 5 |
| 2−1-2−3-2−6-2−8 | 0.0045 | 6 |
| 2−1-2−3-2−6-2−8-2−11 | 0.0043 | 7 |
| C11 | δ1 [dB] | N1 |
|---|---|---|
| 2−1-2−3 | 0.0157 | 4 |
| 2−1-2−3-2−5 | 0.0015 | 5 |
| 2−1-2−3-2−5-2−11 | 0.0012 | 6 |
| C11 | δ1 [dB] | N1 |
|---|---|---|
| 2−1 + 2−5 | 0.0056 | 4 |
| 2−1 + 2−5-2−8 | 0.0042 | 5 |
| 2−1 + 2−5-2−7 + 2−9 | 0.0036 | 6 |
| 2−1 + 2−5-2−7 + 2−9-2−11 | 0.0035 | 7 |
| C31 | C32 | δ3 [dB] | N3 |
|---|---|---|---|
| 2−1-2−13 | 2−1-2−4 | 0.0101 | 9 |
| 2−1-2−13-2−16 | 2−1-2−4 | 0.0100 | 11 |
| 2−1-2−11-2−13 | 2−1-2−4 + 2−10 | 0.0098 | 12 |
| C11 | δ1 [dB] | N1 |
|---|---|---|
| 2−1-2−3 | 0.0169 | 4 |
| 2−1-2−3-2−6 | 0.0064 | 5 |
| 2−1-2−3-2−6-2−8 | 0.0045 | 6 |
| 2−1-2−3-2−6-2−8-2−11 | 0.0043 | 7 |
| C31 | C32 | δ3 [dB] | N3 |
|---|---|---|---|
| 20-2−3 | 21-2−3 | 0.0776 | 9 |
| 20-2−3 + 2−7 | 21-2−3 | 0.0601 | 11 |
| 20-2−3 + 2−6-2−9 | 21-2−3-2−7 | 0.0587 | 13 |
| 20-2−3 + 2−6 + 2−9 | 21-2−2 + 2−4 + 2−7 | 0.0555 | 16 |
| C21 | C22 | δ2 [dB] | N2 |
|---|---|---|---|
| 2−1 + 2−5 | 2−2 + 2−6 | 0.0307 | 11 |
| 2−1-2−5 + 2−8 | 2−1 + 2−7 | 0.0276 | 12 |
| 2−1-2−5 + 2−8 | 2−1 + 2−7 + 2−9 | 0.0273 | 13 |
| 2−1-2−5 + 2−8 + 2−10 | 2−1 + 2−7-2−11 | 0.0266 | 14 |
| 2−1 + 2−5 + 2−7-2−9-2−11 | 2−1 + 2−7-2−9 + 2−11 | 0.0262 | 16 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jovanovic Dolecek, G.; Fernandez-Vazquez, A. Improving Passband Characteristics in Chebyshev Sharpened Comb Decimation Filters. Appl. Sci. 2024, 14, 11421. https://doi.org/10.3390/app142311421
Jovanovic Dolecek G, Fernandez-Vazquez A. Improving Passband Characteristics in Chebyshev Sharpened Comb Decimation Filters. Applied Sciences. 2024; 14(23):11421. https://doi.org/10.3390/app142311421
Chicago/Turabian StyleJovanovic Dolecek, Gordana, and Alfonso Fernandez-Vazquez. 2024. "Improving Passband Characteristics in Chebyshev Sharpened Comb Decimation Filters" Applied Sciences 14, no. 23: 11421. https://doi.org/10.3390/app142311421
APA StyleJovanovic Dolecek, G., & Fernandez-Vazquez, A. (2024). Improving Passband Characteristics in Chebyshev Sharpened Comb Decimation Filters. Applied Sciences, 14(23), 11421. https://doi.org/10.3390/app142311421






