Accuracy of Dynamic Modulus Models of Asphalt Mixtures Containing Reclaimed Asphalt (RA)
Abstract
1. Introduction
2. Theoretical Background: Predictive Models
2.1. Witczak Model: Sigmoid Function
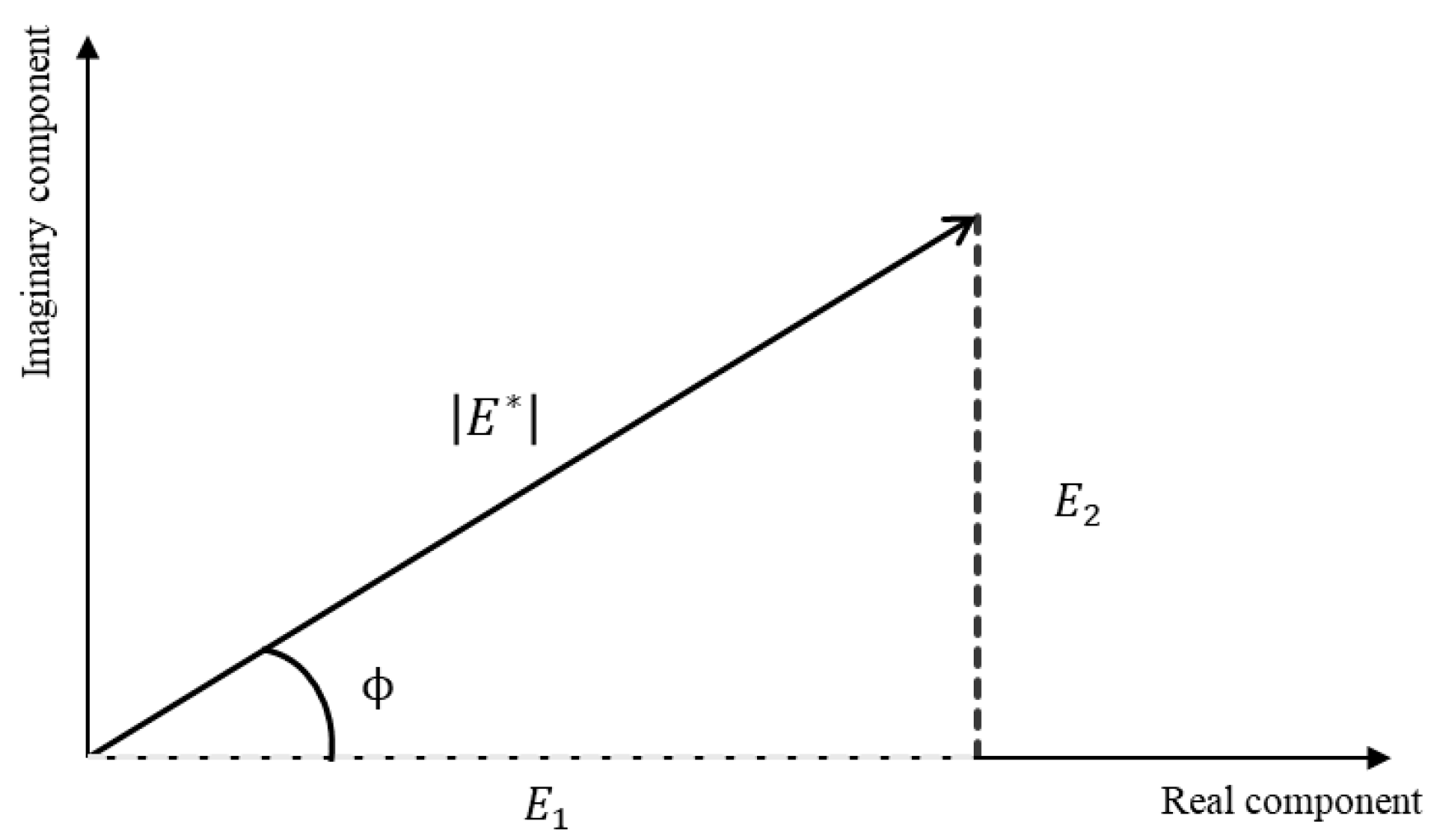
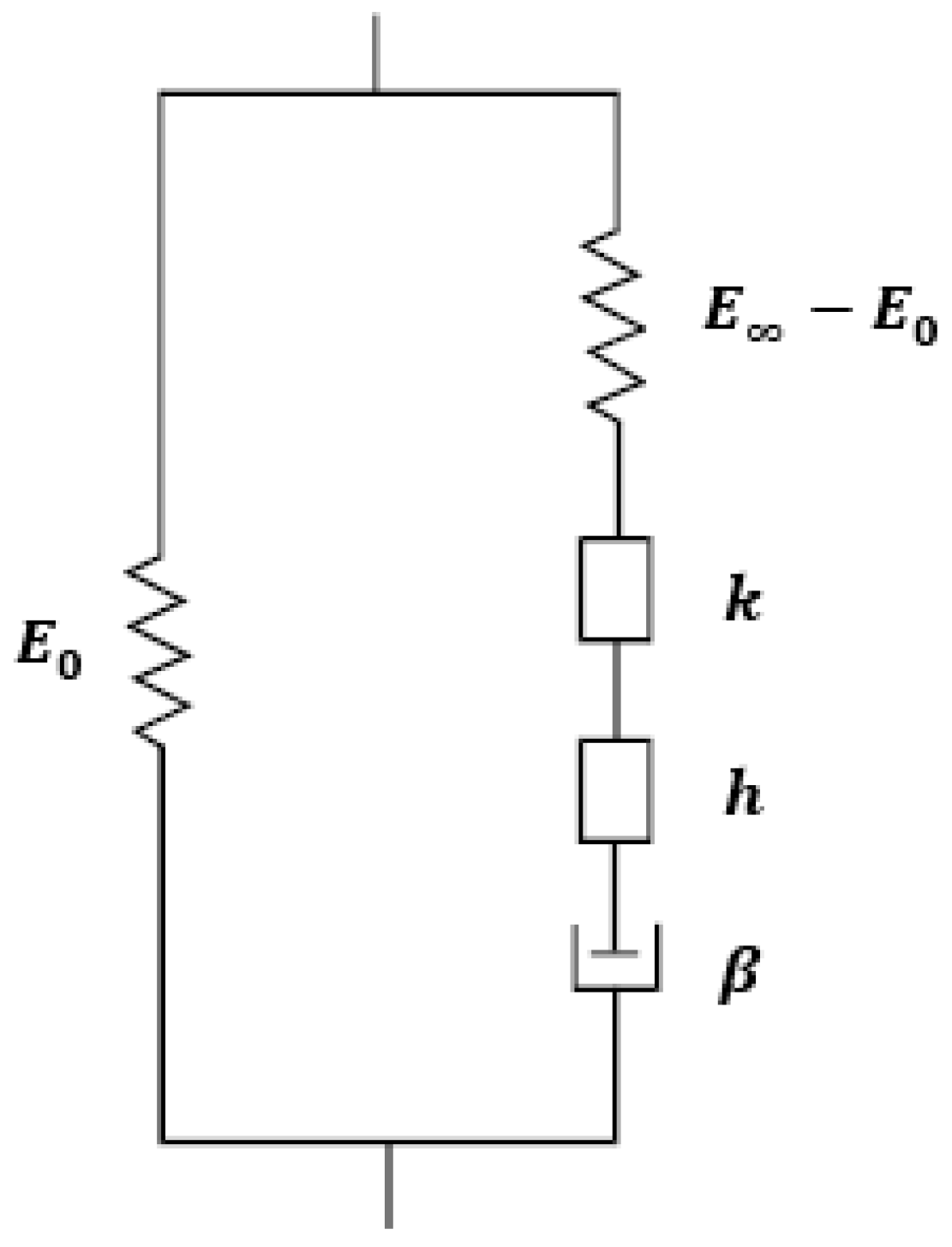
2.2. Generalized Huet–Sayegh Model (2S2P1D)
3. Experimental Materials and Testing
| 0% | 30% | 40% | 50% | EN Standard | |
|---|---|---|---|---|---|
| Bulk density [g.cm−3] | 2.392 | 2.368 | 2.400 | 2.390 | EN 12697-6 [20] |
| Maximum density [g.cm−3] | 2.538 | 2.520 | 2.524 | 2.553 | EN 12697-5 [21] |
| Voids content [%-vol.] | 5.7 | 6.0 | 4.9 | 6.4 | |
| Moisture susceptibility, ITSR [%] | 94.0 | 77.4 | 71.4 | 79.6 | EN 12697-12 [22] |
| Stiffness @15 °C [MPa] | 7654 | 10,760 | 11,159 | 17,497 | EN 12697-26, annex C [3] |
| Fracture toughness [N/mm3/2] | 35 | 39 | 38 | 39 | EN 12697-44 [23] |
| Added virgin asphalt binder [% mass] | 0 | 2.7 | 2.0 | 1.6 | |
| Total asphalt binders in the mix [% mass] | 4.5 | 4.5 | 4.5 | 4.5 |
| 50/70 Asphalt Binder Properties | EN Standard | |
|---|---|---|
| Penetration @25 °C [0.1 mm] | 54 | EN 1426 [24] |
| Softening point (R&B) [°C] | 50 | EN 1427 [25] |

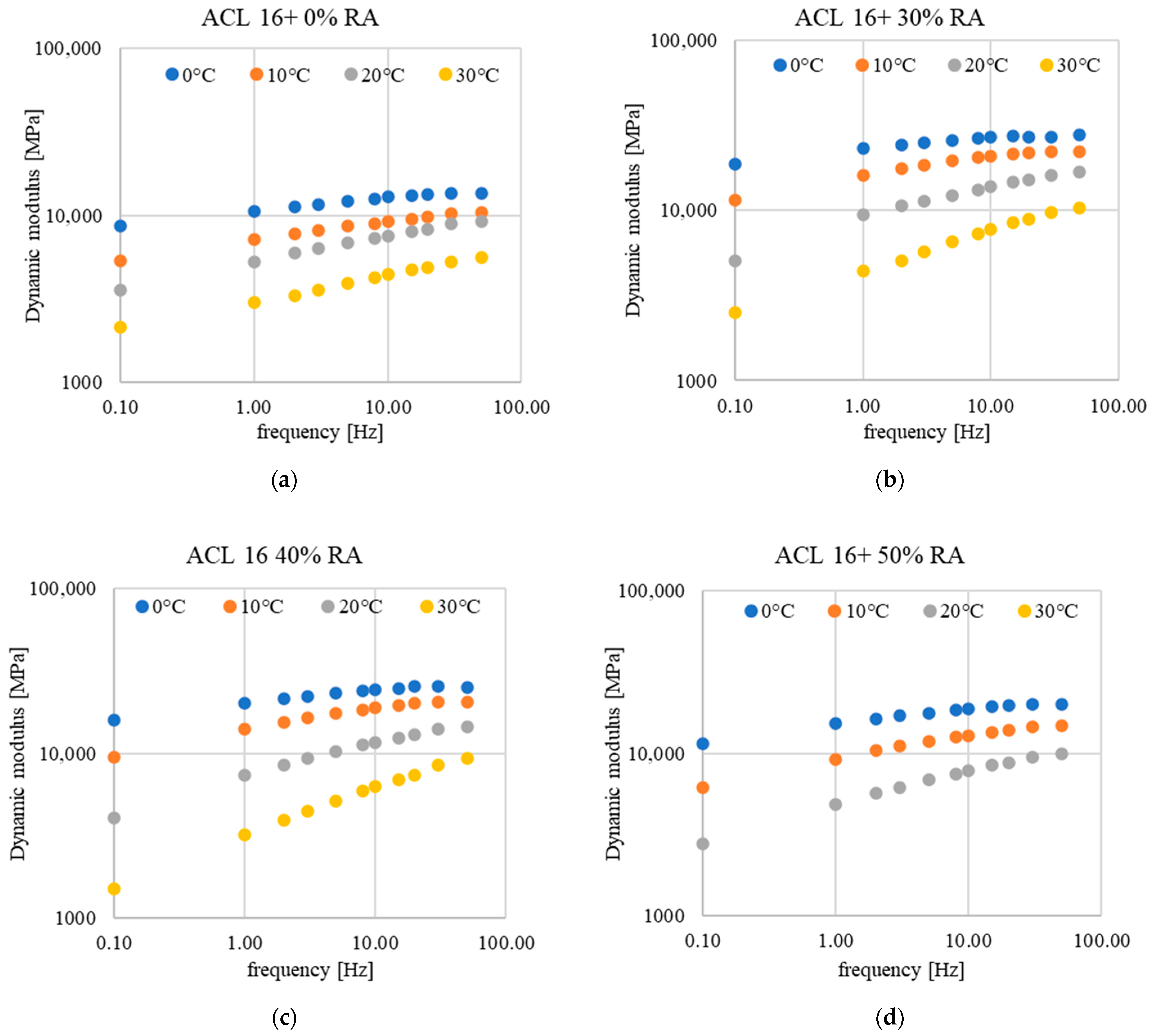
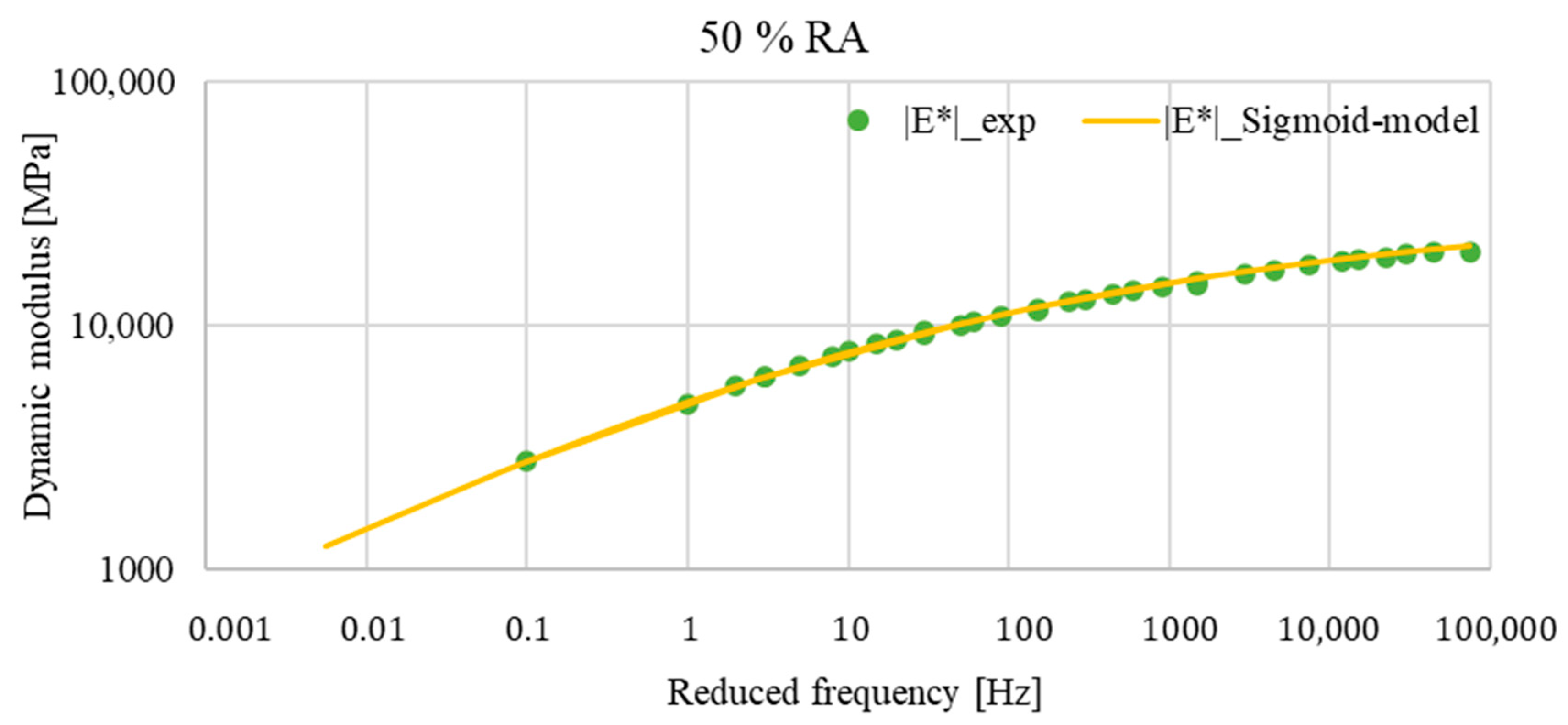
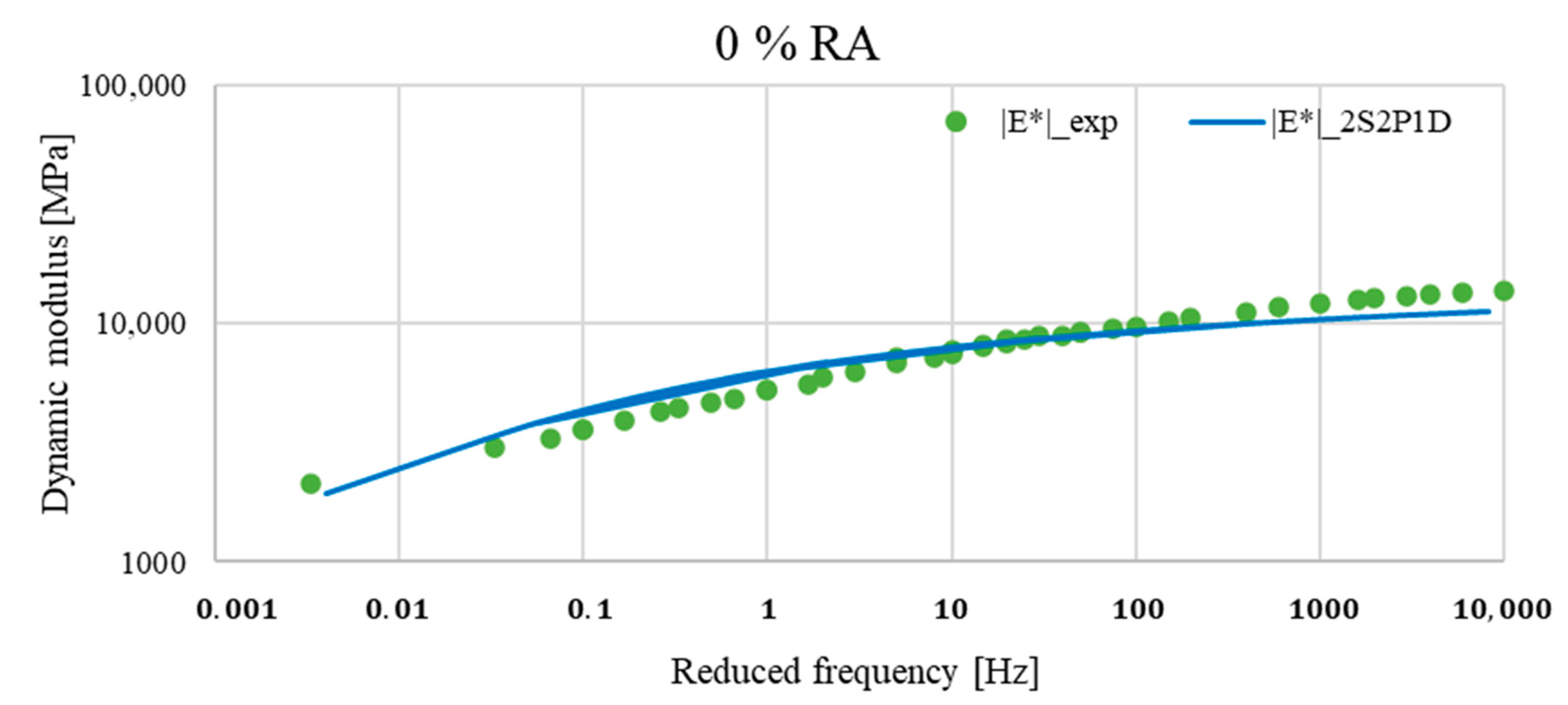
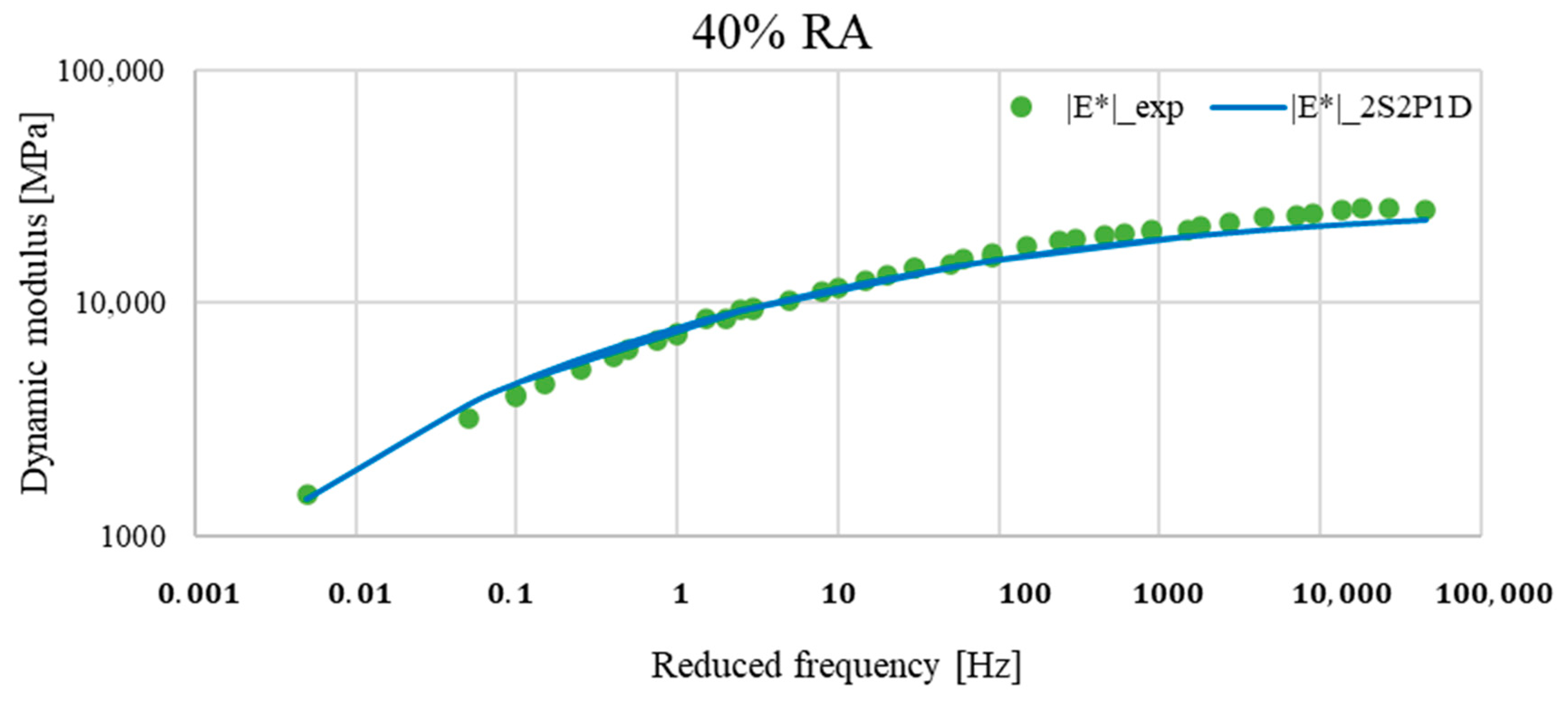
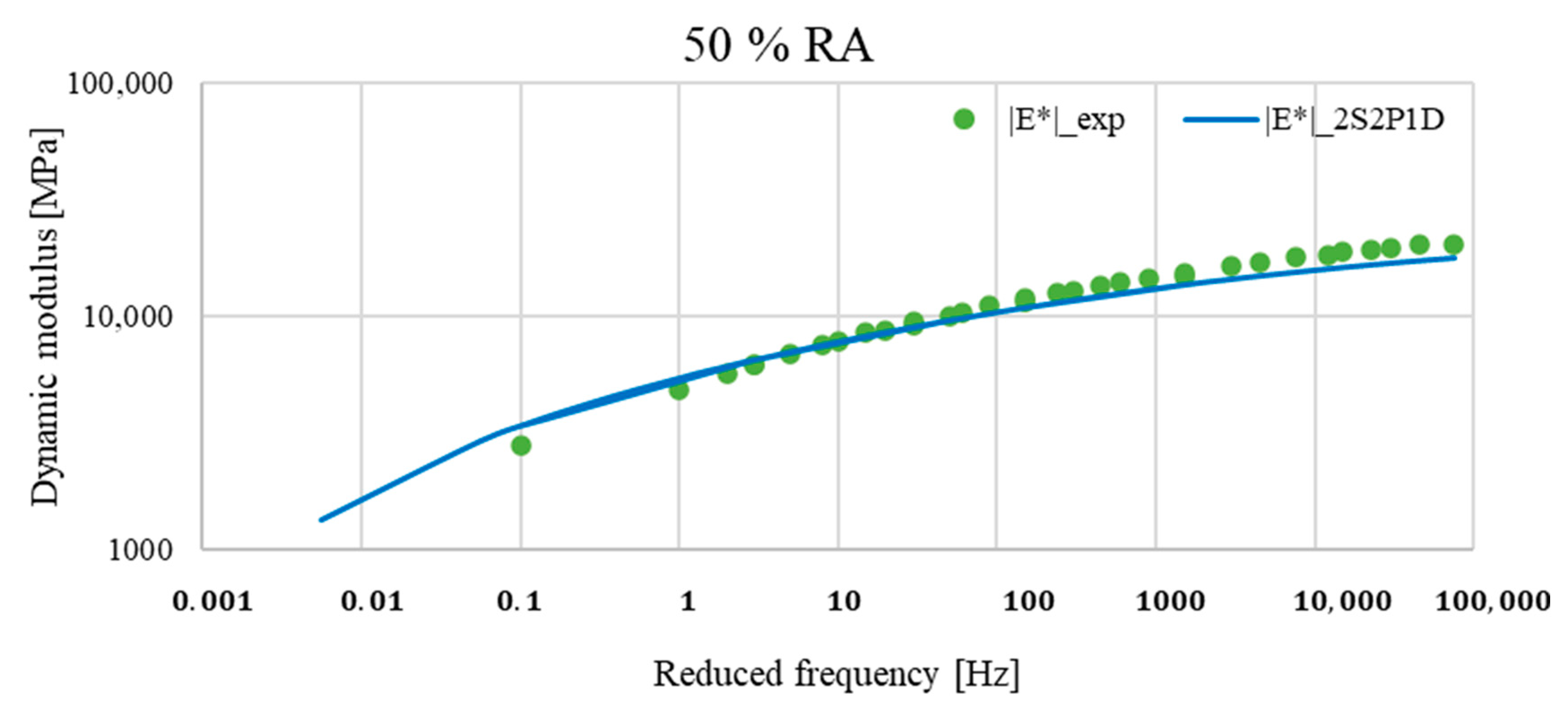
4. Experimental Results: Master Curve Construction
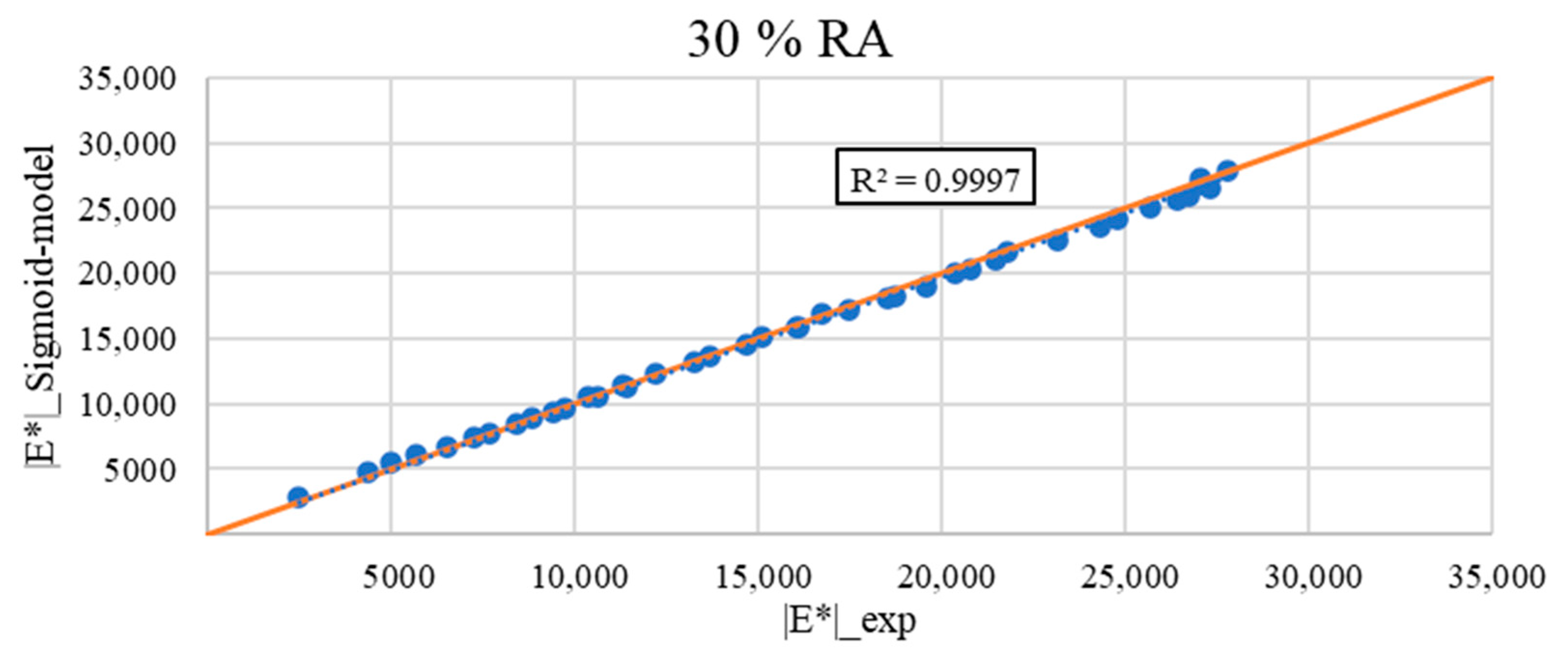
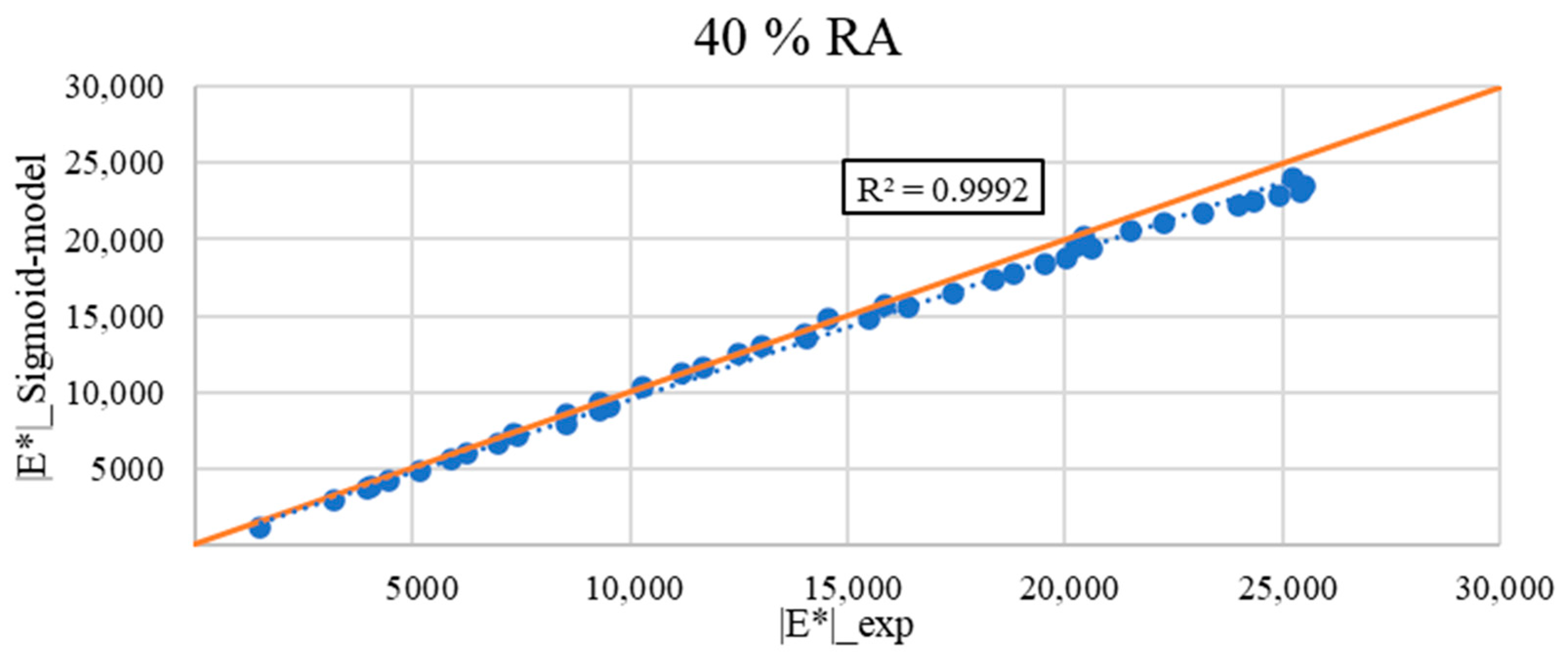
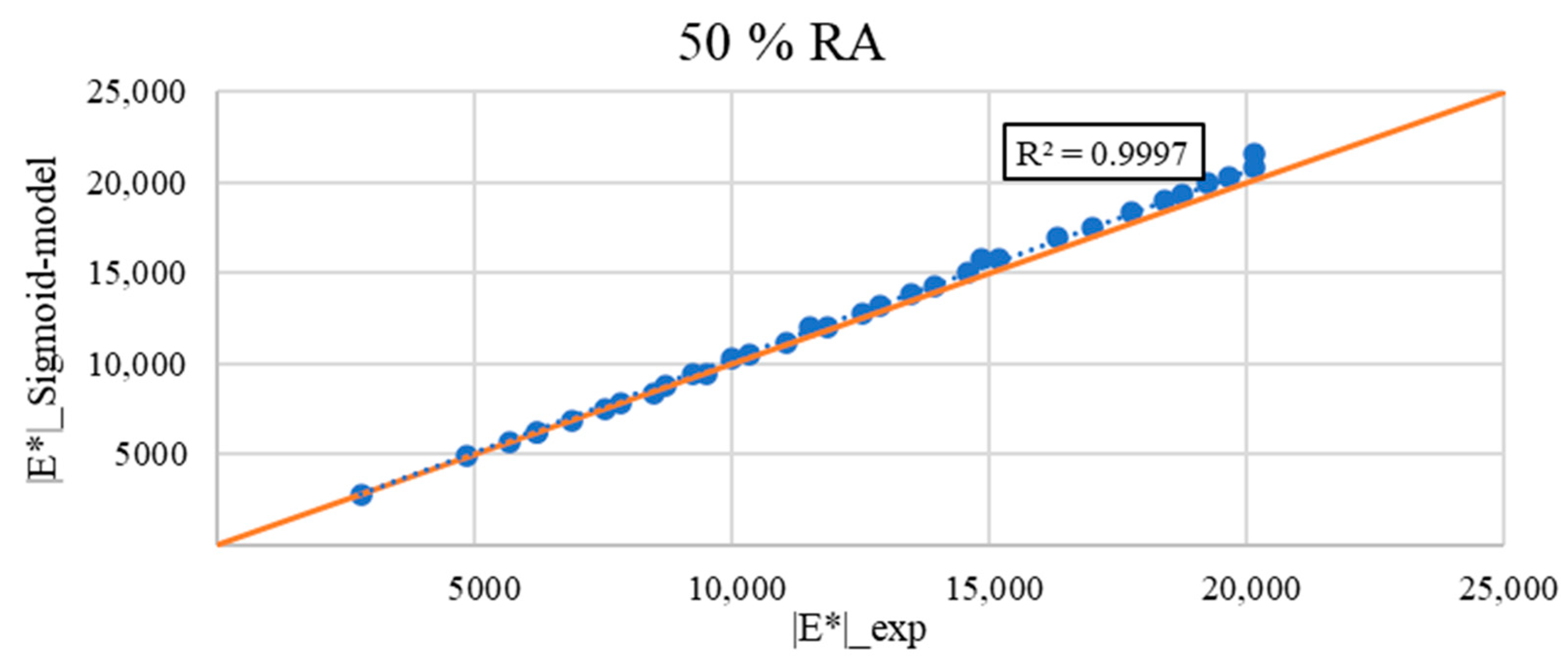
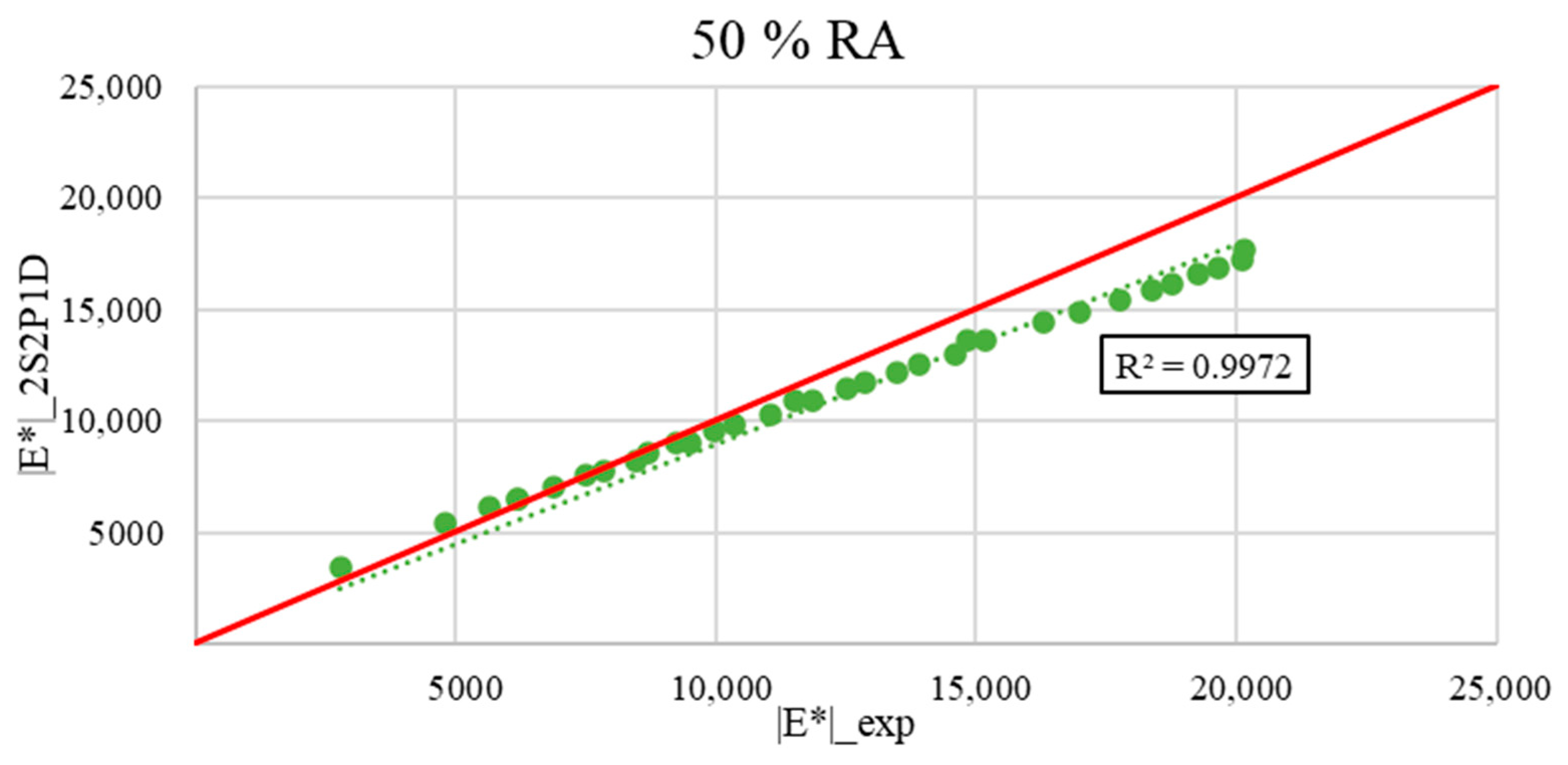
5. Parameter Acquisition
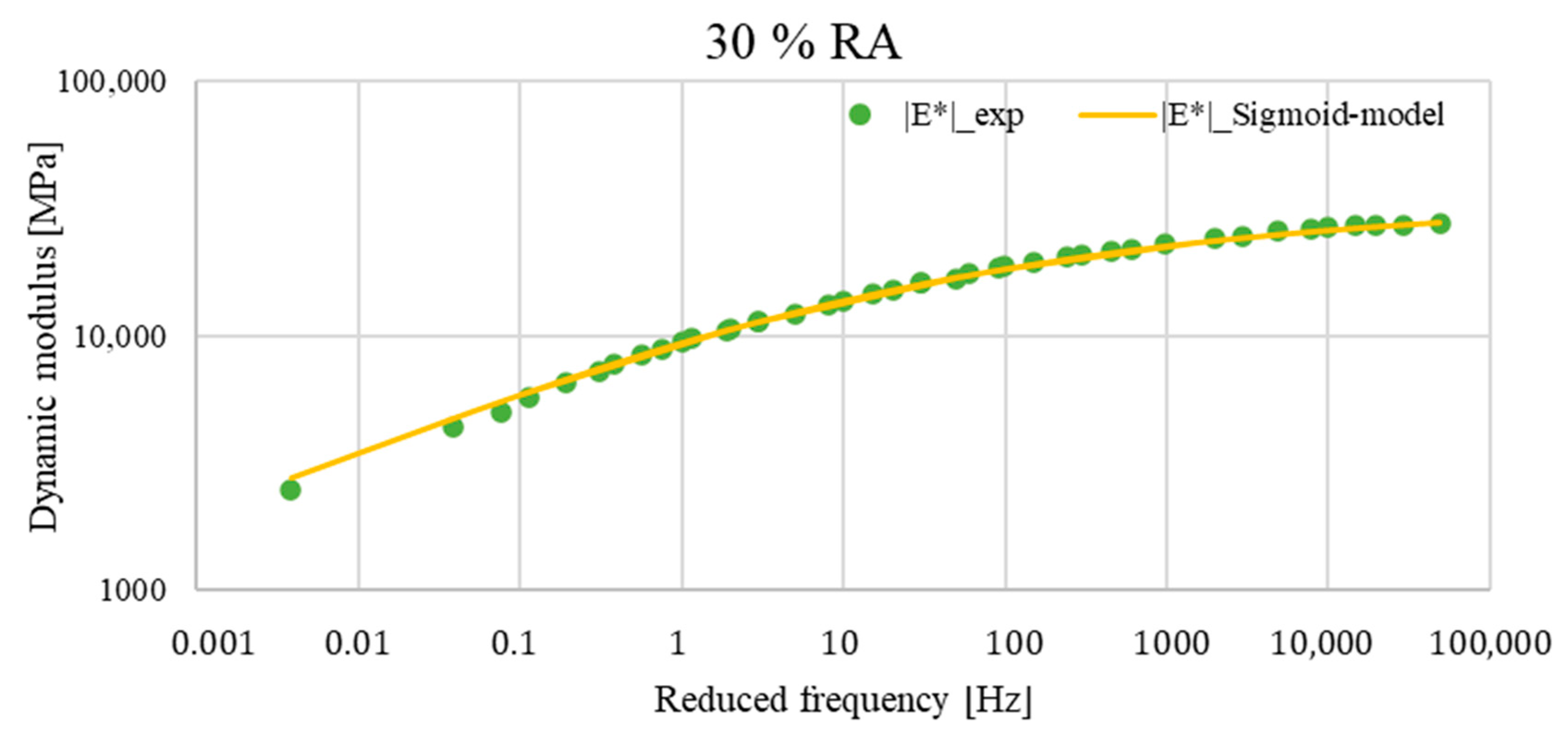
5.1. Time–Temperature Superposition
5.2. Witczak Model: Sigmoid Function
5.3. Generalized Huet–Sayegh Model (2S2P1D)
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pellinen, T.K. Investigation of the Use of Dynamic Modulus as an Indicator of Hot Mix Asphalt; Arizona State University: Tempe, AZ, USA, 2001. [Google Scholar]
- Witzcak, M.W. Simple Performance Test for Superpave Mix Design; Transportation Research Board: Washington, DC, USA, 2002; Volume 465. [Google Scholar]
- EN 12697-26; Bituminous Mixtures—Test Methods for Hot Mix Asphalt—Part 26: Stiffness. European Committee for Standardization: Brussels, Belgium, 2012.
- Witczak, M.W.; Bari, J. Development of a Master Curve (E*) Database for Lime Modified Asphaltic Mixtures; Arizona State University Research Report; Arizona State University: Tempe, AZ, USA, 2004. [Google Scholar]
- Bari, J. Development of a New Revised Version of the Witczak E* Predictive Models for Hot Mix Asphalt Mixtures. Ph.D. Dissertation, Arizona State University, Tempe, AZ, USA, 2005. [Google Scholar]
- Shu, X.; Huang, B. Micromechanics-based dynamic modulus prediction of polymeric asphalt concrete mixtures. Compos. Part B Eng. 2008, 39, 704–713. [Google Scholar] [CrossRef]
- Cho, Y.H.; Park, D.W.; Hwang, S.D. A predictive equation for dynamic modulus of asphalt mixtures used in Korea. Constr. Build. Mater. 2010, 24, 513–519. [Google Scholar] [CrossRef]
- Zhu, H.; Sun, L.; Yang, J.; Chen, Z.; Gu, W. Developing master curves and predicting dynamic modulus of polymer-modified asphalt mixtures. J. Mater. Civ. Eng. 2011, 23, 131–137. [Google Scholar] [CrossRef]
- Wu, H.; Zhan, Y.; Song, W.; Xu, S.; Chen, X.; Liao, H. Prediction modelling on dynamic modulus of recycled asphalt mixtures based on meso-mechanical analysis. J. Clean. Prod. 2024, 469, 143200. [Google Scholar] [CrossRef]
- Hossain, Z.; Zaman, M. Prediction of Dynamic Modulus of Hot Mix Asphalts with Reclaimed Asphalt Pavement. Adv. Civ. Eng. 2020, 2020, 8672654. [Google Scholar] [CrossRef]
- Dao, D.V.; Nguyen, N.L.; Ly, H.B.; Pham, B.T.; Le, T.T. Cost-effective approaches based on machine learning to predict dynamic modulus of warm mix asphalt with high reclaimed asphalt pavement. Materials 2020, 13, 3272. [Google Scholar] [CrossRef] [PubMed]
- Al-Khateeb, G.; Shenoy, A.; Gibson, N.; Harman, T. A new simplistic model for dynamic modulus predictions of asphalt paving mixtures. Assoc. Asph. Paving Technol. 2006, 75. [Google Scholar]
- Witczak, M.W.; Fonseca, O.A. Revised predictive model for dynamic (complex) modulus of asphalt mixtures. Transp. Res. Rec. 1996, 1540, 15–23. [Google Scholar] [CrossRef]
- Far, M.S.S. Development of New Dynamic Modulus (E*) Predictive Models for Hot Mix Asphalt Mixtures; North Carolina State University ProQuest Dissertations Publishing: Raleigh, NC, USA, 2011. [Google Scholar]
- Schwartz, C.W. Evaluation of the Witczak dynamic modulus prediction model. In Proceedings of the 84th Annual Meeting of the Transportation Research Board, Washington, DC, USA, 9–13 January 2005. [Google Scholar]
- Ceylan, H.; Schwartz, C.W.; Kim, S.; Gopalakrishnan, K. Accuracy of predictive models for dynamic modulus of hot-mix asphalt. J. Mater. Civ. Eng. 2009, 21, 286–293. [Google Scholar] [CrossRef]
- Garcia, G.; Thompson, M. HMA Dynamic Modulus Predictive Models (A Review); FHWA-ICT-07-005; Illinois Center for Transportation: Rantoul, IL, USA, 2007. [Google Scholar]
- Tóth, C.; Ureczky, J. Determination of master curves for asphalt mixtures by means of IT-CY tests. Period. Polytech. Civ. Eng. 2010, 54, 137–142. [Google Scholar] [CrossRef][Green Version]
- Benedetto, H.D. Modelling the rheological properties of bituminous binders using the 2S2P1D Model. Constr. Build. Mater. 2013, 38, 395–406. [Google Scholar]
- EN 12697-6; Bituminous Mixtures—Test Methods—Part 6: Determination of Bulk Density of Bituminous Specimens. European Committee for Standardization: Brussels, Belgium, 2018.
- EN 12697-5; Bituminous Mixtures—Test Methods—Part 5: Determination of the Maximum Density. European Committee for Standardization: Brussels, Belgium, 2018.
- EN 12697-12; Bituminous Mixtures—Test Methods—Part 12: Determination of the Water Sensitivity of Bituminous Specimens. European Committee for Standardization: Brussels, Belgium, 2018.
- EN 12697-44; Bituminous Mixtures—Test Methods—Part 44: Crack Propagation by Semi-Circular Bending Test. European Committee for Standardization: Brussels, Belgium, 2019.
- EN 1426; Bitumen and Bituminous Binders—Determination of Needle Penetration. European Committee for Standardization: Brussels, Belgium, 2015.
- EN 1427; Bitumen and Bituminous Binders—Determination of the Softening Point—Ring and Ball Method. European Committee for Standardization: Brussels, Belgium, 2015.
- ČSN 73 6121:2019; Road Building—Asphalt Pavement Courses—Construction and Conformity Assessment. Česká agentura pro Standardizaci: Prague, Czech Republic, 2019.
- EN 13 108-1; Bituminous Mixtures—Material Specifications–Part 1: Asphalt Concrete. European Committee for Standardization: Brussels, Belgium, 2017.
- EN 12697-33; Bituminous Mixtures—Test Method—Part 33: Specimen Prepared by Roller Compactor. European Committee for Standardization: Brussels, Belgium, 2019.
- Booshehrian, A.; Mogawer, W.S.; Bonaquist, R. How to Construct an Asphalt Binder Master Curve and Assess the Degree of Blending between RAP and Virgin Binders. J. Mater. Civ. Eng. 2013, 25, 1813–1821. [Google Scholar] [CrossRef]
- Adams, Y.E.; Zeghal, M.; El Hussein, H.M. Complex Modulus Test Protocol and Procedure for Determining Huet-Sayegh Model Parameters; NRC-CNRC; National Research Council of Canada: Ottawa, ON, Canada, 2006. [Google Scholar]











| Reclaimed Asphalt | |
|---|---|
| Asphalt binder content | 5.7–5.9% |
| Asphalt binder properties | PEN 16 to 24 and softening point 65 to 72 °C |
| Grading | 0/8 mm |
| RA (%) | 0 °C | 10 °C | 20 °C | 30 °C | ||||
|---|---|---|---|---|---|---|---|---|
| a(T) | log(a(T)) | a(T) | log(a(T)) | a(T) | log(a(T)) | a(T) | log(a(T)) | |
| 0 | 200 | 2.301 | 5 | 0.699 | 1 | 0 | 0.033 | −1.481 |
| 30 | 980 | 2.991 | 30 | 1.477 | 1 | 0 | 0.038 | −1.420 |
| 40 | 900 | 2.954 | 30 | 1.477 | 1 | 0 | 0.05 | −1.301 |
| 50 | 1500 | 3.176 | 30 | 1.477 | 1 | 0 | - | - |
| RA (%) | Regression Parameters Representing the Material Characteristics (Polynomial Second Degree) | |||
|---|---|---|---|---|
| a | b | c | R² | |
| 0 | 2.217 | −0.130 | 0.000301 | 0.98 |
| 30 | 2.992 | −0.154 | 0.000235 | 1.00 |
| 40 | 2.963 | −0.156 | 0.000440 | 1.00 |
| 50 | 3.176 | −0.181 | 0.001109 | 1.00 |
| RA (%) | Regression Parameters of the Sigmoid Function | |||
|---|---|---|---|---|
| δ | α | β | γ | |
| 0 | 2.373 | 2.032 | −0.775 | −0.361 |
| 30 | 2.447 | 2.087 | −0.996 | −0.451 |
| 40 | 0.004 | 4.451 | −1.862 | −0.473 |
| 50 | 1.353 | 3.149 | −1.055 | −0.373 |
| RA (%) | Generalized Huet–Sayegh Model Parameters | |||||
|---|---|---|---|---|---|---|
| E0 | Einf | k | h | α | β | |
| 0 | 760 | 13,966 | 0.157 | 0.539 | 1.469 | 2,259,955 |
| 30 | 1154 | 27,717 | 0.215 | 0.749 | 2.728 | 2,259,953 |
| 40 | 214 | 26,105 | 0.242 | 0.725 | 3.559 | 2,259,961 |
| 50 | 16 | 23,935 | 0.195 | 0.670 | 4.644 | 2,259,953 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belhaj, M.; Valentin, J.; Baldo, N. Accuracy of Dynamic Modulus Models of Asphalt Mixtures Containing Reclaimed Asphalt (RA). Appl. Sci. 2024, 14, 10505. https://doi.org/10.3390/app142210505
Belhaj M, Valentin J, Baldo N. Accuracy of Dynamic Modulus Models of Asphalt Mixtures Containing Reclaimed Asphalt (RA). Applied Sciences. 2024; 14(22):10505. https://doi.org/10.3390/app142210505
Chicago/Turabian StyleBelhaj, Majda, Jan Valentin, and Nicola Baldo. 2024. "Accuracy of Dynamic Modulus Models of Asphalt Mixtures Containing Reclaimed Asphalt (RA)" Applied Sciences 14, no. 22: 10505. https://doi.org/10.3390/app142210505
APA StyleBelhaj, M., Valentin, J., & Baldo, N. (2024). Accuracy of Dynamic Modulus Models of Asphalt Mixtures Containing Reclaimed Asphalt (RA). Applied Sciences, 14(22), 10505. https://doi.org/10.3390/app142210505









