Evaluating Rock Brittleness Through a Novel Statistical Damage Theory-Based Index: A Case Study on Sandstones
Abstract
1. Introduction
2. The Proposed Rock Brittleness Index
3. Verification
3.1. Case 1: Verification of Rock Brittleness Under Different Confining Pressures Using Proposed Index
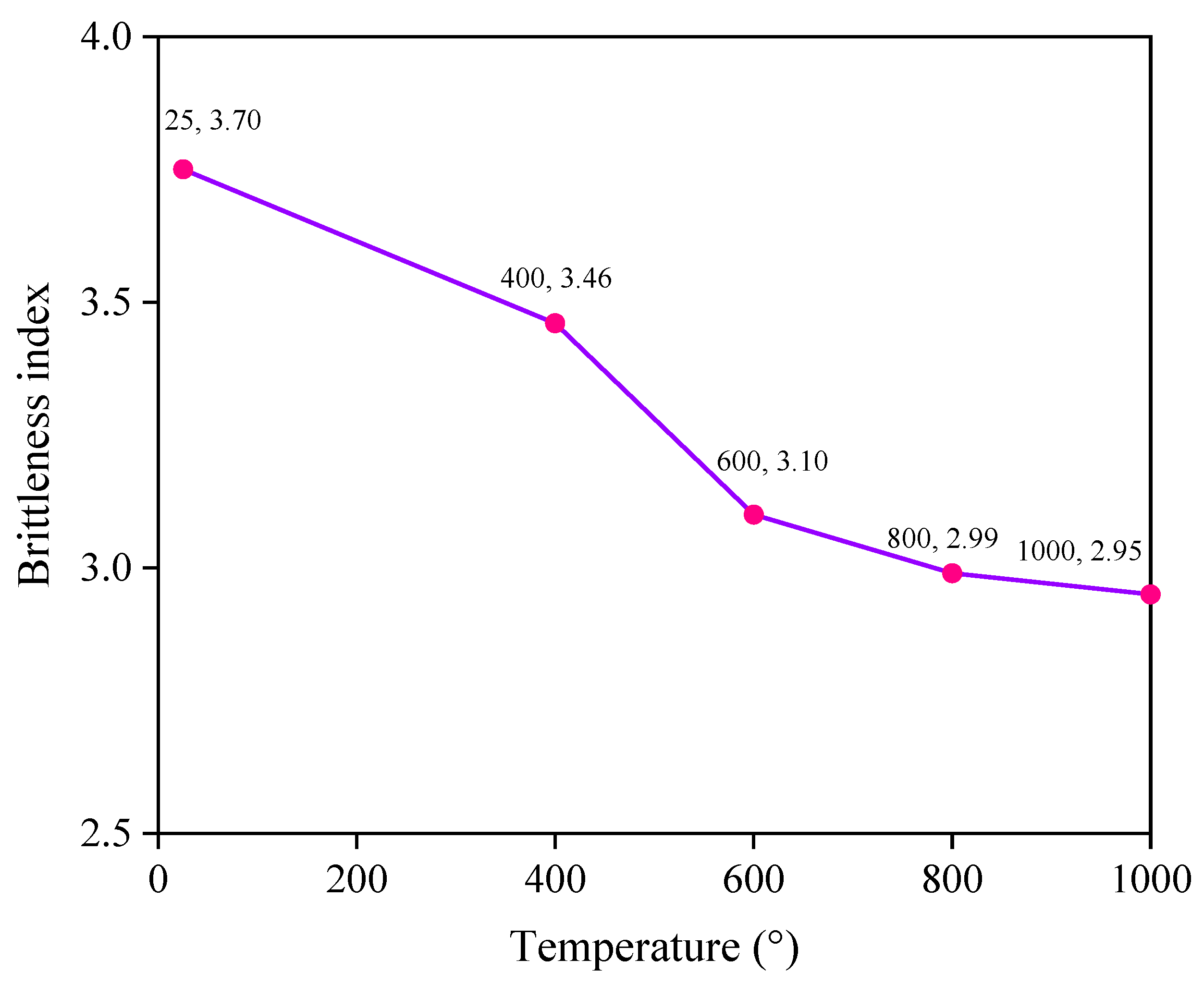
3.2. Case 2: Verification of Rock Brittleness Under Varying Temperatures Using Proposed Index
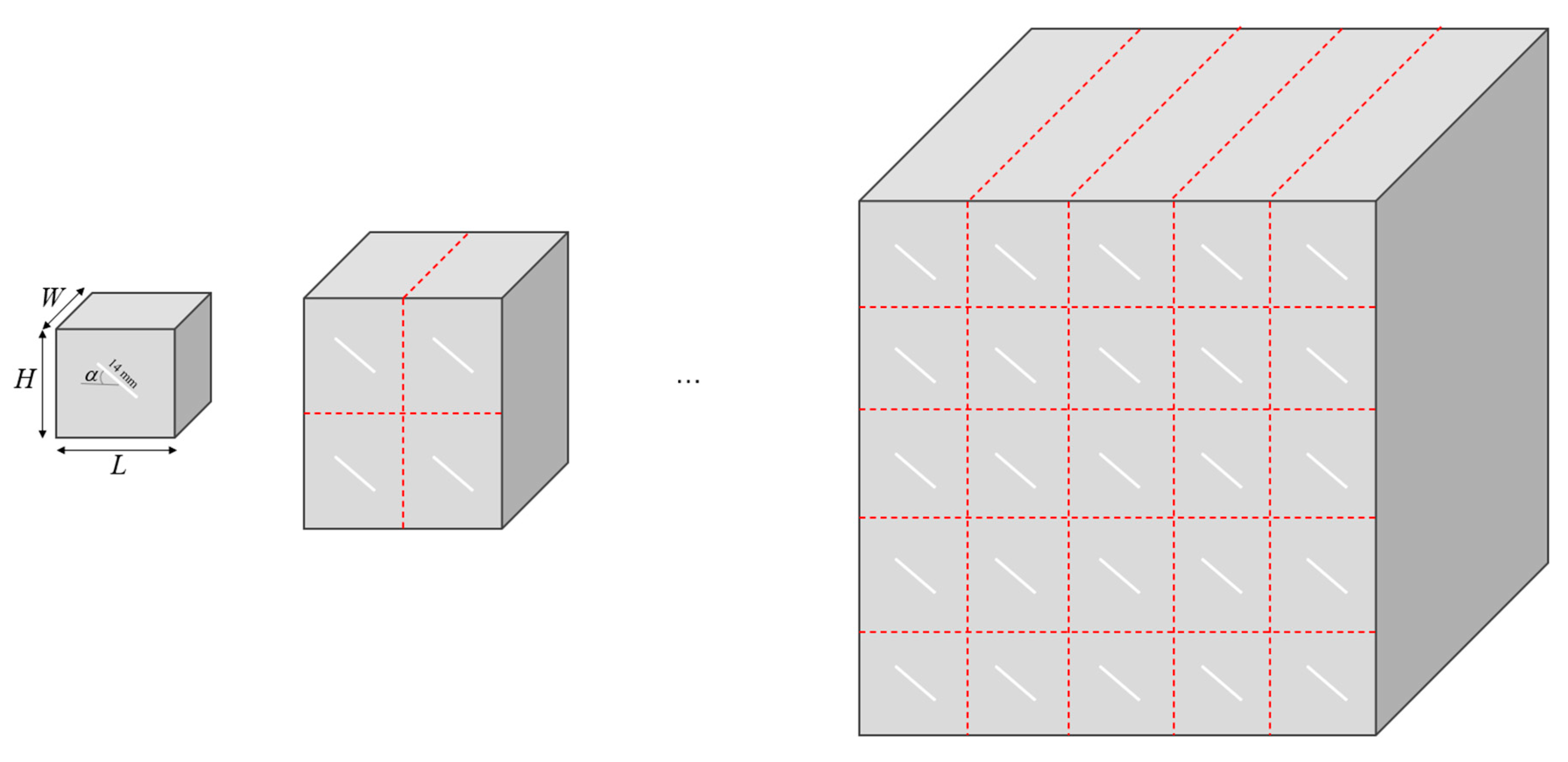
4. Investigation of Influence of Model Size and Joint Dip Angle on Rock Brittleness
4.1. Experimental Scheme and Design
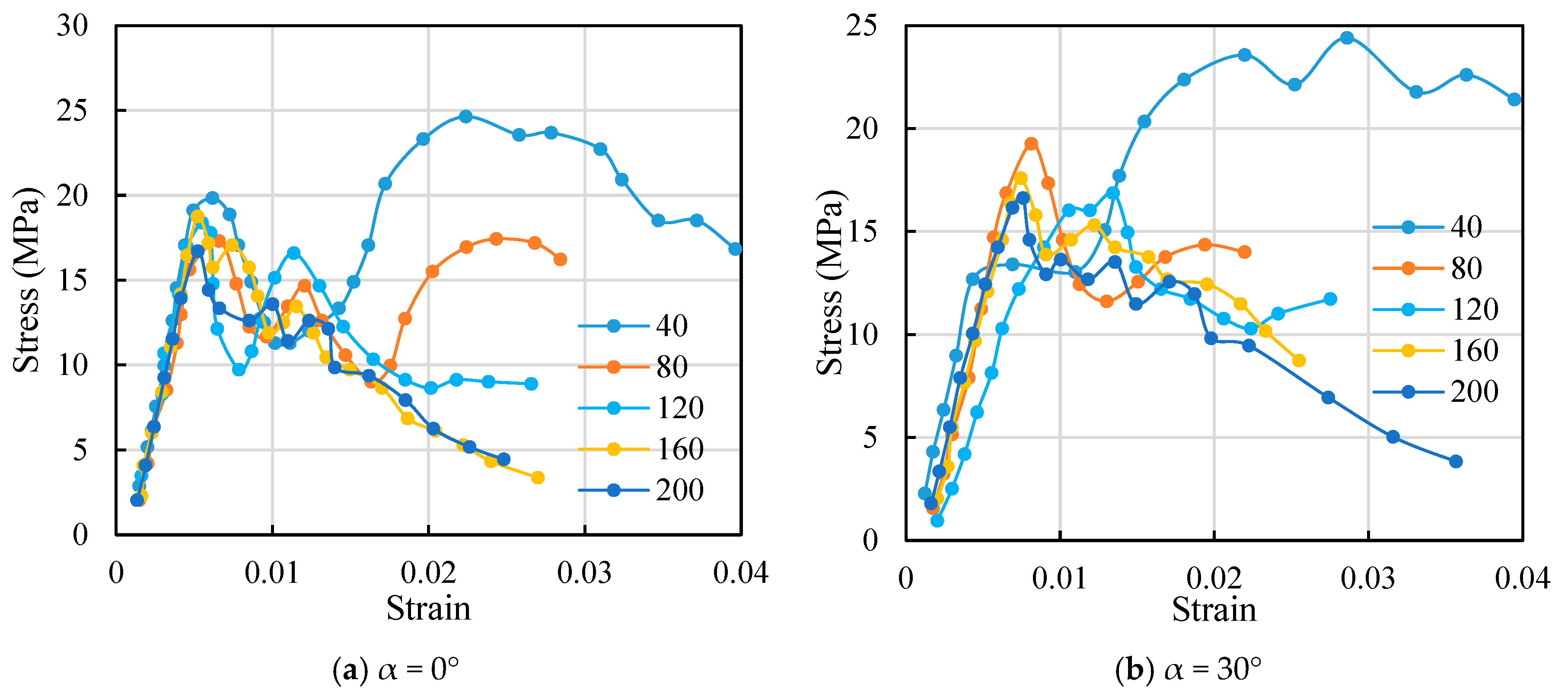
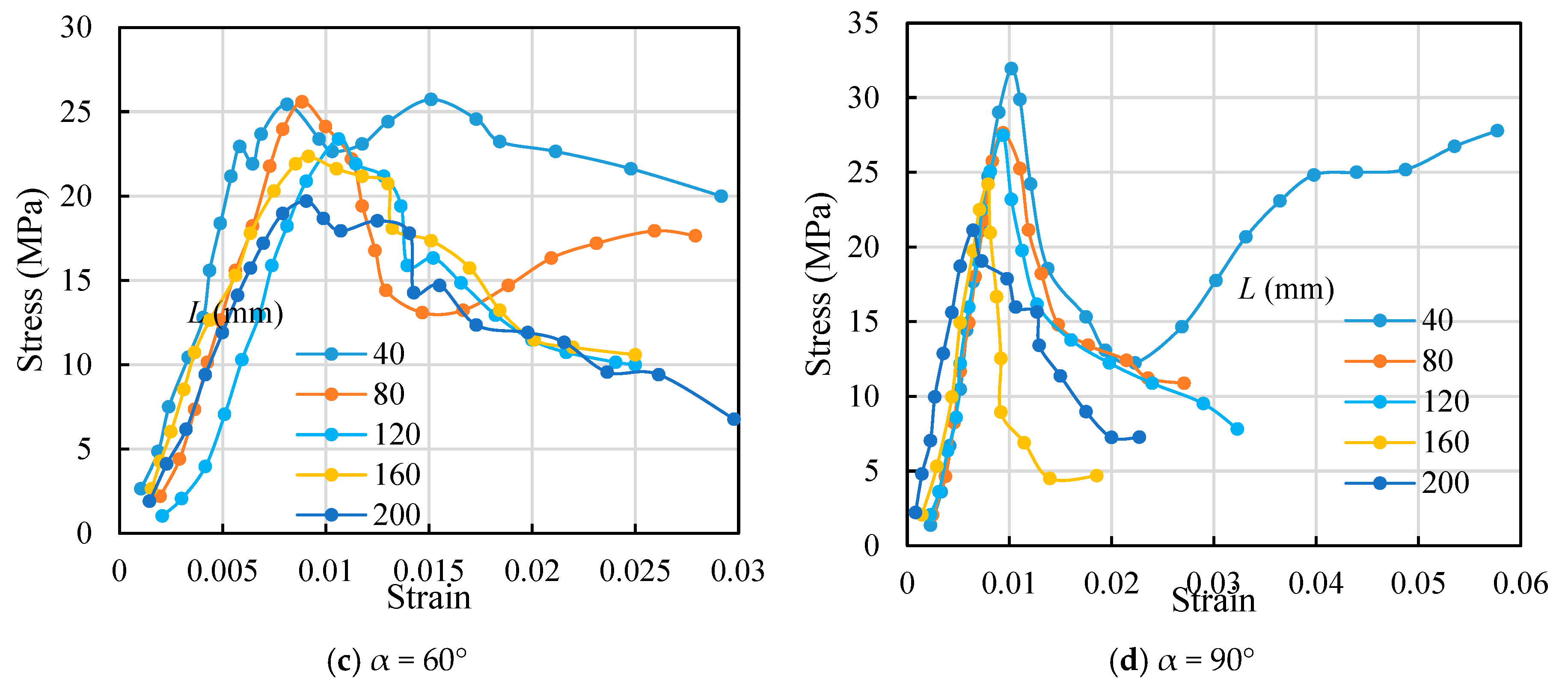
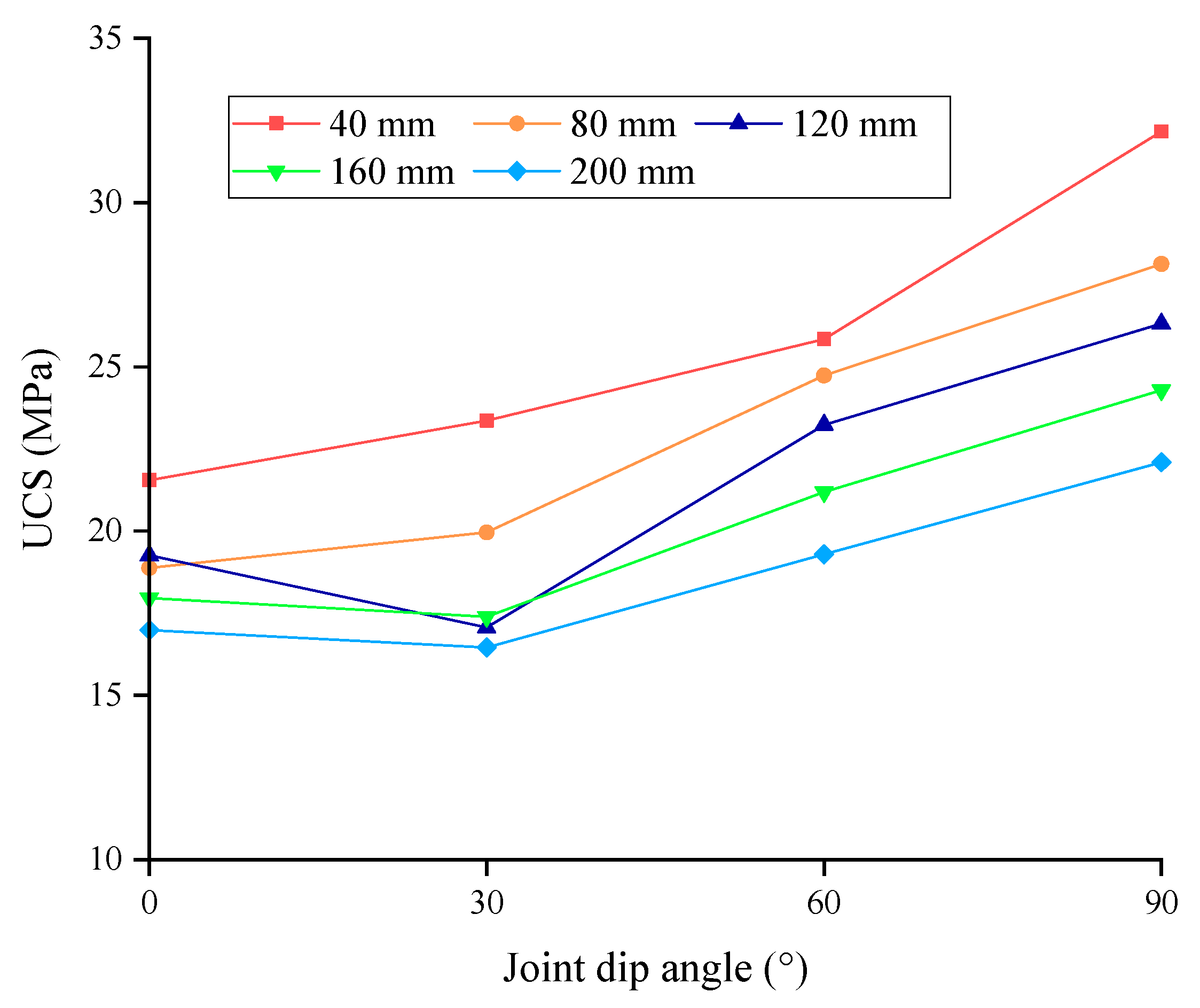
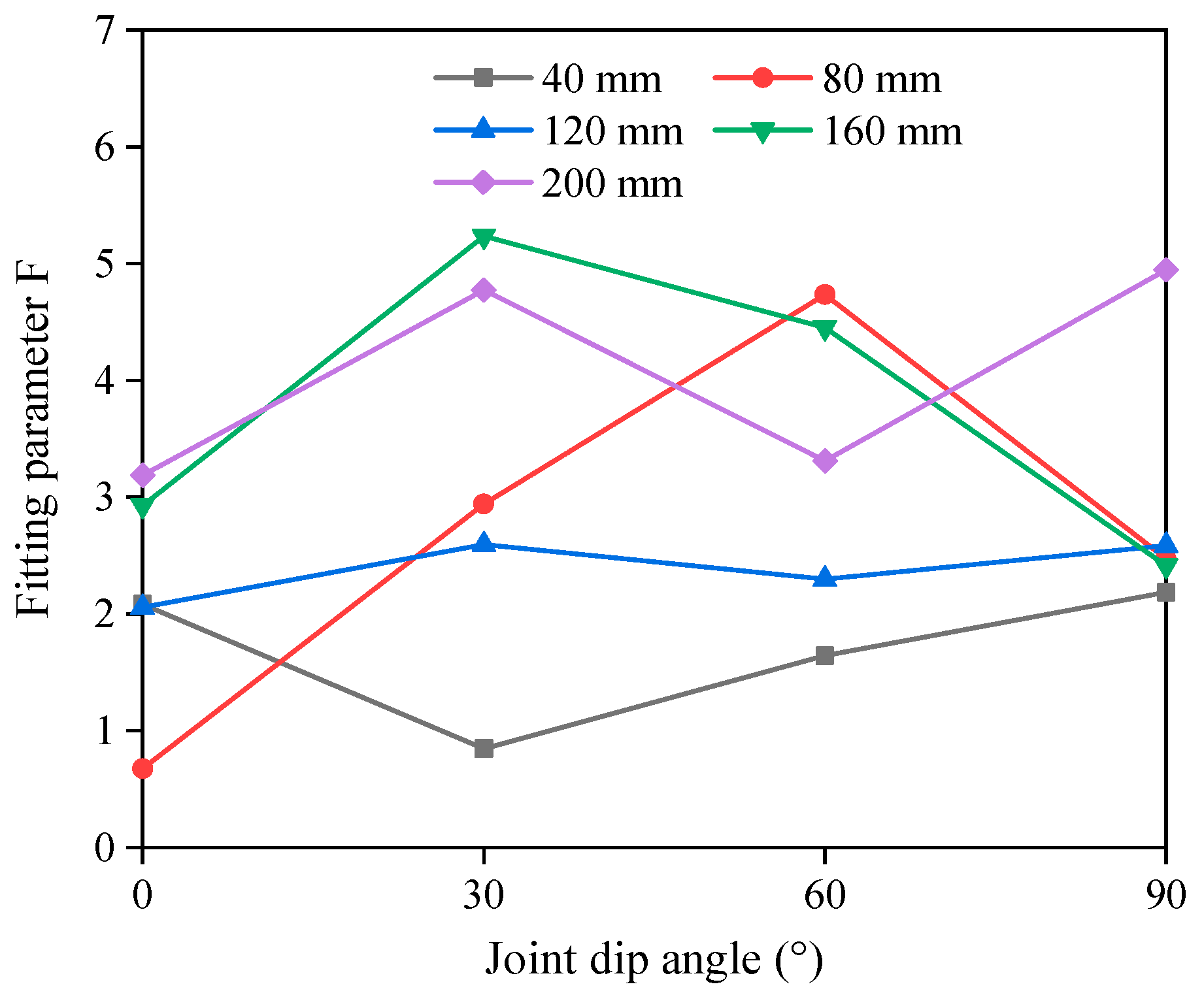
4.2. Experimental Results
4.3. Analysis of Brittleness Characteristics
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Stress | |
| Effective stress | |
| εi | Strain |
| Peak stress | |
| Peak strain | |
| D | Damage variable |
| ν | Poisson’s ratio |
| E | Elastic modulus |
| B | Brittleness index |
| i | Stress/strain direction |
| m | Fitting parameter of damage variable |
| F | Fitting parameter of damage variable |
| Dp | Damage value at the peak stress |
| L | Length of specimen |
| H | Height of specimen |
| W | Width of specimen |
| α | Dip angle of the joint |
| UCS | Uniaxial compressive strength |
References
- Munoz, H.; Taheri, A.; Chanda, E.K. Fracture Energy-Based Brittleness Index Development and Brittleness Quantification by Pre-peak Strength Parameters in Rock Uniaxial Compression. Rock Mech. Rock Eng. 2016, 49, 4587–4606. [Google Scholar] [CrossRef]
- Zou, C.J.; Cheng, Y.; Li, J.C. Strain rate and size effects on the brittleness indexes of Carrara marble. Int. J. Rock Mech. Min. Sci. 2021, 146, 104860. [Google Scholar] [CrossRef]
- Peng, J.; Xu, C.H.; Dai, B.B.; Sun, L.J.; Feng, J.J.; Huang, Q.S. Numerical Investigation of Brittleness Effect on Strength and Microcracking Behavior of Crystalline Rock. Int. J. Geomech. 2022, 22, 04022178. [Google Scholar] [CrossRef]
- Wang, W.; Wang, Y.; Chai, B.; Du, J.; Xing, L.X.; Xia, Z.H. An Energy-Based Method to Determine Rock Brittleness by Considering Rock Damage. Rock Mech. Rock Eng. 2022, 55, 1585–1597. [Google Scholar] [CrossRef]
- Meng, F.Z.; Zhou, H.; Zhang, C.Q.; Xu, R.C.; Lu, J.J. Evaluation Methodology of Brittleness of Rock Based on Post-Peak Stress-Strain Curves. Rock Mech. Rock Eng. 2015, 48, 1787–1805. [Google Scholar] [CrossRef]
- Meng, F.Z.; Wong, L.N.Y.; Zhou, H. Rock brittleness indices and their applications to different fields of rock engineering: A review. J. Rock Mech. Geotech. Eng. 2021, 13, 221–247. [Google Scholar] [CrossRef]
- Kuang, Z.H.; Qiu, S.L.; Li, S.J.; Du, S.H.; Huang, Y.; Chen, X.Q. A New Rock Brittleness Index Based on the Characteristics of Complete Stress-Strain Behaviors. Rock Mech. Rock Eng. 2021, 54, 1109–1128. [Google Scholar] [CrossRef]
- Xu, R.C.; Li, Z.; Jin, Y.D. Brittleness Effect on Acoustic Emission Characteristics of Rocks Based on a New Brittleness Evaluation Index. Int. J. Geomech. 2022, 22, 04022185. [Google Scholar] [CrossRef]
- Xie, J.Y.; Zhang, J.J.; Fang, Y.P.; Cao, J.X.; Deng, J.X. Quantitative Evaluation of Shale Brittleness Based on Brittle-Sensitive Index and Energy Evolution-Based Fuzzy Analytic Hierarchy Process. Rock Mech. Rock Eng. 2023, 56, 3003–3021. [Google Scholar] [CrossRef]
- Tarasov, B.G.; Stacey, T.R. Features of the Energy Balance and Fragmentation Mechanisms at Spontaneous Failure of Class I and Class II Rocks. Rock Mech. Rock Eng. 2017, 50, 2563–2584. [Google Scholar] [CrossRef]
- Liu, X.B.; Zhang, Z.Y.; Ge, Z.L.; Zhong, C.L.; Liu, L. Brittleness Evaluation of Saturated Coal Based on Energy Method from Stress-Strain Curves of Uniaxial Compression. Rock Mech. Rock Eng. 2021, 54, 3193–3207. [Google Scholar] [CrossRef]
- Zhang, Y.; Feng, X.T.; Yang, C.X.; Han, Q.; Wang, Z.F.; Kong, R. Evaluation Method of Rock Brittleness under True Triaxial Stress States Based on Pre-peak Deformation Characteristic and Post-peak Energy Evolution. Rock Mech. Rock Eng. 2021, 54, 1277–1291. [Google Scholar] [CrossRef]
- Gong, F.Q.; Wang, Y.L. A New Rock Brittleness Index Based on the Peak Elastic Strain Energy Consumption Ratio. Rock Mech. Rock Eng. 2022, 55, 1571–1582. [Google Scholar] [CrossRef]
- Gong, F.Q.; Zhang, P.L.; Luo, S.; Li, J.C.; Huang, D. Theoretical damage characterisation and damage evolution process of intact rocks based on linear energy dissipation law under uniaxial compression. Int. J. Rock Mech. Min. Sci. 2021, 146, 104858. [Google Scholar] [CrossRef]
- Ren, C.H.; Yu, J.; Liu, S.Y.; Yao, W.; Zhu, Y.L.; Liu, X.Y. A Plastic Strain-Induced Damage Model of Porous Rock Suitable for Different Stress Paths. Rock Mech. Rock Eng. 2022, 55, 1887–1906. [Google Scholar] [CrossRef]
- Li, X.; Cao, W.G.; Su, Y.H. A statistical damage constitutive model for softening behavior of rocks. Eng. Geol. 2012, 143, 1–17. [Google Scholar] [CrossRef]
- Huang, S.B.; Liu, Q.S.; Cheng, A.P.; Liu, Y.Z. A statistical damage constitutive model under freeze-thaw and loading for rock and its engineering application. Cold Reg. Sci. Tech. 2018, 145, 142–150. [Google Scholar] [CrossRef]
- Xie, S.J.; Han, Z.Y.; Hu, H.H.; Lin, H. Application of a novel constitutive model to evaluate the shear deformation of discontinuity. Eng. Geol. 2022, 304, 106693. [Google Scholar] [CrossRef]
- Li, Y.W.; Jia, D.; Rui, Z.H.; Peng, J.Y.; Fu, C.K.; Zhang, J. Evaluation method of rock brittleness based on statistical constitutive relations for rock damage. J. Petrol. Sci. Eng. 2017, 153, 123–132. [Google Scholar] [CrossRef]
- Li, Y.W.; Long, M.; Zuo, L.H.; Li, W.; Zhao, W.C. Brittleness evaluation of coal based on statistical damage and energy evolution theory. J. Petrol. Sci. Eng. 2019, 172, 753–763. [Google Scholar] [CrossRef]
- Lemaitre, J.; Chaboche, J.-L. Mechanics of Solid Materials; Cambridge University Press: London, UK, 1994. [Google Scholar]
- Deng, J.A.; Cu, D.S. On a statistical damage constitutive model for rock materials. Comput. Geosci. 2011, 37, 122–128. [Google Scholar] [CrossRef]
- Liu, H.Y.; Lv, S.R.; Zhang, L.M.; Yuan, X.P. A dynamic damage constitutive model for a rock mass with persistent joints. Int. J. Rock Mech. Min. Sci. 2015, 75, 132–139. [Google Scholar] [CrossRef]
- Cao, W.; Zhao, H.; Zhang, L.; Zhang, Y. Damage statistical softening constitutive model for rock considering effect of damage threshold and its parameters determination method. Chinese J. Rock Mech. Eng. 2008, 27, 1148–1154. [Google Scholar]
- Nicksiar, M.; Martin, C.D. Crack initiation stress in low porosity crystalline and sedimentary rocks. Eng. Geol. 2013, 154, 64–76. [Google Scholar] [CrossRef]
- Gao, F.Q.; Stead, D.; Kang, H.P. Numerical Simulation of Squeezing Failure in a Coal Mine Roadway due to Mining-Induced Stresses. Rock Mech. Rock Eng. 2015, 48, 1635–1645. [Google Scholar] [CrossRef]
- Khan, J.A.; Padmanabhan, E.; Ul Haq, I.; Franchek, M.A. Hydraulic fracturing with low and high viscous injection mediums to investigate net fracture pressure and fracture network in shale of different brittleness index. Geomech. Energy Environ. 2023, 33, 100416. [Google Scholar] [CrossRef]
- Liu, S.F.; Ma, P.; Meng, F.F.; Dintwe, T.K.M.; Mao, P.; Moses, D. Strain softening and brittleness characteristics of gangue-containing coal samples. Geomech. Geophys. Geo-Energy Geo-Resour. 2021, 7, 28. [Google Scholar] [CrossRef]
- Wang, X.K.; Wei, W.S.; Niu, Y.; Xia, C.C.; Song, L.B.; Han, G.S.; Zhu, Z.M. Triaxial Creep Mechanical Behaviors and Creep Damage Model of Dolomitic Limestone Material under Multi-Stage Incremental Loading. Materials 2023, 16, 1918. [Google Scholar] [CrossRef]
- Zhang, J.; Ai, C.; Li, Y.W.; Che, M.G.; Gao, R.; Zeng, J. Energy-Based Brittleness Index and Acoustic Emission Characteristics of Anisotropic Coal Under Triaxial Stress Condition. Rock Mech. Rock Eng. 2018, 51, 3343–3360. [Google Scholar] [CrossRef]
- Wei, S.J.; Yang, Y.S.; Su, C.D.; Cardosh, S.R.; Wang, H. Experimental Study of the Effect of High Temperature on the Mechanical Properties of Coarse Sandstone. Appl. Sci. 2019, 9, 2424. [Google Scholar] [CrossRef]
- Zhang, D.C.; Ranjith, P.G.; Perera, M.S.A. The brittleness indices used in rock mechanics and their application in shale hydraulic fracturing: A review. J. Petrol. Sci. Eng. 2016, 143, 158–170. [Google Scholar] [CrossRef]
- Wu, N. Study on the Scale Effect and Anisotropy of Mechanical Properties of Jointed Rock Masses. Ph.D. Thesis, Dalian University of Technology, Dalian, China, 2020. [Google Scholar]
- Chen, X.; Liao, Z.; Li, D. Experimental study of effects of joint inclination angle and connectivity rate on strength and deformation properties of rock masses under uniaxial compression. Chinese J. Rock Mech. Eng. 2011, 30, 781–789. [Google Scholar]
- Ghazvinian, A.; Hadei, M.R. Effect of discontinuity orientation and confinement on the strength of jointed anisotropic rocks. Int. J. Rock Mech. Min. Sci. 2012, 55, 117–124. [Google Scholar] [CrossRef]
- Wu, N.; Liang, Z.Z.; Zhang, Z.H.; Li, S.H.; Lang, Y.X. Development and verification of three-dimensional equivalent discrete fracture network modelling based on the finite element method. Eng. Geol. 2022, 306, 106759. [Google Scholar] [CrossRef]






| Confining Pressure (MPa) | Peak Strength (MPa) | Peak Strain | Elastic Modulus (MPa) | Poisson’s Ratio |
|---|---|---|---|---|
| 0 | 23.99 | 0.0075 | 3220 | 0.26 |
| 6 | 34.33 | 0.0125 | 3310 | 0.26 |
| 12 | 43.74 | 0.0152 | 3770 | 0.26 |
| 18 | 64.92 | 0.021 | 3910 | 0.26 |
| 24 | 68.44 | 0.023 | 4350 | 0.26 |
| 30 | 80.03 | 0.029 | 4510 | 0.26 |
| Temperature (°C) | Peak Strength (MPa) | Peak Strain | Elastic Modulus (MPa) | Poisson’s Ratio |
|---|---|---|---|---|
| 25 | 139.6 | 0.011 | 18,290 | 0.28 |
| 400 | 155.7 | 0.013 | 19,730 | 0.28 |
| 600 | 165.79 | 0.018 | 16,480 | 0.28 |
| 800 | 176.72 | 0.02 | 15,840 | 0.28 |
| 1000 | 148.07 | 0.022 | 10,650 | 0.28 |
| Specimen No. | L (mm) | α (°) | Specimen No. | L (mm) | α (°) | Specimen No. | L (mm) | α (°) | Specimen No. | L (mm) | α (°) |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 40 | 0 | 6 | 40 | 30 | 11 | 40 | 60 | 16 | 40 | 90 |
| 2 | 80 | 0 | 7 | 80 | 30 | 12 | 80 | 60 | 17 | 80 | 90 |
| 3 | 120 | 0 | 8 | 120 | 30 | 13 | 120 | 60 | 18 | 120 | 90 |
| 4 | 160 | 0 | 9 | 160 | 30 | 14 | 160 | 60 | 19 | 160 | 90 |
| 5 | 200 | 0 | 10 | 200 | 30 | 15 | 200 | 60 | 20 | 200 | 90 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, N.; Jiang, B.; Ai, T.; Liang, Z. Evaluating Rock Brittleness Through a Novel Statistical Damage Theory-Based Index: A Case Study on Sandstones. Appl. Sci. 2024, 14, 10153. https://doi.org/10.3390/app142210153
Wu N, Jiang B, Ai T, Liang Z. Evaluating Rock Brittleness Through a Novel Statistical Damage Theory-Based Index: A Case Study on Sandstones. Applied Sciences. 2024; 14(22):10153. https://doi.org/10.3390/app142210153
Chicago/Turabian StyleWu, Na, Bei Jiang, Ting Ai, and Zhengzhao Liang. 2024. "Evaluating Rock Brittleness Through a Novel Statistical Damage Theory-Based Index: A Case Study on Sandstones" Applied Sciences 14, no. 22: 10153. https://doi.org/10.3390/app142210153
APA StyleWu, N., Jiang, B., Ai, T., & Liang, Z. (2024). Evaluating Rock Brittleness Through a Novel Statistical Damage Theory-Based Index: A Case Study on Sandstones. Applied Sciences, 14(22), 10153. https://doi.org/10.3390/app142210153







