Shear Mechanical Behaviours and Size Effect of Band–Bedrock Interface: Discrete Element Method Simulation Insights
Abstract
1. Introduction
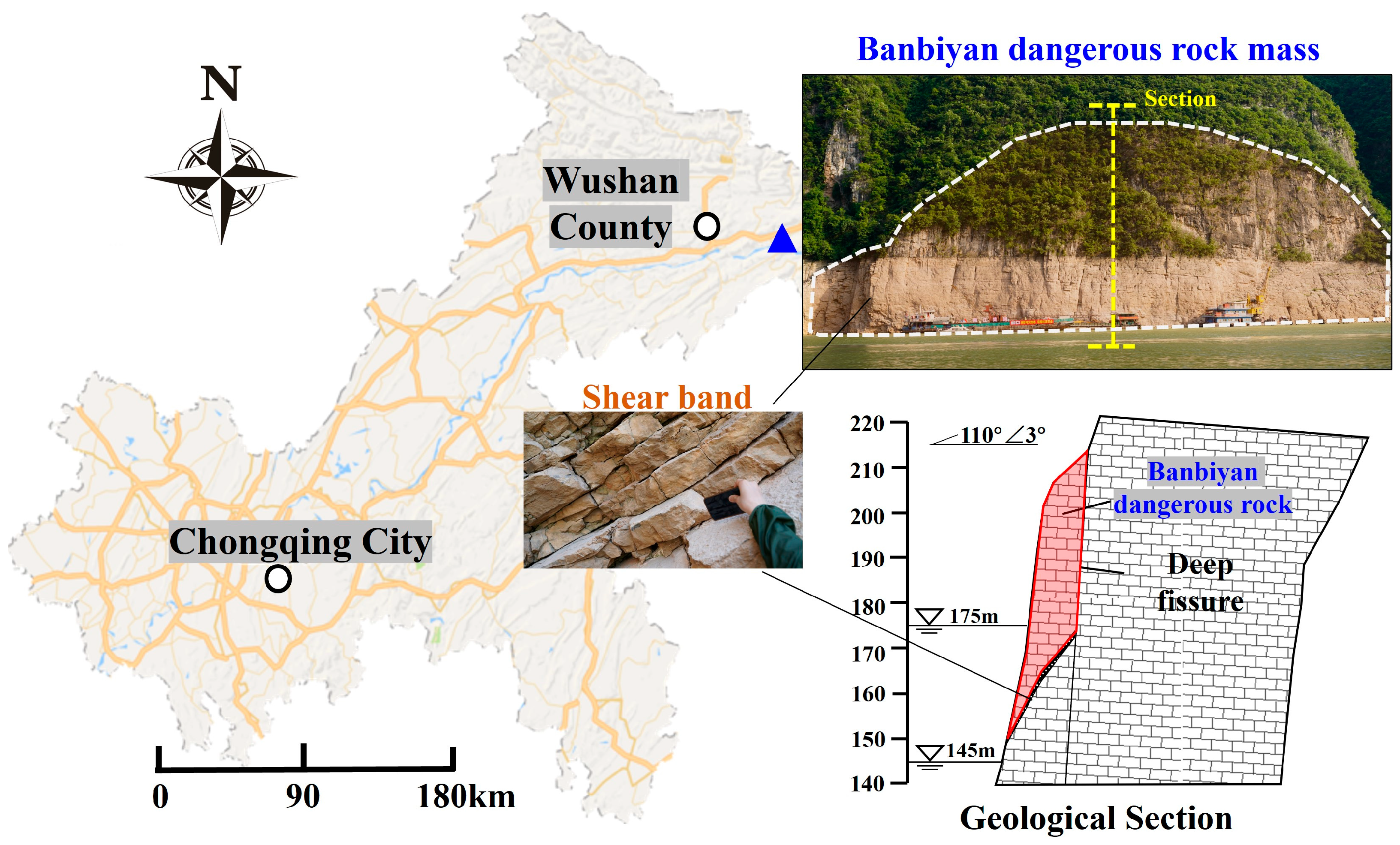
2. Characteristics of Shear Bands
3. Laboratory and DEM Modelling Establishment
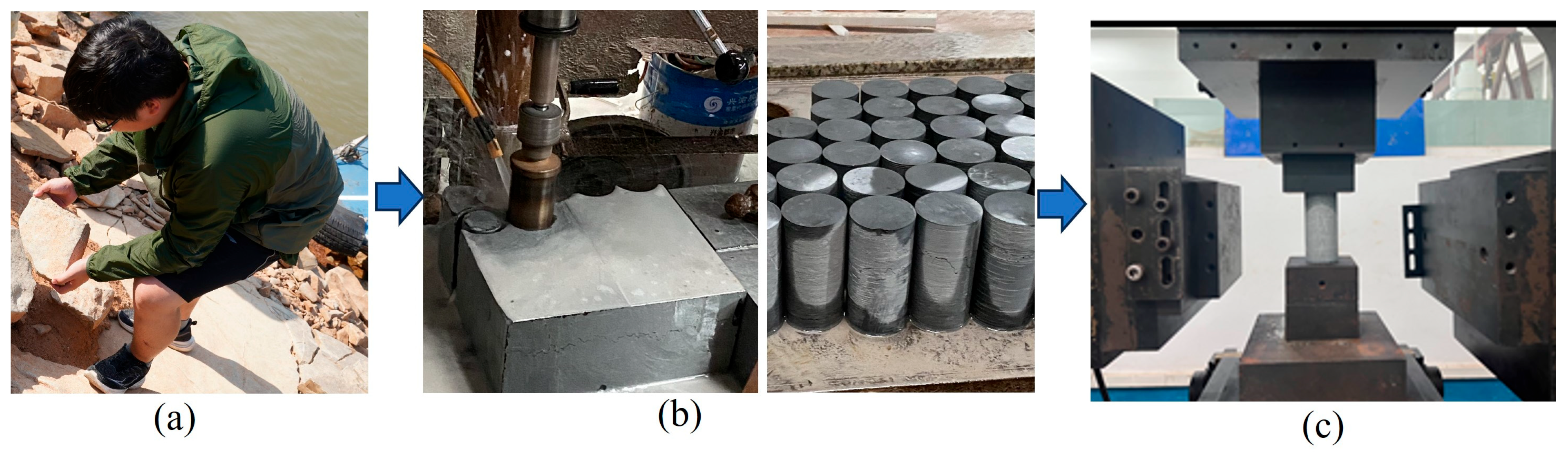
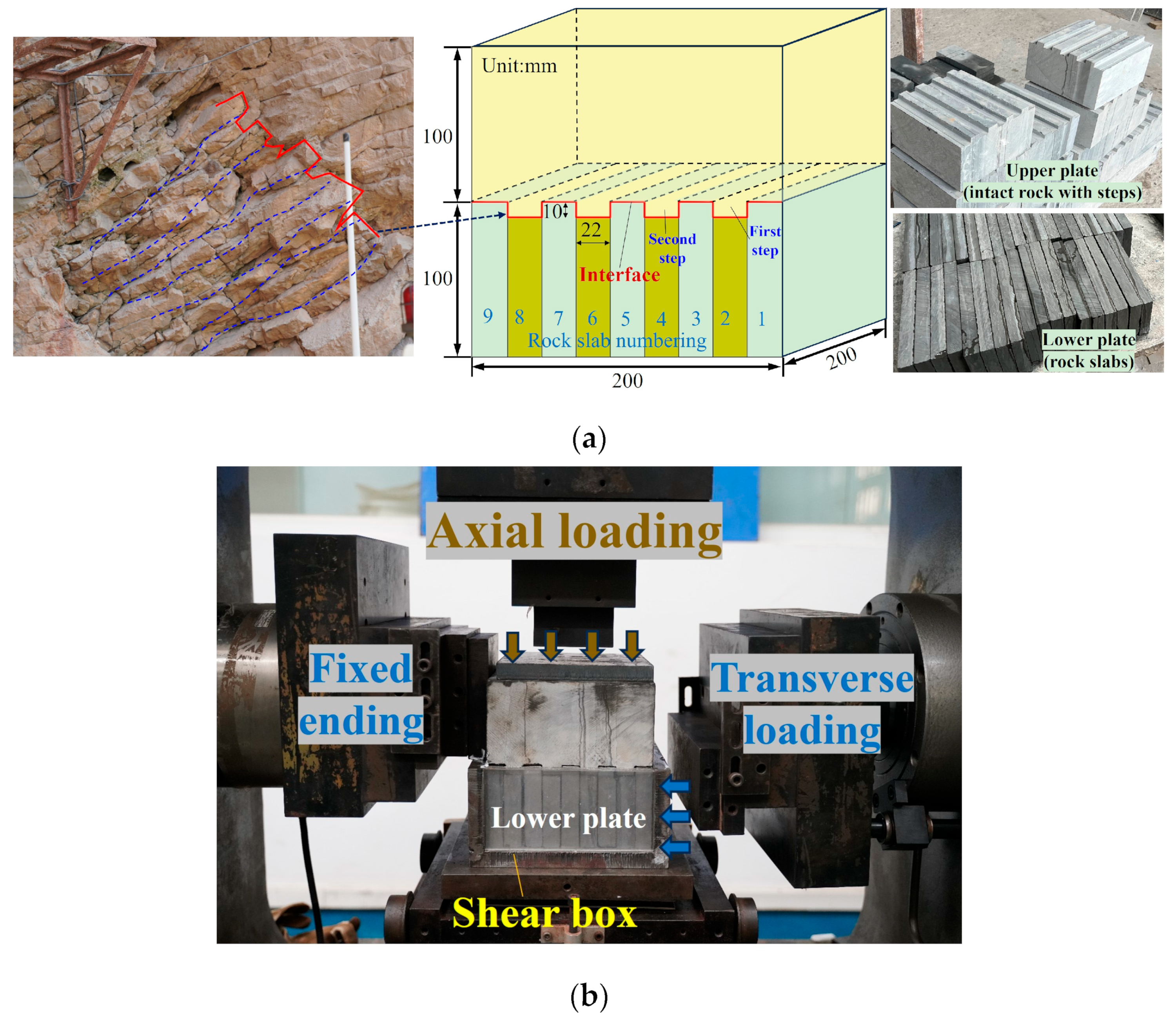
3.1. Sample Preparation
3.2. Testing System and Procedure
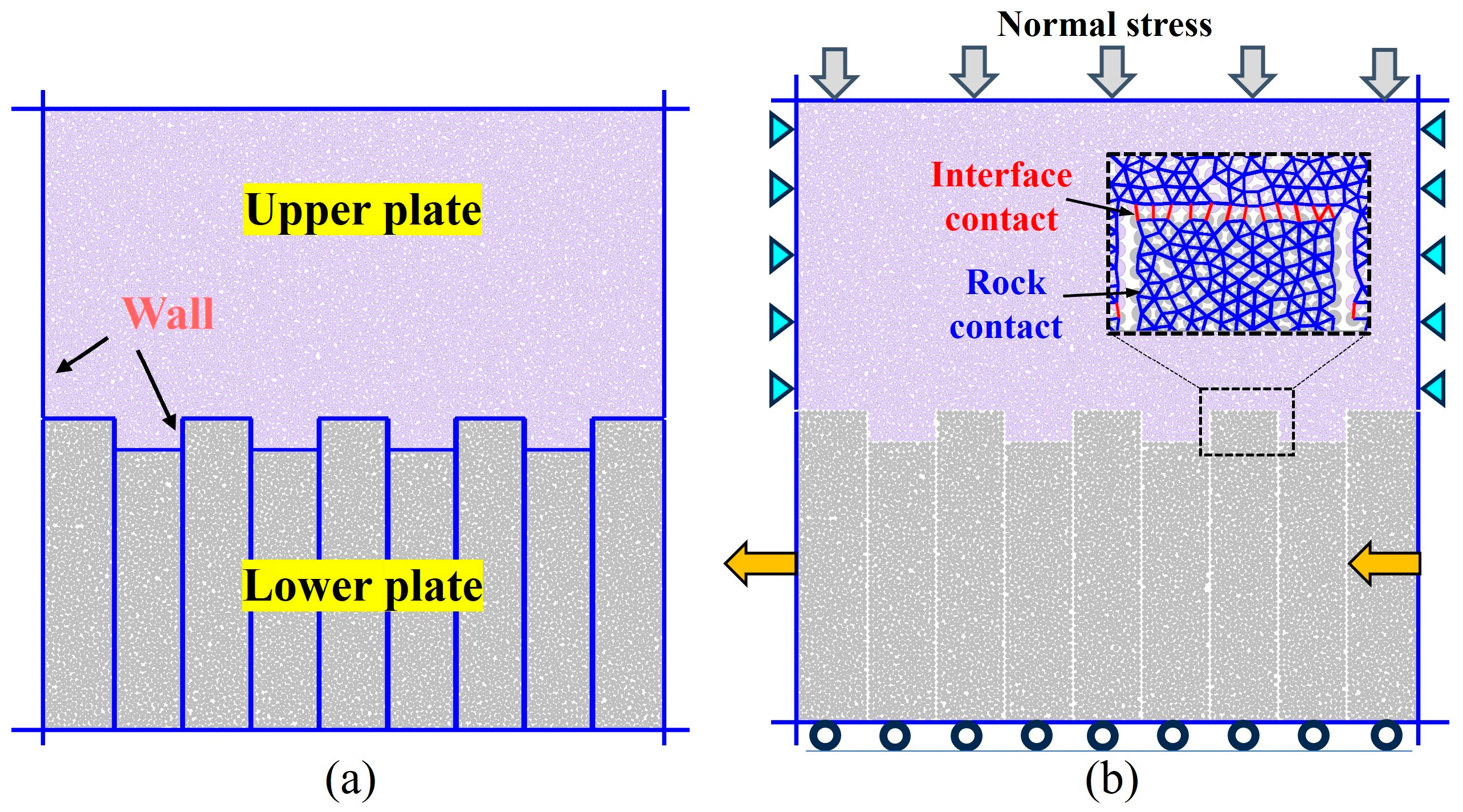
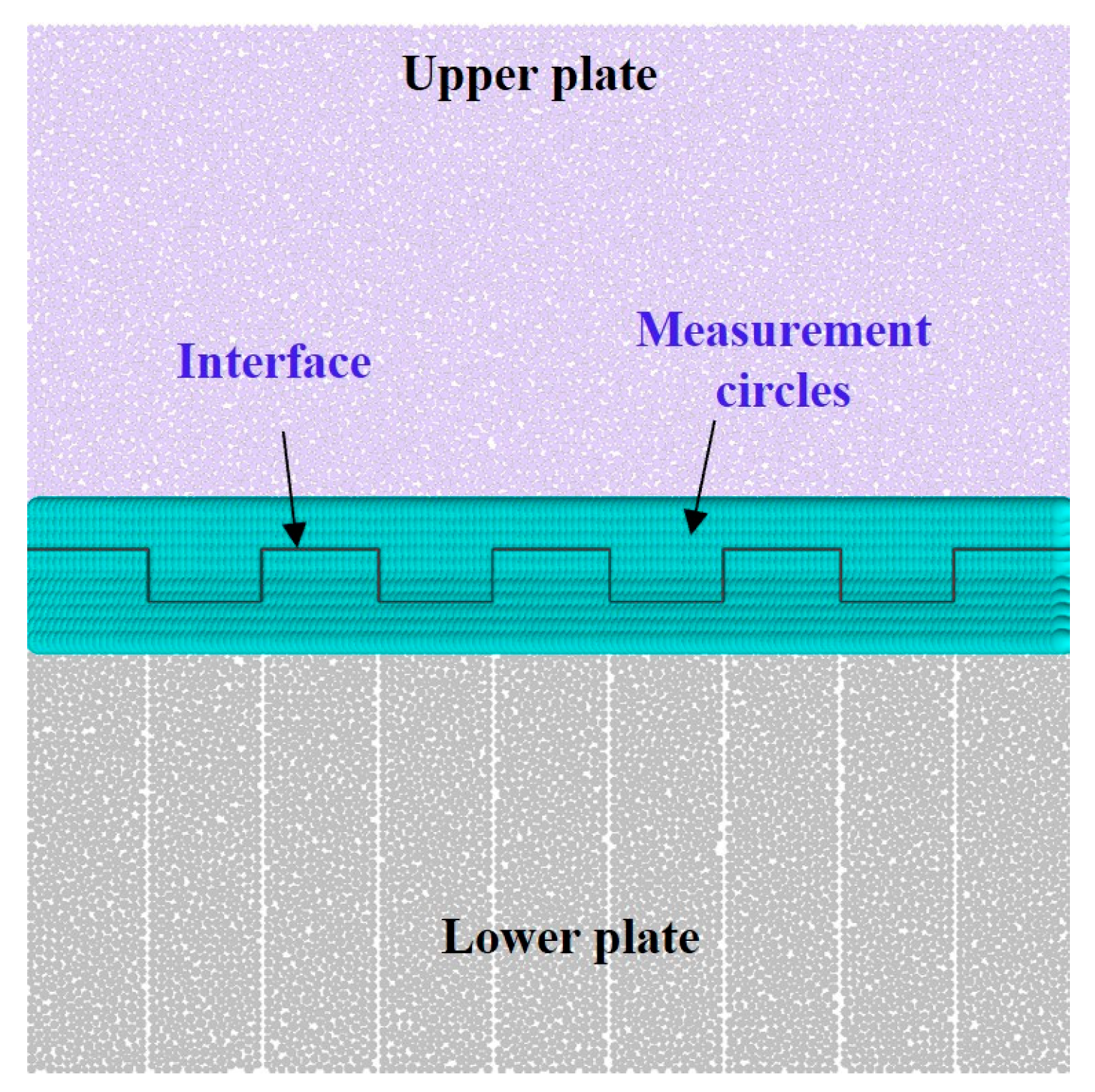
3.3. DEM Modelling Establishment
3.4. Contact Model and Parameter Calibration
3.5. Experimental and Numerical Simulation Schemes
4. Results Analysis
4.1. Failure Mode and Curve Characteristics
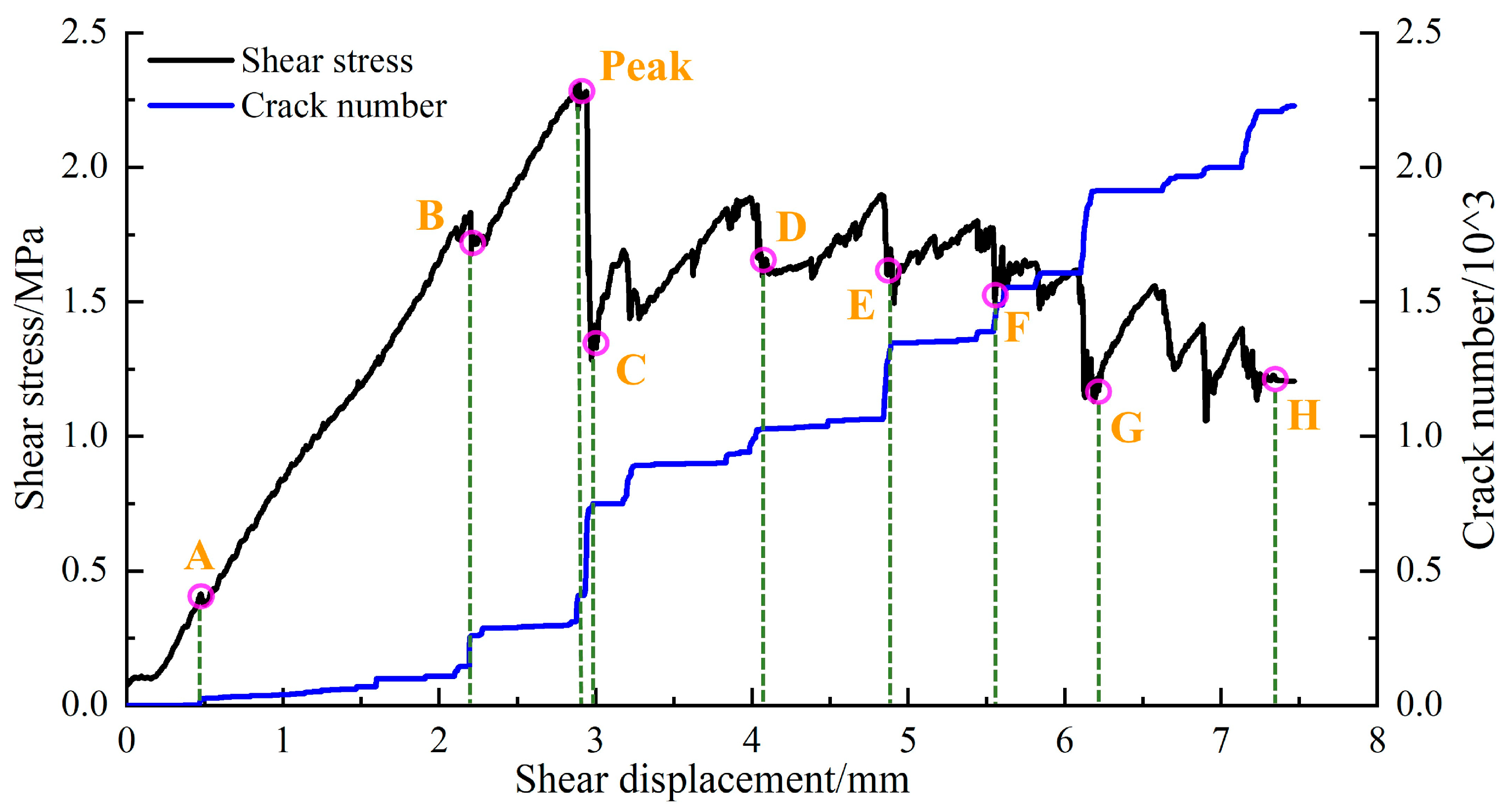
4.2. Failure and Crack Evolution Process Analysis
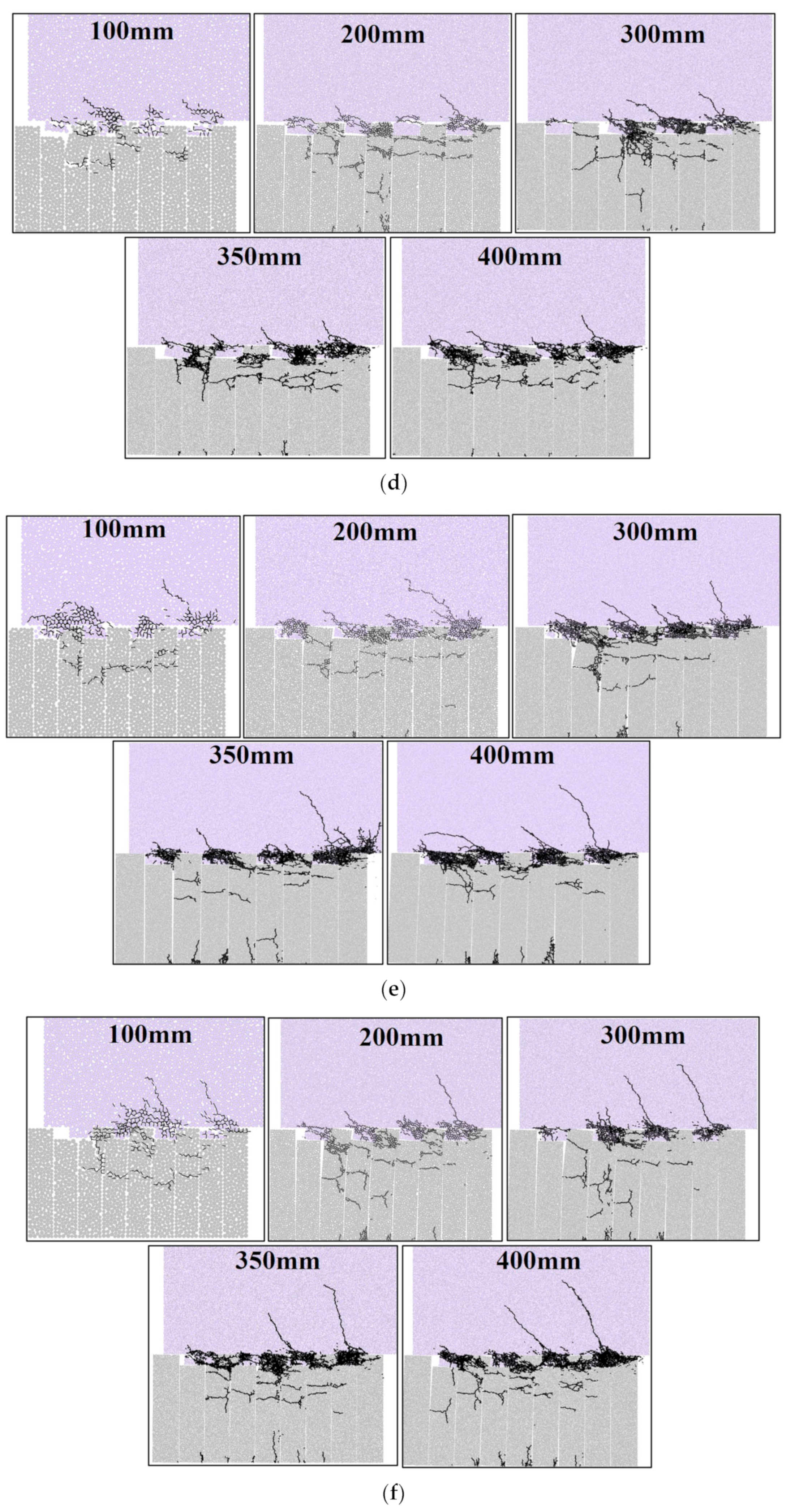
4.3. Displacement Field and Crack Type Analysis
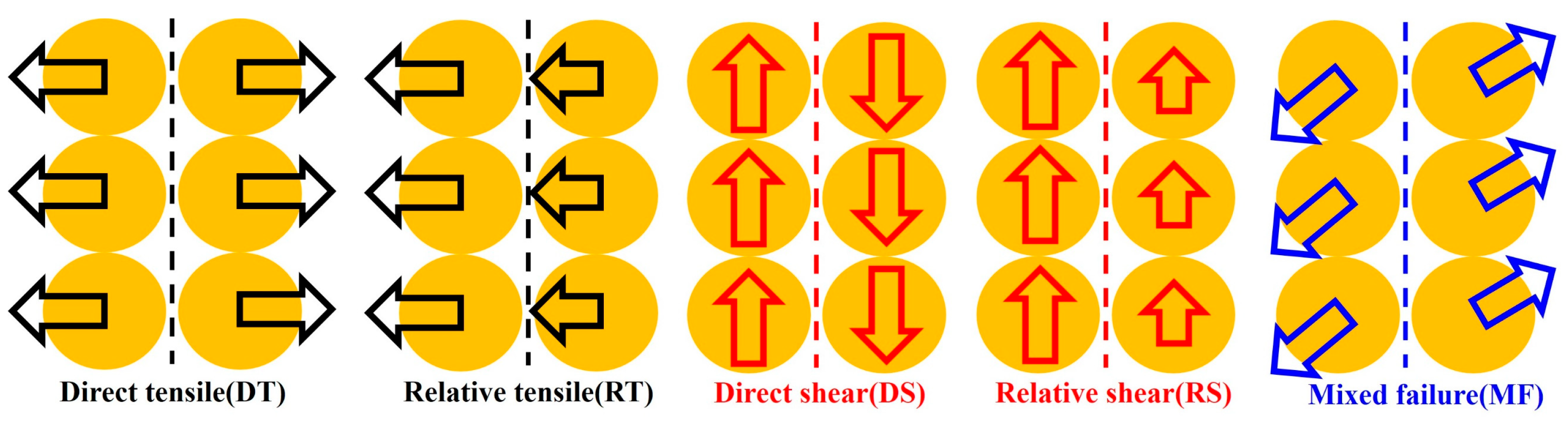
4.3.1. Description of Crack Types
- (1)
- Direct tensile (DT). This type of crack forms when the displacement directions of two particles are opposite and parallel to the line connecting their centres.
- (2)
- Relative tensile (RT). The displacement directions of the two particles are aligned and parallel to the line connecting their centres; however, the magnitudes of the displacements differ.
- (3)
- Direct shear (DS). The directions of the two particles’ displacement are opposite and perpendicular to the line connecting their centres.
- (4)
- Relative shear (RS). The directions of the two particles’ displacement are uniform and perpendicular to the line connecting their centres, but with differing displacement magnitudes.
- (5)
- Mixed failure (MF). This mode of failure is characterised by the simultaneous presence of relative displacements in both the normal and tangential directions for the two particles.
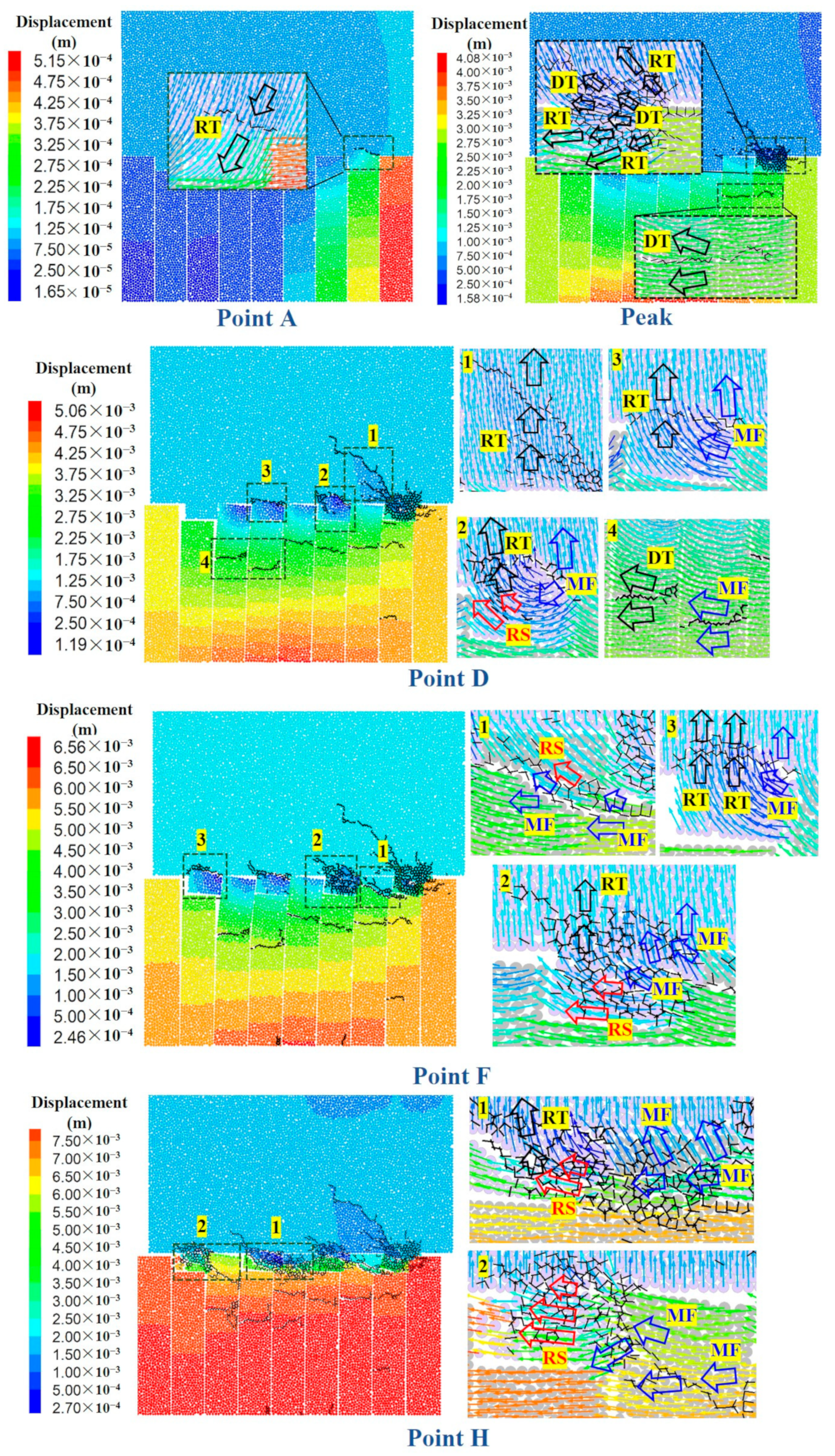
4.3.2. Displacement Field Evolution and Crack Types
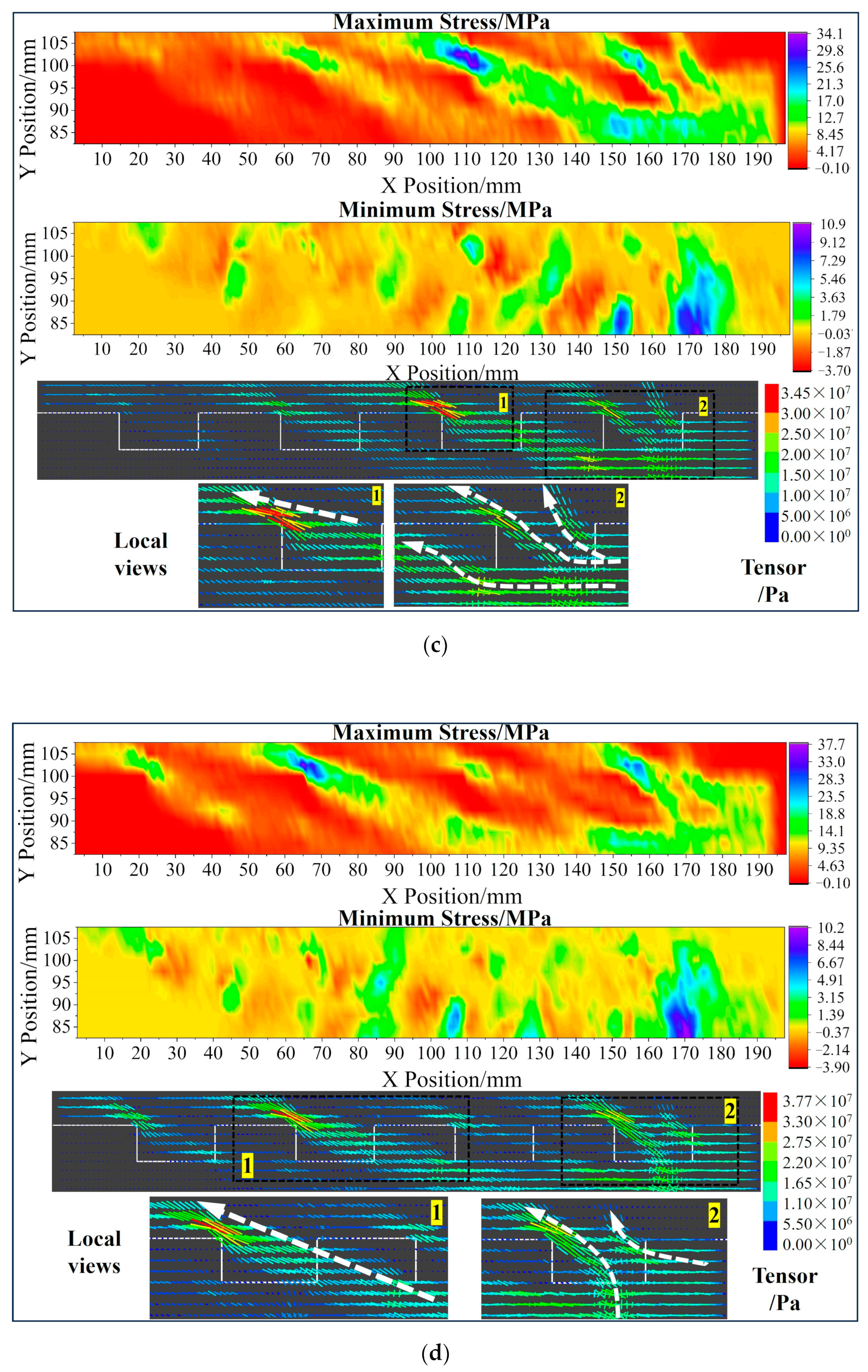
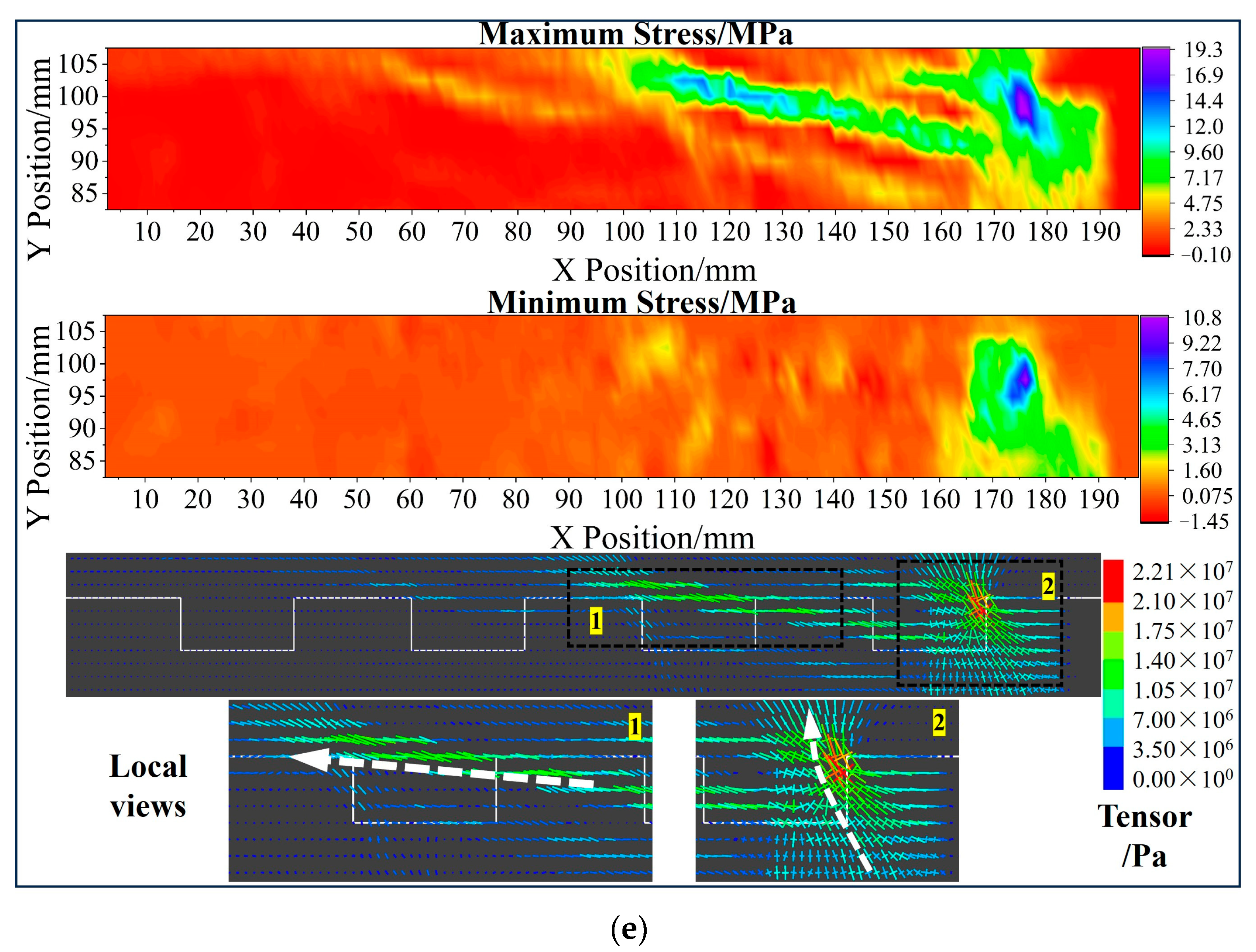
4.4. Stress Evolution and Failure Mechanism
5. Discussion
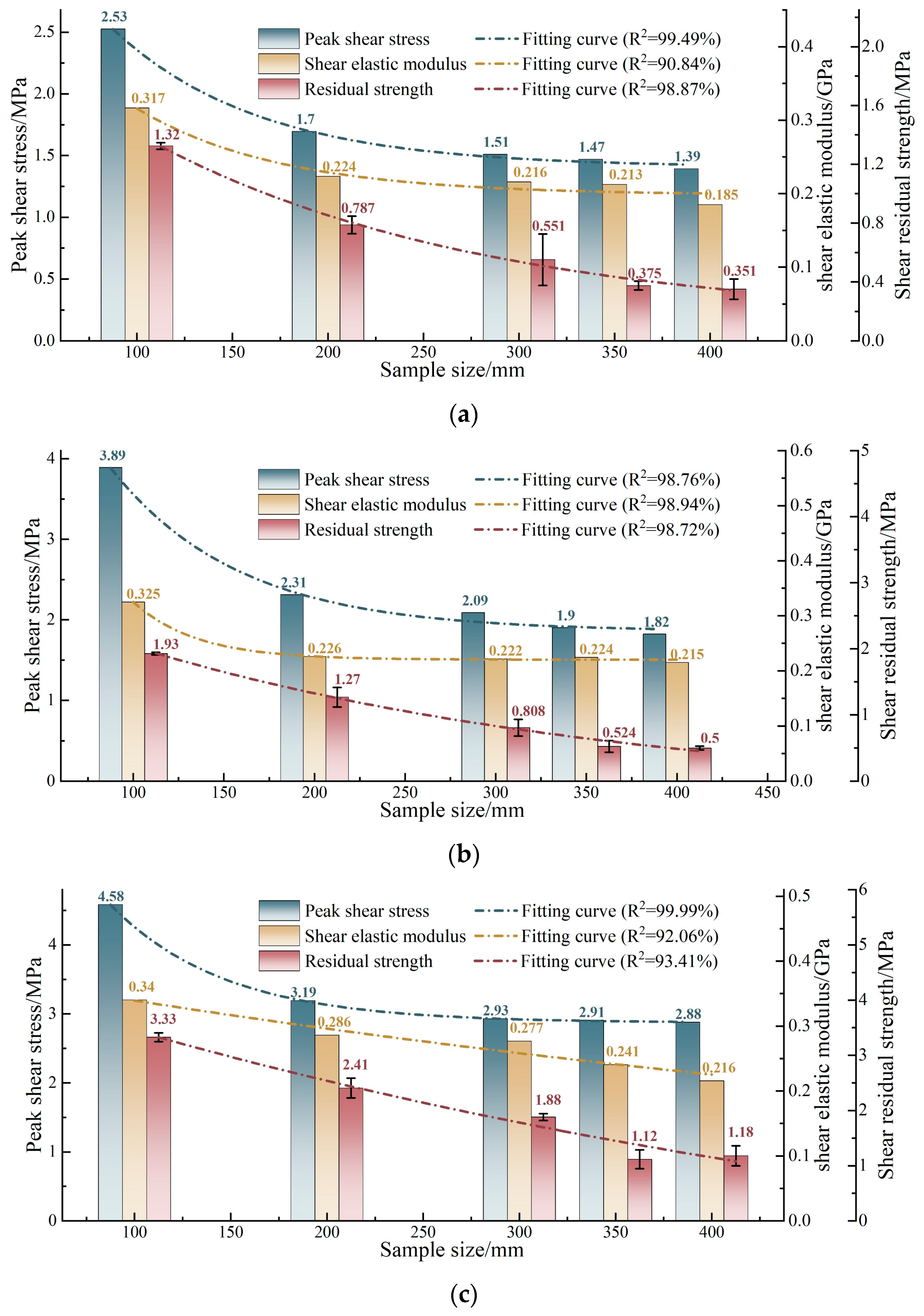
5.1. Effect of Model Size on Shear Characteristics
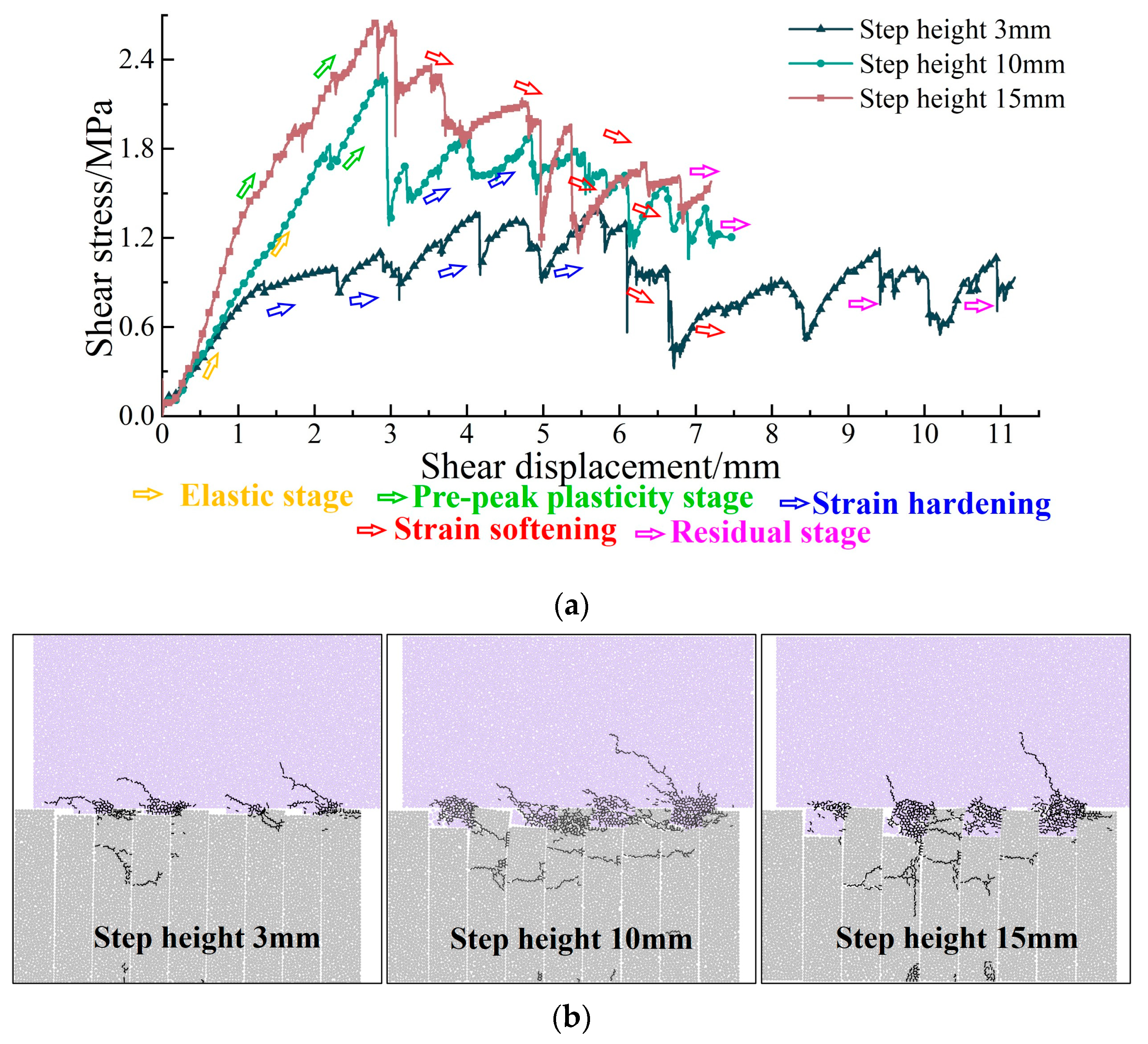
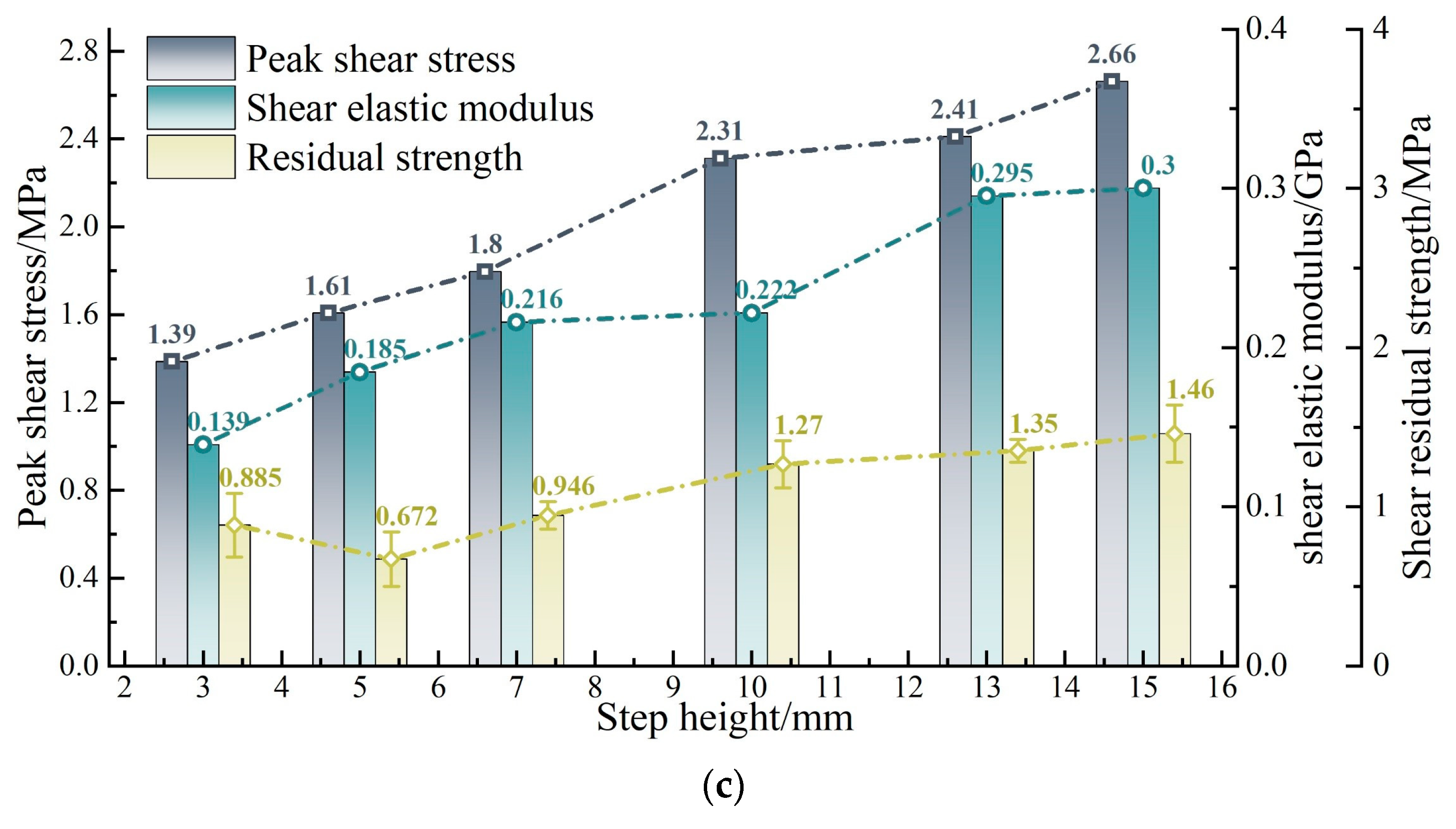
5.2. Effect of Rock Step Height on Shear Characteristics
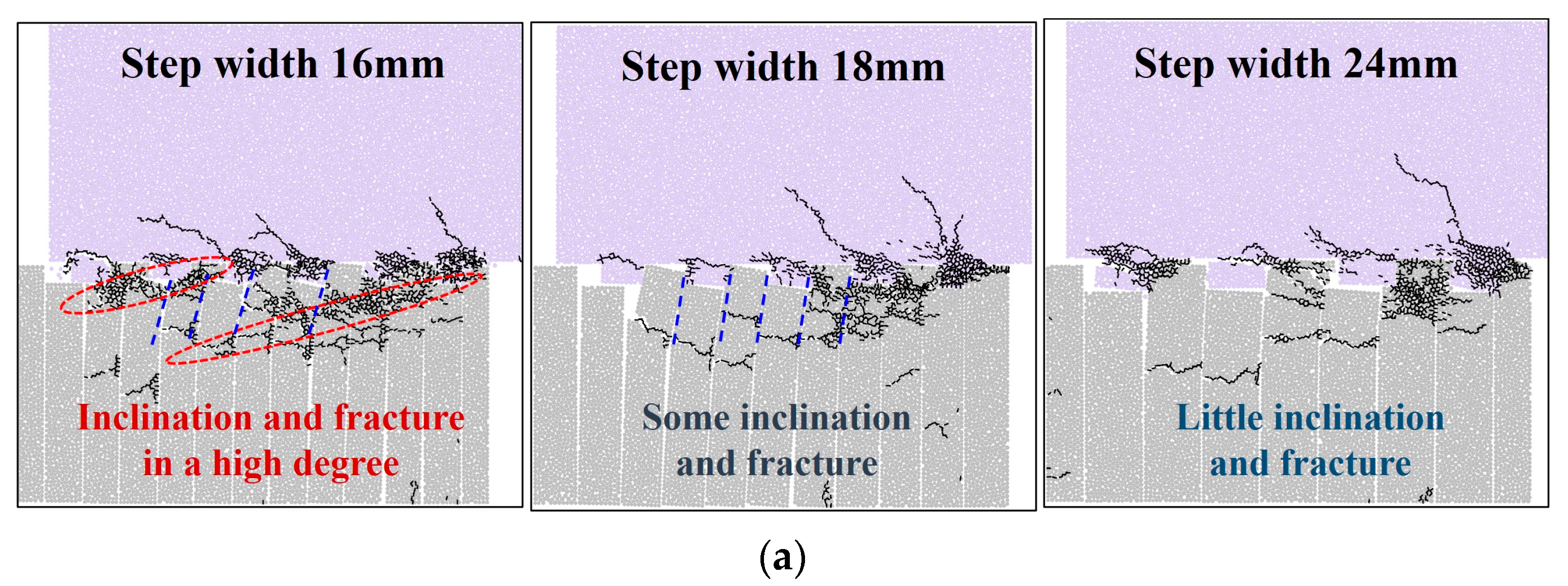
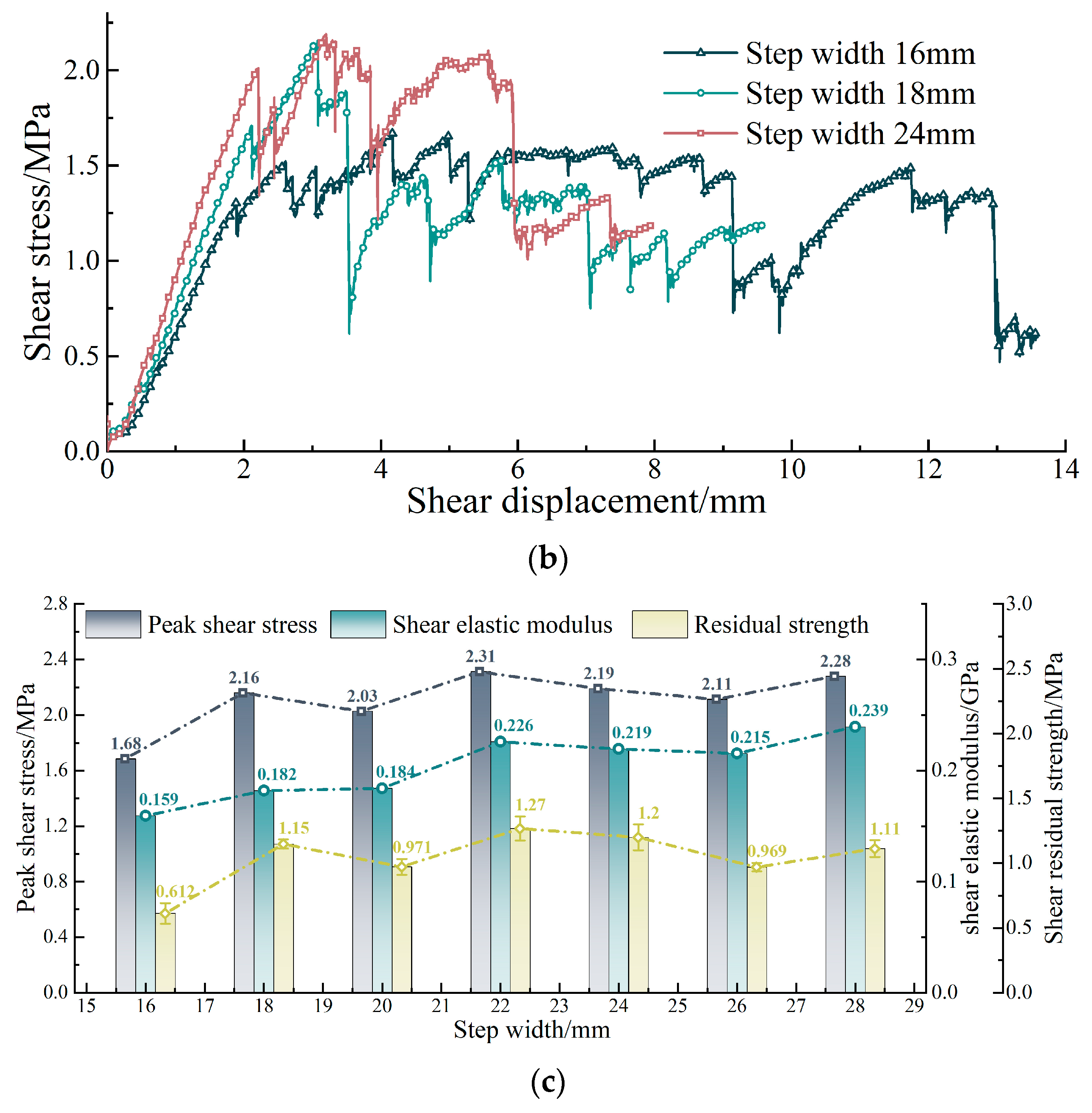
5.3. Effect of Step Width on Shear Characteristics
6. Conclusions
- (1)
- The initiation and failure cracks in the first rock step are predominantly tensile. As the induced shear displacement increases, the influence of shear stress becomes more pronounced, leading to shear and mixed types of failure cracks in the subsequent rock slabs and steps.
- (2)
- The failure of the first rock step occurs before the peak, primarily due to tensile stress, resulting in a point-load stress state that induces splitting failure. The remaining steps transition into a complex compressive–tensile stress state, with their failure occurring progressively.
- (3)
- With the increase in the sample size, the post-peak plasticity of the stress curves with strain softening becomes the predominant characteristic when the sample size exceeds 200 mm. The variation in the shear parameters with sample size can be described by a negative exponential function. Once the sample size surpasses 350 mm, the shear parameter values approach consistency. The representative elementary volume of the shear parameters can be considered as a sample with a geometry size of 350 mm.
- (4)
- The rock step height significantly influences the failure mode and stress curve characteristics by affecting the stress concentration and shear dilation. The peak shear strength and shear elastic modulus increase with rock step height, with respective amplifications of 91.37% and 115.83%. The residual strength initially decreases and then gradually increases with step height, exhibiting amplitude reductions and amplifications of 23.73% and 116.94%.
- (5)
- A smaller step width results in a higher number of fractures during shearing, maintaining shear resistance through point contacts between rock slabs and step blocks. As the rock step width increases, the stress concentration is mitigated due to the reduced inclination of the broken rock slab, leading to the stabilisation of the shear parameter values.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shang, J.; West, L.; Hencher, S.; Zhao, Z. Geological discontinuity persistence: Implications and quantification. Eng. Geol. 2018, 241, 41–54. [Google Scholar] [CrossRef]
- Chen, C.; Yang, H.; Song, K.; Liang, D.; Zhang, Y.; Ni, J. Dissolution feature differences of carbonate rock within hydro-fluctuation belt located in the Three Gorges Reservoir Area. Eng. Geol. 2023, 327, 107362. [Google Scholar] [CrossRef]
- Tang, H.; Wasowski, J.; Juang, C. Geohazards in the three Gorges Reservoir Area, China—Lessons learned from decades of research. Eng. Geol. 2019, 261, 105267. [Google Scholar] [CrossRef]
- Yin, Y.; Huang, B.; Wang, W.; Wei, Y.; Ma, X.; Ma, F.; Zhao, C. Reservoir-induced landslides and risk control in Three Gorges Project on Yangtze River, China. J. Rock Mech. Geotech. Eng. 2016, 8, 557–595. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Y.; Xu, B.; Zhou, X.; Guo, X.; Miao, L. Dynamic damage evolution of bank slopes with serrated structural planes considering the deteriorated rock mass and frequent reservoir induced earthquakes. Int. J. Min. Sci. Technol. 2023, 33, 1131–1145. [Google Scholar] [CrossRef]
- Yin, Y.; Wang, L.; Huang, B.; Zhang, Z.; Dai, Z. Evolution analysis of the Banbiyan dangerous rock mass in the three gorges reservoir area. Georisk-Assess. Manag. Risk Eng. Syst. Geohazards 2023, 17, 376–386. [Google Scholar] [CrossRef]
- Patton, F. Multiple modes of shear failure in rock. In Proceeding of the Congress of 1st International Society of Rock Mechanics, Lisbon, Portugal, 25 September–1 October 1966; International Society for Rock Mechanics and Rock Engineering: Lisbon, Portugal, 1966; pp. 509–513. [Google Scholar]
- Barton, N.; Choubey, V. The shear strength of rock joints in theory and practice. Rock Mech. Rock Eng. 1977, 10, 1–54. [Google Scholar] [CrossRef]
- Zhao, J. A new JRC-JMC shear strength criterion for rock joint. Chin. J. Rock Mech. Eng. 1998, 17, 349–357. [Google Scholar]
- Maksimović, M. The shear strength components of rough rock joint. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1996, 33, 769–783. [Google Scholar] [CrossRef]
- Xia, C.; Tang, Z.; Xiao, W.; Song, Y. New peak shear strength criterion of rock joints based on quantified surface description. Rock Mech. Rock Eng. 2014, 47, 387–400. [Google Scholar] [CrossRef]
- Yang, J.; Rong, G.; Hou, D.; Peng, J.; Zhou, C. Experimental study on peak shear strength criterion for rock joints. Rock Mech. Rock Eng. 2016, 49, 821–835. [Google Scholar] [CrossRef]
- Liu, X.; Li, P.; Guo, X.; Luo, X.; Zhou, X.; Miao, L.; Zhou, F.; Wang, H. A statistical damage-based constitutive model for shearing of rock joints in brittle drop mode. Int. J. Min. Sci. Technol. 2024. [Google Scholar] [CrossRef]
- Liu, X.; Xu, B.; Huang, J.; Lin, G.; Zhou, X.; Wang, J.; Xiong, F. Macro-meso shear mechanical behaviors of coalescent rock joints with different morphologies. Chin. J. Geotech. Eng. 2021, 43, 406–415. [Google Scholar]
- Liu, R.; Lou, S.; Li, X.; Han, G.; Jiang, Y. Anisotropic surface roughness and shear behaviors of rough-walled plaster joints under constant normal load and constant normal stiffness conditions. J. Rock Mech. Geotech. Eng. 2022, 12, 338–352. [Google Scholar] [CrossRef]
- Zhang, G.; Zhang, J.; Zou, B.; Zou, J.; Xiong, F.; Lin, Z.; Zhang, Z. An Empirical Study on Water-Induced Shear Weakening Behaviors of Rough-Walled Sandstone Joints. Rock Mech. Rock Eng. 2023, 56, 3475–3497. [Google Scholar] [CrossRef]
- Le, H.; Wei, J.; Sun, S.; Zhang, C. Cracking behaviors and strength of rock-like samples with steps of different geometries under shear stress. J. Mt. Sci. 2021, 18, 1352–1370. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, G.; Jiang, Y.; Wang, C.; Xu, F. Shear behaviours and roughness degeneration based on a quantified rock joint surface description. Int. J. Min. Sci. Technol. 2023, 33, 1301–1316. [Google Scholar] [CrossRef]
- Jiao, F.; Xu, J.; Peng, S.; Hei, M.; Zhang, X.; Cheng, L. Experimental study on shear mechanical properties and damage evolution of artificial structural plane under constant normal stiffness. J. China Coal Soc. 2023, 48, 4065–4077. [Google Scholar]
- Bao, H.; Hu, Q.; Lan, H.; Yan, C.; Sun, W.; Liu, S.; Ma, K.; Wang, J. Spatio-temporal evolution law of anisotropic shear damage on rock mass joint surface affected by joint morphology. Eng. Fail. Anal. 2024, 165, 108820. [Google Scholar] [CrossRef]
- Zhang, Q.; Luo, Z.; Shen, D.; Shi, Z.; Pan, Q. Shear stress relaxation behavior of rock joints considering 3D morphological characteristics. Bull. Eng. Geol. Environ. 2023, 84, 429. [Google Scholar] [CrossRef]
- Song, L.; Jiang, Q.; Li, Y.; Yang, C.; Liu, C.; Yang, B.; Li, S. Improved JRC-JCS shear strength formula for soft-hard natural joints. Rock Soil Mech. 2017, 38, 2789–2798. [Google Scholar]
- Ghazvinian, A.; Taghichian, A.; Hashemi, M.; Mar’ashi, S. The shear behaviour of bedding planes of weakness between two different rock types with high strength difference. Rock Mech. Rock Eng. 2010, 43, 69–87. [Google Scholar] [CrossRef]
- Hu, Z.; Wang, Q.; Ma, Y.; Lv, H.; Liu, W.; Yan, R.; Wang, K.; Shao, T.; Sun, Y. Study on shear failure characteristics of fiber-reinforced shotcrete-granite interface based on surface scanning. Case Stud. Constr. Mater. 2024, 21, e03486. [Google Scholar] [CrossRef]
- Bahaaddini, M.; Sharrock, G.; Hebblewhite, B. Numerical direct shear tests to model the shear behaviour of rock joints. Comput. Geotech. 2013, 51, 101–115. [Google Scholar] [CrossRef]
- Yang, K.; Hu, Q.; Zhao, H.; Zhao, M.; Zhou, S. Numerical study on the shear behavior of concrete-rock joints with similar triangular asperities. Comput. Geotech. 2023, 159, 105468. [Google Scholar] [CrossRef]
- Cao, R.; Yao, R.; Lin, H.; Lin, Q.; Meng, Q.; Li, T. Shear behaviour of 3D nonpersistent jointed rock-like specimens: Experiment and numerical simulation. Comput. Geotech. 2022, 148, 104858. [Google Scholar] [CrossRef]
- Tai, D.; Qi, S.; Zheng, B.; Wang, C.; Guo, S.; Luo, G. Shear mechanical properties and energy evolution of rock-like samples containing multiple combinations of non-persistent joints. J. Rock Mech. Geotech. Eng. 2023, 15, 1651–1670. [Google Scholar] [CrossRef]
- Chen, M.; Yang, S.; Ranjith, P.; Zhang, Y. Cracking behavior of rock containing non-persistent joints with various joints inclinations. Theor. Appl. Fract. Mech. 2020, 109, 102701. [Google Scholar] [CrossRef]
- Song, Z.; Yang, Z.; Zhang, M.; Wang, F.; Herbst, M.; Konietzky, H. Effect of heterogeneity on mechanical and micro-seismic behaviors of sandstone subjected to multi-level cyclic loading: A discrete element method investigation. J. Rock Mech. Geotech. Eng. 2023, 15, 2556–2581. [Google Scholar] [CrossRef]
- Shao, X.; Chi, S.; Tao, Y.; Zhou, X. DEM simulation of the size effect on the wetting deformation of rockfill materials based on single-particle crushing tests. Comput. Geotech. 2020, 123, 103429. [Google Scholar] [CrossRef]
- Bahaaddini, M.; Hagan, P.; Mitra, R.; Hebblewhite, B. Scale effect on the shear behaviour of rock joints based on a numerical study. Eng. Geol. 2014, 181, 212–223. [Google Scholar] [CrossRef]
- Lin, H.; Kang, W.; Oh, J.; Canbulat, I.; Hebblewhite, B. Numerical simulation on borehole breakout and borehole size effect using discrete element method. Int. J. Min. Sci. Technol. 2020, 30, 623–633. [Google Scholar] [CrossRef]
- Sun, Y.; Kwok, C.; Duan, K. Size effects on crystalline rock masses: Insights from grain-based DEM modeling. Comput. Geotech. 2024, 171, 106376. [Google Scholar] [CrossRef]
- Ye, Y.; Li, C.; Zeng, Y.; Tang, H. 3D DEM simulations of the variability of rock mechanical behaviour based on random rock microcracks. Int. J. Rock Mech. Min. Sci. 2023, 167, 105395. [Google Scholar] [CrossRef]
- Wei, Y.; Feng, Y.; Tian, Z.; Yang, T.; Li, X.; Dai, Z.; Deng, J. Borehole stability in naturally fractured rocks with drilling mud intrusion and associated fracture strength weakening: A coupled DFN-DEM approach. J. Rock Mech. Geotech. Eng. 2024, 16, 1565–1581. [Google Scholar] [CrossRef]
- Jiang, S.; Chen, J.; Wang, Z.; Zheng, J.; Huang, J.; Lv, Q. Three-Dimensional Discrete Element Analysis of Jointed Rock Slope Stability Based on the Universal Elliptical Disc Model. Rock Mech. Rock Eng. 2024, 57, 505–525. [Google Scholar] [CrossRef]
- Vaziri, M.; Tavakoli, H.; Bahaaddini, M. Statistical analysis on the mechanical behaviour of non-persistent jointed rock masses using combined DEM and DFN. Bull. Eng. Geol. Environ. 2022, 81, 177. [Google Scholar] [CrossRef]
- Zhang, Y.; Jiang, Y.; Asahina, D.; Wang, C. Experimental and numerical investigation on shear failure behavior of rock-like samples containing multiple non-persistent joints. Rock Mech. Rock Eng. 2020, 53, 4717–4744. [Google Scholar] [CrossRef]
- ICG (Itasca Consulting Group). PFC-Particle Flow Code Documentation, Version 7.0; Itasca Consulting Group Inc.: Minneapolis, MN, USA, 2019. [Google Scholar]
- Lei, X.; Gong, W.; Li, B.; Zhao, C.; Tang, H.; Wang, L. Probabilistic Analysis of Earthquake-induced Failure and Runout Behaviors of Rock Slopes with Discrete Fracture Network. Comput. Geotech. 2023, 159, 105487. [Google Scholar]
- Ni, X.; Ma, J.; Zhang, F. Mechanism of the variation in axial strain of sand subjected to undrained cyclic triaxial loading explained by DEM with non-spherical particles. Comput. Geotech. 2024, 165, 105846. [Google Scholar] [CrossRef]
- Rafiee, R.; Ataei, M.; KhalooKakaie, R.; Jalali, S.; Sereshki, F.; Noroozi, M. Numerical modeling of influence parameters in cavabililty of rock mass in block caving mines. Int. J. Rock Mech. Min. Sci. 2018, 105, 22–27. [Google Scholar] [CrossRef]
- Zhang, X.; Wong, L. Loading rate effects on cracking behavior of flaw-contained specimens under uniaxial compression. Int. J. Fract. 2013, 180, 93–110. [Google Scholar] [CrossRef]
- Xu, X.; Wu, S.; Gao, Y.; Xu, M. Effects of Micro-structure and Micro-parameters on Brazilian Tensile Strength Using Flat-Joint Model. Rock Mech. Rock Eng. 2016, 49, 3575–3595. [Google Scholar] [CrossRef]
- Wu, S.; Xu, X. A Study of Three intrinsic problems of the classic discrete element method using flat-joint model. Rock Mech. Rock Eng. 2016, 49, 1813–1830. [Google Scholar] [CrossRef]
- Yang, Y.; Qiao, W. Numerical investigation of the shear behavior of granite materials containing discontinuous joints by utilizing the flat-joint model. Comput. Geotech. 2018, 104, 69–80. [Google Scholar] [CrossRef]
- Chen, Y.; Wong, L. A study on mechanical properties and fracturing behavior of Carrara marble with the flat-jointed model. Int. J. Numer. Anal. Methods Geomech. 2020, 44, 803–822. [Google Scholar] [CrossRef]
- Cao, R.; Yao, R.; Dai, H.; Qiu, X.; Lin, H.; Li, K. Fracture behaviour of transversely isotropic rocks under pure mode III fracture: Experiment and numerical simulation. Theor. Appl. Fract. Mech. 2024, 129, 104208. [Google Scholar] [CrossRef]
- Xie, S.; Lin, H.; Duan, H.; Liu, H.; Liu, B. Numerical study on cracking behavior and fracture failure mechanism of fractured rocks under shear loading. Comput. Part. Mech. 2024, 11, 903–920. [Google Scholar] [CrossRef]
- Cao, R.; Cao, P.; Lin, H.; Ma, G.; Chen, Y. Failure characteristics of intermittent fissures under a compressive-shear test: Experimental and numerical analyses. Theor. Appl. Fract. Mech. 2018, 96, 740–757. [Google Scholar] [CrossRef]
- Lin, Q.; Cao, P.; Meng, J.; Cao, R.; Zhao, Z. Strength and failure characteristics of jointed rock mass with double circular holes under uniaxial compression: Insights from discrete element method modelling. Theor. Appl. Fract. Mech. 2020, 109, 102692. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, B.; Wu, S.; Chen, L. Failure mechanism of brittle rock with 3D parallel preset flaws based on the particle displacement trend method. Theor. Appl. Fract. Mech. 2022, 117, 103193. [Google Scholar] [CrossRef]
- Zhang, X.; Wong, L. Displacement field analysis for cracking processes in bonded-particle mode. Bull. Eng. Geol. Environ. 2014, 71, 13–21. [Google Scholar] [CrossRef]
- Sharafisafa, M.; Aliabadian, Z.; Tahmasebinia, F.; Shen, L. Experimental and numerical investigations on crack development in 3D printed rock-like specimens with pre-existing flaws. Eng. Fract. Mech. 2021, 241, 107396. [Google Scholar]
- Luo, X.; Cao, P.; Lin, Q.; Li, S. Mechanical behaviour of fracture-filled rock-like specimens under compression-shear loads: An experimental and numerical study. Theor. Appl. Fract. Mech. 2021, 113, 102935. [Google Scholar] [CrossRef]
- Cai, W.; Li, Y.; Gao, K.; Wang, K. Crack propagation mechanism in rock-like specimens containing intermittent flaws under shear loading. Theor. Appl. Fract. Mech. 2022, 117, 103187. [Google Scholar] [CrossRef]
- Zhao, Y.; Chen, C.; Qi, Q.; Wu, S. Rotation and deflection of 3D principal stress axes induced by a prefabricated single flaw in sandstone: A numerical investigation based on DEM. Theor. Appl. Fract. Mech. 2022, 120, 103430. [Google Scholar] [CrossRef]
- Chen, C.; Zhao, Y.; Wu, S. Influences of true 3D twin flaws on principal stress status and crack occurrence of brittle rock-like samples under uniaxial compression: Insights from experimental and DEM studies. Theor. Appl. Fract. Mech. 2023, 127, 104057. [Google Scholar] [CrossRef]
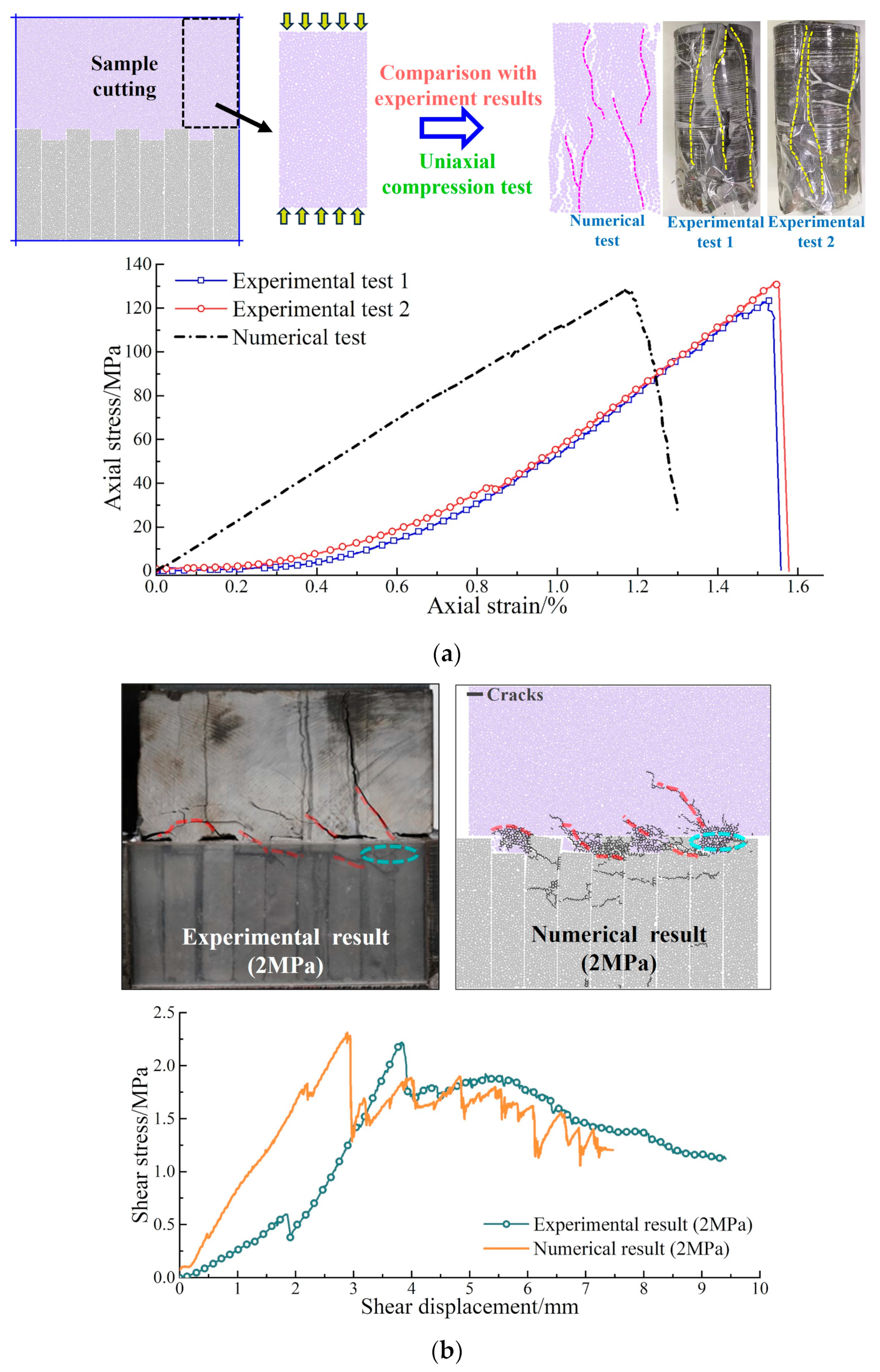





| Limestone | Density (kg/m3) | Elastic Modulus (GPa) | Uniaxial Compressive Strength (MPa) | Poisson’s Ratio |
|---|---|---|---|---|
| Value | 2580 | 13.99 | 131.74 | 0.24 |
| Microscopic Parameters | Limestone | Interface |
|---|---|---|
| Particle minimum radius Rmin (mm) | 0.70 | / |
| Particle maximum radius Rmax (mm) | 1.05 | / |
| Deformability modulus E* (GPa) | 10.20 | 0.11 |
| Normal to shear stiffness ratio k* | 2.00 | 0.50 |
| Tensile strength fj_ten (MPa) | 18.50 | 0.00 |
| Bond strength fj_coh (MPa) | 60.00 | 0.00 |
| Friction angle fj_fa (°) | 35.00 | 55.00 |
| Friction coefficient fj_fric | 0.45 | 0.80 |
| Mechanical Parameters | Experimental Result | Simulation Result | Error |
|---|---|---|---|
| Elastic Modulus (GPa) | 13.99 | 13.64 | 2.50% |
| UCS (Mpa) | 131.74 | 128.51 | 2.45% |
| Poisson’s Ratio | 0.24 | 0.25 | 4.17% |
| Scheme Name | Description | Scheme Name | Description |
|---|---|---|---|
| L-1 | normal stress 1 MPa model size 200 mm step height 10 mm step width 22 mm | L-2 | normal stress 2 MPa model size 200 mm step height 10 mm step width 22 mm |
| L-4 | normal stress 4 MPa model size 200 mm step height 10 mm step width 22 mm | S-1 | normal stress 1 MPa model size 200 mm step height 10 mm step width 22 mm |
| S-2 | normal stress 2 MPa model size 200 mm step height 10 mm step width 22 mm | S-4 | normal stress 4 MPa model size 200 mm step height 10 mm step width 22 mm |
| SS-100 | model size 100 mm normal stress 1, 2, 4 MPa | SS-300 | model size 300 mm normal stress 1, 2, 4 MPa |
| SS-350 | model size 350 mm normal stress 1, 2, 4 MPa | SS-400 | model size 400 mm normal stress 1, 2, 4 MPa |
| SH-3 | Step height 3 mm normal stress 2 MPa | SH-5 | Step height 5 mm normal stress 2 MPa |
| SH-7 | Step height 7 mm normal stress 2 MPa | SH-13 | Step height 13 mm normal stress 2 MPa |
| SH-15 | Step height 15 mm normal stress 2 MPa | SW-16 | Step width 16 mm normal stress 2 MPa |
| SW-18 | Step width 18 mm normal stress 2 MPa | SW-20 | Step width 20 mm normal stress 2 MPa |
| SW-24 | Step width 24 mm normal stress 2 MPa | SW-26 | Step width 26 mm normal stress 2 MPa |
| SW-28 | Step width 28 mm normal stress 2 MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Guo, X.; Liu, X.; Zhou, X.; Xu, B. Shear Mechanical Behaviours and Size Effect of Band–Bedrock Interface: Discrete Element Method Simulation Insights. Appl. Sci. 2024, 14, 9481. https://doi.org/10.3390/app14209481
Wang H, Guo X, Liu X, Zhou X, Xu B. Shear Mechanical Behaviours and Size Effect of Band–Bedrock Interface: Discrete Element Method Simulation Insights. Applied Sciences. 2024; 14(20):9481. https://doi.org/10.3390/app14209481
Chicago/Turabian StyleWang, Hao, Xueyan Guo, Xinrong Liu, Xiaohan Zhou, and Bin Xu. 2024. "Shear Mechanical Behaviours and Size Effect of Band–Bedrock Interface: Discrete Element Method Simulation Insights" Applied Sciences 14, no. 20: 9481. https://doi.org/10.3390/app14209481
APA StyleWang, H., Guo, X., Liu, X., Zhou, X., & Xu, B. (2024). Shear Mechanical Behaviours and Size Effect of Band–Bedrock Interface: Discrete Element Method Simulation Insights. Applied Sciences, 14(20), 9481. https://doi.org/10.3390/app14209481





