A Comparative Study for Evaluating the Groundwater Inflow and Drainage Effect of Jinzhai Pumped Storage Power Station, China
Abstract
1. Introduction
2. Study Site
2.1. Description of Study Area
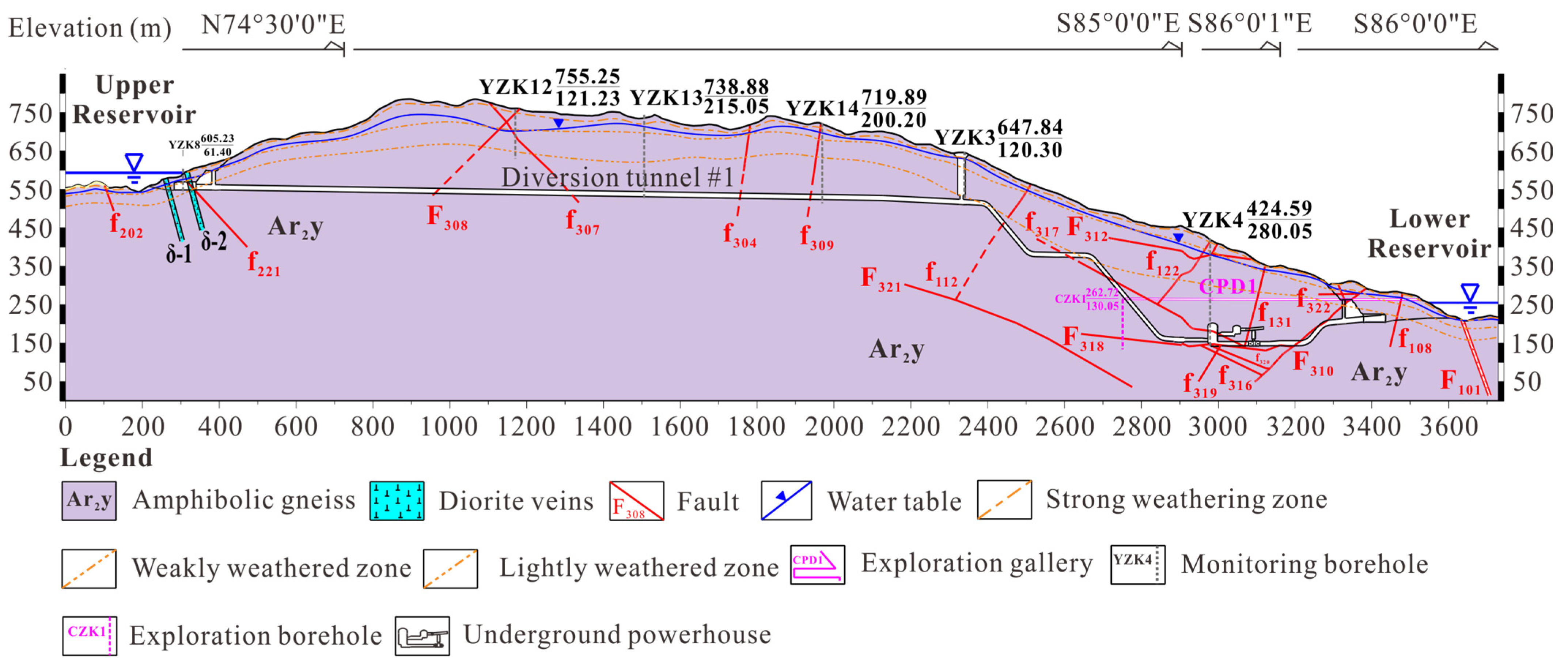
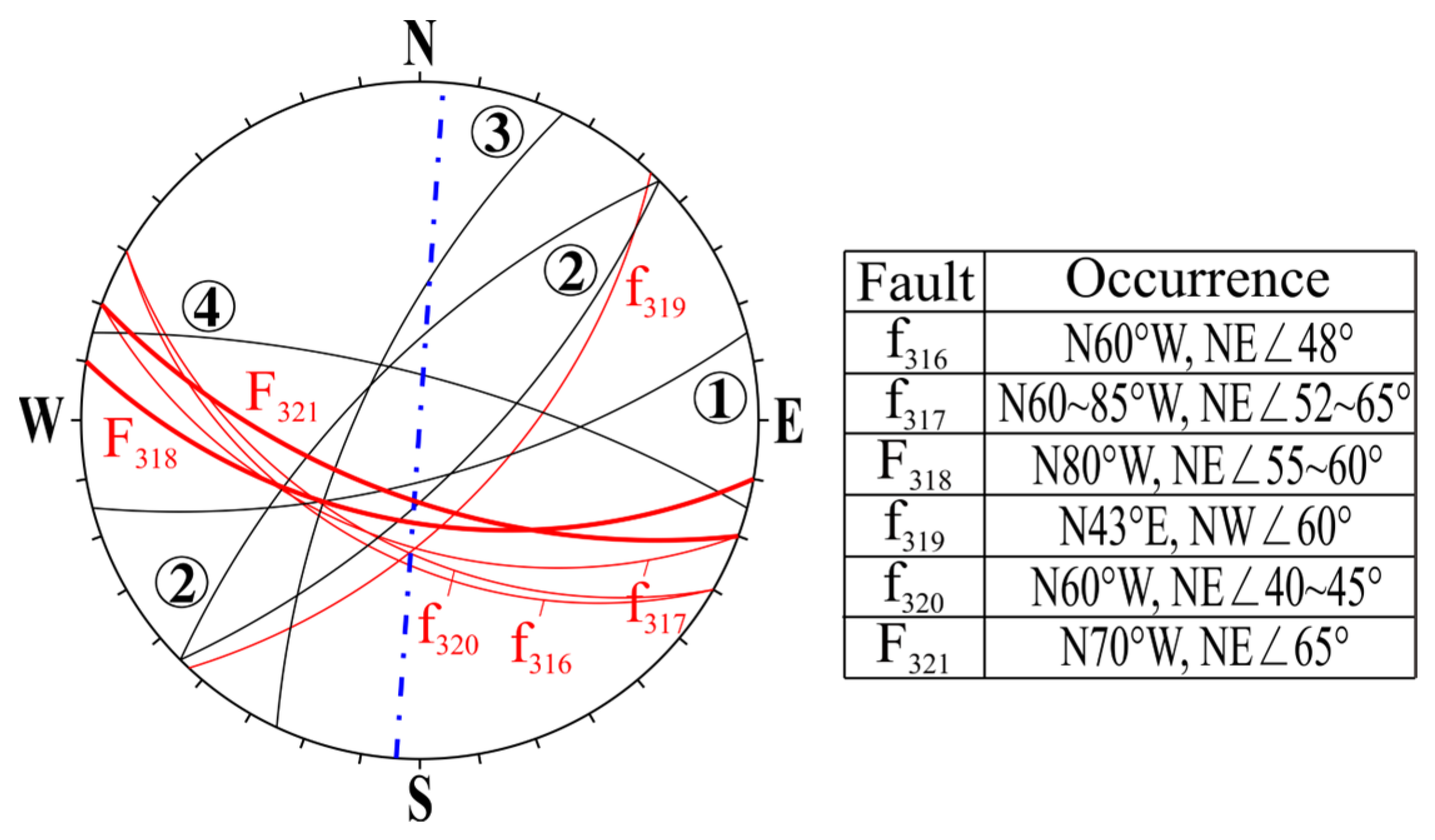
2.2. Engineering Geological Conditions
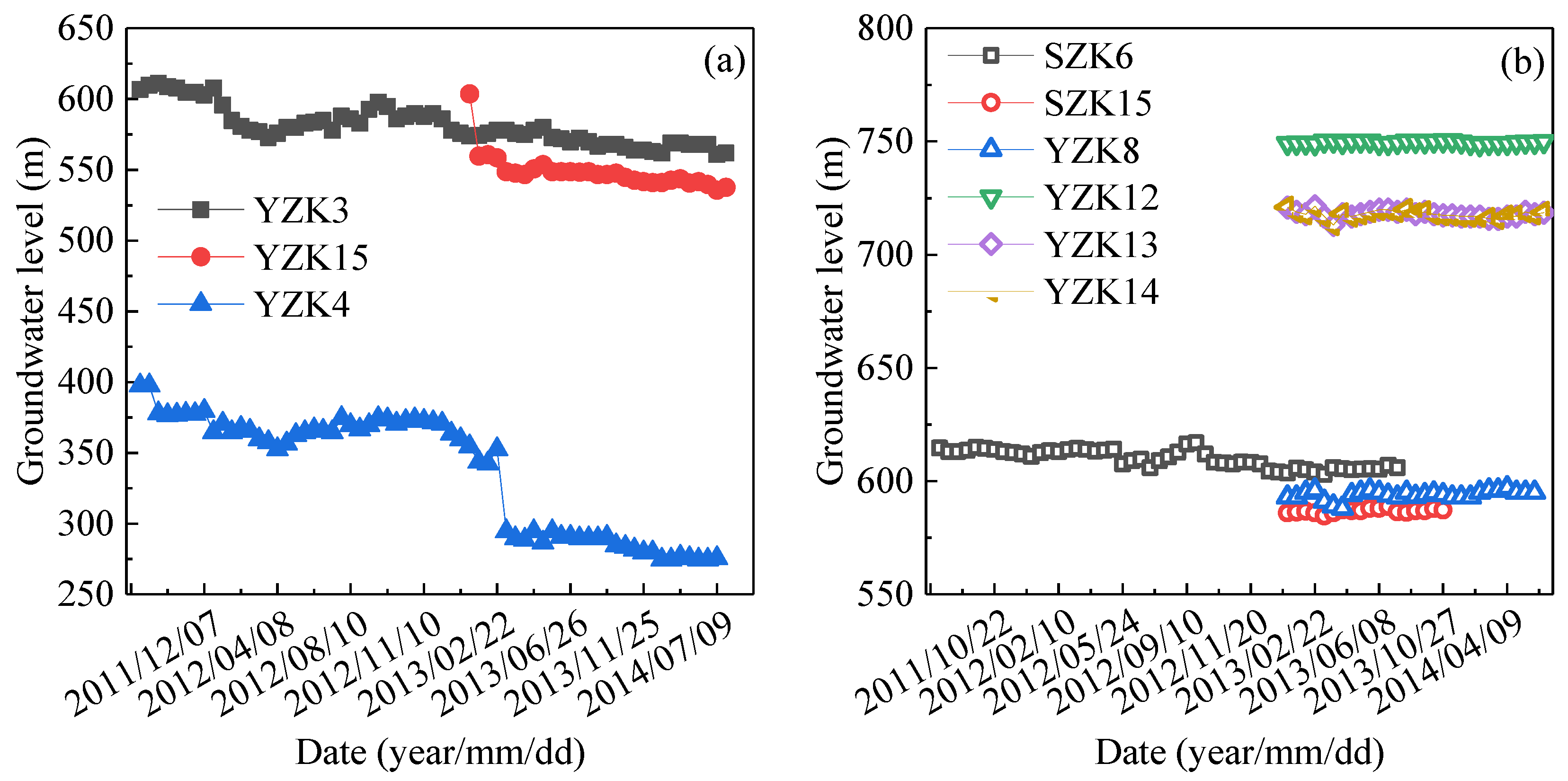
2.3. Hydrogeological Conditions
3. Methods
3.1. Analytical Methods
3.1.1. Analytical Methods Considering Stable Water Table
3.1.2. Analytical Methods Considering Water Table Drawdown
3.2. Empirical Method
3.3. Numerical Modelling
3.3.1. Finite-Element Model
3.3.2. Model Parameters and Synthetic Cases
4. Results and Discussion
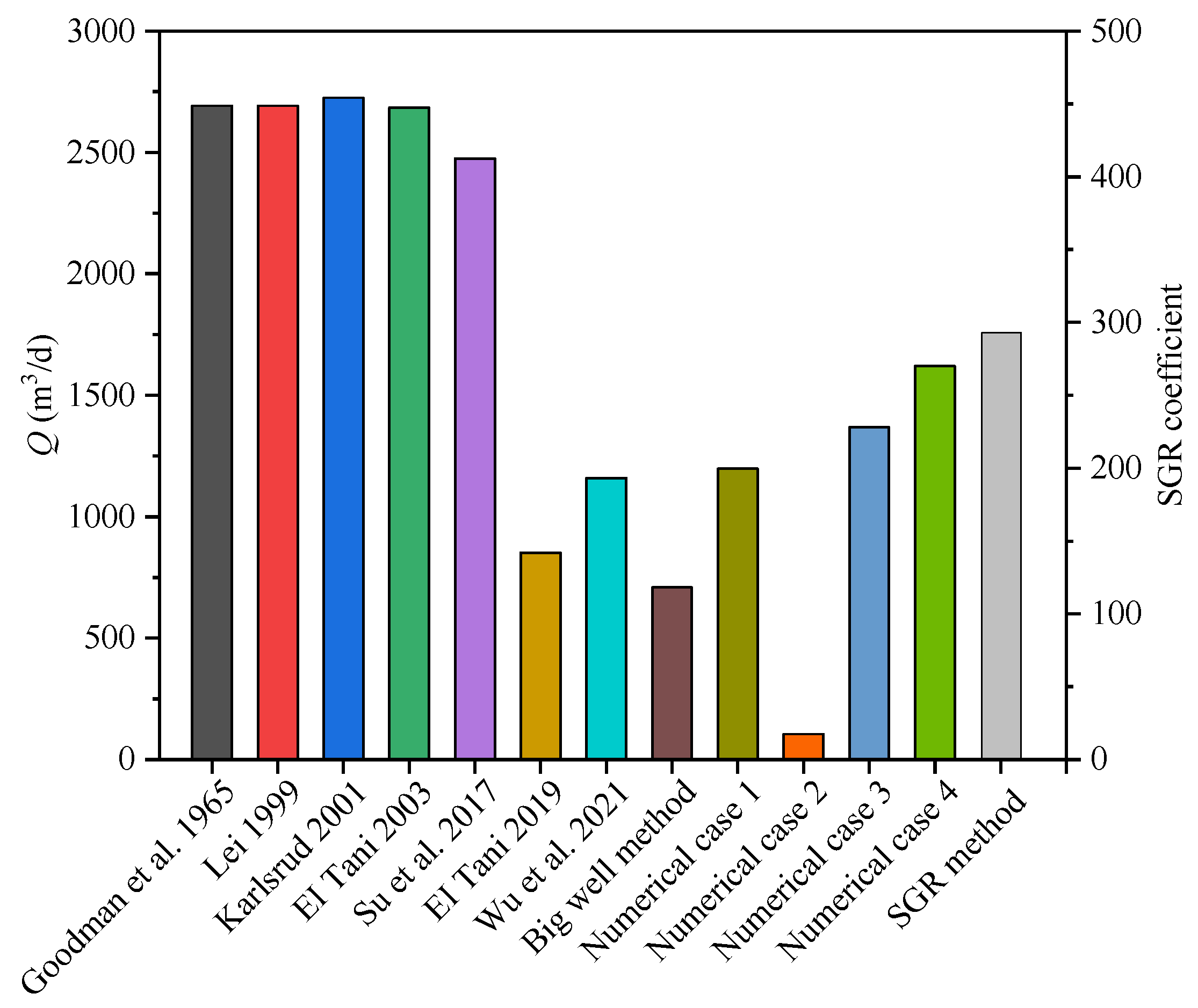
4.1. Groundwater Inflow into Underground Powerhouse
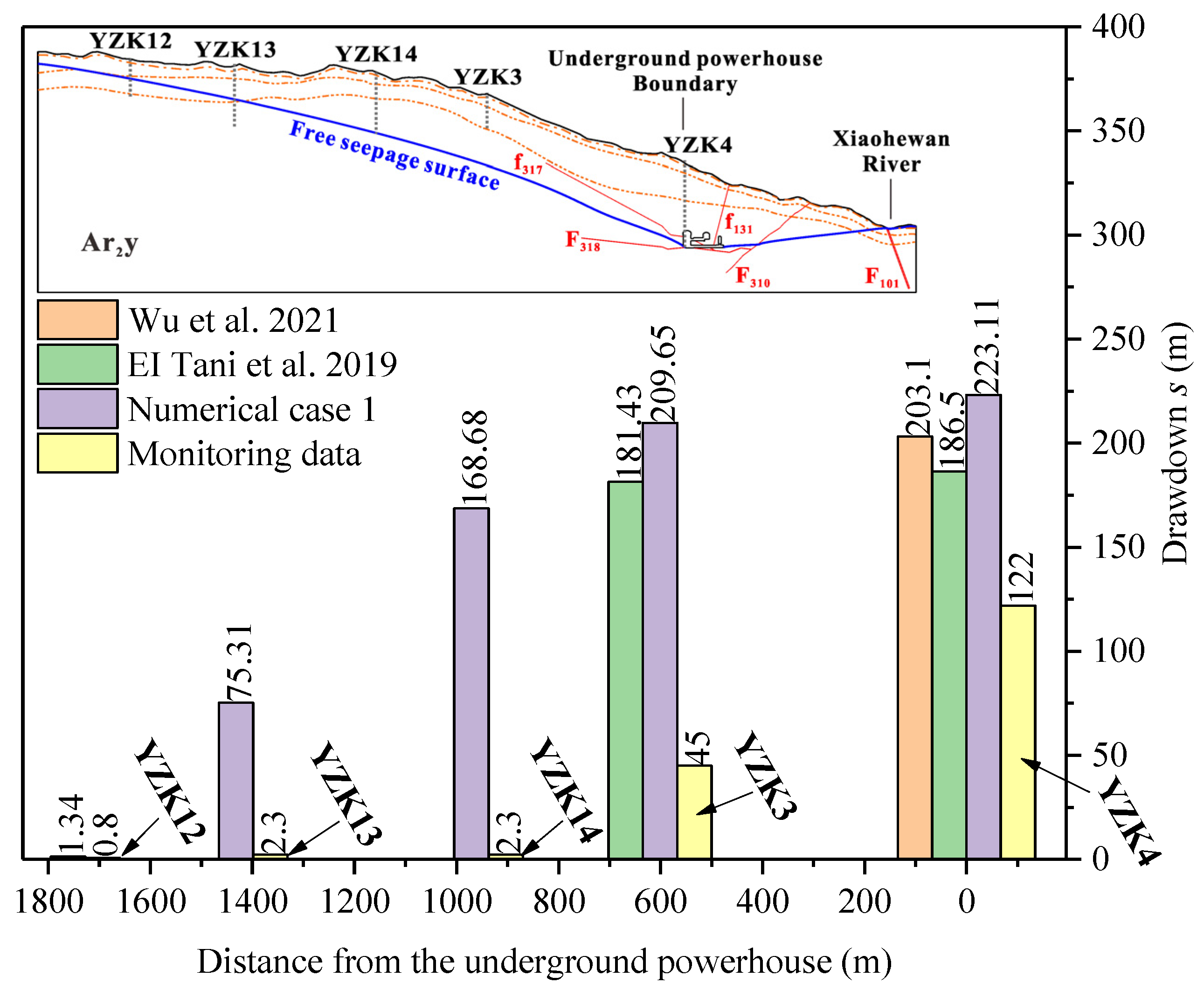
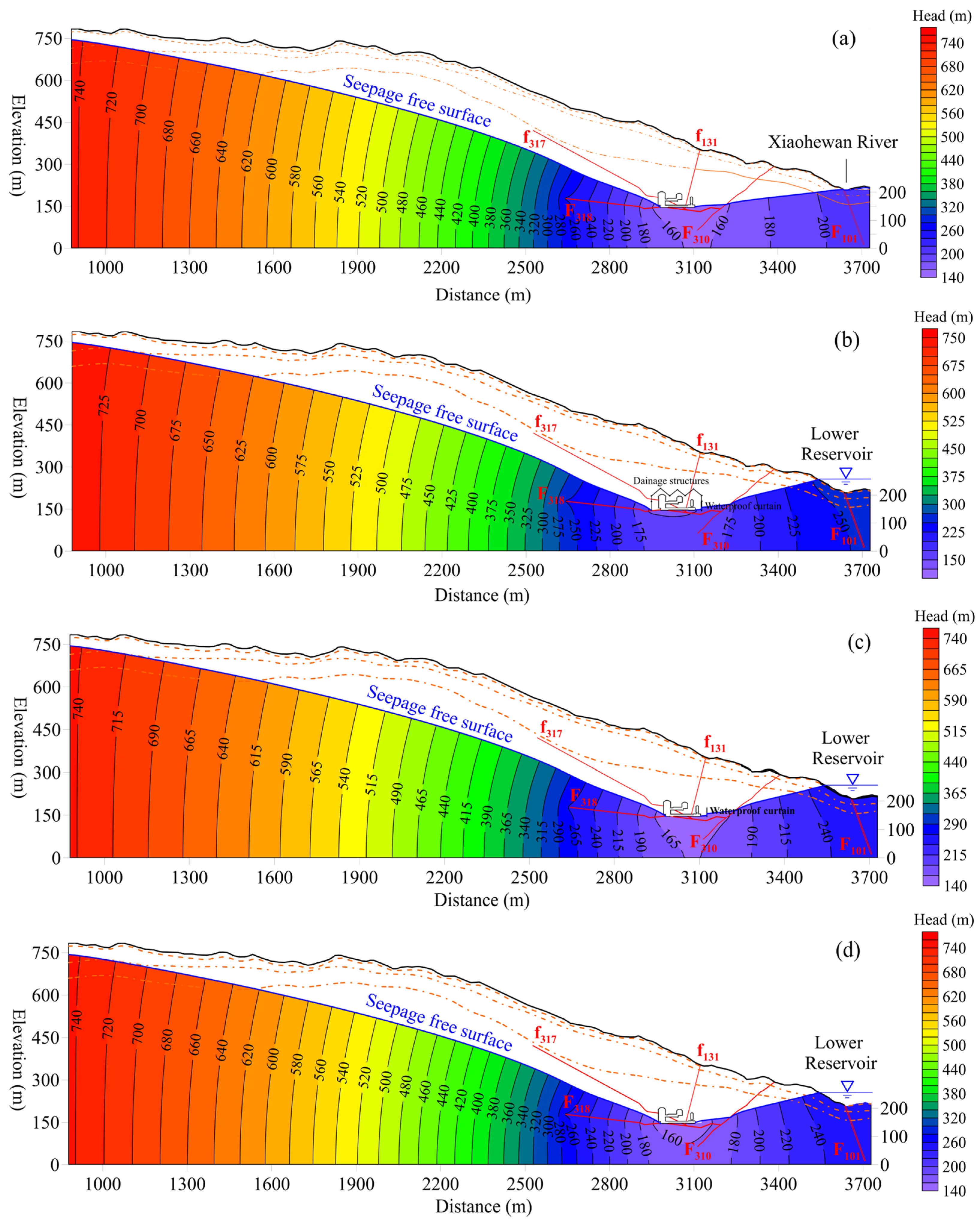
4.2. Water Table Drawdown of Underground Powerhouse
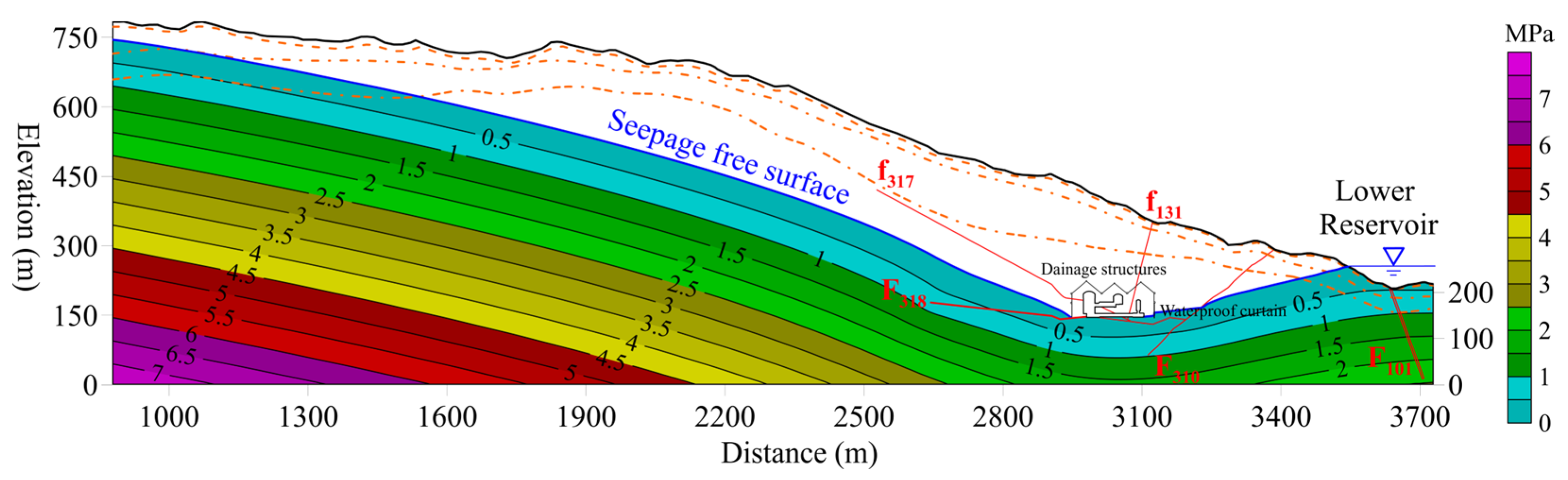
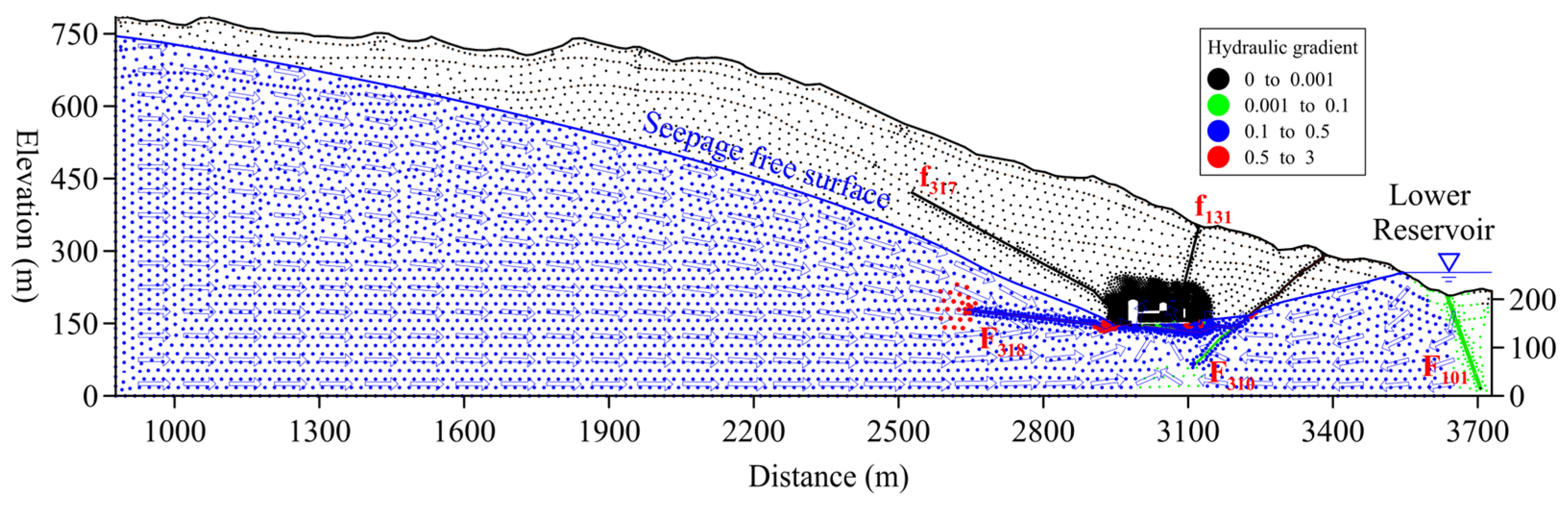
4.3. Water Pressure Redistribution and Hydraulic Gradient
5. Conclusions
- (1)
- The analytical methods considering a stable water table may mostly overestimate the water inflow rates of the underground powerhouse, with an approximate value of 3000 m3/d. Conversely, the analytical results considering the drainage effect show a decent fit with the numerical solution in the excavation condition. Based on the refined numerical simulation of seepage control measures in the operation condition, the water inflow rate is significantly reduced to 104.47 m3/d. Using the SGR method, the underground powerhouse is in “Low Risk” class, with a SGR coefficient of 292.86.
- (2)
- The seepage-free surface intersects precisely on the boundaries of the main and auxiliary powerhouse and tailrace gate chamber, which indicates that the groundwater above these cavern roofs is completely drained. The drainage structures combined with waterproof curtain can reduce the water inflow rates, but cause an increase in the water table drawdown and depression cone. A comparison between analytical and numerical methods and monitoring data of water table drawdown can not only highlight the drainage effect but also show the complexity of the study site.
- (3)
- The underground caverns associated with drainage structures significantly change the pore water pressure distribution and hydraulic gradient. The seepage control measures including drainage holes and tunnels and waterproof curtain prevent groundwater from entering caverns, resulting in a decrease in hydraulic head and water pressure. However, the high relative permeability brings an increase in the inflow rates and hydraulic gradient within the faults (F318, f317). Hence, it is necessary to pay attention to the water inrush in faults during excavation.
- (4)
- Although the capabilities of various methods for tunnel inflow have been effectively identified and the drainage effects caused by cavern excavation have been comprehensively evaluated, the framework proposed in this study has some limitations, especially with homogeneous isotropic media, 2D numerical modeling, steady-state Dracy flow, and simplifying assumptions of analytical formulas. Therefore, the future research advances are necessary to focus on the interactive effects of heterogeneous anisotropy of fractured rock, faults, and seepage control measures on tunnel drainage using a three-dimensional transient numerical simulation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kong, Y.; Kong, Z.; Liu, Z.; Wei, C.; Zhang, J.; An, G. Pumped Storage Power Stations in China: The Past, the Present, and the Future. Renew. Sustain. Energy Rev. 2017, 71, 720–731. [Google Scholar] [CrossRef]
- Mahmoodzadeh, A.; Ghafourian, H.; Mohammed, A.H.; Rezaei, N.; Ibrahim, H.H.; Rashidi, S. Predicting Tunnel Water Inflow Using a Machine Learning-Based Solution to Improve Tunnel Construction Safety. Transp. Geotech. 2023, 40, 100978. [Google Scholar] [CrossRef]
- Wu, J.; Zhifang, Z.; Chao, Z. A Combined Analytical-Numerical Method for Groundwater Inflow into Circular Tunnels in Drained Conditions. Hydrogeol. J. 2021, 29, 2529–2543. [Google Scholar] [CrossRef]
- Gokdemir, C.; Rui, Y.; Rubin, Y.; Li, X. A Framework for Assessing Tunnel Drainage-Induced Impact on Terrestrial Vegetation. Tunn. Undergr. Sp. Technol. 2023, 132, 104917. [Google Scholar] [CrossRef]
- Li, S.; Gao, C.; Zhou, Z.; Li, L.; Wang, M.; Yuan, Y.; Wang, J. Analysis on the Precursor Information of Water Inrush in Karst Tunnels: A True Triaxial Model Test Study. Rock Mech. Rock Eng. 2019, 52, 373–384. [Google Scholar] [CrossRef]
- Hamidi, J.K.; Shahriar, K.; Rezai, B.; Rostami, J.; Bejari, H. Risk Assessment Based Selection of Rock TBM for Adverse Geological Conditions Using Fuzzy-AHP. Bull. Eng. Geol. Environ. 2010, 69, 523–532. [Google Scholar] [CrossRef]
- Zhou, Z.; Zhou, Z.; Xu, H.; Li, M. The Hydrochemical and Isotopic Evolution of the Surface Water and Groundwater for Impoundment in the Xiluodu Reservoir, Jinsha River, China. Sustainability 2020, 12, 5805. [Google Scholar] [CrossRef]
- Lei, S. An Analytical Solution for Steady Flow into a Ttonnel. Ground Water 1999, 37, 23–26. [Google Scholar] [CrossRef]
- Goodman, R.E.; Moye, D.G.; Schalkwyk, A.; Javandel, I. Groundwater Inflow during Tunnel Driving. Eng. Geol. 1965, 2, 39–56. [Google Scholar]
- Karlsrud, K. Water Control When Tunneling under Urban Areas in the Olso Region. NFF Publ. 2001, 12, 27–33. [Google Scholar]
- Hwang, J.; Lu, C. A Semi-Analytical Method for Analyzing the Tunnel Water Inflow. Tunn. Undergr. Sp. Technol. 2007, 22, 39–46. [Google Scholar] [CrossRef]
- Shen, H.; Huang, Y.; Tang, Y.; Qiu, H.; Wang, P. Impact Analysis of Karst Reservoir Construction on the Surrounding Environment: A Case Study for the Southwest of China. Water 2019, 11, 2327. [Google Scholar] [CrossRef]
- Verruijt, A.; Booker, J.R. Complex Variable Analysis of Mindlin’s Tunnel Problem. In Proceedings of the Developments in Theoretical Geomechanics: Proceedings of the Booker Memorial Symposium, Sydney, Australia, 16–17 November 2000; pp. 1–20. [Google Scholar]
- Zhang, Y.; Zhang, D.; Fang, Q.; Xiong, L.; Yu, L.; Zhou, M. Analytical Solutions of Non-Darcy Seepage of Grouted Subsea Tunnels. Tunn. Undergr. Sp. Technol. 2020, 96, 103182. [Google Scholar] [CrossRef]
- Huangfu, M.; Wang, M.S.; Tan, Z.S.; Wang, X.Y. Analytical Solutions for Steady Seepage into an Underwater Circular Tunnel. Tunn. Undergr. Sp. Technol. 2010, 25, 391–396. [Google Scholar] [CrossRef]
- Aalianvari, H.K. Development of a New Method for Tunnel Site Rating from Groundwater Hasard Point of View. J. Appl. Sci. 2009, 9, 1496–1502. [Google Scholar]
- Zarei, H.R.; Uromeihy, A.; Sharifzadeh, M. A New Tunnel Inflow Classification (TIC) System through Sedimentary Rock Masses. Tunn. Undergr. Sp. Technol. 2013, 34, 1–12. [Google Scholar] [CrossRef]
- Rasouli Maleki, M. Groundwater Seepage Rate (GSR); a New Method for Prediction of Groundwater Inflow into Jointed Rock Tunnels. Tunn. Undergr. Sp. Technol. 2018, 71, 505–517. [Google Scholar] [CrossRef]
- Nikvar Hassani, A.; Farhadian, H.; Katibeh, H. A Comparative Study on Evaluation of Steady-State Groundwater Inflow into a Circular Shallow Tunnel. Tunn. Undergr. Sp. Technol. 2018, 73, 15–25. [Google Scholar] [CrossRef]
- Mojiri-Khozani, A.; Nassery, H.R.; Nikpeyman, Y.; Abedian, H. Assessing the Impact of Koohrang III Tunnel on the Hydrogeological Settings Using Stable Isotopes and Hydrochemical Methods. Bull. Eng. Geol. Environ. 2023, 82, 219. [Google Scholar] [CrossRef]
- Abedian, H.; Karami, G.H.; Karimi, H. The Effect of Scale on the Water Leakage from the Reservoir and Abutment of Beheshtabad Dam. Bull. Eng. Geol. Environ. 2019, 78, 5569–5581. [Google Scholar] [CrossRef]
- Tran, T.; Tapas, M.R.; Do, S.K.; Etheridge, R. Investigating the Impacts of Climate Change on Hydroclimatic Extremes in the Tar-Pamlico River Basin, North Carolina. J. Environ. Manage. 2024, 363, 121375. [Google Scholar] [CrossRef] [PubMed]
- Tran, T.; Nguyen, B.Q. The Role of Reservoirs under the Impacts of Climate Change on the Srepok River Basin, Central Highlands of Vietnam. Front. Environ. Sci. 2023, 11, 1304845. [Google Scholar] [CrossRef]
- Tapas, M.R.; Etheridge, R.; Tran, T.; Finlay, C.G.; Peralta, A.L.; Bell, N.; Xu, Y.; Lakshmi, V. A Methodological Framework for Assessing Sea Level Rise Impacts on Nitrate Loading in Coastal Agricultural Watersheds Using SWAT+: A Case Study of the Tar-Pamlico River Basin, North Carolina, USA. Sci. Total Environ. 2024, 951, 175523. [Google Scholar] [CrossRef]
- Desai, C.S.; Li, G.C. A Residual Flow Procedure and Application for Free Surface Flow in Porous Media. Adv. Water Resour. Res. 1983, 6, 27–35. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, P.; Wang, L. Initial Flow Method for Seepage Analysis with Free Surface. Chinese J. Hydraul. Eng. 1988, 8, 18–26. [Google Scholar]
- Bathe, K.J.; Khoshgoftaar, M.R. Finite Element Free Surface Seepage Analysis without Mesh Iteration. Int. J. Numer. Anal. Methods Geomech. 1979, 3, 13–22. [Google Scholar] [CrossRef]
- Kikuchi, N. Seepage Flow Problems by Variational Inequalities: Theory and Approximation. Int. J. Numer. Anal. Methods Geomech. 1977, 1, 283–297. [Google Scholar] [CrossRef]
- Zheng, H.; Liu, D.F.; Lee, C.F.; Tham, L.G. A New Formulation of Signorini’s Type for Seepage Problems with Free Surfaces. Int. J. Numer. Methods Eng. 2005, 64, 1–16. [Google Scholar] [CrossRef]
- Tang, F.; Yang, J.; Li, H.N.; Liu, F.; Wang, N.; Jia, P.; Chen, Y. Field Validation of a Magnetic Sensor to Monitor Borehole Deviation during Tunnel Excavation. Materials 2018, 11, 1511. [Google Scholar] [CrossRef]
- El Tani, M. Circular Tunnel in a Semi-Infinite Aquifer. Tunn. Undergr. Sp. Technol. 2002, 18, 49–55. [Google Scholar] [CrossRef]
- Fernandez, G.; Moon, J. Excavation-Induced Hydraulic Conductivity Reduction around a Tunnel–Part 2: Verification of Proposed Method Using Numerical Modeling. Tunn. Undergr. Sp. Technol. 2010, 25, 567–574. [Google Scholar] [CrossRef]
- Su, K.; Zhou, Y.; Wu, H.; Shi, C.; Zhou, L. An Analytical Method for Groundwater Inflow into a Drained Circular Tunnel. Groundwater 2017, 55, 712–721. [Google Scholar] [CrossRef]
- El, M.; Abbas, T.; Mohamed, K.; Gholami, A. Analytic Assessment of the Water Table Drawdown, Seepage, and Back Pressure at Rudbar PSPP. Rock Mech. Rock Eng. 2019, 52, 2227–2243. [Google Scholar]
- NB/T 10073-2018; Specification for Engineering Geological Investigation of Pumped Storage Power Stations. National Energy Administration of the People’s Republic of China: Beijing, China, 2018; pp. 1–74.
- Chen, Y.; Zhou, C.; Zheng, H. A Numerical Solution to Seepage Problems with Complex Drainage Systems. Comput. Geotech. 2008, 35, 383–393. [Google Scholar] [CrossRef]
- Dai, Y.; Zhou, Z. Steady Seepage Simulation of Underground Oil Storage Caverns Based on Signorini Type Variational Inequality Formulation. Geosci. J. 2015, 19, 341–355. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.; Jiang, Q.; Hu, R.; Zhou, C. Performance Assessment and Optimization of Seepage Control System: A Numerical Case Study for Kala Underground Powerhouse. Comput. Geotech. 2014, 55, 306–315. [Google Scholar] [CrossRef]
- Zhuang, C.; Zhou, Z.; Illman, W.A.; Wang, J. Geostatistical Inverse Modeling for the Characterization of Aquitard Heterogeneity Using Long-Term Multi-Extensometer Data. J. Hydrol. 2019, 578, 124024. [Google Scholar] [CrossRef]
- Luo, M.; Chen, J.; Jakada, H.; Li, N.; Guo, X.; Zhou, H. Identifying and Predicting Karst Water Inrush in a Deep Tunnel, South China. Eng. Geol. 2022, 305, 106716. [Google Scholar] [CrossRef]
- Mar, J.; Lanini, S.; Aunay, B.; Perrochet, P. Analytical Solution for Modeling Discharge into a Tunnel Drilled in a Heterogeneous Unconfined Aquifer. Groundwater 2014, 52, 597–605. [Google Scholar]
- Zhang, D.M.; Xie, X.C.; Zhou, M.L.; Huang, Z.K.; Zhang, D.M. An Incident of Water and Soil Gushing in a Metro Tunnel Due to High Water Pressure in Sandy Silt. Eng. Fail. Anal. 2021, 121, 105196. [Google Scholar] [CrossRef]
- Lv, Y.; Jiang, Y.; Hu, W.; Cao, M.; Mao, Y. A Review of the Effects of Tunnel Excavation on the Hydrology, Ecology, and Environment in Karst Areas: Current Status, Challenges, and Perspectives. J. Hydrol. 2020, 586, 124891. [Google Scholar] [CrossRef]
- Su, K.; Zhang, Y.J.; Chang, Z.H.; Wu, H.G.; Wang, T.; Zhou, W. Transverse Extent of Numerical Model for Deep Buried Tunnel Excavation. Tunn. Undergr. Sp. Technol. 2019, 84, 373–380. [Google Scholar] [CrossRef]
- Chen, Y.F.; Liao, Z.; Zhou, J.Q.; Hu, R.; Yang, Z.; Zhao, X.J.; Wu, X.L.; Yang, X.L. Non-Darcian Flow Effect on Discharge into a Tunnel in Karst Aquifers. Int. J. Rock Mech. Min. Sci. 2020, 130, 104319. [Google Scholar] [CrossRef]

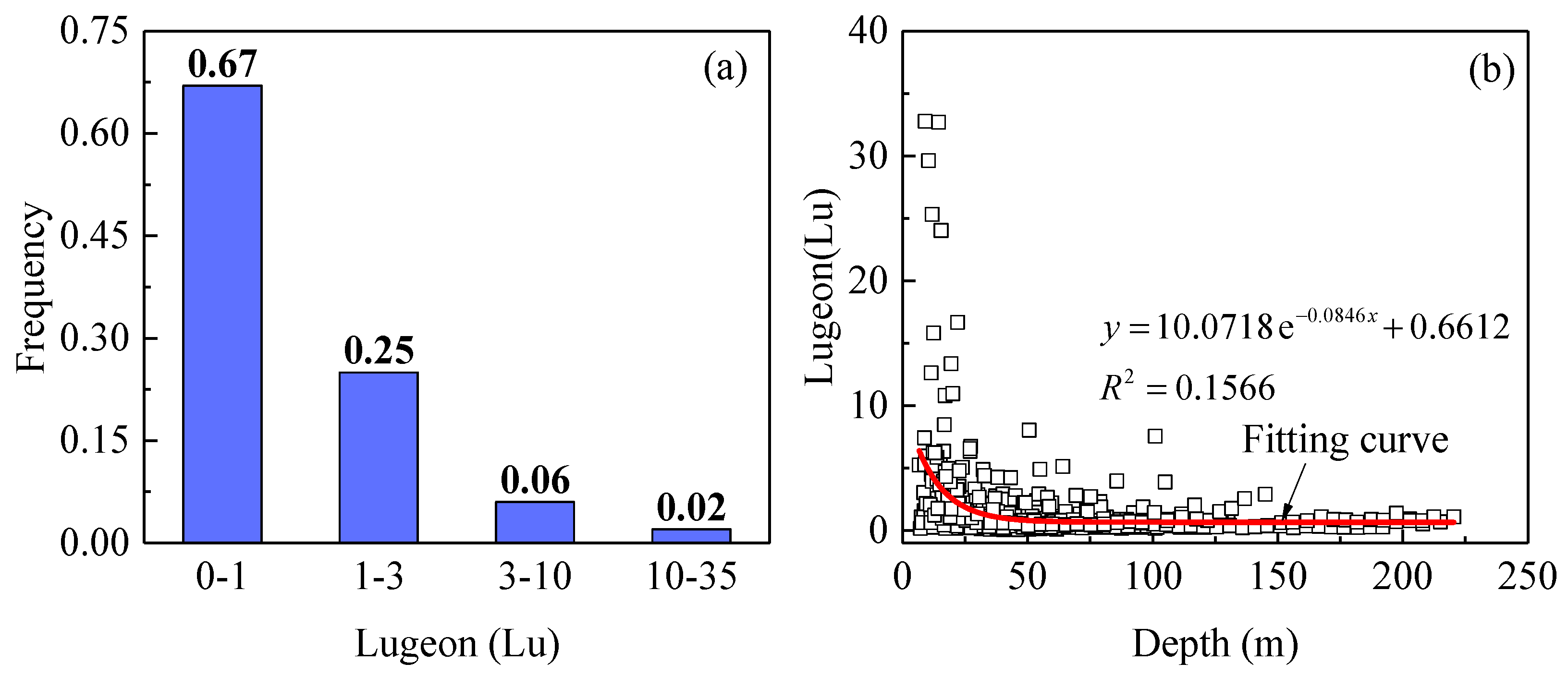
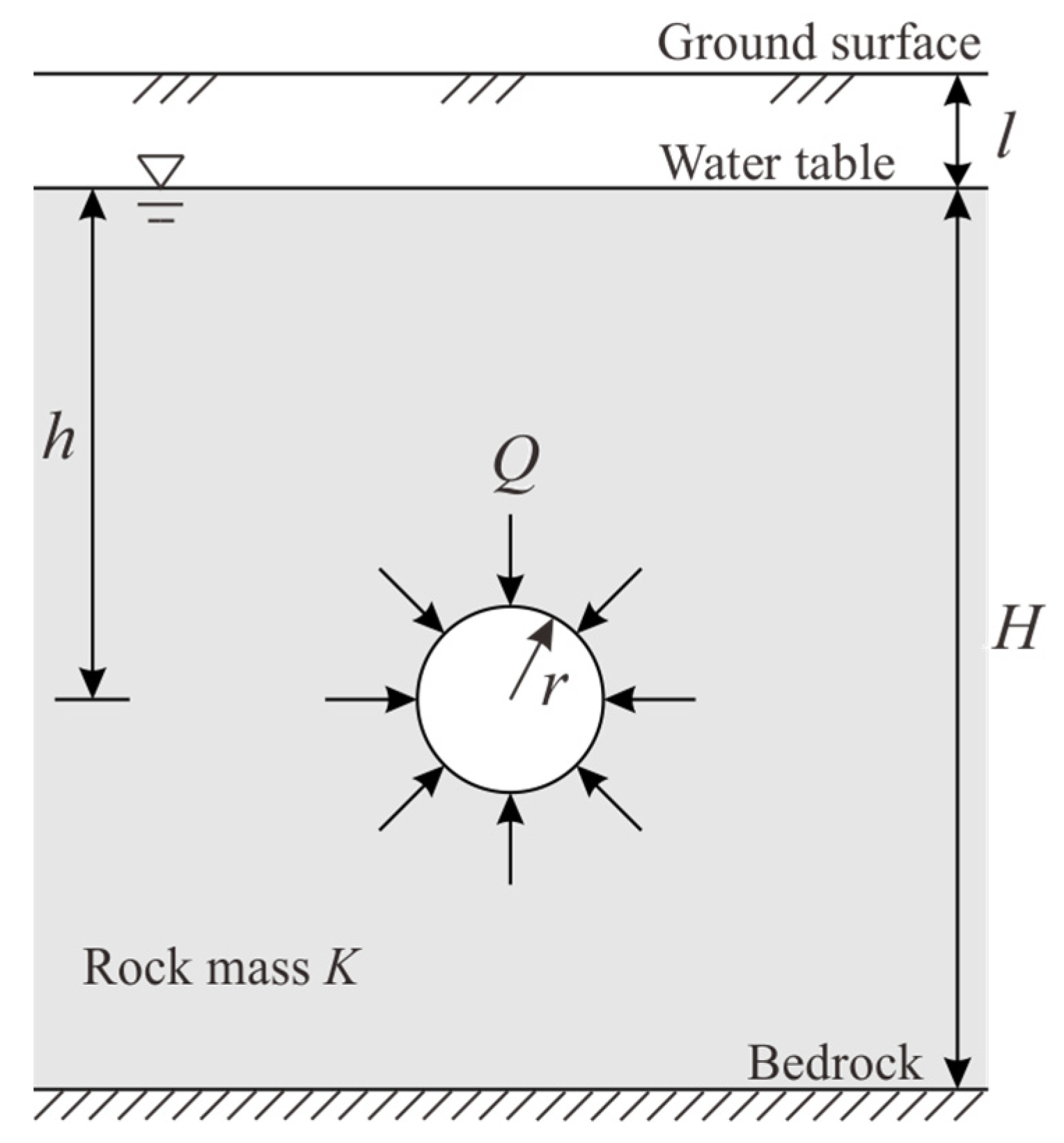
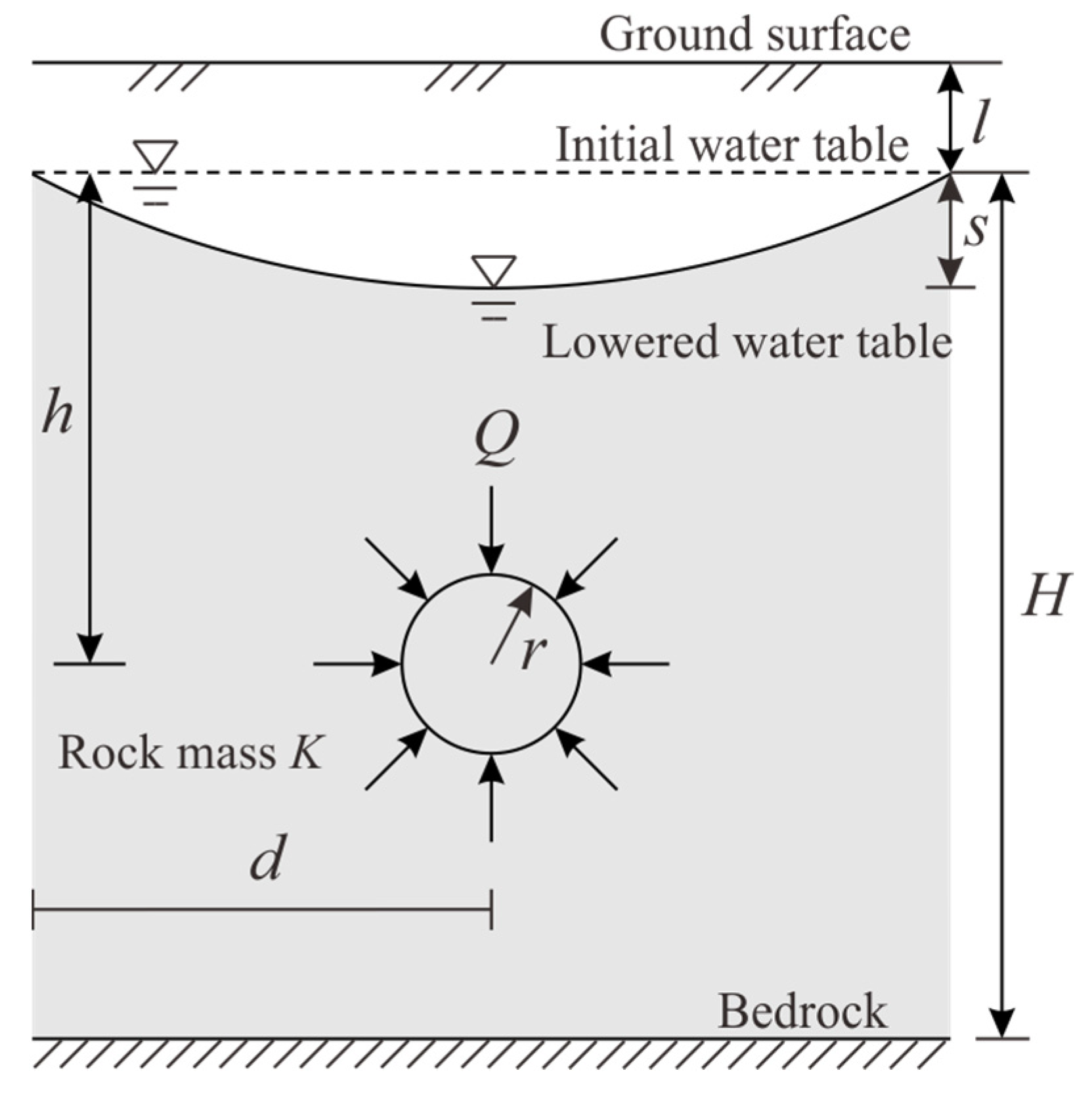
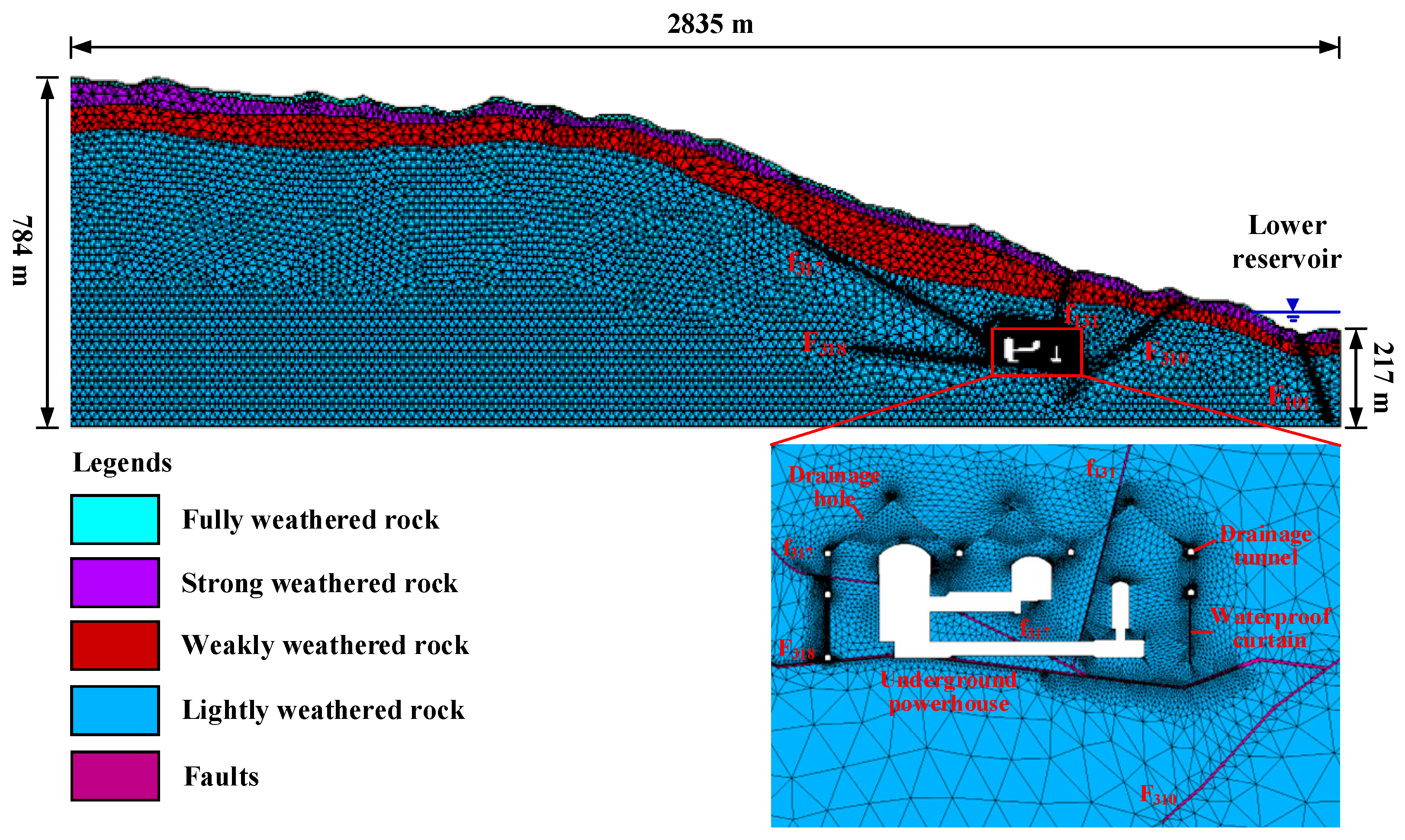
| SGR | Tunnel Rating | Class | Probable Conditions for Groundwater Inflow into Tunnel (L/s/m) |
|---|---|---|---|
| 0–100 | No risk | I | 0–0.04 |
| 100–300 | Low risk | II | 0.04–0.1 |
| 300–500 | Moderate risk | III | 0.1–0.16 |
| 500–700 | Risky | IV | 0.16–0.28 |
| 700–1000 | High risk | V | Q > 0.28, inflow of groundwater and mud from crashed zones is probable |
| 1000< | Critical | VI | Inflow of groundwater and mud is highly probable |
| Model Parameters | Formations | Faults | Waterproof Curtain | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| CW Rock | SW Rock | WW Rock | LW Rock | F318 | F310 | f317 | f131 | F101 | ||
| Permeability K (m/d) | 4.97 × 10−2 | 1.42 × 10−2 | 8.36 × 10−3 | 7.41 × 10−3 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 8.64 × 10−5 |
| Thickness d (m) | 0~17.3 | 6.5~58.8 | 22.5~109.9 | 156.4~668.9 | 0.7 | 1.0 | 0.5 | 0.5 | 4.0 | 1.0 |
| Specific yield μ | 0.1 | 0.02 | 0.01 | 0.001 | 0.05 | 0.05 | 0.01 | 0.01 | 0.05 | 0.0001 |
| No. | Water Head (m) | Seepage Control Structures | Working Conditions | |||
|---|---|---|---|---|---|---|
| Left Boundary | Right Boundary | Drainage Holes | Drainage Tunnels | Waterproof Curtain | ||
| Case 1 | 745 | 210 | None | None | None | Excavation condition |
| Case 2 | 745 | 255 | Effective | Effective | Effective | Operation condition |
| Case 3 | 745 | 255 | Failure | Failure | Effective | Risk condition |
| Case 4 | 745 | 255 | Failure | Failure | Failure | Risk condition |
| Underground Powerhouse | K (m/d) | h (m) | H (m) | r (m) | L (m) | d (m) | R (m) |
|---|---|---|---|---|---|---|---|
| Main and auxiliary powerhouse | 0.016 | 211.46 | 378.19 | 21.23 | 176.3 | 651 | 602.67 |
| Main transformed cavern | 0.016 | 186.96 | 360.61 | 11.47 | 168.0 | 500.1 | 588.50 |
| Tailrace gate chamber | 0.016 | 186.95 | 347.61 | 7.47 | 111.0 | 455.1 | 577.79 |
| Underground Powerhouse | Water Inflow Rates Q (m3/d) | Drawdown s (m) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Main and auxiliary powerhouse | 1252.72 | 1253.78 | 1274.66 | 1247.44 | 1233.97 | 325.99 | 330.07 | 288.82 | 186.50 | 203.10 | 122 |
| Main transformed cavern | 906.24 | 906.48 | 914.41 | 904.77 | 786.50 | 308.06 | 480.03 | 230.39 | 146.31 | 175.08 | 122 |
| Tailrace gate chamber | 533.15 | 533.20 | 535.91 | 532.77 | 454.85 | 217.23 | 348.19 | 189.90 | 146.79 | 151.23 | 122 |
| Total | 2692.10 | 2693.46 | 2724.98 | 2684.99 | 2475.32 | 851.28 | 1158.30 | 709.11 | 186.50 | 203.10 | 122 |
| Underground Powerhouse | h (m) | λi (1/m) | ei (m) | CZW (m) | S1 | S2 | S3 | S4 | S5 | S6 | S7 | SGR | Tunnel Rating |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Main and auxiliary powerhouse | 211.46 | 1.98 | 0.005 | 0.44 | 1.03 | 2 | 1.28 | 0 | 0 | 39.50 | 1.0 | 170.27 | Low risk |
| Main transformed cavern | 186.96 | 1.11 | 0.003 | 0 | 0.21 | 2 | 0 | 0 | 0 | 35.74 | 1.0 | 78.99 | No risk |
| Tailrace gate chamber | 186.95 | 1.18 | 0.003 | 0 | 0.22 | 1 | 0 | 0 | 0 | 35.74 | 1.0 | 43.60 | No risk |
| Total | / | / | / | / | / | / | / | / | / | / | / | 292.86 | Low risk |
| No. | Water Inflow into Underground Powerhouse Q (m3/d/m) | Water Inflow into Drainage Structures (m3/d/m) | Drawdown s (m) | |||||
|---|---|---|---|---|---|---|---|---|
| Main and Auxiliary Powerhouse | Main Transformed Cavern | Tailrace Gate Chamber | Total | Drainage Holes | Drainage Tunnels | Total | ||
| Case 1 | 753.15 | 0 | 474.70 | 1227.85 | None | None | None | 231.8 |
| Case 2 | 60.48 | 0 | 43.99 | 104.47 | 875.55 | 1035.68 | 1911.23 | 234.3 |
| Case 3 | 820.47 | 0 | 607.69 | 1428.16 | None | None | None | 231.8 |
| Case 4 | 830.23 | 0 | 689.56 | 1519.79 | None | None | None | 231.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Zhou, Z.; Wang, H.; Chen, B.; Wang, J. A Comparative Study for Evaluating the Groundwater Inflow and Drainage Effect of Jinzhai Pumped Storage Power Station, China. Appl. Sci. 2024, 14, 9123. https://doi.org/10.3390/app14199123
Wu J, Zhou Z, Wang H, Chen B, Wang J. A Comparative Study for Evaluating the Groundwater Inflow and Drainage Effect of Jinzhai Pumped Storage Power Station, China. Applied Sciences. 2024; 14(19):9123. https://doi.org/10.3390/app14199123
Chicago/Turabian StyleWu, Jian, Zhifang Zhou, Hao Wang, Bo Chen, and Jinguo Wang. 2024. "A Comparative Study for Evaluating the Groundwater Inflow and Drainage Effect of Jinzhai Pumped Storage Power Station, China" Applied Sciences 14, no. 19: 9123. https://doi.org/10.3390/app14199123
APA StyleWu, J., Zhou, Z., Wang, H., Chen, B., & Wang, J. (2024). A Comparative Study for Evaluating the Groundwater Inflow and Drainage Effect of Jinzhai Pumped Storage Power Station, China. Applied Sciences, 14(19), 9123. https://doi.org/10.3390/app14199123