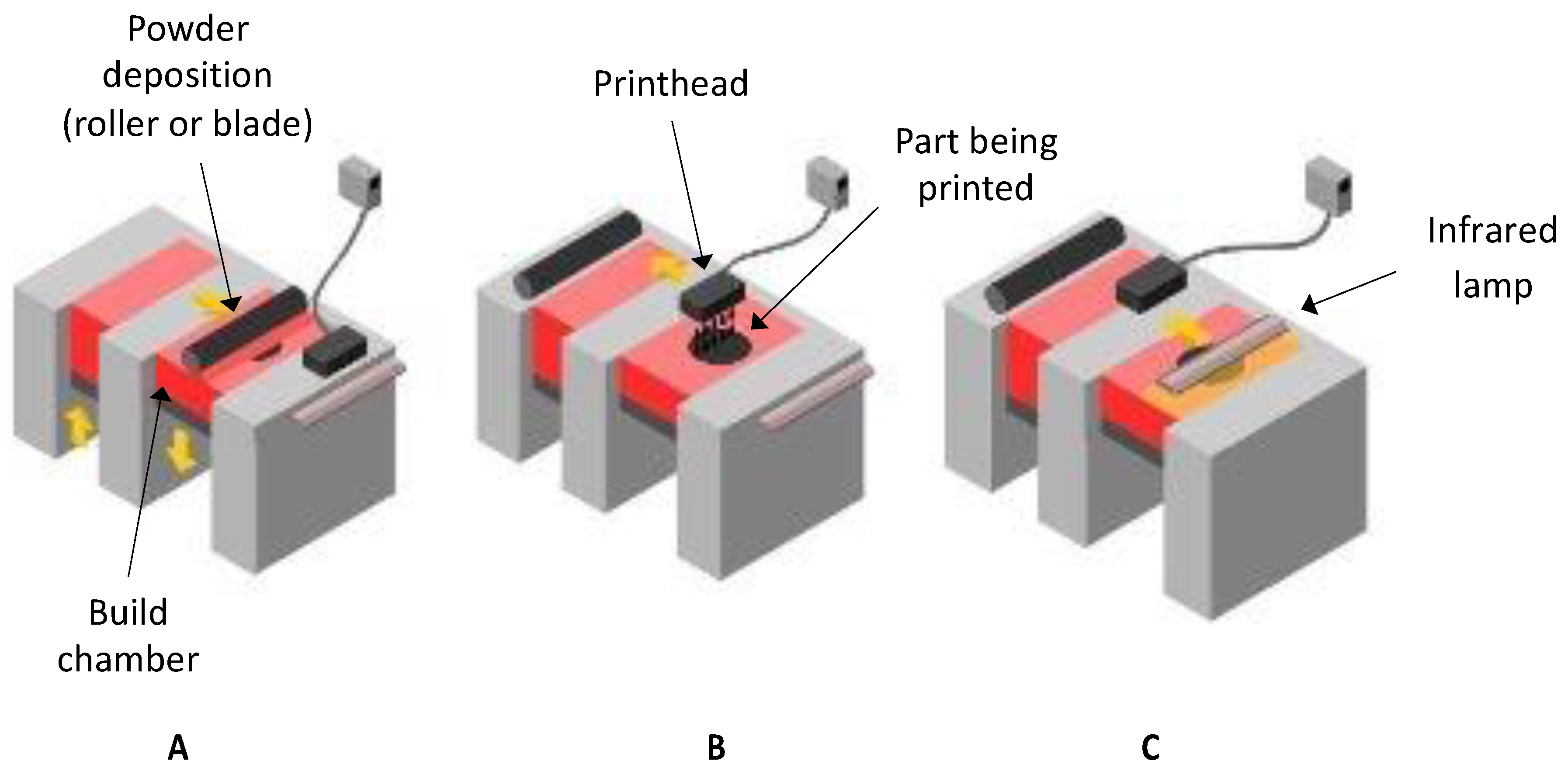
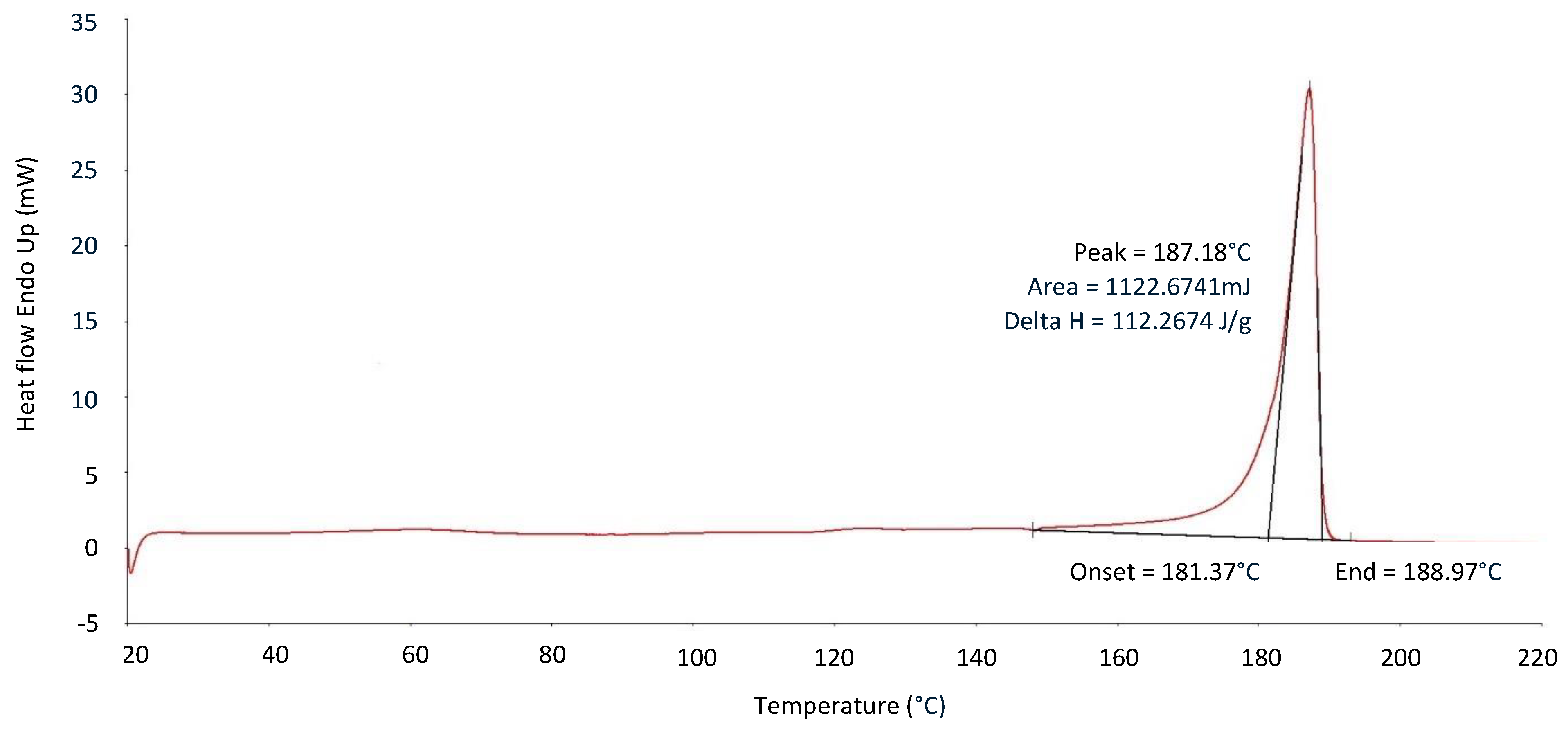
4. Discussion
The trends seen here correlate well with the other literature focused on powdered polymer additive manufacturing, much of which has been based on selective laser sintering. Research on PA12, presented in [
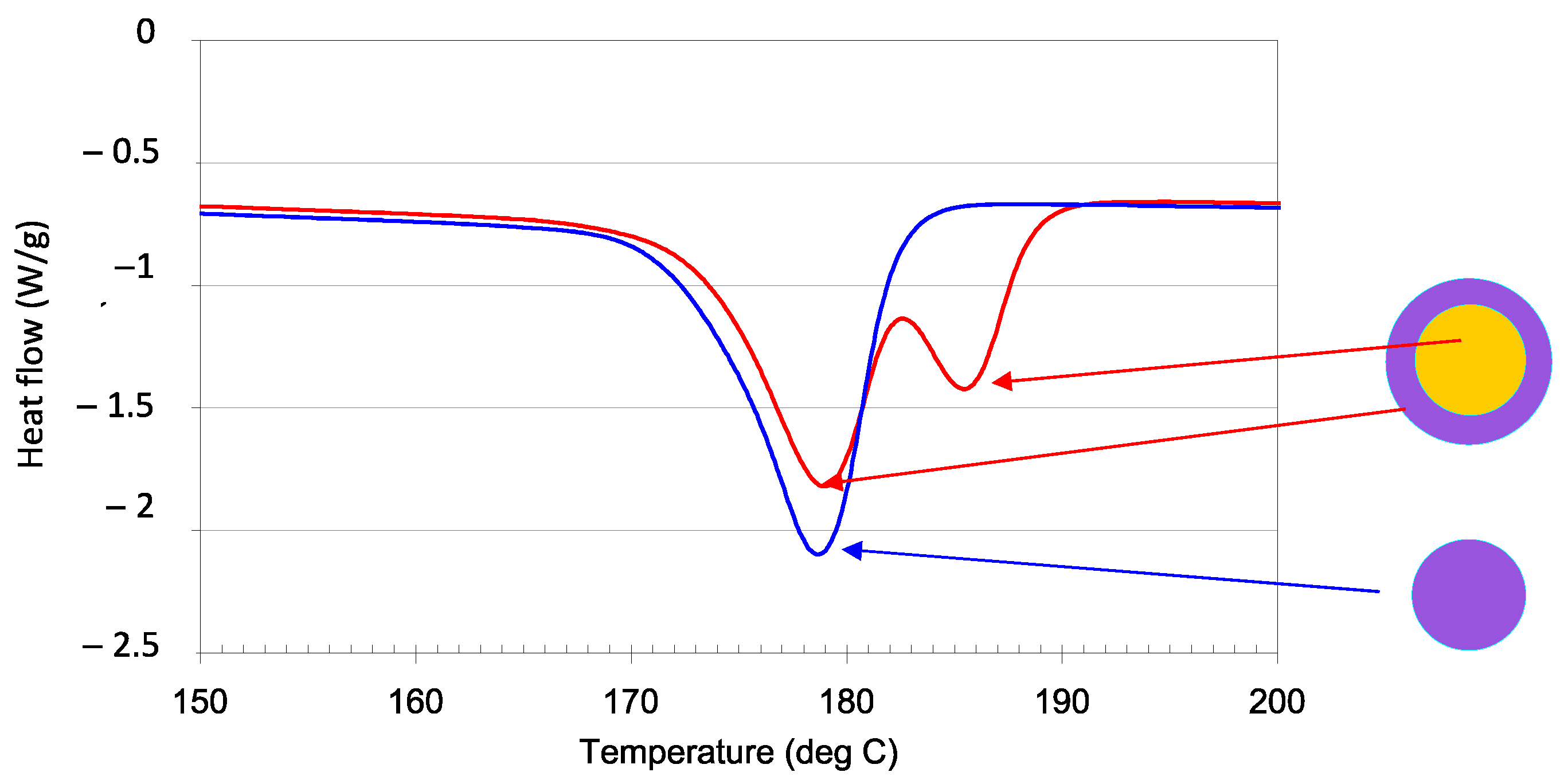
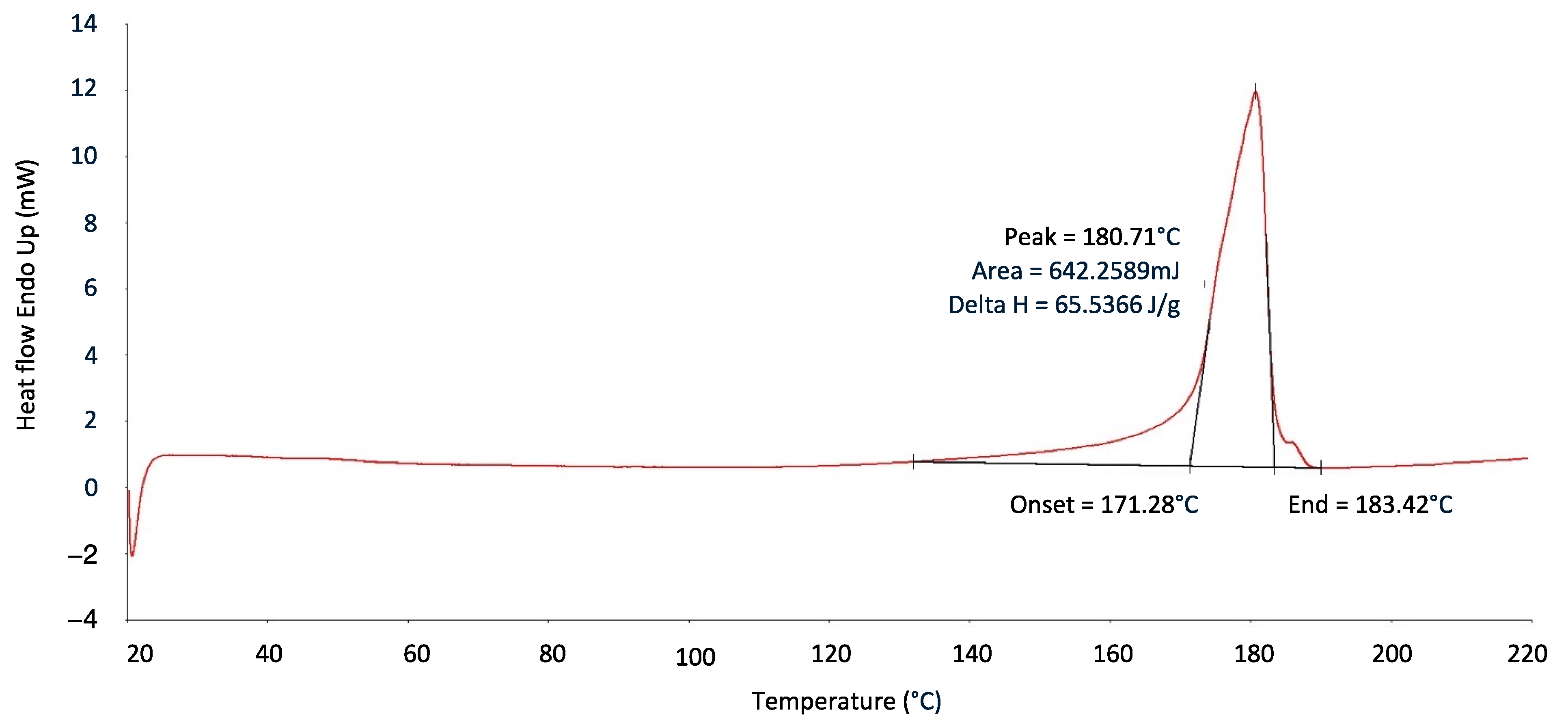
22], identified two peaks in the DSC traces, corresponding to the peaks of the raw powder and SEM images showing unmelted or partially melted particles.
The results presented in
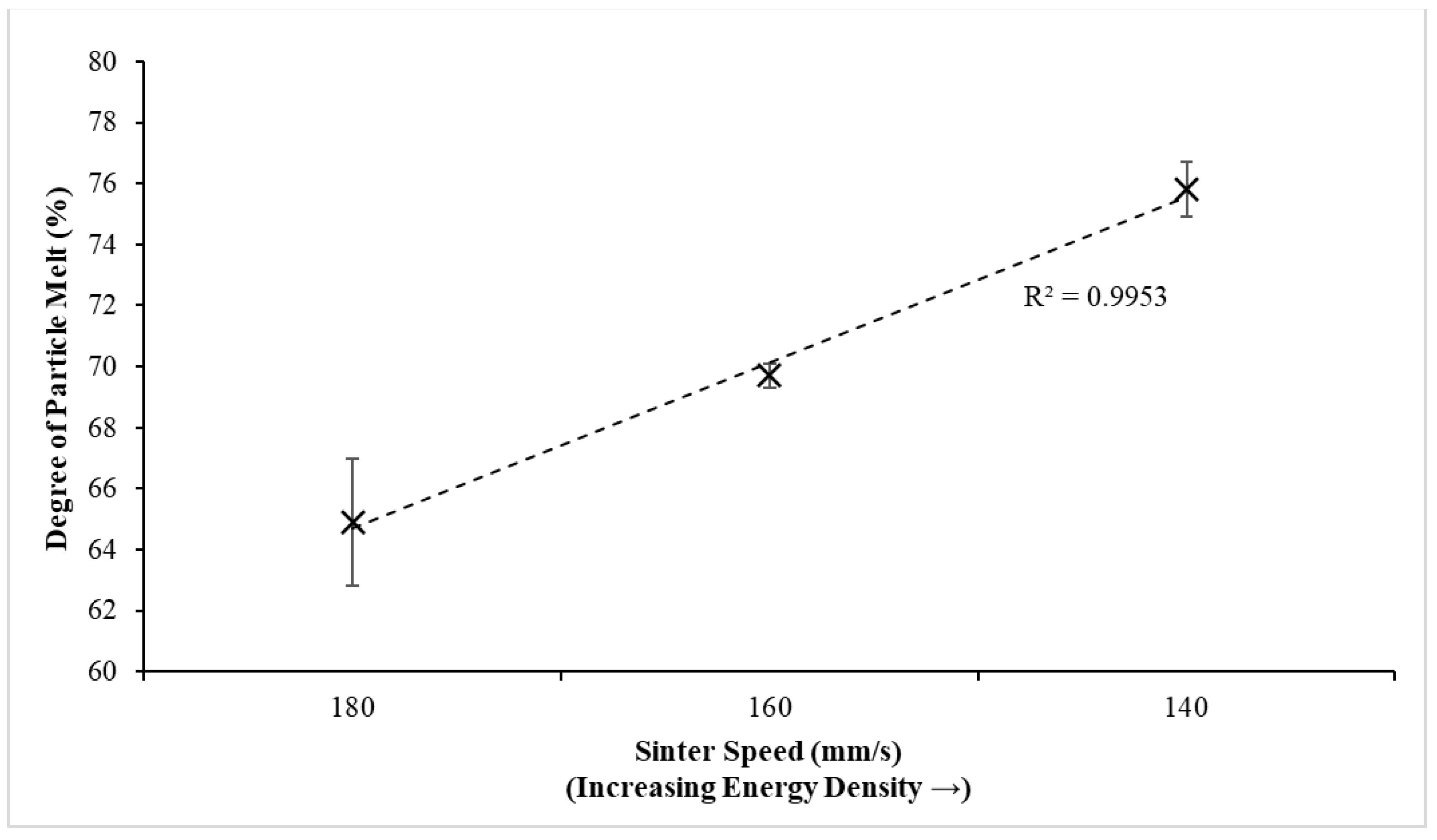
Table 8 indicate a linear increase in the mechanical properties as energy density increases. This is to be expected based on the existing literature relating to polymer powder bed fusion; early research into the laser sintering process [
23] demonstrated increases in the tensile properties with increasing energy densities. Further research [
24] showed similar effects, also identifying that the strength and modulus approach a maximum as the energy input exceeds the amount needed to fully melt the applied powder.
More recent research into the use of polyether block amide in the HSS process [
25] demonstrated an increase in the tensile properties with an increasing energy density, in this case induced via increases in the IR lamp power. In the work presented here, it can be seen that, as would be expected, an increase in the sinter speed, which corresponds to a decrease in the energy input, led to a subsequent decrease in the mechanical properties. While not the key focus of this work, it is worth noting that the significance of this effect would be dependent on the specific application of the parts and that the increase in the sinter speed would also lead to a decrease in the build time. For example, assuming the sinter stroke ~40% of the total layer time, an increase of 10% in the sinter speed would lead to a decrease in the build time of 9.1% following warm-up.
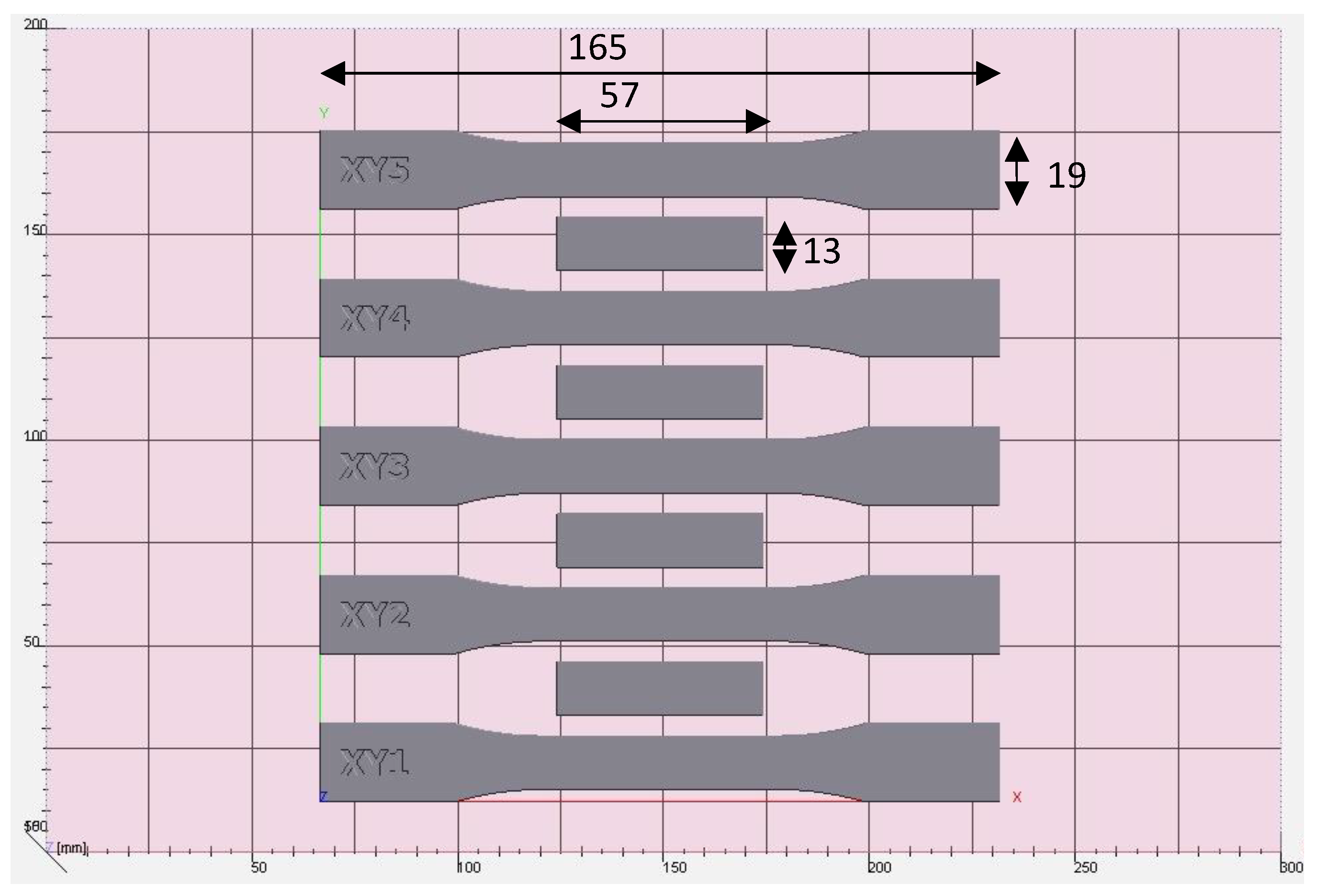
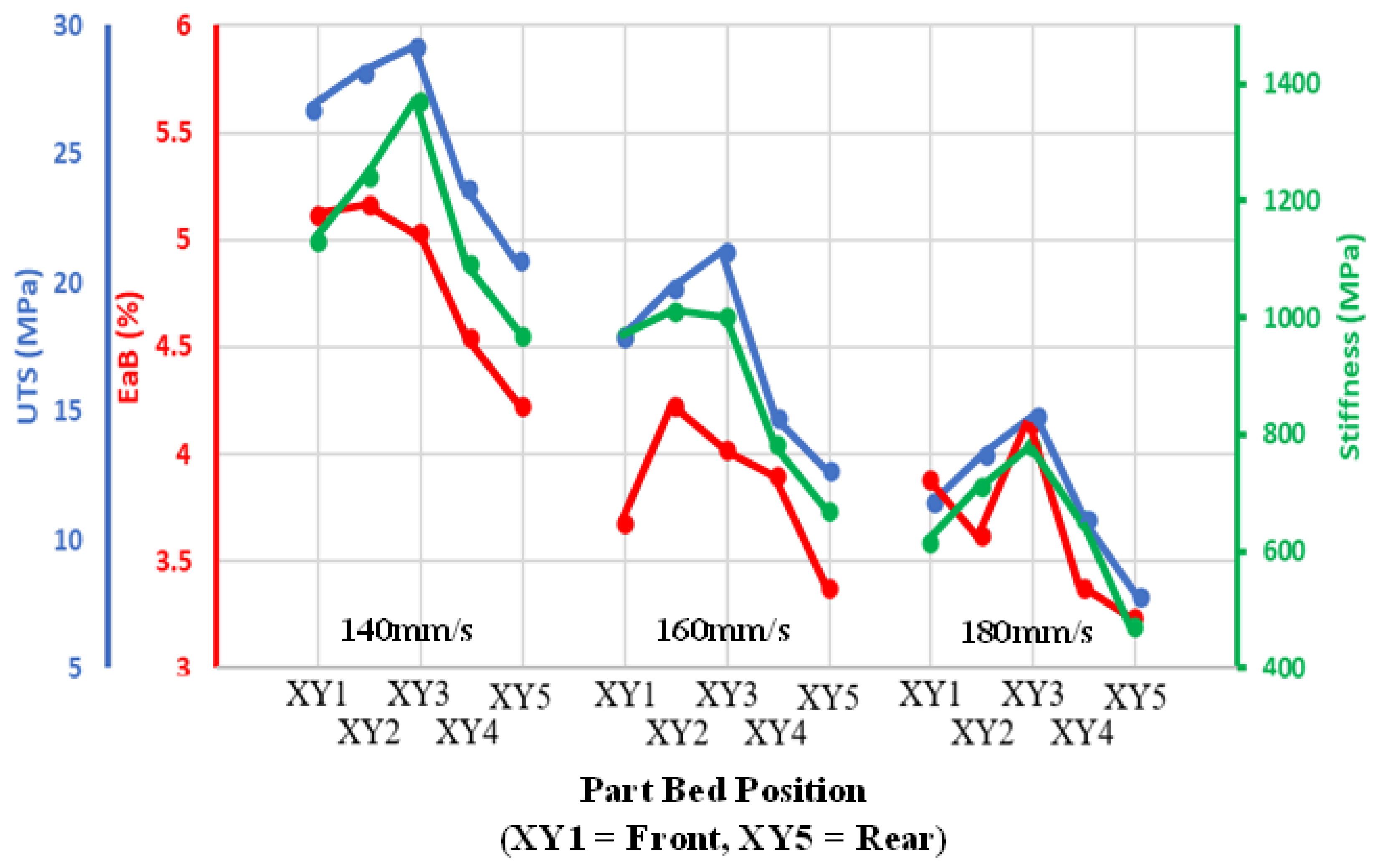
A closer inspection of the results identified a significant variation in the mechanical properties based on a part’s position within the build volume, with centrally located parts outperforming those closer to the edge (
Figure 10). Whilst variations in the data are exaggerated compared to what would be expected in a commercial setting (due to the fact that the HSS machine used was a prototype version of a now commercialised machine), this is a well-known phenomenon in PBF caused by variations in the part bed temperature, and it highlights the importance of identifying mechanical properties at as many points as possible throughout the build volume in order to predict part performance with confidence.
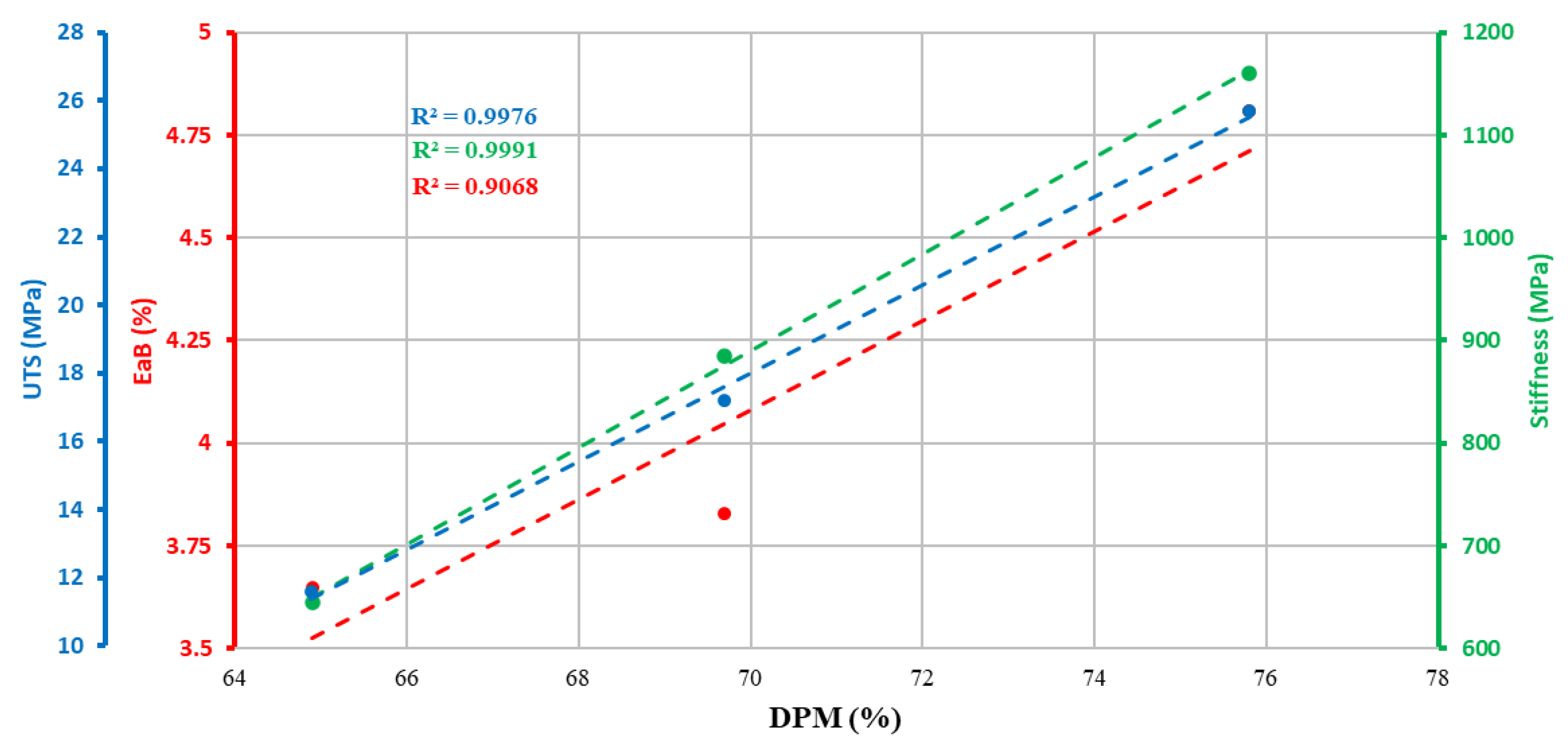
As noted in
Section 3, the tensile properties showed strong correlations with the DPM. This matches existing LS research [
16], whereby increases in strength and stiffness are seen despite the general appreciation that lower crystallinities (higher DPMs) should result in lower part strength and stiffnesses [
15]. Whilst both UTS and YM demonstrate an incredibly strong correlation with the DPM (R
2 values of 0.998 and 0.999, respectively), the EaB shows a weaker (although still >0.9) correlation. This is likely due to the brittle nature of the parts manufactured, with small changes in ductility affecting the average results more significantly. It has also been noted in the literature [
26] that samples with less favourable microstructure and crystallinity may also contain higher levels of porosity, exacerbating the effects on the mechanical properties and, in particular, the elongation at break. Research into SLS PA12 [
27] identified two melting peaks in samples produced at 0.096 J/mm
3 energy density, transitioning to a single peak at 0.270 J/mm
3, with an XRD analysis showing that higher proportions of the α crystal form at lower LS energy densities, suggesting a less effective melting of the PA12 particles.
Whilst the work presented in this paper focuses on PA12, there is reason to believe that this may extend to other materials; for example, the research in [
28] identified residual powder melt peaks at low energy densities in PA11 for SLS. The evaluation of polyethylene terephthalate in the HSS process [
29] identified lower properties for HSS than for SLS, due to unmelted areas within the HSS parts. This was thought to be a result of an insufficient energy input, in this case due to the lower temperature achievable in the machine.
We also expect that this work could be translated to other similar processes. Investigations into the MJF process [
30] identified the presence of powder particles or unsintered particle cores in the interior of the parts, as opposed to LS, in which these were almost entirely melted. Anther research [
31] produced MJF parts without these DSC shoulders, perhaps as a result of increased melting and possibly due to the thermal fusing agent used. Research into carbon black/PA12 composites in SLS [
32] also demonstrated multiple DSC peaks, disappearing as the energy density increased, noting the effect of carbon black causing differences in heat transmission, which may be relevant when comparing processes such as HSS/MJF with the SLS process.
Having established that both the DPM and the mechanical properties are proportional to the energy density applied to the parts in a given HSS build, it follows that, provided that the samples used for DPM analysis are close enough to the parts of interest, the DPM should be able to predict the performance of those parts. For example, when producing multiple parts throughout a build volume (e.g., manufacturing high numbers of small connectors), DPM samples can be included throughout the build volume to give an indication of potential variations in the mechanical properties of the parts. The same could be applied when producing smaller numbers of large parts, in particular in proximity to areas of the part where the mechanical properties are critical.
5. Conclusions
The research presented here identified sources of variability in established methods for processing raw DSC data to determine DPM values, including the calculation of MCM and core crystallinities, before proposing an alternative method with improved processing standardisation. Using this method, the DPM of sacrificial parts was found to correlate strongly with the mechanical properties of adjacent tensile samples (R-Squared values of 0.9976, 0.9991, and 0.9068 for ultimate tensile strength, Young’s modulus, and elongation at break, respectively) when sinter speed was used to alter the energy density.
These results confirm that the approach previously established for laser sintering can also be expanded to the high-speed sintering process, with practical implications for quality control/assessment. Users of high-speed sintering systems may be able to confirm the quality and repeatability of the parts they produce through the inclusion of small, sacrificial samples positioned close to the main parts, to be subjected to DSC analysis.
Further work in this area should include the expansion of the range of energy densities tested by varying other key HSS parameters such as the part bed temperature and the lamp power, including combinations of these parameters, to confirm that the DPM is linked to the overall energy density imparted during the HSS process and not to these individual parameters. An expanded build containing multiple smaller DPM samples and tensile samples throughout should also be tested to determine the repeatability of the proposed DPM processing method, in addition to identifying the accuracy and reliability of mechanical property prediction. The effect of the proximity of any sacrificial DSC samples to parts whose properties require prediction should also be considered, as the increased separation of adjacent parts will improve powder removal. Finally, expansion to other materials should be considered to improve the overall usefulness of the proposed method in a commercial setting.