Suitable Model for Rehydration of Dried Red Beets: Effect of Solid-to-Liquid Ratio on Rehydration Characteristics
Abstract
1. Introduction
- To determine the effect of the solid-to-liquid ratio on rehydration characteristics of dried red beets (mass gain, dry matter loss, water gain, moisture content increase, and volume increase);
- To fit the experimental rehydration data achieved to the Peleg model widely applied to describe rehydration kinetics of food products;
- To investigate the effect of the solid-to-liquid ratio on the Peleg model parameters which have physical meanings and helped explain the rehydration process.
2. Materials and Methods
- Mass determination
- Dry matter determination
- Mass of water determination
- Moisture content determination
- Moisture content M (in dry basis) was calculated applying Equation (2):
- Volume determination
3. Results and Discussion
- Constant A1 for mass gain, water gain, moisture content increase, and volume increase was described using Equation (5):
- Constant A1 for fry matter loss was determined with Equation (6):
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Solid-to-Liquid Ratio | Rehydration Liquid | Applications |
|---|---|---|
| 1:333 | distilled water | pumpkin [11] |
| 1:200 | distilled water | bananas [27], broccoli [44], and pumpkin [21] |
| 1:125 | distilled water | kiwi [37] |
| 1:120 | water | carrots [45] |
| 1:100 | distilled water | celery [46], kiwi [37], and mangoes [47] |
| 1:75 | distilled water | kiwi [37] |
| 1:50 | distilled water | apples [31], jackfruit [48], kiwi [37], parsley [31], and red bell pepper [49] |
| 1:40 | distilled water | apples [19] and hawthorn fruit [50] |
| 1:30 | distilled water | apples [31,32], oranges [20], parsley [31], potatoes [35], red pepper [25], and wormwood [51] |
| water | lablab bean seeds [28] and mangoes [52] | |
| 1:25 | distilled water | date palm [10], kiwi [37], potatoes [40] |
| water | carrots [33,34], and coriander leaves [53] | |
| 1:20 | distilled water | apples [31,54,55], broccoli [56], and parsley [31] |
| water | potatoes [9] | |
| 0.5% solution of citric acid, apple, juice | apples [54] | |
| 1:15 | distilled water | amaranth grain [57] and okra [58] |
| SO2 aqueous solution (0.01% and 0.02% (w/v)) SO2 solution (0.02% w/v) with variable concentrations of lactic acid (0.0025% and 0.0050% (v/v)) | amaranth grain [57] | |
| 1:14 | distilled water | pear [59] |
| 1:10 | tap water | apples [31], carrots [60], and parsley [31] |
| 1:7.5 | distilled water | sesame seeds [61] |
| 1:5 | distilled water and vegetable stock | potatoes [62] |
| 1:1.125, 1:0.512 | drinking water | pork tenderloins [63] |
References
- Chen, S.; Wu, W.; Yang, Y.; Wang, H.; Zhang, H. Experimental Study of a Novel Vacuum Sublimation–Rehydration Thawing for Frozen Pork. Int. J. Refrig. 2020, 118, 392–402. [Google Scholar] [CrossRef]
- Nejatdarabi, S.; Mohebbi, M. Predicting the Rehydration Process of Mushroom Powder by Multiple Linear Regression (MLR) and Artificial Neural Network (ANN) in Different Rehydration Medium. J. Food Meas. Charact. 2023, 17, 1962–1973. [Google Scholar] [CrossRef]
- Ratti, C.; Kudra, T. Drying of Foamed Biological Materials: Opportunities and Challenges. Dry. Technol. 2006, 24, 1101–1108. [Google Scholar] [CrossRef]
- Khan, M.I.H.; Farrell, T.; Nagy, S.A.; Karim, M.A. Fundamental Understanding of Cellular Water Transport Process in Bio-Food Material during Drying. Sci. Rep. 2018, 8, 15191. [Google Scholar] [CrossRef] [PubMed]
- Abbaspour-Gilandeh, Y.; Kaveh, M.; Fatemi, H.; Khalife, E.; Witrowa-Rajchert, D.; Nowacka, M. Effect of Pretreatments on Convective and Infrared Drying Kinetics, Energy Consumption and Quality of Terebinth. Appl. Sci. 2021, 11, 7672. [Google Scholar] [CrossRef]
- Biswas, R.; Hossain, M.A.; Zzaman, W. Thin Layer Modeling of Drying Kinetics, Rehydration Kinetics and Color Changes of Osmotic Pre-Treated Pineapple (Ananas comosus) Slices during Drying: Development of a Mechanistic Model for Mass Transfer. Innov. Food Sci. Emerg. Technol. 2022, 80, 103094. [Google Scholar] [CrossRef]
- Wang, B.; Li, Y.; Lv, Y.; Jiao, X.; Wang, Z.; He, Y.; Wen, L. Dehydration–Rehydration Mechanism of Vegetables at the Cell-Wall and Cell-Membrane Levels and Future Research Challenges. Crit. Rev. Food Sci. Nutr. 2023, 1–17. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Zhao, F.; Li, C.; Ban, X.; Gu, Z.; Li, Z. Acceleration Mechanism of the Rehydration Process of Dried Rice Noodles by the Porous Structure. Food Chem. 2024, 431, 137050. [Google Scholar] [CrossRef] [PubMed]
- Ravindra, M.R.; Chattopadhyay, P.K. Optimisation of Osmotic Preconcentration and Fluidised Bed Drying to Produce Dehydrated Quick-Cooking Potato Cubes. J. Food Eng. 2000, 44, 5–11. [Google Scholar] [CrossRef]
- Falade, K.O.; Abbo, E.S. Air-Drying and Rehydration Characteristics of Date Palm (Phoenix dactylifera L.) Fruits. J. Food Eng. 2007, 79, 724–730. [Google Scholar] [CrossRef]
- Rojas, M.L.; Augusto, P.E.D. Microstructure Elements Affect the Mass Transfer in Foods: The Case of Convective Drying and Rehydration of Pumpkin. LWT 2018, 93, 102–108. [Google Scholar] [CrossRef]
- Janiszewska, E. Microencapsulated Beetroot Juice as a Potential Source of Betalain. Powder Technol. 2014, 264, 190–196. [Google Scholar] [CrossRef]
- Kumar, Y.; Khan, M.A.; Patel, K.K. Effect of Microwave on Fluidized Bed Drying of Beetroot (Beta vulgaris L.). Am. J. Eng. Res. 2014, 3, 267–273. [Google Scholar]
- Latorre, M.E.; De Escalada Plá, M.F.; Rojas, A.M.; Gerschenson, L.N. Blanching of Red Beet (Beta vulgaris L. Var. Conditiva) Root. Effect of Hot Water or Microwave Radiation on Cell Wall Characteristics. LWT-Food Sci. Technol. 2013, 50, 193–203. [Google Scholar] [CrossRef]
- Kaleta, A.; Górnicki, K. Some Remarks on Evaluation of Drying Models of Red Beet Particles. Energy Convers. Manag. 2010, 51, 2967–2978. [Google Scholar] [CrossRef]
- Horwitz, W. (Ed.) Official Methods of Analysis of AOAC International, 18th ed.; AOAC International: Gaithersburg, MD, USA, 2005; ISBN 978-0-935584-75-2. [Google Scholar]
- Mazza, G. Dehydration of Carrots.: Effects of Pre-Drying Treatments on Moisture Transport and Product Quality. Int. J. Food Sci. Technol. 2007, 18, 113–123. [Google Scholar] [CrossRef]
- Peleg, M. An Empirical Model for the Description of Moisture Sorption Curves. J. Food Sci. 1988, 53, 1216–1219. [Google Scholar] [CrossRef]
- Tepe, T.K.; Tepe, B. The Comparison of Drying and Rehydration Characteristics of Intermittent-Microwave and Hot-Air Dried-Apple Slices. Heat Mass Transf. 2020, 56, 3047–3057. [Google Scholar] [CrossRef]
- Özkan-Karabacak, A.; Acoğlu, B.; Yolci Ömeroğlu, P.; Çopur, Ö.U. Microwave Pre-treatment for Vacuum Drying of Orange Slices: Drying Characteristics, Rehydration Capacity and Quality Properties. J. Food Process Eng. 2020, 43, e13511. [Google Scholar] [CrossRef]
- Benseddik, A.; Azzi, A.; Zidoune, M.N.; Khanniche, R.; Besombes, C. Empirical and Diffusion Models of Rehydration Process of Differently Dried Pumpkin Slices. J. Saudi Soc. Agric. Sci. 2019, 18, 401–410. [Google Scholar] [CrossRef]
- Nayi, P.; Kumar, N.; Kachchadiya, S.; Chen, H.; Singh, P.; Shrestha, P.; Pandiselvam, R. Rehydration Modeling and Characterization of Dehydrated Sweet Corn. Food Sci. Nutr. 2023, 11, 3224–3234. [Google Scholar] [CrossRef] [PubMed]
- Lopez-Quiroga, E.; Prosapio, V.; Fryer, P.J.; Norton, I.T.; Bakalis, S. Model Discrimination for Drying and Rehydration Kinetics of Freeze-dried Tomatoes. J. Food Process Eng. 2020, 43, e13192. [Google Scholar] [CrossRef]
- Demir, H.; Demir, H.; Lončar, B.; Nićetin, M.; Pezo, L.; Yilmaz, F. Artificial Neural Network and Kinetic Modeling of Capers during Dehydration and Rehydration Processes. J. Food Process Eng. 2023, 46, e14249. [Google Scholar] [CrossRef]
- Demiray, E.; Tulek, Y. Effect of Temperature on Water Diffusion during Rehydration of Sun-Dried Red Pepper (Capsicum annuum L.). Heat Mass Transf. 2017, 53, 1829–1834. [Google Scholar] [CrossRef]
- García-Pascual, P.; Sanjuán, N.; Melis, R.; Mulet, A. Morchella esculenta (Morel) Rehydration Process Modelling. J. Food Eng. 2006, 72, 346–353. [Google Scholar] [CrossRef]
- Maskan, M. Drying, Shrinkage and Rehydration Characteristics of Kiwifruits during Hot Air and Microwave Drying. J. Food Eng. 2001, 48, 177–182. [Google Scholar] [CrossRef]
- Pervin, S.; Islam, M.; Islam, M. Study on Rehydration Characteristics of Dried Lablab Bean (Lablab purpureus) Seeds. J. Agric. Rural Dev. 2008, 6, 157–163. [Google Scholar] [CrossRef]
- Maté, J.I.; Zwietering, M.; Van’T Riet, K. The Effect of Blanching on the Mechanical and Rehydration Properties of Dried Potato Slices. Eur. Food Res. Technol. 1999, 209, 343–347. [Google Scholar] [CrossRef]
- Sopade, P.A.; Xun, P.Y.; Halley, P.J.; Hardin, M. Equivalence of the Peleg, Pilosof and Singh–Kulshrestha Models for Water Absorption in Food. J. Food Eng. 2007, 78, 730–734. [Google Scholar] [CrossRef]
- Górnicki, K. Modelling of Selected Vegetables and Fruits Rehydration Process; Wydawnictwo SGGW: Warsaw, Poland, 2011. (In Polish) [Google Scholar]
- Taiwo, K.A.; Angersbach, A.; Knorr, D. Rehydration Studies on Pretreated and Osmotically Dehydrated Apple Slices. J. Food Sci. 2002, 67, 842–847. [Google Scholar] [CrossRef]
- Rastogi, N.K.; Nayak, C.A.; Raghavarao, K.S.M.S. Influence of Osmotic Pre-Treatments on Rehydration Characteristics of Carrots. J. Food Eng. 2004, 65, 287–292. [Google Scholar] [CrossRef]
- Nayak, C.A.; Suguna, K.; Rastogi, N.K. Combined Effect of Gamma-Irradiation and Osmotic Treatment on Mass Transfer during Rehydration of Carrots. J. Food Eng. 2006, 74, 134–142. [Google Scholar] [CrossRef]
- McMinn, W.A.M.; Magee, T.R.A. Physical Characteristics of Dehydrated Potatoes—Part II. J. Food Eng. 1997, 33, 49–55. [Google Scholar] [CrossRef]
- Mujaffar, S.; Lee Loy, A. The Rehydration Behavior of Microwave-dried Amaranth (Amaranthus dubius) Leaves. Food Sci. Nutr. 2017, 5, 399–406. [Google Scholar] [CrossRef]
- Ergün, K.; Çalışkan, G.; Dirim, S.N. Determination of the Drying and Rehydration Kinetics of Freeze Dried Kiwi (Actinidia deliciosa) Slices. Heat Mass Transf. 2016, 52, 2697–2705. [Google Scholar] [CrossRef]
- Moreira, R.; Chenlo, F.; Chaguri, L.; Fernandes, C. Water Absorption, Texture, and Color Kinetics of Air-Dried Chestnuts during Rehydration. J. Food Eng. 2008, 86, 584–594. [Google Scholar] [CrossRef]
- Vetter, S.; Kunzek, H. The Influence of Suspension Solution Conditions on the Rehydration of Apple Cell Wall Material. Eur. Food Res. Technol. 2003, 216, 39–45. [Google Scholar] [CrossRef]
- Markowski, M.; Bondaruk, J.; Błaszczak, W. Rehydration Behavior of Vacuum-Microwave-Dried Potato Cubes. Dry. Technol. 2009, 27, 296–305. [Google Scholar] [CrossRef]
- Solomon, W.K. Hydration Kinetics of Lupin (Lupinus albus) Seeds. J. Food Process Eng. 2007, 30, 119–130. [Google Scholar] [CrossRef]
- Noshad, M.; Mohebbi, M.; Shahidi, F.; Mortazavi, S.A. Kinetic Modeling of Rehydration in Air-Dried Quinces Pretreated with Osmotic Dehydration and Ultrasonic: Rehydration in Dried Quinces. J. Food Process. Preserv. 2012, 36, 383–392. [Google Scholar] [CrossRef]
- Ohaco, E.H.; Ichiyama, B.; Lozano, J.E.; De Michelis, A. Rehydration of Rosa rubiginosa Fruits Dried with Hot Air. Dry. Technol. 2015, 33, 696–703. [Google Scholar] [CrossRef]
- Doymaz, İ.; Sahin, M. Effect of Temperature and Pre-Treatment on Drying and Rehydration Characteristics of Broccoli Slices. J. Food Meas. Charact. 2016, 10, 364–373. [Google Scholar] [CrossRef]
- Doymaz, İ. Drying Kinetics, Rehydration and Colour Characteristics of Convective Hot-Air Drying of Carrot Slices. Heat Mass Transf. Waerme-Stoffuebertragung 2017, 53, 25–35. [Google Scholar] [CrossRef]
- Nowak, D.; Piechucka, P.; Witrowa-Rajchert, D.; Wiktor, A. Impact of Material Structure on the Course of Freezing and Freeze-Drying and on the Properties of Dried Substance, as Exemplified by Celery. J. Food Eng. 2016, 180, 22–28. [Google Scholar] [CrossRef]
- Link, J.V.; Tribuzi, G.; Laurindo, J.B. Improving Quality of Dried Fruits: A Comparison between Conductive Multi-Flash and Traditional Drying Methods. LWT-Food Sci. Technol. 2017, 84, 717–725. [Google Scholar] [CrossRef]
- Kaushal, P.; Sharma, H.K. Osmo-Convective Dehydration Kinetics of Jackfruit (Artocarpus heterophyllus). J. Saudi Soc. Agric. Sci. 2016, 15, 118–126. [Google Scholar] [CrossRef]
- Vega-Gálvez, A.; Lemus-Mondaca, R.; Bilbao-Sáinz, C.; Fito, P.; Andrés, A. Effect of Air Drying Temperature on the Quality of Rehydrated Dried Red Bell Pepper (Var. Lamuyo). J. Food Eng. 2008, 85, 42–50. [Google Scholar] [CrossRef]
- Aral, S.; Bese, A.V. Convective Drying of Hawthorn Fruit (Crataegus Spp.): Effect of Experimental Parameters on Drying Kinetics, Color, Shrinkage, and Rehydration Capacity. Food Chem. 2016, 210, 577–584. [Google Scholar] [CrossRef] [PubMed]
- Beigi, M. Thin Layer Drying of Wormwood (Artemisia absinthium L.) Leaves: Dehydration Characteristics, Rehydration Capacity and Energy Consumption. Heat Mass Transf. 2017, 53, 2711–2718. [Google Scholar] [CrossRef]
- Maldonado, S.; Arnau, E.; Bertuzzi, M.A. Effect of Temperature and Pretreatment on Water Diffusion during Rehydration of Dehydrated Mangoes. J. Food Eng. 2010, 96, 333–341. [Google Scholar] [CrossRef]
- Kaur, P.; Kumar, A.; Arora, S.; Singh Ghuman, B. Quality of Dried Coriander Leaves as Affected by Pretreatments and Method of Drying. Eur. Food Res. Technol. 2006, 223, 189–194. [Google Scholar] [CrossRef]
- Winiczenko, R.; Górnicki, K.; Kaleta, A.; Janaszek-Mańkowska, M.; Choińska, A.; Trajer, J. Apple Cubes Drying and Rehydration. Multiobjective Optimization of the Processes. Sustainability 2018, 10, 4126. [Google Scholar] [CrossRef]
- Górnicki, K.; Choińska, A.; Kaleta, A. Effect of Variety on Rehydration Characteristics of Dried Apples. Processes 2020, 8, 1454. [Google Scholar] [CrossRef]
- Femenia, A.; Bestard, M.J.; Sanjuan, N.; Rosselló, C.; Mulet, A. Effect of Rehydration Temperature on the Cell Wall Components of Broccoli (Brassica oleracea L. Var. Italica) Plant Tissues. J. Food Eng. 2000, 46, 157–163. [Google Scholar] [CrossRef]
- Resio, A.C.; Aguerre, R.J.; Suarez, C. Hydration Kinetics of Amaranth Grain. J. Food Eng. 2006, 72, 247–253. [Google Scholar] [CrossRef]
- Kocabay, Ö.G.; İsmail, O. Investigation of Rehydration Kinetics of Open-Sun Dried Okra Samples. Heat Mass Transf. 2017, 53, 2155–2163. [Google Scholar] [CrossRef]
- Komes, D.; Lovrić, T.; Kovačević Ganić, K. Aroma of Dehydrated Pear Products. LWT-Food Sci. Technol. 2007, 40, 1578–1586. [Google Scholar] [CrossRef]
- Marabi, A.; Thieme, U.; Jacobson, M.; Saguy, I.S. Influence of Drying Method and Rehydration Time on Sensory Evaluation of Rehydrated Carrot Particulates. J. Food Eng. 2006, 72, 211–217. [Google Scholar] [CrossRef]
- Khazaei, J.; Mohammadi, N. Effect of Temperature on Hydration Kinetics of Sesame Seeds (Sesamum indicum L.). J. Food Eng. 2009, 91, 542–552. [Google Scholar] [CrossRef]
- Severini, C.; Baiano, A.; De Pilli, T.; Carbone, B.F.; Derossi, A. Combined Treatments of Blanching and Dehydration: Study on Potato Cubes. J. Food Eng. 2005, 68, 289–296. [Google Scholar] [CrossRef]
- Chen, S.; Wu, W.; Mao, S.; Li, K.; Zhang, H. Optimization of a Novel Vacuum Sublimation–Rehydration Thawing Process. J. Food Sci. 2023, 88, 259–272. [Google Scholar] [CrossRef] [PubMed]
 ), 1:15 (
), 1:15 ( ), 1:10 (
), 1:10 ( ), 1:5, (
), 1:5, ( ), 1:3 (
), 1:3 ( ), and 1:1 (
), and 1:1 ( ).
).
 ), 1:15 (
), 1:15 ( ), 1:10 (
), 1:10 (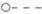 ), 1:5, (
), 1:5, (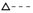 ), 1:3 (
), 1:3 (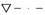 ), and 1:1 (
), and 1:1 (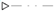 ).
).
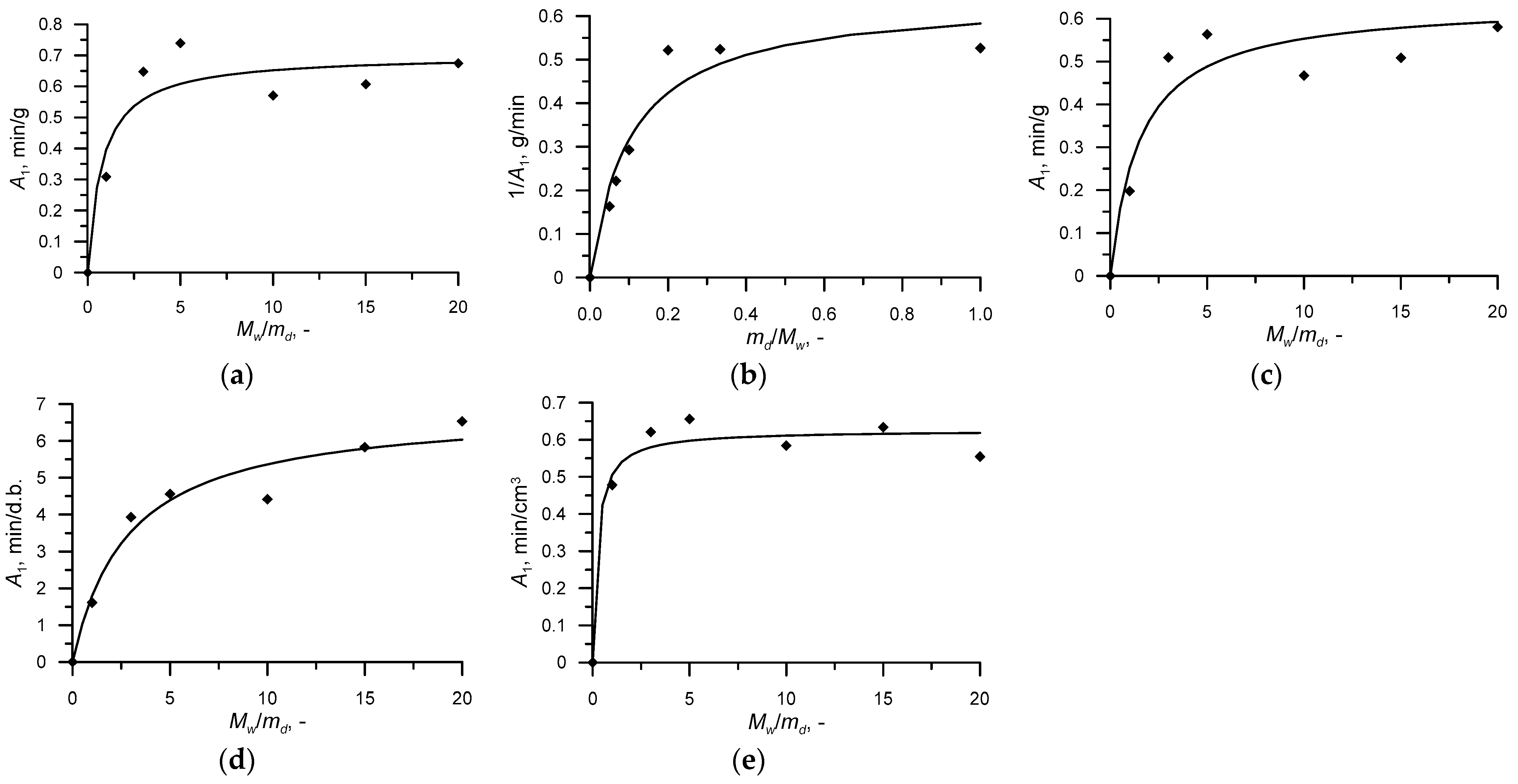
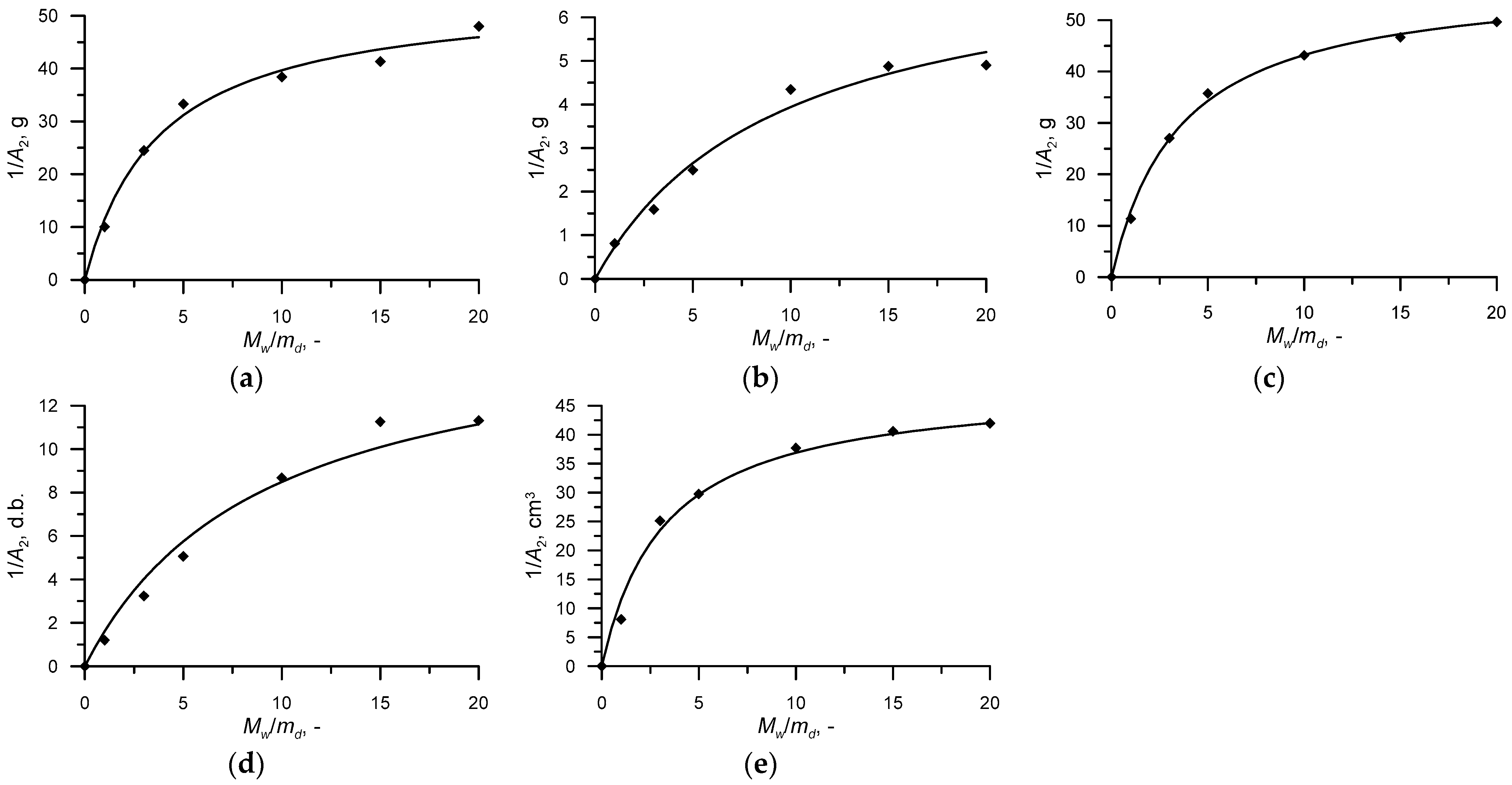
| Solid-to-Liquid Ratio | Statistical Criterion | Mass Gain | Dry Matter Loss | Water Gain | Moisture Content Increase | Volume Increase |
|---|---|---|---|---|---|---|
| 1:20 | R2 | 0.977841 | 0.990122 | 0.980034 | 0.986391 | 0.978582 |
| RMSE | 2.761468 | 0.160082 | 2.801446 | 0.494571 | 1.985095 | |
| 1:15 | R2 | 0.976974 | 0.988429 | 0.978578 | 0.979364 | 0.983792 |
| RMSE | 1.986113 | 0.167964 | 2.180706 | 0.480982 | 1.641184 | |
| 1:10 | R2 | 0.989993 | 0.991571 | 0.991465 | 0.993521 | 0.979681 |
| RMSE | 1.218165 | 0.127637 | 1.293332 | 0.216352 | 1.715386 | |
| 1:5 | R2 | 0.973097 | 0.934743 | 0.978194 | 0.993253 | 0.985869 |
| RMSE | 1.728302 | 0.213659 | 1.692072 | 0.131513 | 1.157729 | |
| 1:3 | R2 | 0.97692 | 0.72837 | 0.981604 | 0.988882 | 0.957971 |
| RMSE | 1.281404 | 0.296452 | 1.184785 | 0.109865 | 1.676851 | |
| 1:1 | R2 | 0.993796 | 0.708077 | 0.994371 | 0.993351 | 0.950684 |
| RMSE | 0.261170 | 0.15826 | 0.366403 | 0.035141 | 0.704667 |
| Solid-to-Liquid Ratio | Parameter | Mass Gain (1) | Dry Matter Loss (2) | Water Gain (3) | Moisture Content Increase (4) | Volume Increase (5) |
|---|---|---|---|---|---|---|
| 1:20 | A1 | 0.674 | 6.125 | 0.581 | 6.533 | 0.555 |
| A2 | 0.021 | 0.204 | 0.019 | 0.080 | 0.024 | |
| 1:15 | A1 | 0.607 | 4.512 | 0.509 | 5.834 | 0.634 |
| A2 | 0.024 | 0.205 | 0.021 | 0.089 | 0.025 | |
| 1:10 | A1 | 0.570 | 3.415 | 0.467 | 4.418 | 0.584 |
| A2 | 0.026 | 0.230 | 0.023 | 0.115 | 0.027 | |
| 1:5 | A1 | 0.739 | 1.917 | 0.563 | 4.558 | 0.656 |
| A2 | 0.030 | 0.401 | 0.028 | 0.198 | 0.034 | |
| 1:3 | A1 | 0.647 | 1.910 | 0.510 | 3.930 | 0.621 |
| A2 | 0.041 | 0.629 | 0.037 | 0.309 | 0.040 | |
| 1:1 | A1 | 0.309 | 1.900 | 0.198 | 1.613 | 0.478 |
| A2 | 0.100 | 1.235 | 0.088 | 0.833 | 0.124 |
| Parameter | Constant | Mass Gain | Dry Matter Loss | Water Gain | Moisture Content Increase | Volume Increase |
|---|---|---|---|---|---|---|
| A1 | a1 | 1.10290 | 0.16104 | 2.40345 | 0.41313 | 0.38017 |
| a2 | 1.42394 | 1.55455 | 1.56684 | 0.14512 | 1.59827 | |
| A2 | a1 | 0.011832 | 1.23212 | 0.06008 | 0.56068 | 0.06615 |
| a2 | 0.06886 | 0.13049 | 0.011713 | 0.06174 | 0.02050 |
| Parameter | Statistical Criterion | Mass Gain | Dry Matter Loss | Water Gain | Moisture Content Increase | Volume Increase |
|---|---|---|---|---|---|---|
| A1 | R2 | 0.8957 | 0.9350 | 0.9040 | 0.9571 | 0.9654 |
| RMSE | 0.0002 | 4 × 10−5 | 0.0001 | 0.2320 | 2 × 10−5 | |
| A2 | R2 | 0.9904 | 0.9845 | 0.9977 | 0.9860 | 0.9920 |
| RMSE | 39.3976 | 0.0182 | 3.4162 | 0.9160 | 29.7406 |
| Variable | Extreme Solid-to-Liquid Ratio | A1 | A2 |
|---|---|---|---|
| Mass grain | Mw (1)/md (2)→∞ | 1.4239 | 0.0183 1/g |
| Dry matter loss | md/Mw→∞ | 1.5546 | - |
| Mw/md→∞ | - | 0.1305 1/g | |
| Water gain | Mw/md→∞ | 0.6382 | 0.0171 1/g |
| Moisture content increase | Mw/md→∞ | 6.8909 | 0.0617 1/d.b. |
| Volume increase | Mw/md→∞ | 0.6257 | 0.0205 1/cm3 |
| Solid-to-Liquid Ratio | Statistical Criterion | Mass Gain | Dry Matter Loss | Water Gain | Moisture Content Increase | Volume Increase |
|---|---|---|---|---|---|---|
| 1:20 | R2 | 0.9775 | 0.9846 | 0.9794 | 0.9822 | 0.9800 |
| RMSE | 2.2519 | 0.3707 | 2.3039 | 0.4363 | 2.1207 | |
| 1:15 | R2 | 0.9773 | 0.9849 | 0.9796 | 0.9770 | 0.9837 |
| RMSE | 2.1673 | 0.1876 | 2.3298 | 0.6443 | 1.6507 | |
| 1:10 | R2 | 0.9895 | 0.9881 | 0.9913 | 0.9923 | 0.9797 |
| RMSE | 1.4871 | 0.2557 | 1.7627 | 0.4141 | 1.8390 | |
| 1:5 | R2 | 0.9662 | 0.9131 | 0.9733 | 0.9929 | 0.9847 |
| RMSE | 1.9451 | 0.2526 | 1.8239 | 0.4098 | 1.3127 | |
| 1:3 | R2 | 0.9717 | 0.7042 | 0.9765 | 0.9878 | 0.9589 |
| RMSE | 1.3763 | 0.3616 | 1.3829 | 0.5338 | 1.6643 | |
| 1:1 | R2 | 0.9874 | 0.7253 | 0.9913 | 0.9887 | 0.9374 |
| RMSE | 1.0075 | 0.1681 | 1.2462 | 0.3295 | 2.6472 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaleta, A.; Górnicki, K.; Obranović, M.; Kosiorek, K. Suitable Model for Rehydration of Dried Red Beets: Effect of Solid-to-Liquid Ratio on Rehydration Characteristics. Appl. Sci. 2024, 14, 6132. https://doi.org/10.3390/app14146132
Kaleta A, Górnicki K, Obranović M, Kosiorek K. Suitable Model for Rehydration of Dried Red Beets: Effect of Solid-to-Liquid Ratio on Rehydration Characteristics. Applied Sciences. 2024; 14(14):6132. https://doi.org/10.3390/app14146132
Chicago/Turabian StyleKaleta, Agnieszka, Krzysztof Górnicki, Marko Obranović, and Krzysztof Kosiorek. 2024. "Suitable Model for Rehydration of Dried Red Beets: Effect of Solid-to-Liquid Ratio on Rehydration Characteristics" Applied Sciences 14, no. 14: 6132. https://doi.org/10.3390/app14146132
APA StyleKaleta, A., Górnicki, K., Obranović, M., & Kosiorek, K. (2024). Suitable Model for Rehydration of Dried Red Beets: Effect of Solid-to-Liquid Ratio on Rehydration Characteristics. Applied Sciences, 14(14), 6132. https://doi.org/10.3390/app14146132







