Multi-Frequency Fringe Projection Profilometry: Phase Error Suppression Based on Cycle Count Adjustment

Abstract

Fringe projection profilometry is one of the most widely used three-dimensional measurement techniques at present, in which phase is the key factor for the accuracy of dimensional measurements. Jumping errors may occur due to improper handling of truncation points in phase unwrapping. Meanwhile, projective dual-frequency grating has the shortcomings of a narrow measurement range and coarse projection fringe due to the requirements of an overlapping grid. To address the above problems, this paper puts forward an improved multi-frequency heterodyne phase unwrapping approach. Firstly, the phase principal values of three frequencies are obtained by the standard four-step phase-shifting approach, and two wrapped phases with lower frequencies are obtained through the dual-frequency heterodyne phase unwrapping approach. Then, the decimal part of the fringe order is again calculated using the dual-frequency heterodyne principle, and the actual value of the current decimal part is calculated from the phase principal values of the grating fringe corresponding to the fringe order. Then, a threshold is set according to the error of the phase principal value itself, and the differences between this threshold and the above calculated and theoretical values are compared. Finally, the absolute phase is corrected by adjusting the number of cycles according to the judgment results. Experiments show that the improved approach can achieve a correction rate of more than 96.8% for the jumping errors that occur in phase unwrapping, and it is also highly resistant to noise in the face of different noises. Furthermore, the approach can measure the three-dimensional morphology of objects with different surface morphologies, indicating the certain universality of the approach.

1. Introduction

As a representative structured light three-dimensional (3D) measurement technique, fringe projection profilometry [1,2,3] is widely applied in medical imaging, the industrial field, and online measurement due to its non-contact measurement, high precision, and low cost. Phase-shifting projected fringe profilometry mainly consists of four steps: projecting grating stripes with a certain phase difference onto the target, extracting the principal phase value of the target, unwrapping the phase, and 3D reconstruction. The phase-shifting approach is a common approach to extract the phase, but the acquired phase is wrapped in [−π, π], which is not unique at this time, so the wrapped phase must be unwrapped to obtain the full-field real phase field. Currently, approaches of unwrapping phases are classified in principle into the time domain and the space domain. Spatial phase unwrapping [4] can be completed with only one phase figure, but the phase unwrapping operation of each point is related to its surrounding adjacent points. Once the phase of one point is unwrapped incorrectly because of phase aliasing and phase noise, the error will be transmitted to the phase unwrapping process of all subsequent points, resulting in a reduction in measurement accuracy. On the other hand, temporal phase unwrapping refers to the phase unwrapping of the phase diagrams obtained for the same pixel point at different times to obtain the absolute phase. In temporal phase unwrapping, the phase of each point is only related to the point at the same position on the phase diagrams at different frequencies, not to its adjacent points, so even if the phase of one point is unwrapped incorrectly due to the phase mixing and phase noise, these errors are not transmitted. The multi-frequency heterodyne phase unwrapping approach, as a kind of temporal phase unwrapping approach, is widely used because of its wide applicability and high precision. In the heterodyne phase-solving approach, three grating fringes of different frequencies are used to obtain phase values at three frequencies by a three-step phase-shifting methodology. Then, the heterodyne operation is performed on the phase values of these three frequencies to obtain two heterodyne phase maps. These two heterodyne phase maps can be further processed to obtain a phase solution wrap. Finally, the absolute phase value is calculated by the phase solution parcel diagram.

However, the phase jump will occur after directly utilizing this methodology to solve the wrapped phase, so the results need to be corrected. Aiming at the jumping errors generated in unwrapping by the multi-frequency heterodyne methodology, Lei et al. [5] modified the principle of multi-frequency heterodyne to correct the unwrapping phase error, but this methodology is only limited to the dual-frequency heterodyne, and the phase errors generated by the multi-frequency heterodyne cannot be completely eliminated. Zhang et al. [6] extended the methodology in [5] and derived the methodology of the multi-frequency heterodyne phase solution, but the phase jumping error after using the solution cannot be completely avoided. Chen et al. [7] improved the methodology proposed in the literature [5], but there exist many constraints, and the grating fringe of the third frequency has a large pitch, which could easily lead to the loss of details and could not improve the measurement accuracy. Chen et al. [8] analyzed the neighborhood of the phase error points and corrected the errors. This methodology works better for objects with simple and gentle surfaces, but it ignores the independence of each pixel point during phase unwrapping, so it is not suitable for measuring objects with complex surfaces. Cheng et al. [9] proposed a phase unwrapping methodology for phase-shifting projected fringe profilometry that does not require additional projections to identify fringe orders. The pattern used for phase extraction can be directly used for phase unwrapping. By encoding the fringe pattern used in the binary-coded phase-shifting technique spatially, the fringe order can be identified unambiguously, even for spatially isolated objects or surfaces with large depth discontinuities. It can also distinguish fringe orders well even when the surface color or reflectivity varies periodically with the position. However, this methodology has high requirements for encoding images, and once there is a coding error, it will lead to a large error in the subsequent dephasing. Xu et al. [10] proposed a multi-frequency projection fringe profilometry for measuring large-depth discontinuous objects. A fringe pattern with multiple spatial frequencies is projected onto the target object. The phase map of the package at different spatial frequencies is obtained by phase shifting. By finding the peak value of the parcel phase index and the peaks of different spatial frequencies, the height of each point on the object surface can be obtained independently. Several measurements on real objects are given. However, the surface topographies of some complex surfaces cannot be completely measured. Li Y et al. [1] proposed a methodology based on a three-stream neural network to reduce motion error and introduced a general dynamic scene dataset establishment methodology to complete the 3D shape measurement of a virtual fringe projection system. The model is optimized with a large amount of automatically generated data for various motion types. The three-step phase-shifting fringe map captured along the timeline is divided into three groups and processed by the trained three-stream neural network to generate an accurate phase map. However, this methodology is complex and difficult to implement. Fu G et al. [11] proposed a dynamic phase measurement profilometry (PMP) based on the principle of three-color binary fringe combination time division multiplexing. By combining red (R), green (G), and blue (B) stripes into a three-color stripe of the same width without any color overlap, it can be pre-fed into the flash memory of a high-speed digital optical projector (HDLP). Special time division multiplexing timing was designed to control the HDLP to project the three-color binary stripes stored in the flash memory to the dynamic object under test in sequence at 234 frames per second, and set the projected light source mode to monochromatic mode, that is, all RGB LEDs remain luminous. At the same time, it triggers a high frame rate monochrome camera synced with the HDLP to capture the corresponding anamorphic patterns in the R, G, and B channels. By filtering, an almost complete phase-shift sinusoidal deformation pattern can be extracted from the captured three-step PMP phase-shift sinusoidal deformation pattern. It is equivalent to 3D shape reconstruction of the dynamic object under test at 78 frames per second. Experimental results show that the methodology is feasible and effective. This methodology is effective for the measurement of dynamic objects and can effectively avoid color crosstalk. This methodology has certain feasibility, but the experimental steps proposed by this methodology are complicated and difficult to implement. Yang C et al. [12] proposed a new single 3D measurement methodology based on four-element grating projection. In traditional binary grating PMP, sinusoidal fringes are extracted from multi-step or color fringe patterns. In this methodology, grayscale is fourth order by using DLP4500’s two-bit grayscale encoding mode. The three non-zero gray levels are arranged in a cycle of equal width, and the fourth gray value is zero, which is not encoded in the fringe pattern, but represents shadow information in the deformation pattern, where a fourth-order raster is encoded. When the DLP4500 projects the four-element grating onto the object under test, the charge-coupled device (CCD) synchronously captures the corresponding deformation pattern. The algorithm proposed in this paper can be used to segment three-frame binary deformation maps with a duty ratio of 1/3 and relative displacement of a 1/3 period. Three sinusoidal deformation modes with 2π/3 shift phase can be obtained by extracting the fundamental frequencies of the three binary deformation modes, and the 3D shape of the object can be reconstructed by using PMP methodology. Mohammadi et al. [13] achieved a multi-wavelength digital phase-shifting moiré by determining multiple moiré wavelengths through system calibration over the full operating depth range. In this methodology, the extended noise phase diagram is used as reference, and the phase diagram is expanded at a shorter wavelength to obtain a continuous phase diagram with lower noise and more accuracy moiré wavelength calibration determines the relationship between moiré wavelength and height, allowing pixel-level refinement of moiré wavelength and height during 3D reconstruction. Only one mode needs to be projected, so only one image needs to be captured to calculate each phase map with different wavelengths to perform a digital phase-shift moiré time phase unrolling. Two-wavelength phase unwrapping requires only two capture images, and three-wavelength phase unwrapping requires only three capture images. This methodology has been verified in the 3D surface shape measurement of objects with surface discontinuity and space isolation. However, the accuracy of moiré in this methodology is too high for experiment. Luo et al. [14] proposed a square-root volume Kalman filtering phase unwrapped (SCKFPU) procedure. By combining SCKF algorithm with phase gradient estimator, a phase unwrapping method for discrete Markov jump systems is proposed for tracking maneuvering targets. The phase quality estimation function is designed and the optimal path tracking strategy is proposed. This strategy ensures that the SCKFPU method performs both noise suppression and InSAR image expansion along pixel points, and has highly dependent and low-dependence pixels in the image. However, this procedure has many steps and increases the computational burden. Lei et al. [15] calculate the bar based on the heterodyne principle. The fractional part of the grain series, and then the actual value of the current fractional part is calculated from the phase principal value of the raster fringe of the frequency corresponding to the fringe series. Then, according to the phased master, the error of the value itself sets the judgment threshold, and the threshold is used to judge the difference between the calculated value and the actual value. Finally, according to the judgment results, the period of the intermediate parameters. The number of times is adjusted to add or subtract to correct the absolute phase. However, there are still some jump points.

According to the above problems, a phase unwrapping correction procedure based on multi-frequency heterodyne procedure is proposed to solve the jumping error in phase unwrapping. Firstly, the phase principal values under three different frequencies are obtained by the four-step phase shifting method. Then, the dual-frequency heterodyne phase unwrapping method is used to obtain the phase principal values of the two adjacent frequencies, and the two lower frequency wrapped phases are obtained. Then the threshold is set for the absolute difference between the actual value of the decimal part with certain error calculated from the phase principal value and the theoretical value of the decimal part derived from the three-frequency heterodyne phase principle, so that the number of cycles for phase unwrapping can be added by one, subtracted by one, or the original value may be left unchanged to realize absolute phase correction. The experimental results show that this procedure can correct the jumping error effectively and has high noise resistance.

2. Principle of Heterodyne Phase Unwrapping

2.1. Solution of Phase Principal Value

The four-step phase-shifting procedure [9,10,11] is the most commonly used method to obtain the phase principal value at present. The basic principle of the phase-shifting procedure is that the projection device is usually controlled chronologically. If more than three fringe patterns are projected, the grayscale distribution of the fringe patterns must conform to the sinusoidal transformation. In the law of transformation, the phase starting values of two adjacent fringe patterns differ by a fixed phase, that is, the constant phase. Displacement is also known as phase shift. The camera synchronously acquires these images, and after least squares or arctangent processing, phase information can be extracted. The distribution of the gray values of the four fringe images at coordinates (m,n) is shown in Formula (1):

$$\{\begin{array}{l}{I}_1(m,n)=I^{\prime }(m,n)+I^{″}(m,n)\cos [\phi (m,n)]\\ I_2(m,n)=I^{\prime }(m,n)+I^{″}(m,n)\cos [\phi (m,n)+{\displaystyle \frac{\pi }{2}}]\\ I_3(m,n)=I^{\prime }(m,n)+I^{″}(m,n)\cos [\phi (m,n)+\pi ]\\ I_4(m,n)=I^{\prime }(m,n)+I^{″}(m,n)\cos [\phi (m,n)+{\displaystyle \frac{3\pi }{2}}]\end{array}$$

(1)

where I_i (m, n) is the gray value of the i-th fringe pattern at the point (m, n); I′ (m, n) is the gray value of the image background; I″ (m, n) is the grayscale modulation of image; φ (m, n) is the phase principal value needed to be solved. Then, the phase solution of the four-step phase-shifting method is shown in Formula (2):

$$\phi (m,n)\hspace{0.17em}=\hspace{0.17em}\arctan [\frac{I_4(m,n)-I_2(m,n)}{I_1(m,n)-I_3(m,n)}]$$

(2)

According to Formula (2) and the trigonometric function property [12,13,14], the phase is wrapped in the interval [−π, π] and exhibits a serrated distribution. The phase unwrapping is necessary to obtain the complete and continuous distribution of absolute phases.

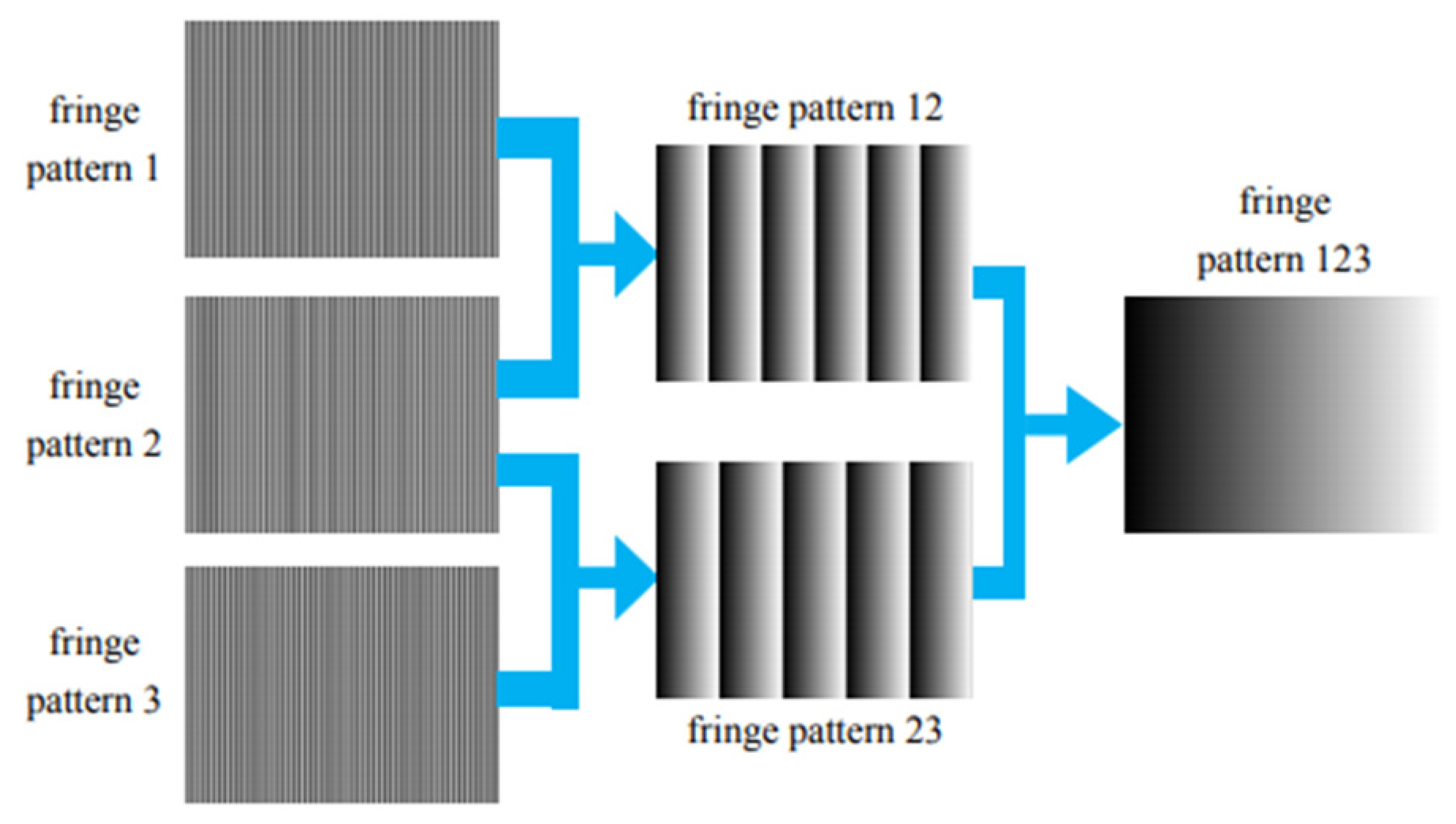
2.2. Principle of Heterodyne Phase Unwrapping

Multi-frequency heterodyne phase unwrapping [15] is a phase measurement method based on the principle of interference, which is an improvement of the heterodyne phase unwrapping method. Compared to dual-frequency heterodyne unwrapping methods, it can use optical signals with more frequencies to improve the accuracy and reliability of phase measurements.

In multi-frequency heterodyne phase unwrapping, three or more optical signals with similar frequencies are used for interference, and the phase information on the measured object surface or medium can be obtained by calculating and processing the interference signals of different frequencies. Specifically, these optical signals with similar frequencies are mixed to obtain a different frequency signal at several frequencies. Phase solving and synthesis of these differential frequency signals can obtain the absolute phase distribution of the measured object. In this paper, the three-frequency heterodyne phase unwrapping is utilized to solve the absolute phase, as shown in Figure 1:

Firstly, three sinusoidal gratings with pitches P₁, P₂, and P₃ are projected onto the measured object, respectively. Then, after superposition, P₁ and P₂ generate fringe pattern 12 with pitch P₁₂, and P₂ and P₃ generate fringe pattern 23 with pitch P₂₃, where P₁ < P₂ < P₃ < P₁₂ < P₂₃. For a certain point on the measured object [15], the following relationships exist:

$$P_{23}=\frac{P_2{P}_3}{P_3-P_2}, P_{23}=\frac{P_2{P}_3}{P_3-P_2}$$

(3)

$$P_1{n}_1=P_2{n}_2=P_3{n}_3=P_{12}{n}_{12}=P_{23}{n}_{23}$$

(4)

$$\mathsf{\Delta }{n}_i={\displaystyle \frac{{\phi }_i}{2\pi }}, i = 1,2,12,23,123$$

(5)

$${\theta }_i=2\pi n_i=2\pi (\mathsf{\Delta }{n}_i+N_i)\hspace{1em}{N}_i\in Z$$

(6)

$$\{\begin{array}{l}\mathsf{\Delta }{n}_{12}=\{\begin{array}{c}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\mathsf{\Delta }{n}_1 - \mathsf{\Delta }{n}_2,\hspace{1em}\hspace{0.17em}\mathsf{\Delta }{n}_1 \ge \mathsf{\Delta }{n}_2\\ \mathsf{\Delta }{n}_1 - \mathsf{\Delta }{n}_2 + 1,\hspace{1em} \mathsf{\Delta }{n}_1 < \mathsf{\Delta }{n}_2\end{array}\\ \mathsf{\Delta }{n}_{23}=\{\begin{array}{c}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\mathsf{\Delta }{n}_2 - \mathsf{\Delta }{n}_3,\hspace{1em}\hspace{0.17em}\mathsf{\Delta }{n}_{{}_2} \ge \mathsf{\Delta }{n}_3\\ \mathsf{\Delta }{n}_2 - \mathsf{\Delta }{n}_3 + 1,\hspace{1em} \mathsf{\Delta }{n}_2 < \mathsf{\Delta }{n}_3\end{array}\end{array}$$

(7)

where, φ_i and θ_i refer to the phase principal value and absolute phase of the corresponding fringe pattern, respectively; n_i is the fringe order of the corresponding fringe pattern; Δn_i and N represent the decimal part and integer part of the fringe order, respectively. Fringe pattern 123 is generated by the superposition of fringe patterns 12 and 23. A suitable choice of P₁, P₂, and P₃ allows the fringe pitch of fringe pattern 123 to cover the full field. At this time [16,17,18], N = 0, so

$$\mathsf{\Delta }{n}_{123}=\{\begin{array}{c}\hspace{1em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\mathsf{\Delta }{n}_{12} - \mathsf{\Delta }{n}_{23},\hspace{1em}\hspace{0.17em}\mathsf{\Delta }{n}_{12} \ge \mathsf{\Delta }{n}_{23}\\ \mathsf{\Delta }{n}_{12} - \mathsf{\Delta }{n}_{23} + 1,\hspace{1em} \mathsf{\Delta }{n}_{12} < \mathsf{\Delta }{n}_{23}\end{array}$$

(8)

$$n_{12}=\frac{P_{23}(N_{123} + \mathsf{\Delta }{n}_{123})}{P_{23}-P_{12}},$$

(9)

N₁₂ = floor(n₁₂)

(10)

$${\theta }_{12}=2\pi \times N_{12}+{\phi }_{12},$$

(11)

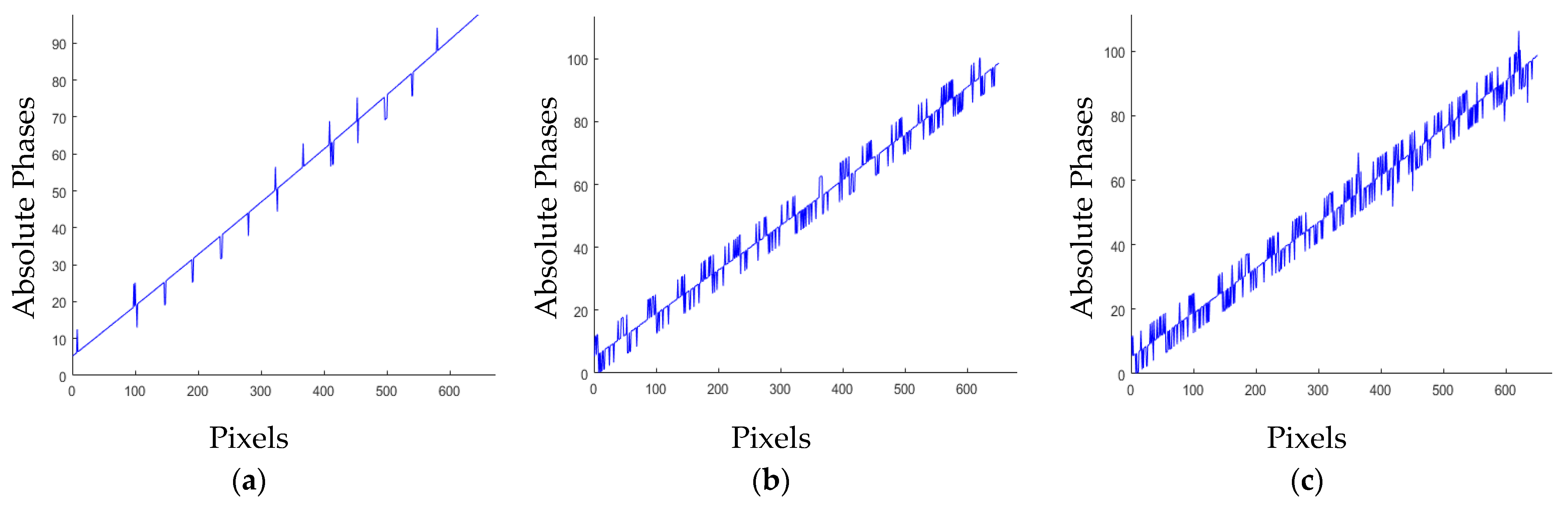
2.3. Error Analysis

Formulas (7) and (8) show that when Δ1 and Δ2 or Δ2 and Δ3 are close to each other, even if the values are extremely small, it will lead to a jump from 0 to 1 in Δ12 and Δ23 and the errors of the phase principal values obtained by grating 1, grating 2, and grating 3 are independent of each other. Therefore, for the three-frequency heterodyne phase unwrapping, each solution creates an error that eventually leads to an increase in the error of Δ123. When the error is multiplied by the coefficient P₁₂/(P₂₃ − P₁₂), the error will be magnified significantly. Assume that

$$H=\frac{P_{12}}{P_{23}-P_{12}}$$

(12)

According to Formula (9), when Δ123 = c/H, c = 1, 2, 3, 4, …, N₁₂ will jump, that is, the phase unwrapping will jump. According to Formulas (5) and (6), the theoretical value of the decimal part Δn_12t of the fringe order derived using heterodyne should be equal to the actual value of the decimal part Δn_12r calculated based on the principal value of the current phase. The theoretical value of the decimal part is

Δn_12t = n₁₂ − N₁₂,

(13)

In fact, due to the error of the phase principal value, the two values are not equal. According to the analysis above, if the number of cycles changes, the absolute value of the difference between the theoretical value and the actual value must be bigger or smaller than a certain value, and this value is related to the error of the phase principal value itself, set this value as threshold T. Let the error of Δn₁ refer to q₁, the error of Δn₂ refer to q₂, and the error of Δn₃ refer to q₃, then the error of Δn₁₂₃ is (−q₁ − q₂ − q₃,q₁ + q₂ + q₃). Thus, the value of threshold T is

$$T=H·(q_1+q_2+q_3)$$

(14)

The maximum error of the phase principal value extracted by the uncorrected four-step phase-shifting procedure is no more than 0.08 rad [15], and the value of H can be calculated from the grating pitch, so all quantities in Formula (14) are known.

In summary, the method of adjusting the abnormal jump in the cycle is as follows:

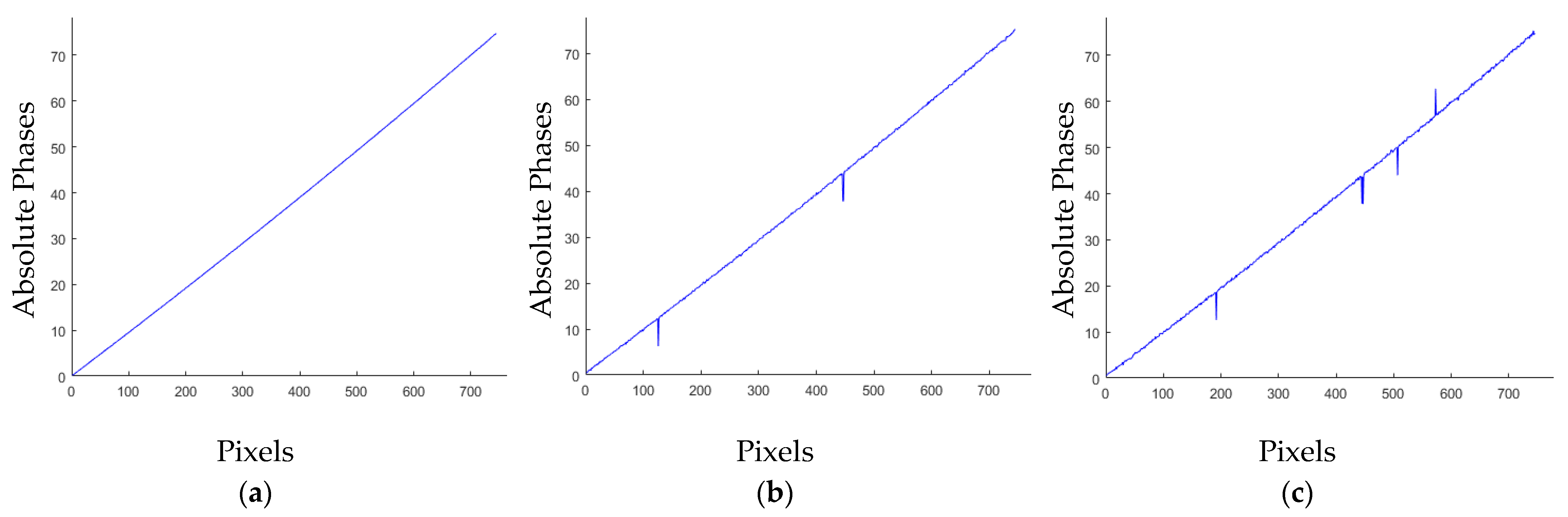
• If |Δn_12r − Δn_12t| < T, the cycle will jump accurately and no adjustment is needed.
• If |Δn_12r − Δn_12t| ≥ T and Δn_12r − Δn_12t > 0, the cycle will jump upward and the number of cycles will be minus one.
• If |Δn_12r − Δn_12t| ≥ T and Δn_12r − Δn_12t ≤ 0, the cycle will jump downward and the number of cycles will be plus one.

3. Experiments and Result Analysis

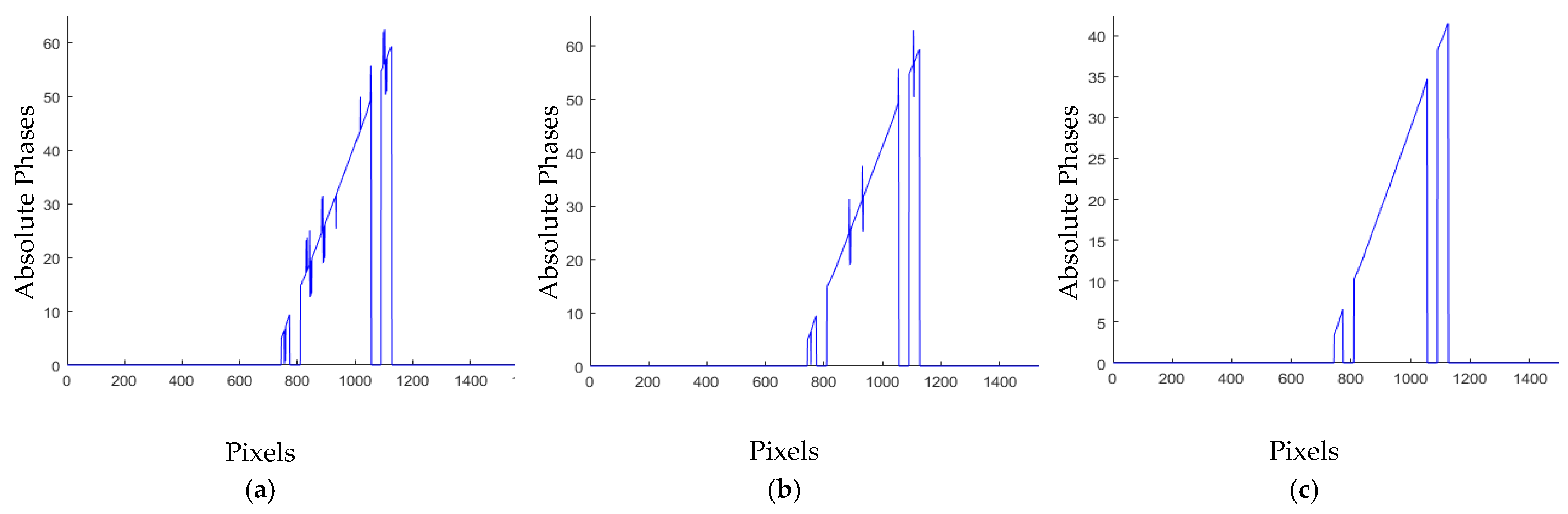
3.1. Simulation Experiments

To verify the feasibility of the above method, three sets of four-step phase-shifting patterns with grating pitches of 16, 20, and 26 pixels are designed. Different Gaussian noises are added to the phase-shifting patterns, and the phase principal values of three sets of patterns are obtained according to the four-step phase-shifting method. The phase principal values are unwrapped by the three-frequency heterodyne principle, the absolute phase is corrected by the procedure in this paper, and the threshold value H is set as 0.459. As a comparison, two sets of phase-shifting patterns with grating pitches of 34 and 36 pixels are designed [19,20,21], while adding the same Gaussian noise. The phase principal values of the two sets of patterns are obtained according to the four-step phase-shifting method, and the phase principal values are unwrapped by the traditional method (the dual-frequency heterodyne procedure) and the method of [15], respectively. The numbers of calculated jumping points are shown in

Table 1. Jumping error points.

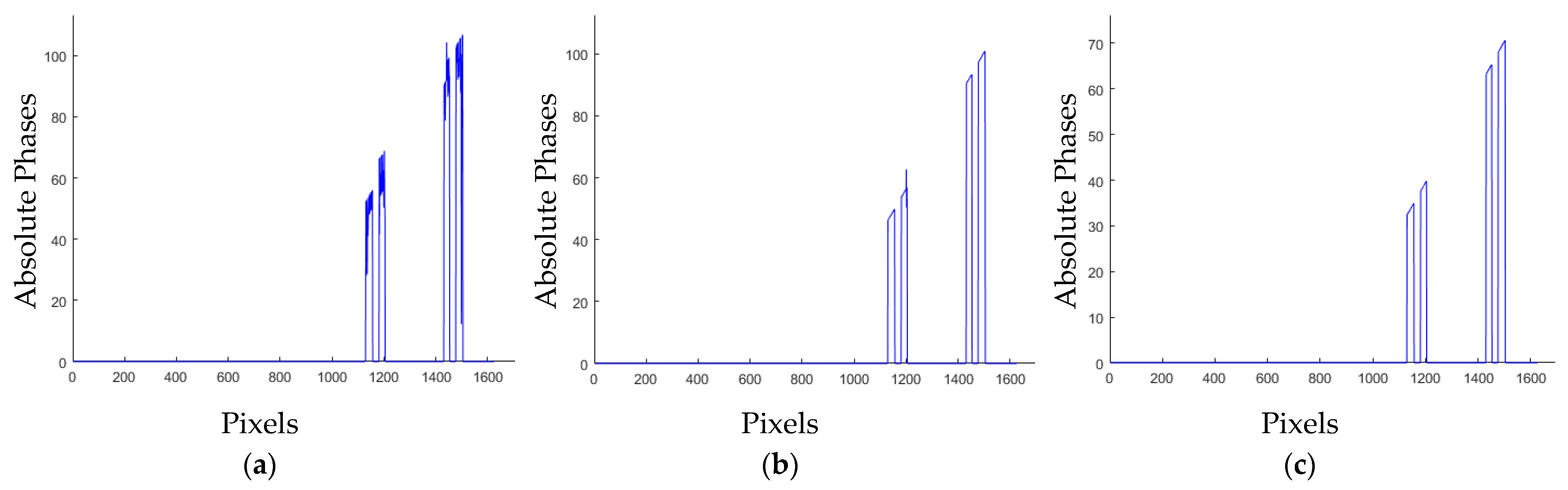
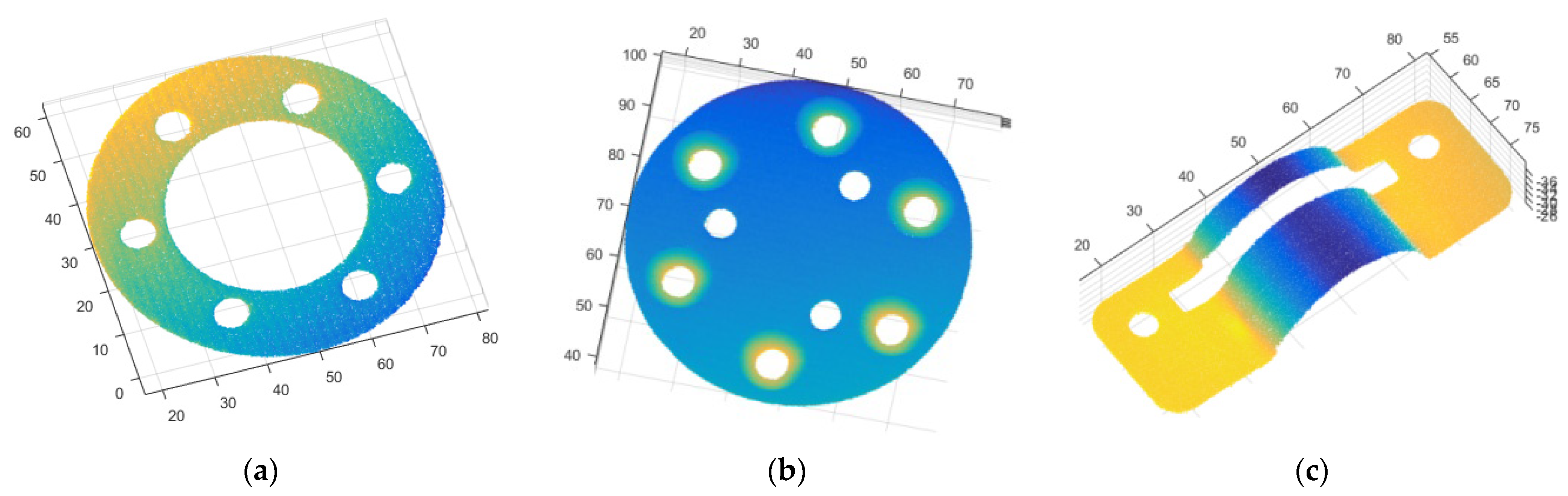
Gaussian Noise		Jumping Point			Correction Rate/%
Mean Value	Variance	Traditional Method	Method of Literature [15]	Method of this Paper
0	0	40,339	1668	1305	96.8
0.01	0.002	112,654	2345	2249	98.0
0.03	0.004	142,195	3705	2762	98.1

. The absolute phases obtained by different methods are shown in the Figure 2, Figure 3 and Figure 4.

As shown in Table 1, compared with the traditional method, the correction rate of absolute phase jumping points can reach more than 96.8%, and in the face of Gaussian noise with different mean values and variances, only a few jumping points are not corrected. Compared with the method in the literature [15], the number of jumping points corrected by the method in this paper is also bigger.

Figure 2, Figure 3 and Figure 4 show the absolute phase diagrams after the wrapped phase expansion by the traditional method of phase solving, the method of [15] and the improved method of this paper, respectively. Figure (a), Figure (b), and Figure (c) represent the absolute phase diagrams without Gaussian noise, with Gaussian noise of mean 0.01 and variance 0.002 (the first set of Gaussian noise), and with Gaussian noise of mean 0.03 and variance 0.004 (the second set of Gaussian noise), respectively, in the phase shift pattern using the current three sets of wrapped phase patterns solved by the method.

It is evident in Figure 2 that the phase unwrapped by the traditional dual-frequency heterodyne method undergoes many jumps; especially after the addition of noise, the number of jumping points increases dramatically, which introduces a lot of errors and the obtained absolute phase is not accurate. Meanwhile, it will also cause a great impact on the 3D reconstruction.

As can be observed from Figure 3, the method in [15] improves the threshold value setting for the jumping errors in the unwrapping phase. Adding fringe images with less noise can basically remove the jumps completely, but when faced with an environment with more noise, there will still be more jumping points.

As shown in Figure 4, the jumps can be basically removed when the wrapping phase is unwrapped by the method in this paper, and the absolute phase rarely jumps, even in the face of varying noises, providing high noise immunity.

3.2. Real Experiments

In order to verify the practicality of the proposed method, flange, oil gasket, and U-card are selected as the tested samples. The traditional method, the method of [15], and the method of this paper are, respectively, applied to detect the 3D contour of the above three samples. Firstly, the projection device projects the gratings with pitches of 34 and 36 pixels to the measured object, and eight phase-shifting images are obtained using the four-step phase-shifting procedure and the dual-frequency heterodyne method. The traditional method and the method of literature [15] are used to unwrap the wrapped phase and process the number of cycles correspondingly. Then the projection device projects gratings with pitches of 16, 20, and 26 pixels, respectively, to the measured object, and 12 phase-shifting images are obtained by the four-step phase-shifting procedure and three-frequency heterodyne technique. The technique in this paper is utilized to unwrap the wrapped phase and adjust the number of cycles by determining the threshold value. A group of data is taken across the center of the measured object to compare the absolute phase of the three techniques, as shown in Figure 5, Figure 6 and Figure 7.

From Figure 5, Figure 6 and Figure 7, it can be seen that when the absolute phase of the measured object is obtained by different methods, the traditional method will have many jumping points when the phase is expanded, resulting in the inaccuracy of the absolute phase, which leads to the decrease of the accuracy when the point cloud is calculated subsequently; while the method of [15] can correct part of the phase jumping compared with the traditional method, but there will still be part of the phase inaccuracy, which will still cause the decrease in the accuracy when the point cloud is calculated subsequently. Compared with the traditional method and the method of [15], the principle of three-frequency outlier deconvolution has a longer absolute phase period and more accurate data, and the combination of the improved method proposed in this paper can effectively remove the phase jump error, ensure the accuracy of the absolute phase, and improve the guarantee for the subsequent calculation of the point cloud, and it has certain anti-noise capability in the face of different noises. Figure 8a–c show the 3D reconstructed point clouds of the above three samples, respectively.

In this paper, the internal and external parameters of the camera are obtained by using Zhang Zhengyou’s camera calibration technique [22]. Then the grating fringe is projected to the measured object, and the phase principal value of the measured object is obtained. The technique in this paper is used to expand the phase principal value, and finally the reconstructed 3D point cloud image of the measured object is obtained combined with the calibration parameters. In order to prove the applicability and universality of the technique, three mechanical workpieces including flange, oil gasket, and U-shaped card were selected for 3D profile measurement. Figure 8a–c are the 3D point cloud images representing the three kinds of mechanical workpiece. It can be clearly observed that the surface of the figure is flat and continuous, and the hollow, concave, and convex parts on the measured surface can be reconstructed appropriately, which indicates that the proposed technique has a good suppression effect on the jumping error of phase, and the improved technique has certain effectiveness.

4. Conclusions

Aiming at the jumping error in the traditional multi-frequency heterodyne phase unwrapping, the phase principal values of three frequencies are obtained by the four-step phase-shifting technique, and the corresponding threshold value is set according to the difference between the actual value of the decimal part calculated from the phase principal value and the theoretical value of the decimal part derived from the principle of multi-frequency heterodyne phase unwrapping. The number of cycles is adjusted by the threshold value, so as to reduce the jumping of the absolute phase. The experimental results show that compared with the traditional dual-frequency heterodyne phase unwrapping, the elimination rate of the jumping points of the technique proposed in this paper can reach more than 96.8%, and the technique is not susceptible to noise interference, which has strong noise resistance and practicality. Furthermore, whether the measured object has a simple continuous surface or a complex steep surface, this method can measure its complete 3D contour surface, which proves the certain universality of the proposed method and provides a certain reference value for the subsequent 3D reconstruction based on fringe projection.