Assessment of Deformation Flow in 1050 Aluminum Alloy by the Implementation of Constitutive Model Parameters
Abstract
Featured Application
Abstract
1. Introduction
2. Materials, Experiments, and Numerical Methods
2.1. Material and Experimental Procedures
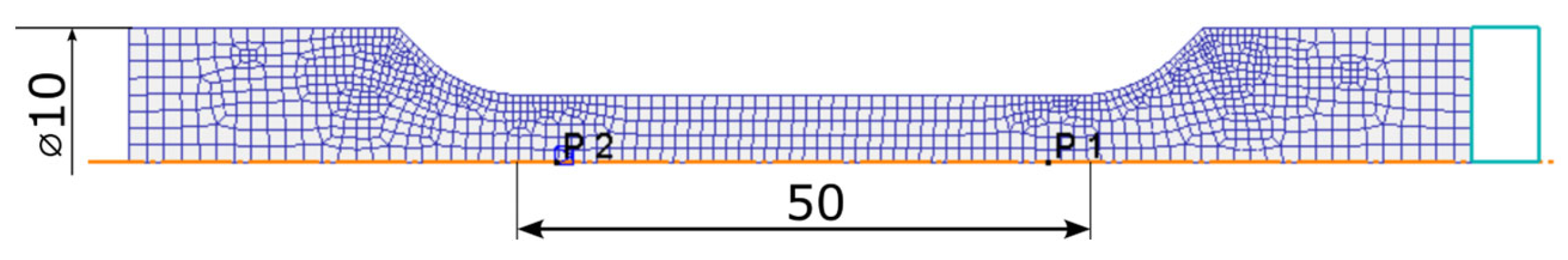
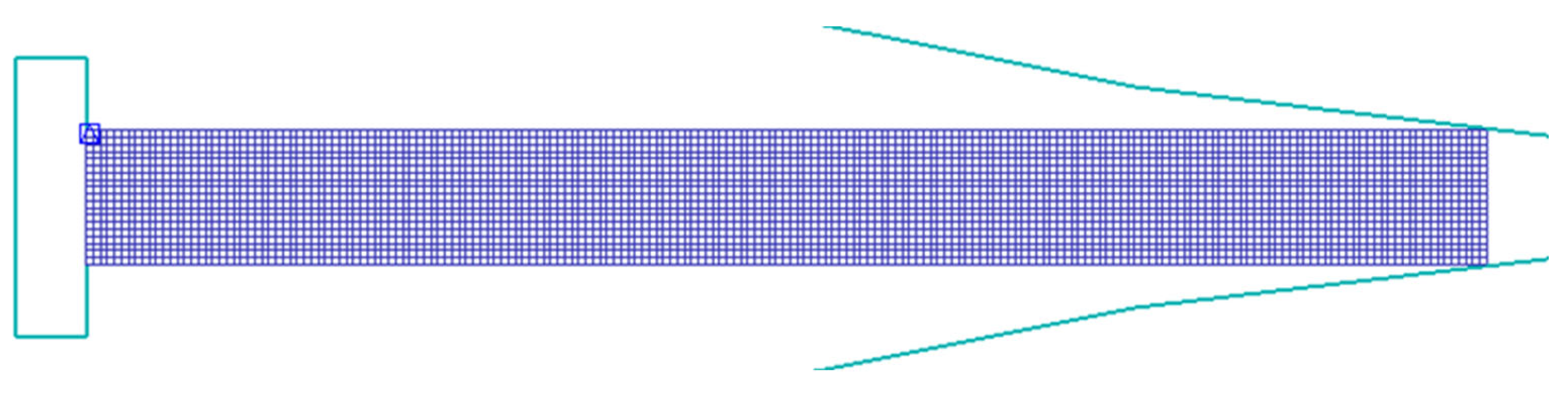
2.2. Finite Element Modeling of Tensile Test
2.3. Finite Element Modeling of Hardness Measurement
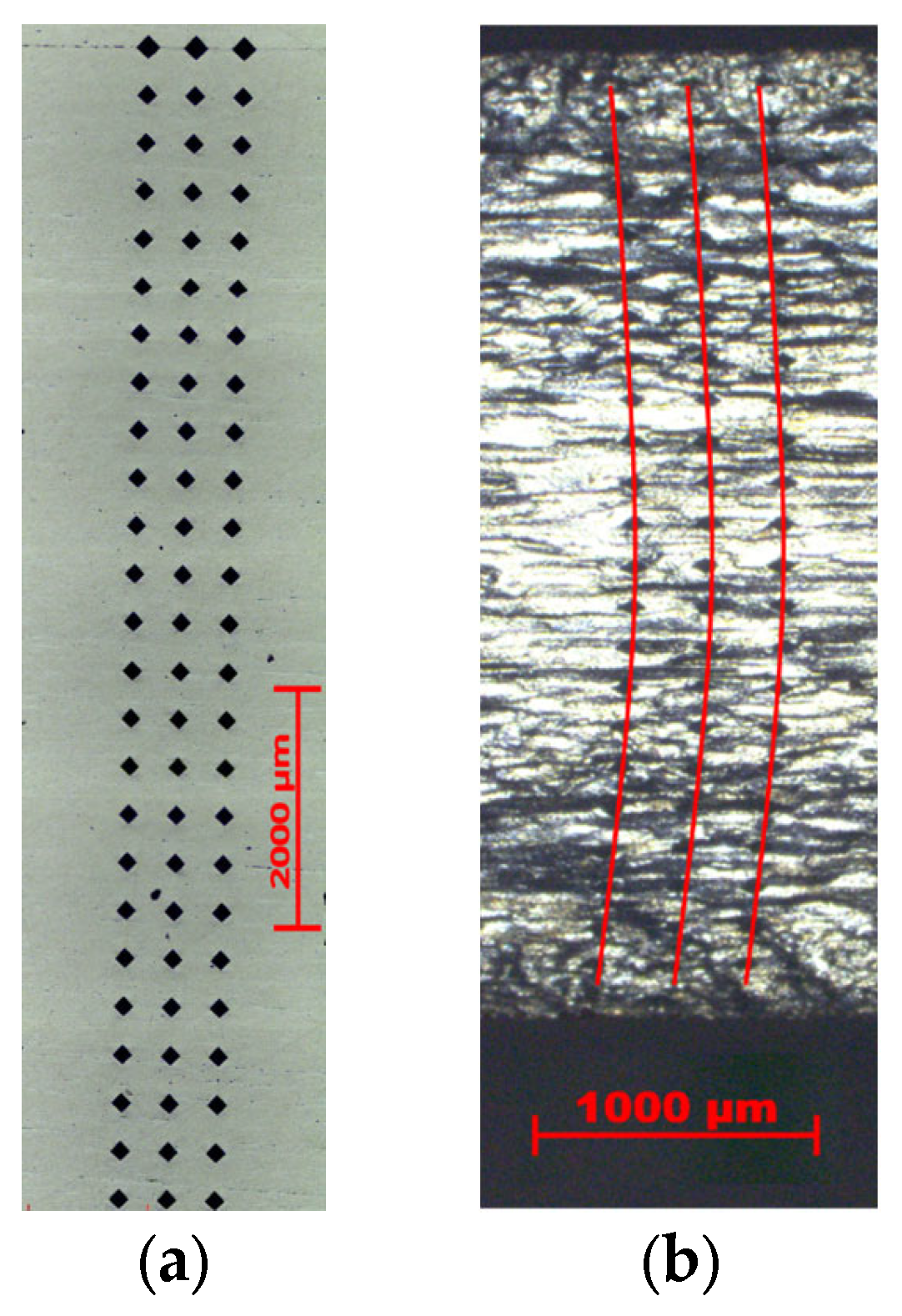
2.4. Finite Element Modeling of Symmetric and Asymmetric Rolling
3. Results and Discussion
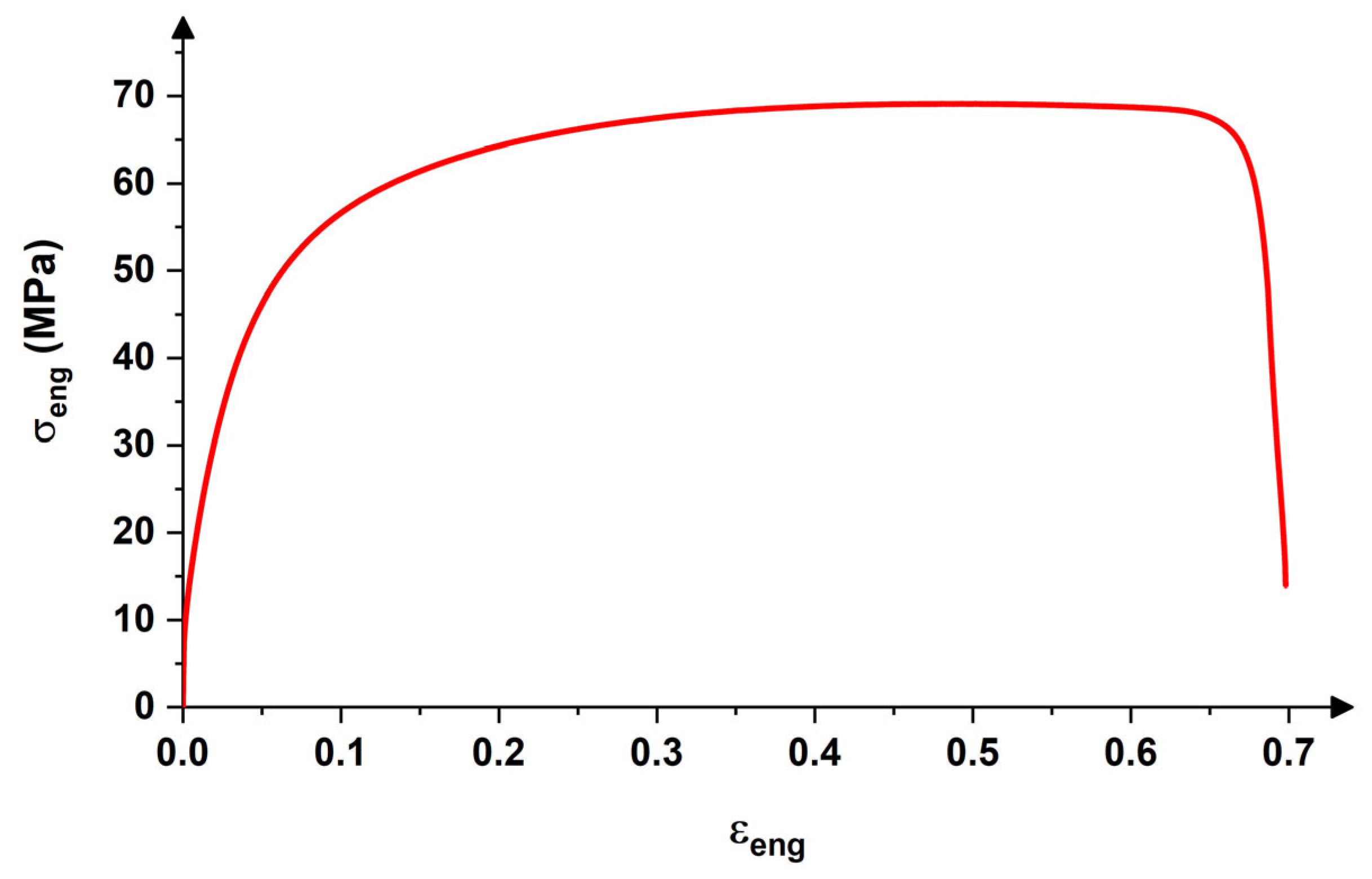
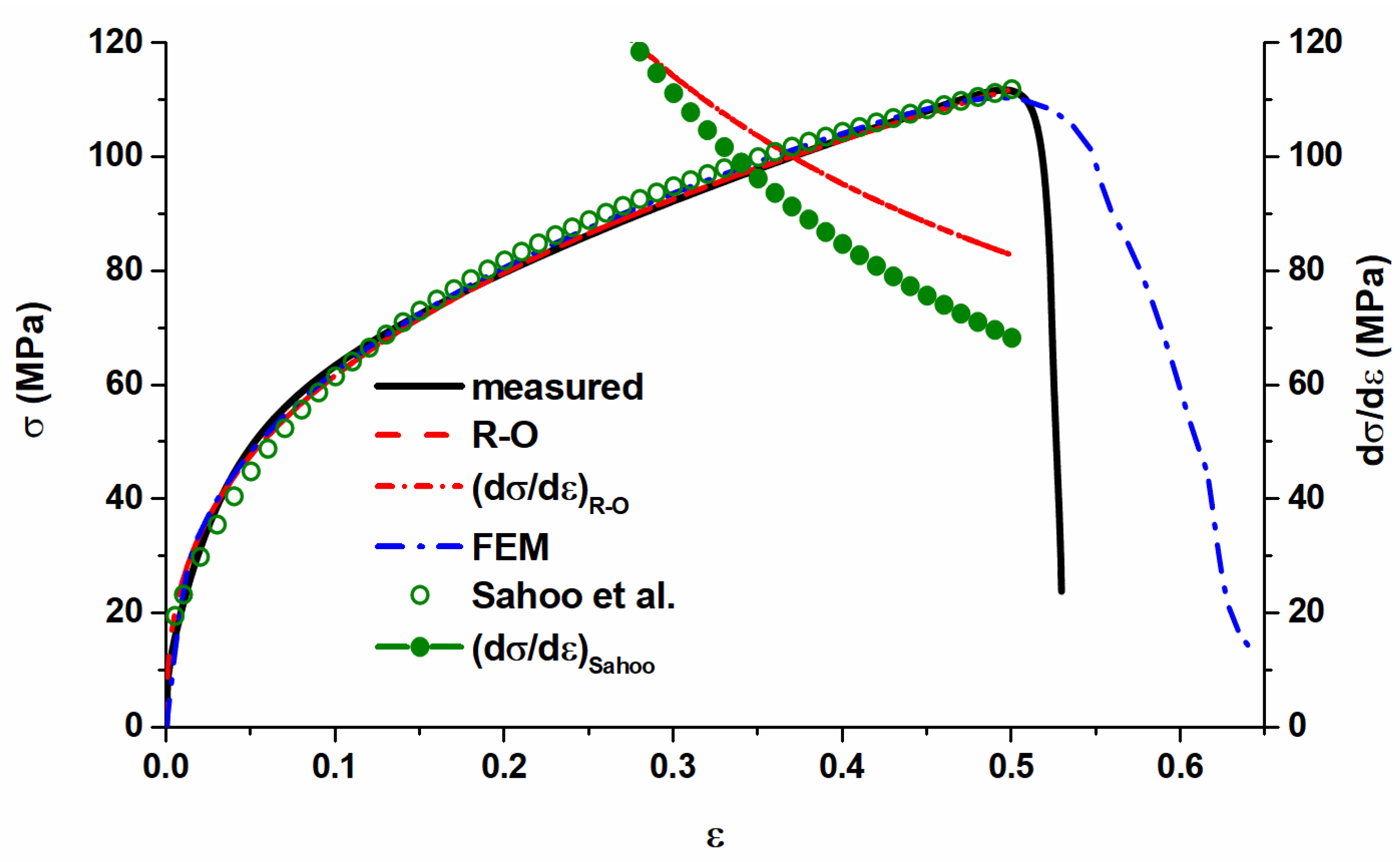
3.1. Tensile Test
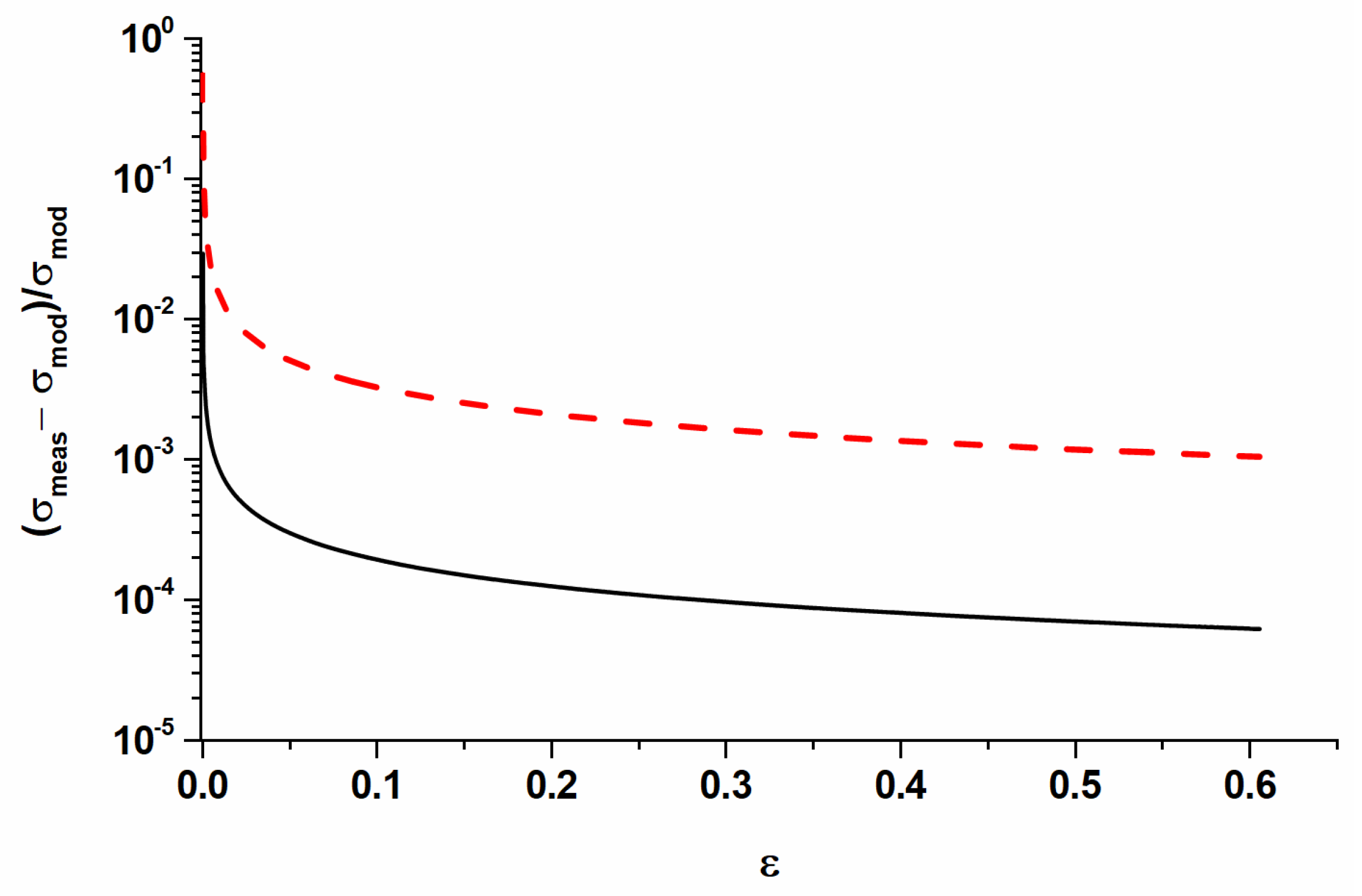
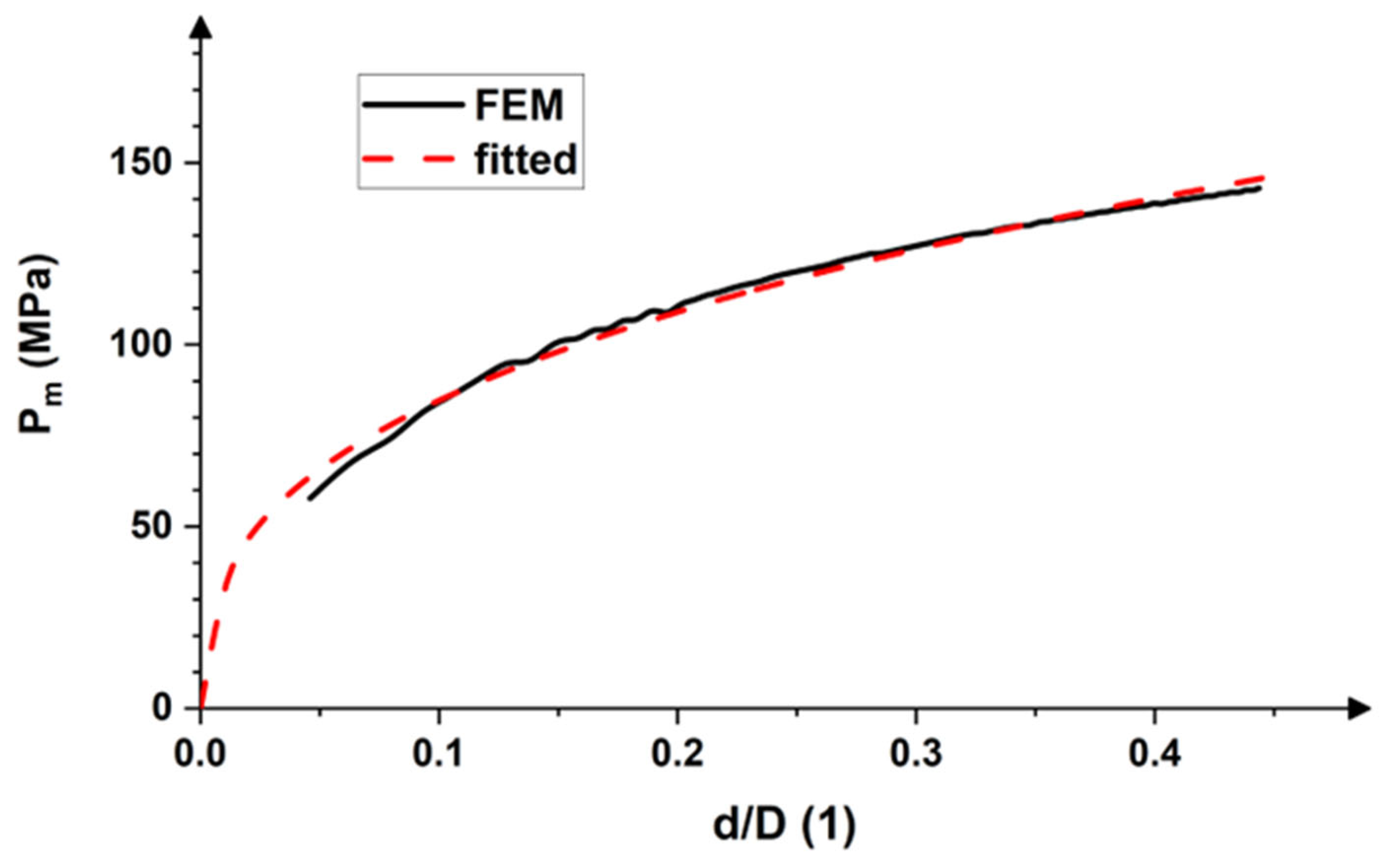
3.2. Defining the Model Parameters from Hardness Simulations
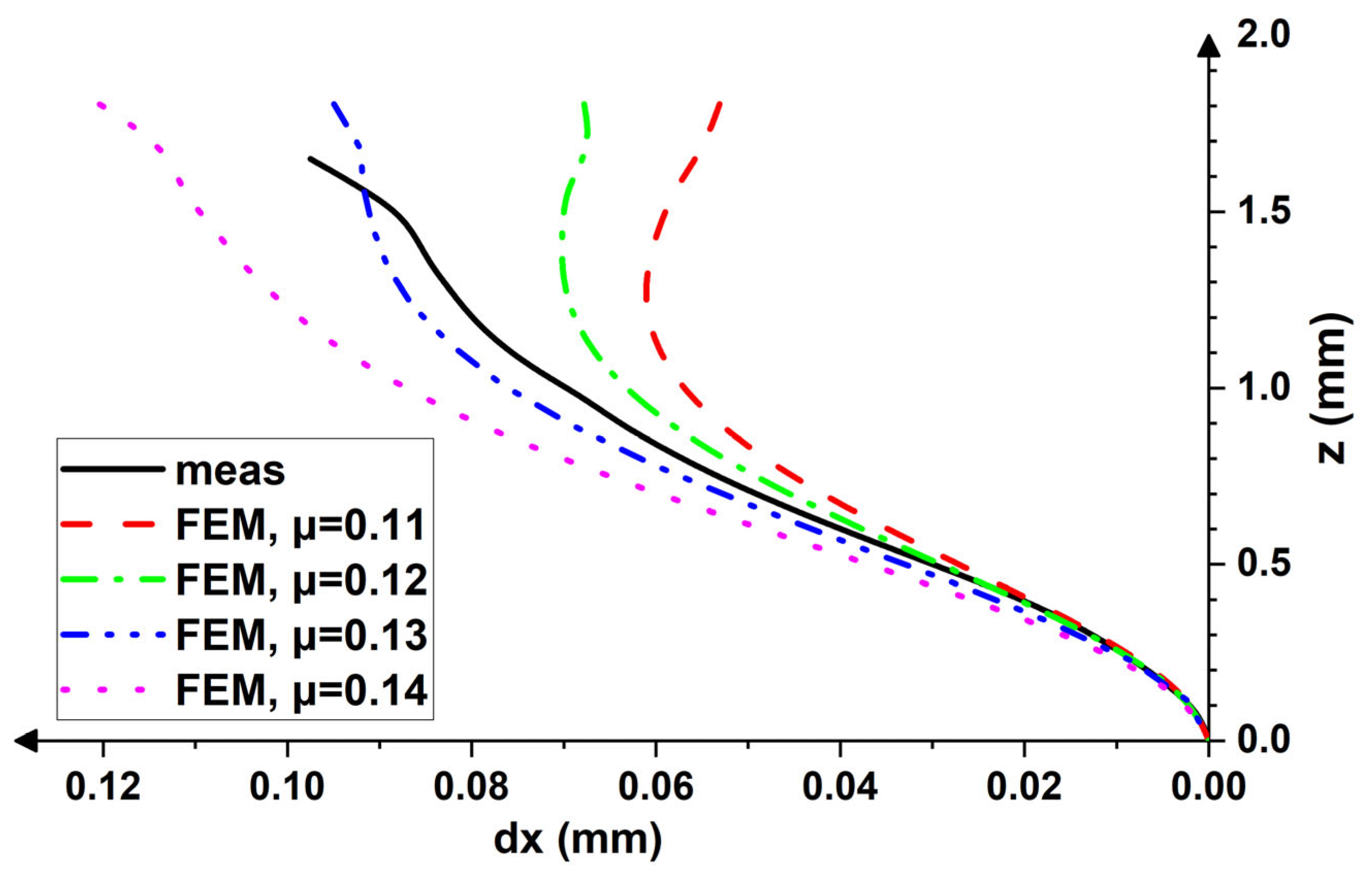
3.3. Rolling Processes: Simulation and Experiment
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pierazzo, E.; Artemieva, N.; Asphaug, E.; Baldwin, E.C.; Cazamias, J.; Coker, R.; Collins, G.S.; Crawford, D.A.; Davison, T.; Elbeshausen, D.; et al. Validation of Numerical Codes for Impact and Explosion Cratering: Impacts on Strengthless and Metal Targets. Meteorit. Planet. Sci. 2008, 43, 1917–1938. [Google Scholar] [CrossRef]
- Schulze, V.; Boev, N.; Zanger, F. Simulation of Metal Cutting Process with Variable Cutting Thickness During Broaching. Procedia CIRP 2012, 1, 437–442. [Google Scholar] [CrossRef]
- Ramberg, W.; Osgood, W.R. Description of Stress-Strain Curves by Three Parameters Technical Notes. National Advisory Committee for Aeronautics. Report: NACA-TN- 902. Washington, July 1943. Available online: https://ntrs.nasa.gov/citations/19930081614 (accessed on 5 January 2023).
- Gadamchetty, G.; Pandey, A.; Gawture, M. On Practical Implementation of the Ramberg-Osgood Model for FE Simulation. SAE Int. J. Mater. Manf. 2016, 9, 200–205. [Google Scholar] [CrossRef]
- Muhanna, R.L.; Mullen, R.L.; Rao, M.R. Nonlinear Interval Finite Elements for Beams. In Proceedings of the Second International Conference on Vulnerability and Risk Analysis and Management (ICVRAM2014), Liverpool, UK, 13–16 July 2014. [Google Scholar]
- Donohue, B.R.; Ambrus, A.; Kalidindi, S.R. Critical Evaluation of the Indentation Data Analyses Methods for the Extraction of Isotropic Uniaxial Mechanical Properties Using Finite Element Models. Acta Mater. 2012, 60, 3943–3952. [Google Scholar] [CrossRef]
- Imai, T.; Utsunomiya, H.; Matsumoto, R. Finite Element Analysis of Plastic Instability Phenomenon in Cold Rolling of Clad Sheets. Procedia Eng. 2017, 184, 306–312. [Google Scholar] [CrossRef]
- Kumar Reddy Sirigiri, V.; Yadav Gudiga, V.; Shankar Gattu, U.; Suneesh, G.; Mohan Buddaraju, K. A Review on Johnson Cook Material Model. Mater. Today Proc. 2022, 62, 3450–3456. [Google Scholar] [CrossRef]
- Jebri, L.; Lazghab, T.; Toumi, M.; Soula, M. The Behavior and Forming Performance of Vapor Hydroforming Process of Thin Aluminum Sheets: Numerical and Experimental Analysis. Adv. Mech. Eng. 2022, 14, 168781322210893. [Google Scholar] [CrossRef]
- Schulze, V.; Vöhringer, O. Plastic Deformation: Constitutive Description. In Encyclopedia of Materials: Science and Technology; Elsevier: Amsterdam, The Netherlands, 2001; pp. 7050–7064. ISBN 978-0-08-043152-9. [Google Scholar]
- Allazadeh, M.R. Computing Strain Rate Sensitivity of Aluminium Alloy 1050. Int. Sci. Surv. J. 2020, 3, 9–19. [Google Scholar]
- Yasnikov, I.S.; Vinogradov, A.; Estrin, Y. Revisiting the Considère Criterion from the Viewpoint of Dislocation Theory Fundamentals. Scr. Mater. 2014, 76, 37–40. [Google Scholar] [CrossRef]
- Jin, H.; Lloyd, D.J. The Tensile Response of a Fine-Grained AA5754 Alloy Produced by Asymmetric Rolling and Annealing. Metall. Mater. Trans. A 2004, 35, 997–1006. [Google Scholar] [CrossRef]
- Reihle, M. Ein einfaches Verfahren zur Aufnahme der Fließkurven von Stahl bei Raumtemperatur. Archiv. Eisenhüttenwesen 1961, 32, 331–336. [Google Scholar] [CrossRef]
- Sahoo, S.K.; Dhinwal, S.S.; Vu, V.Q.; Toth, L.S. A New Macroscopic Strain Hardening Function Based on Microscale Crystal Plasticity and Its Application in Polycrystal Modeling. Mater. Sci. Eng. A 2021, 823, 141634. [Google Scholar] [CrossRef]
- Gyurkó, Z.; Borosnyói, A. Brinell-Hardness Testing and Discrete Element Modelling of Hardened Concrete. Epa. J. Silic. Based Compos. Mater. 2015, 67, 8–11. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, L.; Lu, Z.; Teng, G.; Liu, S.; Hu, Z.; He, A. The Effect of Annealing Temperature on the Recrystallization and Mechanical Properties of Severe Plastic Deformed Commercial Pure Aluminium during Ultra-Fast Annealing. Mater. Res. Express 2021, 8, 046515. [Google Scholar] [CrossRef]
- Cerbu, C.; Teodorescu-Draghicescu, H. Aspects On modeling the Mechanical Behavior of Aluminum Alloys with Different heat Treatments. J. Comput. Appl. Mech. 2017, 12, 85–98. [Google Scholar] [CrossRef]
- Joun, M.; Choi, I.; Eom, J.; Lee, M. Finite Element Analysis of Tensile Testing with Emphasis on Necking. Comput. Mater. Sci. 2007, 41, 63–69. [Google Scholar] [CrossRef]
- Sonmez, F.O.; Demir, A. Analytical Relations between Hardness and Strain for Cold Formed Parts. J. Mater. Process. Technol. 2007, 186, 163–173. [Google Scholar] [CrossRef]
- Oliver, W.C.; Pharr, G.M. An Improved Technique for Determining Hardness and Elastic Modulus Using Load and Displacement Sensing Indentation Experiments. J. Mater. Res. 1992, 7, 1564–1583. [Google Scholar] [CrossRef]
- Tabor, D. The Hardness of Metals; Oxford Classic Texts in the Physical Sciences; Clarendon Press: Oxford, UK; Oxford University Press: New York, NY, USA, 2000; ISBN 978-0-19-850776-5. [Google Scholar]
- Sakai, M. Indentation Contact Mechanics. Online Textbook. 2020. Available online: https://indentpt.com/pdf/IndentationContactMechanics_En_Revised2020.pdf (accessed on 10 January 2023).
- Yazdanshenas, A.; Goh, C.-H. Rockwell Hardness Testing on an Aluminum Specimen Using Finite Element Analysis. SSRG Int. J. Mech. Eng. 2020, 7, 1–10. [Google Scholar] [CrossRef]
- Rule, W.K. Finite Element Modeling of Brinell and Rockwell Hardness Testing of Metals. In Proceedings of the Problems Involving Thermal Hydraulics, Liquid Sloshing, and Extreme Loads on Structures, ASMEDC, San Diego, CA, USA, 1 January 2004; pp. 191–196. [Google Scholar]
- Mišović, M.; Tadić, N.; Jaćimović, M.; Janjić, M. Deformations and Velocities during the Cold Rolling of Aluminium Alloys. Mater. Tehnol. 2016, 50, 59–67. [Google Scholar] [CrossRef]
- Fluhrer, J. DEFORM(TM) 2D Version 8.1 User’s Manual; Scientific Forming Technologies Corporation: Columbus, OH, USA, 2015. [Google Scholar]
- Sidor, J.; Miroux, A.; Petrov, R.; Kestens, L. Controlling the Plastic Anisotropy in Asymmetrically Rolled Aluminium Sheets. Philos. Mag. 2008, 88, 3779–3792. [Google Scholar] [CrossRef]
- Cui, Q.; Ohori, K. Grain Refinement of High Purity Aluminium by Asymmetric Rolling. Mater. Sci. Technol. 2000, 16, 1095–1101. [Google Scholar] [CrossRef]
- Ma, C.Q.; Hou, L.G.; Zhang, J.S.; Zhuang, L.Z. Experimental and Numerical Investigations of the Plastic Deformation during Multi-Pass Asymmetric and Symmetric Rolling of High-Strength Aluminum Alloys. Mater. Sci. Forum 2014, 794–796, 1157–1162. [Google Scholar] [CrossRef]
- Inoue, T. Strain Variations on Rolling Condition in Accumulative Roll-Bonding by Finite Element Analysis. In Finite Element Analysis; Moratal, D., Ed.; InTech, Sciyo: Rijeka, Croatia, 2010; ISBN 978-953-307-123-7. [Google Scholar]
- Inoue, T.; Qiu, H.; Ueji, R. Through-Thickness Microstructure and Strain Distribution in Steel Sheets Rolled in a Large-Diameter Rolling Process. Metals 2020, 10, 91. [Google Scholar] [CrossRef]
- Hirt, G.; Senge, S. Selected Processes and Modeling Techniques for Rolled Products. Procedia Eng. 2014, 81, 18–27. [Google Scholar] [CrossRef]
- Ma, C.Q.; Hou, L.G.; Zhang, J.S.; Zhuang, L.Z. Strain Analysis during the Symmetric and Asymmetric Rolling of 7075 Al Alloy Sheets. In Light Metals 2015; Hyland, M., Ed.; Springer International Publishing: Cham, Switzerland, 2015; pp. 445–449. ISBN 978-3-319-48610-9. [Google Scholar]
- Avitzur, B. Friction-Aided Strip Rolling with Unlimited Reduction. Int. J. Mach. Tool Des. Res. 1980, 20, 197–210. [Google Scholar] [CrossRef]
- Sidor, J.J. Assessment of Flow-Line Model in Rolling Texture Simulations. Metals 2019, 9, 1098. [Google Scholar] [CrossRef]
- Szűcs, M.; Krallics, G.; Lenard, J.G. The Difficulties of Predicting the Coefficient of Friction in Cold Flat Rolling. J. Tribol. 2021, 143, 101703. [Google Scholar] [CrossRef]
- MiŁek, T. The Influence of Friction Coefficient on Forward Slip in Experimental Research on Cold Longitudinal Flat Rolling. In Proceedings of the Terotechnology: 10th Conference on Terotechnology, Brisbane, Australia, 25 August 2018; pp. 67–72. [Google Scholar]
- Wang, Q.Y.; Zhu, Y.; Zhao, Y. Friction and Forward Slip in High-Speed Cold Rolling Process of Aluminum Alloys. Appl. Mech. Mater. 2012, 229–231, 361–364. [Google Scholar] [CrossRef]
- Sidor, J.J. Crystal Plasticity and Continuum Mechanics-Based Modelling of Deformation and Recrystallization Textures in Aluminum Alloys. IOP Conf. Ser. Mater. Sci. Eng. 2018, 375, 012028. [Google Scholar] [CrossRef]
- Sidor, J.J. Deformation Texture Simulation in Al Alloys: Continuum Mechanics and Crystal Plasticity Aspects. Model. Simul. Mater. Sci. Eng. 2018, 26, 085011. [Google Scholar] [CrossRef]
- Alvi, M.H.; Cheong, S.W.; Suni, J.P.; Weiland, H.; Rollett, A.D. Cube Texture in Hot-Rolled Aluminum Alloy 1050 (AA1050)—Nucleation and Growth Behavior. Acta Mater. 2008, 56, 3098–3108. [Google Scholar] [CrossRef]
- Barlat, F. A Simple Model for Dislocation Behavior, Strain and Strain Rate Hardening Evolution in Deforming Aluminum Alloys. Int. J. Plast. 2002, 18, 919–939. [Google Scholar] [CrossRef]
- Hariharan, K.; Barlat, F. Modified Kocks–Mecking–Estrin Model to Account Nonlinear Strain Hardening. Metall. Mater. Trans. A 2019, 50, 513–517. [Google Scholar] [CrossRef]
- Sidor, J.; Petrov, R.H.; Kestens, L. Texture Control in Aluminum Sheets by Conventional and Asymmetric Rolling. In Comprehensive Materials Processing; Elsevier: Amsterdam, The Netherlands, 2014; pp. 447–498. ISBN 9780080965338. [Google Scholar]
- Pustovoytov, D.; Pesin, A.; Biryukova, O. Finite Element Analysis of Strain Gradients in Aluminium Alloy Sheets Processed by Asymmetric Rolling. Procedia Manuf. 2018, 15, 129–136. [Google Scholar] [CrossRef]
- Serajzadeh, S.; Karimi Taheri, A.; Nejati, M.; Izadi, J.; Fattahi, M. An Investigation on Strain Inhomogeneity in Hot Strip Rolling Process. J. Mater. Process. Technol. 2002, 128, 88–99. [Google Scholar] [CrossRef]




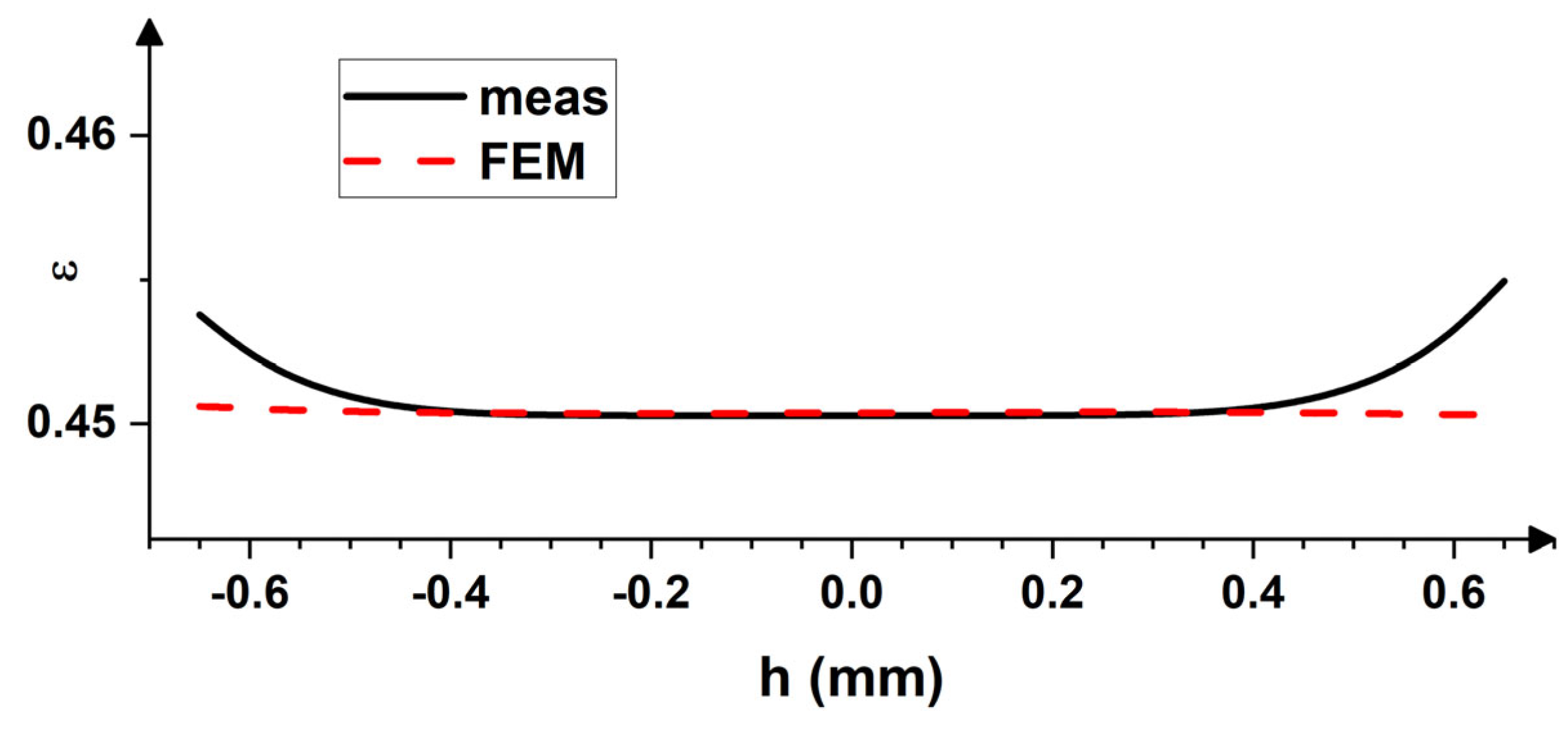

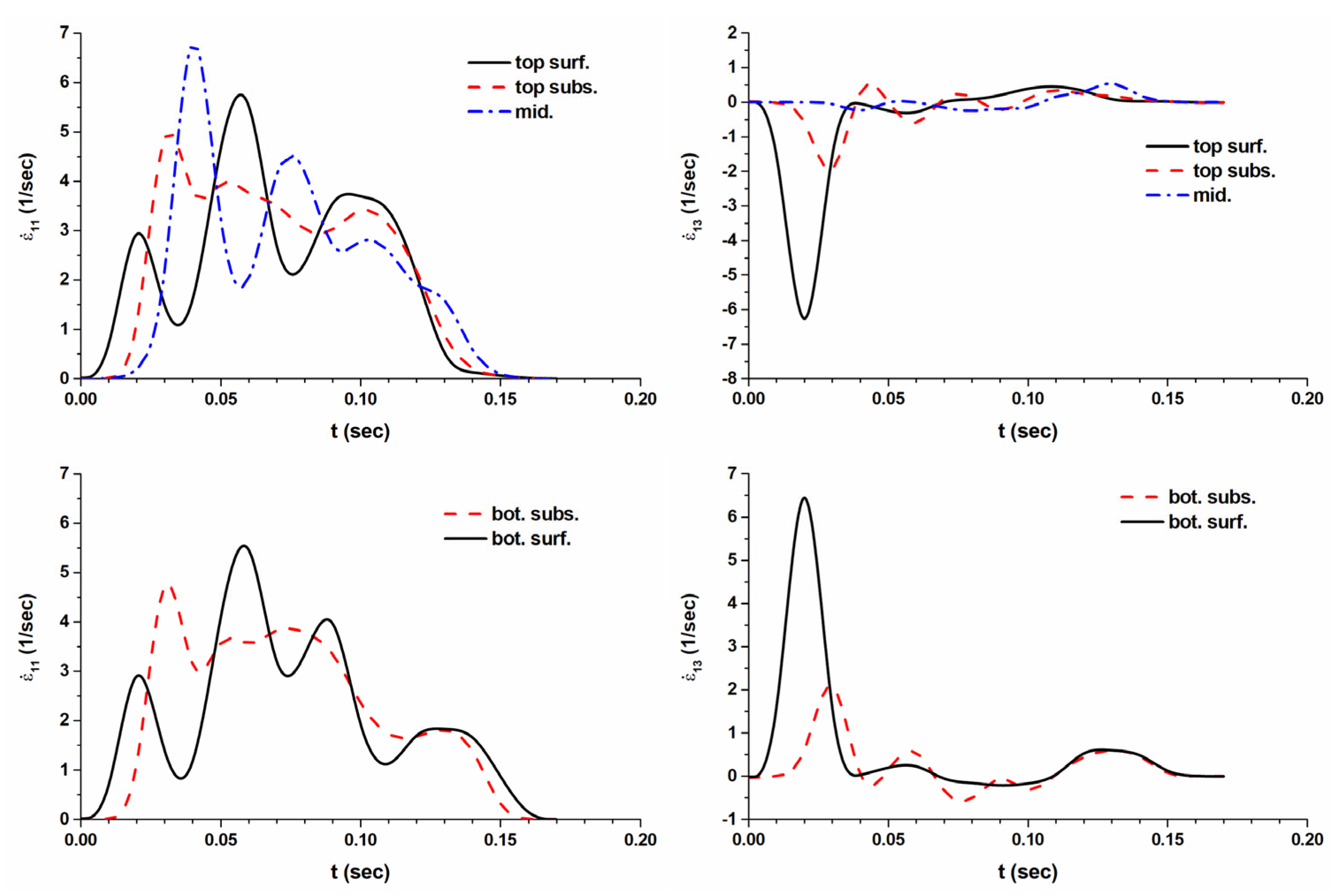
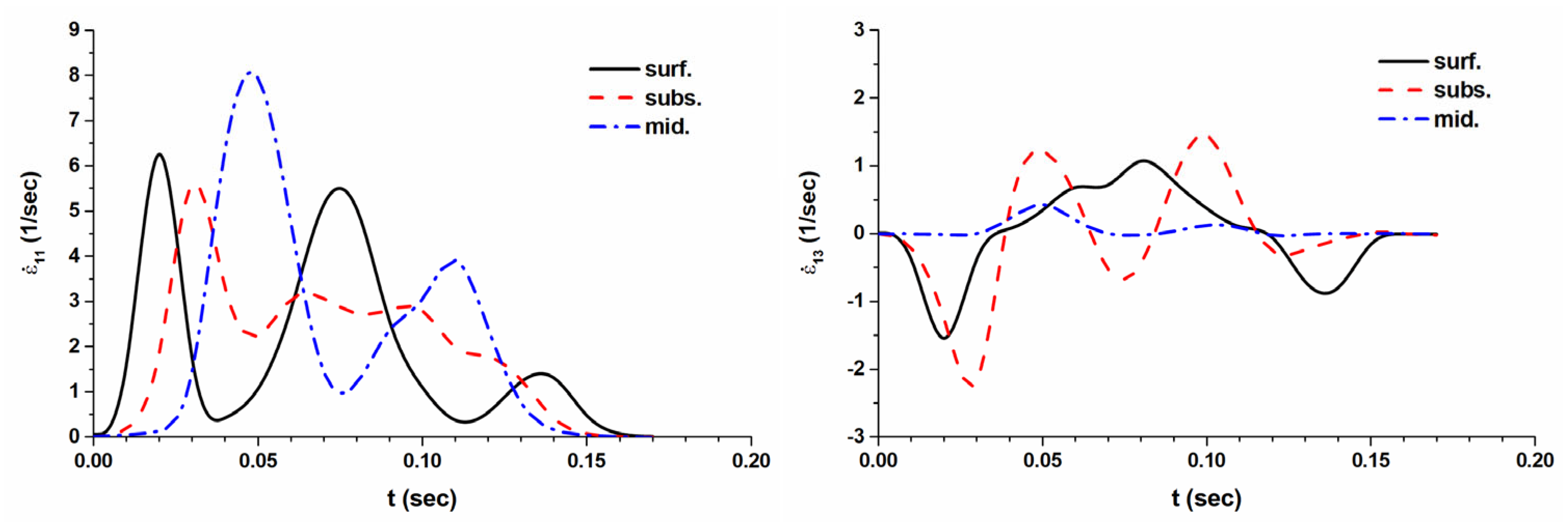
| Description | Parameter | Value | |
|---|---|---|---|
| Asym. | Sym. | ||
| Roll radii | R (mm) | 75 | 75 |
| Initial thickness of the sheet | hi (mm) | 1.92 | 5.02 |
| Final thickness of the sheet | hf (mm) | 1.3 | 3.61 |
| Initial sheet length | L (mm) | 20 | 20 |
| Material | - | Al-1050 | Al-1050 |
| Minimal friction coefficient necessary for rolling | μmin | 0.0672 | 0.118 |
| Angular velocity of the top roll | ωt (rad/s) | 1.1023 | 1.1023 |
| Angular velocity of the bottom roll | ωb (rad/s) | 0.748 | 1.1023 |
| Velocity rate | ωt/ωb | 1.474 | 1.000 |
| Model Parameter | Ramberg–Osgood Model | Hollomon Model |
|---|---|---|
| E (MPa) | 69,854 | 69,519 |
| K (MPa) | 144.56 | 144.58 |
| n | 0.3691 | 0.3703 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bátorfi, J.G.; Pál, G.; Chakravarty, P.; Sidor, J.J. Assessment of Deformation Flow in 1050 Aluminum Alloy by the Implementation of Constitutive Model Parameters. Appl. Sci. 2023, 13, 4359. https://doi.org/10.3390/app13074359
Bátorfi JG, Pál G, Chakravarty P, Sidor JJ. Assessment of Deformation Flow in 1050 Aluminum Alloy by the Implementation of Constitutive Model Parameters. Applied Sciences. 2023; 13(7):4359. https://doi.org/10.3390/app13074359
Chicago/Turabian StyleBátorfi, János György, Gyula Pál, Purnima Chakravarty, and Jurij J. Sidor. 2023. "Assessment of Deformation Flow in 1050 Aluminum Alloy by the Implementation of Constitutive Model Parameters" Applied Sciences 13, no. 7: 4359. https://doi.org/10.3390/app13074359
APA StyleBátorfi, J. G., Pál, G., Chakravarty, P., & Sidor, J. J. (2023). Assessment of Deformation Flow in 1050 Aluminum Alloy by the Implementation of Constitutive Model Parameters. Applied Sciences, 13(7), 4359. https://doi.org/10.3390/app13074359









