Analysis of the Failure Area of the Slope Using the Slip Line Method
Abstract
1. Introduction
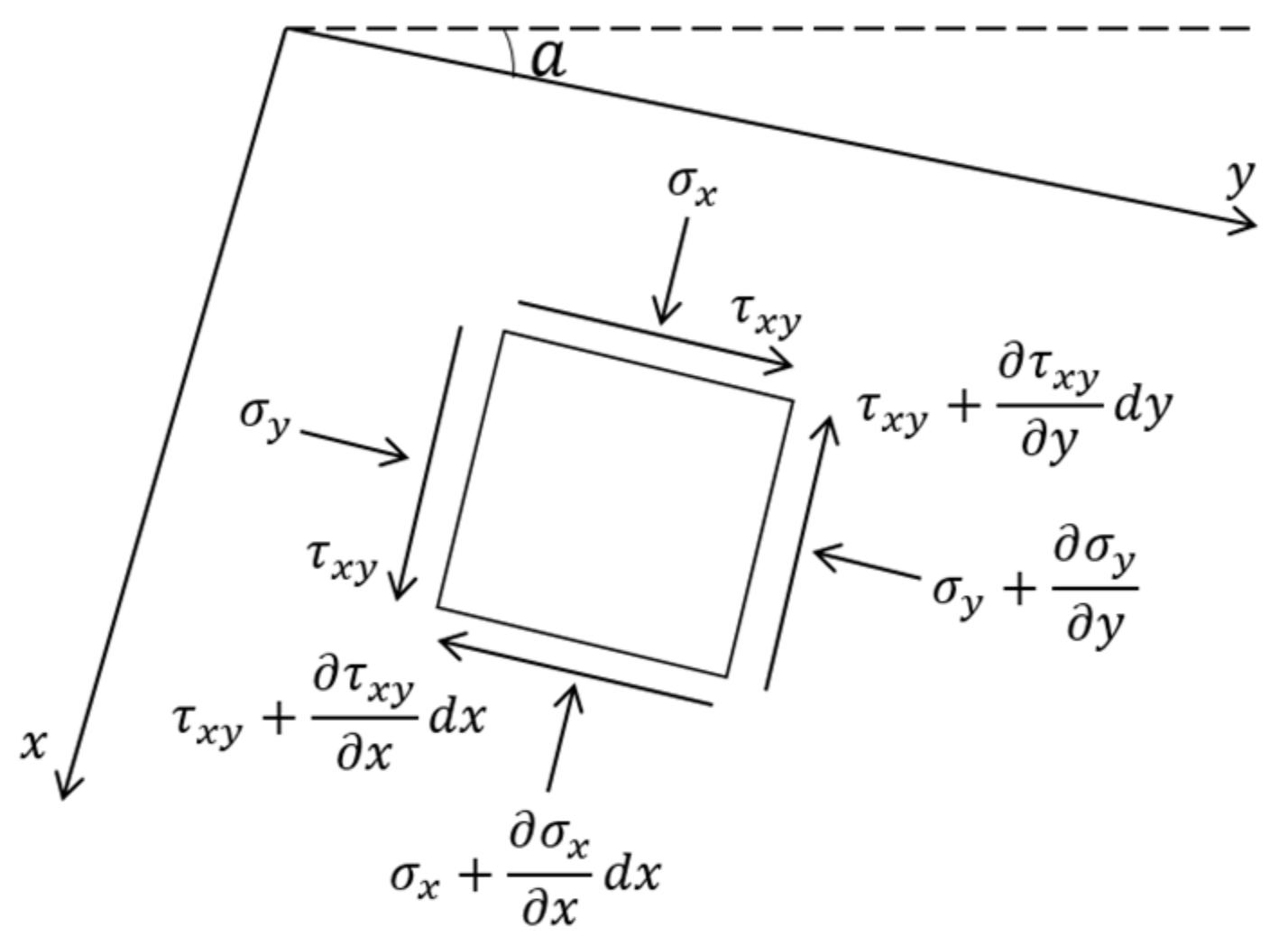
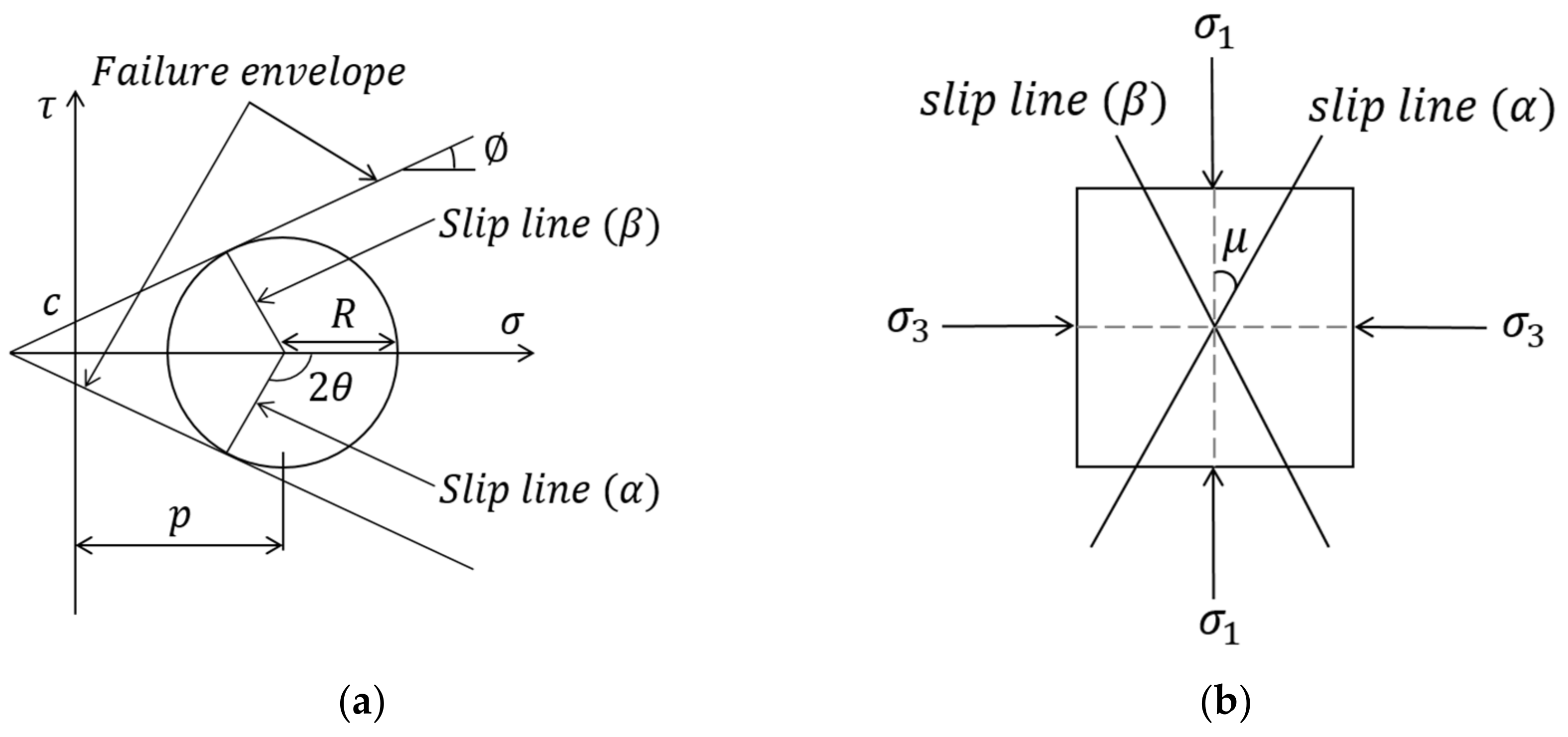
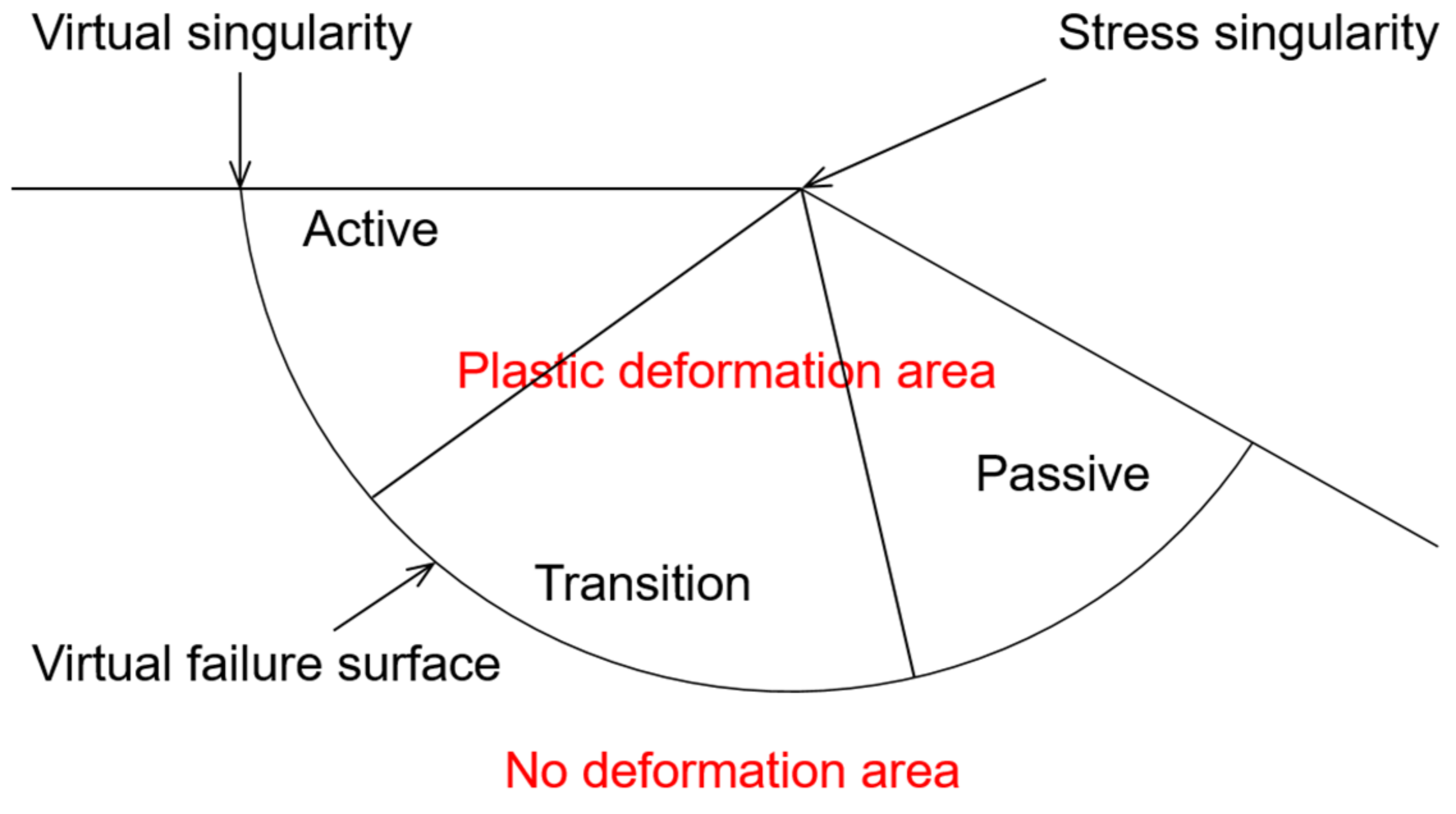
2. Slip Line Method
2.1. Application of Slip Line Method of Coulomb Material
- The soil body is an ideal hard plastic body.
- Mohr–Coulomb yield criteria are followed.
- When a load is applied, the plastic area of the ground can move freely.
- The plastic deformation of the ground is large; therefore, elasticity can be neglected.
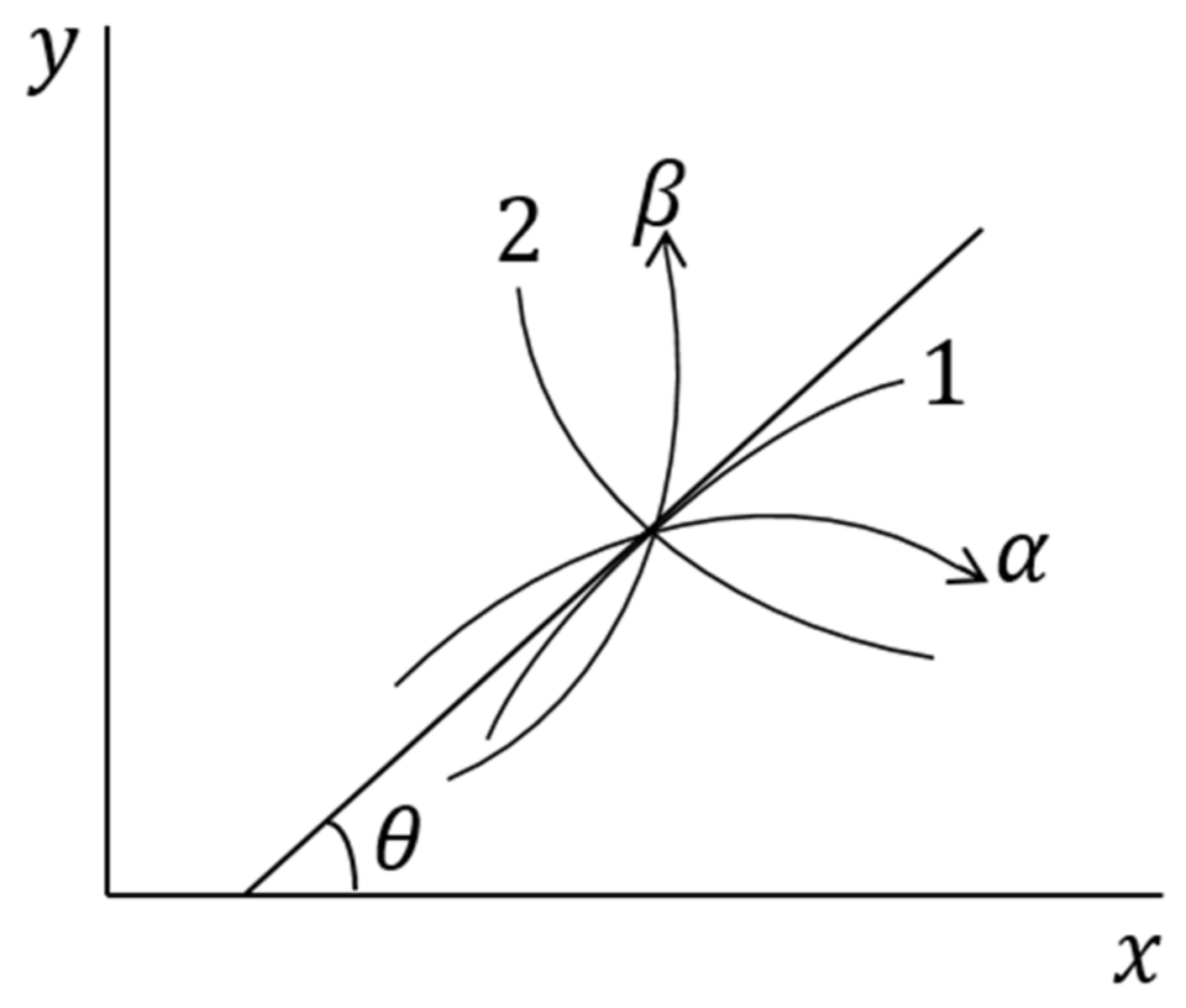
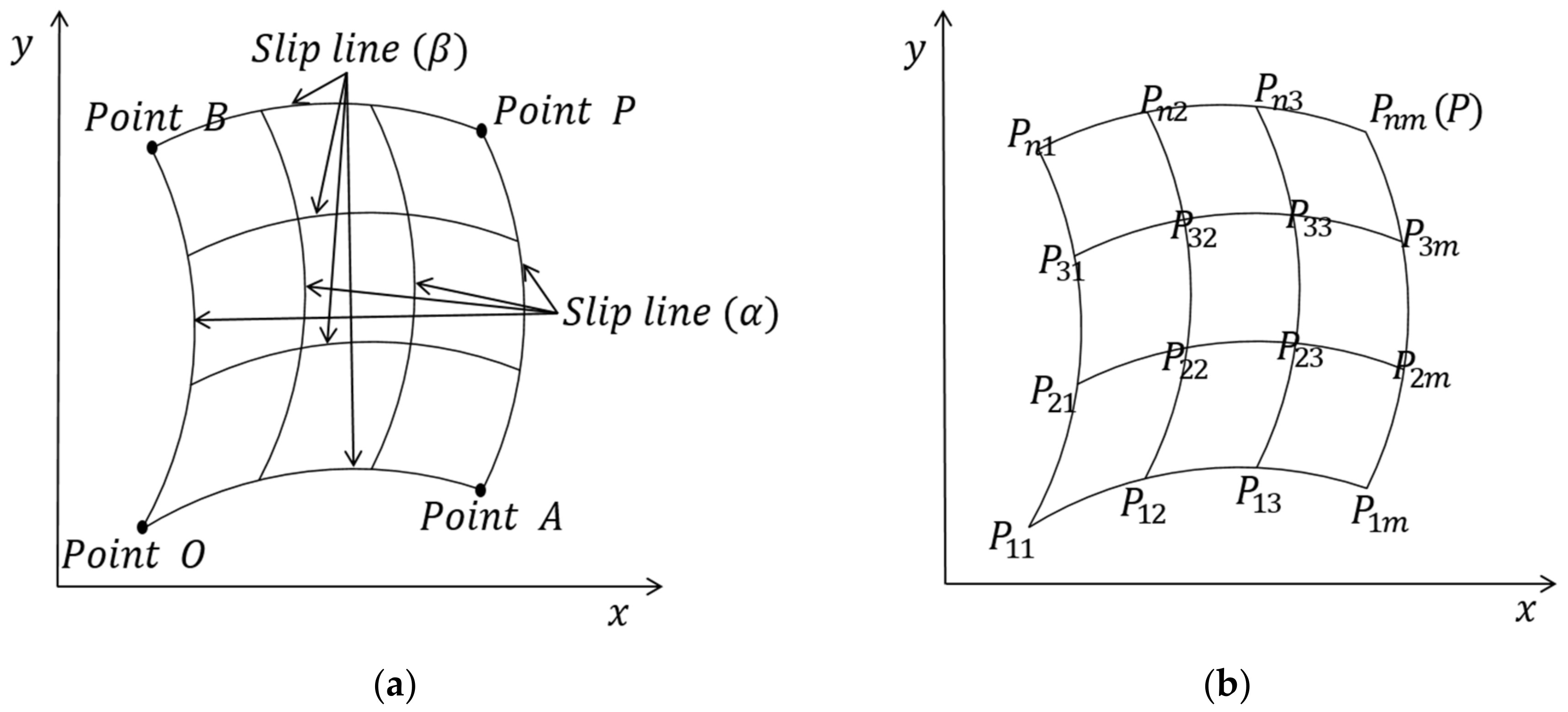
2.2. Recurrence Formula of the Slip Line Method
3. Analysis of Failure Area
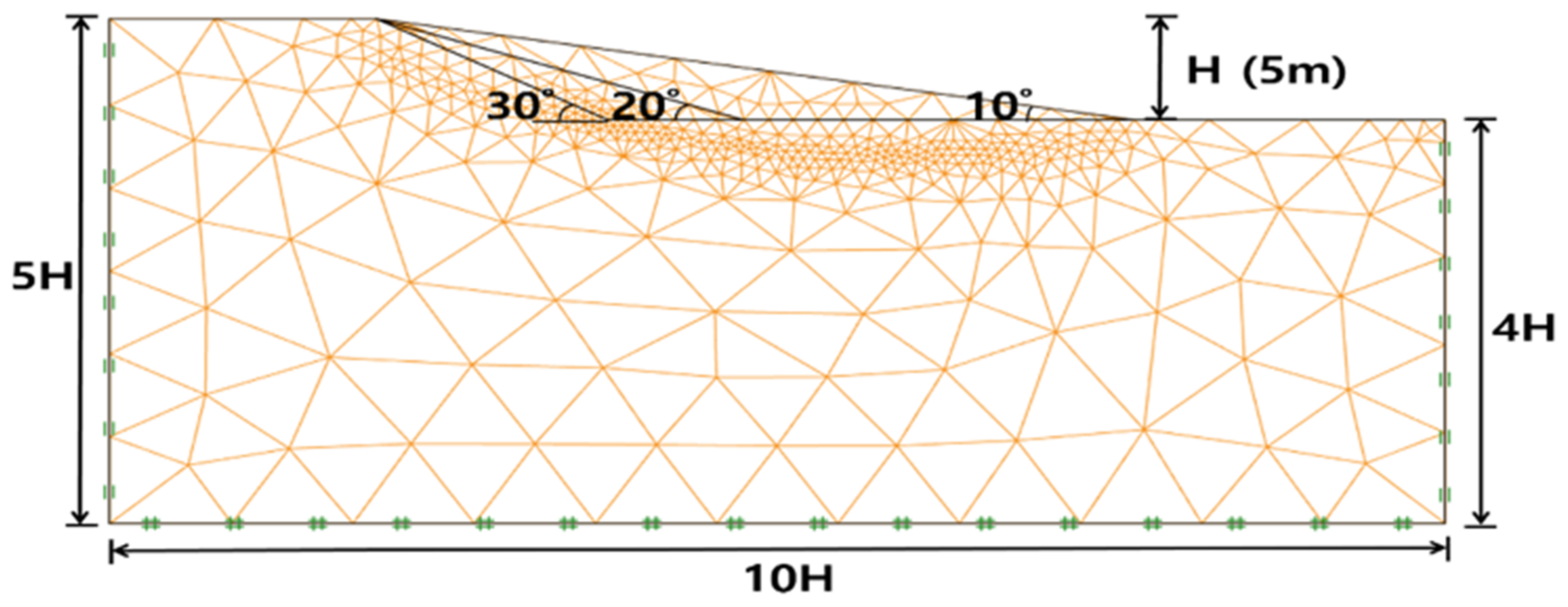
3.1. Analysis Condition of Slope Failure Area
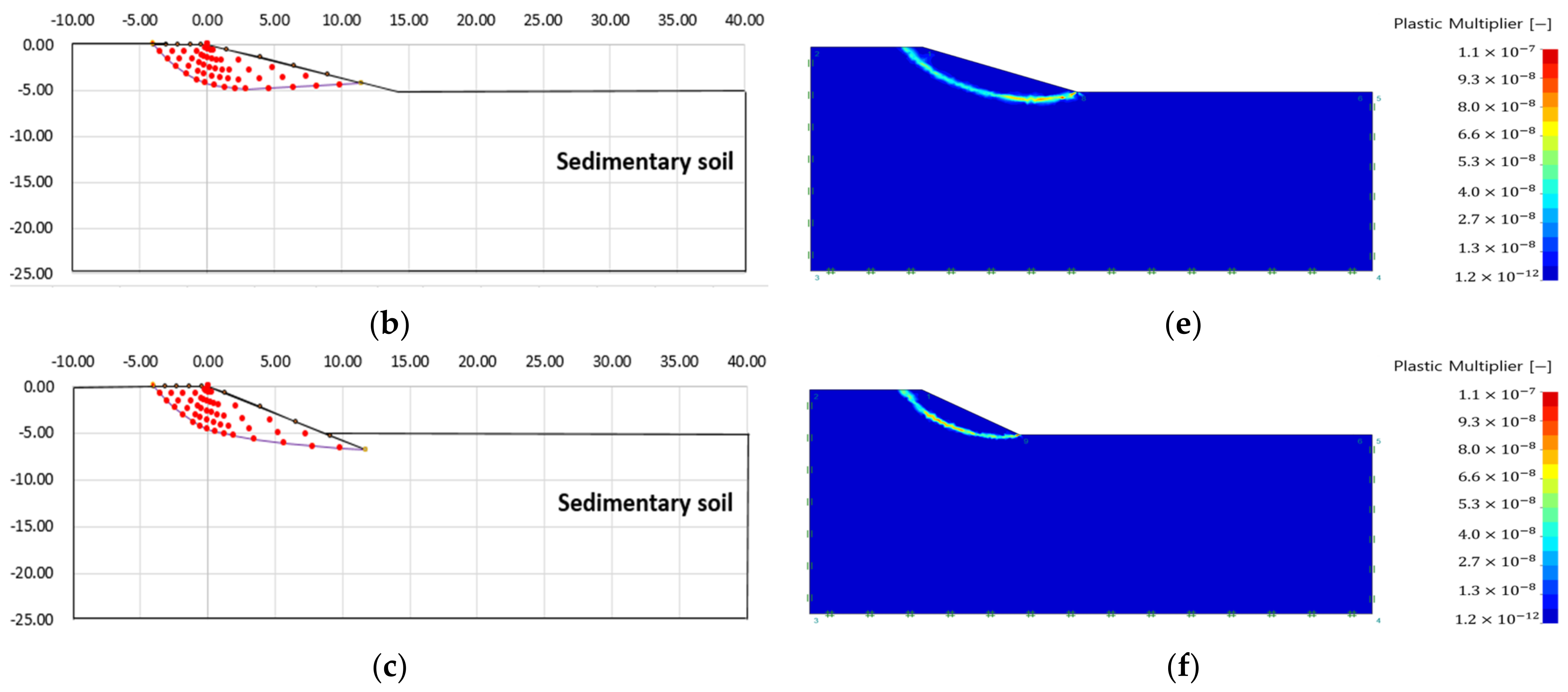
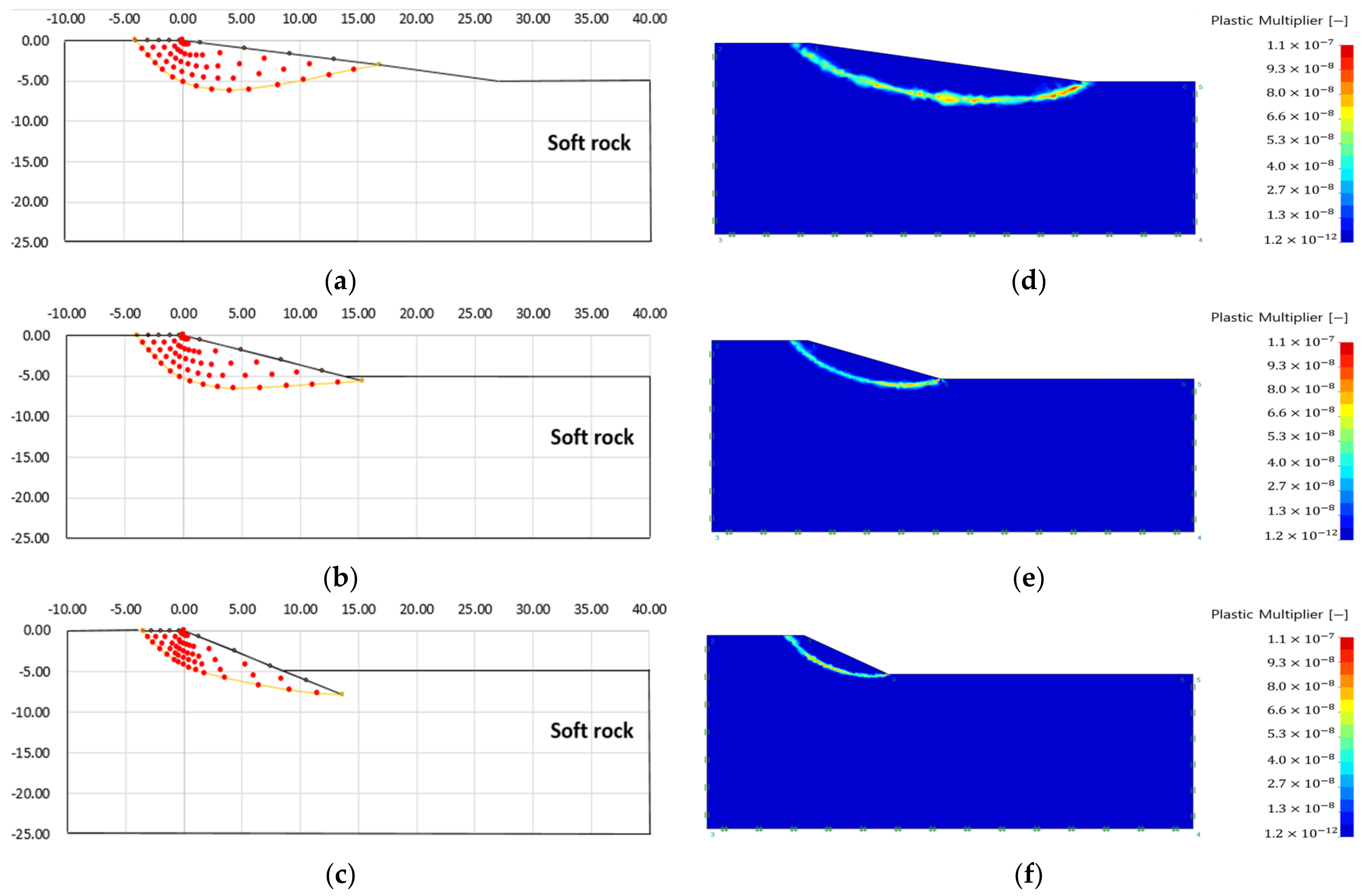
3.2. Analysis of the Failure Area of a Single Ground Slope
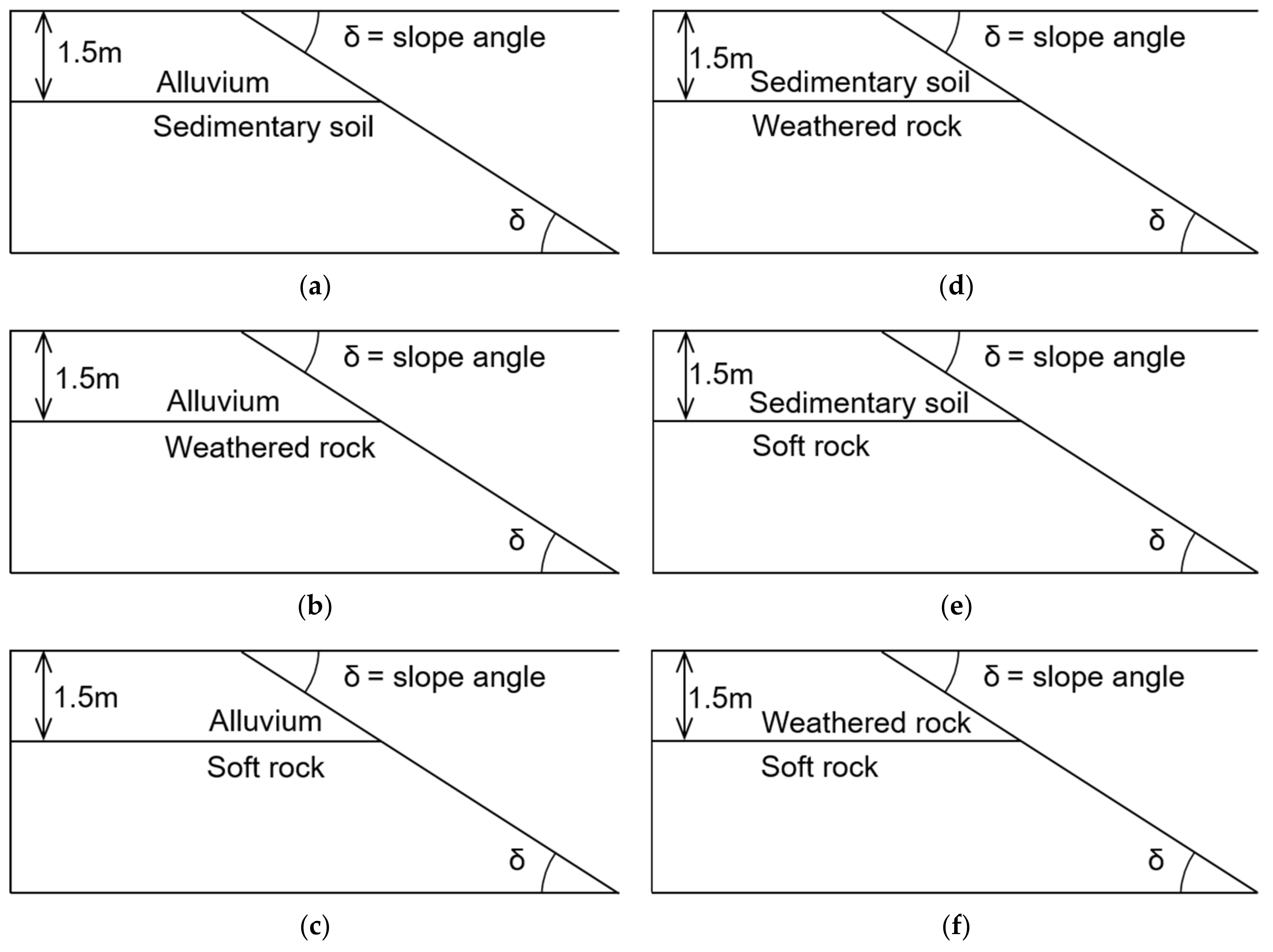
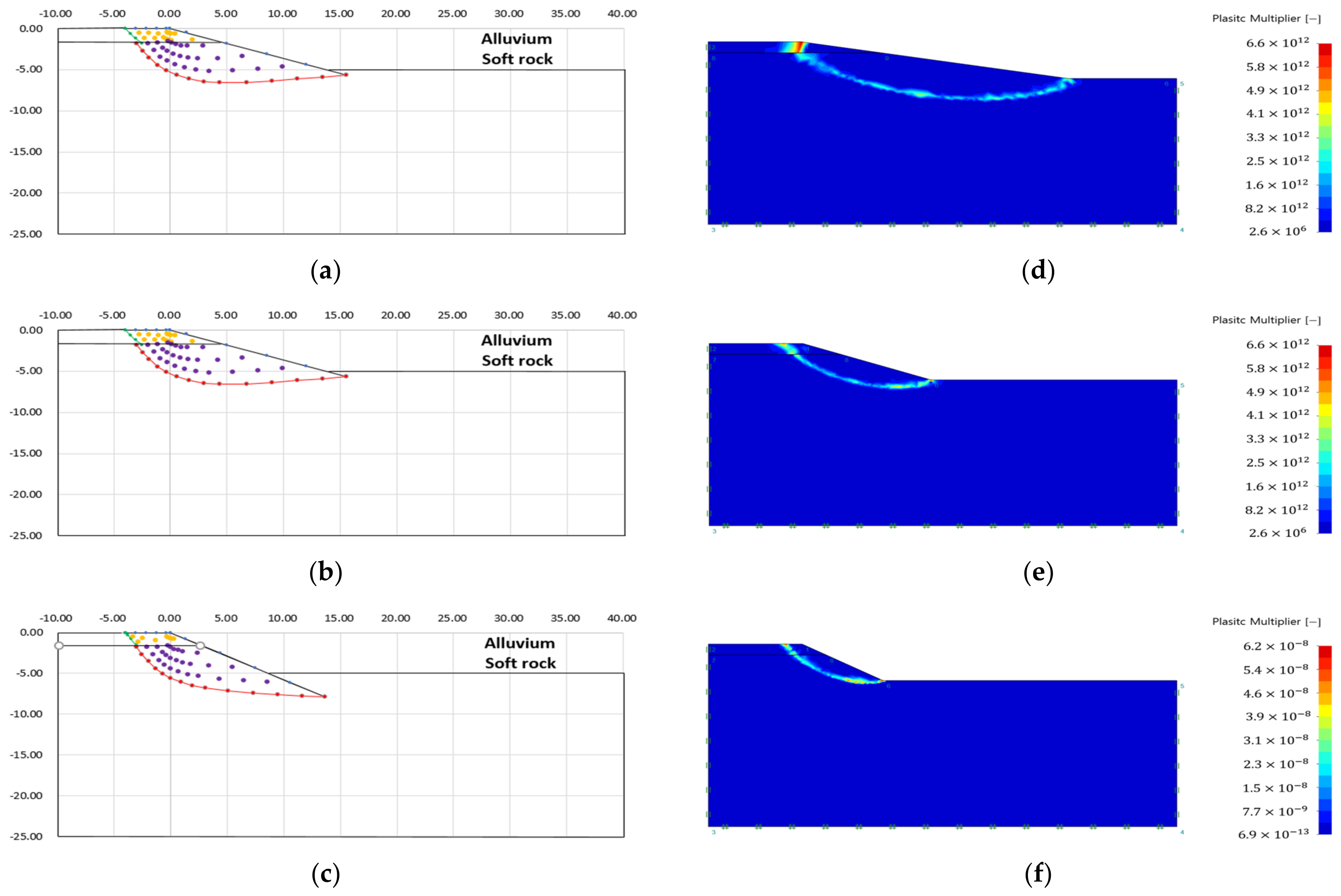
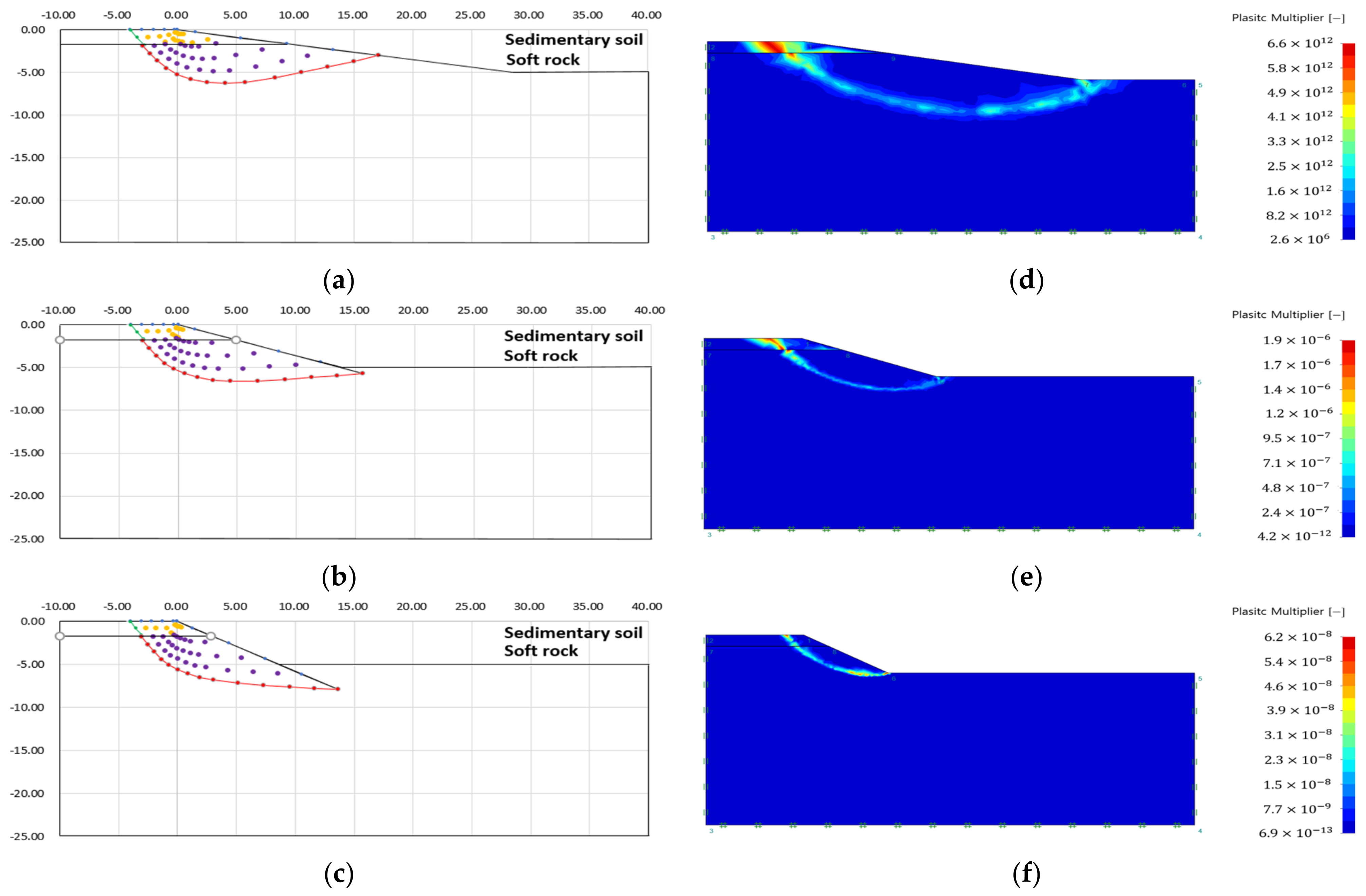
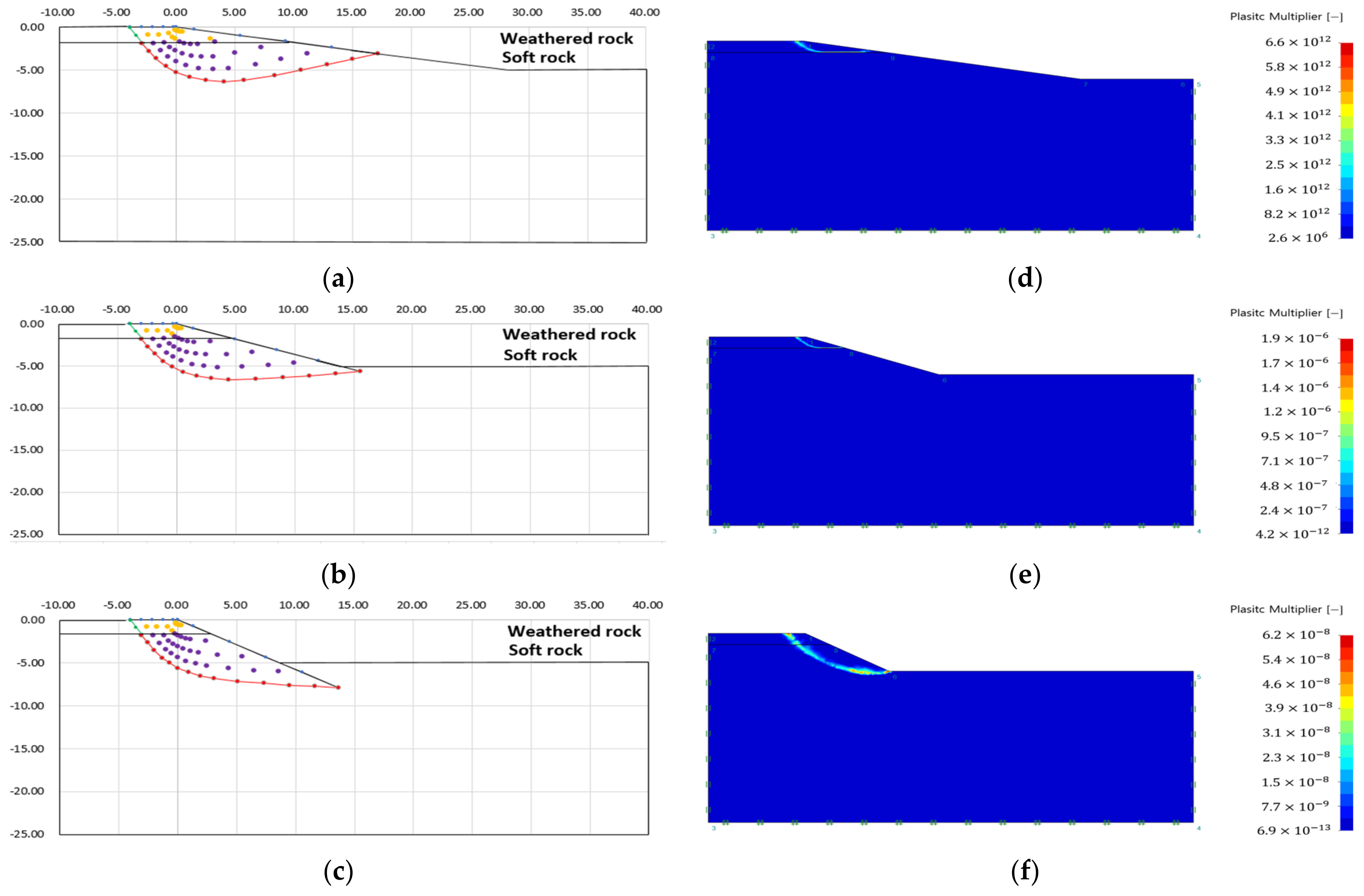
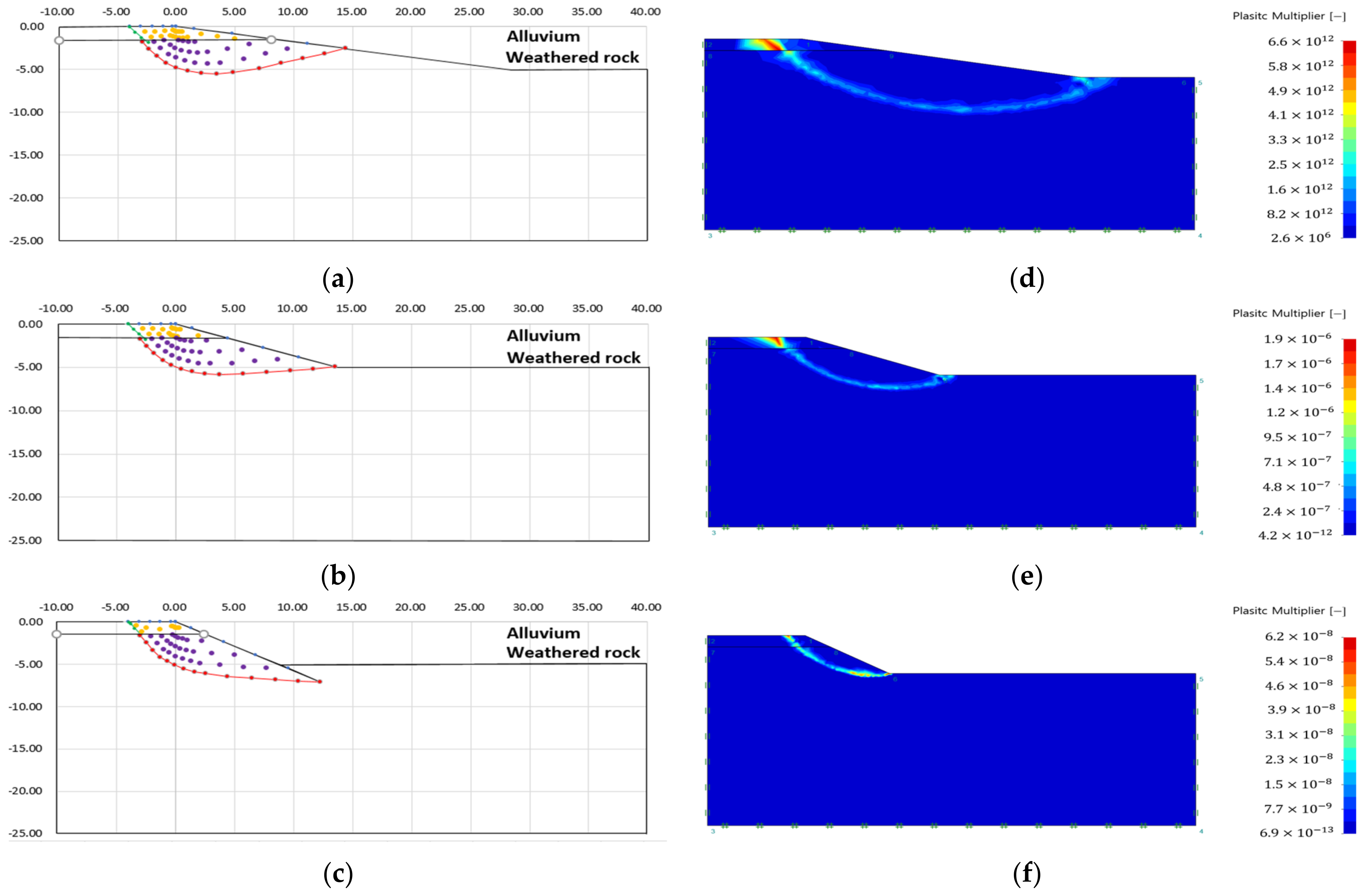
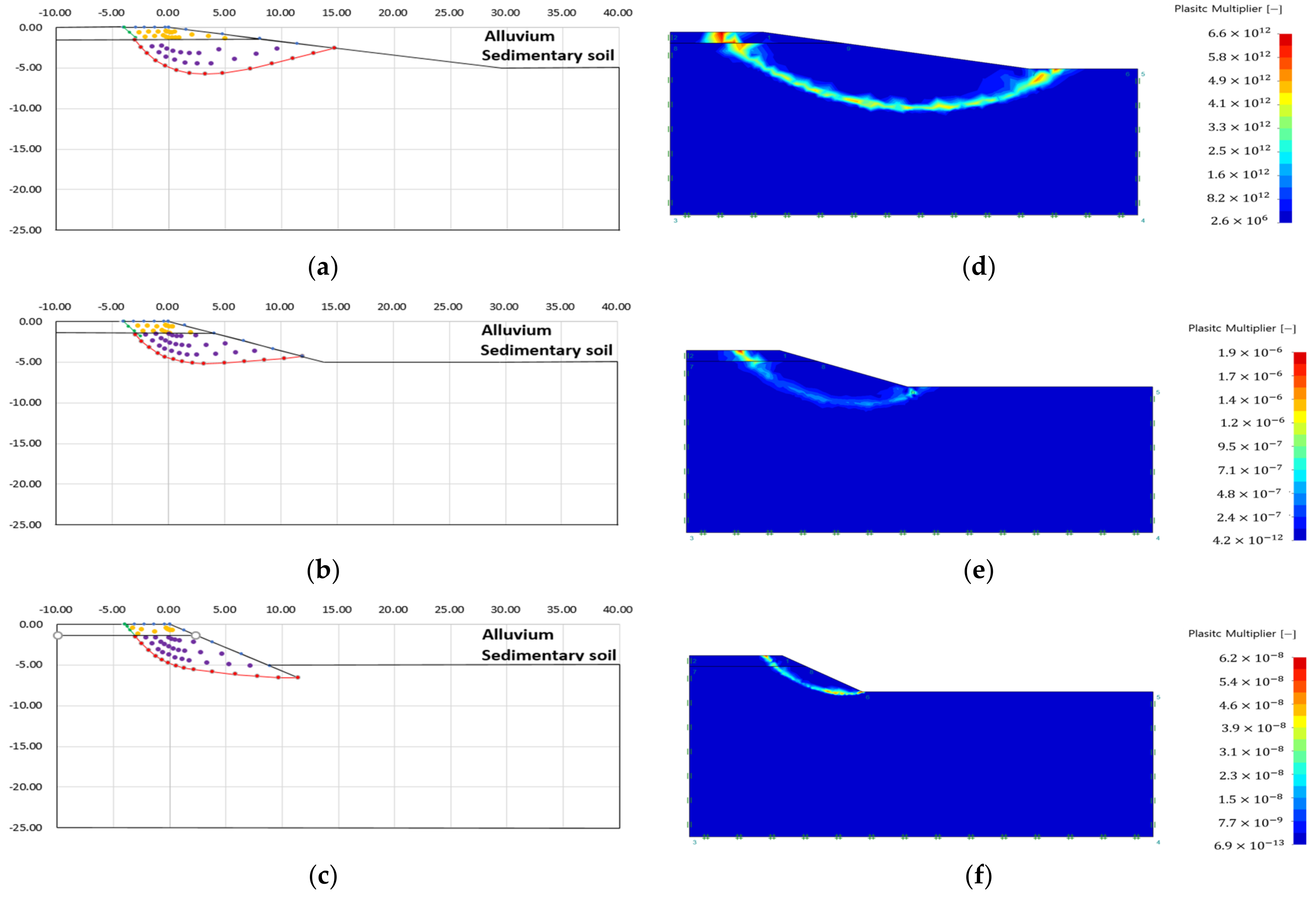
3.3. Analysis of Failure Area of Heterogeneous Ground Slope
- The failure surface of the upper and lower ground changed according to the internal friction angle of each ground. The green line is the failure surface according to the internal friction angle of the upper ground, and the red line is the failure surface according to the internal friction angle of the lower ground.
- There was no significant difference in the failure area, because there is small variation in the internal friction angle according to each analysis condition. However, it could be confirmed that the difference in internal stress was different because of the difference in other ground properties (cohesion, unit weight) (see Table 3).
- 3.
- The plastic area appeared over the entire slope when the difference in ground properties between the upper and lower ground was small, or the slope angle was large.
- 4.
- Case 1, in which the difference in ground properties between the upper and lower ground was the largest, showed a plastic area limited to the upper ground. This is because the upper ground, which had the lowest ground properties, failed before the lower ground, because the strength reduction method was applied without external load.
- 5.
- As with the analysis result of the single ground, there was no stress singularity, such as an external load. Therefore, the failure area was formed at the start and end points of the slope, which were the other stress singular points.
- 6.
- As with the analysis results of the slip line method, it can be seen that the failure surface was discontinuously connected to the ground boundary layer.
4. Conclusions
- The slip line method showed a clear virtual failure surface regardless of the ground strength and slope. In addition, it is possible to calculate the internal stress of the coordinates according to the coordinates (x, y) expressed in the Cartesian coordinate system.
- In the case of finite element limit analysis, the plastic area analyzed by the strength reduction method was similar to the analysis result of the slip line method. However, it should be noted that the strength reduction method reduces the strength of the entire ground properties. Therefore, the plastic area was over-analyzed because the analysis was performed using the strength reduction method in the absence of stress singularities such as load.
- As a result of analyzing the failure area of a single ground, the slip line method increased the scale of the failure surface as the slope of the soft ground (alluvium, sedimentary layer) increased. In the case of finite element limit analysis, it was confirmed that there was no significant difference in the plastic region depending on the properties of the ground and the slope angle.
- As a result of the analysis of the failure area of the heterogeneous ground, the slip line method showed that the shape of the failure surface changed according to the change of the ground characteristic value, and the failure area and the virtual failure surface were clearly expressed regardless of the ground condition and slope angle. In addition, in the case of heterogeneous ground, the upper ground was regarded as a surcharge load and applied to the lower ground for analysis, showing a difference from the analysis results of a single ground.
- In the case of finite element limit analysis, it can be seen that the failure surface is discontinuously connected to the ground boundary layer. In addition, when the difference in soil properties between the upper ground and the lower ground was the greatest, the failure area was limited to the upper ground. This is because the upper ground with the lowest ground properties failed before the lower ground because the strength reduction method was applied without external load. It should be noted that the plastic area was over-analyzed by considering the shape of the slope as a stress singular point, because the analysis was performed using the strength reduction method in the absence of stress singularity such as load.
- The slip line method analyzes the plastic area based on boundary conditions. Since the Riemann problem applied to the slope analysis sets the slip line as the boundary condition, the boundary condition can change. Because of these features, there is a risk that the plastic area and failure surface may be analyzed to be larger than the shape of the slope. Therefore, it is thought that a combination of boundary conditions according to the structure or geotechnical problem is necessary.
- The slip line method can determine the virtual failure surface, failure area (active area, transition area, passive area), and internal stress if only the basic boundary conditions and ground characteristics are given. In addition, in this paper, it was shown that analysis of heterogeneous ground is possible. Through these features, the applicability and development potential of the slip line method to various geotechnical problems or structures were confirmed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bolton, H.; Heymann, G.; Groenwold, A. Global search for critical failure surface in slope stability analysis. Eng. Optim. 2003, 35, 51–65. [Google Scholar] [CrossRef]
- Cheng, Y. Location of critical failure surface and some further studies on slope stability analysis. Comput. Geotech. 2003, 30, 255–267. [Google Scholar] [CrossRef]
- Cheng, Y.; Li, L.; Chi, S.-C.; Wei, W. Particle swarm optimization algorithm for the location of the critical non-circular failure surface in two-dimensional slope stability analysis. Comput. Geotech. 2007, 34, 92–103. [Google Scholar] [CrossRef]
- Kahatadeniya, K.; Nanakorn, P.; Neaupane, K. Determination of the critical failure surface for slope stability analysis using ant colony optimization. Eng. Geol. 2009, 108, 133–141. [Google Scholar] [CrossRef]
- Himanshu, N.; Kumar, V.; Burman, A.; Maity, D.; Gordan, B. Grey wolf optimization approach for searching critical failure surface in soil slopes. Eng. Comput. 2020, 37, 2059–2072. [Google Scholar] [CrossRef]
- Khajehzadeh, M.; Keawsawasvong, S.; Sarir, P.; Khailany, D.K. Seismic Analysis of Earth Slope Using a Novel Sequential Hybrid Optimization Algorithm. Period. Polytech. Civ. Eng. 2022, 66, 355–366. [Google Scholar] [CrossRef]
- Zolfaghari, A.R.; Heath, A.C.; McCombie, P.F. Simple genetic algorithm search for critical non-circular failure surface in slope stability analysis. Comput. Geotech. 2005, 32, 139–152. [Google Scholar] [CrossRef]
- Li, S.; Shangguan, Z.; Duan, H.; Liu, Y.; Luan, M. Searching for critical failure surface in slope stability analysis by using hybrid genetic algorithm. Géoméch. Eng. 2009, 1, 85–96. [Google Scholar] [CrossRef]
- Sengupta, A.; Upadhyay, A. Locating the critical failure surface in a slope stability analysis by genetic algorithm. Appl. Soft Comput. 2009, 9, 387–392. [Google Scholar] [CrossRef]
- Zhang, T.; Zhang, J.-Z. Numerical estimate of critical failure surface of slope by ordinary state-based peridynamic plastic model. Eng. Fail. Anal. 2022, 140, 106556. [Google Scholar] [CrossRef]
- Li, W.; Zhang, C.; Zhang, D.; Ye, Z.; Tan, Z. Face stability of shield tunnels considering a kinematically admissible velocity field of soil arching. J. Rock Mech. Geotech. Eng. 2022, 14, 505–526. [Google Scholar] [CrossRef]
- Zhang, C.; Han, K.; Zhang, D. Face stability analysis of shallow circular tunnels in cohesive–frictional soils. Tunn. Undergr. Space Technol. 2015, 50, 345–357. [Google Scholar] [CrossRef]
- Tu, S.; Li, W.; Zhang, C.; Chen, W. Effect of inclined layered soils on face stability in shield tunneling based on limit analysis. Tunn. Undergr. Space Technol. 2023, 131, 104773. [Google Scholar] [CrossRef]
- Shiau, J.; Lai, V.Q.; Keawsawasvong, S. Multivariate adaptive regression splines analysis for 3D slope stability in anisotropic and heterogenous clay. J. Rock Mech. Geotech. Eng. 2022. [Google Scholar] [CrossRef]
- Yodsomjai, W.; Keawsawasvong, S.; Thongchom, C.; Lawongkerd, J. Undrained stability of unsupported conical slopes in two-layered clays. Innov. Infrastruct. Solutions 2020, 6, 15. [Google Scholar] [CrossRef]
- Yodsomjai, W.; Keawsawasvong, S.; Likitlersuang, S. Stability of Unsupported Conical Slopes in Hoek-Brown Rock Masses. Transp. Infrastruct. Geotechnol. 2020, 8, 279–295. [Google Scholar] [CrossRef]
- Lai, V.Q.; Lai, F.; Yang, D.; Shiau, J.; Yodsomjai, W.; Keawsawasvong, S. Determining Seismic Bearing Capacity of Footings Embedded in Cohesive Soil Slopes Using Multivariate Adaptive Regression Splines. Int. J. Geosynth. Ground Eng. 2022, 8, 46. [Google Scholar] [CrossRef]
- Martin, C.M. User Guide for ABC—Analysis of Bearing Capacity (Version 1.0); OUEL Report No. 2261/03; University of Oxford: Oxford, UK, 2003. [Google Scholar]
- Gao, F.-P.; Wang, N.; Zhao, B. A general slip-line field solution for the ultimate bearing capacity of a pipeline on drained soils. Ocean Eng. 2015, 104, 405–413. [Google Scholar] [CrossRef]
- Liu, D.; Chen, X. Shearing characteristics of slip zone soils and strain localization analysis of a landslide. Géoméch. Eng. 2015, 8, 33–52. [Google Scholar] [CrossRef]
- Keshavarz, A.; Fazeli, A.; Sadeghi, S. Seismic bearing capacity of strip footings on rock masses using the Hoek–Brown failure criterion. J. Rock Mech. Geotech. Eng. 2016, 8, 170–177. [Google Scholar] [CrossRef]
- Zhou, A.; Li, C.; Jiang, P.; Yao, K.; Li, N.; Wang, W. Slip Line Theory Based Stability Analysis on the Influence of Deep Excavation on Adjacent Slope. Math. Probl. Eng. 2018, 2018, 2041712. [Google Scholar] [CrossRef]
- Tsegaya, A.B. Bearing capacity of shallow foundations that are situated at a varying distance from slopes. In Proceedings of the XVII European Conference ESCMGE-2019, Reykjavik, Iceland, 1–6 September 2019. [Google Scholar]
- Park, S.Y.; Han, H.S. Earth Pressure Analysis of Tunnel Ceiling according to Tunnel Plastic Zone. J. Korea Acad. -Ind. Coop. Soc. 2020, 21, 753–764. [Google Scholar] [CrossRef]
- Lee, Y.K. Calculation of Failure Load of V-shaped Rock Notch Using Slip-line Method. Tunn. Undergr. Space 2020, 30, 404–416. [Google Scholar] [CrossRef]
- Hill, R. The Mathematical Theory of Plasticity; Clarendon Press & Oxford: Oxford, UK, 1950. [Google Scholar]
- Sokolovskii, V.V.; Kushner, J.K. Statics of Granular Media. J. Appl. Mech. 1966, 33, 239. [Google Scholar] [CrossRef]
- Wang, R.; Skirrow, R.K.; Jianping, S. Slip line soiltion by spreadsheet. In Proceedings of the GeoEdmonton’08: 61st Canadian Geotechnical Conference and 9th Joint CGS/IAH-CNC Groundwater Conference, Edmonton, AB, Canada, 21–24 September 2008. [Google Scholar]
- Soilworks. SoilWorks Ground Property Database. MIDAS IT Co., Ltd., 2022. Available online: https://www.midasuser.com (accessed on 12 July 2022).







| Ground Property | Alluvium | Sedimentary Soil | Weathered Rock | Soft Rock |
|---|---|---|---|---|
| Cohesion (c, kPa) | 15 | 17.5 | 50 | 100 |
| Unit Weight () | 17 | 18.5 | 21 | 24 |
| Inner friction angle ( | 20 | 31 | 33 | 35.5 |
| Ground | Alluvium | Sedimentary Soil | Weathered Rock | Soft Rock | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Property | |||||||||||||
| Slope angle () | 10 | 20 | 30 | 10 | 20 | 30 | 10 | 20 | 30 | 10 | 20 | 30 | |
| Failure area depth (m) | 3.36 | 3.81 | 7.36 | 4.46 | 4.83 | 6.76 | 5.31 | 5.59 | 7.06 | 6.17 | 6.5 | 7.86 | |
| Failure area length (m) | 7.25 | 8.18 | 12.8 | 11.4 | 11.5 | 11.7 | 13.9 | 13.2 | 12.2 | 16.8 | 15.4 | 13.6 | |
| Maximum internal stress (kPa) | 296.16 | 203.19 | 130.36 | 1038.51 | 677.26 | 401.20 | 2200.07 | 1478.94 | 952.53 | 4476.83 | 3016.08 | 1985.89 | |
| Ground | Case 1 | Case 2 | Case 3 | Case 4 | Case 5 | Case 5 | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Property | |||||||||||||||||||
| Slope angle () | 10 | 20 | 30 | 10 | 20 | 30 | 10 | 20 | 30 | 10 | 20 | 30 | 10 | 20 | 30 | 10 | 20 | 30 | |
| Failure area depth (m) | 6.29 | 6.6 | 7.89 | 6.29 | 6.62 | 7.90 | 6.3 | 6.63 | 7.90 | 5.77 | 5.18 | 6.60 | 5.51 | 5.85 | 7.08 | 5.53 | 5.85 | 7.08 | |
| Failure area length (m) | 17.1 | 15.6 | 13.7 | 17.1 | 15.6 | 13.7 | 17.2 | 15.6 | 13.7 | 14.7 | 11.9 | 11.4 | 14.4 | 13.5 | 12.3 | 14.5 | 13.5 | 12.3 | |
| Maximum internal stress (kPa) | 4967.76 | 3386.2 | 2267.53 | 5007.52 | 3420.49 | 2292.87 | 5078.84 | 3472.64 | 2333.81 | 2910.29 | 936.70 | 605.46 | 2587.84 | 1776.37 | 1183.25 | 2621.87 | 1801.17 | 1203.34 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shin, J.; Baek, Y.; Song, J. Analysis of the Failure Area of the Slope Using the Slip Line Method. Appl. Sci. 2023, 13, 3863. https://doi.org/10.3390/app13063863
Shin J, Baek Y, Song J. Analysis of the Failure Area of the Slope Using the Slip Line Method. Applied Sciences. 2023; 13(6):3863. https://doi.org/10.3390/app13063863
Chicago/Turabian StyleShin, JunWoo, Yong Baek, and JungHo Song. 2023. "Analysis of the Failure Area of the Slope Using the Slip Line Method" Applied Sciences 13, no. 6: 3863. https://doi.org/10.3390/app13063863
APA StyleShin, J., Baek, Y., & Song, J. (2023). Analysis of the Failure Area of the Slope Using the Slip Line Method. Applied Sciences, 13(6), 3863. https://doi.org/10.3390/app13063863






