Method for Theoretical Assessment of Safety against Derailment of New Freight Wagons

Abstract

The assessment of safety against freight wagons’ derailment is a mandatory element of the documents provided to the EU notifying authorities of the entry into service of new freight wagons. The assessment methodology is presented in EN 14363:2019. It is mainly aimed at the experimental measurement of certain parameters, and the data are used to calculate the safety criterion. The practical implementation of the tests is accompanied by many difficulties: finding a track with a proper radius, ensuring free access to the railway infrastructure for a long period of time, waiting for suitable metrological conditions, preparing the curve and the test wagon, etc. These difficulties are well known to the European legislators, and as a solution, they propose a large set of reference wagons that have undergone real tests. It is sufficient to demonstrate that the parameters of the new wagon relate to some of the reference wagon parameters to avoid such a requirement. Proving the “convergence” of the parameters of the new and the reference wagons is a lengthy, complex, and, in many cases, subjective process. To introduce an objective assessment, the authors set themselves the task of developing a theoretical method to assess safety against derailment.

1. Introduction

The methods for assessing safety against derailment for new vehicle acceptance are mostly performed using some combination of testing and theoretical mathematical calculations or simulations. Most methods are based on dynamic tests, which can be performed as controlled tests in a laboratory or as long runs under representative service conditions or under the described test conditions, using exactly defined track characteristics and statistical assessment methods. All three test types have their advantages and disadvantages, as described in [1].

There are many methods used for evaluating safety against derailments worldwide. A good overview of these methods is given in [1]. Theoretical mathematical calculations have a long tradition since the first attempts from Hertz [2], Klingel [3], and Nadal [4] to mathematically describe the processes occurring in the wheel–rail interface. In particular, the Nadal criterion [4] sets the basics for further developing theoretical methods to assess safety against derailment [5,6,7,8]. Today, it is applied in different international and European normative documents [9,10].

On the other hand, the experimental measurement of certain parameters relevant to derailment safety is still a standard procedure for approving new freight wagons for operational commissioning. The measured values of these parameters are then used to calculate the safety criterion; if the limit values are not exceeded, the new wagon is approved. The performance of these tests is strictly regulated by international and European authorities in standards and regulatory works [9,10]. Even if these test procedures and conditions are strictly described, some new approaches and methods for measuring forces and displacements are used nowadays [11,12]. However, the practical implementation of the tests is accompanied by many difficulties: finding a track with a proper radius, ensuring free access to the railway infrastructure for a long period of time, waiting for suitable metrological conditions, preparing the curve and the test wagon, etc. For the wagon manufacturers, these difficulties mean long-term approval procedures and higher expenses. This is why most wagon manufacturers attempt to avoid dynamic tests since this is possible according to EN 14363:2019 [9]. The required evidence and procedure for acceptance are well defined in this European standard, and, thus, it is possible to compare the resulting parameters from the theoretical calculations or simulations of safety against derailment with parameters of reference wagons that have undergone real tests. This approach is allowed according to clause 6.1.5.2.6 of EN 14363:2019 [9] when using Method 2 for the assessment. This method is described in detail in Section 2 of this paper.

When using Method 2 of EN 14363:2019 [9], it is necessary to determine the vertical and transversal forces acting in wheel–rail contact. In tests, these parameters are measured directly. It is not explicitly pointed out which theoretical method or type of simulation should be used. The only condition is that the method used for calculation or simulation delivers sufficiently reliable results that are close enough to the results from dynamic tests. Depending on the mechanism that causes derailment of wagons, many methods that determine forces and displacements exist. As mentioned above, a very good overview of these methods is given in [1].

Many methods are based on the flange climb in the wheel–rail contact [13,14,15,16], which is the most frequent reason for derailment. Many researchers in this field deal with geometrical properties of wheel–rail contact on straight tracks or in curves [13,15], including dynamic properties of suspension, while others propose methods to control these processes [14,16].

Some researchers deal with derailment caused by other mechanisms: passing through an S-shaped curve [17], passing through turnouts and switches [18], or due to track failure [19]. There is also research on the effects of wind loads on dynamic behavior and derailment of the train composition [20]. New methodologies are also presented with some non-standard approaches, like a response surface methodology [21].

Over the last 70 years, computer development has allowed their very intense use in numerical and multibody simulations, as well as in testing and analysis techniques. There is much research using multibody dynamic approaches to assess safety against derailment [22,23]. Also, other types of simulations are used for this purpose [24,25]. Many simulation methods have their advantages and problems [1,24,25,26]. The main issue for simulations and theoretical calculations in the last decades is that a vehicle model must be validated in tests; it is still unclear what can be determined as a validated model. In [10], computer simulations were introduced to supplement or replace testing and to be used as an alternative assessment method. The same thing happened in the last version of [9], but with some restrictions regarding the validation procedure: in Europe, for every new freight wagon that should enter the service across European railways, mandatory documents provided to the EU notifying authorities is an assessment of safety against derailment. According to Method 2 of EN 14363:2019 [9], it is sufficient to demonstrate that the parameters of the new wagon relate to some of the reference wagon parameters to avoid such a requirement. Proving the “convergence” of the parameters of the new and the reference wagons is a lengthy, complex, and, in many cases, subjective process.

The final decision is met by the notified bodies, independent of the method used for theoretical calculations or simulations. The notified body will accept a new freight wagon for commissioning if safety against derailment is proven and assessed with appropriate methods or simulation. Moreover, the multibody approaches and different simulations and theoretical methods can be very complex, as well as costly and time-consuming. From the perspective of the wagon manufacturers, the used method must be objective enough, fast in fulfillment, not expensive, and capable of delivering reliable results.

This work introduces an objective theoretical method to assess safety against derailment. Consequently, it is possible to shorten the time and the costs of the approval procedure. The results obtained from theoretical analyses, as described in Section 3, were compared and validated with results from experimental tests carried out on real objects to achieve a sufficient degree of validation.

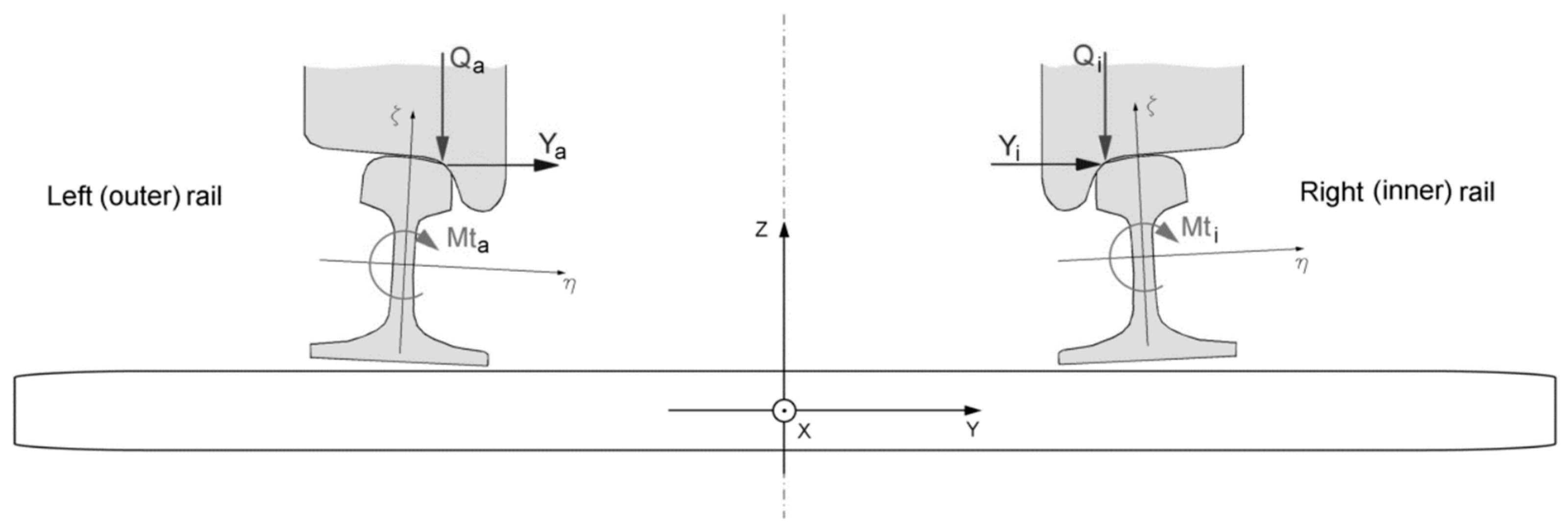
2. Method for Assessment of Theoretical Safety against Freight Wagon Derailment

In accordance with EN 14363:2019 [9], Section 6.1, Method 2 (paragraph 6.1.5.2), when analyzing the safety criterion against derailment, Equation (1) must be considered:

$${\left(\frac{Y}{Q}\right)}_{\mathit{ja}}=\frac{Y_{\mathit{ja}}}{Q_{\mathit{jk},\min }{+\Delta Q}_{\mathit{jH}}}; \frac{Y_{\mathit{ja}}}{Q_{\mathit{jk},\min }{+\Delta Q}_{\mathit{jH}}}{\le \left(\frac{Y}{Q}\right)}_{\lim }$$

(1)

where Y_ja is the reaction of the rail at its contact with the attacking wheel, Q_jk,_min is the lowest value of the vertical reaction of the wheel calculated when the frame of the wagon is twisted, and ΔQ_jH is a load on the wheel from the moment of the forces acting on the 2 wheels of the examined wheel axle (Figure 1). The value of (Y/Q)_lim is equal to 1.2, and the railway vehicle is safe against derailment if (Y/Q)_ja ≤ 1.2.

The component ΔQ_jH is determined using Equation (2):

$$\mathsf{\Delta }{Q}_{\mathit{jH}}=(Y_{\mathit{ja}}+Y_{\mathit{ji}}).\frac{h}{2b_0}$$

(2)

where Y_ja is the total reaction of the rail at its contact with the attacking (outer) wheel; h is the effective height above the rail of the journal box suspension (for the most commonly used bogie, Y25, h = 365 mm is assumed); Y_ji is horizontal load force between the inner (non-attacking) wheel of the examined axle and the inner rail (Figure 1); 2b₀ is the nominal transverse distance between the contact points of the wheels (2b₀ = 1500 mm is assumed); j is index (number) of the examined axle; a is index (number) of the outer wheel; and i is index of the inner wheel.

For the theoretical assessment of the criterion from Equation (1), it is necessary to apply appropriate methods for the theoretical determination of the following parameters:

• Y_ja—the total reaction of the rail in contact with the attacking (outer) wheel. The parameter is involved in Equations (1) and (2).
• Y_ji—the horizontal load force between the inner (non-attacking) wheel of the examined track axle and the inner rail. The parameter is involved in Equation (2).
• Q_jk,min—the lowest value of the vertical reaction of the wheel calculated when the frame of the wagon is twisted. The parameter is involved in Equation (1).

The methods for determining these three important parameters are given in the next three subsections and represent the main goal of our paper.

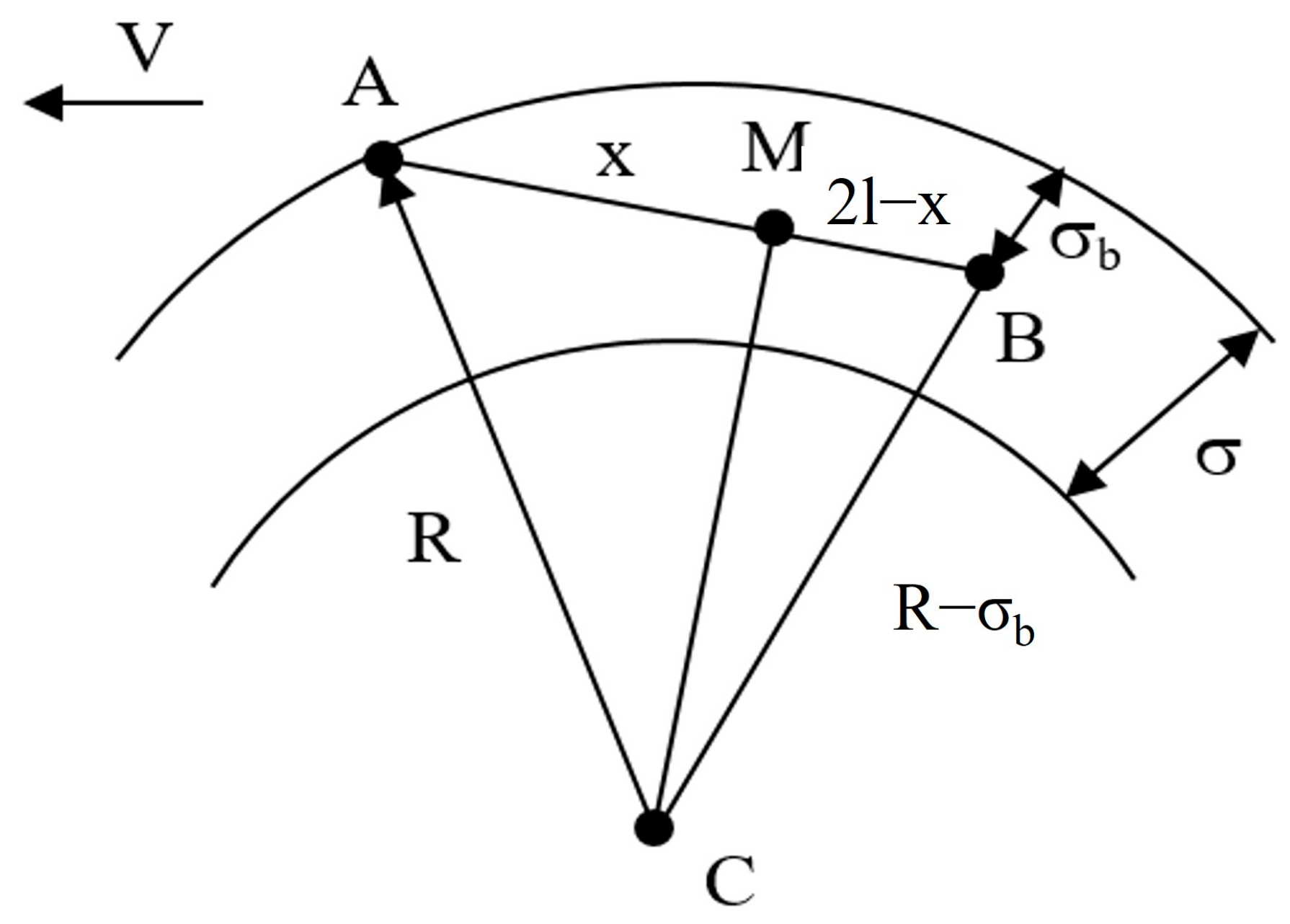
2.1. Methodology for Theoretical Determination of Leading Forces, Y_a, on Axles of Railway Vehicles with Bogies

In [27], a theoretical method for determining the total reaction of the rail on the wheels of a bogie is proposed. Briefly, the method consists of the following: when moving on a curved section of the rail track, the wagon performs two movements—translational and rotational. The rotation occurs around the center of rotation, M (Figure 2), characterized by the pole distance, x, which can be determined using Equation (3):

$$x= l+\frac{R{.\mathsf{\sigma }}_b}{2.l}$$

(3)

where 2.l is the distance between wheelsets of the bogie (for compliance with European standards, it should be noted that 2.l = 2a⁺), R is the radius of the calculation curve, and σ_b is the current coordinate.

The current coordinate, σ_b, depends on the position of the bogie when passing a curved section of the track. In Figure 3, the bogie is represented by section AB (AB′ or AB″). For this purpose, the transverse dimensions of the track with gauge 2s and of the bogie are reduced by the constant amount, 2d, defining the transverse distance between the bases of the wheel flanges of the same axle.

The bases of the flanges of the two wheels merge and are represented in Figure 3 as points A and B (B′ and B″). The same points depict the attacking (A) and the non-attacking (B) axle, respectively.

The reduced gauge is determined via Equation (4):

$$\sigma =2s-2d=\Delta +\delta$$

(4)

where Δ is the total clearance between the flange and the rail, equal to 0.01 m; and δ is an additional expansion of the rail track in a curved section depending on the radius of the calculation curve, as determined with data from

Table 1. Additional expansion of the rail track in a curved section depends on the radius of the calculation curve [13].

Radius R (m)	δ (mm)
125–150	20
150–180	15
180–250	10
250–300	5
Over 300	0

[27].

In this way, the calculated value represents the maximum total clearance between the rails and the axle in a curved section of the rail track. In Figure 3, the attacking axle (point A) always contacts the outer rail. Depending on the movement speed and the radius of the curve, the second axle (point B or B’ or B”) can take one of the following positions:

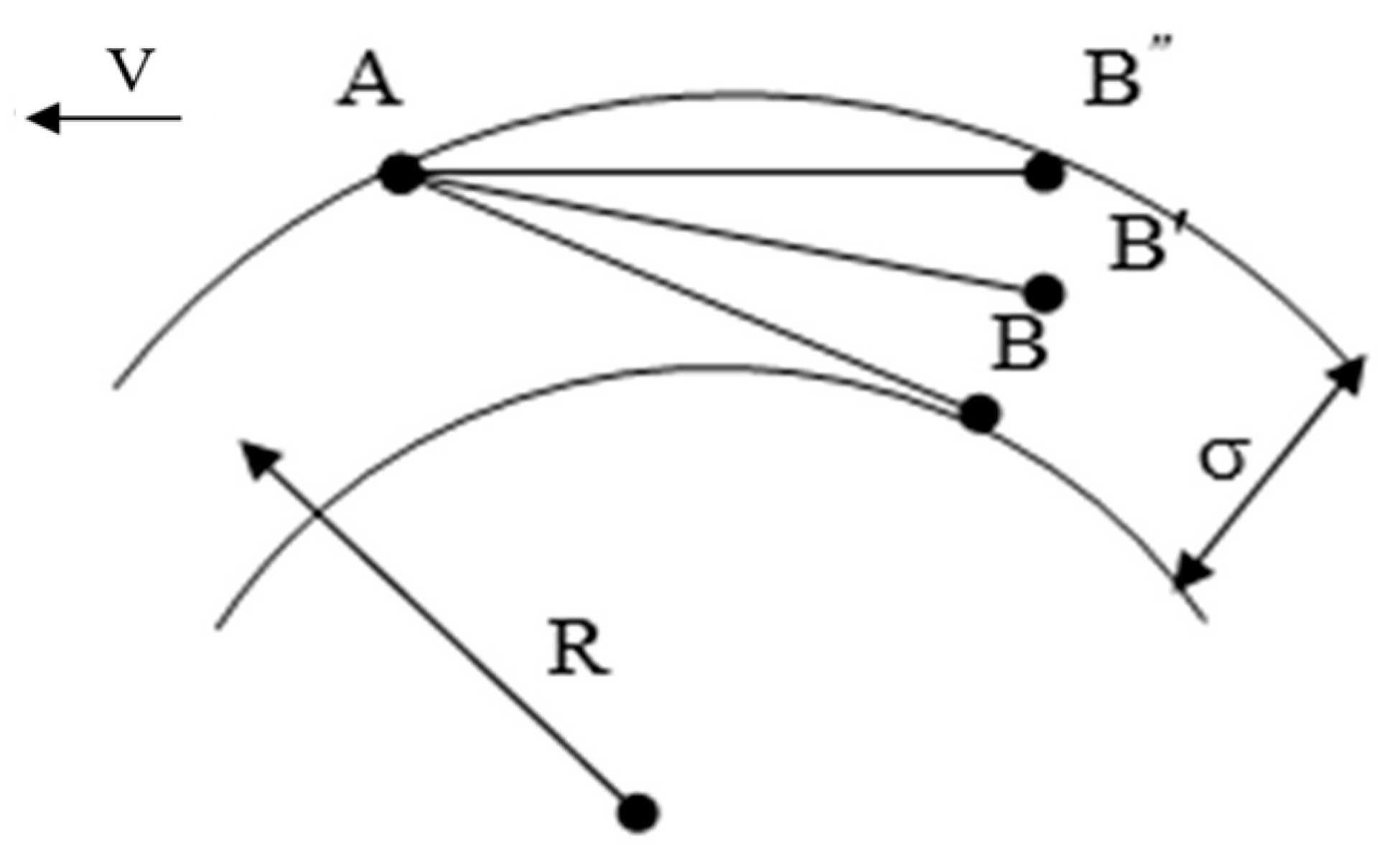
• AB—maximum crossing (σ_b = σ = Δ + δ);
• AB′—free settling (0 ≤ σ_b ≤ δ);
• AB″—maximum displacement (σ_b = 0).

When moving in a curve, the following forces act on the bogie (Figure 4):

• The transverse force, H, is induced by the centrifugal (H_c) and wind (H_w) forces. It is applied at the mass center of the wagon and is determined via Equation (5):

$$H=H_c+ H_w$$

(5)

2.

The centrifugal force is defined using Equation (6):

$$H_c=P.(\frac{v^2}{R.g}- \frac{h}{2.s})$$

(6)

where P (in [N]) is the weight of the wagon, v (in [m/s]) is the movement speed, g (in [m/s²]) is the gravitational acceleration, R (in [m]) is the curve radius, 2.s (in [mm]) is the distance between wheels rolling circles (normal track width 2.s = 1500 mm), and h (in [mm]) is the overhang of the outer rail determined from the table in Figure 5 [27].

3.

The wind force is determined via Equation (7):

$$H_w=F.W$$

(7)

where F is the surface of the wagon on which the wind is acting (in [m²]), and W is the wind pressure (in [N/m²]).

4.

The frictional forces Φ obtained because of the rotation around the pole M are determined using Equation (8):

$$\Phi =\mu .N_{st}$$

(8)

where µ is the coefficient of friction between the wheel and the rail, and N_st is the static vertical load on one wheel, as determined using Equation (9):

$$N_{st}=\frac{P}{N}$$

(9)

where N is the number of wheels. For compliance with European standards, it should be noted that N_st = Q_nom.

5.

The total reaction Y_i from rails on the wheelset i are obtained from the equilibrium conditions ΣY = 0 and ΣM_M = 0, according to Equation (10):

$$\left|\begin{array}{c}{Y}_1-Y_2-H-2.\Phi .cos{\alpha }_1+2\Phi \mathit{cos}{\alpha }_2=0\\ Y_{1}x+Y_2\left(2l-x\right)-H\left(x-l\right)-2\Phi .r_1-2\Phi .r_2=0\end{array}\right.$$

(10)

where Φ_yi is the component of force Φ along the y-axis, and r_i is the distance from pole M to the corresponding contact point between the rail and wheel of the i-th wheel axle.

In Equation (10), there are four unknown terms implicitly set by the centrifugal forces: Y₁, Y₂, x, and speed v. Therefore, the total reactions, Y₁ and Y₂, are determined according to the following methodology, and the graphical representation is shown in Figure 6.

Step 1. It is assumed that the bogie is in the limit state between “maximum overshoot” and “free settling”. It is possible at a precisely determined but unknown speed, v₁. From the condition for the considered boundary condition, it follows that the distance from pole M, according to Equation (11), is

$$x=x_{max }=l+\frac{R{.\mathsf{\sigma }}_b}{2.l}$$

(11)

which is typical for the “maximum overshoot” position. It also follows that the total reaction of the second wheel axle is zero, e.g.,

$$Y_2=0$$

(12)

which is typical for the “free settling” position.

This allows Equation (10) to be solved and to obtain specific values for Y₁ and v₁, which are typical for the limited state. When solving Equation (10), it is possible for Y₁ or for v₁ to obtain negative values. This indicates that the boundary condition is not valid for the specified track and bogie parameters. In this case, it is necessary to go to Step 3 of the current methodology.

Step 2. When the bogie is in the “maximum overshoot” state, it will move with a speed in the interval from 0 to v₁, and the pole distance will be x = x_max. Therefore, the system of Equation (10) can be solved concerning Y₁ and Y₂ by setting discrete movement speed values in the specified interval.

Step 3. It is assumed that the bogie is in a limited state between free settling and maximum displacement. From this, the next conditions are shown in the following Equation (13):

$$x=x_{min}=l \mathrm{a}\mathrm{n}\mathrm{d} Y_2=0$$

(13)

Movement speed v₂ and force Y₁, in this case, can be found by solving Equation (10) under the conditions of Equation (13).

Step 4. If the bogie is freely fixed (Y₂ = 0), then, in Equation (10), there are three unknowns: Y₁, v, and x. In this case, the condition in Equation (14) is relevant:

$${v_{1 }<v<v_{2 } \mathrm{a}\mathrm{n}\mathrm{d} x}_{min}< x < x_{max}$$

(14)

Therefore, it is possible to obtain the remaining two unknowns by setting discrete values of v or x in Equation (10). The calculation process is greatly simplified when setting values of the parameter x.

Step 5. If the design speed of the wagon, v_k, is higher than v₂, it is necessary to build the third zone of the horizontal dynamic calculations, i.e., the zone of maximum displacement. In this case, the condition in Equation (15) is valid:

$$x=x_{min}=l$$

(15)

Therefore, by setting movement speed values in the interval between v₂ and v_k, the full reaction forces, Y₁ and Y₂, can be determined.

The methodology proposed above allows us to determine the full reactions, Y₁ and Y₂, of the first- and second-wheel axles of each bogie at different speeds, curve radii, specific track parameters, different wheel loads, different bogie wheel axle distances, and other parameters.

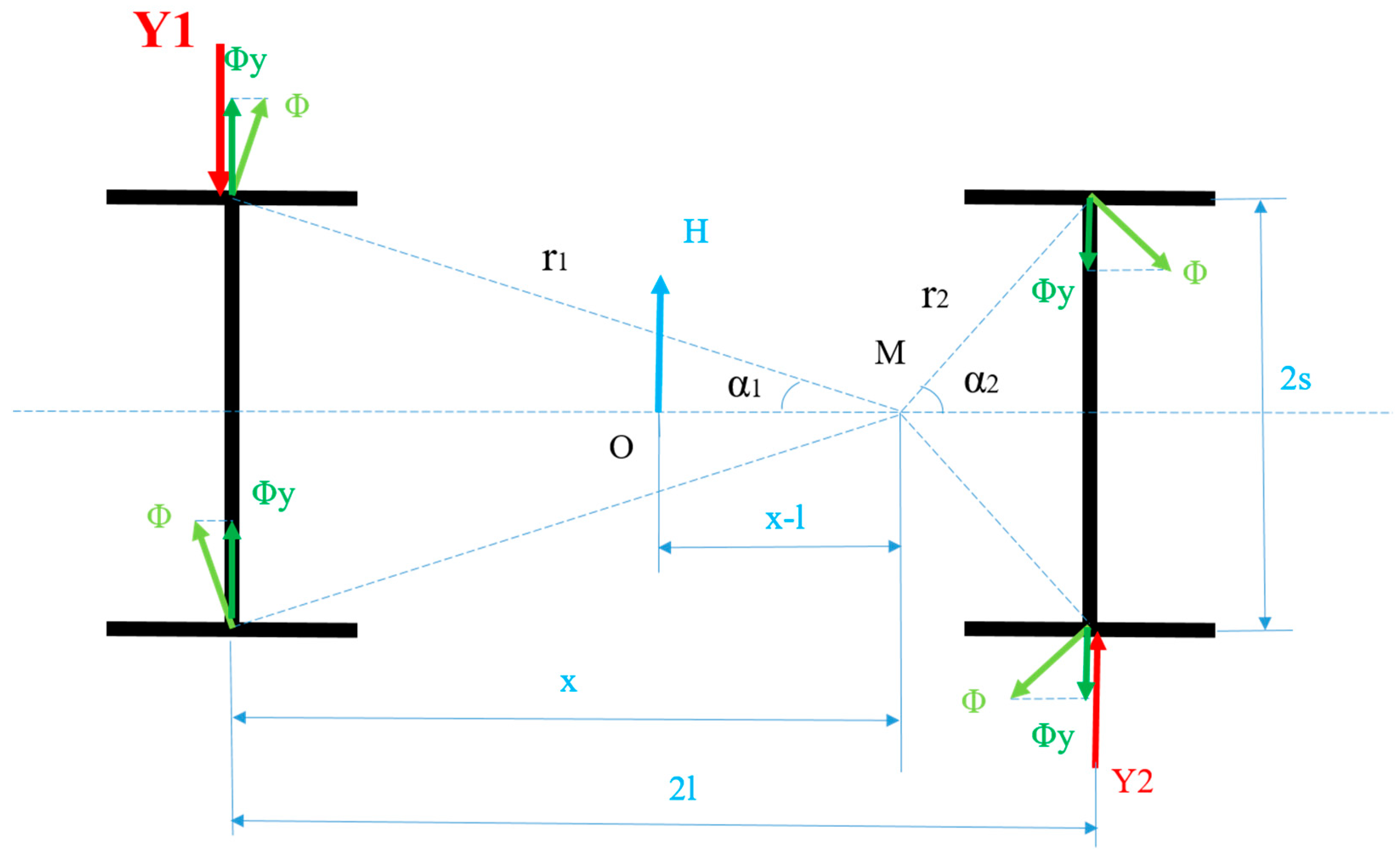
2.2. Methodology for Theoretical Determination of the Horizontal Load Force between the Inner (Non-Attacking) Wheel Y_ji of the Investigated Wheel Axle and the Inner Rail

The inner wheel of the examined wheel axle does not contact its flange with the corresponding rail. Therefore, the horizontal force acting between them arises from the frictional forces, which are determined via Equation (16):

$$Y_{ji}=\mu .Q_{ji}=\mu .(2.Q_{nom}-Q_{ja,min})$$

(16)

where μ is the coefficient of friction between the wheel and the rail, assumed to be equal to 0.4 for clean and dry rails, Q_ji is the vertical load force of the inner wheel (index i) on axle j, Q_nom is the nominal vertical load force of the wagon wheels, and Q_ja,min is the minimum vertical force acting on the outer (attacking) wheel of axle j. It is determined in accordance with the methodology given in Section 2.3. of this paper. Q_nom is determined using the ratio of the force from the weight of the wagon Q and the number of wheels of the vehicle N, as given in Equation (17):

$$Q_{nom}=\frac{Q}{N}$$

(17)

2.3. Methodology for Theoretical Determination of the Smallest Value of the Vertical Reaction of the Wheel, Q_jk,,min, Calculated during Torsion of the Wagon Frame

The proposed methodology for the theoretical determination of the minimum value of the vertical reaction of the wheels, Q_jk,,min, allows us to obtain the corresponding maximum value of this parameter, Q_jk,,max. The calculations are carried out in the following sequence:

• The frame of the wagon is loaded with an arbitrary force, ΔF_p (Figure 7), according to UIC Leaflet 432 [28], and the deflection of the frame, Δz_p, in the area around the lateral supports is determined (Figure 8).

2.

In accordance with EN 14363 [9], the minimum deflection of the frame Δz* is determined, which should be reached during the real (in situ) testing of the wagon. It is determined via Equation (18), subject to the requirement in Equation (19). In this case, 2a* is valid for wagon frames with pivot distances between 4 and 30 m.

$$∆z^{\ast }=g^{\ast }.2a^{\ast }$$

(18)

$$g^{\ast }=\frac{15}{2a^{\ast }}+2$$

(19)

3.

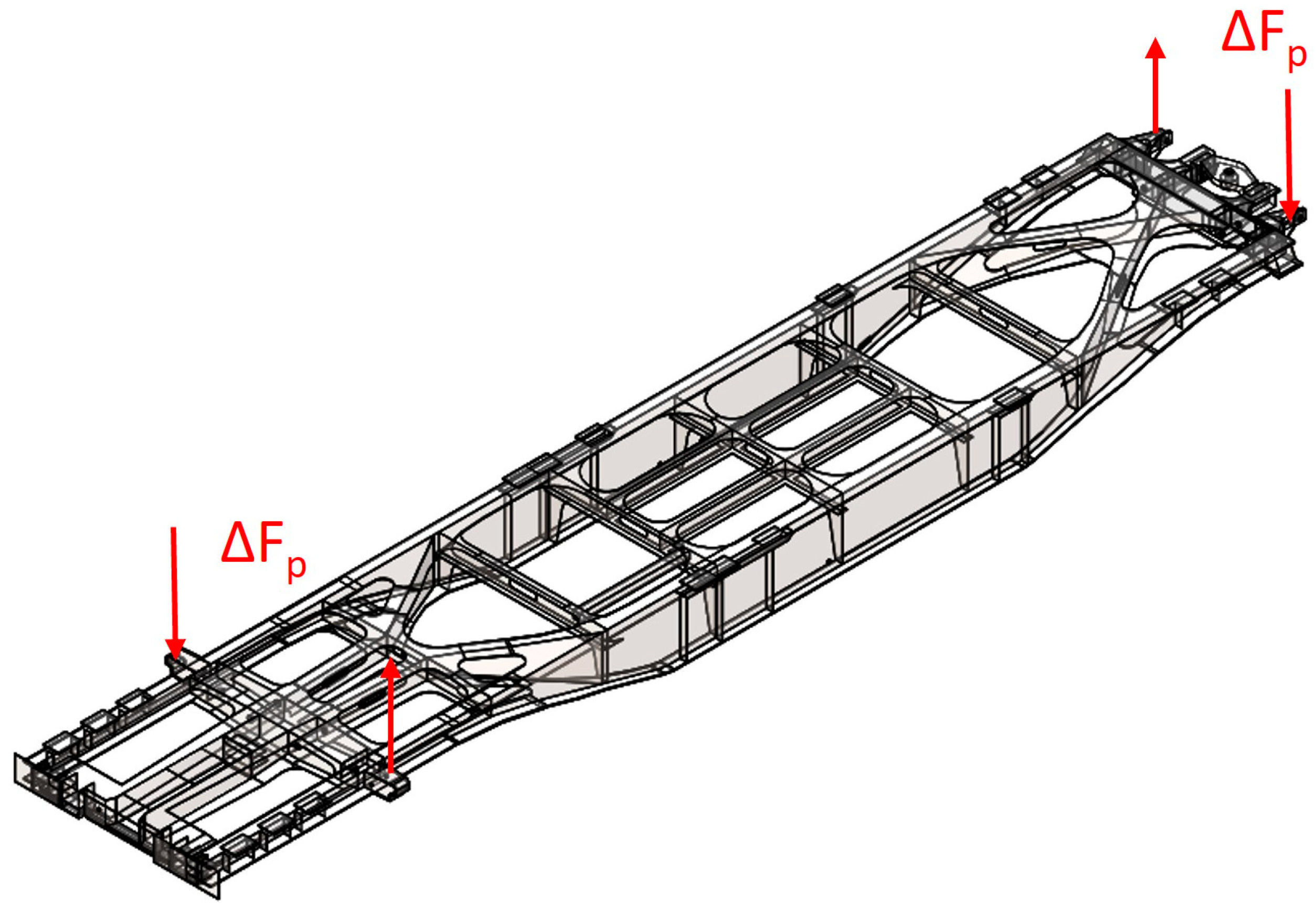
Recalculation of the force ΔF_p from step 1 for loading the wagon frame to achieve the minimum deflection Δz* according to Equation (20):

$$∆F_{z\ast }=\frac{∆F_p.∆z^{\ast }}{∆z_p}$$

(20)

The result of Equation (20) gives the force that acts on one side of the bogie in the area around the lateral support in Figure 8. This means that force ΔF_z* significantly loads the two unilaterally located wheels and significantly less for the other two.

4.

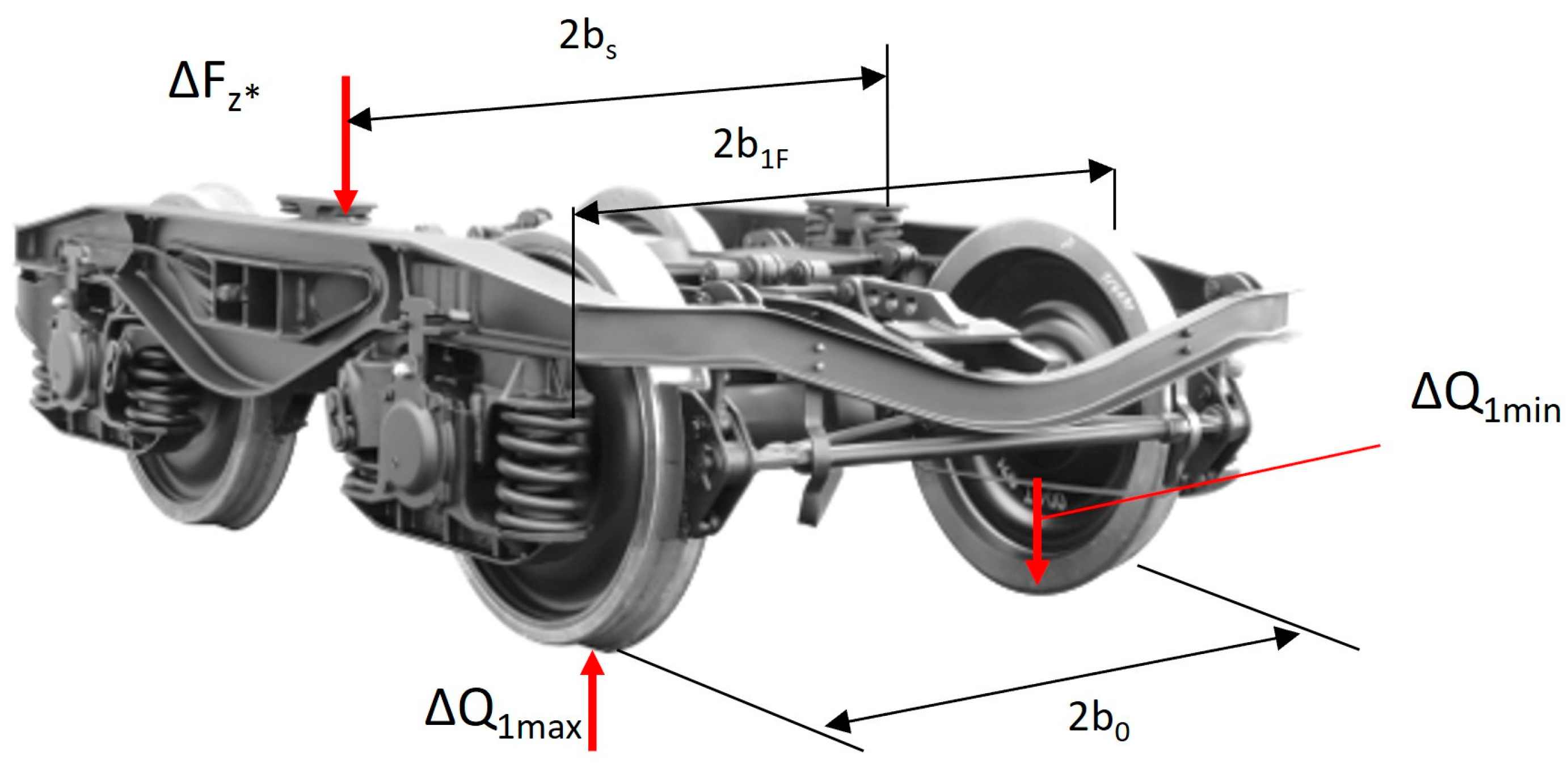
The force ΔF_z* is then transmitted from the lateral support to the side beams of the bogie with a value of ΔF′_z*max and ΔF′_z*min according to Equations (21) and (22). The corresponding distances, b_1F and b_s, are shown in Figure 9.

$$∆{F^{\prime }}_{z\ast ,max}=\frac{∆F_{z\ast }.(b_{1F }+b_s)}{2b_{1F }}$$

(21)

$$∆{F^{\prime }}_{z\ast ,min}=∆F_{z\ast }-∆{F^{\prime }}_{z\ast ,max}$$

(22)

From the side beam, the forces ΔF′_z*max and ΔF′_z*min are distributed between the two axle journals of the overloaded and the two axle journals of the unloaded wheels, with the forces ΔF′_z*max and ΔF′_z*min acting on the first (attacking) wheel axle, defined using Equations (23) and (24):

$$∆{F^{\prime }}_{1z\ast ,max}=\frac{∆{F^{\prime }}_{z\ast ,max}}{2}$$

(23)

$$∆{F^{\prime }}_{1z\ast ,min}=\frac{∆{F^{\prime }}_{z\ast ,min}}{2}$$

(24)

From the corresponding axle journal, the forces from Equations (23) and (24) cause additional reactions in the two wheels, with values defined in Equations (25) and (26):

$$∆Q_{1,max}=\frac{∆{F^{\prime }}_{1z\ast ,max .}\left(b_{1F }+b_0\right)-∆{F^{\prime }}_{1z\ast ,min}.\left(b_{1F }-b_0\right) }{2b_0}$$

(25)

$$∆Q_{1,min}=∆{F^{\prime }}_{1z\ast ,max}+∆{F^{\prime }}_{1z\ast ,min}-∆Q_{1,max}$$

(26)

5.

The minimum value of the wheel reaction, Q_jk,,min, is determined using Equation (27), and the maximum value is evaluated using Equation (28), respectively:

$$Q_{jk,min}=Q_{nom}+∆Q_{1,min}$$

(27)

$$Q_{jk,max}=Q_{nom}+∆Q_{1,max}$$

(28)

where the force Q_nom is determined via Equation (17).

3. Results from the Theoretical Derailment Safety Assessment

This study was conducted only for the first bogie axle of a Sggmrss series wagon (90 feet) in an unloaded condition equipped with a standard Y25 bogie. The reason for this is that theoretical analyses categorically state that the first wheel axle of an unloaded wagon is most at risk of derailment. This conclusion is also confirmed in the test results of all wagons from the reference list given in UIC Leaflet 530-2 [29]. This was also found during field tests of the same wagon [11,30]. The initial data used in the theoretical study are as follows:

• Tare weight of the wagon, 27.5 t;
• Curve radius, R = 150 m;
• Clearance between flanges and rail threads in a straight section of the track, equal to δ = 0.01 m;
• Additional tracks widening in a curved section, δ = 0.002 m (in accordance with the test data of the wagon [30]);
• Coefficient of friction between the rail and the wheel, μ = 0.4;
• Wheel axle distance, a⁺ = 1.8 m;
• Pivot distance (for one wagon section only), a* = 11.995 m;
• Speed of passing through the curve, v = 7 km/h (in accordance with the test data of the wagon [30]);
• Wind pressure, W = 0 N/m² (in accordance with the test data of the wagon [30]);
• Distance between the rolling circles of the two wheels of the same axle, 2b₀ = 1.5 m;
• Transverse distance between the axle journals, 2b_jF = 2.0 m;
• Distance between the side supports on the bogie, 2b_s = 1.7 m;
• Overhang of the outer rail, h = 0.15 m;
• Earth acceleration, g = 9.81 m/s².

For the theoretical determination of the safety criterion against derailment of a Sggmrss wagon (90 feet), the methods described in detail in Section 2 of this paper were applied. The results from the calculations conducted with the mentioned methodology are given in

Table 2. Results from the calculation needed for determination of safety against derailment.

Parameter	Value	Remark
v1	58.3 km/h	Methodology from Section 2.1.
Y1 = Y1a	24.718 kN	Methodology from Section 2.1.
Y1i	−14.024 kN	Methodology from Section 2.2.
g*	3.251‰	Equation (19)
Δz*	39 mm	Equation (18)
ΔFp	50 kN	The selected load value for the torsional stiffness test [30]
Δzp	0.08265 mm	Deflection of the frame under the Load, ΔFp, determined in [31]
ΔFz*	23.59 kN	Equation (20)
ΔF’z*,max	21.82 kN	Equation (21)
ΔF’z*,min	1.769 kN	Equation (22)
ΔF’1z*,max	10.909 kN	Equation (23)
ΔF’1z*,min	0.885 kN	Equation (24)
ΔQ1,max	12.58 kN	Equation (25)
ΔQ1,min	−0.7862 kN	Equation (26)
Qnom	22.48 kN	Equation (17)
Qjk,min	21.695 kN	Equation (27)
Qjk,max	35.061 kN	Equation (28)

.

With the data from Table 2, the final assessment of safety against derailment can be conducted. This is performed using Equation (1), and the calculated value is equal to 1.017. According to [9], when using the theoretical assessment methods, the limit value of 1.2 is reduced by 10%, which means that the limit value of Nadal’s criterion should be set to 1.08 and compared with the calculated value, as shown in Equation (29).

$${\left(\frac{Y}{Q}\right)}_{\mathit{ja}}=1.017; 1.017 \le 1.08$$

(29)

The obtained value of the safety criterion, 1.017, is lower than the limit value, 1.08, meaning that, for wagon series Sggmrss, the requirement for safety against derailment is fulfilled.

4. Discussion

The partial results from the experimental study are given in [11,30]. In [11], as well as in this paper, only an excerpt of the results is presented due to the sensitivity and confidentiality of the data contained in the report [30], as requested by the wagon manufacturer. For the final assessment in tests, not all parameters from Table 2 were determined, but only a few of them, which are necessary for the assessment and measured directly or indirectly. These parameters are Y_1i, Δz*, Q_nom, and Q_jk,,min. A comparison of the results from tests and calculations is given in

Table 3. Comparison of results from the calculation and test.

Parameter	Value from Calculation	Value from Test
Y1i	−14.024 kN	−13.5 kN
Δz*	39 mm	40.1 mm
Qnom	22.48 kN	22.10 kN
Qjk,min	21.695 kN	19.81 kN

.

The values for the lateral force, Y_1i, were measured in the test on the flat track with a radius of 150 m, while forces Q_nom and Q_jk,min were measured on the twist test rig, as well as Δz* [11,30]. It should be noted that all values of parameters measured in the tests are the average values from different numbers of tests (seven tests on twist rig and three on flat track) conducted. In [30], the measurement uncertainty was determined at 1.4% for vertical forces and 1.2% for displacements and twists. This, along with wagon imperfections caused by production, welding, and other influential factors, explains the deviation from the theoretical assumptions.

The results from the tests also confirm that the safety against derailment for this wagon fulfills the requirements. The value obtained in the tests is equal to 1.03 (Table 1 in [11]). It should be mentioned that the limit value in this case is set to 1.2, as stated in Equation (1) and Reference [9].

Table 3 shows that the values of measured and calculated parameters are close enough (in order of ±10%). This gives reason to claim that the proposed theoretical safety assessment method delivers very good results and can be used for the safety assessment of similar wagons.

The advantages of our method compared to similar methods are mainly the use of fewer input parameters, simplicity, and no need for complex simulations. On the other hand, the proposed method uses some initial parameters for which assumptions are made (the value of the coefficient of friction, µ) or for which their values are determined in tests (additional tracks widening in a curved section (δ) or wind pressure (W)). With other values for these parameters, safety against derailment would have values other than the calculated values, and the final fulfillment could be questionable. This is the reason why it would be necessary to conduct more assessments using the proposed method and on different wagon series for future research. This would help to additionally verify the results from calculations.

5. Conclusions

It is possible to save costs and time for the acceptance procedure by using the resulting parameters from the theoretical calculations of safety against derailment with the proposed method and by comparing them with the parameters of the reference wagons that have undergone real tests. This approach is allowed by clause 6.1.5.2.6 of EN 14363:2019 [9]. In this paper, we show that the proposed methodology for calculating safety against derailment gives good results and is verified in a test on a real object, the wagon Sggmrss series. For future research, it would be necessary to conduct more theoretical assessments using the proposed method on different wagon series, as doing so would help to verify the calculation results. The proposed theoretical safety assessment method can be used to study the safety regarding derailment for other new wagons and railway vehicles.

However, the final decision on whether the proposed method is appropriate for the assessment of safety in terms of preventing derailment lies with the notified body. If the authorities are satisfied with the results obtained in the theoretical calculation, this method could be allowed to be used for the assessment.