Effect of Rock Dissolution on Two-Phase Relative Permeabilities: Pore-Scale Simulations Based on Experimental Data
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Description
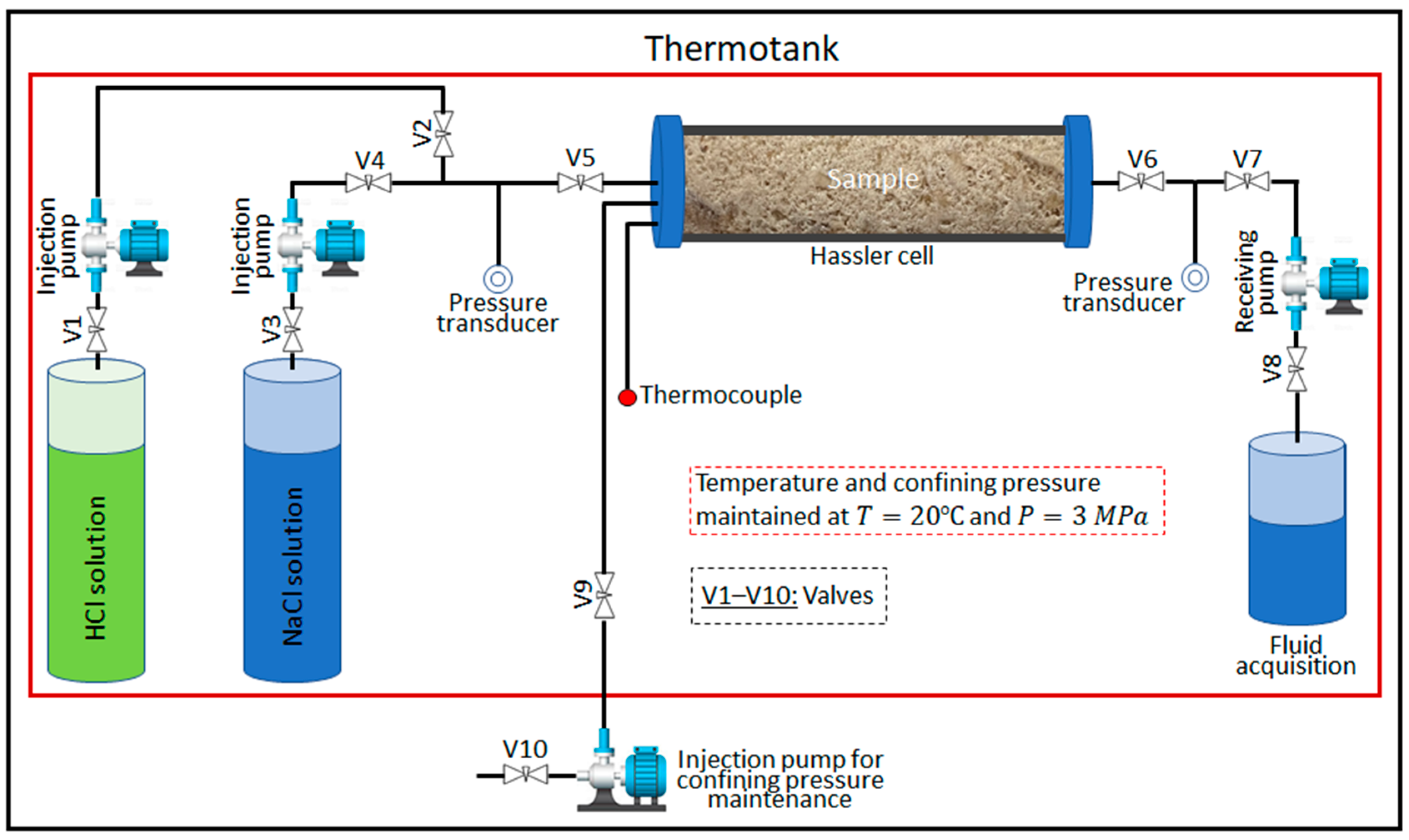
2.2. Experimental Procedure
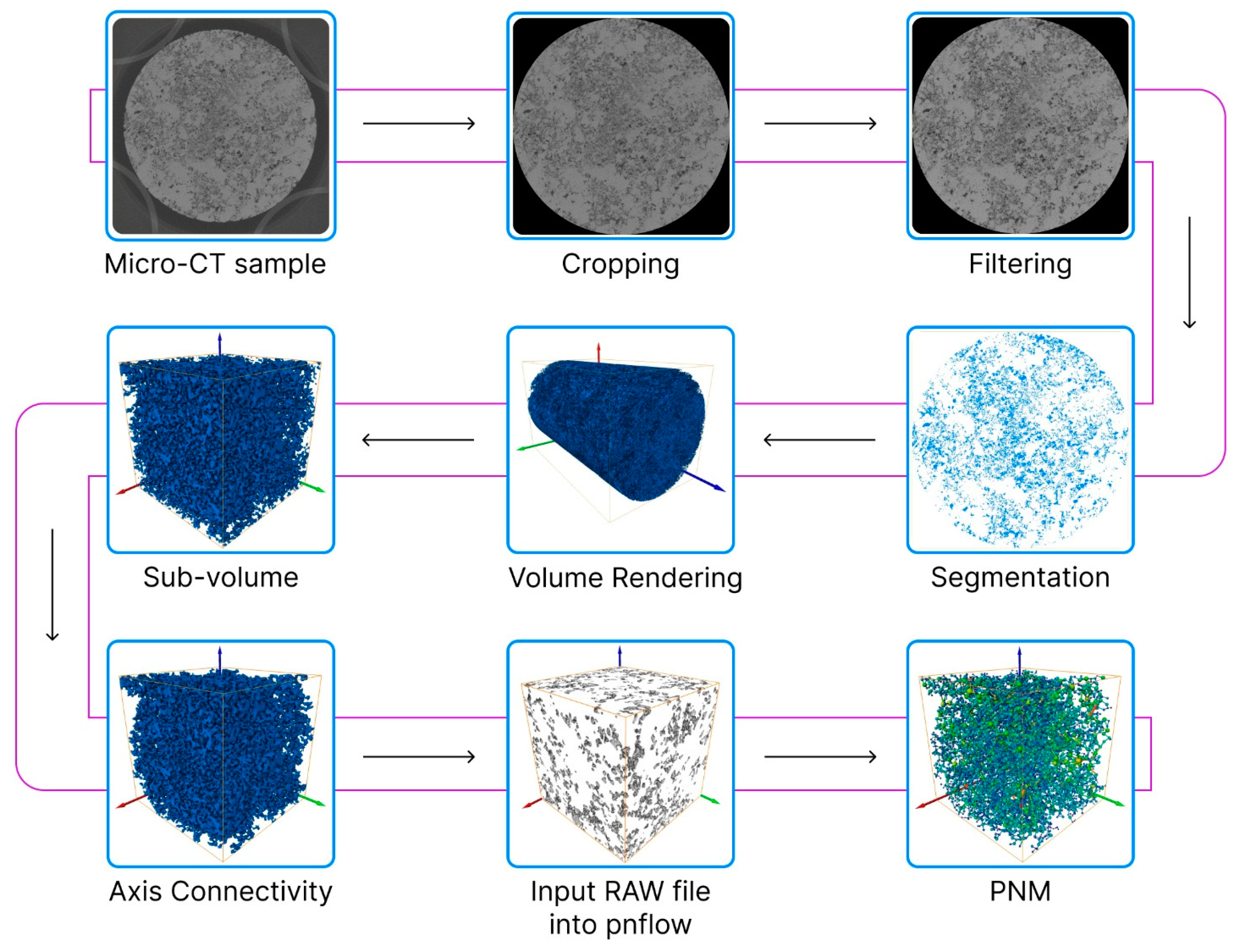
2.3. Simulation of Two-Phase Fluid Flow
3. Results and Discussion
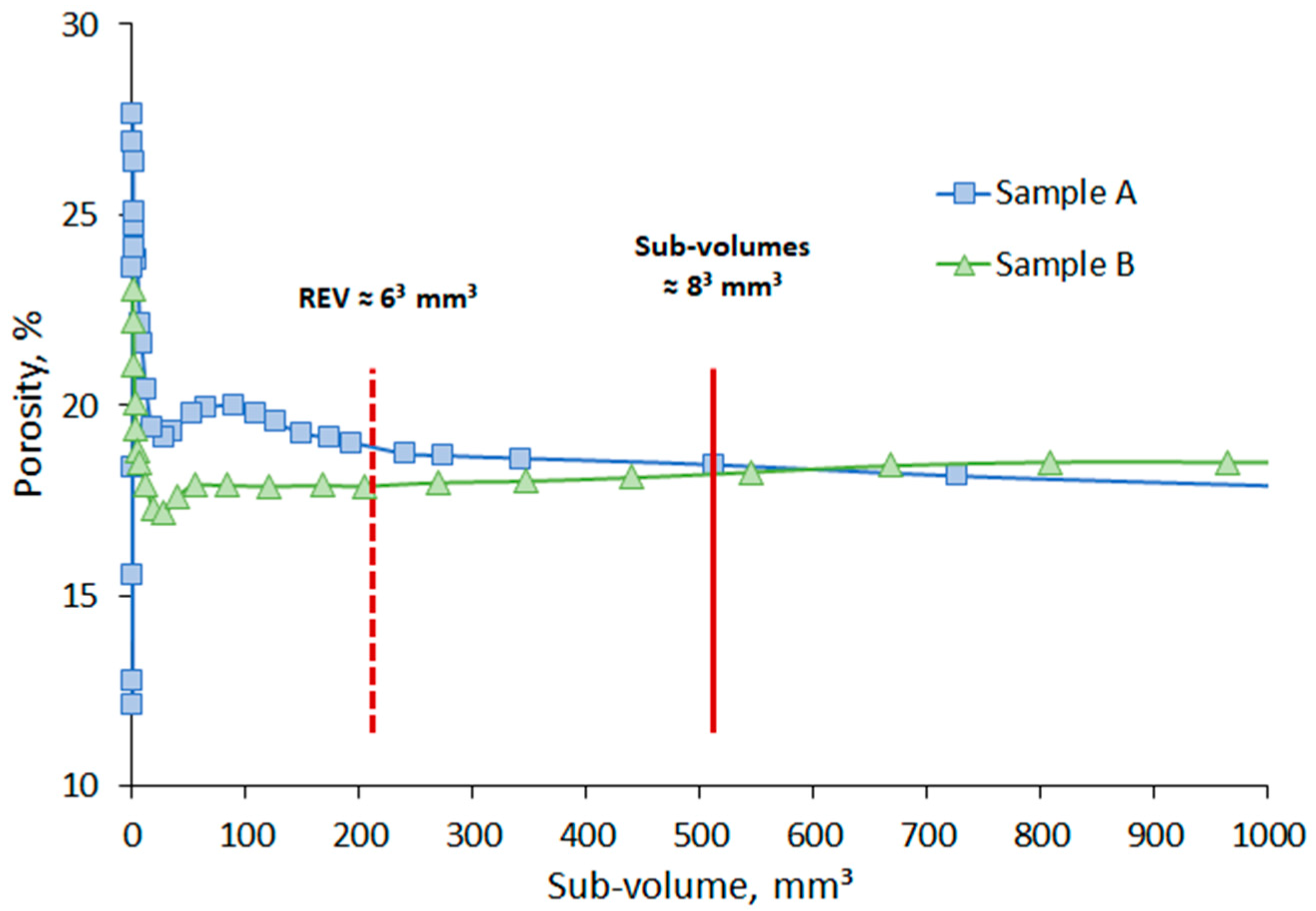
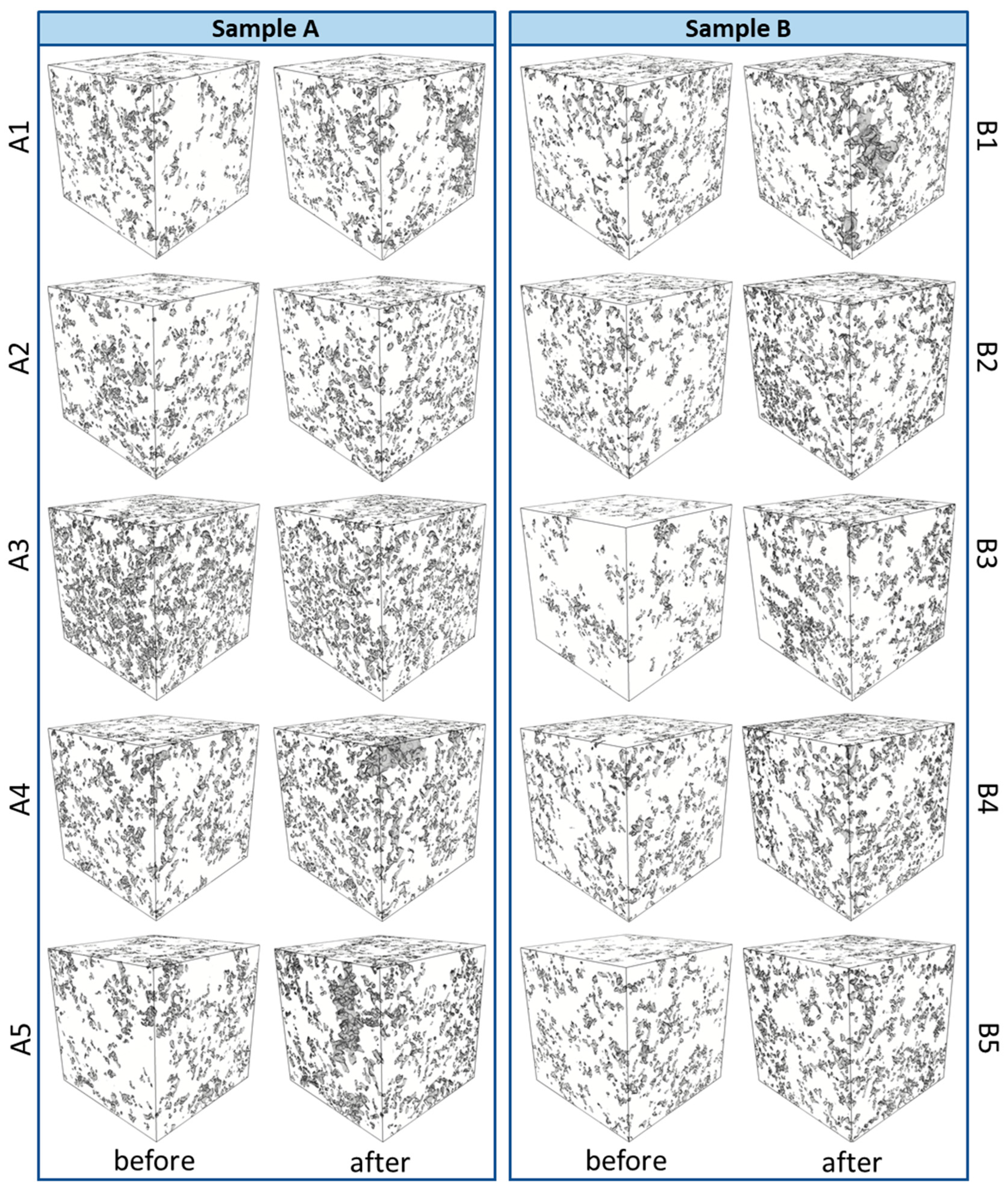
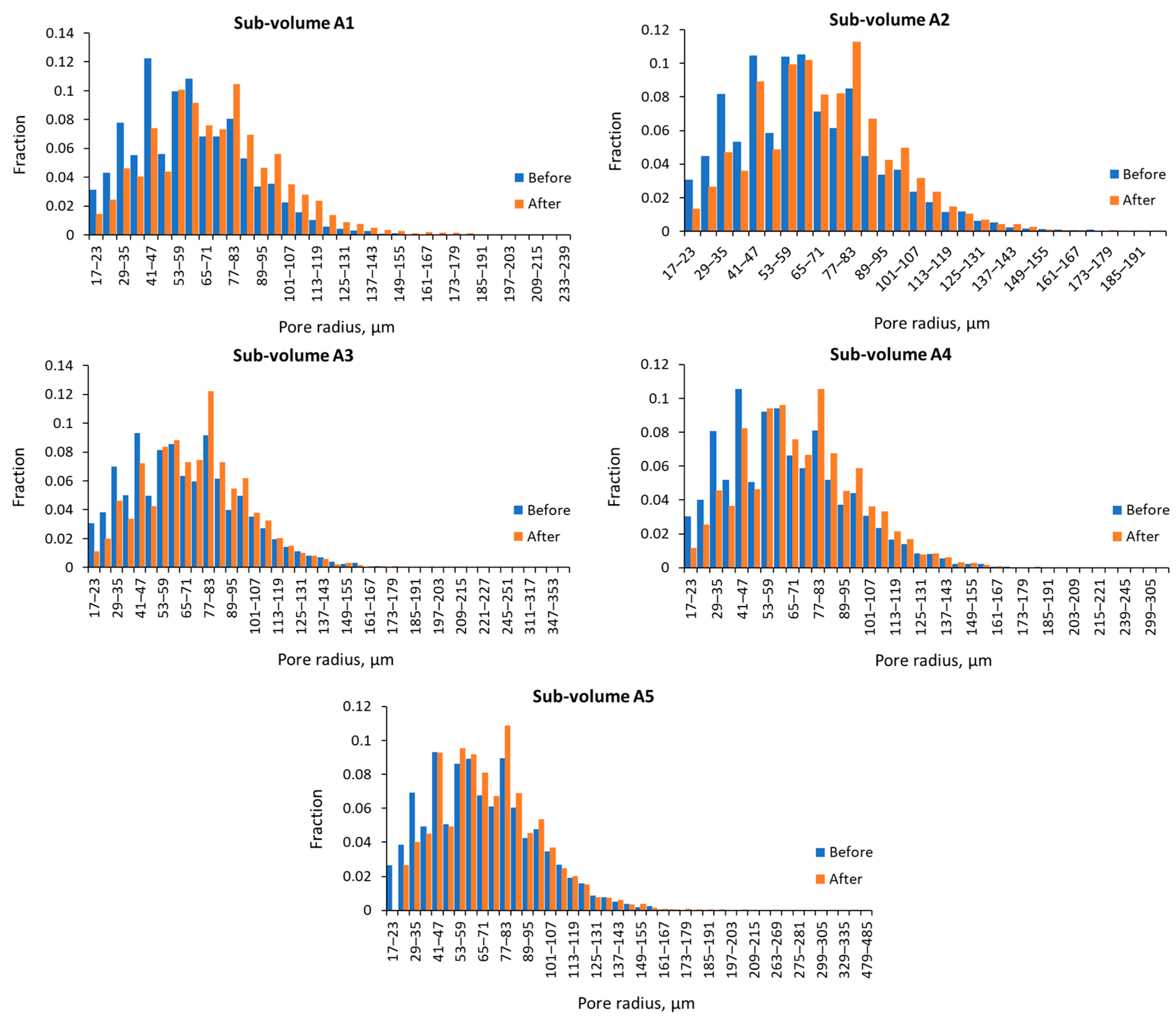
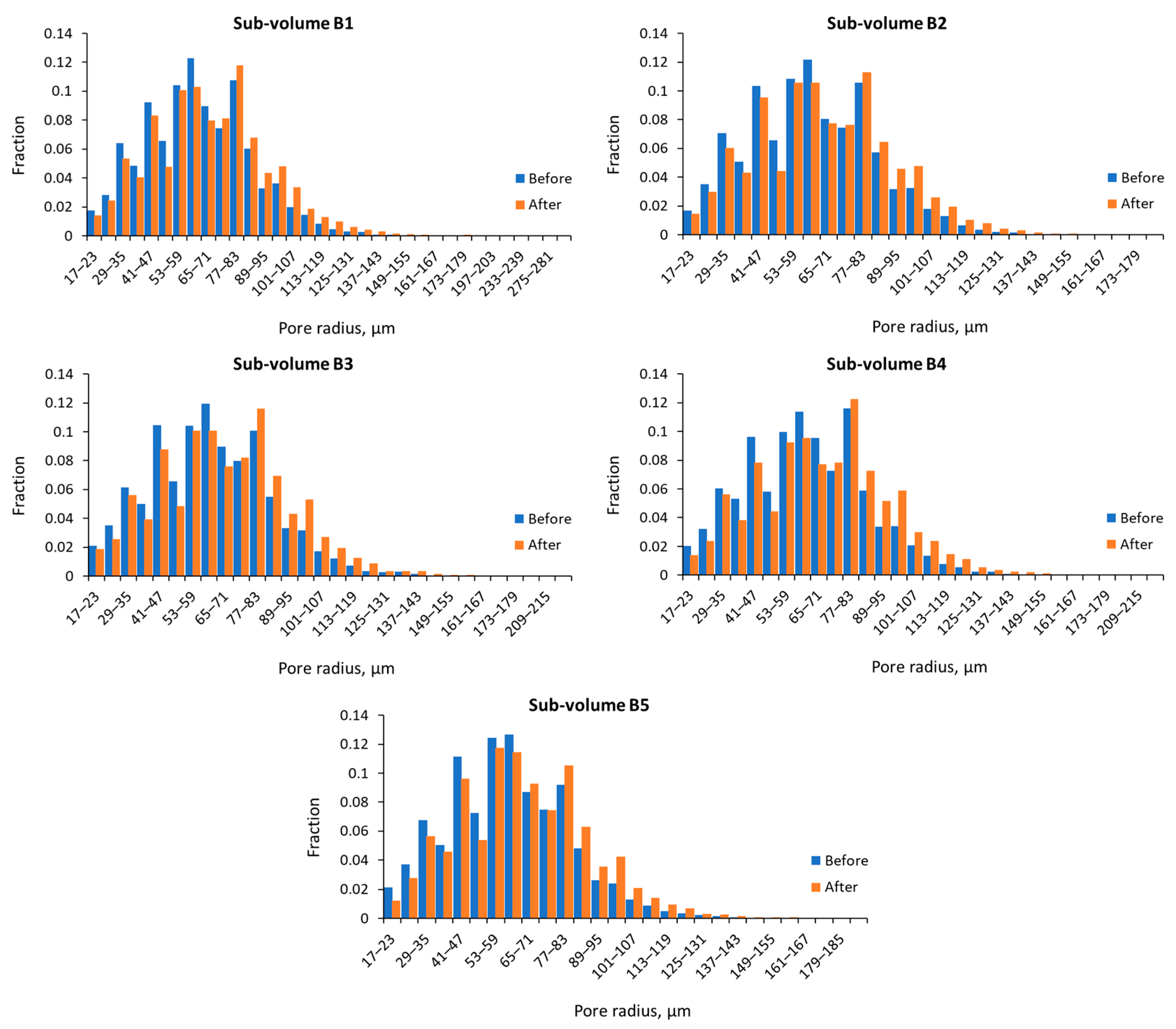
3.1. Pore Size Distribution, Porosity, and Absolute Permeability
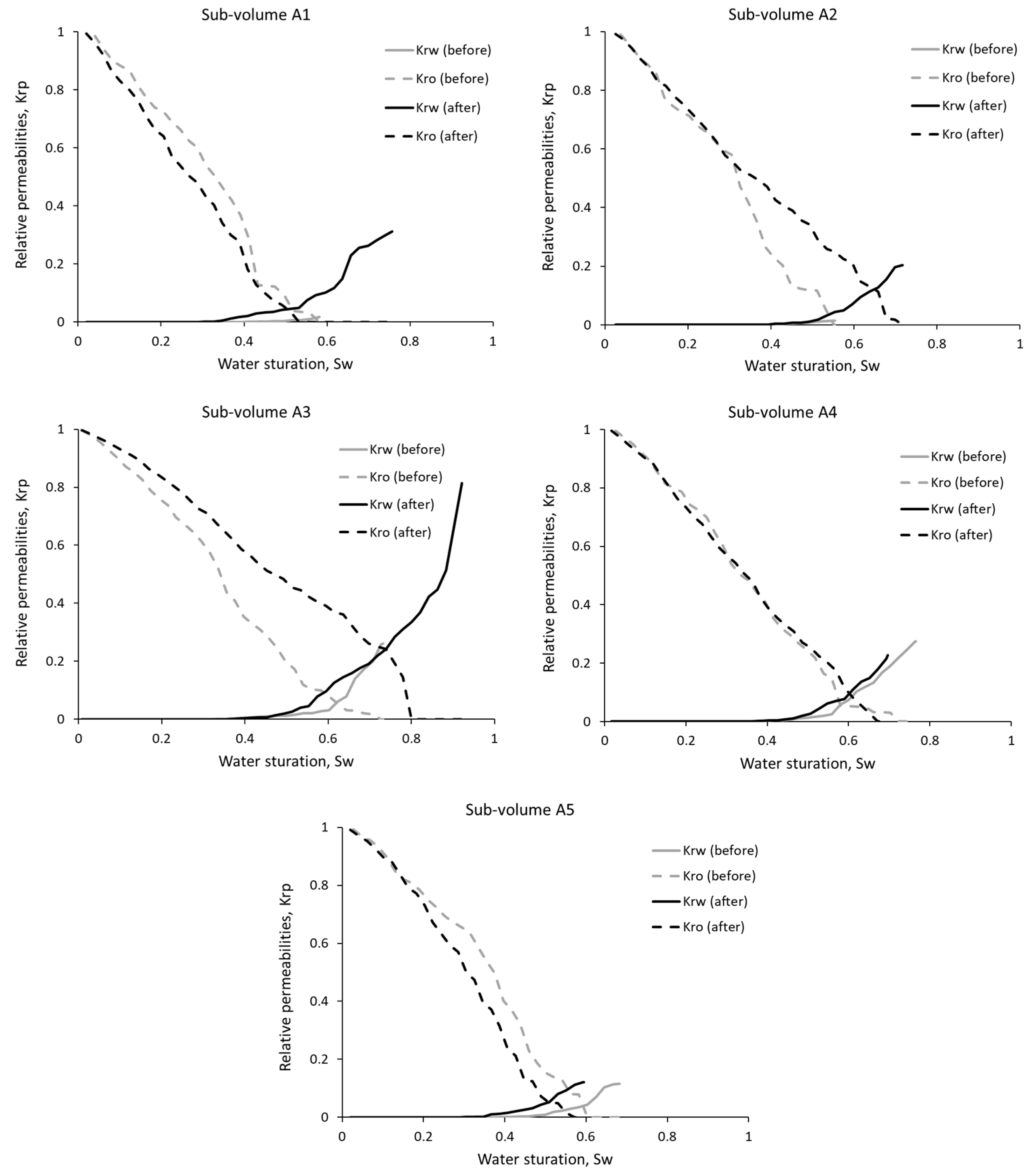
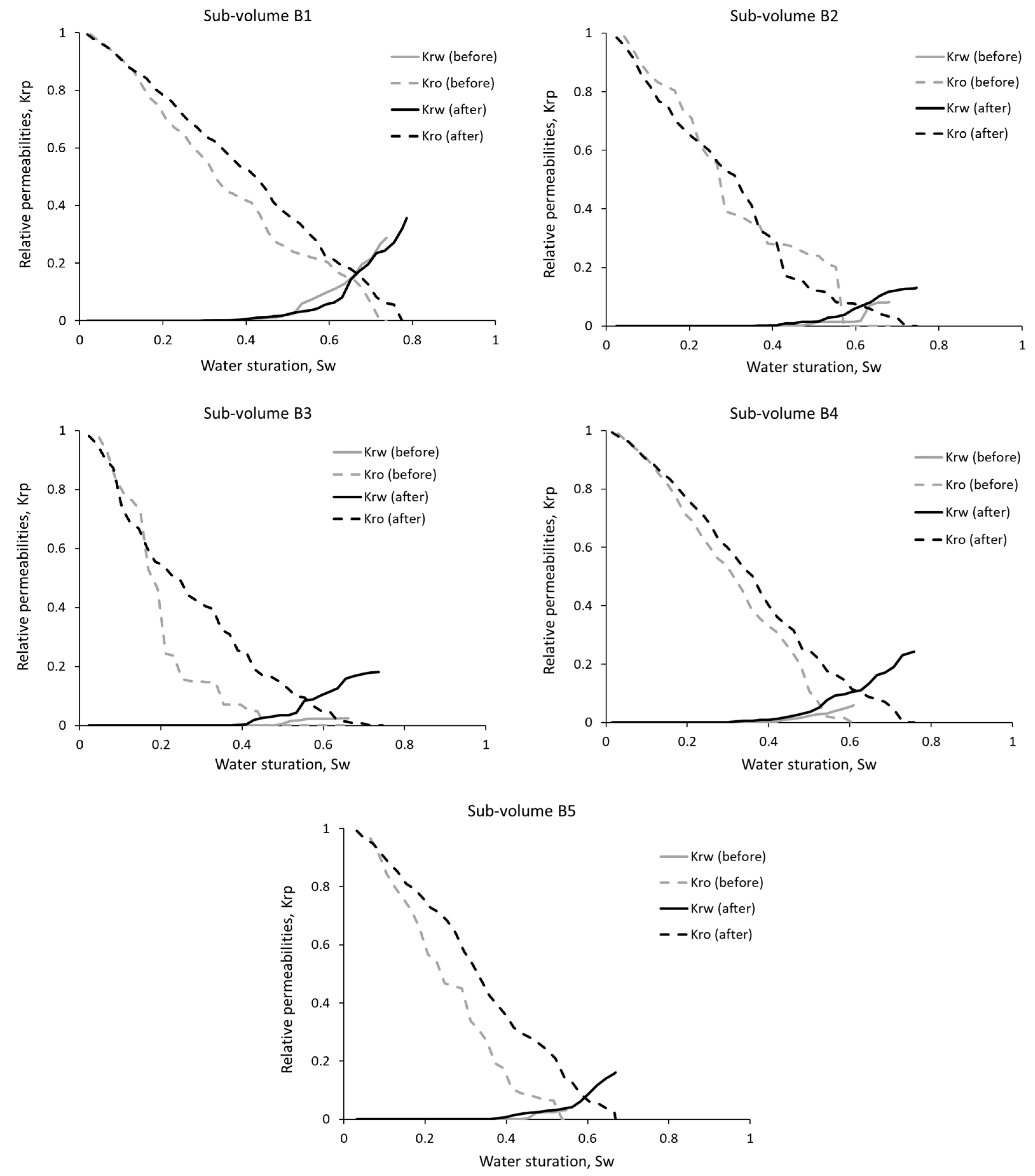
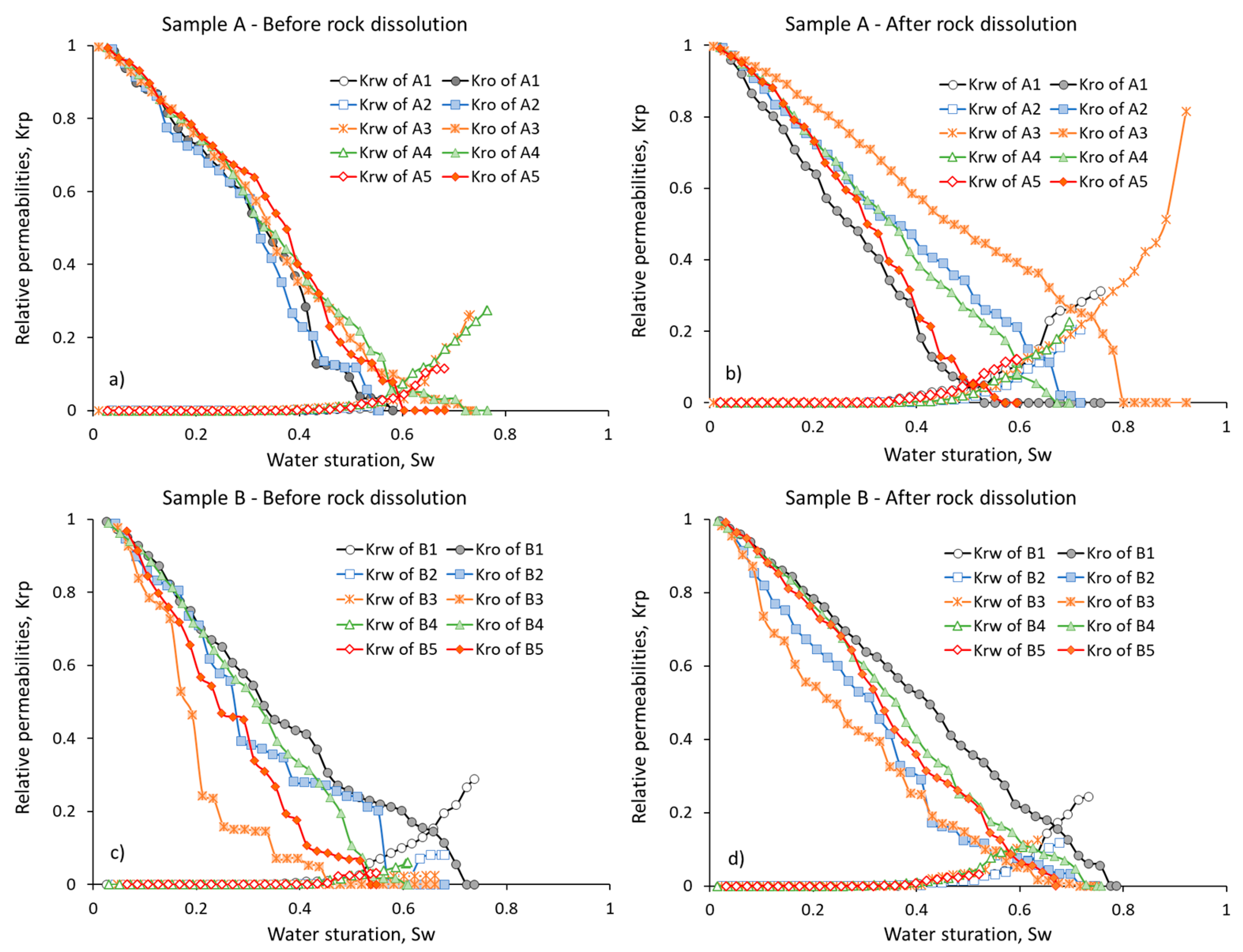
3.2. Relative Phase Permeabilities
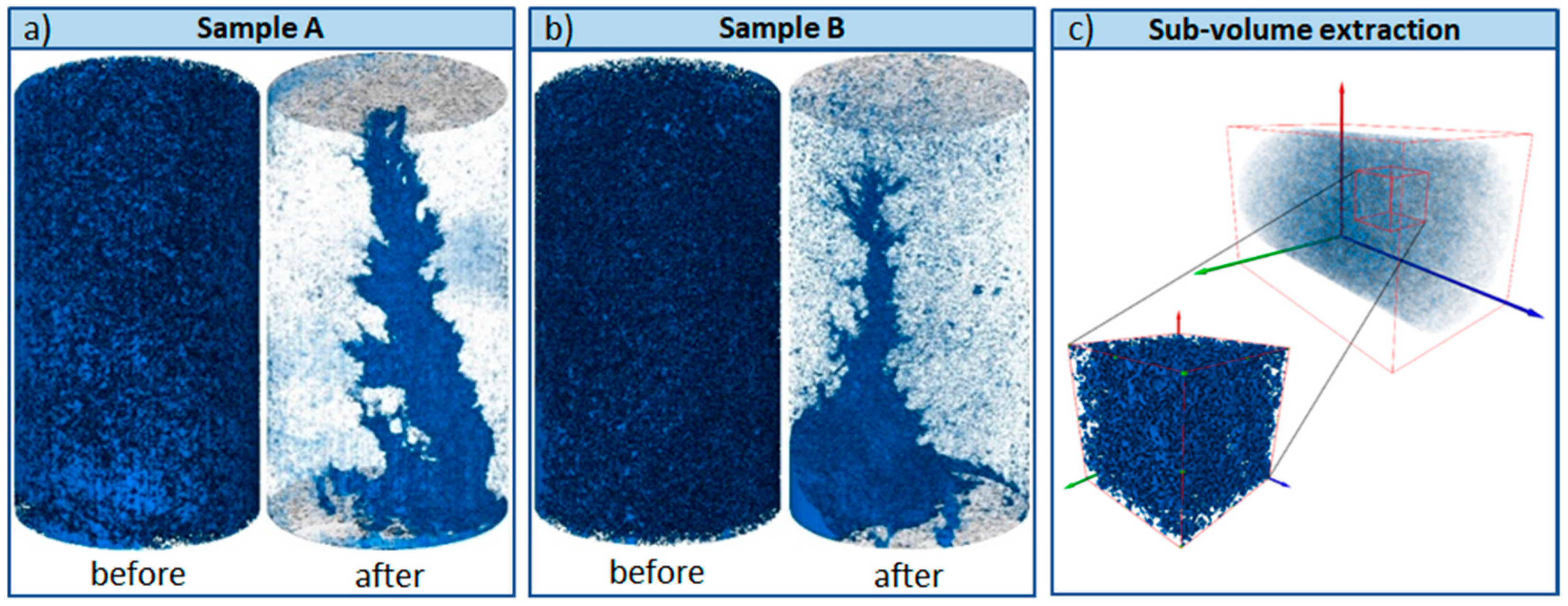
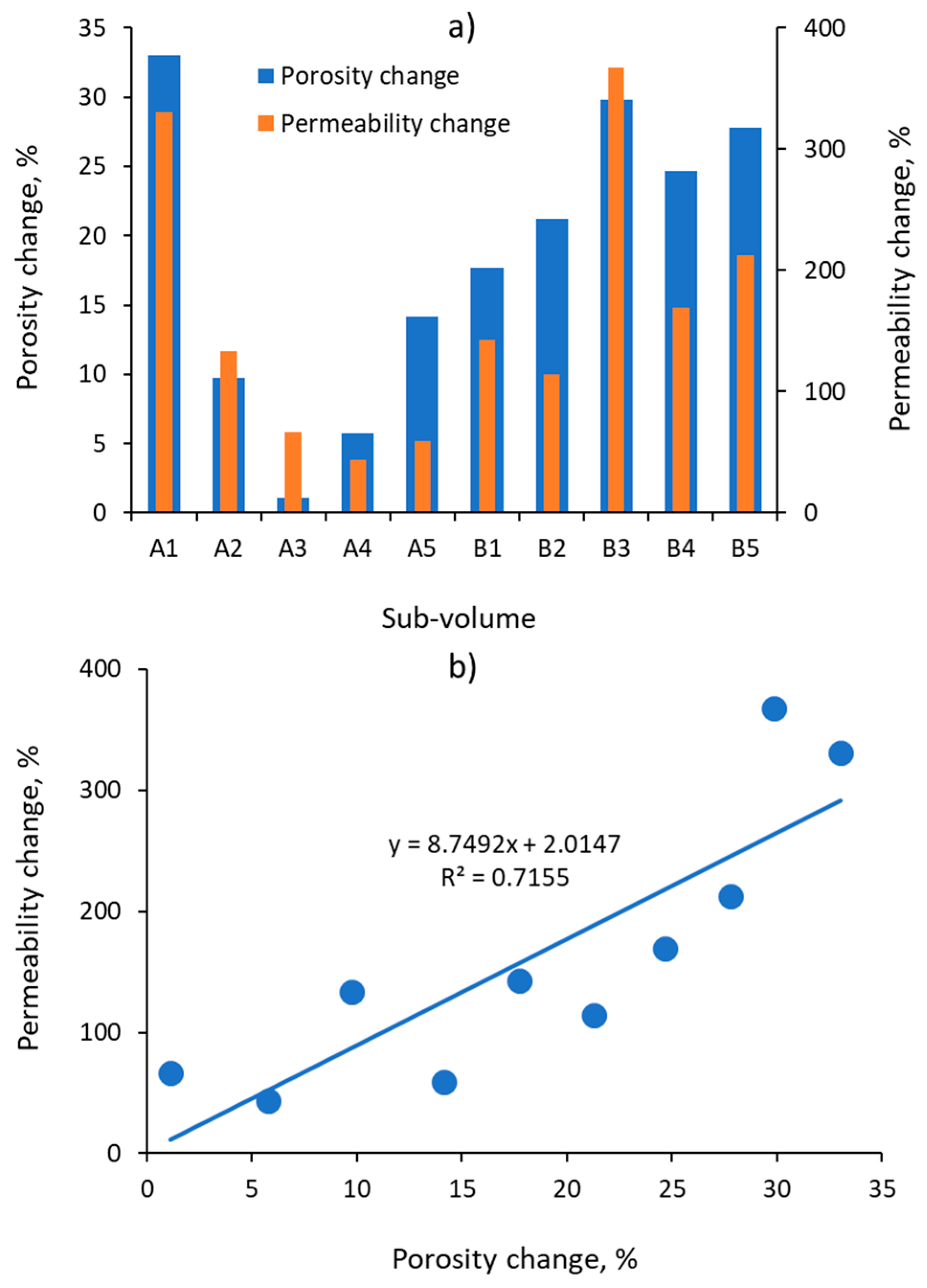
3.3. Spatial Variability of Relative Permeabilities
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Blunt, M.J.; Bijeljic, B.; Dong, H.; Gharbi, O.; Iglauer, S.; Mostaghimi, P.; Paluszny, A.; Pentland, C. Pore-scale imaging and modelling. Adv. Water Resour. 2013, 51, 197–216. [Google Scholar] [CrossRef]
- Cnudde, V.; Boone, M.N. High-resolution X-ray computed tomography in geosciences: A review of the current technology and applications. Earth-Sci. Rev. 2013, 123, 1–17. [Google Scholar] [CrossRef]
- Sadeghnejad, S.; Enzmann, F.; Kersten, M. Digital rock physics, chemistry, and biology: Challenges and prospects of pore-scale modelling approach. Appl. Geochem. 2021, 131, 105028. [Google Scholar] [CrossRef]
- Niu, B.; Krevor, S. The Impact of Mineral Dissolution on Drainage Relative Permeability and Residual Trapping in Two Carbonate Rocks. Transp. Porous Media 2020, 131, 363–380. [Google Scholar] [CrossRef]
- Van der Land, C.; Wood, R.; Wu, K.; van Dijke, M.I.; Jiang, Z.; Corbett, P.W.; Couples, G. Modelling the permeability evolution of carbonate rocks. Mar. Pet. Geol. 2013, 48, 1–7. [Google Scholar] [CrossRef]
- Jeong, G.S.; Ki, S.; Lee, D.S.; Jang, I. Effect of the Flow Rate on the Relative Permeability Curve in the CO2 and Brine System for CO2 Sequestration. Sustainability 2021, 13, 1543. [Google Scholar] [CrossRef]
- Juanes, R.; Spiteri, E.J.; Orr, F.M.; Blunt, M.J. Impact of relative permeability hysteresis on geological CO2 storage. Water Resour. Res. 2006, 42, W12418. [Google Scholar] [CrossRef]
- Krevor, S.C.M.; Pini, R.; Zuo, L.; Benson, S.M. Relative permeability and trapping of CO2 and water in sandstone rocks at reservoir conditions. Water Resour. Res. 2012, 48, W02532. [Google Scholar] [CrossRef]
- Rodríguez de Castro, A.; Goyeau, B. A pore network modelling approach to investigate the interplay between local and Darcy viscosities during the flow of shear-thinning fluids in porous media. J. Colloid Interface Sci. 2021, 590, 446–457. [Google Scholar] [CrossRef]
- Song, R.; Liu, J.; Cui, M. Single- and two-phase flow simulation based on equivalent pore network extracted from micro-CT images of sandstone core. Springerplus 2016, 5, 817. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, K.; Zhang, L.; Sun, H.; Zhang, K.; Ma, J. Pore-scale simulation of shale oil flow based on pore network model. Fuel 2019, 251, 683–692. [Google Scholar] [CrossRef]
- Zhao, J.; Qin, F.; Derome, D.; Kang, Q.; Carmeliet, J. Improved pore network models to simulate single-phase flow in porous media by coupling with lattice Boltzmann method. Adv. Water Resour. 2020, 145, 103738. [Google Scholar] [CrossRef]
- Gao, Y.; Raeini, A.Q.; Selem, A.M.; Bondino, I.; Blunt, M.J.; Bijeljic, B. Pore-scale imaging with measurement of relative permeability and capillary pressure on the same reservoir sandstone sample under water-wet and mixed-wet conditions. Adv. Water Resour. 2020, 146, 103786. [Google Scholar] [CrossRef]
- Masihi, M.; Shams, R.; King, P.R. Pore level characterization of Micro-CT images using percolation theory. J. Pet. Sci. Eng. 2022, 211, 110113. [Google Scholar] [CrossRef]
- Zhang, G.; Foroughi, S.; Raeini, A.Q.; Blunt, M.J.; Bijeljic, B. The impact of bimodal pore size distribution and wettability on relative permeability and capillary pressure in a microporous limestone with uncertainty quantification. Adv. Water Resour. 2023, 171, 104352. [Google Scholar] [CrossRef]
- Gray, F.; Anabaraonye, B.; Shah, S.; Boek, E.; Crawshaw, J. Chemical mechanisms of dissolution of calcite by HCl in porous media: Simulations and experiment. Adv. Water Resour. 2018, 121, 369–387. [Google Scholar] [CrossRef]
- Soulaine, C.; Roman, S.; Kovscek, A.; Tchelepi, H.A. Mineral dissolution and wormholing from a pore-scale perspective. J. Fluid Mech. 2017, 827, 457–483. [Google Scholar] [CrossRef]
- Steinwinder, J.; Beckingham, L.E. Role of Pore and Pore-Throat Distributions in Controlling Permeability in Heterogeneous Mineral Dissolution and Precipitation Scenarios. Water Resour. Res. 2019, 55, 5502–5517. [Google Scholar] [CrossRef]
- Yang, Y.; Li, Y.; Yao, J.; Iglauer, S.; Luquot, L.; Zhang, K.; Sun, H.; Zhang, L.; Song, W.; Wang, Z. Dynamic Pore-Scale Dissolution by CO2-Saturated Brine in Carbonates: Impact of Homogeneous Versus Fractured Versus Vuggy Pore Structure. Water Resour. Res. 2020, 56, e2019WR026112. [Google Scholar] [CrossRef]
- Bianchi Janetti, E.; Riva, M.; Guadagnini, A. Effects of Pore-Scale Geometry and Wettability on Two-Phase Relative Permeabilities within Elementary Cells. Water 2017, 9, 252. [Google Scholar] [CrossRef]
- Wu, Y.; Tahmasebi, P.; Lin, C.; Munawar, M.J.; Cnudde, V. Effects of micropores on geometric, topological and transport properties of pore systems for low-permeability porous media. J. Hydrol. 2019, 575, 327–342. [Google Scholar] [CrossRef]
- Aziz, R.; Joekar-Niasar, V.; Martínez-Ferrer, P.J.; Godinez-Brizuela, O.E.; Theodoropoulos, C.; Mahani, H. Novel insights into pore-scale dynamics of wettability alteration during low salinity waterflooding. Sci. Rep. 2019, 9, 9257. [Google Scholar] [CrossRef] [PubMed]
- Sagbana, P.I.; Sarkodie, K.; Nkrumah, W.A. A critical review of carbonate reservoir wettability modification during low salinity waterflooding. Petroleum 2023, 9, 317–330. [Google Scholar] [CrossRef]
- Drexler, S.; Hoerlle, F.; Godoy, W.; Boyd, A.; Couto, P. Wettability Alteration by Carbonated Brine Injection and Its Impact on Pore-Scale Multiphase Flow for Carbon Capture and Storage and Enhanced Oil Recovery in a Carbonate Reservoir. Appl. Sci. 2020, 10, 6496. [Google Scholar] [CrossRef]
- Singh, K.; Menke, H.; Andrew, M.; Lin, Q.; Rau, C.; Blunt, M.J.; Bijeljic, B. Dynamics of snap-off and pore-filling events during two-phase fluid flow in permeable media. Sci. Rep. 2017, 7, 5192. [Google Scholar] [CrossRef]
- Mahmud, W.M.; Nguyen, V.H. Effects of Snap-Off in Imbibition in Porous Media with Different Spatial Correlations. Transp. Porous Media 2006, 64, 279–300. [Google Scholar] [CrossRef]
- Yousef, A.A.; Al-Saleh, S.; Al-Jawfi, M. Smart water flooding for carbonate reservoirs: Salinity and role of ions. In Proceedings of the SPE Middle East Oil and Gas Show and Conference, Manama, Bahrain, 25–28 September 2011; SPE: Richardson, TX, USA, 2011. [Google Scholar]
- Fredd, C.N.; Scott Fogler, H. Influence of transport and reaction on wormhole formation in porous media. AIChE J. 1998, 44, 1933–1949. [Google Scholar] [CrossRef]
- Thermo Fisher Scientific. User’s Guide Avizo Software; Thermo Fisher Scientific: Waltham, MA, USA, 2019. [Google Scholar]
- Raeini, A.Q. Pnflow Simulation Code. Available online: https://github.com/aliraeini/pnflow (accessed on 27 January 2023).
- Dong, H.; Blunt, M.J. Pore-network extraction from micro-computerized-tomography images. Phys. Rev. E 2009, 80, 036307. [Google Scholar] [CrossRef]
- Raeini, A.Q.; Bijeljic, B.; Blunt, M.J. Generalized network modeling: Network extraction as a coarse-scale discretization of the void space of porous media. Phys. Rev. E 2017, 96, 013312. [Google Scholar] [CrossRef]


| Sample | A | B | ||||
|---|---|---|---|---|---|---|
| Length, cm | 5.01 | 5.02 | ||||
| Diameter, cm | 2.98 | 2.98 | ||||
| Porosity, % | 20.9 | 19.0 | ||||
| Composition, % | Calcite | Dolomite | Quartz | Calcite | Dolomite | Quartz |
| 99 | - | 1 | 99 | - | 1 | |
| Sample | HCl Concentration, % | Injection Rate, mL/min | Permeability, D | |
|---|---|---|---|---|
| Before | After | |||
| A | 12 | 8 | 0.72 | 3.90 |
| B | 18 | 2 | 0.29 | 0.99 |
| Sub-Volume | Min, µm | Max, µm | Mean, µm | |||
|---|---|---|---|---|---|---|
| Before | After | Before | After | Before | After | |
| A1 | 17.13 | 17.17 | 173.7 | 236.3 | 61.6 | 71.9 |
| A2 | 17.13 | 17.17 | 194.9 | 185.9 | 62.7 | 69.0 |
| A3 | 17.12 | 17.18 | 238.2 | 439.1 | 68.0 | 73.5 |
| A4 | 17.12 | 17.18 | 220.7 | 340.8 | 65.4 | 72.0 |
| A5 | 17.13 | 23.23 | 279.6 | 480.9 | 67.8 | 72.1 |
| B1 | 17.10 | 17.18 | 178.8 | 392.4 | 63.9 | 68.6 |
| B2 | 17.10 | 17.18 | 167.2 | 190.2 | 62.3 | 66.4 |
| B3 | 17.09 | 17.18 | 211.3 | 216.3 | 62.6 | 67.53 |
| B4 | 17.09 | 17.17 | 211.3 | 219.8 | 63.6 | 69.45 |
| B5 | 17.10 | 17.18 | 180.9 | 193.0 | 60.3 | 65.17 |
| Sub-Volume | Porosity, % | Absolute Permeability, D | ||
|---|---|---|---|---|
| Before | After | Before | After | |
| A1 | 16.7 | 22.2 | 2.58 | 11.13 |
| A2 | 19.4 | 21.3 | 3.86 | 9.01 |
| A3 | 29.1 | 29.4 | 24.53 | 40.94 |
| A4 | 21.8 | 23.1 | 8.95 | 12.86 |
| A5 | 19.8 | 22.6 | 7.49 | 11.88 |
| B1 | 19.0 | 22.4 | 6.22 | 15.07 |
| B2 | 16.7 | 20.3 | 3.04 | 6.50 |
| B3 | 14.5 | 18.8 | 0.90 | 4.23 |
| B4 | 18.4 | 22.9 | 5.77 | 15.52 |
| B5 | 14.7 | 18.8 | 2.04 | 6.37 |
| Sub-Volume | Residual Oil Saturation, % | Residual Water Saturation, % | Water Saturation Range, % | |||
|---|---|---|---|---|---|---|
| Before | After | Before | After | Before | After | |
| A1 | 44 | 49 | 4 | 3 | 4–58 | 2–76 |
| A2 | 47 | 30 | 4 | 3 | 4–55 | 2–72 |
| A3 | 29 | 22 | 1 | 1 | 1–73 | 1–92 |
| A4 | 30 | 35 | 3 | 3 | 3–77 | 2–70 |
| A5 | 42 | 45 | 3 | 3 | 3–68 | 2–59 |
| B1 | 30 | 24 | 4 | 2 | 3–74 | 2–79 |
| B2 | 41 | 30 | 4 | 2 | 4–68 | 2–75 |
| B3 | 56 | 30 | 5 | 2 | 5–66 | 2–75 |
| B4 | 42 | 27 | 3 | 2 | 3–61 | 1–76 |
| B5 | 48 | 33 | 7 | 3 | 7–55 | 3–67 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bolysbek, D.A.; Kuljabekov, A.B.; Uzbekaliyev, K.S.; Assilbekov, B.K. Effect of Rock Dissolution on Two-Phase Relative Permeabilities: Pore-Scale Simulations Based on Experimental Data. Appl. Sci. 2023, 13, 11385. https://doi.org/10.3390/app132011385
Bolysbek DA, Kuljabekov AB, Uzbekaliyev KS, Assilbekov BK. Effect of Rock Dissolution on Two-Phase Relative Permeabilities: Pore-Scale Simulations Based on Experimental Data. Applied Sciences. 2023; 13(20):11385. https://doi.org/10.3390/app132011385
Chicago/Turabian StyleBolysbek, Darezhat A., Alibek B. Kuljabekov, Kenbai Sh Uzbekaliyev, and Bakytzhan K. Assilbekov. 2023. "Effect of Rock Dissolution on Two-Phase Relative Permeabilities: Pore-Scale Simulations Based on Experimental Data" Applied Sciences 13, no. 20: 11385. https://doi.org/10.3390/app132011385
APA StyleBolysbek, D. A., Kuljabekov, A. B., Uzbekaliyev, K. S., & Assilbekov, B. K. (2023). Effect of Rock Dissolution on Two-Phase Relative Permeabilities: Pore-Scale Simulations Based on Experimental Data. Applied Sciences, 13(20), 11385. https://doi.org/10.3390/app132011385







