A Framework to Determine the Utilization of Vacant Taxis on HOV Lanes with the Optimal Deployment
Abstract
:1. Introduction
2. Preliminaries
- the demand between OD pair
- the demand for travel mode between OD pair
- the demand for vacant taxis between OD pair
- the value of time of travelers
- the value of waiting time of taxi passengers
- the area of origin
- the length of link
- the capacity of link
- the operation cost per unit distance of solo-driving travelers
- the flag–fall price per ride
- the mileage-based fare per ride
- the delay-based fare per ride
- the operation cost per unit distance of vacant taxis
- travel time function on link , which is strictly increasing, convex, and continuous with the flow on link
- A binary constant that equals 1 when link is on the path between OD pair and 0 otherwise.
- the flow of travel mode on path between OD pair
- the flow of vacant taxis on path between OD pair
- the vehicle flow on link
- the vehicle flow of mode on link
- the vehicle flow of vacant taxis on link
3. Formulations
3.1. Cost Functions
3.2. Model Constraints
3.3. Travel Mode Split and Path Choice
3.4. Varational Inequality
4. The Network Performance
5. Optimal Deployment of HOV Lanes
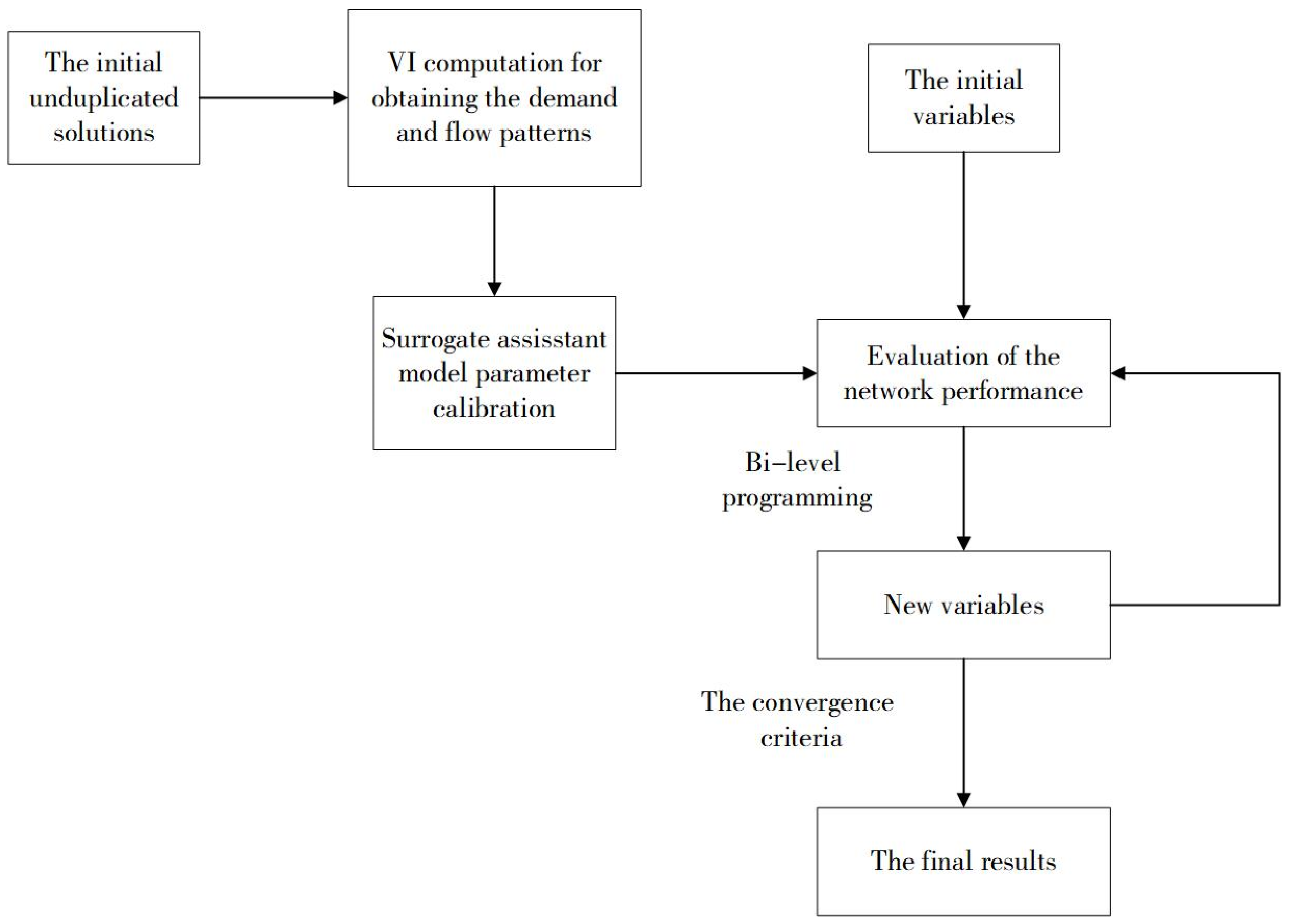
6. Algorithm
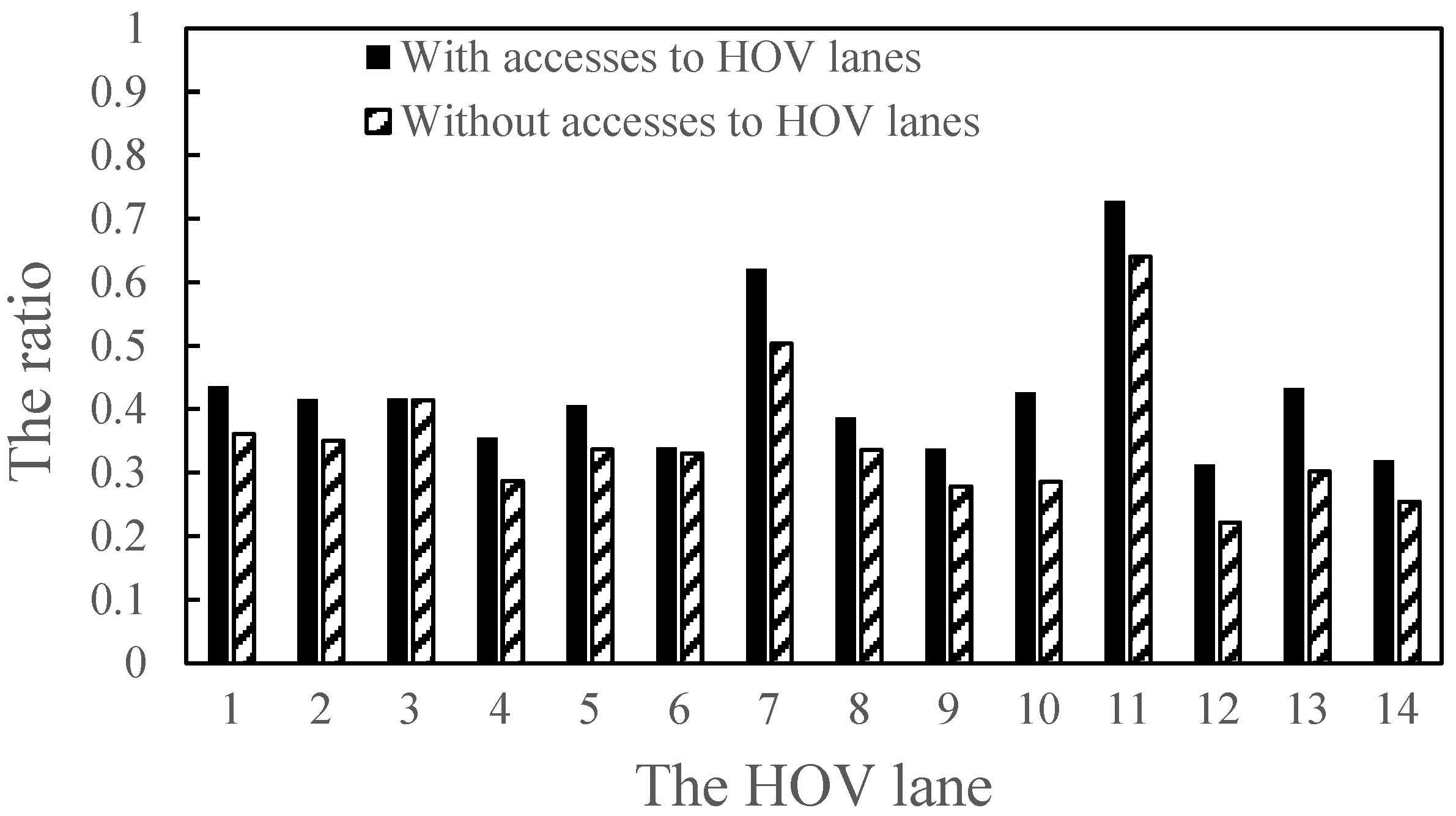
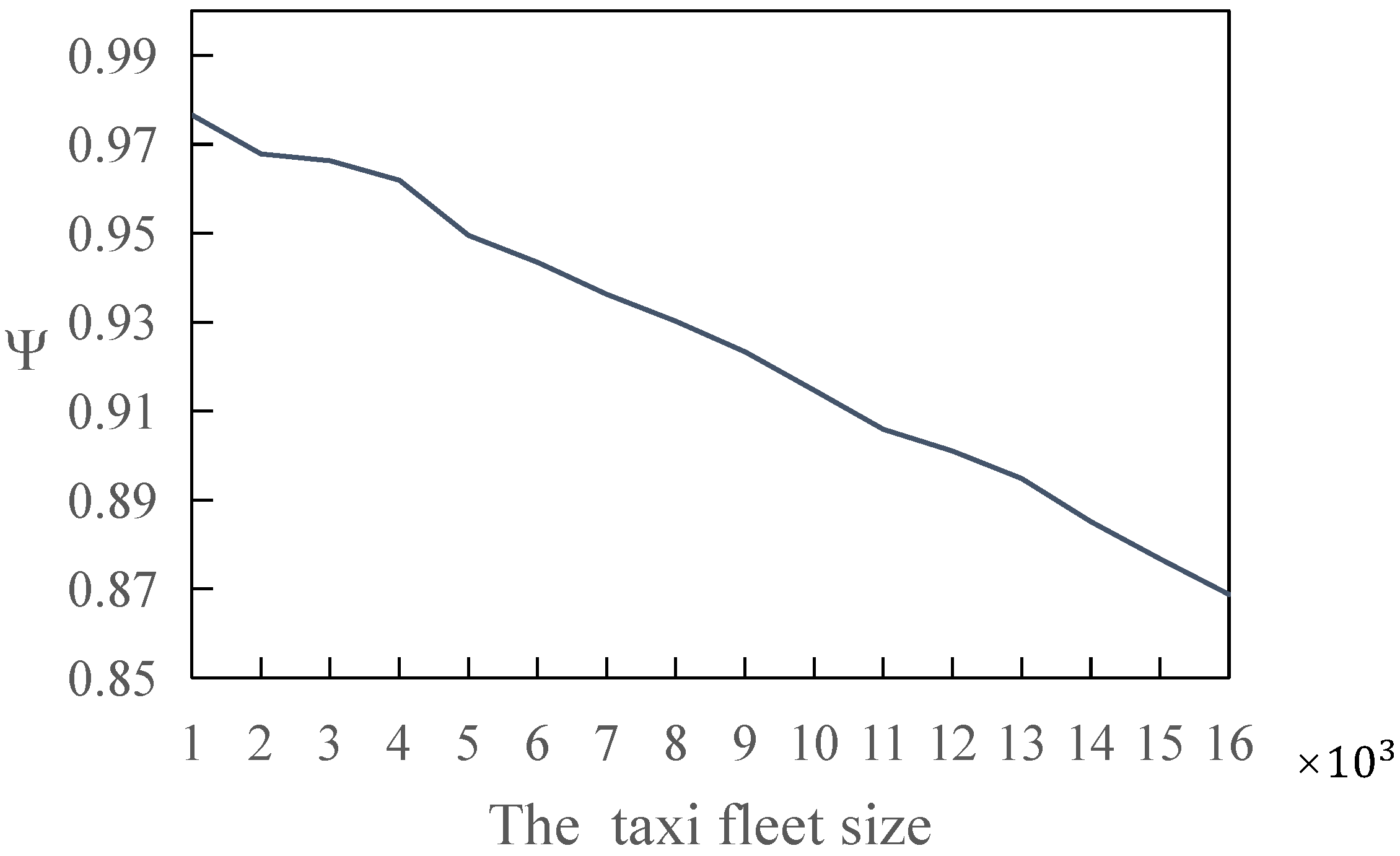
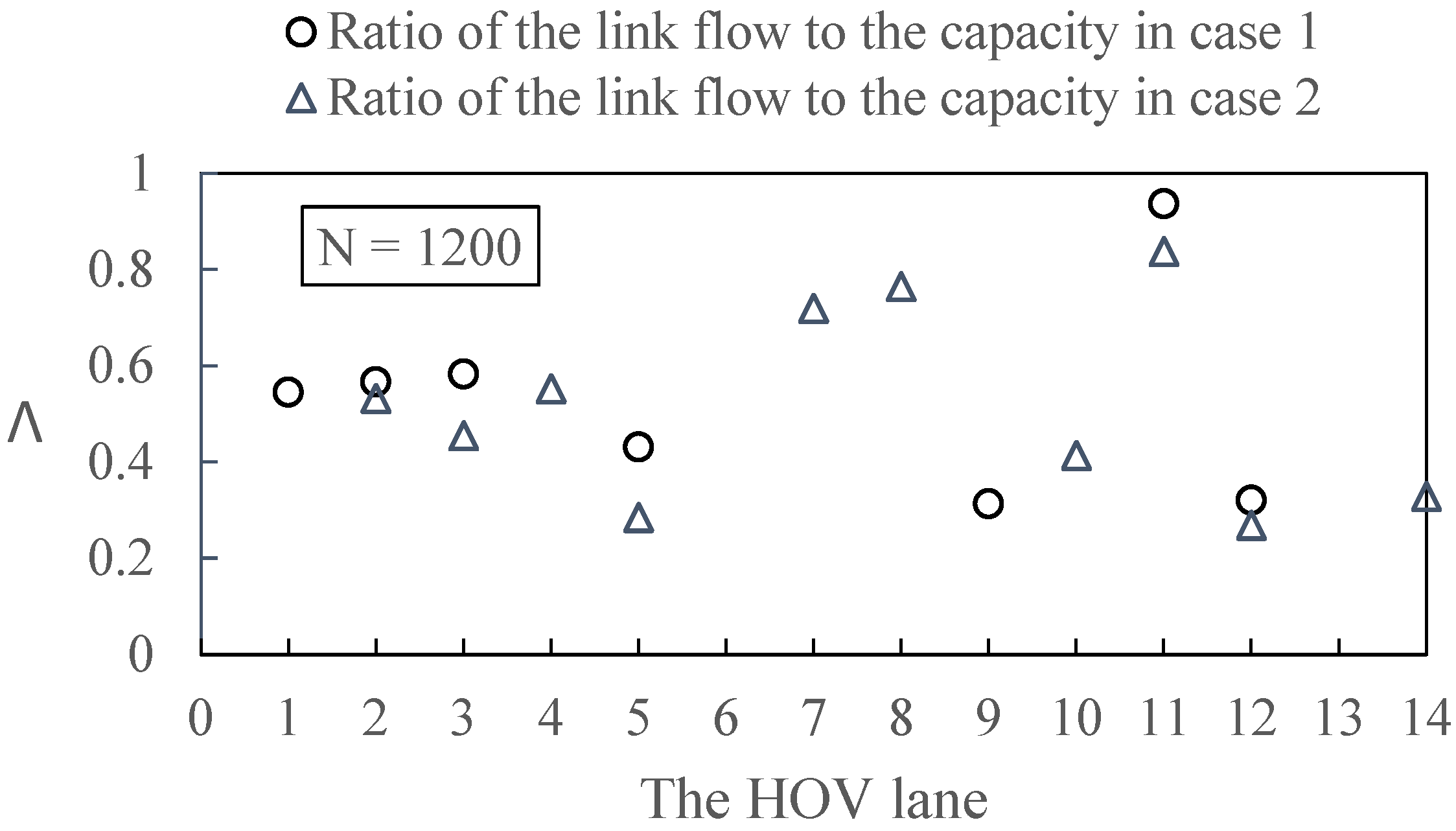
7. Numerical Examples
7.1. Simple Network Example
7.2. Sioux Falls Network Example
8. Conclusions
- (1)
- A paradox similar to the Braess paradox is revealed. Given HOV lane deployment, allowing vacant taxis to use HOV lanes may be negative for maximization of social welfare with intensive usage of HOV lanes. This paradox may derive from the congestion in GP lanes and the capacity limits of the bottlenecks, instead of the congestion in HOV lanes. Allowing vacant taxis to use HOV lanes can intensify the utilization of the HOV lanes while decreasing social welfare.
- (2)
- Considering optimization of HOV lane deployment, allowing vacant taxis to use HOV lanes is more likely negative to social welfare due to the paradox, although the optimal deployments are different in two cases.
- (3)
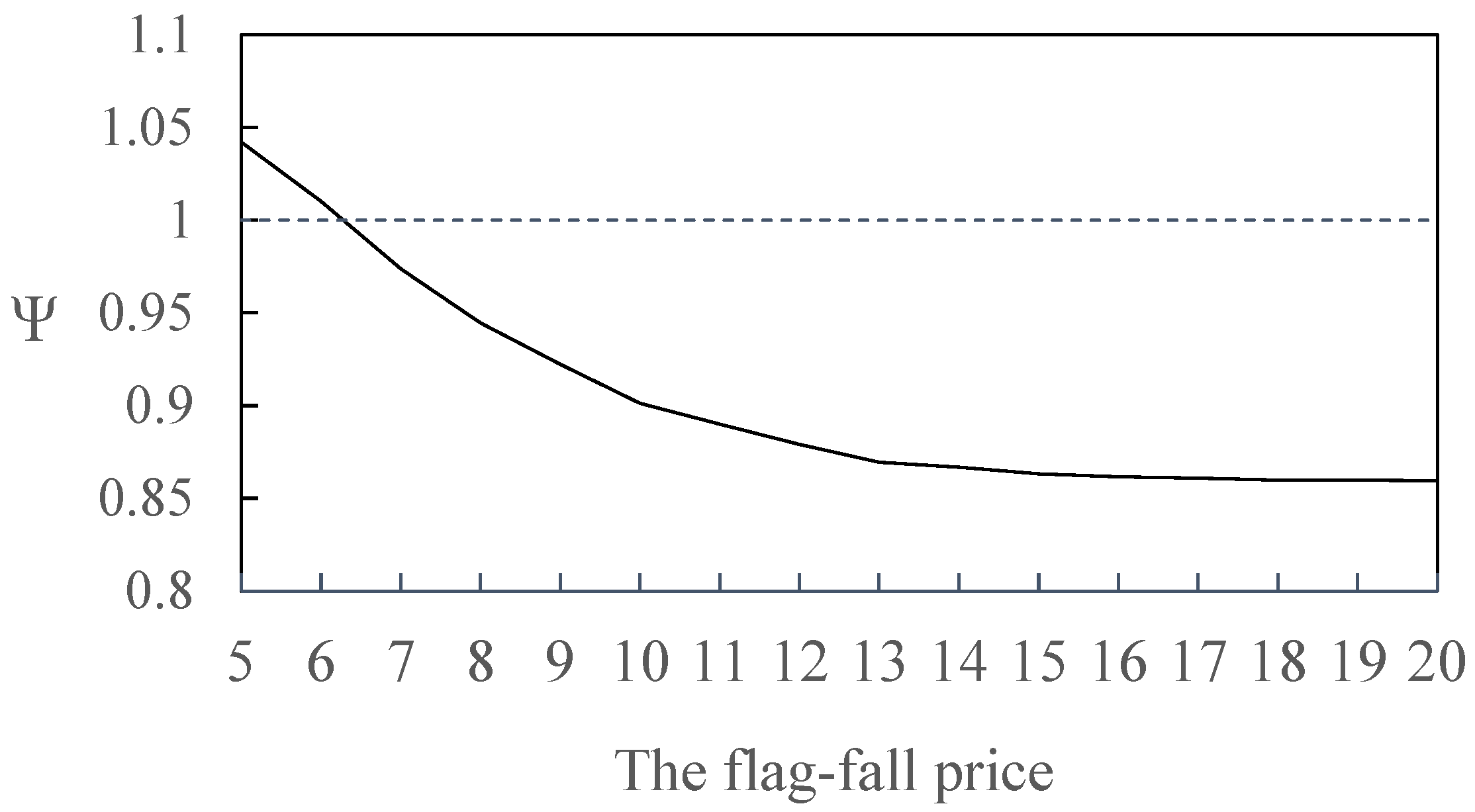
- The paradox depends on the topology and the parameters of the network, e.g., the flag–fall price of taxis. If the paradox is inexistent, the scheme may be beneficial to the traffic system. Therefore, specific information and analysis are necessary to determine whether to forbid or allow vacant taxis to use HOV lanes.
- (4)
- The models and the algorithms can be used in reality.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schroeter, J.R. A model of taxi service under fare structure and fleet size regulation. Bell J. Econ. 1983, 14, 81–96. [Google Scholar] [CrossRef]
- Moore, A.T.; Balaker, T. Do economists reach a conclusion on taxi deregulation? Econ. J. Watch. 2006, 3, 109. [Google Scholar]
- Loo, B.P.Y.; Leung, B.S.Y.; Wong, S.C.; Yang, H. Taxi license premiums in Hong Kong: Can their fluctuations be explained by taxi as a mode of public transport? Int. J. Sustain. Transp. 2007, 1, 249–266. [Google Scholar] [CrossRef]
- Douglas, G.W. Price regulation and optimal service standards: The taxicab industry. J. Transp. Econ. Policy 1972, 6, 116–127. [Google Scholar]
- Daganzo, C.F. An approximate analytic model of many-to-many demand responsive transportation systems. Transp. Res. 1978, 12, 325–333. [Google Scholar] [CrossRef]
- Arnott, R. Taxi travel should be subsidized. J. Urban Econ. 1996, 40, 316–333. [Google Scholar] [CrossRef]
- Yang, H.; Wong, S.C. A network model of urban taxi services. Transp. Res. B-Methodol. 1998, 32, 235–246. [Google Scholar] [CrossRef]
- Wong, K.I.; Wong, S.C.; Yang, H. Modeling urban taxi services in congested road networks with elastic demand. Transp. Res. B-Methodol. 2001, 35, 819–842. [Google Scholar] [CrossRef]
- Yang, H.; Ye, M.; Tang, W.H.; Wong, S.C. Regulating taxi services in the presence of congestion externality. Transp. Res. A-Policy 2005, 39, 17–40. [Google Scholar] [CrossRef]
- Wong, K.I.; Wong, S.C.; Yang, H.; Wu, J.H. Modeling urban taxi services with multiple user classes and vehicle modes. Transp. Res. B-Methodol. 2008, 42, 985–1007. [Google Scholar] [CrossRef]
- Yang, H.; Fung, C.S.; Wong, K.I.; Wong, S.C. Nonlinear pricing of taxi services. Transp. Res. A-Policy 2010, 44, 337–348. [Google Scholar] [CrossRef]
- Yang, H.; Leung, C.W.Y.; Wong, S.C.; Bell, M.G. Equilibria of bilateral taxi–customer searching and meeting on networks. Transp. Res. B-Methodol. 2010, 44, 1067–1083. [Google Scholar] [CrossRef]
- Li, B.; Szeto, W.Y. Taxi service area design: Formulation and analysis. Transp. Res. E-Logist. 2019, 125, 308–333. [Google Scholar] [CrossRef]
- He, F.; Shen, Z.-J.M. Modeling taxi services with smartphone-based e-hailing applications. Transp. Res. C-Emerg. 2015, 58, 93–106. [Google Scholar] [CrossRef]
- Wang, X.; He, F.; Yang, H.; Gao, H.O. Pricing strategies for a taxi-hailing platform. Transp. Res. E-Logist. 2016, 93, 212–231. [Google Scholar] [CrossRef]
- Nie, Y.M. How can the taxi industry survive the tide of ridesourcing? Evidence from Shenzhen, China. Transp. Res. C-Emerg. 2017, 79, 242–256. [Google Scholar] [CrossRef]
- McMullen, B.S.; Gut, T. HOV lane effectiveness in controlling traffic congestion. Transp. Q. 1992, 46, 429–434. [Google Scholar]
- Stamos, I.; Kitis, G.; Basbas, S.; Tzevelekis, I. Evaluation of a high occupancy vehicle lane in central business district Thessaloniki. Procedia-Soc. Behav. Sci. 2012, 48, 1088–1096. [Google Scholar] [CrossRef] [Green Version]
- Giuliano, G.; Levine, D.W.; Teal, R.F. Impact of high occupancy vehicle lanes on carpooling behavior. Transportation 1990, 17, 159–177. [Google Scholar] [CrossRef]
- Chang, M.; Wiegmann, J.; Smith, A.; Bilotto, C. A Review of HOV Lane Performance and Policy Options in the United States; No. FHWA-HOP-09-029; Federal Highway Administration: Washington, DC, USA, 2008.
- Kwon, J.; Varaiya, P. Effectiveness of California’s high occupancy vehicle (HOV) system. Transp. Res. C-Emerg. 2008, 16, 98–115. [Google Scholar] [CrossRef] [Green Version]
- Dahlgren, J. High occupancy vehicle lanes: Not always more effective than general purpose lanes. Transp. Res. A-Policy 1998, 32, 99–114. [Google Scholar] [CrossRef] [Green Version]
- Fontes, T.; Fernandes, P.; Rodrigues, H.; Bandeira, J.M.; Pereira, S.R.; Khattak, A.J.; Coelho, M.C. Are HOV/eco-lanes a sustainable option to reducing emissions in a medium-sized European city? Transp. Res. A-Policy 2014, 63, 93–106. [Google Scholar] [CrossRef]
- Nesamani, K.S.; Lianyu, C.H.U.; Recker, W. Policy implications of incorporating hybrid vehicles into high-occupancy vehicle lanes. J. Transp. Syst. Eng. Inf. Technol. 2010, 10, 30–41. [Google Scholar] [CrossRef]
- Sheldon, T.L.; DeShazo, J.R. How does the presence of HOV lanes affect plug-in electric vehicle adoption in California? A generalized propensity score approach. J. Environ. Econ. Manag. 2017, 85, 146–170. [Google Scholar] [CrossRef]
- Tal, G.; Nicholas, M.A.; Davies, J.; Woodjack, J. Charging behavior impacts on electric vehicle miles traveled: Who is not plugging in? Transp. Res. Rec. 2014, 2454, 53–60. [Google Scholar] [CrossRef]
- Song, Z.; Yin, Y.; Lawphongpanich, S. Optimal deployment of managed lanes in general networks. Int. J. Sustain. Transp. 2015, 9, 431–441. [Google Scholar] [CrossRef]
- Gardner, L.M.; Bar-Gera, H.; Boyles, S.D. Development and comparison of choice models and tolling schemes for high-occupancy/toll (HOT) facilities. Transp. Res. B-Methodol. 2013, 55, 142–153. [Google Scholar] [CrossRef]
- Dahlgren, J. High-occupancy/toll lanes: Where should they be implemented? Transp. Res. A-Policy 2002, 36, 239–255. [Google Scholar] [CrossRef]
- Yang, H.; Huang, H.J. Carpooling and congestion pricing in a multilane highway with high-occupancy-vehicle lanes. Transp. Res. A-Policy 1999, 33, 139–155. [Google Scholar] [CrossRef]
- Dafermos, S. Traffic equilibrium and variational inequalities. Transp. Sci. 1980, 14, 42–54. [Google Scholar] [CrossRef] [Green Version]
- Nagurney, A. Network Economics: A Variational Inequality Approach; Springer Science & Business Media: Berlin, Germany, 1998. [Google Scholar]
- Ma, J.; Xu, M.; Meng, Q.; Cheng, L. Ridesharing user equilibrium problem under OD-based surge pricing strategy. Transp. Res. B-Methodol. 2020, 134, 1–24. [Google Scholar] [CrossRef]
- Ma, J.; Meng, Q.; Cheng, L.; Liu, Z. General stochastic ridesharing user equilibrium problem with elastic demand. Transp. Res. B-Methodol. 2022, 162, 162–194. [Google Scholar] [CrossRef]
- Ni, L.; Chen, C.; Wang, X.C.; Chen, X.M. Modeling network equilibrium of competitive ride-sourcing market with heterogeneous transportation network companies. Transp. Res. C-Emerg. 2021, 130, 103277. [Google Scholar] [CrossRef]
- Xie, T.; Liu, Y. Impact of connected and autonomous vehicle technology on market penetration and route choices. Transp. Res. C-Emerg. 2022, 139, 103646. [Google Scholar] [CrossRef]
- Yang, H.; Wong, S.C.; Wong, K.I. Demand–supply equilibrium of taxi services in a network under competition and regulation. Transp. Res. B-Methodol. 2002, 36, 799–819. [Google Scholar] [CrossRef]
- Zhu, J.; Xiao, F.; Liu, X. Taxis in road pricing zone: Should they pay the congestion charge? J. Adv. Transp. 2015, 49, 69–113. [Google Scholar] [CrossRef]
- Wang, X.; Wang, J.; Guo, L.; Liu, W.; Zhang, W. A convex programming approach for ridesharing user equilibrium under fixed driver/rider demand. Transp. Res. B-Methodol. 2021, 149, 33–51. [Google Scholar] [CrossRef]
- Long, J.; Gao, Z.; Zhang, H.; Szeto, W.Y. A turning restriction design problem in urban road networks. Eur. J. Oper. Res. 2010, 206, 569–578. [Google Scholar] [CrossRef] [Green Version]
- Gao, Z.; Wu, J.; Sun, H. Solution algorithm for the bi-level discrete network design problem. Transp. Res. B-Methodol. 2005, 39, 479–495. [Google Scholar] [CrossRef]
- Wu, J.J.; Sun, H.J.; Gao, Z.Y.; Zhang, H.Z. Reversible lane-based traffic network optimization with an advanced traveller information system. Eng. Optim. 2009, 41, 87–97. [Google Scholar] [CrossRef]
- Farahani, R.Z.; Miandoabchi, E.; Szeto, W.Y.; Rashidi, H. A review of urban transportation network design problems. Eur. J. Oper. Res. 2013, 229, 281–302. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Zhang, L.; He, X.; Xiong, C.; Li, Z. Surrogate-based optimization of expensive-to-evaluate objective for optimal highway toll charges in transportation network. Comput.-Aided Civ. Infrastruct. Eng. 2014, 29, 359–381. [Google Scholar] [CrossRef]
- Yan, C.Y.; Jiang, R.; Gao, Z.Y.; Shao, H. Effect of speed limits in degradable transport networks. Transp. Res. C-Emerg. 2015, 56, 94–119. [Google Scholar] [CrossRef]
- Tam, M.L.; Lam, W.H.K. Analysis of demand for road-based transport facilities: A bi-level programming approach. Transp. Res. Rec. 1999, 1685, 73–80. [Google Scholar] [CrossRef]


| D | 1 | 2 | 3 | 4 | 5 | 6 | |
|---|---|---|---|---|---|---|---|
| O | |||||||
| 1 | 0 | 600 | 800 | 600 | 600 | 600 | |
| 2 | 600 | 0 | 600 | 600 | 600 | 600 | |
| 3 | 800 | 600 | 0 | 600 | 800 | 600 | |
| 4 | 600 | 600 | 600 | 0 | 600 | 600 | |
| 5 | 800 | 600 | 800 | 600 | 0 | 600 | |
| 6 | 600 | 600 | 800 | 600 | 600 | 0 | |
| Link | (h) | (veh/h) | (km) | Link | (h) | (veh/h) |
|---|---|---|---|---|---|---|
| 1-2(GP) | 0.03 | 2400 | 2.1 | 4-2(GP) | 0.02 | 3600 |
| 2-1(GP) | 0.03 | 2400 | 2.1 | 3-5(GP) | 0.03 | 2400 |
| 1-3(GP) | 0.03 | 2400 | 2.1 | 5-3(GP) | 0.03 | 2400 |
| 3-1(GP) | 0.03 | 2400 | 2.1 | 5-6(GP) | 0.04 | 2400 |
| 3-4(GP) | 0.04 | 2400 | 2.8 | 6-5(GP) | 0.04 | 2400 |
| 4-3(GP) | 0.04 | 2000 | 2.8 | 4-6(GP) | 0.04 | 2400 |
| 2-4(GP) | 0.02 | 3600 | 1.4 | 6-4(GP) | 0.04 | 2400 |
| 1-2(HOV) | 0.03 | 600 | 2.1 | 4-2(HOV) | 0.02 | 900 |
| 2-1(HOV) | 0.03 | 600 | 2.1 | 3-5(HOV) | 0.03 | 600 |
| 1-3(HOV) | 0.03 | 600 | 2.1 | 5-3(HOV) | 0.03 | 600 |
| 3-1(HOV) | 0.03 | 600 | 2.1 | 5-6(HOV) | 0.04 | 600 |
| 3-4(HOV) | 0.04 | 600 | 2.8 | 6-5(HOV) | 0.04 | 600 |
| 4-3(HOV) | 0.04 | 500 | 2.8 | 4-6(HOV) | 0.04 | 600 |
| 2-4(HOV) | 0.02 | 900 | 1.4 | 6-4(HOV) | 0.04 | 600 |
| Social Welfare | Solo-Driving Demand | Carpooling Demand | Occupied Taxi Demand | |
|---|---|---|---|---|
| Case 1 | 413,638 | 11,849 | 909 | 1399 |
| Case 2 | 421,983 | 11,808 | 939 | 1443 |
| Social Welfare | Solo-Driving Demand | Carpooling Demand | Occupancy Taxi Demand | |
|---|---|---|---|---|
| Case 1 | 160,739 | 6822 | 189 | 3030 |
| Case 2 | 162,542 | 6820 | 188 | 3036 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, F.; Liu, K.; Ding, D. A Framework to Determine the Utilization of Vacant Taxis on HOV Lanes with the Optimal Deployment. Appl. Sci. 2023, 13, 913. https://doi.org/10.3390/app13020913
Gao F, Liu K, Ding D. A Framework to Determine the Utilization of Vacant Taxis on HOV Lanes with the Optimal Deployment. Applied Sciences. 2023; 13(2):913. https://doi.org/10.3390/app13020913
Chicago/Turabian StyleGao, Fawen, Kun Liu, and Dong Ding. 2023. "A Framework to Determine the Utilization of Vacant Taxis on HOV Lanes with the Optimal Deployment" Applied Sciences 13, no. 2: 913. https://doi.org/10.3390/app13020913
APA StyleGao, F., Liu, K., & Ding, D. (2023). A Framework to Determine the Utilization of Vacant Taxis on HOV Lanes with the Optimal Deployment. Applied Sciences, 13(2), 913. https://doi.org/10.3390/app13020913




