Observer-Based Model Reference Tracking Control of the Markov Jump System with Partly Unknown Transition Rates
Abstract
:1. Introduction
2. Problem Description and Preliminaries
3. Observer and Control Law Design
3.1. Observer Design
| (1), | (2), |
| (3), | (4), |
| (5), | (6). |
3.2. Control Law Design
4. Parameter Solutions
4.1. State Feedback Control Law Design
4.2. Feedforward Control Law Design
4.3. Algorithm for Solving the Controller
- According to Theorem 2, compute the state feedback gain matrix.
- Judge whether the matrix pair is controllable. If it is controllable, solve Lemma 3, and go on to the next step; otherwise, the feedforward compensator does not exist.
- Compute and based on Lemma 4, then compute the gain matrix of the feedforward compensator.
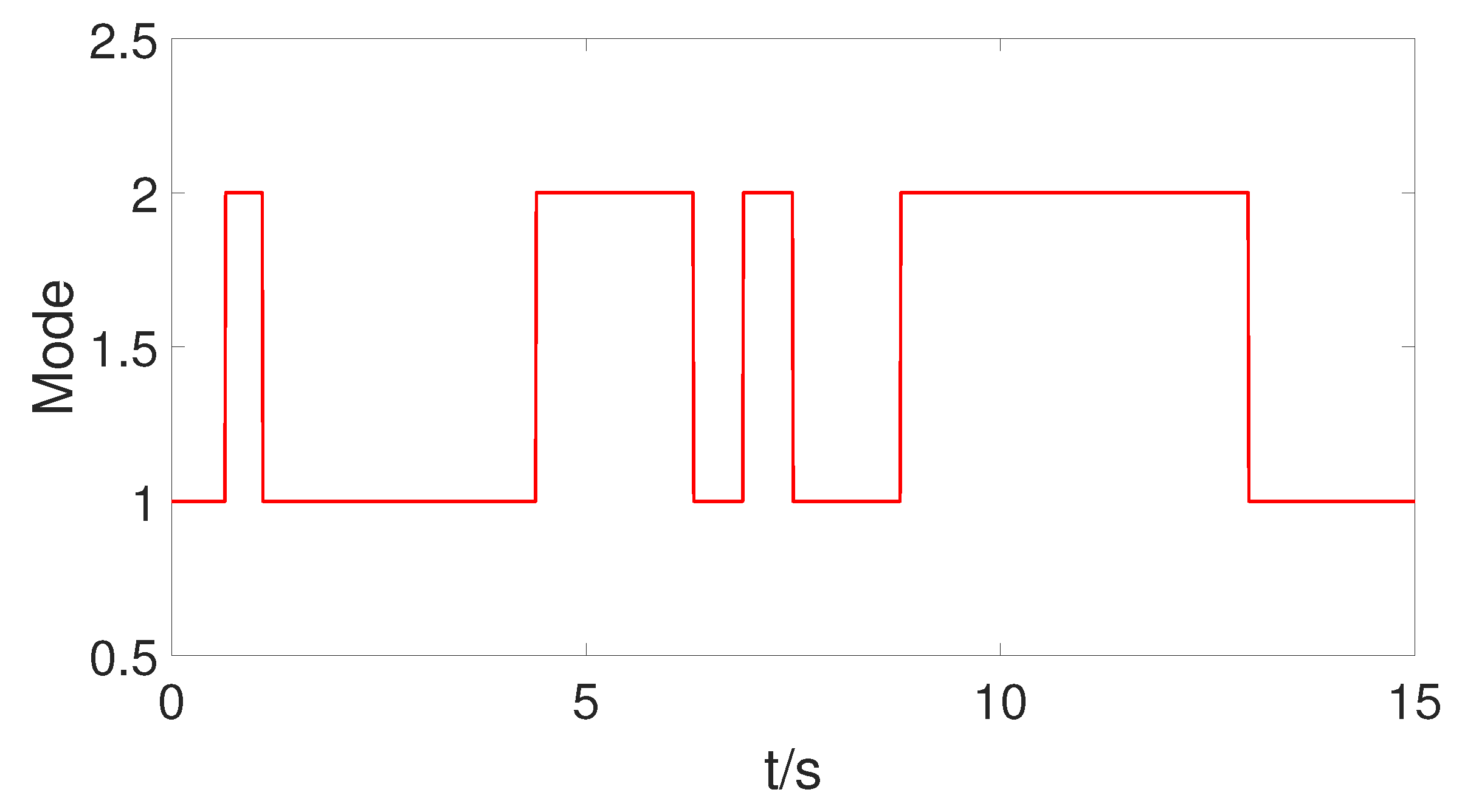
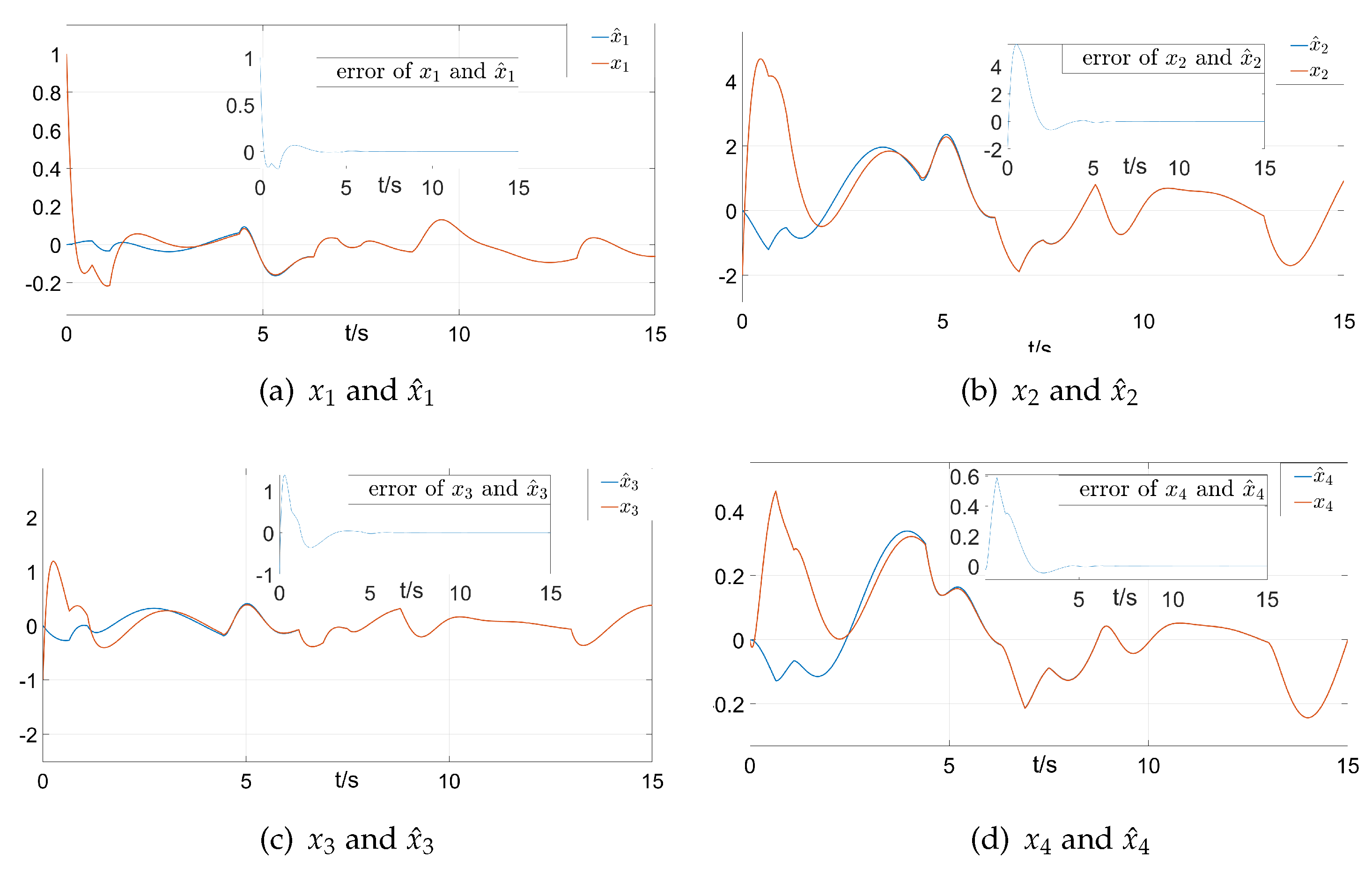
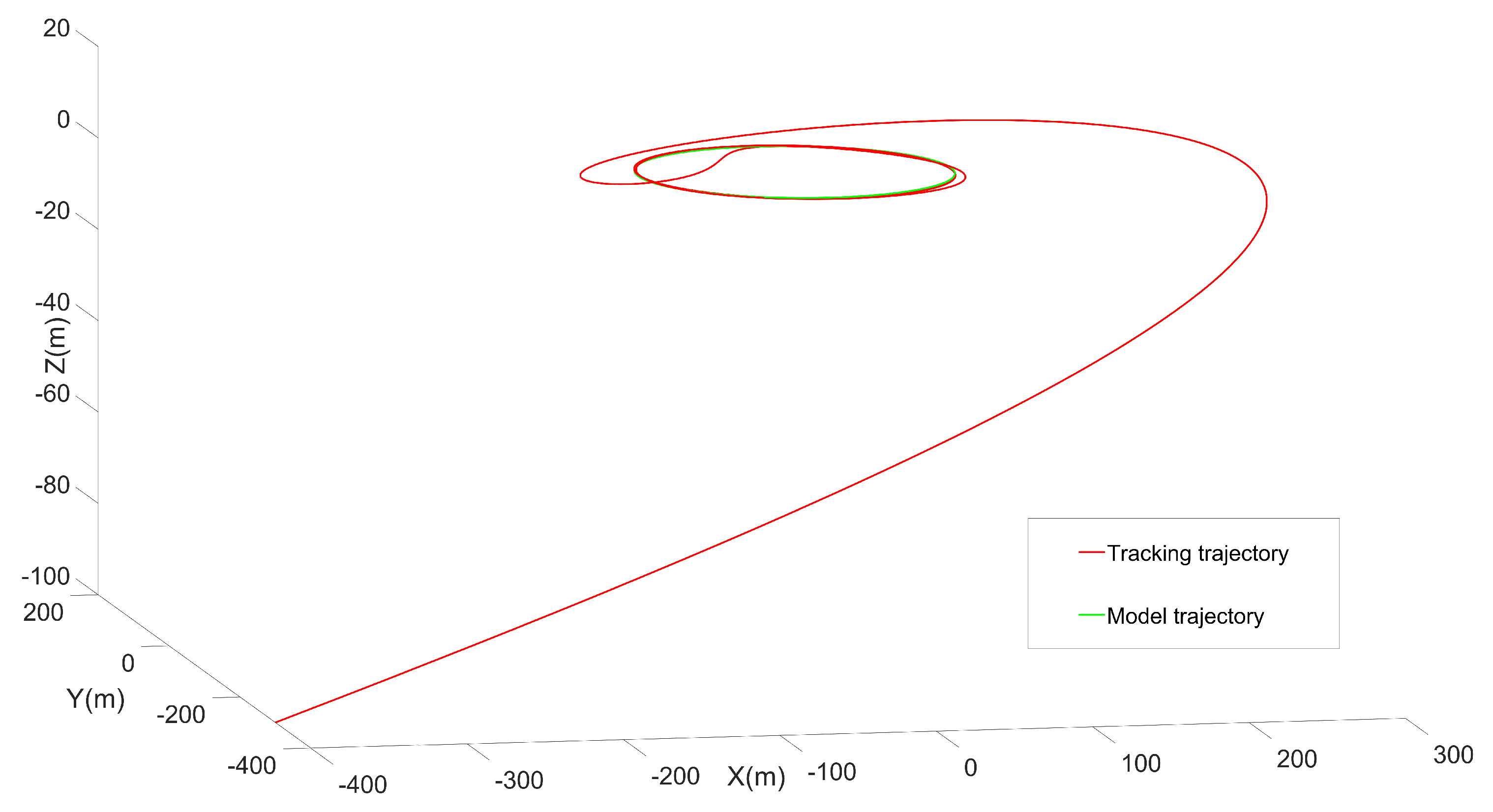
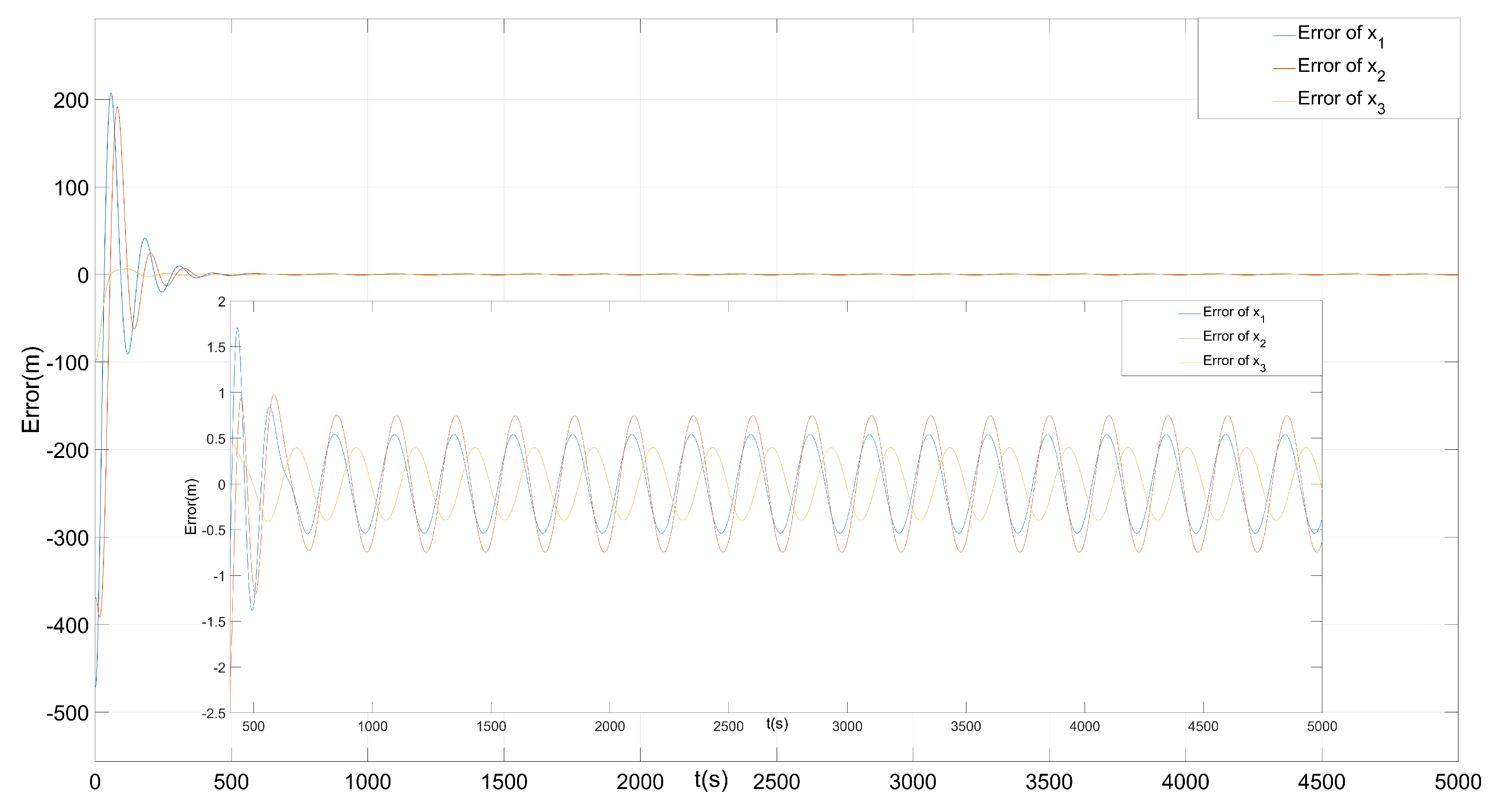
5. Numerical Example
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| (1), | (2), |
| (3), | (4), |
| (5), | (6), |
| (7), | (8). |
References
- Ji, Y.; Chizeck, H.J. Controllability, stabilizability, and continuous-time Markovian jump linear quadratic control. IEEE Trans. Autom. Control 1990, 35, 777–788. [Google Scholar] [CrossRef]
- Hou, T.; Liu, Y.; Deng, F. Stability for discrete-time uncertain systems with infinite Markov jump and time-delay. Sci. China Inf. Sci. 2021, 64, 152202. [Google Scholar] [CrossRef]
- Wang, P.; Wang, W.; Su, H.; Feng, J. Stability of stochastic discrete-time piecewise homogeneous Markov jump systems with time delay and impulsive effects. Nonlinear Anal. Hybrid Syst. 2020, 38, 100916. [Google Scholar] [CrossRef]
- Rakkiyappan, R.; Chandrasekar, A.; Lakshmanan, S.; Park, J. Exponential stability for markovian jumping stochastic BAM neural networks with mode-dependent probabilistic time-varying delays and impulse control. Complex 2015, 20, 39–65. [Google Scholar] [CrossRef]
- Zhang, L.; Boukas, E.K. Stability and stabilization of Markovian jump linear systems with partly unknown transition probabilities. Automatica 2009, 45, 463–468. [Google Scholar] [CrossRef]
- Xia, Y.; Boukas, E.K.; Shi, P.; Zhang, J. Stability and stabilization of continuous-time singular hybrid systems. Automatica 2009, 45, 1504–1509. [Google Scholar] [CrossRef]
- Zhang, X.; Baron, L.; Liu, Q.; Boukas, E.K. Design of Stabilizing Controllers With a Dynamic Gain for Feedforward Nonlinear Time-Delay Systems. IEEE Trans. Autom. Control 2011, 56, 692–697. [Google Scholar] [CrossRef]
- Boukas, E.K. Stabilization of stochastic singular nonlinear hybrid systems. Nonlinear-Anal.-Theory Methods Appl. 2006, 64, 217–228. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, Q.; Baron, L.; Boukas, E.K. Feedback stabilization for high order feedforward nonlinear time-delay systems. Automatica 2011, 47, 962–967. [Google Scholar] [CrossRef]
- Ji, H.; Cui, B.; Liu, X. Adaptive control of Markov jump distributed parameter systems via model reference. Fuzzy Sets Syst. 2020, 392, 115–135. [Google Scholar]
- Tan, M.; Mai, J.; Song, Z. Exponential H∞ output tracking control for coupled switched systems with states of different dimensions. Int. J. Control 2021, 94, 190–201. [Google Scholar] [CrossRef]
- Carravetta, F.; Sorge, M.M. Model reference adaptive expectations in Markov-switching economies. Econ. Model. 2013, 32, 551–559. [Google Scholar] [CrossRef]
- Chen, Z.; Hu, H.; Wu, Y.; Zhang, Y.; Li, G.; Liu, Y. Stochastic model predictive control for energy management of power-split plug-in hybrid electric vehicles based on reinforcement learning. Energy 2020, 211, 118931. [Google Scholar] [CrossRef]
- Zhao, X.; Guo, G. Model Reference Adaptive Control of Vehicle Slip Ratio Based on Speed Tracking. Appl. Sci. 2020, 10, 3459. [Google Scholar] [CrossRef]
- Peng, L.; Yin, A.; Song, W.; Yao, W.; Ren, H.; Yang, L. Sleep Monitoring With Hidden Markov Model for Physical Conditions Tracking. IEEE Sens. J. 2021, 21, 14232–14239. [Google Scholar] [CrossRef]
- Nguyen, H.Q. Observer-Based Tracking Control for Polysolenoid Linear Motor with Unknown Disturbance Load. Actuators 2020, 9, 23. [Google Scholar] [CrossRef] [Green Version]
- Lala, T.; Chirla, D.; Radac, M. Model Reference Tracking Control Solutions for a Visual Servo System Based on a Virtual State from Unknown Dynamics. Energies 2021, 15, 267. [Google Scholar] [CrossRef]
- Boukas, E.K. On reference model tracking for Markov jump systems. Int. J. Syst. Sci. 2009, 40, 393–401. [Google Scholar] [CrossRef]
- Fu, Y.; Lu, Y.; Zhang, M. Model reference tracking control of continuous-time periodic linear systems with actuator jumping fault and its applications in orbit maneuvering. Int. J. Control. Autom. Syst. 2017, 15, 2182–2192. [Google Scholar] [CrossRef]
- Afri, C.; Andrieu, V.; Bako, L.; Dufour, P. State and Parameter Estimation: A Nonlinear Luenberger Observer Approach. IEEE Trans. Autom. Control 2017, 62, 973–980. [Google Scholar] [CrossRef] [Green Version]
- CastroRego, F.; Pu, Y.; Alessandretti, A.; Aguiar, A.; Pascoal, A.M.; Jones, C.N. A Distributed Luenberger Observer for Linear State Feedback Systems With Quantized and Rate-Limited Communications. IEEE Trans. Autom. Control 2021, 66, 3922–3937. [Google Scholar]
- Yin, Z.; Bai, C.; Du, N.; Du, C.; Liu, J. Research on Internal Model Control of Induction Motors Based on Luenberger Disturbance Observer. IEEE Trans. Power Electron. 2021, 36, 8155–8170. [Google Scholar] [CrossRef]
- Bejarano, F.J.; Mera, M. Robust Luenberger-like observer for control of linear switched systems under arbitrary unknown switched function. Asian J. Control. 2020, 23, 2527–2536. [Google Scholar] [CrossRef]
- Rincón, A.; Restrepo, G.M.; Velasco, F.E. A Robust Observer-Based Adaptive Control of Second—Order Systems with Input Saturation via Dead-Zone Lyapunov Functions. Computation 2021, 5, 82. [Google Scholar] [CrossRef]
- Al-Gabalawy, M.; Mahmoud, K.; Darwish, M.M.; Dawson, J.A.; Lehtonen, M.; Hosny, N.S. Reliable and Robust Observer for Simultaneously Estimating State-of-Charge and State-of-Health of LiFePO4 Batteries. Appl. Sci. 2021, 11, 3609. [Google Scholar] [CrossRef]
- Hua, H.; Fang, Y.; Zhang, X.; Lu, B. A Novel Robust Observer-Based Nonlinear Trajectory Tracking Control Strategy for Quadrotors. IEEE Trans. Control. Syst. Technol. 2021, 29, 1952–1963. [Google Scholar] [CrossRef]
- Yao, D.; Lu, R.; Xu, Y.; Ren, H. Observer-based sliding mode control of Markov jump systems with random sensor delays and partly unknown transition rates. Int. J. Syst. Sci. 2017, 48, 2985–2996. [Google Scholar] [CrossRef]
- Jiang, B.; Shi, P.; Mao, Z. Sliding Mode Observer-Based Fault Estimation for Nonlinear Networked Control Systems. Circuits, Syst. Signal Process. 2011, 30, 1–16. [Google Scholar] [CrossRef]
- Vijayakumar, M.; Sakthivel, R.; Mohammadzadeh, A.; Karthick, S.A.; Anthoni, S.M. Proportional integral observer based tracking control design for Markov jump systems. Appl. Math. Comput. 2021, 410, 126467. [Google Scholar] [CrossRef]
- Wu, L.; Shi, P.; Gao, H. State Estimation and Sliding-Mode Control of Markovian Jump Singular Systems. IEEE Trans. Autom. Control 2010, 55, 1213–1219. [Google Scholar]
- Huo, S.; Ge, L.; Li, F. Robust H∞ Consensus for Markov Jump Multiagent Systems Under Mode-Dependent Observer and Quantizer. IEEE Syst. J. 2020, 15, 2443–2450. [Google Scholar] [CrossRef]
- Yang, H.; Yin, S. Descriptor Observers Design for Markov Jump Systems with Simultaneous Sensor and Actuator Faults. IEEE Trans. Autom. Control 2019, 64, 3370–3377. [Google Scholar] [CrossRef]
- Lei, C.; Zhang, H.; Wang, L.; Liu, L.; Ma, D. Incomplete information Markov game theoretic approach to strategy generation for moving target defense. Comput. Commun. 2018, 116, 184–199. [Google Scholar] [CrossRef]
- Ashkenazi-Golan, G.; Rainer, C.; Solan, E. Solving two-state Markov games with incomplete information on one side. Games Econ. Behav. 2020, 122, 83–104. [Google Scholar] [CrossRef] [Green Version]
- Cui, D.; Wang, Y.; Su, H.; Xu, Z.; Que, H. Fuzzy-model-based tracking control of Markov jump nonlinear systems with incomplete mode information. J. Frankl. Inst. 2021, 358, 3633–3650. [Google Scholar] [CrossRef]
- Fang, H.; Zhu, G.; Stojanovic, V.; Nie, R.; He, S.; Luan, X.; Liu, F. Adaptive optimization algorithm for nonlinear Markov jump systems with partial unknown dynamics. Int. J. Robust Nonlinear Control 2021, 31, 2126–2140. [Google Scholar] [CrossRef]
- Li, F.; Xu, S.; Shen, H.; Ma, Q. Passivity-Based Control for Hidden Markov Jump Systems With Singular Perturbations and Partially Unknown Probabilities. IEEE Trans. Autom. Control 2020, 65, 3701–3706. [Google Scholar] [CrossRef]
- Zhang, L.; Lam, J. Necessary and Sufficient Conditions for Analysis and Synthesis of Markov Jump Linear Systems With Incomplete Transition Descriptions. IEEE Trans. Autom. Control 2010, 55, 1695–1701. [Google Scholar] [CrossRef] [Green Version]
- Li, L.; Zhang, Q. Finite-time H∞ control for singular Markovian jump systems with partly unknown transition rates. Appl. Math. Model. 2016, 40, 302–314. [Google Scholar] [CrossRef]
- Shen, M.; Park, J.; Ye, D. A Separated Approach to Control of Markov Jump Nonlinear Systems with General Transition Probabilities. IEEE Trans. Cybern. 2016, 46, 2010–2018. [Google Scholar] [CrossRef]
- Kao, Y.; Xie, J.; Wang, C. Stabilization of Singular Markovian Jump Systems With Generally Uncertain Transition Rates. IEEE Trans. Autom. Control 2014, 59, 2604–2610. [Google Scholar] [CrossRef]
- Park, C.; Kwon, N.K.; Park, I.S.; Park, P. H∞ filtering for singular Markovian jump systems with partly unknown transition rates. Automatica 2019, 109, 108528. [Google Scholar] [CrossRef]
- Shen, A.; Li, L.; Li, C. H∞ Filtering for Discrete-Time Singular Markovian Jump Systems with Generally Uncertain Transition Rates. Circuits, Syst. Signal Process. 2021, 40, 3204–3226. [Google Scholar] [CrossRef]
- Boukas, E.K. Stochastic Switching Systems: Analysis and Design; Birkhauser: Basel, Switzerland; Berlin, Germany, 2005. [Google Scholar]
- Horn, R.A.; Johnson, C.R. Matrix analysis. In Statistical Inference for Engineers and Data Scientists; Cambridge University Press: Cambridge, UK, 2018. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, W.; Jin, A. Observer-Based Model Reference Tracking Control of the Markov Jump System with Partly Unknown Transition Rates. Appl. Sci. 2023, 13, 914. https://doi.org/10.3390/app13020914
Song W, Jin A. Observer-Based Model Reference Tracking Control of the Markov Jump System with Partly Unknown Transition Rates. Applied Sciences. 2023; 13(2):914. https://doi.org/10.3390/app13020914
Chicago/Turabian StyleSong, Weiqiang, and Aijuan Jin. 2023. "Observer-Based Model Reference Tracking Control of the Markov Jump System with Partly Unknown Transition Rates" Applied Sciences 13, no. 2: 914. https://doi.org/10.3390/app13020914
APA StyleSong, W., & Jin, A. (2023). Observer-Based Model Reference Tracking Control of the Markov Jump System with Partly Unknown Transition Rates. Applied Sciences, 13(2), 914. https://doi.org/10.3390/app13020914





