Predictive Modeling of Spring-Back Behavior in V-Bending of SS400 Steel Sheets under Elevated Temperatures Using Combined Hardening Laws
Abstract
1. Introduction
2. Materials and Hardening Model
2.1. Materials
2.2. Hardening Models
3. Experiment and Simulation of V-Shaped Bending at Room Temperature
3.1. Experimental Procedure
3.2. Simulation Procedure
3.3. Combined Hardening Law
4. Experiment and Simulation of V-Shaped Bending at Elevated Temperatures
4.1. Experimental Procedure
4.2. Simulation Procedure for V-Bending at Elevated Temperatures
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Marciniak, S.J.H.Z.; Ducan, J.L. Mechanics of Sheet Metal Forming; Elsevier: Amsterdam, The Netherlands, 2002. [Google Scholar]
- Ahmed, G.M.S.; Ahmed, H.; Mohiuddin, M.V.; Sajid, S.M.S. Experimental Evaluation of Springback in Mild Steel and its Validation Using LS-DYNA. Procedia Mater. Sci. 2014, 6, 1376–1385. [Google Scholar] [CrossRef][Green Version]
- Yoshida, F.; Uemori, T. A model of large-strain cyclic plasticity describing the Bauschinger effect and work hardening stagnation. Int. J. Plast. 2002, 18, 661–686. [Google Scholar] [CrossRef]
- Eggertsen, P.A.; Mattiasson, K. On constitutive modeling for spring-back analysis. Int. J. Mech. Sci. 2010, 52, 804–818. [Google Scholar] [CrossRef]
- Eggertsen, P.A.; Mattiasson, K. On the modelling of the bending-unbending behaviour for accurate spring-back predictions. Int. J. Mech. Sci. 2009, 51, 547–563. [Google Scholar] [CrossRef]
- Yu, H.Y. Variation of elastic modulus during plastic deformation and its influence on spring-back. Mater. Des. 2009, 30, 846–850. [Google Scholar] [CrossRef]
- Armstrong, C.O.; Frederick, P.J. A Mathematical Representation of the Multiaxial Bauschinger Effect; G.E.G.B. Report RD/B/N 731; Berkeley Nuclear Laboratories: Berkeley, CA, USA, 1966. [Google Scholar]
- Stachowicz, F.; Trzepieciński, T.; Pieja, T. Warm forming of stainless steel sheet. Arch. Civ. Mech. Eng. 2010, 10, 85–94. [Google Scholar] [CrossRef]
- Nguyen, D.T.; Park, J.G.; Kim, Y.S. A Study on Yield Function for Ti–6Al–4V Titanium Alloy Sheets at Elevated Temperatures. Trans. Indian Inst. Met. 2016, 69, 1343–1350. [Google Scholar] [CrossRef]
- Bruni, C.; Forcellese, A.; Gabrielli, F.; Simoncini, M. Air bending of AZ31 magnesium alloy in warm and hot forming conditions. J. Mater. Process. Technol. 2006, 177, 373–376. [Google Scholar] [CrossRef]
- Wang, A.; Zhong, K.; El Fakir, O.; Liu, J.; Sun, C.; Wang, L.-L.; Lin, J.; Dean, T.A. Springback analysis of AA5754 after hot stamping: Experiments and FE modelling. Int. J. Adv. Manuf. Technol. 2017, 89, 1339–1352. [Google Scholar] [CrossRef]
- Duc-Toan, N.; Seung-Han, Y.; Dong-Won, J.; Tien-Long, B.; Young-Suk, K. A study on material modeling to predict spring-back in V-bending of AZ31 magnesium alloy sheet at various temperatures. Int. J. Adv. Manuf. Technol. 2012, 62, 551–562. [Google Scholar] [CrossRef]
- Poh, K.W. Stress-strain-temperature relationship for structural steel. J. Mater. Civ. Eng. 2001, 13, 371–379. [Google Scholar] [CrossRef]
- Fei, D.; Hodgson, P. Experimental and numerical studies of springback in air v-bending process for cold rolled TRIP steels. Nucl. Eng. Des. 2006, 236, 1847–1851. [Google Scholar] [CrossRef]
- Garcia-Romeu, M.L.; Ciurana, J.; Ferrer, I. Springback determination of sheet metals in an air bending process based on an experimental work. J. Mater. Process. Technol. 2007, 191, 174–177. [Google Scholar] [CrossRef]
- Zhan, M.; Yang, H.; Huang, L.; Gu, R. Springback analysis of numerical control bending of thin-walled tube using numerical-analytic method. J. Mater. Process. Technol. 2006, 177, 197–201. [Google Scholar] [CrossRef]
- Tekaslan, Ö.; Şeker, U.; Özdemir, A. Determining springback amount of steel sheet metal has 0.5 mm thickness in bending dies. Mater. Des. 2006, 27, 251–258. [Google Scholar] [CrossRef]
- Tekiner, Z. An experimental study on the examination of springback of sheet metals with several thicknesses and properties in bending dies. J. Mater. Process. Technol. 2004, 145, 109–117. [Google Scholar] [CrossRef]
- Hsu, T.C.; Shien, I.R. Finite element modeling of sheet forming process with bending effects. J. Mater. Process. Technol. 1997, 63, 733–737. [Google Scholar] [CrossRef]
- Muderrisoglu, T.; Livatyali, A.; Ahmetoglu, H.; Altan, M. Computer Aided Design for Bending, Flanging and Hemming of Steels and Aluminum Alloys. Proc. PMA Metealform 1997, 97, 151–158. [Google Scholar]
- Nishino, S.; Ohya, K.; Naruishi, K. Proposal for reducing press working load and highly accurate evaluation of springback error in bending automobile sheet metal. JSAE Rev. 2003, 24, 283–288. [Google Scholar] [CrossRef]
- Chou, I.N.; Hung, C. Finite element analysis and optimization on springback reduction. Int. J. Mach. Tools Manuf. 1999, 39, 517–536. [Google Scholar] [CrossRef]
- Karafillis, A.P.; Boyce, M.C. Tooling design in sheet metal forming using springback calculations. Int. J. Mech. Sci. 1992, 34, 113–131. [Google Scholar] [CrossRef]
- Said, L.B.; Wali, M.; Khedher, N.; Kessentini, A.; Algahtani, A.; Dammak, F. Efficiency of rubber-pad cushion in bending process of a thin aluminum sheet. J. Rubber Res. 2020, 23, 89–99. [Google Scholar] [CrossRef]
- Ben Said, L.; Bouhamed, A.; Wali, M.; Ayadi, B.; Betrouni, S.A.; Hajji, H.; Dammak, F. SPIF Manufacture of a Dome Part Made of AA1060-H14 Aluminum Alloy Using CNC Lathe Machine: Numerical and Experimental Investigations. Arab. J. Sci. Eng. 2021, 46, 12207–12220. [Google Scholar] [CrossRef]
- Zhao, K.M.; Lee, J.K. Finite element analysis of the three-point bending of sheet metals. J. Mater. Process. Technol. 2002, 122, 6–11. [Google Scholar] [CrossRef]
- Gan, W.; Wagoner, R.H. Die design method for sheet springback. Int. J. Mech. Sci. 2004, 46, 1097–1113. [Google Scholar] [CrossRef]
- Nguyen, D.; Kim, Y.; Jung, D. Finite Element Method Study to Predict Spring-back in Roll-Bending of Pre-Coated Material and Select Bending Parameters. Int. J. Precis. Eng. Manuf. 2012, 13, 1425–1432. [Google Scholar] [CrossRef]
- Luyen, T.; Tong, V.; Nguyen, D. A simulation and experimental study on the deep drawing process of SPCC sheet using the graphical method. Alex. Eng. J. 2021, 61, 2472–2483. [Google Scholar] [CrossRef]
- Luyen, T.T.; Nguyen, D.T. Improved uniformity in cylindrical cup wall thickness at elevated temperatures using deep drawing process for SPCC sheet steel. J. Braz. Soc. Mech. Sci. Eng. 2023, 45, 348. [Google Scholar] [CrossRef]
- Luyen, T.T.; Mac, T.B.; Banh, T.L.; Nguyem, D.T. Investigating the impact of yield criteria and process parameters on fracture height of cylindrical cups in the deep drawing process of SPCC sheet steel. Int. J. Adv. Manuf. Technol. 2023, 128, 2059–2073. [Google Scholar] [CrossRef]
- Luyen, T.T.; Mac, T.B.; Nguyen, D.T. Simulation and experimental comparison study based on predicting forming limit curve of SUS304 sheet material. Mod. Phys. Lett. B 2023, 37, 2340001. [Google Scholar] [CrossRef]
- Dong, T.; Toan, N.; Dung, N. Influence of heat treatment process on the hardness and material structure of SKD61 tool steel. Mod. Phys. Lett. B 2023, 37, 2340014. [Google Scholar] [CrossRef]
- Mac, T.-B.; Luyen, T.-T.; Nguyen, D.-T. Assessment of the Effect of Thermal-Assisted Machining on the Machinability of SKD11 Alloy Steel. Metals 2023, 13, 699. [Google Scholar] [CrossRef]
- Tong, V.-C.; Nguyen, D.-T. A study on spring-back in U-draw bending of DP350 high-strength steel sheets based on combined isotropic and kinematic hardening laws. Adv. Mech. Eng. 2018, 10, 168781401879743. [Google Scholar] [CrossRef]
- Hai, V.G.; Minh, N.T.H.; Nguyen, D.T. A study on experiment and simulation to predict the spring-back of SS400 steel sheet in large radius of V-bending process. Mater. Res. Express 2020, 7, 016562. [Google Scholar] [CrossRef]
- JIS G3101 SS400; Steel Plate/Sheet for General Purpose Structural Steels. Hebei Haihao High Pressure Flange & Pipe Fitting Group Co., Ltd.: Cangzhou, China; p. 86011881.
- ISO 6892-1:2019; Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature. ISO: Geneva, Switzerland, 2019. Available online: https://www.iso.org/standard/78322.html (accessed on 1 January 2023).
- Voce, E. The relationship between stress and strain for homogeneous deformation. J. Inst. Met. 1948, 74, 537–562. [Google Scholar]
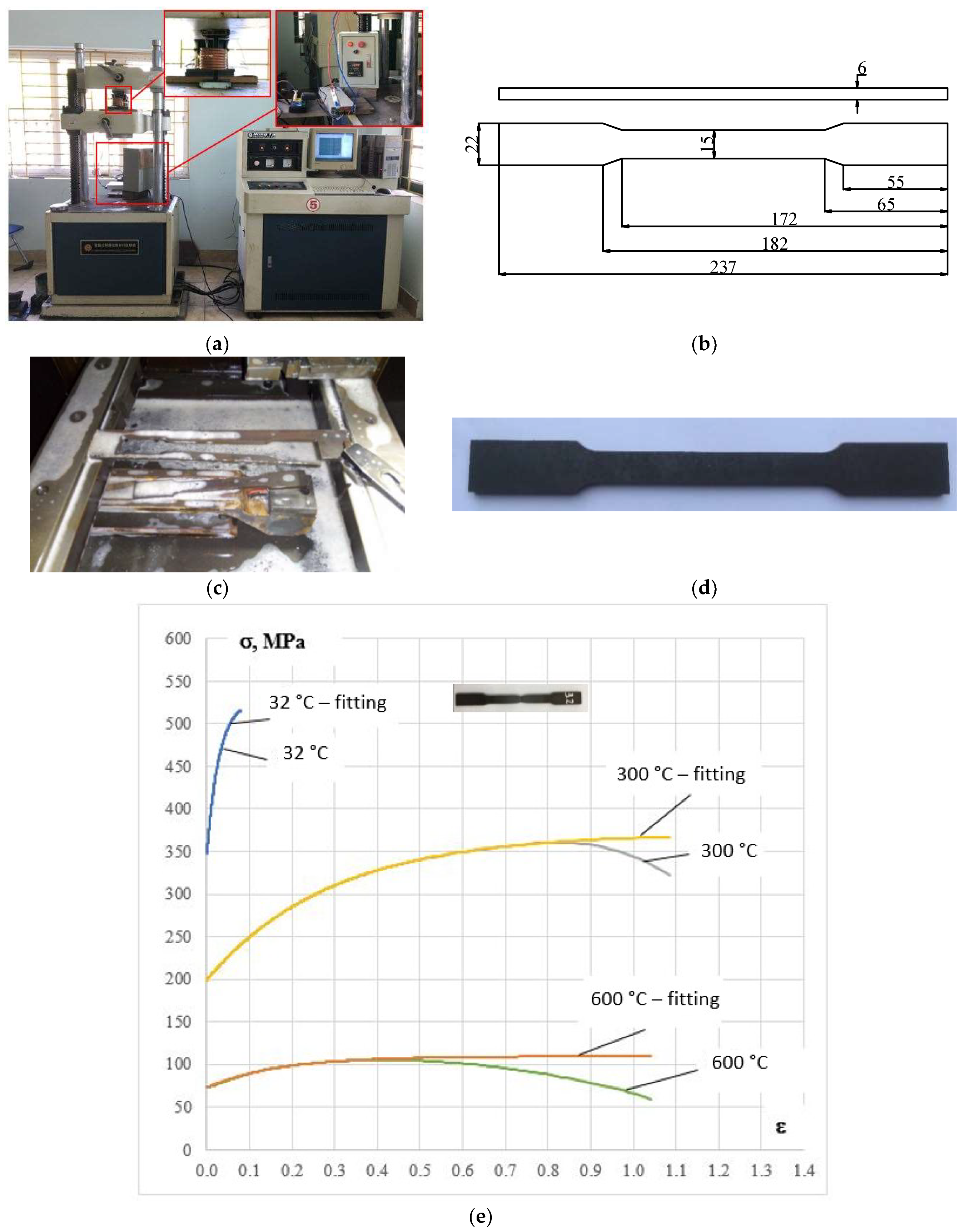
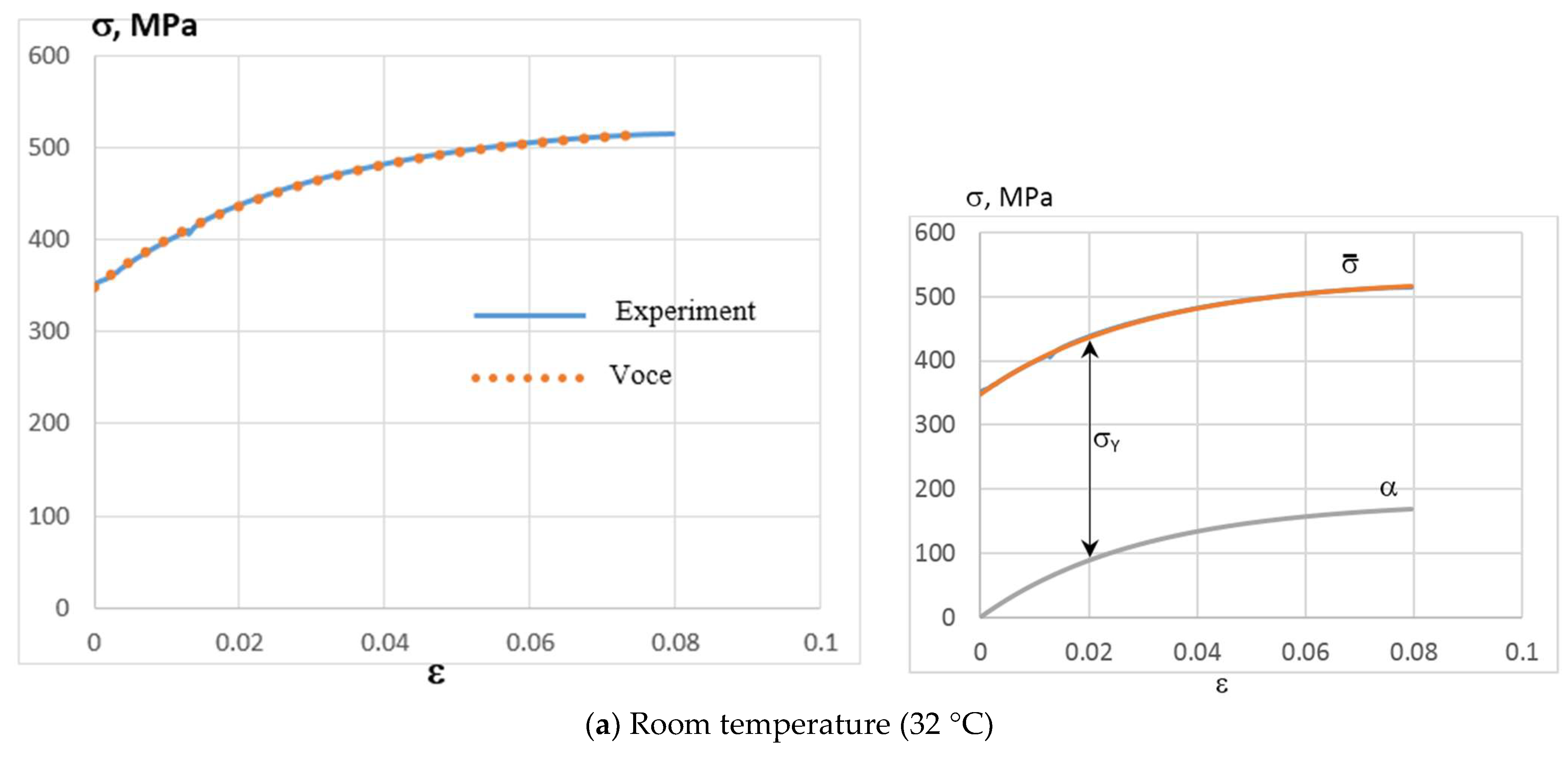
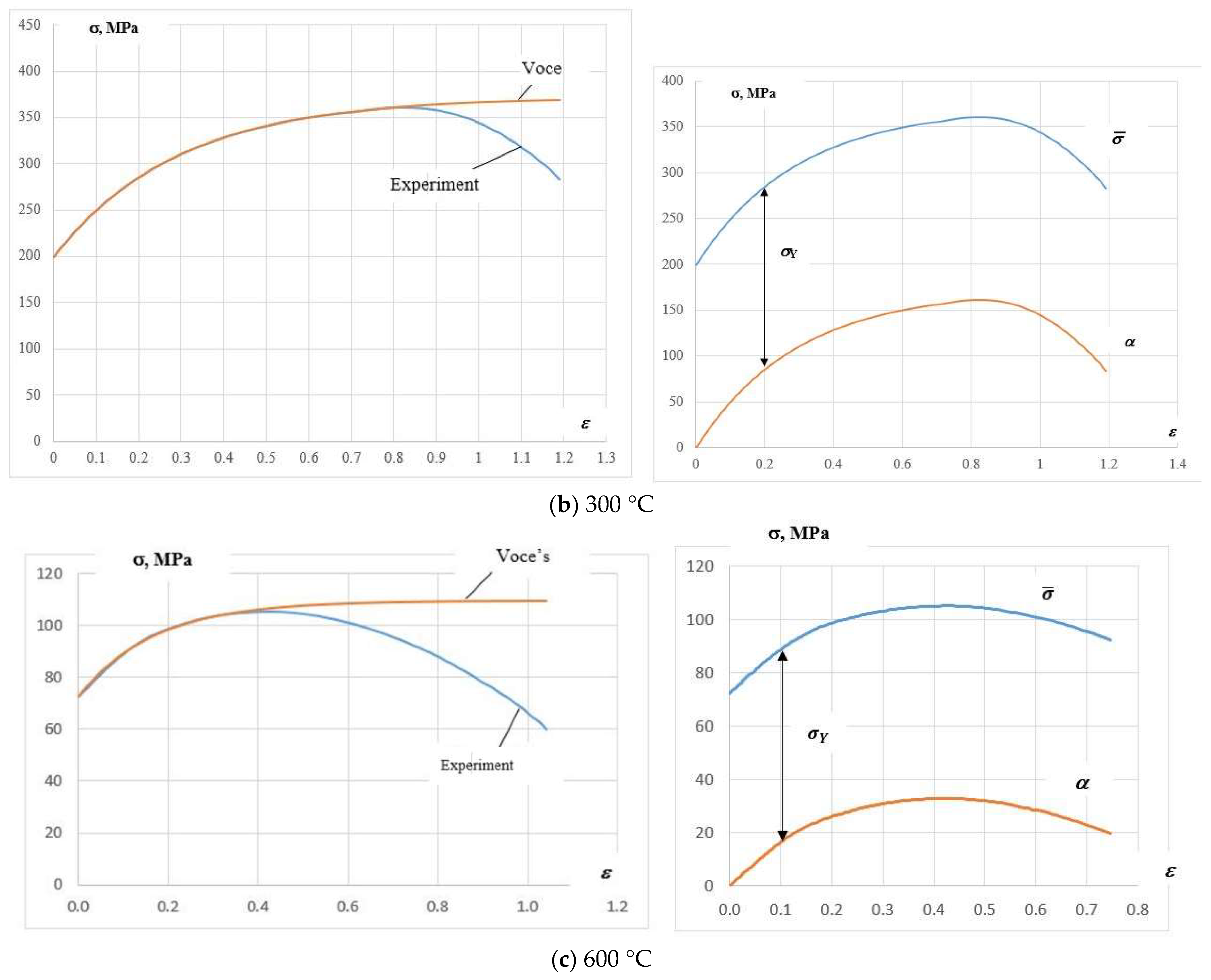

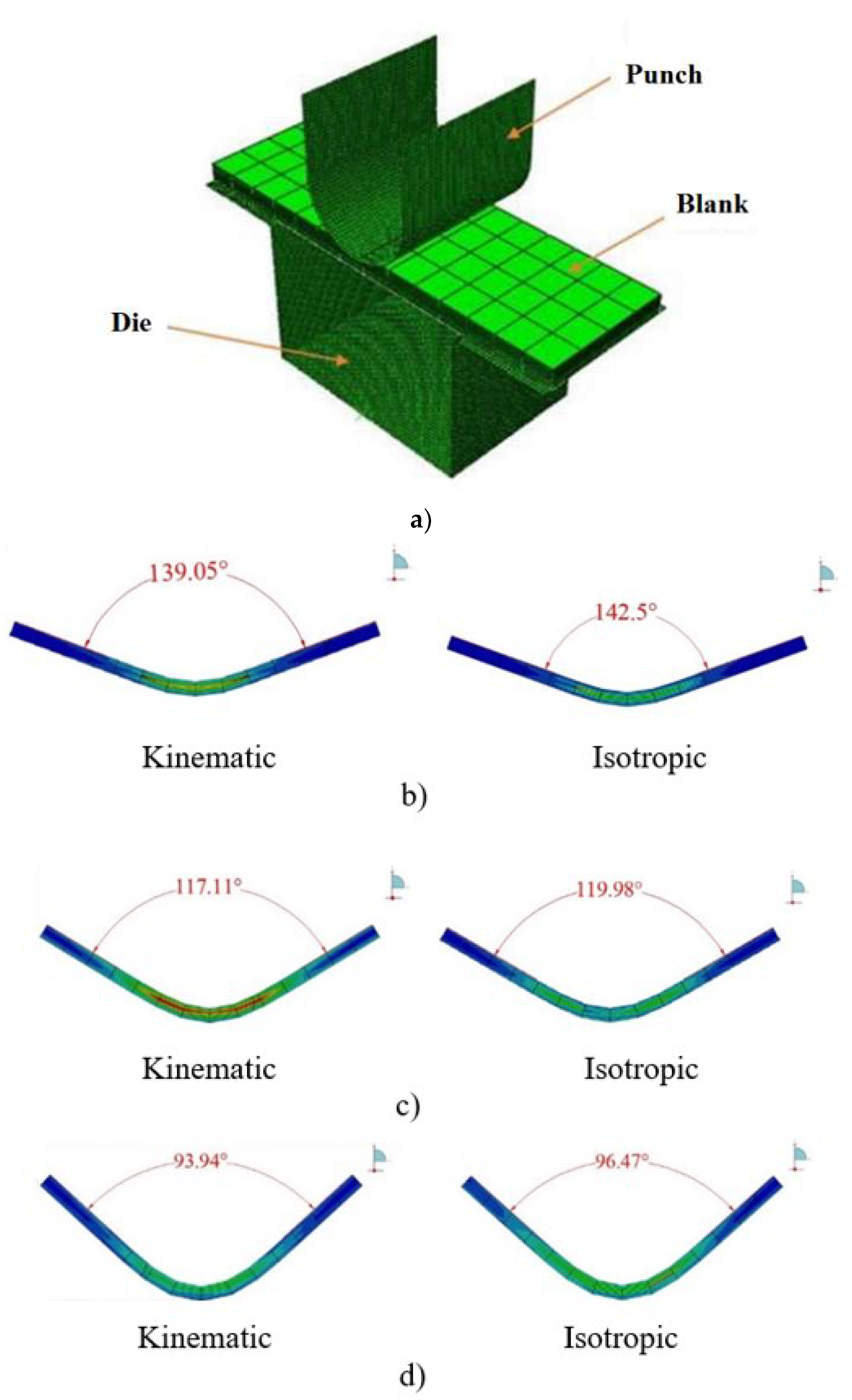

| C | Si | Mn | P | S | Cr |
|---|---|---|---|---|---|
| 0.19–0.21 | 0.05–0.17 | 0.4–0.6 | 0.04 | 0.05 | ≤0.3 |
| Parameters | Levels |
|---|---|
| Temperature (°C) | 32; 300; 600 |
| Bending speed (mm/s) | 1 |
| Thickness sheet (mm) | 6 |
| Poisson’s ratio | 0.3 |
| Young’s modulus (MPa) | 213,000 (32 °C); 184,410 (300 °C); 107,640 (600 °C) |
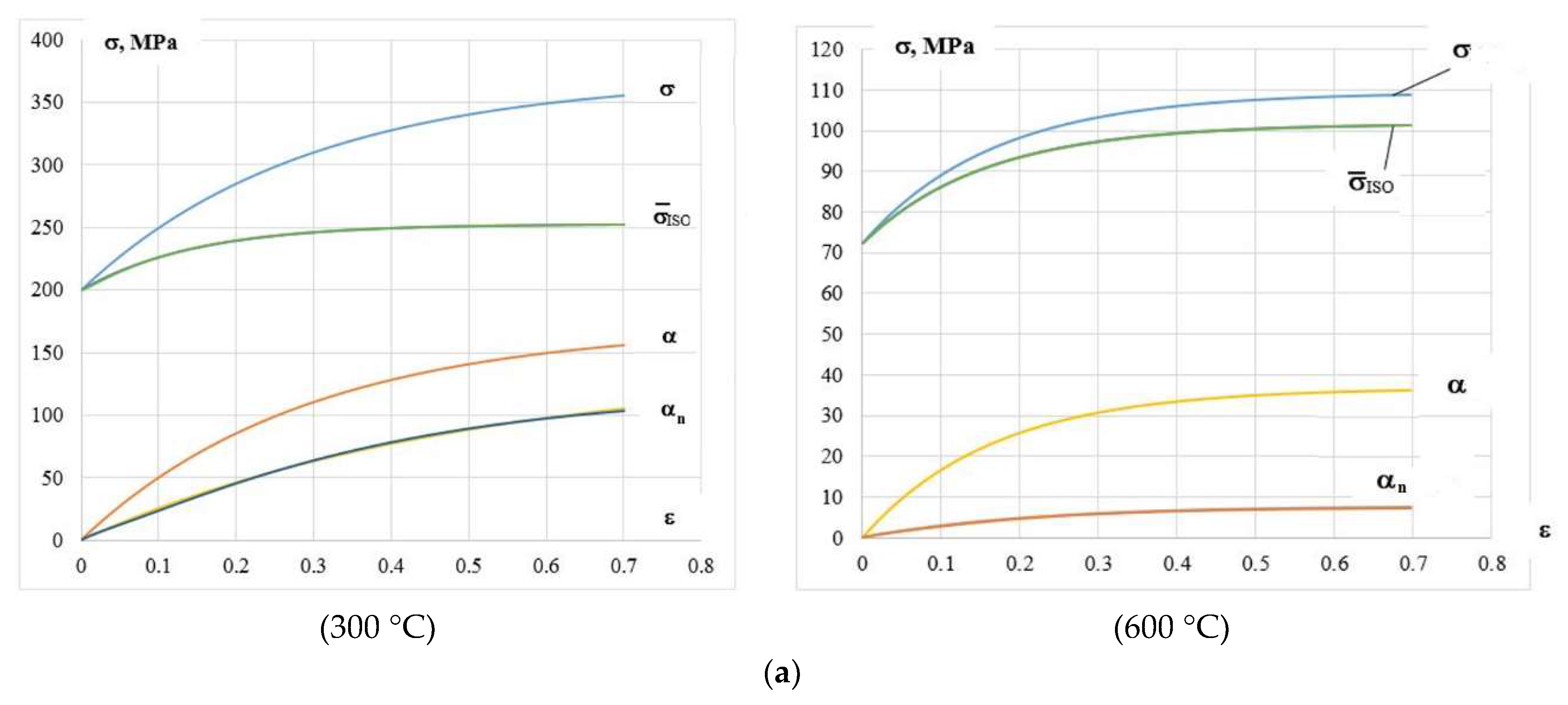
| Temperature (°C) | (MPa) | A (MPa) | B | C (MPa) | γ |
|---|---|---|---|---|---|
| 32 | 348 | 188.86 | 28.3293 | 5350 | 28.3293 |
| 300 | 199.3 | 171.56 | 3.452 | 592.2251 | 3.452 |
| 600 | 72.43 | 36.89 | 6.0145 | 221.8749 | 6.0145 |
| H (mm) | Bending Angle (°) | Measure Angle θexp (°) | Spring-Back (°) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Levels | Average | Levels | Average | ||||||
| 1 | 2 | 3 | 1 | 2 | 3 | ||||
| 10 | 136 | 141.5 | 142.5 | 141.5 | 141.833 | 5.5 | 6.5 | 5.5 | 5.833 |
| 16 | 112 | 117.67 | 117.88 | 119.72 | 118.423 | 5.67 | 5.88 | 7.72 | 6.423 |
| 22 | 88 | 96 | 95 | 94.5 | 95.167 | 8.0 | 7.0 | 6.5 | 7.167 |
| H (mm) | Bending Angle (°) | Measure Angle (°) | Deviation (Δθ)(°) | |||
|---|---|---|---|---|---|---|
| Experiment () | Simulation () | |||||
| Isotropic | Kinematic | Isotropic ( ) | Kinematic ( ) | |||
| 10 | 136 | 141.83 | 142.50 | 139.05 | 0.667 | −2.783 |
| 16 | 112 | 118.42 | 119.98 | 117.11 | 1.557 | −1.313 |
| 22 | 88 | 95.17 | 96.47 | 93.94 | 1.303 | −1.227 |
| Bending Angle (°) | Measure Angle (°) | Deviation (Δθ) (°) | |
|---|---|---|---|
| Experiment () | Simulation () | ||
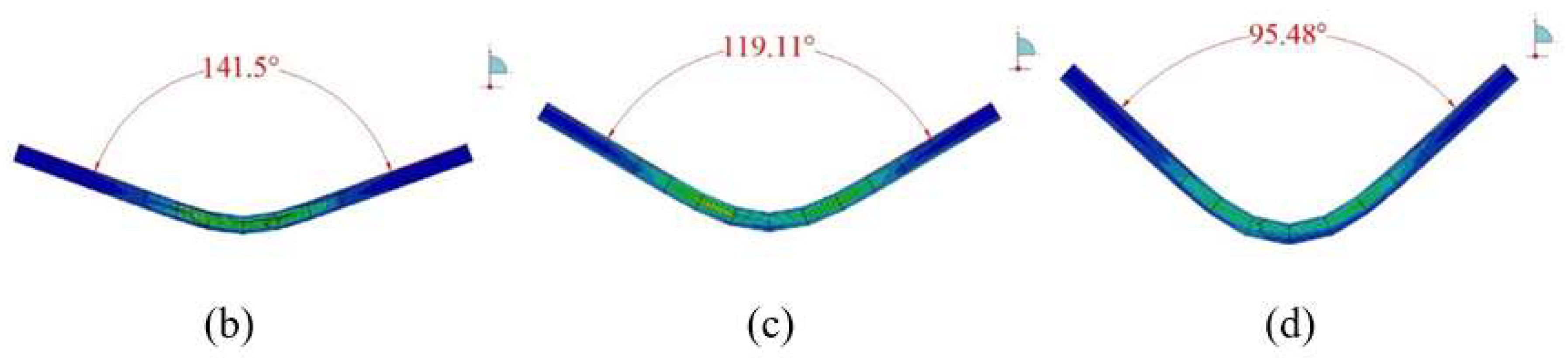
| 88 | 95.170 | 95.48 | −0.313 |
| 112 | 118.423 | 119.11 | 0.686 |
| 136 | 141.833 | 141.50 | 0.330 |
| H (mm) | Bending Angle (°) | Measure Angle (°) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 300 °C | 600 °C | ||||||||
| Level | Avg. | Level | Avg. | ||||||
| 1 | 2 | 3 | 1 | 2 | 3 | ||||
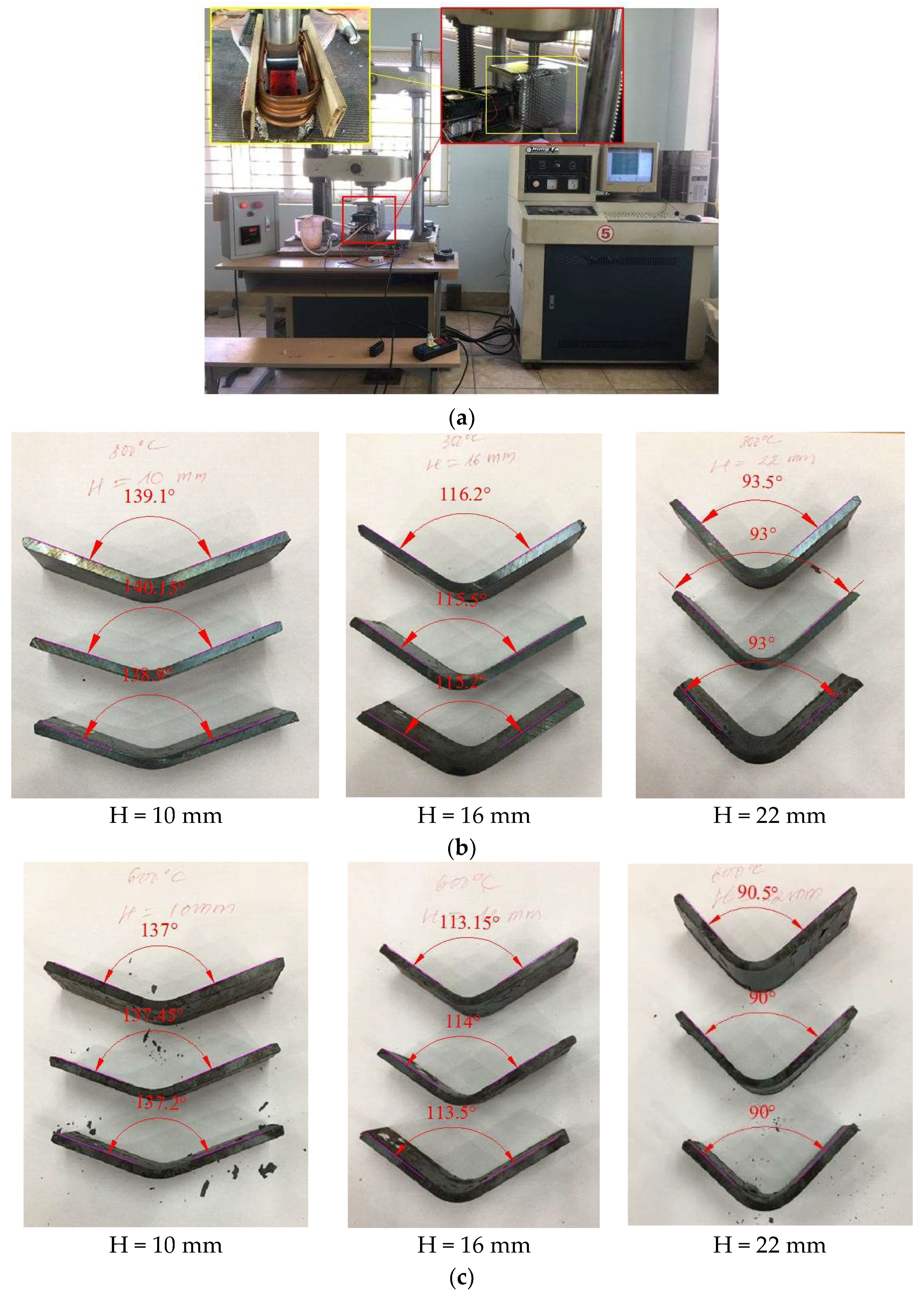
| 10 | 136 | 139.1 | 140.15 | 138.9 | 139.38 | 137 | 137.45 | 137.2 | 137.22 |
| 16 | 112 | 116.2 | 115.5 | 115.2 | 115.63 | 113.15 | 114 | 113.5 | 113.55 |
| 22 | 88 | 93.5 | 93.0 | 93.0 | 93.167 | 90.5 | 90 | 90 | 90.167 |
| T (°C) | (MPa) | (MPa) | (Mpa) | ||
|---|---|---|---|---|---|
| 300 | 199.3 | 53.3422 | 6.9049 | 280.9023 | 2.0133 |
| 600 | 72.43 | 29.2536 | 6.3814 | 36.5690 | 4.7288 |
| Bending Angle (°) | Measure Angle (°) | |||||
|---|---|---|---|---|---|---|
| 300 °C | 600 °C | |||||
| Experiment () | Simulation () | Δθ (°) | Experiment () | Simulation () | Δθ (°) | |
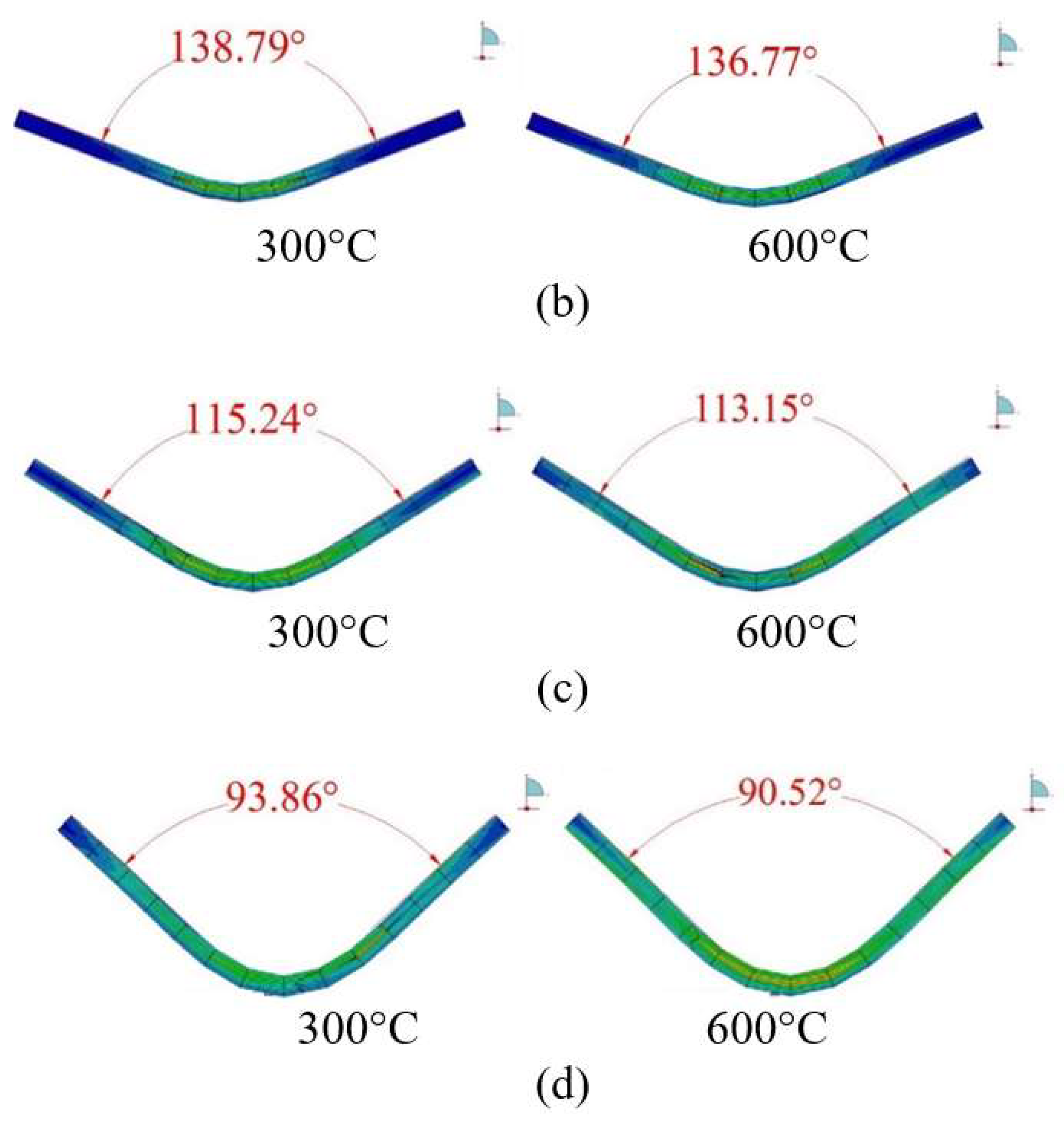
| 88 | 93.167 | 93.86 | 0.693 | 90.167 | 90.52 | 0.353 |
| 112 | 115.63 | 115.24 | −0.393 | 113.55 | 113.15 | −0.4 |
| 136 | 139.38 | 138.79 | −0.593 | 137.22 | 136.77 | −0.45 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trieu, Q.-H.; Vuong, G.-H.; Nguyen, D.-T. Predictive Modeling of Spring-Back Behavior in V-Bending of SS400 Steel Sheets under Elevated Temperatures Using Combined Hardening Laws. Appl. Sci. 2023, 13, 10347. https://doi.org/10.3390/app131810347
Trieu Q-H, Vuong G-H, Nguyen D-T. Predictive Modeling of Spring-Back Behavior in V-Bending of SS400 Steel Sheets under Elevated Temperatures Using Combined Hardening Laws. Applied Sciences. 2023; 13(18):10347. https://doi.org/10.3390/app131810347
Chicago/Turabian StyleTrieu, Quy-Huy, Gia-Hai Vuong, and Duc-Toan Nguyen. 2023. "Predictive Modeling of Spring-Back Behavior in V-Bending of SS400 Steel Sheets under Elevated Temperatures Using Combined Hardening Laws" Applied Sciences 13, no. 18: 10347. https://doi.org/10.3390/app131810347
APA StyleTrieu, Q.-H., Vuong, G.-H., & Nguyen, D.-T. (2023). Predictive Modeling of Spring-Back Behavior in V-Bending of SS400 Steel Sheets under Elevated Temperatures Using Combined Hardening Laws. Applied Sciences, 13(18), 10347. https://doi.org/10.3390/app131810347







