Screw Dynamics of a Multibody System by a Schoenflies Manipulator
Abstract
:1. Introduction
2. Momentum Screw
3. Screw Kinematics and Dynamics of a Limb of a Schoenflies Manipulator
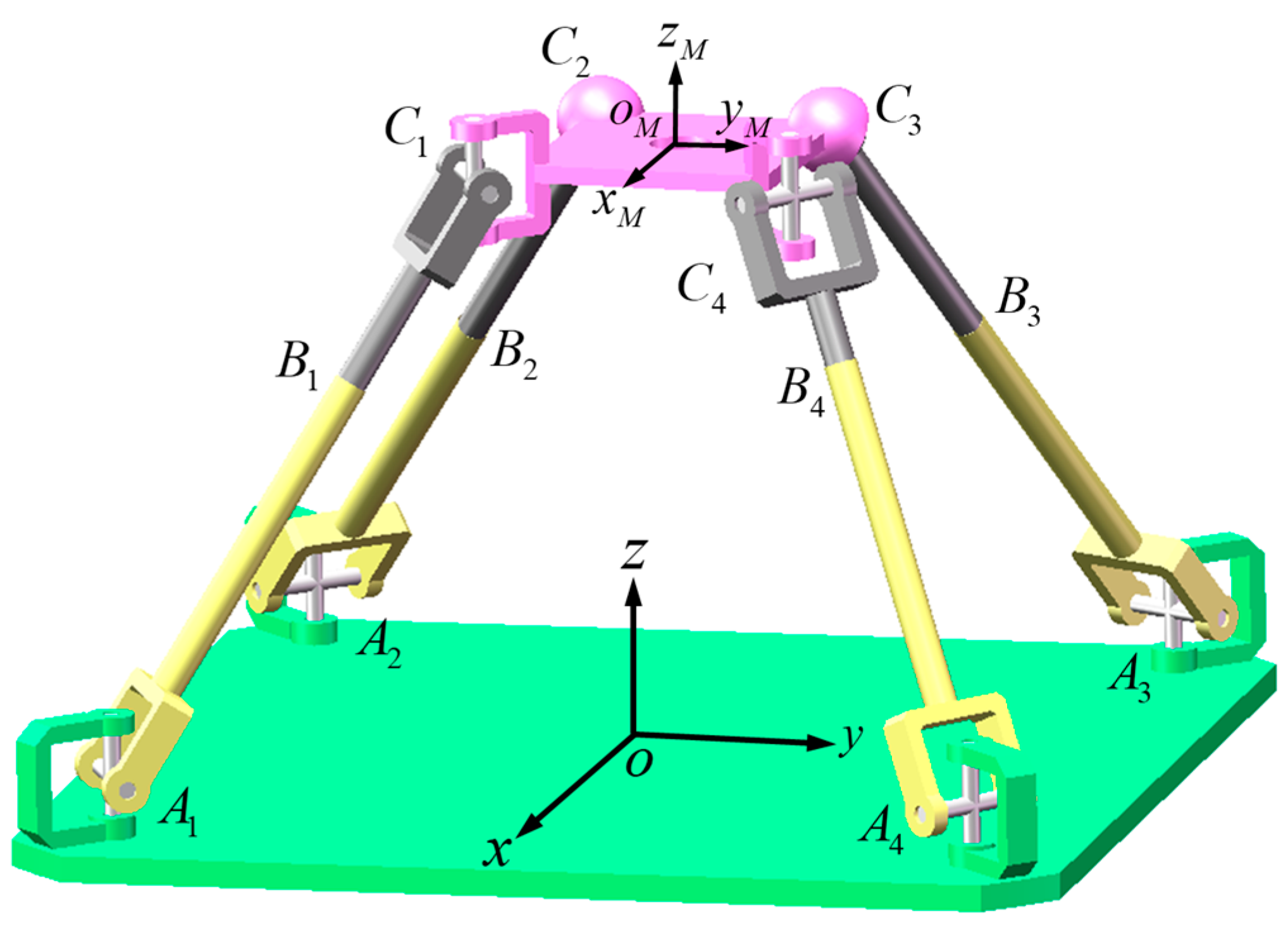
3.1. Description of the Schoenflies Manipulator
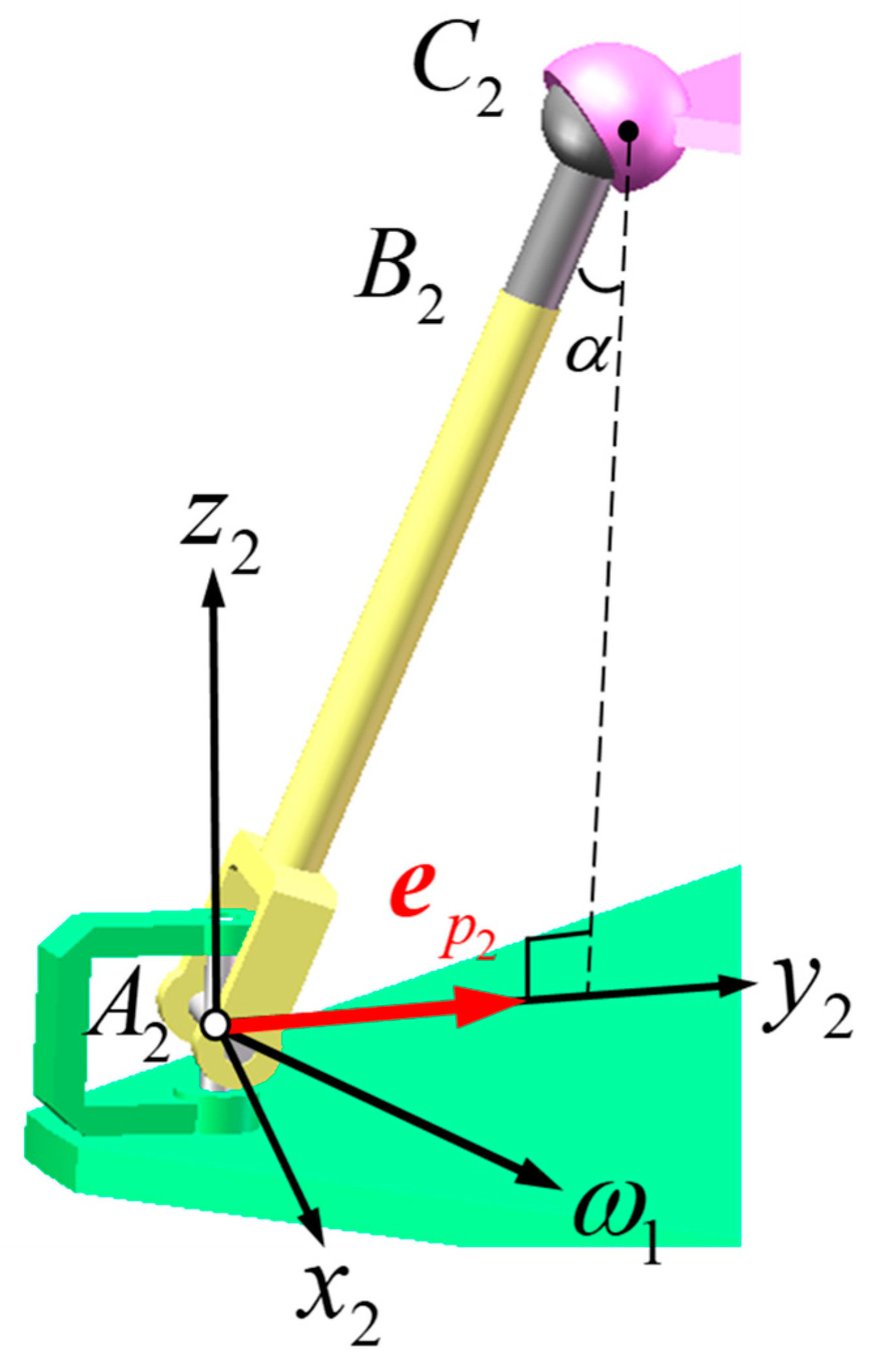
3.2. Kinematic Analysis of a Limb
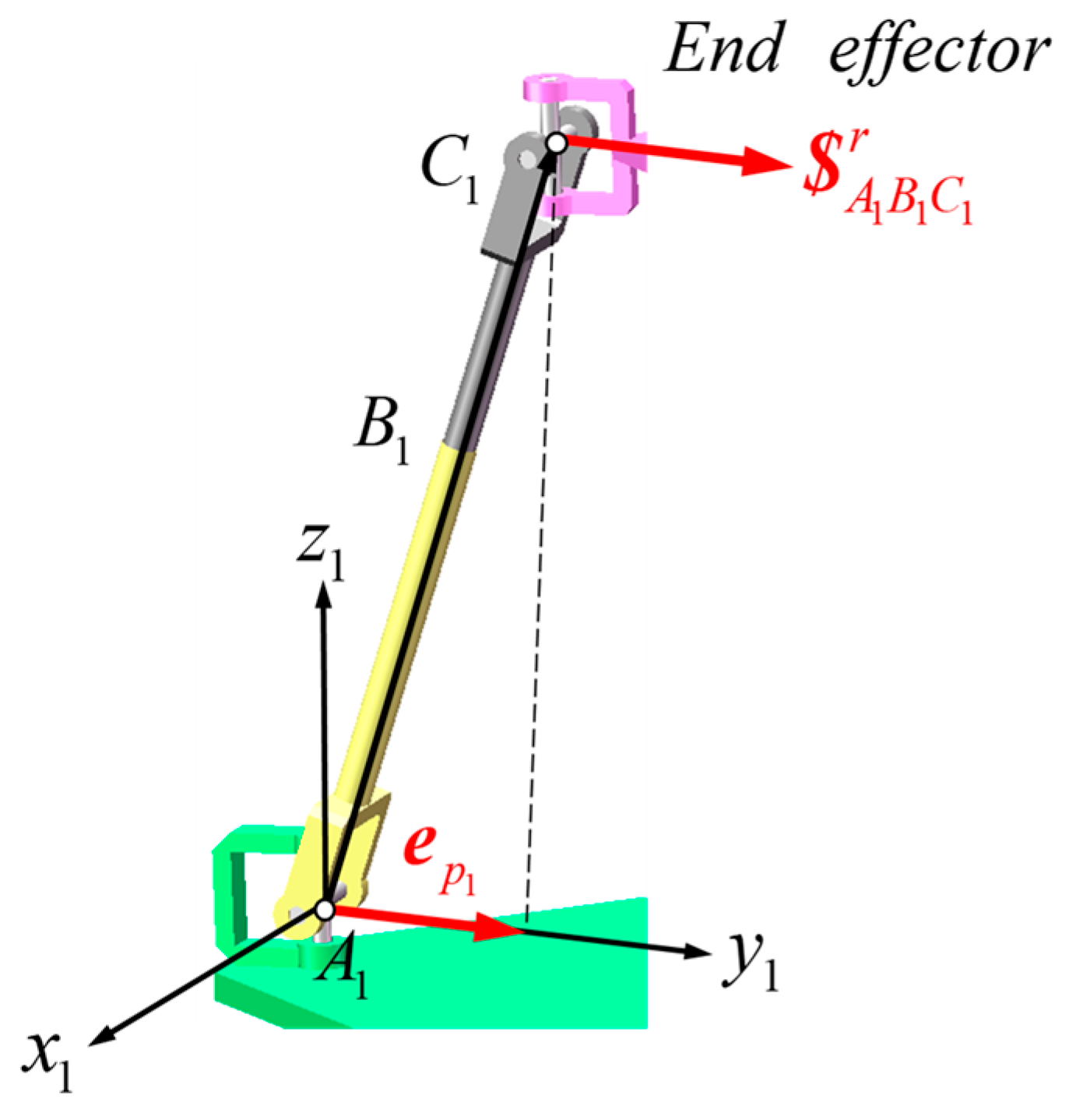
3.3. Screw Dynamics Analysis of a Limb
- UPU kinematic chains
- 2.
- UPS kinematic chain
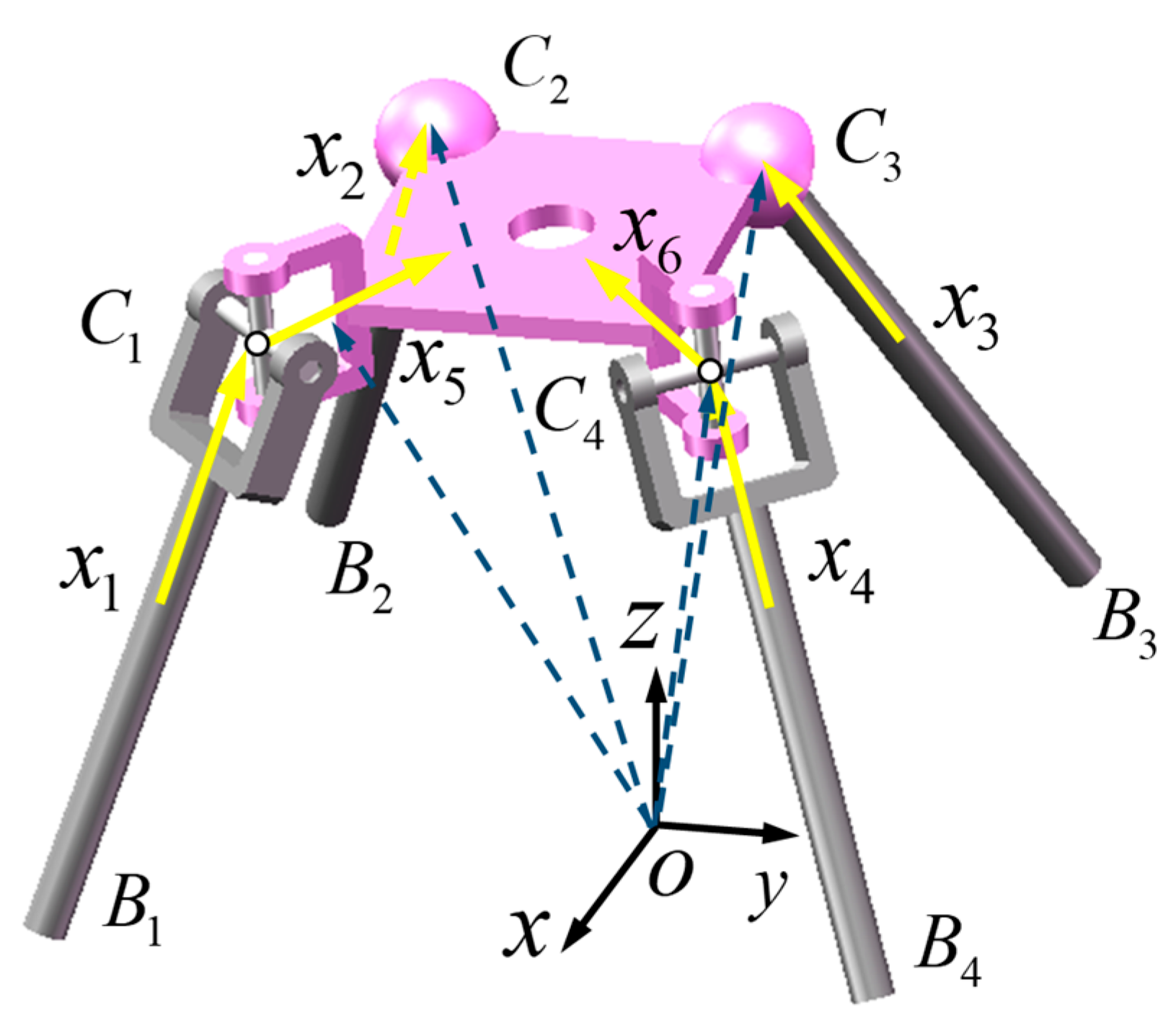
3.4. Screw Dynamics Analysis of the Moving Platform
3.5. Solution of the Driving Forces
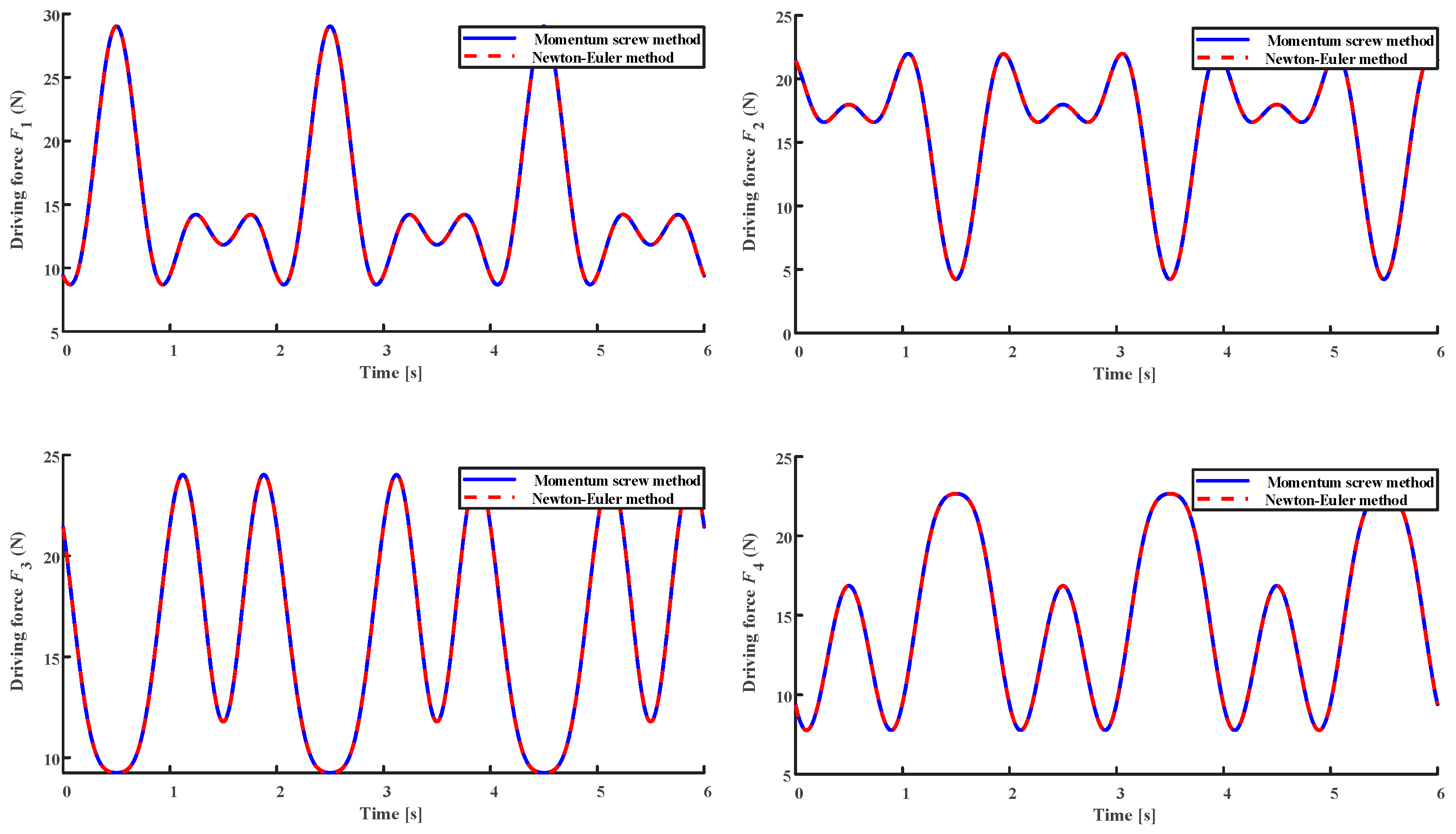
4. Simulation and Discussion
- (1)
- Computational results discussion
- (2)
- The real-time capabilities
- (3)
- Comparison of computational advantages
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Notation | Meanings of the Notation |
| Velocity of the differential mass | |
| Position vector of the differential mass in the absolute coordinate | |
| Momentum screw | |
| Velocity screw (twist) | |
| Coordinate space of the rigid body | |
| Linear velocity of the center of mass | |
| Force screw (wrench) | |
| Mass moment of inertia of a rigid body relative to its center of mass | |
| Density of a rigid body | |
| Momentum | |
| Moment of momentum | |
| Unit axial vector | |
| Reciprocal screws of the limb | |
| Position vector of the point in the absolute coordinate frame | |
| Rotation transformation matrix | |
| Angular velocity of the limb | |
| Linear velocity of point in the absolute coordinate frame | |
| Unit direction vector of the limb | |
| plane | |
| Constraint torque of joint | |
| Mass of the lower part | |
| Mass of the upper part | |
| Angular velocity of the moving platform around the z-axis | |
| Gravity acceleration |
Appendix A
References
- Xin, G.; Deng, H.; Zhong, G. Closed-form dynamics of a 3-DOF spatial parallel manipulator by combining the Lagrangian formulation with the virtual work principle. Nonlinear Dyn. 2016, 86, 1329–1347. [Google Scholar] [CrossRef]
- Tsai, L.W. Robot Analysis and Design: The Mechanics of Serial and Parallel Manipulators; Wiley: New York, NY, USA, 1999. [Google Scholar]
- Dasgupta, B.; Mruthyunjaya, T.S. A Newton-Euler formulation for the inverse dynamics of the Stewart platform manipulator. Mech. Mach. Theory 1998, 33, 1135–1152. [Google Scholar] [CrossRef]
- Fass, T.H.; Hao, G.; Cantillon-Murphy, P. Vectorized Formulation of Newton-Euler Dynamics for Efficiently Computing Three-Dimensional Folding Chains. ASME J. Mech. Robot. 2022, 14, 060911. [Google Scholar] [CrossRef]
- Peng, J.; Wu, H.; Lau, D. Operational Space Iterative Learning Control of Coupled Active/Passive Multilink Cable-Driven Hyper-Redundant Robots. ASME J. Mech. Robot. 2022, 15, 011013. [Google Scholar] [CrossRef]
- Tafrishi, S.A.; Svinin, M.; Yamamoto, M. Inverse dynamics of underactuated planar manipulators without inertial coupling singularities. Multibody Syst. Dyn. 2021, 52, 407–429. [Google Scholar] [CrossRef]
- Dopico Dopico, D.; López Varela, Á.; Luaces Fernández, A. Augmented Lagrangian index-3 semi-recursive formulations with projections. Multibody Syst. Dyn. 2021, 52, 377–405. [Google Scholar] [CrossRef]
- Quental, C.; Simões, F.; Sequeira, M.; Ambrósio, J.; Vilas-Boas, J.P.; Nakashima, M. A multibody methodological approach to the biomechanics of swimmers including hydrodynamic forces. Multibody Syst. Dyn. 2022, 57, 413–426. [Google Scholar] [CrossRef]
- Nguyen, C.C.; Pooran, F.J. Dynamic analysis of a 6 DOF CKCM robot end-effector for dual-arm telerobot systems. Robot. Auton. Syst. 1989, 5, 377–394. [Google Scholar] [CrossRef]
- Calogero, J.; Frecker, M.; Hasnain, Z.; Hubbard, J.E., Jr. Tuning of a Rigid-Body Dynamics Model of a Flapping Wing Structure With Compliant Joints. ASME J. Mech. Robot. 2017, 10, 011007. [Google Scholar] [CrossRef]
- Geng, Z.; Haynes, L.S.; Lee, J.D.; Carroll, R.L. On the dynamic model and kinematic analysis of a class of Stewart platforms. Robot. Auton. Syst. 1992, 9, 237–254. [Google Scholar] [CrossRef]
- Guo, H.B.; Li, H.R. Dynamic analysis and simulation of a six degree of freedom Stewart platform manipulator. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2006, 220, 61–72. [Google Scholar]
- Kumar, S.; Szadkowski, K.A.v.; Mueller, A.; Kirchner, F. An Analytical and Modular Software Workbench for Solving Kinematics and Dynamics of Series-Parallel Hybrid Robots. ASME J. Mech. Robot. 2020, 12, 021114. [Google Scholar] [CrossRef]
- Kane, T.R.; Levinson, D.A. The Use of Kane’s Dynamical Equations in Robotics. Int. J. Robot. Res. 1983, 2, 1–21. [Google Scholar] [CrossRef]
- Enferadi, J.; Jafari, K. A Kane’s based algorithm for closed-form dynamic analysis of a new design of a 3RSS-S spherical parallel manipulator. Multibody Syst. Dyn. 2020, 49, 377–394. [Google Scholar] [CrossRef]
- Liu, S.; Huang, T.; Mei, J.; Zhao, X.; Wang, P.; Chetwynd, D.G. Optimal Design of a 4-DOF SCARA Type Parallel Robot Using Dynamic Performance Indices and Angular Constraints. ASME J. Mech. Robot. 2012, 4, 031005. [Google Scholar] [CrossRef]
- Lafi, W.; Djemal, F.; Akrout, A.; Walha, L.; Haddar, M. Effects of the interval geometric deviation and crowning parameters on the automotive differential dynamics. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2021, 235, 602–625. [Google Scholar] [CrossRef]
- Li, K.; Liu, M.; Yu, Z.; Lan, P.; Lu, N. Multibody system dynamic analysis and payload swing control of tower crane. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2022, 236, 407–421. [Google Scholar] [CrossRef]
- Chen, G.; Yu, W.; Li, Q.; Wang, H. Dynamic modeling and performance analysis of the 3-PRRU 1T2R parallel manipulator without parasitic motion. Nonlinear Dyn. 2017, 90, 339–353. [Google Scholar]
- Kamman, J.W.; Huston, R.L. Dynamics of Constrained Multibody Systems. ASME J. Appl. Mech. 1984, 51, 899–903. [Google Scholar] [CrossRef]
- Giacaglia, G.E.O.; de Queiroz Lamas, W. Notes on Newton–Euler formulation of robotic manipulators. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2011, 226, 61–71. [Google Scholar] [CrossRef]
- Chadaj, K.; Malczyk, P.; Frączek, J. A parallel Hamiltonian formulation for forward dynamics of closed-loop multibody systems. Multibody Syst. Dyn. 2017, 39, 51–77. [Google Scholar] [CrossRef]
- Plöchl, M.; Heinzl, P.; Mack, W.; Lugner, P. Dynamic analysis of an articulated interurban train by means of a symbolic-numerical method for closed-loop mechanisms. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2000, 214, 243–252. [Google Scholar] [CrossRef]
- Craig, J.J. Introduction to Robotics: Mechanics and Control, 4th ed.; Pearson Education, Inc.: London, UK, 2017. [Google Scholar]
- Milica, L.; Năstase, A.; Andrei, G. A novel algorithm for the absorbed power estimation of HEXA parallel mechanism using an extended inverse dynamic model. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2019, 234, 185–197. [Google Scholar] [CrossRef]
- Zhao, J.S.; Zhou, K.; Mao, D.Z.; Gao, Y.F.; Fang, Y.F. A new method to study the degree of freedom of spatial parallel mechanisms. Int. J. Adv. Manuf. Technol. 2004, 23, 288–294. [Google Scholar] [CrossRef]
- Zhao, J.S.; Fu, Y.Z.; Zhou, K.; Feng, Z.J. Mobility properties of a Schoenflies-type parallel manipulator. Robot. Comput. Integr. Manuf. 2006, 22, 124–133. [Google Scholar] [CrossRef]
- Featherstone, R. Rigid Body Dynamics Algorithms; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Zhao, J.-S.; Wei, S.-T.; Sun, X.-C. Computational Dynamics of Multi-Rigid-Body System in Screw Coordinate. Appl. Sci. 2023, 13, 6341. [Google Scholar] [CrossRef]



| Component of the Manipulator | Meaning of the Components | Parameter | Value |
|---|---|---|---|
| Moving platform | Mass | 4.196 | |
| Inertia matrix | |||
| Circumradius | 0.212 | ||
| Lower limb I | Mass | 0.367 | |
| Inertia matrix | |||
| length | 0.6 | ||
| Upper limb II | Mass | 0.367 | |
| Inertia matrix | |||
| length | 0.7 | ||
| Base platform | Circumradius | 0.707 |
| Parameter | Momentum Screw Method | Newton–Euler Method |
|---|---|---|
| Time interval (ms) | 0.001 | 0.001 |
| Number of period node | 6000 | 6000 |
| Elapsed times (s) | 0.768 | 0.923 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, J.-S.; Sun, H.-L.; Li, H.-Y.; Zhao, D.-J. Screw Dynamics of a Multibody System by a Schoenflies Manipulator. Appl. Sci. 2023, 13, 9732. https://doi.org/10.3390/app13179732
Zhao J-S, Sun H-L, Li H-Y, Zhao D-J. Screw Dynamics of a Multibody System by a Schoenflies Manipulator. Applied Sciences. 2023; 13(17):9732. https://doi.org/10.3390/app13179732
Chicago/Turabian StyleZhao, Jing-Shan, Han-Lin Sun, Hao-Yang Li, and Dong-Jie Zhao. 2023. "Screw Dynamics of a Multibody System by a Schoenflies Manipulator" Applied Sciences 13, no. 17: 9732. https://doi.org/10.3390/app13179732
APA StyleZhao, J.-S., Sun, H.-L., Li, H.-Y., & Zhao, D.-J. (2023). Screw Dynamics of a Multibody System by a Schoenflies Manipulator. Applied Sciences, 13(17), 9732. https://doi.org/10.3390/app13179732







