A Numerical Method-Based Analysis of the Structural Deformation Behaviour of a Turkish String Instrument (Cura Baglama) under Varying String Tensions
Abstract
:1. Introduction
2. Materials and Methods
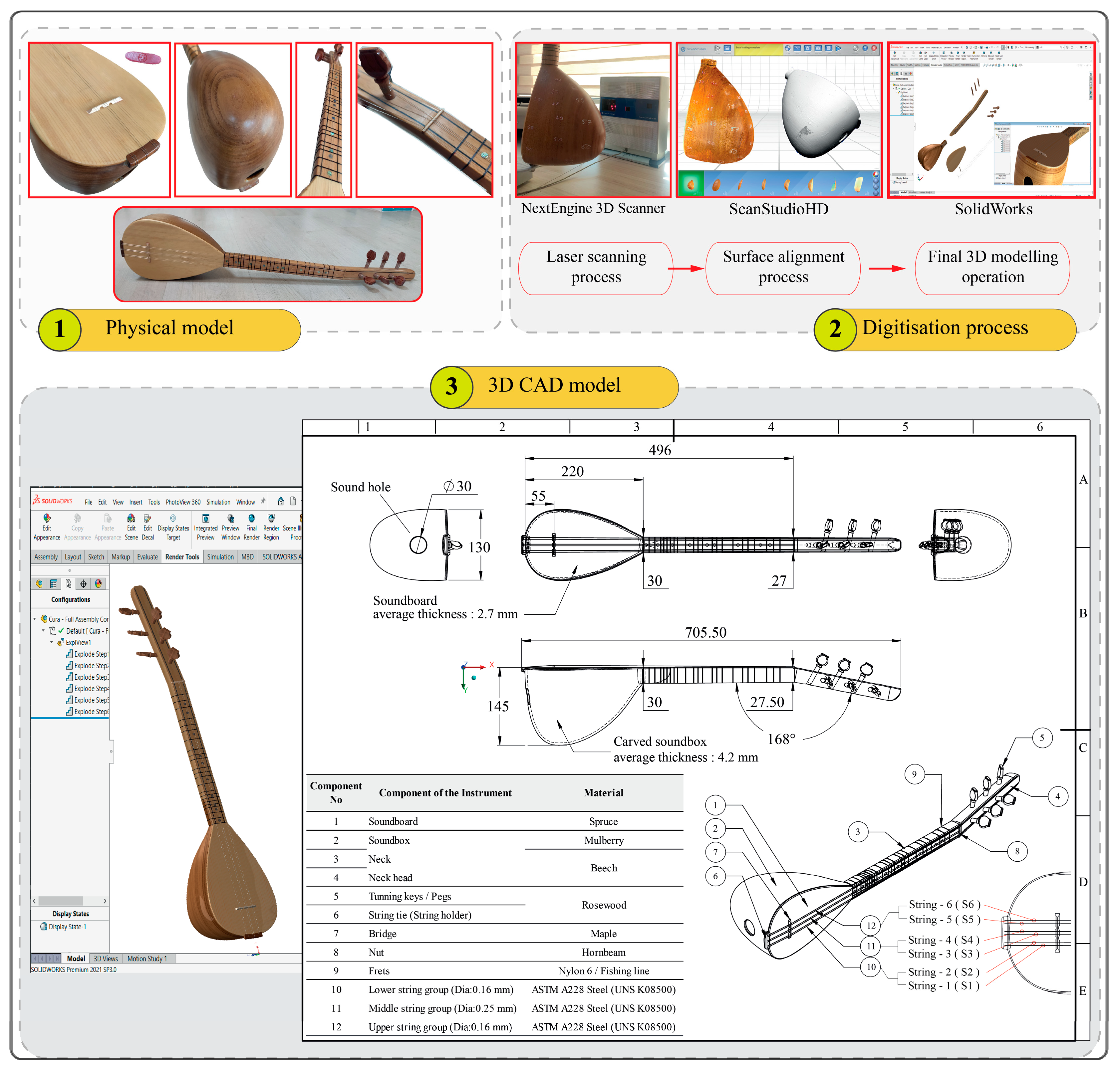
2.1. Cura Baglama and Three-Dimensional Modelling Procedure
2.2. Frequency Measurement and Calculations for String Tension Force
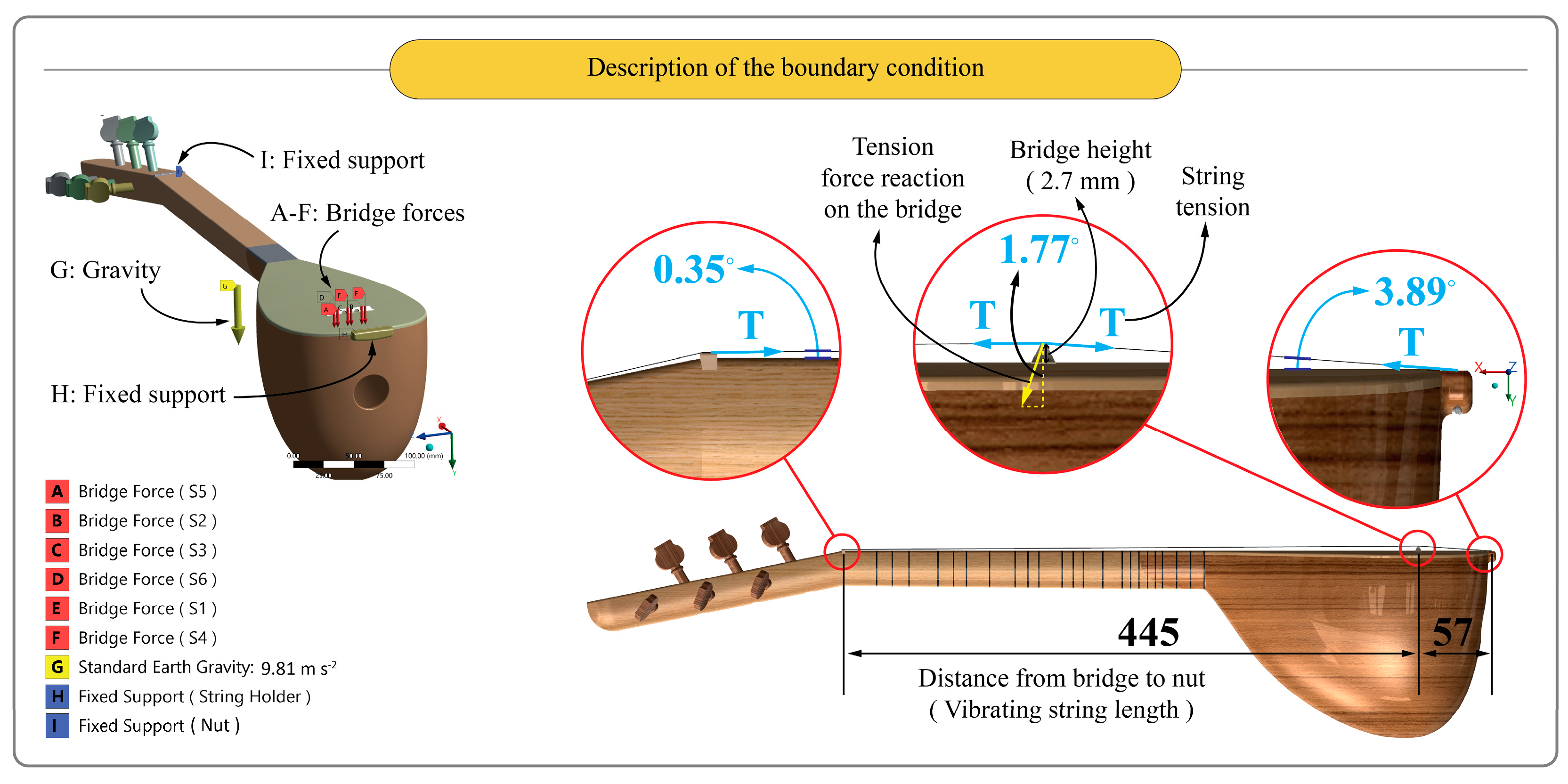
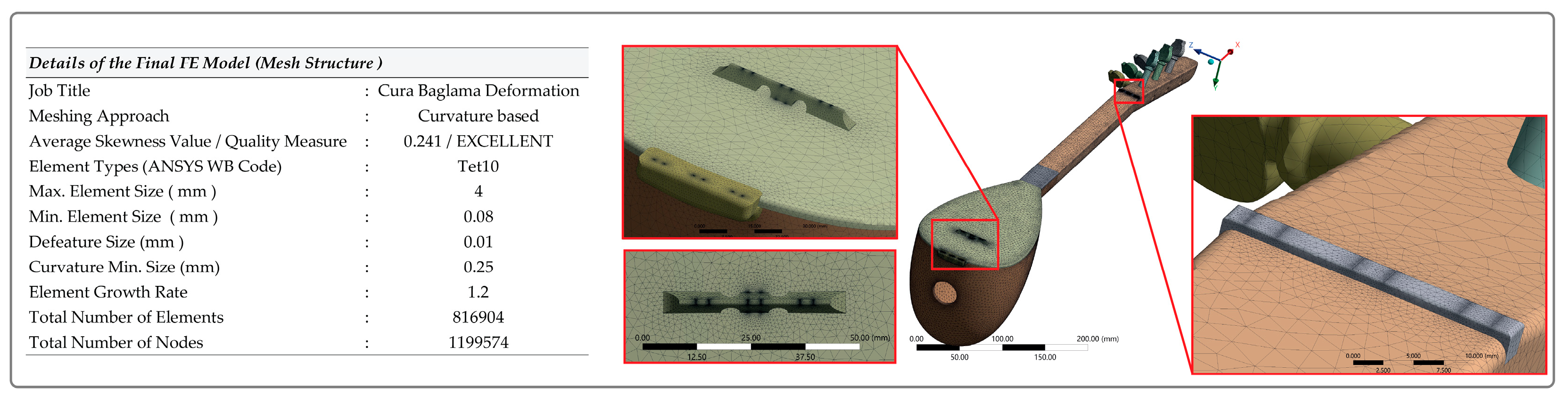
2.3. Finite Element Analysis Procedure
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Winternitz, E. Musical Instruments and Their Symbolism in Western Art, 2nd ed.; Yale University Press: New Haven, CT, USA, 1979; ISBN 9780300023244. [Google Scholar]
- de Zeeuw, H. The Turkish Long-Necked Lute Saz or Bağlama; Archaeopress Publishing Ltd.: Oxford, UK, 2020; ISBN 9781789694338. [Google Scholar]
- de Zeeuw, H. Tanbûr Long-Necked Lutes along the Silk Road and Beyond; Archaeopress Publishing Ltd.: Oxford, UK, 2019; ISBN 9781789691702. [Google Scholar]
- de Zeeuw, H. The Ottoman Tanbûr: The Long-Necked Lute of Ottoman Art Music; Archaeopress Publishing Ltd.: Oxford, UK, 2022; ISBN 9781803271064. [Google Scholar]
- Saz Kopuz’dan Türemiş Olamaz. Yildiray Erdener. Available online: https://www.sanattanyansimalar.com/yazarlar/yildiray-erdener/saz-kopuzdan-turemis-olamaz/1395/ (accessed on 25 April 2023). (In Turkish).
- Ekim, G. Historical Progression of Baglama; Ege University: İzmir, Turkey, 2002. (In Turkish) [Google Scholar]
- Mert, B. “Baglama” with New Expression Opportunities from the Past to the Present; Mimar Sinan Fine Arts University: Istanbul, Turkey, 2018. (In Turkish) [Google Scholar]
- Yilmaz, A. Current Changes in Professional Baglama Performance; Halic University: Istanbul, Turkey, 2011. (In Turkish) [Google Scholar]
- Demirbas, T. Stringed and Bowed Turkish Folk Music Instruments in Terms of Instrumentation; Istanbul Technical University: Istanbul, Turkey, 2020. (In Turkish) [Google Scholar]
- Erbil, K. An Investigation of the Usability of Burdur Three Strings (Üçtelli) Bağlama in Music Teaching Programs in the Line of Expert Opinions; Necmettin Erbakan University: Konya, Turkey, 2021. (In Turkish) [Google Scholar]
- Tetik Isik, S. Organology Studies in Turkey. Mukaddime 2015, 6, 197–220. (In Turkish) [Google Scholar] [CrossRef]
- Degirmenli, E. An Investigation of the Sound Production in the Turkish Musical Instrument Oud and a Proposal for Determining the Design of Stringed Instruments from the Aspect of Their Sound Characteristics; Gazi University: Ankara, Turkey, 2018. (In Turkish) [Google Scholar]
- Carlson, M. Application of Finite Element Analysis for an Improved Musical Instrument Design; Fender Musical Instruments Corporation, Guitar Products Research & Development Department: Corona, CA, USA, 2011. [Google Scholar]
- Narasaiah, G.L. Finite Element Analysis; B.S. Publications: Hyderabad, India, 2008; ISBN 9788178001401. [Google Scholar]
- Kuna, M. Finite Elements in Fracture Mechanics; Gladwell, G.M.L., Ed.; Springer: London, UK, 2004; ISBN 9789400766792. [Google Scholar]
- Chakrabarty, B.K. Integrated CAD by Optimization; Springer International Publishing: Cham, Switzerland, 2022; ISBN 978-3-030-99305-4. [Google Scholar]
- Szabó, B.; Babuška, I. Finite Element Analysis: Method, Verification and Validation, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2021; ISBN 9781119426479. [Google Scholar]
- Cook, R.D.; Robert, D. Finite Element Modeling for Stress Analysis; Wiley: Hoboken, NJ, USA, 1995; Volume 32, ISBN 978-0-471-10774-3. [Google Scholar]
- Kim, N.-H.; Sankar, B.V.; Kumar, A.V. Introduction to Finite Element Analysis and Design, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2018; p. 552. [Google Scholar]
- McIntyre, M.E.; Woodhouse, J. The Acoustics of Stringed Musical Instruments. Interdiscip. Sci. Rev. 1978, 3, 157–173. [Google Scholar] [CrossRef]
- Bülbül, F. Microscopic Examination of Play-Worn Steel Music Strings. Frict. Wear Res. 2015, 3, 1. [Google Scholar] [CrossRef]
- Alm, J.F.; Walker, J.S. Time-Frequency Analysis of Musical Instruments. SIAM Rev. 2002, 44, 457–476. [Google Scholar] [CrossRef]
- Brahmi, M.; Banerjee, S.D.A.; Banerjee, S. Frequency Analysis of Plucked Musical Instruments. In The Surprising Case of Suppressed Fundamental; Indian Institute of Science Education and Research Kolkata: Mohanpur, India, 2017. [Google Scholar]
- Meyer, J. Acoustics and the Performance of Music, 5th ed.; Springer: New York, NY, USA, 2009; ISBN 978-0-387-09516-5. [Google Scholar]
- Ozbek, M. Turkish Folk Music Handbook-I: Glossary of Terms; Ataturk Culture Center: Ankara, Turkey, 1998. (In Turkish) [Google Scholar]
- Say, A. Music Encyclopedia; Music Encyclopedia Publisher: Ankara, Turkey, 2002; ISBN 975-7436-29-1. (In Turkish) [Google Scholar]
- Howard, D.M.; Angus, J.A. Acoustics and Psychoacoustics, 4th ed.; Routledge: London, UK, 2009; ISBN 9780240521756. [Google Scholar]
- Ugural, A.C.; Fenster, S.K. Advanced Strength and Applied Elasticity; Prentice Hall: Hoboken, NJ, USA, 2003; Volume 1, ISBN 9780130473929. [Google Scholar]
- Deta, U.A.; Suprapto, N.; Mubarok, H.; Adam, A.S.; Kholiq, A. The Comparison of Static Friction Coefficient on Wood between the Combination of Wood-Metal Load System and Wood-Sand Load System. In International Conference on Science and Technology (ICST 2018); Atlantis Press: Amsterdam, The Netherlands, 2018; Volume 1, pp. 887–890. [Google Scholar] [CrossRef]
- SolidWorks Doc. SolidWorks Simulation Premium: Nonlinearity Training Manual Document No. 22658021044-ENG0001; Dassault Systemes SolidWorks Corporation: Waltham, MA, USA, 2010. [Google Scholar]
- Wakabayashi, N.; Ona, M.; Suzuki, T.; Igarashi, Y. Nonlinear Finite Element Analyses: Advances and Challenges in Dental Applications. J. Dent. 2008, 36, 463–471. [Google Scholar] [CrossRef] [PubMed]
- ANSYS Product Doc. ANSYS Meshing User’s Guide: Skewness (Release 2019 R2); ANSYS Inc.: Canonsburg, PA, USA, 2019. [Google Scholar]
- Brys, G.; Hubert, M.; Struyf, A. A Robust Measure of Skewness. J. Comput. Graph. Stat. 2004, 13, 996–1017. [Google Scholar] [CrossRef]
- Obara, P. Verification of Orthotropic Model of Wood. Arch. Civ. Eng. 2018, 64, 31–44. [Google Scholar] [CrossRef]
- Pedrammehr, S.; Aghdam, N.J.; Pakzad, S.; Ettefagh, M.M.; Sadeghi, M.H. A Study on Vibration of Setar: Stringed Persian Musical Instrument. J. Vibroengineering 2018, 20, 2680–2689. [Google Scholar] [CrossRef]
- Ross, R.J. Wood Handbook: Wood as an Engineering Material; U.S. Department of Agriculture, Forest Service, Forest Products Laboratory: Madison, WI, USA, 2010.
- Higuchi, K.; Takai, H. Stress-Strain Diagram, Young’s Modulus and Poisson’s Ratio of Textile Fibers. J. Text. Mach. Soc. Jpn. 1961, 7, 4–12. [Google Scholar] [CrossRef]
- Fu, Y.; Fang, H.; Dai, F. Study on the Properties of the Recombinant Bamboo by Finite Element Method. Compos. Part B Eng. 2017, 115, 151–159. [Google Scholar] [CrossRef]
- Gao, Z.; Zhang, X.; Wang, Y.; Yang, R.; Wang, G.; Wang, Z. Measurement of the Poisson’s Ratio of Materials Based on the Bending Mode of the Cantilever Plate. BioResources 2016, 11, 5703–5721. [Google Scholar] [CrossRef]
- Meier, E. WOOD! Identifying and Using Hundreds of Woods Worldwide. The Wood Database. 2015. Available online: https://www.wood-database.com/book/ (accessed on 25 January 2023).
- MatWeb. MatWeb: Online Materials Information Resource. Available online: https://www.matweb.com/ (accessed on 3 March 2023).
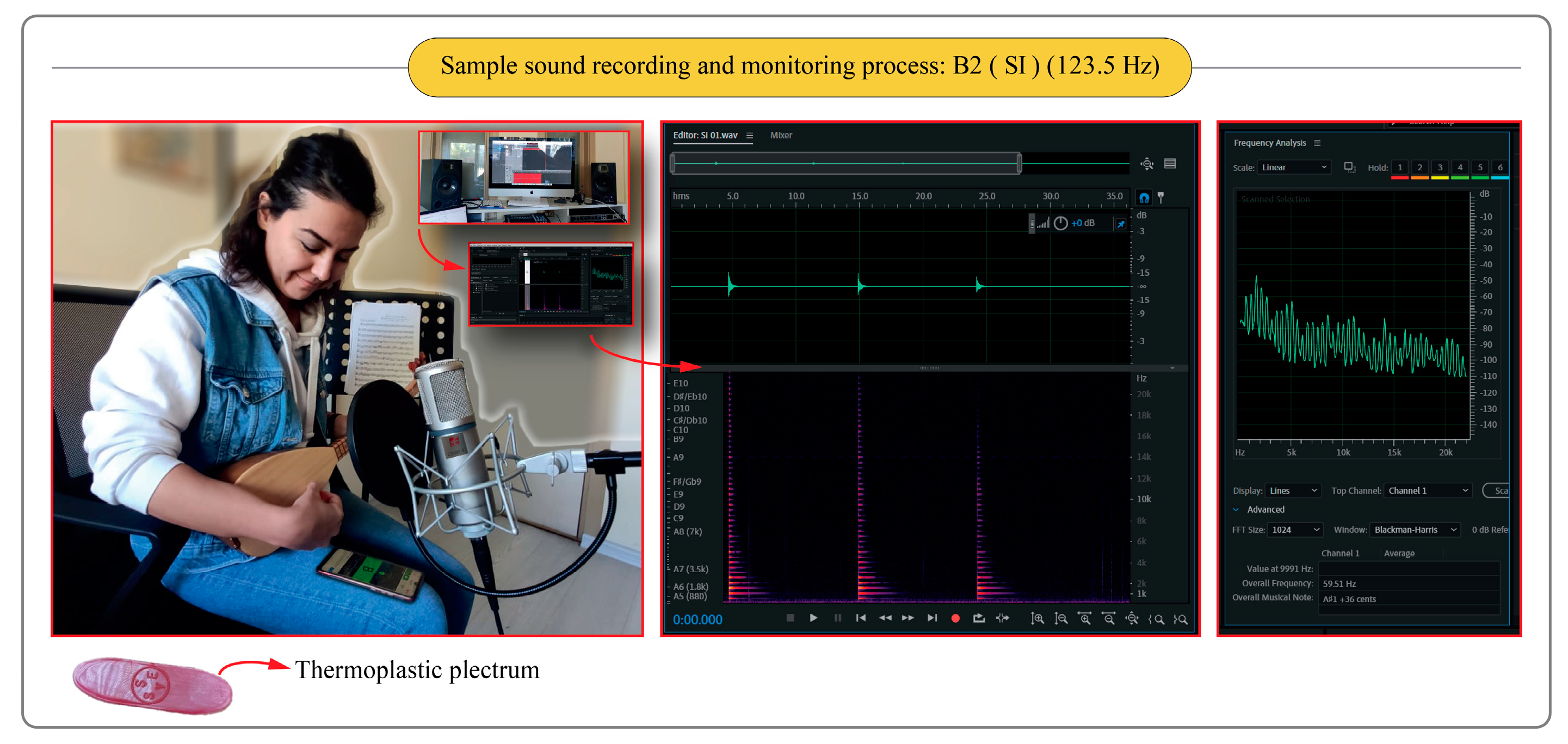
| String Group | Playing Tuning (End Tune) | String Diameter | String Note | Measured Fequency (FFT Size 1024-Blackman-Haarris) | Corresponding String Tension Force | Corresponding Cross Section String Stress |
|---|---|---|---|---|---|---|
| (Reference Tune: A4 (LA) @ 440 Hz) | (mm) | (-) | (Hz) | (N) | (MPa) | |
| Lower String Group (S1 and S2) | B2 (SI) (123.5 Hz) | 0.16 | B4 (SI) | 493.14 ± 0.10 | 30.194 | 1502.488 |
| C3 (DO) (130.8 Hz) | 0.16 | C5 (DO) | 523.54 ± 3.24 | 34.032 | 1693.471 | |
| D3 (RE) (146.8 Hz) | 0.16 | D5 (RE) | 587.42 ± 0.43 | 42.843 | 2131.917 | |
| Middle String Group (S3 and S4) | B2 (SI) (123.5 Hz) | 0.25 | E4 (MI) | 329.10 ± 0.25 | 32.831 | 669.160 |
| C3 (DO) (130.8 Hz) | 0.25 | F4 (FA) | 349.9 ± 2.15 | 37.112 | 756.415 | |
| D3 (RE) (146.8 Hz) | 0.25 | G4 (SOL) | 392.78 ± 1.95 | 46.765 | 953.162 | |
| Upper String Group (S5 and S6) | B2 (SI) (123.5 Hz) | 0.16 | A4 (LA) | 440.5 ± 0.20 | 24.092 | 1198.846 |
| C3 (DO) (130.8 Hz) | 0.16 | B4b (SI b) | 466.11 ± 3.30 | 26.975 | 1342.307 | |
| D3 (RE) (146.8 Hz) | 0.16 | C5 (DO) | 523.32 ± 0.14 | 34.003 | 1692.028 |
| Component No. | Component of the Instrument | Material | Component Coordinates | Modulus of Elasticity | Poisson’s Ratio | Density | Crushing/Tensile Yield Strength |
|---|---|---|---|---|---|---|---|
| (GPa) | (-) | (kg m−3) | (MPa) | ||||
| 1 | Soundboard | Spruce | (Orthotropic) | EL (X): 9.800 | Vxy: 0.462 | 385 | 31.50 |
| ET (Y): 0.580 | Vyz: 0.422 | ||||||
| ER (Z): 1.250 | Vxz: 0.255 | ||||||
| 2 | Soundbox | Mulberry | (Isotropic) | 9.32 | 0.33 | 690 | 48.20 |
| 3 | Neck | Beech | (Orthotropic) | EL (X): 13.900 | Vxy: 0.24 | 710 | 57.00 |
| ET (Y): 0.606 | Vyz: 0.27 | ||||||
| ER (Z): 1.900 | Vxz: 0.27 | ||||||
| 4 | Neck head | (Isotropic) | 13.90 | 0.24 | |||
| 5 | Tunning keys/Pegs | Rosewood | (Isotropic) | 13.93 | 0.37 | 835 | 67.20 |
| 6 | String tie (String holder) | ||||||
| 7 | Bridge | Maple | (Isotropic) | 12.62 | 0.35 | 705 | 54.00 |
| 8 | Nut | Hornbeam | (Isotropic) | 12.10 | 0.38 | 735 | 50.50 |
| 9 | Frets | Nylon 6/Fishing line | (Isotropic) | 1.3–4.2 | 0.39 | 1110–1170 | 40–100 |
| 10 | Lower string group (Dia:0.16 mm) | ASTM A228 Steel (UNS K08500) | (Isotropic) | 210.00 | 0.31 | 7800 | 2860–3165 |
| 11 | Middle string group (Dia:0.25 mm) | ASTM A228 Steel (UNS K08500) | 2670–2950 | ||||
| 12 | Upper string group (Dia:0.16 mm) | ASTM A228 Steel (UNS K08500) | 2860–3165 |
| Playing Tuning (End Tune) | Related Strings | Corresponding String Tension Force | Tension Force Components on the Bridge | Resultant Tension Force on Bridge by Single String | Total Resultant Tension Force on the Bridge | Resultant Tension Force Angle on the Bridge | |
|---|---|---|---|---|---|---|---|
| (T) | T.FX (←) | T.FY (↓) | |||||
| (N) | (N) | (N) | (N) | (N) | (o) | ||
| B2 (SI) (123.5 Hz) | S1 = S2 | 30.194 | 0.069 | 2.233 | 2.234 | 12.89 | 1.77 |
| S3 = S4 | 32.831 | 0.075 | 2.428 | 2.429 | |||
| S5 = S6 | 24.092 | 0.055 | 1.782 | 1.782 | |||
| C3 (DO) (130.8 Hz) | S1 = S2 | 34.032 | 0.078 | 2.517 | 2.518 | 14.52 | 1.77 |
| S3 = S4 | 37.112 | 0.085 | 2.744 | 2.746 | |||
| S5 = S6 | 26.975 | 0.062 | 1.995 | 1.996 | |||
| D3 (RE) (146.8 Hz) | S1 = S2 | 42.843 | 0.098 | 3.168 | 3.170 | 18.29 | 1.77 |
| S3 = S4 | 46.765 | 0.107 | 3.458 | 3.460 | |||
| S5 = S6 | 34.003 | 0.078 | 2.515 | 2.516 | |||
| Structural Components | Material Reference to Failure | Playing Tuning (End Tune) | |||||
|---|---|---|---|---|---|---|---|
| B2 (SI) (123.5 Hz) | C3 (DO) (130.8 Hz) | D3 (RE) (146.8 Hz) | |||||
| FEA Eq. Stress Output | Factor of Safety (FoS) | FEA Eq. Stress Output | Factor of Safety (FoS) | FEA Eq. Stress Output | Factor of Safety (FoS) | ||
| (MPa) | (MPa) | (-) | (MPa) | (-) | (MPa) | (-) | |
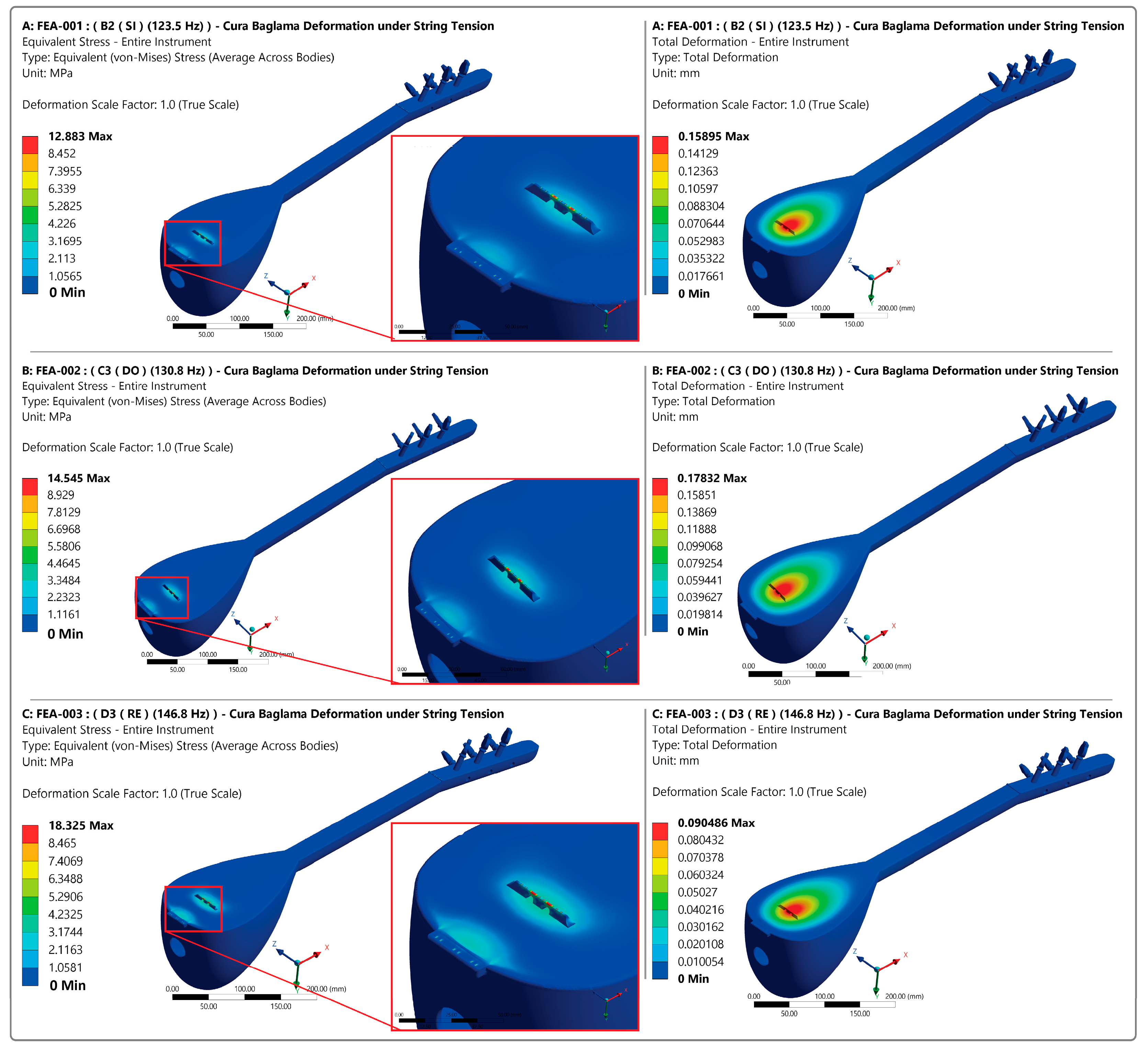
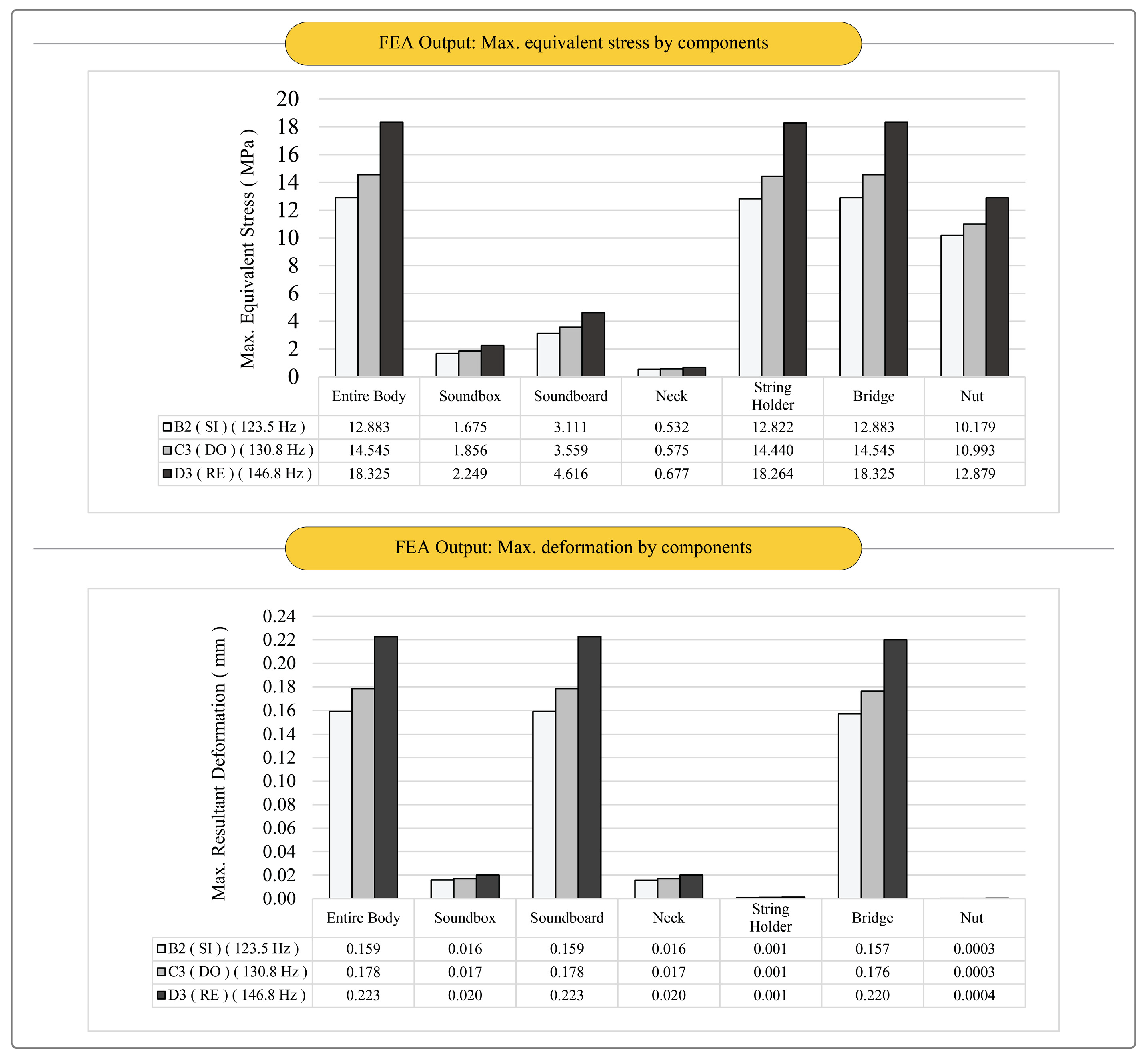
| Soundbox | 48.20 | 1.675 | 28.78 | 1.856 | 25.97 | 2.249 | 21.43 |
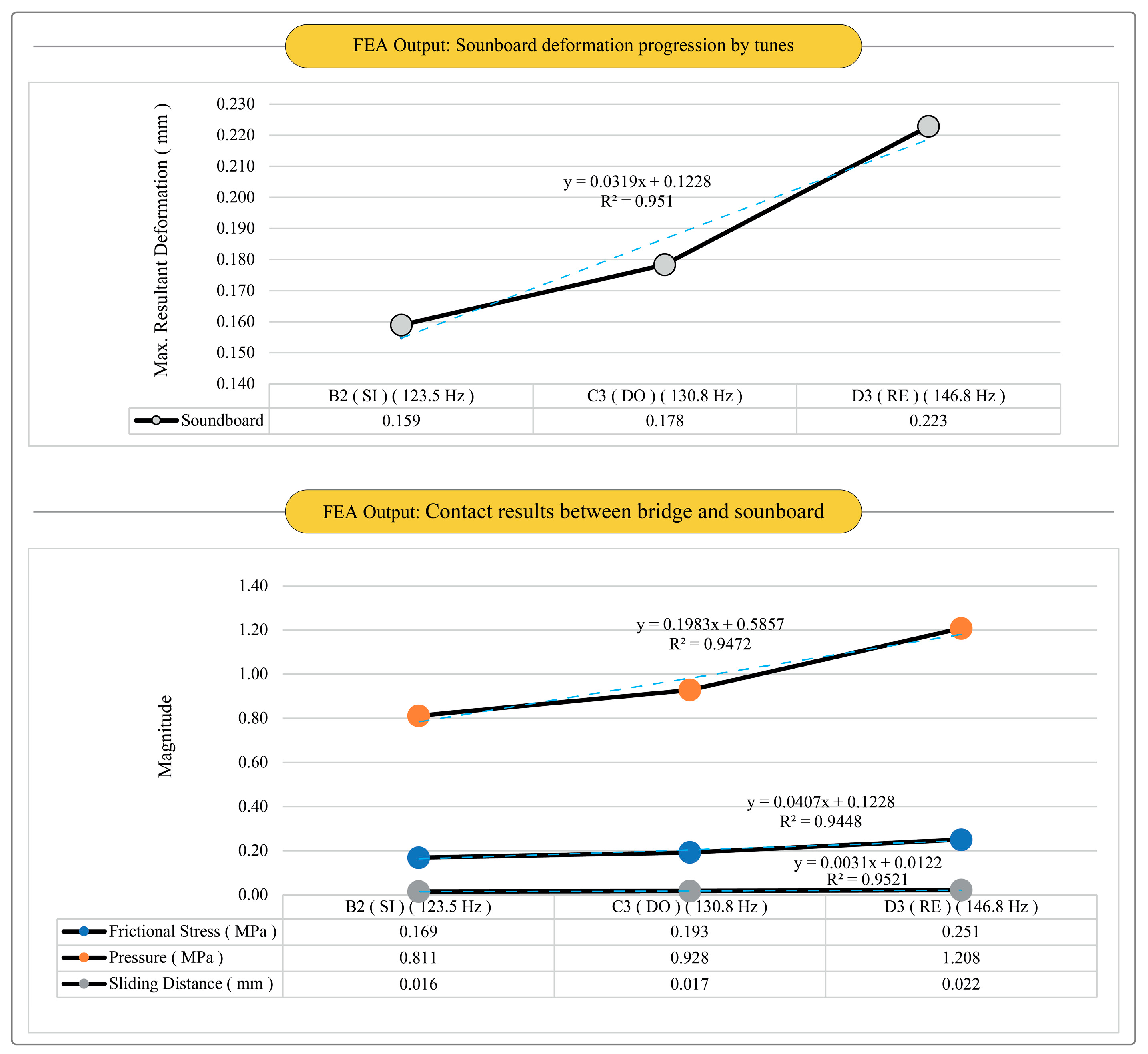
| Soundboard | 31.50 | 3.111 | 10.12 | 3.559 | 8.85 | 4.616 | 6.82 |
| Neck | 57.00 | 0.532 | 107.16 | 0.575 | 99.06 | 0.677 | 84.20 |
| String tie (String holder) | 67.20 | 12.822 | 5.24 | 14.440 | 4.65 | 18.264 | 3.68 |
| Bridge | 54.00 | 12.883 | 4.19 | 14.545 | 3.71 | 18.325 | 2.95 |
| Nut | 50.50 | 10.179 | 4.96 | 10.993 | 4.59 | 12.879 | 3.92 |
| Strings (ASTM A228 Steel (UNS K08500)) | Material Reference to Failure | Playing Tuning (End Tune) | |||||
| B2 (SI) (123.5 Hz) | C3 (DO) (130.8 Hz) | D3 (RE) (146.8 Hz) | |||||
| Section Stress | Factor of Safety (FoS) | Section Stress | Factor of Safety (FoS) | Section Stress | Factor of Safety (FoS) | ||
| (MPa) | (MPa) | (-) | (MPa) | (-) | (MPa) | (-) | |
| Lower string group (Dia:0.16 mm) | 2860 | 1502.49 | 1.90 | 1693.47 | 1.69 | 2131.92 | 1.34 |
| Middle string group (Dia:0.25 mm) | 2670 | 669.16 | 3.99 | 756.42 | 3.53 | 953.16 | 2.80 |
| Upper string group (Dia:0.16 mm) | 2860 | 1198.85 | 2.39 | 1342.31 | 2.13 | 1692.03 | 1.69 |
| Component | Playing Tuning (End Tune) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| B2 (SI) (123.5 Hz) | C3 (DO) (130.8 Hz) | D3 (RE) (146.8 Hz) | ||||||||||
| RX | RY | RResultant | Resultant Reaction Force Angle | RX | RY | RResultant | Resultant Reaction Force Angle | RX | RY | RResultant | Resultant Reaction Force Angle | |
| (N) | (N) | (N) | (o) | (N) | (N) | (N) | (o) | (N) | (N) | (N) | (o) | |
| String tie (String holder) | 7.940 | 13.600 | 15.75 | 30.28 | 8.670 | 15.100 | 17.41 | 29.86 | 10.400 | 18.500 | 21.22 | 29.34 |
| Nut | 7.540 | 3.830 | 8.46 | 63.07 | 8.220 | 3.990 | 9.14 | 64.11 | 9.800 | 4.350 | 10.72 | 66.06 |
| Bridge-Contact surface | 0.398 | 12.900 | 12.91 | 1.77 | 0.45 | 14.500 | 14.51 | 1.77 | 0.566 | 18.300 | 18.31 | 1.77 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Celik, H.K.; Gok, S.; Caglayan, N.; Rennie, A.E.W. A Numerical Method-Based Analysis of the Structural Deformation Behaviour of a Turkish String Instrument (Cura Baglama) under Varying String Tensions. Appl. Sci. 2023, 13, 9682. https://doi.org/10.3390/app13179682
Celik HK, Gok S, Caglayan N, Rennie AEW. A Numerical Method-Based Analysis of the Structural Deformation Behaviour of a Turkish String Instrument (Cura Baglama) under Varying String Tensions. Applied Sciences. 2023; 13(17):9682. https://doi.org/10.3390/app13179682
Chicago/Turabian StyleCelik, H. Kursat, Sevilay Gok, Nuri Caglayan, and Allan E. W. Rennie. 2023. "A Numerical Method-Based Analysis of the Structural Deformation Behaviour of a Turkish String Instrument (Cura Baglama) under Varying String Tensions" Applied Sciences 13, no. 17: 9682. https://doi.org/10.3390/app13179682
APA StyleCelik, H. K., Gok, S., Caglayan, N., & Rennie, A. E. W. (2023). A Numerical Method-Based Analysis of the Structural Deformation Behaviour of a Turkish String Instrument (Cura Baglama) under Varying String Tensions. Applied Sciences, 13(17), 9682. https://doi.org/10.3390/app13179682









