Research on Dynamic Response of a Single-Tower Cable-Stayed Bridge with Successive Cable Breaks Based on a 3D Index
Abstract
1. Introduction
2. Evaluation Index and Analysis Method of Broken Cable
2.1. Evaluation Index
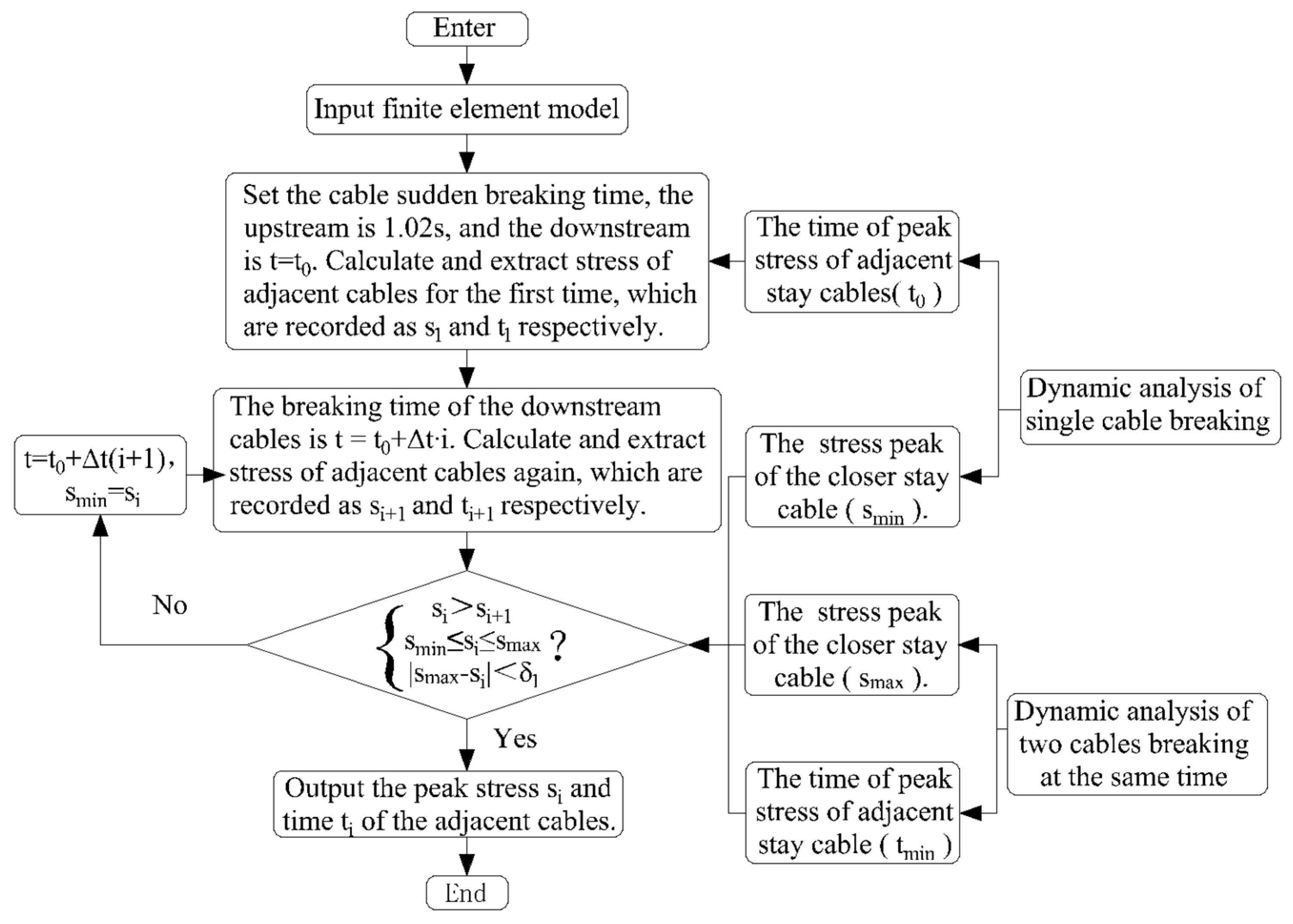
2.2. Analytical Method
- (1)
- Enter ANSYS and input the finite element model.
- (2)
- Set an appropriate static analysis step size. The static analysis of the structure is carried out to obtain the initial stress and deformation of the structural cable before breaking.
- (3)
- Set the step size of the dynamic analysis and perform the dynamic calculation. First, the cable element is broken in an instant, and the appropriate analysis step and integration time are specified. Second, in order to obtain the maximum dynamic response of the structure, the analysis sub-step is extended. Finally, the analysis step is extended again, and the integration time is increased.
- (4)
- Extract the dynamic response time history of the structure.
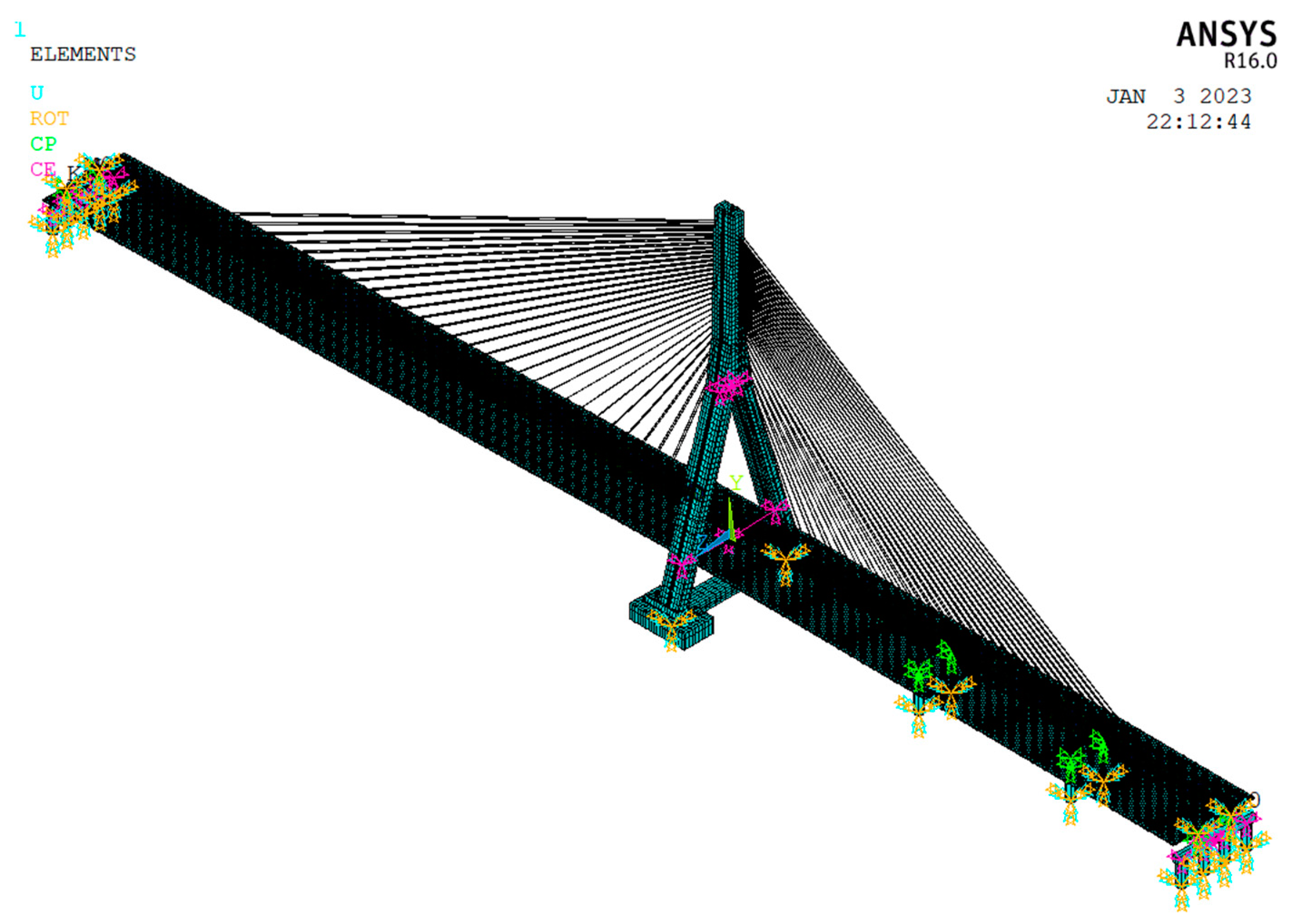
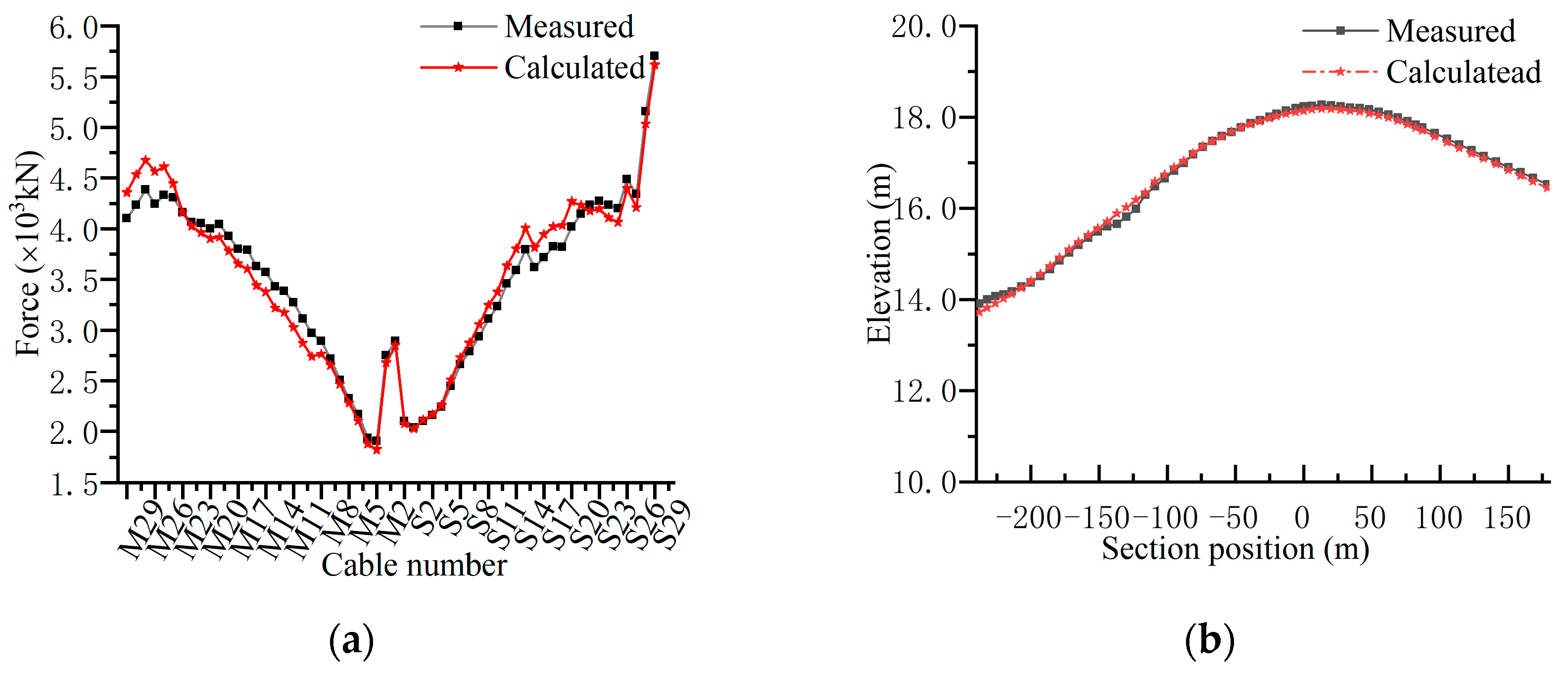
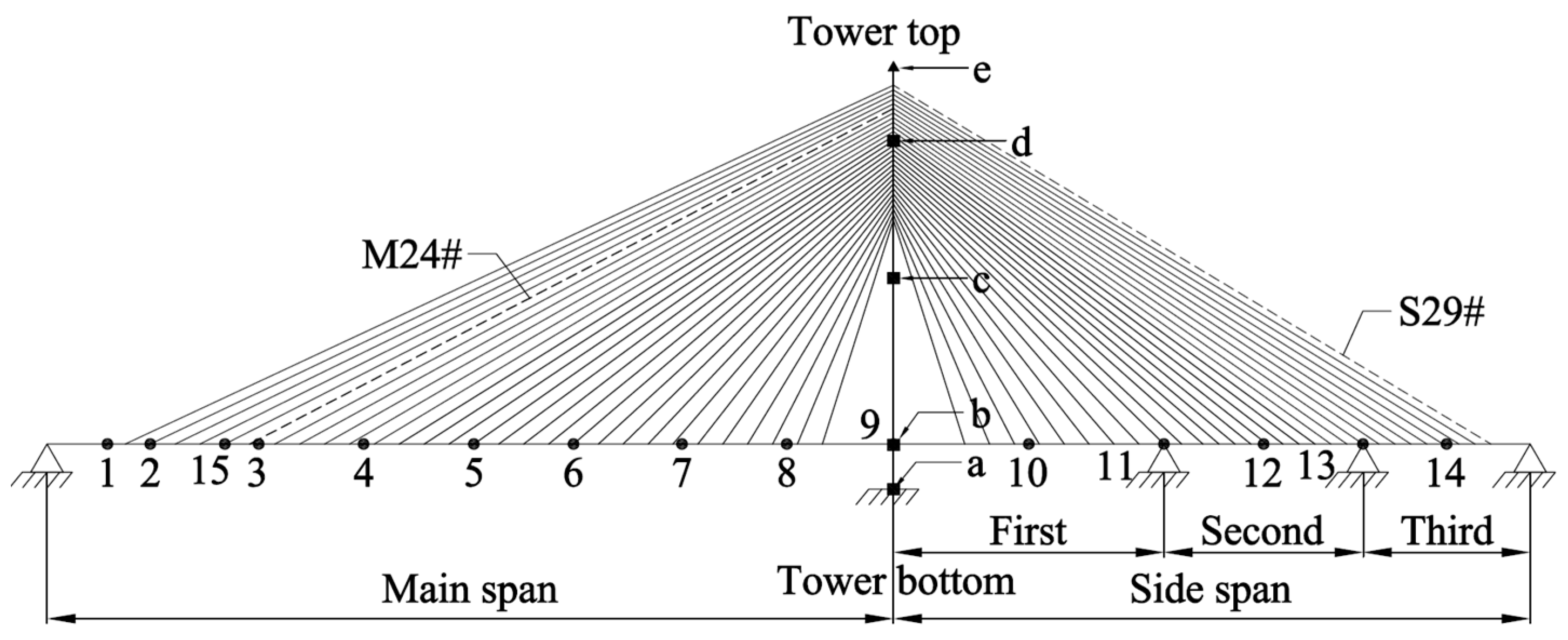
3. Benchmark Finite Element Model
3.1. Case Study
3.2. Finite Element Model
4. Cable-Breaking Simulation
4.1. Cable-Breaking Time
4.2. Analysis Condition Selection
5. Results
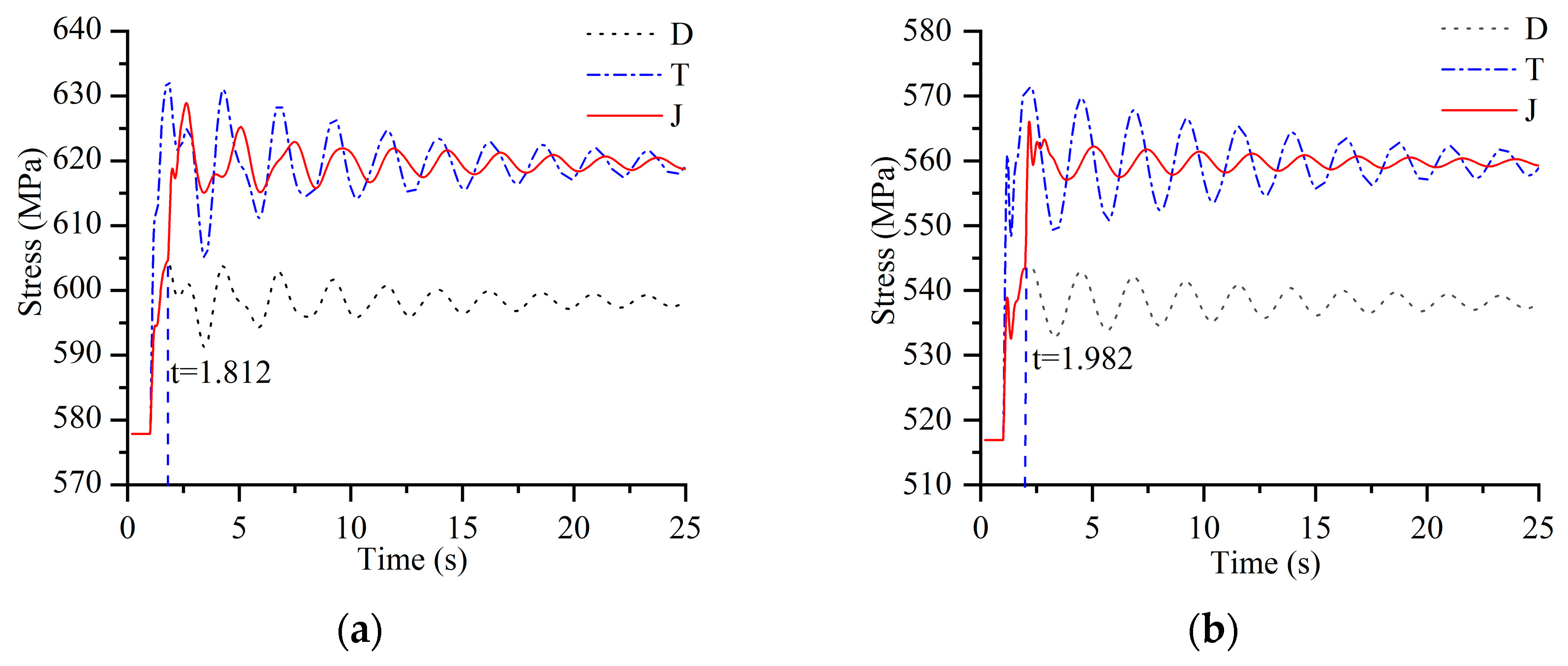
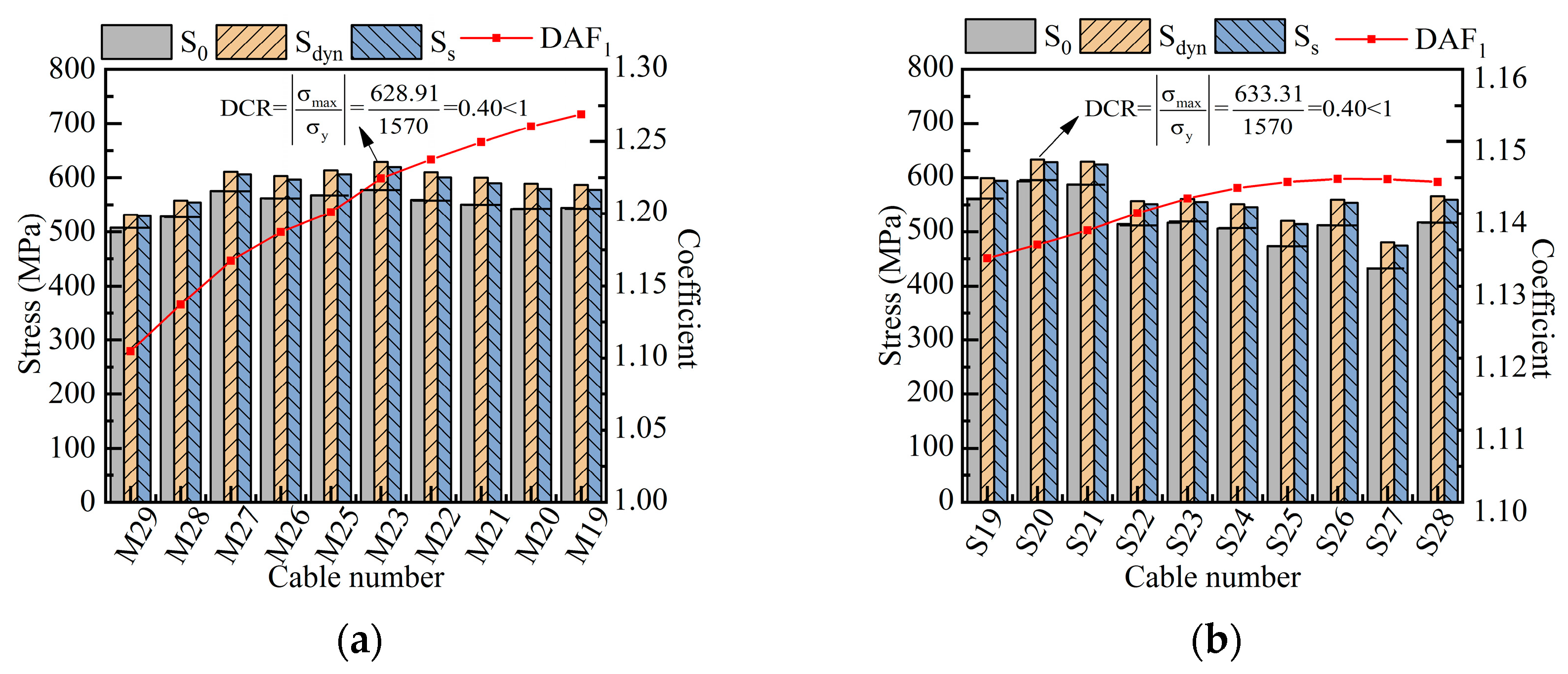
5.1. Cable Stress
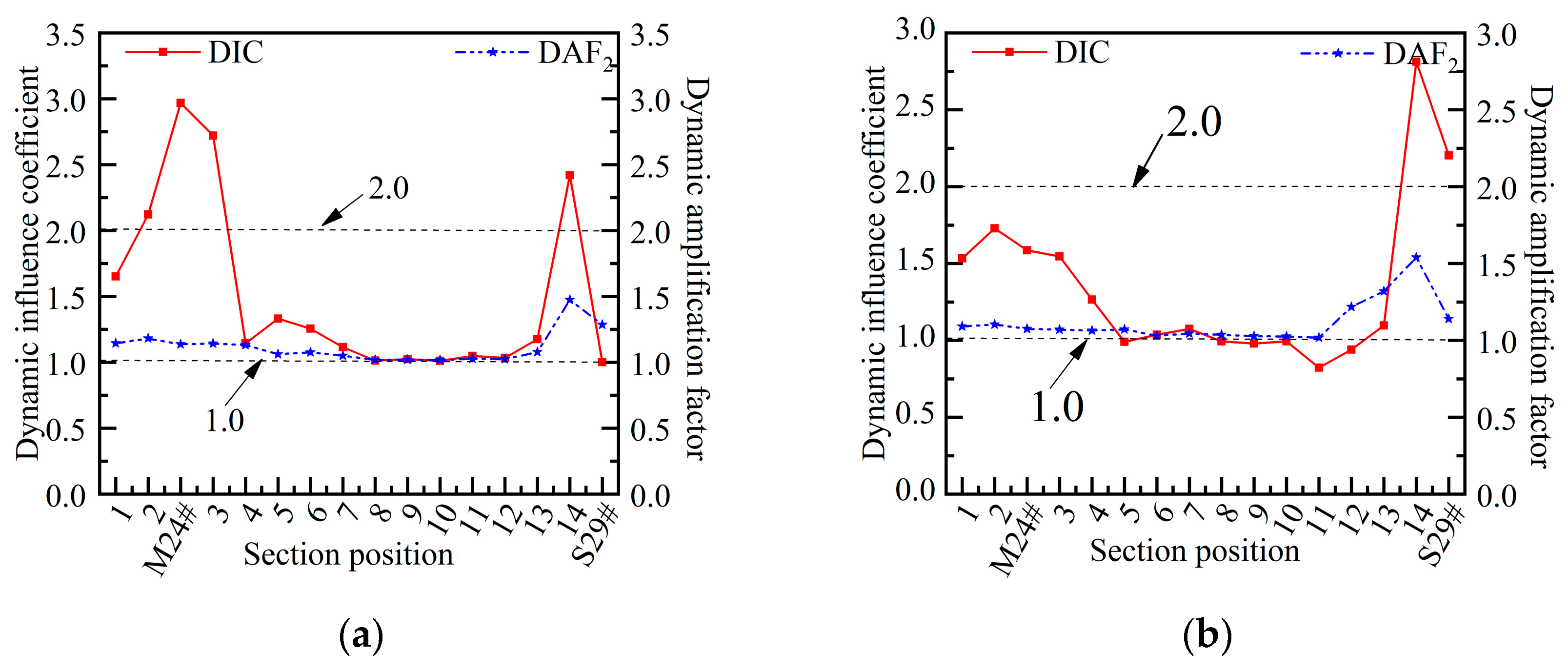
5.2. Stress of the Main Beam
5.3. Tower Stress
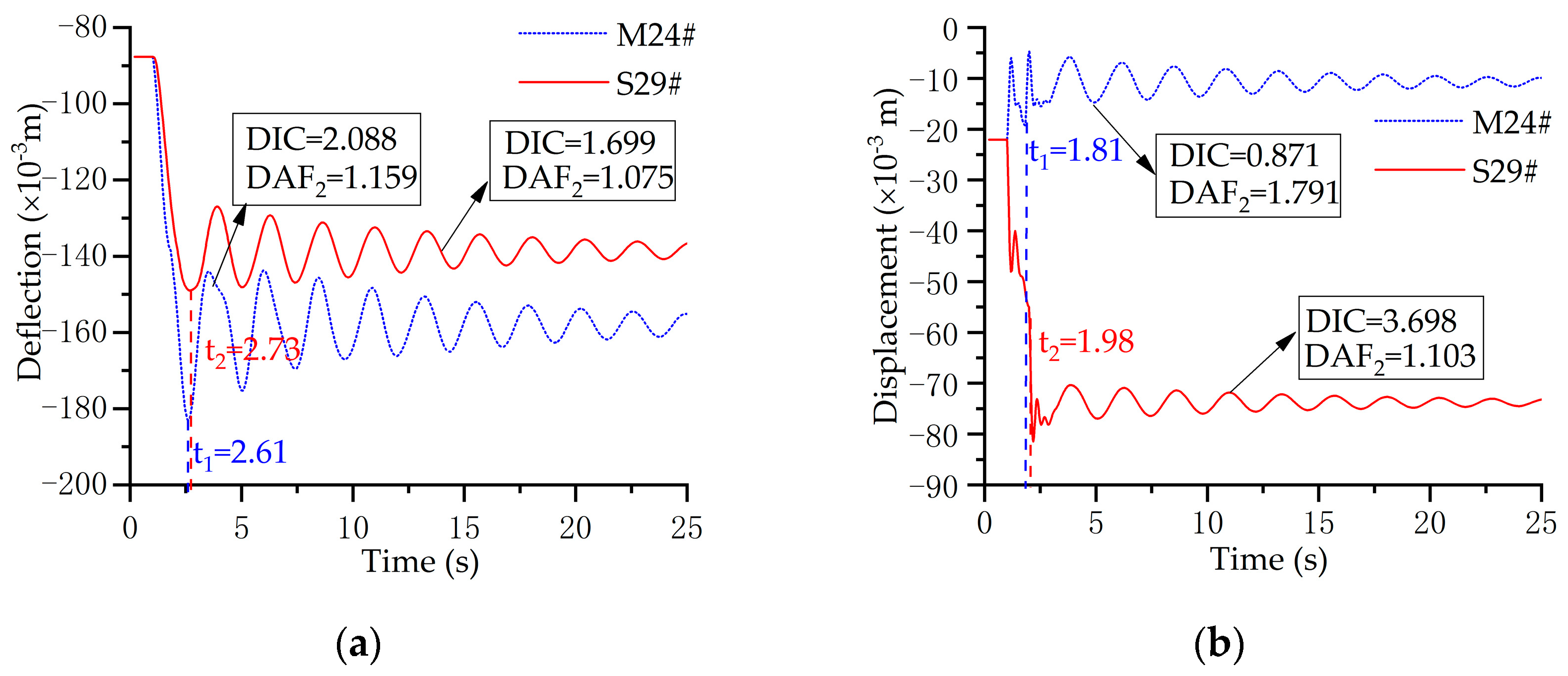
5.4. Structural Displacement
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Broken Object | Cable Number | Stress (MPa) | Sdyn − S0 | Ss − S0 | DAF1 | DCR | ||
|---|---|---|---|---|---|---|---|---|
| S0 | Sdyn | Ss | ||||||
| #M24 | #M29 | 507.78 | 531.26 | 529.04 | 23.48 | 21.26 | 1.105 | 0.34 |
| #M28 | 528.48 | 557.78 | 554.25 | 29.30 | 25.76 | 1.137 | 0.36 | |
| #M27 | 575.66 | 610.95 | 605.89 | 35.30 | 30.23 | 1.167 | 0.39 | |
| #M26 | 562.01 | 602.94 | 596.49 | 40.93 | 34.47 | 1.187 | 0.38 | |
| #M25 | 567.70 | 613.56 | 605.89 | 45.86 | 38.19 | 1.201 | 0.39 | |
| #M23 | 577.87 | 628.91 | 619.56 | 51.04 | 41.68 | 1.224 | 0.40 | |
| #M22 | 559.20 | 610.05 | 600.29 | 50.85 | 41.09 | 1.237 | 0.39 | |
| #M21 | 550.45 | 599.72 | 589.88 | 49.27 | 39.43 | 1.250 | 0.38 | |
| #M20 | 542.37 | 588.90 | 579.28 | 46.53 | 36.92 | 1.260 | 0.38 | |
| #M19 | 544.13 | 586.97 | 577.89 | 42.84 | 33.76 | 1.269 | 0.37 | |
| #S29 | #S19 | 560.33 | 598.84 | 594.30 | 38.51 | 33.96 | 1.134 | 0.38 |
| #S20 | 593.28 | 633.31 | 628.53 | 40.03 | 35.25 | 1.136 | 0.40 | |
| #S21 | 587.72 | 629.26 | 624.23 | 41.54 | 36.51 | 1.138 | 0.40 | |
| #S22 | 513.88 | 556.74 | 551.47 | 42.85 | 37.59 | 1.140 | 0.35 | |
| #S23 | 516.63 | 560.75 | 555.26 | 44.11 | 38.62 | 1.142 | 0.36 | |
| #S24 | 505.98 | 551.29 | 545.60 | 45.31 | 39.62 | 1.144 | 0.35 | |
| #S25 | 473.51 | 519.93 | 514.08 | 46.42 | 40.57 | 1.144 | 0.33 | |
| #S26 | 512.23 | 559.67 | 553.67 | 47.44 | 41.44 | 1.145 | 0.36 | |
| #S27 | 432.28 | 480.58 | 474.47 | 48.30 | 42.19 | 1.145 | 0.31 | |
| #S28 | 516.92 | 565.88 | 559.70 | 48.96 | 42.78 | 1.144 | 0.36 | |
| Broken Object | Section Position | Stress (MPa) | DIC | DAF2 | DCR | ||
|---|---|---|---|---|---|---|---|
| S0 | Sdyn | Ss | |||||
| #M24 | #1 | 1.36 | 2.25 | 1.96 | 1.653 | 1.144 | 0.07 |
| #2 | 1.42 | 3.02 | 2.56 | 2.123 | 1.182 | 0.09 | |
| M24# | 1.80 | 5.33 | 4.69 | 2.968 | 1.137 | 0.16 | |
| #3 | 1.87 | 5.10 | 4.46 | 2.721 | 1.142 | 0.16 | |
| #4 | 2.55 | 2.92 | 2.58 | 1.144 | 1.131 | 0.09 | |
| #5 | −4.72 | −6.28 | −5.92 | 1.331 | 1.060 | 0.19 | |
| #6 | −6.68 | −8.39 | −7.79 | 1.255 | 1.076 | 0.26 | |
| #7 | −6.60 | −7.36 | −7.01 | 1.116 | 1.050 | 0.23 | |
| #8 | 7.41 | 7.51 | 7.37 | 1.012 | 1.018 | 0.23 | |
| #9 | −6.18 | −6.32 | −6.19 | 1.023 | 1.021 | 0.20 | |
| #10 | 7.33 | 7.42 | 7.29 | 1.012 | 1.017 | 0.23 | |
| #11 | −4.52 | −4.74 | −4.61 | 1.049 | 1.028 | 0.15 | |
| #12 | 2.47 | 2.55 | 2.49 | 1.033 | 1.023 | 0.08 | |
| #13 | −2.43 | −2.86 | −2.65 | 1.176 | 1.078 | 0.09 | |
| #14 | 0.16 | 0.39 | 0.27 | 2.420 | 1.473 | 0.01 | |
| #S29 | −0.478 | −0.479 | −0.37 | 1.002 | 1.285 | 0.01 | |
| #S29 | #1 | 1.36 | 2.08 | 1.91 | 1.534 | 1.090 | 0.06 |
| #2 | 1.42 | 2.46 | 2.23 | 1.728 | 1.103 | 0.08 | |
| #M24 | 1.80 | 2.85 | 2.65 | 1.585 | 1.074 | 0.09 | |
| #3 | 1.87 | 2.90 | 2.71 | 1.546 | 1.069 | 0.09 | |
| #4 | 2.55 | 3.23 | 3.04 | 1.266 | 1.063 | 0.10 | |
| #5 | −4.72 | −4.67 | −4.36 | 0.990 | 1.072 | 0.14 | |
| #6 | −6.68 | −6.92 | −6.71 | 1.036 | 1.031 | 0.21 | |
| #7 | −6.60 | −7.10 | −6.80 | 1.075 | 1.044 | 0.22 | |
| #8 | 7.41 | 7.37 | 7.12 | 0.994 | 1.036 | 0.23 | |
| #9 | −6.18 | −6.05 | −5.89 | 0.979 | 1.027 | 0.19 | |
| #10 | 7.33 | 7.28 | 7.10 | 0.994 | 1.026 | 0.22 | |
| #11 | −4.52 | −3.72 | −3.65 | 0.822 | 1.018 | 0.11 | |
| #12 | 2.47 | 2.32 | 1.91 | 0.940 | 1.217 | 0.07 | |
| #13 | −2.43 | −2.66 | −2.02 | 1.097 | 1.319 | 0.08 | |
| #14 | 0.16 | 0.46 | 0.30 | 2.812 | 1.539 | 0.01 | |
| #S29 | −0.48 | 1.05 | 0.92 | 2.203 | 1.140 | 0.03 | |
References
- Zhao, X. Effect Study of Cable Damage to Structural Performance of the Cable-Stayed Bridge. Ph.D. Thesis, Southeast University, Nanjing, China, June 2005; pp. 85–105. [Google Scholar]
- Mozosa, C.M.; Aparicio, A.C. Numerical and experimental study on the interaction cable structure during the failure of a stay in a cable stayed bridge. Eng. Struct. 2011, 33, 2330–2341. [Google Scholar] [CrossRef]
- Yafei, M.; Anyin, P.; Lei, W.; Dai, L. Model test on static performance degradation of cable-stayed bridge with cable rupture and main girder damage. Arch. Appl. Mech. 2022, 53, 653–664. (In Chinese) [Google Scholar]
- Raftoyiannis, I.G.; Konstantakopoulos, T.G.; Michaltsos, G.T. Dynamic response of cable-stayed bridges subjected to sudden failure of stays—The 2D problem. Coupled Syst. Mech. 2014, 3, 345–365. [Google Scholar] [CrossRef]
- Michaltsos, G.T.; Sophianopoulos, D.S.; Avraam, T.P. Dynamic response of cable-stayed bridges due to sudden failure of stays: The 3D problem. Arch. Appl. Mech. 2020, 90, 1431–1456. [Google Scholar] [CrossRef]
- Sheng, K.C.; Huan, K.C. The influence of broken cables on the structural behavior of long-span cable-stayed bridges. J. Mar. Sci. Technol. 2010, 18, 395–404. [Google Scholar]
- Kim, S.; Kang, Y.J. Structural behavior of cable-stayed bridges after cable failure. Struct. Eng. Mech. 2016, 59, 1095–1120. [Google Scholar] [CrossRef]
- Ruiz-Teran, A.M.; Aparicio, A.C. Response of under-deck cable-stayed bridges to the accidental breakage of stay cables. Eng. Struct. 2009, 31, 1425–1434. [Google Scholar] [CrossRef][Green Version]
- Mozosa, C.M.; Aparicio, A.C. Parametric study on the dynamic response of cable stayed bridges to the sudden failure of a stay, Part I: Bending moment acting on the deck. Eng. Struct. 2010, 32, 3328–3330. [Google Scholar] [CrossRef]
- Mozosa, C.M.; Aparicio, A.C. Parametric study on the dynamic response of cable stayed bridges to the sudden failure of a stay, Part II: Bending moment acting on the pylons and stress on the stays. Eng. Struct. 2010, 32, 3301–3312. [Google Scholar] [CrossRef]
- Cai, J.-G.; Xu, Y.-X.; Zhang, L.-P.; Feng, J.; Zhang, J. Comparison of various procedures for progressive collapse analysis of cable-stayed bridges. J. Zhejiang Univ. 2012, 13, 323–334. [Google Scholar] [CrossRef]
- Aoki, Y.; Valipour, H.; Samali, B.; Saleh, A. A Study on Potential Progressive Collapse Responses of Cable-Stayed Bridges. Adv. Struct. Eng. 2013, 16, 689–706. [Google Scholar] [CrossRef]
- Zhou, Y.; Chen, S. Time-Progressive Dynamic Assessment of Abrupt Cable-Breakage Events on Cable-Stayed Bridges. J. Bridge Eng. 2013, 19, 159–171. [Google Scholar] [CrossRef]
- Zhou, Y.; Chen, S. Reliability Assessment Framework of the Long-Span Cable-Stayed Bridge and Traffic System Subjected to Cable Breakage Events. J. Bridge Eng. 2017, 22, 1–18. [Google Scholar] [CrossRef]
- Zheng, X.-B.; Zhang, G.; Song, Y.-F. Dynamic response of cable breakage in double-tower cable-stayed bridge with steel truss girder. J. Chang. Univ. 2017, 37, 70–77. (In Chinese) [Google Scholar]
- Zhang, Y.; Fang, Z.; Lu, J.; Xiang, Y.; Long, H. Broken cable-induced dynamic response of long-span concrete cable stayed bridge during construction. J. Vib. Shock. 2021, 40, 237–246. [Google Scholar]
- Wang, T.; Zhang, X.; Wang, L. The Investigation on dynamic response of cable-stayed bridge and train on bridge under cable break conditions. J. Southwest Jiaotong Univ. 2022, 30, 1023–1041. (In Chinese) [Google Scholar]
- Recommendations for Stay Cable Design, Testing and Lnstallation; Post-Tensioning Institute: Phoenix, AZ, USA, 2007.
- Hong, G.; Zhaohui, C.; Lai, Y.; Chung, T.M. Analysis of Cable Rupture Dynamic Amplification Factor of Cable-stayed Bridge. In Proceedings of the 11th National Symposium on the Theory and Application of Random Vibration, Yichang, China, 12–14 October 2018; pp. 328–339. (In Chinese). [Google Scholar]
- JTG D64-2015; Specifications for Design of Highway Steel Bridge. People’s Communications Press: Beijing, China, 2015.
- Zhaole, Q.; Xuefei, S.; Li, X.; Ruan, X. Research on Dynamic Simulation Methodology for Cable Loss of Cable-Stayed Bridges. Struct. Eng. 2009, 25, 89–92. (In Chinese) [Google Scholar]
- JTG/T 3360-01—2018; Wind-Resistant Design Specification for Highway Bridges. People’s Communications Press: Beijing, China, 2018.
- JTG/T 3365-01—2020; Design Specifications of Highway Cable Stayed Bridge. People’s Communications Press: Beijing, China, 2020.




| Broken Object | Observation Point | Stress (MPa) | DIC | DAF2 | DCR | ||
|---|---|---|---|---|---|---|---|
| S0 | Sdyn | Ss | |||||
| #M24 | a | −7.93 | −8.29 | −7.80 | 1.045 | 1.063 | 0.26 |
| b | −13.40 | −13.55 | −13.15 | 1.011 | 1.030 | 0.42 | |
| c | −6.94 | −7.02 | −6.70 | 1.012 | 1.048 | 0.22 | |
| d | −7.59 | −7.25 | −6.98 | 0.955 | 1.039 | 0.22 | |
| #S29 | a | −7.93 | −8.94 | −8.28 | 1.127 | 1.081 | 0.28 |
| b | −13.40 | −14.43 | −13.94 | 1.077 | 1.035 | 0.45 | |
| c | −6.94 | −8.10 | −7.75 | 1.167 | 1.044 | 0.25 | |
| d | −7.59 | −10.09 | −9.73 | 1.329 | 1.036 | 0.31 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Wang, S.; Huang, K.; Zhong, J.; Cheng, H. Research on Dynamic Response of a Single-Tower Cable-Stayed Bridge with Successive Cable Breaks Based on a 3D Index. Appl. Sci. 2023, 13, 9197. https://doi.org/10.3390/app13169197
Chen Y, Wang S, Huang K, Zhong J, Cheng H. Research on Dynamic Response of a Single-Tower Cable-Stayed Bridge with Successive Cable Breaks Based on a 3D Index. Applied Sciences. 2023; 13(16):9197. https://doi.org/10.3390/app13169197
Chicago/Turabian StyleChen, Yongjian, Song Wang, Kai Huang, Jiwei Zhong, and Hui Cheng. 2023. "Research on Dynamic Response of a Single-Tower Cable-Stayed Bridge with Successive Cable Breaks Based on a 3D Index" Applied Sciences 13, no. 16: 9197. https://doi.org/10.3390/app13169197
APA StyleChen, Y., Wang, S., Huang, K., Zhong, J., & Cheng, H. (2023). Research on Dynamic Response of a Single-Tower Cable-Stayed Bridge with Successive Cable Breaks Based on a 3D Index. Applied Sciences, 13(16), 9197. https://doi.org/10.3390/app13169197





