Influence of Cavitation and Shaft Deformation in the Analysis of Lubrication of the Stern Bearing
Abstract
:1. Introduction
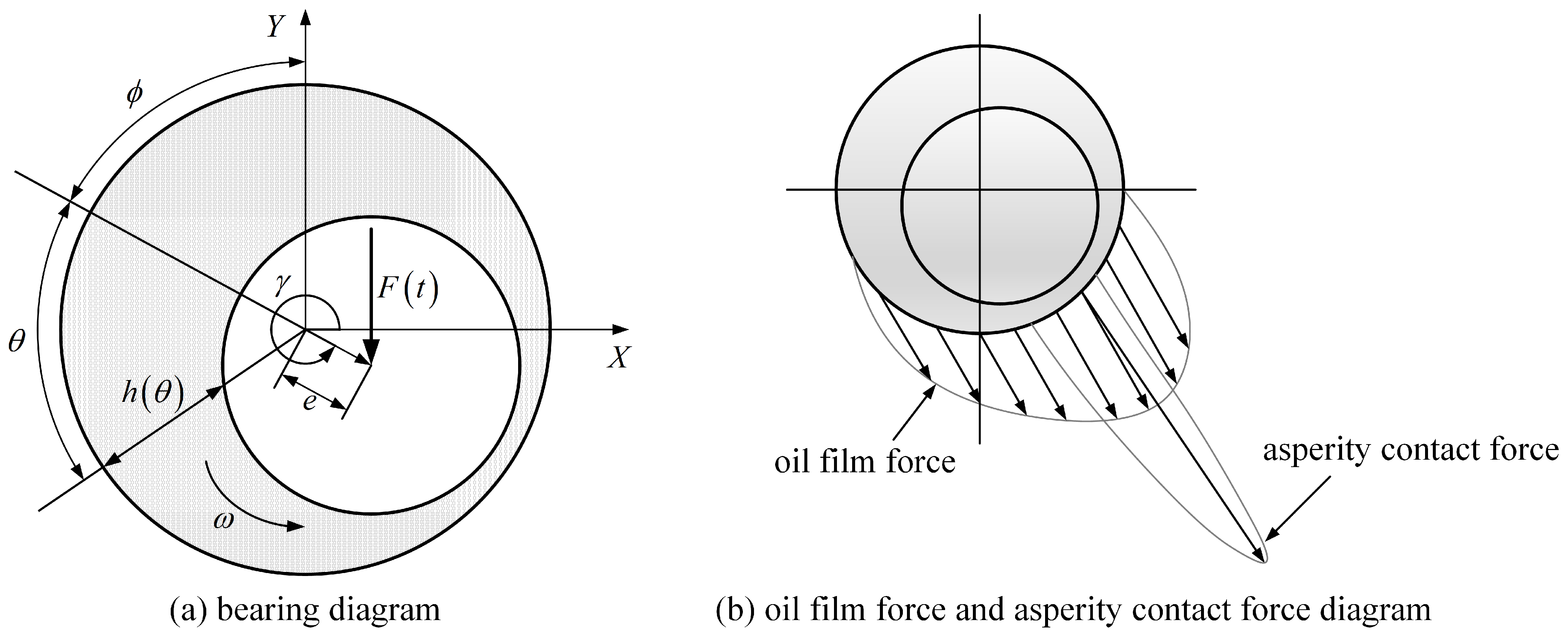
2. Governing Equations of Lubrication Model
2.1. Force Balance Model of Shaft
2.2. Coefficient of Friction
2.3. Mixed Lubrication Model Considering Surface Roughness
2.4. Contact Force of Micro-Convex Body
3. General Equation Considering Cavitation Effect
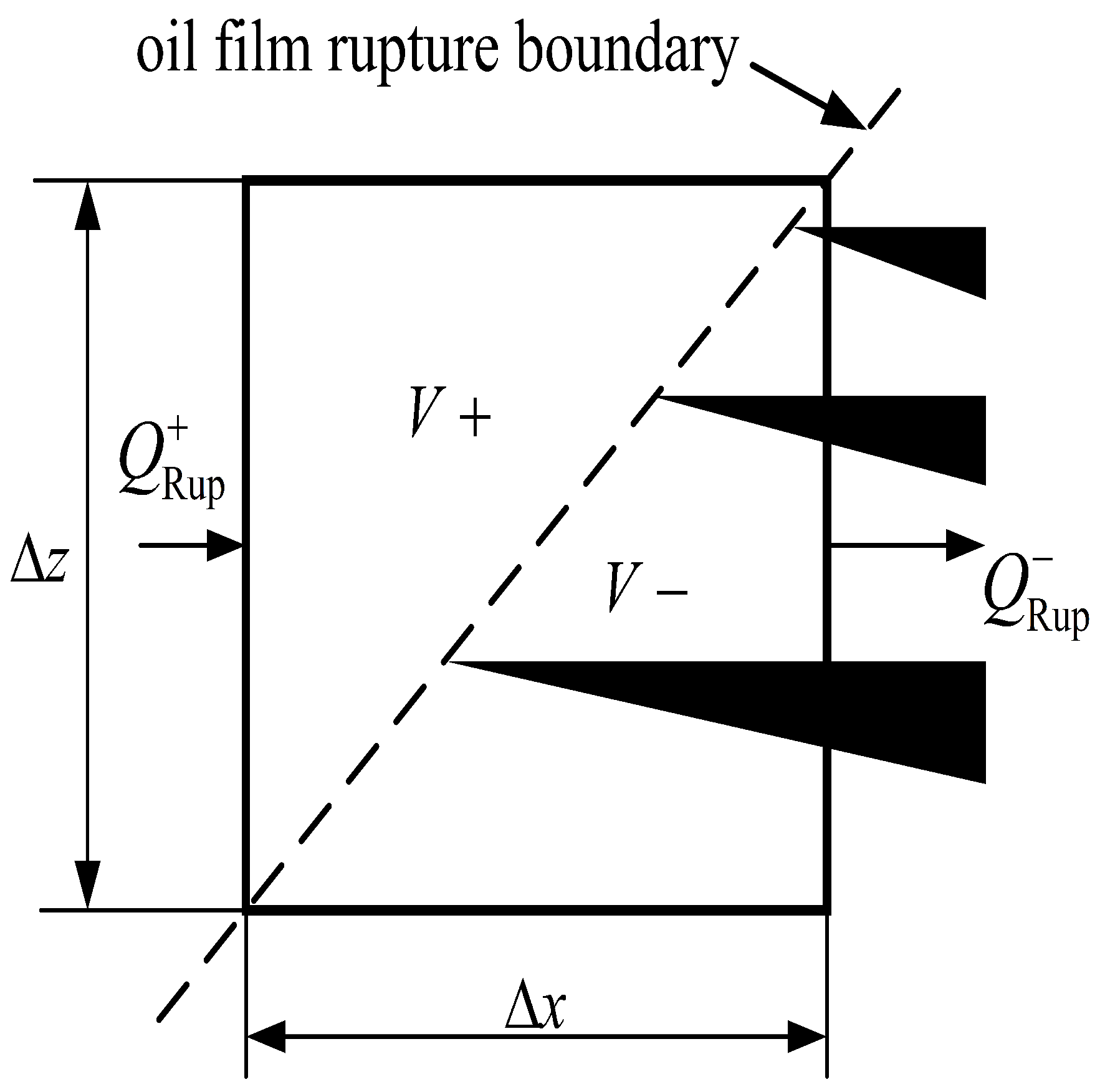
3.1. Boundary Conditions of Oil Film Rupture
3.2. Boundary Conditions of Oil Film Reformation
3.3. Control Equation of Cavitation Zone
4. Oil Film Thickness Equation Considering Shaft Deflection
- (1)
- The basic parameters of the bearing are input, such as bearing diameter, bearing radius clearance, bearing width, external load, lubricating oil viscosity, etc. The surface roughness of the bearing and the shaft and the pressure of the cavitation zone are input. The initial value of the eccentricity and the deviation angle are given, and the switching function (g) is set to 1;
- (2)
- The general Reynolds Equation (37) is solved to obtain the distribution of and update the value of g. The finite difference method and the successive over-relaxation algorithm are applied for the calculation. The contact force of the asperity is solved. Then, the oil film force and the contact force of the asperity are substituted into the following formula to determine if the calculation is convergent:where , is the convergence precision in the calculation. A typical value for the convergence criteria is 0.003. If the above convergence condition is not satisfied, the eccentricity, offset angle, and oil film thickness are adjusted until the convergence condition is satisfied;
- (3)
5. Results and Analysis
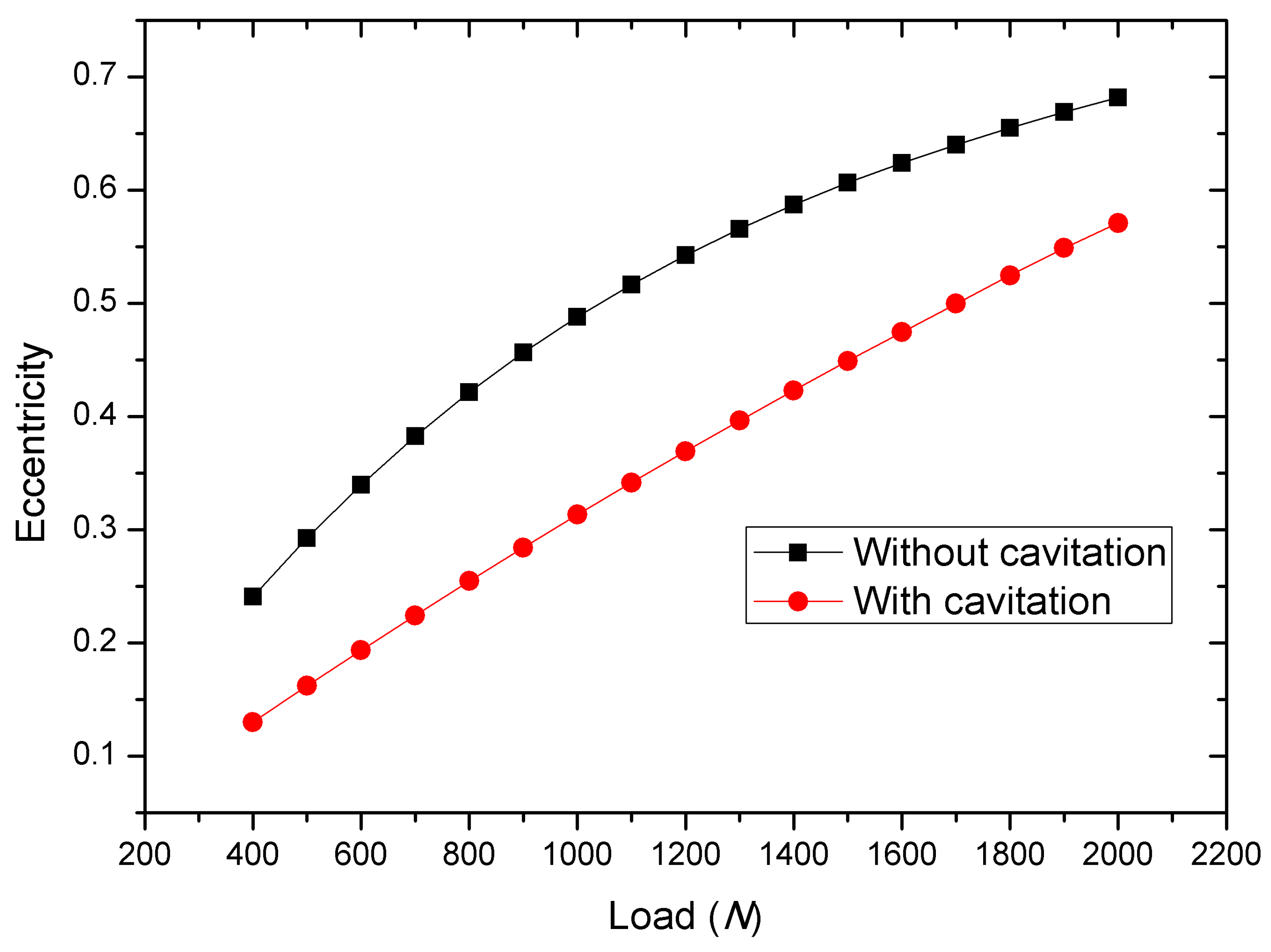
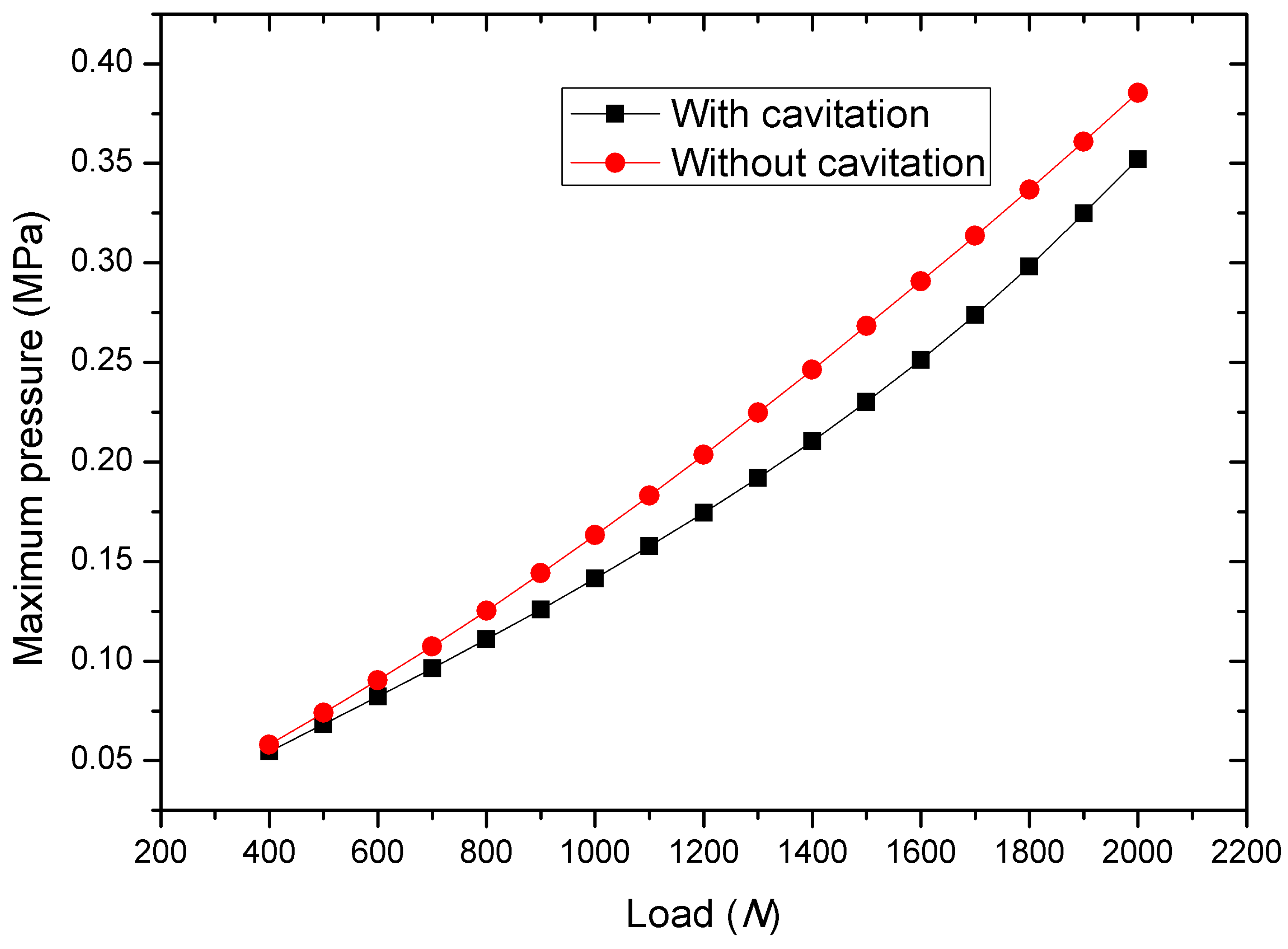
5.1. The Influence of Cavitation
5.2. The Mixed Lubrication State
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wei, B.; Jiao, Y.; Wu, X. Numerical Calculation of Fluid Film Force on Journal Bearings Based on a Biconjugate Gradient-Stabilized Algorithm. J. Tribol. Trans. ASME 2022, 144, 114502. [Google Scholar] [CrossRef]
- Xie, Z.; Shen, N.; Zhu, W.; Tian, W.; Hao, L. Theoretical and experimental investigation on the influences of misalignment on the lubrication performances and lubrication regimes transition of water lubricated bearing. Mech. Syst. Signal Process. 2021, 149, 107211. [Google Scholar] [CrossRef]
- Xu, W.; Tian, Y.; Li, K.; Zhang, M.; Yang, J. Reynolds boundary condition realization in journal bearings: Location of oil film rupture boundary with layering-sliding mesh method. Tribol. Int. 2022, 165, 107330. [Google Scholar] [CrossRef]
- Jakobsson, B. The Finite Journal Bearing, Considering Vaporization. Trans. Chalmers Univ. Technol. 1957, 190, 117. [Google Scholar]
- Olsson, K.O. Cavitation in dynamically loaded bearings. In Akademiförlaget-Gumpert; Scandinavian University Books: Oslo, Norway, 1965. [Google Scholar]
- Hansen, E.; Kacan, A.; Frohnapfel, B.; Codrignani, A. An EHL Extension of the Unsteady FBNS Algorithm. Tribol. Lett. 2022, 70, 80. [Google Scholar] [CrossRef]
- Narwat, K.; Kumar, V.; Singh, S.J.; Kumar, A.; Sharma, S.C. Performance of rough surface hydrodynamic circular and multi-lobe journal bearings in turbulent regimes. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2023, 237, 860–880. [Google Scholar] [CrossRef]
- Huang, B.; Qiu, S.C.; Li, X.B.; Wu, Q.; Wang, G.Y. A review of transient flow structure and unsteady mechanism of cavitating flow. J. Hydrodyn. 2019, 31, 429–444. [Google Scholar] [CrossRef]
- Floberg, L. Sub-Cavity Pressures and Number of Oil Streamers in Cavitation Regions with Special Reference to the Infinite Journal Bearing; Royal Swedish Academy of Engineering Sciences: Stockholm, Sweden, 1968. [Google Scholar]
- Pan, C.H.T. Dynamic Analysis of Rupture in Thin Fluid Films. I—A Noninertial Theory. J. Lubr. Technol. 1983, 105, 96–104. [Google Scholar] [CrossRef]
- Brewe, D.E. Theoretical Modeling of the Vapor Cavitation in Dynamically Loaded Journal Bearings. J. Tribol. 1986, 108, 628–637. [Google Scholar] [CrossRef] [Green Version]
- Kumar, A.; Booker, J.F. A Mass and Energy Conserving Finite Element Lubrication Algorithm. J. Tribol. 1994, 116, 667–671. [Google Scholar] [CrossRef]
- Elrod, H.G. A Cavitation Algorithm. J. Lubr. Technol. 1981, 103, 350–354. [Google Scholar] [CrossRef]
- Vijayaraghavan, D.; Keith, T. Development and evaluation of a cavitation algorithm. Tribol. Trans. 1989, 32, 225–233. [Google Scholar] [CrossRef]
- Vincent, B.; Maspeyrot, P.; Frene, J. Cavitation in dynamically loaded journal bearings using mobility method. Wear 1996, 193, 155–162. [Google Scholar] [CrossRef]
- Tucker, P.G.; Keogh, P.S. A generalized computational fluid dynamics approach for journal bearing performance prediction. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 1995, 209, 99–108. [Google Scholar] [CrossRef]
- Yan-Ming, C.; Mongis, J. Cavitation wear in plain bearing: Case study. Mec. Ind. 2005, 6, 195–201. [Google Scholar] [CrossRef]
- Flores, P.; Ambrósio, J.; Claro, J.C.P.; Lankarani, H.M.; Koshy, C.S. Lubricated revolute joints in rigid multibody systems. Nonlinear Dyn. 2009, 56, 277–295. [Google Scholar] [CrossRef] [Green Version]
- Song, Y.; Gu, C.w.; Ren, X. Development and validation of a gaseous cavitation model for hydrodynamic lubrication. PRoceedings Inst. Mech. Eng. Part-J. Eng. Tribol. 2015, 229, 1227–1238. [Google Scholar] [CrossRef]
- Shahmohamadi, H.; Rahmani, R.; Rahnejat, H.; Garner, C.P.; Dowson, D. Big End Bearing Losses with Thermal Cavitation Flow Under Cylinder Deactivation. Tribol. Lett. 2015, 57, 2. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.; Zeng, Q.; Lu, C.; Liang, P. A numerical analysis and experimental investigation of three oil grooves sleeve bearing performance. Ind. Lubr. Tribol. 2019, 71, 181–187. [Google Scholar] [CrossRef]
- Jiang, S.; Ji, H.; Feng, D.; Li, Q.; Wu, S.; Chen, Z. Analysis and optimisation of grooved parallel slider bearings with cavitation. Meccanica 2020, 55, 1379–1391. [Google Scholar] [CrossRef]
- Zhang, C.; Cheng, H.S. Transient Non-Newtonian Thermohydrodynamic Mixed Lubrication of Dynamically Loaded Journal Bearings. J. Tribol. 1999, 122, 156–161. [Google Scholar] [CrossRef]
- Xu, W.; Zhao, S.; Xu, Y.; Li, K. Reynolds Model versus JFO Theory in Steadily Loaded Journal Bearings. Lubricants 2021, 9, 111. [Google Scholar] [CrossRef]
- Ding, A.; Ren, X.; Li, X.; Gu, C. A new gaseous cavitation model in a tilting-pad journal bearing. Sci. Prog. 2021, 104, 368504211029431. [Google Scholar] [CrossRef] [PubMed]
- Sobhi, S.; Nabhani, M.; Zarbane, K.; El Khlifi, M. Cavitation in oscillatory porous squeeze film: A numerical approach. Ind. Lubr. Tribol. 2022, 74, 636–644. [Google Scholar] [CrossRef]
- Sobhi, S.; El Khlifi, M.; Nabhani, M. Effects of both cavitation and non-Newtonian behavior on the performance of oscillatory anisotropic poroelastic squeeze film. Ind. Lubr. Tribol. 2023, 75, 145–156. [Google Scholar] [CrossRef]
- Tieu, A.K.; Freund, N.O. A high performance journal bearing with controlled elastic deflection. J. Tribol.-Trans. Asme 1995, 117, 702–708. [Google Scholar] [CrossRef]
- Liu, X.; He, T.; Yan, Y.; Meng, L.; Dong, J.; Guo, Y.; Zhou, P. Effects of axial offset and deflection on load-bearing characteristics of the permanent magnet bearing. Eng. Fail. Anal. 2023, 146, 107123. [Google Scholar] [CrossRef]
- He, T.; Zou, D.; Lu, X.; Guo, Y.; Wang, Z.; Li, W. Mixed-lubrication analysis of marine stern tube bearing considering bending deformation of stern shaft and cavitation. Tribol. Int. 2014, 73, 108–116. [Google Scholar] [CrossRef]
- Félix Quiñonez, A.; Morales-Espejel, G.E. Surface roughness effects in hydrodynamic bearings. Tribol. Int. 2016, 98, 212–219. [Google Scholar] [CrossRef]
- Mishra, P.C.; Rahnejat, H. Tribology of big-end bearings. In Tribology and Dynamics of Engine and Powertrain; Rahnejat, H., Ed.; Woodhead Publishing: Sawston, UK, 2010; pp. 635–659. [Google Scholar] [CrossRef]
- Allmaier, H.; Priestner, C.; Six, C.; Priebsch, H.H.; Forstner, C.; Novotny-Farkas, F. Predicting friction reliably and accurately in journal bearings—A systematic validation of simulation results with experimental measurements. Tribol. Int. 2011, 44, 1151–1160. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Tripp, J.H. The Contact of Two Nominally Flat Rough Surfaces. Proc. Inst. Mech. Eng. 1970, 185, 625–633. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, H.S. An Average Flow Model for Determining Effects of Three-Dimensional Roughness on Partial Hydrodynamic Lubrication. J. Lubr. Technol. 1978, 100, 12–17. [Google Scholar] [CrossRef]
- Wu, C.; Zheng, L. An Average Reynolds Equation for Partial Film Lubrication With a Contact Factor. J. Tribol. 1989, 111, 188–191. [Google Scholar] [CrossRef]
- Hong, S. Determining the Value of Pressure in the Cavitation Zone for Steady-state Journal Bearing. Mech. Eng. 2007, 5, 88–90. [Google Scholar]
- Feng, Z.; Ding, Q.; Cai, Y.; Sun, W. Experimental Investigation of the Dynamic Response of a Sliding Bearing System under Different Oil Pressure Levels. Appl. Sci. 2022, 12, 9759. [Google Scholar] [CrossRef]
- Lloyd, T.; Horsnell, R.; McCallion, H. Paper 8: An Investigation into the Performance of Dynamically Loaded Journal Bearings: Design Study. Proc. Inst. Mech. Eng. Conf. Proc. 1966, 181, 28–34. [Google Scholar]
- Dowson, D.; Taylor, C.M. Cavitation in Bearings. Annu. Rev. Fluid Mech. 1979, 11, 35–65. [Google Scholar] [CrossRef]
- Shen, C.; Khonsari, M.M. On the Magnitude of Cavitation Pressure of Steady-State Lubrication. Tribol. Lett. 2013, 51, 153–160. [Google Scholar] [CrossRef]




| Radius (mm) | Radius Clearance (mm) | Length–Diameter Ratio | Cavitation Pressure (kPa, Gage) |
|---|---|---|---|
| 50 | 0.1455 | 1.333 | −72 |
| Angular Velocity (rad/s) | Dynamic Viscosity (Pa·s) | Ambient Pressure (kPa, Gage) | Density (kg/m) |
| 48.1 | 0.0127 | 0 | 950 |
| Inner radius of bearing (mm) | 100 | Outer radius of bearing (mm) | 160 |
| Radius clearance (mm) | 0.3 | Width of Bearing(mm) | 240 |
| Rotational speed (rpm) | 20–60 | Lubricating oil viscosity (Pa·s) | 0.082 |
| Roughness of bushing ( m) | 8 | Roughness of journal ( m) | 2 |
| Elastic modulus of bushing (GPa) | 100 | Elastic modulus of journal (GPa) | 210 |
| Poisson ratio of bushing | 0.29 | Poisson ratio of journal | 0.3 |
| Load of bearing (N) | 38,000 | Initial Bearing-journal contact friction coefficient | 0.1 |
| Speed (rpm) | Eccentricity | Film Load (N) | Contact Load (N) | Friction Force (N) | Friction Coefficient |
|---|---|---|---|---|---|
| 20 | 0.95360 | 19,066.50 | 18,943.96 | 1970.07 | 0.05183 |
| 30 | 0.94960 | 26,172.52 | 11,639.29 | 1261.44 | 0.03336 |
| 40 | 0.94480 | 31,588.07 | 6231.27 | 729.33 | 0.01928 |
| 50 | 0.93930 | 35,510.86 | 2815.49 | 388.55 | 0.01014 |
| 60 | 0.93175 | 37,494.50 | 831.29 | 188.50 | 0.00492 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, T.; Zhou, Y.; Liu, Y.; Xia, Y. Influence of Cavitation and Shaft Deformation in the Analysis of Lubrication of the Stern Bearing. Appl. Sci. 2023, 13, 9033. https://doi.org/10.3390/app13159033
He T, Zhou Y, Liu Y, Xia Y. Influence of Cavitation and Shaft Deformation in the Analysis of Lubrication of the Stern Bearing. Applied Sciences. 2023; 13(15):9033. https://doi.org/10.3390/app13159033
Chicago/Turabian StyleHe, Tao, Yingzhi Zhou, Yong Liu, and Yang Xia. 2023. "Influence of Cavitation and Shaft Deformation in the Analysis of Lubrication of the Stern Bearing" Applied Sciences 13, no. 15: 9033. https://doi.org/10.3390/app13159033
APA StyleHe, T., Zhou, Y., Liu, Y., & Xia, Y. (2023). Influence of Cavitation and Shaft Deformation in the Analysis of Lubrication of the Stern Bearing. Applied Sciences, 13(15), 9033. https://doi.org/10.3390/app13159033







