A Solution Procedure to Improve 3D Solid Finite Element Analysis with an Enrichment Scheme
Abstract
1. Introduction
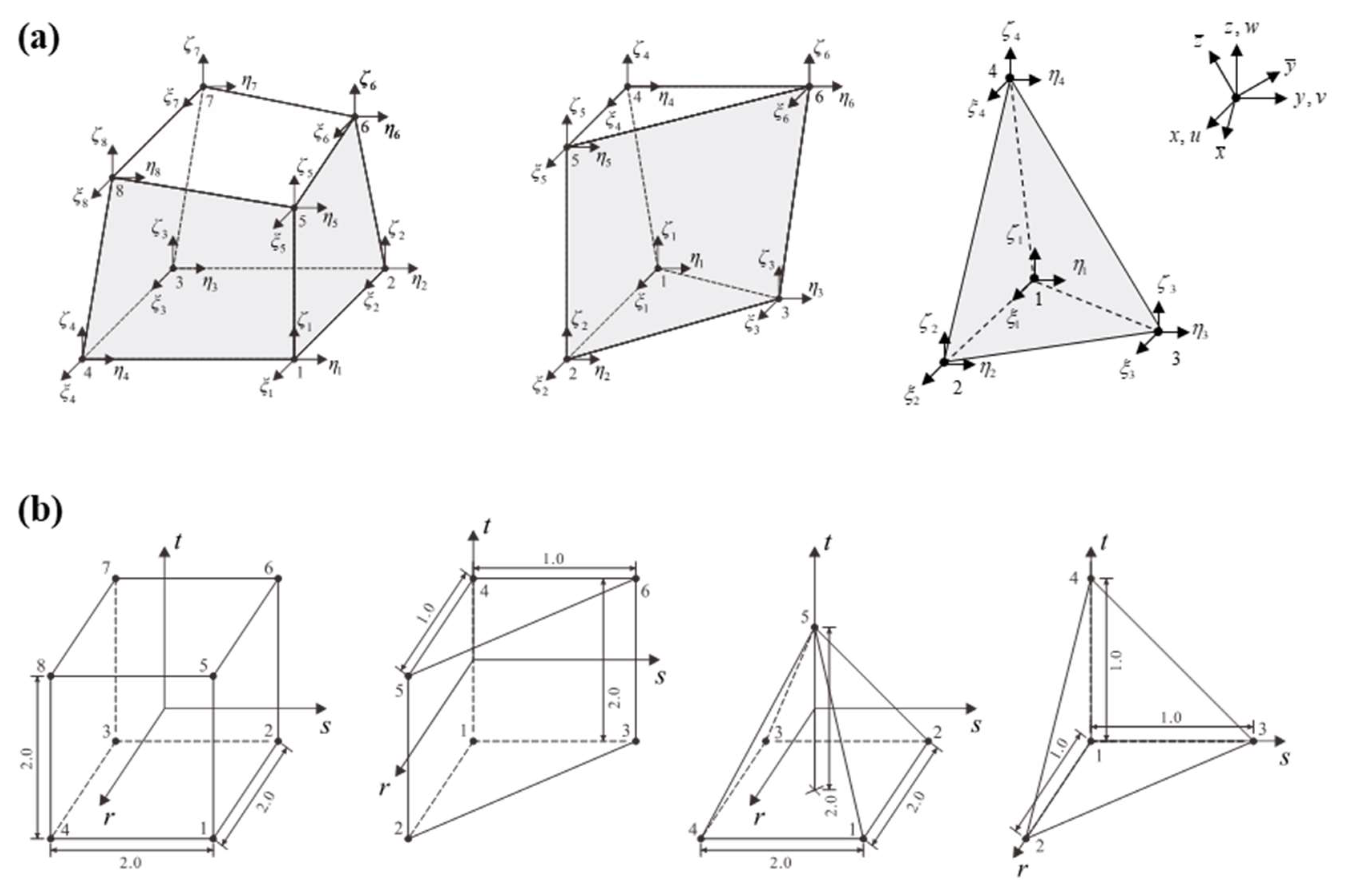
2. Finite Element Formulation Enriched by Cover Functions
3. Improving 3D Solid FE Solutions
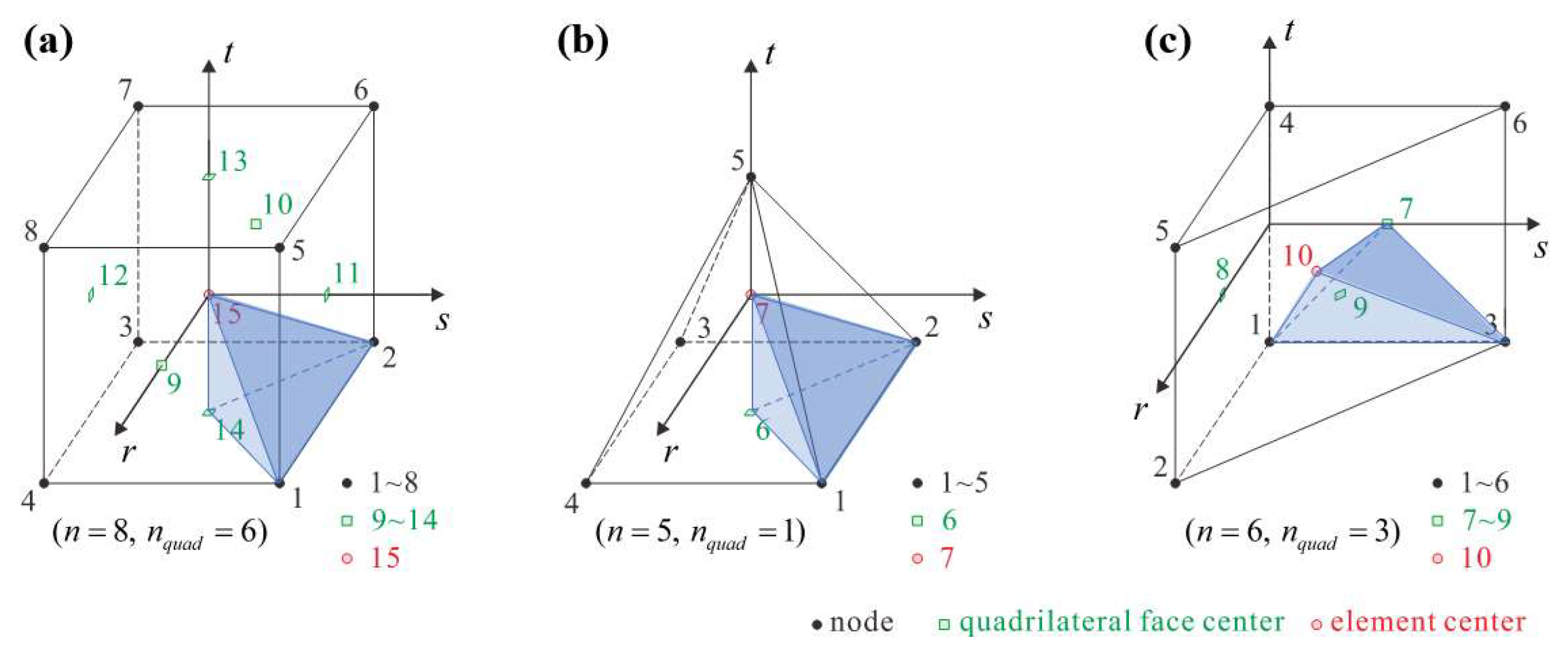
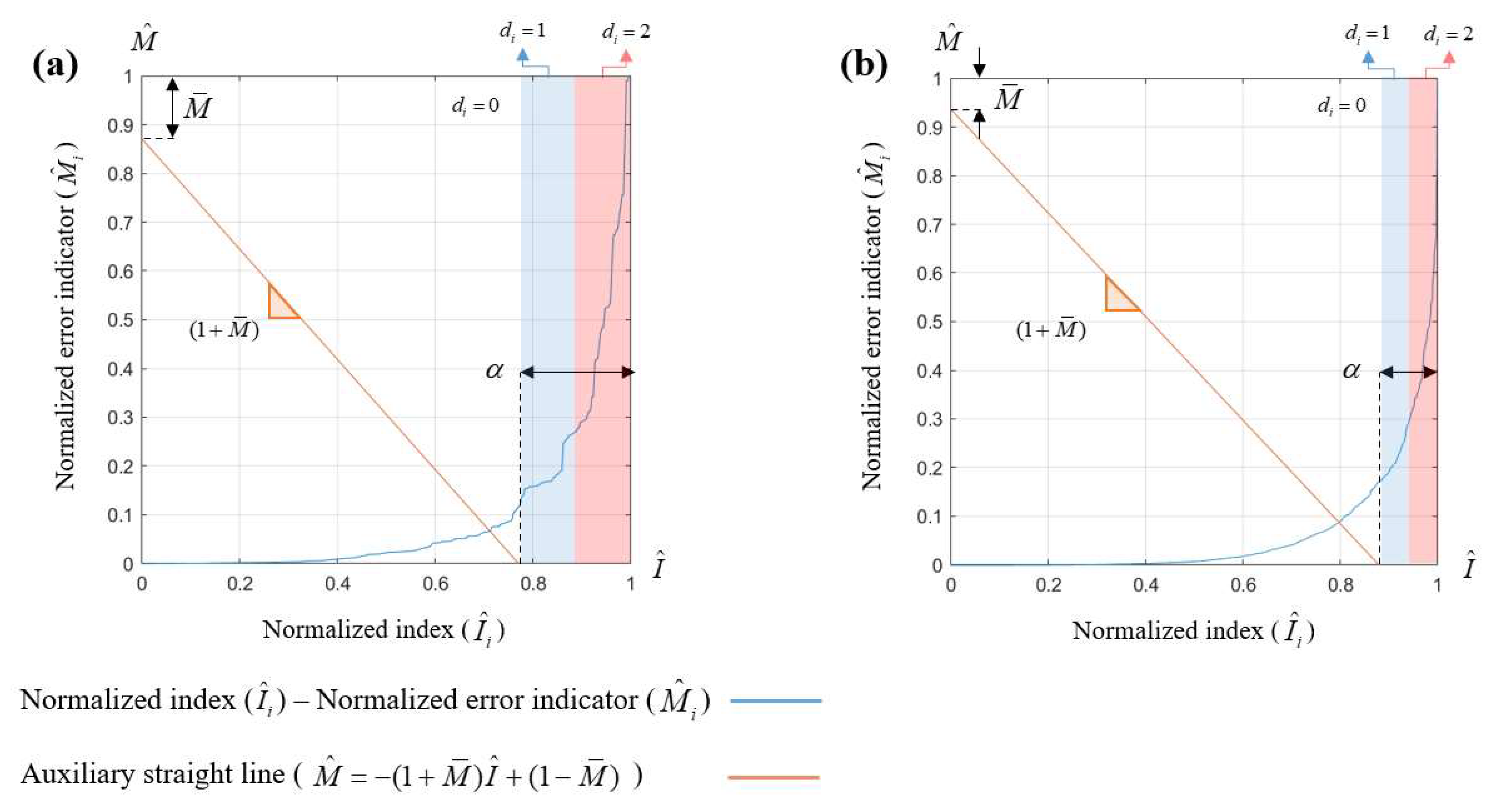
3.1. Error Indicator and Cover Function Selection Scheme
3.2. Cover Function Selection Scheme
4. Numerical Examples
- HEX8: standard 8-node hexahedral element
- PRI6: standard 6-node prismatic element
- PYR5: standard 5-node pyramidal element
- TET4: standard 4-node tetrahedral element
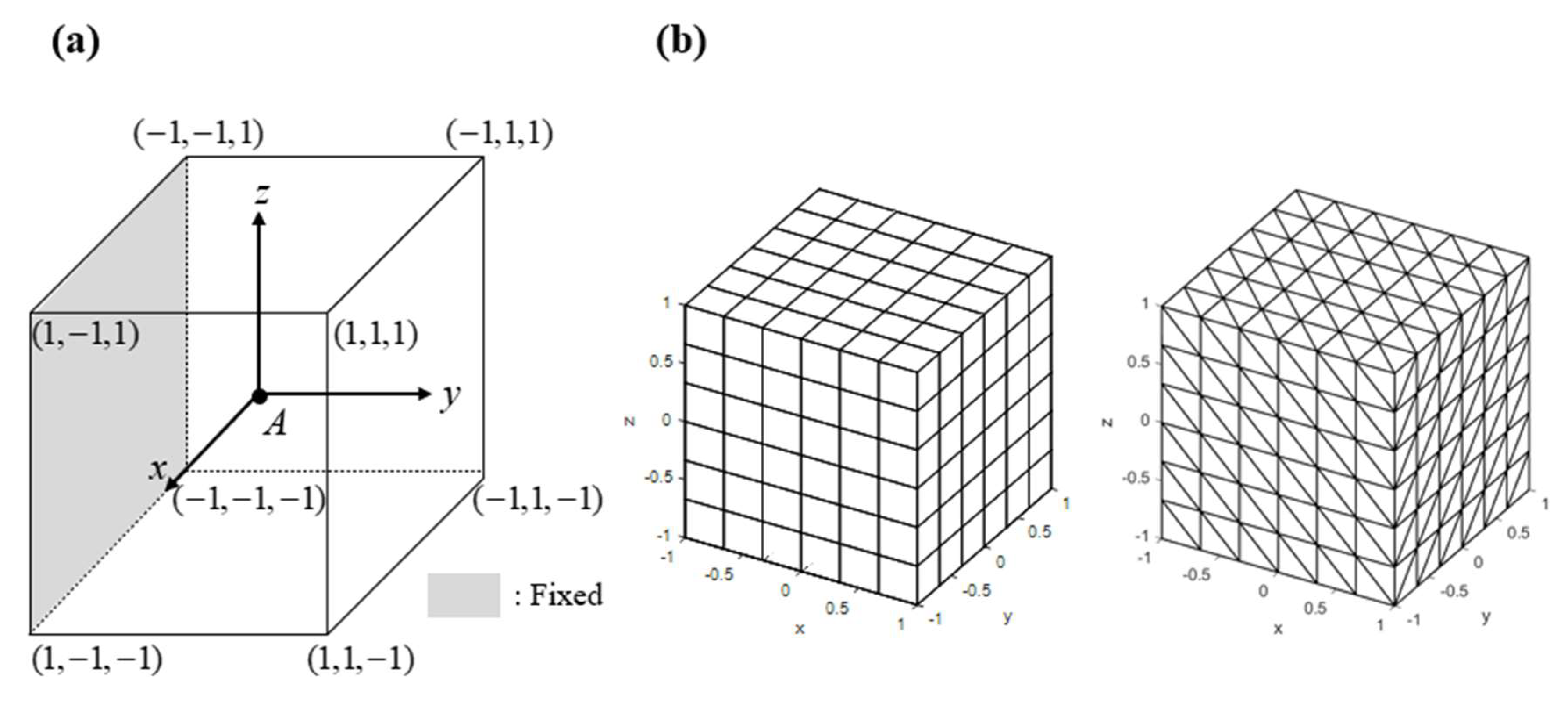
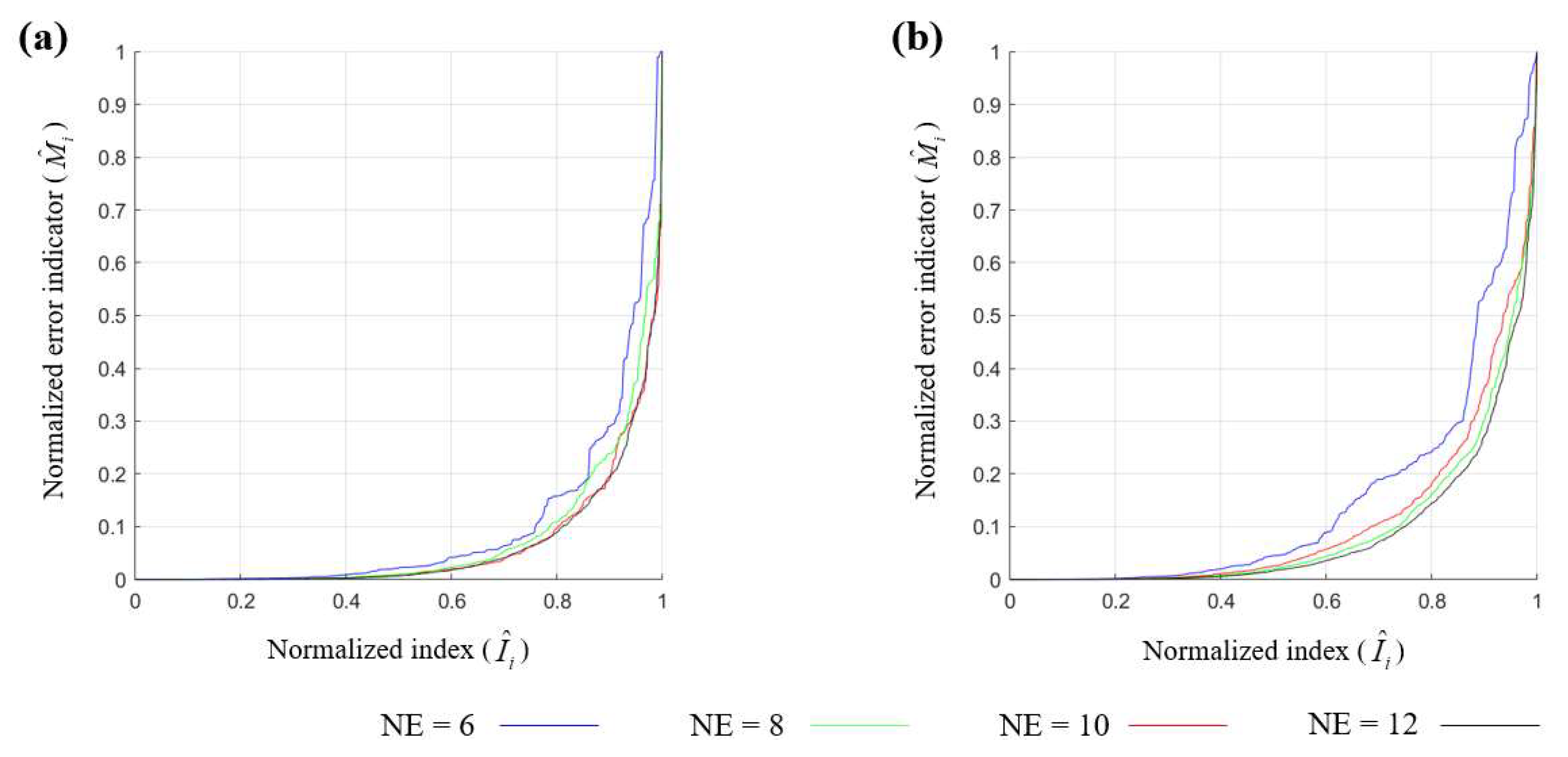
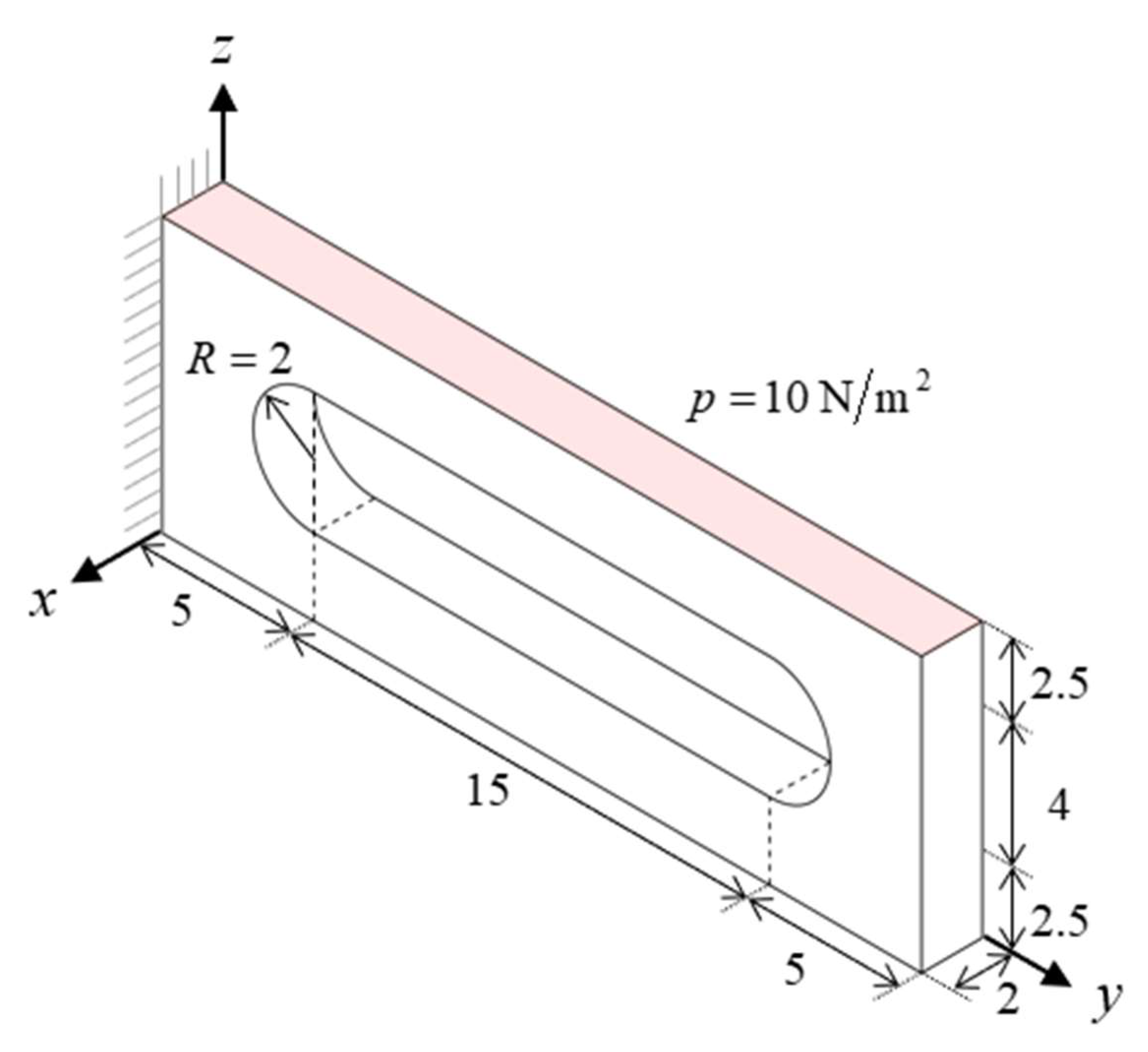
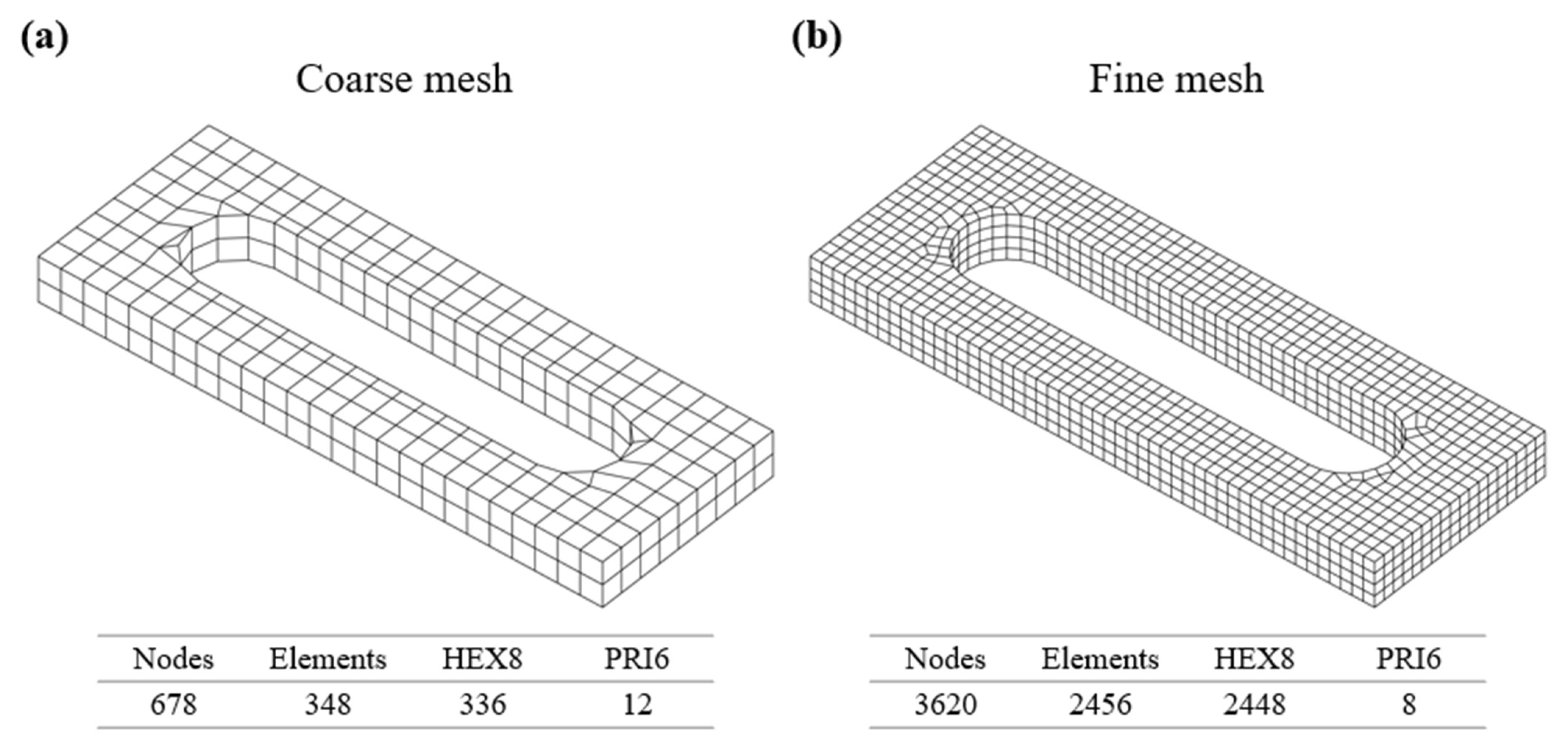
4.1. Ad Hoc Problem
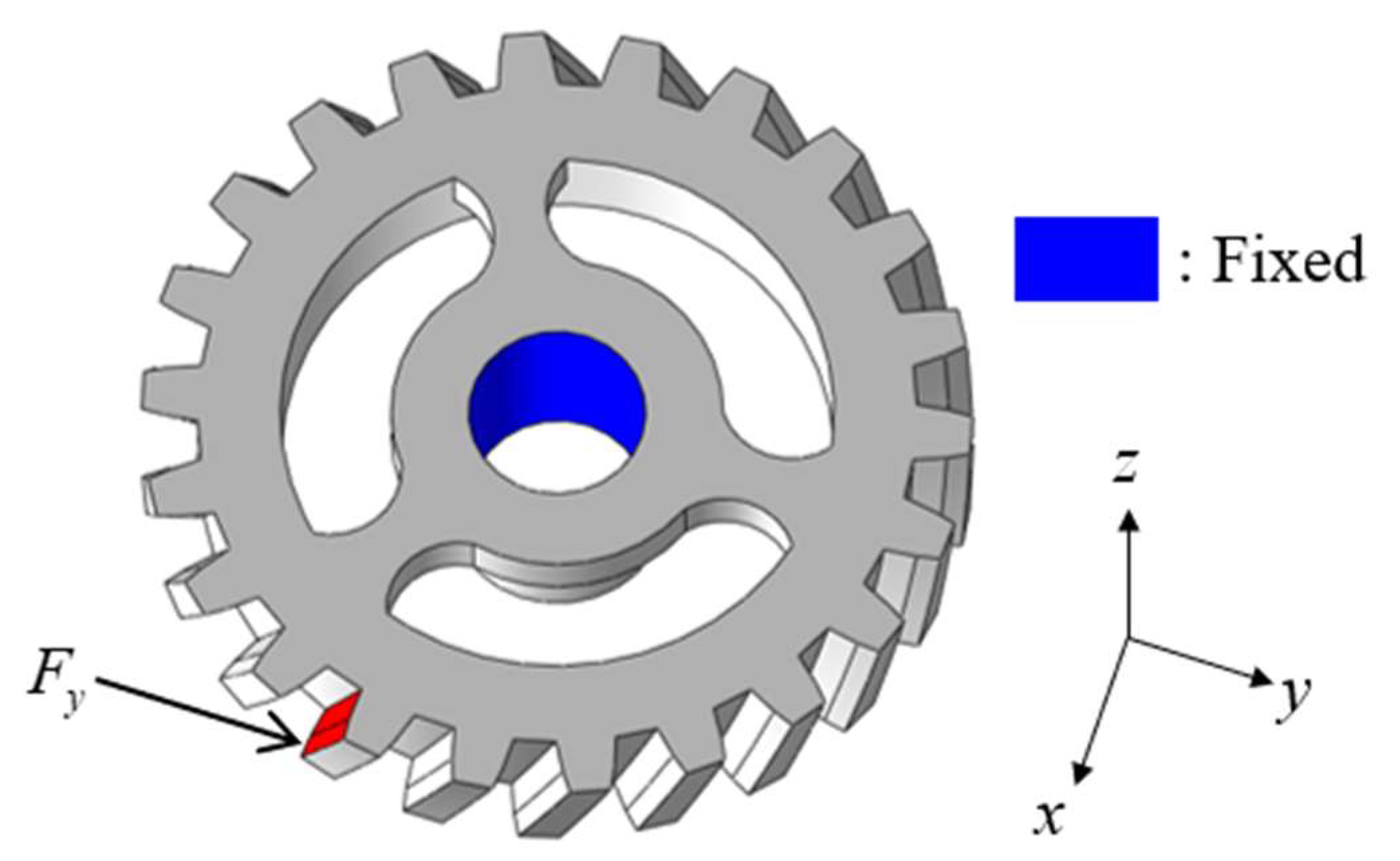
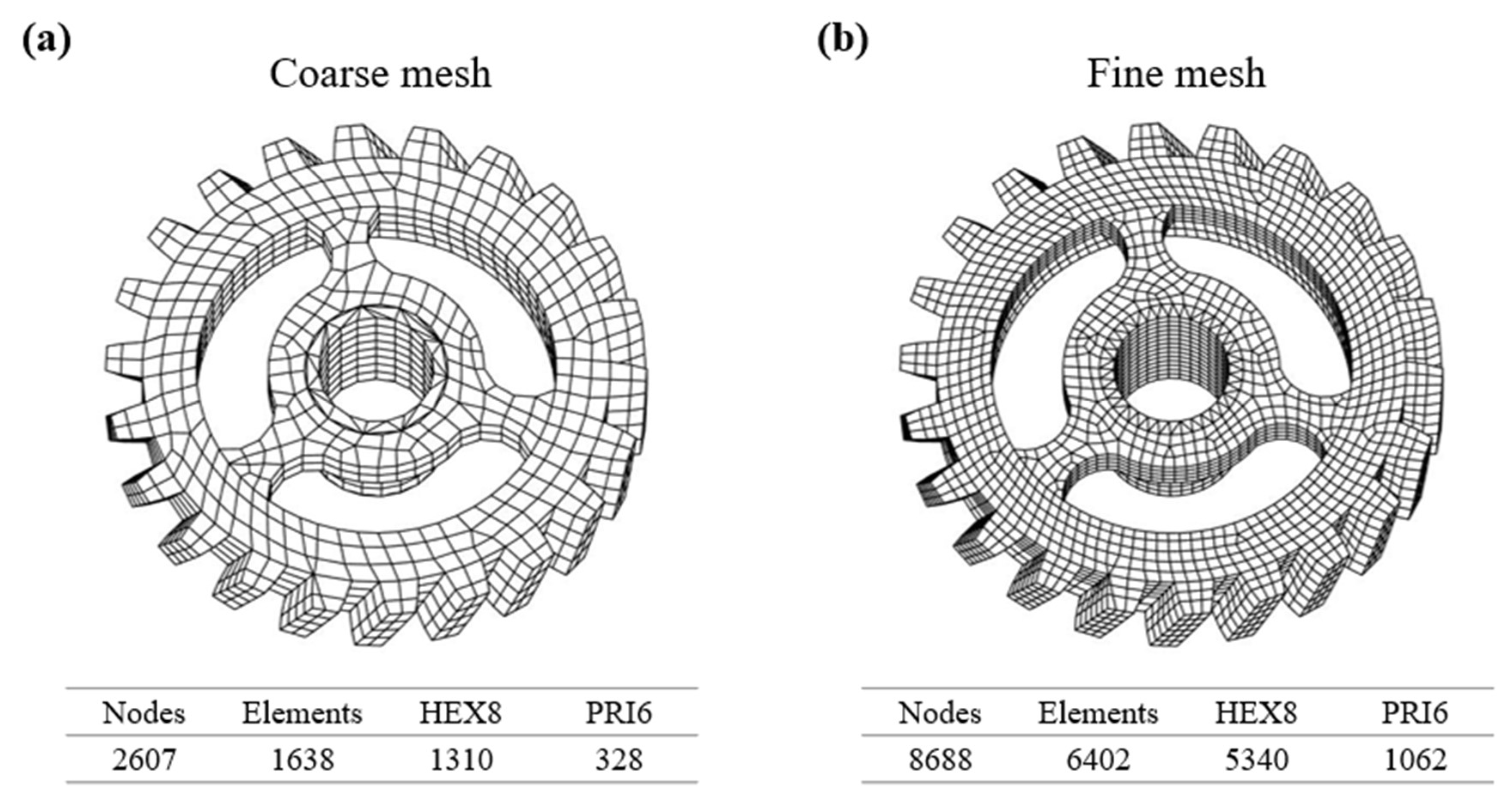
4.2. Gear Problem
4.3. Tool Jig Problem
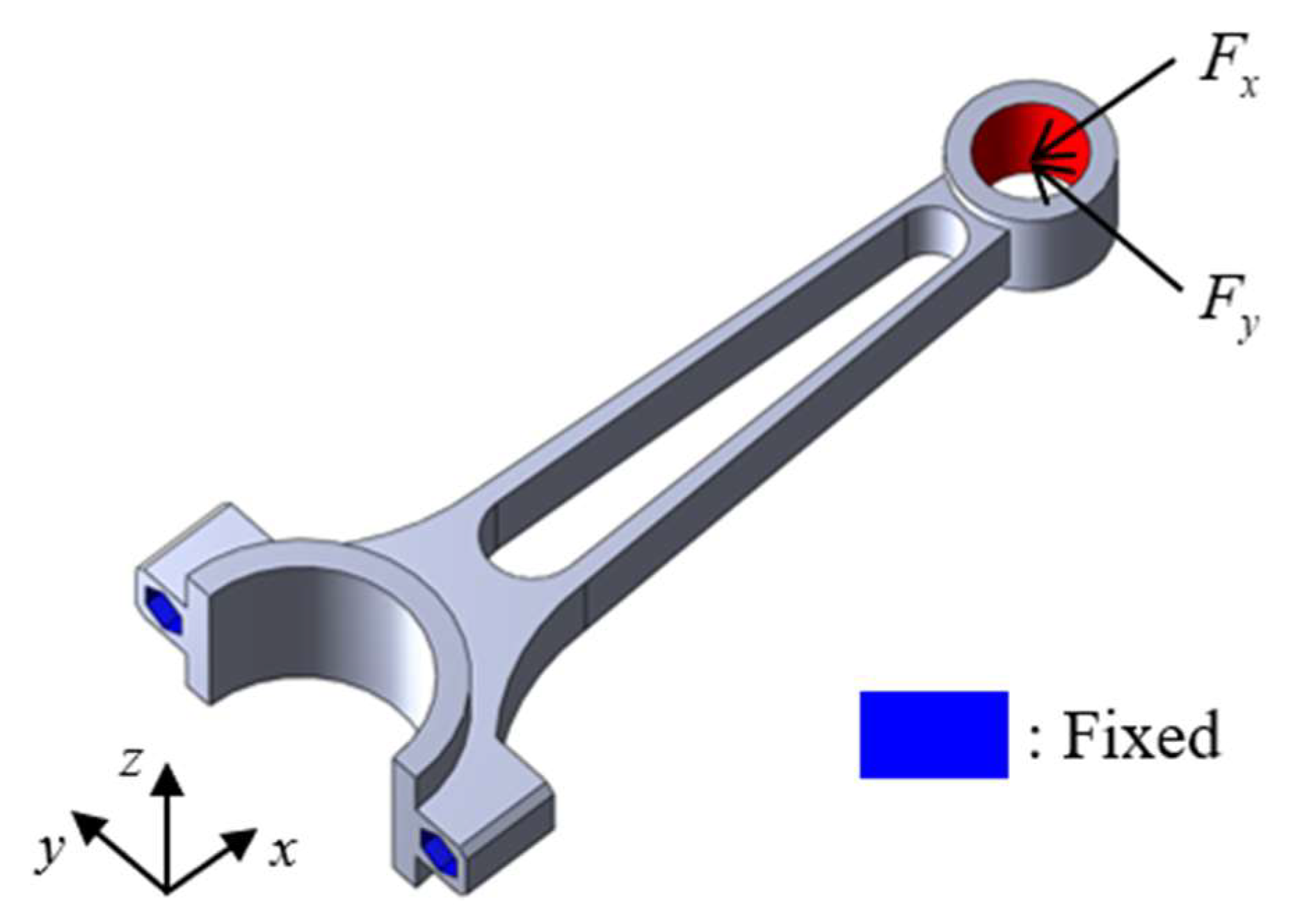
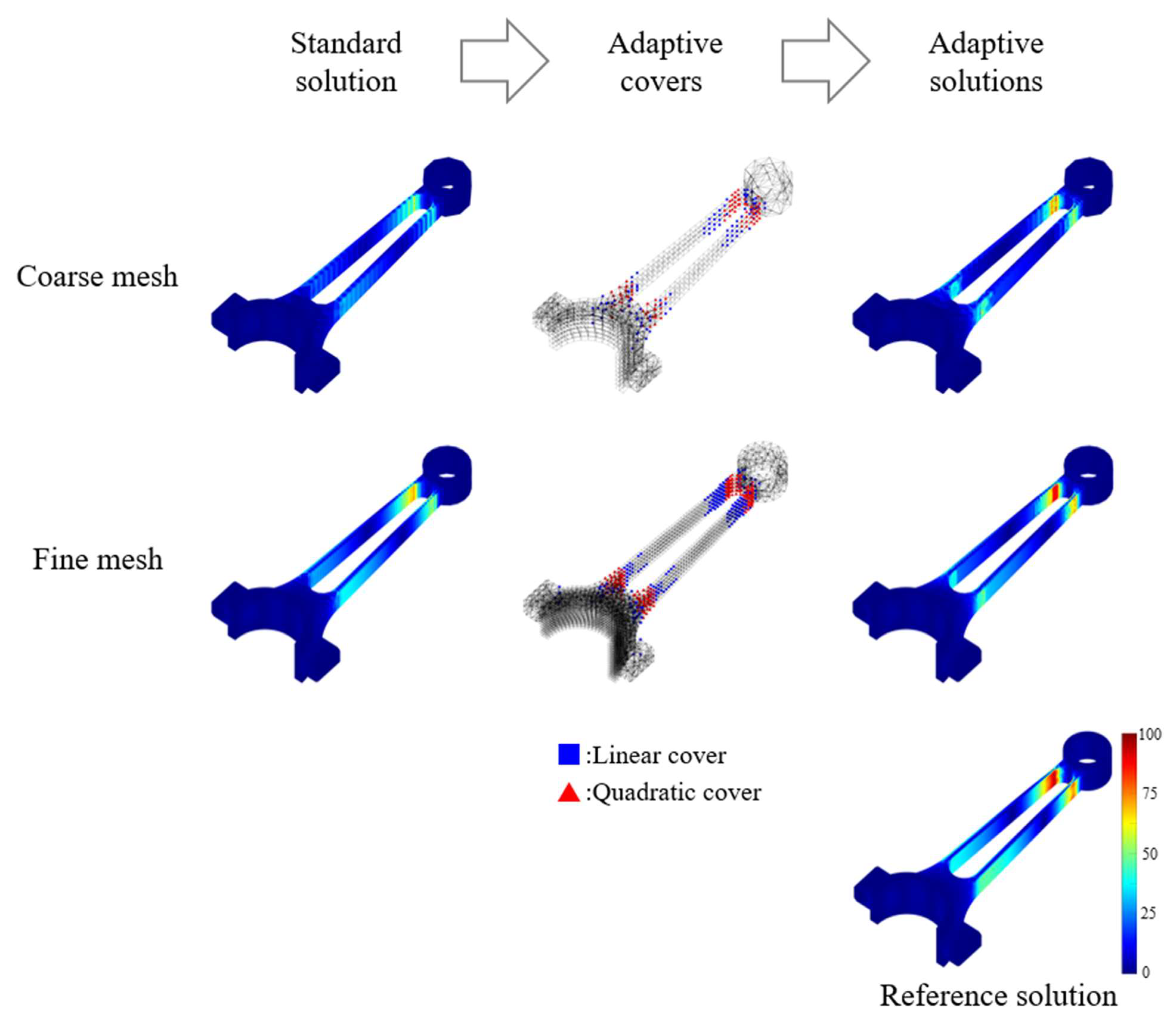
4.4. Connecting Rod Problem
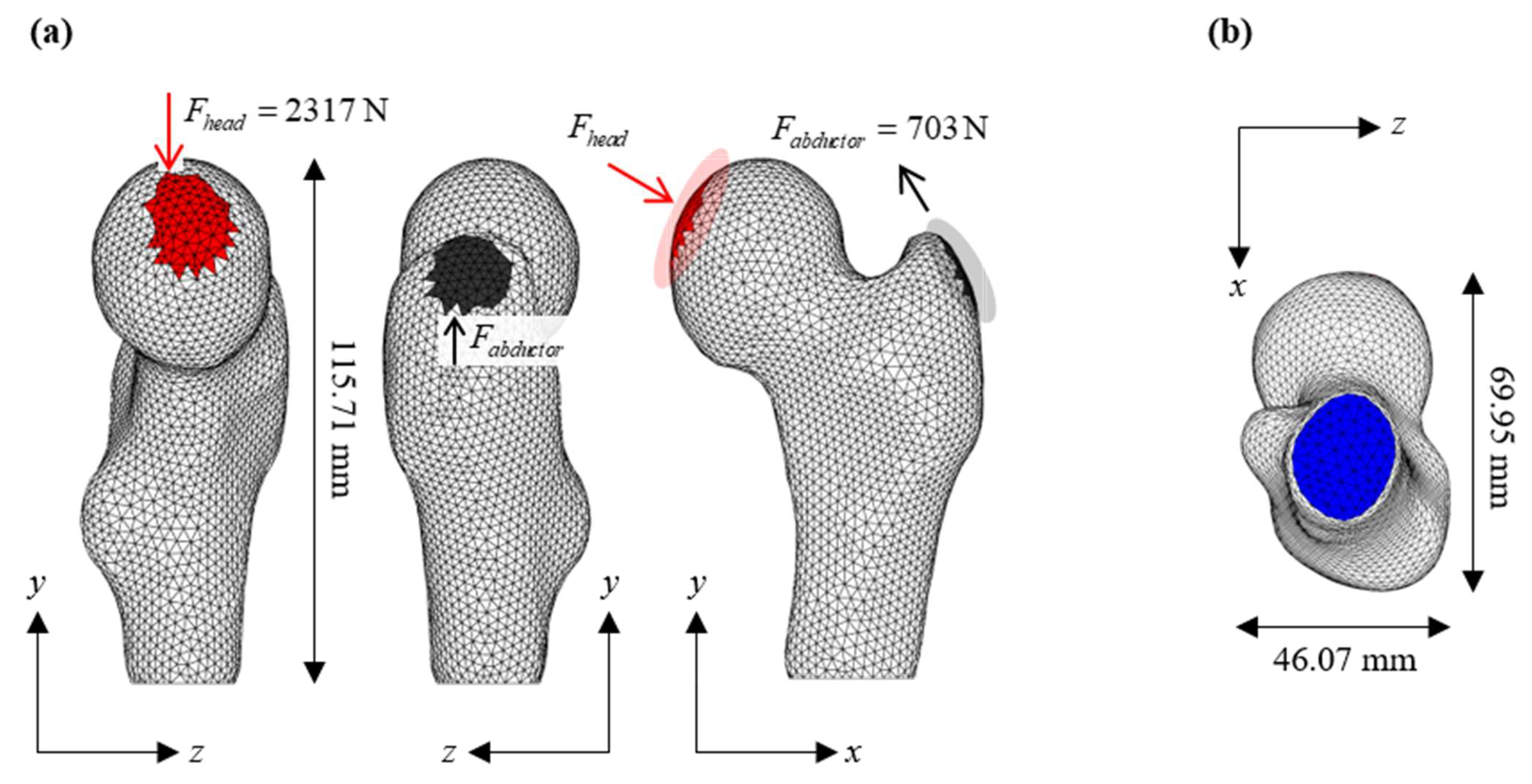
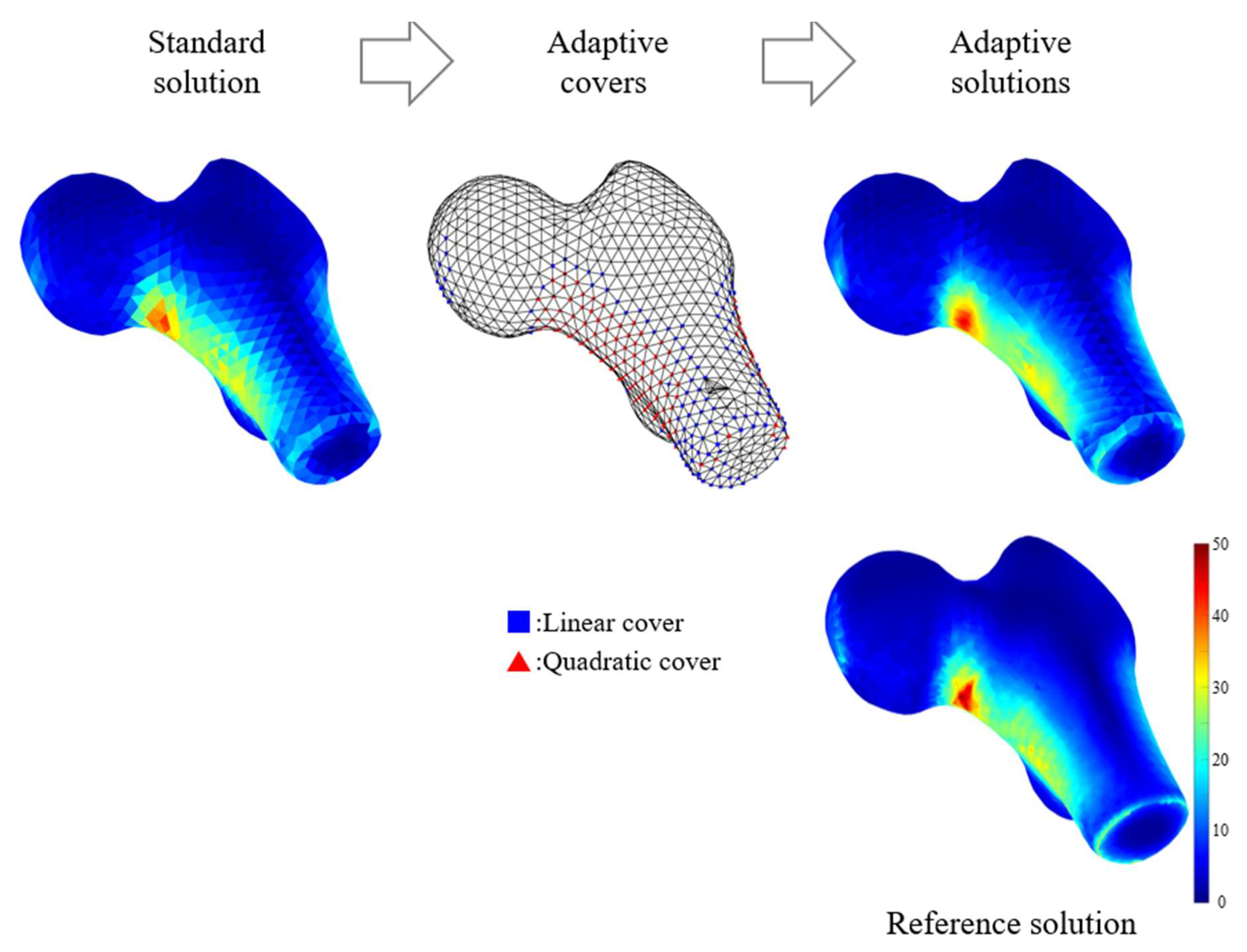
4.5. Proximal Femur Problem
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zienkiewicz, O.C. The Finite Element Method; McGraw-Hill: New York, NY, USA, 1991. [Google Scholar]
- Bathe, K.J. Finite Element Procedures; Prentice Hall: New York, NY, USA, 1996. [Google Scholar]
- Hughes, T.J.R. The Finite Element Method-Linear Static and Dynamic Finite Element Analysis; Dover Publications: New York, NY, USA, 2000. [Google Scholar]
- Cook, R.D. Concepts and Applications of Finite Element Analysis; John Wiley & Sons: New York, NY, USA, 2007. [Google Scholar]
- Babuška, I.; Melenk, J.M. The partition of unity method. Int. J. Numer. Methods Eng. 1997, 40, 727–758. [Google Scholar] [CrossRef]
- Liu, G.R. The smoothed finite element method (S-FEM): A framework for the design of numerical models for desired solutions. Front. Struct. Civ. Eng. 2019, 13, 456–477. [Google Scholar] [CrossRef]
- Fries, T.P.; Belytschko, T. The extended/generalized finite element method: An overview of the method and its applications. Int. J. Numer. Methods Eng. 2010, 84, 253–304. [Google Scholar] [CrossRef]
- Choi, H.G.; Lee, P.S. Towards improving the 2D-MITC4 element for analysis of plane stress and strain problems. Comput. Struct. 2023, 275, 106933. [Google Scholar] [CrossRef]
- Chen, J.S.; Wu, C.T.; Yoon, S.; You, Y. A stabilized conforming nodal integration for Galerkin mesh-free methods. Int. J. Numer. Methods Eng. 2001, 50, 435–466. [Google Scholar] [CrossRef]
- Jung, J.; Park, S.; Lee, C. A Posteriori Error Estimation via Mode-Based Finite Element Formulation Using Deep Learning. Struct. Eng. Mech. 2022, 2, 273–282. [Google Scholar]
- Ham, S.; Bathe, K.J. A finite element method enriched for wave propagation problems. Comput. Struct. 2012, 94, 1–12. [Google Scholar] [CrossRef]
- Hong, W.T.; Lee, P.S. Coupling flat-top partition of unity method and finite element method. Finite Elem. Anal. Des. 2013, 67, 43–55. [Google Scholar]
- Kim, J.; Bathe, K.J. The finite element method enriched by interpolation covers. Comput. Struct. 2013, 116, 35–49. [Google Scholar] [CrossRef]
- Lee, C.; Lee, P.S. A new strain smoothing method for triangular and tetrahedral finite elements. Comput. Methods Appl. Mech. Eng. 2018, 341, 939–955. [Google Scholar] [CrossRef]
- Lee, C.; Kim, S.; Lee, P.S. The strain-smoothed 4-node quadrilateral finite element. Comput. Methods Appl. Mech. Eng. 2021, 373, 113481. [Google Scholar] [CrossRef]
- Lee, C.; Park, J. A variational framework for the strain-smoothed element method. Comput. Math. Appl. 2021, 94, 76–93. [Google Scholar] [CrossRef]
- Lee, C.; Park, J. Preconditioning for Finite Element Methods with Strain Smoothing. Comput. Math. Appl. 2023, 130, 41–57. [Google Scholar] [CrossRef]
- Belytschko, T.; Black, T. Elastic crack growth in finite elements with minimal remeshing. Int. J. Numer. Methods Eng. 1999, 45, 602–620. [Google Scholar] [CrossRef]
- Kim, J.; Bathe, K.J. Towards a procedure to automatically improve finite element solutions by interpolation covers. Comput. Struct. 2014, 131, 81–97. [Google Scholar] [CrossRef]
- Tian, R.; Yagawa, G.; Terasaka, H. Linear dependence problems of partition of unity-based generalized FEMs. Comput. Methods Appl. Mech. Eng. 2006, 195, 4768–4782. [Google Scholar] [CrossRef]
- An, X.M.; Li, L.X.; Ma, G.W.; Zhang, H.H. Prediction of rank deficiency in partition of unity-based methods with plane triangular or quadrilateral meshes. Comput. Methods Appl. Mech. Eng. 2011, 200, 665–674. [Google Scholar] [CrossRef]
- An, X.M.; Zhao, Z.Y.; Zhang, H.H.; Li, L.X. Investigation of linear dependence problem of three-dimensional partition of unity-based finite element methods. Comput. Methods Appl. Mech. Eng. 2012, 233, 137–151. [Google Scholar] [CrossRef]
- Kim, S.; Lee, P.S. A new enriched 4-node 2D solid finite element free from the linear dependence problem. Comput. Struct. 2018, 202, 25–43. [Google Scholar] [CrossRef]
- Kim, S.; Lee, P.S. New enriched 3D solid finite elements: 8-node hexahedral, 6-node prismatic, and 5-node pyramidal elements. Comput. Struct. 2019, 216, 40–63. [Google Scholar] [CrossRef]
- Lee, C.; Kim, S. Towards improving finite element solutions automatically with enriched 2D solid elements. Struct. Eng. Mech. 2020, 76, 379–393. [Google Scholar]
- Jeon, H.M.; Lee, P.S.; Bathe, K.J. The MITC3 shell finite element enriched by interpolation covers. Comput. Struct. 2014, 134, 128–142. [Google Scholar] [CrossRef]
- Jun, H.; Yoon, K.; Lee, P.S.; Bathe, K.J. The MITC3+ shell element enriched in membrane displacements by interpolation covers. Comput. Methods Appl. Mech. Eng. 2018, 337, 458–480. [Google Scholar] [CrossRef]
- Lee, Y.; Lee, P.S.; Bathe, K.J. The MITC3+ shell element and its performance. Comput. Struct. 2014, 138, 12–23. [Google Scholar] [CrossRef]
- Ko, Y.; Lee, Y.; Lee, P.S.; Bathe, K.J. Performance of the MITC3+ and MITC4+ shell elements in widely-used benchmark problems. Comput. Struct. 2017, 193, 187–206. [Google Scholar] [CrossRef]
- Lee, C.; Lee, P.S. The strain-smoothed MITC3+ shell finite element. Comput. Struct. 2019, 223, 106096. [Google Scholar] [CrossRef]
- Jun, H.M.; Paul, M.; Kim, S. Benchmark tests of MITC triangular shell elements. Struct. Eng. Mech. 2018, 68, 17–38. [Google Scholar]
- Lee, S.; Bathe, K.J. An enhancement of overlapping finite elements. Comput. Struct. 2022, 260, 106704. [Google Scholar] [CrossRef]
- Lee, C.; Lee, D.-H.; Lee, P.-S. The strain-smoothed MITC3+ shell element in nonlinear analysis. Comput. Struct. 2022, 266, 106768. [Google Scholar] [CrossRef]
- Lee, C.; Moon, M.; Park, J. A gradient smoothing method and its multiscale variant for flows in heterogeneous porous media. Comput. Methods Appl. Mech. Eng. 2022, 395, 1–25. [Google Scholar] [CrossRef]
- Kim, W.; Choi, H.G.; Kwon, S. Higher-order accurate explicit time schemes with improved dissipation properties. Int. J. Struct. Stab. Dyn. 2023, 2350166. [Google Scholar] [CrossRef]
- Nicoletti, V.; Gara, F. Modelling strategies for the updating of infilled RC building FEMs considering the construction phases. Buildings. 2023, 13, 598. [Google Scholar] [CrossRef]
- Kim, S.; Lee, C.H. Hydrodynamic analysis of floating structures with baffled ARTs. Struct. Eng. and Mech. 2018, 68, 1–15. [Google Scholar]
- Kim, S.; Oh, M.H. Strategy to improve edge-based smoothed finite element solutions using enriched 2D solid finite elements. Appl. Sci. 2021, 11, 3476. [Google Scholar]
- Boo, S.H.; Lee, P.S. A dynamic condensation method using algebraic substructuring. Int. J. Numer. Methods Eng. 2017, 109, 1701–1720. [Google Scholar] [CrossRef]
- Boo, S.H.; Kim, J.H.; Lee, P.S. Towards improving the enhanced Craig-Bampton method. Comput. Struct. 2018, 196, 63–75. [Google Scholar] [CrossRef]
- Hyun, C.; Boo, S.H.; Lee, P.S. Improving the computational efficiency of the enhanced AMLS method. Comput. Struct. 2020, 228, 106158. [Google Scholar] [CrossRef]


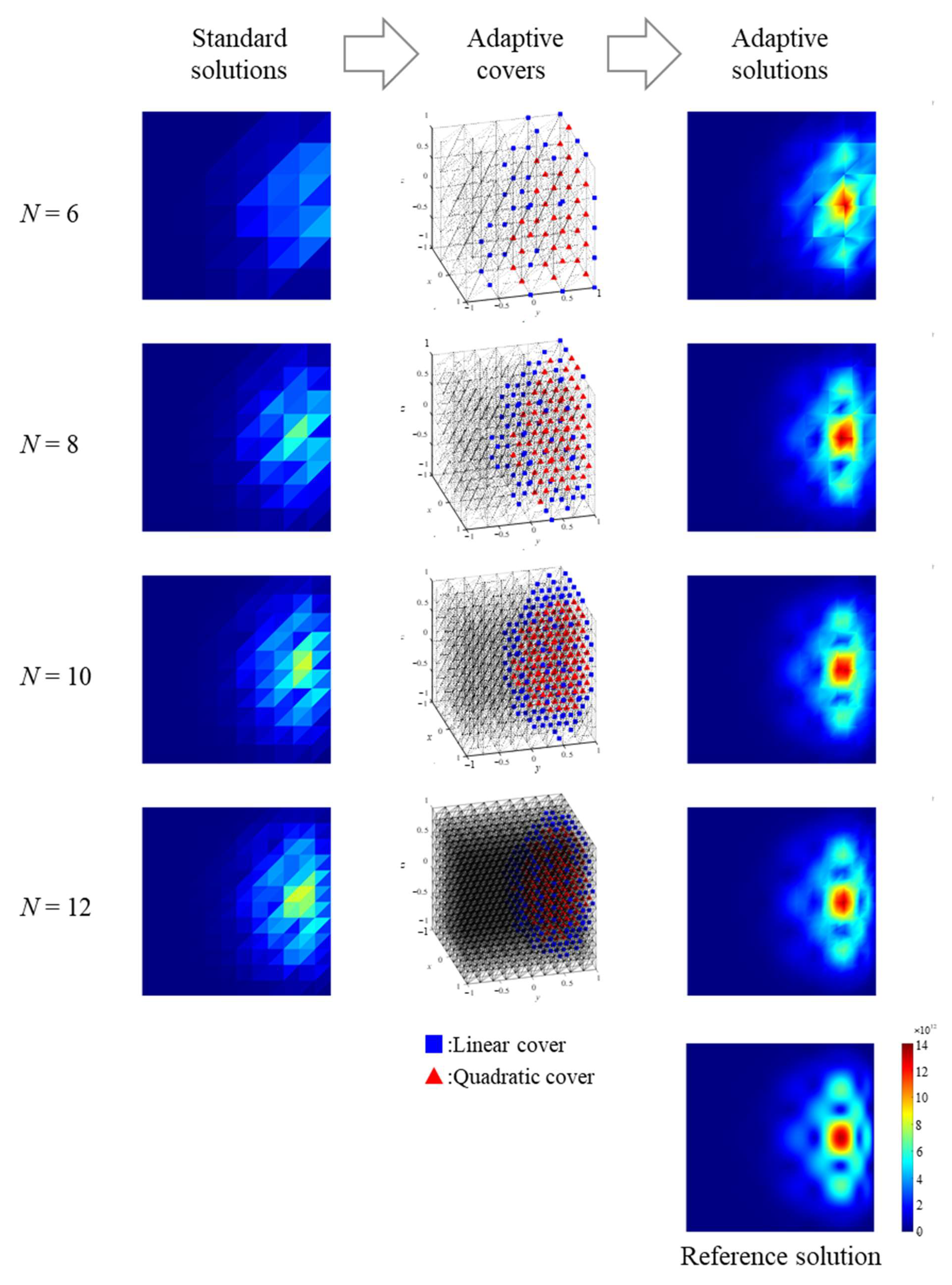
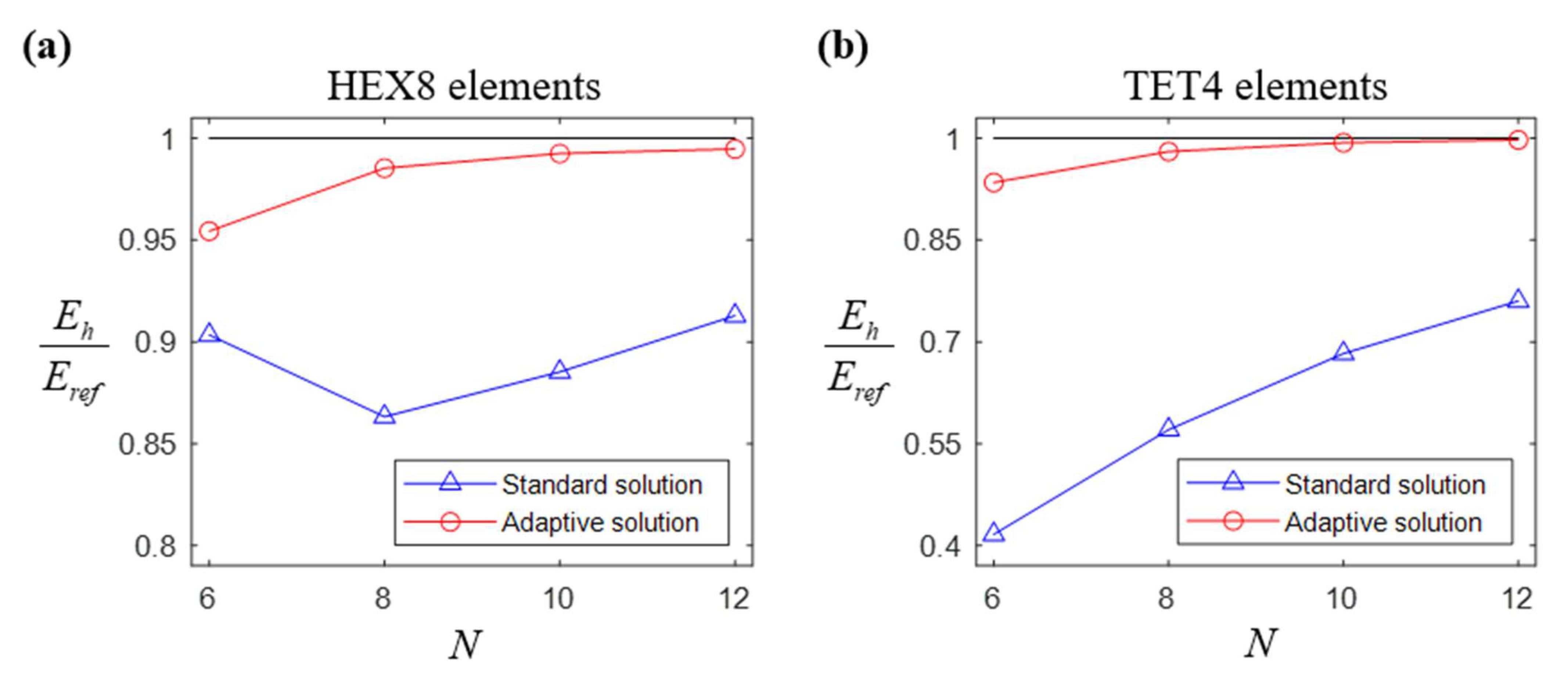




| Element | NE | Energy | von Mises Stress | ||
|---|---|---|---|---|---|
| Standard Solution | Adaptive Solution | Standard Solution | Adaptive Solution | ||
| HEX8 | 6 | 9.66% | 4.56% | 60.48% | 19.39% |
| 8 | 13.68% | 1.46% | 16.21% | 12.10% | |
| 10 | 11.48% | 0.75% | 8.03% | 5.57% | |
| 12 | 8.72% | 0.53% | 5.05% | 4.03% | |
| TET4 | 6 | 58.36% | 6.51% | 46.86% | 13.98% |
| 8 | 42.94% | 1.96% | 39.86% | 9.72% | |
| 10 | 31.72% | 0.65% | 32.98% | 4.26% | |
| 12 | 23.98% | 0.25% | 26.96% | 1.86% | |
| Reference solution | |||||
| Mesh | Strain Energy | von Mises Stress | ||
|---|---|---|---|---|
| Standard Solution | Adaptive Solution | Standard Solution | Adaptive Solution | |
| Coarse mesh | 19.06% | 9.84% | 25.40% | 9.65% |
| Fine mesh | 7.44% | 4.27% | 12.72% | 6.18% |
| Reference solution | ||||
| Mesh | DOFs | Number of Nodes | ||||
|---|---|---|---|---|---|---|
| Standard Solution | Adaptive Solution | None | Linear Cover | Quadratic Cover | ||
| Coarse mesh | 7308 | 17,469 | 0.22 | 2021 | 293 | 293 |
| Fine mesh | 24,579 | 47,637 | 0.15 | 7396 | 646 | 646 |
| Mesh | von Mises Stress | |
|---|---|---|
| Standard Solution | Adaptive Solution | |
| Coarse mesh | 39.46% | 14.73% |
| Fine mesh | 28.83% | 5.60% |
| Reference solution | ||
| Mesh | DOFs | Number of Nodes | ||||
|---|---|---|---|---|---|---|
| Standard Solution | Adaptive Solution | None | Linear Cover | Quadratic Cover | ||
| Coarse mesh | 1944 | 5040 | 0.25 | 506 | 86 | 86 |
| Fine mesh | 10,575 | 21,762 | 0.17 | 2999 | 310 | 311 |
| Mesh | von Mises Stress | |
|---|---|---|
| Standard Solution | Adaptive Solution | |
| Coarse mesh | 39.11% | 19.32% |
| Fine mesh | 23.73% | 8.35% |
| Reference solution | ||
| Mesh | DOFs | Number of Nodes | ||||
|---|---|---|---|---|---|---|
| Standard Solution | Adaptive Solution | None | Linear Cover | Quadratic Cover | ||
| Coarse mesh | 3435 | 7179 | 0.19 | 898 | 104 | 104 |
| Fine mesh | 13,854 | 23,268 | 0.11 | 4178 | 262 | 262 |
| DOFs | Number of Nodes | von Mises Stress | |||||
|---|---|---|---|---|---|---|---|
| Standard Solution | Adaptive Solution | None | Linear Cover | Quadratic Cover | Standard Solution | Adaptive Solution | |
| 9627 | 24,189 | 0.25 | 2429 | 415 | 415 | 33.3% | 8.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Choi, H.-G.; Byun, Y.I.; Song, C.K.; Jun, M.B.G.; Lee, C.; Kim, S. A Solution Procedure to Improve 3D Solid Finite Element Analysis with an Enrichment Scheme. Appl. Sci. 2023, 13, 7114. https://doi.org/10.3390/app13127114
Choi H-G, Byun YI, Song CK, Jun MBG, Lee C, Kim S. A Solution Procedure to Improve 3D Solid Finite Element Analysis with an Enrichment Scheme. Applied Sciences. 2023; 13(12):7114. https://doi.org/10.3390/app13127114
Chicago/Turabian StyleChoi, Hyung-Gyu, Young Il Byun, Chul Ki Song, Martin B.G. Jun, Chaemin Lee, and San Kim. 2023. "A Solution Procedure to Improve 3D Solid Finite Element Analysis with an Enrichment Scheme" Applied Sciences 13, no. 12: 7114. https://doi.org/10.3390/app13127114
APA StyleChoi, H.-G., Byun, Y. I., Song, C. K., Jun, M. B. G., Lee, C., & Kim, S. (2023). A Solution Procedure to Improve 3D Solid Finite Element Analysis with an Enrichment Scheme. Applied Sciences, 13(12), 7114. https://doi.org/10.3390/app13127114





