Spatial Deformation Calculation and Parameter Analysis of Pile–Anchor Retaining Structure
Abstract
1. Introduction
2. Spatial Deformation and Soil Pressure Distribution of Retaining Structure
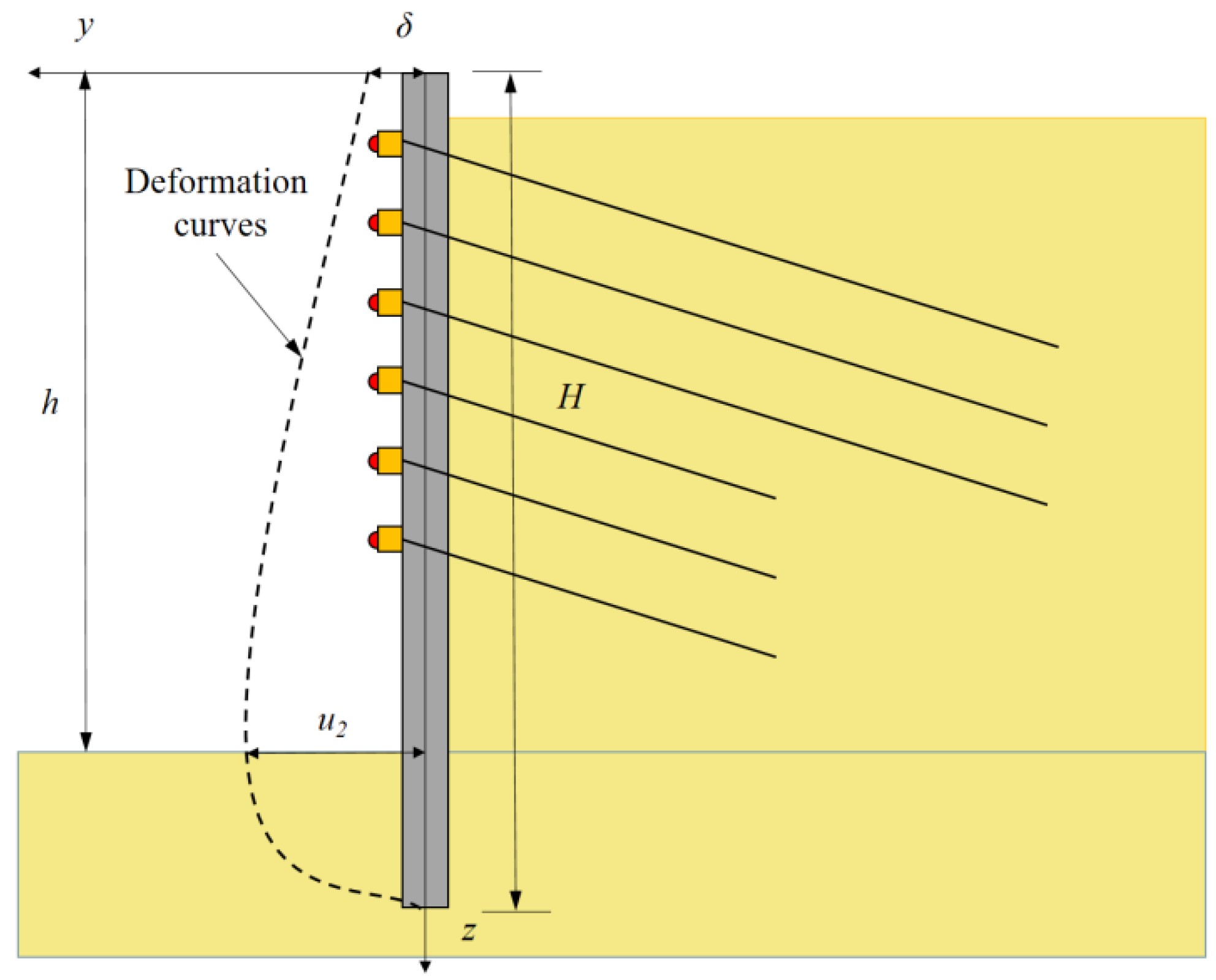
2.1. Spatial Deformation of Retaining Structure
2.1.1. Crown Beam Deformation
2.1.2. Pile Deformation
2.1.3. Waist Beam Deformation
2.1.4. Anchor Cable Deformation
2.2. Distribution of Soil Pressure behind the Structure
- c: Cohesive force of the soil mass (kPa).
- γ: Density of the soil mass (kN/m3).
- q: Overload on the slope top (Pa).
- ka: Active soil pressure coefficient.
- φ: Internal friction angle of the soil mass (°).
- m: Foundation resistance coefficient.
- H: Excavation depth of the foundation pit (m).
3. Calculation of Retaining Structure Deformation
3.1. Calculation of Strain Energy of Support Structure
3.2. Deformation Prediction Formula
4. Engineering Background and Numerical Simulation Model
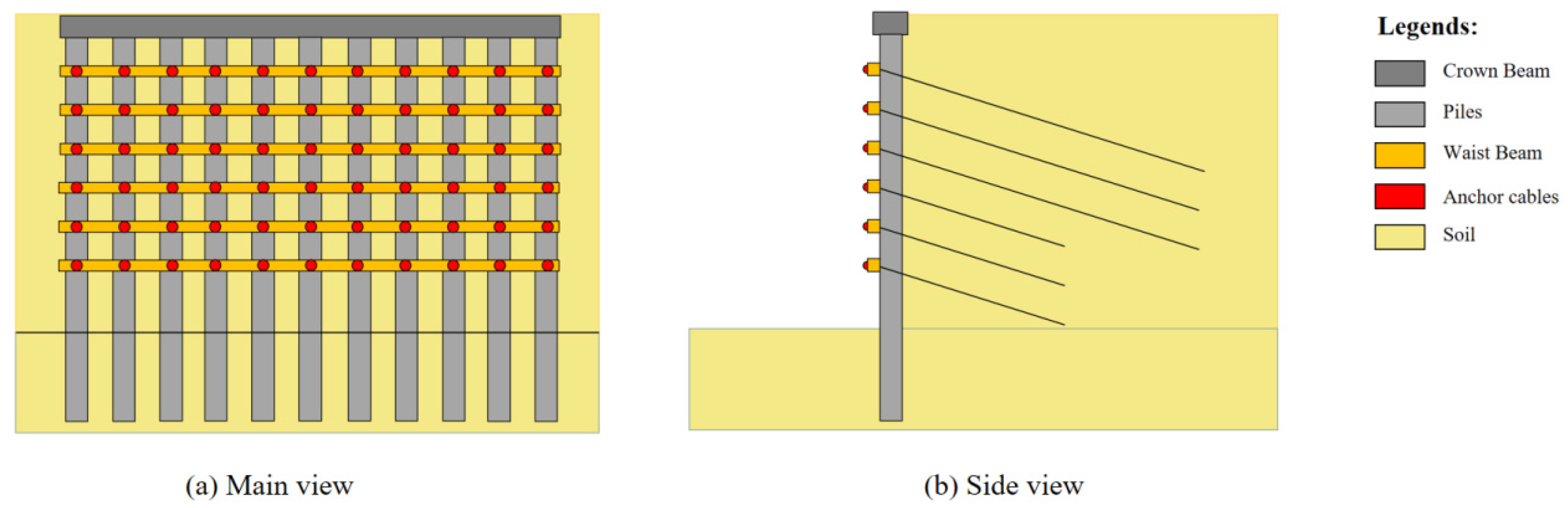
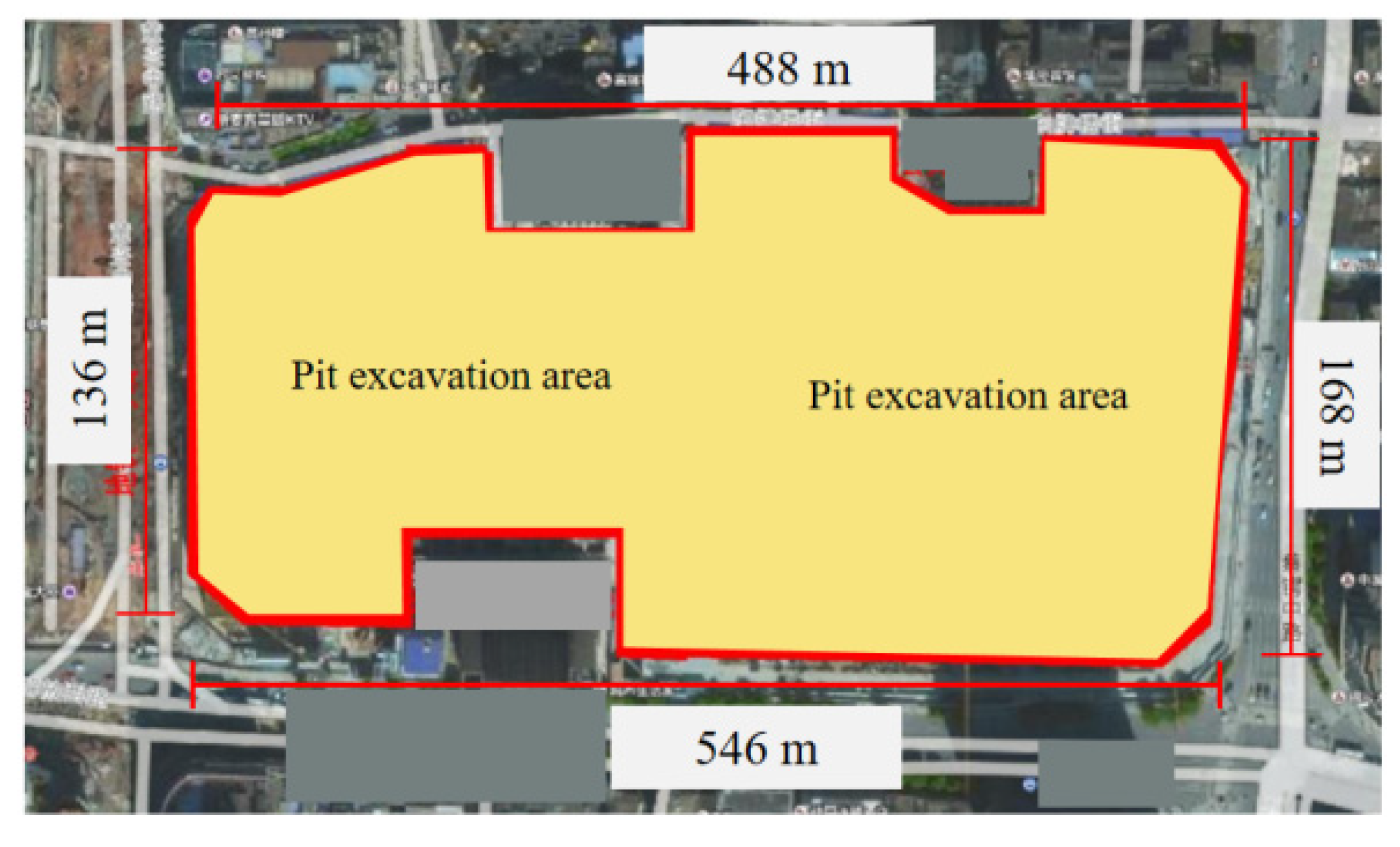
4.1. Project Profile
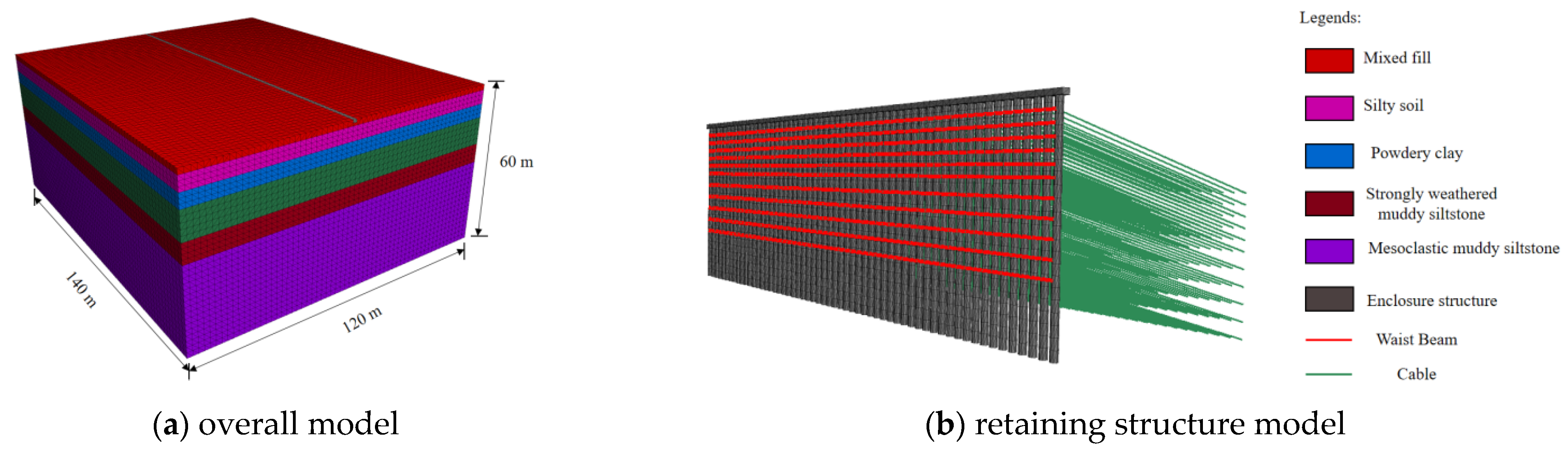
4.2. Numerical Simulation Model
4.2.1. Model Size and Boundary Conditions
4.2.2. Material Parameters
4.2.3. Calculation Steps
4.3. Comparative Analysis of Results
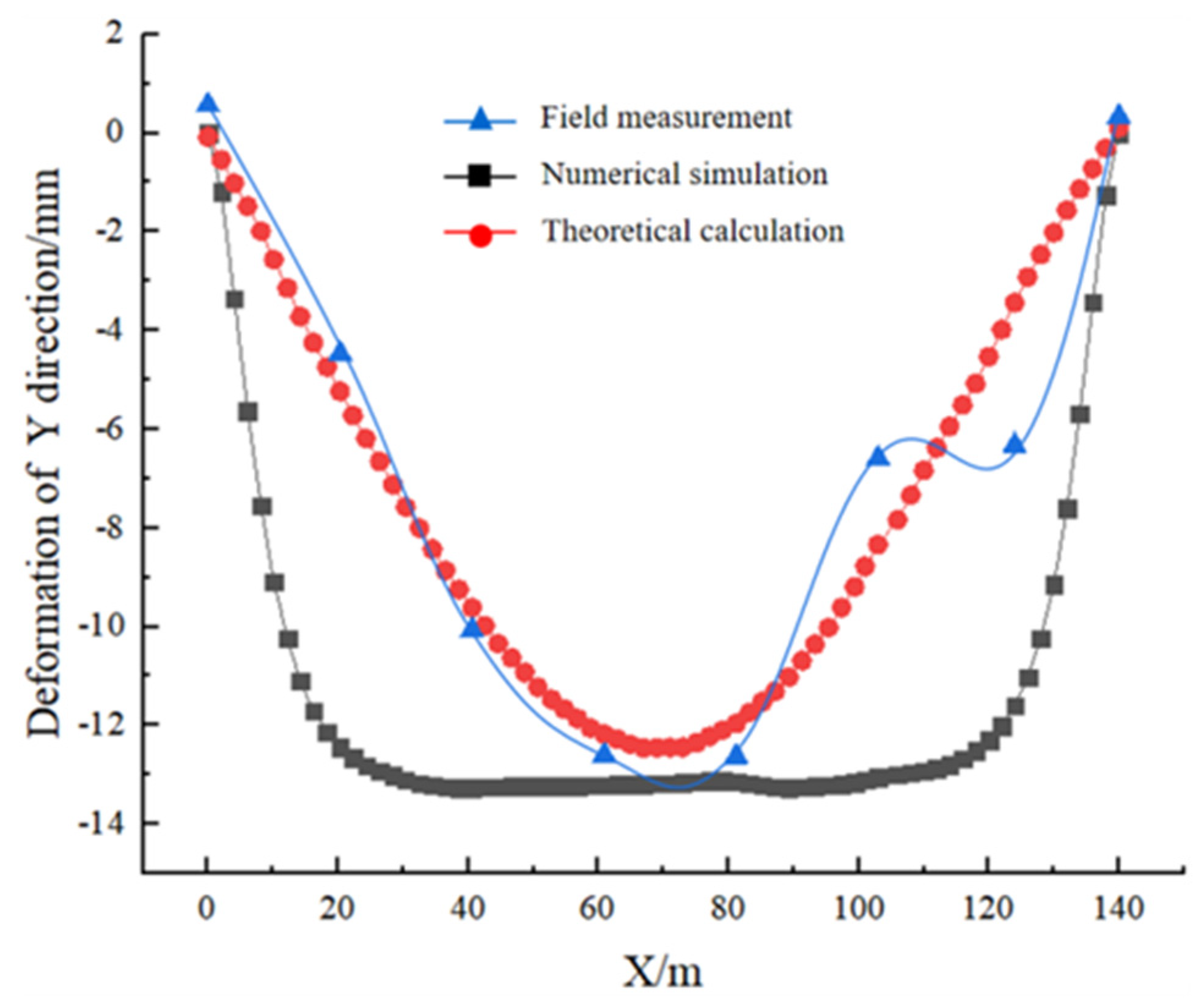
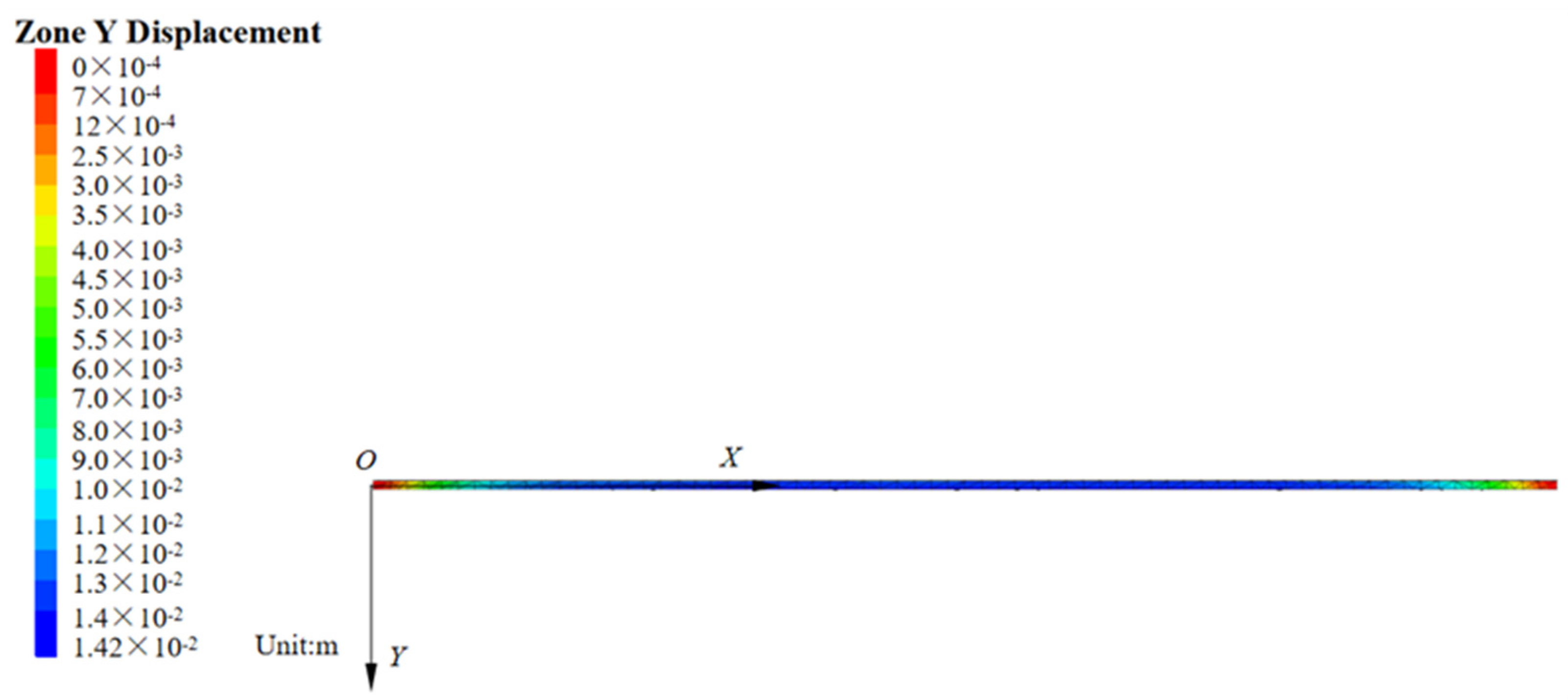
4.3.1. Comparison of Crown Beam Deformation
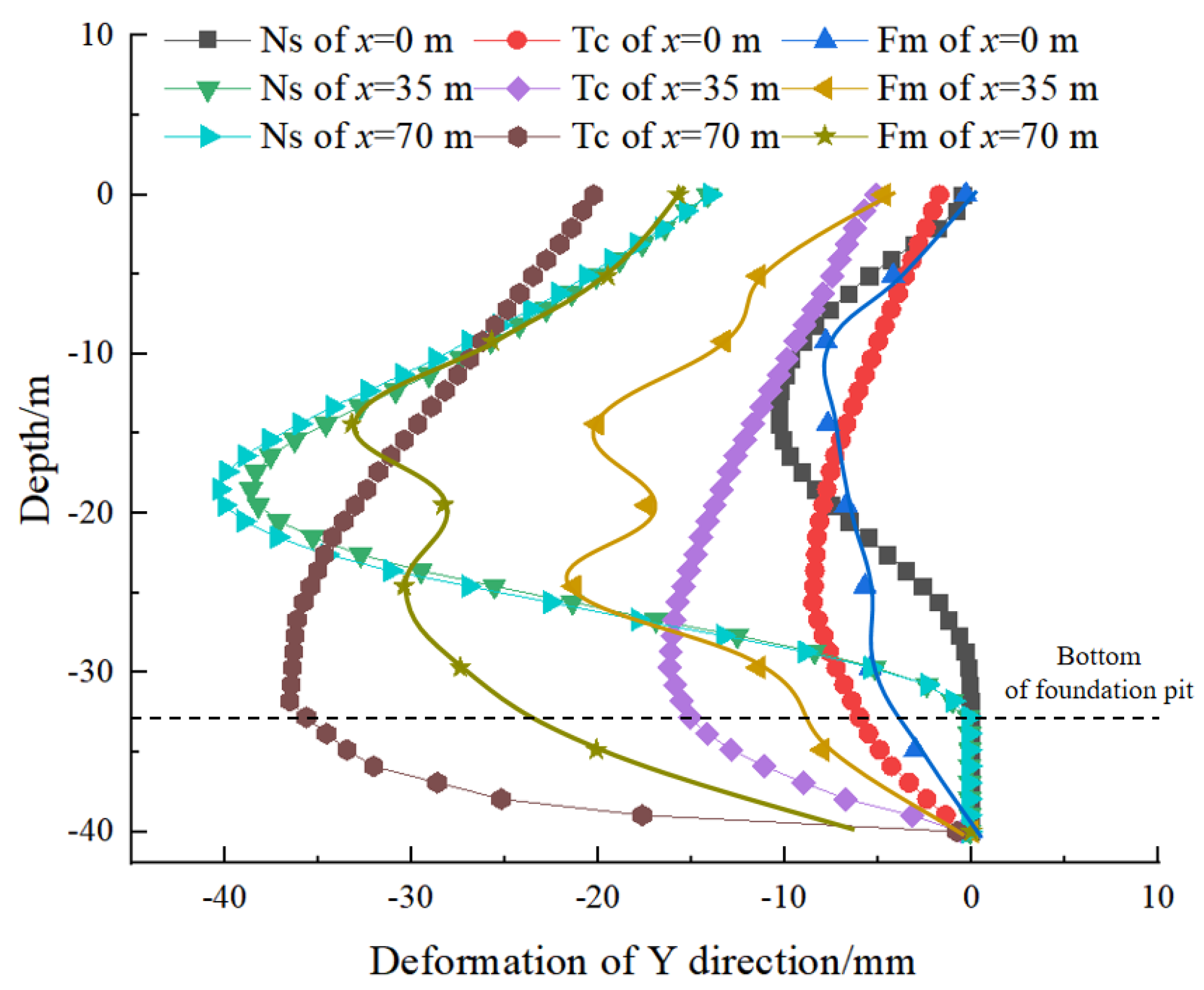
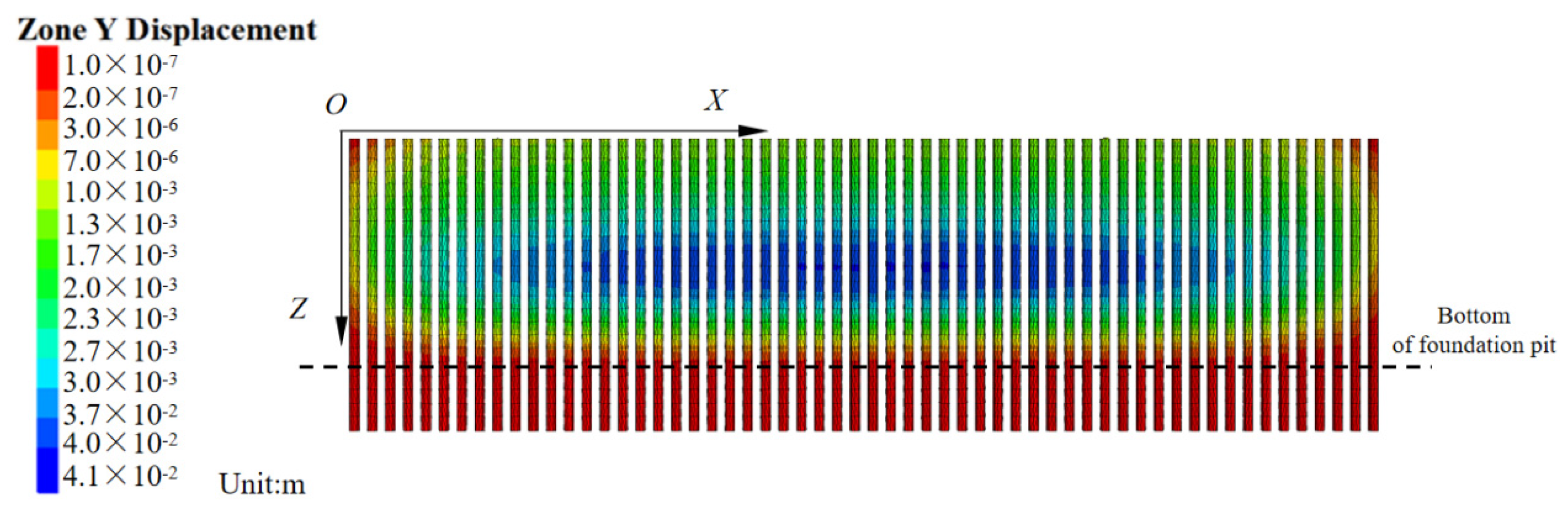
4.3.2. Comparison of Pile Deformation
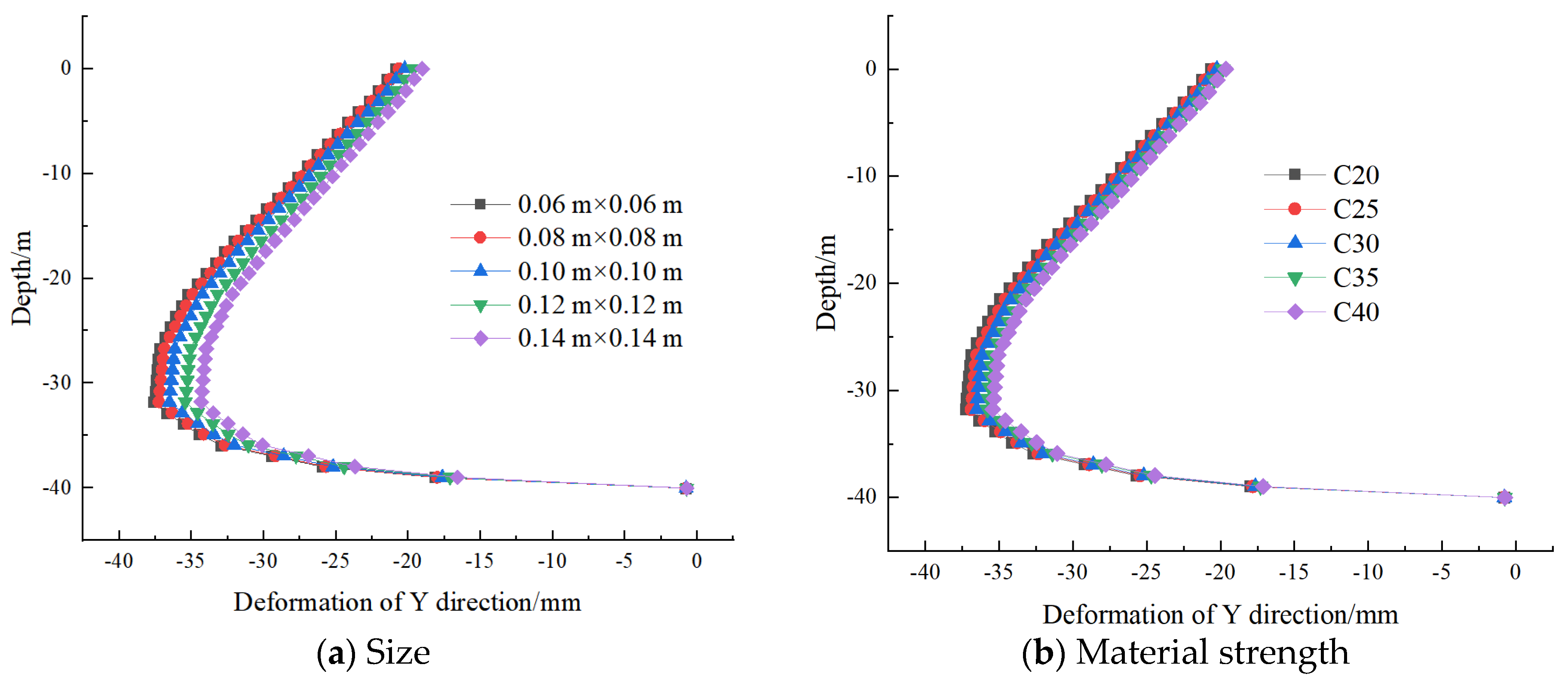
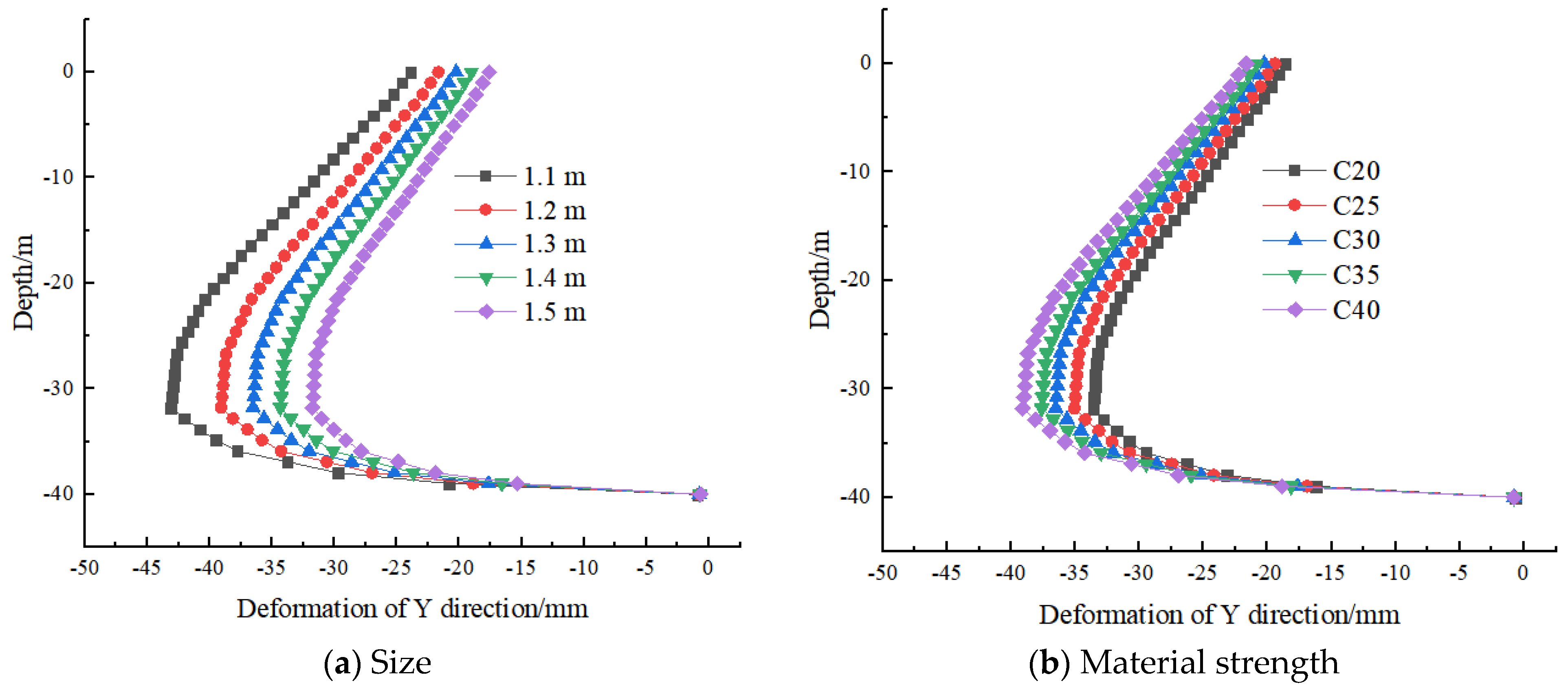
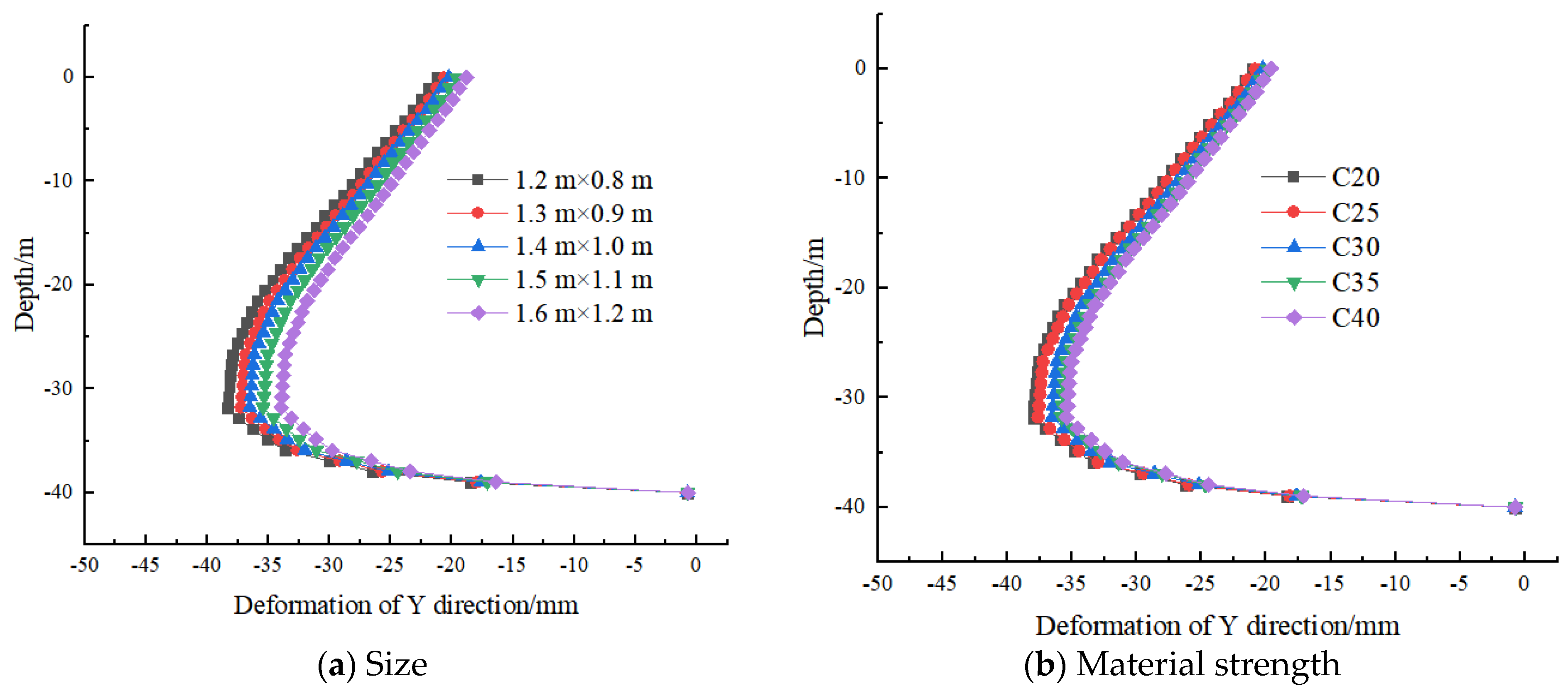
5. Parameters Analysis on Deformation
5.1. Waist Beam Size and Strength
5.2. Pile Size and Strength
5.3. Crown Beam Size and Strength
6. Conclusions
- (1)
- The theoretical prediction formula and numerical simulation model demonstrate practical applicability, with small errors observed when compared to on-site monitoring results.
- (2)
- The crown beam exhibits a ‘V’-shaped horizontal deformation curve, and the proximity to the center line of the foundation pit correlates with greater horizontal deformation. The maximum horizontal deformation of the crown beam is measured at 13.23 mm (theoretical), 12.44 mm (numerical simulation), and 12.60 mm (on-site monitoring). The differences in the horizontal distribution arise due to the treatment of the crown beam and pile as a whole in the numerical simulation model.
- (3)
- The horizontal deformation curve of the pile also exhibits a ‘V’-shaped distribution. The maximum horizontal deformation of the pile is observed at different depths (z = 33 m, z = 20 m, and z = 26 m) in the theoretical calculation, numerical simulation, and on-site monitoring, respectively. This discrepancy is attributed to the simplifications made in the theoretical calculation and numerical simulation, which do not account for the actual excavation steps. Future research can focus on modifying the spatial deformation curve of the retaining structure based on these findings.
- (4)
- The size and material strength of the waist beam and crown beam have negligible influence on the horizontal deformation of the pile. However, increasing the size and material strength of the pile significantly enhances the overall stiffness of the retaining structure, thereby reducing the horizontal deformation of the pile. Therefore, improving the size and material strength of the pile is crucial in minimizing the deformation of the retaining structure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Goh, A.T.; Zhang, W.; Wong, K. Deterministic and reliability analysis of basal heave stability for excavation in spatial variable soils. Comput. Geotech. 2019, 108, 152–160. [Google Scholar] [CrossRef]
- Choosrithong, K.; Schweiger, H.F. Numerical investigation of sequential strut failure on performance of deep excavations in soft soil. Int. J. Geomech. 2020, 20, 04020063. [Google Scholar] [CrossRef]
- Maleki, J.; Pak, A.; Yousefi, M.; Aghakhani, N. A comprehensive FE study for design of anchored wall systems for deep excavations. Tunn. Undergr. Space Technol. 2022, 122, 104340. [Google Scholar] [CrossRef]
- Momeni, E.; Poormoosavian, M.; Tehrani, H.S.; Fakher, A. Reliability analysis and risk assessment of deep excavations using random-set finite element method and event tree technique. Transp. Geotech. 2021, 29, 100560. [Google Scholar] [CrossRef]
- Lin, S.S.; Zhang, N.; Zhou, A.; Shen, S.L. Risk evaluation of excavation based on fuzzy decision-making model. Autom. Constr. 2022, 136, 104143. [Google Scholar] [CrossRef]
- Sun, J.; Sun, K.; Gong, L.; Gu, S.; Hao, H. Health Assessment of Foundation Pit Based on the Fuzzy Analytical Hierarchy Process. Adv. Civ. Eng. 2022, 2022, 3245305. [Google Scholar] [CrossRef]
- Long, L.; Li, Z. An assessment model of monitoring risk in deep excavation based on fuzzy theory. Indoor Built Environ. 2020, 29, 221–229. [Google Scholar] [CrossRef]
- Wu, C.; Yu, J.; Cao, X.; Shen, W. Study on Design Method of Pile Wall Combination Structure in a Deep Foundation Pit Considering Deformation Induced by Excavation. Front. Earth Sci. 2022, 10, 837950. [Google Scholar] [CrossRef]
- Wang, K.; Li, W.; Sun, H.; Pan, X.; Diao, H.; Hu, B. Lateral Deformation Characteristics and Control Methods of Foundation Pits Subjected to Asymmetric Loads. Symmetry 2021, 13, 476. [Google Scholar] [CrossRef]
- Li, Y.; Wang, C.; Sun, Y.; Wang, R.; Shao, G.; Yu, J. Analysis of Corner Effect of Diaphragm Wall of Special-Shaped Foundation Pit in Complex Stratum. Front. Earth Sci. 2022, 10, 238. [Google Scholar] [CrossRef]
- Su, T.; Zhou, Y.; Wang, Z.; Ye, S. Large Scale Model Test Study of Foundation Pit Supported by Pile Anchors. Appl. Sci. 2022, 12, 9792. [Google Scholar] [CrossRef]
- Zhao, X.; Wang, H.; Li, Z.; Dai, G.; Yin, Z.; Cao, S.; Zhou, J. Numerical Study on the Deformation of Tunnels by Excavation of Foundation Pit Adjacent to the Subway. Appl. Sci. 2022, 12, 4752. [Google Scholar] [CrossRef]
- Li, Z. Displacement monitoring during the excavation and support of deep foundation pit in complex environment. Adv. Civ. Eng. 2021, 2021, 5715306. [Google Scholar] [CrossRef]
- Zheng, Y.; Hu, Z.; Ren, X.; Wang, R.; Zhang, E. The influence of partial pile cutting on the pile-anchor supporting system of deep foundation pit in loess area. Arab. J. Geosci. 2021, 14, 1229. [Google Scholar] [CrossRef]
- Huang, M.; Liu, X.R.; Zhang, N.Y.; Shen, Q.W. Calculation of foundation pit deformation caused by deep excavation considering influence of loading and unloading. J. Cent. South Univ. 2017, 24, 2164–2171. [Google Scholar] [CrossRef]
- Song, D.; Chen, Z.; Dong, L.; Tang, G.; Zhang, K.; Wang, H. Monitoring analysis of influence of extra-large complex deep foundation pit on adjacent environment: A case study of Zhengzhou City, China, Geomatics. Nat. Hazards Risk 2020, 11, 2036–2057. [Google Scholar] [CrossRef]
- Ou, Q.; Zhang, L.; Zhao, M.; Wang, Y. Lateral displacement and internal force in diaphragm walls based on principle of minimum potential energy. Int. J. Geomech. 2019, 19, 04019055. [Google Scholar] [CrossRef]
- Bouazza, M.; Benseddiq, N.; Zenkour, A.M. Thermal buckling analysis of laminated composite beams using hyperbolic refined shear deformation theory. J. Therm. Stress. 2019, 42, 332–340. [Google Scholar] [CrossRef]
- Qin, S.; Wei, L.; Dai, Z. Simplified Calculation of Lateral Displacement of a Cement-Soil Gravity Retaining Wall. Math. Probl. Eng. 2023, 2023, 3443699. [Google Scholar] [CrossRef]
- Qin, Z.; Tao, Z.; Chen, Z.; Zhang, Z.; Tang, C.; Liu, H.; Ren, Q. Deformation Analysis and Prediction of Foundation Pit in Soil-Rock Composite Stratum. Front. Phys. 2022, 9, 817429. [Google Scholar] [CrossRef]
- Wei, G.; Guo, B.; Wang, Z.; Diao, H.; Wang, X. Analysis of influence of deformation modes of retaining structures on deformation of a side shield tunnel. Sci. Rep. 2022, 12, 19974. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.-S.; Lee, D.S.; Oh, S.-H. Experimental Investigation of the Earth Pressure on Horizontally Composite Breakwaters According to Different Shoulder Widths of Rubble Mounds. Water 2022, 14, 2273. [Google Scholar] [CrossRef]
- Ashtiani, M.; Nowkandeh, M.J.; Kayhani, A. Numerical modeling of the interaction of normal fault and shallow embedded foundation. Bull. Earthq. Eng. 2021, 19, 4805–4832. [Google Scholar] [CrossRef]
- Sun, J.; Yu, T.; Dong, P. Evaluation of 3D slope stability based on the minimum potential energy principle. Comput. Geotech. 2022, 146, 104717. [Google Scholar] [CrossRef]
- Wang, G.; Fang, Q.; Du, J.; Yang, X.; Wang, J. Estimating Volume Loss for Shield-Driven Tunnels Based on the Principle of Minimum Total Potential Energy. Appl. Sci. 2022, 12, 1794. [Google Scholar] [CrossRef]
- Commend, S.; Geiser, F.; Crisinel, J. Numerical simulation of earthworks and retaining system for a large excavation. Adv. Eng. Softw. 2004, 35, 669–678. [Google Scholar] [CrossRef]
- Deng, H.-S.; Fu, H.-L.; Yue, S.; Huang, Z.; Zhao, Y.-Y. Ground loss model for analyzing shield tunneling-induced surface settlement along curve sections. Tunn. Undergr. Space Technol. 2022, 119, 104250. [Google Scholar] [CrossRef]
- Deng, H.-S.; Fu, H.-L.; Shi, Y.; Zhao, Y.-Y.; Hou, W.-Z. Countermeasures against large deformation of deep-buried soft rock tunnels in areas with high geostress: A case study. Tunn. Undergr. Space Technol. 2022, 119, 104238. [Google Scholar] [CrossRef]
- Cao, L.; Zhang, D.; Fang, Q. Semi-analytical prediction for tunnelling-induced ground movements in multi-layered clayey soils. Tunn. Undergr. Space Technol. 2020, 102, 103446. [Google Scholar] [CrossRef]
- Wen-Juan, Y.; Hua-Cong, Z. Research on the Horizontal Displacement Coefficient of Soil Surrounding Pile in Layered Foundations by Considering the Soil Mass’s Longitudinal Continuity. Math. Probl. Eng. 2013, 2013, 948917. [Google Scholar] [CrossRef]
- Medina, C.; Álamo, G.M.; Aznárez, J.J.; Padron, L.A.; Maeso, O. Influence of soil non-homogeneity on the base shear force of piled structures subjected to harmonic seismic waves. Eng. Struct. 2020, 215, 110658. [Google Scholar] [CrossRef]



| Stratum | Natural Gravity γ (KN/m3) | Cohesion c (kPa) | Friction Angle φ (°) | Elastic Modulus (MPa) | Poisson’s Ratio | Thickness (m) |
|---|---|---|---|---|---|---|
| Plain fill | 19.5 | 12 | 8 | 8 | 0.3 | 2 |
| Mucky soil | 18.2 | 12 | 6 | 4 | 0.35 | 5 |
| Silty clay | 19.5 | 30 | 18 | 16 | 0.25 | 5 |
| Coarse sand | 20.2 | 0 | 35 | 40 | 0.25 | 11 |
| Round gravel | 20.5 | 0 | 40 | 60 | 0.25 | 7 |
| Argillaceous Siltstone | 21.9 | 40 | 40 | 82 | 0.25 | - |
| Material | Elastic Modulus (MPa) | Poisson’s Ratio | Bending Stiffness (N·m2) |
|---|---|---|---|
| Crown beam | 30,000 | 0.25 | 6.86 × 106 |
| Waist beam | 30,000 | 0.25 | 25,000 |
| Pile | 30,000 | 0.25 | 4.25 × 106 |
| Anchor cable | 200,000 | 0.20 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, Q.; Fu, H.; Zhou, Y. Spatial Deformation Calculation and Parameter Analysis of Pile–Anchor Retaining Structure. Appl. Sci. 2023, 13, 6637. https://doi.org/10.3390/app13116637
Yin Q, Fu H, Zhou Y. Spatial Deformation Calculation and Parameter Analysis of Pile–Anchor Retaining Structure. Applied Sciences. 2023; 13(11):6637. https://doi.org/10.3390/app13116637
Chicago/Turabian StyleYin, Quan, Helin Fu, and Yi Zhou. 2023. "Spatial Deformation Calculation and Parameter Analysis of Pile–Anchor Retaining Structure" Applied Sciences 13, no. 11: 6637. https://doi.org/10.3390/app13116637
APA StyleYin, Q., Fu, H., & Zhou, Y. (2023). Spatial Deformation Calculation and Parameter Analysis of Pile–Anchor Retaining Structure. Applied Sciences, 13(11), 6637. https://doi.org/10.3390/app13116637





