The Effect of Scaling Building Configuration Blast Experiments on Positive Phase Blast Wave Parameters
Abstract
1. Introduction
2. Materials and Methods
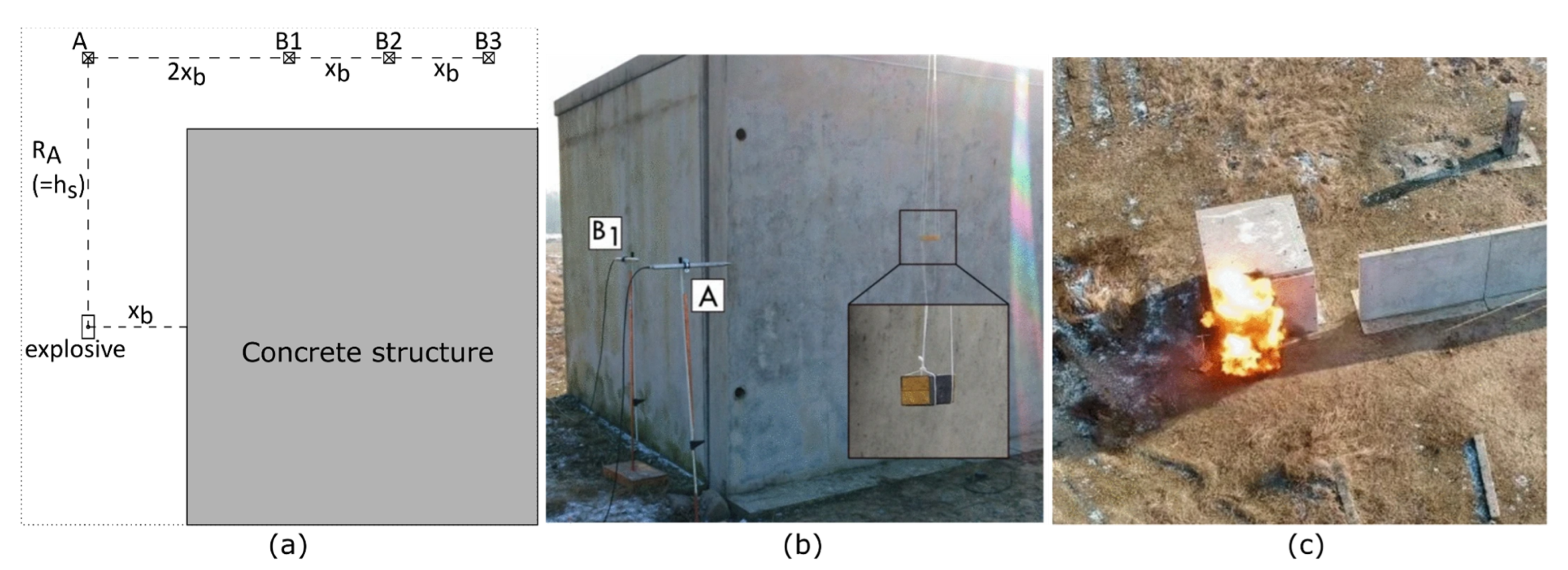
2.1. Experimental Design
2.1.1. Scaling Charge Mass
2.1.2. Scaling Corner Feature
2.2. Instrumentation
3. Numerical Modelling Approach
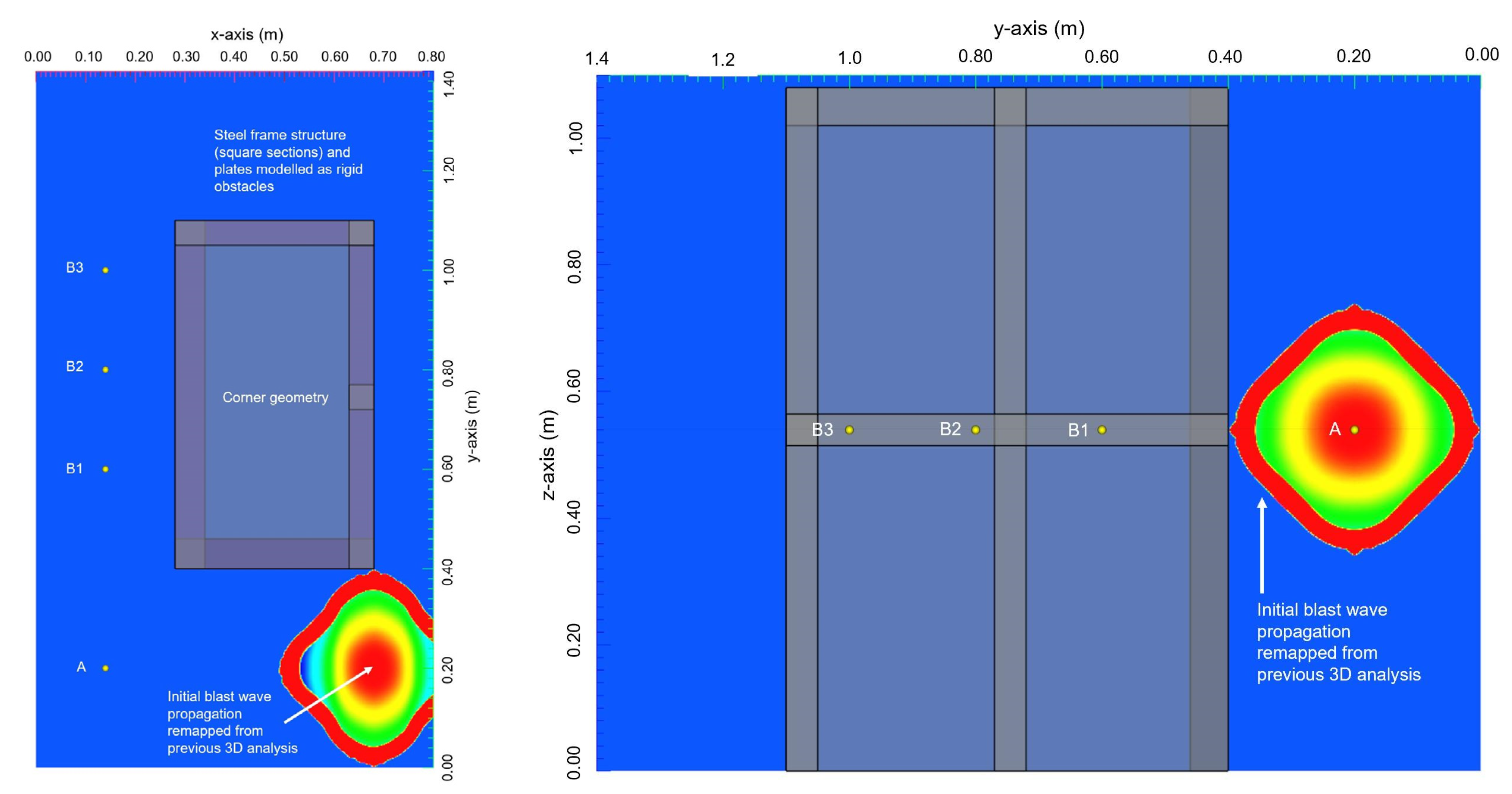
3.1. CFD Methodology
3.2. Detonation and Near-Field Blast Propagation (Stage 1)
| Air | PE-4 | |
|---|---|---|
| Equation of State (EOS) | Ideal gas | JWL |
| Initial Conditions | γ = 1.4 e = 2.068 × 105 kJ/kg ρ = 1.225 kg/m3 | A = 9.593 × 105 MPa B = 4.914 GPa R1 = 5.616 R2 = 1.804 ω = 0.136 |
3.3. Blast Wave Propagation and Structural Interaction (Stage 2)
4. Results and Discussion
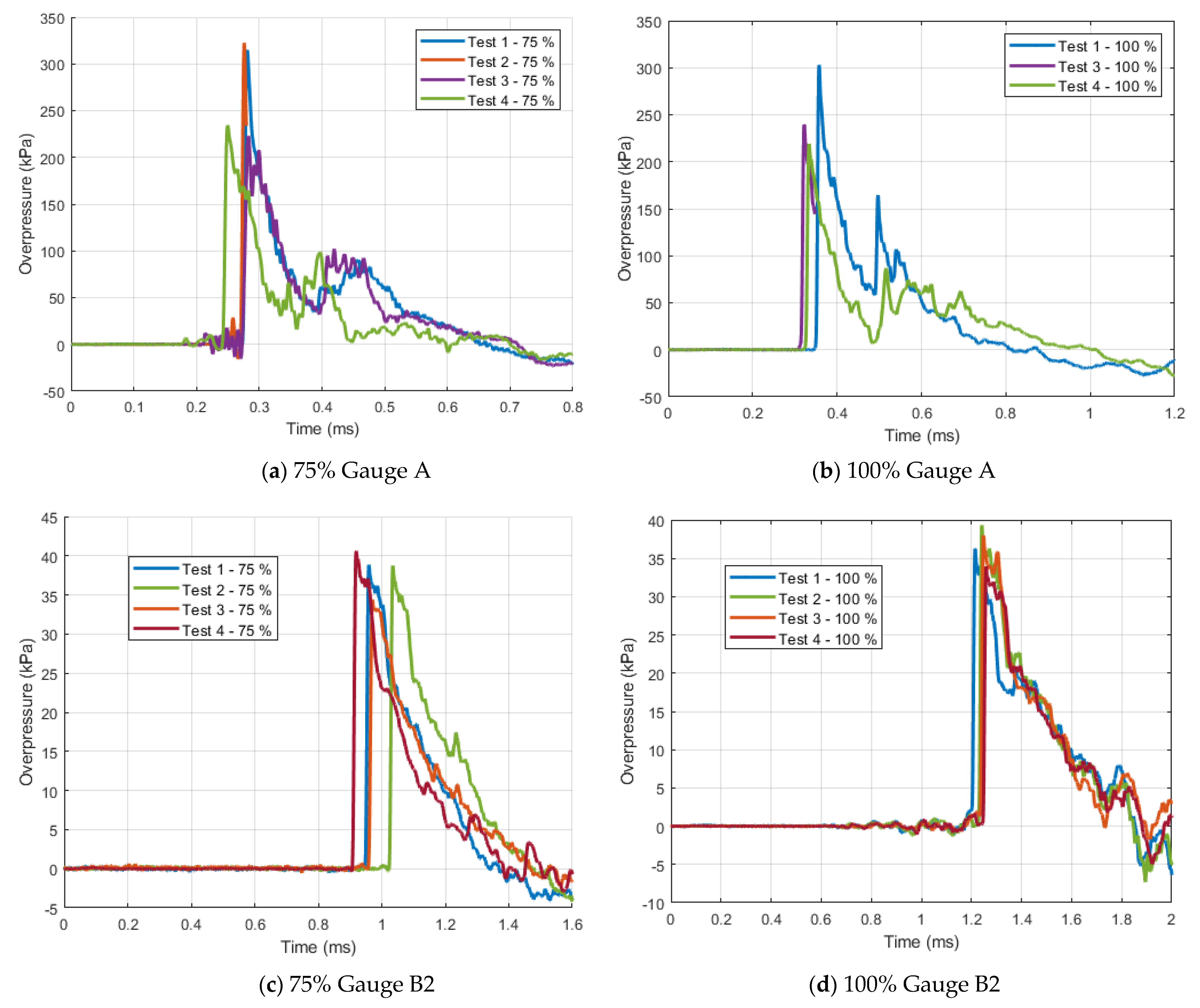
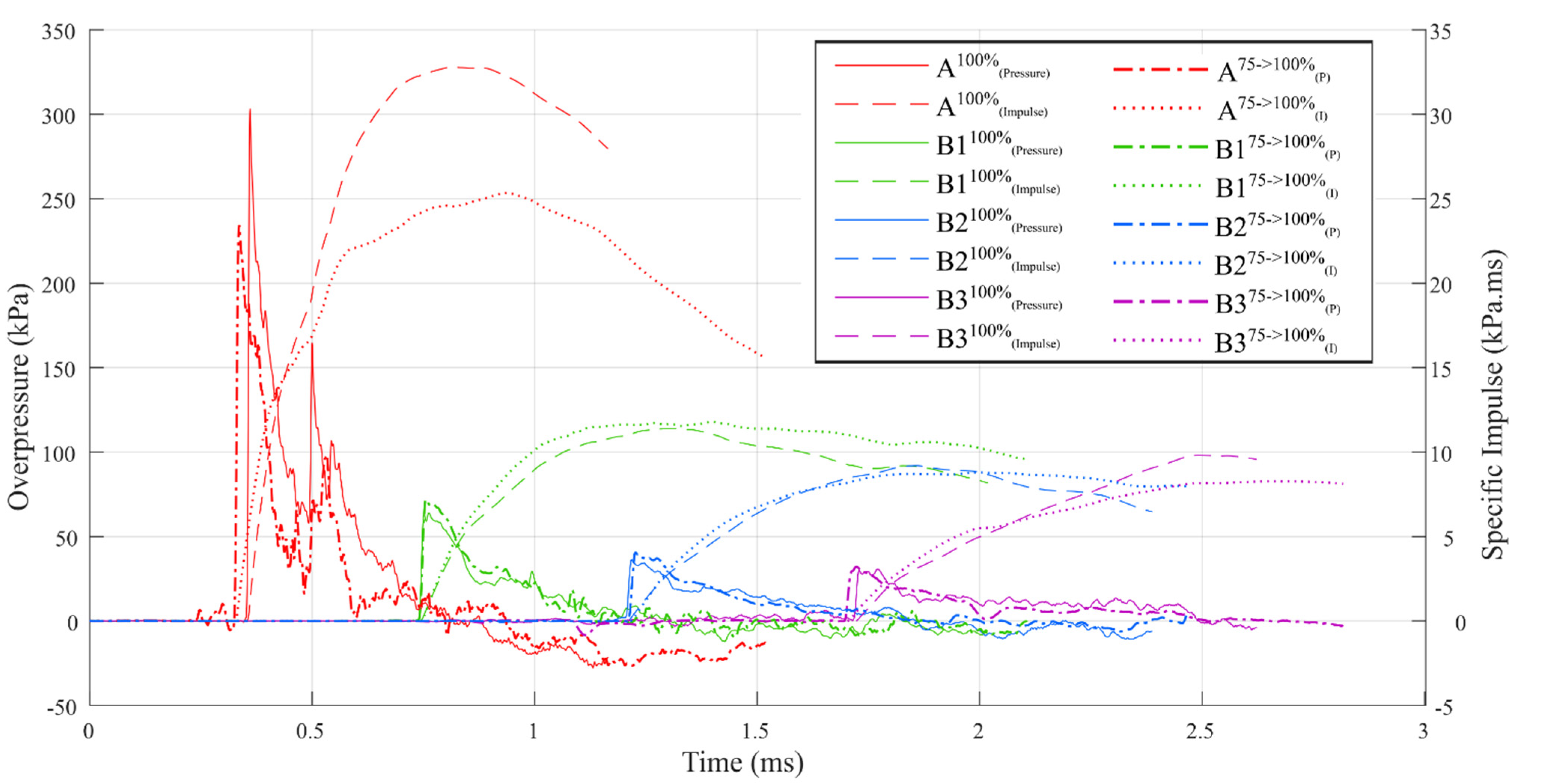
4.1. Experimental Results
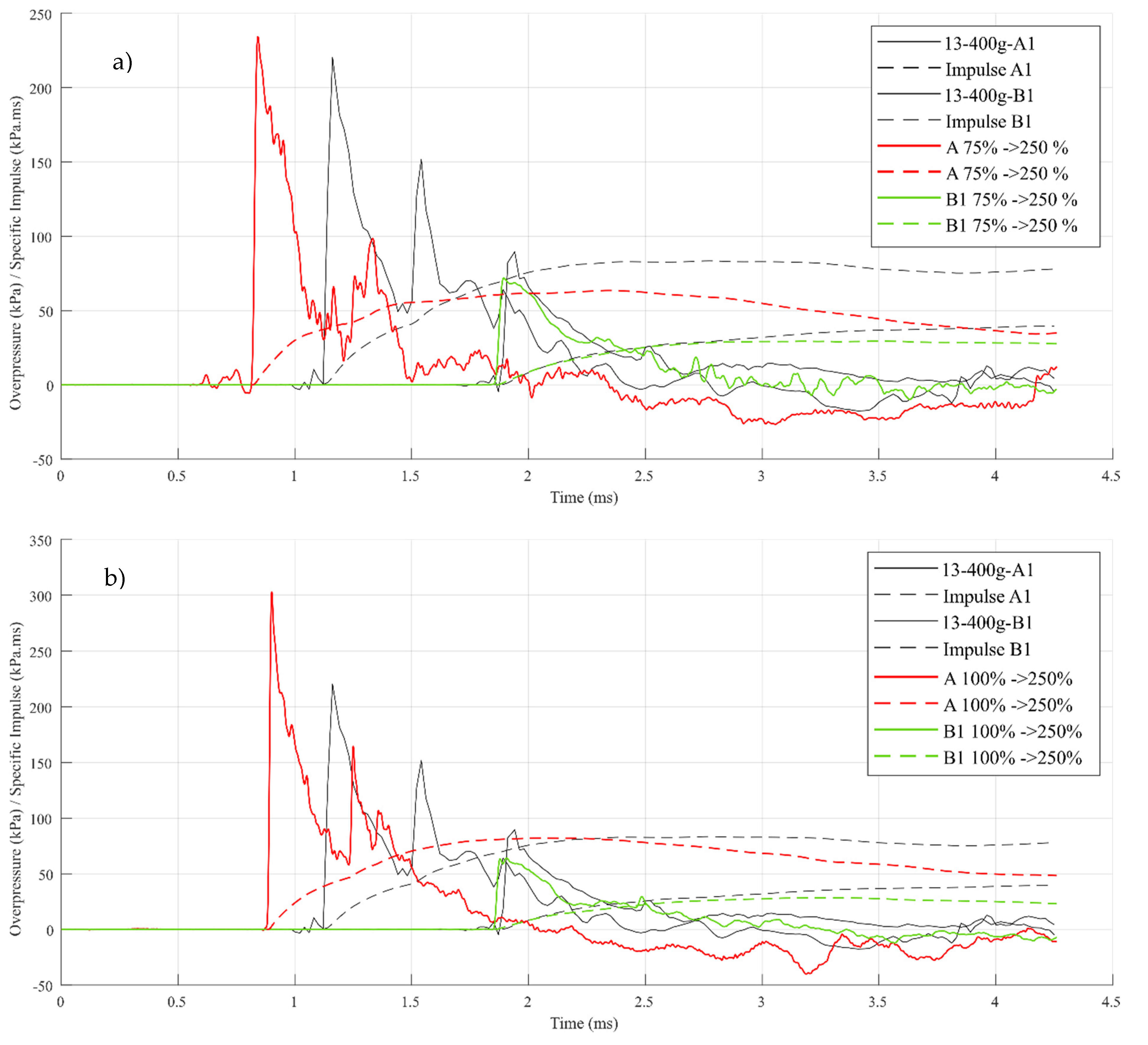
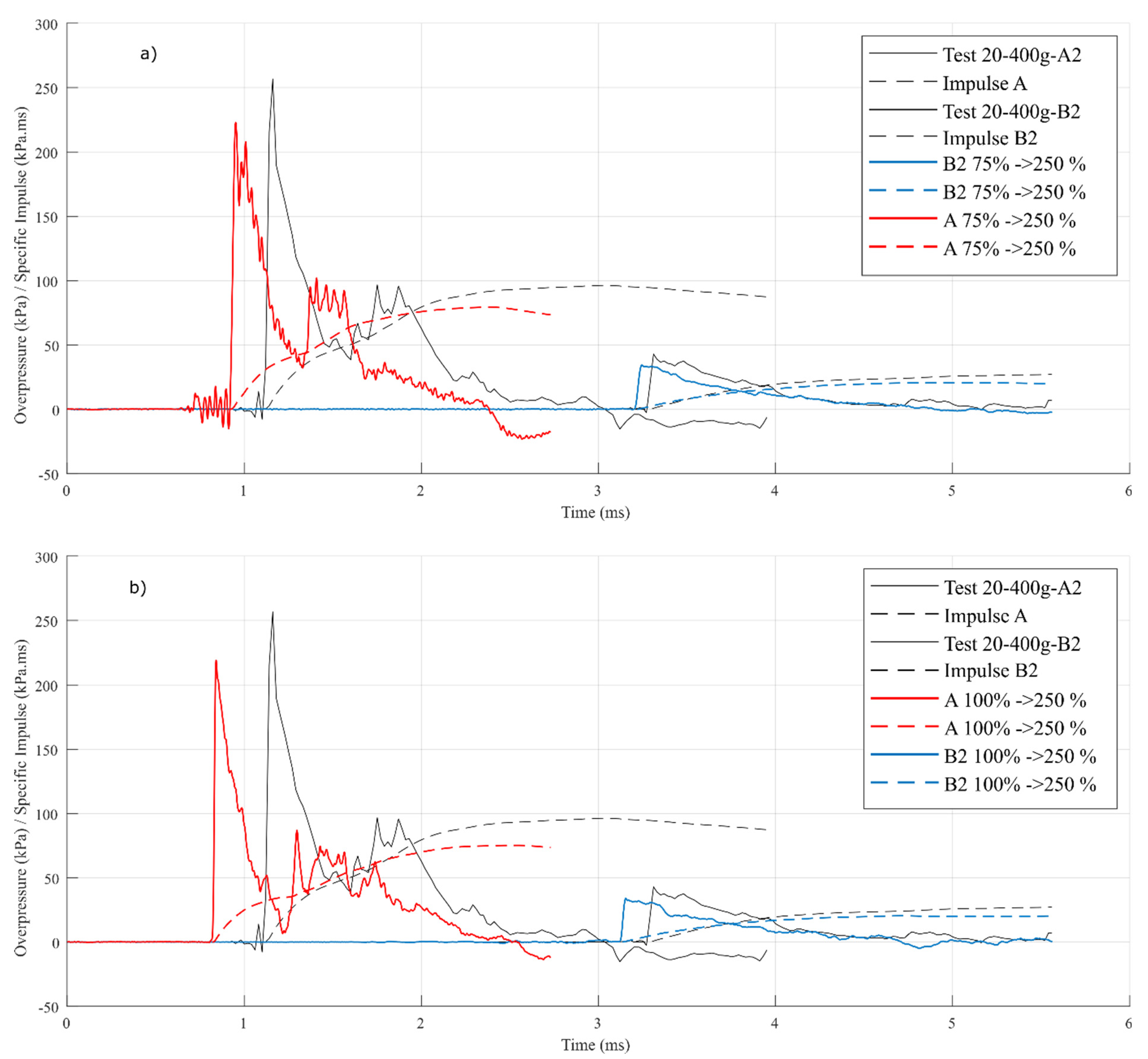
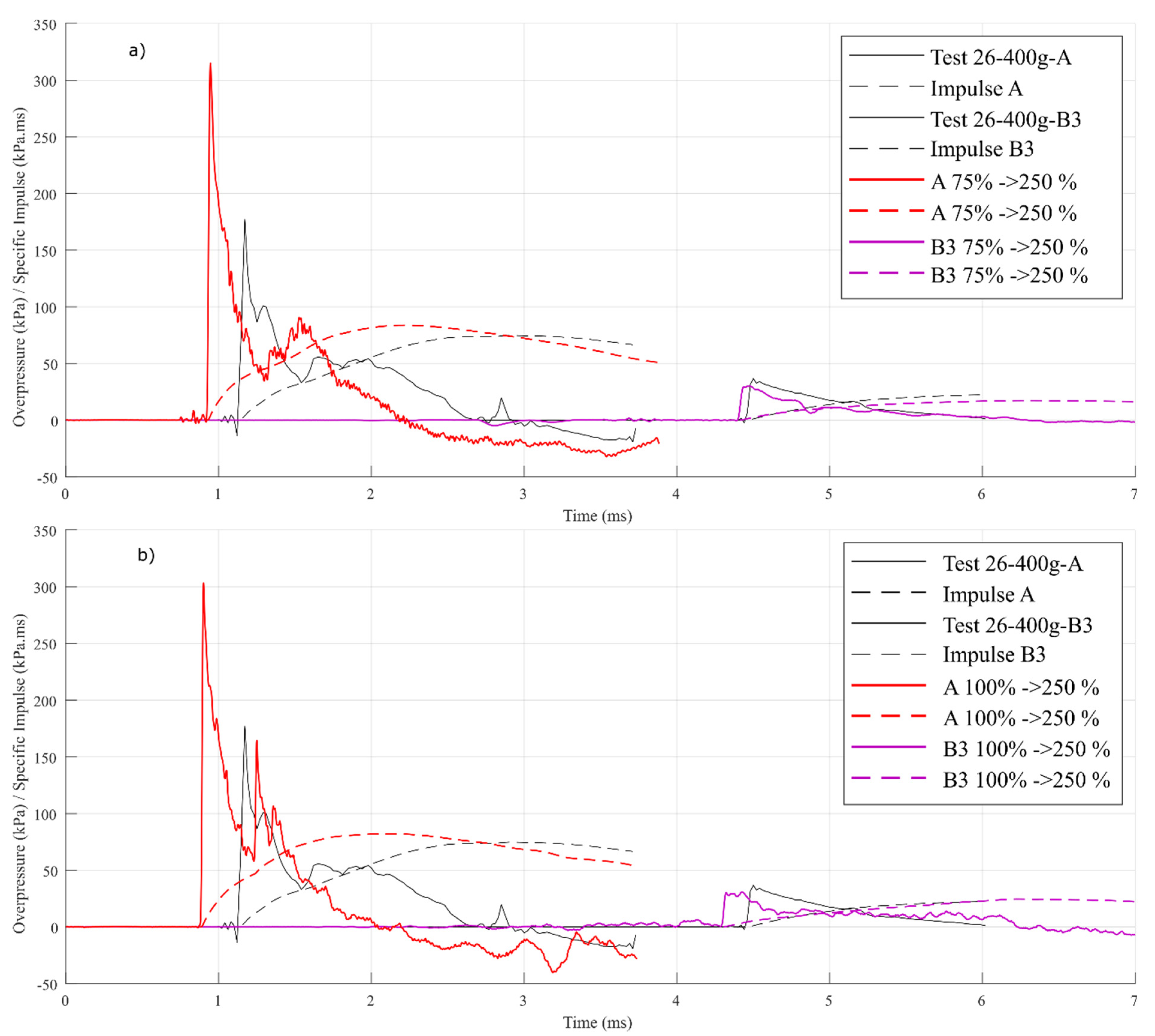
4.2. Scaling 75% Results to Predict Blast Parameters at 100% Scale
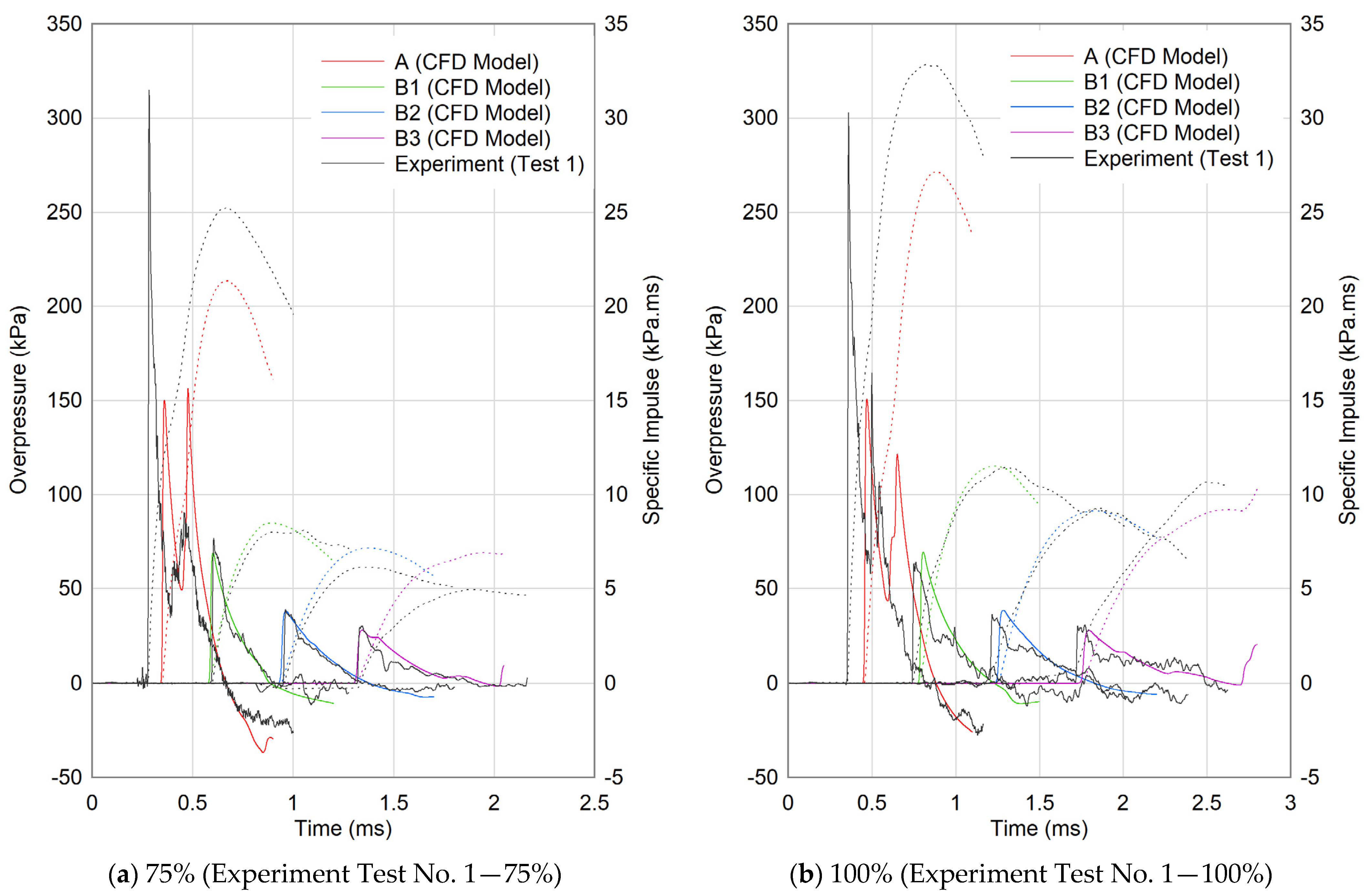
4.3. Numerical Modelling Results and Comparison to Experimental Work
4.4. Using Laboratory Results to Predict Parameters Found for Field Tests (at 250% Scale)
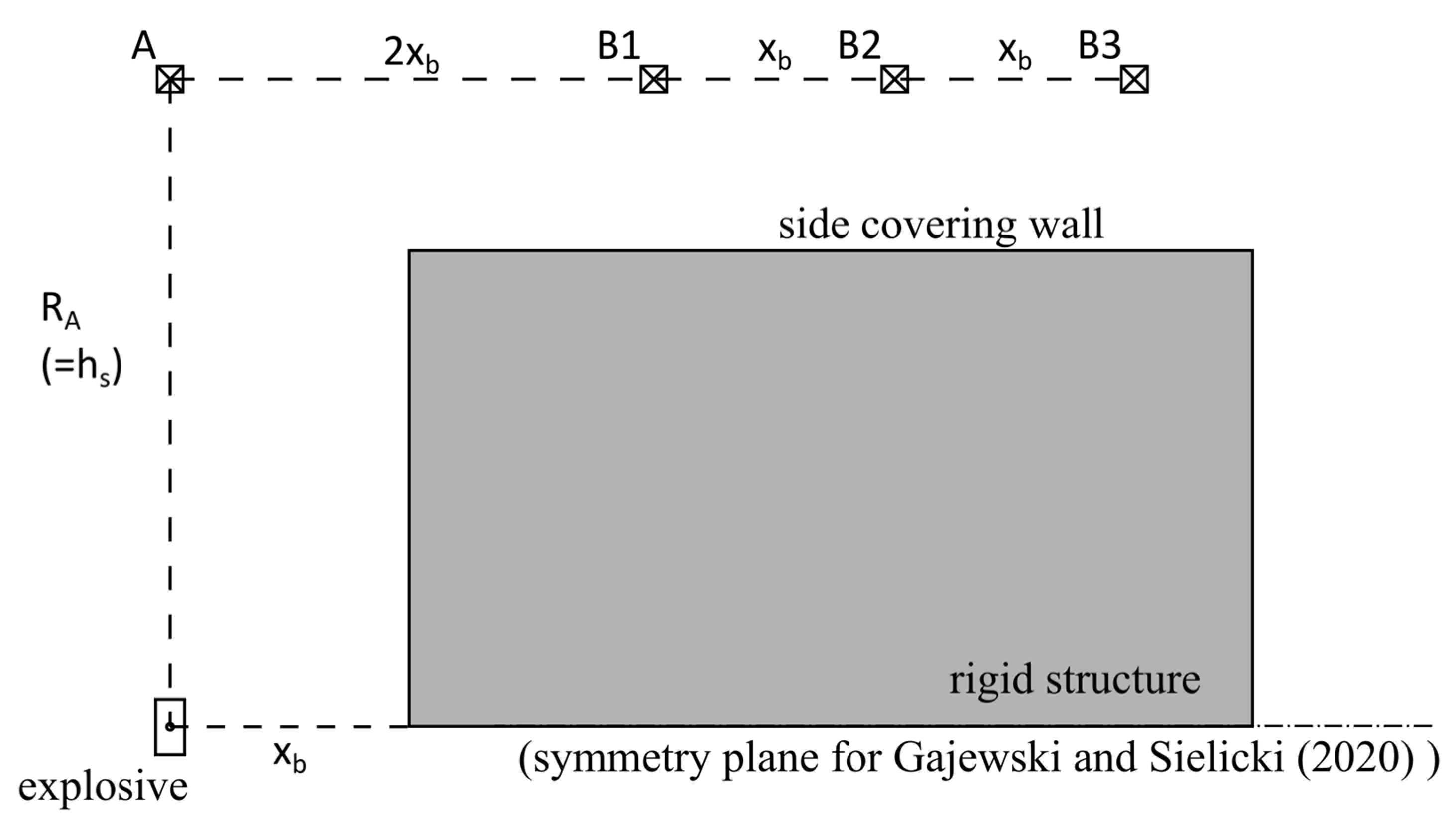
- The detonator position was not identical—for the field trials, the explosive was rear detonated at the cross-sectional centre of the bottom of the two 200 g TNT blocks (referred to as the rear–below centre). However, in the current work, the PE-4 cuboid was a single moulded part with a centrally located detonator (referred to as middle–centre detonated);
- Instead of a relatively smooth corner structure manufactured from 12 mm plated steel and a lightweight steel frame, a reinforced concrete structure with a similar corner geometry was used. A slight gap between the side covering wall and the front wall is evident in the photographs of the field trials (Figure 9) [11]. The current work used a steel baseplate for the lab-scale indoor tests rather than earth (this is likely to be less important as the charges were detonated considerably high above the ground).
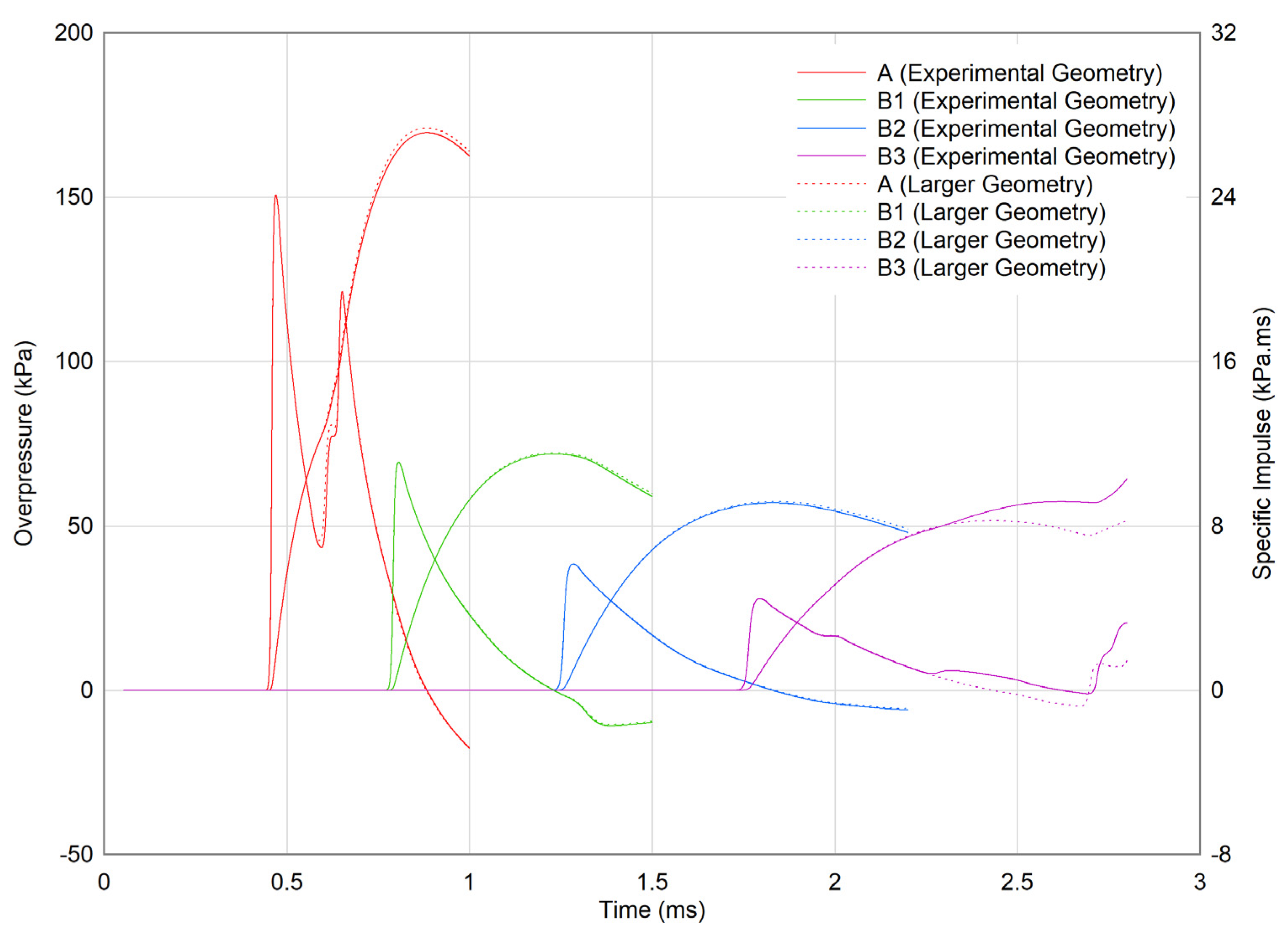
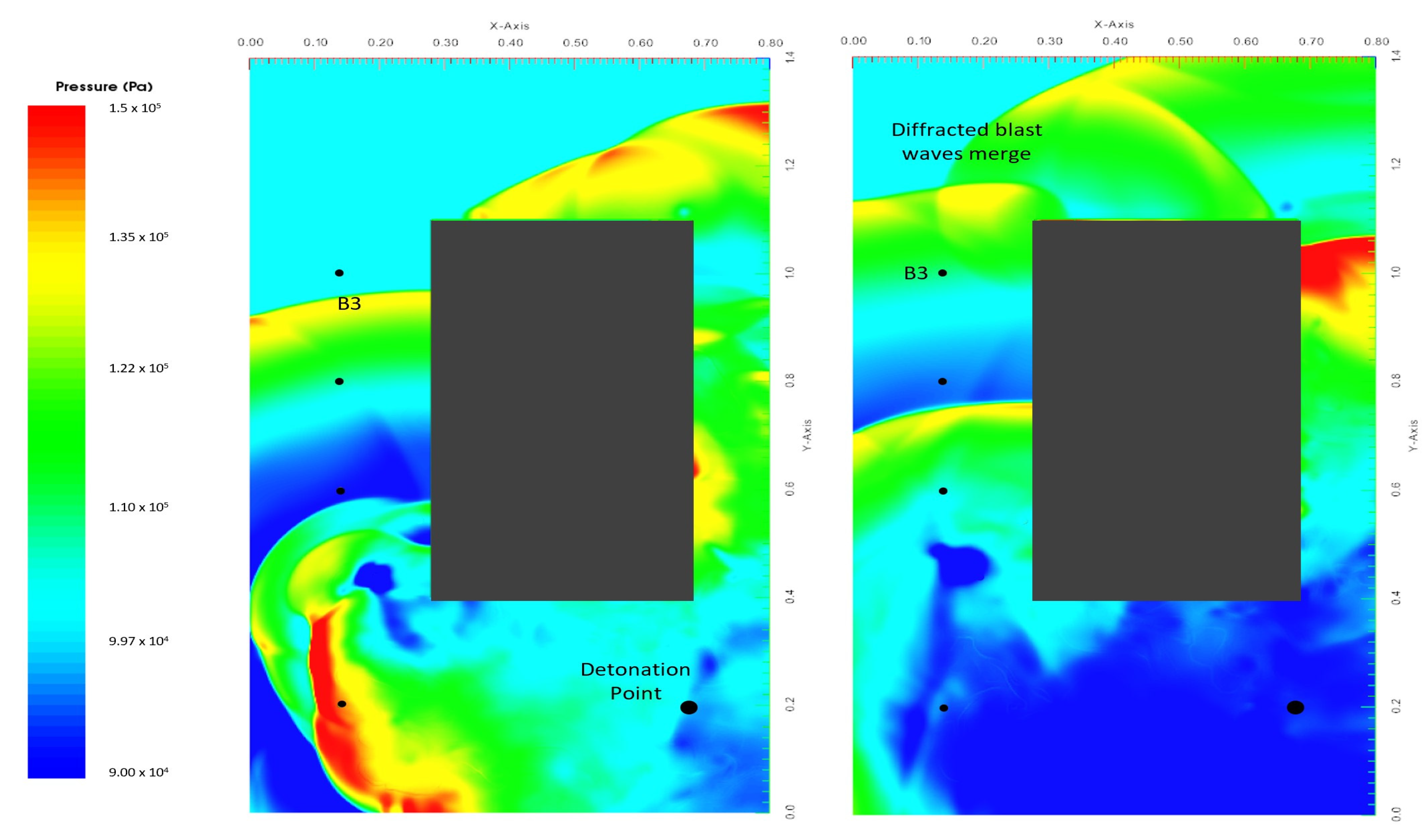
- The geometry is not quite identical—in the current tests, the front wall was simplified to facilitate efficient numerical simulations by enabling a half-symmetry assumption (Figure 10). The implications of this assumption are considered in the following discussion.
4.4.1. Assessing the Effect of Employing Half-Symmetry
4.4.2. Comparing Experimental Values between Field and Laboratory Tests
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Test Data for Laboratory-Scaled Tests Conducted
| Test 1 | Test 2 | |||||||
|---|---|---|---|---|---|---|---|---|
| Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | |
| Gauge A | 303.054 | 0.351 | 0.464 | 32.783 | ||||
| Gauge A * | 164.514 | 0.500 | 0.315 | 19.128 | ||||
| Gauge B1 | 63.373 | 0.739 | 0.562 | 11.388 | 74.219 | 0.758 | 0.434 | 11.620 |
| Gauge B2 | 36.282 | 1.205 | 0.653 | 9.194 | 39.320 | 1.233 | 0.617 | 8.747 |
| Gauge B3 | 30.215 | 1.715 | 0.779 | 9.817 | 32.620 | 1.742 | 0.734 | 7.897 |
| Test 3 | Test 4 | |||||||
| Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | |
| Gauge A | 239.755 | 0.318 | 219.157 | 0.323 | 0.692 | 29.534 | ||
| Gauge A * | 86.324 | 0.519 | 0.497 | 14.006 | ||||
| Gauge B1 | 70.960 | 0.775 | 0.418 | 10.495 | 66.417 | 0.776 | 0.419 | 10.062 |
| Gauge B2 | 38.000 | 1.235 | 0.655 | 8.591 | 33.955 | 1.245 | 0.641 | 8.166 |
| Gauge B3 | 29.347 | 1.748 | ||||||
| Test 1 | Test 2 | |||||||
|---|---|---|---|---|---|---|---|---|
| Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | |
| Gauge A | 314.921 | 0.276 | 0.391 | 25.128 | 322.520 | 0.269 | ||
| Gauge A * | 89.891 | 0.491 | 0.206 | 18.098 | ||||
| Gauge B1 | 76.629 | 0.592 | 0.319 | 79.826 | 65.551 | 0.665 | 0.327 | 8.322 |
| Gauge B2 | 38.858 | 0.950 | 0.426 | 60.993 | 38.733 | 1.027 | 0.467 | 6.375 |
| Gauge B3 | 29.400 | 1.318 | 0.572 | 5.175 | 29.514 | 1.397 | 0.572 | 6.065 |
| Test 3 | Test 4 | |||||||
| Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | Pi (kPa) | ta (ms) | t+ (ms) | Ii (kPa.ms) | |
| Gauge A | 222.878 | 0.275 | 0.441 | 23.837 | 234.297 | 0.240 | 0.361 | 18.474 |
| Gauge A * | 101.926 | 0.423 | 0.293 | 14.617 | 98.505 | 0.400 | 0.202 | 14.507 |
| Gauge B1 | 67.522 | 0.591 | 0.354 | 8.161 | 71.873 | 0.556 | 0.349 | 8.740 |
| Gauge B2 | 34.380 | 0.960 | 0.510 | 6.226 | 40.625 | 0.910 | 0.488 | 9.544 |
| Gauge B3 | 27.580 | 1.335 | 0.608 | 6.150 | 32.034 | 1.276 | 0.626 | 6.143 |
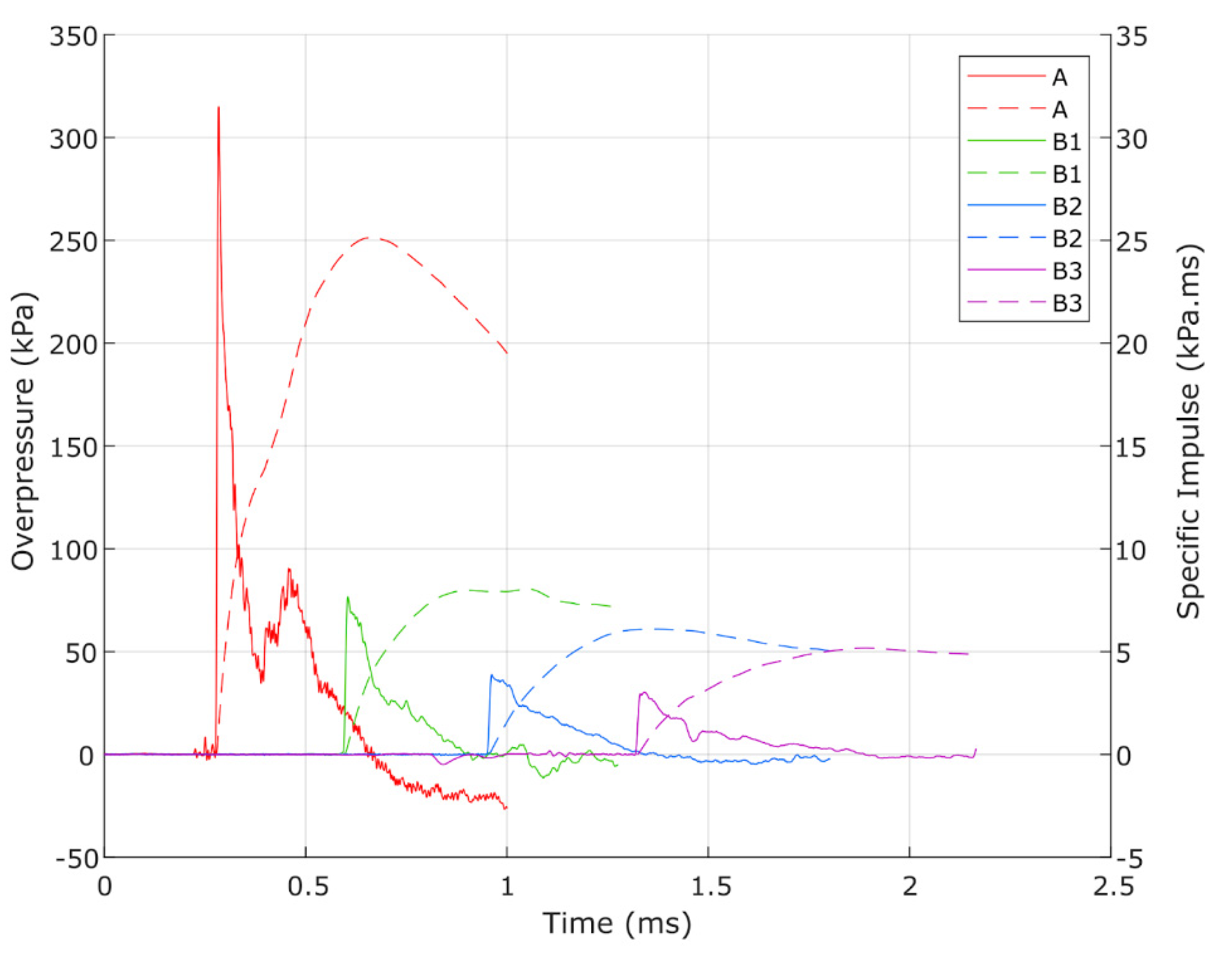
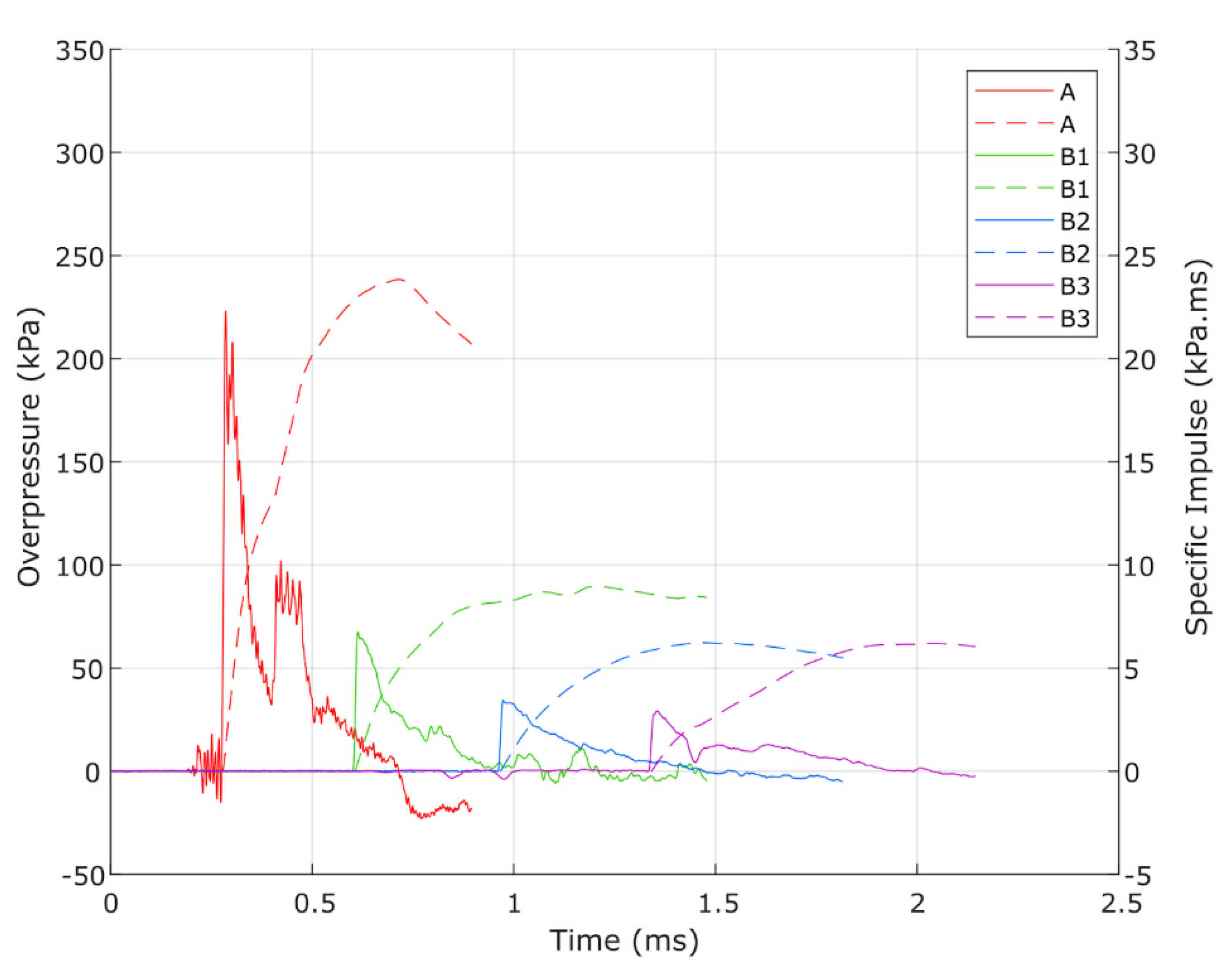
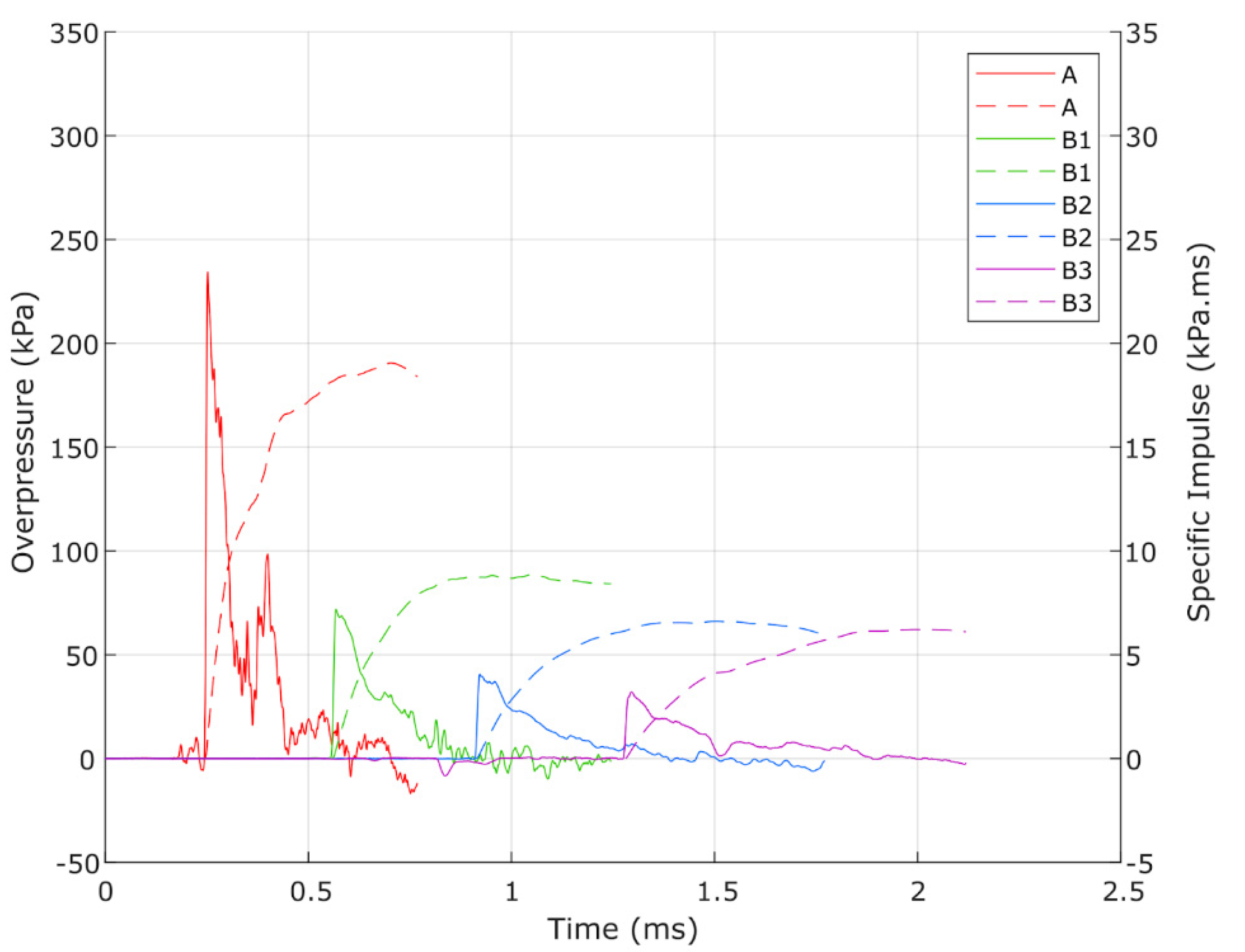
Appendix B. Overpressure–Time Histories and Cumulative Specific Impulse at Each Gauge Location Measured in the 100% Experiments
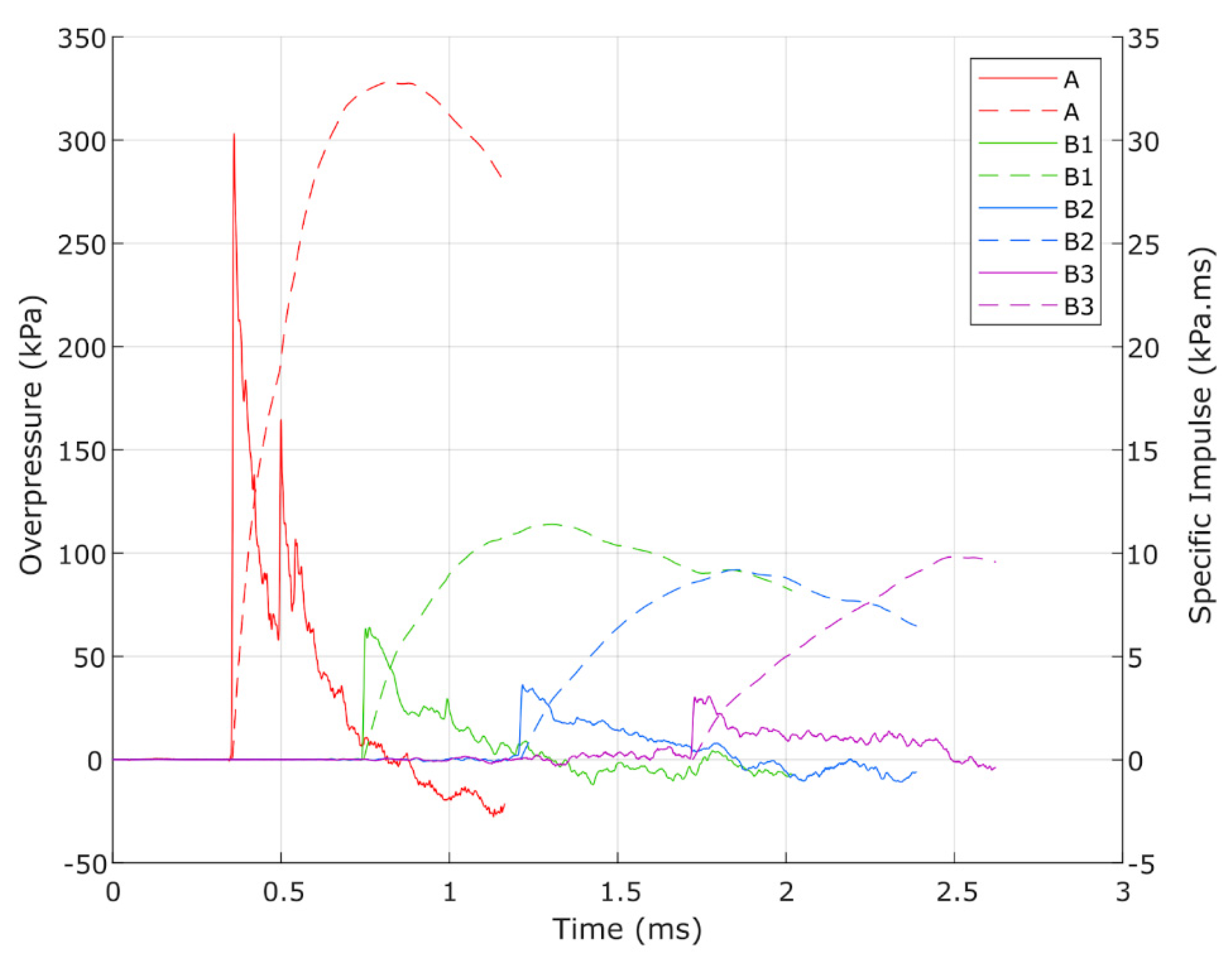
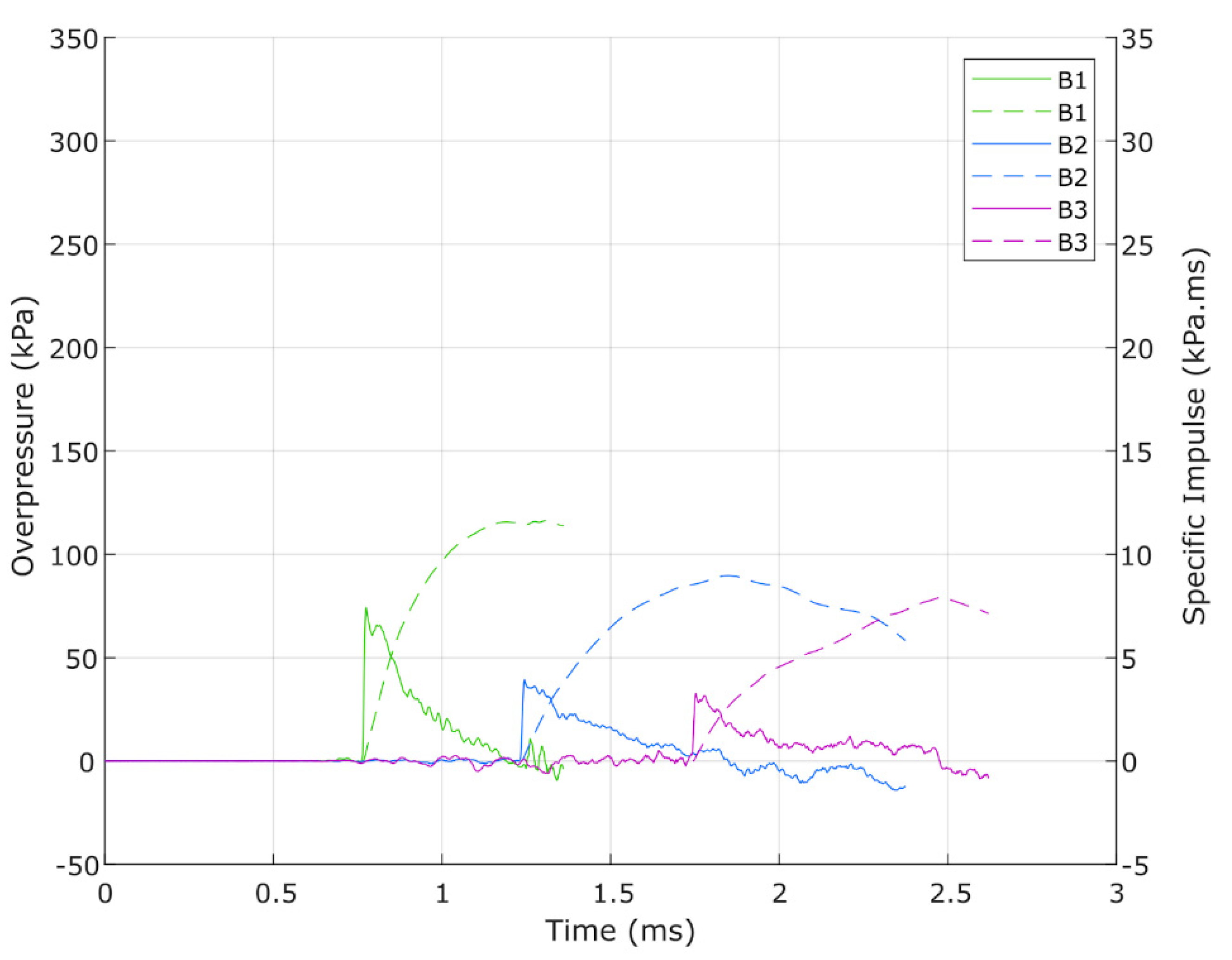

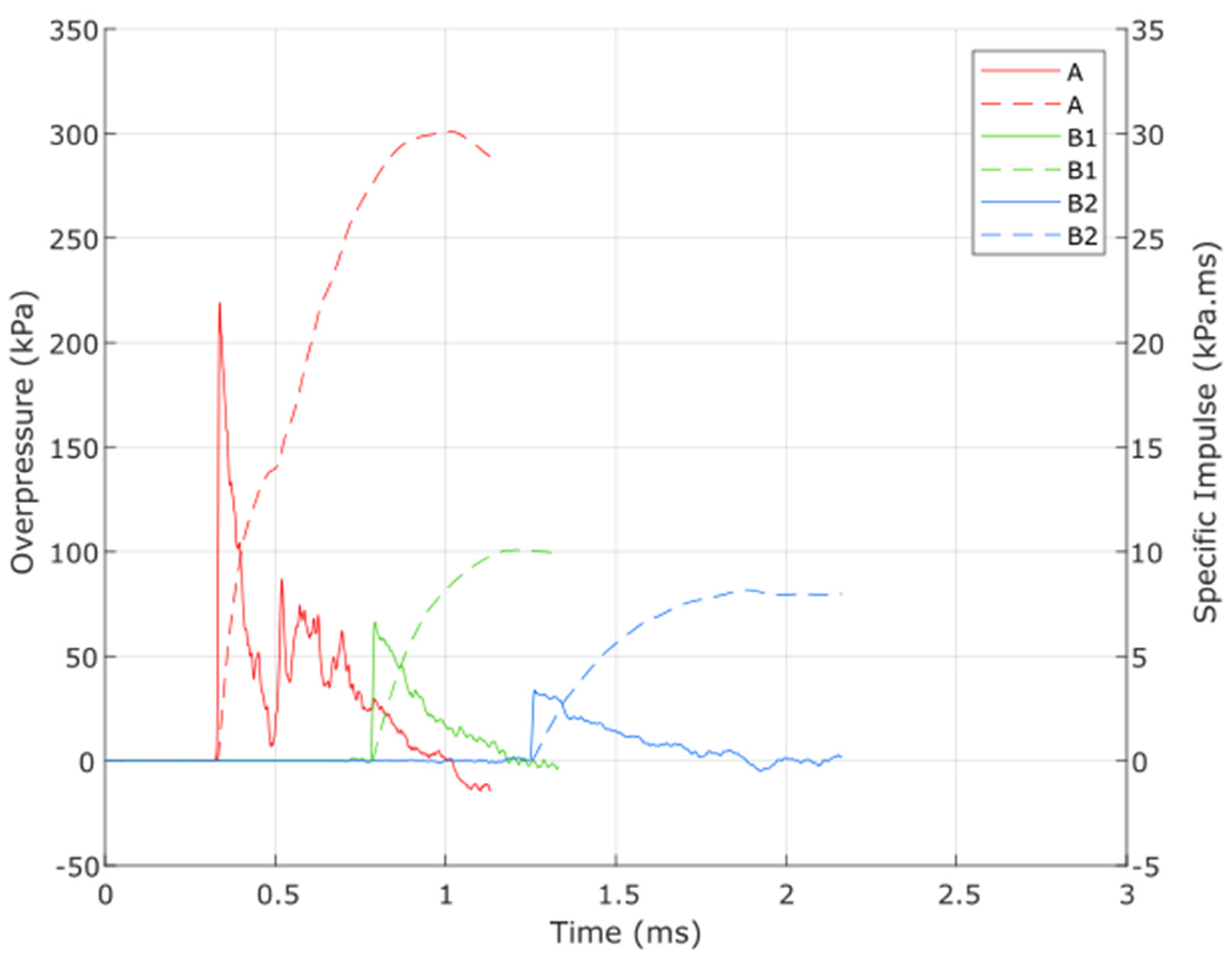
Appendix C. Overpressure–Time Histories and Cumulative Specific Impulse at Each Gauge Location Measured in the 75% Experiments

References
- United Nations. World Urbanization Prospects 2018: Highlights (ST/ESA/SER.A/421). 2020. Available online: https://www.un.org/development/desa/pd/sites/www.un.org.development.desa.pd-/files/wpp2022_summary_of_results.pdf (accessed on 10 November 2022).
- United Nations. World population Prospects 2022: Summary of Results. UN DESA/POP/2022/TR/NO. 3, Department of Economic and Social Affairs, Population Division, 2022. 2022. Available online: https://www.un.org/development/desa/pd/sites/www.un.org.development.desa.pd/files/wpp2022_sum-mary_of_results.pdf (accessed on 10 October 2022).
- Hayda, J.; Beaubien, J.; Lonsdorf, K.; Maynes, C.; Deadly Missile Strikes Hit Kyiv as Explosions Reported in Other Cities Across Ukraine. National Public Radio (NPR), 10 October 2022. Available online: https://www.npr.org/2022/10/10/1127794708/explosions-hit-kyiv-and-other-cities (accessed on 10 November 2022).
- Rigby, S.E.; Lodge, T.J.; Alotaibi, S.; Barr, A.D.; Clarke, S.D.; Langdon, G.S.; Tyas, A. Preliminary yield estimation of the 2020 Beirut explosion using video footage from social media. Shock Waves 2020, 30, 671–675. [Google Scholar] [CrossRef]
- Valsamos, G.; Larcher, M.; Casadei, F. Beirut explosion 2020: A case study for a large-scale urban blast simulation. Saf. Sci. 2021, 137, 105190. [Google Scholar] [CrossRef]
- Banda, M. Death Toll in Boksburg Gas Tanker Explosion Rises to 41 as Class Action Gathers Steam, Daily Maverick South Africa, Boksburg, 23 January 2023. Available online: https://www.dailymaverick.co.za-/article/2023-01-23-death-toll-in-boksburg-gas-tanker-explosion-rises-to-41-as-class-action-gathers-steam/ (accessed on 29 March 2023).
- AOAV. Action on Armed Violence, ‘Explosive Vviolence Monitor 2019: Initial Findings’. 2019. Available online: https://aoav.org.uk/2020/explosive-violence-in-2019/ (accessed on 10 October 2022).
- Baker, W.E.; Cox, P.A.; Westine, P.S.; Kulesz, J.J.; Strehlow, R.A. Chapter 2—Free-field explosions and their characteristics. In Fundamental Studies in Engineering; Elsevier: Amsterdam, The Netherlands, 1983; pp. 106–221. [Google Scholar]
- Held, M. Blast Waves in Free Air. Propellants Explos. Pyrotech. 1983, 8, 1–7. [Google Scholar] [CrossRef]
- Kinney, G.F.; Graham, K.J. Explosive Shocks in Air; Springer: Berlin/Heidelberg, Germany, 1985. [Google Scholar] [CrossRef]
- Gajewski, T.; Sielicki, P.W. Experimental study of blast loading behind a building corner. Shock Waves 2020, 30, 385–394. [Google Scholar] [CrossRef]
- Heggelund, S.; Brekken, K.; Ingier, P.; Christensen, S.O. Global response of a three-story building exposed to blast loading. Proceedings 2018, 2, 386. [Google Scholar] [CrossRef]
- Rose, T.A.; Smith, P.D.; May, J.H. The interaction of oblique blast waves with buildings. Shock Waves 2006, 16, 35–44. [Google Scholar] [CrossRef]
- Xiao, W.; Andrae, M.; Steyerer, M.; Gebbeken, N. Investigations of blast loads on a two-storeyed building with a gable roof: Full-scale experiments and numerical study. J. Build. Eng. 2021, 43, 103111. [Google Scholar] [CrossRef]
- Sugiyama, Y.; Izumo, M.; Ando, H.; Matsuo, A. Two-dimensional explosion experiments examining the interaction between a blast wave and a sand hill. Shock Waves 2018, 28, 627–630. [Google Scholar] [CrossRef]
- Jin, M.; Hao, Y.; Hao, H. Numerical study of fence type blast walls for blast load mitigation. Int. J. Impact Eng. 2019, 131, 238–255. [Google Scholar] [CrossRef]
- Warnstedt, P.; Gebbeken, N. Innovative protection of urban areas—Experimental research on the blast mitigating potential of hedges. Landsc. Urban Plan. 2020, 202, 103876. [Google Scholar] [CrossRef]
- Ghajari, Y.E.; Alesheikh, A.A.; Modiri, M.; Hosnavi, R.; Abbasi, M.; Sharifi, A. Urban vulnerability under various blast loading scenarios: Analysis using GIS-based multi-criteria decision analysis techniques. Cities 2018, 72, 102–114. [Google Scholar] [CrossRef]
- Shi, Y.; Liu, S.; Li, Z.; Ding, Y. Review on quick safety assessment of building structures in complex urban environment after extreme explosion events. In International Journal of Protective Structures; Sage Publications Inc.: Thousand Oaks, CA, USA, 2022. [Google Scholar] [CrossRef]
- Smith, P.D.; Rose, T.A. Blast wave propagation in city streets—An overview. Prog. Struct. Eng. Mater. 2005, 8, 16–28. [Google Scholar] [CrossRef]
- Rose, T.; Smith, P. Influence of the principal geometrical parameters of straight city streets on positive and negative phase blast wave impulses. Int. J. Impact Eng. 2002, 27, 359–376. [Google Scholar] [CrossRef]
- Isaac, O.S.; Alshammari, O.G.; Pickering, E.G.; Clarke, S.D.; Rigby, S.E. Blast wave interaction with structures—An overview. In International Journal of Protective Structures; Sage Publications Inc.: Thousand Oaks, CA, USA, 2022. [Google Scholar] [CrossRef]
- Ratcliff, A.; Rigby, S.; Clarke, S.; Fay, S. A Review of Blast Loading in the Urban Environment. Appl. Sci. 2023, 13, 5349. [Google Scholar] [CrossRef]
- Denny, J.; Langdon, G.; Rigby, S.; Dickinson, A.; Batchelor, J. A numerical investigation of blast-structure interaction effects on primary blast injury risk and the suitability of existing injury prediction methods. Int. J. Prot. Struct. 2022, Preprint. [Google Scholar] [CrossRef]
- Cranz, C.; Poppenberg, O.; von Eberhard, O. (Eds.) Innere Ballistik; Springer: Berlin/Heidelberg, Germany, 1926. [Google Scholar] [CrossRef]
- Hopkinson, B. British Ordnance Board Minutes, 13565; The National Archive: Kew, UK, 1926. [Google Scholar]
- Rigby, S.E.; Sielicki, P.W. An investigation of TNT equivalence of hemispherical pe4 charges. Eng. Trans. 2014, 62, 423–435. [Google Scholar]
- PCB Piezotronics, ICP Pressure Sensor Model 113B28 Product Specifications. 2021. Available online: https://www.pcb.com-/contentStore/docs/pcb_corporate/pressure/products/specsheets/113b28_f.pdf (accessed on 1 March 2022).
- PCB Piezotronics, ICP Pressure Sensor Model 113B27 Product Specifications. 2021. Available online: https://www.pcb.com-/contentStore/-docs/pcb_corporate/pressure/products/specsheets/113b27_f.pdf (accessed on 1 March 2022).
- PCB Piezotronics, ICP Pressure Sensor Model 113B21 Product Specifications. 2021. Available online: https://www.pcb.com-/contentStore/-docs/pcb_corporate/pressure/products/specsheets/113b21_f.pdf (accessed on 1 March 2022).
- Stirling, C. Viper::Blast; Stirling Simulation Services Limited: Edinburgh, Scotland, UK, 2021. [Google Scholar]
- Rose, T.A. An Approach to the Evaluation of Blast Loads on Finites and Semi-Infinite Structures. Ph.D. Thesis, Cranfield University, Cranfield, UK, 2010. [Google Scholar]
- Wada, Y.; Liou, M.-S. An Accurate and Robust Flux Splitting Scheme for Shock and Contact Discontinuities. SIAM J. Sci. Comput. 1997, 18, 633–657. [Google Scholar] [CrossRef]
- Dobratz, B.M.; Crawford, P.C. LLNL Explosives handbook, properties of chemical explosives and simulants. In Report UCRL-52997; Livermore National Laboratory: Livermore, CA, USA, 1985. [Google Scholar]
- Lee, E.L.; Hornig, H.C.; Kury, J.W. Adiabatic Expansion of High Explosive Detonation Products; University of California Radiation Laboratory at Livermore: Livermore, CA, USA, 1968. [Google Scholar]
- Zhou, X.; Hao, H. Prediction of airblast loads on structures behind a protective barrier. Int. J. Impact Eng. 2008, 35, 363–375. [Google Scholar] [CrossRef]
- Sung, S.-H.; Chong, J.-W. A fast-running method for blast load prediction shielding by a protective barrier. Def. Technol. 2020, 16, 308–315. [Google Scholar] [CrossRef]
- Rigby, S.E.; Tyas, A.; Bennett, T.; Fay, S.D.; Clarke, S.; Warren, J.A. A Numerical Investigation of Blast Loading and Clearing on Small Targets. Int. J. Prot. Struct. 2014, 5, 253–274. [Google Scholar] [CrossRef]
- Xiao, W.; Andrae, M.; Gebbeken, N. Influence of charge shape and point of detonation of high explosive cylinders detonated on ground surface on blast-resistant design. Int. J. Mech. Sci. 2020, 181, 105697. [Google Scholar] [CrossRef]
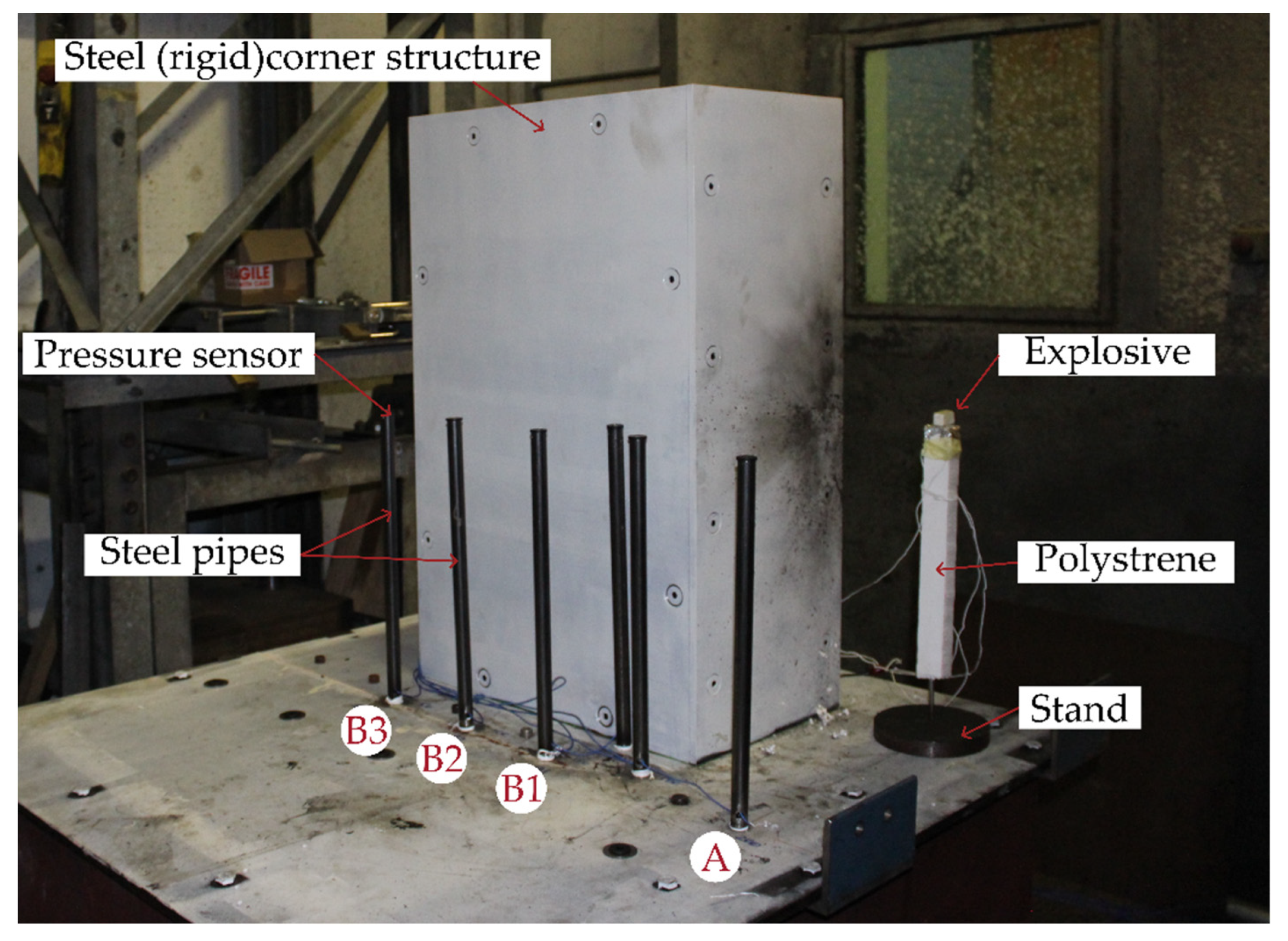
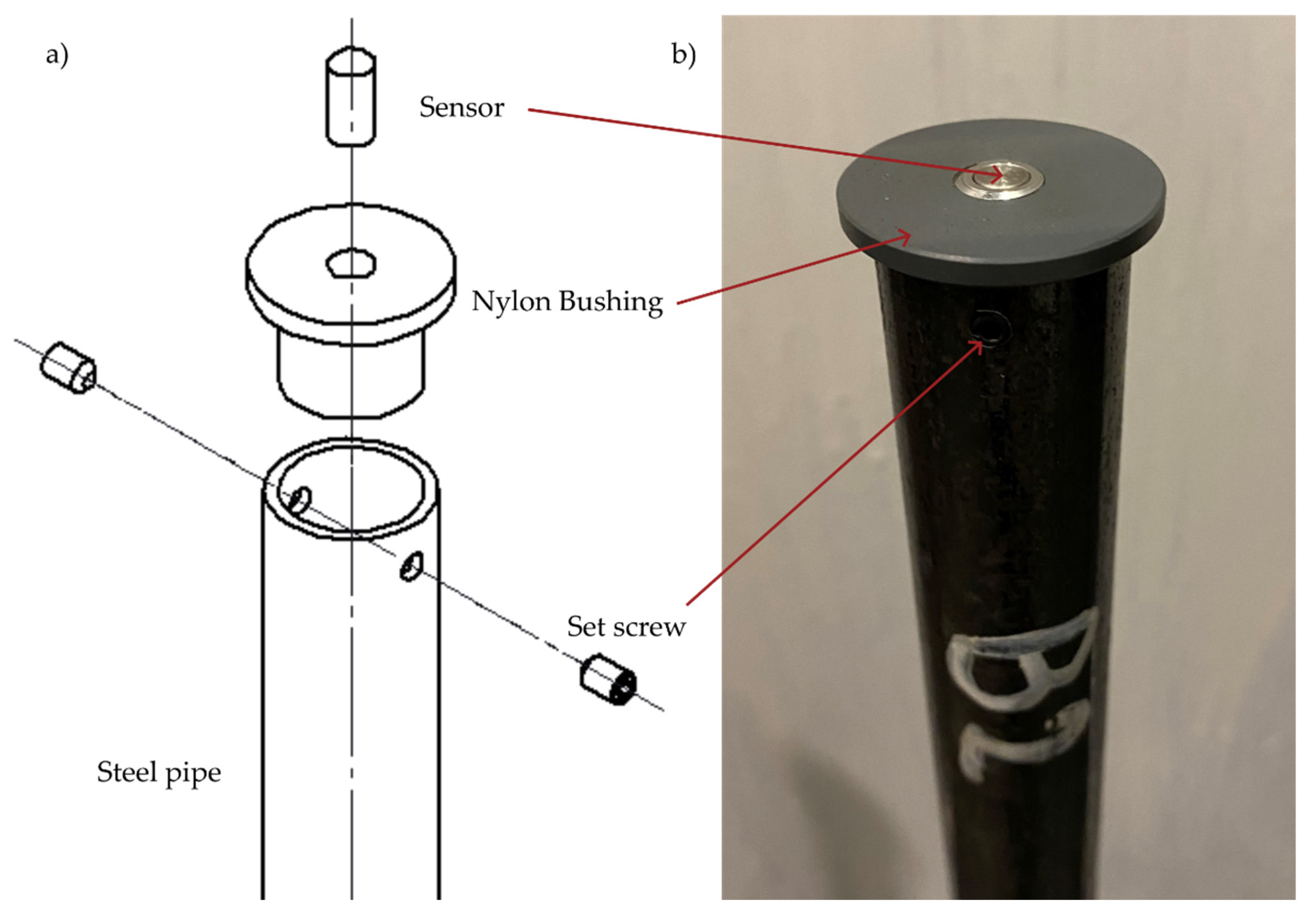



| Sensor Model | Maximum Operating Pressure | Sensitivity | Allocated Gauge | |
|---|---|---|---|---|
| PCB 113B28 [28] | 50 psi | 344 kPa | 100 mV/psi | B3 |
| PCB 113B27 [29] | 100 psi | 689 kPa | 50 mV/psi | B1, B2 |
| PCB 113B21 [30] | 200 psi | 1378 kPa | 25 mV/psi | A |
| (a) 75% | Mean [Range], Standard Deviation | |||
|---|---|---|---|---|
| Gauge | Pi (kPa) | ta (ms) | t+ (ms) | I+ (kPa.ms) |
| A | 274 [323–223], 52 | 0.26 [0.28–0.24], 0.02 | 0.40 [0.44–0.36], 0.04 | 22.5 [25.1–18.5], 3.5 |
| A(2nd peak) | 97 [102–90], 6 | 0.43 [0.46–0.40], 0.03 | 0.23 [0.29–0.20], 0.05 1 | 15.7 [18.1–14.5], 2.0 2 |
| B1 | 70 [77–66], 5 | 0.60 [0.67–0.56], 0.05 | 0.34 [0.35–0.32], 0.02 | 8.3 [8.7–8.0], 0.3 |
| B2 | 38 [41–34], 3 | 0.96 [1.03–0.91], 0.05 | 0.47 [0.51–0.43], 0.04 | 6.3 [6.5–6.1], 0.2 |
| B3 | 30 [32–28], 2 | 1.33 [1.40–1.28], 0.05 | 0.59 [0.63–0.57], 0.03 | 5.9 [6.2–5.2], 0.5 |
| (b) 100% | Mean [Range], Standard Deviation | |||
| Gauge | Pi (kPa) | ta (ms) | t+ (ms) | I+ (kPa.ms) |
| A | 254 [303–219], 44 | 0.33 [0.35–0.32], 0.02 | 0.58 [0.69–0.46], 0.16 | 31.2 [32.8–29.5], 2.3 |
| A(2nd peak) | 125 [165–86], 55 | 0.51 [0.52–0.50], 0.01 | 0.41 [0.52–0.32], 0.13 1 | 16.6 [19.1–14.0], 3.6 2 |
| B1 | 69 [74–63], 5 | 0.76 [0.78–0.74], 0.02 | 0.46 [0.56–0.42], 0.07 | 10.9 [11.6–10.1], 0.7 |
| B2 | 37 [39–34], 2 | 1.23 [1.24–1.21], 0.02 | 0.64 [0.65–0.62], 0.02 | 8.7 [9.2–8.2], 0.4 |
| B3 | 31 [33–29], 2 | 1.73 [1.75–1.72], 0.02 | 0.76 [0.78–0.73], 0.03 | 8.9 [9.8–7.9], 1.4 |
| Gauge | Pi (kPa) | ta (ms) | t+ (ms) | I+ (kPa.ms) |
|---|---|---|---|---|
| Experiment A 100% mean [range] | 254 [303–219] | 0.33 [0.35–0.32] | 0.58 [0.69–0.46] | 31.2 [32.8–29.5] |
| Expected A from 75% | 274 | 0.35 | 0.53 | 30.0 |
| Difference | 8%; 20 | 7%; 0.02 | 8%; 0.05 | −4%; 1.2 |
| Experiment A2 100% mean [range] | 125 [165–86] | 0.51 [0.52–0.50] | 0.41 [0.52–0.32] 1 | 16.6 [19.1–14.0] 2 |
| Expected A2 from 75% | 97 | 0.57 | 0.31 1 | 21.0 2 |
| Difference | −23%; 29 | 12%; 0.06 | −23%; 0.09 | 27%; 4.4 |
| Experiment B1 100% mean [range] | 69 [74–63] | 0.76 [0.78–0.74] | 0.46 [0.56–0.42] | 10.9 [11.6–10.1] |
| Expected B1 from 75% | 70 | 0.80 | 0.45 | 11.1 |
| Difference | 2%; 2 | 5%; 0.04 | −2%; 0.01 | 2%; 0.2 |
| Experiment B2 100% mean [range] | 37 [39–34] | 1.23 [1.24–1.21] | 0.64 [0.65–0.62] | 8.7 [9.2–8.2] |
| Expected B2 from 75% | 38 | 1.28 | 0.63 | 8.4 |
| Difference | 3%; 1 | 4%; 0.05 | −2%; 0.01 | −3%; 0.3 |
| Experiment B3 100% mean [range] | 31 [33–29] | 1.73 [1.75–1.72] | 0.76 [0.78–0.73] | 8.9 [9.8–7.9] |
| Expected B3 from 75% | 30 | 1.77 | 0.79 | 7.8 |
| Difference | −4%; 1 | 2%; 0.04 | 5%; 0.04 | −11%; 1.0 |
| Gauge | Pi (kPa) | ta (ms) | t+ (ms) | I+ (kPa.ms) |
|---|---|---|---|---|
| Experiment A 75% mean [range] | 274 [323–223] | 0.26 [0.28–0.24] | 0.40 [0.44–0.36] | 22.5 [25.1–18.5] |
| CFD Model | 150 | 0.33 | 0.34 | 21.3 |
| Difference | −45%; −124 | 27%; 0.07 | −16%; −0.06 | −5.3%; −1.2 |
| Experiment B1 75% mean [range] | 70 [77–66] | 0.60 [0.67–0.56] | 0.34 [0.35–0.32] | 8.3 [8.7–8.0] |
| CFD Model | 69 | 0.57 | 0.32 | 8.5 |
| Difference | −1%; −1 | −5%; −0.03 | −7%; −0.02 | 2%; 0.2 |
| Experiment B2 75% mean [range] | 38 [41–34] | 0.96 [1.03–0.91] | 0.47 [0.51–0.43] | 6.3 [6.5–6.1] |
| CFD Model | 38 | 0.92 | 0.46 | 7.2 |
| Difference | 0%; 0 | −4%; −0.04 | −2.4%; −0.01 | 14%; 0.9 |
| Experiment B3 75% mean [range] | 30 [32–28] | 1.33 [1.40–1.28] | 0.59 [0.63–0.57] | 5.9 [6.2–5.2] |
| CFD Model | 28 | 1.30 | 0.65 | 6.9 |
| Difference | −7%; −2 | 2%; 0.03 | 10%; 0.06 | 17%; 1.0 |
| Gauge | Pi (kPa) | ta (ms) | t+ (ms) | I+ (kPa.ms) |
|---|---|---|---|---|
| Experiment A 100% mean [range] | 254 [303–219] | 0.33 [0.35–0.32] | 0.58 [0.69–0.46] | 31.2 [32.8–29.5] |
| CFD Model | 151 | 0.44 | 0.44 | 27.1 |
| Difference | −41%; −103 | 33%; 0.11 | −24%; −0.14 | −13%; 4.1 |
| Experiment B1 100% mean [range] | 69 [74–63] | 0.76 [0.78–0.74] | 0.46 [0.56–0.42] | 10.9 [11.6–10.1] |
| CFD Model | 69 | 0.77 | 0.46 | 11.5 |
| Difference | 0%; 0 | 1%; 0.01 | −0%; 0 | 6%; 0.6 |
| Experiment B2 100% mean [range] | 37 [39–34] | 1.23 [1.24–1.21] | 0.64 [0.65–0.62] | 8.7 [9.2–8.2] |
| CFD Model | 38 | 1.23 | 0.60 | 9.1 |
| Difference | 3%; 1 | 0%; 0 | −6%; −0.04 | 5%; 0.4 |
| Experiment B3 100% mean [range] | 31 [33–29] | 1.73 [1.75–1.72] | 0.76 [0.78–0.73] | 8.9 [9.8–7.9] |
| CFD Model | 28 | 1.73 | 0.89 | 9.2 |
| Difference | −10%; −3 | 0%; 0 | 17%; 0.13 | 3%; 0.3 |
| Gauge | Pi (kPa) | ta (ms) | t+ (ms) | I+ (kPa.ms) |
|---|---|---|---|---|
| Experiment A 250% mean [range] | 206 [336–134] | 1.15 [1.17–1.12] | 1.38 [1.89–0.92] | 75.9 [95.7–58.3] |
| Expected A from 100% | 254 | 0.83 | 1.45 | 77.9 |
| Expected A from 75% | 274 | 0.88 | 1.33 | 74.9 |
| Percentage Difference | 23%; 33% | −28%; −23% | 5%; −4% | 3%; −1% |
| Experiment A2 250% mean [range] 1 | 106 [152–56|N/A] | 1.58 [1.75–1.44|N/A] | 0.93 [1.34–0.44|N/A] 2 | 42.0 [59.7–32.1|N/A] 3 |
| Expected A2 from 100% | 125 | 1.27 | 1.01 2 | 41.4 3 |
| Expected A2 from 75% | 97 | 1.43 | 0.78 2 | 52.5 3 |
| Percentage Difference | 18%; −9% | −19%; −10% | 9%; −16% | −1%; 25% |
| Experiment B1 250% mean [range] | 70 [90–74] | 1.93 [1.94–1.92] | 2.04 [1.75–2.34] | 38.5 [41.1–35.8] |
| Expected B1 from 100% | 69 | 1.90 | 1.14 | 27.2 |
| Expected B1 from 75% | 70 | 2.00 | 1.12 | 27.7 |
| Percentage Difference | −2%; 0% | −1%; 4% | −44%, −45% | −29%; −28% |
| Experiment B2 250% mean [range] | 42 [42–41] | 2.98 [3.31–2.58] | 2.15 [2.50–1.87] | 29.7 [34.4–25.8] |
| Expected B2 from 100% | 37 | 3.07 | 1.60 | 21.7 |
| Expected B2 from 75% | 38 | 3.21 | 1.57 | 21.0 |
| Percentage Difference | −12%; −9% | 3%; 7% | −25%; −27% | −27%; −29% |
| Experiment B3 250% mean [range] | 36 [37–35] | 4.22 [4.50–3.93] | 1.49 [single data point] | 24.2 [single data point] |
| Expected B3 from 100% | 31 | 4.34 | 1.89 | 22.14 |
| Expected B3 from 75% | 30 | 4.44 | 1.98 | 19.81 |
| Percentage Difference | −14%; −17% | 3%; 5% | 27%; 33% | −4%; −15% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gabriel, S.; Denny, J.; Chung Kim Yuen, S.; Langdon, G.S.; Govender, R.A. The Effect of Scaling Building Configuration Blast Experiments on Positive Phase Blast Wave Parameters. Appl. Sci. 2023, 13, 5956. https://doi.org/10.3390/app13105956
Gabriel S, Denny J, Chung Kim Yuen S, Langdon GS, Govender RA. The Effect of Scaling Building Configuration Blast Experiments on Positive Phase Blast Wave Parameters. Applied Sciences. 2023; 13(10):5956. https://doi.org/10.3390/app13105956
Chicago/Turabian StyleGabriel, Sherlyn, Jack Denny, Steeve Chung Kim Yuen, Genevieve S. Langdon, and Reuben A. Govender. 2023. "The Effect of Scaling Building Configuration Blast Experiments on Positive Phase Blast Wave Parameters" Applied Sciences 13, no. 10: 5956. https://doi.org/10.3390/app13105956
APA StyleGabriel, S., Denny, J., Chung Kim Yuen, S., Langdon, G. S., & Govender, R. A. (2023). The Effect of Scaling Building Configuration Blast Experiments on Positive Phase Blast Wave Parameters. Applied Sciences, 13(10), 5956. https://doi.org/10.3390/app13105956







