Bending Behavior of a Frictional Single-Layered Spiral Strand Subjected to Multi-Axial Loads: Numerical and Experimental Investigation
Abstract
:1. Introduction
2. Materials and Methods
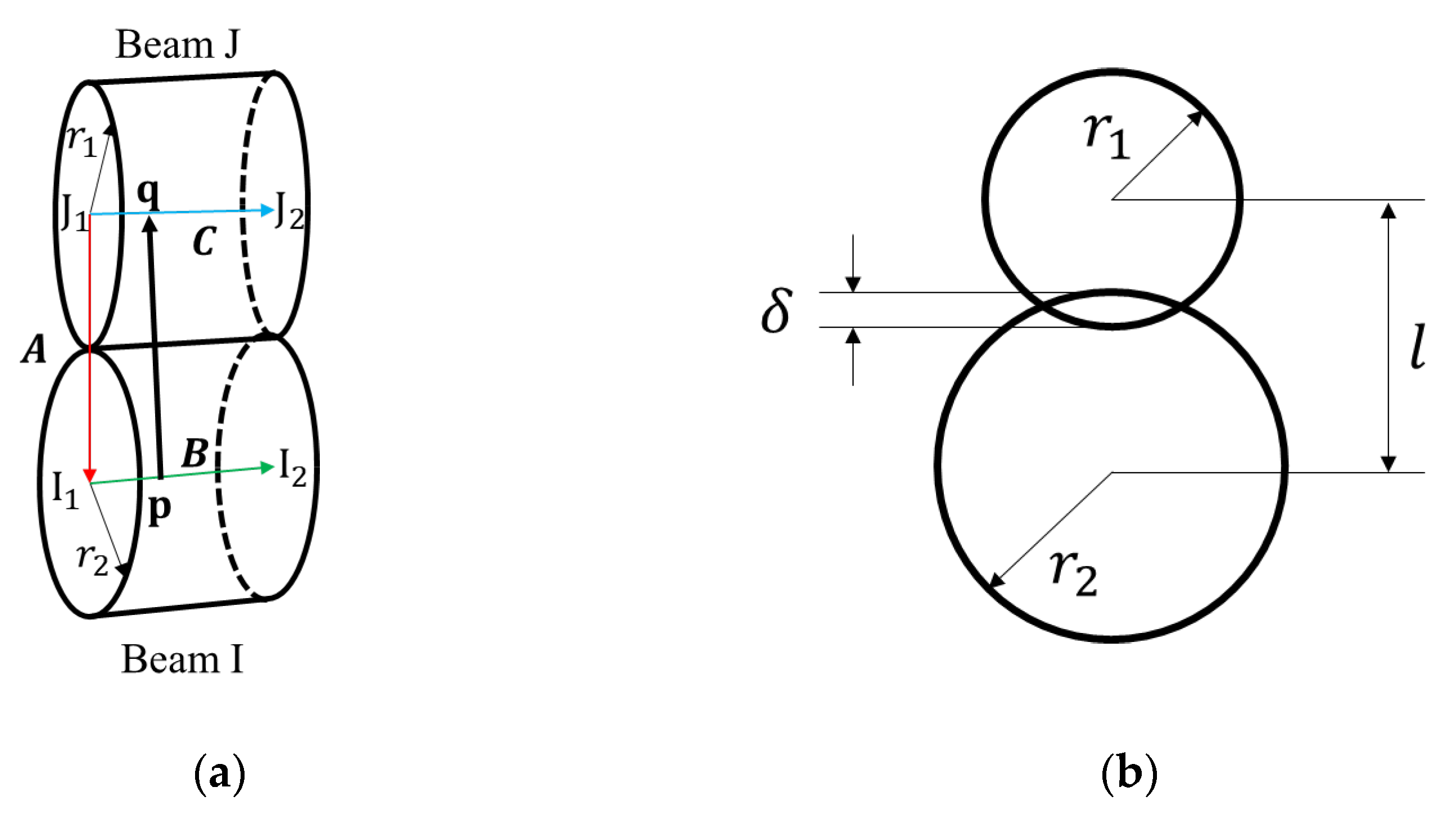
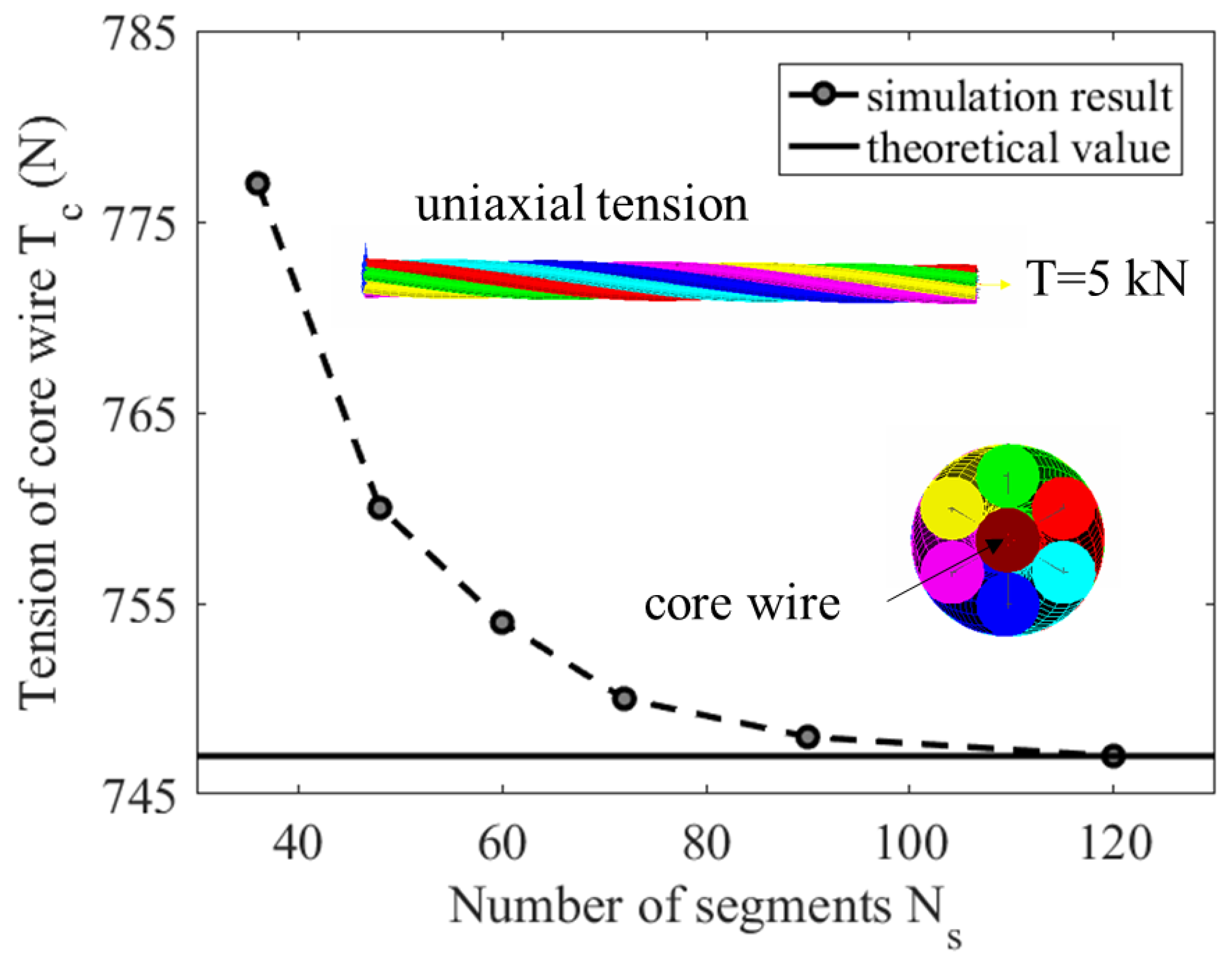
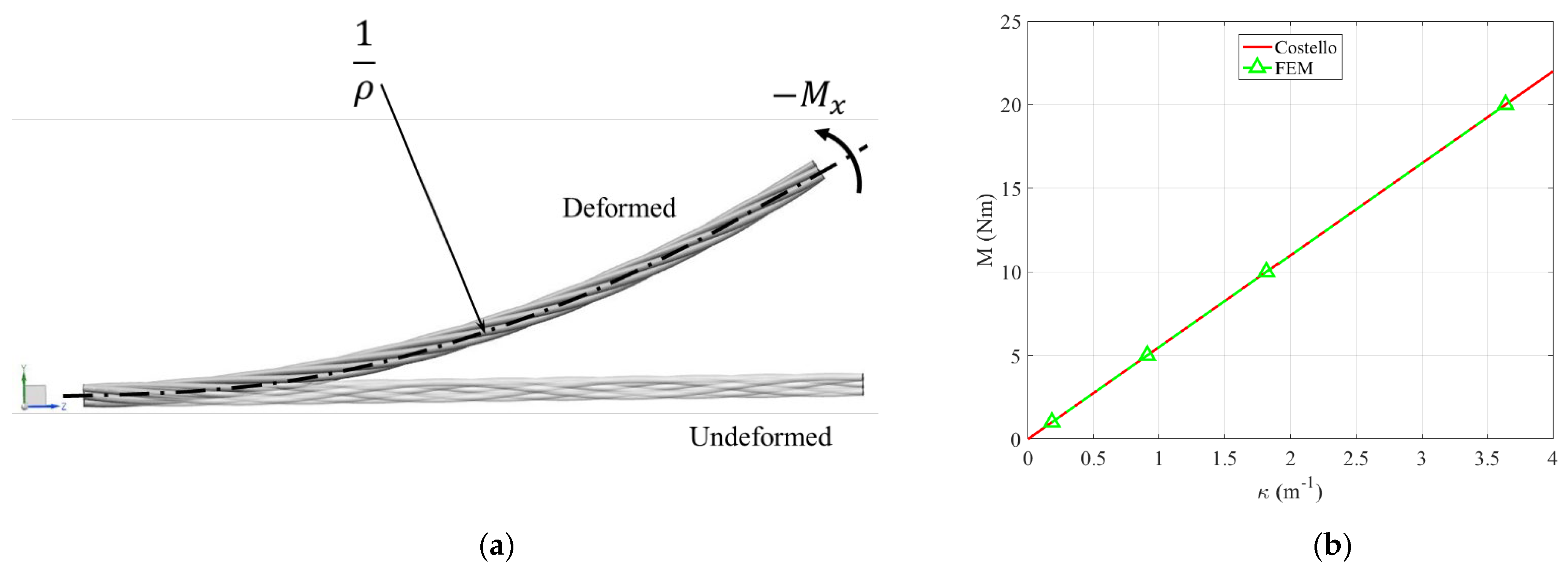
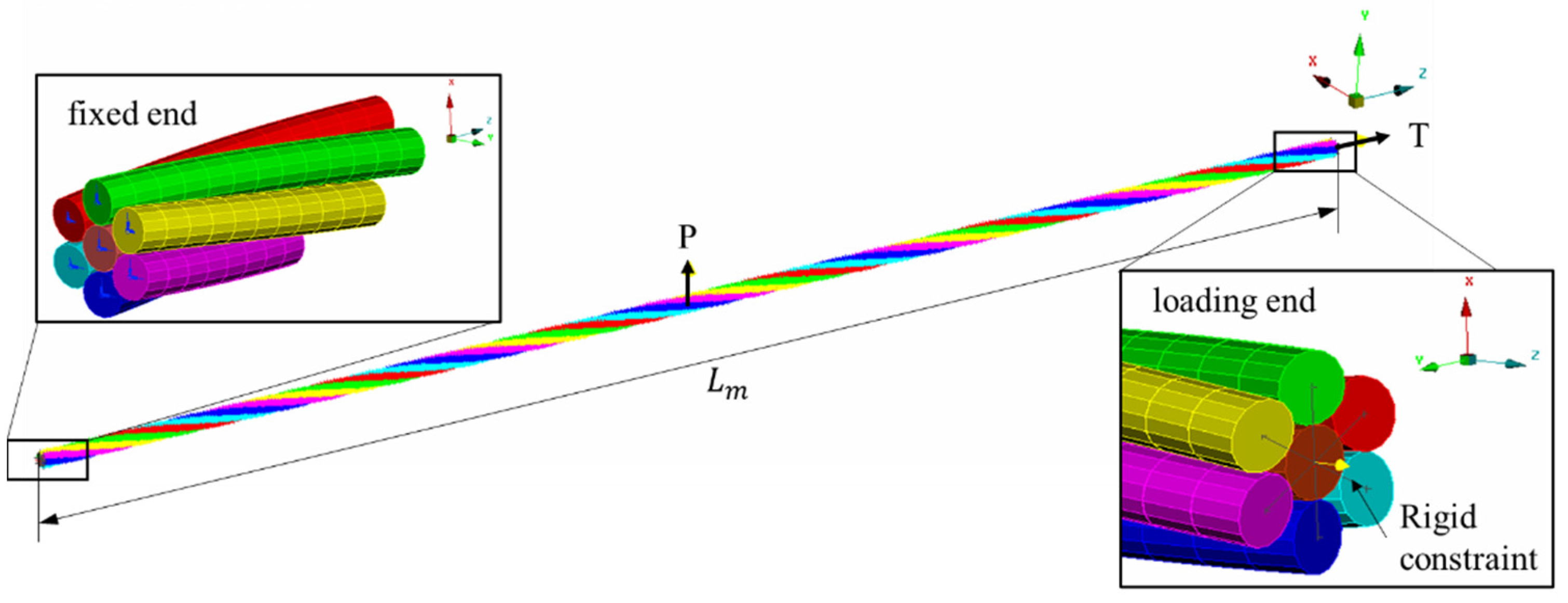
2.1. A Beam FE Modeling Strategy for a Simple Strand under Multi-Axial Loads
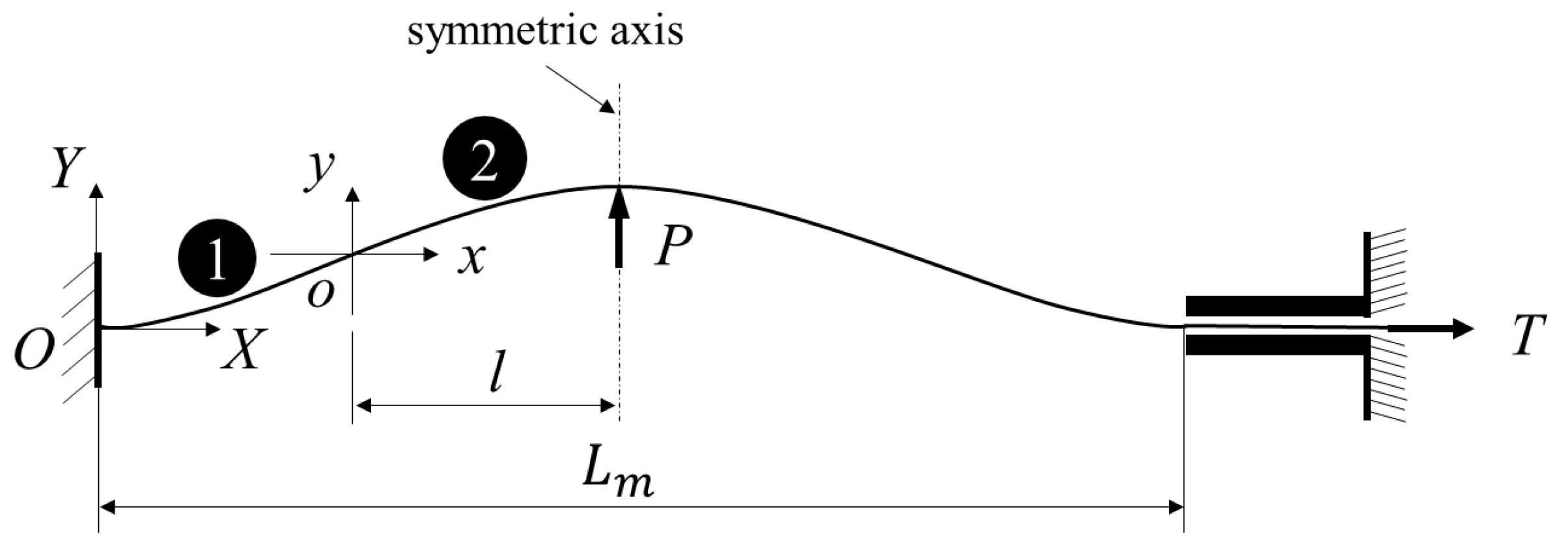
2.2. Approximation of the Deflection of the Simple Strand under Multi-Axial Loadings
2.3. Approximation of Tension for Individual Wire of the Simple Strand under Multi-Axial Loadings
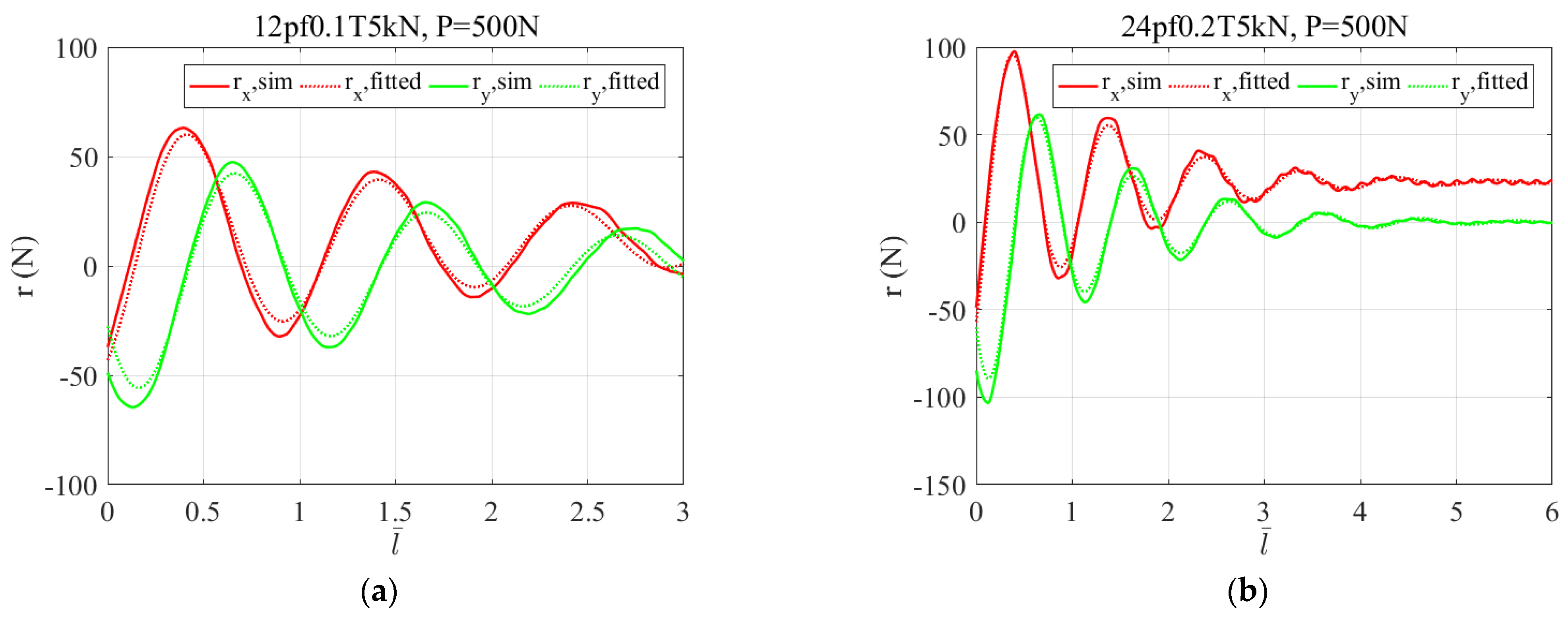
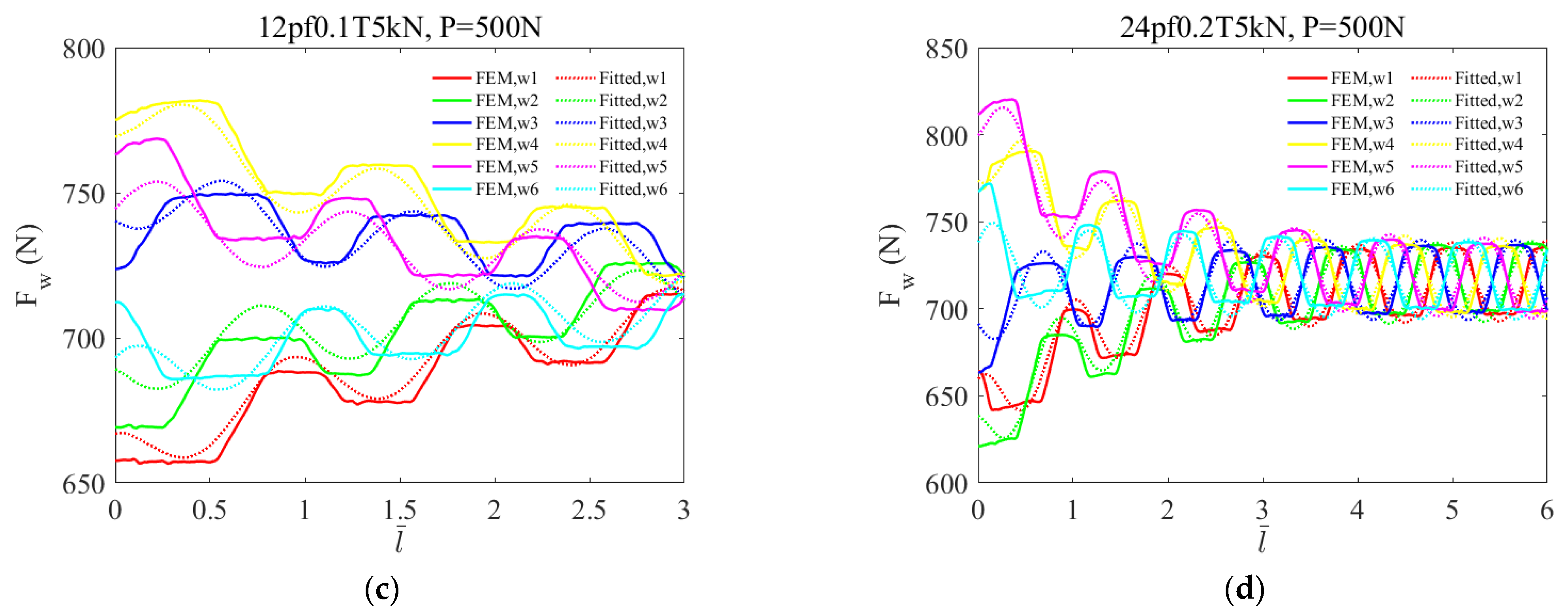
2.4. Experimental Measurement
3. Results and Discussion
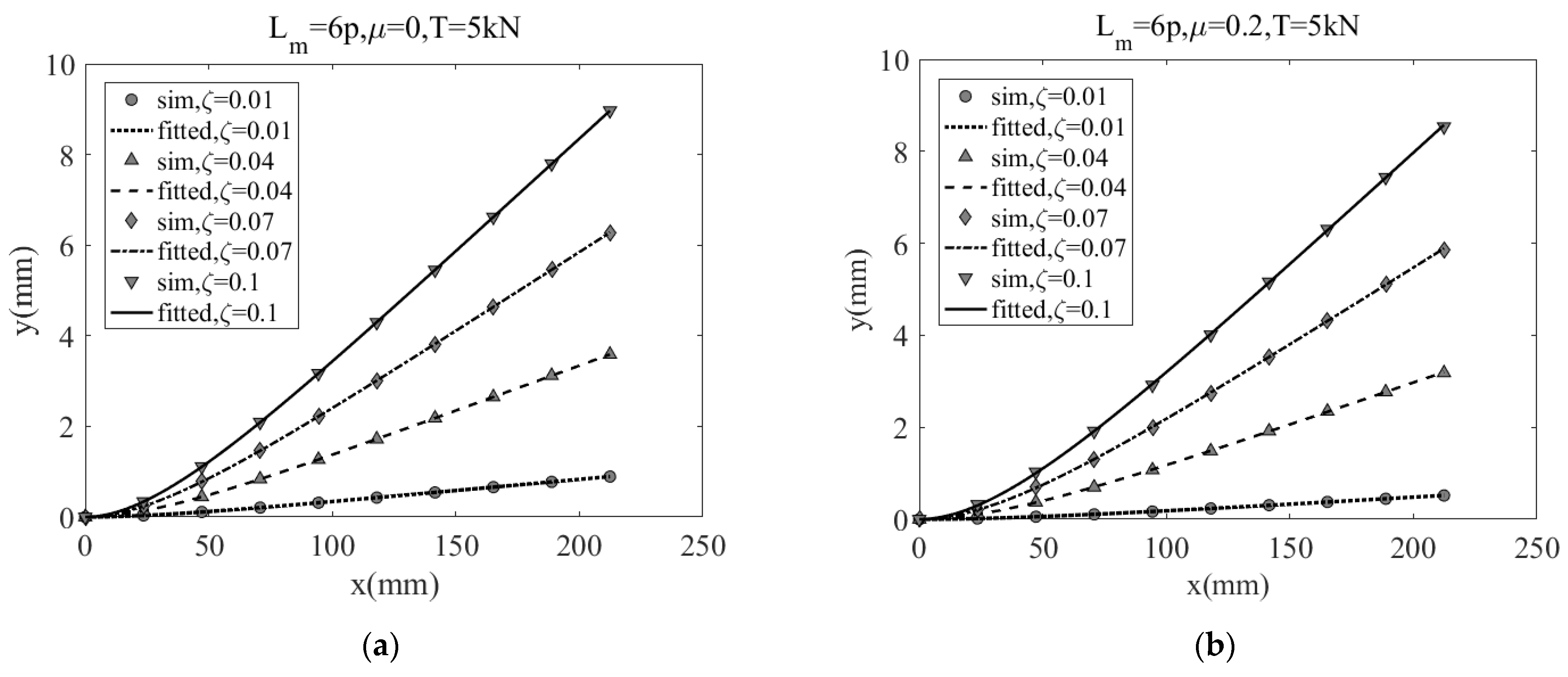
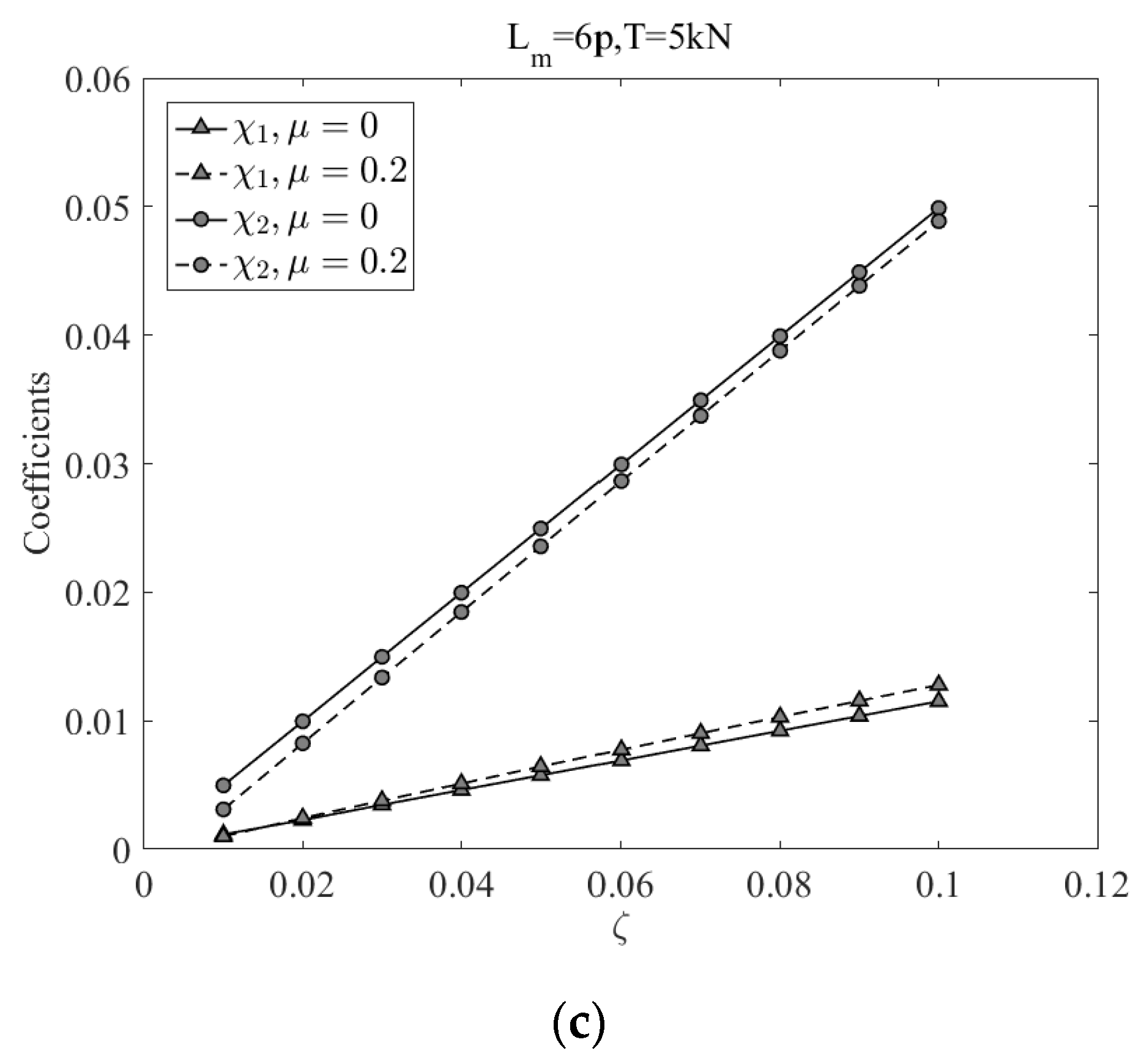
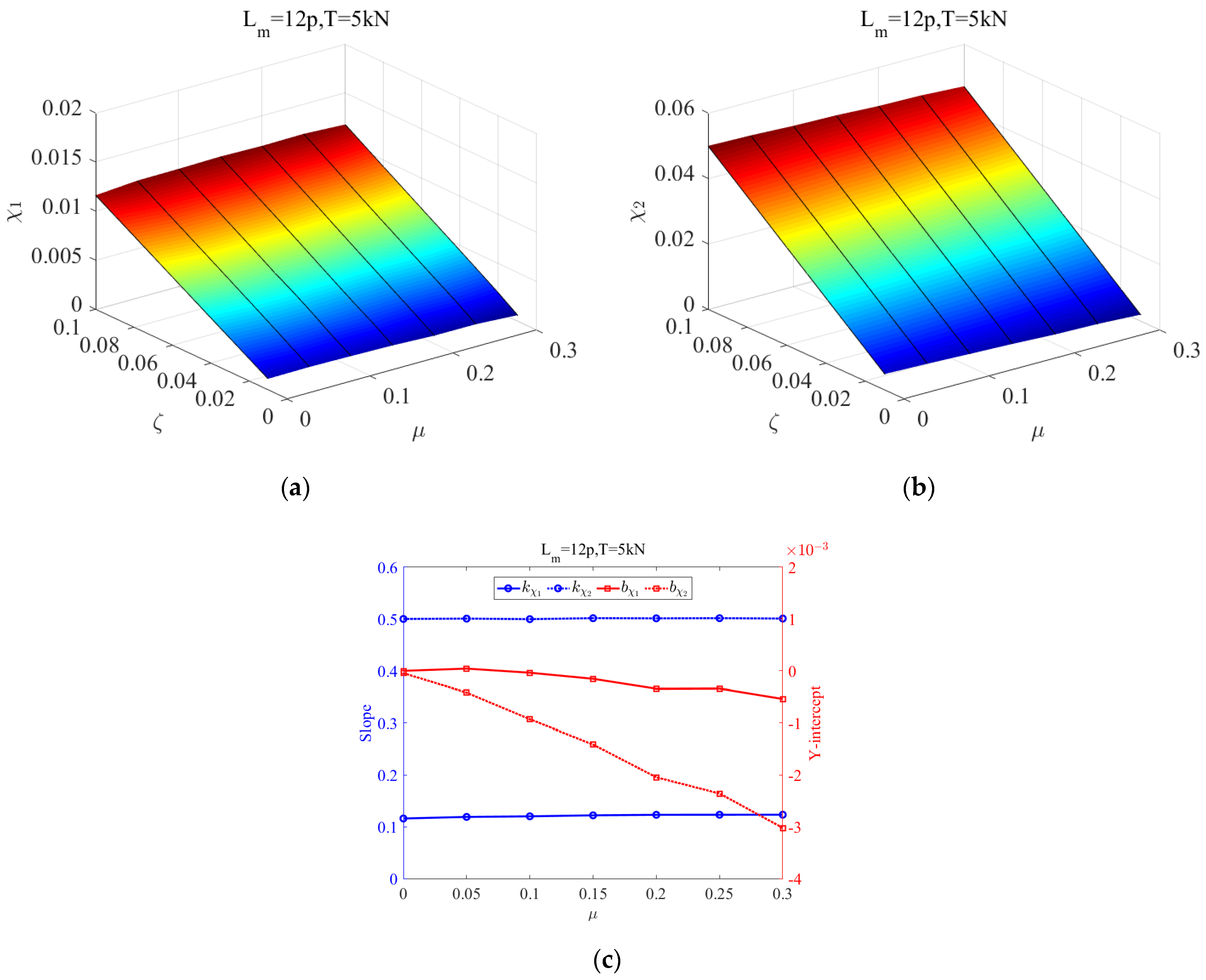
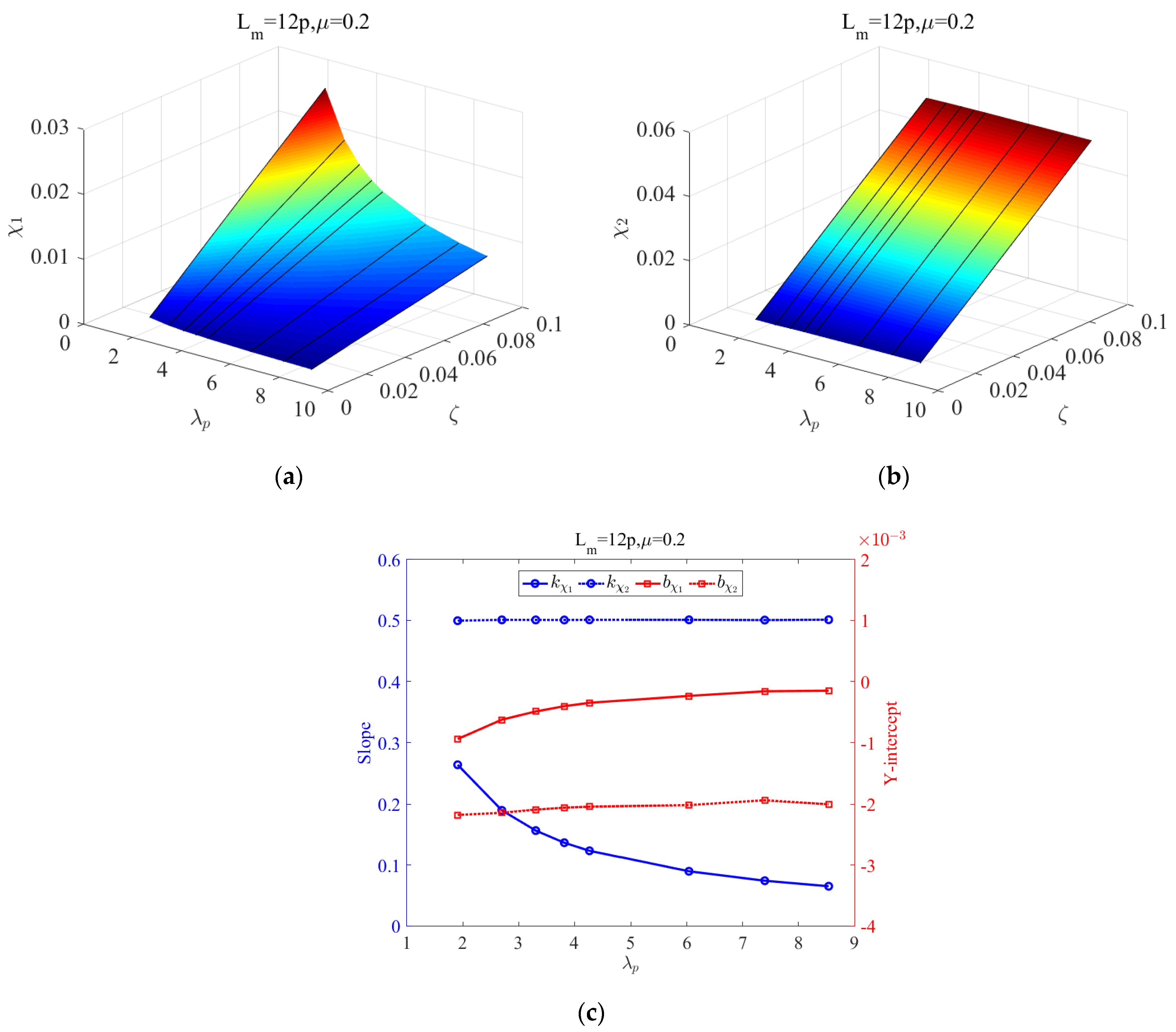
3.1. Effects of Length, Friction and Tension on the Deflection for Simple Strands
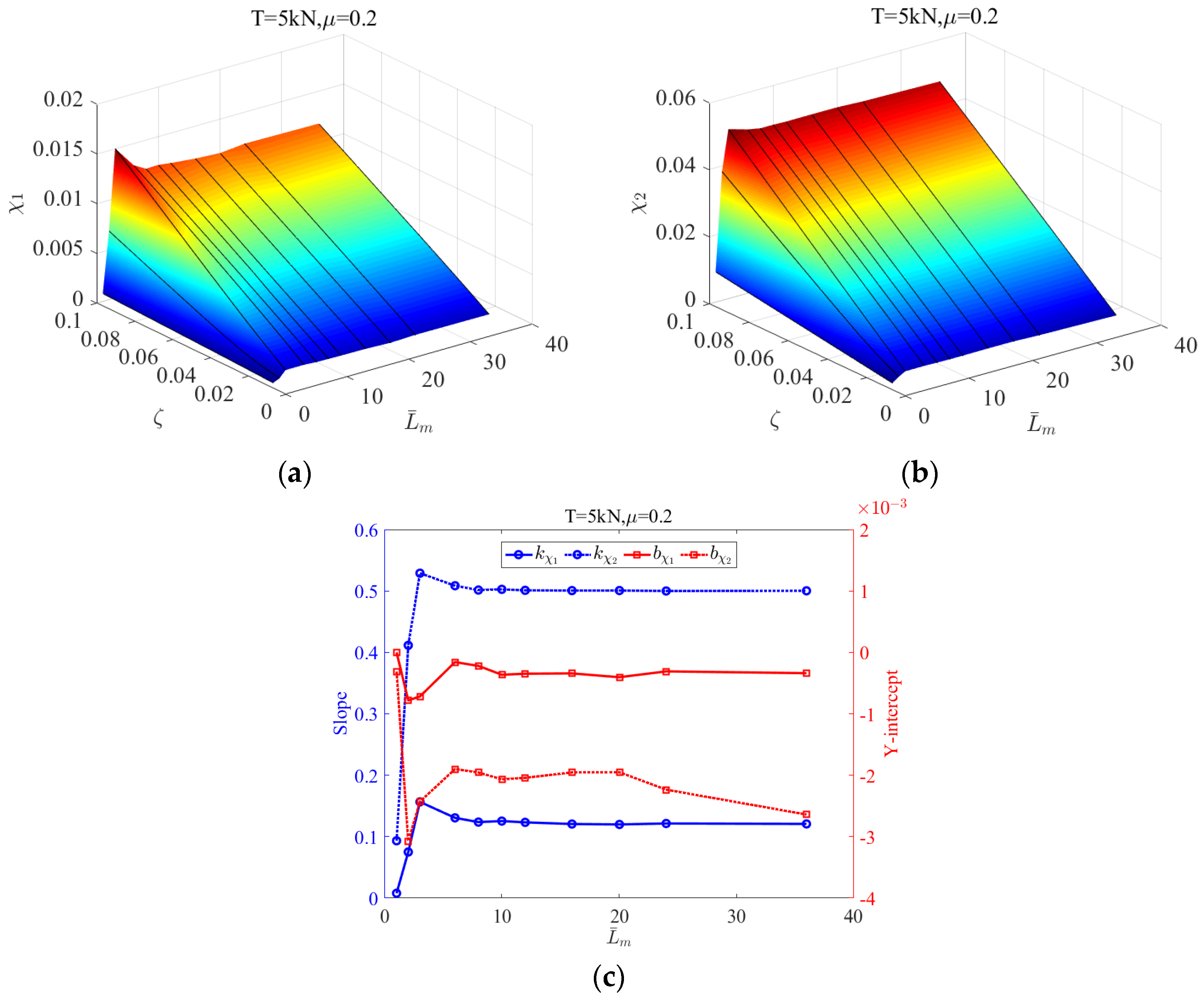
3.2. An Analytical Model from Papailiou’s Theory and Comparision Analysis
3.3. Tension Estimation for Frictional Strand under Different Loads
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ni, Y.Q.; Ko, J.M.; Zheng, G. Dynamic analysis of large-diameter sagged cables taking into account flexural rigidity. J. Sound Vib. 2002, 257, 301–319. [Google Scholar] [CrossRef]
- Levesque, F.; Goudreau, S.; Langlois, S.; Legeron, F. Experimental study of dynamic bending stiffness of ACSR overhead conductors. IEEE Trans. Power Deliv. 2015, 30, 2252–2259. [Google Scholar] [CrossRef] [Green Version]
- Langlois, S.; Legeron, F.; Levesque, F. Time history modeling of vibrations on overhead conductors with variable bending stiffness. IEEE Trans. Power Deliv. 2014, 29, 607–614. [Google Scholar] [CrossRef]
- Zhu, Z.H.; Meguid, S.A. Nonlinear FE-based investigation of flexural damping of slacking wire cables. Int. J. Solids Struct. 2007, 44, 5122–5132. [Google Scholar] [CrossRef] [Green Version]
- Foti, F.; Martinelli, L.; Perotti, F. A new approach to the definition of self-damping for stranded cables. Meccanica 2016, 51, 2827–2845. [Google Scholar] [CrossRef]
- Dastous, J.B. Nonlinear finite-element analysis of stranded conductors with variable bending stiffness using the tangent stiffness method. IEEE Trans. Power Deliv. 2005, 20, 328–338. [Google Scholar] [CrossRef]
- McConnell, K.G.; Zemke, W.P. The measurement of flexural stiffness of multistranded electrical conductors while under tension. Exp. Mech. 1980, 20, 198–204. [Google Scholar] [CrossRef]
- Filiatrault, A.; Christopher, S. Flexural properties of flexible conductors interconnecting electrical substation equipment. J. Struct. Eng. 2005, 131, 151–159. [Google Scholar] [CrossRef]
- Chen, Z.; Yu, Y.; Wang, X.; Wu, X.; Liu, H. Experimental research on bending performance of structural cable. Constr. Build. Mater. 2015, 96, 279–288. [Google Scholar] [CrossRef]
- Love, A.E.H. A Treatise on the Mathematical Theory of Elasticity; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Costello, G.A. Theory of Wire Rope, 2nd ed.; Springer: New York, NY, USA, 1997. [Google Scholar]
- Cao, X.; Wu, W.G. The establishment of a mechanics model of multi-strand wire rope subjected to bending load with finite element simulation and experimental verification. Int. J. Mech. Sci. 2018, 142, 289–303. [Google Scholar] [CrossRef]
- LeClair, R.A.; Costello, G.A. Axial, bending and torsional loading of a strand with friction. J. Offshore Mech. Arct. Eng. 1988, 110, 38–42. [Google Scholar] [CrossRef]
- Chen, Y.; Meng, F.; Gong, X. Study on performance of bended spiral strand with interwire frictional contact. Int. J. Mech. Sci. 2017, 128, 499–511. [Google Scholar] [CrossRef]
- Ramsey, H. A theory of thin rods with application to helical constituent wires in cables. Int. J. Mech. Sci. 1988, 30, 559–570. [Google Scholar] [CrossRef]
- Raoof, M.; Hobbs, R. The bending of spiral strand and armored cables close to terminations. J. Energy Resour. Technol. 1984, 106, 349–355. [Google Scholar] [CrossRef]
- Jolicoeur, C.; Cardou, A. Semicontinuous mathematical model for bending of multilayered wire strands. J. Eng. Mech. 1996, 122, 643–650. [Google Scholar] [CrossRef]
- Cardou, A.; Jolicoeur, C. Mechanical models of helical strands. Appl. Mech. Rev. 1997, 50, 1–14. [Google Scholar] [CrossRef]
- Lanteigne, J. Theoretical estimation of the response of helically armored cables to tension, torsion, and bending. J. Appl. Mech. 1985, 52, 423–432. [Google Scholar] [CrossRef]
- Papailiou, K.O. On the bending stiffness of transmission line conductors. IEEE Trans. Power Deliv. 1997, 12, 1576–1588. [Google Scholar] [CrossRef]
- Hobbs, R.E.; Ghavani, K. The fatigue of structural wire strands. Int. J. Fatigue 1982, 4, 69–72. [Google Scholar] [CrossRef]
- Hong, K.J.; Kiureghian, A.D.; Sackman, J.L. Bending behavior of helically wrapped cables. J. Eng. Mech. 2005, 131, 500–511. [Google Scholar] [CrossRef]
- Foti, F.; Martinelli, L. Mechanical modeling of metallic strands subjected to tension, torsion and bending. Int. J. Solids Struct. 2016, 91, 1–17. [Google Scholar] [CrossRef]
- Foti, F.; Martinelli, L. An analytical approach to model the hysteretic bending behavior of spiral strands. Appl. Math. Model. 2016, 40, 6451–6467. [Google Scholar] [CrossRef]
- Khan, S.W.; Gencturk, B.; Shahzada, K.; Ullah, A. Bending behaviour of axially preloaded multilayered spiral strands. J. Eng. Mech. 2018, 144, 04018112. [Google Scholar] [CrossRef]
- Zheng, X.; Hu, Y.; Zhou, B.; Li, J. Modelling of the hysteretic bending behavior for helical strands under multi-axial loads. Appl. Math. Model. 2021, 97, 536–558. [Google Scholar] [CrossRef]
- Nawrocki, A.; Labrosse, M. A finite element model for simple straight wire rope strands. Comput. Struct. 2000, 77, 345–359. [Google Scholar] [CrossRef]
- Yu, Y.J.; Chen, Z.H.; Liu, H.B.; Wang, X.D. Finite element study of behavior and interface force conditions of seven-wire strand under axial and lateral loading. Constr. Build. Mater. 2014, 66, 10–18. [Google Scholar] [CrossRef]
- Judge, R.; Yang, Z.; Jones, S.W.; Beattie, G. Full 3D finite element modelling of spiral strand cables. Constr. Build. Mater. 2012, 35, 452–459. [Google Scholar] [CrossRef]
- Kmet, S.; Stanova, E.; Fedorko, G.; Fabian, M.; Brodniansky, J. Experimental investigation and finite element analysis of a four-layered spiral strand bent over a curved support. Eng. Struct. 2013, 57, 475–483. [Google Scholar] [CrossRef]
- Xing, E.; Zhou, C. Analysis of the bending behavior of a cable structure under microgravity. Int. J. Mech. Sci. 2016, 114, 132–140. [Google Scholar] [CrossRef]
- Zhang, D.; Martin, O.S. Finite element solutions to the bending stiffness of a single-layered helically wound cable with internal friction. J. Appl. Mech. 2016, 83, 031003. [Google Scholar] [CrossRef]
- Jiang, W.G.; Yao, M.S.; Walton, J.M. A concise finite element model for simple straight wire rope strand. Int. J. Mech. Sci. 1999, 41, 143–161. [Google Scholar] [CrossRef]
- Jiang, W.G.; Henshall, J.L.; Walton, J.M. A concise finite element model for three-layered straight wire rope strand. Int. J. Mech. Sci. 2000, 42, 63–86. [Google Scholar] [CrossRef]
- Kim, S.-Y.; Lee, P.-S. Modeling of helically stranded cables using multiple beam finite elements and its application to torque balance design. Constr. Build. Mater. 2017, 151, 591–606. [Google Scholar] [CrossRef]
- Yu, Y.; Wang, X.; Chen, Z. A simplified finite element model for structural cable bending mechanism. Int. J. Mech. Sci. 2016, 113, 196–210. [Google Scholar] [CrossRef]
- Zhou, W.; Tian, H.Q. A novel finite element model for single-layered wire strand. J. Cent. South Univ. 2013, 20, 1767–1771. [Google Scholar] [CrossRef]
- Lalonde, S.; Guilbault, R.; Légeron, F. Modeling multilayered wire strands, a strategy based on 3D finite element beam-to-beam contacts—Part I: Model formulation and validation. Int. J. Mech. Sci. 2016, 126, 281–296. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Rajabzadeh-Safaei, N.; Masoodi, A.R. An efficient mixed interpolated curved beam element for geometrically nonlinear analysis. Appl. Math. Model. 2019, 76, 252–273. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Mokhtari, M.; Masoodi, A.R. A novel cable element for nonlinear thermo-elastic analysis. Eng. Struct. 2018, 167, 431–444. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Rajabzadeh-Safaei, N.; Masoodi, A.R. An efficient curved beam element for thermo-mechanical nonlinear analysis of functionally graded porous beams. Structures 2020, 28, 1035–1049. [Google Scholar] [CrossRef]
- Hughes, T.J.R.; Liu, W.K. Nonlinear fitite element analysis of shells: Part I. Three-dimensional shells. Comput. Methods Appl. Mech. Eng. 1981, 26, 331–362. [Google Scholar] [CrossRef]











| Symbol | Physical Meaning | Value |
|---|---|---|
| Rc | Radius of core wire | 1.52 mm |
| Rw | Radius of outer wire | 1.50 mm |
| p | Pitch length | 141.58 mm |
| α | Layer angle | 0.1331 rad |
| m | Number of outer wires | 6 |
| E | Youngs modulus | 197,950 MPa |
| ν | Poisson ratio | 0.3 |
| P = 4 kg | P = 8 kg | P = 12 kg | P = 16 kg | P = 20 kg | |||||
|---|---|---|---|---|---|---|---|---|---|
| T/kg | /mm | T/kg | /mm | T/kg | /mm | T/kg | /mm | T/kg | /mm |
| 102 | 5.74 | 100 | 11.97 | 101 | 18.57 | 99.5 | 24.79 | 101 | 30.57 |
| 127 | 4.79 | 123 | 10.21 | 126 | 15.33 | 125 | 20.64 | 126 | 25.70 |
| 149 | 4.18 | 150 | 8.62 | 151 | 13.10 | 150.5 | 17.58 | 152 | 22.12 |
| 175 | 3.68 | 175 | 7.58 | 176 | 11.45 | 175 | 15.48 | 174 | 19.73 |
| 202 | 3.33 | 201 | 6.87 | 200 | 10.33 | 200 | 13.85 | 201 | 17.35 |
| 230.8 | 2.95 | 221 | 6.35 | 225 | 9.41 | 225.5 | 12.59 | 227 | 15.70 |
| 253 | 2.65 | 250 | 5.64 | 250 | 8.53 | 250.5 | 11.59 | 252 | 14.39 |
| 287 | 2.30 | 275.5 | 5.14 | 272 | 7.92 | 275 | 10.64 | 278 | 13.21 |
| 304 | 2.16 | 299 | 4.70 | 300 | 7.25 | 298 | 9.92 | 301 | 12.38 |
| 331 | 1.96 | 325 | 4.31 | 326 | 6.70 | 325 | 9.17 | 326.5 | 11.46 |
| 352 | 1.83 | 349 | 4.00 | 350 | 6.23 | 350 | 8.55 | 351 | 10.70 |
| 381 | 1.67 | 365 | 3.83 | 376 | 5.77 | 375 | 7.92 | 377 | 9.99 |
| 402.5 | 1.58 | 399 | 3.48 | 401 | 5.38 | 399 | 7.38 | 402 | 9.36 |
| 426 | 1.51 | 424.5 | 3.27 | 425.5 | 5.09 | 424 | 6.90 | 427 | 8.76 |
| 454 | 1.44 | 450 | 3.11 | 450 | 4.85 | 450.5 | 6.51 | 452 | 8.22 |
| 484 | 1.40 | 474.5 | 2.98 | 475 | 4.64 | 475 | 6.21 | 477 | 7.80 |
| 528 | 1.34 | 499 | 2.87 | 500 | 4.44 | 500 | 5.96 | 502 | 7.43 |
| Factors | Levels |
|---|---|
| Nominal length | 1, 2, 3, 6, 8, 10, 12, 16, 24, 36 |
| Friction coefficients μ | 0, 0.05, 0.1, 0.15, 0.2, 0.25, 0.3 |
| Axial tension T (kN) | 1, 2, 3, 4, 5, 10, 15, 20 |
| Nominal lateral loading factor ζ | 0.01, 0.02, 0.03, 0.04, 0.05, 0.06, 0.07, 0.08, 0.09, 0.1 |
| C1 | C2 | C3 | C4 | |
|---|---|---|---|---|
| c1 | 29.0682 | 81.2622 | 3.9885 | -- |
| c2 | 3.8947 | −10.5819 | 0.0387 | -- |
| c3 | 21.0599 | 195.6334 | 0.1034 | -- |
| c4 | −33.1358 | 54.2467 | 0.1468 | -- |
| c5 | −33.3216 | −22.2503 | −0.2001 | -- |
| c6 | −49.1485 | −1559.0403 | −27.6557 | -- |
| c7 | 143.9697 | −328.7151 | −0.4003 | -- |
| c8 | −625.9817 | 3172.6300 | 5.4847 | -- |
| c9 | 1.1374 | 2.9707 | −0.0021 | 6.2839 |
| c10 | −2.3024 | 13.5124 | −0.0043 | -- |
| c11 | 1884.4129 | −2257.9039 | 42.2574 | -- |
| c12 | 33.0398 | −180.9647 | 0.3377 | -- |
| c13 | −341.1416 | 588.8275 | 0.2601 | -- |
| R2 | 0.9959 | 0.9909 | 0.9847 | 0.9998 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, B.; Hu, Y.; Zheng, X.; Zhu, H. Bending Behavior of a Frictional Single-Layered Spiral Strand Subjected to Multi-Axial Loads: Numerical and Experimental Investigation. Appl. Sci. 2022, 12, 4792. https://doi.org/10.3390/app12094792
Zhou B, Hu Y, Zheng X, Zhu H. Bending Behavior of a Frictional Single-Layered Spiral Strand Subjected to Multi-Axial Loads: Numerical and Experimental Investigation. Applied Sciences. 2022; 12(9):4792. https://doi.org/10.3390/app12094792
Chicago/Turabian StyleZhou, Biwen, Yumei Hu, Xingyuan Zheng, and Hao Zhu. 2022. "Bending Behavior of a Frictional Single-Layered Spiral Strand Subjected to Multi-Axial Loads: Numerical and Experimental Investigation" Applied Sciences 12, no. 9: 4792. https://doi.org/10.3390/app12094792
APA StyleZhou, B., Hu, Y., Zheng, X., & Zhu, H. (2022). Bending Behavior of a Frictional Single-Layered Spiral Strand Subjected to Multi-Axial Loads: Numerical and Experimental Investigation. Applied Sciences, 12(9), 4792. https://doi.org/10.3390/app12094792







