Linear and Non-Linear Population Retrieval with Femtosecond Optical Pumping of Molecular Crystals for the Generalised Uniaxial and Biaxial Systems
Abstract
:1. Introduction
2. Crystal Orientation and Practical Determination of Principal Indices of Refraction
2.1. Uniaxial Crystals
2.2. Biaxial Crystals
2.3. Cubic Crystals
3. Rotation Matrix for Transition Dipole Moment to the Optical Frame
4. Electric Field Decomposition Using the Indicatrix
5. Dipole Projections
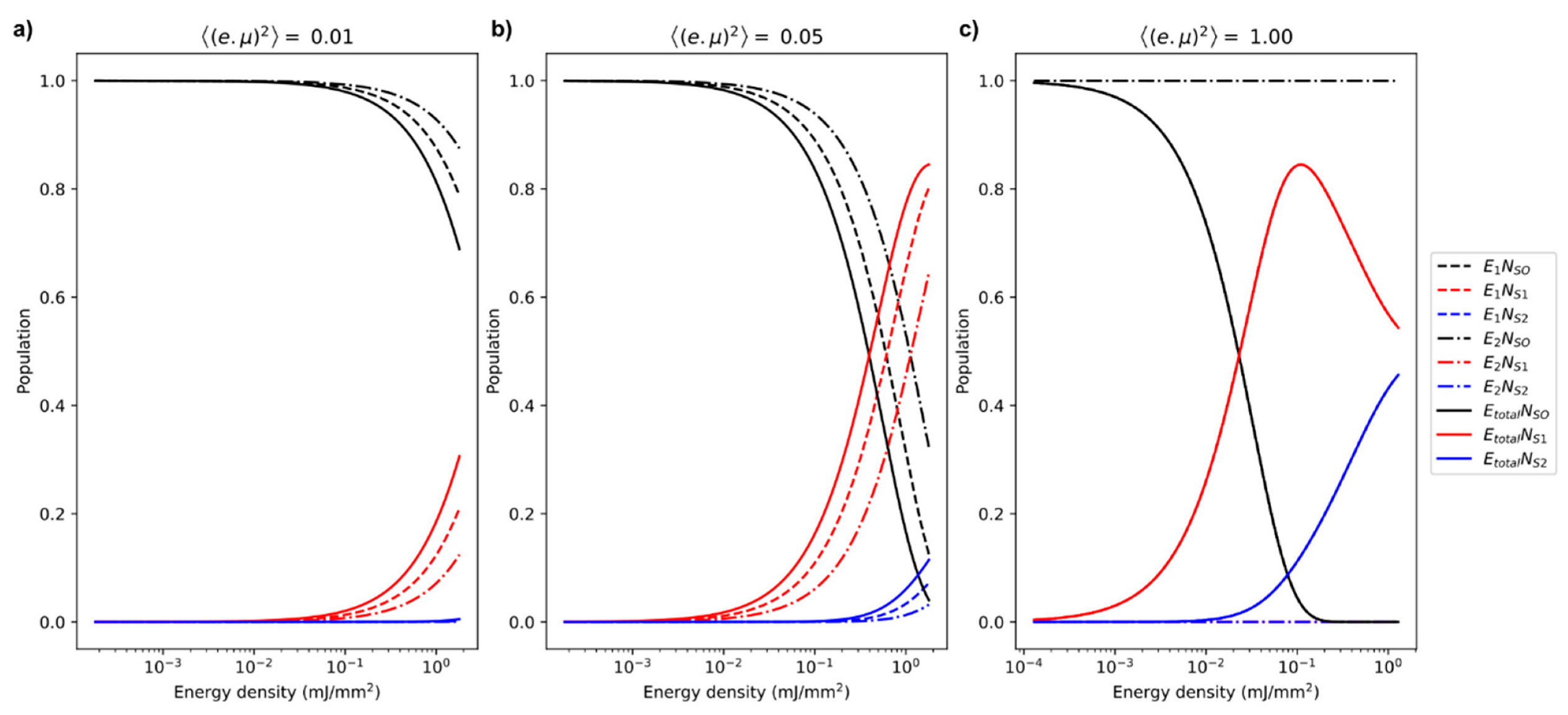
6. Linear and Non-Linear Excitation Calculations
7. Toolkit Program
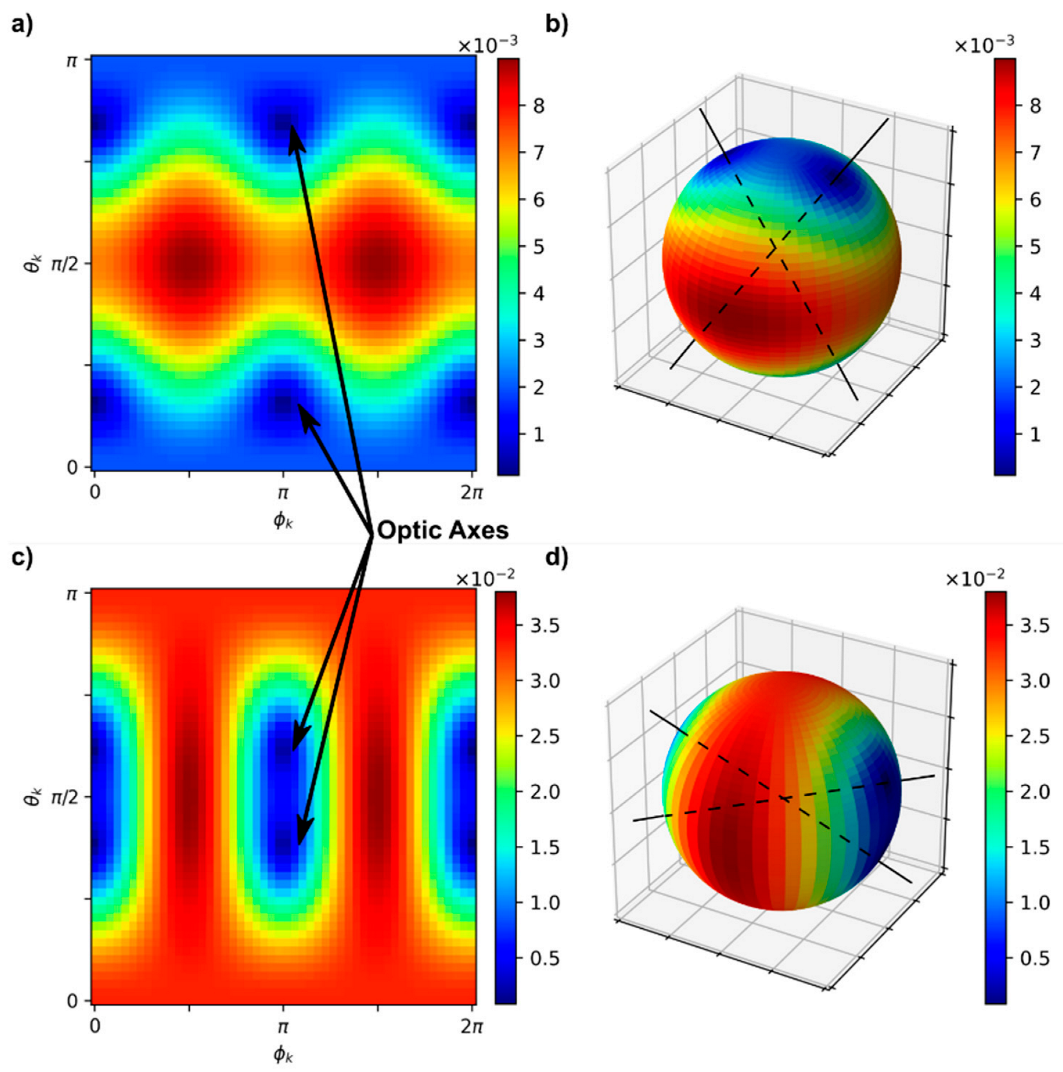
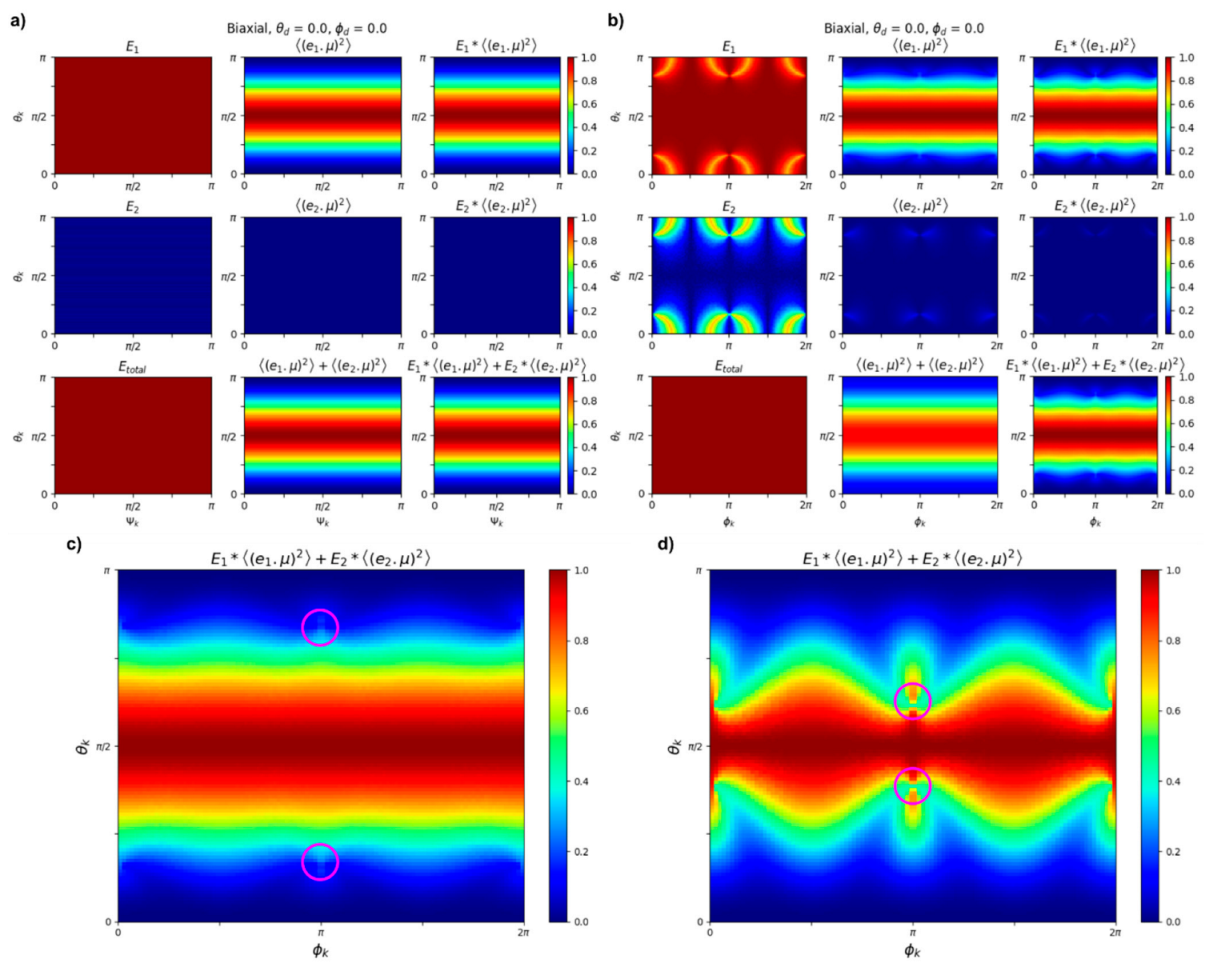
8. Biaxial Crystal Modelling Examples
9. Assumptions, Approximations, and Extensions of the Excitation Model
10. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mukamel, S. Multidimensional Femtosecond Correlation Spectroscopies of Electronic and Vibrational Excitations. Annu. Rev. Phys. Chem. 2000, 51, 691–729. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jonas, D.M. Two-Dimensional Femtosecond Spectroscopy. Annu. Rev. Phys. Chem. 2003, 54, 425–463. [Google Scholar] [CrossRef] [PubMed]
- Jansen, T.L.C.; Saito, S.; Jeon, J.; Cho, M. Theory of coherent two-dimensional vibrational spectroscopy. J. Chem. Phys. 2019, 150, 100901. [Google Scholar] [CrossRef] [PubMed]
- Tiwari, V.; Peters, W.K.; Jonas, D.M. Electronic resonance with anticorrelated pigment vibrations drives photosynthetic energy transfer outside the adiabatic framework. Proc. Natl. Acad. Sci. USA 2013, 110, 1203–1208. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chenu, A.; Scholes, G.D. Coherence in Energy Transfer and Photosynthesis. Annu. Rev. Phys. Chem. 2015, 66, 69–96. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Richter, J.M.; Branchi, F.; Valduga de Almeida Camargo, F.; Zhao, B.; Friend, R.H.; Cerullo, G.; Deschler, F. Ultrafast carrier thermalization in lead iodide perovskite probed with two-dimensional electronic spectroscopy. Nat. Commun. 2017, 8, 376. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- van Thor, J.J. Coherent two-dimensional electronic and infrared crystallography. J. Chem. Phys. 2019, 150, 124113. [Google Scholar] [CrossRef] [PubMed]
- Nye, J.F.; Lindsay, R.B. Physical Properties of Crystals: Their Representation by Tensors and Matrices. Phys. Today 1957, 10, 26. [Google Scholar] [CrossRef]
- Barends, T.R.M.; Foucar, L.; Ardevol, A.; Nass, K.; Aquila, A.; Botha, S.; Doak, R.B.; Falahati, K.; Hartmann, E.; Hilpert, M.; et al. Direct observation of ultrafast collective motions in CO myoglobin upon ligand dissociation. Science 2015, 350, 445–450. [Google Scholar] [CrossRef] [PubMed]
- Pande, K.; Hutchison, C.D.M.; Groenhof, G.; Aquila, A.; Robinson, J.S.; Tenboer, J.; Basu, S.; Boutet, S.; DePonte, D.P.; Liang, M.; et al. Femtosecond structural dynamics drives the trans/cis isomerization in photoactive yellow protein. Science 2016, 352, 725–729. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nogly, P.; Weinert, T.; James, D.; Carbajo, S.; Ozerov, D.; Furrer, A.; Gashi, D.; Borin, V.; Skopintsev, P.; Jaeger, K.; et al. Retinal isomerization in bacteriorhodopsin captured by a femtosecond X-ray laser. Science 2018, eaat0094. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hutchison, C.D.M.; van Thor, J.J. Optical control, selection and analysis of population dynamics in ultrafast protein X-ray crystallography. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2019, 377, 20170474. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Born, M.; Wolf, E. Principles of optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light; Pergamon Press: Oxford, UK, 1994; ISBN 0521642221. [Google Scholar]
- Sage, J.T.; Zhang, Y.; McGeehan, J.; Ravelli, R.B.G.; Weik, M.; Van Thor, J.J. Infrared protein crystallography. Biochim. Biophys. Acta-Proteins Proteom. 2011, 1814, 760–777. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ruiz, T.; Oldenbourg, R. Birefringence of tropomyosin crystals. Biophys. J. 1988, 54, 17–24. [Google Scholar] [CrossRef] [Green Version]
- Perutz, M.F.; Weisz, O. Crystal Structure of Human Carboxyhæmoglobin. Nature 1947, 160, 786–787. [Google Scholar] [CrossRef] [PubMed]
- Michel-Lévy, A.; Lacroix, A. Les Mineraux des Roches; Librairie Polytechnique: Paris, France, 1888. [Google Scholar]
- Stoiber, R.E.; Morse, S.A. Crystal Identification with the Polarizing Microscope; Springer: Boston, MA, USA, 1994; ISBN 978-0-412-04831-9. [Google Scholar]
- Nesse, W. Introduction to Optical Mineralogy, 4th ed.; Oxford University Press: Oxford, UK, 2012. [Google Scholar]
- Holtzer, A.; Clark, R.; Lowey, S. The Conformation of Native and Denatured Tropomyosin B *. Biochemistry 1965, 4, 2401–2411. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics; Academic Press: Amsterdam, The Netherlands, 2008; ISBN 9780123694706. [Google Scholar]
- Zamzam, N.; van Thor, J. Excited State Frequencies of Chlorophyll f and Chlorophyll a and Evaluation of Displacement through Franck-Condon Progression Calculations. Molecules 2019, 24, 1326. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hutchison, C.D.M.; Kaucikas, M.; Tenboer, J.; Kupitz, C.; Moffat, K.; Schmidt, M.; van Thor, J.J. Photocycle populations with femtosecond excitation of crystalline photoactive yellow protein. Chem. Phys. Lett. 2016, 654, 63–71. [Google Scholar] [CrossRef] [Green Version]
- Anaconda Software Distribution; Anaconda, Inc.: Austin, TX, USA, 2016.
- Lincoln, C.N.; Fitzpatrick, A.E.; van Thor, J.J. Photoisomerisation quantum yield and non-linear cross-sections with femtosecond excitation of the photoactive yellow protein. Phys. Chem. Chem. Phys. 2012, 14, 15752–15764. [Google Scholar] [CrossRef] [PubMed]
- Torres-Cavanillas, R.; Morant-Giner, M.; Escorcia-Ariza, G.; Dugay, J.; Canet-Ferrer, J.; Tatay, S.; Cardona-Serra, S.; Giménez-Marqués, M.; Galbiati, M.; Forment-Aliaga, A.; et al. Spin-crossover nanoparticles anchored on MoS2 layers for heterostructures with tunable strain driven by thermal or light-induced spin switching. Nature 2021, 13, 1101–1109. [Google Scholar] [CrossRef] [PubMed]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hutchison, C.D.M.; Fadini, A.; van Thor, J.J. Linear and Non-Linear Population Retrieval with Femtosecond Optical Pumping of Molecular Crystals for the Generalised Uniaxial and Biaxial Systems. Appl. Sci. 2022, 12, 4309. https://doi.org/10.3390/app12094309
Hutchison CDM, Fadini A, van Thor JJ. Linear and Non-Linear Population Retrieval with Femtosecond Optical Pumping of Molecular Crystals for the Generalised Uniaxial and Biaxial Systems. Applied Sciences. 2022; 12(9):4309. https://doi.org/10.3390/app12094309
Chicago/Turabian StyleHutchison, Christopher D. M., Alisia Fadini, and Jasper J. van Thor. 2022. "Linear and Non-Linear Population Retrieval with Femtosecond Optical Pumping of Molecular Crystals for the Generalised Uniaxial and Biaxial Systems" Applied Sciences 12, no. 9: 4309. https://doi.org/10.3390/app12094309
APA StyleHutchison, C. D. M., Fadini, A., & van Thor, J. J. (2022). Linear and Non-Linear Population Retrieval with Femtosecond Optical Pumping of Molecular Crystals for the Generalised Uniaxial and Biaxial Systems. Applied Sciences, 12(9), 4309. https://doi.org/10.3390/app12094309





